High-Frequency Radar Cross Section of Ocean Surface for an FMICW Source
Abstract
:1. Introduction
2. Derivation of the Cross Section
2.1. General First- and Second-Order Electric Field Equations
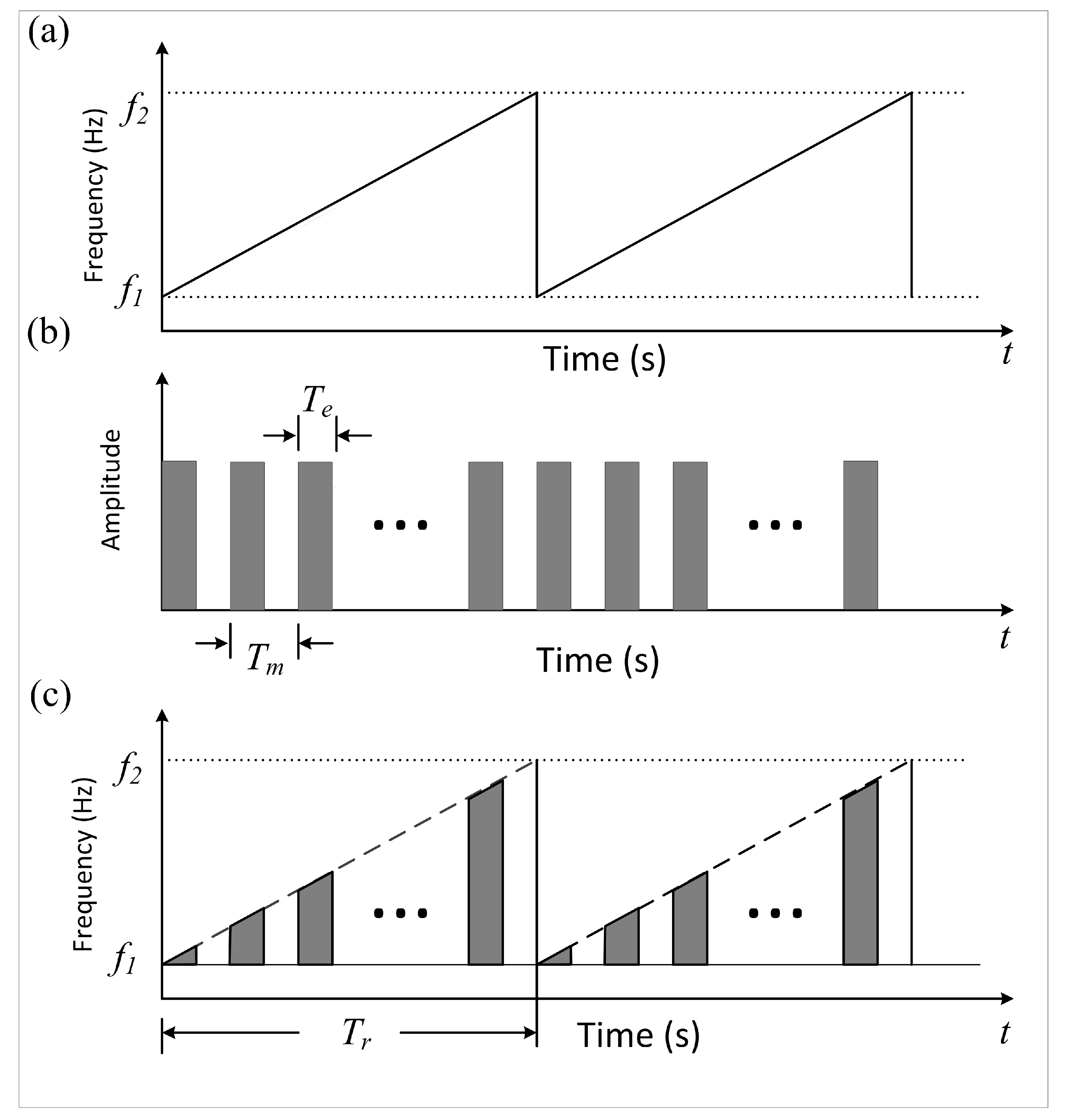
2.2. The FMICW Source
2.3. Electric Field Equations Incorporating the FMICW Source
2.4. Cross Section for the FMICW Source
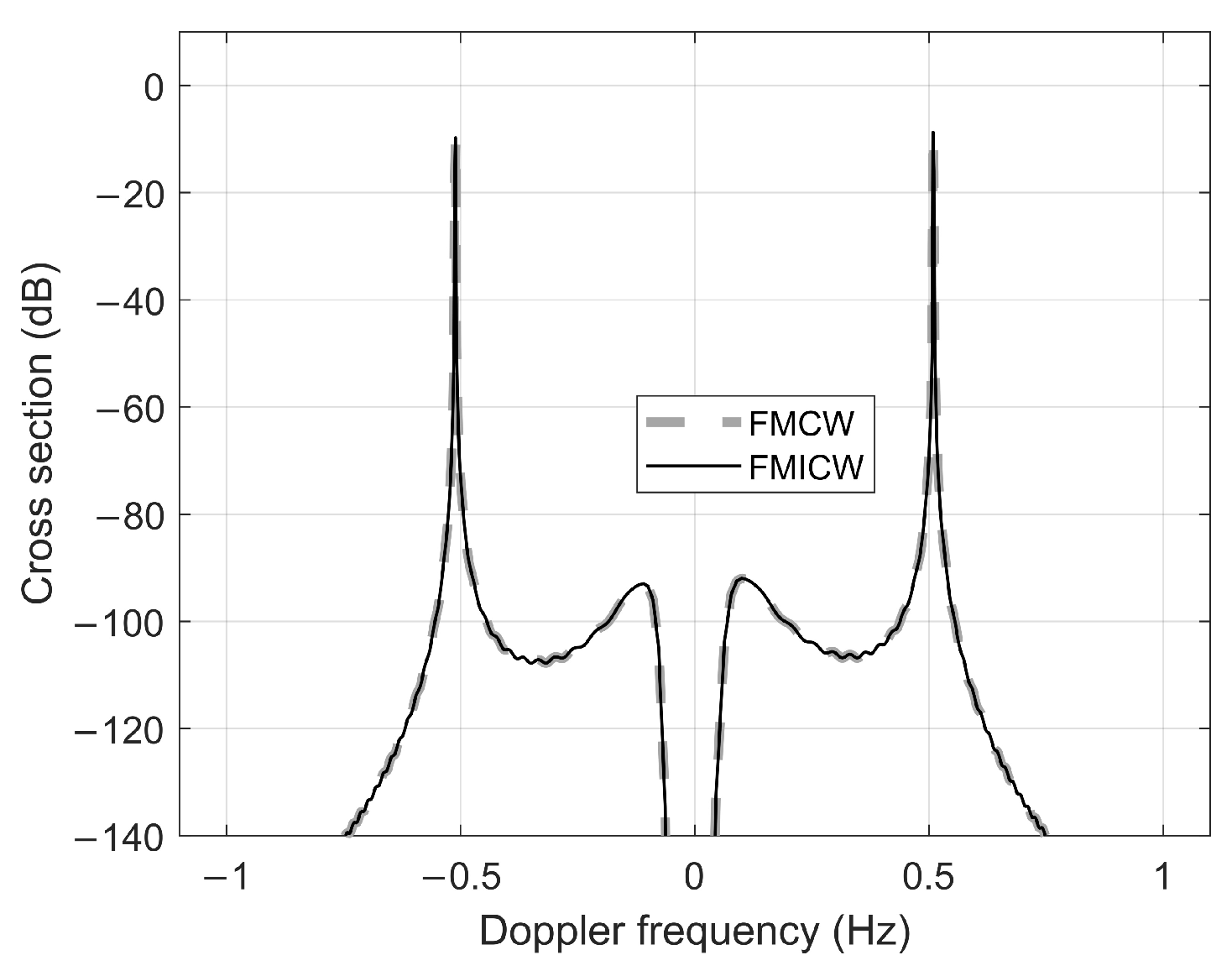
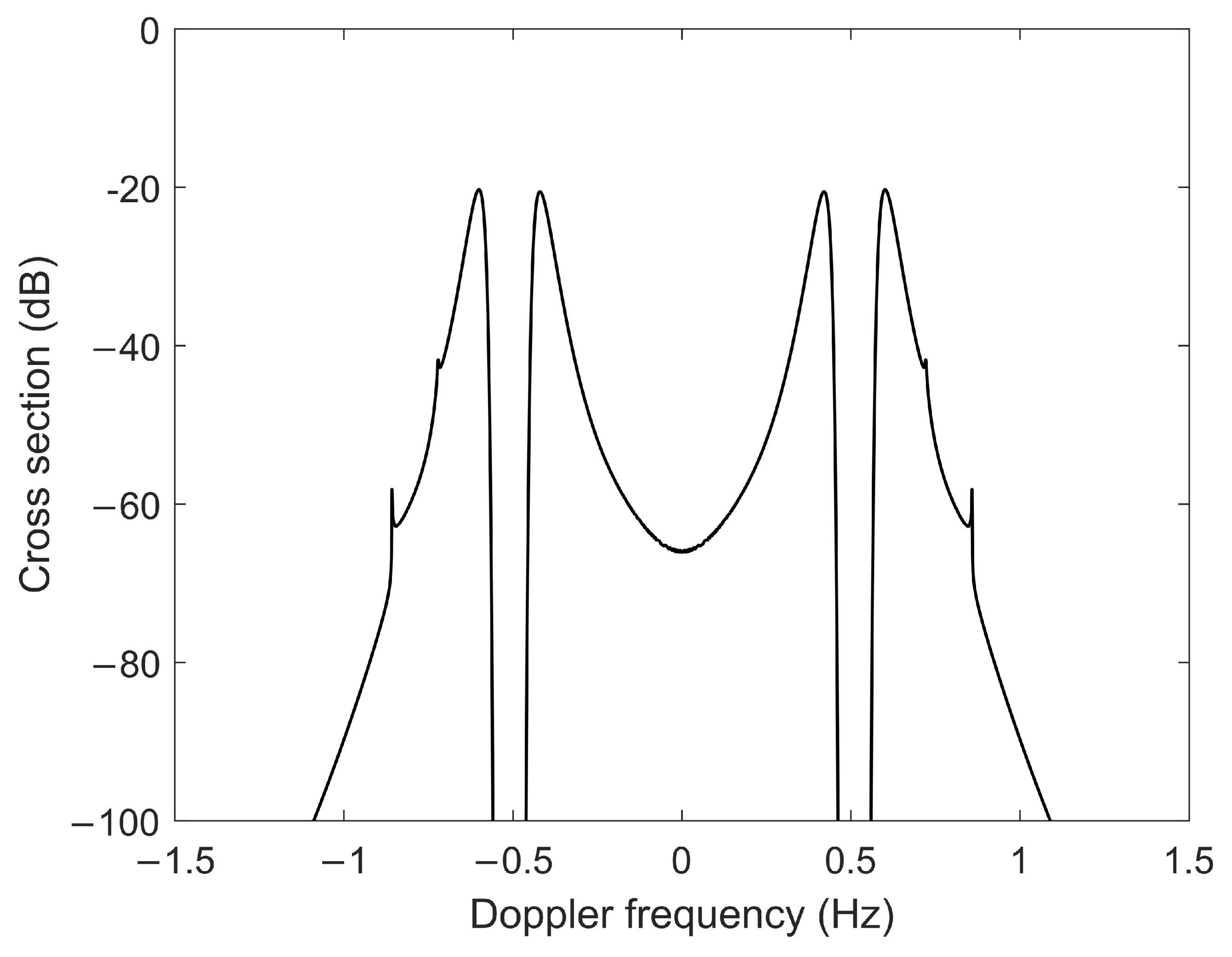
3. Simulation Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mantovani, C.; Corgnati, L.; Horstmann, J.; Rubio, A.; Reyes, E.; Quentin, C.; Cosoli, S.; Asensio, J.; Mader, J.; Griffa, A. Best practices on high frequency radar deployment and operation for ocean current measurement. Front. Mar. Sci. 2020, 7, 210. [Google Scholar] [CrossRef] [Green Version]
- Jena, B.; Arunraj, K.; Suseentharan, V.; Tushar, K.; Karthikeyan, T. Indian coastal ocean radar network. Curr. Sci. 2019, 116, 372–378. [Google Scholar] [CrossRef]
- Paduan, J.D.; Washburn, L. High-frequency radar observations of ocean surface currents. Annu. Rev. Mar. Sci. 2013, 5, 115–136. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Crombie, D.D. Doppler spectrum of sea echo at 13.56 mc./s. Nature 1955, 175, 681–682. [Google Scholar] [CrossRef]
- Gurgel, K.-W.; Essen, H.-H.; Kingsley, S. High-frequency radars: Physical limitations and recent developments. Coast. Eng. 1999, 37, 201–218. [Google Scholar] [CrossRef]
- Barrick, D.E.; Headrick, J.M.; Bogle, R.W.; Crombie, D.D. Sea backscatter at HF: Interpretation and utilization of the echo. Proc. IEEE 1974, 62, 673–680. [Google Scholar] [CrossRef]
- Barrick, D. First-order theory and analysis of MF/HF/VHF scatter from the sea. IEEE Trans. Antennas Propag. 1972, 20, 2–10. [Google Scholar] [CrossRef] [Green Version]
- Walsh, J.; Donnelly, R. Consolidated approach to 2-body electromagnetic scattering. Phys. Rev. A 1987, 36, 4474–4485. [Google Scholar] [CrossRef]
- Walsh, J.; Gill, E.W. An analysis of the scattering of high-frequency electromagnetic radiation from rough surfaces with application to pulse radar operating in backscatter mode. Radio Sci. 2000, 35, 1337–1359. [Google Scholar] [CrossRef] [Green Version]
- Gill, E.W.; Walsh, J. Bistatic form of the electric field equations for the scattering of vertically polarized high-frequency ground wave radiation from slightly rough, good conducting surfaces. Radio Sci. 2000, 35, 1323–1335. [Google Scholar] [CrossRef]
- Gill, E.W.; Walsh, J. High-frequency bistatic cross sections of the ocean surface. Radio Sci. 2001, 36, 1459–1475. [Google Scholar] [CrossRef]
- Walsh, J.; Huang, W.; Gill, E. The first-order high frequency radar ocean surface cross section for an antenna on a floating platform. IEEE Trans. Antennas Propag. 2010, 58, 2994–3003. [Google Scholar] [CrossRef]
- Walsh, J.; Huang, W.; Gill, E. The second-order high frequency radar ocean surface cross section for an antenna on a floating platform. IEEE Trans. Antennas Propag. 2012, 60, 4804–4813. [Google Scholar] [CrossRef]
- Ma, Y.; Gill, E.W.; Huang, W. First-order bistatic high-frequency radar ocean surface cross-section for an antenna on a floating platform. IET Radar Sonar Navig. 2016, 10, 1136–1144. [Google Scholar] [CrossRef] [Green Version]
- Ma, Y.; Huang, W.; Gill, E.W. The second-order bistatic high frequency radar scattering cross section of the ocean surface for the case of floating platform. In Proceedings of the OCEANS 2015—MTS/IEEE, Washington, DC, USA, 19–22 October 2015; pp. 1–5. [Google Scholar]
- Yao, G.; Xie, J.; Huang, W. First-order ocean surface cross-section for shipborne HFSWR incorporating a horizontal oscillation motion model. IET Radar Sonar Navig. 2018, 12, 973–978. [Google Scholar] [CrossRef]
- Walsh, J.; Zhang, J.; Gill, E.W. High-frequency radar cross section of the ocean surface for an FMCW waveform. IEEE J. Ocean. Eng. 2011, 36, 615–626. [Google Scholar] [CrossRef]
- Ma, Y.; Huang, W.; Gill, E.W. Bistatic high frequency radar ocean surface cross section for an FMCW source with an antenna on a floating platform. Int. J. Antennas Propag. 2016, 2016, 9. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.; Gill, E.W.; Huang, W. A first-order HF radar cross-section model for mixed-path ionosphere-ocean propagation with an FMCW source. IEEE J. Ocean. Eng. 2016, 41, 982–992. [Google Scholar] [CrossRef]
- Barrick, D.E.; Lipa, B.J.; Lilleboe, P.M.; Isaacson, J. Gated FMCW DF Radar and Signal Processing for Range/Doppler/Angle Determination. U.S. Patent 5,361,072, 1 November 1994. [Google Scholar]
- Silva, M.T.; Huang, W.; Gill, E.W. High-frequency radar cross-section of the ocean surface with arbitrary roughness scales: A generalized functions approach. IEEE Trans. Antennas Propag. 2021, 69, 1643–1657. [Google Scholar] [CrossRef]
- Lipa, B.J.; Barrick, D.E. Extraction of sea state from HF radar sea echo: Mathematical theory and modeling. Radio Sci. 1986, 21, 81–100. [Google Scholar] [CrossRef]
- Pierson, W.J.; Moskowitz, L. A proposed spectral form for fully developed wind seas based on the similarity theory of s. a. kitaigorodskii. J. Geophys. Res. Atmos. 1964, 69, 5181–5190. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Lai, Y.; Wang, Y.; Zhou, H. High-Frequency Radar Cross Section of Ocean Surface for an FMICW Source. J. Mar. Sci. Eng. 2021, 9, 427. https://doi.org/10.3390/jmse9040427
Zhao H, Lai Y, Wang Y, Zhou H. High-Frequency Radar Cross Section of Ocean Surface for an FMICW Source. Journal of Marine Science and Engineering. 2021; 9(4):427. https://doi.org/10.3390/jmse9040427
Chicago/Turabian StyleZhao, Hangyu, Yeping Lai, Yuhao Wang, and Hao Zhou. 2021. "High-Frequency Radar Cross Section of Ocean Surface for an FMICW Source" Journal of Marine Science and Engineering 9, no. 4: 427. https://doi.org/10.3390/jmse9040427
APA StyleZhao, H., Lai, Y., Wang, Y., & Zhou, H. (2021). High-Frequency Radar Cross Section of Ocean Surface for an FMICW Source. Journal of Marine Science and Engineering, 9(4), 427. https://doi.org/10.3390/jmse9040427





