Numerical Prediction of the Vertical Responses of Planing Hulls in Regular Head Waves
Abstract
1. Introduction
2. Methodology
2.1. Planing Hull Geometries
2.2. Numerical Setup
2.2.1. Physics Modelling
2.2.2. Mesh Generation and Computational Domain
3. CFD Verification Study
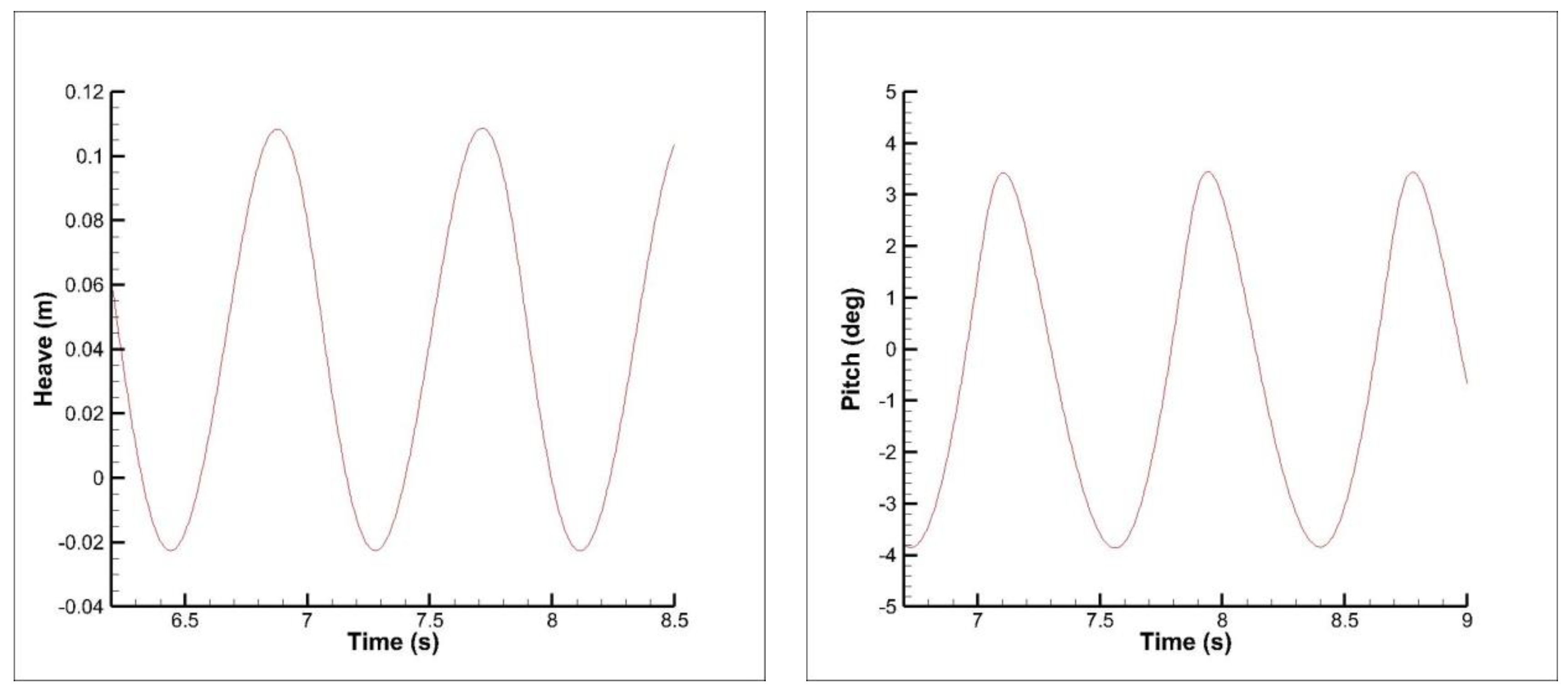
4. Seakeeping Analyses
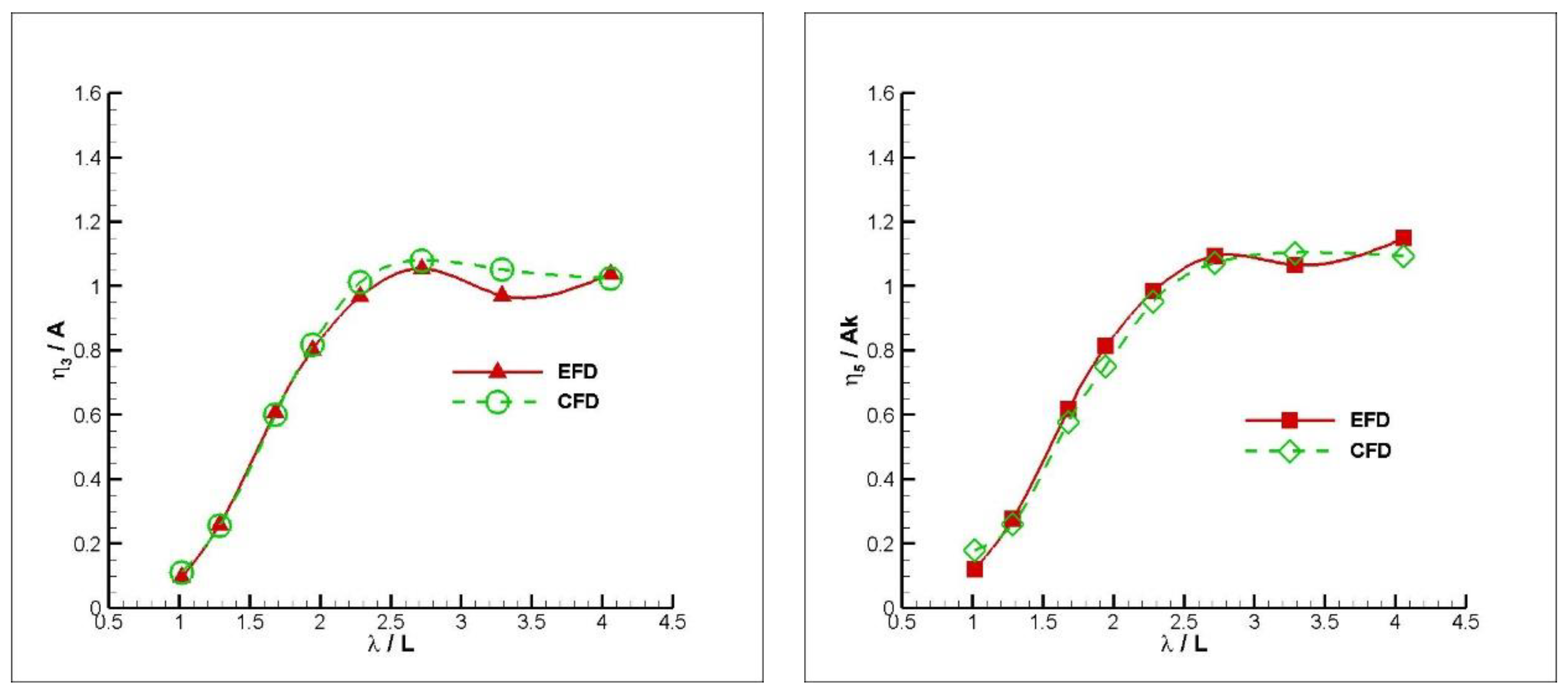
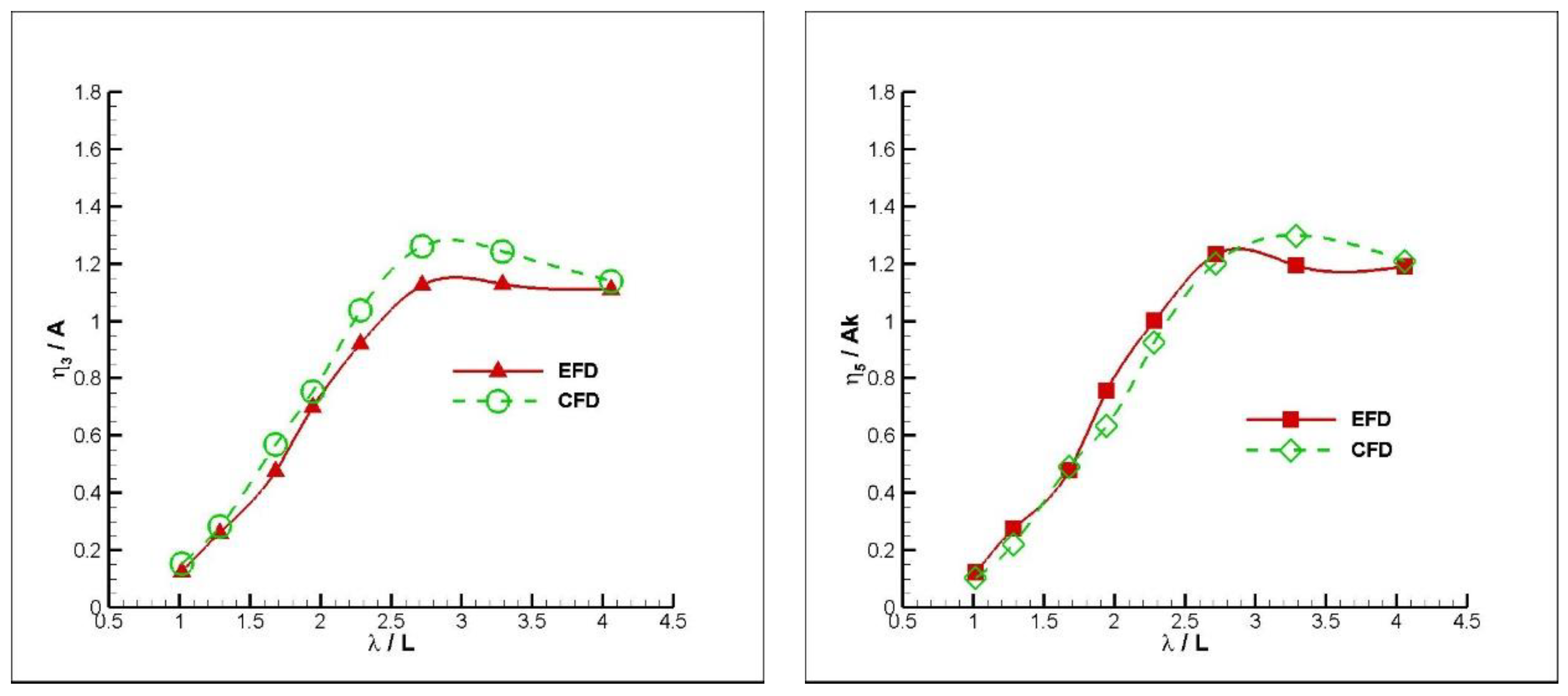
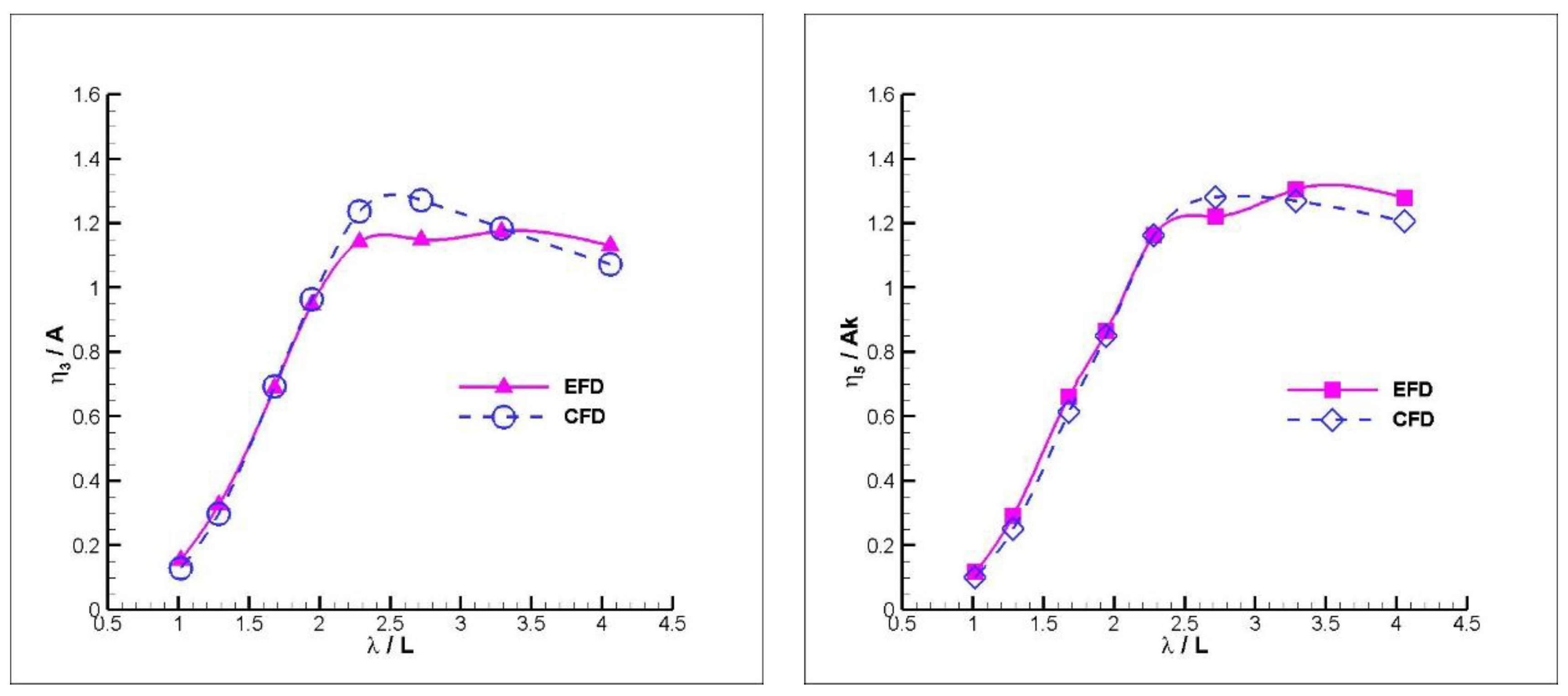
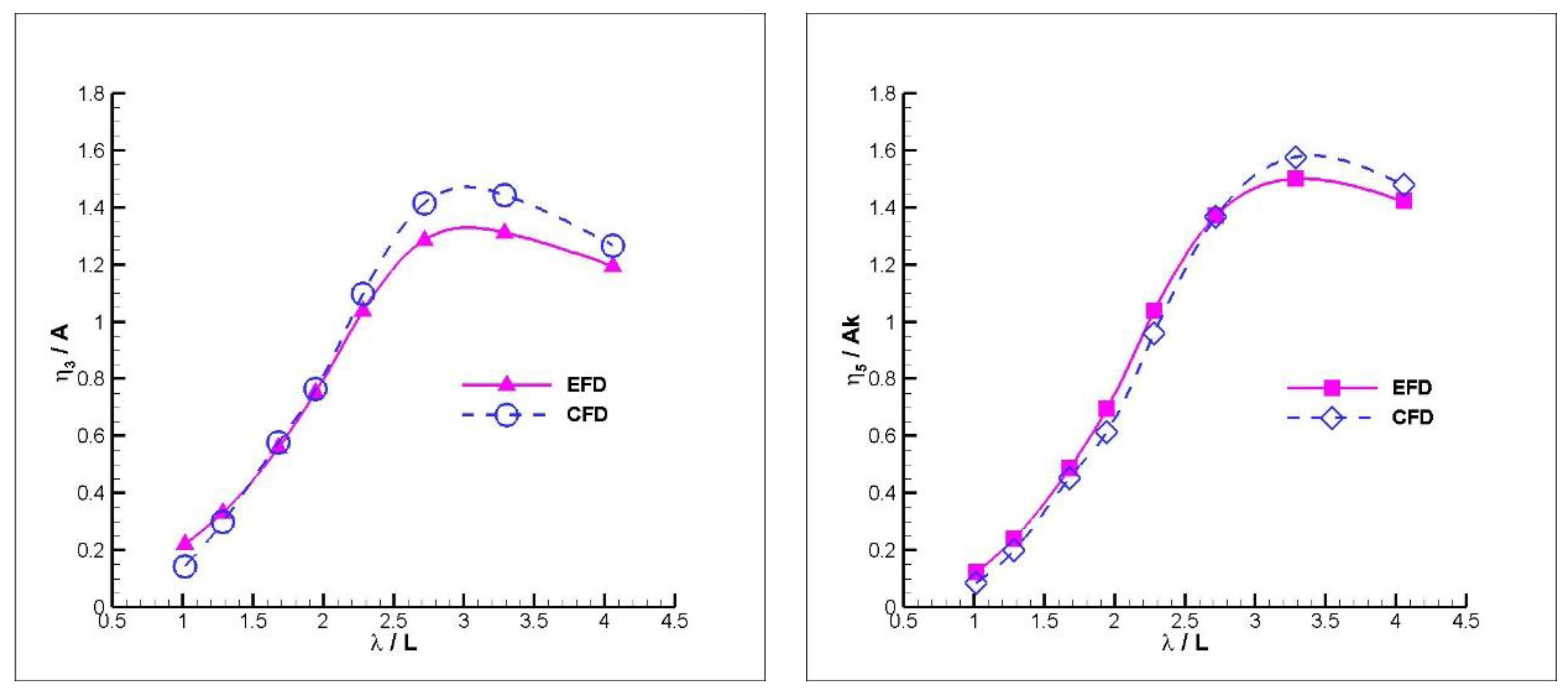
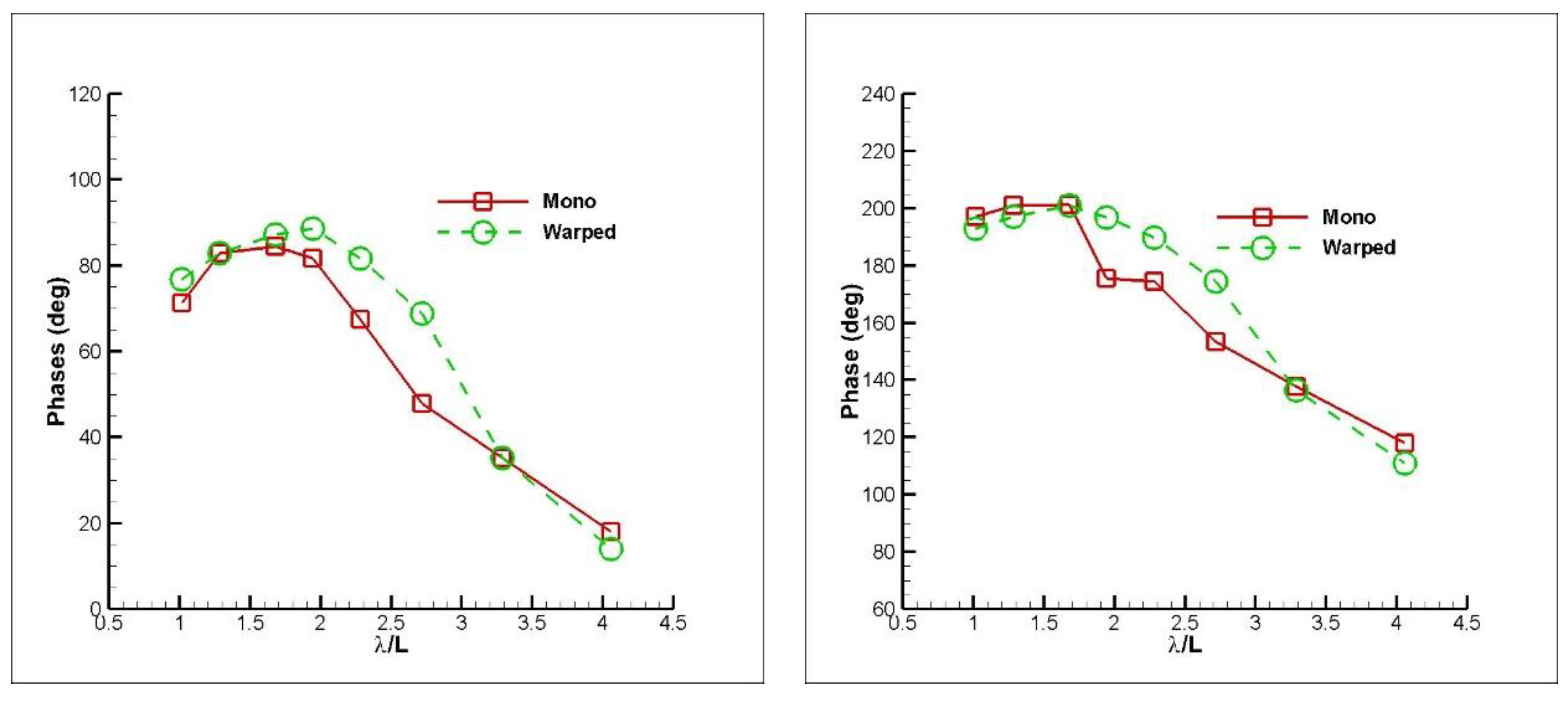
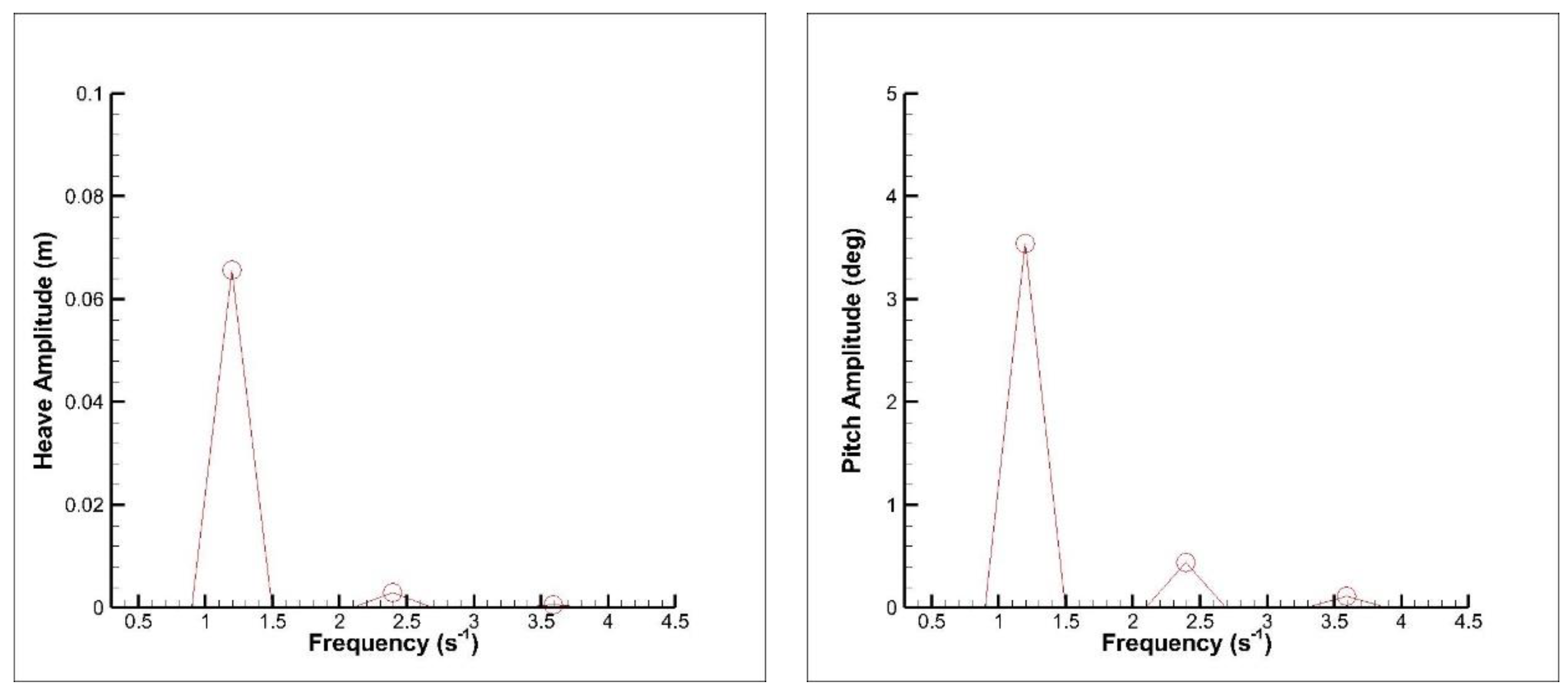
4.1. Motion RAOs
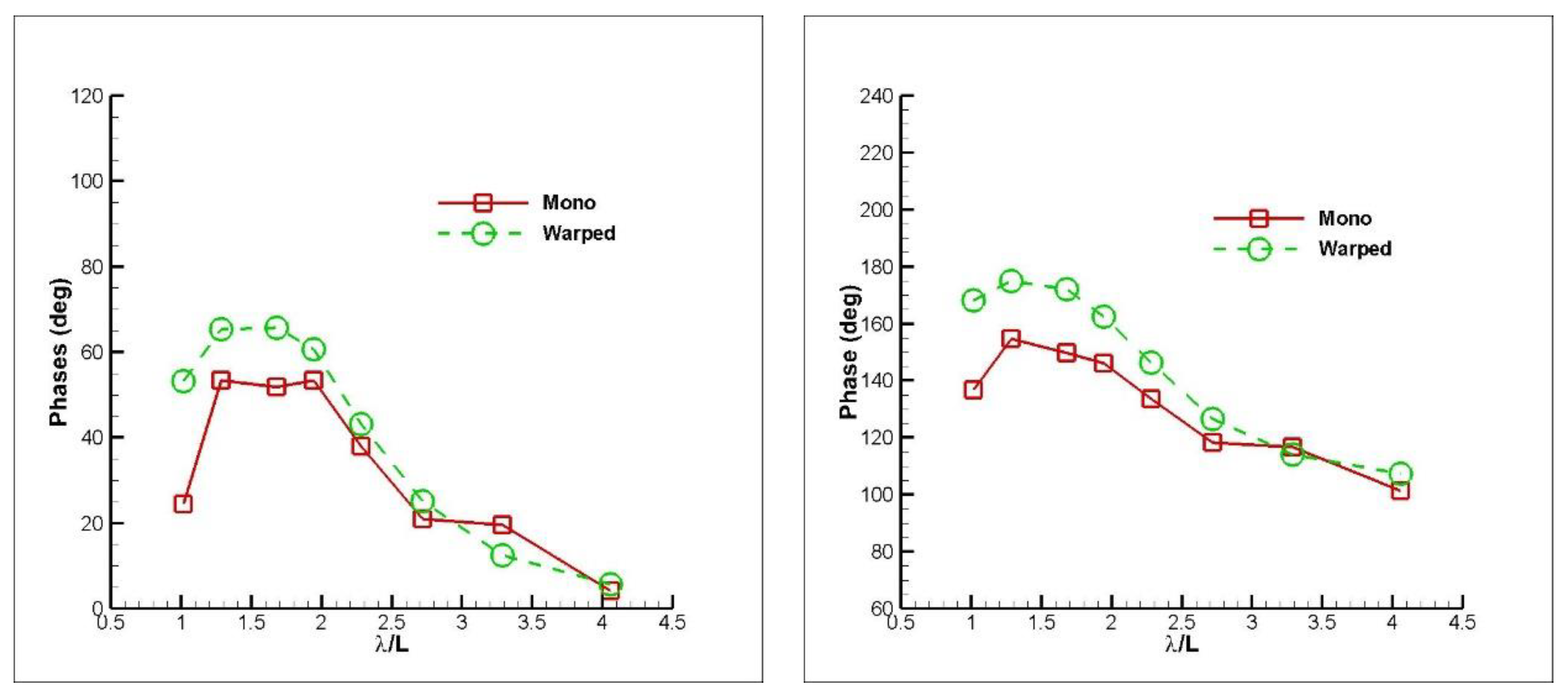
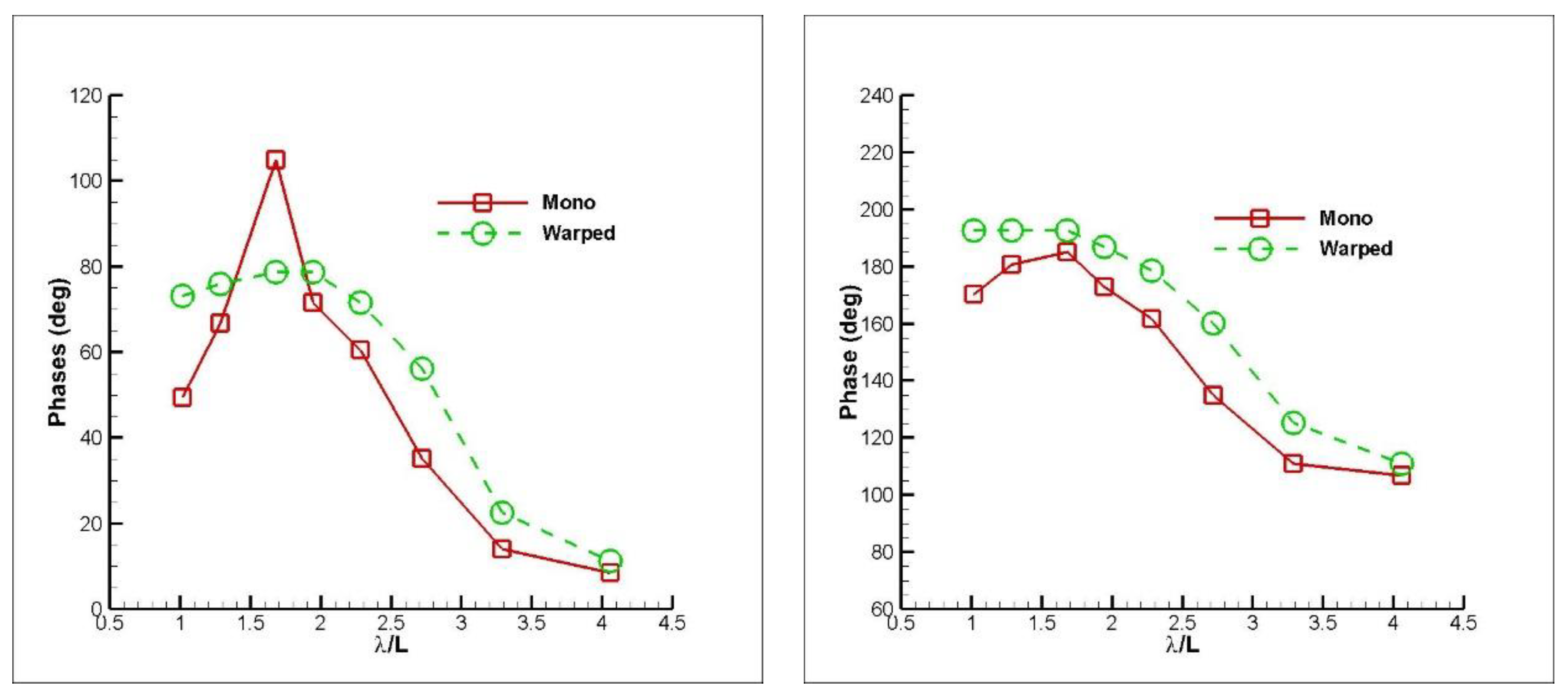
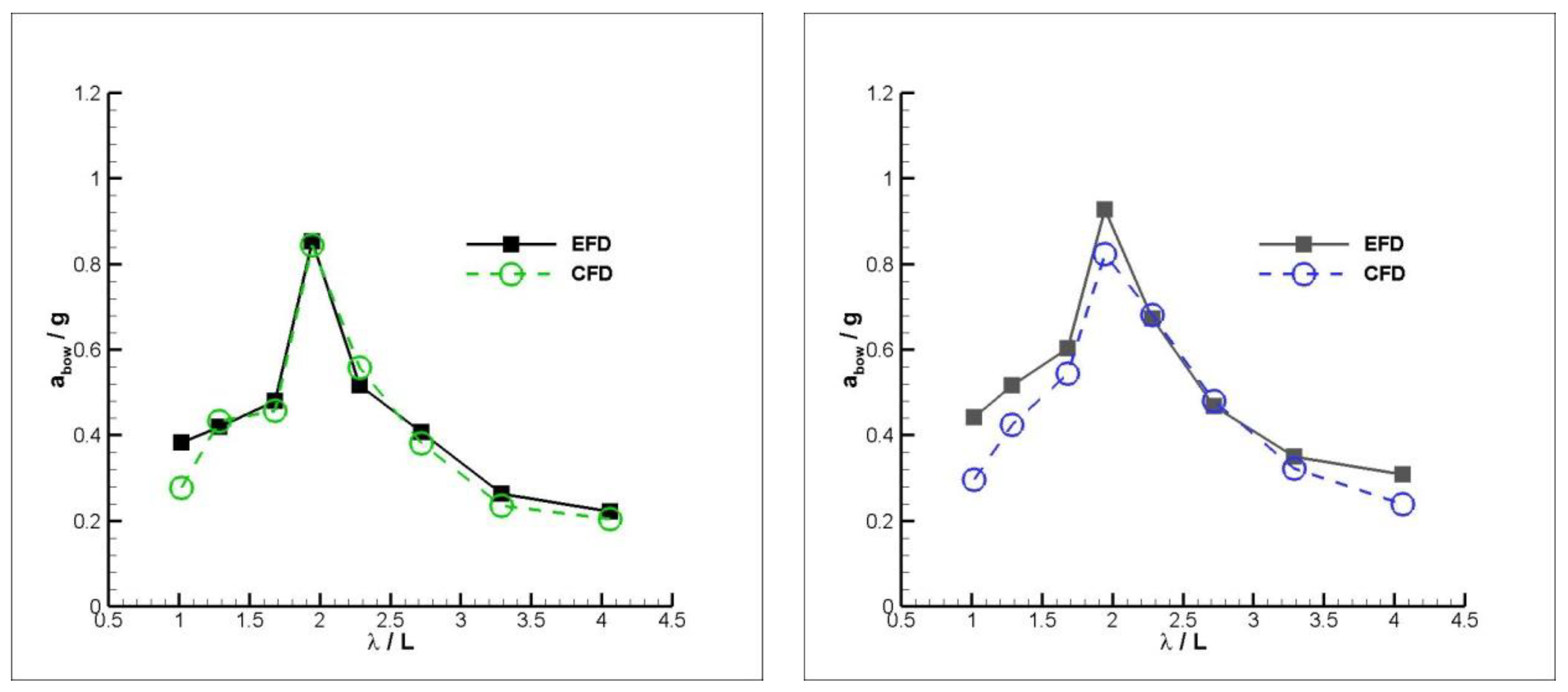
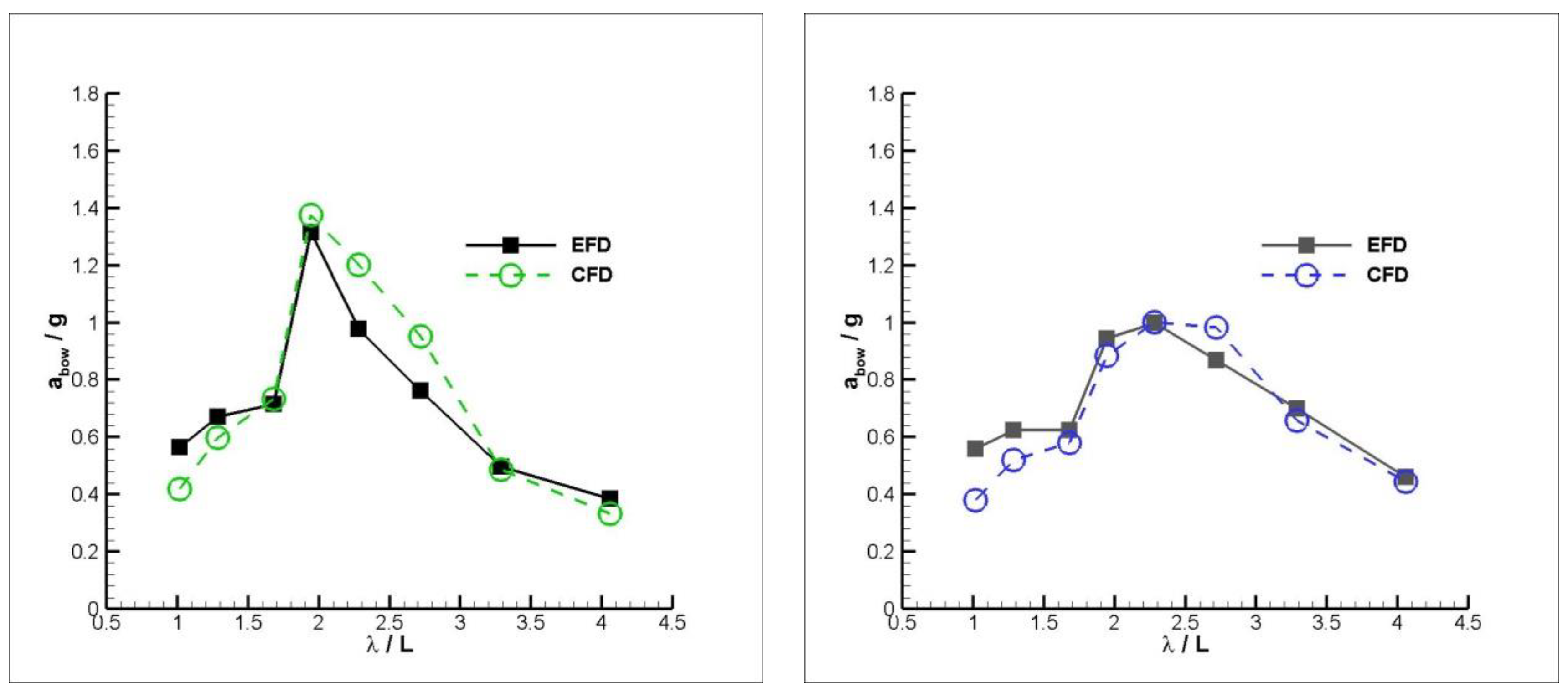
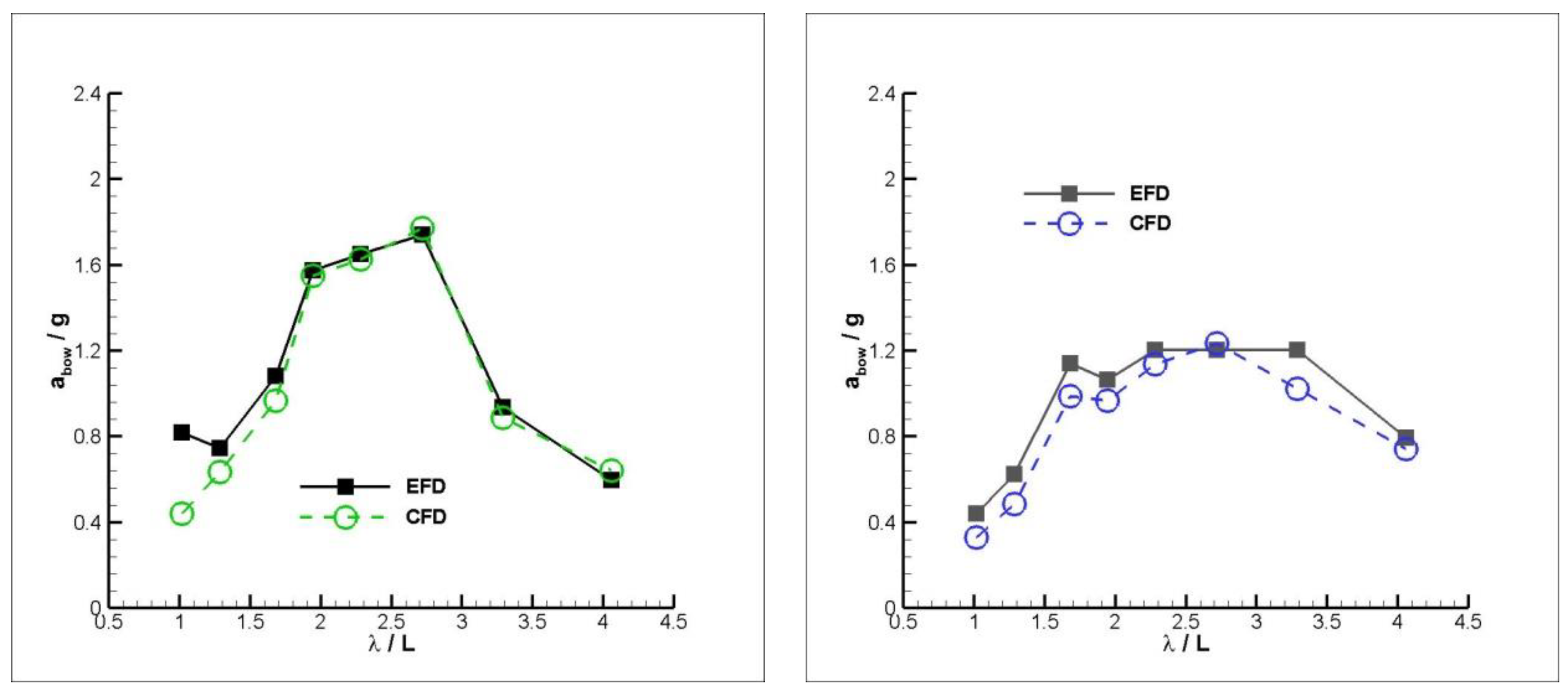
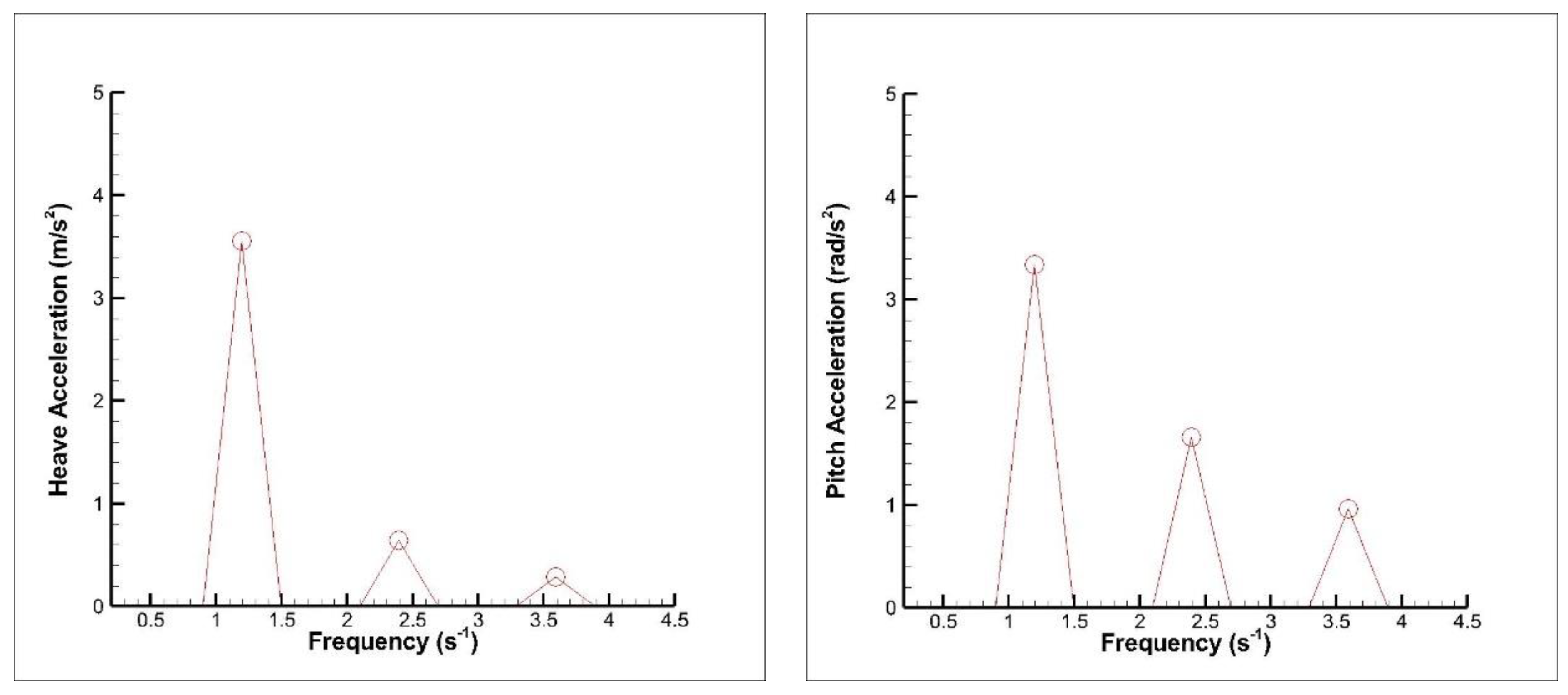
4.2. Acceleration RAOs
5. Conclusions
- An employed viscous solver is reliable in predicting heave and pitch motions in regular waves and bow accelerations for each case.
- In general, the heave motion is overestimated, while the pitch motion is underestimated at almost all frequencies and advance velocities for both hull forms.
- The deviations between the numerical and experimental results are getting larger with the increase in the wavelength, especially for the heave motion.
- The largest deviations are observed in the minimum wavelength cases (Case 1), for both models at all advance velocities. Since the motions are reasonably smaller in the inertia region, these deviations might be directly related to the measurement difficulties in the experiments.
- Similar to the experiments, more than one dominant frequency is observed in the accelerations. Therefore, the comparison of acceleration RAOs is made with through to peak analysis.
- Since there is more than one dominant frequency in the acceleration signal, the RAO calculation technique might cause a small variation in these results.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Savitsky, D. Hydrodynamic Design of Planing Hulls. Mar. Technol. SNAME News 1964, 1, 71–95. [Google Scholar]
- Fridsma, G. A Systematic Study of the Rough-Water Performance of Planing Boats; Stevens Inst of Tech Hoboken NJ Davidson Lab.: Hoboken, NJ, USA, 1969. [Google Scholar]
- De Luca, F.; Pensa, C. The Naples warped hard chine hulls systematic series. Ocean Eng. 2017, 139, 205–236. [Google Scholar] [CrossRef]
- De Luca, F.; Pensa, C. The Naples Systematic Series—Second part: Irregular waves, seakeeping in head sea. Ocean Eng. 2019, 194, 106620. [Google Scholar] [CrossRef]
- Begovic, E.; Bertorello, C.; Pennino, S. Experimental seakeeping assessment of a warped planing hull model series. Ocean Eng. 2014, 83, 1–15. [Google Scholar] [CrossRef]
- Begovic, E.; Bertorello, C.; Pennino, S.; Piscopo, V.; Scamardella, A. Statistical analysis of planing hull motions and accelerations in irregular head sea. Ocean Eng. 2016, 112, 253–264. [Google Scholar] [CrossRef]
- Santoro, N.; Begovic, E.; Bertorello, C.; Bove, A.; De Rosa, S.; Franco, F. Experimental Study of the Hydrodynamic Loads on High Speed Planing Craft. Procedia Eng. 2014, 88, 186–193. [Google Scholar] [CrossRef]
- Zarnick, E.E. A Nonlinear Mathematical Model of Motions of a Planing Boat in Regular Waves; David W Taylor Naval Ship Research and Development Center Bethesda Md Dtnsrdc-78/032, USA. 1978. Available online: https://apps.dtic.mil/docs/citations/ADA052039 (accessed on 18 March 2020).
- Zarnick, E.E. A Nonlinear Mathematical Model of Motions of a Planing Boat in Irregular Waves; David W Taylor Naval Ship Research and Development Center Bethesda Md, Dtnsrdc/Spd-0867-91, USA. 1979. Available online: https://apps.dtic.mil/docs/citations/ADA052039 (accessed on 18 March 2020).
- Akers, R.H. Dynamic Analysis of Planing Hulls in the Vertical Plane; Meeting of New England Section of SNAME. 1999, p. 19. Available online: https://d1wqtxts1xzle7.cloudfront.net/32221394/nes42999.pdf?1383459146=&response-content-disposition=inline%3B+filename%3DDynamic_Analysis_of_Planing_Hulls_in_the.pdf&Expires=1592664320&Signature=d7eiv564cfspF2x346l1qvXMyEZocxjwSCdcQydINtQAyJd5ISA2eRPai8ANcOt2Q1icLfbIlPmf~dAQFDLMiwzMB4PszT6BUnmzAlk3NR2t0KzKQPKTbV5DSA70UCG4S5hYmu9v75LUxL6g1ihBrjQOFzJ4ortZYnkO3YTIRUMMAdJzKSaeEsEnnmnGKVFC1AUIJ1VYtk~8yrY7~T2t7udCkCIVqhR2USA4J7ZLNdg-06KakhDagZ2CmS5xeL~3o04FX8dMR98I~Hvl6~2q1RCqhVd1Xfkjr-1uOuJvPKiY7Os0r1HsNy4NXfw69dvvdHaO8lLEZuPg2N8Qb9VG1g__&Key-Pair-Id=APKAJLOHF5GGSLRBV4ZA (accessed on 18 March 2020).
- van Deyzen, A. A Nonlinear Mathematical Model of Motions of a Planing Monohull in Head Seas. In Proceedings of the HIPER08, Naples, Italy, 18–19 September 2008; p. 14. [Google Scholar]
- Keuning, J.A. Non-Linear Behaviour of Fast Mono-Hulls in Head-Waves. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1994. [Google Scholar]
- Ghadimi, P.; Tavakoli, S.; Dashtimanesh, A. Coupled heave and pitch motions of planing hulls at non-zero heel angle. Appl. Ocean Res. 2016, 59, 286–303. [Google Scholar] [CrossRef]
- Algarín, R.; Tascón, O. Hydrodynamic Modeling of Planing Boats with Asymmetry and Steady Condition. In Proceedings of the 9th Conference on High Sped Marine Vehicles, Naples, Italy, 26–27 May 2011. [Google Scholar]
- Algarín, R.; Tascón, O. Analysis of Dynamic Stability of Planing Craft on the Vertical Plane. Ship Sci. Technol. 2014, 8, 35–43. [Google Scholar] [CrossRef]
- Tavakoli, S.; Dashtimanesh, A.; Sahoo, P.K. Prediction of Hydrodynamic Coefficients of Coupled Heave and Pitch Motions of Heeled Planing Boats by Asymmetric 2D+T Theory. In Proceedings of the 37th International Conference on Ocean, Offshore & Arctic Engineering (OMAE2018), Madrid, Spain, 17–22 June 2018; p. V07AT06A009. [Google Scholar] [CrossRef]
- De Luca, F.; Mancini, S.; Miranda, S.; Pensa, C. An Extended Verification and Validation Study of CFD Simulations for Planing Hulls. J. Ship Res. 2016, 60, 101–118. [Google Scholar] [CrossRef]
- Mancini, S.; De Luca, F.; Ramolini, A. Towards CFD Guidelines for Planing Hull Simulations Based on the Naples Systematic Series. In Proceedings of the Computational Methods in Marine Engineering VII (Marine 2017), Nantes, France, 15–17 June 2017. [Google Scholar]
- Sukas, O.F.; Kinaci, O.K.; Cakici, F.; Gokce, M.K. Hydrodynamic assessment of planing hulls using overset grids. Appl. Ocean Res. 2017, 65, 35–46. [Google Scholar] [CrossRef]
- Judge, C.; Mousaviraad, M.; Stern, F.; Lee, E.; Fullerton, A.; Geiser, J.; Schleicher, C.; Merrill, C.; Weil, C.; Morin, J.; et al. Experiments and CFD of a high-speed deep-V planing hull––Part I: Calm water. Appl. Ocean Res. 2020, 96, 102060. [Google Scholar] [CrossRef]
- Fu, T.C.; Brucker, K.A.; Mousaviraad, S.M.; Ikeda, C.M.; Lee, E.J.; O’Shea, T.T.; Wang, Z.; Stern, F.; Judge, C.Q. An Assessment of Computational Fluid Dynamics Predictions of the Hydrodynamics of High-Speed Planing Craft in Calm Water and Waves. In Proceedings of the 30th Symposium on Naval Hydrodynamics, Tasmania, Australia, 2–7 November 2014. [Google Scholar]
- Mousaviraad, S.M.; Wang, Z.; Stern, F. URANS studies of hydrodynamic performance and slamming loads on high-speed planing hulls in calm water and waves for deep and shallow conditions. Appl. Ocean Res. 2015, 51, 222–240. [Google Scholar] [CrossRef]
- Masumi, Y.; Nikseresht, A.H. 2DOF numerical investigation of a planing vessel in head sea waves in deep and shallow water conditions. Appl. Ocean Res. 2019, 82, 41–51. [Google Scholar] [CrossRef]
- Judge, C.; Mousaviraad, M.; Stern, F.; Lee, E.; Fullerton, A.; Geiser, J.; Schleicher, C.; Merrill, C.; Weil, C.; Morin, J.; et al. Experiments and CFD of a high-speed deep-V planing hull—Part II: Slamming in waves. Appl. Ocean Res. 2020, 97, 102059. [Google Scholar] [CrossRef]
- Azcueta, R. Steady and Unsteady RANSE Simulations for Planing Craft. In Proceedings of the 7th Conference on Fast Sea Transportation FAST’03, Ischia, Italy, 7–10 October 2003. [Google Scholar]
- Wang, S.; Su, Y.; Zhang, X.; Yang, J. RANSE simulation of high-speed planning craft in regular waves. J. Mar. Sci. Appl. 2012, 11, 447–452. [Google Scholar] [CrossRef]
- Ling, H.; Wang, Z. Numerical prediction and analysis of motion response of high speed planing craft in regular waves. J. Jiangsu Univ. Sci. Technol. Nat. Sci. Ed. 2019, 33, 1–8. [Google Scholar]
- Bi, X.; Shen, H.; Zhou, J.; Su, Y. Numerical analysis of the influence of fixed hydrofoil installation position on seakeeping of the planing craft. Appl. Ocean Res. 2019, 90, 101863. [Google Scholar] [CrossRef]
- Bi, X.; Zhuang, J.; Su, Y. Seakeeping Analysis of Planing Craft under Large Wave Height. Water 2020, 12, 1020. [Google Scholar] [CrossRef]
- Begovic, E.; Bertorello, C. Resistance assessment of warped hull form. Ocean Eng. 2012, 56, 28–42. [Google Scholar] [CrossRef]
- Wilcox, D.C. Turbulence Modeling for CFD, 3rd ed.; DCW Industries: La Cãnada, CA, USA, 2006. [Google Scholar]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- 7.5-03-02-03 Practical Guidelines for Ship CFD Applications. In Proceedings of the ITTC—Recommended Procedures and Guidelines, Copenhagen, Denmark, 31 August–6 September 2014.
- Richardson, L.F. The Approximate Arithmetical Solution by Finite Differences of Physical Problems Involving Differential Equations, with an Application to the Stresses in a Masonry Dam. Philos. Trans. R. Soc. Lond. 1910, 210, 307–357. [Google Scholar] [CrossRef]
- Roache, P.J. Verification of Codes and Calculations. AIAA J. 1998, 36, 696–702. [Google Scholar] [CrossRef]
- Stern, F.; Wilson, R.V.; Coleman, H.W.; Paterson, E.G. Comprehensive Approach to Verification and Validation of CFD Simulations—Part 1: Methodology and Procedures. J. Fluids Eng. 2001, 123, 793–802. [Google Scholar] [CrossRef]
- Wilson, R.V.; Stern, F.; Coleman, H.W.; Paterson, E.G. Comprehensive Approach to Verification and Validation of CFD Simulations—Part 2: Application for Rans Simulation of a Cargo/Container Ship. J. Fluids Eng. 2001, 123, 803. [Google Scholar] [CrossRef]
- Stern, F.; Wilson, R.; Shao, J. Quantitative V&V of CFD simulations and certification of CFD codes. Int. J. Numer. Methods Fluids 2006, 50, 1335–1355. [Google Scholar] [CrossRef]
- Çelik, I.; Ghia, U.; Roache, P.; Fretias, C.J.; Coleman, H.; Raad, P.E. Procedure for Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008, 130, 078001. [Google Scholar] [CrossRef]
- Sezen, S.; Dogrul, A.; Delen, C.; Bal, S. Investigation of self-propulsion of DARPA Suboff by RANS method. Ocean Eng. 2018, 150, 258–271. [Google Scholar] [CrossRef]
- Tezdogan, T.; Incecik, A.; Turan, O. Full-scale unsteady RANS simulations of vertical ship motions in shallow water. Ocean Eng. 2016, 123, 131–145. [Google Scholar] [CrossRef]

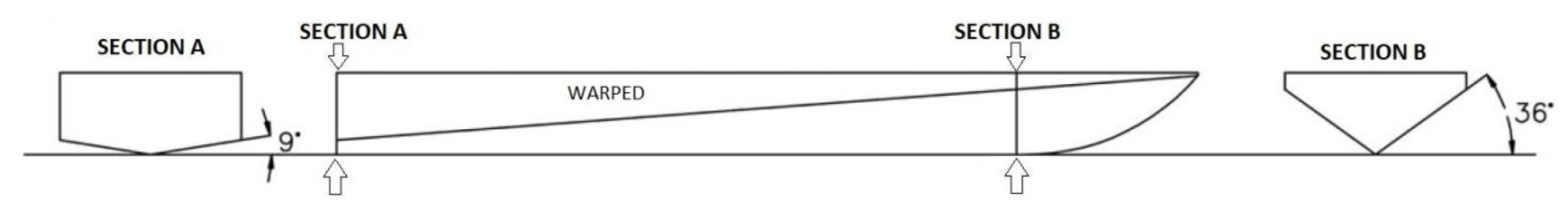

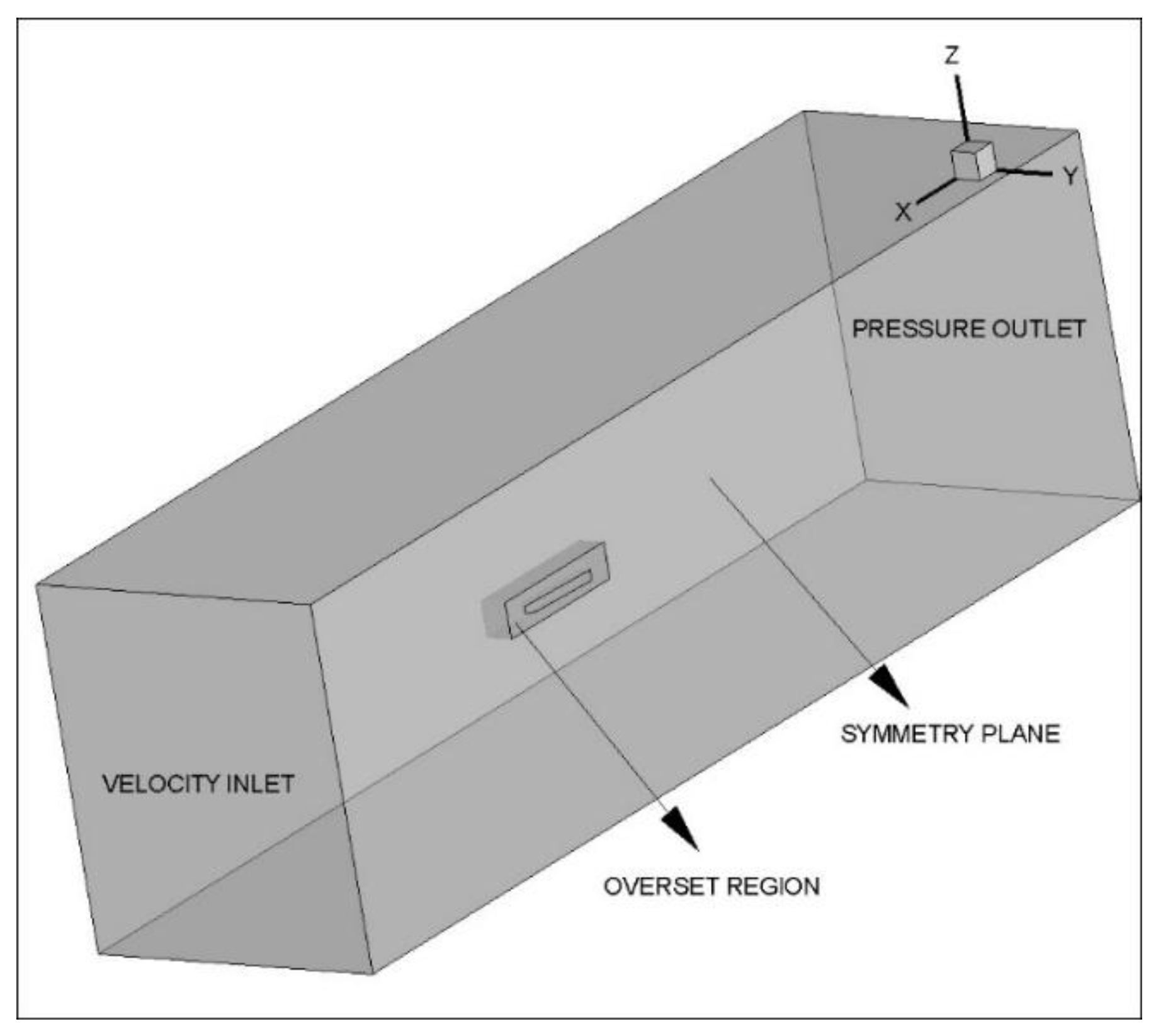




| Main Particular | Mono | Warped |
|---|---|---|
| LOA (m) | 1.900 | 1.900 |
| B (m) | 0.424 | 0.424 |
| Δ (N) | 319.7 | 318.5 |
| LCG (m) | 0.697 | 0.586 |
| VCG (m) | 0.143 | 0.156 |
| kyy (m) | 0.583 | 0.519 |
| TAP (m) | 0.096 | 0.108 |
| β (deg) | 16.70 | 9.09–35.75 |
| FnB (-) | 1.67, 2.26, 2.82 | |
| VOF scheme | Second-order |
| Convectional discretization | Second-order |
| Temporal discretization | First-order |
| Interpolation option | Linear |
| Iteration per one time-step | 10 |
| Parameter | Grid Spacing | Time Step |
|---|---|---|
| R | ||
| X1 (Fine) | 1.080 | 1.109 |
| X2(Medium) | 1.114 | 1.080 |
| X3 (Coarse) | 1.184 | 1.022 |
| R | 0.49 | 0.51 |
| GCI FINE | 3.90% | 3.42% |
| Models | FnB (-) | Case Code | ω (rad/s) | k (rad/m) | λ (m) | A (m) | λ/L |
|---|---|---|---|---|---|---|---|
| Mono and Warped | 1.67 2.26 2.82 | C1 | 5.65 | 3.260 | 1.928 | 0.020 | 1.014 |
| C2 | 5.03 | 2.576 | 2.440 | 0.020 | 1.284 | ||
| C3 | 4.40 | 1.972 | 3.186 | 0.020 | 1.677 | ||
| C4 | 4.08 | 1.700 | 3.695 | 0.032 | 1.945 | ||
| C5 | 3.77 | 1.449 | 4.337 | 0.032 | 2.283 | ||
| C6 | 3.46 | 1.217 | 5.161 | 0.035 | 2.717 | ||
| C7 | 3.14 | 1.006 | 6.245 | 0.035 | 3.287 | ||
| C8 | 2.83 | 0.815 | 7.710 | 0.045 | 4.058 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kahramanoğlu, E.; Çakıcı, F.; Doğrul, A. Numerical Prediction of the Vertical Responses of Planing Hulls in Regular Head Waves. J. Mar. Sci. Eng. 2020, 8, 455. https://doi.org/10.3390/jmse8060455
Kahramanoğlu E, Çakıcı F, Doğrul A. Numerical Prediction of the Vertical Responses of Planing Hulls in Regular Head Waves. Journal of Marine Science and Engineering. 2020; 8(6):455. https://doi.org/10.3390/jmse8060455
Chicago/Turabian StyleKahramanoğlu, Emre, Ferdi Çakıcı, and Ali Doğrul. 2020. "Numerical Prediction of the Vertical Responses of Planing Hulls in Regular Head Waves" Journal of Marine Science and Engineering 8, no. 6: 455. https://doi.org/10.3390/jmse8060455
APA StyleKahramanoğlu, E., Çakıcı, F., & Doğrul, A. (2020). Numerical Prediction of the Vertical Responses of Planing Hulls in Regular Head Waves. Journal of Marine Science and Engineering, 8(6), 455. https://doi.org/10.3390/jmse8060455






