Long-Term Performance of Mega-Nourishments: Role of Directional Wave Climate and Initial Geometry
Abstract
1. Introduction
2. Methodology
2.1. Morphology of the Zm and Prevailing Wave Conditions
2.2. Model Description
2.3. Model Settings
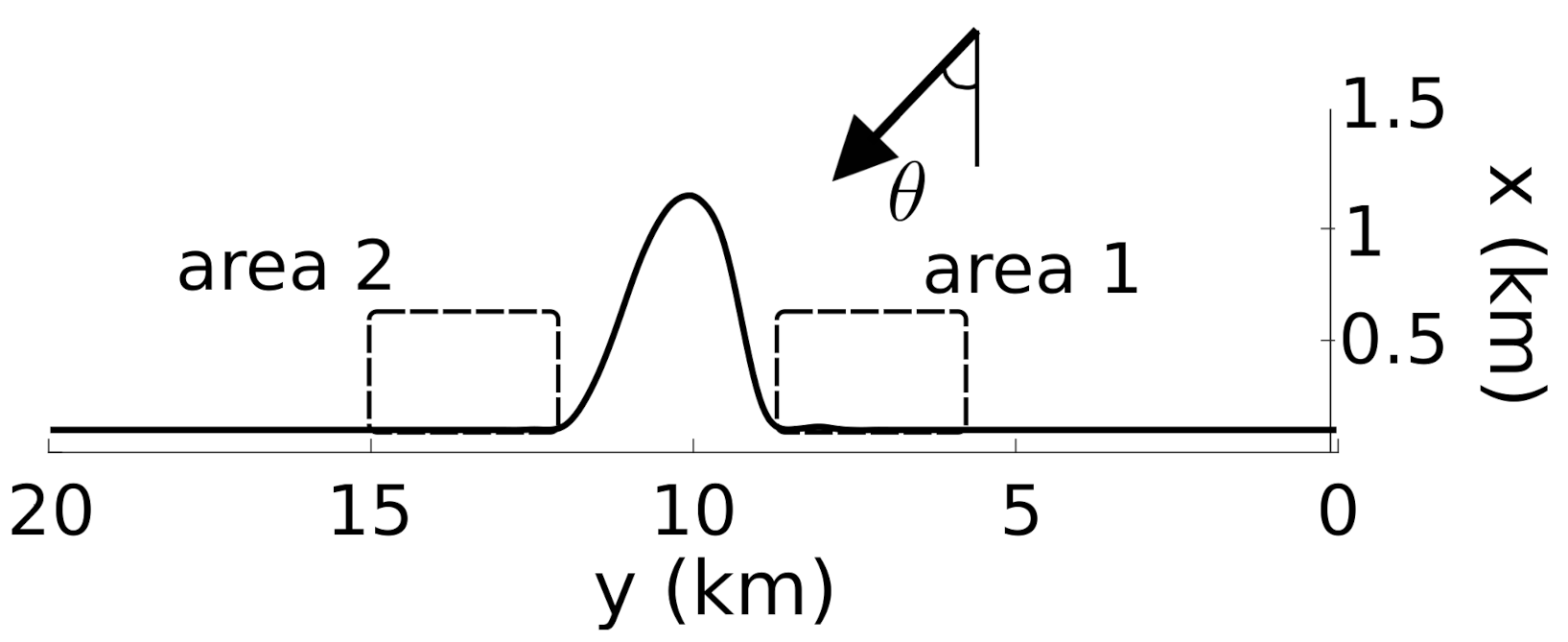
2.4. Metrics for Mega-Nourishment Performance
3. Effect of Varying the Wave Angle
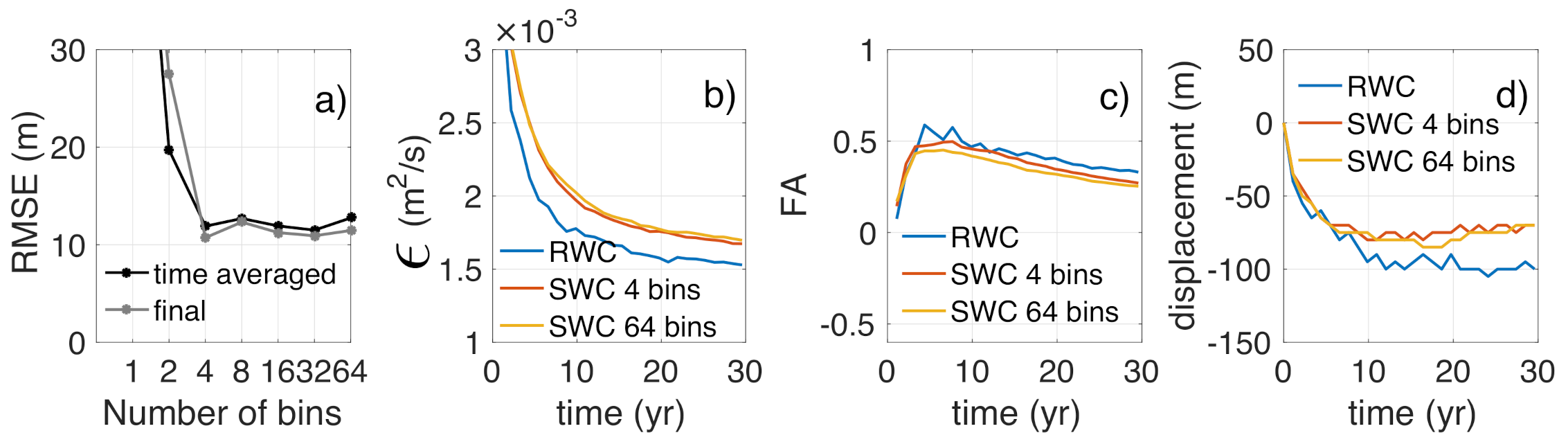
3.1. Design and Validation of the Synthetic Wave Climate
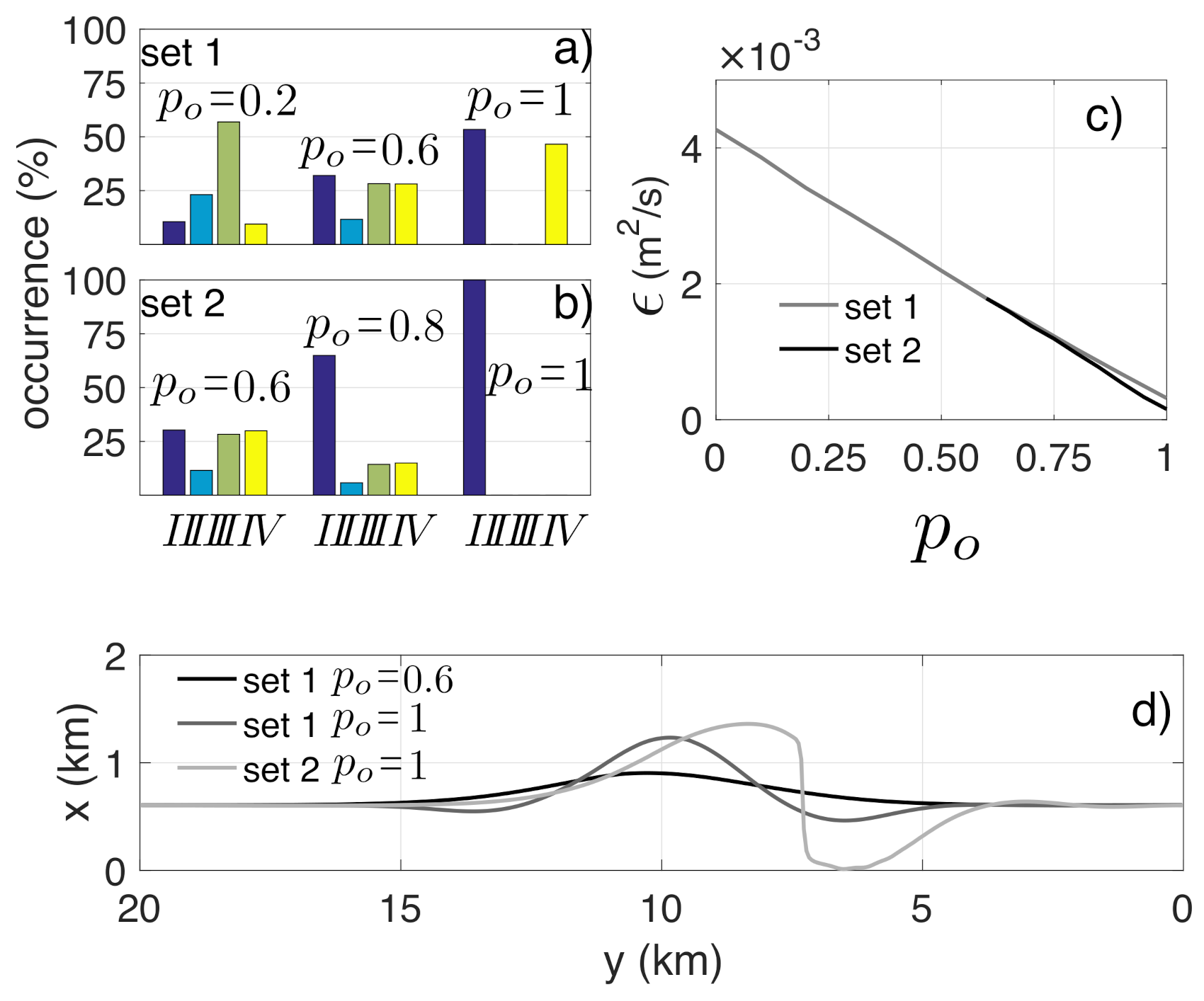
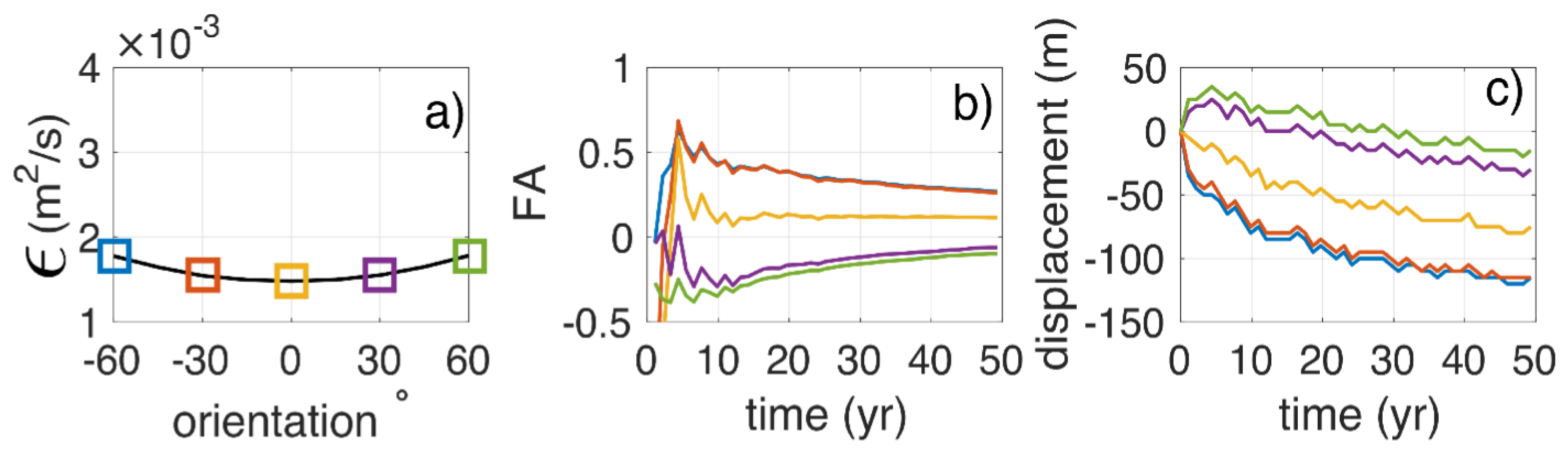
3.2. Sensitivity to the Frequency of High-Angle Waves
4. Effect of Varying the Mega-Nourishment Geometry
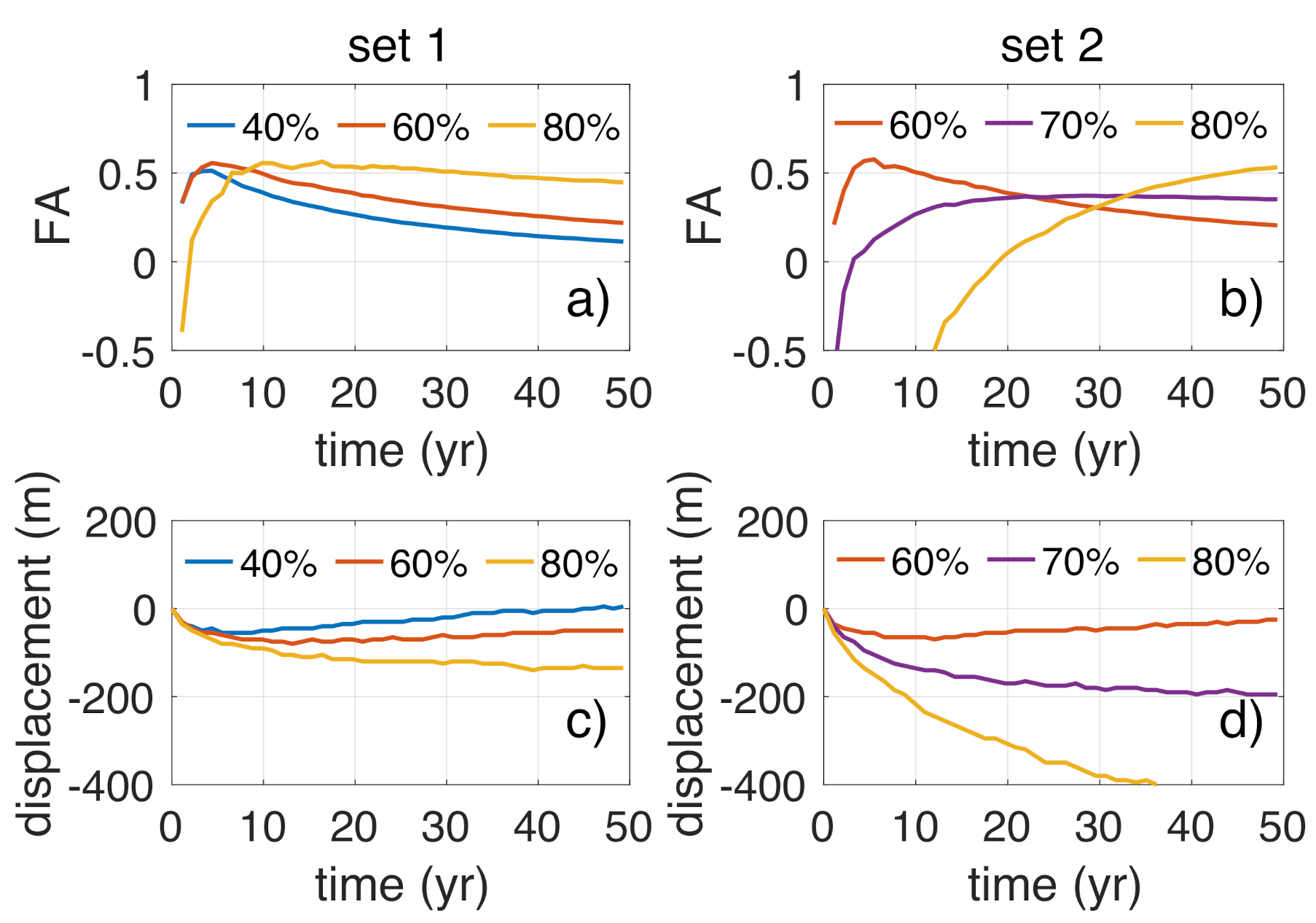
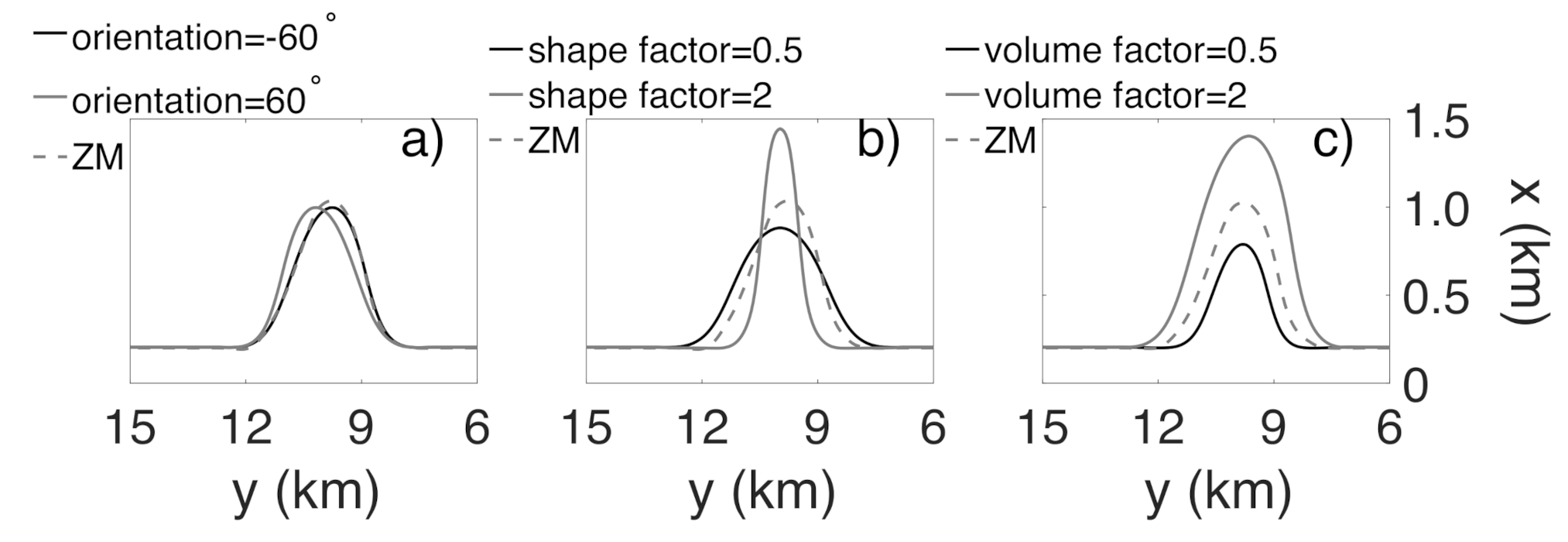
4.1. Sensitivity to the Initial Asymmetry
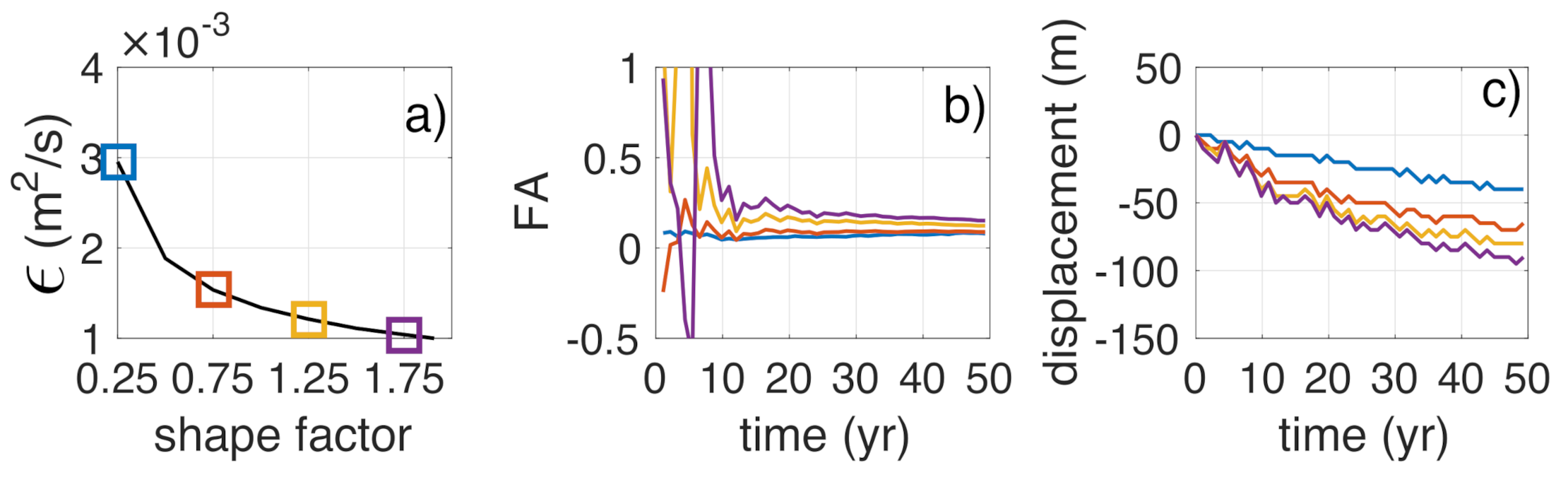
4.2. Sensitivity to the Initial Aspect Ratio
4.3. Sensitivity to The Volume
5. Discussion
5.1. Physical Processes Driving Mega-Nourishment Evolution
5.1.1. Importance of High-Angle Waves
5.1.2. Diffusivity and Feeding Asymmetry
5.2. Design Recommendations
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
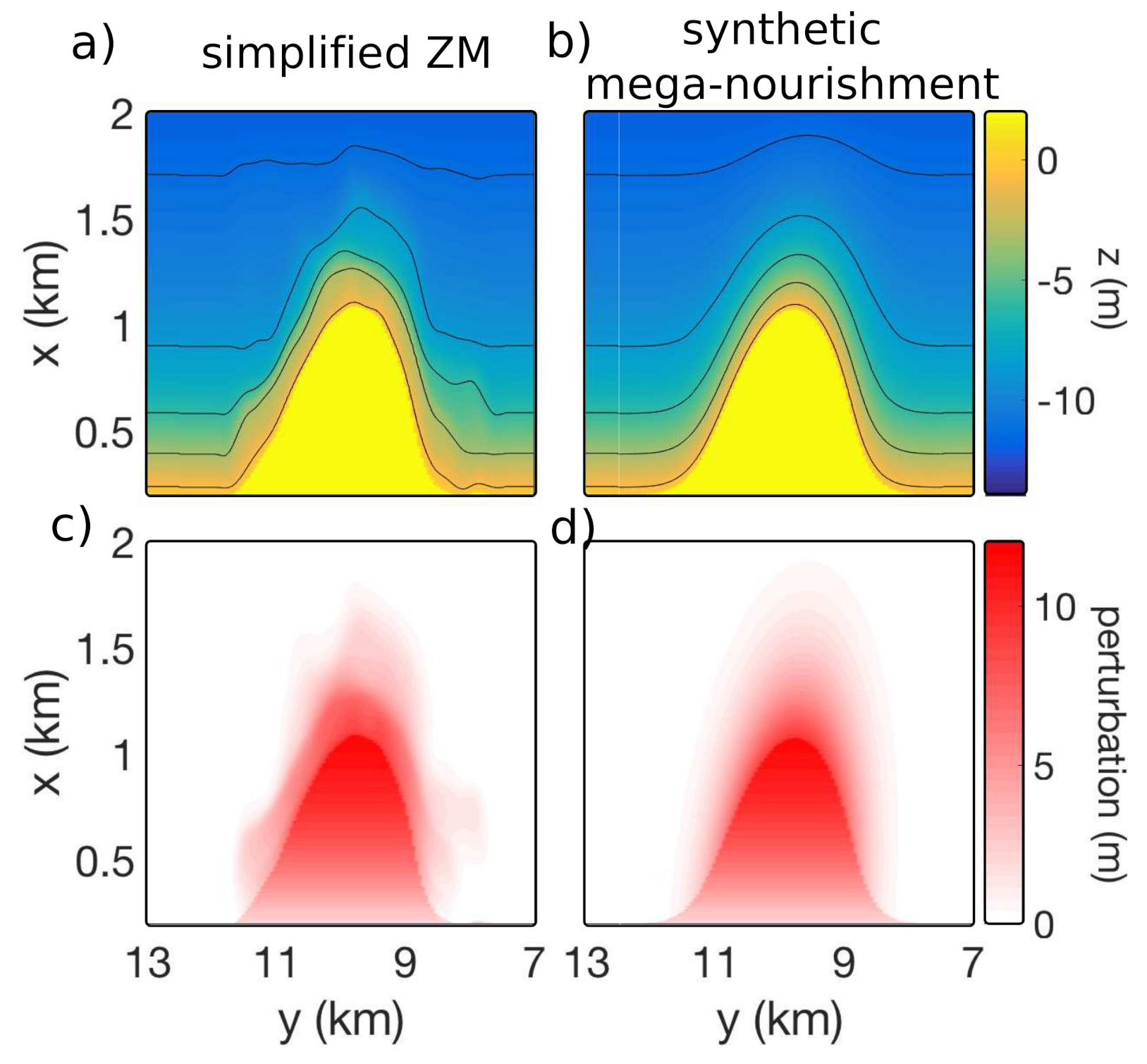
Appendix A. Design of the Synthetic Mega-Nourishment

References
- Stive, M.J.F.; de Schipper, M.A.; Luijendijk, A.P.; Aarninkhof, S.G.J.; van Gelder-Maas, C.; van Thiel de Vries, J.S.M.; de Vries, S.; Henriquez, M.; Marx, S.; Ranasinghe, R. A new alternative to saving our beaches from sea-level rise: The Sand Engine. Coast. Eng. 2013, 29, 1001–1008. [Google Scholar] [CrossRef]
- Hamm, L.; Capobianco, M.; Dette, H.H.; Lechuga, A.; Spanhoff, R.; Stive, M.J.F. A summary of European experience with shore nourishment. Coast. Eng. 2002, 47, 237–264. [Google Scholar] [CrossRef]
- de Schipper, M.A.; De Vries, S.; Ruessink, B.G.; De Zeeuw, R.C.; Rutten, J.; Van Gelder-Maas, C.; Stive, M.J.F. Initial spreading of a mega feeder nourishment: Observations of the Sand Engine pilot project. Coast. Eng. 2016, 111, 23–38. [Google Scholar] [CrossRef]
- Luijendijk, A.P.; Ranasinghe, R.; de Schipper, M.A.; Huisman, B.A.; Swinkels, C.M.; Walstra, D.J.R.; Stive, M.J.F. The initial morphological response of the Sand Engine: A process-based modelling study. Coast. Eng. 2017, 119, 1–14. [Google Scholar] [CrossRef]
- Rutten, J.; Ruessink, B.G.; Price, T.D. Observations on sandbar behaviour along a man-made curved coast. Earth Surf. Process. Landforms 2018, 43, 134–149. [Google Scholar] [CrossRef]
- Lesser, G.R.; Roelvink, J.A.; van Kester, J.A.T.M.; Stelling, G.S. Development and validation of a three-dimensional morphological model. Coastal Eng. 2004, 51, 883–915. [Google Scholar] [CrossRef]
- Ruggiero, P.; Buijsman, M.; Kaminsky, G.M.; Gelfenbaum, G. Modeling the effects of wave climate and sediment supply variability on large-scale shoreline change. Mar. Geol. 2010, 273, 127–140. [Google Scholar] [CrossRef]
- Luijendijk, A.P.; de Schipper, M.A.; Ranasinghe, R. Morphodynamic Acceleration Techniques for Multi-Timescale Predictions of Complex Sandy Interventions. J. Mar. Sci. Eng. 2019, 7, 78. [Google Scholar] [CrossRef]
- Falqués, A.; Garnier, R.; Ojeda, E.; Ribas, F.; Guillén, J. Q2D-morfo: A medium to long term model for beach morphodynamics. In River, Coastal and Estuarine Morphodynamics: RCEM 2007; Dohmen-Jansen, C.M., Hulscher, S.J.M.H., Eds.; Taylor and Francis Group: London, UK, 2008; Volume 1, pp. 71–78. [Google Scholar]
- van den Berg, N.; Falqués, A.; Ribas, F. Long-term evolution of nourished beaches under high angle wave conditions. J. Mar. Syst. 2011, 88, 102–112. [Google Scholar] [CrossRef]
- Arriaga, J.; Rutten, J.; Ribas, F.; Ruessink, B.; Falqués, A. Modeling the longterm diffusion and feeding capability of a mega-nourishment. Coast. Eng. 2017, 121, 1–13. [Google Scholar] [CrossRef]
- Tonnon, P.K.; Huisman, B.J.A.; Stam, G.N.; van Rijn, L.C. Numerical modelling of erosion rates, life span and maintenance volume of mega nourishments. Coast. Eng. 2018, 131, 51–69. [Google Scholar] [CrossRef]
- Falqués, A.; Calvete, D. Large scale dynamics of sandy coastlines. Diffusivity and instability. J. Geophys. Res. 2005, 110. [Google Scholar] [CrossRef]
- Idier, D.; Falqués, A.; Rohmer, J.; Arriaga, J. Self-organized kilometre-scale shoreline sandwave generation: Sensitivity to model and physical parameters. J. Geophys. Res. 2017, 122. [Google Scholar] [CrossRef]
- Ashton, A.; Murray, A.B. High-angle wave instability and emergent shoreline shapes: 1. Modeling of sand waves, flying spits, and capes. J. Geophys. Res. 2006, 111, F04011. [Google Scholar] [CrossRef]
- Kaergaard, K.; Fredsoe, J. Numerical modeling of shoreline undulations part 2: Varying wave climate and comparison with observations. Coastal Eng. 2013, 75, 77–90. [Google Scholar] [CrossRef]
- Kaergaard, K.; Fredsoe, J. Numerical modeling of shoreline undulations part 1: Constant wave climate. Coastal Eng. 2013, 75, 64–76. [Google Scholar] [CrossRef]
- Falqués, A. Wave driven alongshore sediment transport and stability of the Dutch coastline. Coast. Eng. 2006, 53, 243–254. [Google Scholar] [CrossRef]
- Ashton, A.; Murray, A.B.; Arnault, O. Formation of coastline features by large-scale instabilities induced by high-angle waves. Nature 2001, 414, 296–300. [Google Scholar] [CrossRef]
- Falqués, A.; Ribas, F.; Idier, D.; Arriaga, J. Formation mechanisms for self-organized kilometer-scale shoreline sand waves. J. Geophys. Res. Earth Surf. 2017, 122, 10.1002/2016JF003964. [Google Scholar] [CrossRef]
- Portos, L. Effect of Sea Level Variations in the Long-Term Dynamics of the Zandmotor Meganourishment. Bachelor’s Thesis, Universitat Politècnica de Catalunya, Barcelona, Spain, 2020. [Google Scholar]
- van den Berg, N.; Falqués, A.; Ribas, F. Modelling large scale shoreline sand waves under oblique wave incidence. J. Geophys. Res. 2012, 117. [Google Scholar] [CrossRef]
- Komar, P.D. Beach Processes and Sedimentation, 2nd ed.; Prentice Hall: Englewood Cliffs, NJ, USA, 1998. [Google Scholar]
- Battjes, J.A. Modeling of turbulence in the surfzone. Symp. Model. Tech. 1975, 2, 1050–1061. [Google Scholar]
- Pelnard-Considère, R. Essai de theorie de l’Evolution des Formes de Rivage en Plages de Sable et de Galets. Journees L’Hydraulique 1956, 3, 289–298. [Google Scholar]
- Falqués, A. On the diffusivity in coastline dynamics. Geophys. Res. Lett. 2003, 30, 2119. [Google Scholar] [CrossRef]
- Ashton, A.; Murray, A.B. High-angle wave instability and emergent shoreline shapes: 2. Wave climate analysis and comparisons to nature. J. Geophys. Res. 2006, 111, F04012. [Google Scholar] [CrossRef]
- Roelvink, J.A.; Reniers, A.J.H.M. A guide to modeling coastal morphology. In Advances in Coastal and Ocean Engineering; World Scientific: Singapore, 2011. [Google Scholar] [CrossRef]
- Walstra, D.J.R.; Hoekstra, R.; Tonnon, P.K.; Ruessink, B.G. Input reduction for long-term morphodynamic simulations in wave-dominated coastal settings. Coast. Eng. 2013, 77, 57–70. [Google Scholar] [CrossRef]
- Benedet, L.; Dobrochinski, J.P.F.; Walstra, D.J.R.; Klein, A.H.F.; Ranasinghe, R. A morphological modeling study to compare different methods of wave climate schematization and evaluate strategies to reduce erosion losses from a beach nourishment project. Coast. Eng. 2016, 112, 69–86. [Google Scholar] [CrossRef]
- Yu, J.; Slinn, D.N. Effects of wave-current interaction on rip currents. J. Geophys. Res. 2003, 108. [Google Scholar] [CrossRef]



| Sector I (33%) | Sector II (11%) | Sector III (27%) | Sector IV (29%) | Shoreline | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Method | H(m) | (deg) | T (s) | H (m) | (deg) | T (s) | H (m) | (deg) | T (s) | H (m) | (deg) | T (s) | RMSE (m) |
| 1 | 1.4 | −74.2 | 5.8 | 1.3 | −21.4 | 5.7 | 1.2 | 27.6 | 6.3 | 1.0 | 63.3 | 5.8 | 56 |
| 2 | 1.8 | −76.5 | 7.0 | 1.7 | −22.0 | 7.2 | 1.5 | 23.9 | 7.4 | 1.2 | 65.5 | 6.5 | 11 |
| 3 | 1.7 | −72.3 | 6.8 | 1.6 | −21.3 | 7.0 | 1.4 | 24.1 | 7.2 | 1.2 | 62.6 | 6.4 | 17 |
| 4 | 1.9 | −76.7 | 7.2 | 1.8 | −22.1 | 7.4 | 1.7 | 23.1 | 7.6 | 1.3 | 65.9 | 6.7 | 22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arriaga, J.; Ribas, F.; Falqués, A.; Rutten, J.; Ruessink, G. Long-Term Performance of Mega-Nourishments: Role of Directional Wave Climate and Initial Geometry. J. Mar. Sci. Eng. 2020, 8, 965. https://doi.org/10.3390/jmse8120965
Arriaga J, Ribas F, Falqués A, Rutten J, Ruessink G. Long-Term Performance of Mega-Nourishments: Role of Directional Wave Climate and Initial Geometry. Journal of Marine Science and Engineering. 2020; 8(12):965. https://doi.org/10.3390/jmse8120965
Chicago/Turabian StyleArriaga, Jaime, Francesca Ribas, Albert Falqués, Jantien Rutten, and Gerben Ruessink. 2020. "Long-Term Performance of Mega-Nourishments: Role of Directional Wave Climate and Initial Geometry" Journal of Marine Science and Engineering 8, no. 12: 965. https://doi.org/10.3390/jmse8120965
APA StyleArriaga, J., Ribas, F., Falqués, A., Rutten, J., & Ruessink, G. (2020). Long-Term Performance of Mega-Nourishments: Role of Directional Wave Climate and Initial Geometry. Journal of Marine Science and Engineering, 8(12), 965. https://doi.org/10.3390/jmse8120965






