Mechanics and Historical Evolution of Sea Level Blowouts in New York Harbor
Abstract
1. Introduction
2. Background
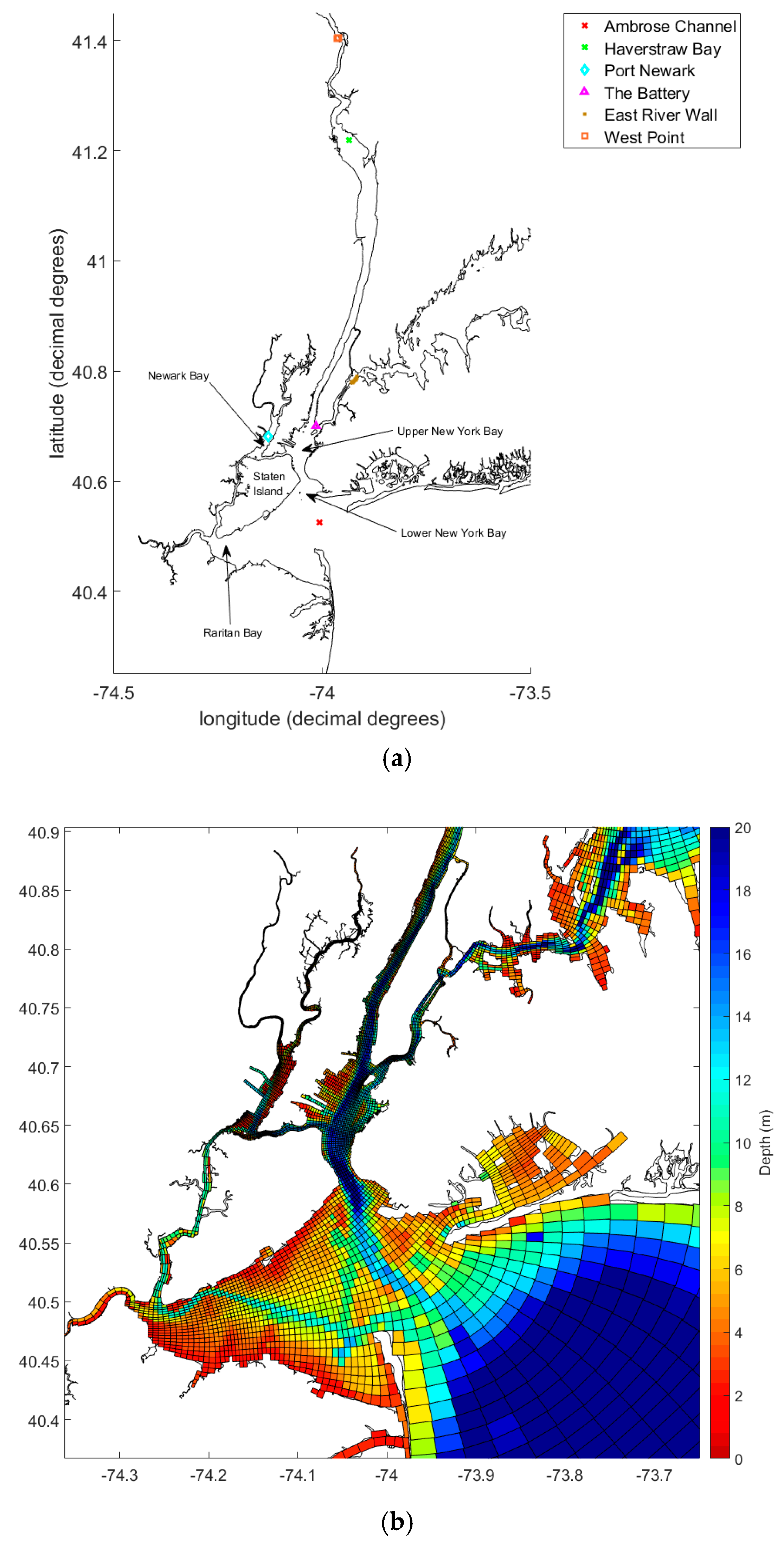
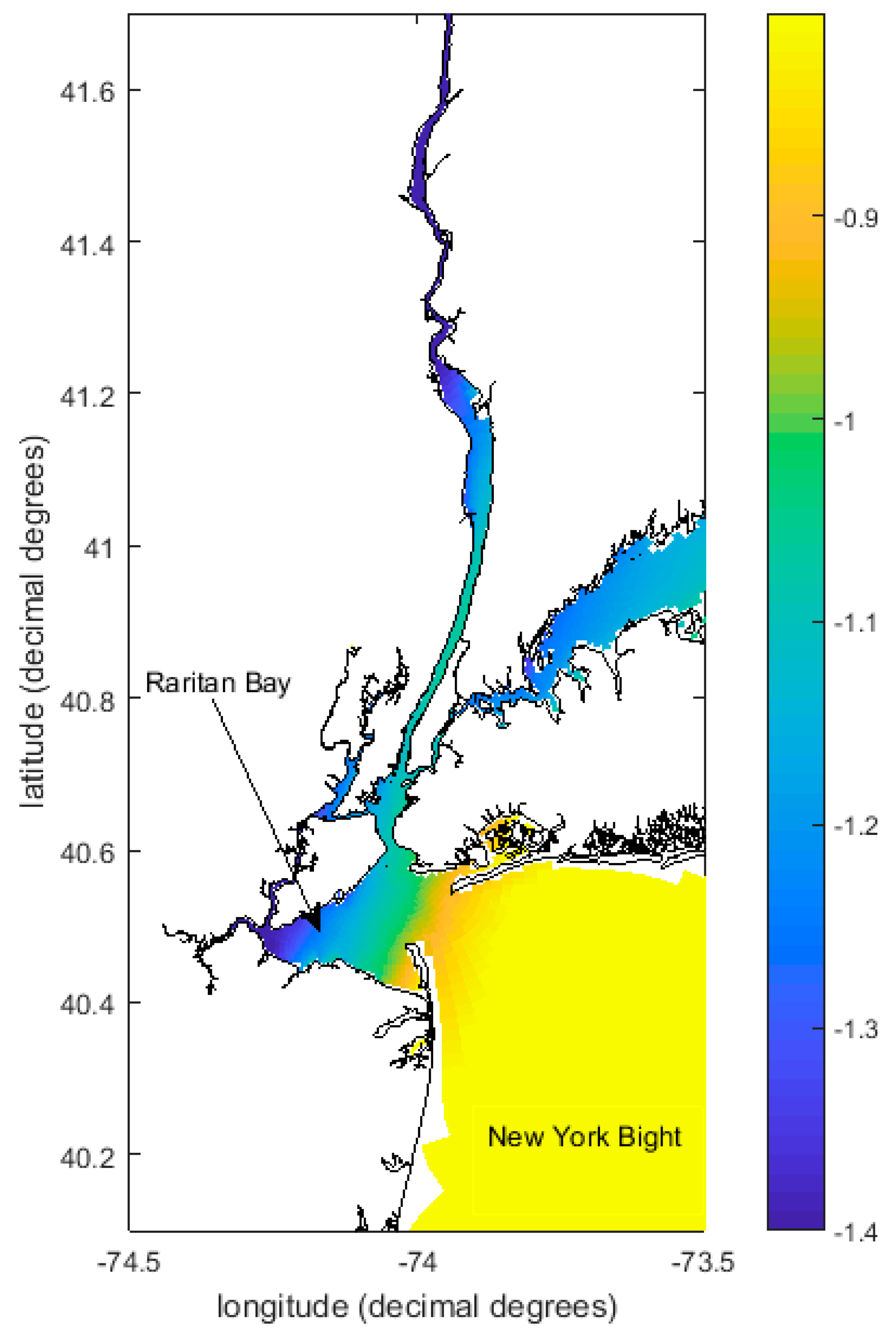
2.1. Shipping Channel Bathymetry
2.2. Reefs
2.3. Ice Formation on the Hudson
3. Methodology
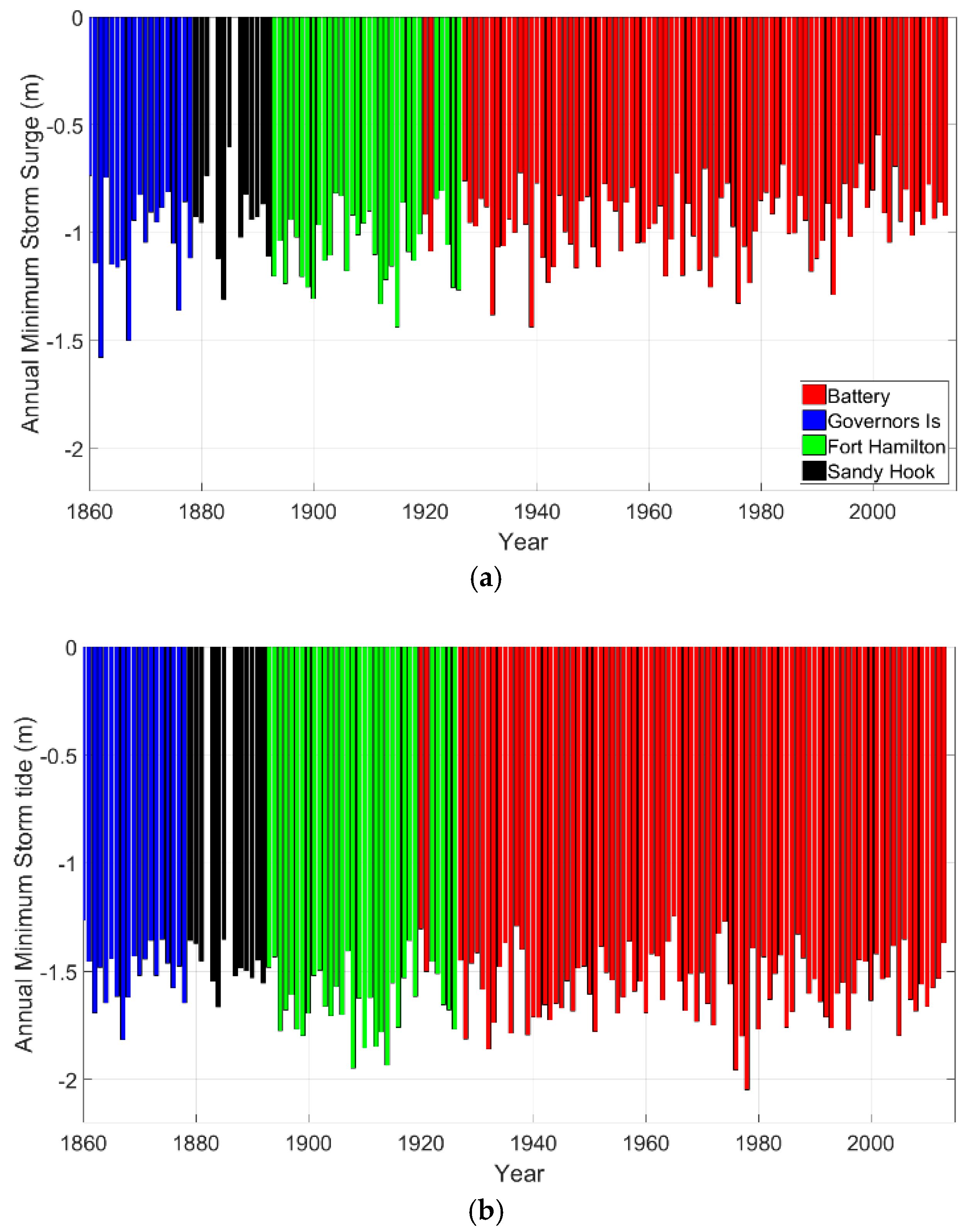
3.1. Historical Data Compilation and Extreme Value Analysis
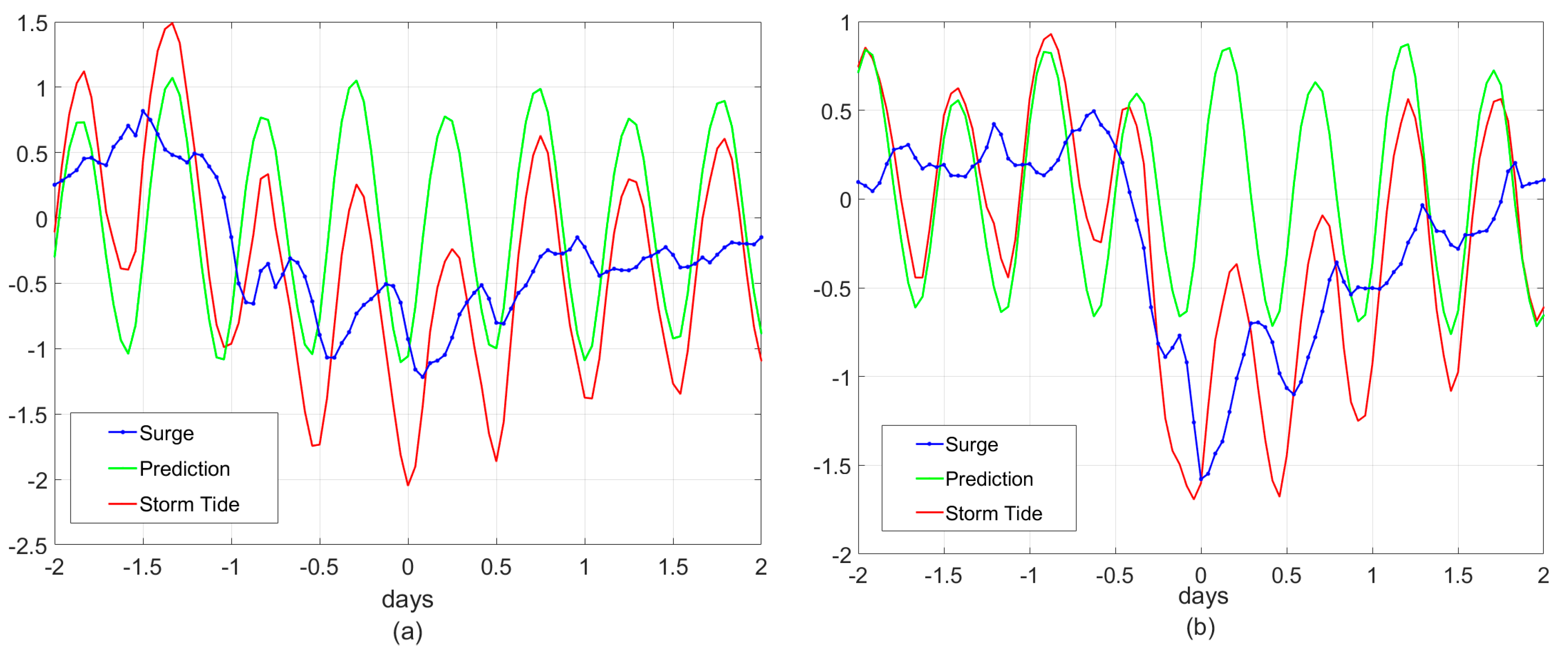
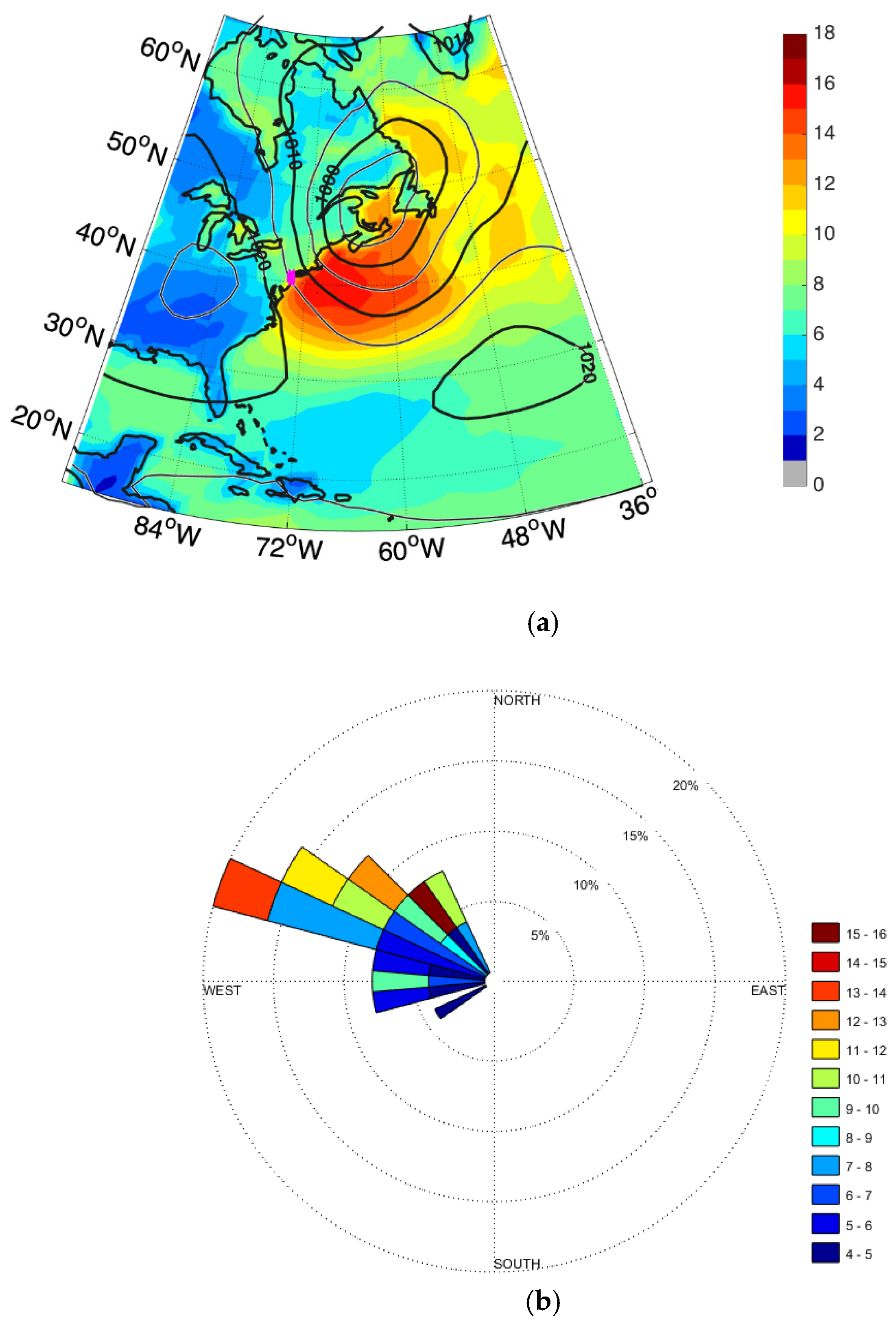
3.2. Meteorological Conditions during Past Blowouts
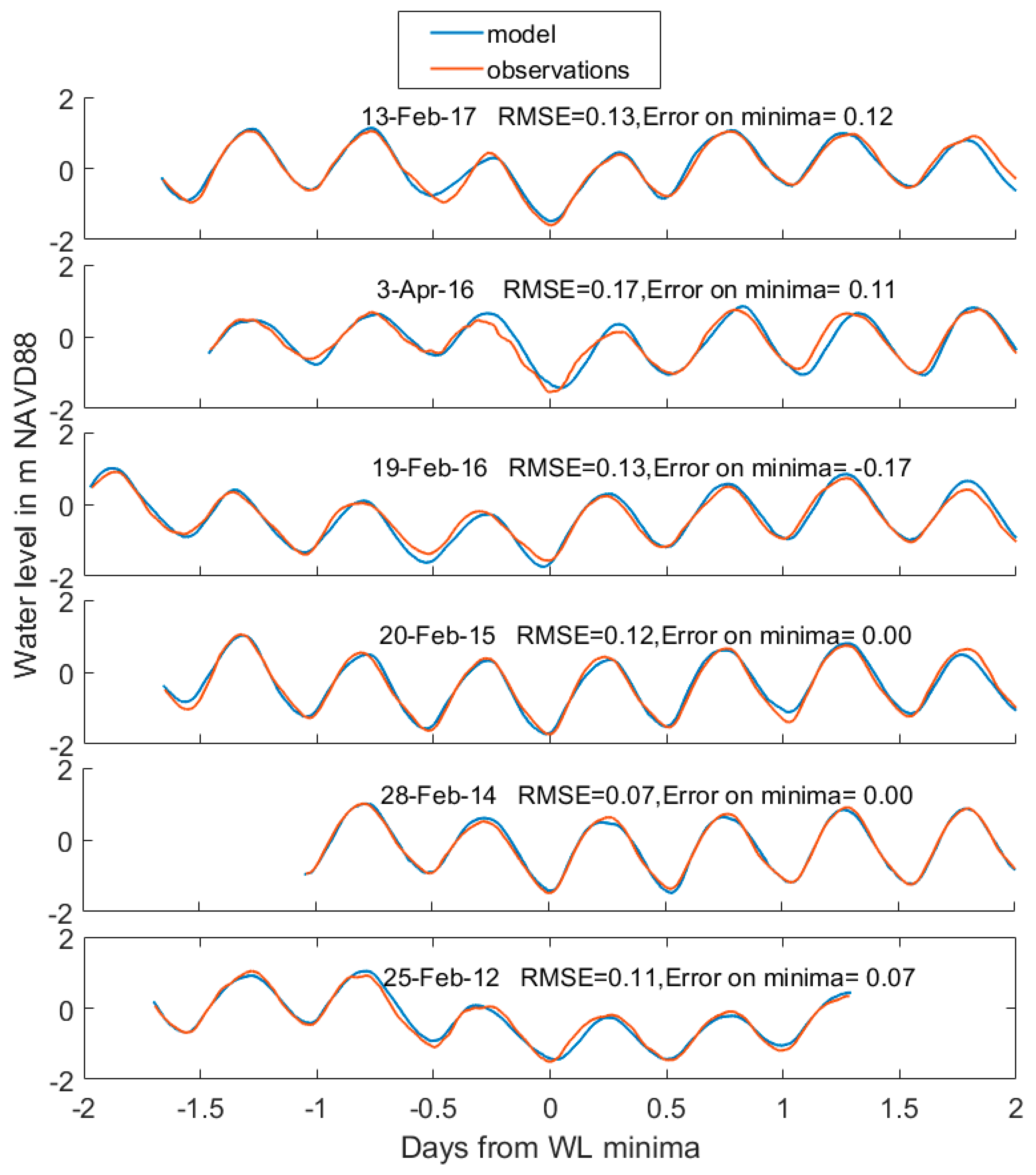
3.3. Hydrodynamic Modeling Methods and Water Level Validation
3.4. Quantifying Blowout Sensitivities
3.4.1. Wind Stress-Tests
3.4.2. Sensitivity to Altered Geometry and Environmental Factors (Emulating the Historical Changes)
- (a)
- An extreme case scenario of the reefs in the East River is emulated by building a wall near Hell Gate (shown as East River wall in Figure 1a). This is done by changing the depth to a negative value to extend out of the free-surface of the river. Hence, this wall stops the flow of water in and out of Hell Gate.
- (b)
- To emulate a choke point constraining the flow of water in the Hudson, an extreme case scenario is modeled in which an ice jam in the river would completely stop the flow of water to the south. The depth is changed to a negative value to replicate a dam in the grid cells across the river near West Point (shown in Figure 1a). West Point is chosen because it has been identified historically as a ‘choke point’ due to its tendency to form shore-fast ice cover which restricts ship traffic.
- (c)
- To mimic the impact of more commonly observed shore-fast ice cover, the surface ice friction is emulated by imposing a nominal horizontal drag coefficient at the free-surface, which is triple the bottom friction drag coefficient, to the grid cells in the Hudson to the north of 41.1° N, where shore-fast ice is commonly observed in wintertime. These surface drag coefficients were used by Georgas [14] to explain the tidal modulations due to ice cover in the Hudson River. Additionally, an extreme case scenario with a surface drag coefficient six times the bottom friction coefficient (twice the nominal value) is also tested.
- (d)
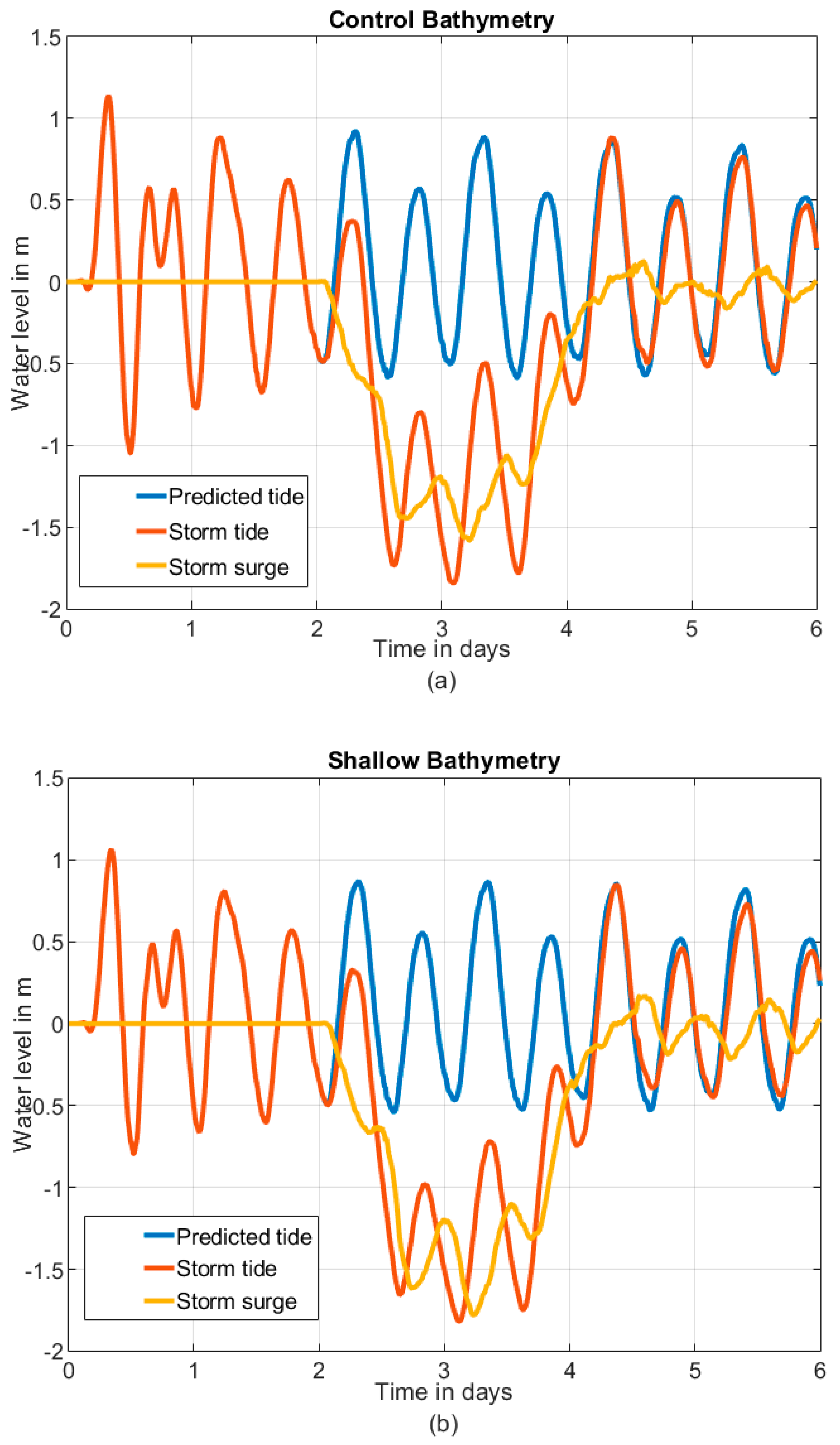
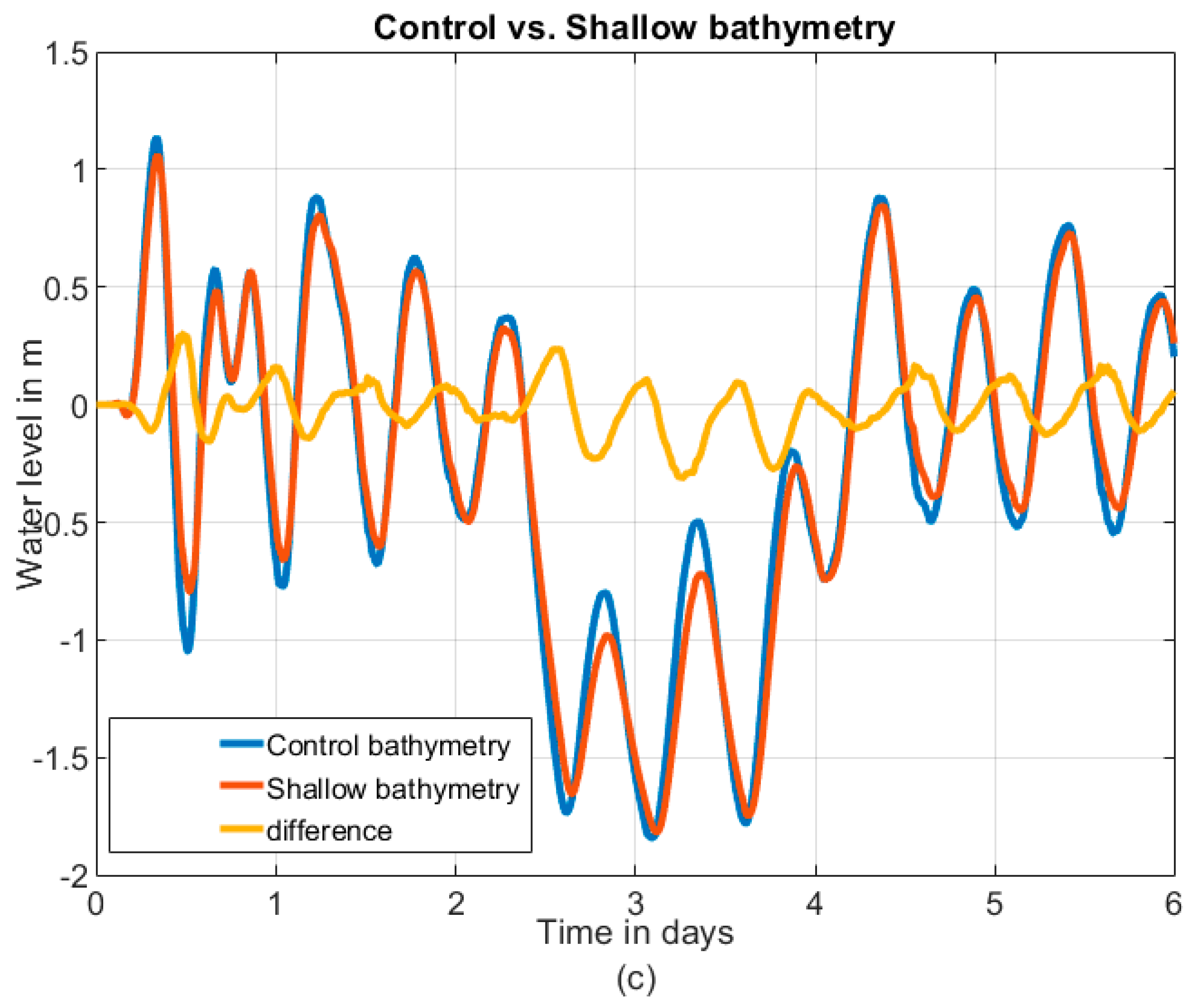
- The historically-dredged shipping channels at the harbor entrance areas of Raritan Bay and Lower New York Bay south of latitude 40.56° N (Figure 1b) are shallowed in the model by setting the depth of the grid cells in the sections of the shipping channels deeper than six meters equal to six meters. This value is the approximate pre-dredging average bathymetric depth across the Ambrose Channel area, estimated from the Hassler Chart of NYH [18].
4. Results and Discussion
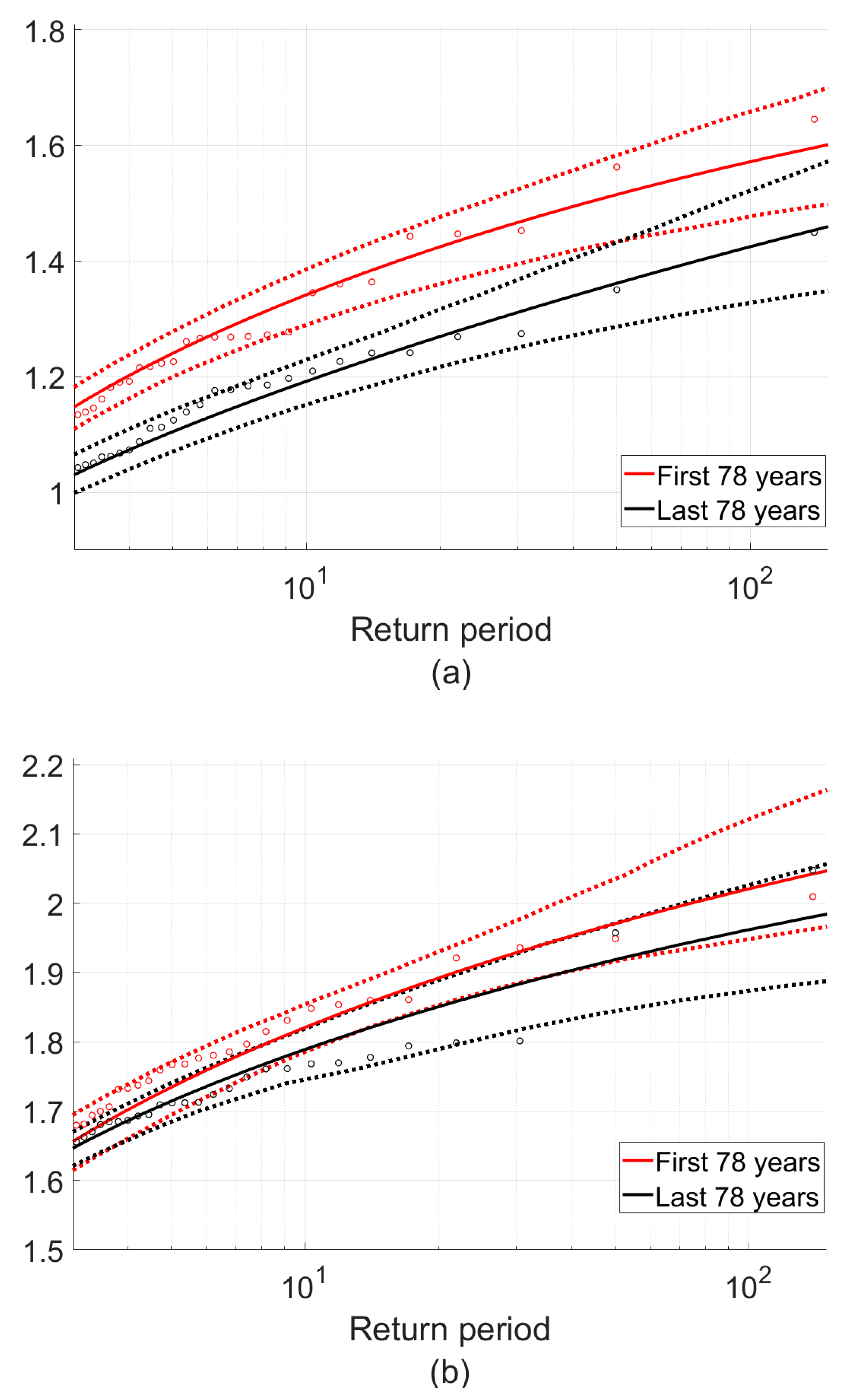
4.1. Historical Evolution of Blowouts
4.2. Observed Meteorological Conditions for Blowout Events
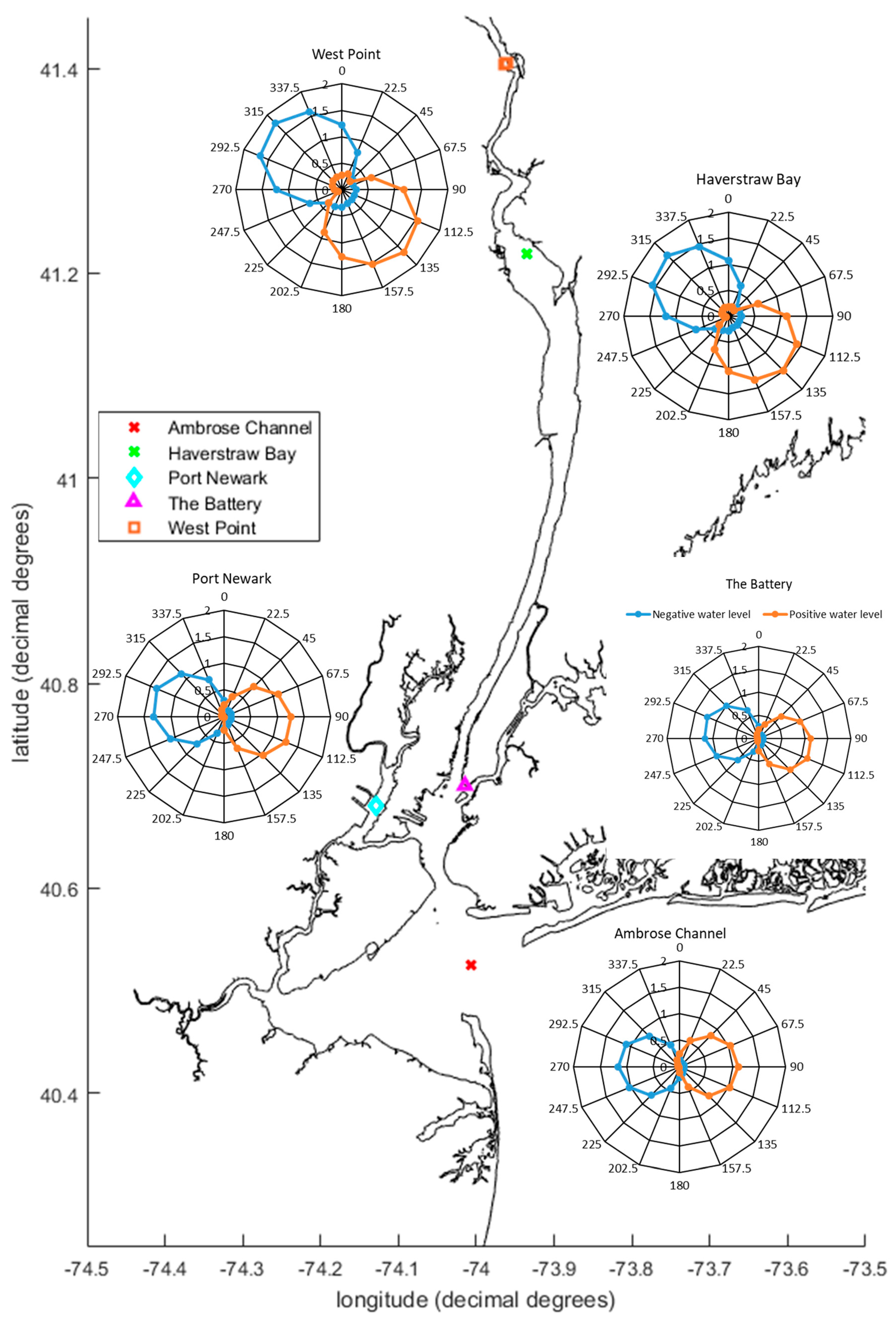
4.3. Model-Based Blowout and Storm Surge Sensitivity to Wind Direction
4.4. Blowout Sensitivity to Environmental Factors
- (a)
- East River wall: in the simulation with a west-northwest (WNW) wind, a wall in the East River increases the minimum water level in The Battery by a small amount (2.6 cm). This could be due to the wind stress driving the water in NYH from the west to east and up the East River. A presence of a wall could lead to accumulation of water in the East River and to a lesser extent near The Battery, hence increasing the water level locally.
- (b)
- Ice jam: in the case of an ice jam at West Point in the Hudson River, the wind driven water level minimum at The Battery also did not change substantially (Table 1). This could be due to the long distance from West Point to The Battery, which could spread out or attenuate any sea surface slope produced by the ice jam.
- (c)
- Ice friction sensitivity: the simulated ice-friction in the Hudson River produced a relatively insignificant effect (1 cm) on the water levels at The Battery (Table 1) even though the surface ice frictional coefficient was twice the normal value. Hence, we conclude that ice-friction did not play a major role in evolution of negative surges over the years in NYH. These results were qualitatively comparable to the findings of Georgas [14].
4.5. Bathymetric Sensitivity
4.6. Amplification of Negative Surge Due to the Inverse Coastal Funneling Effect
5. Summary and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Schureman, P. Tides and Currents in Hudson River; Citeseer: Washington, DC, USA, 1934. [Google Scholar]
- Pittman, C. Why Irma drained the water from Tampa Bay. Available online: http://www.tampabay.com/opinion/columns/pittman-why-irma-drained-the-water-from-tampa-bay/2338244 (accessed on 13 May 2019).
- McWhirter, C.; Campo-Flores, A. U.S. Ports See Costly Delays as Cargo Ships, Volumes Grow. Available online: https://www.wsj.com/articles/u-s-ports-see-costly-delays-as-cargo-ships-volumes-grow-1430340113 (accessed on 13 May 2019).
- Sobey, R.J. Extreme low and high water levels. Coast. Eng. 2005, 52, 63–77. [Google Scholar] [CrossRef]
- Pugh, D.; Woodworth, P. Sea-Level Science: Understanding Tides, Surges, Tsunamis and Mean Sea-Level Changes; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]
- Resio, D.T.; Westerink, J.J. Modeling the physics of storm surges. Phys. Today 2008, 9, 33–38. [Google Scholar] [CrossRef]
- Raicich, F. Recent evolution of sea-level extremes at Trieste (Northern Adriatic). Cont. Shelf Res. 2003, 23, 225–235. [Google Scholar] [CrossRef]
- Pugh, D.T.; Vassie, J.M. Extreme Sea Levels from Tide and Surge Probability. In Coastal Engineering 1978; Institute of Ocwanographic Science: Merseyside, UK, 1978. [Google Scholar]
- Talke, S.; Orton, P.; Jay, D. Increasing storm tides at New York City, 1844–2013. Geophys. Res. Lett. 2014, 41, 3149–3155. [Google Scholar] [CrossRef]
- Chant, R.J.; Sommerfield, C.K.; Talke, S.A. Impact of Channel Deepening on Tidal and Gravitational Circulation in a Highly Engineered Estuarine Basin. Estuaries Coasts 2018, 41, 1587–1600. [Google Scholar] [CrossRef]
- Ralston, D.K.; Talke, S.; Geyer, W.R.; Al-Zubaidi, H.A.; Sommerfield, C.K. Bigger tides, less flooding: Effects of dredging on barotropic dynamics in a highly modified estuary. J. Geophys. Res. Ocean. 2019, 124, 196–211. [Google Scholar] [CrossRef]
- Orton, P.M.; Talke, S.A.; Jay, D.A.; Yin, L.; Blumberg, A.F.; Georgas, N.; Zhao, H.; Roberts, H.J.; MacManus, K. Channel Shallowing as Mitigation of Coastal Flooding. J. Mar. Sci. Eng. 2015, 3, 654–673. [Google Scholar] [CrossRef]
- Familikhalili, R.; Talke, S.A. The effect of channel deepening on tides and storm surge: A case study of Wilmington, NC. Geophys. Res. Lett. 2016, 43, 9138–9147. [Google Scholar] [CrossRef]
- Georgas, N. Large Seasonal Modulation of Tides due to Ice Cover Friction in a Midlatitude Estuary. J. Phys. Oceanogr. 2012, 42, 352–369. [Google Scholar] [CrossRef]
- Marcos, M.; Woodworth, P.L. Spatiotemporal changes in extreme sea levels along the coasts of the North Atlantic and the Gulf of Mexico. J. Geophys. Res. Ocean. 2017, 122, 7031–7048. [Google Scholar] [CrossRef]
- Field, A.M. Port Productivity: The Cooperation Revolution at the Port of New York and New Jersey. J. Commer. 2015. Available online: https://www.joc.com/sites/default/files/u59196/Whitepapers/NYNJ_PortProductivity/WP-NYNJ-v4-agbJAY.pdf (accessed on 23 March 2019).
- Ascher, K. Going Up! A Bridge Makes Way for Bigger Ships. The New York Times, 2014. Available online: https://www.nytimes.com/2014/03/23/nyregion/going-up-a-bridge-makes-way-for-bigger-ships.html (accessed on 23 March 2019).
- Hassler, F.R. Map of New-York Bay and Harbor and the Environs; United States Coast Survey: Washington, DC, USA, 1844. [Google Scholar]
- Keegan, G.C. The Dredging Crisis In New York Harbor: Present and Future Problems, Present and Future Solutions. Fordham Environ. Law J. 1997, 8, 351–388. [Google Scholar]
- United States Army Corps of Engineers. Report of the Chief of Engineers, U.S. Army; U.S. Government Printing Office: Washington, DC, USA, 1920.
- American Society of Civil Engineers. Transactions of the American Society of Civil Engineers; American Society of Civil Engineers: Reston, VA, USA, 1921. [Google Scholar]
- USACE Fact Sheet—New York and New Jersey Harbor Deepening. Available online: http://www.nan.usace.army.mil/Media/Fact-Sheets/Fact-Sheet-Article-View/Article/487407/fact-sheet-new-york-new-jersey-harbor-50-ft-deepening/ (accessed on 13 May 2019).
- Orton, P.; Georgas, N.; Blumberg, A.; Pullen, J. Detailed modeling of recent severe storm tides in estuaries of the New York City region. J. Geophys. Res. 2012, 117, C09030. [Google Scholar] [CrossRef]
- Georgas, N.; Miller, J.; Wang, Y.; Jian, Y.; D’Agostino, D. Tidal Hudson River Ice Cover Climatology; Hudson River Sustainable Shorelines Project: Staatsburg, NY, USA, 2015. [Google Scholar]
- Pawlowicz, R.; Beardsley, B.; Lentz, S. Classical tidal harmonic analysis including error estimates in MATLAB using T_TIDE. Comput. Geosci. 2002, 28, 929–937. [Google Scholar] [CrossRef]
- Leffler, K.E.; Jay, D.A. Enhancing tidal harmonic analysis: Robust (hybrid L1/L2) solutions. Cont. Shelf Res. 2009, 29, 78–88. [Google Scholar] [CrossRef]
- Dee, D.P.; Uppala, S.M.; Simmons, A.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.; Balsamo, G.; Bauer, D.P. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- NOAA Center for Operational Oceanographic Products and Services. Available online: https://tidesandcurrents.noaa.gov/ (accessed on 11 May 2019).
- Blumberg, A.F.; Mellor, G.L. A description of a three-dimensional coastal ocean circulation model. In Three-Dimensional Coastal Ocean Models; Heaps, N.S., Ed.; American Geophysical Union: Washington, DC, USA, 1987; Volume 4, pp. 1–16. [Google Scholar]
- Blumberg, A.F.; Khan, L.A.; St John, J. Three-dimensional hydrodynamic model of New York Harbor region. J. Hydraul. Eng. 1999, 125, 799–816. [Google Scholar] [CrossRef]
- Georgas, N.; Blumberg, A.; Herrington, T. An operational coastal wave forecasting model for New Jersey and Long Island waters. Shore Beach 2007, 75, 30–35. [Google Scholar]
- Taylor, P.K.; Yelland, M.J. The dependence of sea surface roughness on the height and steepness of the waves. J. Phys. Oceanogr. 2001, 31, 572–590. [Google Scholar] [CrossRef]
- Orton, P.; Hall, T.M.; Talke, S.; Blumberg, A.F.; Georgas, N.; Vinogradov, S. A Validated Tropical-Extratropical Flood Hazard Assessment for New York Harbor. J. Geophys. Res. 2016, 121, 8904–8929. [Google Scholar] [CrossRef]
- Georgas, N.; Yin, L.; Jiang, Y.; Wang, Y.; Howell, P.; Saba, V.; Schulte, J.; Orton, P.; Wen, B. An Open-Access, Multi-Decadal, Three-Dimensional, Hydrodynamic Hindcast Dataset for the Long Island Sound and New York/New Jersey Harbor Estuaries. J. Mar. Sci. Eng. 2016, 4, 48. [Google Scholar] [CrossRef]
- Jordi, A.; Georgas, N.; Blumberg, A.; Yin, L.; Chen, Z.; Wang, Y.; Schulte, J.; Ramaswamy, V.; Runnels, D.; Saleh, F. A next-generation coastal ocean operational system: Probabilistic flood forecasting at street scale. Bull. Am. Meteorol. Soc. 2018. [Google Scholar] [CrossRef]
- Georgas, N.; Blumberg, A.F. Establishing confidence in marine forecast systems: The design and skill assessment of the New York Harbor Observation and Prediction System, version 3 (NYHOPS v3). In Proceedings of the 11th International Conference on Estuarine and Coastal Modeling, Seattle, WA, USA, 4–6 November 2009; pp. 660–685. [Google Scholar]
- Di Liberto, T.; Colle, B.A.; Georgas, N.; Blumberg, A.F.; Taylor, A.A. Verification of a multimodel storm surge ensemble around New York City and Long Island for the cool season. Weather Forecast. 2011, 26, 922–939. [Google Scholar] [CrossRef]
- Kuang, L.; Blumberg, A.F.; Georgas, N. Assessing the fidelity of surface currents from a coastal ocean model and HF radar using drifting buoys in the Middle Atlantic Bight. Ocean Dyn. 2012, 62, 1229–1243. [Google Scholar] [CrossRef]
- Georgas, N. Establishing Confidence in Marine Forecast Systems: The Design of a High Fidelity Marine Forecast Model for the NY/NJ Harbor Estuary and Its Adjoining Coastal Waters. Ph.D. Thesis, Department of Civil, Environmental and Ocean Engineering, Stevens Institute of Technology, Hoboken, NJ, USA, 2010. [Google Scholar]
- NOAA National Centers for Environmental Information. Available online: https://www.ncdc.noaa.gov/data-access/model-data/model-datasets/north-american-mesoscale-forecast-system-nam (accessed on 11 May 2019).
- Colle, B.A.; Rojowsky, K.; Buonaito, F. New York City Storm Surges: Climatology and an Analysis of the Wind and Cyclone Evolution. J. Appl. Meteorol. Climatol. 2010, 49, 85–100. [Google Scholar] [CrossRef]
- Kusuda, M.; Alpert, P. Anti-Clockwise Rotation of the Wind Hodograph. Part I: Theoretical Study. J. Atmos. Sci. 1983, 40, 487–499. [Google Scholar] [CrossRef]
- Lin, N.; Emanuel, K.; Smith, J.; Vanmarcke, E. Risk assessment of hurricane storm surge for New York City. J. Geophys. Res 2010, 115. [Google Scholar] [CrossRef]
- Drews, C. Directional Storm Surge in Enclosed Seas: The Red Sea, the Adriatic, and Venice. J. Mar. Sci. Eng. 2015, 3, 356. [Google Scholar] [CrossRef]
- Prandle, D.; Wolf, J. The interaction of surge and tide in the North Sea and River Thames. Geophys. J. Int. 1978, 55, 203–216. [Google Scholar] [CrossRef]
- Horsburgh, K.; Wilson, C. Tide-surge interaction and its role in the distribution of surge residuals in the North Sea. J. Geophys. Res. Oceans 2007, 112, C8. [Google Scholar] [CrossRef]
- Parker, B.B. Frictional Effects on the Tidal Dynamics of a Shallow Estuary. Ph.D. Thesis, Johns Hopkins University, Baltimore, MD, USA, 1984. [Google Scholar]
- Wolf, J. Surge-tide interaction in the North Sea and River Thames. Floods Due High Wind. Tides 1981, 75–94. [Google Scholar]
- Jay, D.A. Green’s law revisited: Tidal long-wave propagation in channels with strong topography. J. Geophys. Res. Ocean. 1991, 96, 20585–20598. [Google Scholar] [CrossRef]
- Rossiter, J.R. Interaction between tide and surge in the Thames. Geophys. J. Int. 1961, 6, 29–53. [Google Scholar] [CrossRef]
- Friedrichs, C.T.; Aubrey, D.G. Non-linear tidal distortion in shallow well-mixed estuaries: a synthesis. Estuar. Coast. Shelf Sci. 1988, 27, 521–545. [Google Scholar] [CrossRef]
- Chernetsky, A.S.; Schuttelaars, H.M.; Talke, S.A. The effect of tidal asymmetry and temporal settling lag on sediment trapping in tidal estuaries. Ocean Dyn. 2010, 60, 1219–1241. [Google Scholar] [CrossRef]
- As-Salek, J.A. Coastal trapping and funneling effects on storm surges in the Meghna estuary in relation to cyclones hitting Noakhali-Cox’s Bazar coast of Bangladesh. J. Phys. Oceanogr. 1998, 28, 227–249. [Google Scholar] [CrossRef]
- Pousa, J.L.; D’Onofrio, E.E.; Fiore, M.M.E.; Kruse, E.E. Environmental impacts and simultaneity of positive and negative storm surges on the coast of the Province of Buenos Aires, Argentina. Environ. Earth Sci. 2013, 68, 2325–2335. [Google Scholar] [CrossRef]

| Experiment | Environmental Change | Anomaly of the Minima in Time Series (cm) |
|---|---|---|
| Surge experiments (Wind Only forcing) | Wall in the East River | +2.6 |
| Ice jam at West Point | +0.3 | |
| Shallowing shipping channels | −7.3 | |
| Tide experiments (Wind + Tide forcing) | Ice jam at West Point | +1.1 |
| Shallowing shipping channels | +5.4 | |
| Surface ice friction with twice nominal drag | +1.1 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gurumurthy, P.; Orton, P.M.; Talke, S.A.; Georgas, N.; Booth, J.F. Mechanics and Historical Evolution of Sea Level Blowouts in New York Harbor. J. Mar. Sci. Eng. 2019, 7, 160. https://doi.org/10.3390/jmse7050160
Gurumurthy P, Orton PM, Talke SA, Georgas N, Booth JF. Mechanics and Historical Evolution of Sea Level Blowouts in New York Harbor. Journal of Marine Science and Engineering. 2019; 7(5):160. https://doi.org/10.3390/jmse7050160
Chicago/Turabian StyleGurumurthy, Praneeth, Philip M. Orton, Stefan A. Talke, Nickitas Georgas, and James F. Booth. 2019. "Mechanics and Historical Evolution of Sea Level Blowouts in New York Harbor" Journal of Marine Science and Engineering 7, no. 5: 160. https://doi.org/10.3390/jmse7050160
APA StyleGurumurthy, P., Orton, P. M., Talke, S. A., Georgas, N., & Booth, J. F. (2019). Mechanics and Historical Evolution of Sea Level Blowouts in New York Harbor. Journal of Marine Science and Engineering, 7(5), 160. https://doi.org/10.3390/jmse7050160





