Wavy Wind-Water Flow Impacts on Offshore Wind Turbine Foundations
Abstract
1. Introduction
2. Mathematical Model
2.1. The Energy Equation Model
2.2. The SST k- Turbulence Model
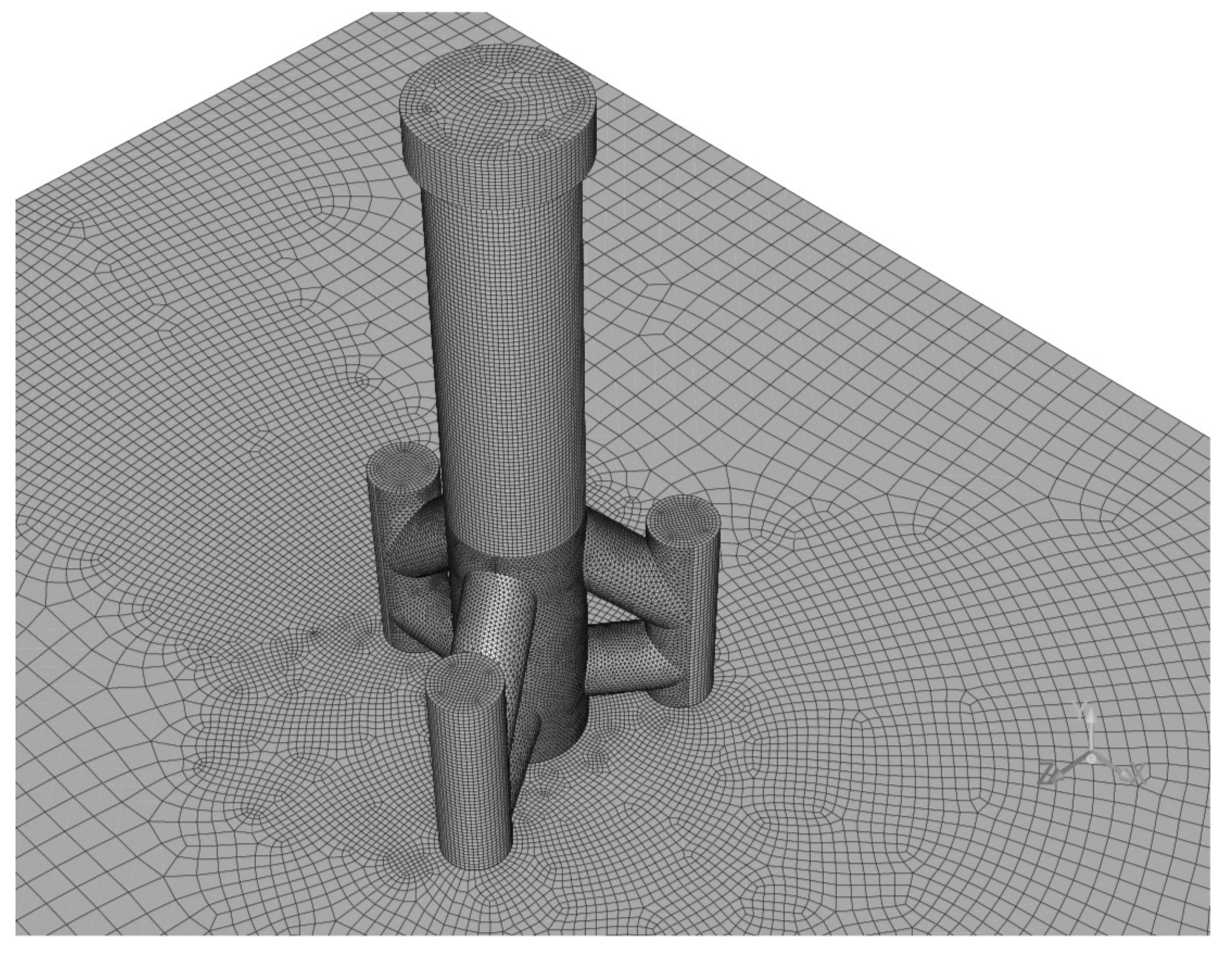
3. Foundations: Designs and Meshing
3.1. Mono-Pile and Tripod Foundations
3.2. Computational Domains and Meshing
3.3. Initial and Boundary Conditions
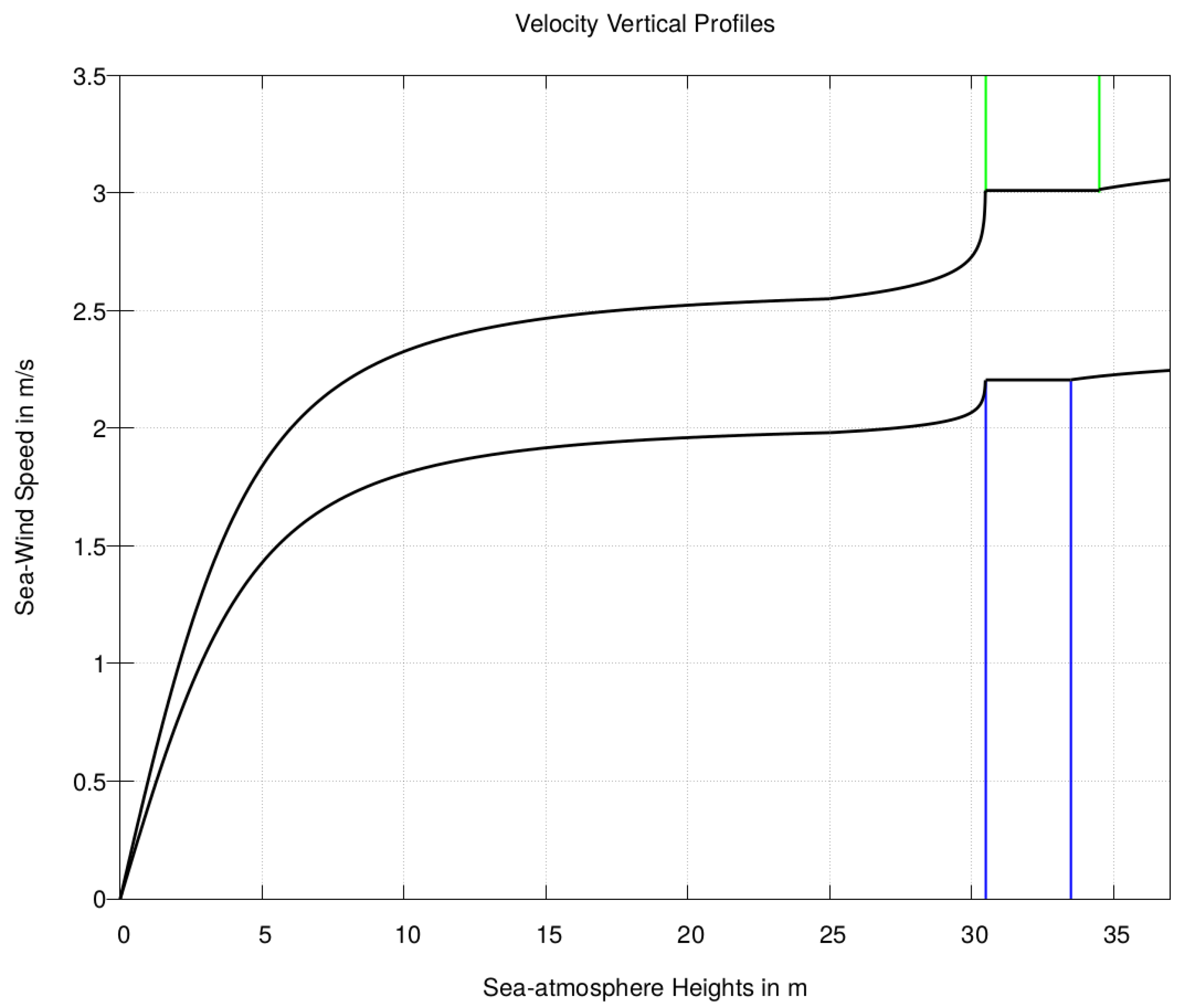
3.4. Inlet Waves Boundary
4. Numerical Results
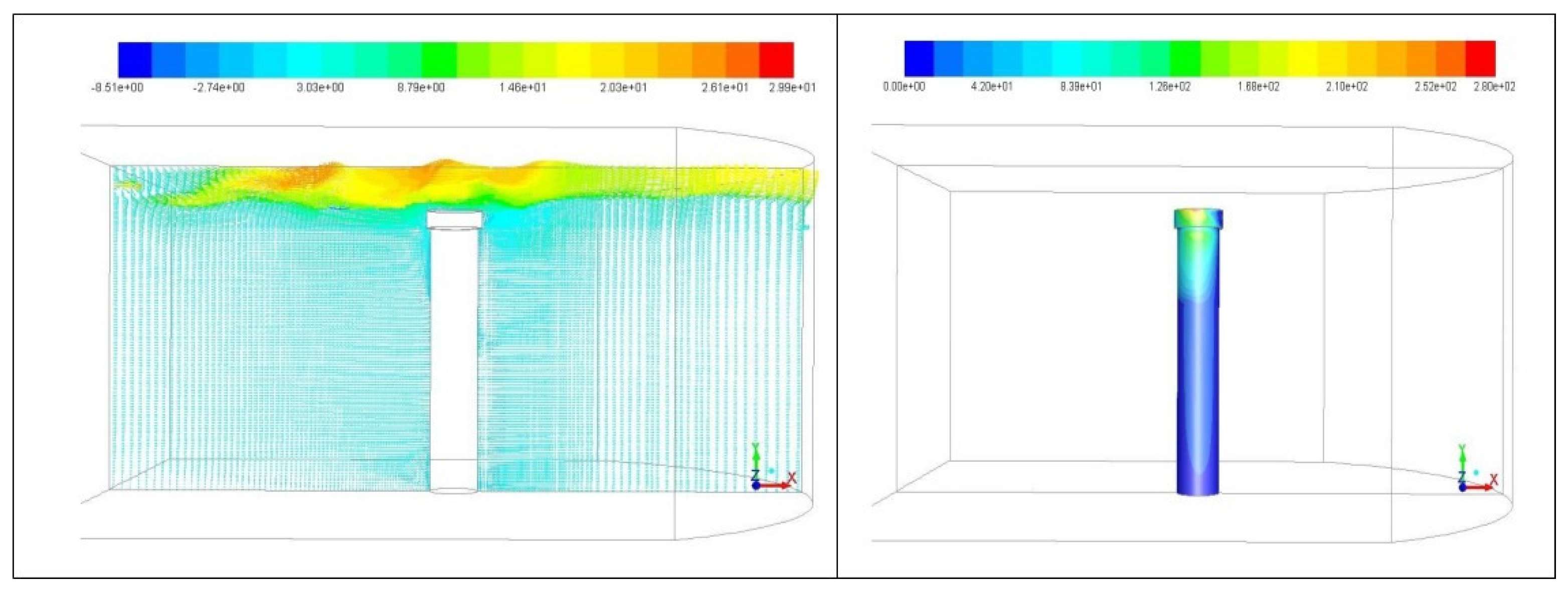
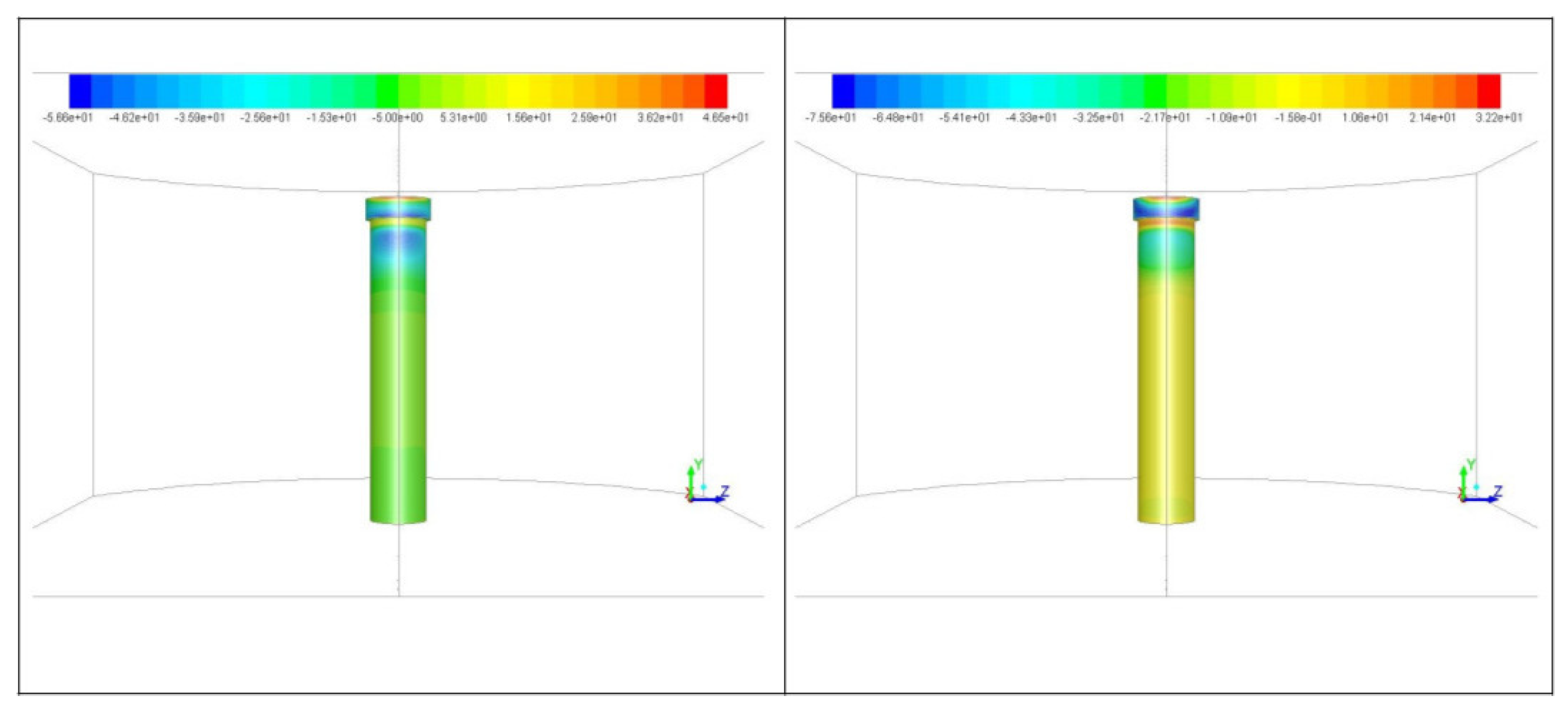
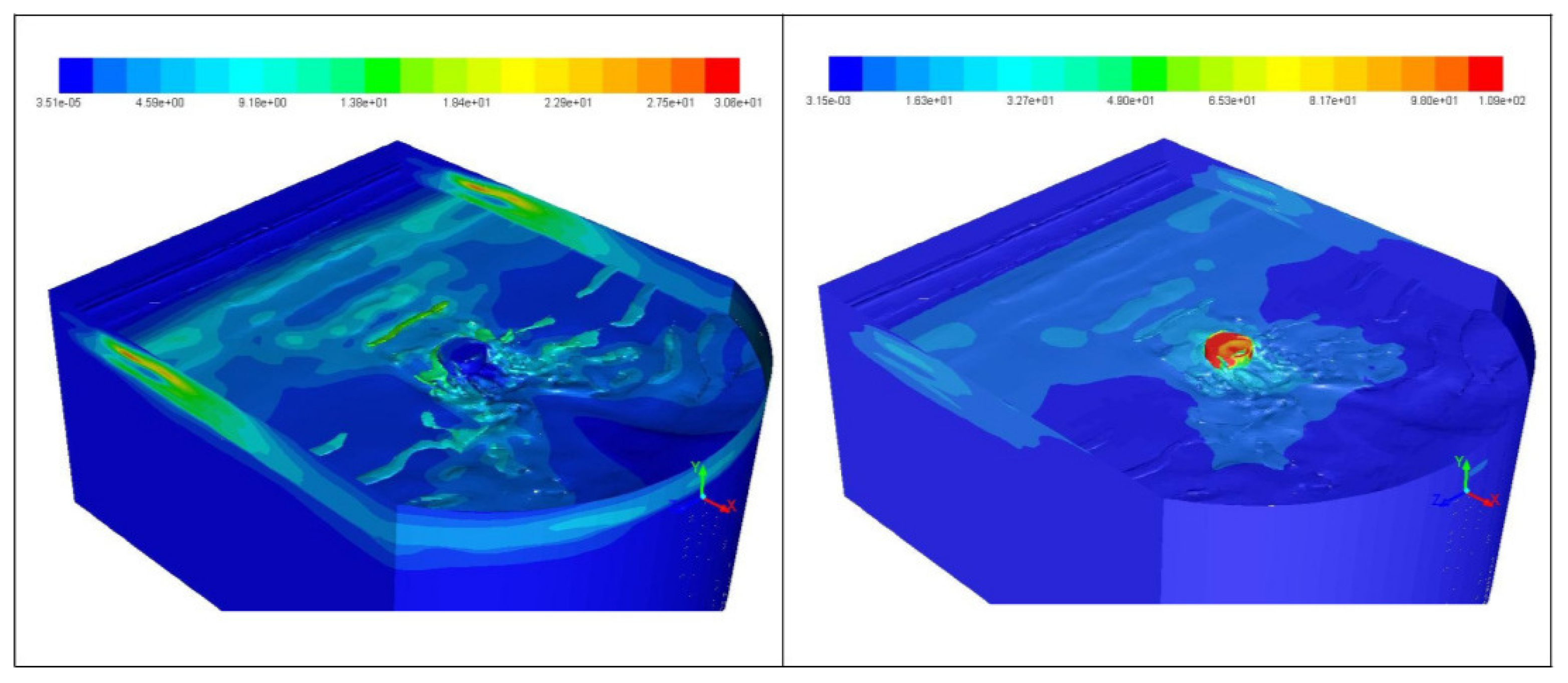
4.1. Flows Around a Mono-Pile Foundation: Cases and m/s
4.2. Flow Around a Tripod Foundation: Case m/s
5. Analyses via Reynolds and Froude Numbers
6. Conclusions
- This study aimed to evaluate the exchange dynamics between wind-driven surface flows and offshore foundation structures, with a focus on the interaction at structural interfaces rather than on small-scale turbulent processes such as those characterized by passive mixing, or detailed turbulence intensity distributions. The flow conditions employed in the simulations do not replicate extreme wind-sea scenarios but serve instead to investigate fundamental hydrodynamic responses under idealized, pseudo-periodic wave inlets. This approach introduces variability in the inflow conditions, enabling the observation of anisotropic flow-structure interactions under steady unidirectional flow.
- The simulation results demonstrate that vertical wall-shear stress distributions exhibit periodic peaks along the structural surfaces, with concentration zones at the neck and upper portions of the foundations—regions where flow-interface interactions are most intense. Analysis of directional drag coefficients reveals high-frequency variations, with each directional component showing a distinct pattern. This indicates that the foundations are subject to anisotropic force distributions, which have implications for structural stability and fatigue over time.
- The behavior of the vertical drag coefficient further reveals the presence of vertical flow structures that facilitate exchange between upper and lower water layers. These vertical dynamics not only influence foundation loading but also affect local hydrodynamics and potentially disrupt the stratification of water density. While the magnitude of these effects warrants careful quantification, their broader implications must be considered in the context of large OWT farms, particularly regarding long-term environmental impacts such as salinity changes and effects on marine ecosystems.
- Additionally, localized phenomena such as down-welling—particularly observed in tripod configurations—suggest that vertical downward flow and flow convergence near the seabed require further investigation to confirm and characterize these dynamics.
- It is important to minimize negative effects of OWT on marine ecosystems while maximizing constructive ones. The uncertainty of long-term effects of OWT remain difficult to grasp or to quantify. All the required studies for understanding the effects of OWT farms on climate and on marine life, must involve sea users, companies, scientists and legislators to establish appropriate and stricter rules for the good of the environment.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Seebai, T.S.; Sundaravadivelu, R. Response analysis of spar platform with wind turbine. Ships Offshore Struct. 2013, 8, 94–101. [Google Scholar]
- Wunsch, C.; Ferrari, R. Is the ocean speeding up? Ocean surface energy trends. J. Phys. Oceanogr. 2020, 50, 3205–3217. [Google Scholar] [CrossRef]
- Kurian, V.J.; Ng, C.Y.; Liew, M.S. Dynamic responses of truss spar due to wave actions. Res. J. Appl. Sci. Eng. Technol. 2013, 5, 812–818. [Google Scholar] [CrossRef]
- Shi, Q.; Borassa, M. Coupling Ocean Currents and Waves with Wind Stress over the Gulf Stream. Remote Sens. 2019, 11, 1476. [Google Scholar] [CrossRef]
- Wang, X.; Zeng, X.; Li, J.; Yang, X.; Wang, H. A review on recent advancements of substructures for offshore wind. Energy Convers. Manag. 2018, 158, 103–119. [Google Scholar] [CrossRef]
- Haider, R.; Shi, W.; Cai, Y.; Lin, Z.; Li, X.; Hu, Z. A comprehensive numerical model for aero-hydro-mooring analysis of a floating offshore wind turbine. Renew. Energy 2024, 237, 121793. [Google Scholar] [CrossRef]
- Haider, R.; Shi, W.; Lin, Z.; Cai, Y.; Zhao, H.; Li, X. Coupled analysis of floating offshore wind turbines with new mooring systems by CFD method. Ocean. Eng. 2024, 312 Pt 1, 119054. [Google Scholar] [CrossRef]
- Christensen, E.D.; Bredmose, H.; Hansen, E.A. Extreme wave forces and wave run-ups on offshore wind turbine foundations. In Proceedings of the Copenhagen Offshore Wind 2005, Copenhagen, Denmark, 26–28 October 2005; pp. 1–10. [Google Scholar]
- Bredmose, H.; Jacobsen, N.G.; Hansen, E.A. Breaking wave impacts on offshore wind turbine foundations:Wave groups and CFD. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, Shanghai, China, 6–11 June 2010; pp. 397–404. [Google Scholar]
- Chen, D.; Huang, K.; Bretel, V.; Hou, L. Comparison of structural properties between mono-pile and tripod offshore wind-turbine support structures. Adv. Mech. Eng. 2013, 2013, 175684. [Google Scholar] [CrossRef]
- Alesbe, I.; Maksoud, M.A.; Aljabair, S. Comparison of structural properties between mono-pile and tripod offshore wind-turbine support structures. J. Renew. Energy 2016, 2, 1–12. [Google Scholar]
- Lai, R.J.; Shemdin, O. Laboratory investigation of air turbulence above simple water wave. J. Geophys. Res. 1971, 76, 7334–7350. [Google Scholar] [CrossRef]
- Veron, F.; Saxena, G.; Misra, S.K. Measurements of the viscous tangential stress in the airflow above wind waves. Geophys. Res. Lett. 2007, 34, 281–314. [Google Scholar] [CrossRef]
- Buckley, M.P.; Veron, F. Structure of the airflow above surface waves. J. Phys. Oceanogr. 2016, 46, 1377–1397. [Google Scholar] [CrossRef]
- Buckley, M.P.; Veron, F. The turbulent airflow over wind generated surface waves. Eur. J. Mech. (B/Fluids) 2019, 73, 132–143. [Google Scholar] [CrossRef]
- Shemer, L. On Evolution of Young Wind Waves in Time and Space. Atmosphere 2019, 10, 562. [Google Scholar] [CrossRef]
- Flugge, M.; Bakhoday-Paskyabi, M.; Reuder, J.; El Guernaoui, O. Wind Stress in the Coastal Zone: Observations from a Buoy in Southwestern Norway. Atmosphere 2019, 10, 491. [Google Scholar] [CrossRef]
- Laxague, N.; Zappa, C. Observations of mean and wave orbital flows in the ocean’s upper centimetres. J. Fluid Mech. 2020, 887, A10. [Google Scholar] [CrossRef]
- Grachev, A.; Fairall, C. Upward momentum transfer in the marine boundary layer. J. Phys. Oceanogr. 2001, 31, 1698–1711. [Google Scholar] [CrossRef]
- Kahma, K.K.; Donelan, M.A.; Drennan, W.M.; Terray, E.A. Evidence of energy and momentum flux from swell to wind. J. Phys. Oceanogr. 2016, 46, 2143–2156. [Google Scholar] [CrossRef]
- Sullivan, P.P.; Mcwilliams, J.C.; Moeng, C.-H. Simulation of turbulent flow over idealized water waves. J. Fluid Mech. 2000, 404, 47–85. [Google Scholar] [CrossRef]
- Yang, D.; Shen, L. Direct numerical simulation of scalar transport in turbulent flows over progressive surface waves. J. Fluid Mech. 2017, 819, 58–103. [Google Scholar] [CrossRef]
- Yang, Z.; Deng, B.Q.; Shen, L. Direct numerical simulation of wind turbulence over breaking waves. J. Fluid Mech. 2018, 850, 120–155. [Google Scholar] [CrossRef]
- Sullivan, P.; Banner, M.L.; Morisson, R.P.; Peirson, W.L. Turbulent Flow over Steep Steady and Unsteady Waves under Strong Wind Forcing. J. Phys. Oceanogr. 2018, 48, 3–27. [Google Scholar] [CrossRef]
- Akervik, E.; Vartdal, M. The role of wave kinematics in turbulent flow over waves. J. Fluid Mech. 2021, 919, 890–915. [Google Scholar] [CrossRef]
- Cao, T.; Shen, L. A numerical and theoretical study of wind over fast-propagating water waves. J. Fluid Mech. 2021, 919, A38. [Google Scholar] [CrossRef]
- Hao, X.; Hen, L. Wind–wave coupling study using LES of wind and phase-resolved simulation of nonlinear waves. J. Fluid Mech. 2019, 874, 391–425. [Google Scholar] [CrossRef]
- Wang, L.; Zhang, W.; Hao, X.; Huang, W.; Shen, L.; Xu, C.; Zhang, Z. Surface wave effects on energy transfer in overlying turbulent flow. J. Fluid Mech. 2020, 951, A21. [Google Scholar] [CrossRef]
- Wu, J.; Popinet, S.; Deike, L. Revisiting wind wave growth with fully-coupled direct numerical simulations. J. Fluid Mech. 2022, 951, A18. [Google Scholar] [CrossRef]
- Cimarelli, A.; Romoli, F.; Stalio, E. On wind–wave interaction phenomena at low Reynolds numbers. J. Fluid Mech. 2023, 956, A13. [Google Scholar] [CrossRef]
- Guth, S.; Katsidoniotaki, E.; Themistoklis, P.; Sapsis, P.T. Statistical modeling of fully nonlinear hydrodynamic loads on offshore wind turbine mono-pile foundations using wave episodes and targeted CFD simulations through active sampling. Wind Energy. 2020, 27, 75–100. [Google Scholar] [CrossRef]
- Eames, I.; Robinson, T. Free-surface channel flow around a square cylinder. J. Fluid Mech. 2024, 980, A16. [Google Scholar] [CrossRef]
- Chen, H.; Zou, Q. Effects of following and opposing vertical current shear on nonlinear wave interactions. Appl. Ocean Res. 2019, 89, 23–35. [Google Scholar] [CrossRef]
- Lange, M.; Focken, U. Physical Approach to Short-Term Wind Power Prediction; Springer: Berlin/Heidelberg, Germany, 2005; ISBN 3-540-25662-8. [Google Scholar] [CrossRef]
- Emeis, S. Wind Energy Meteorology, 2nd ed.; Springer: Cham, Switzerland, 2018; ISBN 978-3-319-72858-2. [Google Scholar] [CrossRef]
- Fluent. In ANSYS Fluent 16.5 User’s Guide; ANSYS, Inc.: Canonsburg, PA, USA, 2015.
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Stewart, R.W. The wave drag of wind over water. J. Fluid Mech. 1961, 10, 189–194. [Google Scholar] [CrossRef]
- Bye, J.A.T. The Dynamics of Air-Sea Boundary Layer. Adv. Environ. Eng. Res. 2023, 4, 1–16. [Google Scholar] [CrossRef]
- Businger, J.A. The Marine Boundary Layer, from Air-Sea Interface to Inversion; Technical Report NCAR/TN-252 + STR NCAR; National Center for Atmospheric Research: Boulder, CO, USA, 1985. [Google Scholar]
- Bye, J.A.T. The coupling of wave drift and wind velocity profiles. J. Mar. Res. 1988, 46, 457–472. [Google Scholar] [CrossRef]
- Bye, J.A.T.; Wolff, J.O. Charnock dynamics: A model for the velocity structure in the wave boundary layer of the air–sea interface. Ocean Dyn. 2008, 58, 31–42. [Google Scholar] [CrossRef]
- Janssen, P. Quasilinear theory of wind-wave generation applied to wave forecasting. J. Phys. Oceanogr. 1991, 21, 1631–1642. [Google Scholar] [CrossRef]
- Abdella, K.; D’Alessio, S. A parameterization of the roughness length for the air-sea interface in free convection. Environ. Fluid Mech. 2003, 3, 55–77. [Google Scholar] [CrossRef]
- Peregrine, D.H.; Jonsson, G.I. Interaction of Waves and Currents; Technical Report NO. 83-6; Coastal Engineering Research Center: Fort Belvoir, VA, USA, 1983. [Google Scholar]
- Wells, M.; Cenedese, C.; Caulfield, C.P. The Relationship between Flux Coefficient and Entrainment Ratio in Density Currents. Am. Meteo. Soc. 2010, 40, 2713–2727. [Google Scholar] [CrossRef]
- Cenedese, C.; Adduce, C. A new parametrization for entrainment in overflows. J. Phys. Oceanogr. 2010, 40, 1835–1850. [Google Scholar] [CrossRef]
- Cenedese, C.; Adduce, C. Mixing in a density-driven current flowing down a slope in a rotating fluid. J. Fluid Mech. 2008, 604, 369–388. [Google Scholar] [CrossRef]
- Faller, A.J. Sources of energy for the ocean circulation and a theory of the mixed layer. In Proceedings of the Fifth United States Congress of Applied Mechanics, American Society of Mechanical Engineers, Minneapolis, MN, USA, 14–17 June 1966; pp. 651–672. [Google Scholar]
- Oort, A.H.; Anderson, L.A.; Peixoto, J.P. Estimates of the energy cycle of the oceans. J. Geophys. Res. 1994, 99, 7665–7688. [Google Scholar] [CrossRef]
- Wunsch, C.; Ferrari, R. Vertical mixing, energy, and the general circulation of the oceans. Annu. Rev. Fluid Mech. 2004, 36, 281–314. [Google Scholar] [CrossRef]
- Large, W.G.; Yeager, S.G. Diurnal to Decadal Global Forcing for Ocean and Sea-Ice Models: The Data Sets and Flux Climatologies; Technical Report 434, NCAR Tech Note NCAR/TN-460+STR; National Center for Atmospheric Research: Boulder, CO, USA, 2004. [Google Scholar]











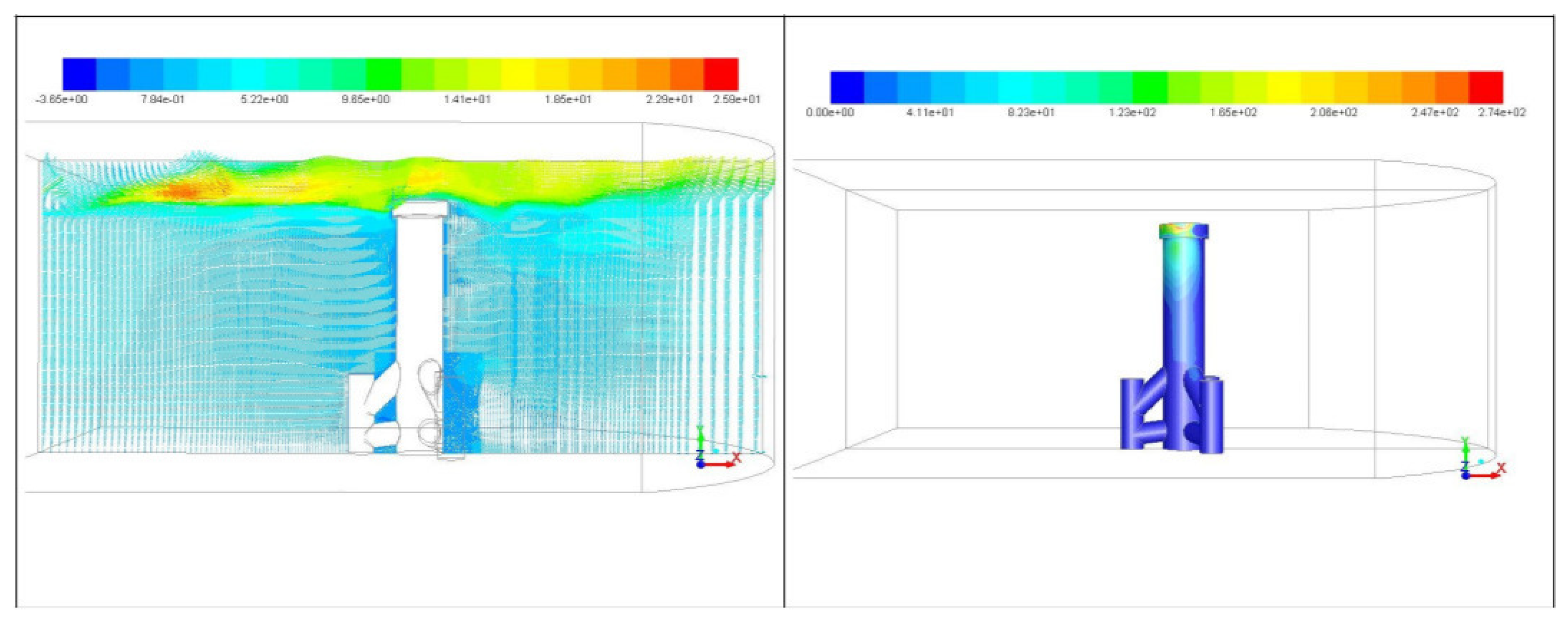
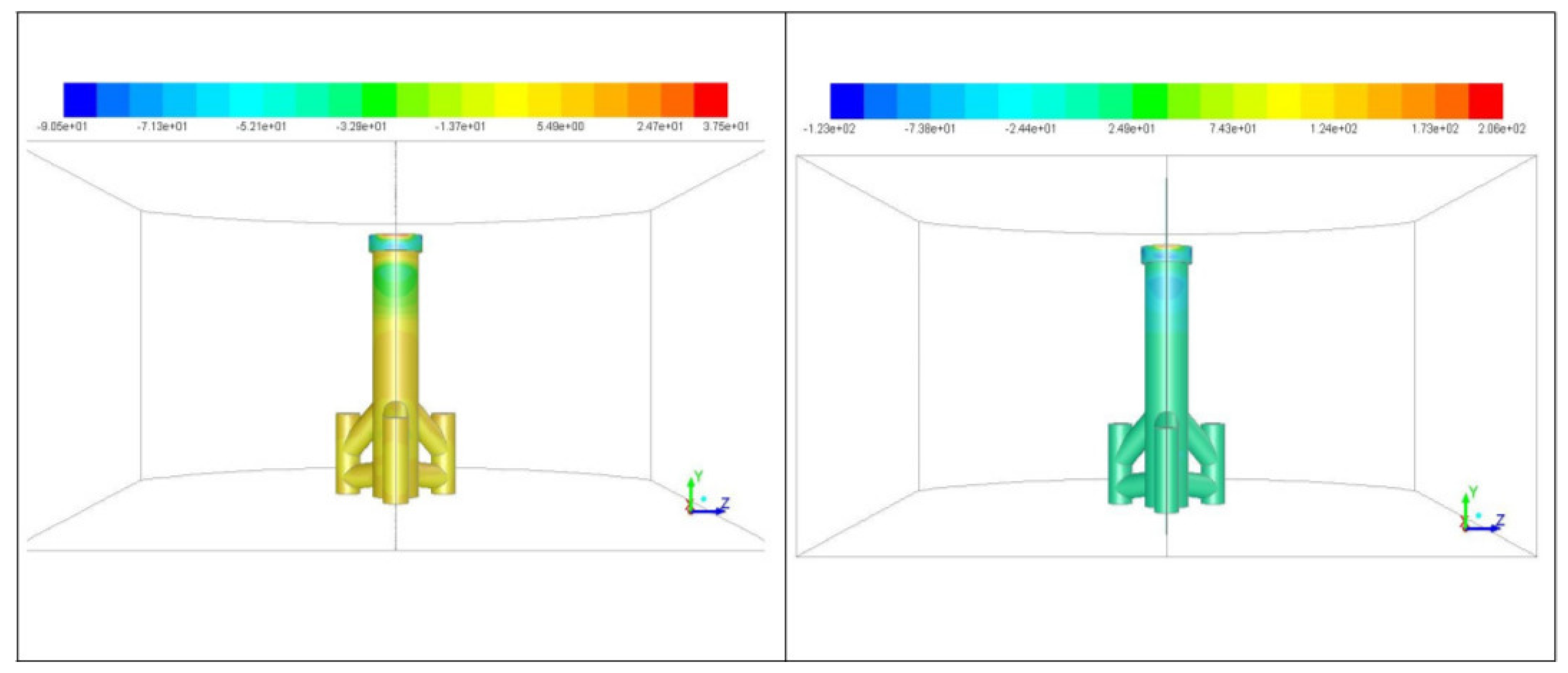
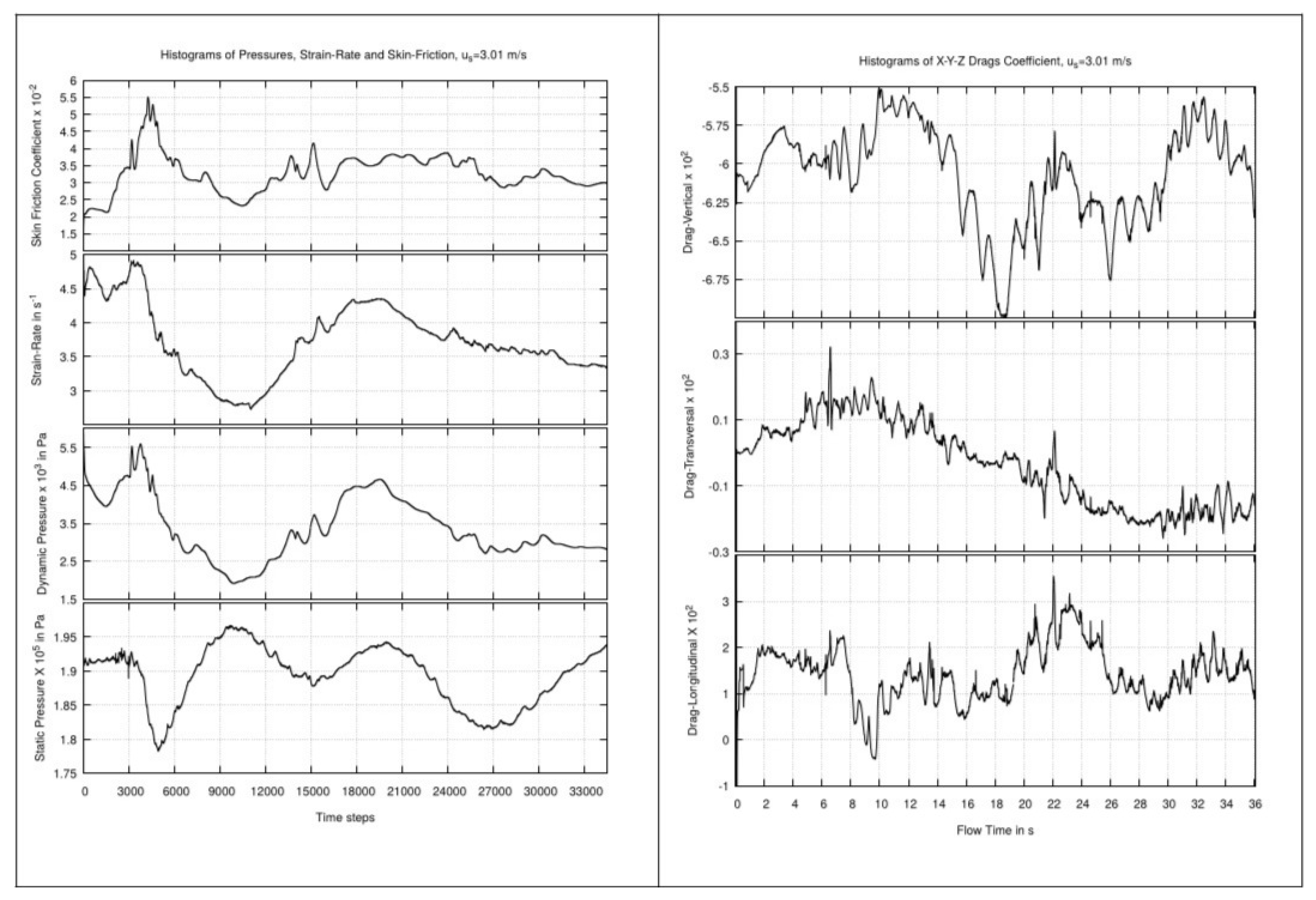
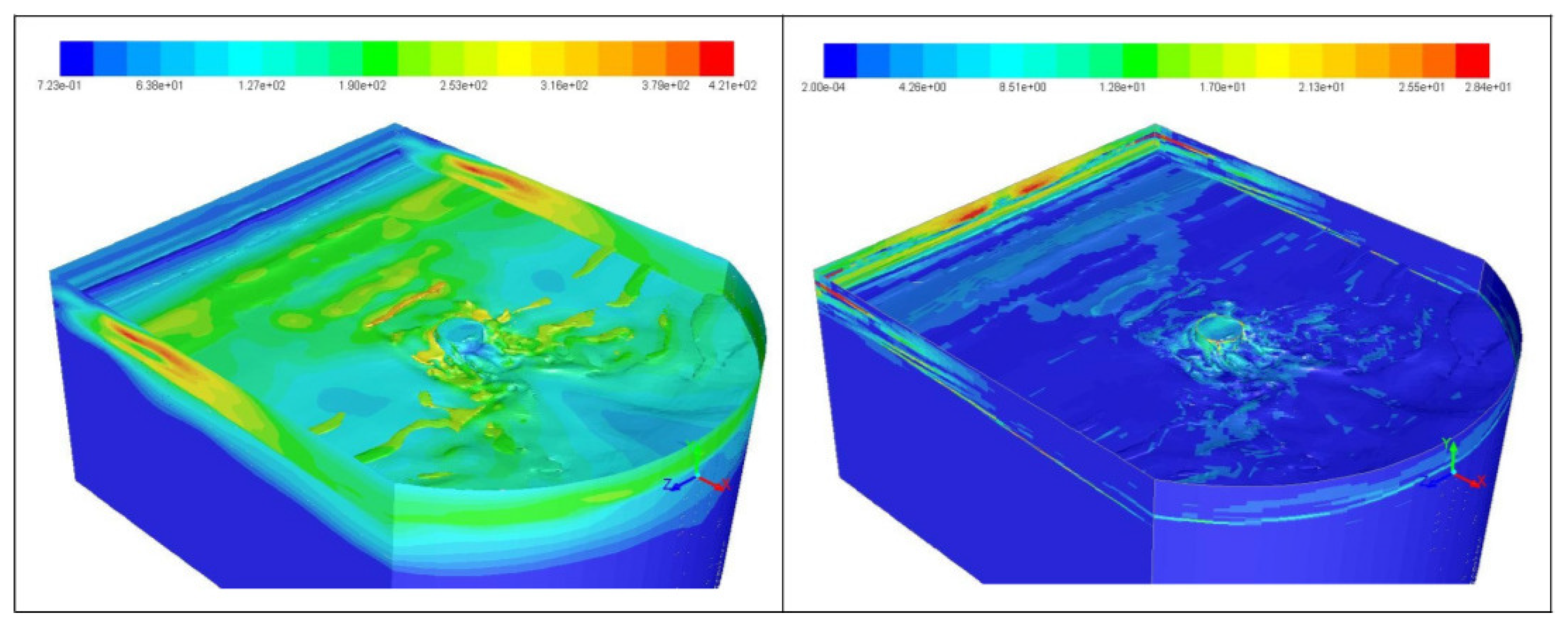
| Foundations | Hexa-Hedral Cells | Quadrilateral Interior Faces |
|---|---|---|
| Mono-pile | 1.36 M | 4.005 M |
| Tripod | 2.45 M | 7.850 M |
| Fluids | Density [kg/m3] | Dyn. Viscosity [Pa·s] | Kin. Viscosity [m2/s] |
|---|---|---|---|
| Air | 1.225 | ||
| Seawater | 998.2 |
| VOF Solver For Transient Open Channel | ||
|---|---|---|
| Pressure-Velocity | Spatial Discret. | Pressure |
| Simple | Least Square Cell Based | Presto |
| Momentum | Turb. Kinetic rate | Turb. Dissip. rate |
| 2nd Upwind/3rd MUSCL | 2nd Upwind/3rd MUSCL | 2nd Upwind/3rd MUSCL |
| Volume Fraction | Level set | Transient Form |
| Compressive | 1st Implicit/2nd Upwind | 1st Order Implicit |
| Maximum Reynolds Phasic Numbers | |||||
|---|---|---|---|---|---|
| Mono-Pile | Mono-Pile | Tripod | |||
| m/s | m/s | m/s | |||
| 20.5 | 26.0 | 26.0 | |||
| max | |||||
| 5.0 | 7.0 | 6.0 | |||
| max | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thomas, R.; Dababneh, O.; Gourma, M. Wavy Wind-Water Flow Impacts on Offshore Wind Turbine Foundations. J. Mar. Sci. Eng. 2025, 13, 941. https://doi.org/10.3390/jmse13050941
Thomas R, Dababneh O, Gourma M. Wavy Wind-Water Flow Impacts on Offshore Wind Turbine Foundations. Journal of Marine Science and Engineering. 2025; 13(5):941. https://doi.org/10.3390/jmse13050941
Chicago/Turabian StyleThomas, Rehil, Odeh Dababneh, and Mustapha Gourma. 2025. "Wavy Wind-Water Flow Impacts on Offshore Wind Turbine Foundations" Journal of Marine Science and Engineering 13, no. 5: 941. https://doi.org/10.3390/jmse13050941
APA StyleThomas, R., Dababneh, O., & Gourma, M. (2025). Wavy Wind-Water Flow Impacts on Offshore Wind Turbine Foundations. Journal of Marine Science and Engineering, 13(5), 941. https://doi.org/10.3390/jmse13050941






