Creep Behavior Research of Deep-Sea Pressure Hull: A Review
Abstract
1. Introduction
2. Creep Test
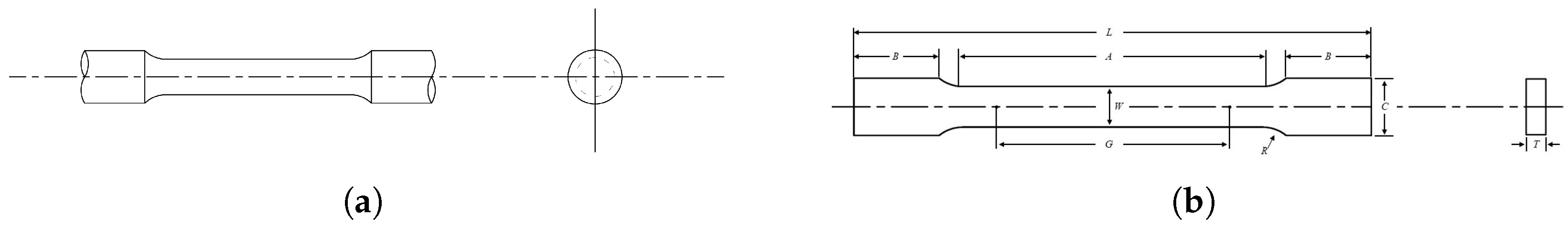
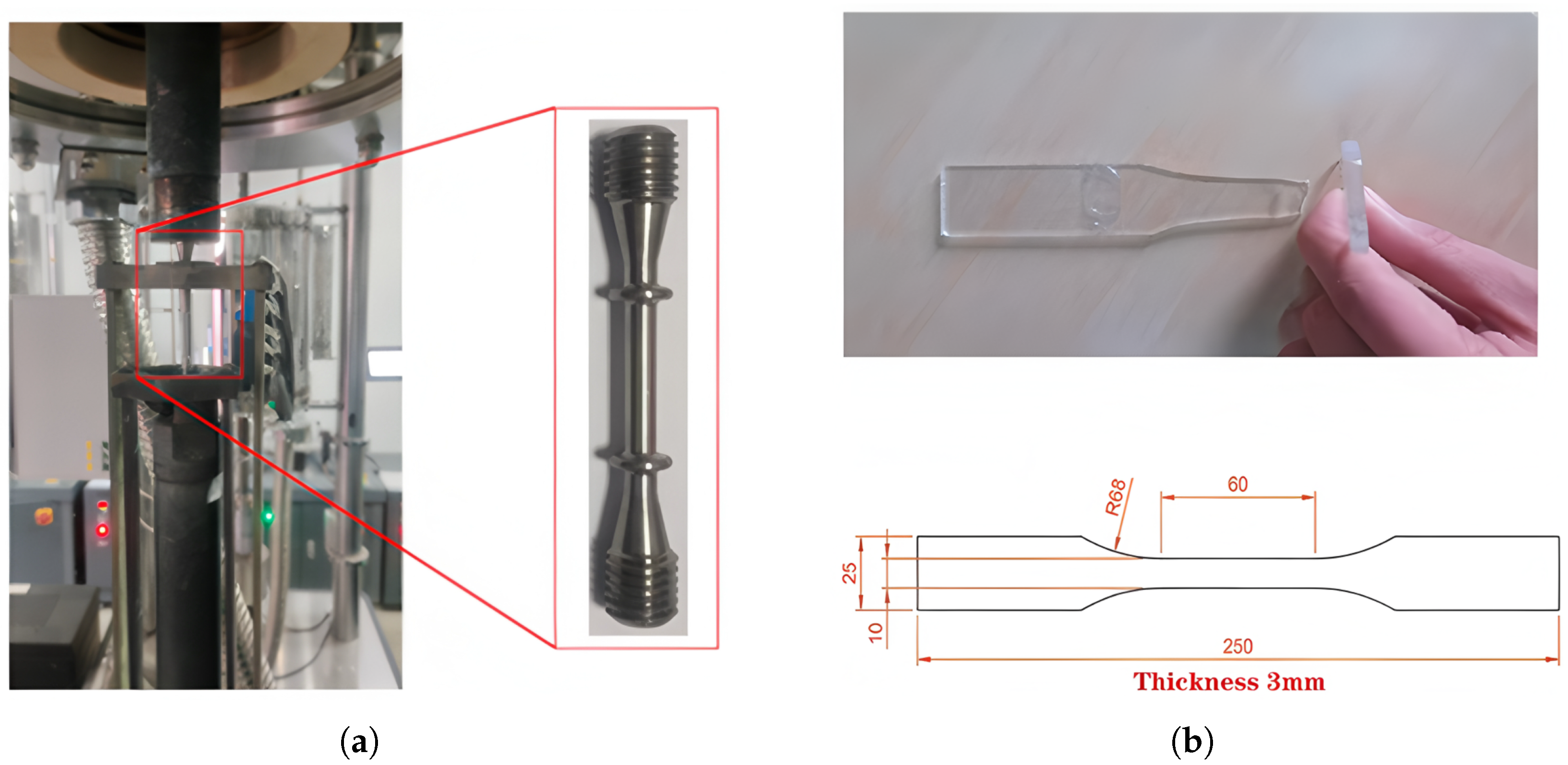
2.1. Material Creep Tests
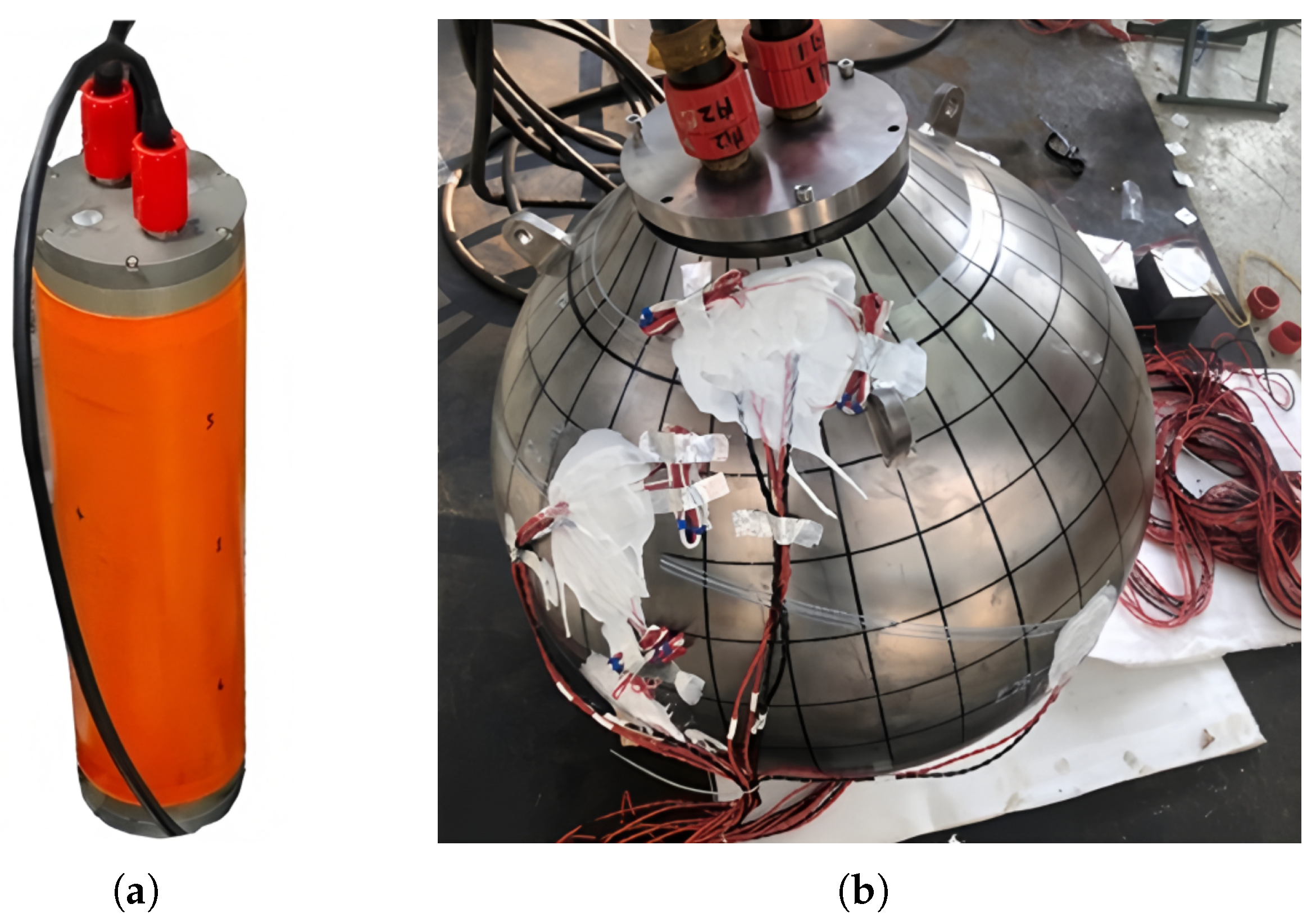
2.2. Creep Test of Pressure Hull Scale Models
3. Creep Constitutive Model
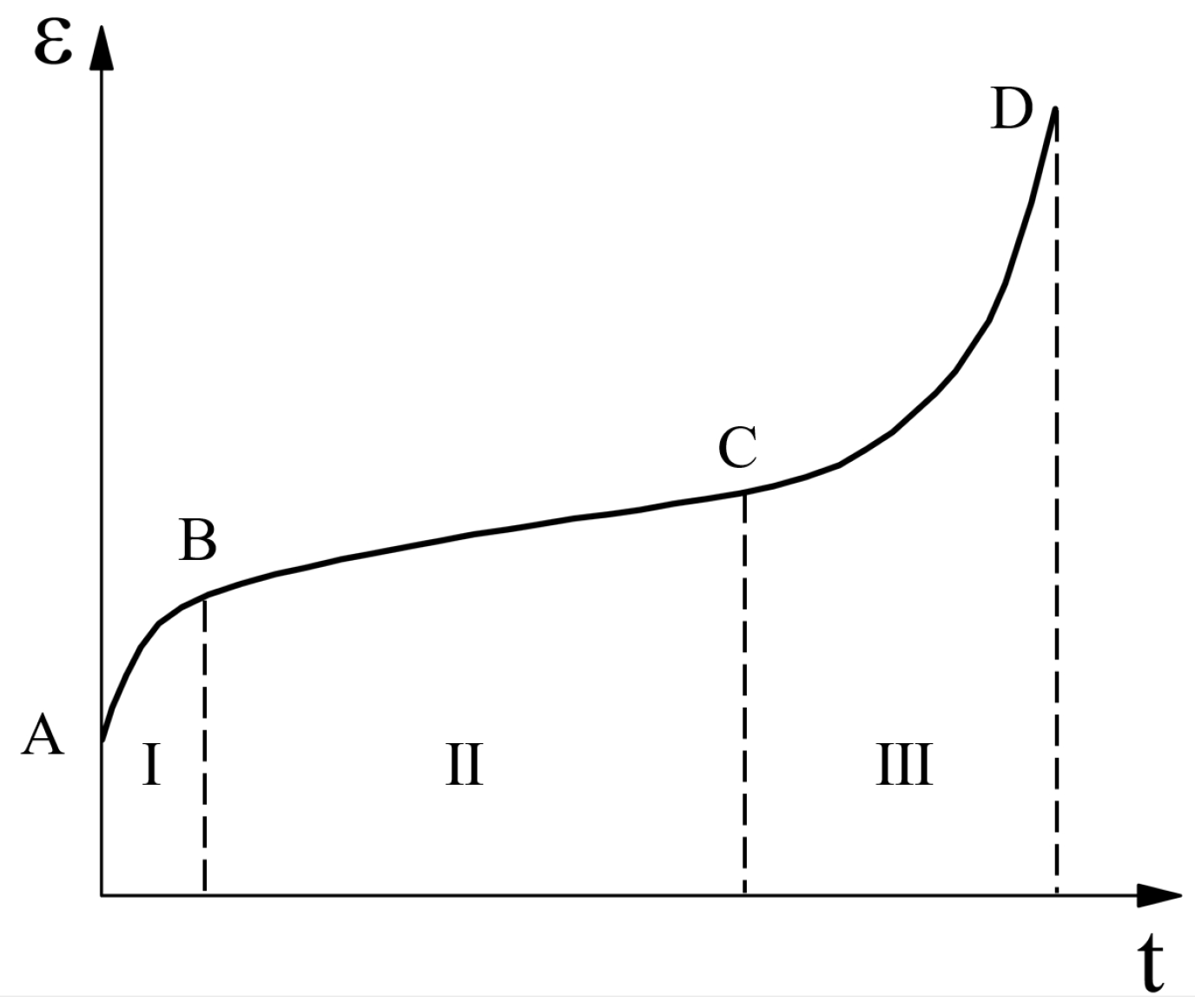
3.1. Uniaxial Creep Constitutive Model
3.2. Modified Creep Constitutive Model
3.3. Multiaxial Creep Constitutive Model
4. Numerical Simulation of Creep
4.1. Creep Simulation of Pressure Hulls
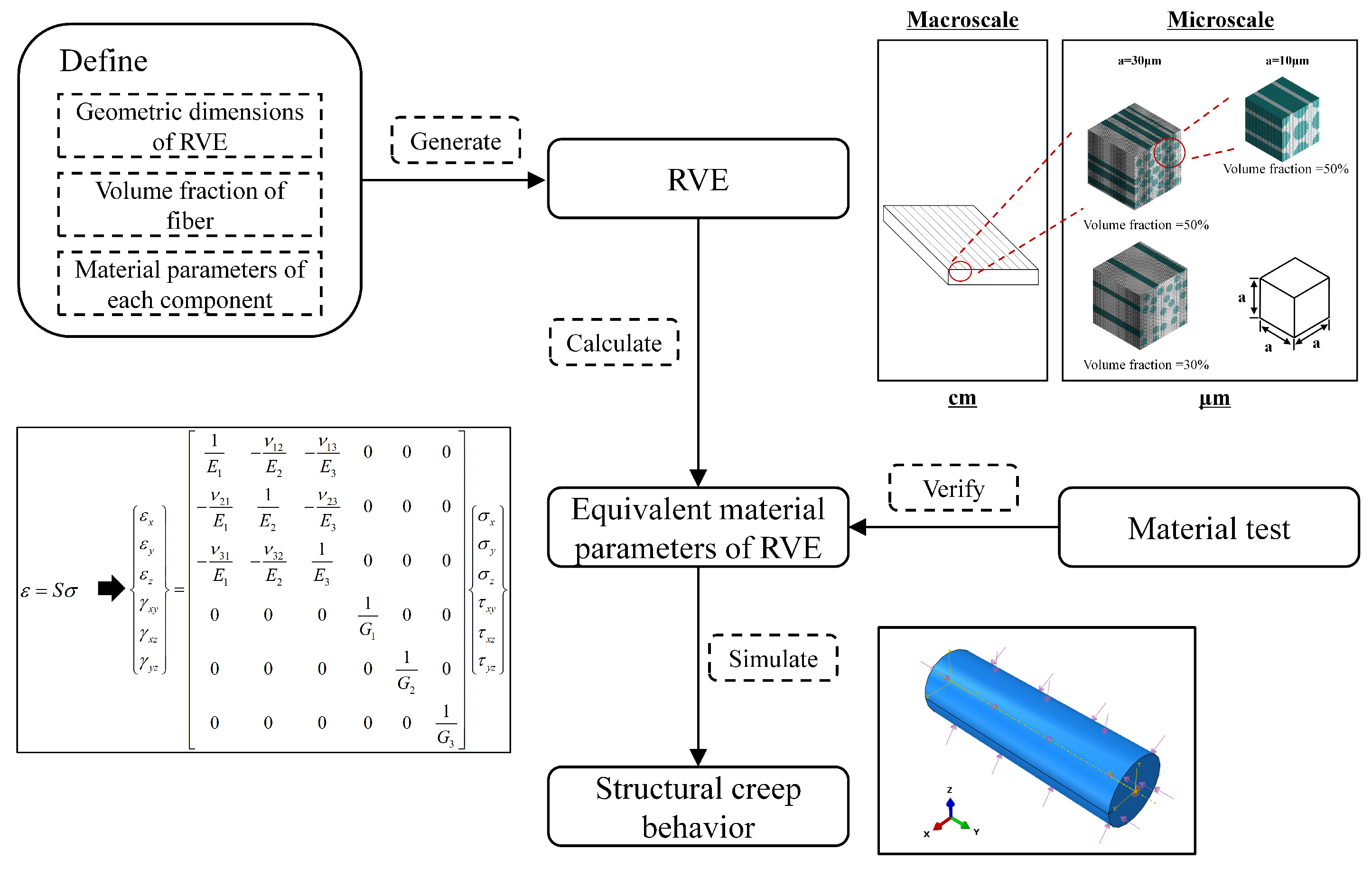
4.2. Multiscale Method of Composite Materials Creep Analysis
5. Conclusions
- Develop numerical methods to quantify and incorporate uncertainty in creep predictions for pressure hulls, addressing variability in environmental factors, material degradation, and fatigue;
- Develop multiscale techniques to predict the creep behavior of high-performance composites, improving reliability in deep-sea environments with limited experimental data;
- Integrate machine learning with multiscale analysis to accelerate predictions of composite creep performance, enhancing the efficiency of traditional design methods.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yang, R.; Ma, Y. Status and Prospect of Key Materials for Deep Submergence Facilities. Sci. Technol. Foresight 2022, 1, 145–156. (In Chinese) [Google Scholar]
- ASTM E139-11; Standard Test Methods for Conducting Creep, Creep-Rupture, and Stress-Rupture Tests of Metallic Materials. ASTM: West Conshohocken, PA, USA, 2018. [CrossRef]
- ASTM D2990-17; Standard Test Methods for Tensile, Compressive, and Flexural Creep and Creep-Rupture of Plastics. ASTM: West Conshohocken, PA, USA, 2017. [CrossRef]
- Yan, Y.; Liu, P.; Wang, A.; Xie, J.; Bian, X.; Wang, Z. Investigation on the Room Temperature Compressive Creep Behavior of TC4 Titanium Alloy Joints with Vacuum Electron Beam Welding. Solid State Commun. 2024, 391, 115641. [Google Scholar] [CrossRef]
- Wang, K.; Gao, L.; Zhang, S.; Huang, X. Experimental Investigations on Tensile Creep of New Titanium Alloy Materials at Room Temperature. J. Ship Mech. 2022, 26, 557–565. (In Chinese) [Google Scholar]
- Yan, S.C.; Wu, Y.K.; Shang, L.; Ren, J.Q.; Zhang, Y.M.; Xin, C.; Wang, Q.; Ai, T.T. Compressive Creep Damage of Ti–6Al–4V ELI Alloy with Bimodal Structure at Room Temperature. J. Mater. Res. Technol. 2024, 33, 4899–4907. [Google Scholar] [CrossRef]
- Xi, G. Studies on Room Temperature Creep and Dwell Fatigue Properties of Marine Engineering Titanium Alloys. Ph.D. Thesis, University of Science and Technology of China, Hefei, China, 2021. (In Chinese) [Google Scholar] [CrossRef]
- GB/T 2039-2024; Metallic Materials-Uniaxial Creep Testing Method in Tension. National Standard of the People’s Republic of China: Beijing, China, 2024.
- GB/T 15048-94; Cellular Plastics, Rigid-Determination of Compressive Creep. National Standard of the People’s Republic of China: Beijing, China, 1994.
- ASTM E8/E8M-21; Standard Test Methods for Tension Testing of Metallic Materials. ASTM: West Conshohocken, PA, USA, 2021.
- Chen, J. Study on Creep Recovery Characteristics of New Titanium Alloy at Room Temperature and Influence of Load Protection-Fatigue Crack Growth Behavior. Master’s Thesis, Jiangsu University of Science and Technology, Zhenjiang, China, 2023. (In Chinese) [Google Scholar] [CrossRef]
- Liu, Z.; Wang, F.; Zhao, B.; Zhang, J.; Gaidai, O.; Sun, Z.; Wang, K. Room-Temperature Creep Deformation of a Pressure-Resistant Cylindrical Structure Made of Dissimilar Titanium Alloys. J. Mar. Sci. Eng. 2024, 12, 1419. [Google Scholar] [CrossRef]
- Liu, P.; Li, J.; Wang, S.; Leng, J. Finite Element Analysis of Viscoelastic Creep Behaviors of Deep-Sea Manned Submersible Viewport Windows. Int. J. Press. Vessel. Pip. 2020, 188, 104218. [Google Scholar] [CrossRef]
- Slaughter, W.S.; Fleck, N.A.; Budiansky, B. Compressive Failure of Fiber Composites: The Roles of Multiaxial Loading and Creep. J. Eng. Mater. Technol. 1993, 115, 308–313. [Google Scholar] [CrossRef]
- Guedes, R.; Vaz, M.; Ferreira, F.; Morais, J.L. Response of CFRP Laminates under High Strain Rate Compression until Failure. Sci. Eng. Compos. Mater. 2005, 12, 145–152. [Google Scholar] [CrossRef]
- Kong, F. Study on Fatigue—Creep Properties of Carbon Fiber Reinforced Composite. Master’s Thesis, Civil Aviation University of China, Tianjing, China, 2022. (In Chinese) [Google Scholar] [CrossRef]
- Lv, J.; Xiao, Y.; Zhou, Y.; Xie, Y. Characterization and Modeling of the Creep Behavior of Fiber Composites with Tension and Compression Asymmetry. Int. J. Mech. Sci. 2020, 170, 105340. [Google Scholar] [CrossRef]
- Jiang, X.; Yu, C.; Li, Y.; Zeng, Y. Ultimate State Assessment of Medium-Thick Composite Cylindrical Pressure Shells Based on Progressive Damage. Ocean Eng. 2023, 289, 116128. [Google Scholar] [CrossRef]
- Wang, L.; Li, Y.; Sun, C.; Qiu, J.; Huang, J.; Jiang, X.; Sun, Z.; Wan, Z. Compressive Creep Behavior of Spherical Pressure Hull Scale Model for Full-Ocean-Depth Manned Submersible. Ocean Eng. 2022, 266, 112831. [Google Scholar] [CrossRef]
- Stachiw, J.D.; Clark, A.; Brenn, C.B. Acrylic Plastic Spherical Pressure Hull for 2439 m (8000 Ft) Design Depth: Phase I. J. Energy Resour. Technol. 1987, 109, 40–47. [Google Scholar] [CrossRef]
- Swindeman, R.; Swindeman, M. A Comparison of Creep Models for Nickel Base Alloys for Advanced Energy Systems. Int. J. Press. Vessel. Pip. 2008, 85, 72–79. [Google Scholar] [CrossRef]
- Bellieud, M. Homogenization of Norton–Hoff Fibered Composites with High Viscosity Contrast. SIAM J. Math. Anal. 2022, 54, 649–692. [Google Scholar] [CrossRef]
- Sattar, M.; Othman, A.R.; Akhtar, M.; Kamaruddin, S.; Khan, R.; Masood, F.; Alam, M.A.; Azeem, M.; Mohsin, S. Curve Fitting for Damage Evolution through Regression Analysis for the Kachanov-Rabotnov Model to the Norton-Bailey Creep Law of SS-316 Material. Materials 2021, 14, 5518. [Google Scholar] [CrossRef]
- Sithickbasha, A.A.; Mahesh, S. The Role of the Constitutive Model in Creep Crack Growth Modelling. Eng. Fract. Mech. 2015, 150, 47–57. [Google Scholar] [CrossRef]
- Hyde, T.H.; Jones, I.A.; Peravali, S.; Sun, W.; Wang, J.G.; Leen, S.B. Anisotropic Creep Behaviour of Bridgman Notch Specimens. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2005, 219, 163–175. [Google Scholar] [CrossRef]
- Arbel, A. Estimating Creep Rates during Tensile Cyclic Loading. J. Test. Eval. 1997, 25, 358–361. [Google Scholar] [CrossRef]
- Jamil, A.; Varshney, V. A User-Defined Subroutine for Numerical Modelling of Failure Due to Creep under Compression in Carbon Fibre Reinforced Composite Materials. Mater. Res. Express 2019, 6, 085609. [Google Scholar] [CrossRef]
- Norton, F.H. The Creep of Steel at High Temperatures; McGraw-Hill: New York, NY, USA, 1929. [Google Scholar]
- Bailey, R.W. The Utilization of Creep Test Data in Engineering Design. Proc. Inst. Mech. Eng. 1935, 131, 131–349. [Google Scholar] [CrossRef]
- Graham, A.; Walles, K.F.A. Relation between Long and Short Time Properties of a Commercial Alloy. J. Lron Steel Inst. 1955, 179, 105–120. [Google Scholar]
- Garofalo, F.; Butrymowicz, D.B. Fundamentals of Creep and Creep-Rupture in Metals. Phys. Today 1966, 19, 100–102. [Google Scholar] [CrossRef]
- Wang, X. Research and Application of High Temperature Creep Theory Model and Numerical Simulation for Uns N10003 Alloy. Ph.D. Thesis, Shanghai Institute of Applied Physics, Chinese Academy of Sciences, Shanghai, China, 2018. (In Chinese). [Google Scholar]
- Gebrehiwot, S.Z.; Espinosa-Leal, L.; Linderbäck, P.; Remes, H. Experimental Investigation and Modelling of the Nonlinear Creep Behaviour of Additive-Manufactured Carbon Fibre-Reinforced Polyethylene Terephthalate (CF-PET). Compos. Part C Open Access 2024, 15, 100530. [Google Scholar] [CrossRef]
- Dong, S.; Gong, Z.; Chen, Z.; Qu, Y.; Chen, R.; Liu, S.; Li, G. High Temperature Tensile Creep Behavior and Microstructure Evolution of Ti60 Alloy Rolled Sheet. Mater. Today Commun. 2024, 41, 110805. [Google Scholar] [CrossRef]
- Lin, L.; Zhao, S.; Zheng, T.; Lin, Y.; Chen, N.Z.; Zhang, J. Fatigue Assessment for Tower Bolts of Floating Offshore Wind Turbine Considering Preload Loss Due to Ambient Temperature Creep. Ocean Eng. 2024, 313, 119527. [Google Scholar] [CrossRef]
- Shi, J.; Qiang, B.; Xie, Y.; Xie, Q.; Liu, X. Determination of Creep Behavior of Vessel Steel Q345R at Elevated Temperatures. Int. J. Press. Vessel. Pip. 2024, 211, 105273. [Google Scholar] [CrossRef]
- He, L.; Zhang, B.; Zhou, L. Influence of Creep Constitutive Models for an AlSiCu Alloy on Load Distribution of Bolted Joint. Eng. Fail. Anal. 2021, 128, 105627. [Google Scholar] [CrossRef]
- Fehér, A.; Linn, S.; Schwienheer, M.; Scholz, A.; Berger, C. An Interactive Approach to Creep Behavior Modeling. Mater. Sci. Eng. A 2009, 510–511, 29–34. [Google Scholar] [CrossRef]
- Rojas-Ulloa, C.; Morch, H.; Tuninetti, V.; Duchêne, L.; Habraken, A.M. Implementation of a Modified Graham-Walles Viscosity Function within a Chaboche Viscoplastic Constitutive Model. Comput. Math. Appl. 2024, 155, 165–175. [Google Scholar] [CrossRef]
- Hou, J. Study on Method of Evaluating the Creep Fatigue Damage and Life for Submersible Pressure Shell. Master’s Thesis, Dalian University of Technology, Dalian, China, 2020. (In Chinese) [Google Scholar] [CrossRef]
- Jiao, T.; Liu, G.; Guo, Y.; Li, Y.; Hu, X.; Zhang, H. Study on Cold Creep-Fatigue Characteristics of Pressure-resistant Shells with Multi-sphere Junction for Deep-sea Submersibles. Shipbuild. China 2023, 64, 157–168. (In Chinese) [Google Scholar]
- Li, Y. Research on Fatigue-Creep Damage and Life Prediction of Pressure Hull. Master’s Thesis, Dalian University of Technology, Dalian, China, 2023. (In Chinese) [Google Scholar] [CrossRef]
- Zhao, B. Failure Mechanism Study for the Windows of the Deep-Sea Human Occupied Vehicle. Ph.D. Thesis, Jimei University, Xiamen, China, 2024. (In Chinese) [Google Scholar] [CrossRef]
- Wang, L.; Qu, P.; Huang, J.; Zhang, A.; Wan, Z. Creep Numerical Calculation Methods and Test Verification for Pressure Structures of Titanium Alloy. J. Ship Mech. 2019, 23, 190–199. (In Chinese) [Google Scholar]
- Liu, D. Study on Creep Properties of Titanium Alloy Ring Ribbed Cylindrical Shell Under Deep Sea Pressure. Master’s Thesis, Harbin Engineering University, Harbin, China, 2023. (In Chinese) [Google Scholar] [CrossRef]
- Findley, W.N.; Davis, F.A. Creep and Relaxation of Nonlinear Viscoelastic Materials; Courier Corporation: North Chelmsford, MA, USA, 2013. [Google Scholar]
- Faraz, M.; Besseling, N.; Korobko, A.; Picken, S. Characterization and Modeling of Creep Behavior of a Thermoset Nanocomposite. Polym. Compos. 2015, 36, 322–329. [Google Scholar] [CrossRef]
- Liang, Y.; Guan, P. Improved Maxwell Model with Structural Dashpot for Characterization of Ultraslow Creep in Concrete. Constr. Build. Mater. 2022, 329, 127181. [Google Scholar] [CrossRef]
- Liu, C.; Liu, X.; Zhang, X.; Wang, Y. Improved Unsteady Maxwell Rock Creep Model and Parameter Identificatio. China Rural. Water Hydropower 2024, 1–10. (In Chinese) [Google Scholar]
- Fliegener, S.; Hohe, J. An Anisotropic Creep Model for Continuously and Discontinuously Fiber Reinforced Thermoplastics. Compos. Sci. Technol. 2020, 194, 108168. [Google Scholar] [CrossRef]
- Militký, J.; Jabbar, A. Comparative Evaluation of Fiber Treatments on the Creep Behavior of Jute/Green Epoxy Composites. Compos. Part B Eng. 2015, 80, 361–368. [Google Scholar] [CrossRef]
- Amir, A.L.; Ishak, M.R.; Yidris, N.; Mohd Zuhri, M.Y.; Asyraf, M.R.M.; Razman, M.R.; Ramli, Z. Full-Scale Evaluation of Creep Coefficients and Viscoelastic Moduli in Honeycomb Sandwich Pultruded GFRP Composite Cross-Arms: Experimental and Numerical Study. Results Eng. 2024, 21, 101850. [Google Scholar] [CrossRef]
- Karabal, M.; Yıldız, A. Creep Resistance Enhancement and Modeling of 3D Printed Polyetherimide/Carbon Black Composites. Compos. Struct. 2024, 345, 118398. [Google Scholar] [CrossRef]
- Yang, Y. A Study of Creep Behavior of Carbon Fiber Reinforced Polypropylene Composites. Master’s Thesis, Donghua University, Shanghai, China, 2023. (In Chinese). [Google Scholar]
- Li, J.; Liu, Z.; Liu, M.; Kuang, Y.; Zhang, Z.; Chen, X. Temperature-Dependent Creep Damage Mechanism and Prediction Model of Fiber-Reinforced Phenolic Resin Composites. Int. J. Mech. Sci. 2024, 278, 109477. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, Q.; Li, x.; Zhu, S. Considering the Influence of Temperature and Stress Levels on the Nonlinear Creep Model of Gfrp in a Water Environment. Acta Mater. Compos. Sin. 2024, 24, 1–9. (In Chinese) [Google Scholar] [CrossRef]
- Almeida, J.H.S.; Ornaghi, H.L.; Lorandi, N.P.; Bregolin, B.P.; Amico, S.C. Creep and Interfacial Behavior of Carbon Fiber Reinforced Epoxy Filament Wound Laminates. Polym. Compos. 2018, 39, E2199–E2206. [Google Scholar] [CrossRef]
- Xu, S.; Gao, H.; Qiu, P.; Shen, W.; Cai, Y.; Liu, D. Stability Analysis of Acrylic Glass Pressure Cylindrical Shell Considering Creep Effect. Thin Walled Struct. 2022, 181, 110033. [Google Scholar] [CrossRef]
- Golan, O.; Arbel, A.; Eliezer, D.; Moreno, D. The Applicability of Norton’s Creep Power Law and Its Modified Version to a Single-Crystal Superalloy Type CMSX-2. Mater. Sci. Eng. A 1996, 216, 125–130. [Google Scholar] [CrossRef]
- He, Z.; Wang, F.; Wang, H.; Zhao, B. Parametric Analysis on Creep Deformation of Deep-Sea PMMA Observation Window. Appl. Sci. 2024, 14, 1040. [Google Scholar] [CrossRef]
- Lan, X. Research on Influence of Multiaxial Creep on Life of Key Components in Ultra-Supercritical Units. Ph.D. Thesis, North China Electric Power University, Beijing, China, 2018. (In Chinese). [Google Scholar]
- Alang, N.; Nikbin, K. A New Approach to Predict Creep Rupture of Grade 92 Steel Under Multiaxial Stress States. Int. J. Mech. Sci. 2019, 163, 105096. [Google Scholar] [CrossRef]
- Nikbin, K. A Unified Multiscale Ductility Exhaustion Based Approach to Predict Uniaxial, Multiaxial Creep Rupture and Crack Growth. Eng. Fract. Mech. 2017, 179, 240–259. [Google Scholar] [CrossRef]
- Zhang, K.; Tan, J.P.; Sun, W.; Nikbin, K.; Tu, S.T. Determination of Multiaxial Stress Rupture Criteria for Creeping Materials: A Critical Analysis of Different Approaches. J. Mater. Sci. Technol. 2023, 137, 14–25. [Google Scholar] [CrossRef]
- Zhang, K.; Zhu, W.B.; Tan, J.P.; Zhang, Y.C.; Zhang, C.C.; Gong, C.Y.; Zhang, X.C.; Tu, S.T. Equivalent Conversion of Different Multiaxial Stress Rupture Criteria for Creep Materials Based on Skeletal Point Stresses. Eng. Fract. Mech. 2024, 310, 110439. [Google Scholar] [CrossRef]
- Yao, H.; Xuan, F.; Wang, Z.; Tu, S. Multi-Axial Creep Design Models Based on the Cavity Growth Theory and Its Applications in Engineering. Nucl. Power Eng. 2007, 28, 72–77. (In Chinese) [Google Scholar]
- Zhang, Y. Creep Damage and Crack Growth Analysis of the Brazed Joint Under Multi-Axial Stress State. Ph.D. Thesis, East China University of Science and Technology, Shanghai, China, 2016. (In Chinese). [Google Scholar]
- Chang, Y. Research on Creep Damage and Microstructual Evolution of Power Station Components Under Complex Stress States. Ph.D. Thesis, North China Electric Power University, Shanghai, China, 2017. (In Chinese). [Google Scholar]
- Zhu, J.; Wang, D.; Bao, S.; Zhou, Q.; Qin, Z.; Zhong, F.; Mao, J. Experimental and Numerical Study on Multiaxial Creep Behavior of 16MND5 Steel at 700 °C. J. Nucl. Mater. 2022, 558, 153387. [Google Scholar] [CrossRef]
- Guo, Y.; Liu, G. Research on Assessment Method of Creep Buckling Strength of Titanium Alloy Pressure Shell for Deep-sea Submersible. Shipbuild. China 2024, 65, 25–36. (In Chinese) [Google Scholar]
- Guo, Y.; Liu, G.; Huang, Y. A Complemented Multiaxial Creep Constitutive Model for Materials with Different Properties in Tension and Compression. Eur. J. Mech. A/Solids 2022, 93, 104510. [Google Scholar] [CrossRef]
- He, Z.; Wang, F.; Li, Y. Analysis on Creep Deformation of Deep-Sea Fullytransparent Pressure-Resistant Hull (in Chinese). Mech. Eng. 2024, 46, 559–569. [Google Scholar]
- Guo, Y.; Liu, G.; Xu, J.; Jiao, T. Numerical Analysis on the Creep-Fatigue Damage of Titanium Alloy Deep-Sea Pressure Hull at Room Temperature. Mar. Struct. 2024, 96, 103639. [Google Scholar] [CrossRef]
- Nguyen, D.; Ogale, A. Compressive and Flexural Creep Behavior of Carbon Fiber/PEEK Composites. J. Thermoplast. Compos. Mater. 1991, 4, 83–99. [Google Scholar] [CrossRef]
- Goertzen, W.K.; Kessler, M. Creep Behavior of Carbon Fiber/Epoxy Matrix Composites. Mater. Sci. Eng. A 2006, 421, 217–225. [Google Scholar] [CrossRef]
- Antin, K.N.; Laukkanen, A.; Andersson, T.; Smyl, D.; Vilaça, P. A Multiscale Modelling Approach for Estimating the Effect of Defects in Unidirectional Carbon Fiber Reinforced Polymer Composites. Materials 2019, 12, 1885. [Google Scholar] [CrossRef]
- Zheng, X.; Zheng, X.; Linhu, G. The Research Progress on Multiscale Method for the Mechanical Analysis of Composites. Adv. Mech. 2010, 40, 41–56. (In Chinese) [Google Scholar]
- Shen, G.; Hu, G.; Liu, B. Mechanics of Composite Materials, 2nd ed.; Tsinghua University Press: Beijing, China, 2013. (In Chinese) [Google Scholar]
- Barbero, E.J. Finite Element Analysis of Composite Materials Using Abaqus®; CRC Press: Boca Raton, FL, USA, 2023. [Google Scholar] [CrossRef]
- Li, S. Study on the Multi-Scale and Refine Design of Composite Materials. Ph.D. Thesis, Hunan University, Changsha, China, 2023. (In Chinese) [Google Scholar] [CrossRef]
- Zhou, J. Numerical Simulation Study of Macroscopic Material Constants of Short Fiber Reinforced Composites. Master’s Thesis, Henan Polytechnic University, Jiaozuo, China, 2023. (In Chinese) [Google Scholar] [CrossRef]
- Naili, C.; Doghri, I.; Kanit, T.; Sukiman, M.S.; Aissa-Berraies, A.; Imad, A. Short Fiber Reinforced Composites: Unbiased Full-Field Evaluation of Various Homogenization Methods in Elasticity. Compos. Sci. Technol. 2020, 187, 107942. [Google Scholar] [CrossRef]
- Spilker, K.; Lebensohn, R.A.; Jacobsen, G.; Capolungo, L. A Mean Field Homogenization Model for the Mechanical Response of Ceramic Matrix Composites. Compos. Struct. 2025, 352, 118630. [Google Scholar] [CrossRef]
- Wang, X. Crystal Plasticity Simulation Study on the Effect of Microstructure of P92 Steel on Mechanical Behavior. Master’s Thesis, Tianjin University of Technology, Tianjin, China, 2022. (In Chinese) [Google Scholar] [CrossRef]
- Ye, F. The Research on the Optimization and Design of Variable Stiffness Composite Material Based on the Surrogate Method. Ph.D. Thesis, Hunan University, Changsha, China, 2021. (In Chinese) [Google Scholar] [CrossRef]
- Qijun, Z. Crystal Plasticity Based Micromechanical Simulation and Investigation of Creep for P91 Steels. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2019. (In Chinese) [Google Scholar] [CrossRef]
- Xiao, H.R.; Cai, L.X.; Han, G.Z. Semi-Analytical Creep Model to Obtain Norton’s Law of Materials under Flat Indentation and Its Applications. J. Mater. Res. Technol. 2023, 25, 905–911. [Google Scholar] [CrossRef]
- Katouzian, M.; Vlase, S.; Scutaru, M.L. Finite Element Method-Based Simulation Creep Behavior of Viscoelastic Carbon-Fiber Composite. Polymers 2021, 13, 1017. [Google Scholar] [CrossRef] [PubMed]
- Katouzian, M.; Vlase, S. Creep Response of Carbon-Fiber-Reinforced Composite Using Homogenization Method. Polymers 2021, 13, 867. [Google Scholar] [CrossRef] [PubMed]

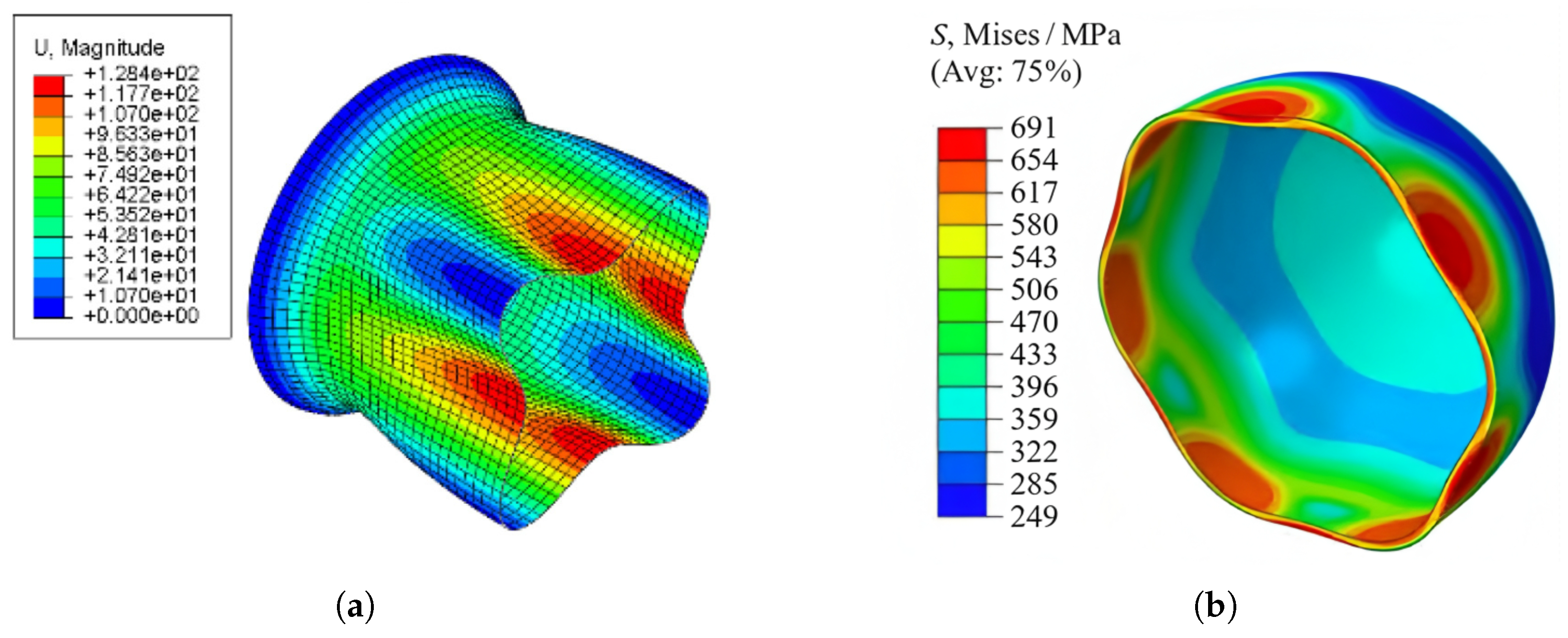
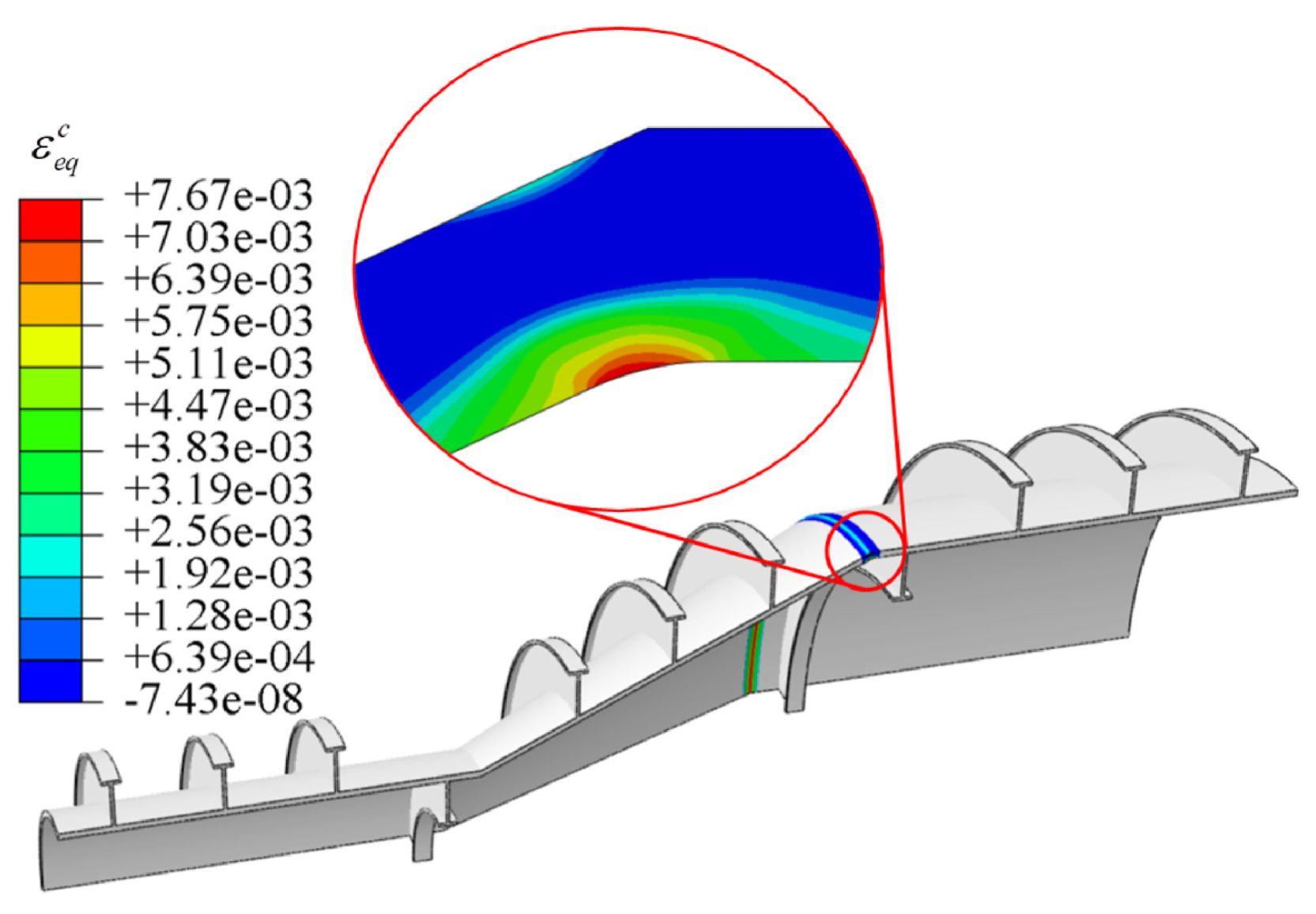
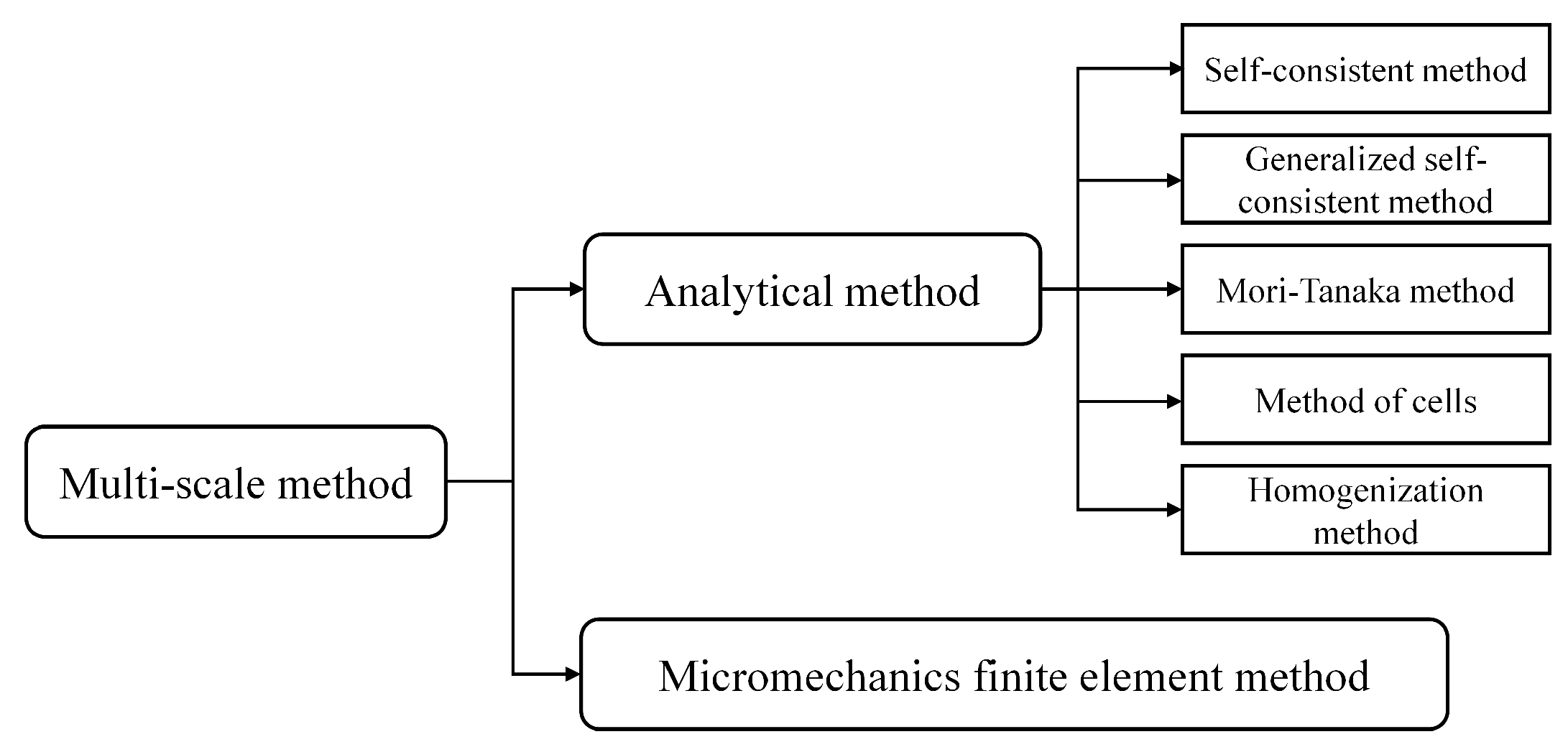
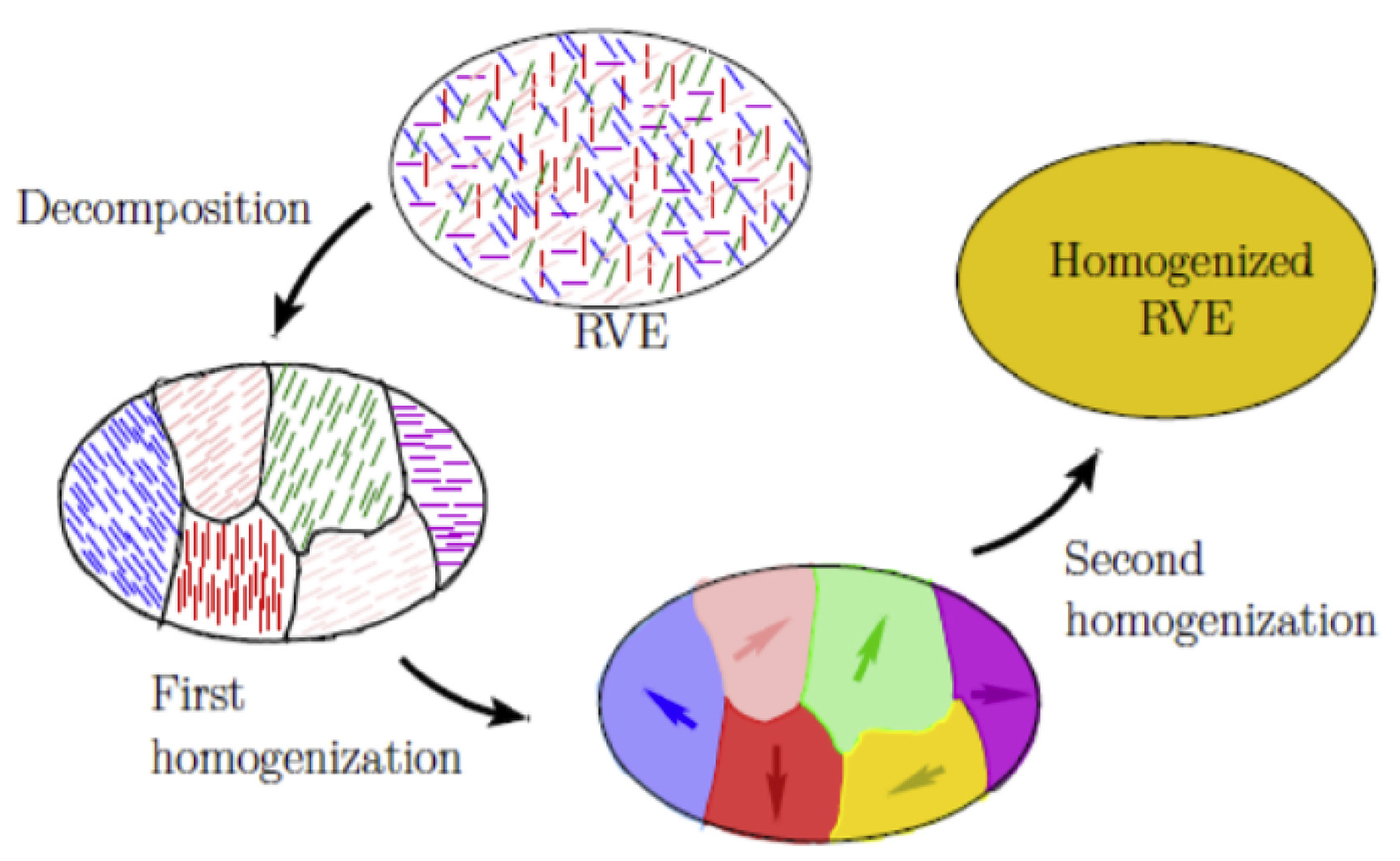
| Model | Equation | Application | Characteristic |
|---|---|---|---|
| Norton | The secondary creep phase | ||
| Norton (General form) | The secondary creep phase | ||
| Time-hardening | The primary creep phase | ||
| Strain-hardening | The primary creep phase | ||
| Norton–Bailey | The primary and secondary creep phase | The model aligns with the creep behavior of titanium alloys but demonstrates limited adaptability to datasets involving significant stress variations [45], making it challenging to describe complex working conditions [43]. | |
| Strain-hardening (Constant temperature) | The primary creep phase | ||
| Time-hardening (Modified) | The primary and secondary creep phase | ||
| Graham | All three phases of creep | It achieves higher fitting accuracy under elevated temperature conditions compared to the Norton–Bailey model [36], but the large number of parameters may impede the convergence of creep numerical calculations [44]. | |
| Findley | The primary and secondary creep phase | It is suitable for viscoelastic materials and exhibits strong fitting performance at high temperatures. | |
| Burger | The primary and secondary creep phase | It is suitable for viscoelastic materials. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zeng, Y.; Yu, C.; Yang, S. Creep Behavior Research of Deep-Sea Pressure Hull: A Review. J. Mar. Sci. Eng. 2025, 13, 749. https://doi.org/10.3390/jmse13040749
Zeng Y, Yu C, Yang S. Creep Behavior Research of Deep-Sea Pressure Hull: A Review. Journal of Marine Science and Engineering. 2025; 13(4):749. https://doi.org/10.3390/jmse13040749
Chicago/Turabian StyleZeng, Yuan, Changli Yu, and Shuo Yang. 2025. "Creep Behavior Research of Deep-Sea Pressure Hull: A Review" Journal of Marine Science and Engineering 13, no. 4: 749. https://doi.org/10.3390/jmse13040749
APA StyleZeng, Y., Yu, C., & Yang, S. (2025). Creep Behavior Research of Deep-Sea Pressure Hull: A Review. Journal of Marine Science and Engineering, 13(4), 749. https://doi.org/10.3390/jmse13040749




