Underwater Target Bearing Estimation Performance of Bottom-Mounted Extended Coprime Sparse Array
Abstract
1. Introduction
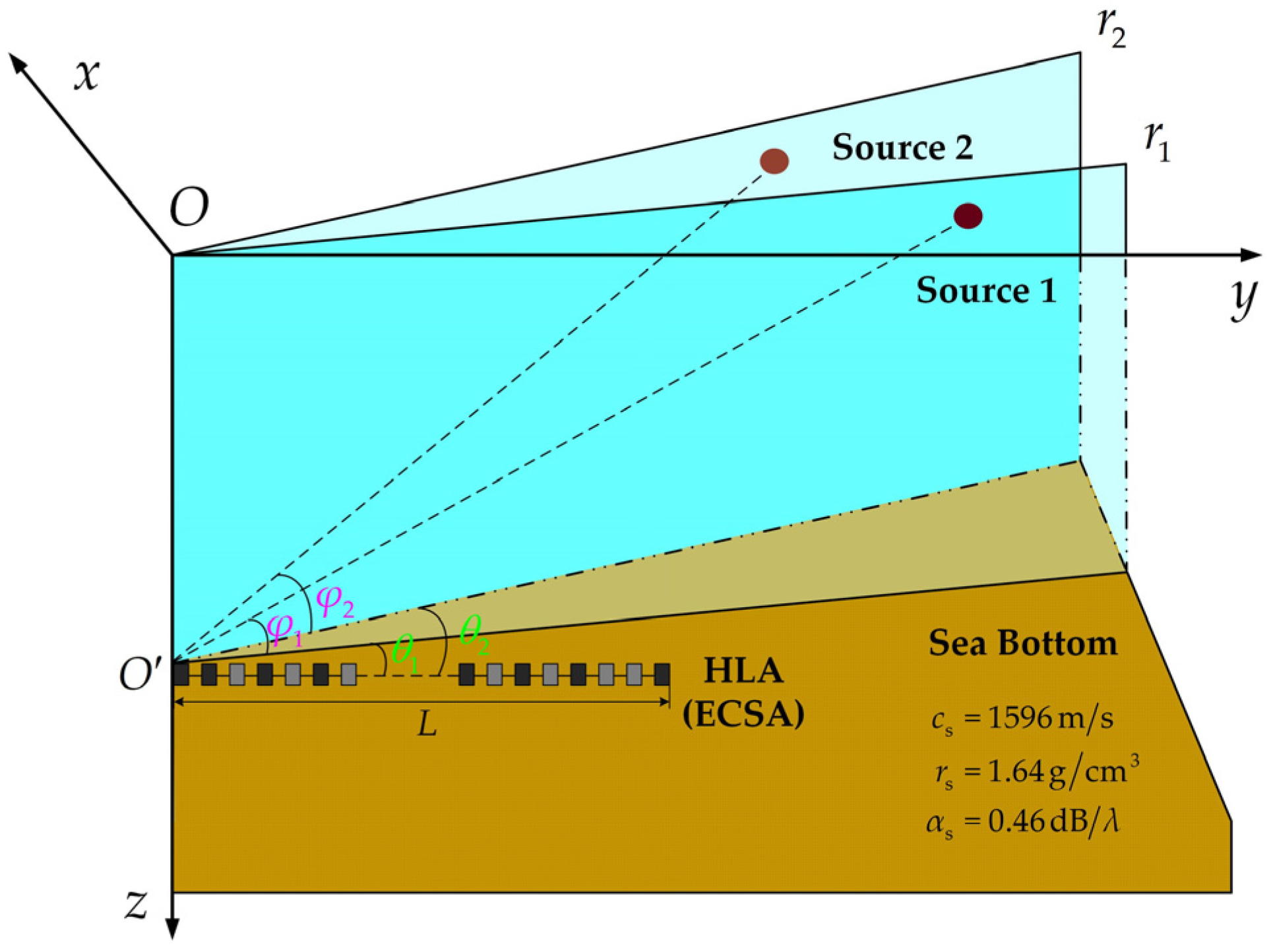
2. ECSA in Underwater Waveguide
2.1. Configuration of ECSA and Spatial Smoothing Technique
2.2. Bearing Deviation of Underwater HLA
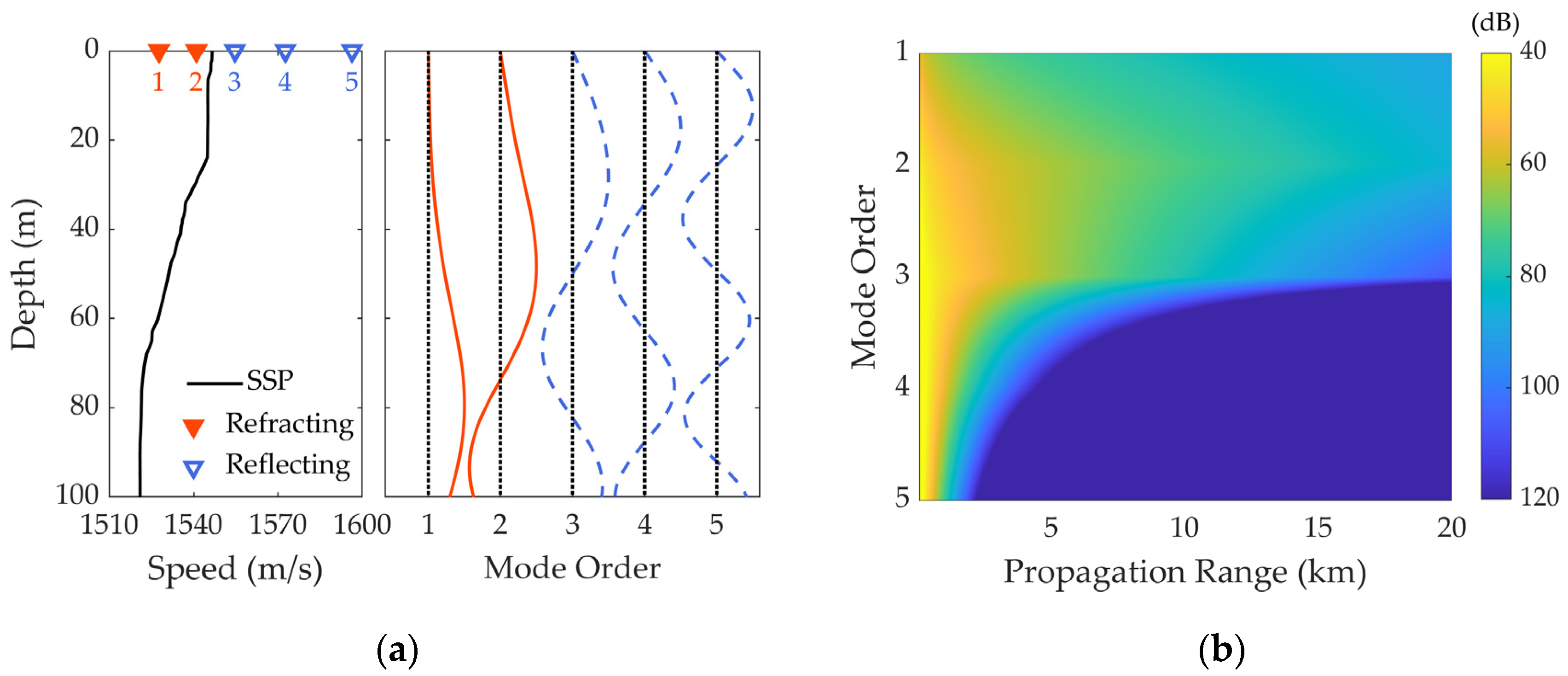
2.3. Energy Trapping of Refracting Modes
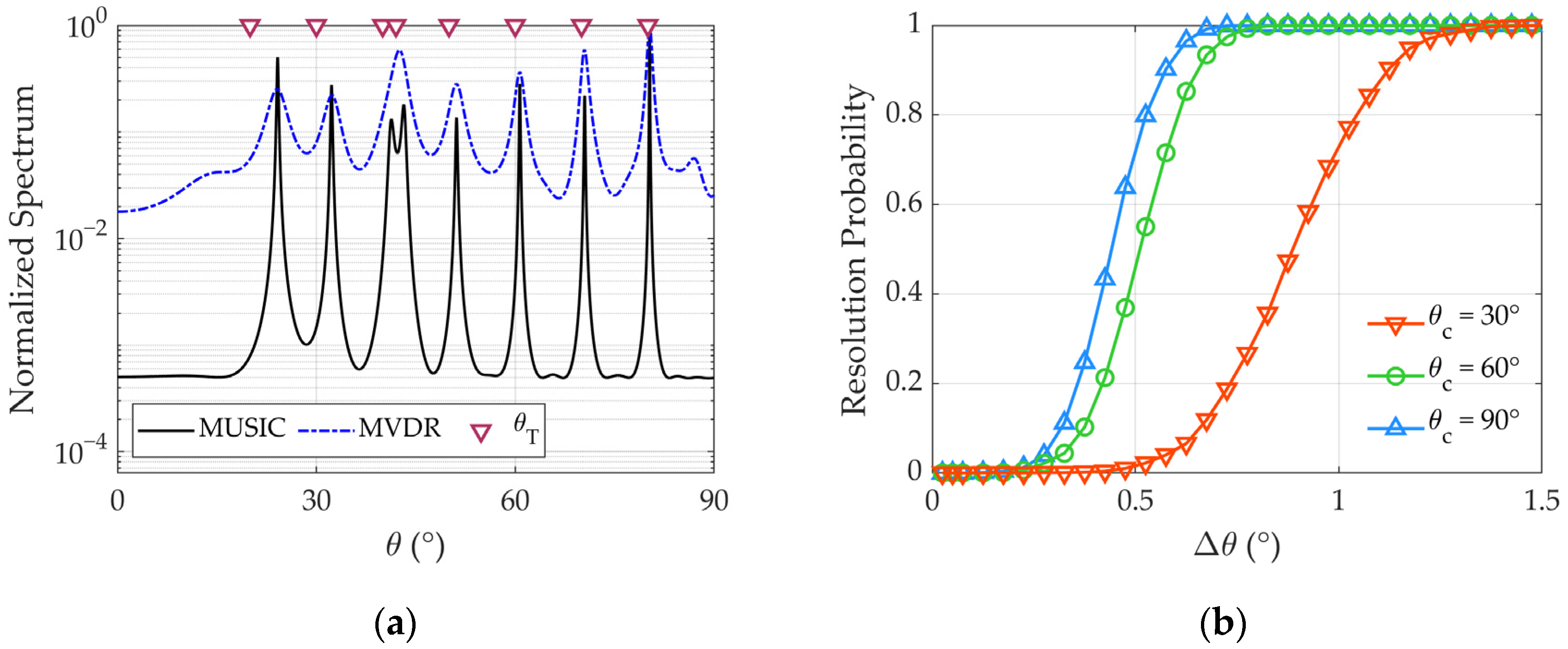
2.4. Performance of the MUSIC Estimator
3. Numerical Analysis
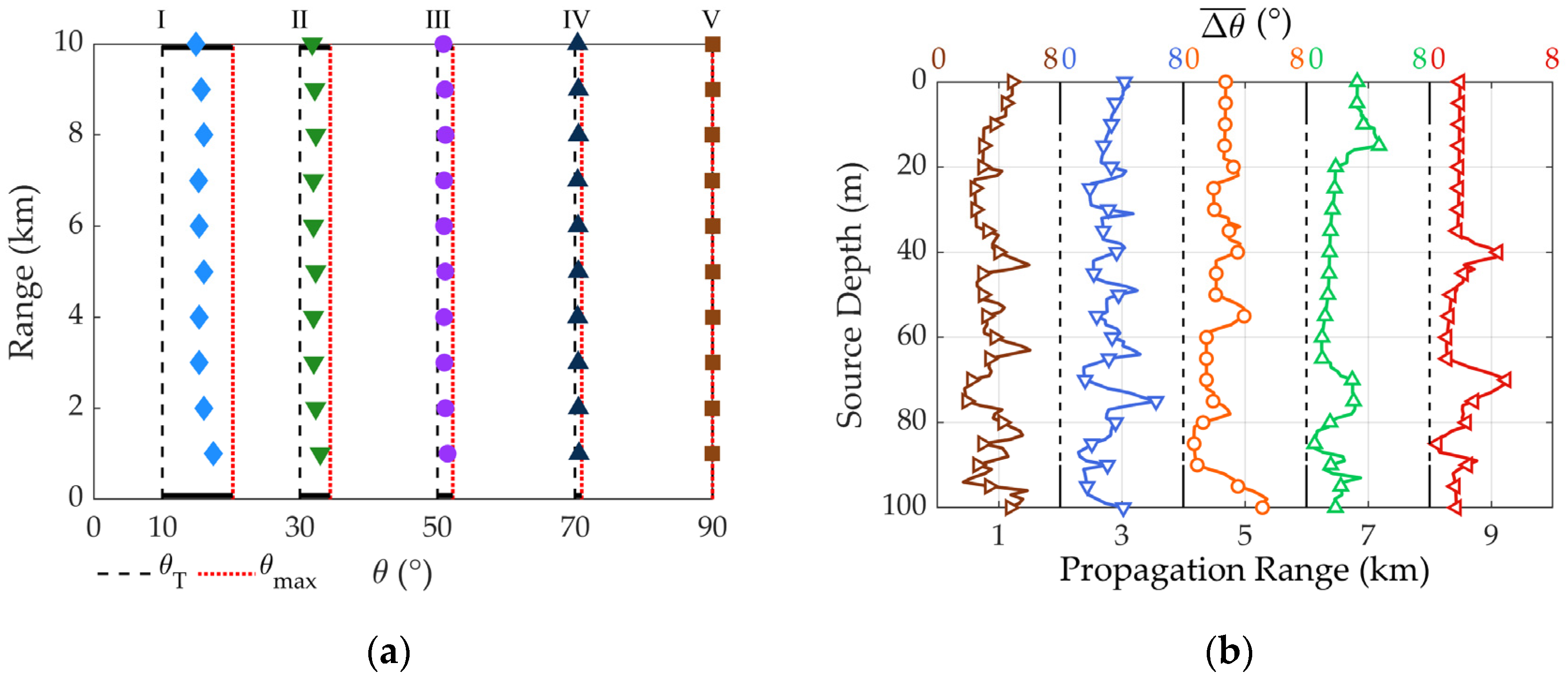
3.1. Bearing Estimation in Underwater Waveguide
3.2. Impact of SSP Structure
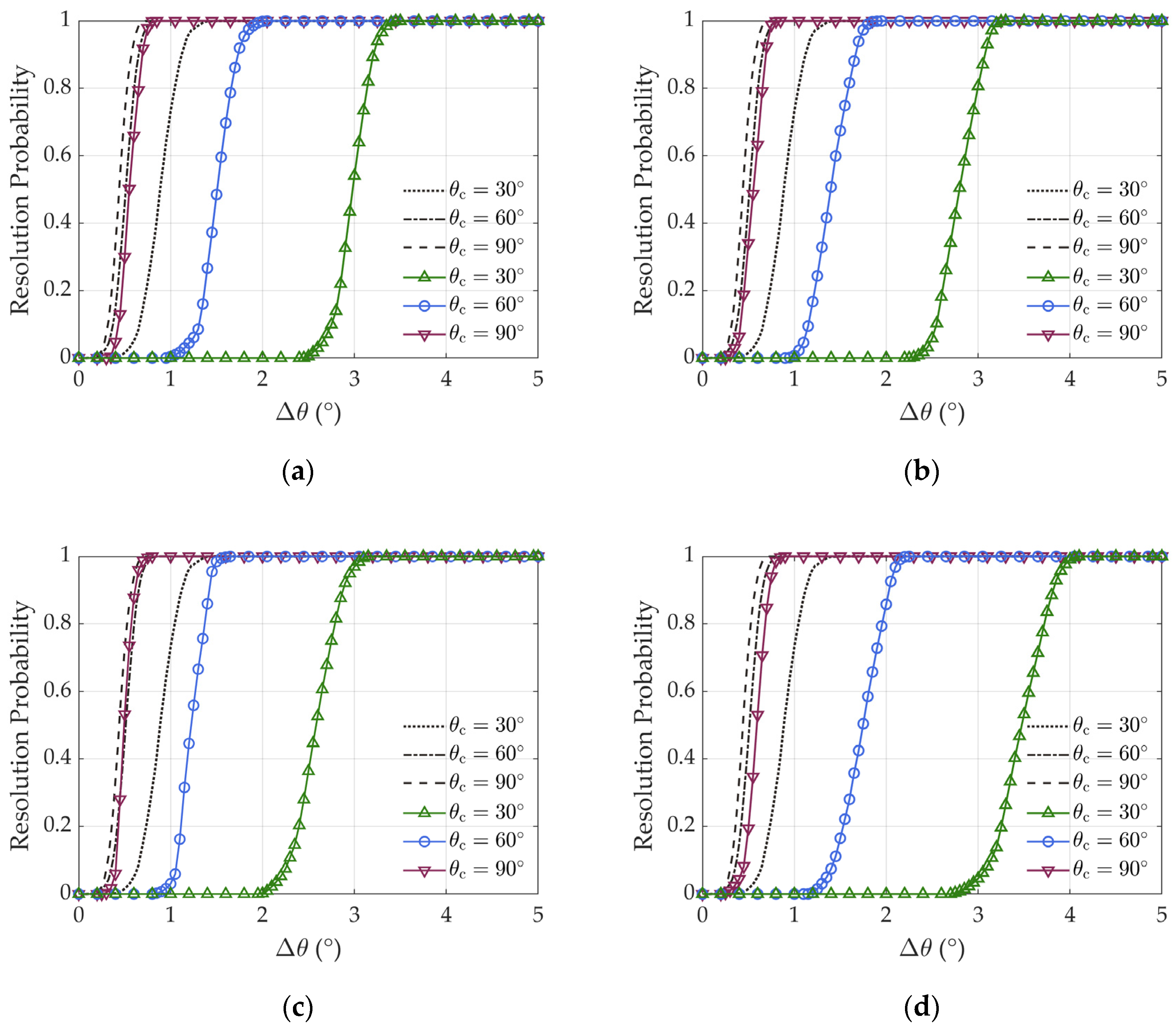
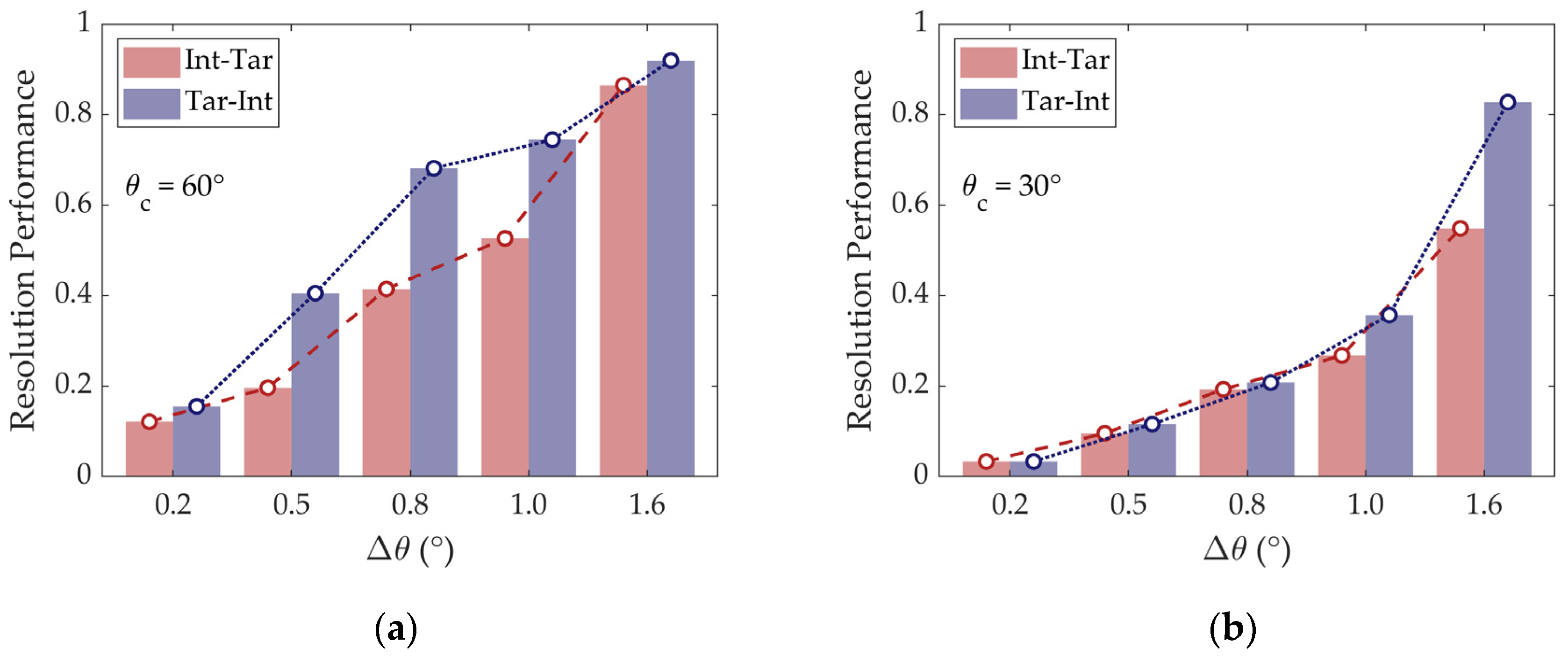
3.3. Performance of Source Resolution
3.4. Impact of Source Position
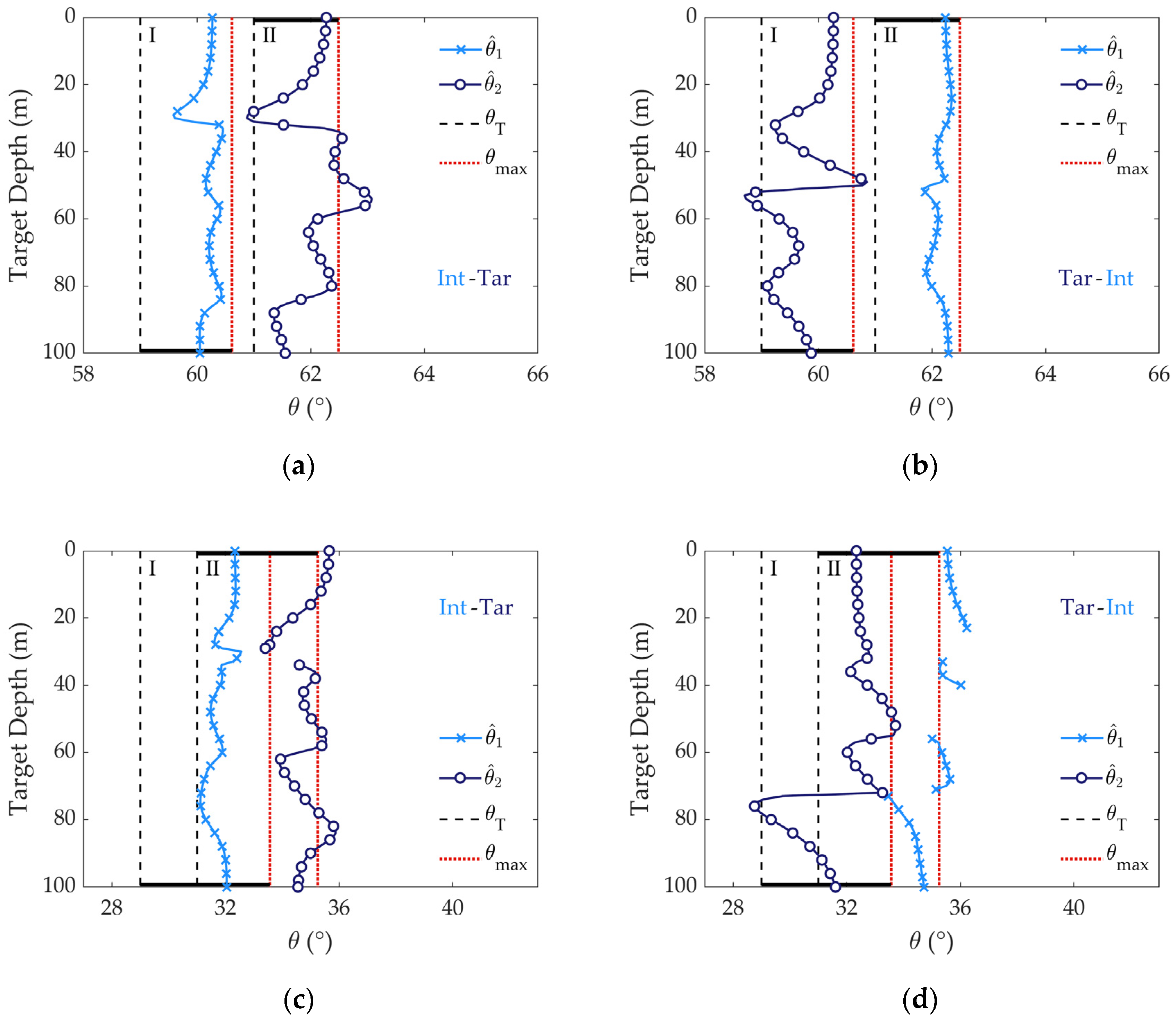
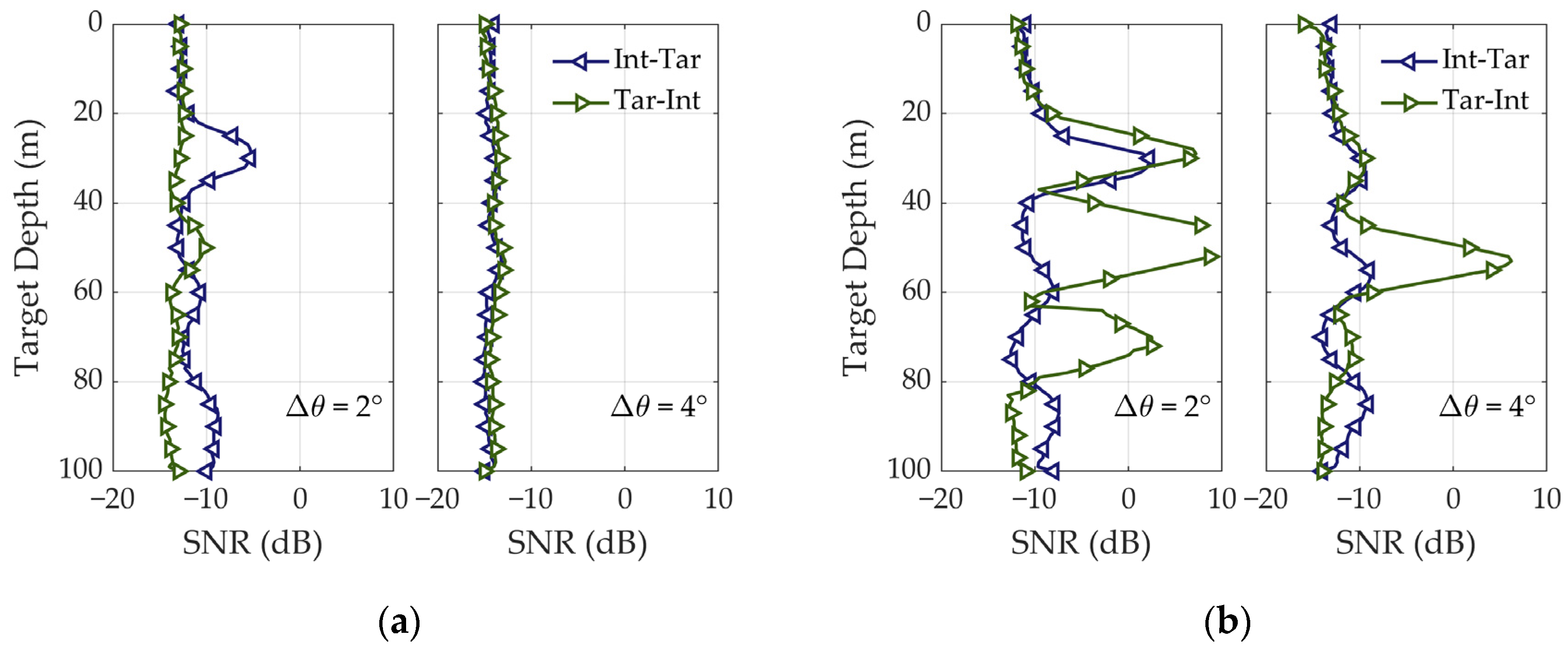
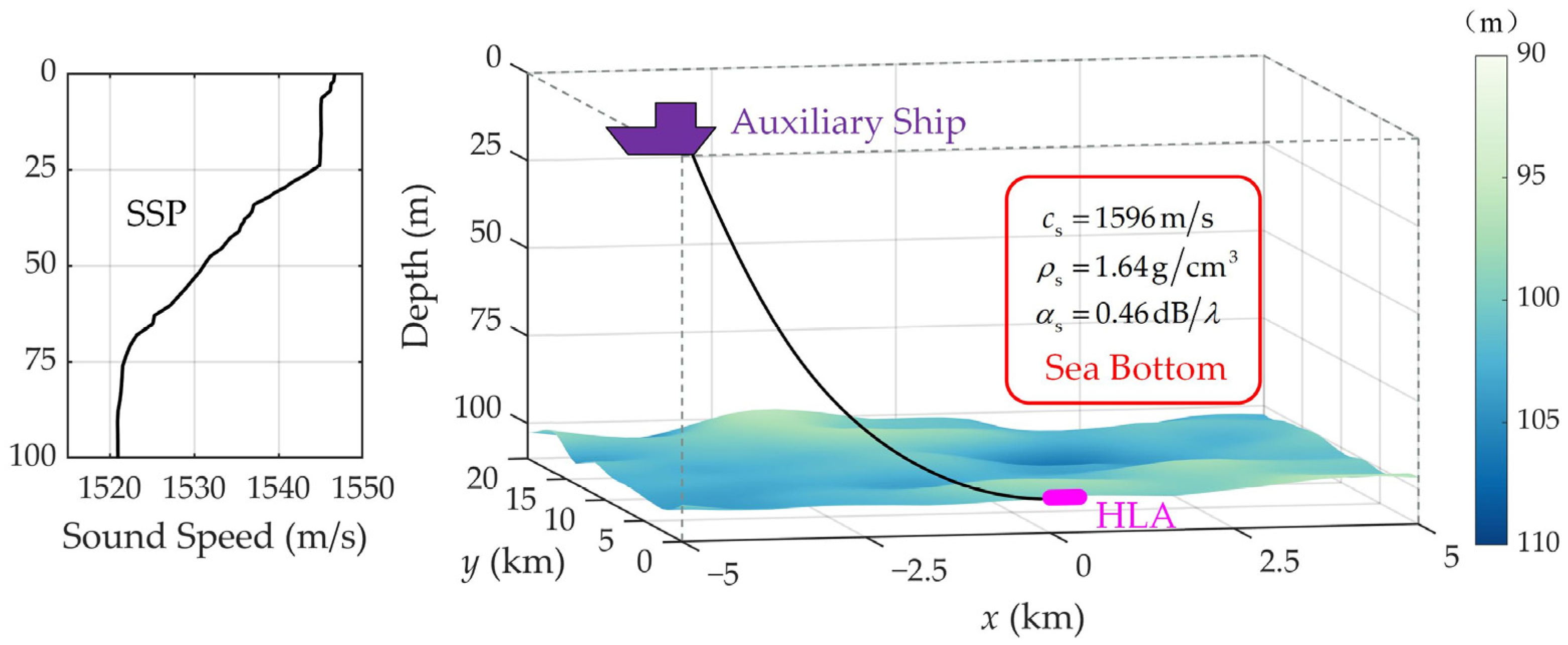
4. Experimental Result
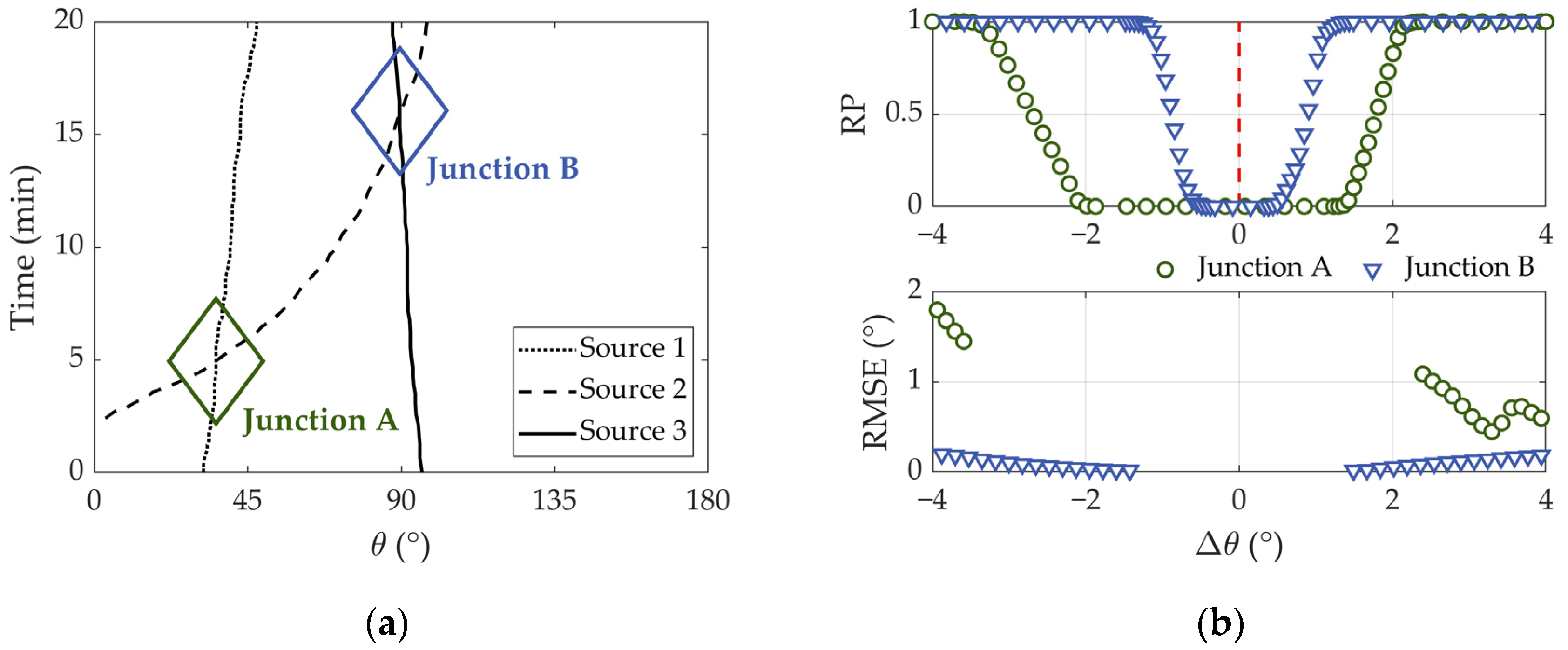
- In the presence of a near-surface interferer, the bearing estimation performance is significantly influenced by the target position. Primarily, it could be attributed to the multimode mechanism and shows distinct dependence on the target–interferer angular arrangement;
- Since junction B is near the broadside direction, the minimum angular separation for complete resolution gets smaller. By designating = 0° as the central axis, symmetrical characteristics can be detected, which indicates that bearing deviation barely occurs. Before and after the track crossing, the minimum angular separation is 1.43° and 1.49°, respectively;
- However, in terms of junction A, the deviating bearing estimation results in asymmetric characteristics. And in order to completely resolve the sources, the required minimum angular separations before and after their track crossing are 3.59° and 2.39°, respectively;
- From the aspect of bearing estimation accuracy of the target, the bearing deviation also governs. It is notable that more significant variation can be observed for bearing estimates around junction A. Before the track crossing, the averaged RMSE is about 1.72°, while it is reduced to about 0.71° after the crossing. In addition, compared with the estimates obtained by the use of measured sound speed, RMSE is decreased by 79% and 62%, respectively. For junction B, the RMSE is no more than 0.2° in the considered interval of [–4, 4]°, and the decrease achieved by reference sound speed substitution is around 54%.
5. Summary and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vaidyanathan, P.P.; Pal, P. Sparse sensing with co-prime samplers and arrays. IEEE Trans. Signal Process. 2010, 59, 573–586. [Google Scholar] [CrossRef]
- Pal, P.; Vaidyanathan, P.P. Coprime sampling and the MUSIC algorithm. In Proceedings of the IEEE DSP/SPE Workshop, Sedona, AZ, USA, 4–7 January 2011; pp. 289–294. [Google Scholar]
- Pal, P.; Vaidyanathan, P.P. Gridless methods for underdetermined source estimation. In Proceedings of the IEEE 48th Asilomar Conference on Signals, Systems and Computers, Pacific Grove, CA, USA, 2–5 November 2014; pp. 111–115. [Google Scholar]
- Zheng, W.; Zhang, X.; Gong, P.; Zhai, H. DOA estimation for coprime linear arrays: An ambiguity-free method involving full DOFs. IEEE Commun. Lett. 2017, 22, 562–565. [Google Scholar] [CrossRef]
- Yang, T.C.; Ye, Z. Array gain of coprime arrays. J. Acoust. Soc. Am. 2019, 146, EL306–EL309. [Google Scholar] [CrossRef] [PubMed]
- Qin, S.; Zhang, Y.D.; Amin, M.G. Generalized coprime array configurations for direction-of-arrival estimation. IEEE Trans. Signal Process. 2015, 63, 1377–1390. [Google Scholar] [CrossRef]
- Adhikari, K.; Buck, J.R.; Wage, K.E. Beamforming with extended co-prime sensor arrays. In Proceedings of the IEEE ICASSP, Vancouver, BC, Canada, 26–31 May 2013; pp. 4183–4186. [Google Scholar]
- Adhikari, K.; Buck, J.R.; Wage, K.E. Extending coprime sensor arrays to achieve the peak side lobe height of a full uniform linear array. EURASIP J. Adv. Signal Process. 2014, 2014, 148. [Google Scholar] [CrossRef]
- Moghadam, G.S.; Shirazi, A.B. Direction of arrival (DOA) estimation with extended optimum co-prime sensor array (EOCSA). Multidimens. Syst. Signal Process. 2022, 33, 17–37. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, H.; Gao, Y.; Wang, Z. DOA estimation of underwater acoustic co-frequency sources for the coprime vector sensor array. Front. Mar. Sci. 2023, 10, 1211234. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, H.; Lv, Y. Improving the beamforming performance of a vector sensor line array with a coprime array configuration. Appl. Acoust. 2023, 207, 109329. [Google Scholar] [CrossRef]
- Wang, L.; Zhao, H.; Wang, Q. Underwater sparse acoustic sensor array design under spacing constraints based on a global enhancement whale optimization algorithm. Appl. Sci. 2022, 12, 11825. [Google Scholar] [CrossRef]
- Dong, F.; Xu, L.; Li, X.; Wang, S.; Xie, X. Particle filter algorithm for underwater acoustic source DOA tracking with co-prime array. In Proceedings of the 2019 IEEE International Conference on Mechatronics and Automation (ICMA), Tianjin, China, 4–7 August 2019; pp. 2445–2450. [Google Scholar]
- Pan, J.; Sun, M.; Dong, X.; Wang, Y.; Wang, Y.; Zhang, X. Enhanced DOA estimation with co-prime array in the scenario of impulsive noise: A pseudo snapshot augmentation perspective. IEEE Trans. Veh. Technol. 2023, 72, 11603–11616. [Google Scholar] [CrossRef]
- Pan, H.; Pan, J.; Zhang, X.; Wang, Y. Time-delay estimation of ground penetrating radar using co-prime sampling strategy via atomic norm minimization. IEEE Trans. Instrum. Meas. 2024, 73, 8502812. [Google Scholar] [CrossRef]
- Su, Y.; Wang, X.; Lan, X. Co-prime array interpolation for DOA estimation using deep matrix iterative network. IEEE Trans. Instrum. Meas. 2024, 73, 2533912. [Google Scholar] [CrossRef]
- Liu, C.L.; Vaidyanathan, P.P. Remarks on the spatial smoothing step in coarray MUSIC. IEEE Signal Process. Lett. 2015, 22, 1438–1442. [Google Scholar]
- Chen, T.; Guo, M.; Guo, L. A direct coarray interpolation approach for direction finding. Sensors 2017, 17, 2149. [Google Scholar] [CrossRef]
- Zheng, Z.; Huang, Y.; Wang, W.Q.; So, H.C. Spatial smoothing PAST algorithm for DOA tracking using difference coarray. IEEE Signal Process. Lett. 2019, 26, 1623–1627. [Google Scholar]
- Wang, M.; Nehorai, A. Coarrays, MUSIC, and the Cramér-Rao bound. IEEE Trans. Signal Process. 2016, 65, 933–946. [Google Scholar]
- Wang, M.; Zhang, Z.; Nehorai, A. Performance analysis of coarray-based MUSIC and the Cramér-Rao bound. In Proceedings of the IEEE ICASSP, New Orleans, LA, USA, 5–9 March 2017; pp. 3061–3065. [Google Scholar]
- Chen, C.; Yang, K.; Duan, R.; Ma, Y. Acoustic propagation analysis with a sound speed feature model in the front area of Kuroshio Extension. Appl. Ocean Res. 2017, 68, 1–10. [Google Scholar] [CrossRef]
- Liu, J.; Piao, S.; Zhang, M.; Zhang, S.; Guo, J.; Gong, L. Characteristics of three-dimensional sound propagation in Western North Pacific fronts. J. Mar. Sci. Eng. 2021, 9, 1035. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, Y.; Meng, Z.; Chen, W. Performance of single empirical orthogonal function regression method in global sound speed profile inversion and sound field prediction. Appl. Ocean Res. 2023, 136, 103598. [Google Scholar]
- Yang, T.C. Beam intensity striations and applications. J. Acoust. Soc. Am. 2003, 113, 1342–1352. [Google Scholar] [CrossRef]
- Lakshmipathi, S.; Anand, G.V. Subspace intersection method of high-resolution bearing estimation in shallow ocean. Signal Process. 2004, 84, 1367–1384. [Google Scholar]
- Yang, K.; Li, H.; He, C.; Duan, R. Error analysis on bearing estimation of a towed array to a far-field source in deep water. Acoust. Aust. 2016, 44, 429–437. [Google Scholar]
- Lu, Y.; Yang, K.; Liu, H.; Huang, C. Effect of emergence angle on acoustic transmission in a shallow sea. Arch. Acoust. 2020, 45, 3–9. [Google Scholar]
- Wu, Y.; Zhang, W.; Hu, Z.; Zhang, W.; Zhang, B.; Wang, J.; Guo, W.; Xu, G.; Zhu, M. Directional response of a horizontal linear array to an acoustic source at close range in deep water. Acoust. Aust. 2022, 50, 91–103. [Google Scholar]
- Jensen, F.B.; Kuperman, W.A.; Porter, M.B.; Schmidt, H. Computational Ocean Acoustics, 2nd ed.; Springer: New York, NY, USA, 2011; pp. 338–341. [Google Scholar]
- Gong, Z.; Lin, J.; Guo, L. The effect of acoustic waves’ phase speed on preciseness of DOA estimation in shallow water. Acta Acust. 2002, 27, 492–496. [Google Scholar]
- Premus, V.E.; Helfrick, M.N. Use of mode subspace projections for depth discrimination with a horizontal line array: Theory and experimental results. J. Acoust. Soc. Am. 2013, 133, 4019–4031. [Google Scholar]
- Porter, M.B. The KRAKEN Normal Mode Program; Naval Research Lab.: Washington, DC, USA, 1992. [Google Scholar]
- Conan, E.; Bonnel, J.; Nicolas, B.; Chonavel, T. Using the trapped energy ratio for source depth discrimination with a horizontal line array: Theory and experimental results. J. Acoust. Soc. Am. 2017, 142, 2776–2786. [Google Scholar]
- Zhang, W.; Wu, Y.; Shi, J.; Leng, H.; Zhao, Y.; Guo, J. Surface and underwater acoustic source discrimination based on machine learning using a single hydrophone. J. Mar. Sci. Eng. 2022, 10, 321. [Google Scholar] [CrossRef]
- Zhai, D.; Zhang, B.; Li, F.; Zhang, Y.; Yang, X. Passive source depth estimation in shallow water using two horizontally separated hydrophones. Appl. Acoust. 2022, 192, 108723. [Google Scholar]
- Jensen, J.K.; Hjelmervik, K.T.; Østenstad, P. Finding acoustically stable areas through Empirical Orthogonal Function (EOF) classification. IEEE J. Ocean. Eng. 2012, 37, 103–111. [Google Scholar]
- Chen, C.; Lei, B.; Ma, Y.L.; Duan, R. Investigating sound speed profile assimilation: An experiment in the Philippine Sea. Ocean Eng. 2016, 124, 135–140. [Google Scholar]





| Method | Accuracy | Resolution | Complexity |
|---|---|---|---|
| MUSIC | High | High | High |
| MVDR | Low | Low | Medium |
| ESPRIT | Medium | Medium | Medium |
| EOF Order | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| Maximum | 9.841 | 6.385 | 4.573 | 2.168 |
| Minimum | −7.710 | −4.862 | −4.951 | −1.881 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Yang, Q.; Yang, K.; Li, X. Underwater Target Bearing Estimation Performance of Bottom-Mounted Extended Coprime Sparse Array. J. Mar. Sci. Eng. 2025, 13, 633. https://doi.org/10.3390/jmse13040633
Zhang Y, Yang Q, Yang K, Li X. Underwater Target Bearing Estimation Performance of Bottom-Mounted Extended Coprime Sparse Array. Journal of Marine Science and Engineering. 2025; 13(4):633. https://doi.org/10.3390/jmse13040633
Chicago/Turabian StyleZhang, Yukun, Qiulong Yang, Kunde Yang, and Xuegang Li. 2025. "Underwater Target Bearing Estimation Performance of Bottom-Mounted Extended Coprime Sparse Array" Journal of Marine Science and Engineering 13, no. 4: 633. https://doi.org/10.3390/jmse13040633
APA StyleZhang, Y., Yang, Q., Yang, K., & Li, X. (2025). Underwater Target Bearing Estimation Performance of Bottom-Mounted Extended Coprime Sparse Array. Journal of Marine Science and Engineering, 13(4), 633. https://doi.org/10.3390/jmse13040633





