Numerical Investigations of a Column Configuration with Towed Super Long Cable in Underwater Environment
Abstract
1. Introduction
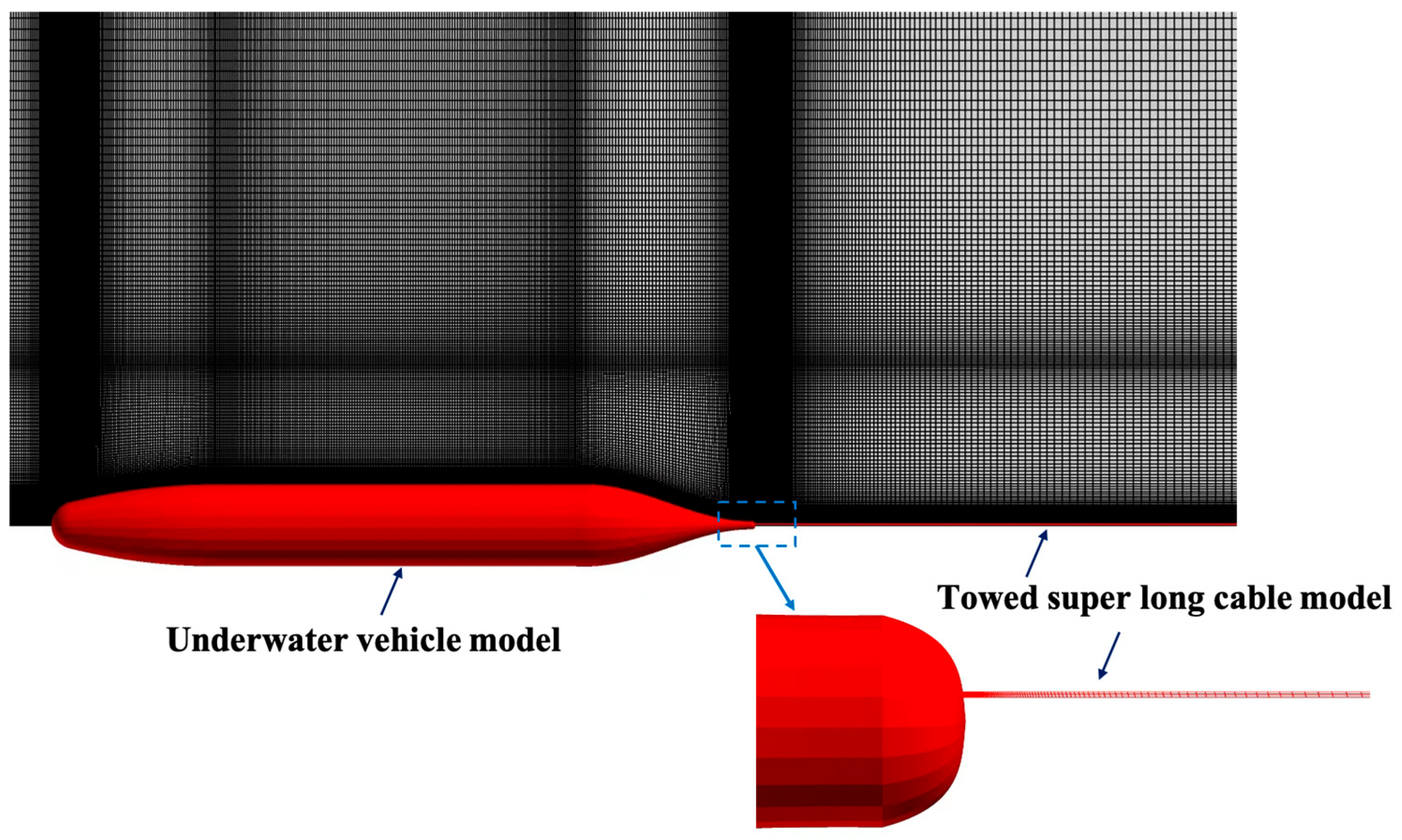
2. Underwater Vehicle-Towing Cable Model
3. Numerical Methods and Grids
3.1. Grids and Freestream Conditions
3.2. Numerical Methods
3.2.1. Flow Calculation
3.2.2. Structural Calculations
3.2.3. Fluid-Structure-Acoustic Coupling Scheme
3.3. Validation of Numerical Methods
4. Results and Discussions
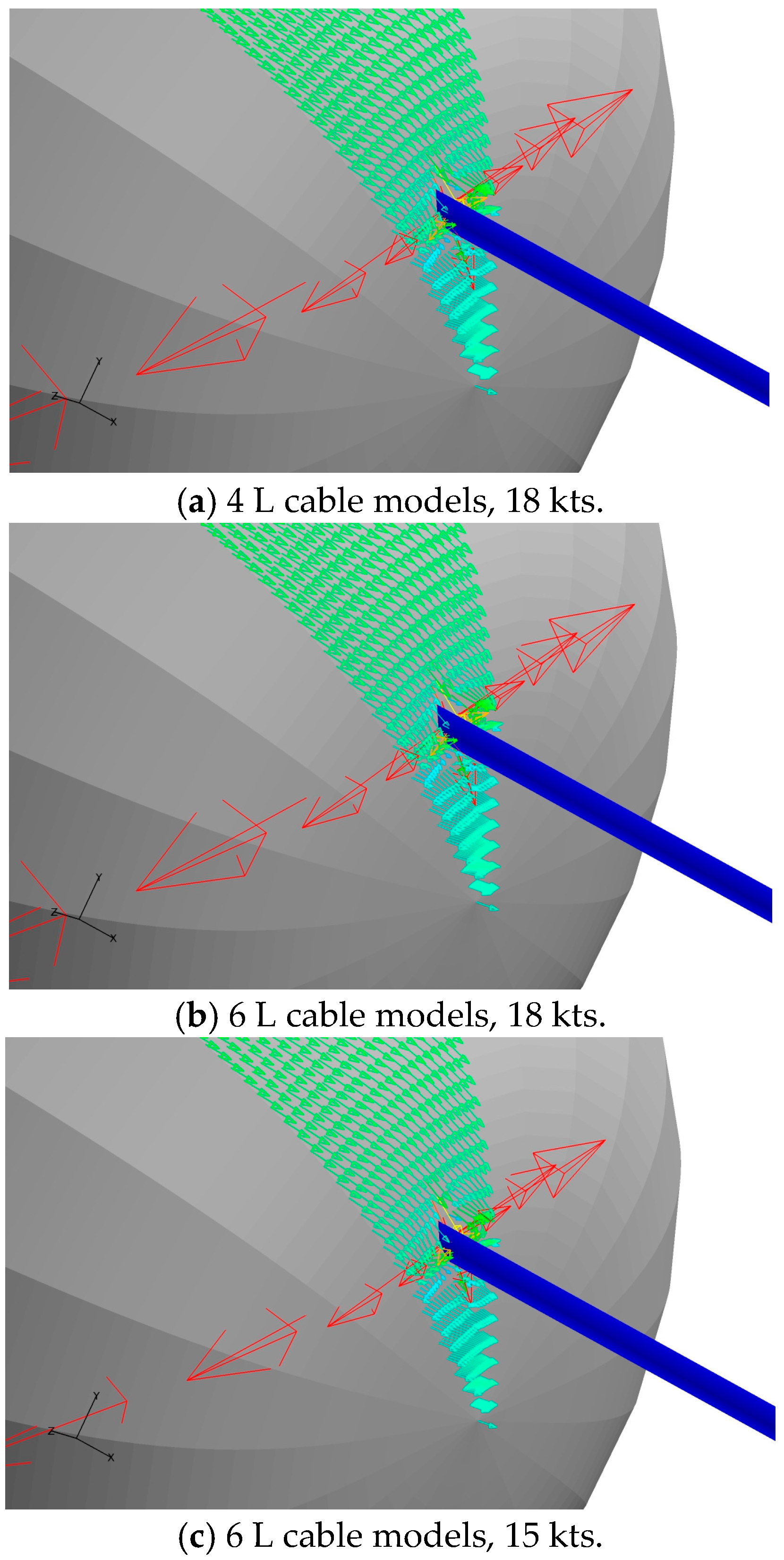
4.1. Models with Different Cable Lengths at Different Freestream Velocities
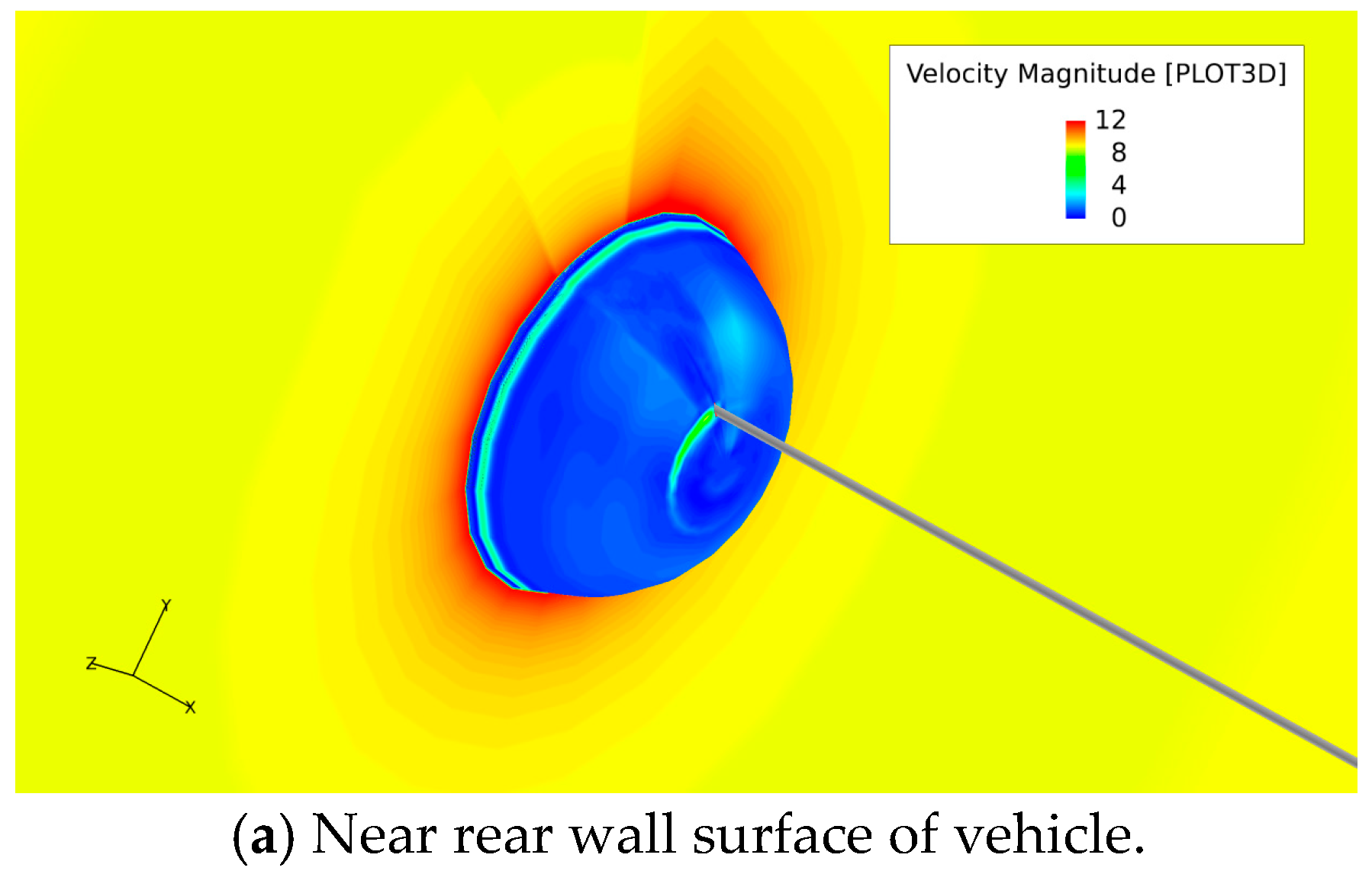
4.1.1. Flow Field Analysis
4.1.2. Flow Noise Analysis
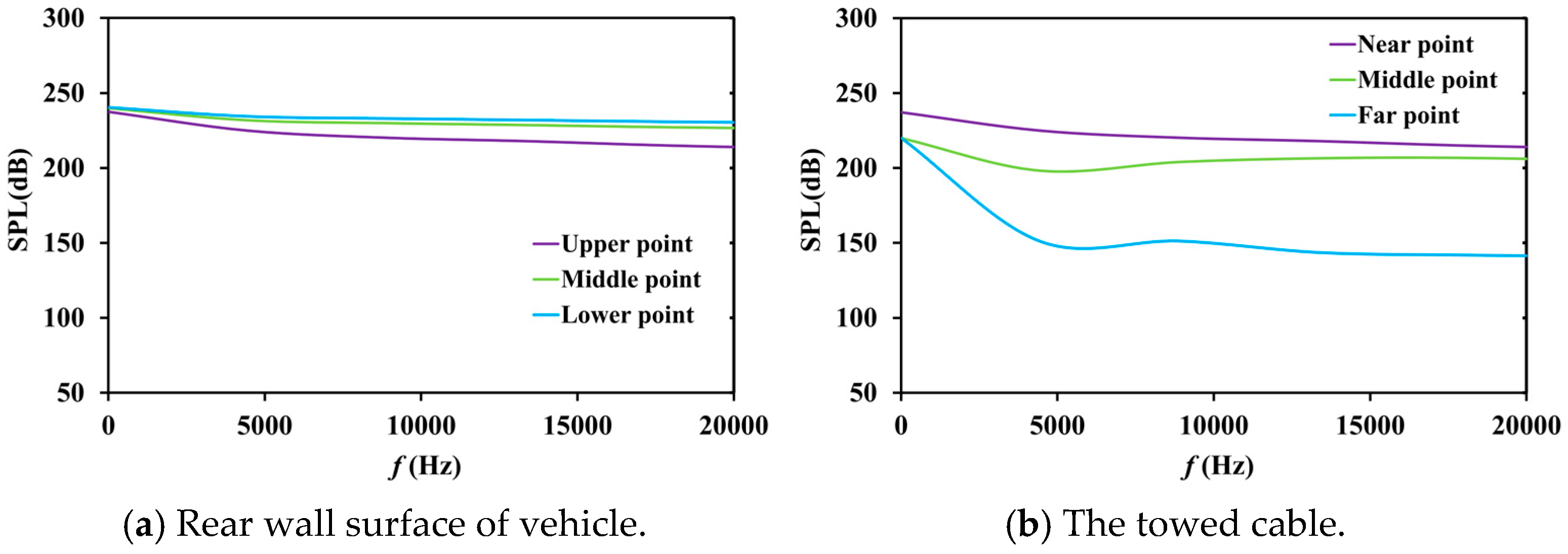
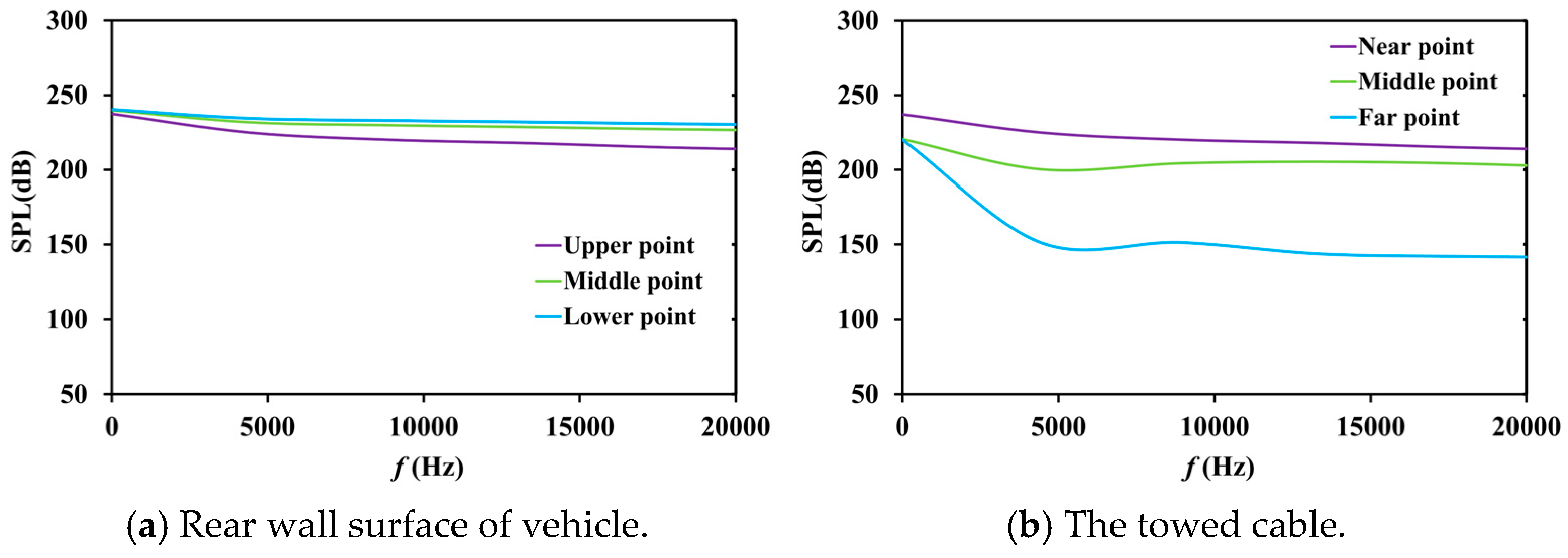
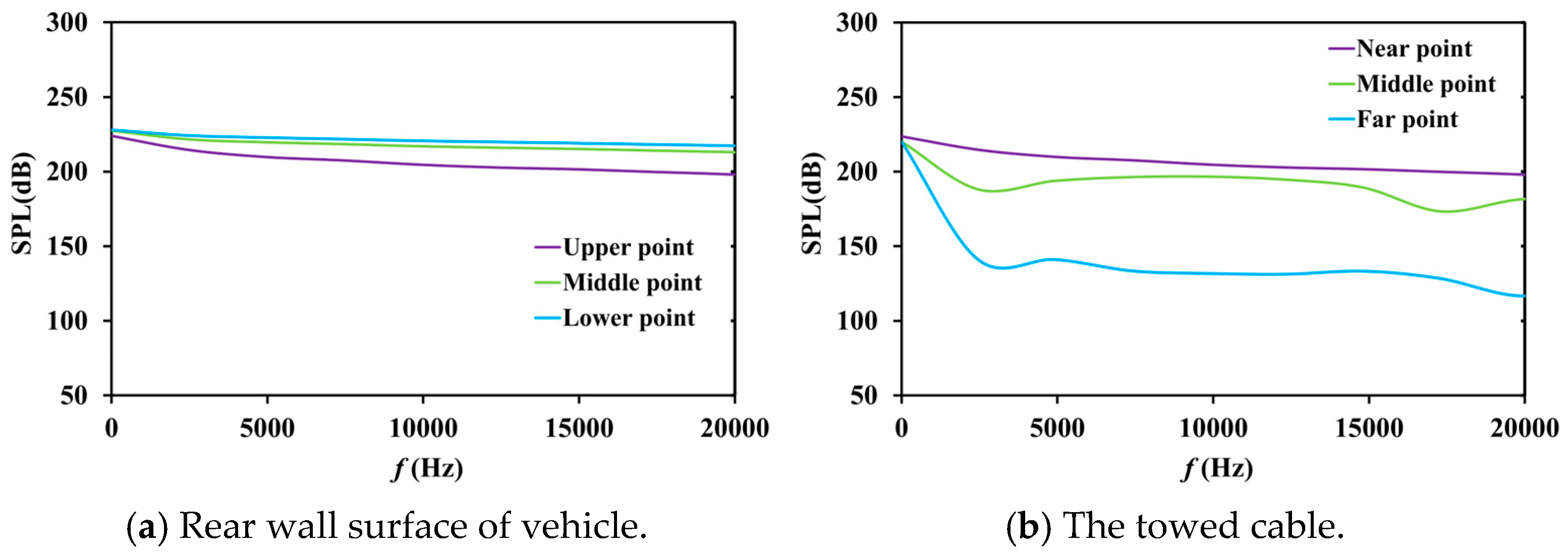
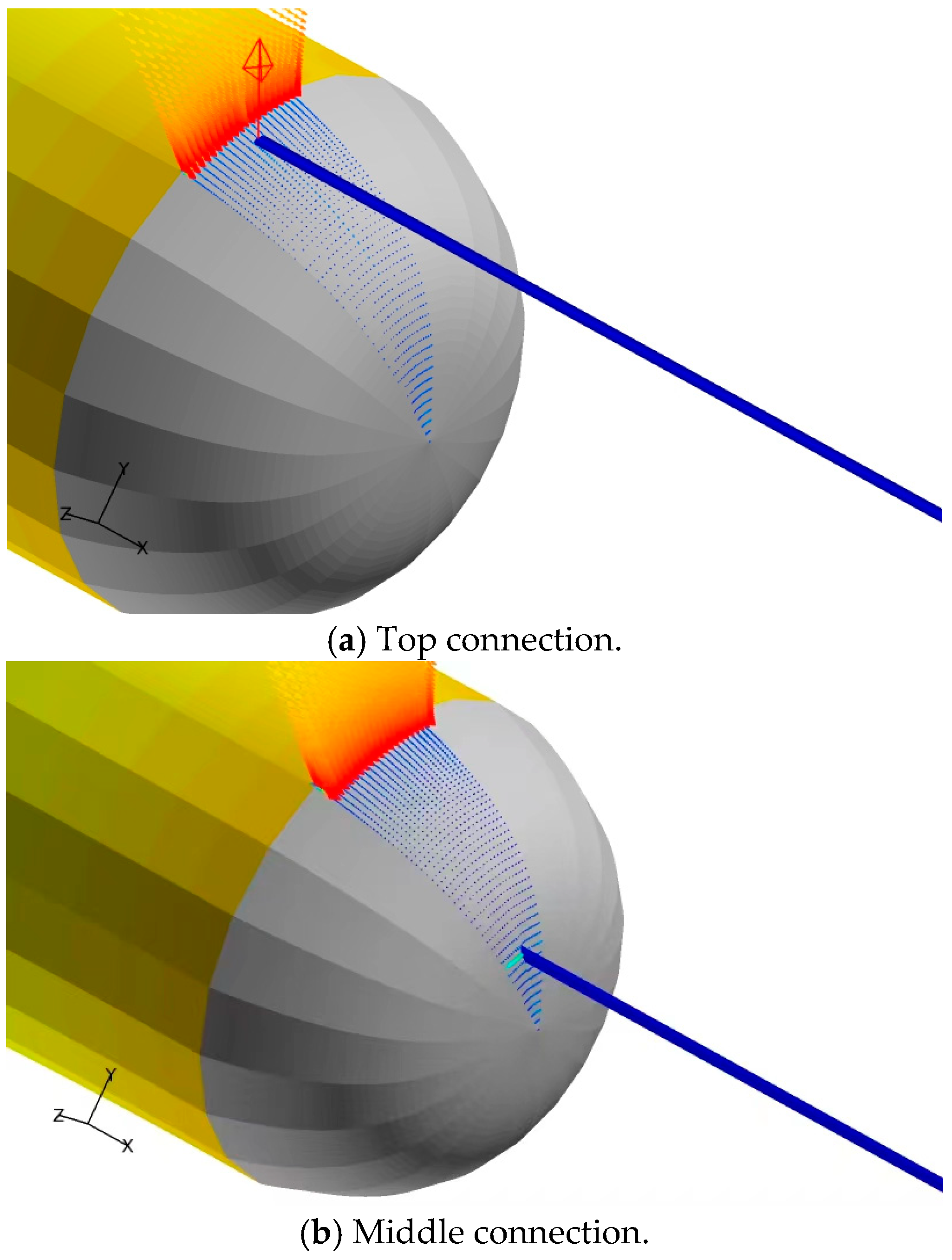
4.2. Models with Different Connection Positions of Towed Cable
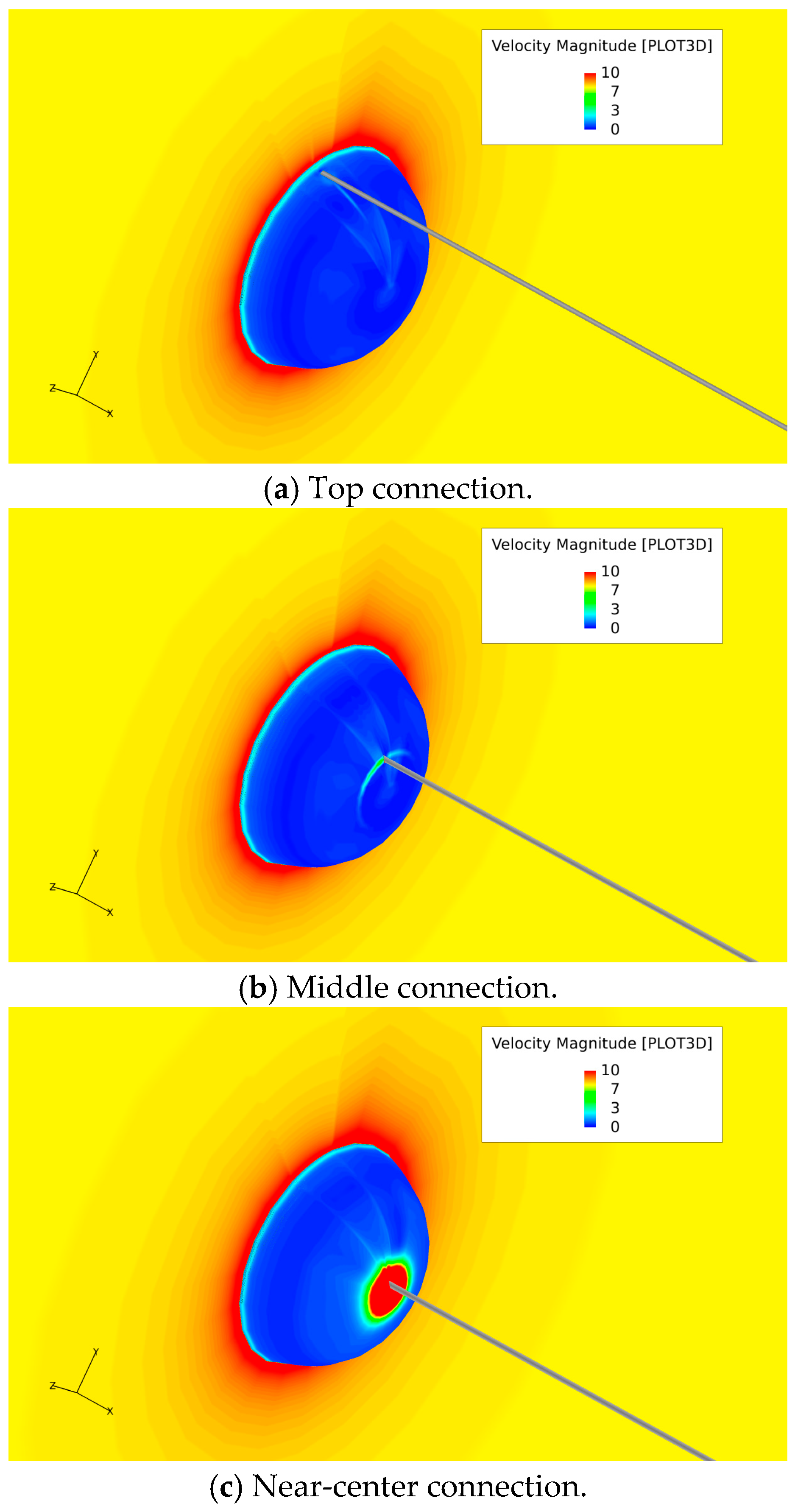
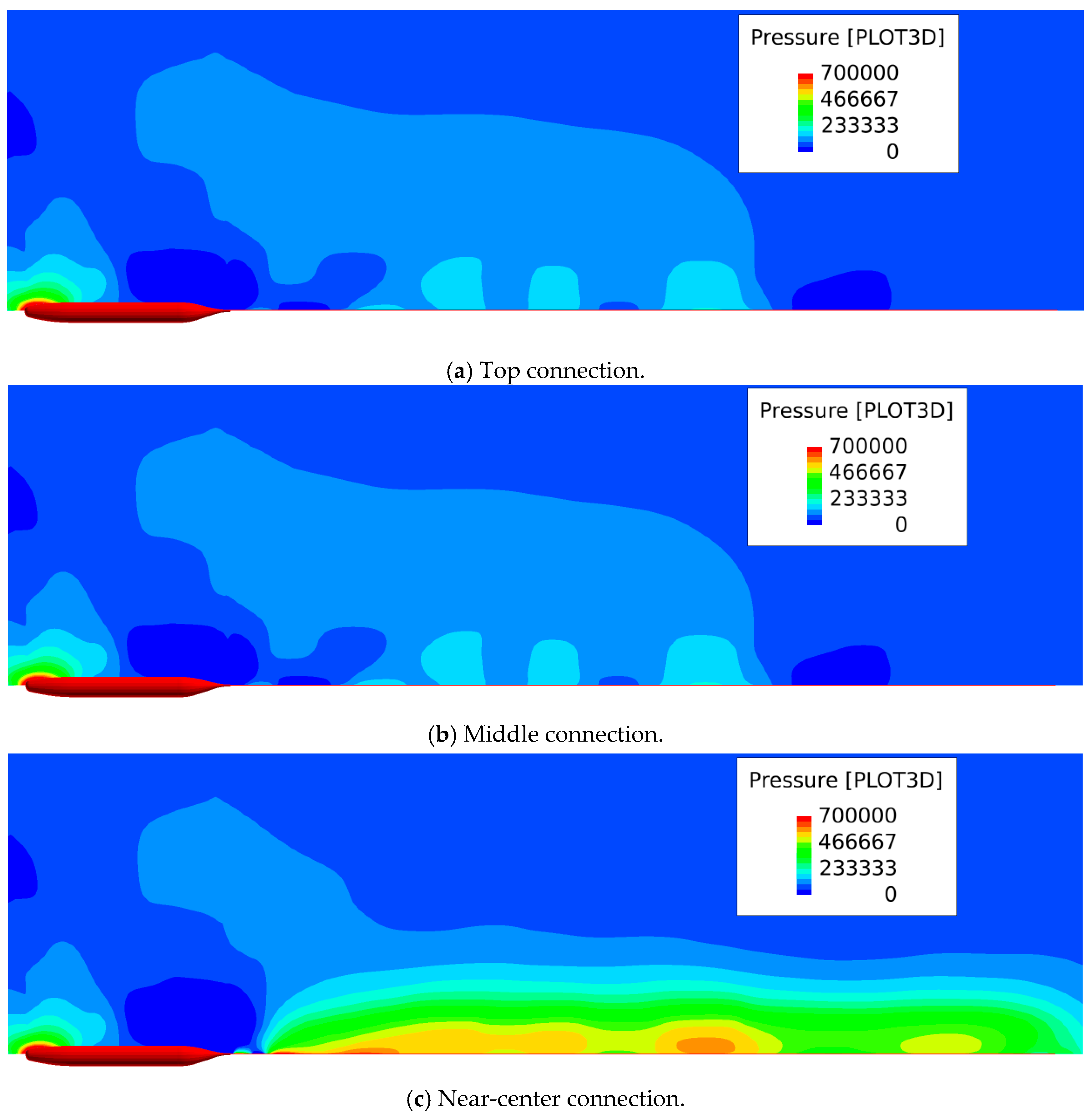
4.2.1. Flow Field Analysis
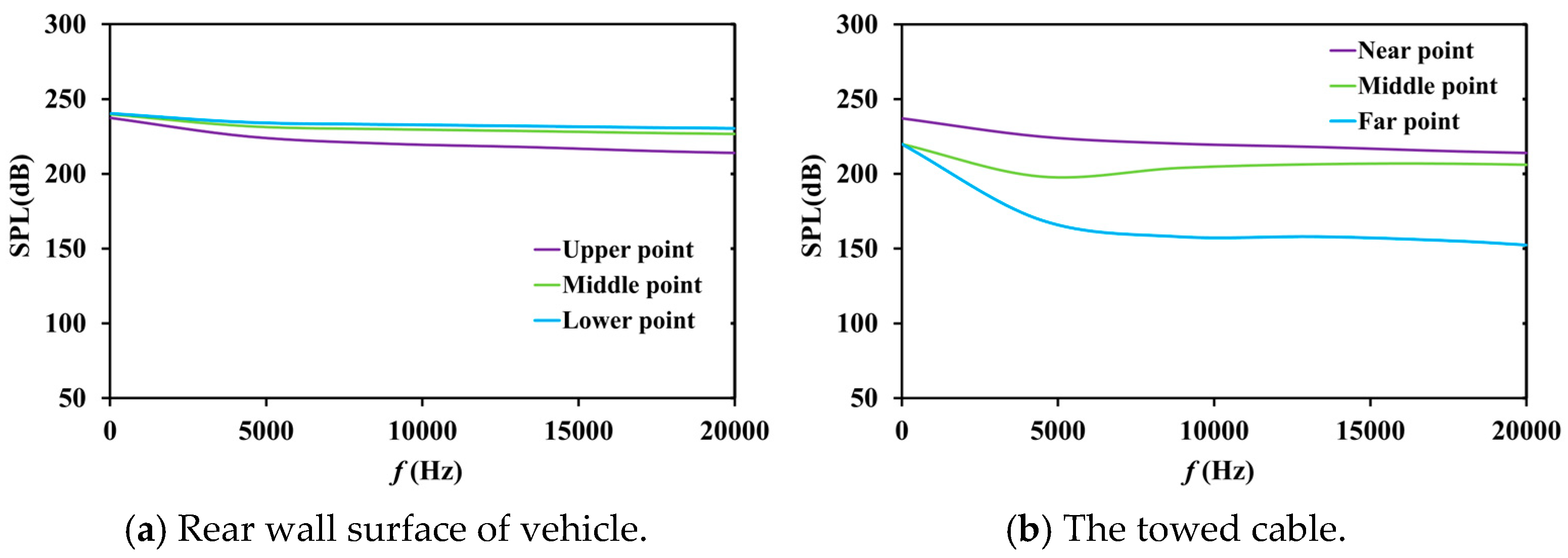
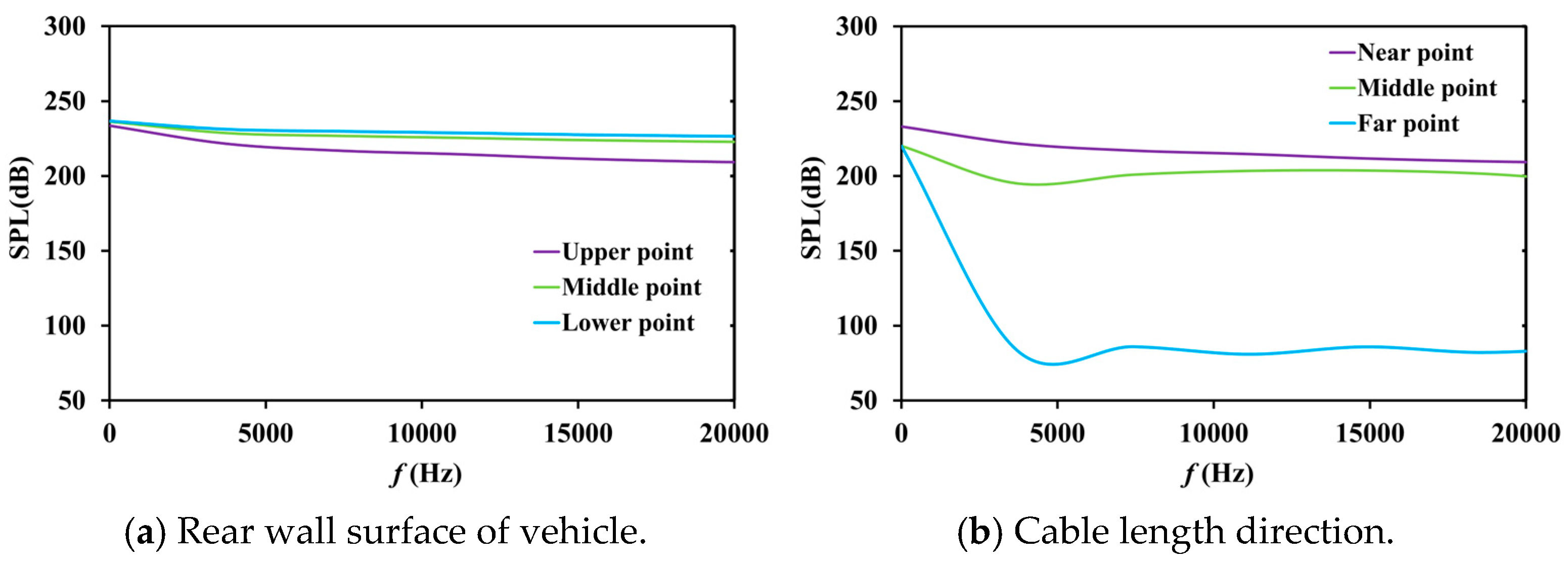
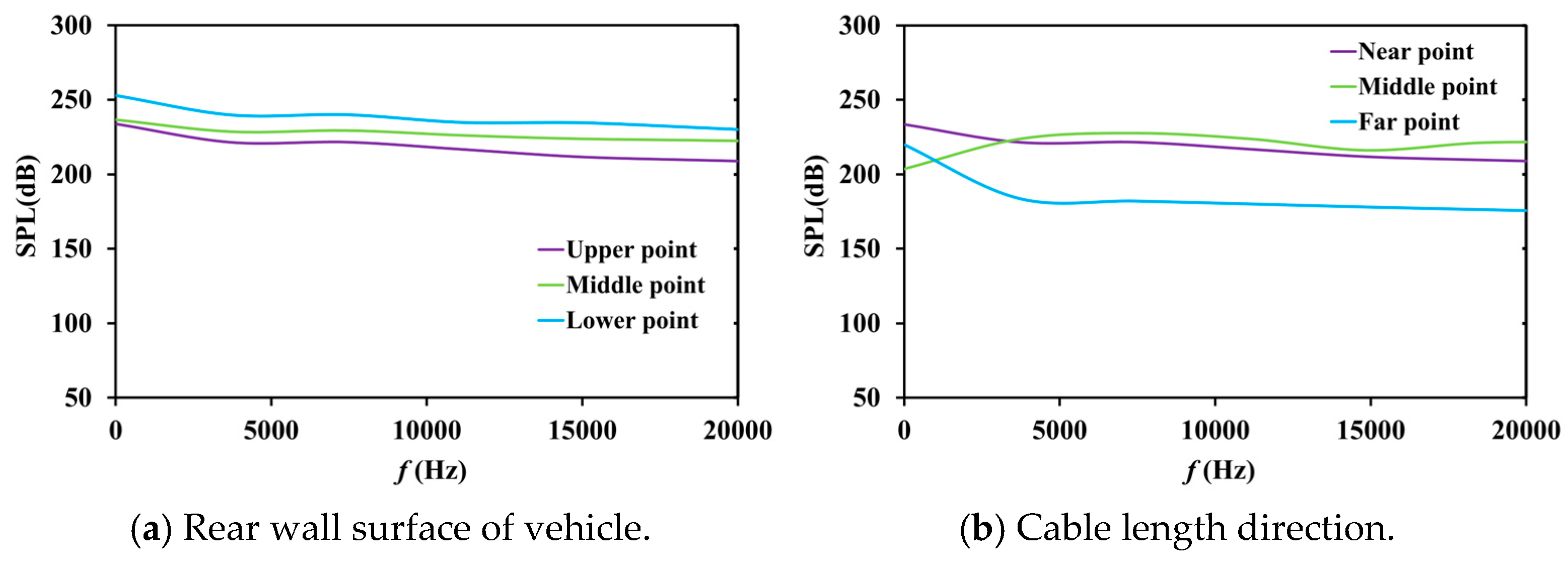
4.2.2. Flow Noise Analysis
5. Conclusions
- (1)
- Unlike traditional IBM approaches limited to low-Re flows, the present developed IBM approach avoids artificial force terms and enables accurate velocity/pressure reconstruction near complex moving boundaries. This is critical for resolving the complex flow field structures and pressure fluctuations for the high-speed towed systems, in which systematic investigation of cable connection positions (top, middle, rear-center) and their impact on transverse flows, pressure pulsations, and flow noise (e.g., 20 dB increase in SPL at rear-center connections). Furthermore, the developed IBM-based approach resolves the slender geometries and adaptive resolution by decoupling grid structure from cable geometry and obtained the pressure fluctuations with noise propagation by the strong fluid-structure coupling framework.
- (2)
- The cable models with different lengths at different freestream velocities have a relatively small impact on the velocity distribution at the rear wall of the system, but there are lateral flow near the cables, which will radiate and affect the velocity distribution around them, thereby affecting the pressure pulsation changes around them and causing significant flow noise problems. Compared to the free stream flow velocity, the impact of cable length variation on flow noise is more significant.
- (3)
- When the towed cable is connected to the towing vehicle from the top to the middle, the cable has little impacts on the velocity distribution in the downstream flow of vehicle, but the impact becomes much significant when the cable is connected near the center of rear surface, where there is a very strong transverse flow around the cable, causing a sharp increase in the velocity near the rear wall.
- (4)
- When connecting the cable system with the towing vehicle, the pressure distribution in the downstream area of the platform is uneven along the cable length direction, indicating significant pressure pulsation around the cable, which in turn generates flow noise. When the cable is connected to the near center position, the uneven pressure distribution intensifies.
- (5)
- When connecting the cable system with the towing vehicle, the sound pressure level at the vehicle rear wall gradually increases from top to bottom (center) along the wall, especially when the connection position is near the center, which cause the sound pressure level near the center to be about 20 dB higher than other connection positions. Along the length direction of the cable, the sound pressure level changes consistently at the top and middle of the connection position, gradually decreasing from the near point to the far one. However, the SPL value at the far point of the middle case is about 75 dB higher than that in the top connecting. In addition, when connected near the center of the rear wall, the SPL value at the middle point is greater than that at the near point in the high-frequency range (about 5–20 kHz) and much greater than at the top and middle connections.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sharif, M.B.; Ghassemi, H.; He, G.; Karimirad, M. A Review of the Flow-Induced Vibrations (FIV) In Marine Circular Cylinder (MCC) Fitted with Various Suppression Devices. Ocean Eng. 2023, 289, 116261. [Google Scholar] [CrossRef]
- Qi, L.; Zou, M.; Lin, C.; Yu, Y.; Liu, S. Directivity and Measurement of Propeller-Shaft-Hull Coupled Acoustic Radiation. Ocean Eng. 2020, 205, 107327. [Google Scholar] [CrossRef]
- Deng, D.; Huang, G.; Lou, L. Numerical Calculation of Towed Array Shape and Attitude. Ocean Eng. 1999, 1, 17–26. (In Chinese) [Google Scholar]
- Zhu, J.; Liu, J.; Deng, Z. Tower Cable Array System Simulations on Turning Manoeuvers. J. Ship Mech. 2003, 7, 33–38. (In Chinese) [Google Scholar]
- Zeng, G.; Zhu, J.; Ge, Y. Predicting the Position and Analyzing the Parameters of a Towed Array During the Maneuvering of a Submarine. J. Harbin Eng. Univ. 2009, 30, 723–727. (In Chinese) [Google Scholar]
- Dong, Y. Study on Single Towed Line Array Shape Estimation and Port/Starboard Rapid Discrimination. Master’s Thesis, Harbin Engineering University, Harbin, China, 2013. (In Chinese). [Google Scholar]
- Beerens, S.P.; IJsselmuide, S.P. Nature and Causes of Flow-Induced Noise on A Sonar with Towed Triplet Array Receiver. NAG Ned. Akoestisch Genoot. J. 2002, 161, 27–37. [Google Scholar]
- Yang, K.; Yang, Q.; Xiao, P.; Li, X.; Duan, R.; Ma, Y. Flow Noise Calculation and Experimental Study for Hydrophones in Fluid-Filled Towed Arrays. Acoust. Aust. 2017, 45, 313–324. [Google Scholar] [CrossRef]
- Howard, B.E. Calculation of The Shape of a Towed Underwater Acoustic Array. IEEE J. Ocean. Eng. 1992, 17, 193–2013. [Google Scholar] [CrossRef]
- Ablow, C.M.; Schechter, S. Numerical Simulation of Undersea Cable Dynamics. Ocean Eng. 1983, 10, 443–457. [Google Scholar] [CrossRef]
- Ma, Y.; Luan, Y.; Xu, W. Hydrodynamic Features of Three Equally Spaced, Long Flexible Cylinders Undergoing Flow-Induced Vibration. Eur. J. Mech.-B/Fluids 2020, 79, 386–400. [Google Scholar] [CrossRef]
- Huera-Huarte, F.J.; Bangash, Z.A.; González, L.M. Towing Tank Experiments on The Vortex-Induced Vibrations of Low Mass Ratio Long Flexible Cylinders. J. Fluids Struct. 2014, 48, 81–92. [Google Scholar] [CrossRef]
- Wang, F.; Ding, W.; Deng, D.; Wu, X. Motion Modeling and Numerical Simulation Study of Underwater Multi-Cable Multi-Body Towed System. J. Shanghai JiaoTong Univ. 2020, 54, 441–450. [Google Scholar]
- Wu, J.; Yang, X.; Xu, S.; Han, X. Numerical Investigation on Underwater Towed System Dynamics Using a Novel Hydrodynamic Model. Ocean Eng. 2022, 247, 110632. [Google Scholar] [CrossRef]
- Peskin, C.S. Flow Patterns Around Heart Valves: A Numerical Method. J. Comput. Phys. 1972, 10, 252–271. [Google Scholar] [CrossRef]
- Yang, F.; Gu, X.; Xia, X.; Zhang, Q. A Peridynamics-Immersed Boundary-Lattice Boltzmann Method for Fluid-Structure Interaction Analysis. Ocean Eng. 2022, 264, 112528. [Google Scholar] [CrossRef]
- Cai, Y. Study on the Numerical Modeling Methods for Underwater Fluid-Structure-Acoustic Wave Interaction Problems Based on Lattice Boltzmann Method. Ph.D. Thesis, Dalian University of Technology, Dalian, China, 2019. (In Chinese). [Google Scholar]
- Miyoshi, T.; Ishii, T.; Hashimoto, A.; Mori, K.; Nakamura, Y. Computational Analysis of Parachute Motion Using the Immersed Boundary Method. In Proceedings of the 46th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 7–10 January 2008. [Google Scholar]
- Miyoshi, M.; Mori, K.; Nakamura, Y. Numerical Simulation of Parachute Inflation Process by IB Method. J. Jpn. Soc. Aeronaut. Space Sci. 2009, 5, 419–425. (In Japanese) [Google Scholar]
- Xue, X.P.; Koyama, H.; Nakamura, Y.; Wen, C.Y. Effects of Suspension Line on Flow Field Around a Supersonic Parachute. Aerosp. Sci. Technol. 2015, 43, 63–77. [Google Scholar] [CrossRef]
- Xue, X.P.; Nakamura, Y.; Mori, K.; Wen, C.Y.; Jia, H. Numerical Investigation of Effects of Angle-Of-Attack on A Parachute-Like Two-Body System. Aerosp. Sci. Technol. 2017, 69, 370–386. [Google Scholar] [CrossRef]
- Xue, X.P.; Nakamura, Y. Numerical Simulation of a Three-Dimensional Flexible Parachute System Under Supersonic Conditions. Trans. JSASS Aerosp. Technol. Jpn. 2013, 11, 99–108. [Google Scholar] [CrossRef]
- Jia, H.; Bao, W.; Rong, W.; Yu, L.; Xue, X.; Fan, J. Numerical Study on Aerodynamic Characteristics of Parachute Models for Future Jupiter Exploration. Space Sci. Technol. 2024, 4, 0116. [Google Scholar] [CrossRef]
- Zhang, N. Research on Mechanism and Hybrid Computation Approach for Cavity Flow and Flow Induced Noise. Ph.D. Thesis, China Ship Scientific Research Center, Wuxi, China, 2010. (In Chinese). [Google Scholar]
- Ochi, A.; Nakamura, Y. A Development of Aerodynamics Analysis Tool Using Cartesian System (First Report). In Proceedings of the Japan Society of Fluid Mechanics 19th CFD Symposium, Tokyo, Japan, 13–15 December 2005. (In Japanese). [Google Scholar]
- Sengupta, A. Fluid Structure Interaction of Parachutes in Supersonic Planetary Entry. In Proceedings of the 21st AIAA Aerodynamic Decelerator Systems Technology Conference and Seminar, Dublin, Ireland, 23–26 May 2011. [Google Scholar]
- Sengupta, A.; Steltzner, A.; Witkowski, A.; Candler, G.; Pantano, C. Findings from the Supersonic Qualification Program of the Mars Science Laboratory Parachute System. In Proceedings of the 20th AIAA Aerodynamic Decelerator Systems Technology Conference and Seminar, Seattle, DC, USA, 4–7 May 2009. [Google Scholar]
- Sengupta, A.; Kelsch, R.; Roeder, J.; Wernet, M.; Witkowski, A.; Kandis, M. Supersonic Performance of Disk-Gap-Band Parachutes Constrained to A 0-Degree Trim Angle. J. Spacecr. Rocket. 2009, 46, 1155–1163. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, X.; Yu, Y.; Zhao, D.; Yang, D.; Qi, L. Numerical Investigations of a Column Configuration with Towed Super Long Cable in Underwater Environment. J. Mar. Sci. Eng. 2025, 13, 592. https://doi.org/10.3390/jmse13030592
Xue X, Yu Y, Zhao D, Yang D, Qi L. Numerical Investigations of a Column Configuration with Towed Super Long Cable in Underwater Environment. Journal of Marine Science and Engineering. 2025; 13(3):592. https://doi.org/10.3390/jmse13030592
Chicago/Turabian StyleXue, Xiaopeng, Yue Yu, Danjun Zhao, Degui Yang, and Libo Qi. 2025. "Numerical Investigations of a Column Configuration with Towed Super Long Cable in Underwater Environment" Journal of Marine Science and Engineering 13, no. 3: 592. https://doi.org/10.3390/jmse13030592
APA StyleXue, X., Yu, Y., Zhao, D., Yang, D., & Qi, L. (2025). Numerical Investigations of a Column Configuration with Towed Super Long Cable in Underwater Environment. Journal of Marine Science and Engineering, 13(3), 592. https://doi.org/10.3390/jmse13030592






