Study on the Effects of Internal Building Layouts on Tsunami-Driven Single-Container Motion
Abstract
:1. Introduction
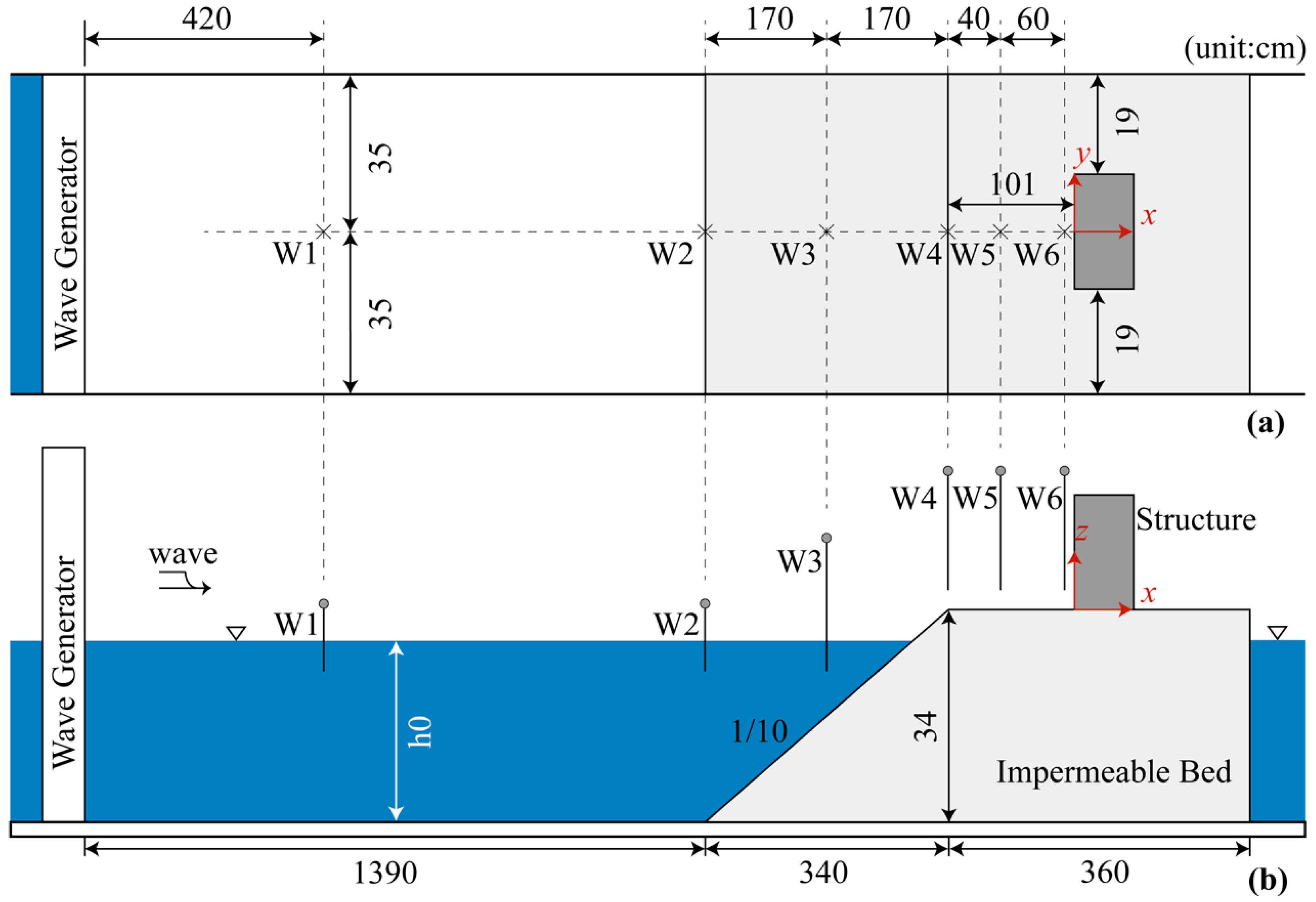
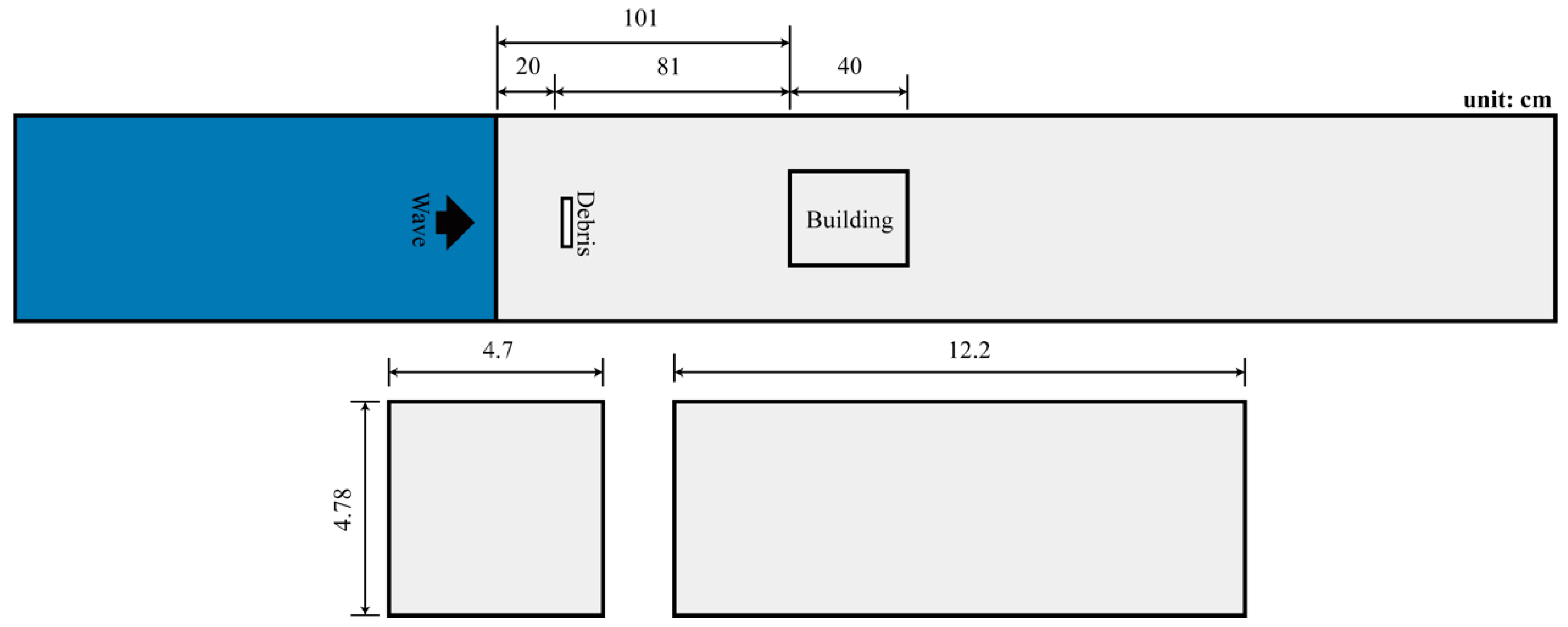
2. Experimental Setup
3. Numerical Model: FS3M
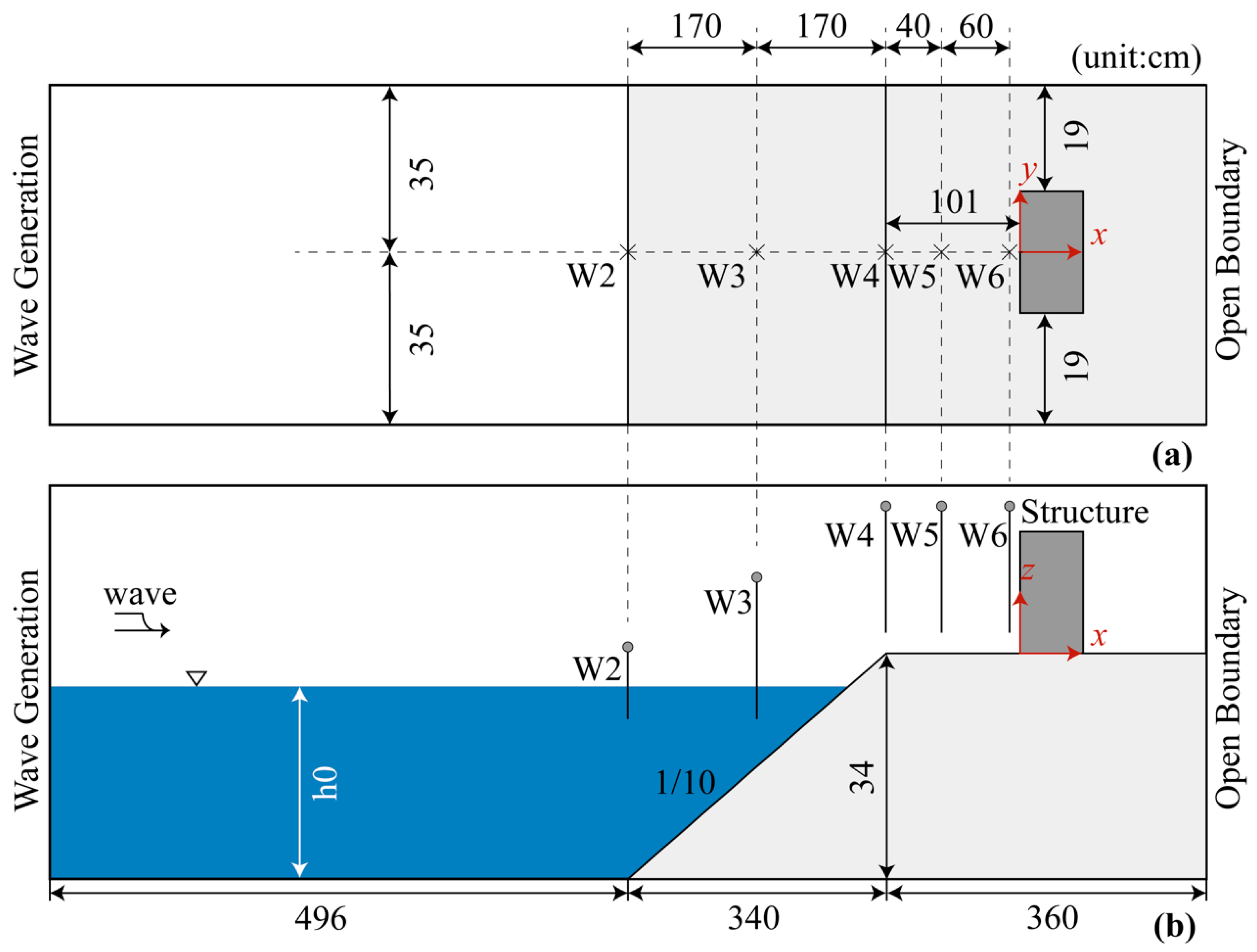
3.1. Numerical Model Setup
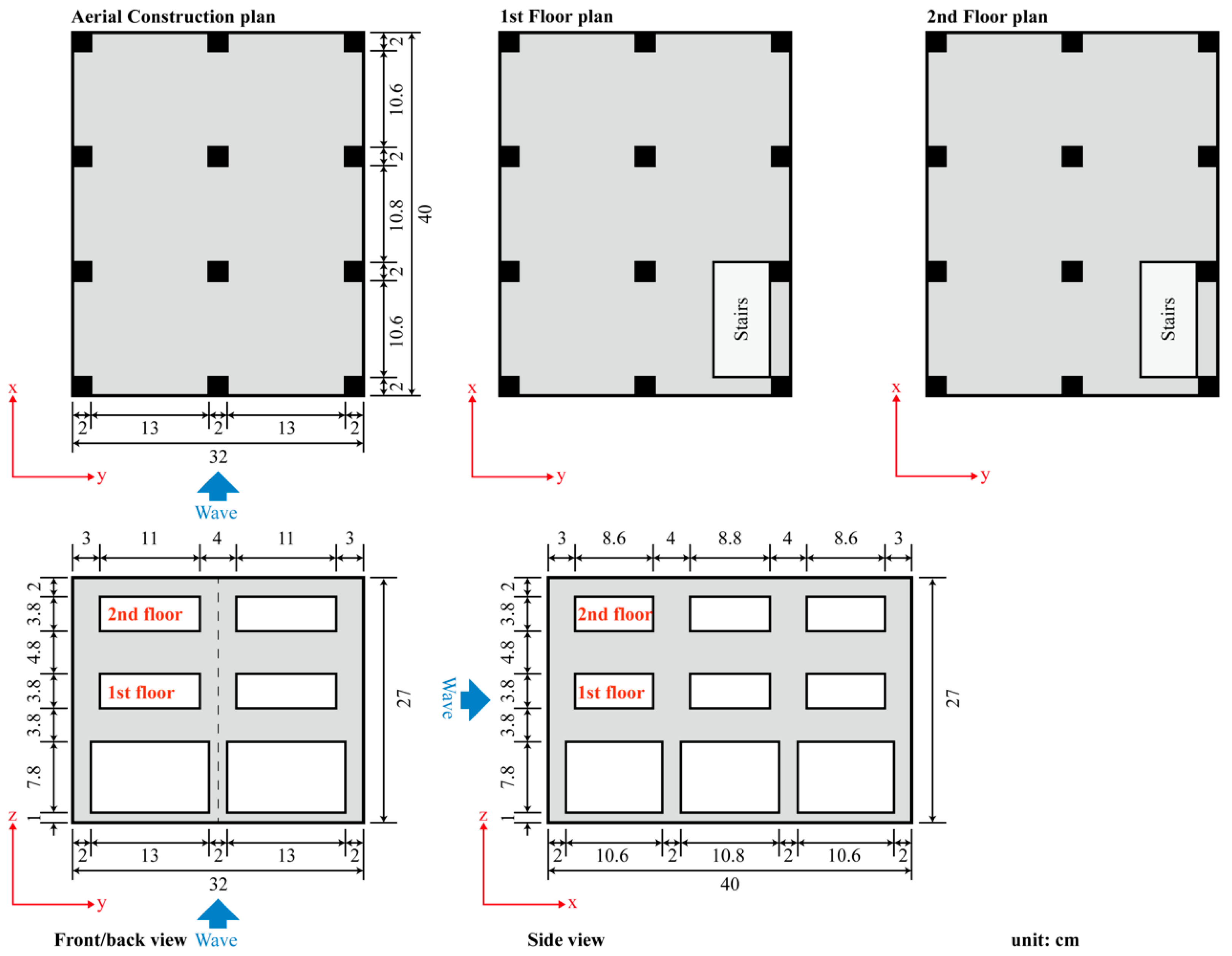
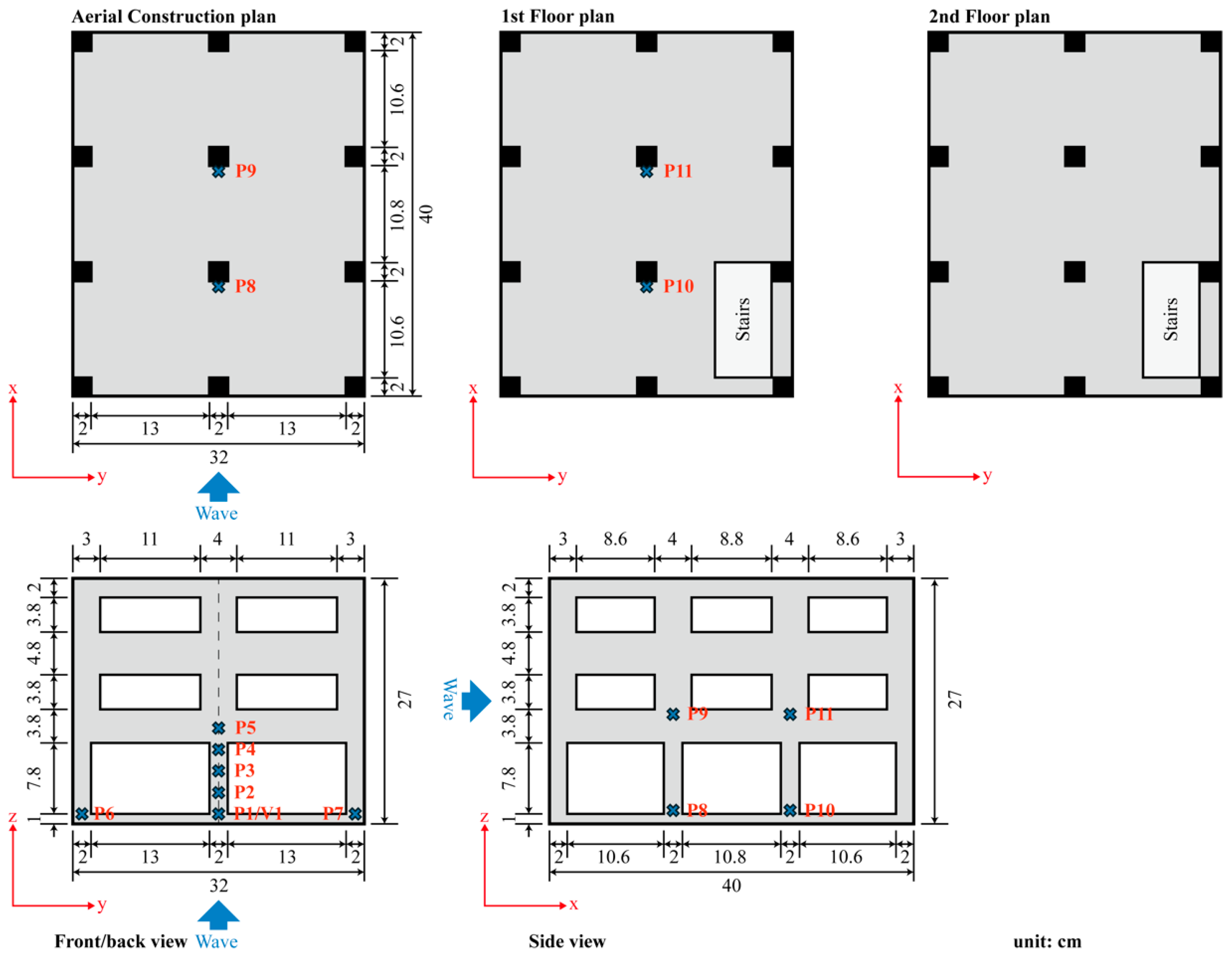
3.2. Building Model and Debris
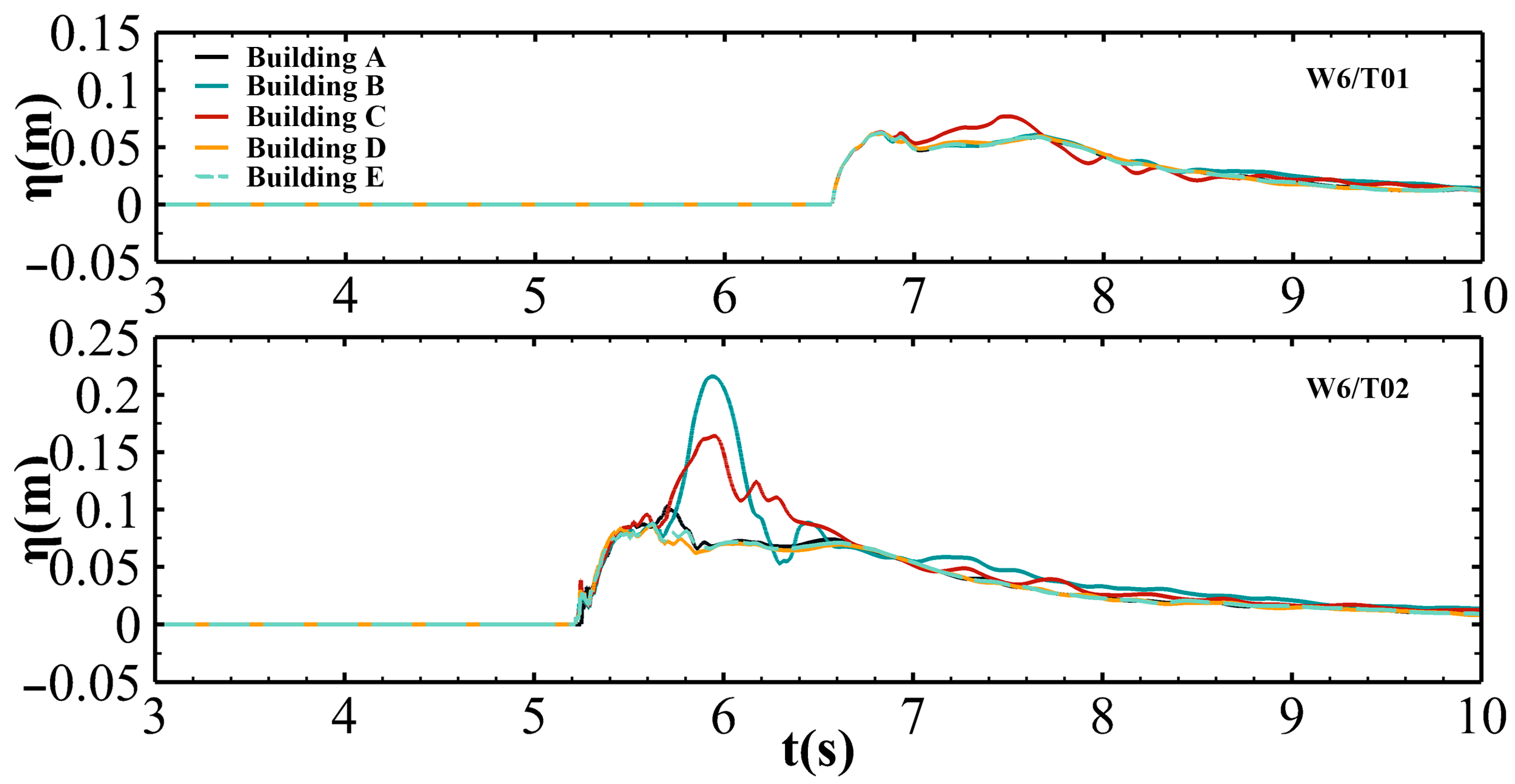
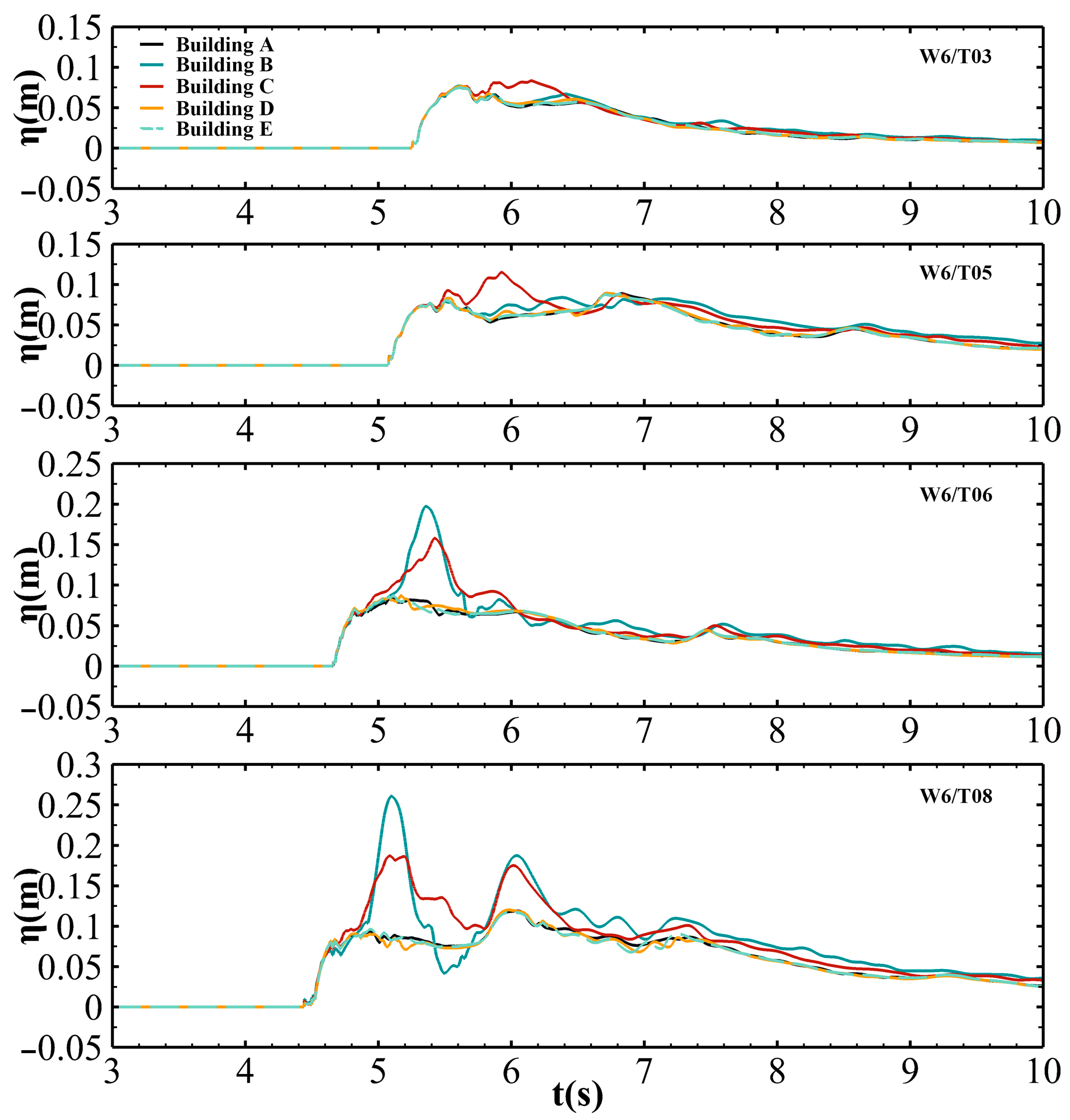
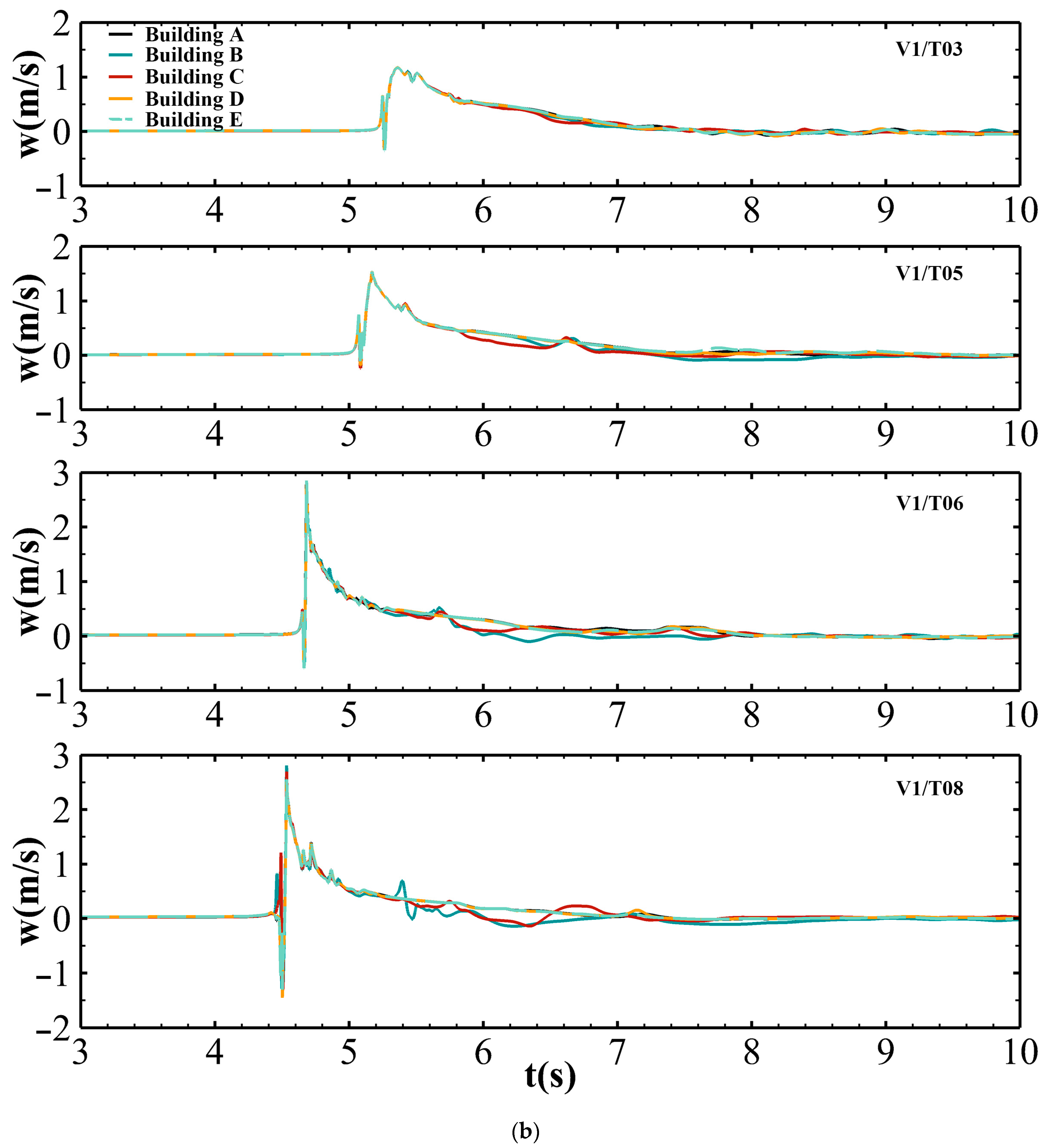
3.3. Validation of Numerical Models
3.4. Numerical Simulation Wave Conditions
4. Simulation Results
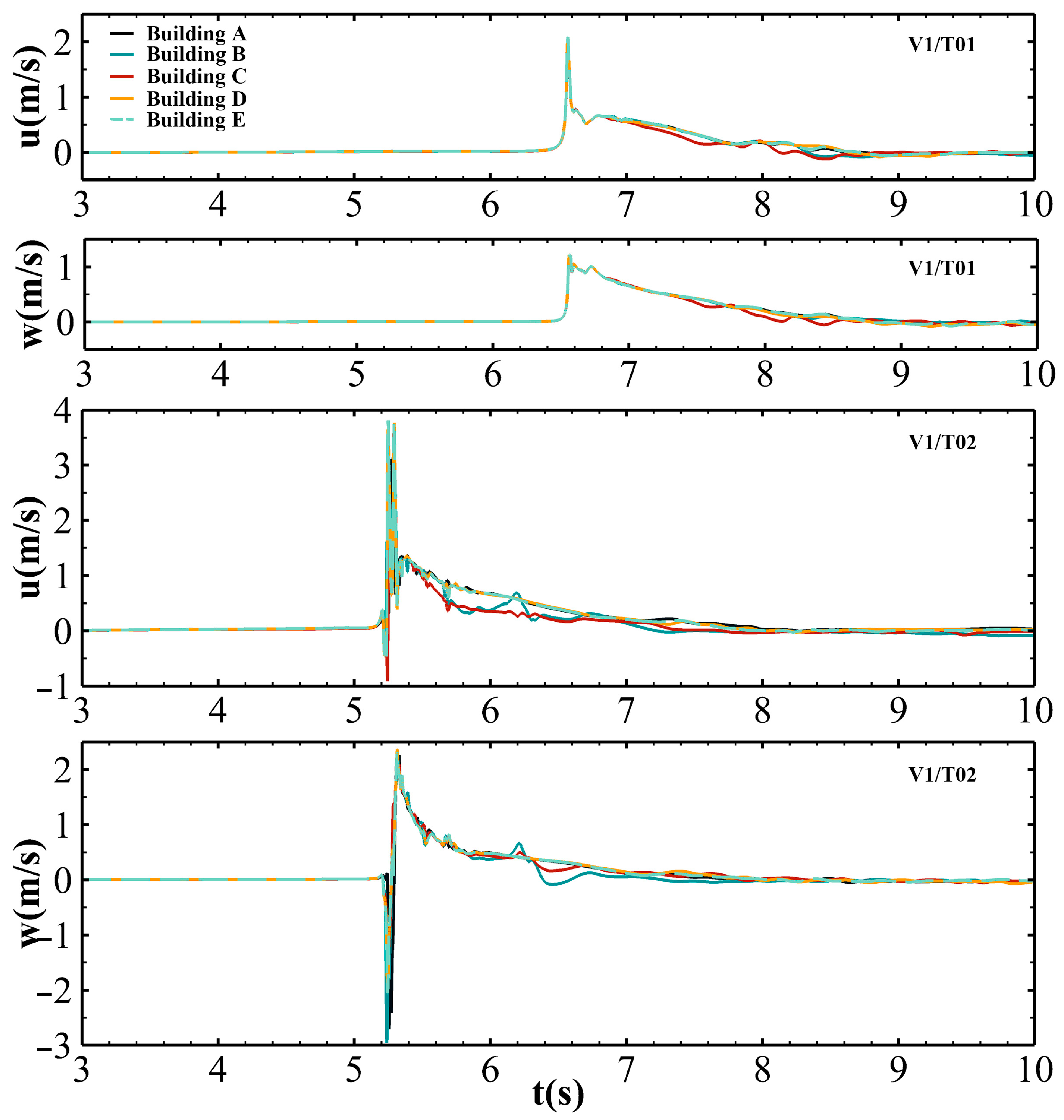
4.1. Solitary Wave (T01 and T02)
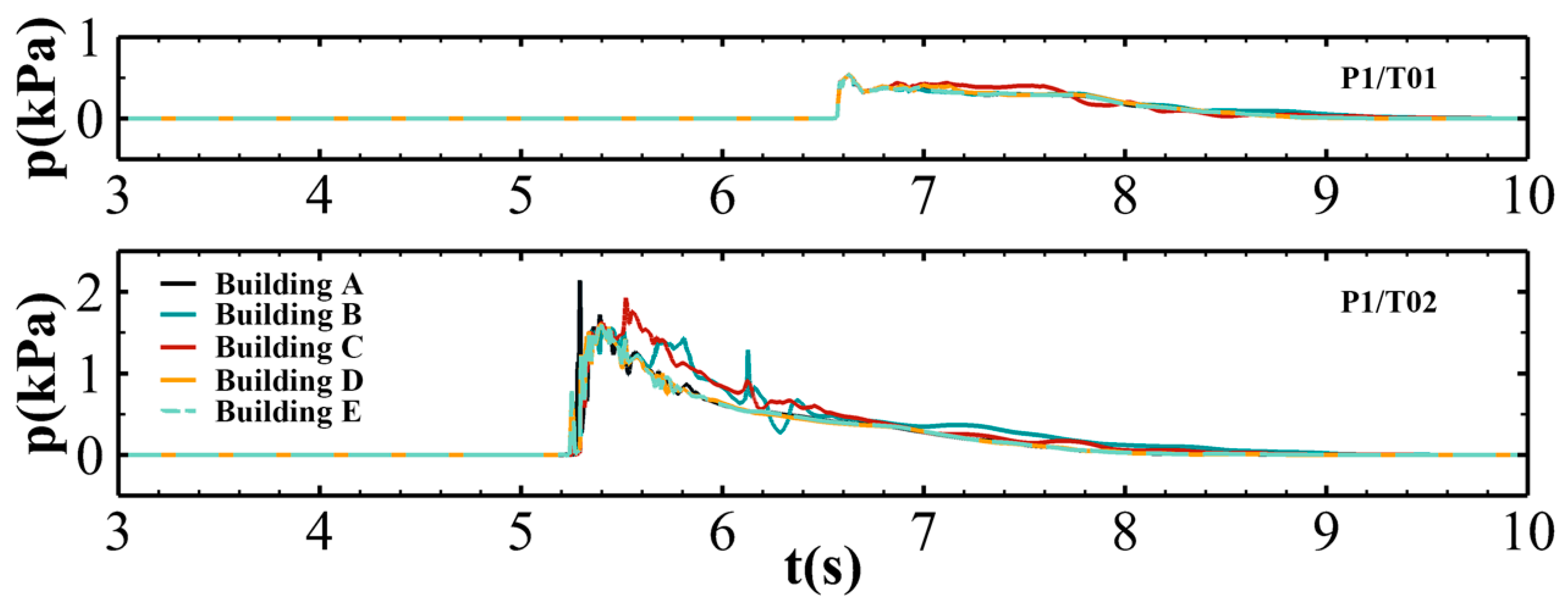
4.2. Instantaneous Pressure Distribution
5. Discussion
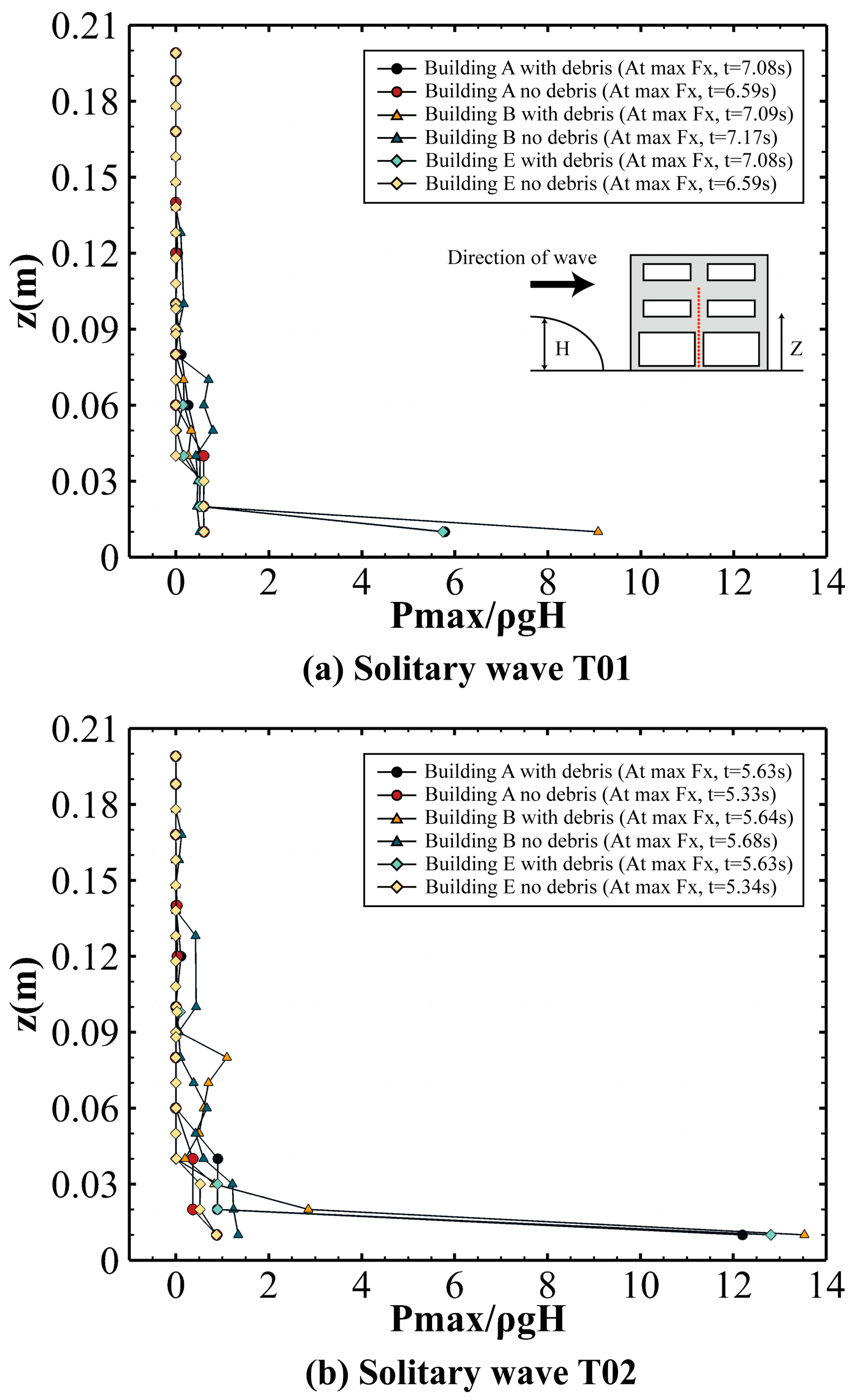
5.1. Maximum Normalized Pressure Distribution on Building
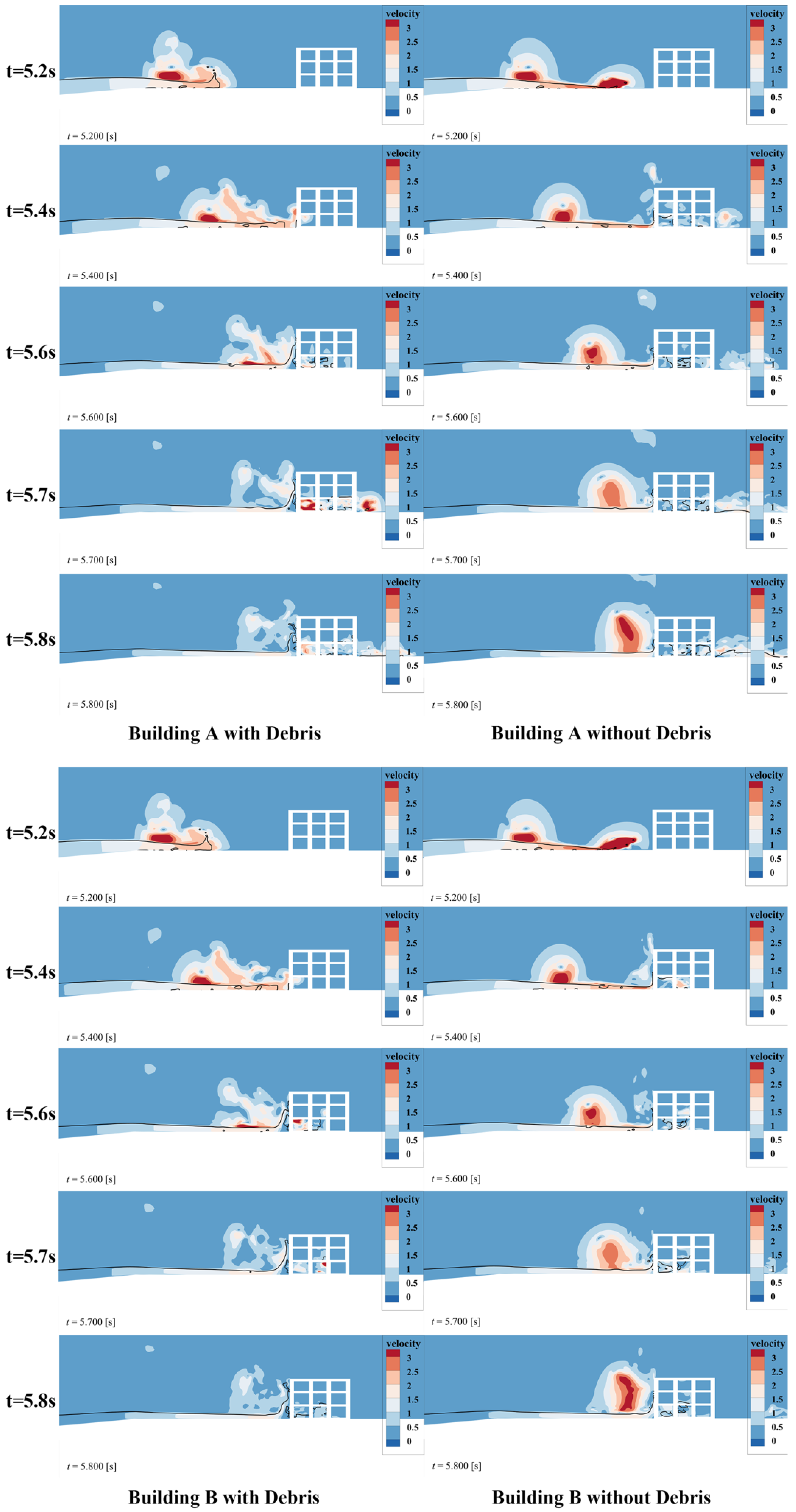
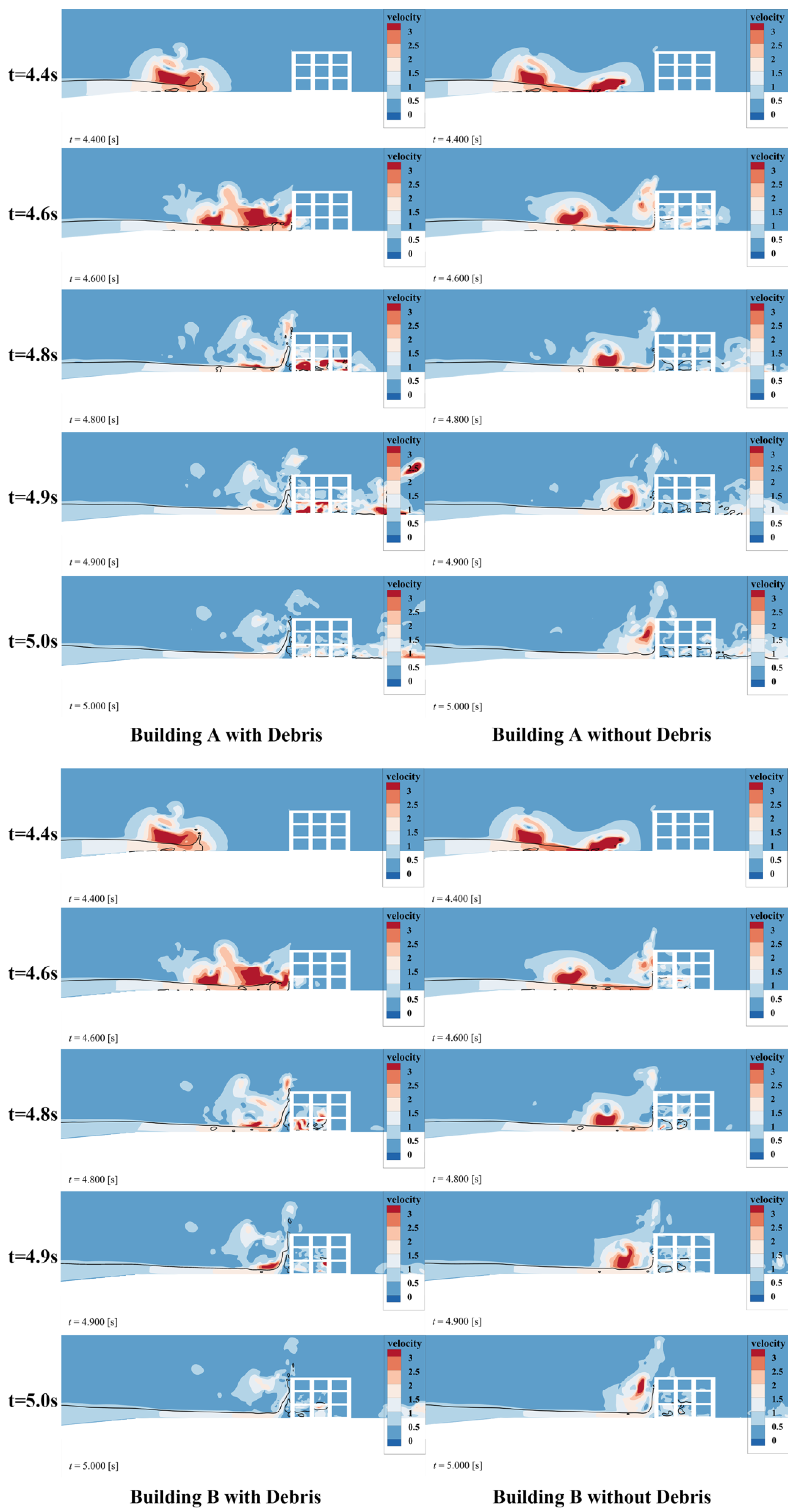
5.2. Instantaneous Resultant Velocity Distribution on Different Building Models
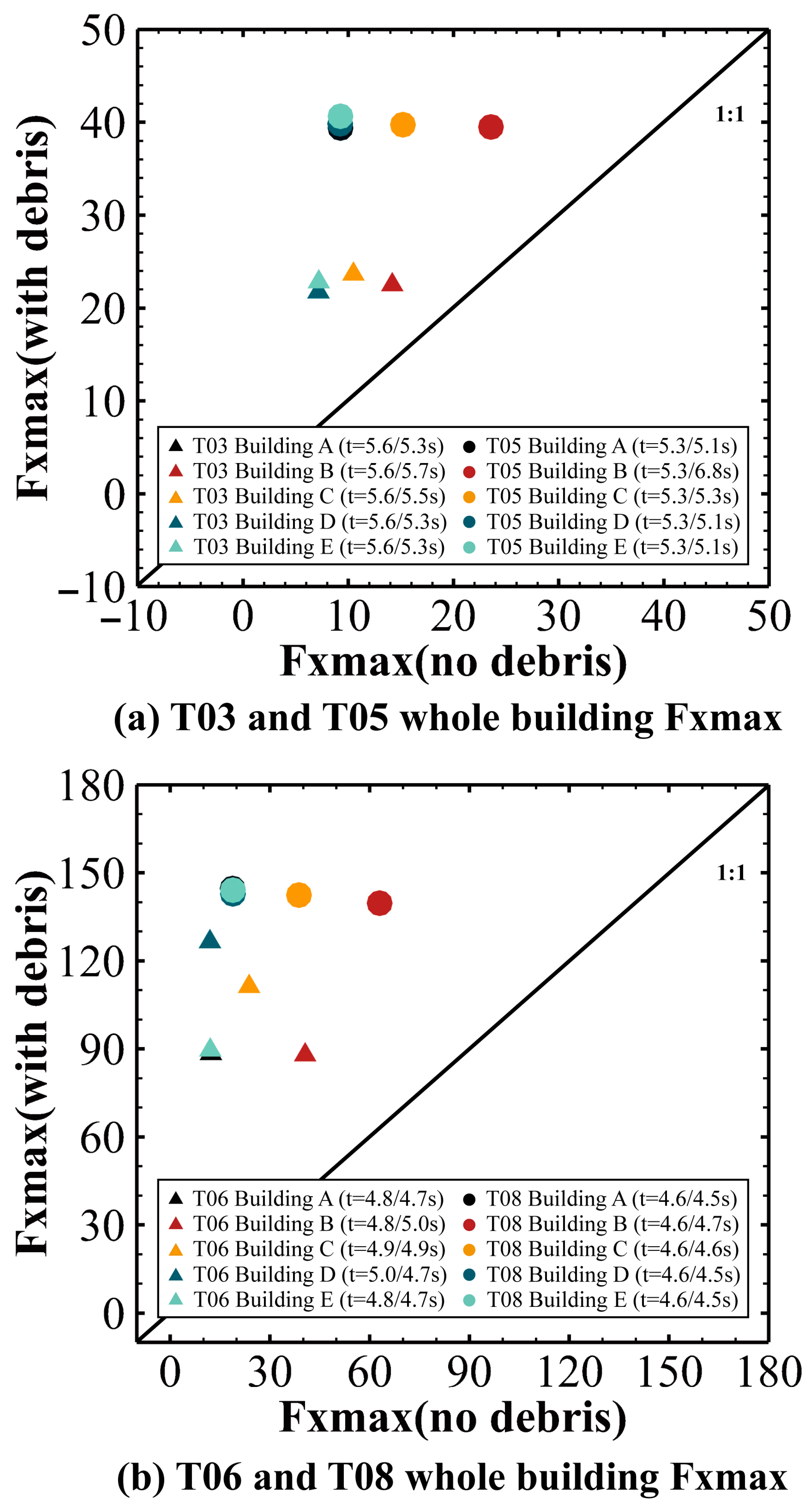
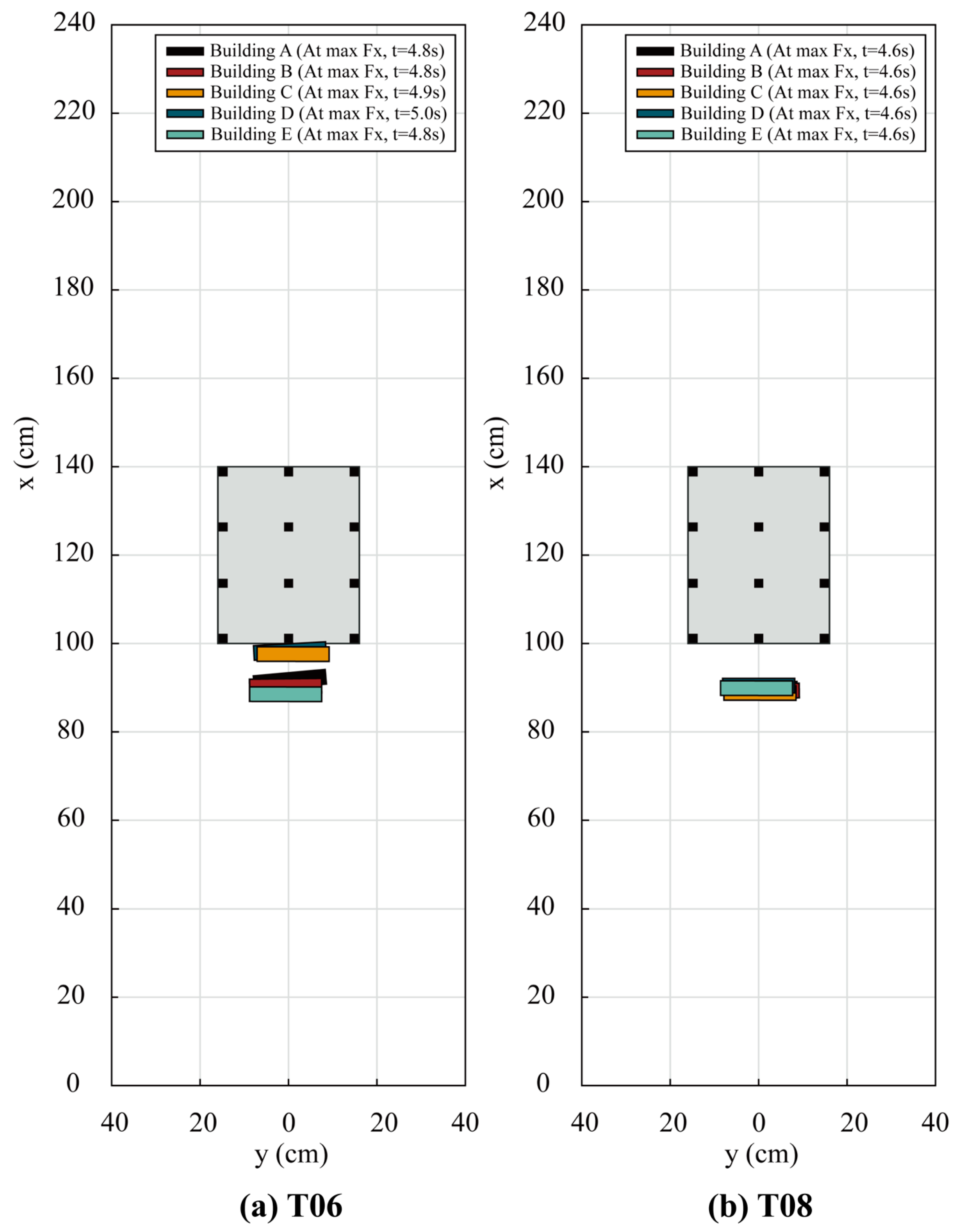
5.3. Effect Elements on Maximum Force Fxmax
6. Summary and Conclusions
- Larger Incident Waves and Fx Dependence on Wave Height
- 2.
- Debris Influence on Fxmax and Pressure Distribution
- 3.
- Wave Period and Force Modulation
- 4.
- Architectural Features and Flow Dynamics
- 5.
- Correlation Between Building Type and Fxmax in Debris-Free Scenarios
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Goff, J.R.; Dominey-Howes, D. Tsunami risk management in the aftermath of the 2004 Indian Ocean tsunami: Lessons learned. Nat. Hazards 2009, 52, 381–393. [Google Scholar]
- FEMA. Guidelines for Design of Structures for Vertical Evacuation from Tsunamis; Federal Emergency Management Agency: Washington, DC, USA, 2012.
- Mori, N.; Takahashi, T.; Yasuda, T.; Yanagisawa, H. Survey of 2011 Tohoku earthquake tsunami inundation and run-up. Geophys. Res. Lett. 2011, 38, L00G14. [Google Scholar] [CrossRef]
- Suppasri, A.; Koshimura, S.; Imai, K.; Mas, E.; Gokon, H.; Muhari, A.; Imamura, F. Damage characteristic and field survey of the 2011 Great East Japan tsunami in Miyagi prefecture. Coast. Eng. J. 2021, 54, 1250005-1–1250005-30. [Google Scholar] [CrossRef]
- Yeh, H.; Sato, S.; Tajima, Y. The 11 March 2011 East Japan earthquake and tsunami: Tsunami effects on coastal infrastructure and buildings. Pure Appl. Geophys. 2012, 170, 1019–1031. [Google Scholar] [CrossRef]
- Kennedy, A.; Rogers, S.; Sallenger, A.; Gravois, U.; Zachry, B.; Dosa, M.; Zarama, F. Building destruction from waves and surge on the Bolivar Peninsula during Hurricane Ike. J. Waterw. Port Coast. Ocean Eng. 2011, 137, 132–141. [Google Scholar] [CrossRef]
- Tomiczek, T.; Kennedy, A.; Rogers, S. Collapse limit state fragilities of wood-framed residences from storm surge and waves during Hurricane Ike. J. Waterw. Port Coast. Ocean Eng. 2014, 140, 43–55. [Google Scholar] [CrossRef]
- Xian, S.; Lin, N.; Hatzikyriakou, A. Storm surge damage to residential areas: A quantitative analysis for Hurricane Sandy in comparison with FEMA Flood Map. Nat. Hazards 2015, 79, 1867–1888. [Google Scholar] [CrossRef]
- Hatzikyriakou, A.; Lin, N.; Gong, J.; Xian, S.; Hu, X.; Kennedy, A. Component-based vulnerability analysis for residential structures subjected to storm surge impact from Hurricane Sandy. Nat. Hazards Rev. 2016, 17, 05015005. [Google Scholar] [CrossRef]
- Hatzikyriakou, A.; Lin, N. Assessing the vulnerability of structures and residential communities to storm surge: An analysis of flood impact during Hurricane Sandy. Front. Built Environ. 2018, 4, 4. [Google Scholar] [CrossRef]
- Mizutani, N.; Aoki, S.; Manawasekara, C.; Nakamura, T. Effect of space configuration of building on Tsunami Force on it. In Proceedings of the 34th Conference on Coastal Engineering, Seoul, Republic of Korea, 15–20 June 2014. [Google Scholar]
- Shafiei, S.; Melville, B.W.; Shamseldin, A.Y. Experimental investigation of tsunami bore impact force and pressure on a square prism. Coast. Eng. 2016, 110, 1–16. [Google Scholar] [CrossRef]
- Wüthrich, D.; Pfister, M.; Nistor, I.; Schleiss, A.J. Experimental study on the hydrodynamic impact of tsunami-like waves against impervious free-standing buildings. Coast. Eng. J. 2018, 60, 180–199. [Google Scholar] [CrossRef]
- Thusyanthan, N.I.; Madabhushi, S.P.G. Tsunami wave loading on coastal houses: A model approach. Proc. Inst. Civ. Eng.-Civ. Eng. 2008, 161, 77–86. [Google Scholar] [CrossRef]
- Wilson, J.S.; Gupta, R.; van de Lindt, J.W.; Clauson, M.; Garcia, R. Behavior of a one-sixth scale wood-framed residential structure under wave loading. J. Perform. Constr. Facil. 2009, 23, 336–345. [Google Scholar] [CrossRef]
- Moon, W.C.; Puay, H.T.; Lau, T.L. Investigations on the effect of roof types on wave impinging on a building: A hybrid experimental-numerical approach. Coast. Eng. 2021, 164, 103836. [Google Scholar] [CrossRef]
- Moon, W.C.; Lau, T.L.; Puay, H.T. Experimental investigations of tsunami loading on internal wall of a building with various openings and wall configurations. Coast. Eng. 2020, 158, 103691. [Google Scholar] [CrossRef]
- Naito, C.; Cercone, C.; Riggs, H.R.; Cox, D. Procedure for site assessment of the potential for Tsunami Debris Impact. J. Waterw. Port Coast. Ocean Eng. 2014, 140, 223–232. [Google Scholar] [CrossRef]
- Yeh, H.; Barbosa, A.; Mason, B.H. Tsunamis effects in man-made environment. In Complexity in Tsunamis, Volcanoes, and Their Hazards; Springer: New York, NY, USA, 2021; pp. 187–211. [Google Scholar]
- Ayca, A.; Lynett, P.J. Modeling the motion of large vessels due to tsunami-induced currents. Ocean Eng. 2021, 236, 109487. [Google Scholar] [CrossRef]
- Lee, K.; Aoki, S.; Mizutani, N.; Ashizawa, S.; Hirakawa, S. A study on tsunami force acting on buildings and the effects of buildings with openings on tsunami loads. J. Jpn. Soc. Civ. Eng. 2012, 68, I_234–I_239. [Google Scholar]
- Abbe, T.B.; David, R.M. Patterns and Processes of Wood Debris Accumulation in the Queets River Basin, Washington. Geomorphology 2003, 51, 81–107. [Google Scholar] [CrossRef]
- Burke, J. Structural Testing of a 1:6 Scaled, Light-Frame Construction, Near-Coastal Residential Structure. Master’s Thesis, Oregon State University, Corvallis, OR, USA, 2018. [Google Scholar]
- FEMA. Determining site-specific loads. In Coastal Construction Manual; FEMA: Washington, DC, USA, 2011; pp. 5–27. [Google Scholar]
- Nakamura, T.; Mizutani, N. Development of fluid-sediment-seabed interaction model and its application. In Proceedings of the 34th Conference on Coastal Engineering, Seoul, Republic of Korea, 15–20 June 2014. [Google Scholar]
- Kunugi, T. MARS for multiphase calculation. Comput. Fluid Dyn. J. 2001, 9, 563–571. [Google Scholar]
- Kajishima, T.; Takiguchi, S.; Hamasaki, H.; Miyake, Y. Turbulence structure of particle-laden flow in a vertical plane channel due to vortex shedding. JSME Int. J. Ser. B 2001, 44, 526–535. [Google Scholar] [CrossRef]
- Mizutani, N.; McDougal, W.G.; Mostafa, A.M. Bem-FEM combined analysis of nonlinear interaction between wave and submerged breakwater. In Coastal Engineering 1996; American Society of Civil Engineers: Reston, VA, USA, 1996; pp. 2377–2390. [Google Scholar]
- Brackbill, J.U.; Kothe, D.B.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Kobayashi, H. The subgrid-scale models based on coherent structures for rotating homogeneous turbulence and turbulent channel flow. Phys. Fluids 2005, 17, 045104. [Google Scholar] [CrossRef]
- Iwata, K.; Kawasaki, K.; Kim, D.S. Numerical analysis of wave breaking due to submerged structures. Proc. Coast. Eng. JSCE 1995, 42, 781–785. [Google Scholar]
- Hou, Y.; Nakamura, T.; Cho, Y.H.; Mizutani, N.; Tomita, T. Influence of tsunami-driven shipping containers’ layout on their motion. J. Mar. Sci. Eng. 2022, 10, 1911. [Google Scholar] [CrossRef]
- Small, C.; Blanpied, T.; Kauffman, A.; O’Neil, C.; Proulx, N.; Rajacich, M.; Simpson, H.; White, J.; Spaulding, M.; Baxter, C.; et al. Assessment of damage and adaptation strategies for structures and infrastructure from storm surge and sea level rise for a coastal community in Rhode Island, United States. J. Mar. Sci. Eng. 2016, 4, 67. [Google Scholar] [CrossRef]
- Derschum, C.; Nistor, I.; Stolle, J.; Goseberg, N. Debris impact under extreme hydrodynamic conditions part 1: Hydrodynamics and impact geometry. Coast. Eng. 2018, 141, 24–35. [Google Scholar] [CrossRef]
- Duncan, S.; Cox, D.; Barbosa, A.R.; Lomónaco, P.; Park, H.; Alam, M.S.; Yu, C. Physical modeling of progressive damage and failure of wood-frame coastal residential structures due to surge and wave forces. Coast. Eng. 2021, 169, 103959. [Google Scholar] [CrossRef]
- Borrero, J.C.; Sieh, K.; Chlieh, M.; Synolakis, C.E. Tsunami inundation modeling for Western Sumatra. Proc. Natl. Acad. Sci. USA 2006, 103, 19673–19677. [Google Scholar] [CrossRef]
- Fritz, H.M.; Phillips, D.A.; Okayasu, A.; Shimozono, T.; Liu, H.; Mohammed, F.; Skanavis, V.; Synolakis, C.E.; Takahashi, T. The 2011 Japan tsunami current velocity measurements from survivor videos at Kesennuma Bay Using Lidar. Geophys. Res. Lett. 2012, 39, L00G23. [Google Scholar] [CrossRef]
- Takahashi, S.; Sugano, T.; Tomita, T.; Arikawa, T.; Tatsumi, D.; Kashima, H.; Murata, S.; Matsuoka, Y.; Nakamura, T. Joint Survey for 2010 Chilean Earthquake and Tsunami Disaster in Ports and Coasts; Port and Airport Research Institute: Kurihama, Japan, 2010.
- Roeber, V.; Yamazaki, Y.; Cheung, K.F. Resonance and impact of the 2009 Samoa tsunami around Tutuila, American Samoa. Geophys. Res. Lett. 2010, 37, 1–9. [Google Scholar] [CrossRef]
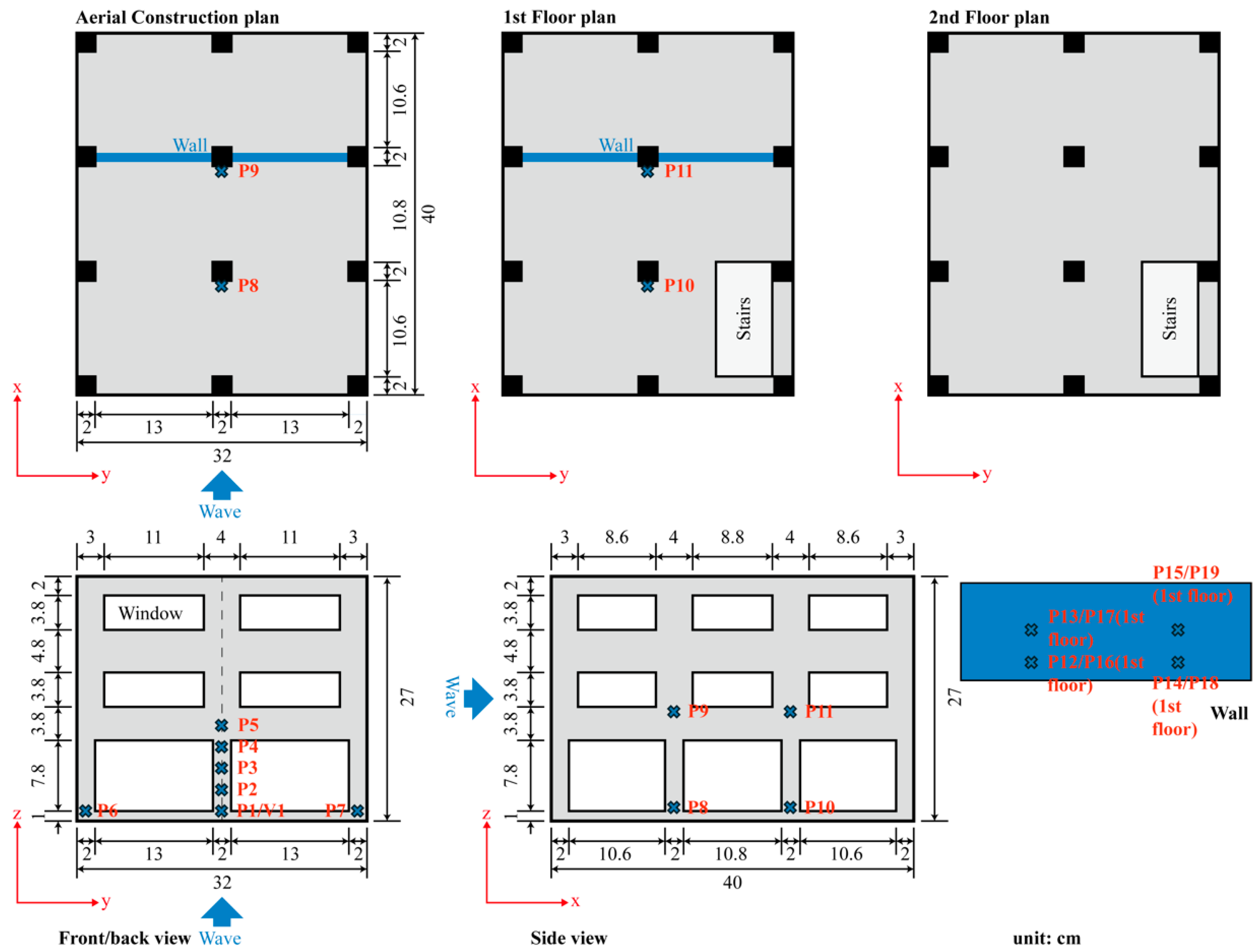
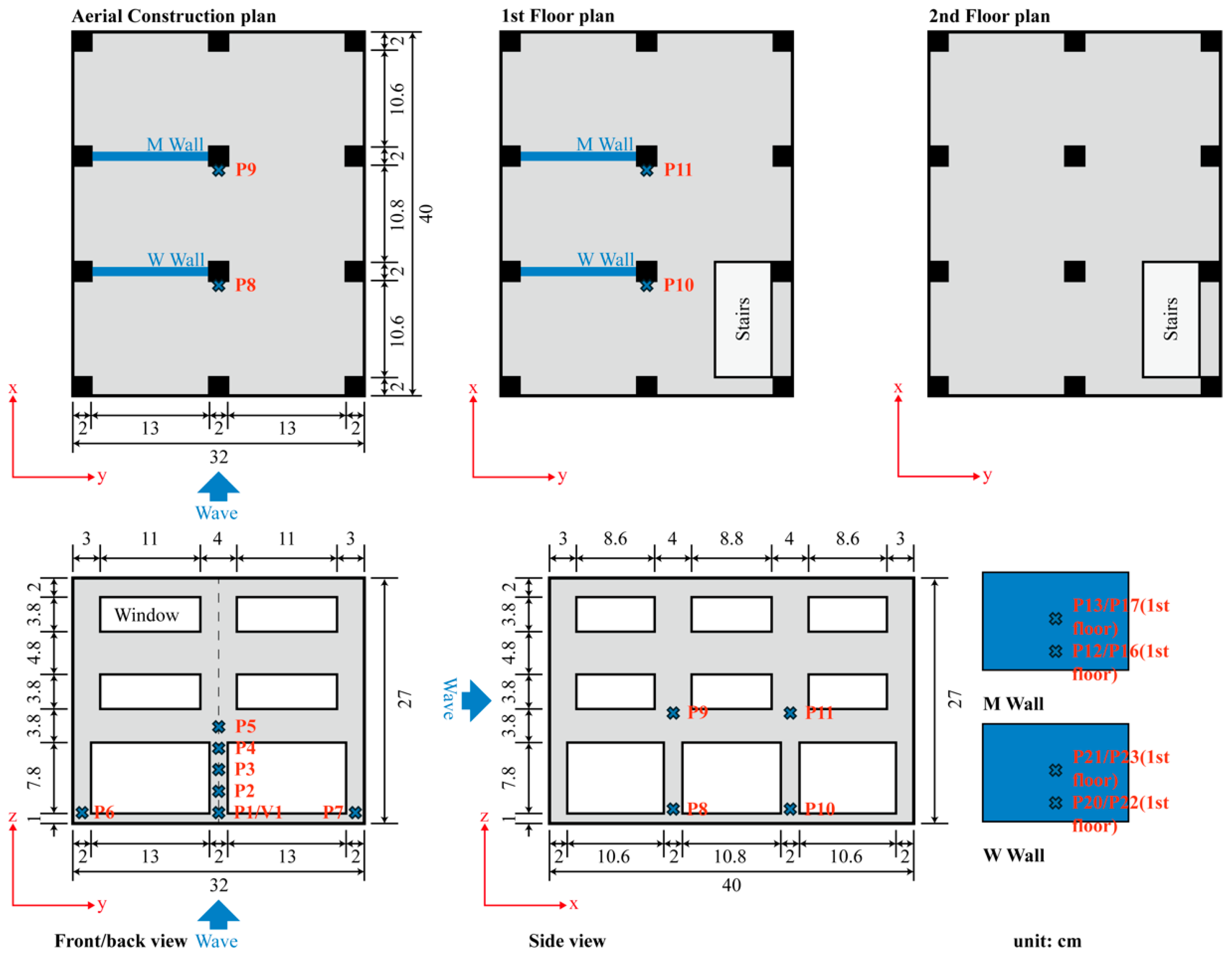
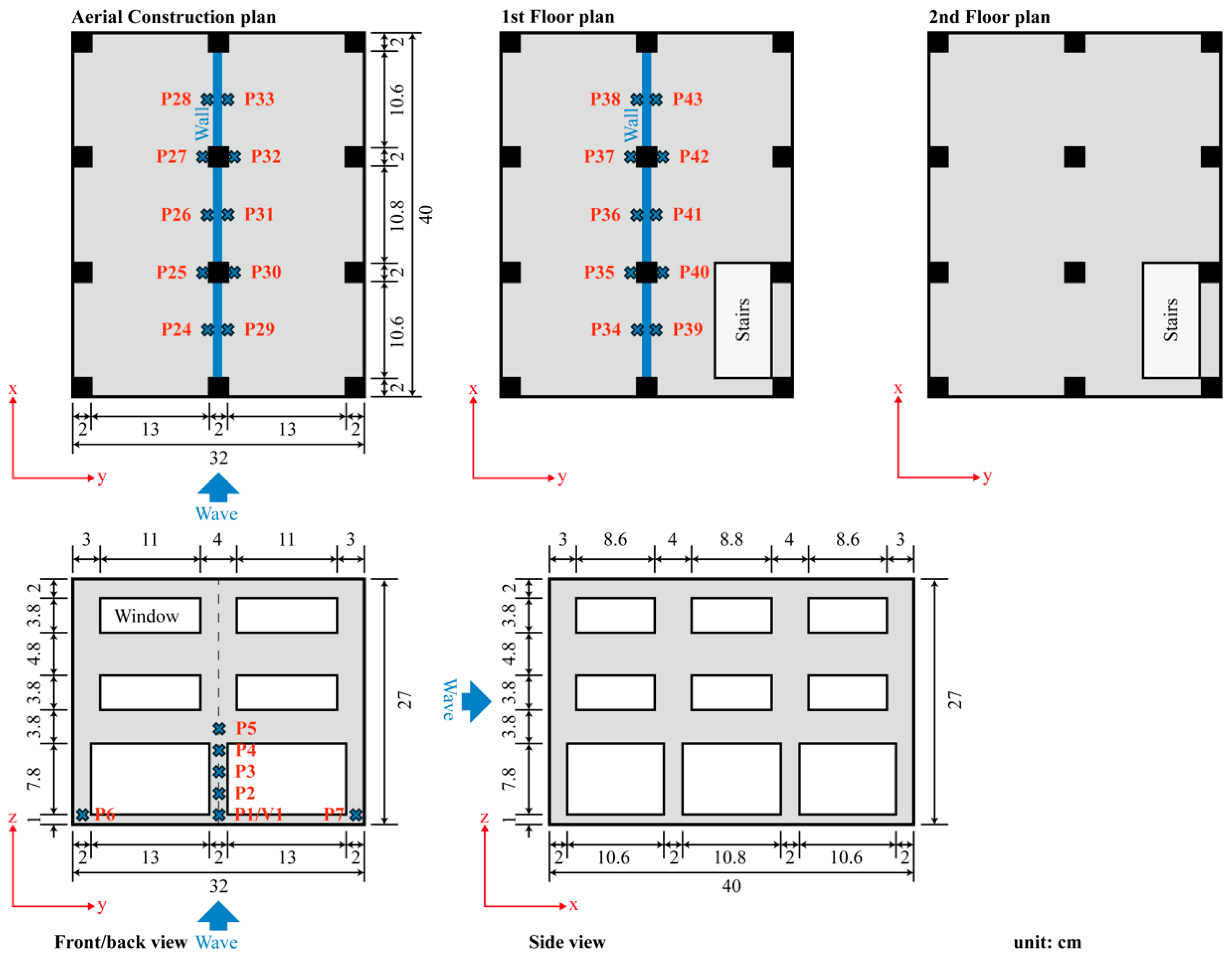
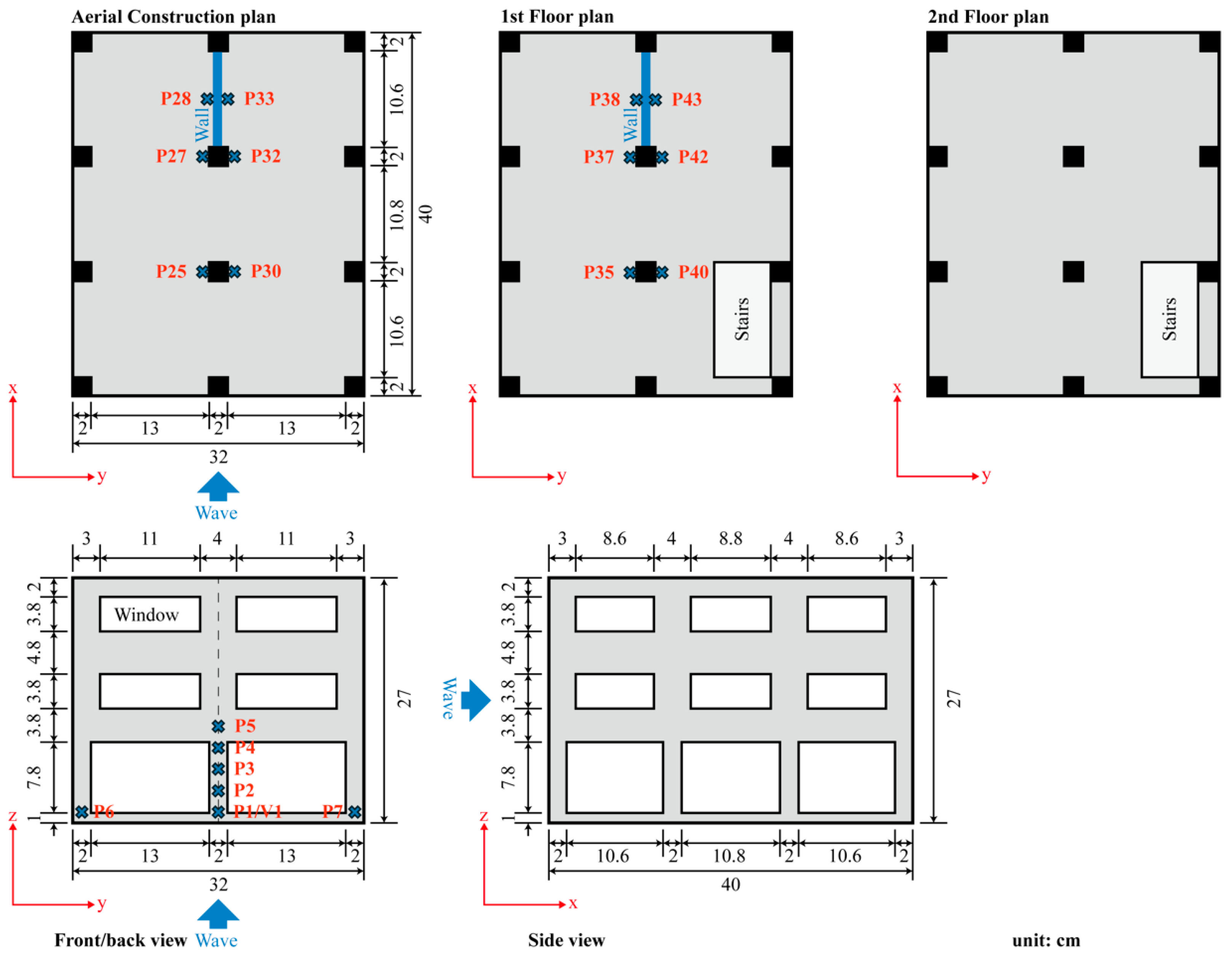





| Case Number | Water Depth (m) | Wave Height (m) | Wave Period (s) | Test Duration (s) | Wave Type |
|---|---|---|---|---|---|
| T01 | 0.32 | 0.064 | N/A | 10 | Solitary wave |
| T02 | 0.32 | 0.16 | N/A | 10 | Solitary wave |
| T03 | 0.32 | 0.128 | 6 | 10 | Long-period wave |
| T04 | 0.32 | 0.128 | 8 | 10 | Long-period wave |
| T05 | 0.32 | 0.128 | 14 | 10 | Long-period wave |
| T06 | 0.32 | 0.192 | 6 | 10 | Long-period wave |
| T07 | 0.32 | 0.192 | 8 | 10 | Long-period wave |
| T08 | 0.32 | 0.192 | 14 | 10 | Long-period wave |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hou, Y.; Nakamura, T.; Cho, Y.-H.; Mizutani, N. Study on the Effects of Internal Building Layouts on Tsunami-Driven Single-Container Motion. J. Mar. Sci. Eng. 2025, 13, 513. https://doi.org/10.3390/jmse13030513
Hou Y, Nakamura T, Cho Y-H, Mizutani N. Study on the Effects of Internal Building Layouts on Tsunami-Driven Single-Container Motion. Journal of Marine Science and Engineering. 2025; 13(3):513. https://doi.org/10.3390/jmse13030513
Chicago/Turabian StyleHou, Yudou, Tomoaki Nakamura, Yong-Hwan Cho, and Norimi Mizutani. 2025. "Study on the Effects of Internal Building Layouts on Tsunami-Driven Single-Container Motion" Journal of Marine Science and Engineering 13, no. 3: 513. https://doi.org/10.3390/jmse13030513
APA StyleHou, Y., Nakamura, T., Cho, Y.-H., & Mizutani, N. (2025). Study on the Effects of Internal Building Layouts on Tsunami-Driven Single-Container Motion. Journal of Marine Science and Engineering, 13(3), 513. https://doi.org/10.3390/jmse13030513







