Delayed Detached-Eddy Simulations of Aerodynamic Variability During Carrier-Based Aircraft Landing with a Domain Precursor Inflow Method
Abstract
1. Introduction
2. CFD Methodology for Carrier-Based Aircraft Landing Simulation
2.1. The Unsteady Flow Solver
2.2. DDES-Based RANS-LES Hybrid Method
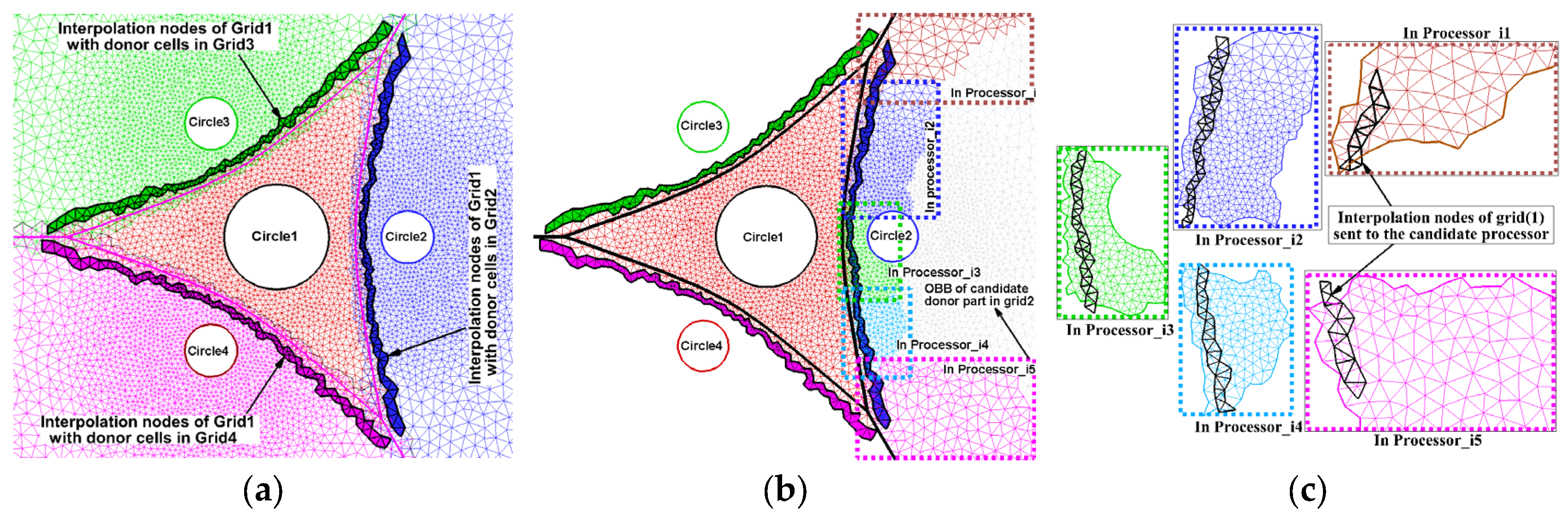
2.3. Dynamic Parallel Overset Grid Method
3. Simulation Model for Aerodynamic Variability in Aircraft Landing
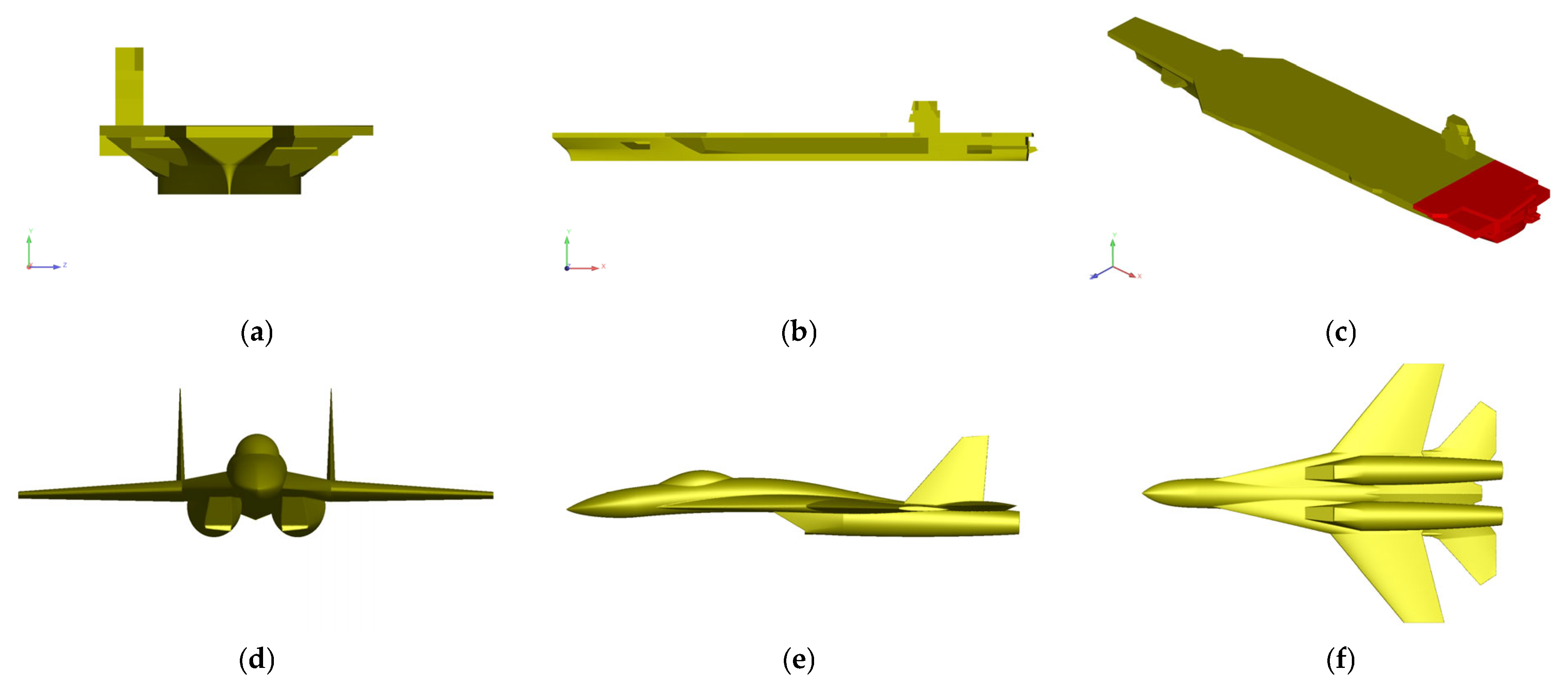
3.1. Profile of Aircraft Landing to Carrier
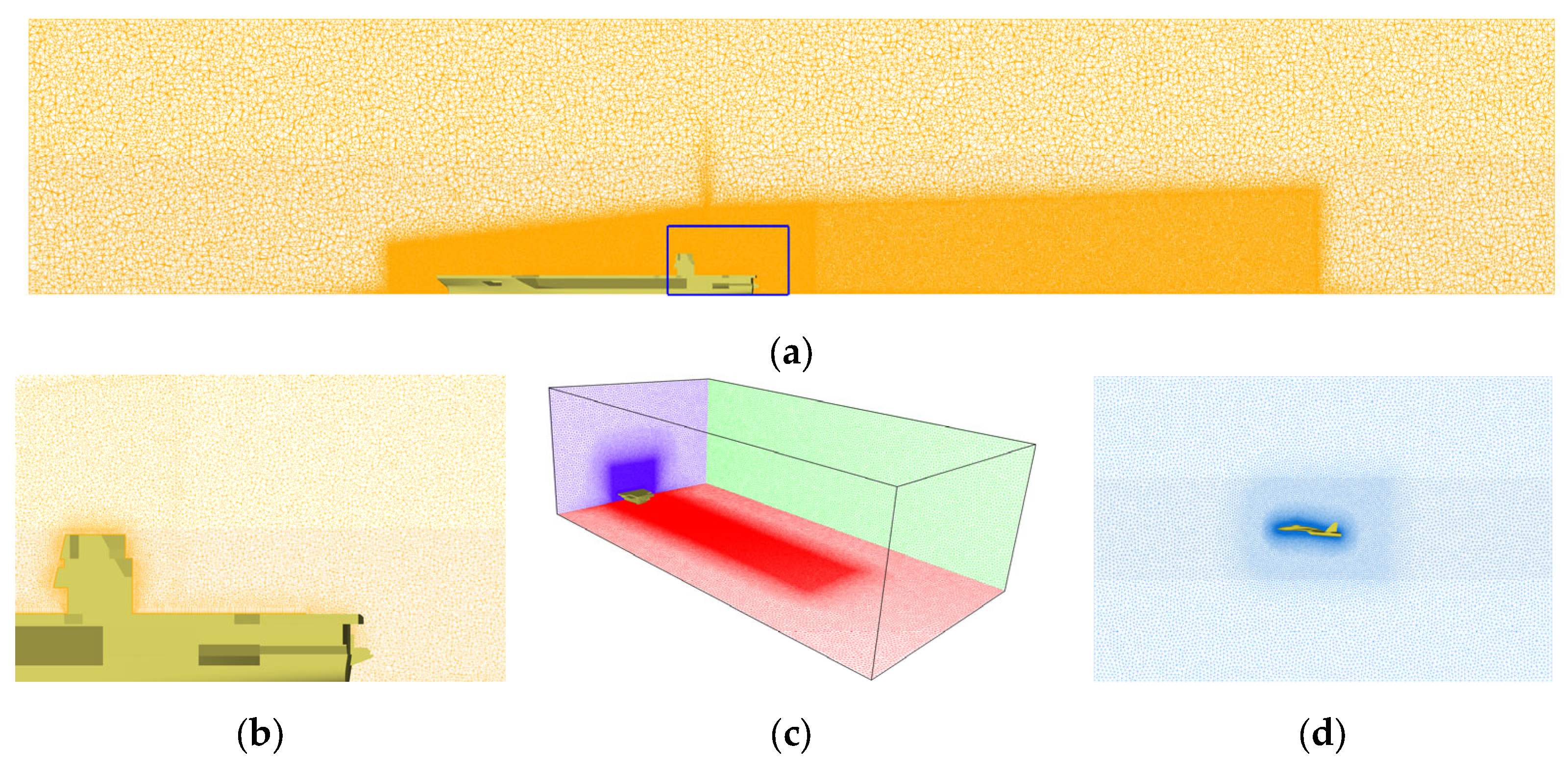
3.2. Domain Precursor Inflow Approach
- (1)
- The entire carrier air-wake domain, the aft carrier air-wake domain, and the aircraft flow field domain are determined, and the meshes in those domains are generated separately.
- (2)
- The unsteady flow field of in the entire carrier air-wake domain is computed by using the RANS-LES hybrid solver to a fully developed state.
- (3)
- The solution of the entire domain at a moment and the transient solution at each physical time step after in the same plane as the inflow of aft air-wake domain are saved.
- (4)
- The steady flow field in the aircraft domain is calculated, and the solution is saved as the initialized state of landing simulation.
- (5)
- The aft carrier air-wake field is initialized by interpolating from the solution of the entire carrier air-wake domain at .
- (6)
- The mesh and solution of the aircraft domain are adjusted to the proper state according to landing simulation condition.
- (7)
- The aircraft landing through the carrier air-wake is simulated on the overset grids of the aft air-wake domain mesh and aircraft mesh with the unsteady precursor inflow boundary condition.
3.3. Landing Simulation Setup
4. Results and Discussion
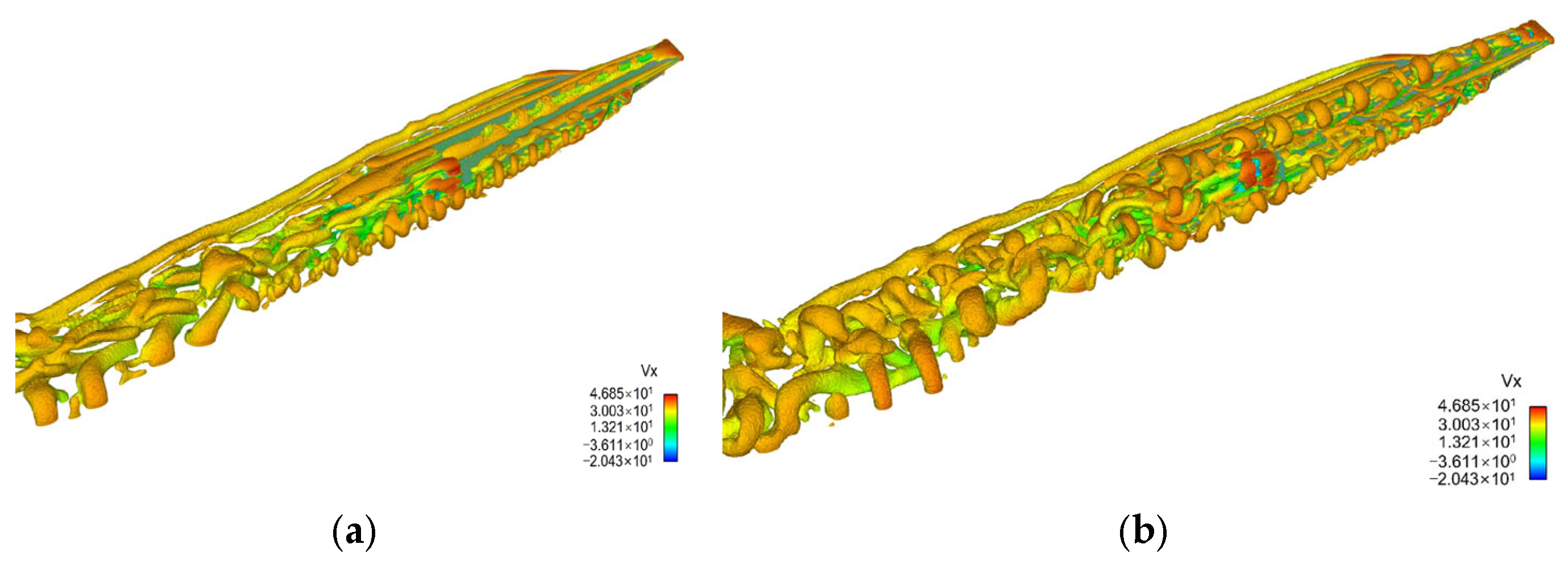
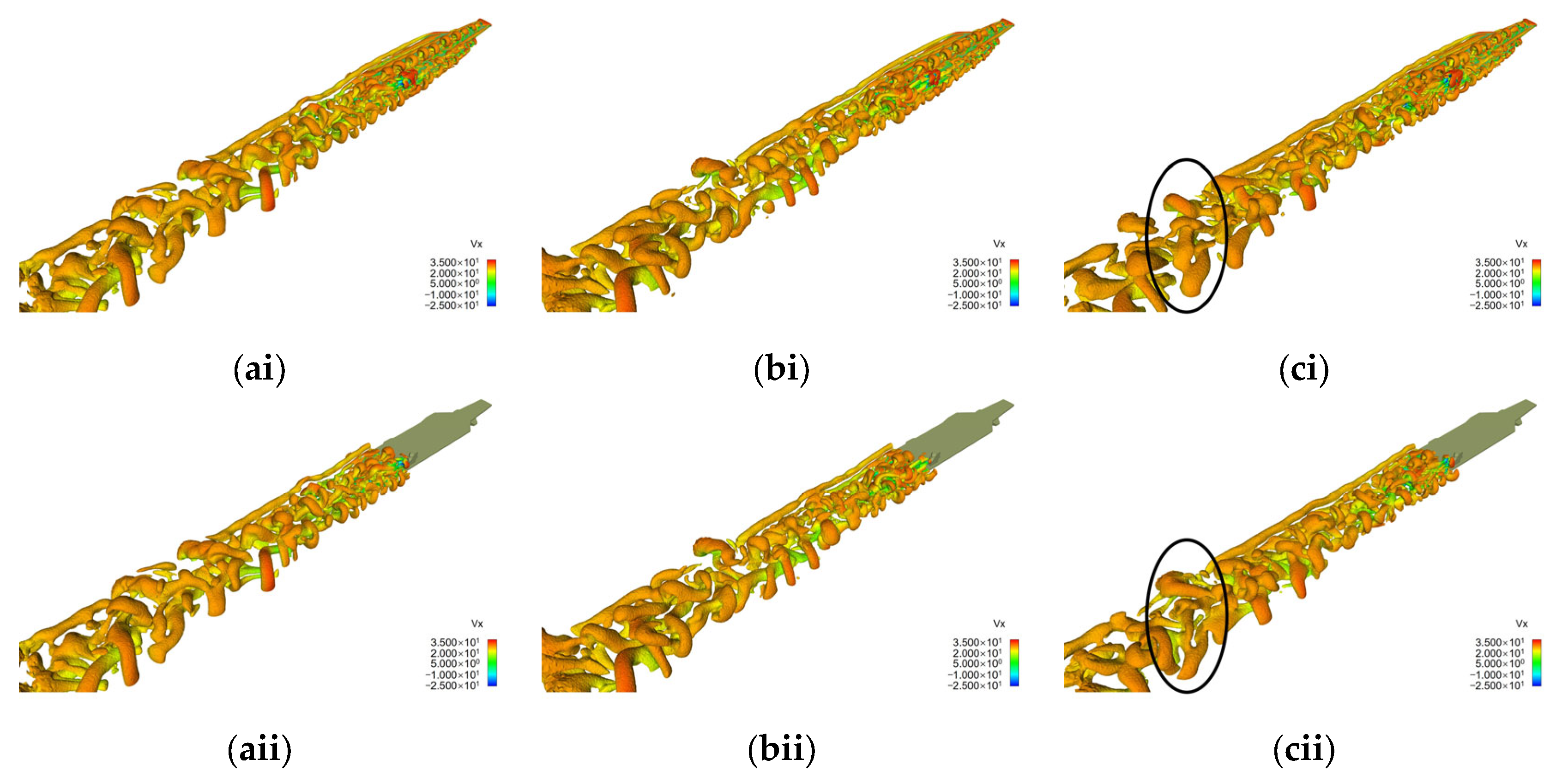
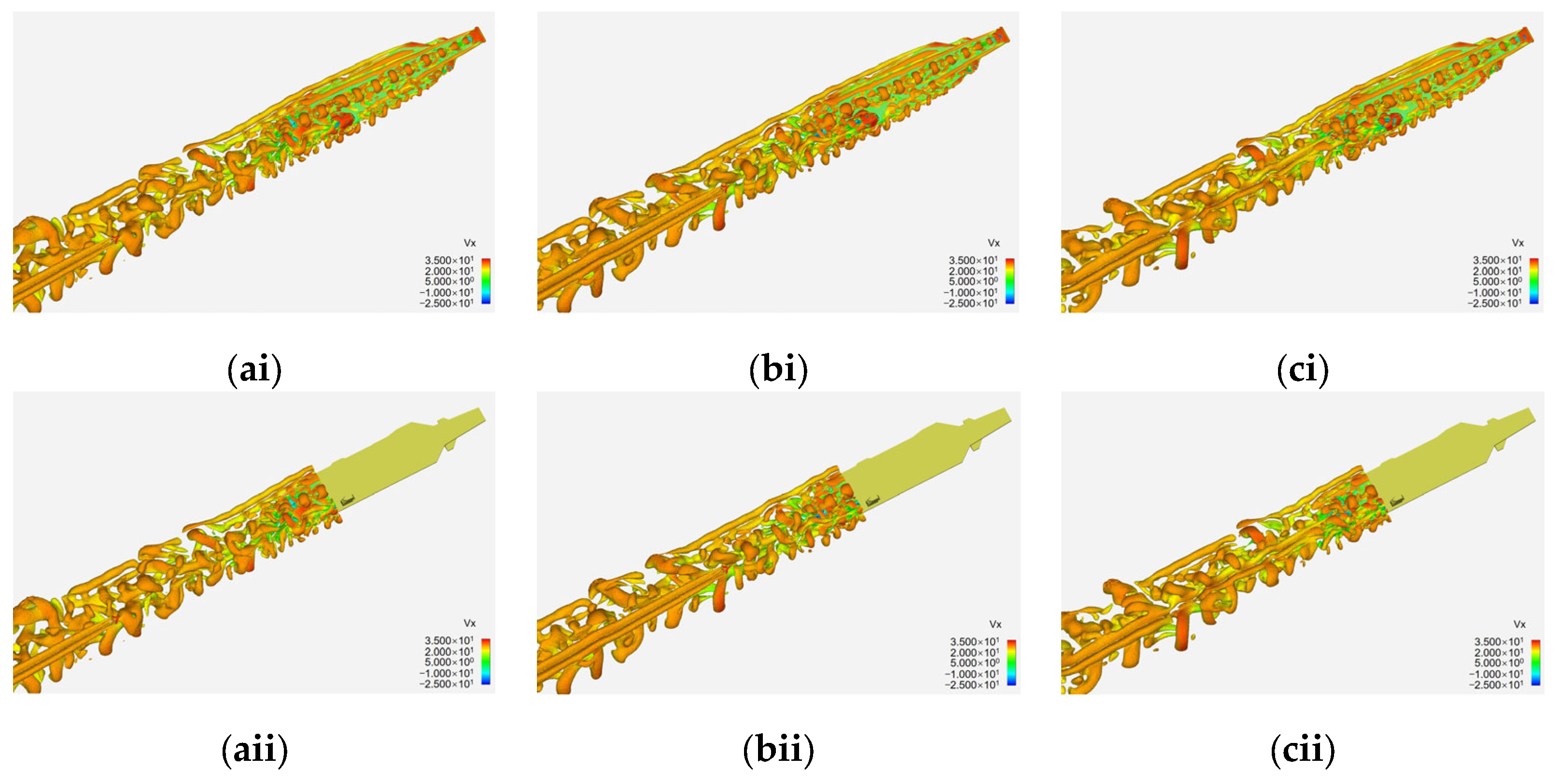
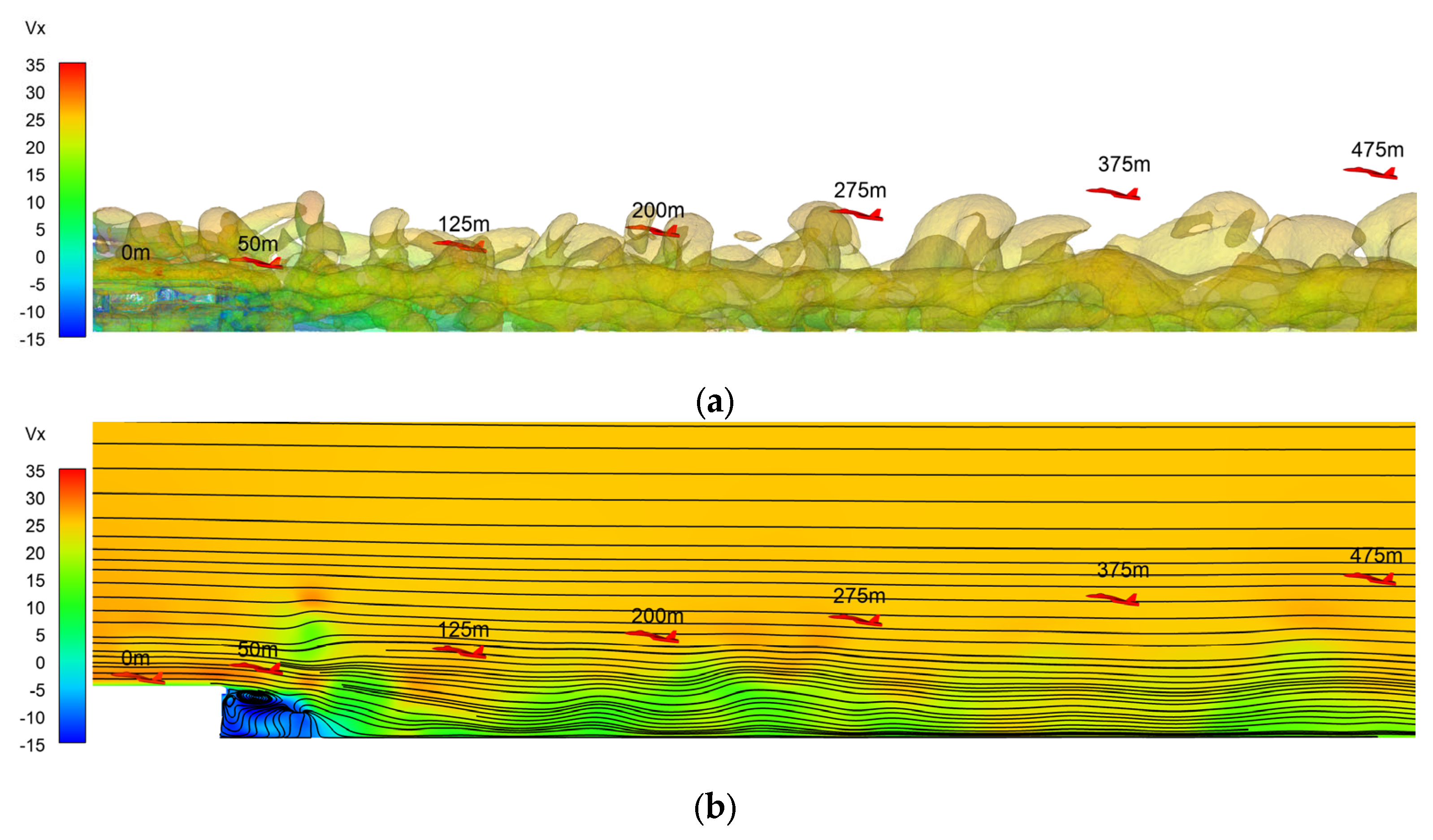
4.1. Validation of the Domain Precursor Inflow Approach
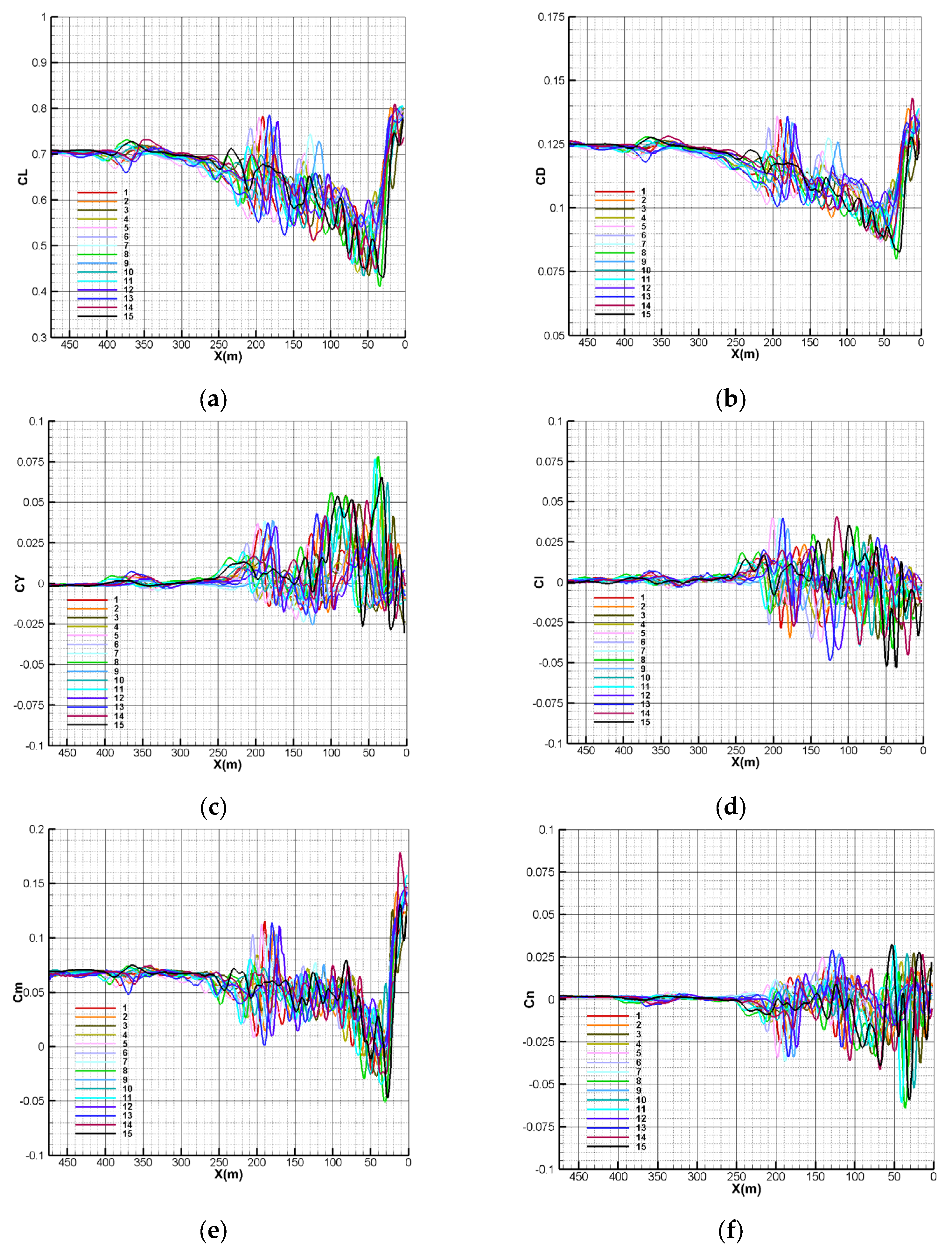
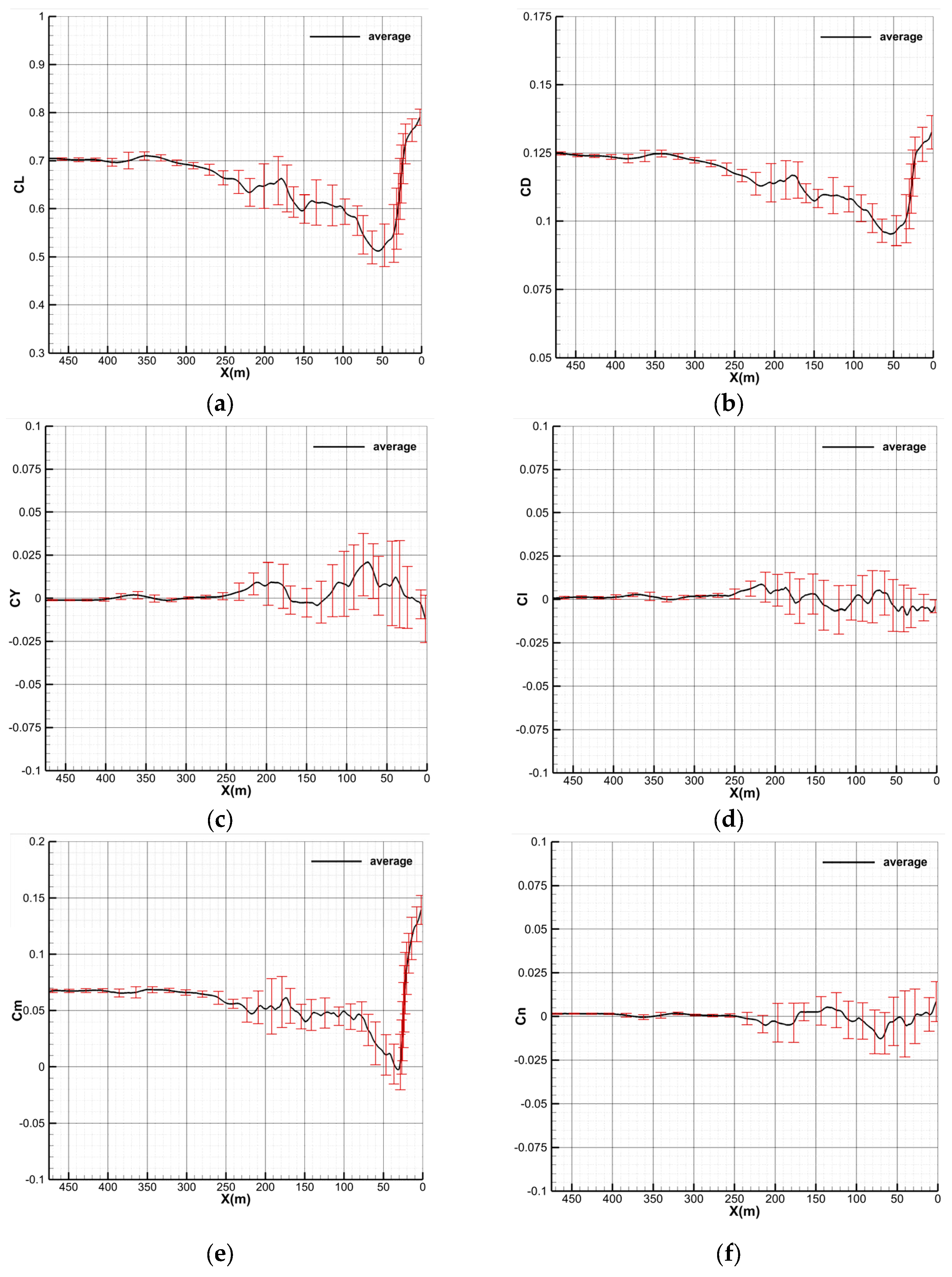
4.2. Variability Analysis of Aerodynamic Loads
5. Conclusions
- (1)
- The domain precursor inflow method can significantly reduce computational costs compared to full-domain simulations while maintaining fidelity in air-wake simulation.
- (2)
- Parametric analyses of 15 distinct landing trajectories reveal significant aerodynamic variability, particularly within 250 m of the carrier, where interactions with island-generated vortices induce fluctuations in lift (up to 25%), drag (18%), and pitching moments (30%).
- (3)
- Ground effects near the deck further amplify load variations by 12–15%, while lateral deviations in landing paths generate asymmetric forces and moments.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Micklos, R.P. Effect of Wind Over Deck Conditions on Aircraft Approach Speeds for Carrier Landings; Naval Air Systems Command: Washington, DC, USA, 1991. [Google Scholar]
- Kelly, M.F. The Development, Validation, and Integration of Aircraft Carrier Airwakes for Piloted Flight Simulation. Ph.D. Thesis, University of Liverpool, Liverpool, UK, 2018. [Google Scholar]
- Jiao, J.; Wei, M.; Yuan, Y.; Zhao, T. Risk Quantification and Analysis of Coupled Factors Based on the DEMATEL Model and a Bayesian Network. Appl. Sci. 2020, 10, 317. [Google Scholar] [CrossRef]
- Li, X. Risk Analysis of Complex System Operation considering Effective Control Time. Math. Probl. Eng. 2022, 2022, 4800559. [Google Scholar] [CrossRef]
- Kumar, A.; Ben-Tzvi, P. Novel wireless sensing platform for experimental mapping and validation of ship air wake. Mechatronics 2018, 52, 58–69. [Google Scholar] [CrossRef]
- Watson, N.A.; Owen, I.; White, M.D. Experimental Validation of the CFD-generated Airwake of an Aircraft Carrier with Simulated Atmospheric Turbulence. In Proceedings of the Virtual AIAA Aviation Forum, Chicago, IL, USA, 27 June–1 July 2022. [Google Scholar]
- Bardera, R.; Matias-Garcia, J.C.; Barderas, E.B. Wind Tunnel Tests for Determining the Aerodynamic Forces and Moments of a Helicopter Operating on an Aircraft Carrier. In Proceedings of the AIAA Aviation Forum and Ascend, Las Vegas, NV, USA, 29 July–2 August 2024. [Google Scholar] [CrossRef]
- Forrest, J.S.; Owen, I. An investigation of ship airwakes using Detached-Eddy Simulation. Comput. Fluids 2010, 39, 656–673. [Google Scholar] [CrossRef]
- Watson, N.A.; White, M.; Owen, I. Experimental Validation of the Unsteady CFD-generated Airwake of the HMS Queen Elizabeth Aircraft Carrier. In Proceedings of the AIAA Aviation 2019 Forum, Dallas, TX, USA, 17–21 June 2019. [Google Scholar]
- Zhang, J.; Minelli, G.; Basara, B.; Bensow, R.; Krajnović, S. Yaw Effect on Bi-stable Air-wakes of a Generic Ship Using Large Eddy Simulation. Ocean Eng. 2021, 219, 108164. [Google Scholar] [CrossRef]
- Zhang, J.; Gidado, F.; Adamu, A.; Guo, Z.; Gao, G. An Investigation on the Wake Flow of a Generic Ship Using IDDES: The Effect of Computational Parameters. Ocean Eng. 2023, 271, 113644. [Google Scholar] [CrossRef]
- Ge, G.; Zhang, W.; Xie, B.; Li, J. Turbulence Model Optimization of Ship Wake Field Based on Data Assimilation. Ocean Eng. 2024, 295, 116929. [Google Scholar] [CrossRef]
- Yang, X.; Li, B.; Ren, Z.; Tian, F. Numerical Simulation of the Unsteady Airwake of the Liaoning Carrier Based on the DDES Model Coupled with Overset Grid. J. Mar. Sci. Eng. 2024, 12, 1598. [Google Scholar] [CrossRef]
- Nehra, A.; Rajagopalan, V. Effect of Twin Island Configuration on Airwake Aerodynamics over Generic Aircraft Carrier Using CFD. Defence Sci. J. 2023, 73, 612–624. [Google Scholar] [CrossRef]
- Watson, N.A.; Kelly, M.F.; Owen, I.; Hodge, S.J.; White, M.D. Computational and Experimental Modelling Study of the Unsteady Airflow over the Aircraft Carrier HMS Queen Elizabeth. Ocean Eng. 2019, 172, 562–574. [Google Scholar] [CrossRef]
- Mathew, M.P.; Singh, S.N.; Sinha, S.S.; Vijayakumar, R. Modeling of Air Flow Over a Generic Aircraft Carrier With and Without Island Structure. Intl. J. Maritime Eng. 2021, 163 Pt A3, 93–110. [Google Scholar] [CrossRef]
- Duque, E.P.N.; Imlay, S.; Ahern, S.; Chen, G.; Kao, D. NASA CFD Vision 2030 Visualization and Knowledge Extraction: Panel Summary from AIAA Aviation 2015 Conference. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016. AIAA Paper 2016-1927. [Google Scholar]
- Polsky, S.; Naylor, S. CVN Airwake Modeling and Integration: Initial Steps in the Creation and Implementation of a Virtual Burble for F-18 Carrier Landing Simulations. In Proceedings of the AIAA Modeling and Simulation Technologies Conference and Exhibit, San Francisco, CA, USA, 15–18 August 2005. AIAA 2005-6298. [Google Scholar]
- Shipman, J.D.; Arunajatesan, S.; Cavallo, P.A.; Sinha, N. Dynamic CFD Simulation of Aircraft Recovery to an Aircraft Carrier. In Proceedings of the 26th AIAA Applied Aerodynamics Conference, Honolulu, HI, USA, 18–21 August 2008. AIAA 2008-6227. [Google Scholar]
- Tian, S.L.; Xu, K.; Xia, J.; Chen, L. Efficient Parallel Dynamic Overset Unstructured Grid for Flow Simulation with Moving Bodies. AIAA J. 2023, 61, 2135–2166. [Google Scholar] [CrossRef]
- Fu, J.; Wang, J.; Wu, J.; Xu, K.; Tian, S. Investigation of the Influence of Wake Field Characteristic Structures on Downstream Targets Using the POD Method. Aerospace 2023, 10, 824. [Google Scholar] [CrossRef]
- Chen, L.; Xia, J.; Tian, S. A New Blend Low-Speed Preconditioning Method for Numerical Simulation of Rotor Interaction Flow Field. Acta Aeron. Astron. Sin. 2018, 39, 121903. [Google Scholar] [CrossRef]
- Blazek, J. Computational Fluid Dynamics: Principles and Applications, 3rd ed.; Butterworth-Heinemann: Oxford, UK, 2001; pp. 15–25. [Google Scholar]
- Einfeldt, B. On Godunov-Type Methods for Gas Dynamics. SIAM J. Numer. Anal. 1988, 25, 294–318. [Google Scholar] [CrossRef]
- Venkatakrishnan, V. Convergence to Steady-State Solutions of the Euler Equations on Unstructured Grids with Limiters. J. Comput. Phys. 1995, 118, 120–130. [Google Scholar] [CrossRef]
- Sharov, D.; Kazuhiro, K. Reordering of 3-D Hybrid Unstructured Grids for Vectorized LU-SGS Navier-Stokes Calculations. In Proceedings of the 13th Computational Fluid Dynamics Conference, Snowmass Village, CO, USA, 29 June–2 July 1997. AIAA Paper 97-2102. [Google Scholar] [CrossRef]
- Wu, D.; Zhao, W.; Wan, D. Comparative Study of SST-SAS and SST-DDES in Predicting Massively Separated Flow. In Proceedings of the 28th International Ocean and Polar Engineering Conference, Sapporo, Japan, 10–15 June 2018. [Google Scholar]
- Strelets, M. Detached Eddy Simulation of Massively Separated Flows. In Proceedings of the 39th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2001. AIAA-2001-0879. [Google Scholar]
- Spalart, P.R. A New Version of Detached-Eddy Simulation Resistant to Ambiguous Grid Densities. Theor. Comput. Fluid Dyn. 2006, 20, 181–195. [Google Scholar] [CrossRef]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten Years of Industrial Experience with the SST Turbulence Model. In Proceedings of the Turbulence Heat and Mass Transfer 4, Antalya, Turkey, 12–17 October 2003. [Google Scholar]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Xia, J.; Fu, H.; Tian, S.; Chen, L. Highly Efficient Wall-Distance-Based Parallel Unstructured Overset Grid Assembly. AIAA J. 2021, 59, 5347–5359. [Google Scholar] [CrossRef]
- Mo, F.; Li, Q.; Zhang, L.; Gao, Z. Direct Numerical Simulation of Hypersonic Wall-Bounded Turbulent Flows: An Improved Inflow Boundary Condition and Applications. Phys. Fluids 2023, 35, 035135. [Google Scholar] [CrossRef]
- Lu, X.; Wang, S.; Sung, H.G.; Shieh, S.Y.; Yang, V. Large-Eddy Simulations of Turbulent Swirling Flows Injected into a Dump Chamber. J. Fluid Mech. 2005, 527, 171–195. [Google Scholar] [CrossRef]
- Wang, J. Research on Order Reduction Methodology and Application of Complex Unsteady Low-Speed Wake Field. Master’s Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2023. [Google Scholar]
- Meng, Y.; Wang, W.; Han, H. Flight Control Method Using Neural Network in Prediction for Suppressing Ship Airwake Impact in Carrier Landing. IEEE Aerosp. Electron. Syst. Mag. 2023, 38, 20–32. [Google Scholar] [CrossRef]
- Chen, L. Numerical Simulation of Rotor Aeroelastics Using CFD/CSD Coupling. Ph.D. Thesis, Nanjing University of Aeronautics and Astronautics, Nanjing, China, 2011. [Google Scholar]
- Hunt, J.C.R.; Wray, A.A.; Moin, P. Eddies, Streams, and Convergence Zones in Turbulent Flows. In Proceedings of the 1988 Summer Program, San Francisco, CA, USA, 20–24 June 1988; Report CTR-S88. NASA Stanford Center for Turbulence Research: Stanford, CA, USA, 1988; pp. 193–208. [Google Scholar]



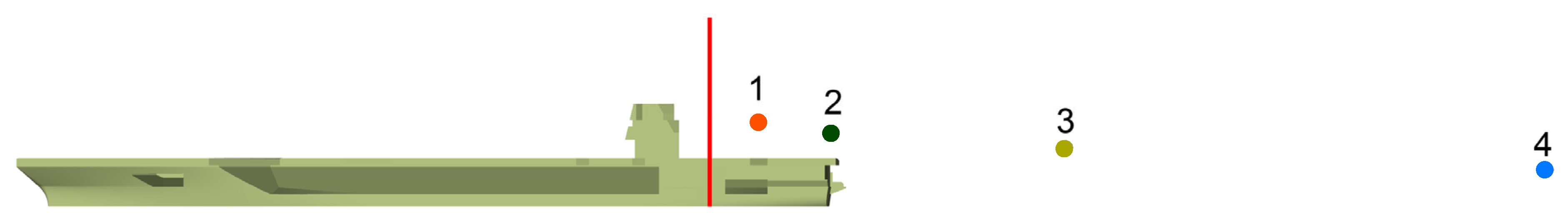
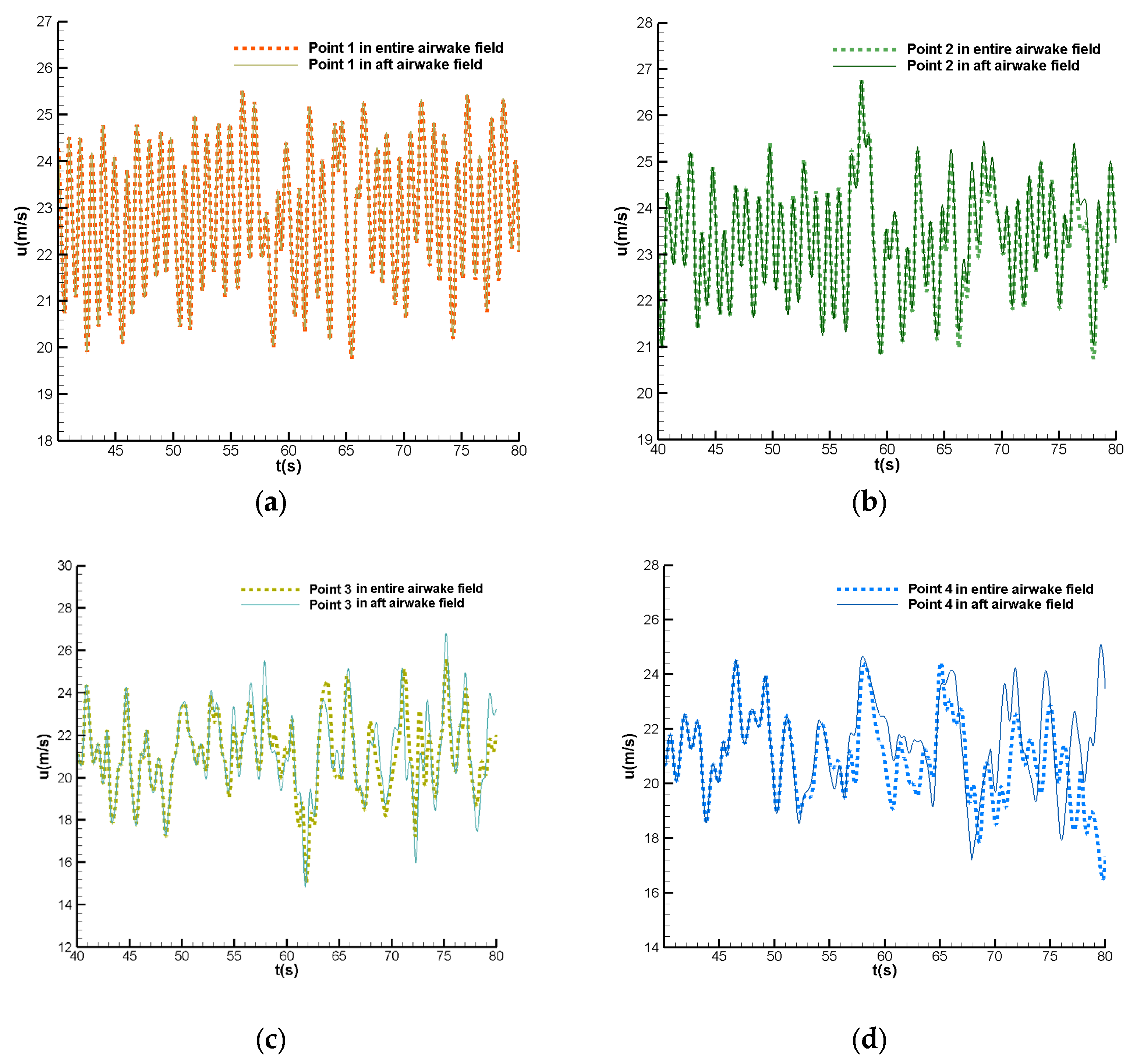


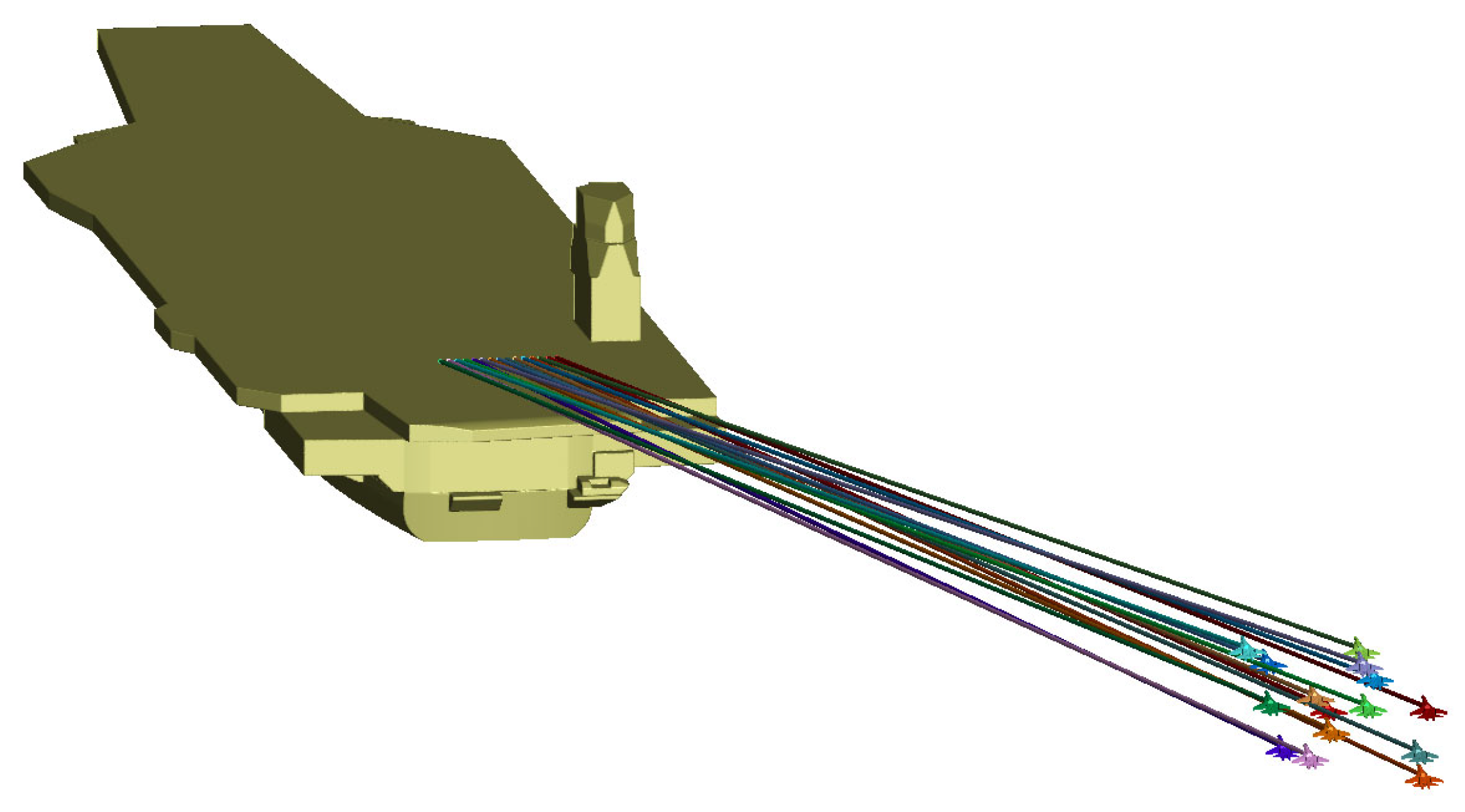




| ID | (°) | (m) | (m) |
|---|---|---|---|
| 1 | 4.6000 | 1.3333 | 550.0000 |
| 2 | 4.8667 | −2.6667 | 523.3333 |
| 3 | 5.8000 | −6.6667 | 536.6667 |
| 4 | 5.9333 | 2.6667 | 576.6667 |
| 5 | 4.3333 | 8.0000 | 563.3333 |
| 6 | 5.4000 | 5.3333 | 590.0000 |
| 7 | 5.0000 | 9.3333 | 543.3333 |
| 8 | 4.2000 | −5.3333 | 583.3333 |
| 9 | 5.6667 | 6.6667 | 516.6667 |
| 10 | 5.5333 | −4.0000 | 556.6667 |
| 11 | 4.7333 | −1.3333 | 596.6667 |
| 12 | 5.2667 | 0.0000 | 503.3333 |
| 13 | 4.0667 | 4.0000 | 530.0000 |
| 14 | 4.4667 | −8.0000 | 510.0000 |
| 15 | 5.1333 | −9.3333 | 570.0000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fu, J.; Hu, R.; Wang, H.; Xu, K.; Tian, S. Delayed Detached-Eddy Simulations of Aerodynamic Variability During Carrier-Based Aircraft Landing with a Domain Precursor Inflow Method. J. Mar. Sci. Eng. 2025, 13, 498. https://doi.org/10.3390/jmse13030498
Fu J, Hu R, Wang H, Xu K, Tian S. Delayed Detached-Eddy Simulations of Aerodynamic Variability During Carrier-Based Aircraft Landing with a Domain Precursor Inflow Method. Journal of Marine Science and Engineering. 2025; 13(3):498. https://doi.org/10.3390/jmse13030498
Chicago/Turabian StyleFu, Jiawei, Ruifan Hu, Hong Wang, Ke Xu, and Shuling Tian. 2025. "Delayed Detached-Eddy Simulations of Aerodynamic Variability During Carrier-Based Aircraft Landing with a Domain Precursor Inflow Method" Journal of Marine Science and Engineering 13, no. 3: 498. https://doi.org/10.3390/jmse13030498
APA StyleFu, J., Hu, R., Wang, H., Xu, K., & Tian, S. (2025). Delayed Detached-Eddy Simulations of Aerodynamic Variability During Carrier-Based Aircraft Landing with a Domain Precursor Inflow Method. Journal of Marine Science and Engineering, 13(3), 498. https://doi.org/10.3390/jmse13030498






