Hydrodynamics and Sediment Transport Under Solitary Waves in the Swash Zone

Abstract

Swash–swash interaction is a common natural phenomenon in the nearshore region, characterized by complex fluid motion. The characteristics of swash–swash interaction are crucial to sediment transport, subsequently affecting the beach morphology. This study investigates the hydrodynamics and sediment transport in swash–swash interaction under two successive solitary waves using a two-phase Smoothed Particle Hydrodynamics (SPH) model. The effects of the time interval between the two waves are examined. It is shown that the time interval has a minor effect on the breaking and swash–swash interacting patterns as well as the final beach morphology but influences the run-up of the second wave and the instantaneous sediment flux. Under wave breaking in the swash–swash interaction, there is significant sediment suspension due to strong vortices, and the suspended sediment forms a plume upward from the bed. The sediment plumes gradually settle down as the vortices decay. These insights enhance the understanding of sediment transport and beach morphology under complex swash–swash interaction.

1. Introduction

The swash zone, a sub-region of the nearshore area, is periodically inundated by wave action. This dynamic zone plays a crucial role in sediment transport and beach morphological changes [1,2,3,4,5,6]. The hydrodynamics of swash flow are characterized by shallow depth [7,8], turbulence generated by wave collapse [9,10], and cross-shore flow accelerations [11,12].

Single swash events induced by dam-break flows [13,14,15] or solitary waves [16,17,18,19] have been focused on, and the relations of hydrodynamics and sediment transport to uprush and backwash behaviors of single swash have been investigated. However, in the field, swash on natural beaches is typically characterized by multiple waves and successive swash events that can interact with each other [20,21,22,23]. The swash–swash interactions are crucial to both suspended sediment transport [24,25] and sheet flow [26,27,28]. Alsina et al. [29] demonstrated the importance of swash–swash interactions in wave-by-wave laboratory experiments. The following wave may catch up with a preceding uprush wave or meet with the backwash of the preceding wave that moves in the opposite direction. Generally, uprush events carry sediment onshore while the backwash transports sediment seaward. Swash–swash interactions may modify these patterns of sediment transport. A wave overrunning a preceding uprush bore reduces the onshore movement of sediment, while the following wave may suppress the seaward sediment transport by a weak backwash flow [23].

However, the hydrodynamics and sediment transport in the swash zone remain incompletely understood [30,31,32,33,34,35,36]. Specifically, the influence of interactions between successive swashes on sheet flow dynamics, sediment suspension, and, hence, bed evolution requires further investigation [27,37,38,39]. Regarding their resemblance to field observations, successive solitary waves were made in laboratory experiments to set up swash–swash interaction cases [23,40]. In this study, two successive solitary waves are numerically produced, and each time, an individual swash–swash interaction event is investigated in detail.

Two-phase flow models, which simultaneously consider the interaction between the water and sediment phases, have shown effectiveness in capturing the complex hydrodynamics and sediment dynamics in nearshore regions [41,42,43,44]. Fluid–sediment and sediment–sediment interactions are carefully formulated in two-phase models [45]. Two-phase models have already been widely applied to submarine landslides [46,47,48,49,50,51,52], scour around coastal structures [53,54,55,56,57,58], and sheet flow sediment transport [59,60,61,62,63]. However, their applications to sediment transport in swash zones are limited due to the difficulties in accurately simulating the complicated hydrodynamic conditions, such as the violent water surface breaking and short flood-dry circles, as well as capturing the thin but intense sediment sheet flow under swashes. Bakhtyar et al. [64] adopted a two-phase flow model with the Reynolds-Averaged Navier–Stokes (RANS) equations for turbulence and the Volume of Fluid (VOF) approach for free surface tracking to simulate the sediment transport in inner-surf and swash zones. In their results, the maximum turbulent kinetic energy and sediment flux were observed near the wave-breaking point. Tofany and Lee [65] applied a two-phase model to investigate the sediment transport and bed evolution under plunging breakers. Despite these valuable contributions, further investigation is needed to fill knowledge gaps in two-phase dynamics, particularly in understanding sediment transport during swash–swash interactions. Therefore, the primary objective of this paper is to apply a two-phase model based on the Smoothed Particle Hydrodynamics (SPH) meshless numerical method to investigate sediment transport and short-term profile evolution in the swash zone of sandy beaches under successive waves.

2. Numerical Method and Validation

2.1. Governing Equations and Closures

In this study, the two-phase SPH model developed by Shi et al. [49] is adopted. The filtered continuity equations and momentum equations are represented by Equations (1) and (2), respectively,

$${\displaystyle \frac{\partial ({\alpha }_k{\rho }_k)}{\partial t}}+{\displaystyle \frac{\partial ({\alpha }_k{\rho }_k{u}_{k,i})}{\partial x_i}}=0,$$

(1)

$${\displaystyle \frac{\partial ({\alpha }_k{\rho }_k{u}_{k,i})}{\partial t}}+{\displaystyle \frac{\partial ({\alpha }_k{\rho }_k{u}_{k,i}{u}_{k,j})}{\partial x_j}}=-{\alpha }_k{\displaystyle \frac{\partial p_f}{\partial x_i}}+{\displaystyle \frac{\partial ({\alpha }_k{\sigma }_{k,ij})}{\partial x_j}}+{\alpha }_k{\rho }_k{g}_i+F_{k,i},$$

(2)

in which $t$ is the time and $x$ denotes the coordinate. $i,j=1,\hspace{0.33em}2,\hspace{0.33em}3$ represent the coordinate directions for which the summation convention is valid. The subscript $k=f, s$ represents the fluid phase and the sediment phase, respectively. $\alpha$, $\rho$, $\mathbf{g}$, $\mathbf{u}$, $p$, and $\mathsf{\sigma }$ are the volume fraction, density, gravitational acceleration, velocity, pressure, and inner-phase stress, respectively. $\mathbf{F}$ is the interphase force.

In the high-concentration sheet-flow layer, the inter-sediment stress results from collisions and frictional enduring contact between sediment particles, expressed in Equation (3) as

$${\sigma }_{s,ij}=-p_s{\delta }_{ij}+{\tau }_{s,ij}^0+{\tau }_{s,ij}^t,$$

(3)

where $p_s$ is the inter-sediment pressure and estimated by Equation (4) as

$$\begin{array}{cc}\hfill p_s& =K{\left({\alpha }_s-{\alpha }_{*}\right)}^{\chi }\left[1+\sin \left({\displaystyle \frac{{\alpha }_s-{\alpha }_{*}}{{\alpha }^{\ast }-{\alpha }_{*}}}\pi -{\displaystyle \frac{\pi }{2}}\right)\right]\hfill \\ & +{\left({\displaystyle \frac{c_1{\alpha }_s}{{\alpha }_0-{\alpha }_s}}\right)}^2\left({\rho }_f{\nu }_f^0+c_2{\rho }_s{d}_s^2\left|{\mathbf{S}}_s\right|\right)\left|{\mathbf{S}}_s\right|\hfill \end{array};$$

(4)

${\mathsf{\tau }}_s^0$ is the sediment shear stress due to inter-sediment collisions and contacts, fulfilling the relation in Equation (5) as

$${\tau }_{s,ij}^0={\displaystyle \frac{\mu p_s}{\left|{\mathbf{S}}_s\right|}}\left(2S_{s,ij}-{\displaystyle \frac{2}{3}}{S}_{s,ll}{\delta }_{ij}\right);$$

(5)

${\mathsf{\tau }}_s^t$ is the stress of the sediment phase induced by fluid turbulence and quantified by Equation (6) as

$${\tau }_{s,ij}^t={\nu }_s^t\left(2S_{s,ij}-{\displaystyle \frac{2}{3}}{S}_{s,ll}{\delta }_{ij}\right);$$

(6)

${\mathbf{S}}_s$ is the strain-rate tensor of the sediment phase and $\left|{\mathbf{S}}_s\right|=\sqrt{2{\mathbf{S}}_s:{\mathbf{S}}_s}$ is its norm. $d_s$ is the sediment diameter, $K$ the coefficient related to Young’s modulus and Poisson’s ratio of sediment particles, ${\alpha }_{*}$ and ${\alpha }^{*}$, respectively, the random loose and close packing volumetric fractions of sediment particles and ${\alpha }_0$ the jamming fraction. $\mu$ is the friction coefficient between sediment particles and is estimated following the μ(K) law [49] by Equation (7) as

$$\mu ={\mu }_1+{\displaystyle \frac{{\mu }_2-{\mu }_1}{1+I_0{c}_1{\alpha }_s/\left({\alpha }_0-{\alpha }_s\right)}}.$$

(7)

${\mu }_1=\mathrm{t}\mathrm{a}\mathrm{n}\varphi$ is the static friction coefficient between sediment particles, and $\varphi$ is their internal friction angle. ${\mu }_2$ is the friction coefficient when sediment particles move extremely fast, and the volumetric fraction approaches zero. ${\nu }_f^0$ is the kinematic viscosity of water and ${\nu }_s^t$ is a sediment viscosity resulting from the turbulent motion. $\chi$, $c_1$, and $c_2$ are model coefficients. These model parameters are divided into two groups: physical material parameters and calibration coefficients, and their suggested ranges can be found in [49,52].

For the water, the stress ${\mathsf{\sigma }}_f$ consists of the viscous and the turbulent shear stresses, respectively symbolized by ${\mathsf{\tau }}_f^0$ and ${\mathsf{\tau }}_f^t$, and are calculated in Equation (8) as

$${\sigma }_{f,ij}={\tau }_{f,ij}^0+{\tau }_{f,ij}^t=2\left({\nu }_f^0+{\nu }_f^t\right)\left(S_{f,ij}-S_{f,ll}{\delta }_{ij}\right),$$

(8)

in which ${\nu }_f^t$ is the water’s turbulent viscosity and ${\mathbf{S}}_f$ is the water strain-rate tensor.

In the present two-phase SPH model, the turbulence is accounted for by adopting the Smagorinsky model, which is simple but has been widely applied to SPH models [66,67]. A slight modification is proposed considering turbulence damping by sediment [61], and the turbulent viscosities of both phases are estimated by Equation (9)

$${\nu }_k^t={\left(C_k\Delta \right)}^2\left|{\mathbf{S}}_k\right|{\left(1-{\displaystyle \frac{{\alpha }_s}{{\alpha }^{\ast }}}\right)}^5,$$

(9)

where $C_k$ are Smagorinsky coefficients, and $\Delta$ is the characteristic length of filtering and set to equal the size of SPH numerical particles.

The primary water–sediment inter-phase force $\mathbf{F}$ is the drag force [49], and in the present model, it is calculated by Equation (10) as

$$F_{s,i}=-F_{f,i}=\beta {\alpha }_s\left(u_{f,i}-u_{s,i}\right),$$

(10)

in which $\beta$ is a coefficient and estimated following the Gidaspow formula [49,68].

2.2. SPH Discretization

The governing equations are numerically discretized by a single-set particle SPH method. In the method, the water–sediment mixture is represented using a single set of SPH particles that move with the water velocity but carry information about the two phases. Accordingly, the substantial derivative of any variable $\Theta$ carried by SPH particles is defined in Equation (11) as

$${\displaystyle \frac{d\Theta }{dt}}\equiv {\displaystyle \frac{\partial \Theta }{\partial t}}+u_{f,i}{\displaystyle \frac{\partial \Theta }{\partial x_i}}.$$

(11)

Details of the method could be found in [49]. Here, for simplicity, only the final discretized governing equations are shown in Equations (12)–(15) as

$${\displaystyle \frac{d{\left.\left({\alpha }_f{\rho }_f\right)\right|}_a}{dt}}=-{\left.\left({\alpha }_f{\rho }_f\right)\right|}_a{\displaystyle \sum _b\left({\left.u_{f,i}\right|}_b-{\left.u_{f,i}\right|}_a\right){\left({\nabla }_a{W}_{ab}\right)}_i{V}_b},$$

(12)

$$\begin{array}{cc}\hfill {\displaystyle \frac{d{\left.{\alpha }_s\right|}_a}{dt}}& =-{\left.{\alpha }_s\right|}_a{\displaystyle \sum _b\left({\left.u_{f,i}\right|}_b-{\left.u_{f,i}\right|}_a\right){\left({\nabla }_a{W}_{ab}\right)}_i{V}_b}\hfill \\ & -{\displaystyle \sum _b{V}_b\left\{{\left.{\alpha }_s\right|}_a\max \right.\left[{\left.\left(u_{s,i}-u_{f,i}\right)\right|}_a{\left({\nabla }_a{W}_{ab}\right)}_i,\hspace{0.33em}0\right]}\hfill \\ & +{\left.{\alpha }_s\right|}_a\max \left[{\left.\left(u_{s,i}-u_{f,i}\right)\right|}_b{\left({\nabla }_a{W}_{ab}\right)}_i,\hspace{0.33em}0\right]\hfill \\ & +{\left.{\alpha }_s\right|}_b\min \left[{\left.\left(u_{s,i}-u_{f,i}\right)\right|}_a{\left({\nabla }_a{W}_{ab}\right)}_i,\hspace{0.33em}0\right]\hfill \\ & \left.+{\left.{\alpha }_s\right|}_b\min \left[{\left.\left(u_{s,i}-u_{f,i}\right)\right|}_b{\left({\nabla }_a{W}_{ab}\right)}_i,\hspace{0.33em}0\right]\right\}\hfill \end{array},$$

(13)

$$\begin{array}{cc}\hfill {\displaystyle \frac{d{\left.u_{f,i}\right|}_a}{dt}}& =-{\displaystyle \frac{1}{{\rho }_{f0}}}{\displaystyle \sum _b\left({\left.p_f\right|}_a+{\left.p_f\right|}_b\right){\left({\nabla }_a{W}_{ab}\right)}_i{V}_b}\hfill \\ & +{\displaystyle \sum _b\left({\left.\frac{{\sigma }_{f,ij}}{{\rho }_f}\right|}_a+{\left.\frac{{\sigma }_{f,ij}}{{\rho }_f}\right|}_b\right)\left[1+\frac{1}{2}\ln \frac{{\left.\left({\alpha }_f{\rho }_f\right)\right|}_b}{{\left.\left({\alpha }_f{\rho }_f\right)\right|}_a}\right]{\left({\nabla }_a{W}_{ab}\right)}_j{V}_b}\hfill \\ & +g_i-{\left.\left[{\displaystyle \frac{\beta {\alpha }_s}{{\alpha }_f{\rho }_f}}\left(u_{f,i}-u_{s,i}\right)\right]\right|}_a\hfill \end{array},$$

(14)

$$\begin{array}{cc}\hfill {\displaystyle \frac{d{\left.u_{s,i}\right|}_a}{dt}}& =-{\displaystyle \frac{1}{{\rho }_s}}{\displaystyle \sum _b\left({\left.p_f\right|}_a+{\left.p_f\right|}_b\right){\left({\nabla }_a{W}_{ab}\right)}_i{V}_b}\hfill \\ & +{\displaystyle \sum _b\left({\left.\frac{{\sigma }_{s,ij}}{{\rho }_s}\right|}_a+{\left.\frac{{\sigma }_{s,ij}}{{\rho }_s}\right|}_b\right)\left[1+\frac{1}{2}\ln \frac{{\left.{\alpha }_s\right|}_b}{{\left.{\alpha }_s\right|}_a}\right]{\left({\nabla }_a{W}_{ab}\right)}_j{V}_b}+g_i+{\left.\left[{\displaystyle \frac{\beta }{{\rho }_s}}\left(u_{f,i}-u_{s,i}\right)\right]\right|}_a\hfill \\ & +{\displaystyle \sum _b\left({\left.u_{s,i}\right|}_a-{\left.u_{s,i}\right|}_b\right)\left\{\min \left[{\left.\left(u_{s,j}-u_{f,j}\right)\right|}_a{\left({\nabla }_a{W}_{ab}\right)}_j,\hspace{0.33em}0\right]\right.}\hfill \\ & \left.+\min \left[{\left.\left(u_{s,j}-u_{f,j}\right)\right|}_b{\left({\nabla }_a{W}_{ab}\right)}_j,\hspace{0.33em}0\right]\right\}{V}_b\hfill \end{array},$$

(15)

in which ${\left.\Theta \right|}_a$ represents the value of any variable $\Theta$ carried by SPH numerical particle a and ${\left.\Theta \right|}_b$ is that by the neighboring particle b. $V_b$ is the volume of the neighboring particle b. ${\nabla }_a{W}_{ab}=\left(\partial W/\partial r\right)\left({\mathbf{x}}_a-{\mathbf{x}}_b\right)/\left|{\mathbf{x}}_a-{\mathbf{x}}_b\right|$ and ${\left({\nabla }_a{W}_{ab}\right)}_i$ is the i-component of ${\nabla }_a{W}_{ab}$, where ${\mathbf{x}}_a$ and ${\mathbf{x}}_b$ are the positions of SPH particles, $W\left(r,h\right)=W(\left|{\mathbf{x}}_a-{\mathbf{x}}_b\right|,h)$ the kernel function, and h is the smoothing length of the kernel function. In this study, the quintic kernel function is adopted [69]. Additionally, in the model, the water is assumed to be weakly compressible, with the water pressure calculated by Equation (16) shown as

$${\left.p_f\right|}_a={\displaystyle \frac{{\rho }_{f0}{c}_0^2}{\xi }}{\left.{\displaystyle \frac{{\alpha }_f{\rho }_f+{\alpha }_s{\rho }_{f0}}{{\alpha }_f{\rho }_f}}\right|}_a\left\{{\left[{\displaystyle \frac{{\left.\left({\alpha }_f{\rho }_f\right)\right|}_a+{\left.{\alpha }_s\right|}_a{\rho }_{f0}}{{\rho }_{f0}}}\right]}^{\xi }-1\right\},$$

(16)

where ${\rho }_{f0}$ is the water density and $c_0$ the numerical speed of sound in water when $p_f=0 \mathrm{P}\mathrm{a}$. $\xi$ is a coefficient quantifying the compressibility of water, and $\xi =7$ is set in the present study.

The predictor-corrector scheme proposed by Monaghan [70] is employed to integrate the equations in time. The time step of the model is restricted by the numerical sound speed, the maximum inertial forces, and the viscous forces of the two phases through the CFL conditions [69] as in Equation (17)

$$\Delta t=\min (\Delta t_c,\Delta t_F,\Delta t_{\nu }).$$

(17)

The Shepard filtering technique for fluid–solid mixtures proposed by Shi et al. [69] is adopted to dampen the numerical oscillation of pressure.

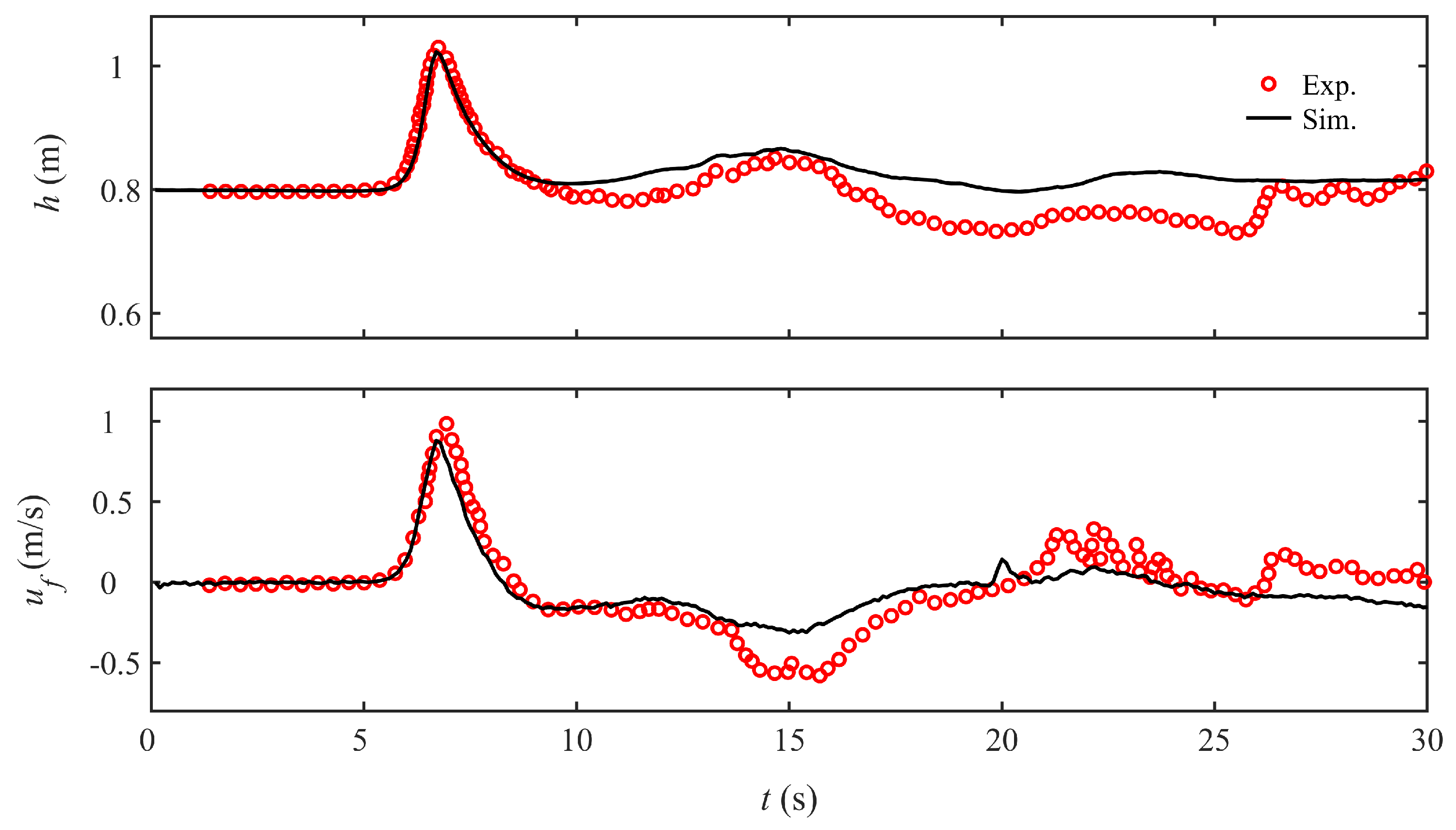
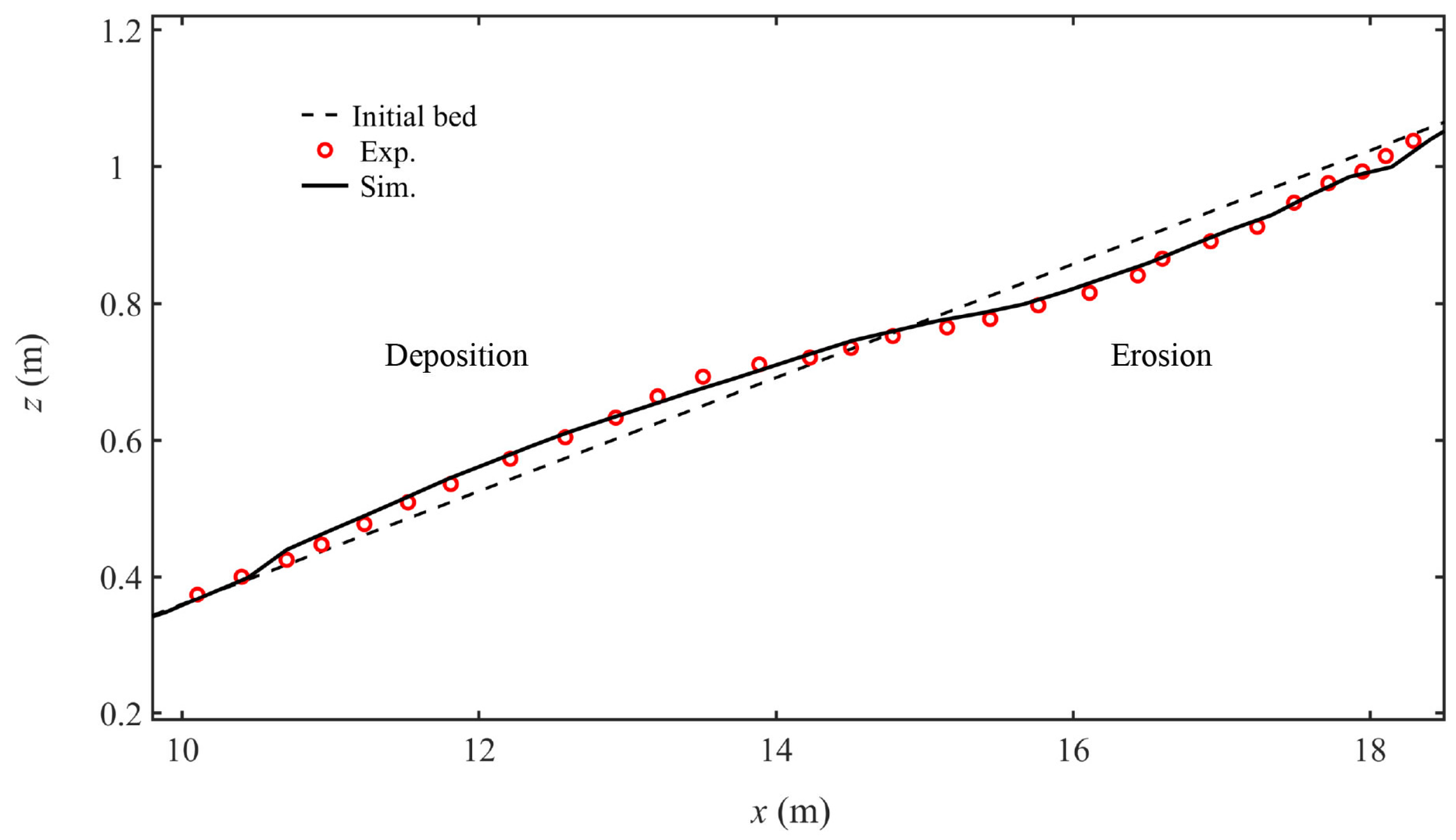
2.3. Model Validation

The model is validated in simulating the experiment of cross-shore sediment transport under breaking solitary waves conducted by Kobayashi and Lawrence [16]. The experiment was conducted in a wave flume of 30 m long, 2.4 m wide, and 1.5 m high. The solitary wave was generated by a piston-type wavemaker at the end of the flume with an initial water depth of 0.8 m. The positive solitary wave case of $H_0=0.216 \mathrm{m}$ was reproduced here. Initially, the movable sandy beach was prepared with a slope of 1:12 composed of well-sorted sand. Figure 1 sketches the experimental setup. The median diameter of the sand was 0.18 mm. The measured specific gravity and fall velocity of the sand were 2.6 and 2.0 cm/s, respectively. The internal friction angle of sediment particles was about 35°. The initial porosity of the beach slope was 0.40.

The experiment can be taken as two-dimensional [16], and the periodic boundary condition is applied to the flume width direction. In the model implementation, four layers of SPH numerical particles are arranged along the flume width to ensure the completeness of the kernel domain. Solid flume walls are dealt with utilizing the dynamic boundary condition, in which they are represented by three layers of fixed SPH numerical particles. The wavemaker is also represented by three layers of SPH particles, but those particles move following the defined trajectory of the wavemaker in the experiment [16]. The initial size of SPH numerical particles is $∆=0.01 \mathrm{m}$ following a sensitivity study. The total number of adopted SPH particles is 779,796. The values of both material parameters and coefficients in the model adopted in the simulations are listed in

Table 1. Model parameters used in this study.

${\mathit{C}}_{\mathit{f}}$	${\mathit{C}}_{\mathit{s}}$	${\mathit{\alpha }}^{*}$	${\mathit{\alpha }}_{*}$	${\mathit{\alpha }}_0$	$\mathit{K}$	${\mathit{\mu }}_2$	${\mathit{c}}_1$	${\mathit{c}}_2$	$\mathit{\chi }$	${\mathit{I}}_0$
0.2	0.2	0.62	0.52	0.61	109 Pa	0.9	1.0	0.1	5.0	0.1

.

The model is validated regarding the temporal variations of water depth and near-bed flow velocity on the sandy slope as well as the final beach profile after the solitary-wave swash event. In Figure 2, the simulated depth and near-bed horizontal flow velocity at $x=11.51 \mathrm{m}$ on the slope generally agreed well with the measured data. The RMSE values in the computed depth and velocity are 0.04 m and 0.13 m/s, respectively. The instants of the peak values, when the solitary wave arrived at the measurement point, are accurately captured by the model. The relative error in the computed peak water depth is −1.0% and that in the peak near-bed velocity is −6.4%.

In Figure 3, the simulated and measured beach profiles after the solitary wave swash event are compared. In the numerical results, the profile of the sandy beach is defined by the contour of ${\alpha }_s=0.30$. The agreement between the computed results and the experimental data is very good, with a RMSE of 0.01 m. Both the erosion of sandy slopes and the deposition of sediment are well captured by the present model, demonstrating its capability to describe hydrodynamics and sediment transport in swash zones under solitary waves.

3. Hydrodynamics and Sediment Transport under Two-Successive Solitary Waves

The validated model is here applied to investigate hydrodynamics and sediment transport under swash–swash interaction, especially the effects of interval periods between successive solitary waves.

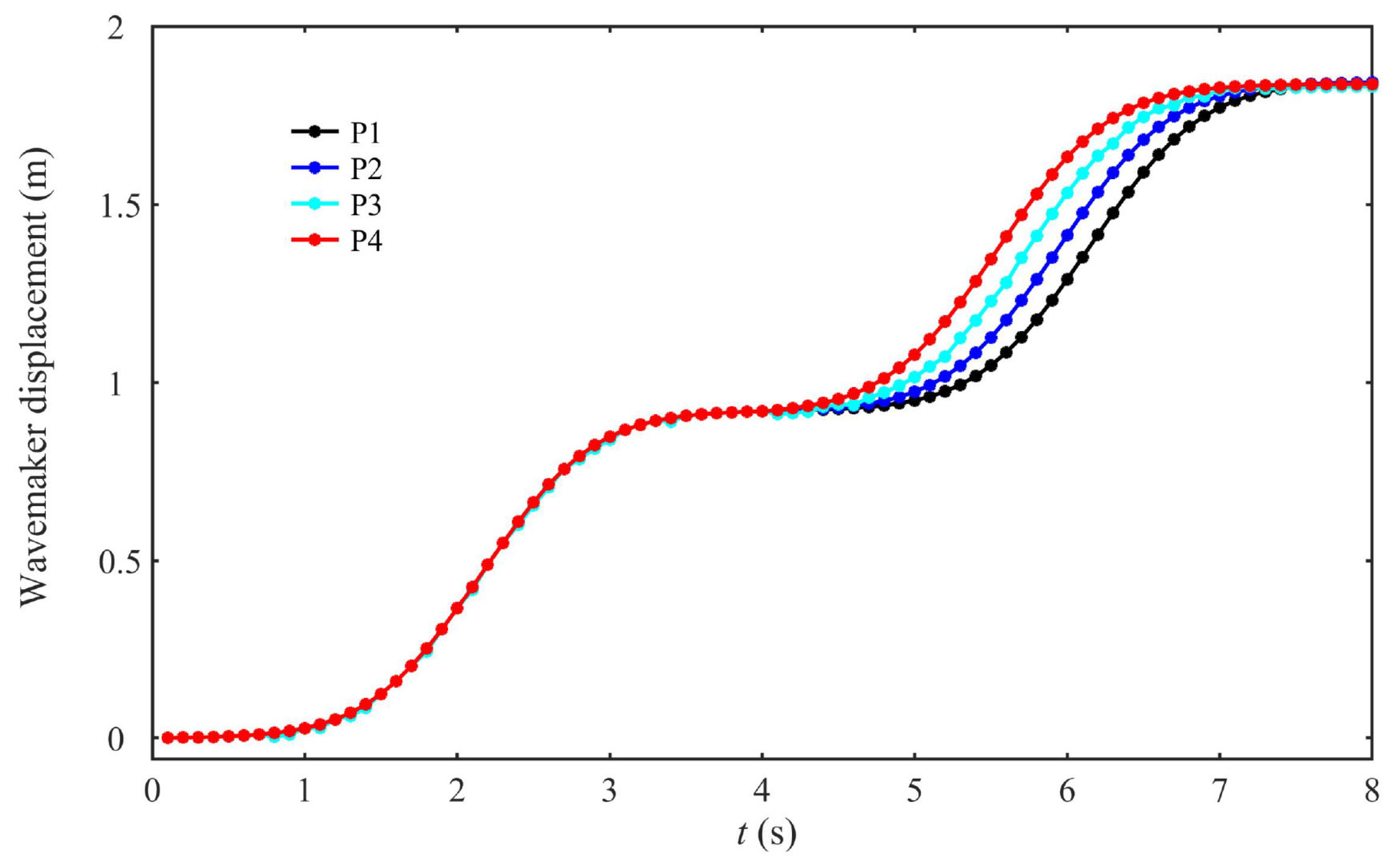
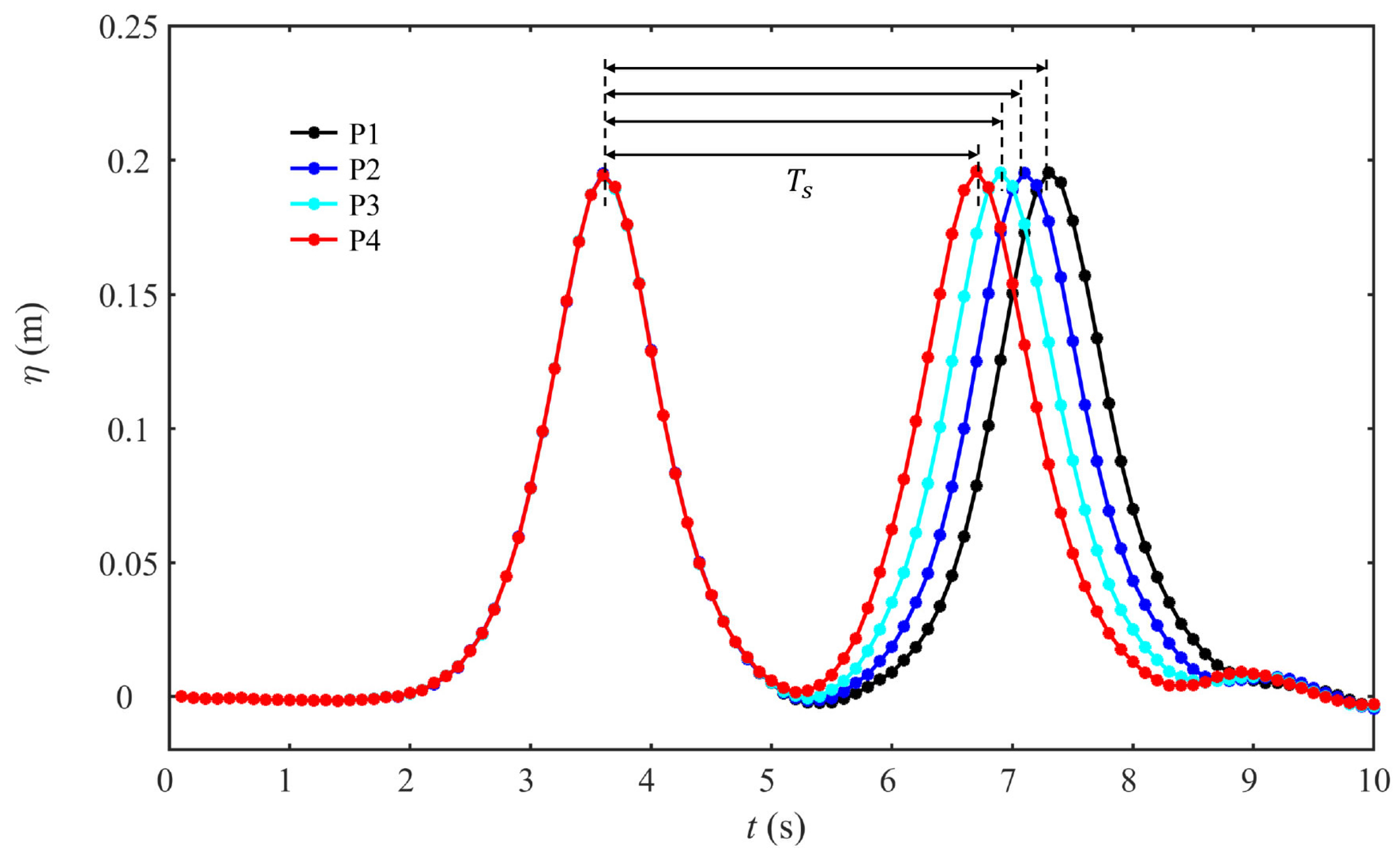
3.1. Setup of Numerical Experiments

There were no laboratory experiments on sediment transport and the evolution of sandy beaches under two successive solitary waves. Here, we extend the numerical experiment in Section 2.3 by making two successive solitary waves. The setup of the numerical cases is sketched in Figure 4. The numerical flume has been extended by 6 m, but the sandy slope is kept the same. The median diameter of the sand is still 0.18 mm. Accordingly, the total number of SPH numerical particles adopted is enlarged to be 1,356,828 with the same initial size of 0.01 m.

Two successive solitary waves of the same height of $H_0=0.2 \mathrm{m}$ are made following the method of Goring [71], similar to the laboratory experiment. The time interval between the peak of the two generated solitary waves is $T_s$. Four cases are set with different values of the time interval, i.e., $T_s=3.7 \mathrm{s}$ in Case P1, $T_s=3.5 \mathrm{s}$ in P2, $T_s=3.3 \mathrm{s}$ in P3, and $T_s=3.1 \mathrm{s}$ in P4. The trajectories of the wavemaker in the four cases are shown in Figure 5. The generated waves, quantified by the temporal variations of free surface displacement above the initial elevation at $x=5.0 \mathrm{m}$, are illustrated in Figure 6.

3.2. Swash–Swash Interaction and Sediment Suspension

It is shown in the results that the patterns of water flow and sediment transport in the four cases are similar, and therefore, mainly the results in Case P1 are illustrated in the figures below.

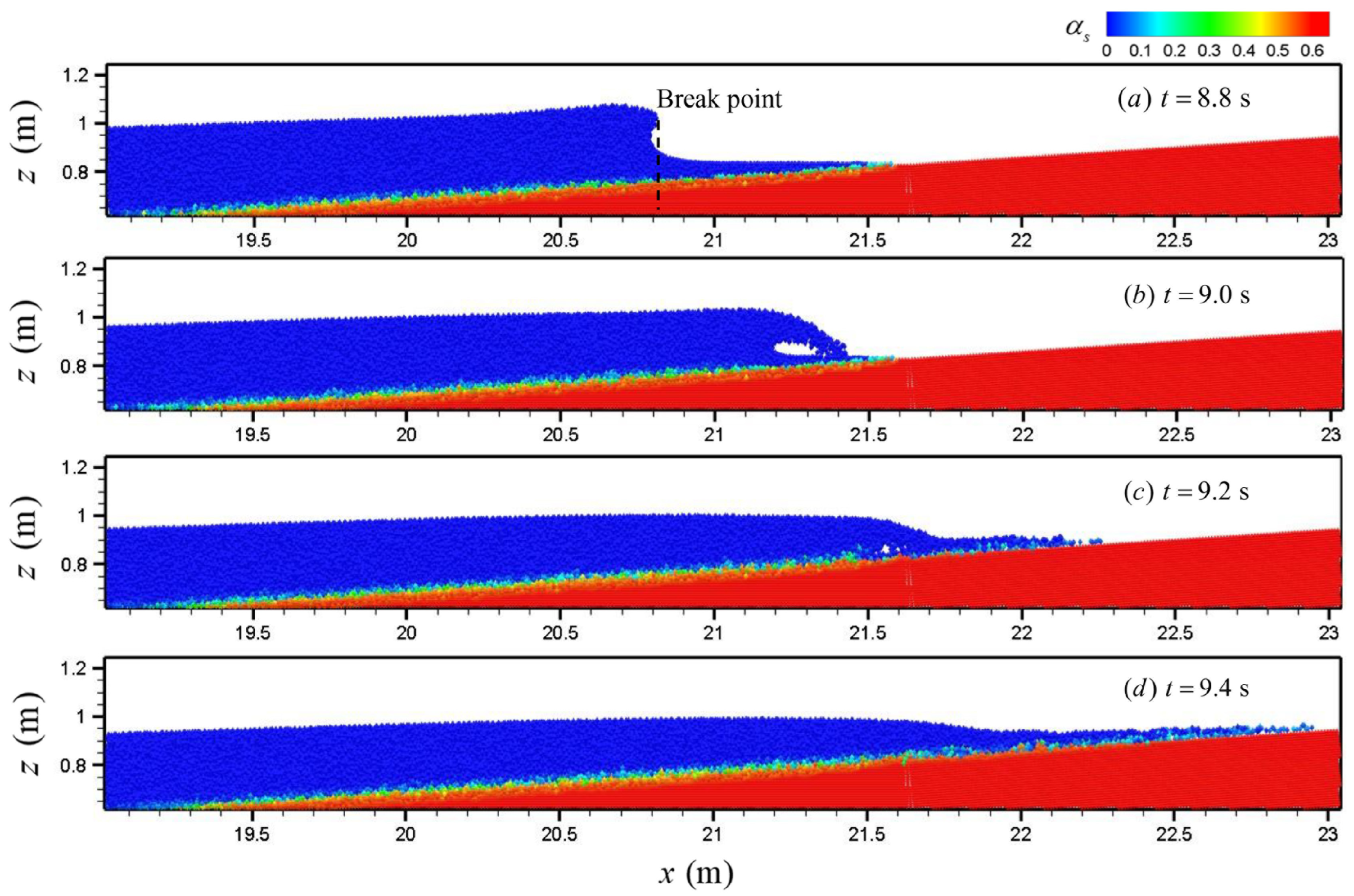
3.2.1. Breaking of the Preceding Wave

Figure 7 shows the snapshots of SPH particle distribution as well as their carried sediment concentration during the breaking of the preceding wave. At about $t=8.8 \mathrm{s}$, the wave starts to break with a forward plunging jet of water forming. The plunging jet impacts the sandy slope and then changes to an uprush current in the swash zone. Along the sandy slope upwards from the toe, there is sediment suspension under the turbulent flow, but no significant bed form is found.

The distance between the two successive waves is far from enough, and the second wave has almost no effect on the breaking of the preceding wave. In all four cases, the breaking point and instant of the first wave are almost the same.

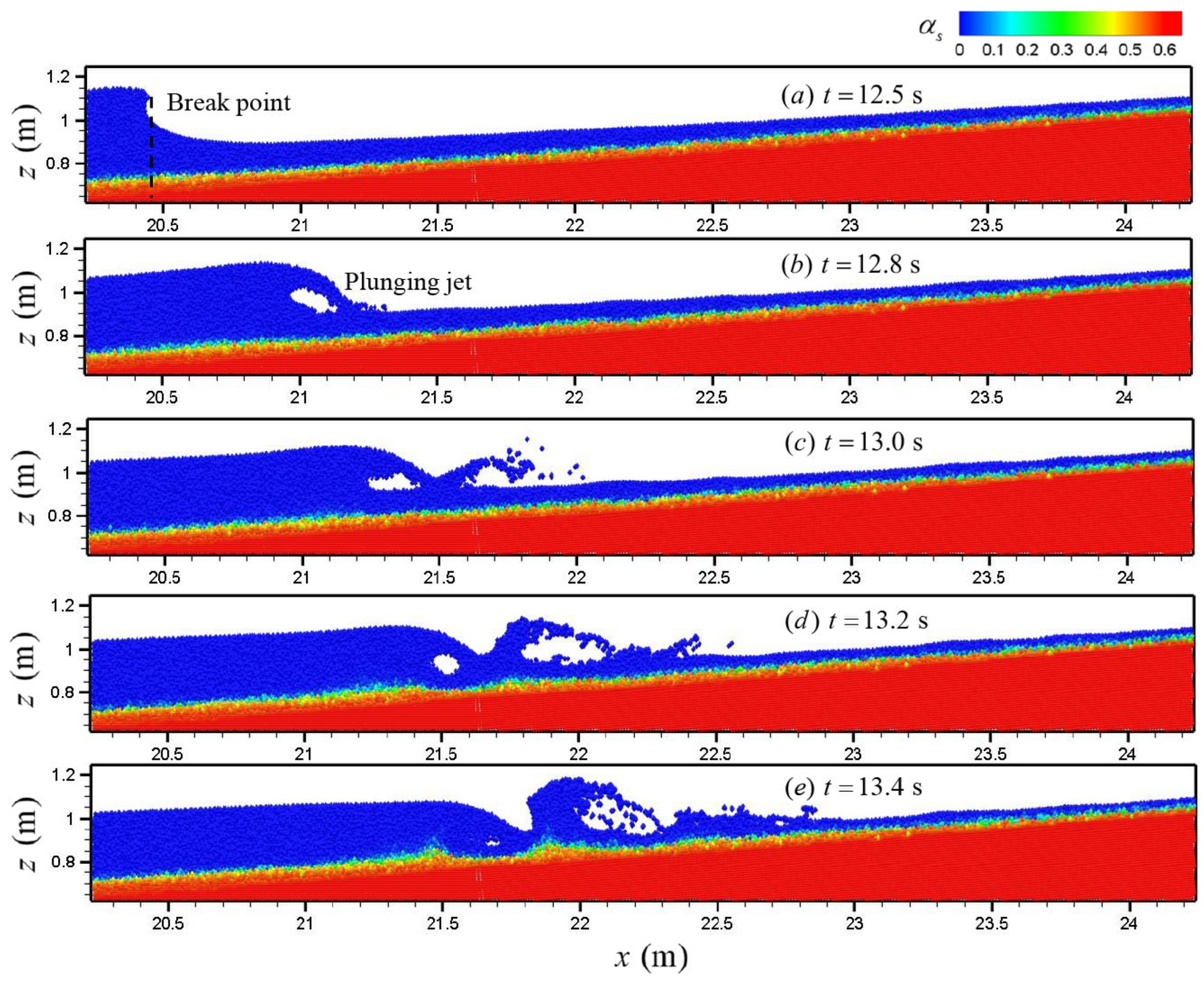
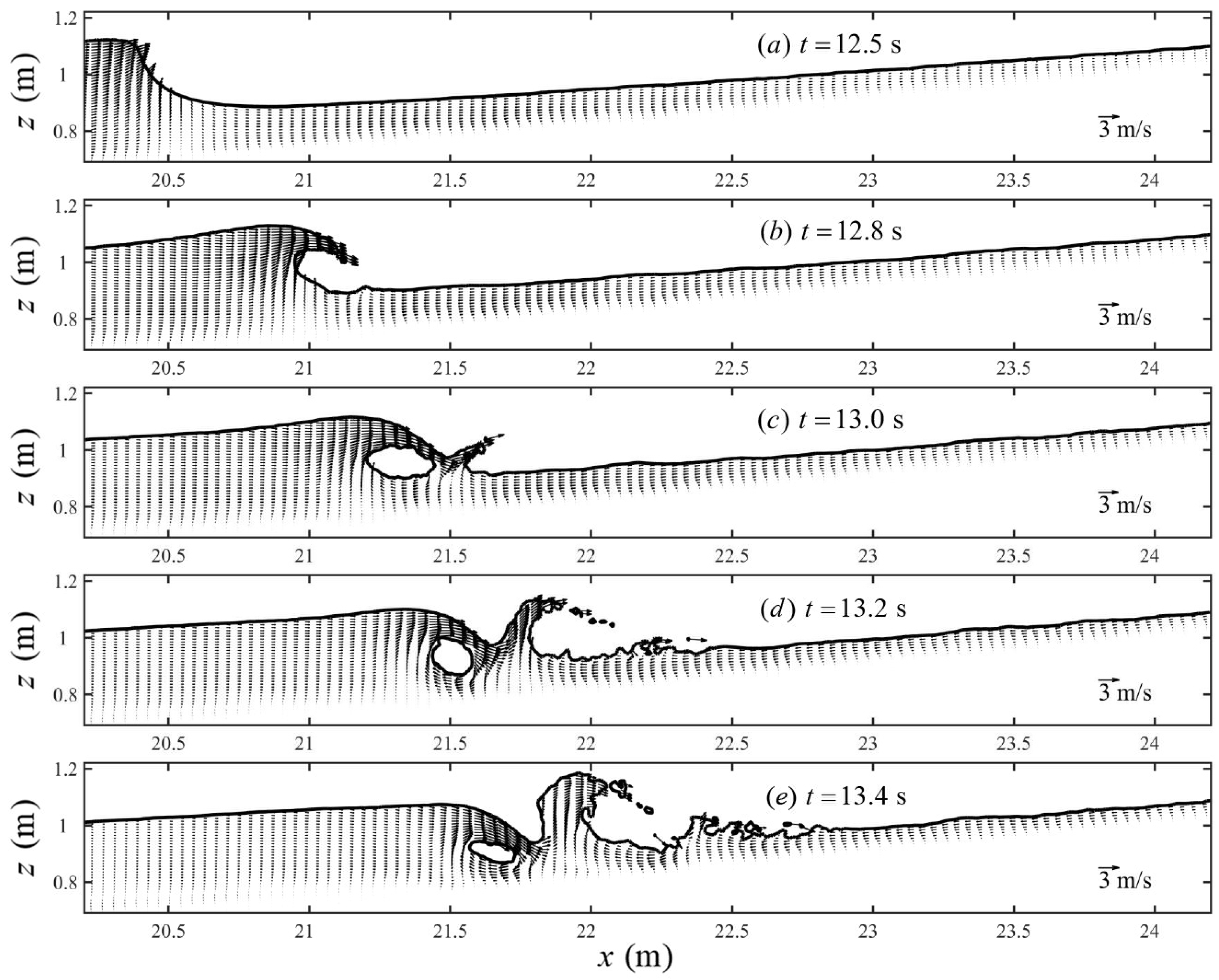
3.2.2. Breaking of the Second Wave

In Case P1, at $t=12.5 \mathrm{s}$, the second wave comes to the critical point of breaking and encounters the backwash of the preceding swash event, as shown in Figure 8a and Figure 9a. The vertical distributions of offshore fluid velocity in the backwash behind $x=21.0 \mathrm{m}$ are similar, firstly increasing linearly upwards from the bed and then generally being uniform until the free surface. The plunging jet forming in the breaking of the second wave impacts the backwashing water and then splashes out with a new upward-forward jet generated, as shown in Figure 8b,c. It is quite different from the breaking of the preceding wave, as shown in Figure 7b,c, where the breaking plunging jet impacts the sandy bed without the second splashing jet. The existence of the backwash is critical to the difference and reduces the energy dissipation during the impact of the plunging jet.

Another interesting phenomenon induced by swash–swash interaction is the tilting backward and thickening of the splashing jet, illustrated in Figure 8e. The splashing jet generated from the impact of the breaking plunge initially has a high forward speed, as shown in Figure 9c,d. However, the onshore propagation of the jet is slower than the plunge behind, and it thickens as more water catches up. The onshore water velocity in the back of the jet reduces almost to zero, and a backward jump seems to form. This phenomenon is highly related to the backwash flow. In Figure 9d,e, below the splashing jet, the flow velocity in the lower part of the water is offshore due to the backwash. The offshore backwash slows down the onshore propagation of the jet. Moreover, the lower offshore backwash clashes with the onshore-downward plunging jet, pushing more water upwards into the splashing jet and thickening it.

Interaction between the upper onshore-moving second wave and the lower offshore backwash flow leads to macroscale vortices. In Figure 9e, at both the offshore and onshore sides of the breaking-plunge impact point on the flow, there is a significant vortex. On the offshore side, the plunging jet and the lower backwash flow form a clockwise vortex, which leads to the bed erosion between $x= 21.5 \mathrm{and} 21.9 \mathrm{m}$ and sediment suspension at $x=21.5 \mathrm{m}$, as shown in Figure 8e. On the onshore side, the clockwise vortex formed by the splashing jet and the backwash flow leads to sediment suspension and generation of bed form around $x=22.0 \mathrm{m}$.

In the present four cases with varying time intervals between the two successive solitary waves, the breaking patterns of the second wave are quite similar. The locations where the second wave breaks are all near $x=20.45 \mathrm{m}$, and the corresponding instants delay with the time interval. The break instants in Cases P2, P3, and P4 are 12.3 s, 12.1 s, and 11.9 s, respectively.

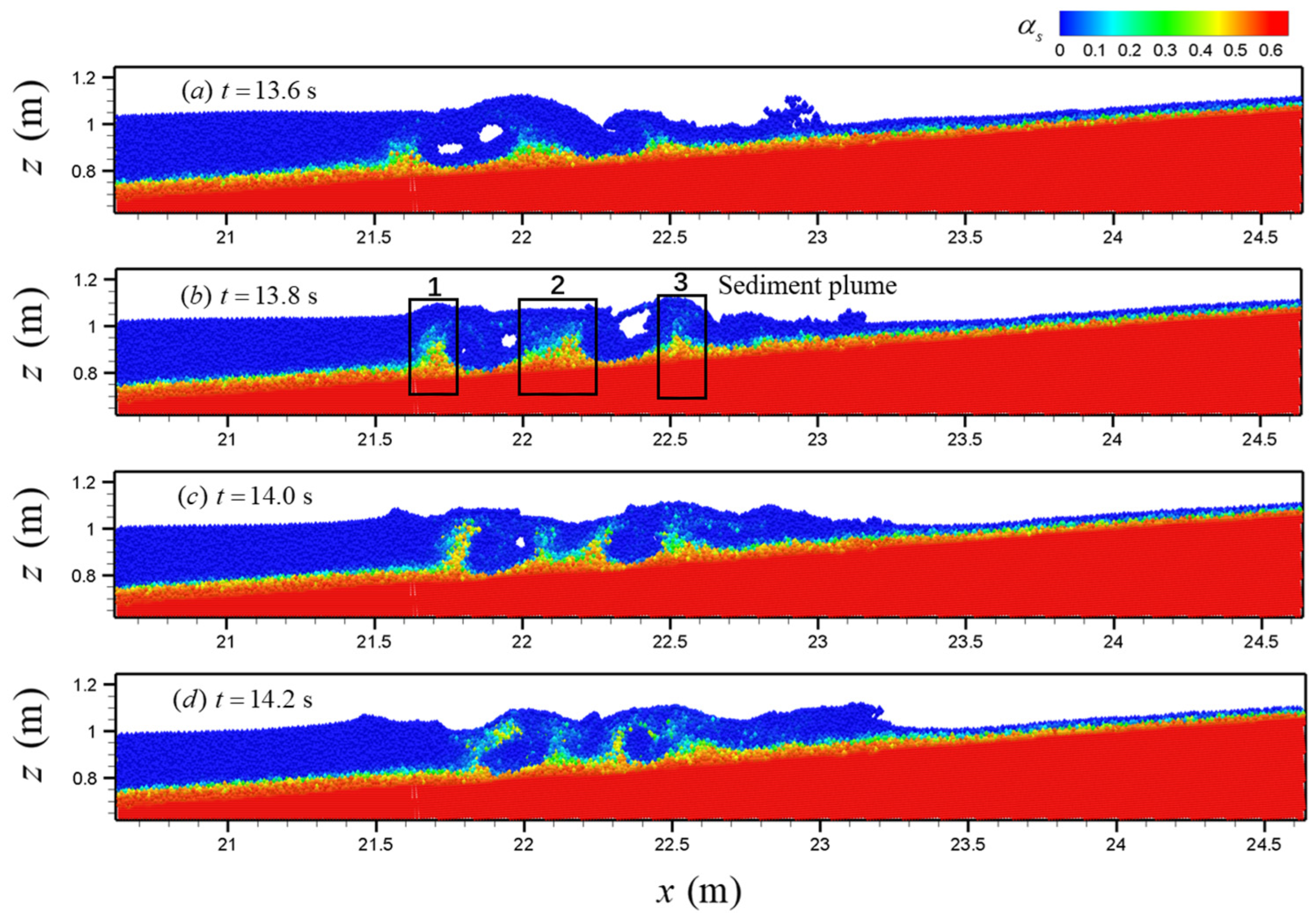
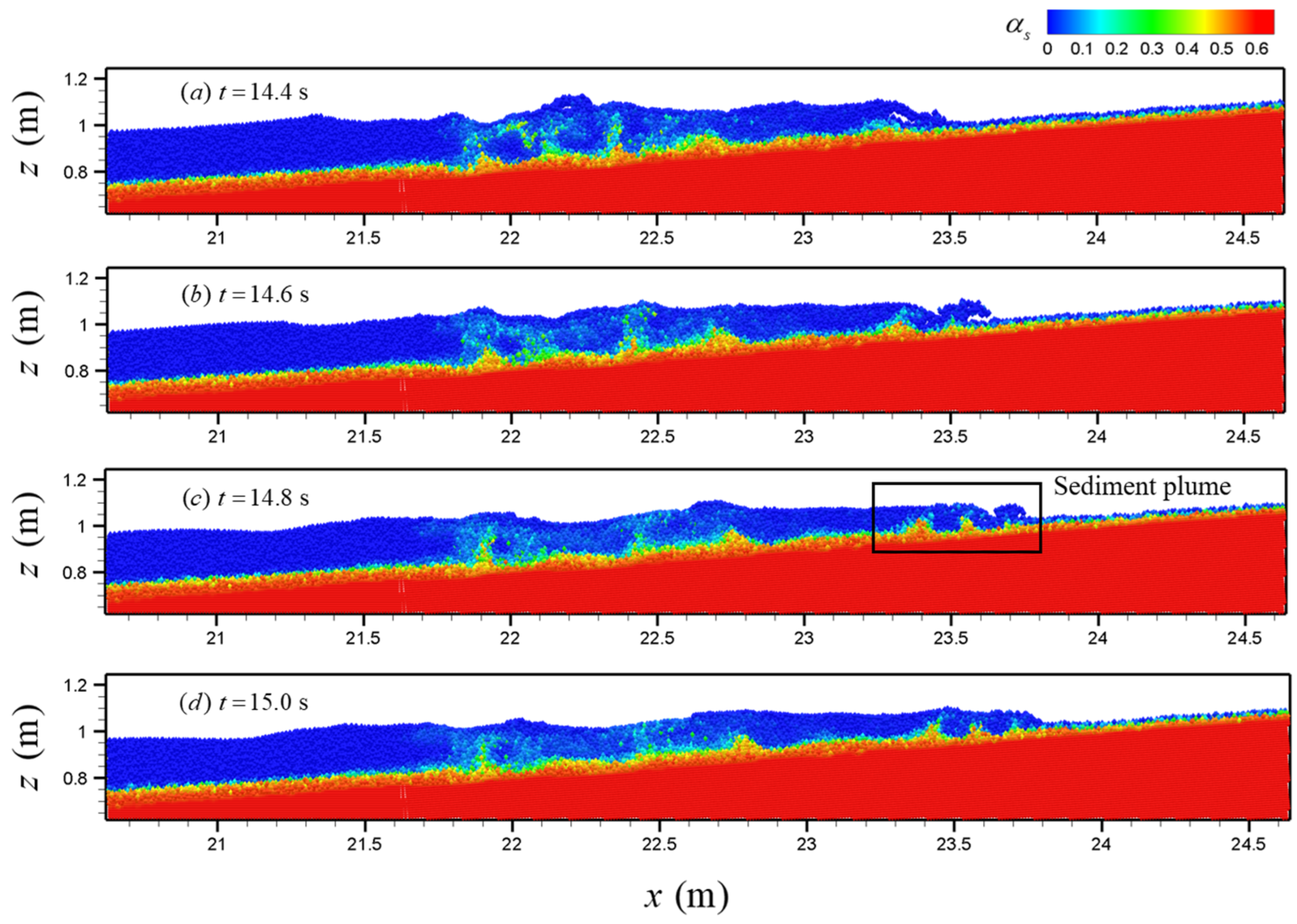
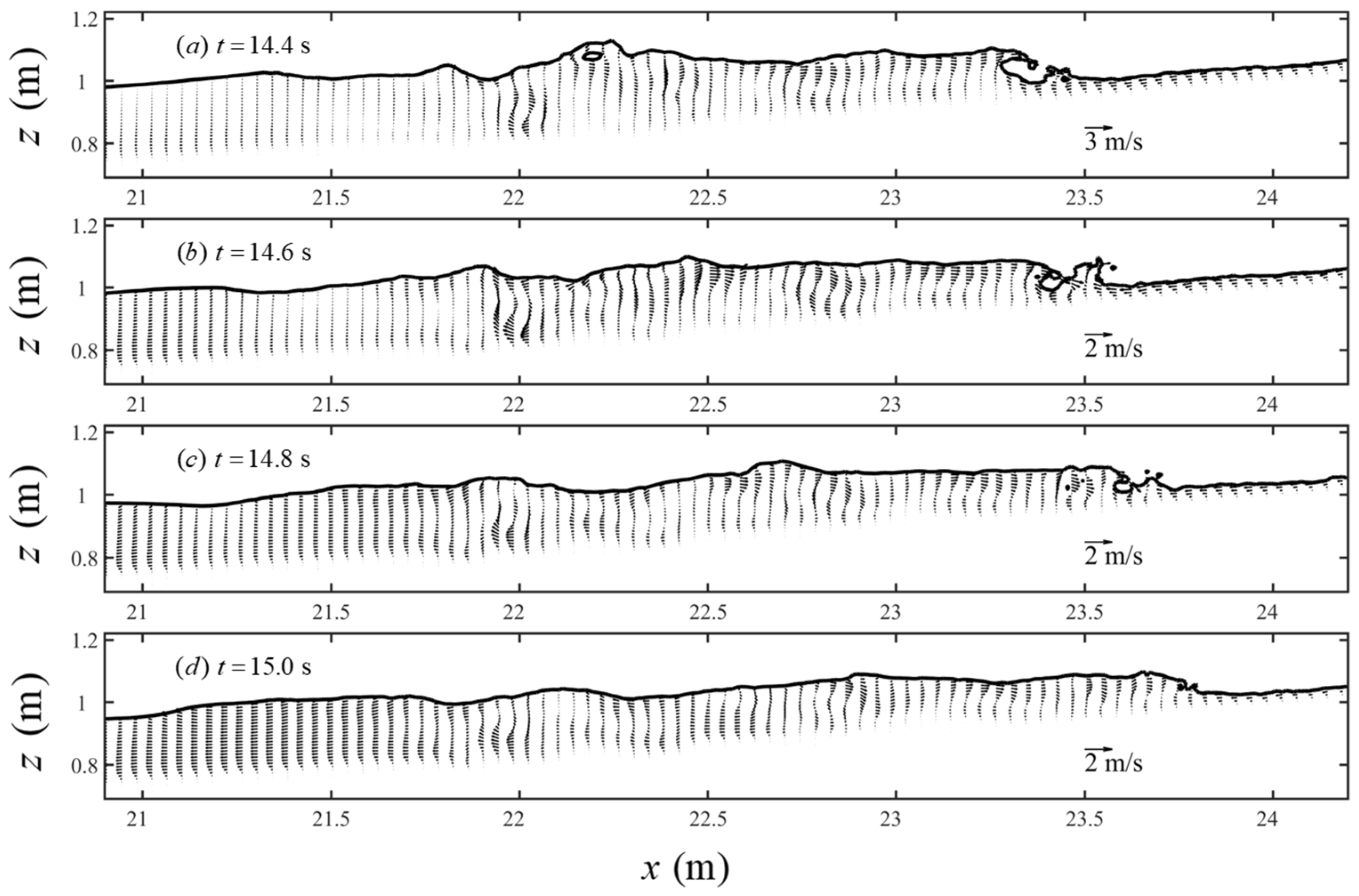
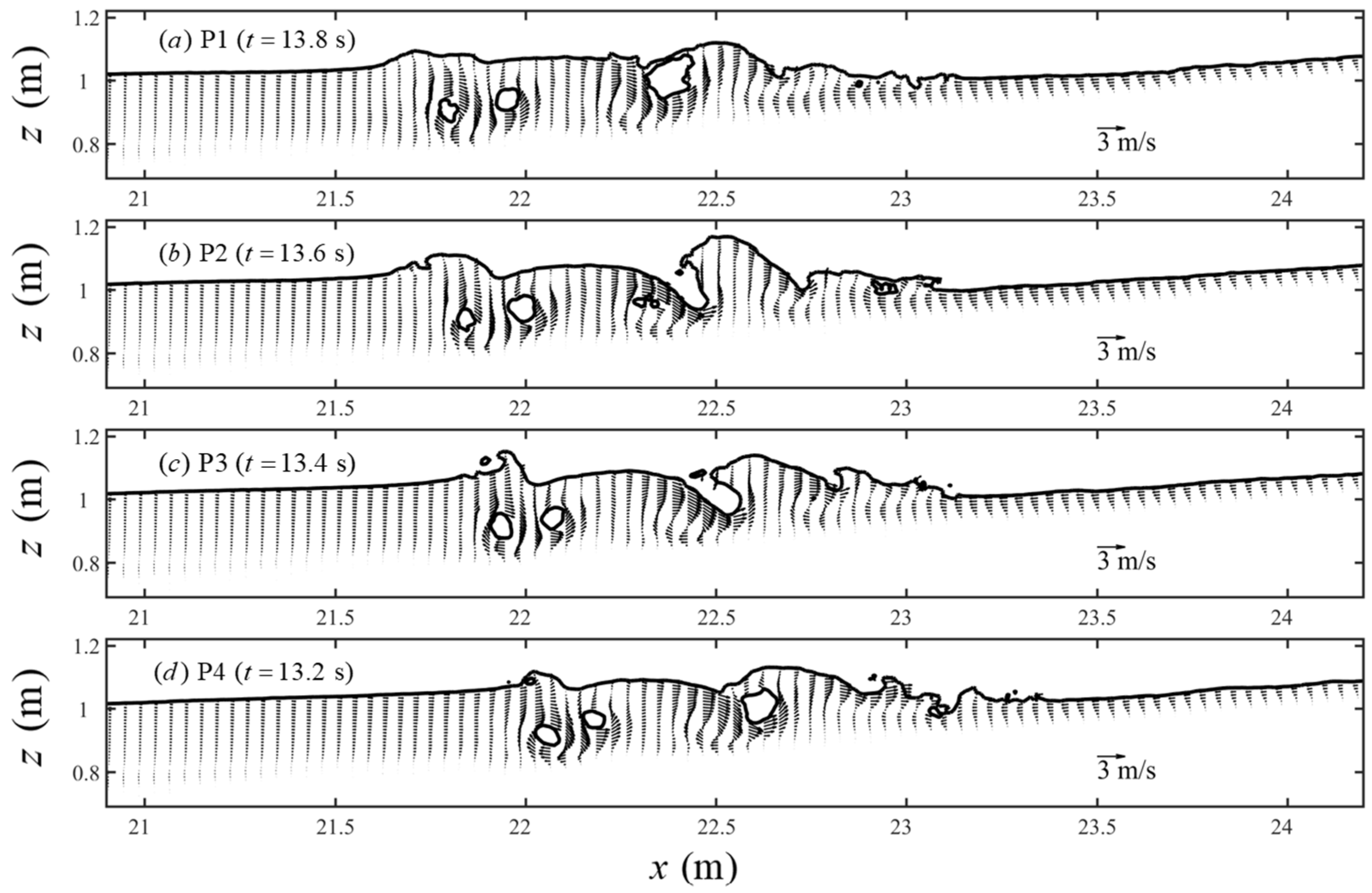
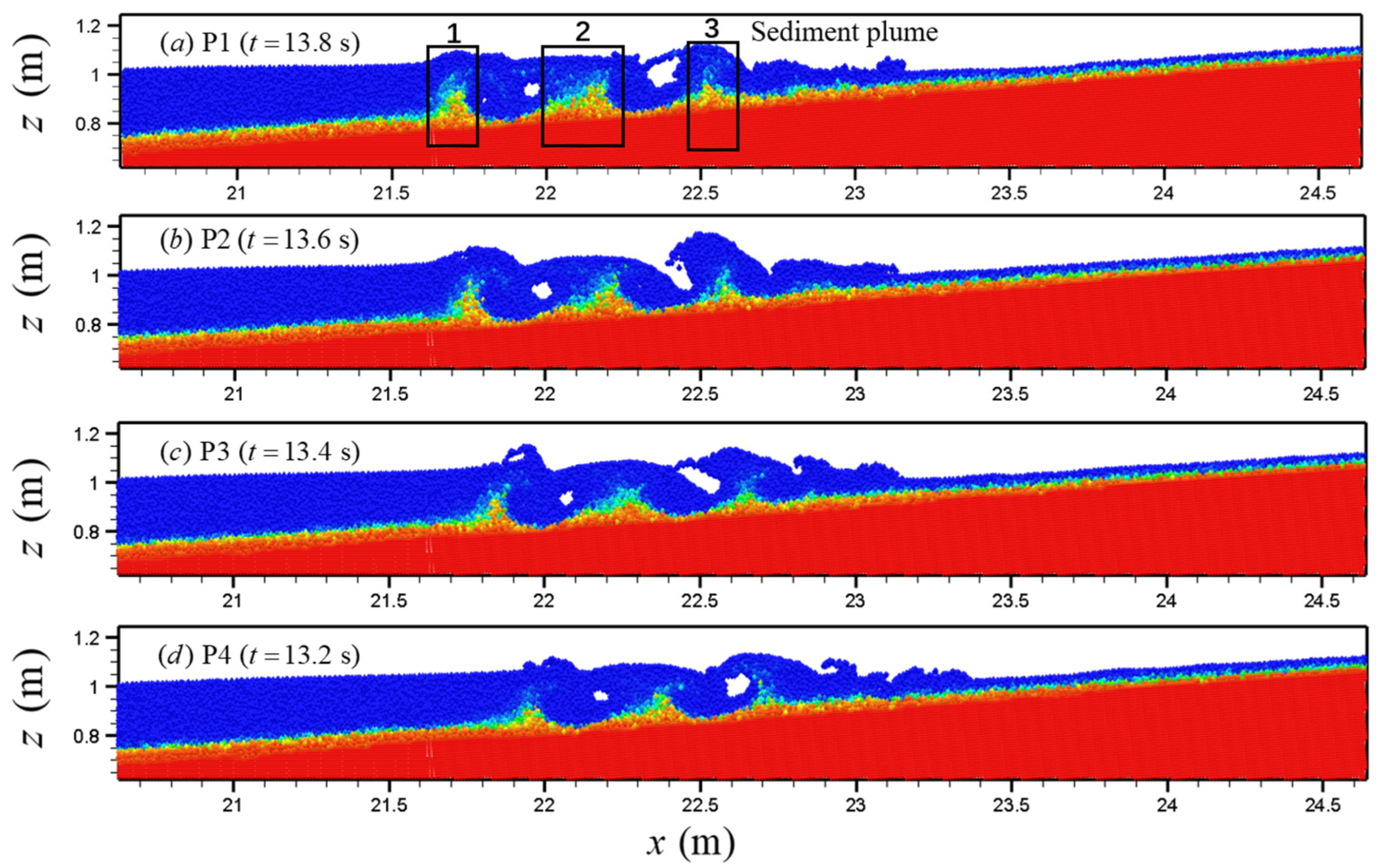
3.2.3. Generation of Sediment Plumes

Three notable plumes of the fine sand, whose median diameter is 0.18 mm, are observed after the breaking of the second wave, as depicted in Figure 10b. These sediment plumes are closely related to vortices generated from wave breaking and swash–swash interactions.

• First sediment plume: It is carried by the vortex that is generated due to the collision between the onshore-moving current under the second wave and the backwash flow of the first swash as well as the backward jet from the impact of the second wave plunge on the flow. On the onshore side of the plume, there is significant bed erosion in the region between $x=21.8 \mathrm{m}$ and $x=22.0 \mathrm{m},$ as shown in Figure 10b. The lower backwash flow carries the sediment downslope and moves upwards when encountering the onshore flow under the second wave.
• Second sediment plume: This plume follows the vortex generated by the splashing jet and the lower backwash current. The splashing jet from the impact of the second-wave breaking plunge on the backwash current rushes upwards and onshore and then jumps down back to the backwash current. The lower backwash takes sediment downslopes and turns upwards when colliding with the upward-onshore splashing jet, also leading to bed erosion before the plume, as observed in the region of $x=22.2~22.4 \mathrm{m}$ in Figure 10b.
• Third sediment plume: The splashing jet jumps down and encounters the backwash current, leading to the formation of the third vortex in Figure 11b. Similarly, the onshore splashing jet impedes the downslope motion of the backwash current as well as the carried sediment and pushes it up.
• It is noted that all the plumes are on the offshore side of the vortices, and there is notable bed erosion on the onshore side of the plumes.

3.2.4. Rolling Bores at the Swash Front

The bores resulted from the breaking of the second wave propagate onshore and further break at the swash front. Sediment plumes also form behind the swash front but on a smaller scale compared with the above-mentioned ones, as shown in Figure 12b–d. Figure 13 shows the distributions of water velocity above the sandy beach during the corresponding period. At $t=14.4 \mathrm{s}$, the upper-layer water velocity in a wide region from about $x=22.0 \mathrm{m}$ to the flow front is still onshore while the lower layer is offshore. Behind the region ($x=21.0~22.0 \mathrm{m}$ in Figure 13a), the water velocity in the upper layer of the water column starts to turn from onshore to offshore, and the backwash emerges. The backwash develops onshore from the slope toe, and, in Figure 13d, the water before about $x=22.7 \mathrm{m}$ all flows offshore.

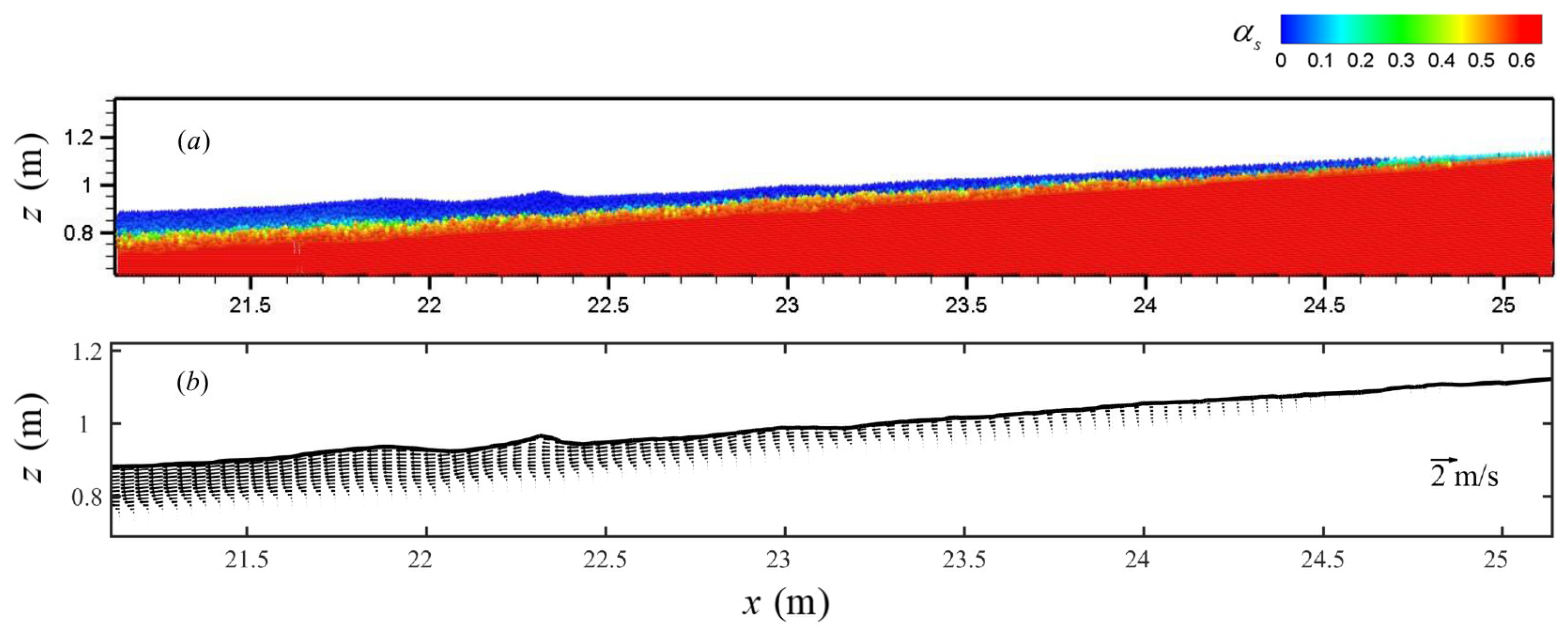
3.2.5. Run-Up and Settling of Sediment Plumes

Figure 14 shows the distributions of SPH particle position and their carried sediment concentration, as well as the flow velocity when the swash front reaches the maximum run-up. At this instant, the water flow in the whole region is backwash. At each section, the offshore flow velocity first increases almost linearly upwards and then keeps uniform until the free surface. There are no notable macroscale vortices anymore. Consequently, the bed forms of the sandy beach are swept out by the backwash flow. The suspended sediment moves offshore along with the backwash and simultaneously settles down vertically. In Figure 14a, there are still two regions with slightly higher sediment concentrations than neighboring domains. Those are the footprints of the sediment plumes. If there are more waves coming up, the sediment could be kept suspended and the water is turbid around the breaking point of waves, as observed in the field.

It is noted that, in the present results, the maximum run-up of the two-solitary-wave swash events is determined by the run-up of the first wave. In all four cases, the run-up of the second wave is lower than the first due to more energy dissipation in the swash–swash interaction. The effect of the time interval between the two solitary waves on the run-up of the first wave is minor as the adopted time intervals are large, and the onshore propagation of the first wave is little affected by the second. However, the run-up of the second wave increases with the decreasing time interval. In Case P1, with a time interval of 3.7 s, the run-up of the second wave is about $x=24.6 \mathrm{m}$. Comparatively, in Case P4, with a time interval of 3.1 s, the run-up of the second wave is about $x=25.0 \mathrm{m}$. The dynamic reason is discussed in Section 4.

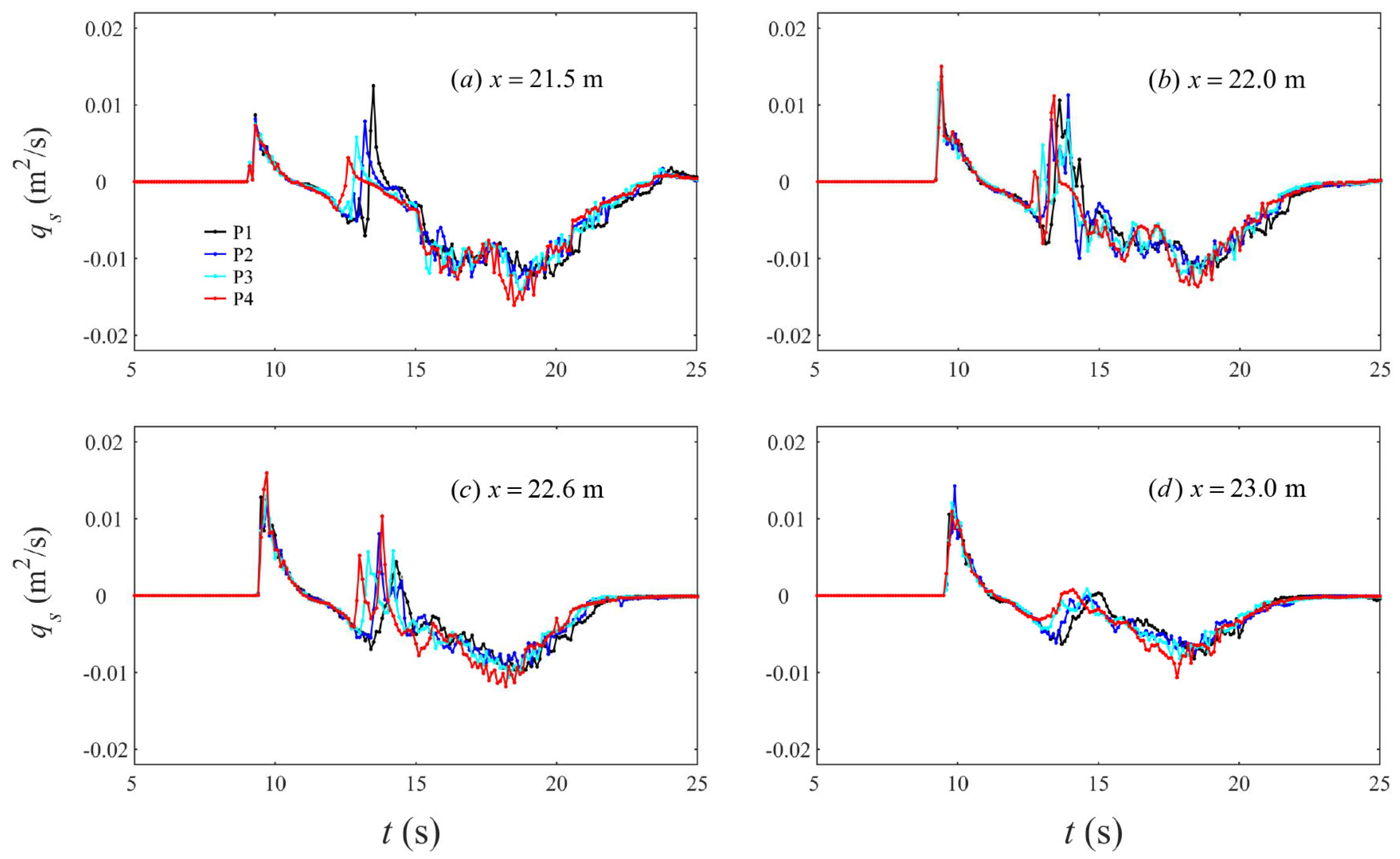
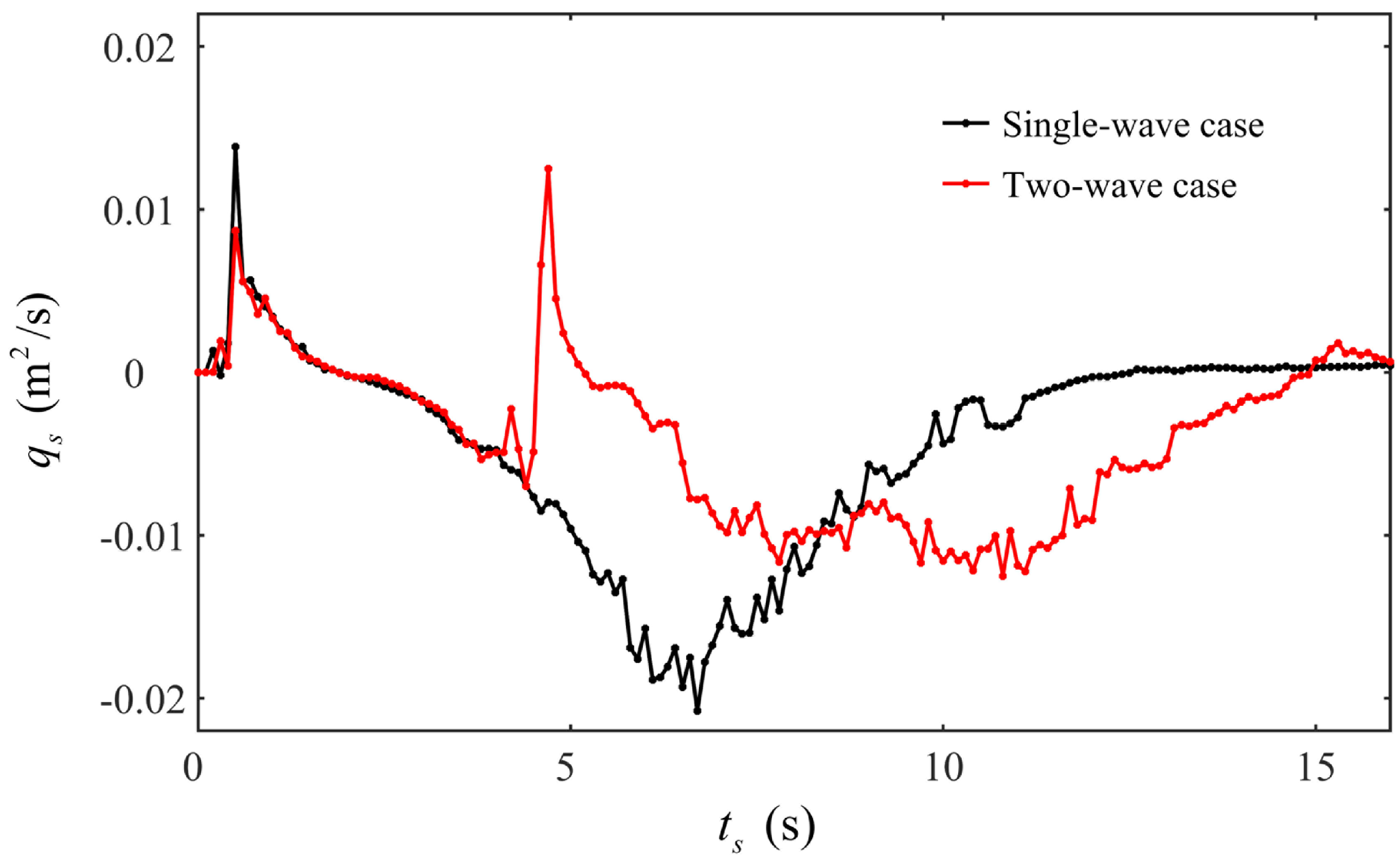
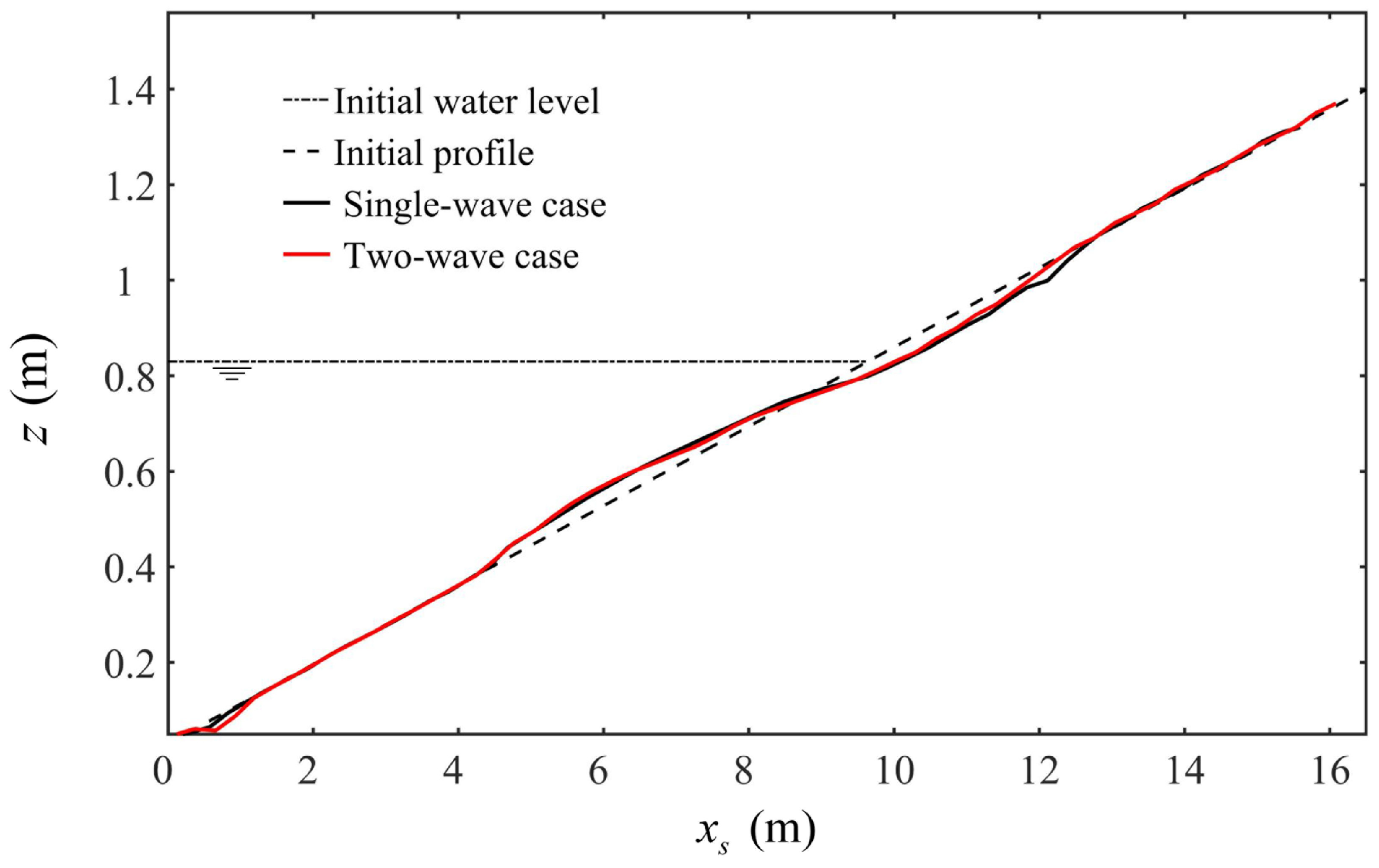
3.3. Sediment Flux and Beach Profile Change

Figure 15 shows the influence of the time interval between two successive solitary waves on temporal variations of the horizontal sediment flux in the swash zone. The sediment flux is defined as

$$q_s={\displaystyle {\int }_{z_b}^h{u}_{s,1}{\alpha }_{s}dz}$$

(18)

where $h$ is the local water depth at the location and $z_b$ is the elevation of the immovable bed. Positive value of $q_s$ indicates the onshore sediment flux.

It is shown that there are two notable peaks (positive) in the temporal variations of sediment transport at the four locations, corresponding to the arrival of the two solitary waves. Before $t=10 \mathrm{s}$, the onshore sediment flux reaches the first peak when the first solitary wave arrives and then decreases to be negative as the flow changes to be backwash. With the arrival of the second wave, the sediment flux turns onshore and reaches another peak. At $x=21.5 \mathrm{m}$, just below the initial water level, the second peak $q_s$ appears later as the time interval between the two solitary waves $T_s$ is larger, and moreover, the absolute value of the second peak $q_s$ increases with $T_s$. In Figure 15b–d, above the initial water level in the swash zone, the time when the second peak $q_s$ still generally moves backward as $T_s$ but the effect of $T_s$ on the absolute value of the second peak $q_s$ is not clear. Furthermore, the values of the second peak $q_s$ are generally lower than those of the first, especially at the locations $x>21.6 \mathrm{m}$ above the initial water level. However, in Case P1 where there is significant sediment suspension as demonstrated in Figure 10b, the second peak in $q_s$ is higher than the first. More dynamic discussions on the dynamic effect of time intervals are given in Section 4.

The simulated beach profiles after the two successive wave swash events are compared in Figure 16. It is illustrated that the time interval between the two successive waves has a minor effect on the final beach profile. The locations of the equilibrium point, where there is no bed erosion or deposition in the four cases, are very close. They are all a little below the initial water level, i.e., the mean water elevation of the swash zone. Above the equilibrium point, there is sediment erosion, while the eroded sediment deposes below the equilibrium point. Even though the effect of time interval $T_s$ on local sediment flux is not negligible, its effect on the final beach morphology is insignificant.

4. Discussion

4.1. Dynamics in the Effects of the Time Interval between Two Successive Waves

In the present limited cases, the time interval between the two successive solitary waves has a minor effect on the propagation and breaking patterns of waves and the final beach profile after the swash event. However, on the sandy beach just below the mean water level, the sediment flux peak induced by the second wave increases notably with the time interval, as shown in Figure 15a. It is also noted in Section 3.2.5 that the run-up of the second wave slightly decreases with the time interval.

The effect of the time interval between the two successive waves mainly lies in the development of the backwash generated from the breaking of the first wave. A larger time interval allows more time for the backwash to intensify, and consequently, the second wave encounters a stronger backwash current during its onshore propagation. Figure 17 shows the distributions of flow velocity in the four cases at a similar stage to that shown in Figure 10b and Figure 11b, when the second wave already breaks and the generated splashing jet jumps down and impacts the flow. The difference in the four instants is set following the given time interval $T_s$. We pay attention to the lower-layer backwash in the region of $x=22.5~23.0 \mathrm{m}$. With the decrease in $T_s$ from Case P1 to P4, the strength of the lower-layer offshore backwash, generated from the second-wave breaking encounter, weakens. Correspondingly, in Figure 17 and Figure 18, the bores in Case P4 experience less resistance from the backwash and move farther toward the shore than those in Case P1. Furthermore, the run-up of the bores in Case P4 is higher than that in Case P1, as reported above.

The intensification of backwash with increasing time intervals is also the reason for the difference in sediment flux shown in Figure 15a. As discussed in Section 3.2.3, the plume of suspended sediment is carried upwards by the lower-layer backwash. Hence, a stronger backwash current leads to a more significant plume with a higher sediment concentration. Figure 18 shows the distributions of SPH particle position and sediment concentration at the corresponding instants in Figure 17. It is clearly presented that the plumes in Case P1 are higher and more notable than those in Case P4. The higher concentration of suspended sediment in these plumes leads to a greater sediment flux. Therefore, in Figure 15a, the second sediment flux peak increases with the time interval. It is noted that, at locations above the initial water level shown in Figure 15b–d, the change in sediment flux with the time interval is not clear as the dynamics are very complex under the violent rolling of bores.

4.2. Differences in Sediment Transport under Single and Two Solitary Waves

The sediment fluxes and resulting changes in beach profile under single and two solitary waves, i.e., respectively, the validation case in Section 2.3 and Case P1 in Section 3, are compared in Figure 19 and Figure 20. In Figure 19, the temporal evolution of sediment flux across the section $x_s=9.47 \mathrm{m}$ away from the slope toe, i.e., the section $x=21.5 \mathrm{m}$ shown in Figure 15a, shows totally different patterns in the two cases. The time for plotting $t_s$ starts from the instant of wave breaking, and the wave-breaking instant is various in the two cases due to the different lengths of the flat part of the flume. $t_s=0 \mathrm{s}$ in Figure 19 corresponds to the instant $t=7.8 \mathrm{s}$ in the single-wave case while $t=8.8 \mathrm{s}$ in the two-wave case. In the single-wave case, once the broken wave arrives at the section, there is a peak of onshore sediment flux. Along with the deceleration of the uprush event, the onshore sediment flux decreases and turns to offshore flux when the lower-layer flow turns to be backwash. The peak absolute value of offshore sediment flux is larger than that of the onshore flux in the uprush stage. The duration of offshore sediment flux is notably longer than that of onshore flux. Comparatively, in the two-wave case, once the second solitary wave arrives at the section, the offshore sediment flux rapidly reverses to be onshore, with a peak even higher than the first one under the uprush of the first solitary wave. The onshore sediment flux then decreases with the deceleration of the uprush of the second solitary wave and also reverses offshore soon. The peak absolute value of the offshore sediment flux in the two-wave case is notably smaller than that in the single-wave case, while the duration of offshore flux is much longer in the two-wave case. Nevertheless, the temporally accumulated volumes of sediment across the section during a whole swash event are close in the two cases. Accordingly, as shown in Figure 20, the profiles of the sandy beach after the swash event are also very close in the two cases.

5. Conclusions

Short-term temporal evolution of water surface level, flow velocity, and beach profile in the swash zone under a solitary wave on a sandy beach is successfully captured by the two-phase SPH model. Numerical results of the flow field, sediment transport, and short-term beach profile change under two successive solitary waves with various time intervals between the two waves are obtained. In the limited cases simulated, the time interval between the two solitary waves has a minor effect on the breaking and swash–swash interaction patterns as well as the maximum run-up of the first wave. On the sandy slope, the second wave encounters the backwash generated by the first wave. The intense swash–swash interaction results in macroscale vortices in the flow field and notable sediment plumes upward from the bed. The sediment plumes are driven by the offshore backwash and pushed upwards by the onshore bores of the second wave. A longer time interval between the two successive solitary waves results in a stronger lower-layer backwash and, thus, a higher and more notable sediment plume. Below the initial water level, the sediment plumes contribute to the increase in local sediment flux, and the peak value of sediment flux induced by the second wave increases with the time interval between the two waves. However, the time interval has a minor effect on the beach morphology after the two-solitary-wave swash event.

This study figures out the dynamics of sediment transport under swash–swash interaction induced by two successive solitary waves. Specifically, the role of the backwash in the lower layer of water flow is uncovered. The backwash from the previous wave, together with the uprush of the later wave, generates macro-vortices, which extend in the whole water depth and lead to sediment plumes from the sandy bed. The time interval between the two successive solitary waves is critical to the temporal variation in sediment flux while having a minor effect on the resulting change in beach profile. All these discussions have not been reported in the literature. However, definitely, the present numerical studies are still quite limited. Further studies on the effects of wave height, waveform, sediment size, and the number of waves on sediment transport are pursued. Additionally, the effects of these wave parameters on the long-term evolution of beach morphology are expected.

It is noted that the presently adopted two-phase SPH model is generally a small-scale dynamic model for short-term changes in hydrodynamics, sediment transport, and beach profile. It has shown, not only in the present paper but also in previous work published by the authors, enhanced capability to capture the violent free-surface water flow and the intense sediment transport. However, its application to practical or long-term experimental cases is limited due to the enormous computational effort. Furthermore, at present, the constitutive law for the sediment phase in the model works for particles with grain sizes larger than fine sand, and the constitutive law should be revised if applied to clay or silt.