Dynamic Response Analysis of a Subsea Rigid M-Shaped Jumper under Combined Internal and External Flows
Abstract
1. Introduction
- (1)
- The internal and external flow fields are segregated for CFD simulation and subsequently loaded onto the FE model. This approach ensures that the influences of multiple flow fields are appropriately considered.
- (2)
- The algorithm selected for each fluid domain and structural model is characterized by a high degree of flexibility, allowing for additional modifications and supplementary calculations to be conducted based on specific requirements as needed.
- (3)
- The quantity of CFD simulations is minimized. Specifically, for combinations of internal and external flow conditions, only two corresponding individual flow conditions are chosen for pairing.
2. Problem Description
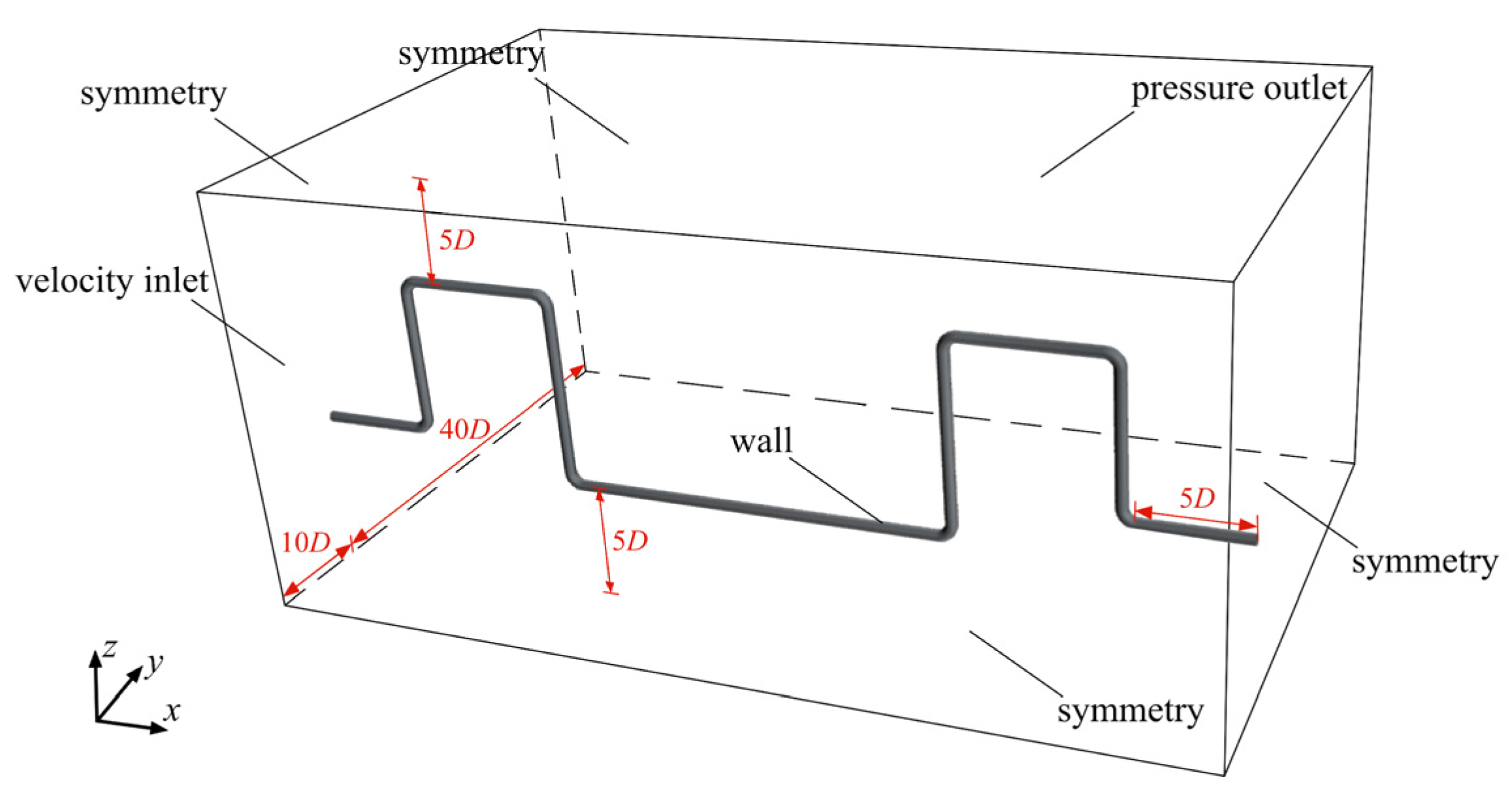
2.1. Analysis Model
2.2. Flow Conditions
3. Method
3.1. Fluid Domain
3.2. Structural Model
3.3. Pressure Interpolation
3.4. Fatigue Damage
4. Convergence Analysis
4.1. Internal Fluid Domain
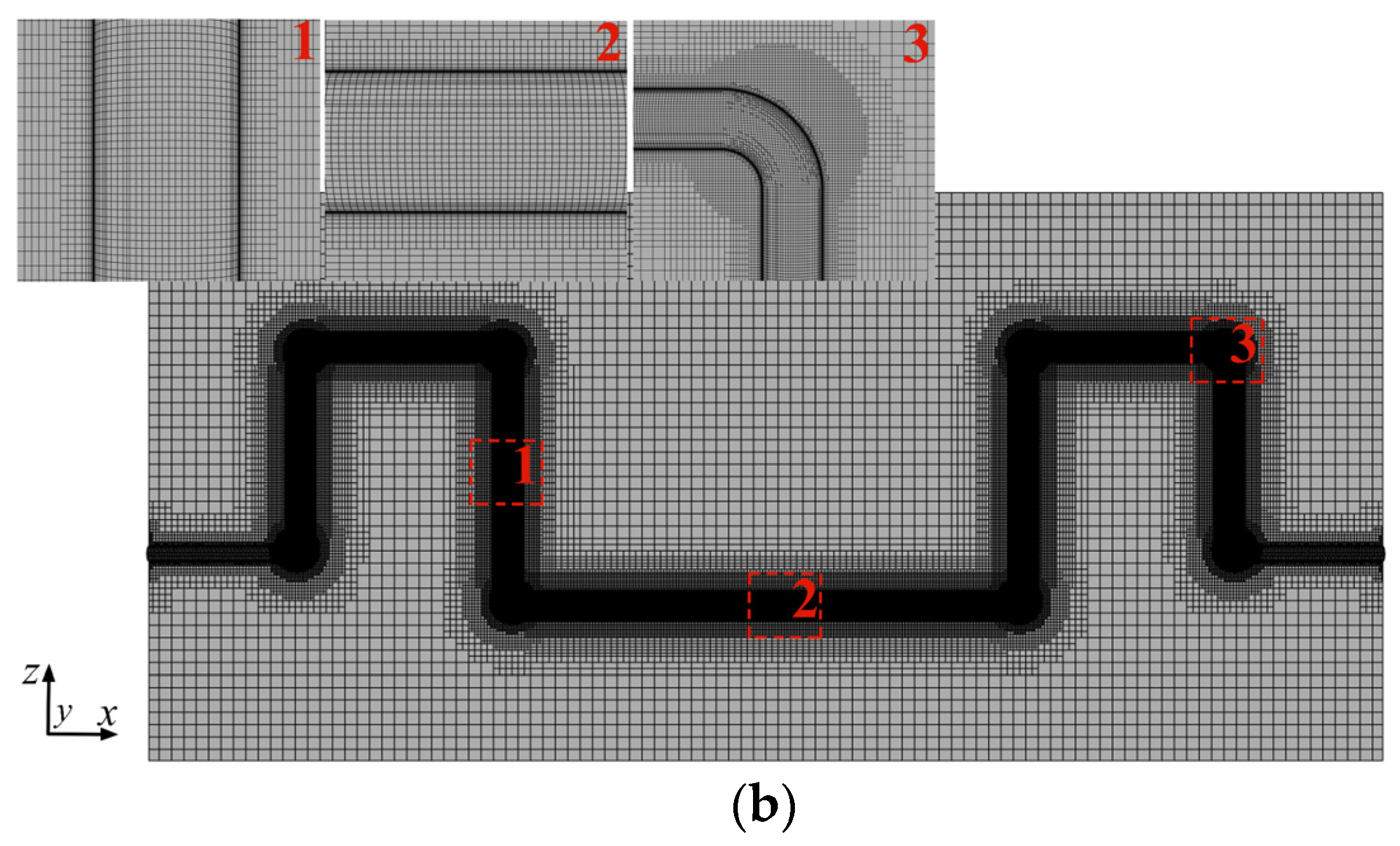
4.1.1. Geometry and Mesh
4.1.2. Mesh and Timestep Analysis
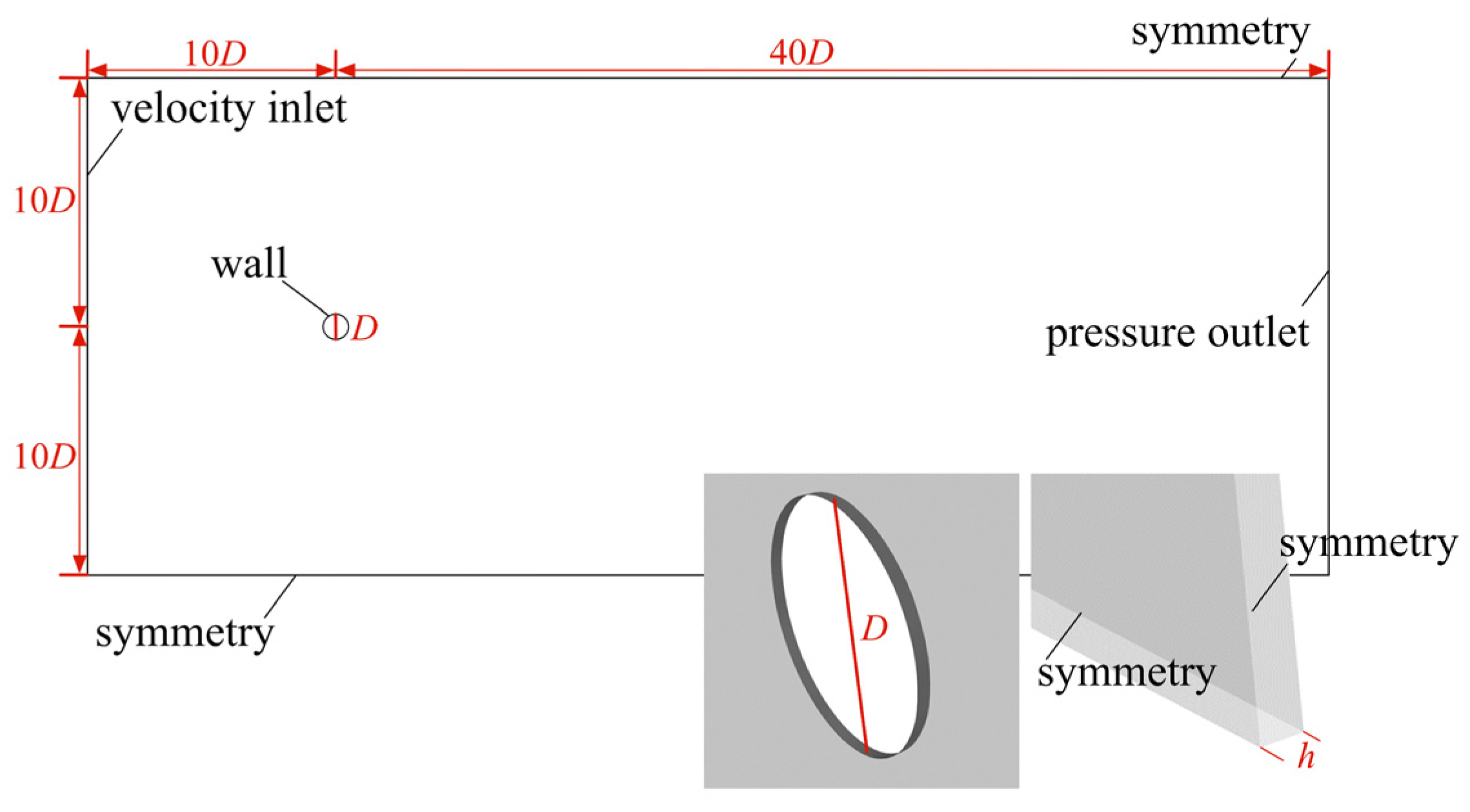
4.2. External Fluid Domain
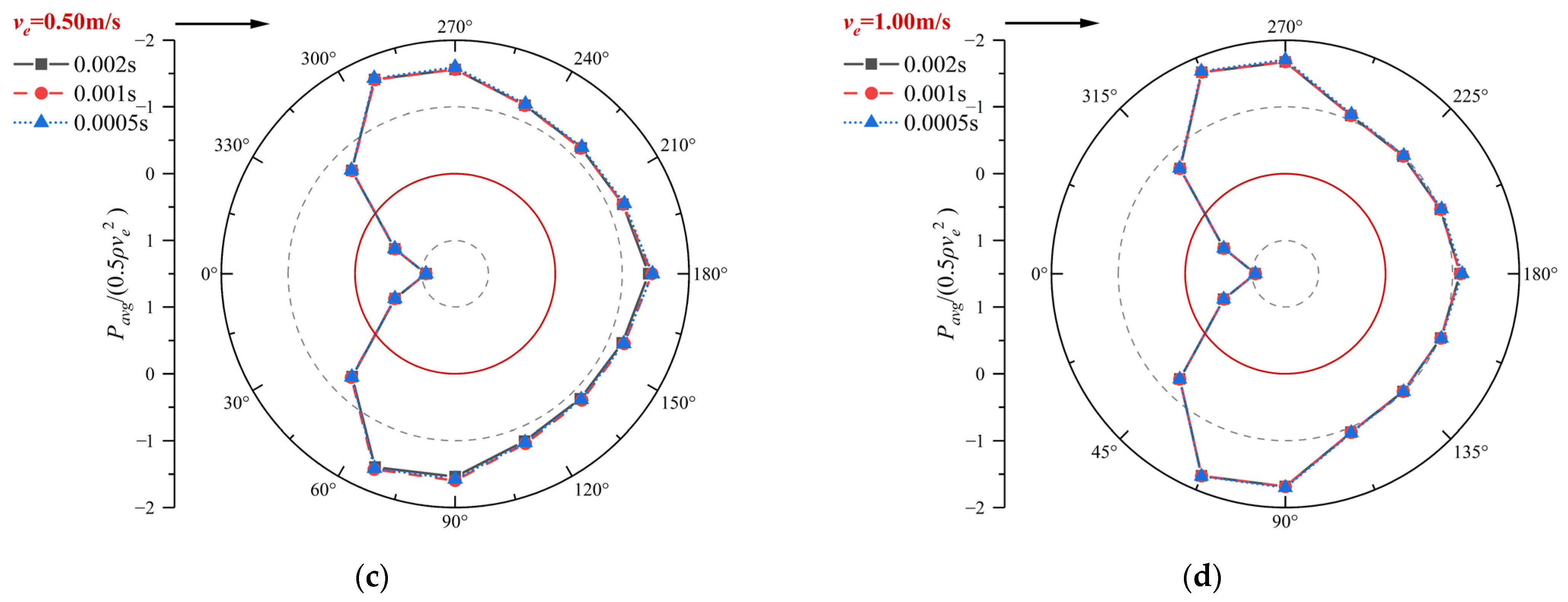
4.2.1. Flow around a Cylinder
4.2.2. Geometry and Mesh
4.3. Structural Model
4.3.1. Geometry and Mesh
4.3.2. Modal
4.3.3. Time History Analysis
5. Results
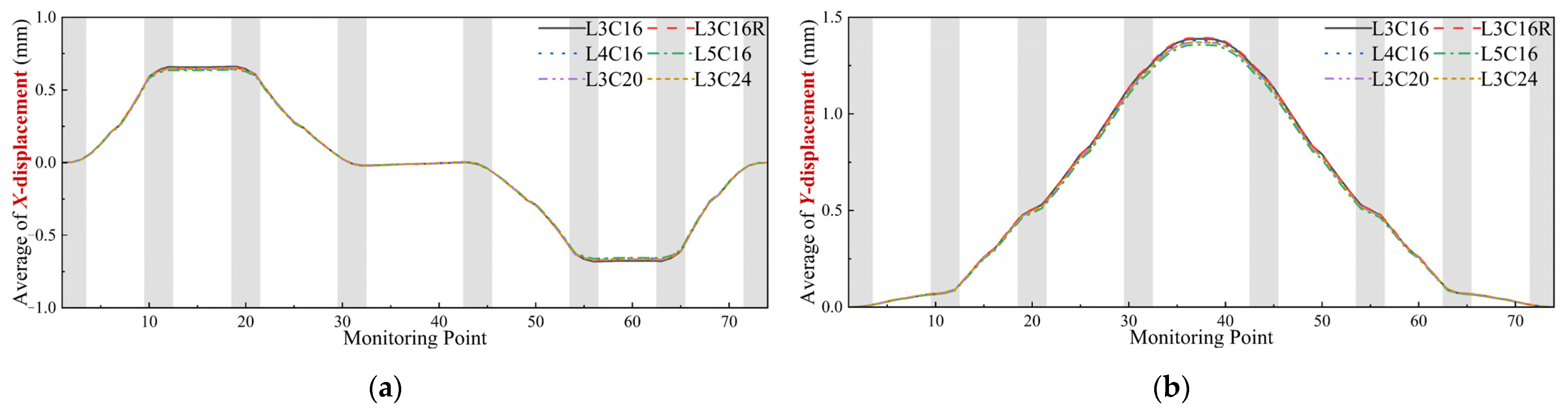
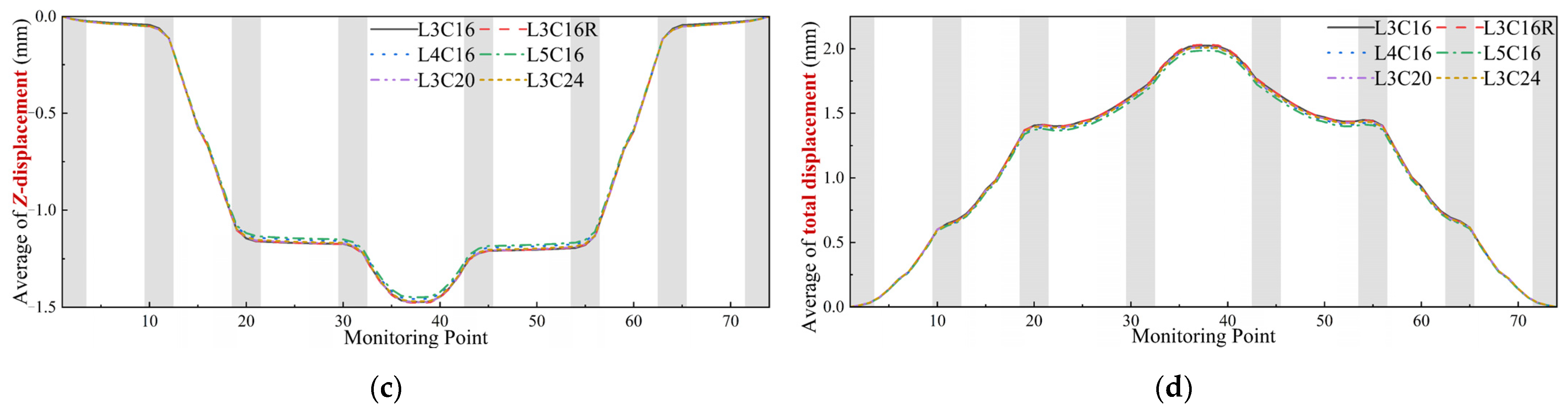
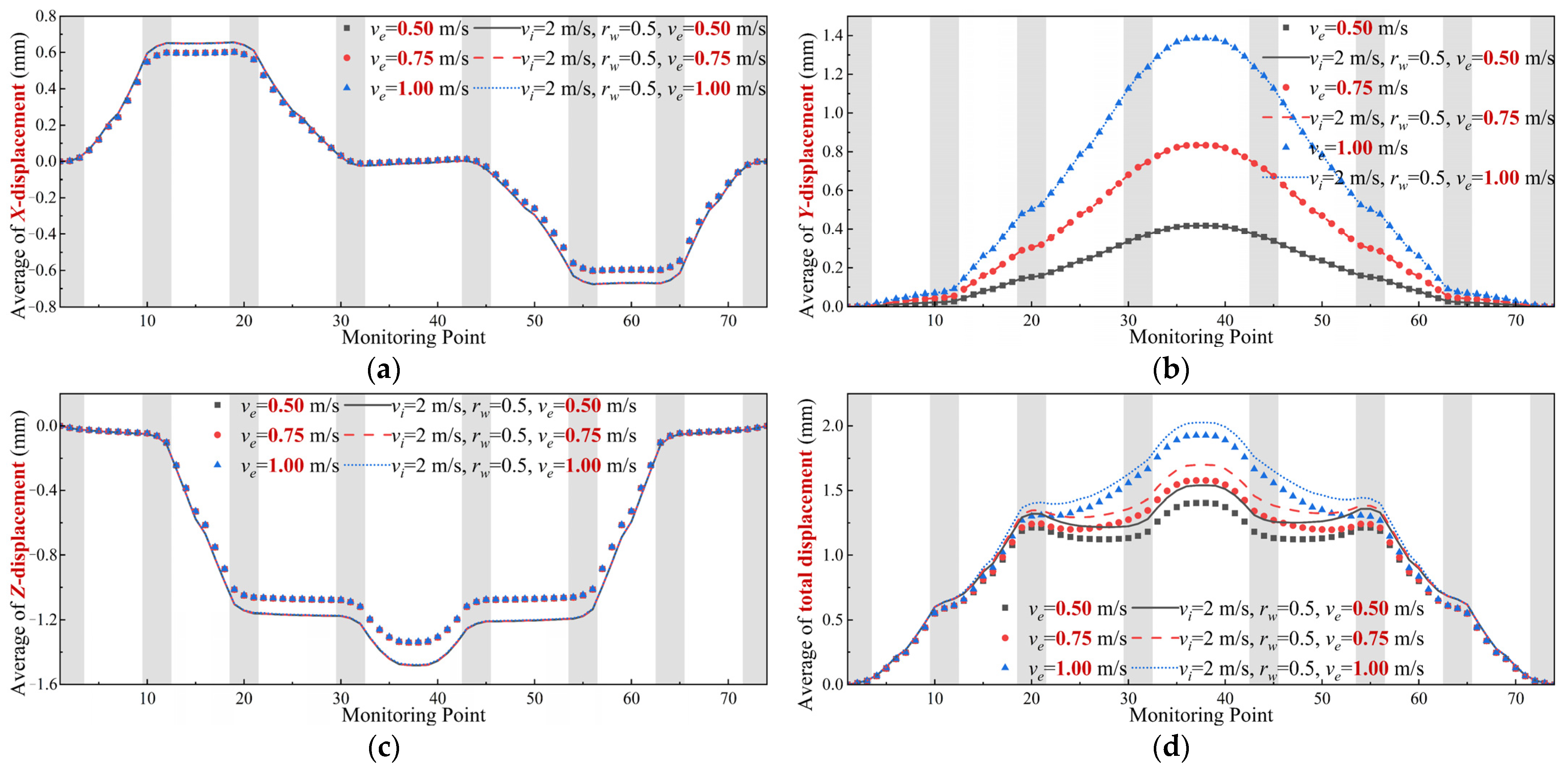
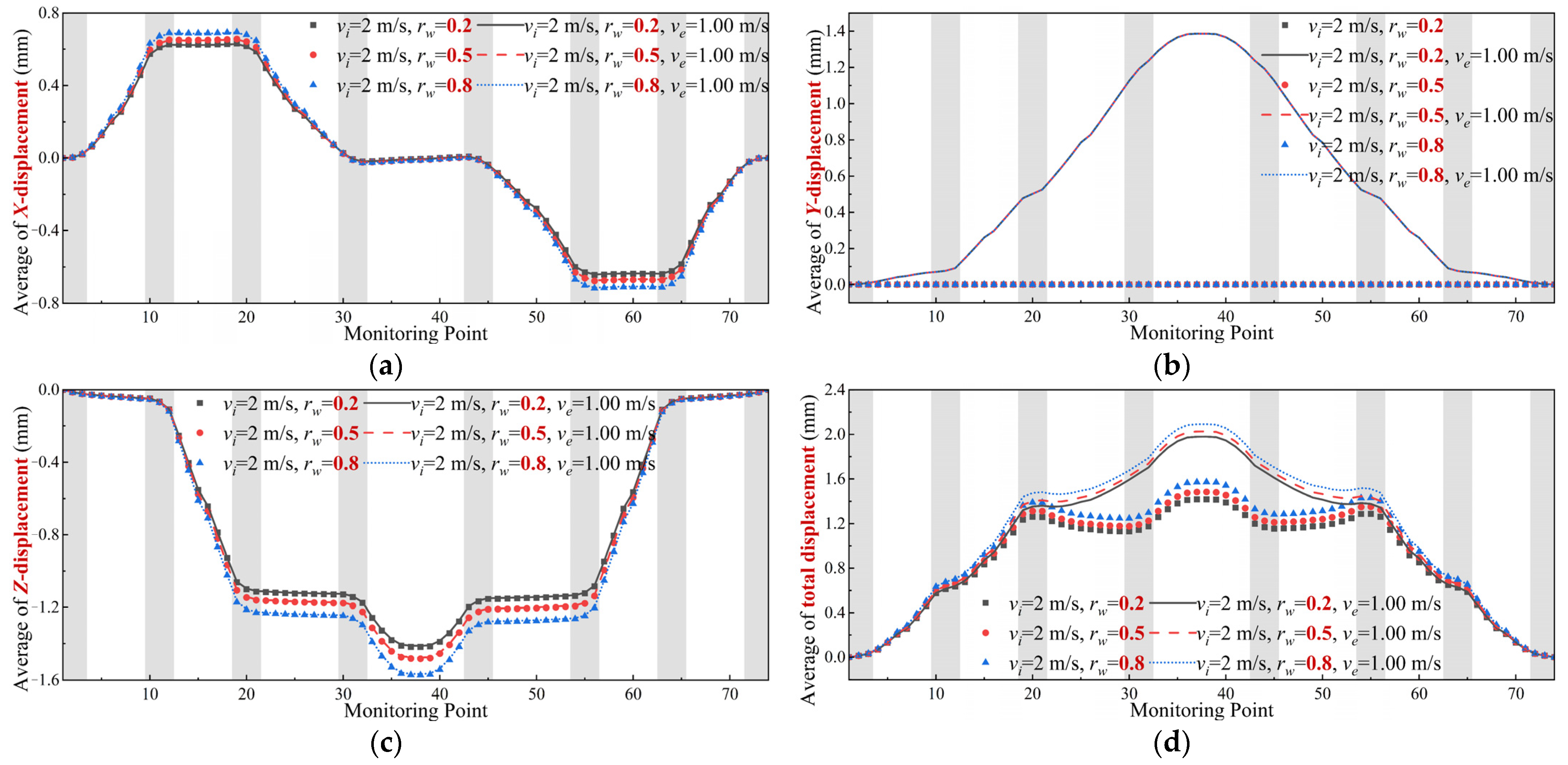
5.1. Displacement
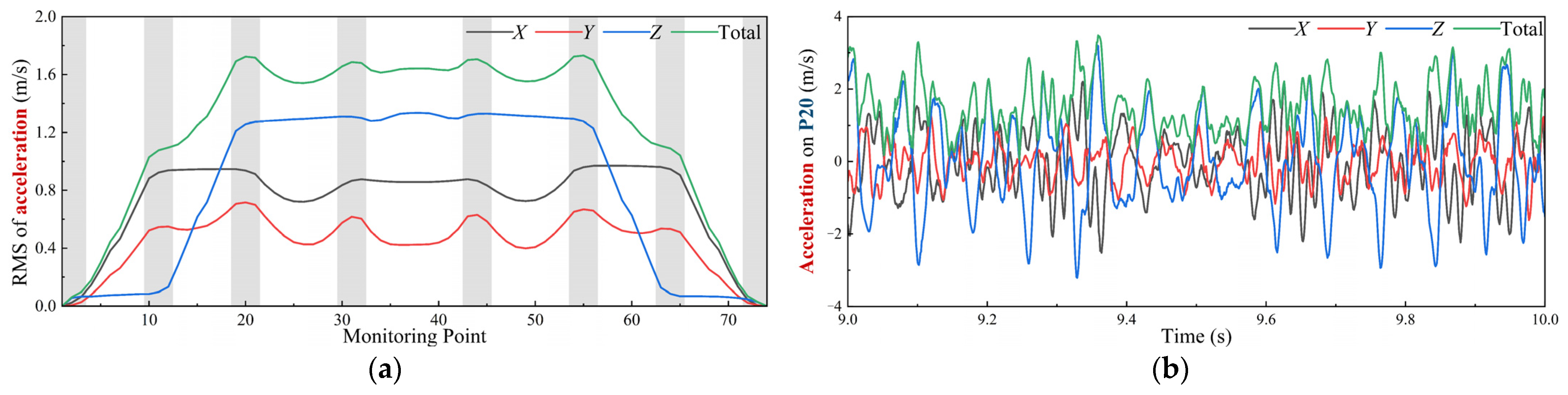
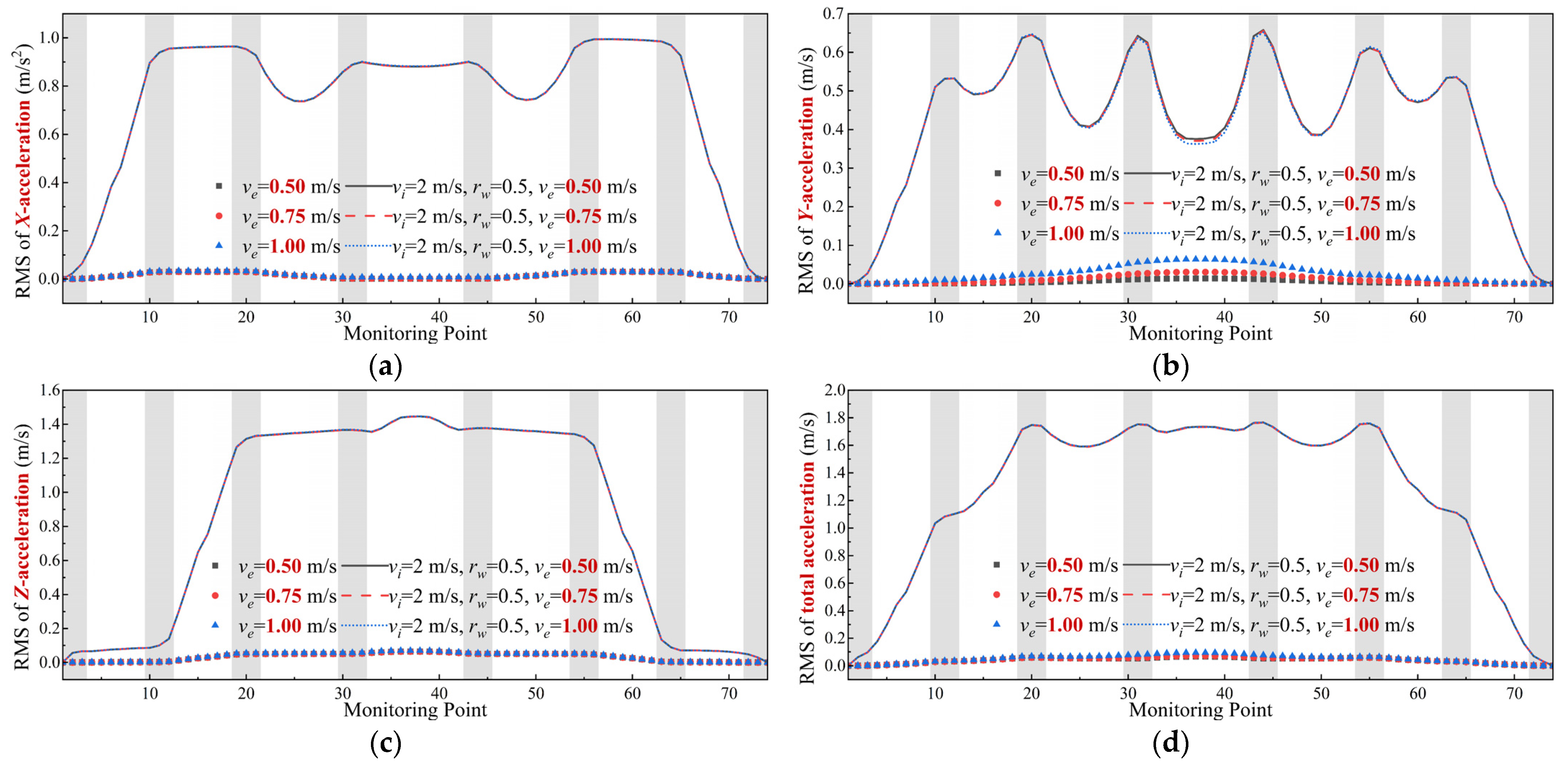
5.2. Vibration
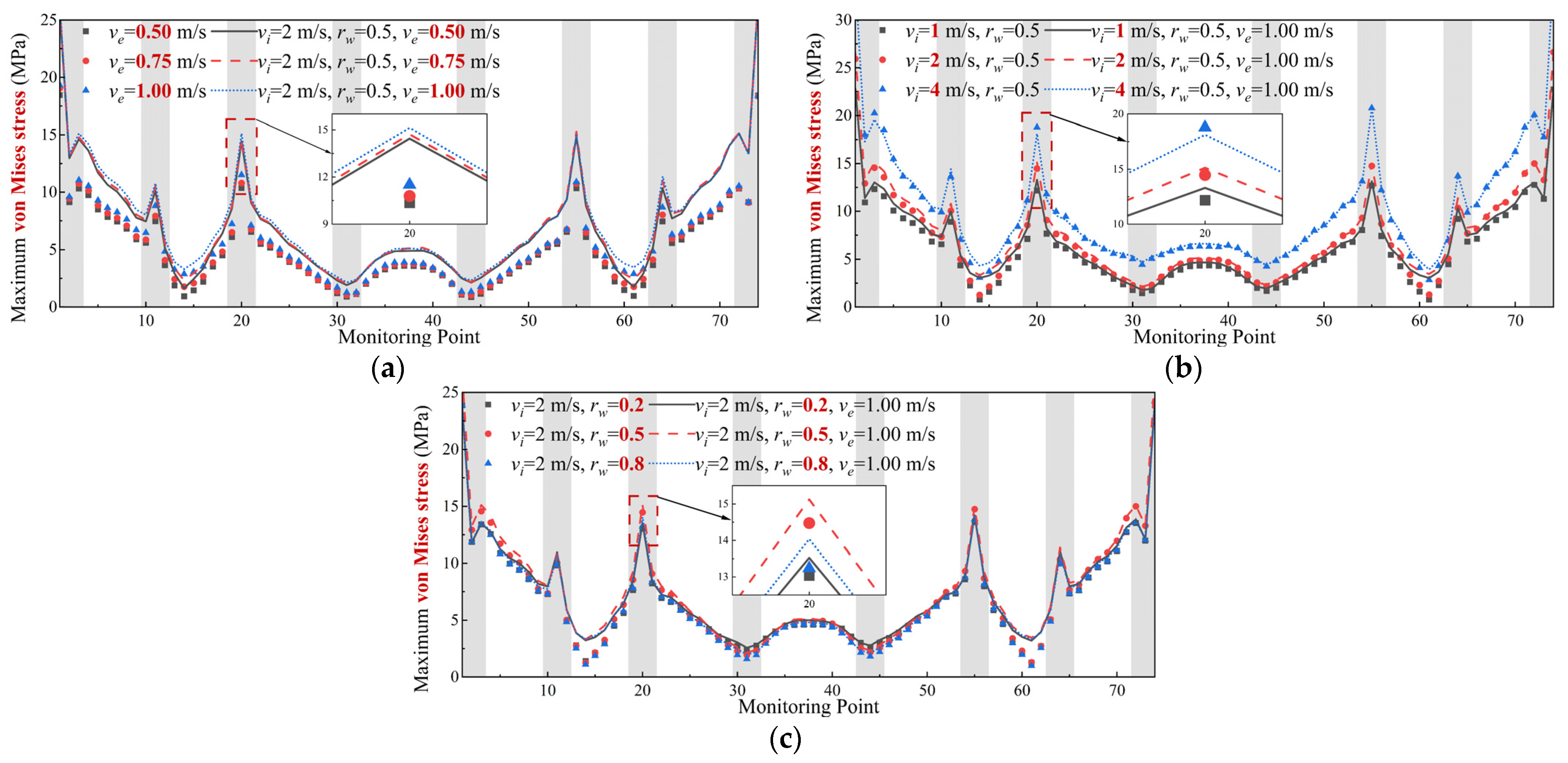
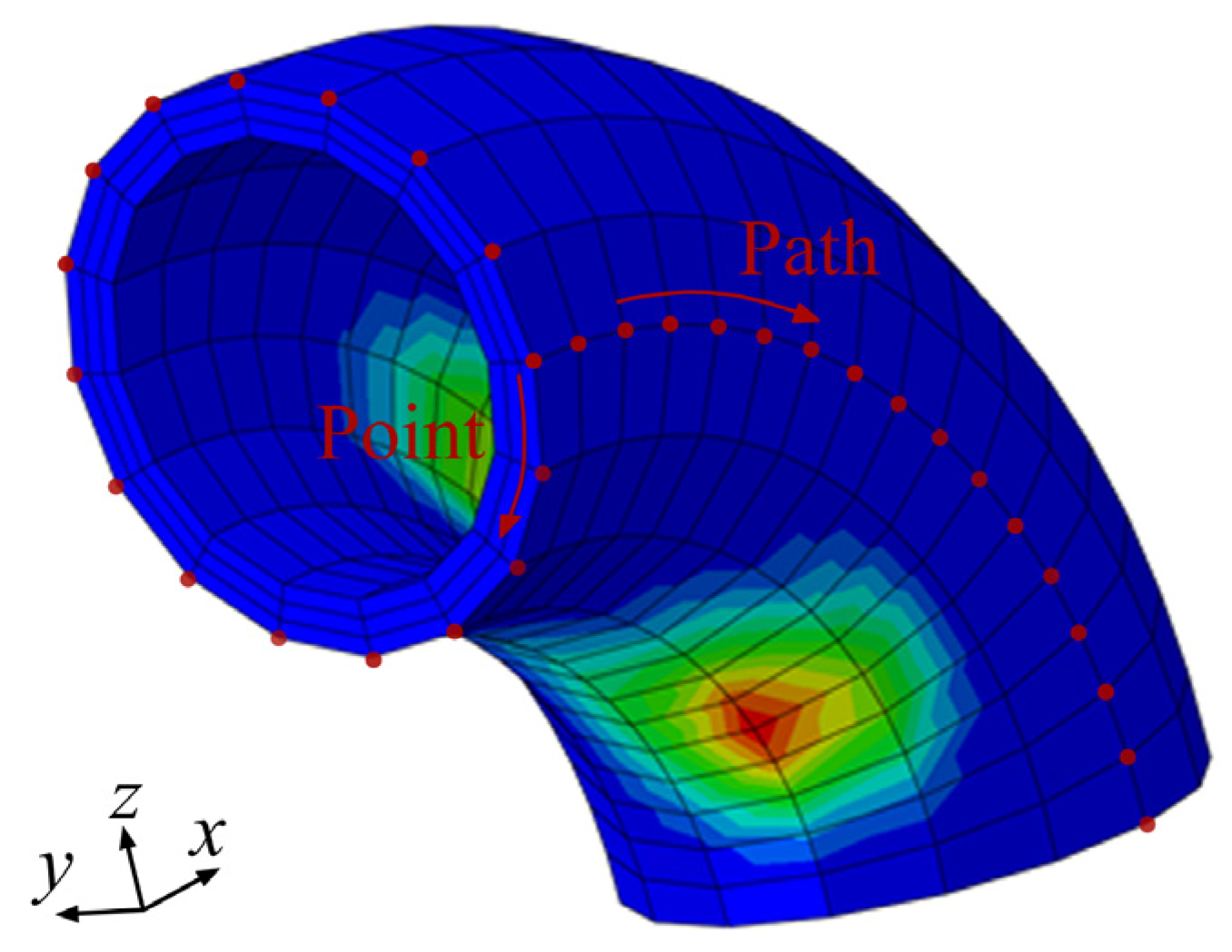
5.3. Stress
5.4. Fatigue Assessment
6. Conclusions
- (1)
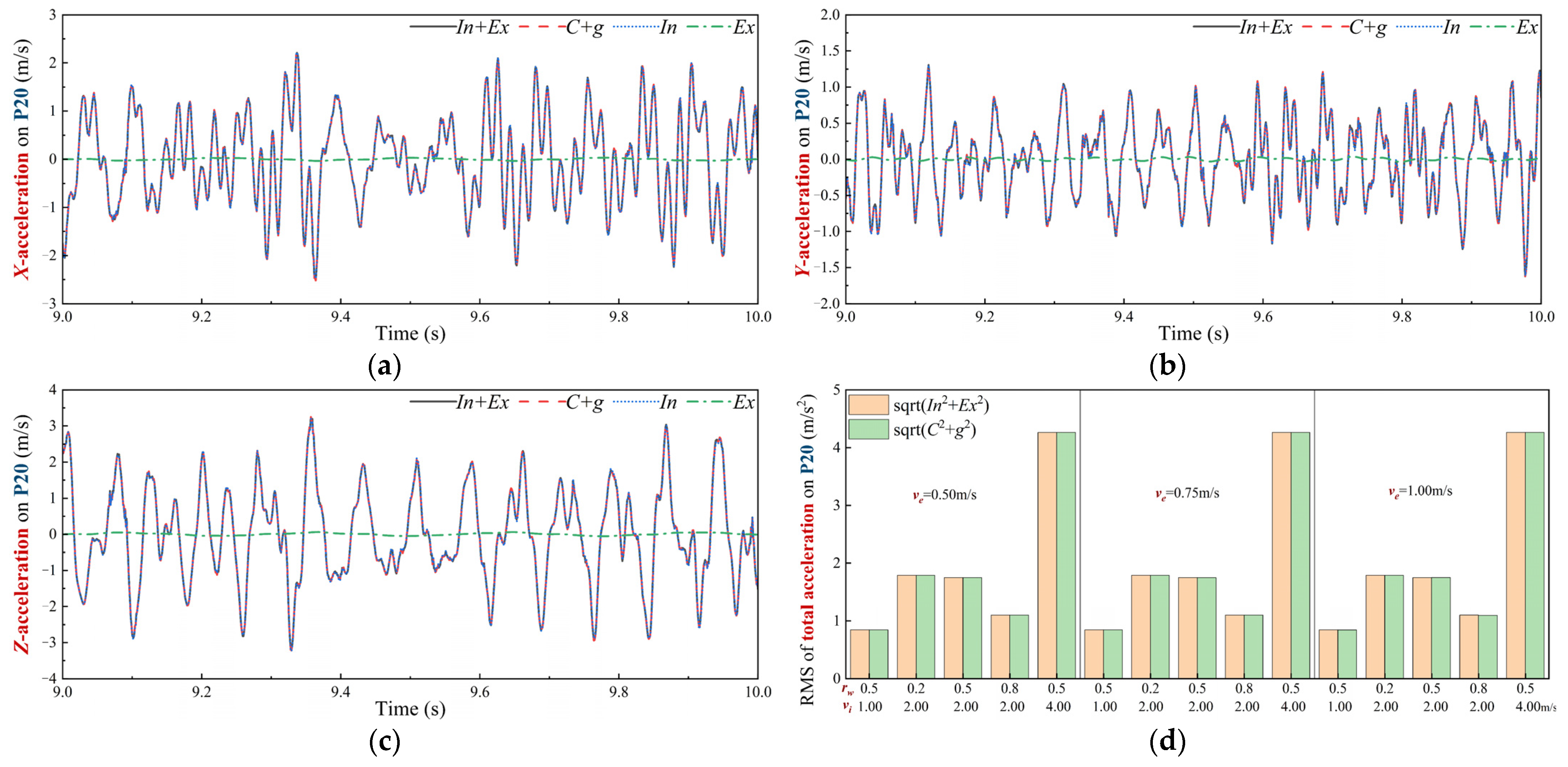
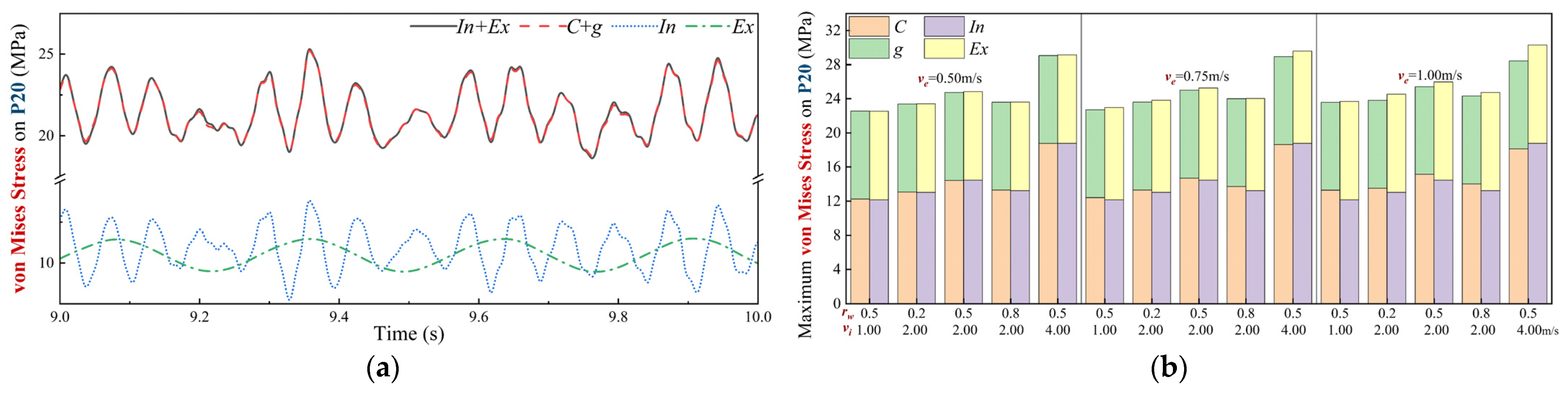
- The overall displacement of the jumper is assessed by averaging point displacements. Analysis of the total displacement and the displacements along the jumper in all three directions from inlet to outlet shows that the long span experiences maximum displacement at the middle, with the external flow velocity dominating the displacement. It is crucial to note that in the FEA, when considering gravity, the displacement results influenced by both internal and external flows, in addition to the results influenced solely by gravity, are equal to the sum of the results influenced solely by internal flow and solely by external flow.
- (2)
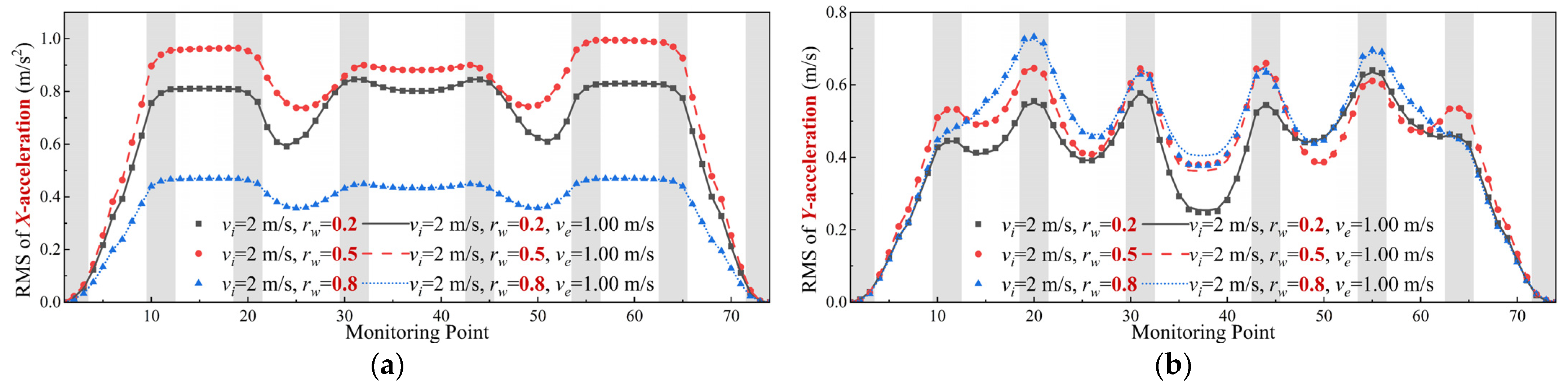
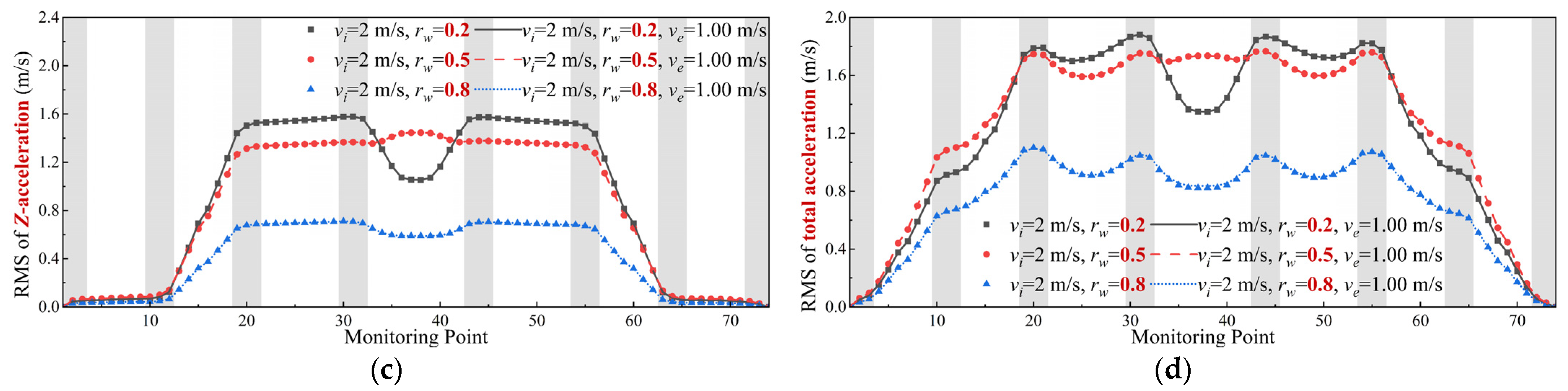
- The vibration of each monitoring point distributed along the jumper is assessed based on the RMS of the historical accelerations. The positions with the most intense vibrations are observed at bend3 and bend6 among the total of eight bends. The effect of external flow velocity is not significant. The variation in internal flow velocity has the greatest impact on vibration, with the amplitude increasing rapidly as the internal flow velocity increased. The variation in amplitude with water volume fraction is more complex, displaying different patterns of change on different segments. The sum of the squares of the internal and external flow acting alone is equal to the sum of the squares of the internal and external flow acting together and gravity acting alone.
- (3)
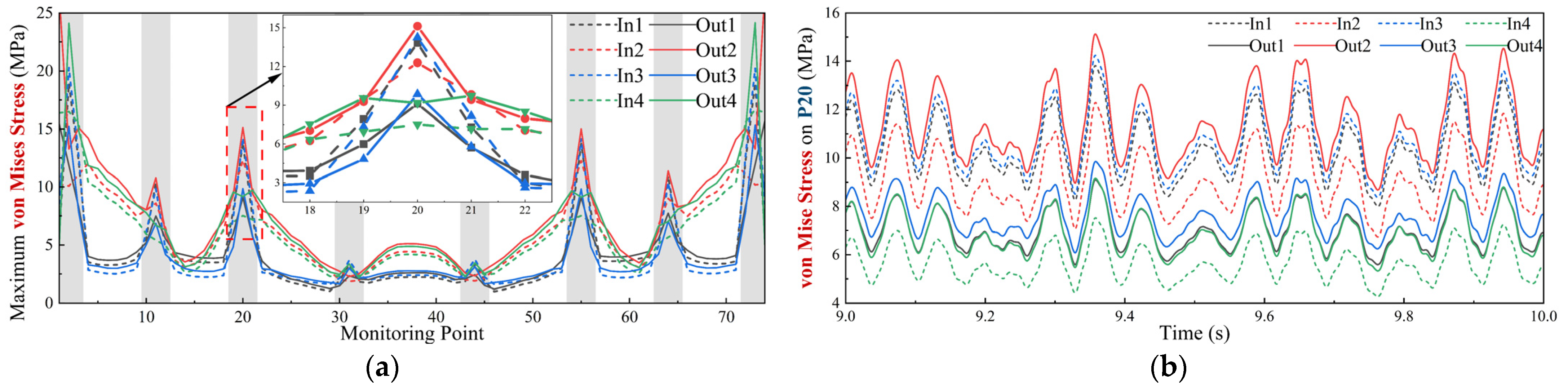
- This analysis focuses on the distribution of maximum von Mises stress on the inner and outer walls of the jumper. The highest stresses are observed at both ends, attributable to the fixed supports. Significant stress concentrations are observed at the bends, particularly at bend3 and bend6. The stress increased significantly with a higher internal flow velocity, identified as the primary influencing factor. According to the historical stress results, the impact on the structure from both internal and external flows, combined with the effects induced by gravity only, is equivalent to the sum of the effects when subjected only to internal flow and when subjected only to external flow.
- (4)
- Fatigue damage assessments are conducted on the inner and outer walls of bend3, where significant stresses and vibrations occur. The location of maximum damage on the inner and outer walls corresponds to the position with a high maximum von Mises stress. This correlation facilitates the quick identification of the location with the maximum damage through the analysis of the von Mises stress.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Srigiriraju, S.; Chakraborty, A.; Ozturk, B.; Rathore, D. FEA based simplified integrated analysis for mudmat design. In Proceedings of the 38th ASME International Conference on Ocean, Offshore and Arctic Engineering (OMAE 2019), Glasgow, UK, 9–14 June 2019. [Google Scholar]
- Silva, L.M.R.; Soares, C.G. An integrated optimization of the floating and subsea layouts. Ocean Eng. 2019, 191, 106557. [Google Scholar] [CrossRef]
- Wang, H.; Huang, J.; Lee, S.; Gioielli, P.; Kan, W.; Spencer, D.; Islam, M. VIV response of a subsea jumper in uniform current. In Proceedings of the 32nd ASME International Conference on Ocean, Offshore and Arctic Engineering, Nantes, France, 9–14 June 2013. [Google Scholar]
- Zheng, H.N.; Slocum, S.T.; Huang, J.Z.; Srivastava, V.; Lee, S.; Wang, H.H. Numerical analysis of experimental data of subsea jumper vortex induced vibrations. In Proceedings of the 34th ASME International Conference on Ocean, Offshore and Arctic Engineering (OMAE2015), St John’s, NL, Canada, 31 May–5 June 2015. [Google Scholar]
- Igeh, L.A.; Liu, Z.H.; Wu, J.; Ong, M.C. A comparative study of fatigue damage assessment methods to a rigid planar jumper. In Proceedings of the 37th ASME International Conference on Ocean, Offshore and Arctic Engineering, Madrid, Spain, 17–22 June 2018. [Google Scholar]
- Kapoor, K.; Liu, Z.H.; Ong, M.C. A method to calculate the multi-axial fatigue of subsea rigid jumper due to VIV. In Proceedings of the 38th ASME International Conference on Ocean, Offshore and Arctic Engineering (OMAE 2019), Glasgow, UK, 9–14 June 2019. [Google Scholar]
- Liu, Z.H.; Igeh, L.A.; Wu, J.; Ong, M.C. Fatigue damage assessment to a rigid planar jumper on model scale. J. Offshore Mech. Arct. Eng. Trans. ASME 2020, 142, 12. [Google Scholar] [CrossRef]
- Qu, Y.; Fu, S.X.; Liu, Z.H.; Xu, Y.W.; Sun, J.Y. Numerical study on the characteristics of vortex-induced vibrations of a small-scale subsea jumper using a wake oscillator model. Ocean Eng. 2022, 243, 17. [Google Scholar] [CrossRef]
- Mobasheramini, M.; Alves, L.; Fernandes, A.C.; Ellwanger, G.B. Behavior evaluation of subsea jumpers exposed to current by experiment and FEM. In Proceedings of the 37th ASME International Conference on Ocean, Offshore and Arctic Engineering, Madrid, Spain, 17–22 June 2018. [Google Scholar]
- Gross, D.; Roux, Y.; Rousse, B.; Pétrié, F.; Assier, L.; Minguez, M. Experimental and numerical study of vortex induced vibrations on a spool model. In Proceedings of the 37th ASME International Conference on Ocean, Offshore and Arctic Engineering, Madrid, Spain, 17–22 June 2018. [Google Scholar]
- Holmes, S.; Constantinides, Y. Vortex induced vibration analysis of a complex subsea jumper. In Proceedings of the 29th ASME International Conference on Ocean, Offshore and Arctic Engineering, Shanghai, China, 6–11 June 2010; pp. 671–678. [Google Scholar]
- Li, G.Z.; Li, W.H.; Han, F.H.; Lin, S.Y.; Zhou, X.K. Experimental investigation and one-way coupled fluid-structure interaction analysis of gas-liquid two-phase flow conveyed by a subsea rigid M-shaped jumper. Ocean Eng. 2023, 285, 15. [Google Scholar] [CrossRef]
- Li, W.H.; Li, J.H.; Yin, G.; Ong, M.C. Experimental and numerical study on the slug characteristics and flow-induced vibration of a subsea rigid M-shaped jumper. Appl. Sci. 2023, 13, 7504. [Google Scholar] [CrossRef]
- Li, W.H.; Song, W.R.; Yin, G.; Ong, M.C.; Han, F.H. Flow regime identification in the subsea jumper based on electrical capacitance tomography and convolution neural network. Ocean Eng. 2022, 266, 11. [Google Scholar] [CrossRef]
- Li, W.H.; Zhou, Q.; Yin, G.; Ong, M.C.; Li, G.; Han, F.H. Experimental investigation and numerical modeling of two-phase flow development and flow-induced vibration of a multi-plane subsea jumper. J. Mar. Sci. Eng. 2022, 10, 1334. [Google Scholar] [CrossRef]
- Zhu, H.J.; Tang, T.; Li, Q.H. Flow-induced vibration of a reversed U-shaped jumper conveying oil-gas two-phase flow. Processes 2023, 11, 1134. [Google Scholar] [CrossRef]
- Zhu, H.J.; Hu, Y.N.; Tang, T.; Ji, C.N.; Zhou, T.M. Evolution of gas-liquid two-phase flow in an M-shaped jumper and the resultant flow-induced vibration response. Processes 2022, 10, 2133. [Google Scholar] [CrossRef]
- Xie, W.D.; Gao, X.F.; Wang, E.H.; Xu, W.H.; Bai, Y.C. An investigation of the nonlinear dynamic response of a flexible pipe undergoing vortex-induced vibrations and conveying internal fluid with variable-density. Ocean Eng. 2019, 183, 453–468. [Google Scholar] [CrossRef]
- Gu, J.J.; Ma, T.Q.; Chen, L.L.; Jia, J.C.; Kang, K. Dynamic analysis of deepwater risers conveying two-phase flow under vortex-induced vibration. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 14. [Google Scholar] [CrossRef]
- Leng, D.X.; Liu, D.; Li, H.Y.; Jin, B.; Liu, G.J. Internal flow effect on the cross-flow vortex-induced vibration of marine risers with different support methods. Ocean Eng. 2022, 257, 20. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Chen, N.Z. Vortex-induced vibration of flexible riser transporting high-speed spiral flow in deep-sea mining. Ocean Eng. 2022, 261, 17. [Google Scholar] [CrossRef]
- Duan, J.L.; Zhou, J.F.; Wang, X.; You, Y.X.; Bai, X.L. Cross-flow vortex-induced vibration of a flexible fluid-conveying riser undergoing external oscillatory flow. Ocean Eng. 2022, 250, 18. [Google Scholar] [CrossRef]
- Xu, A.D.; Chai, Y.Y.; Li, F.M.; Chen, Y.X. Nonlinear vortex-induced vibrations of slightly curved pipes conveying fluid in steady and oscillatory flows. Ocean Eng. 2023, 270, 24. [Google Scholar] [CrossRef]
- Mina, D.; Karampour, H.; Forcellini, D. Resilience of HP/HT pipelines to combined seismic and thermal loadings. Ocean Eng. 2023, 275, 21. [Google Scholar] [CrossRef]
- Chmelko, V.; Kliman, V.; Garan, M. In-time Monitoring of Fatigue Damage. Procedia Eng. 2015, 101, 93–100. [Google Scholar] [CrossRef][Green Version]
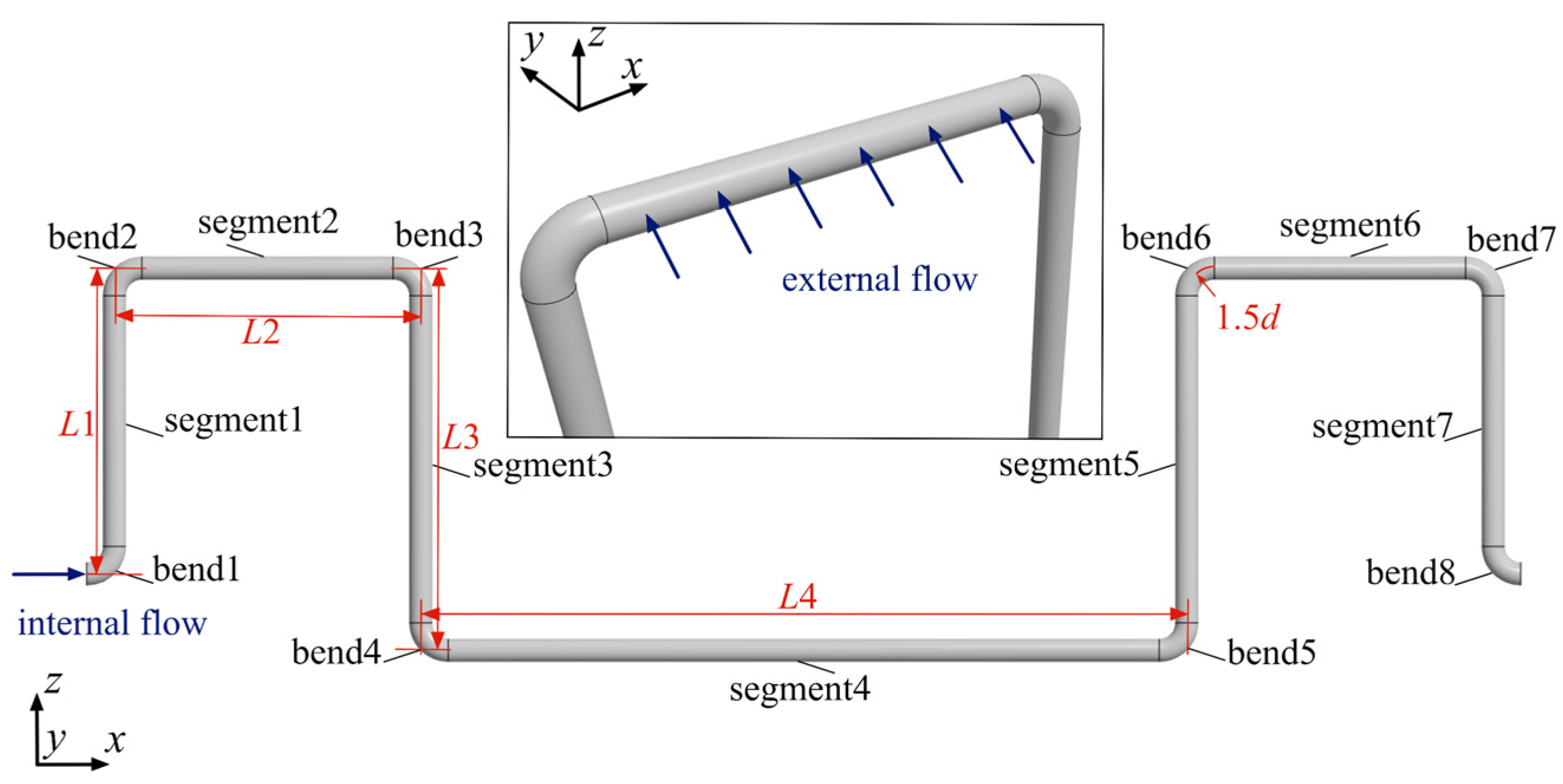
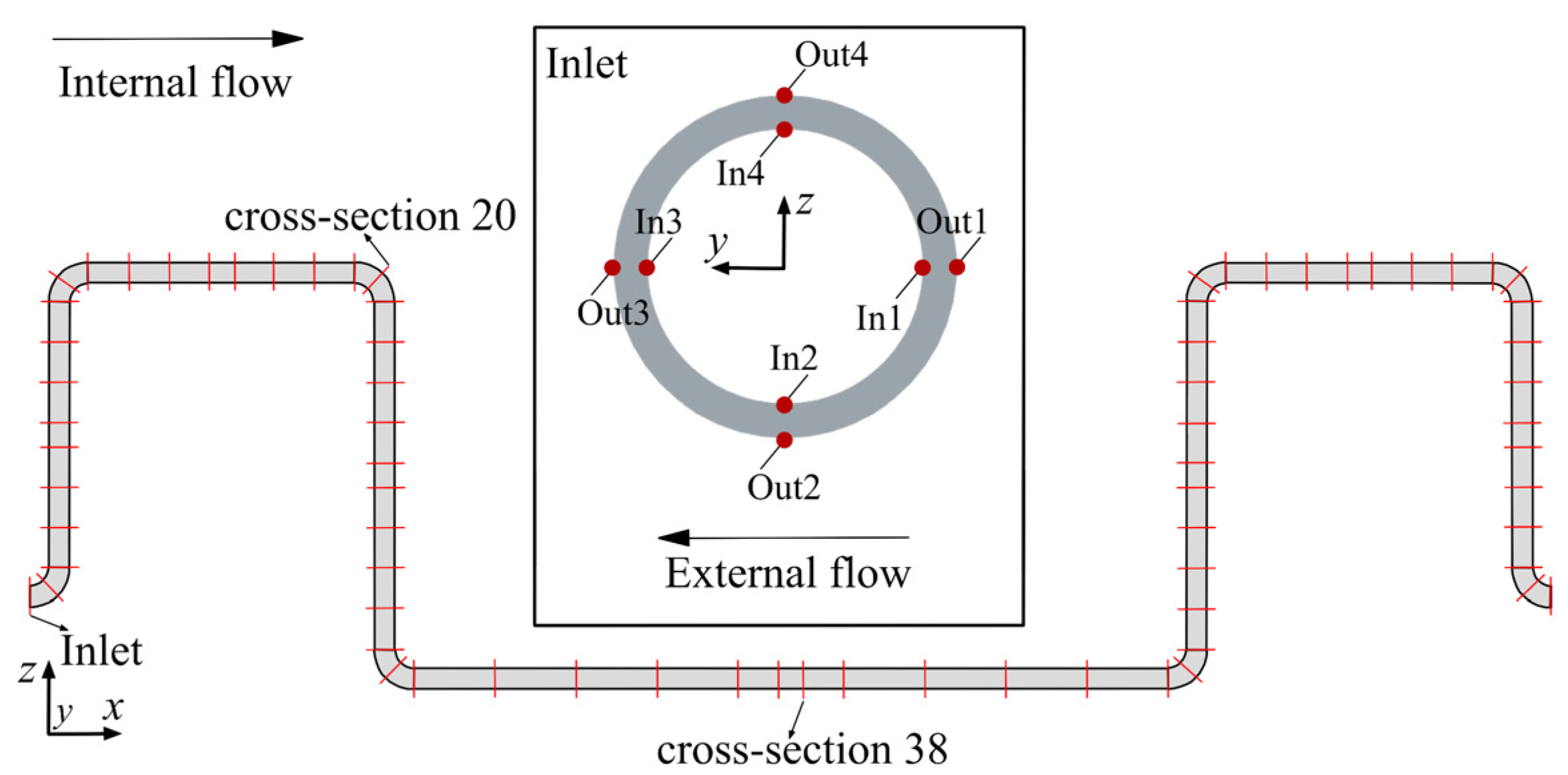

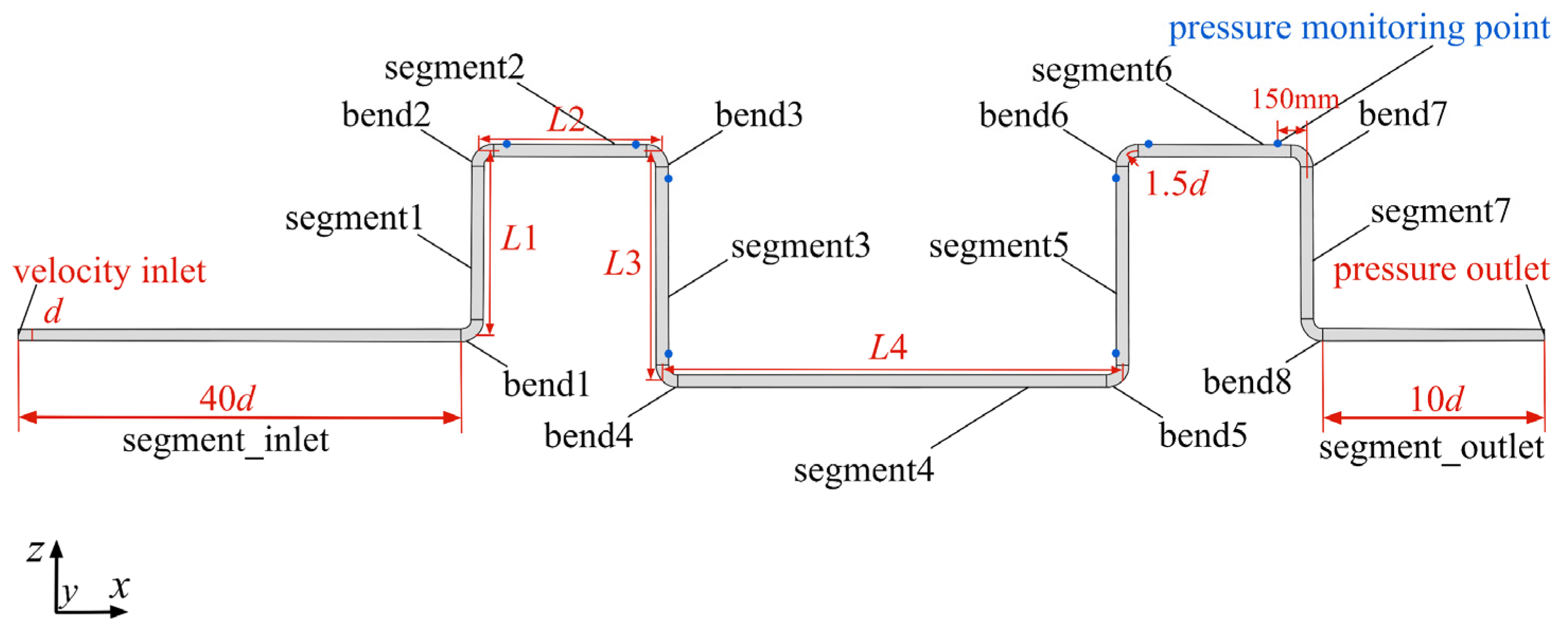
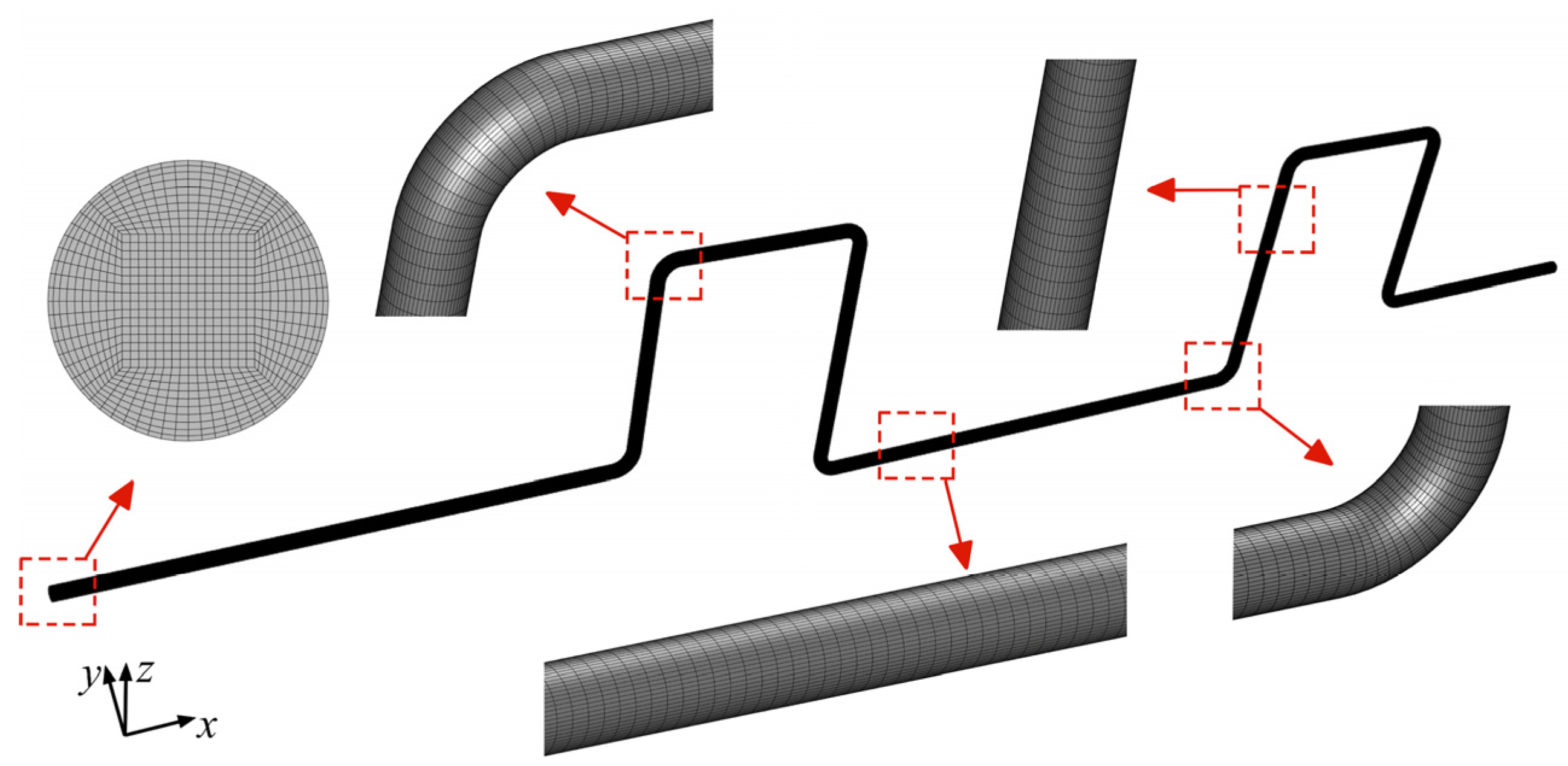





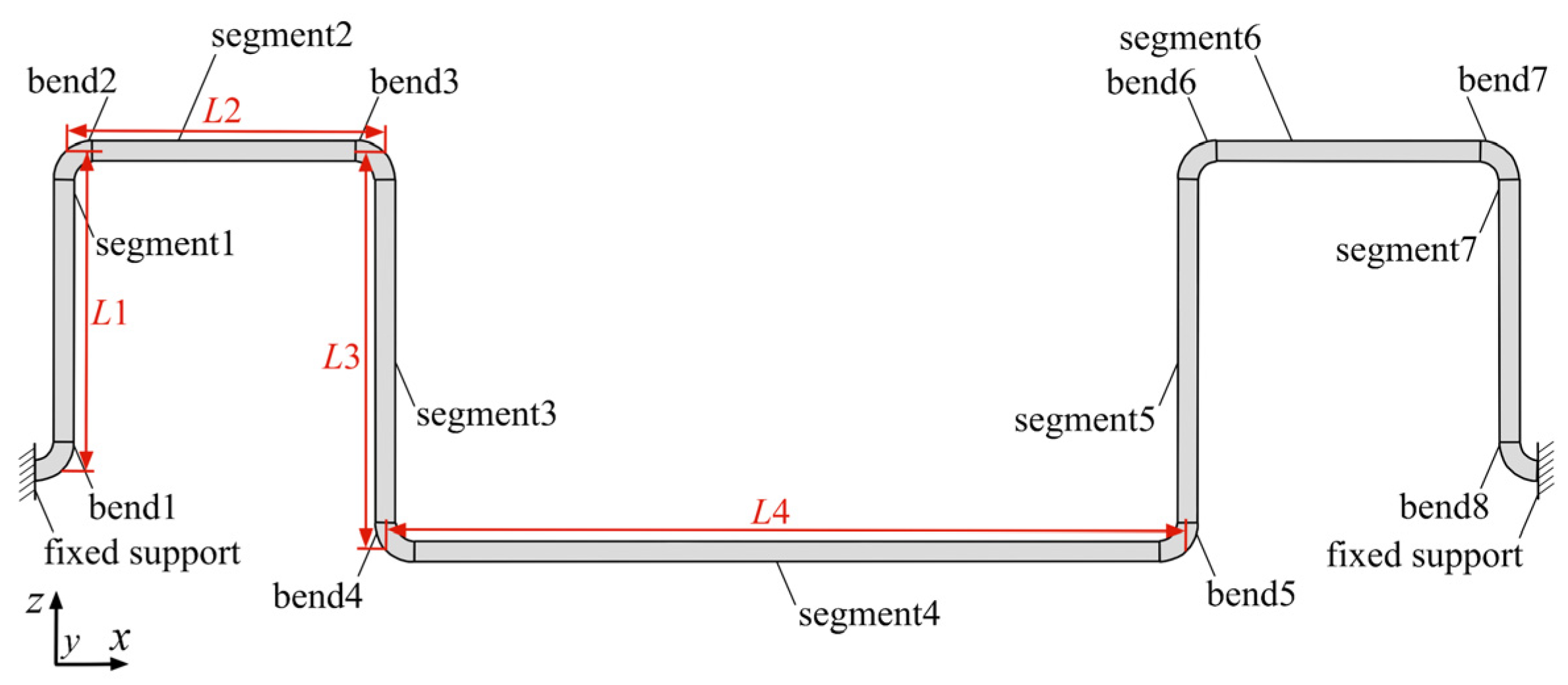








| Parameter | Value (mm) |
|---|---|
| Total length | 3744 |
| Inner diameter () | 48 |
| Outer diameter (D) | 60 |
| Wall thickness | 6 |
| Radius of bends | 72 |
| L1 | 800 |
| L2 | 800 |
| L3 | 1000 |
| L4 | 2000 |
| Internal Flow Conditions | ||||
| Case | Flow condition | Simulation time (s) | ||
| Mixed velocity vi (m/s) | Volume fraction of water rw | Stable state (s) | Pressure export (s) | |
| 1 | 1.00 | 0.5 | 15 | 10 |
| 2 | 2.00 | 0.2 | 15 | 10 |
| 3 | 2.00 | 0.5 | 15 | 10 |
| 4 | 2.00 | 0.8 | 15 | 10 |
| 5 | 4.00 | 0.5 | 15 | 10 |
| External Flow Conditions | ||||
| Case | Flow condition | Simulation time (s) | ||
| Velocity ve (m/s) | Flow direction (°) | Stable state (s) | Pressure export (s) | |
| 1 | 0.50 | 90 | 30 | 10 |
| 2 | 0.75 | 90 | 20 | 10 |
| 3 | 1.00 | 90 | 20 | 10 |
| Combined Internal and External Flow Conditions | ||||
| Case (total) | Flow condition | Simulation time (s) | ||
| 15 (5 × 3) | According to the internal and external flow conditions, respectively | 10 | ||
| Method | Internal Fluid Domain | External Fluid Domain | |
|---|---|---|---|
| Turbulent model | Realizable k-ε | SST k-Ω | |
| Pressure–velocity coupling scheme | PISO | PISO | |
| Spatial discretization | Gradient | Least squares cell-based | Least squares cell-based |
| Pressure | PRESTO! | PRESTO! | |
| Momentum | QUICK | QUICK | |
| Volume fraction | Geo-Reconstruct | - | |
| Turbulent kinetic energy | Second order upwind | Second order upwind | |
| Specific Dissipation Rate | Second order upwind | Second order upwind | |
| Transient formulation | First order implicit | Bounded second order implicit | |
| Mesh | Cross-Section | Path | ||
|---|---|---|---|---|
| Layers of the Wall Thickness | Parts Divided on the Circumference | Straight Segments (m) | Bends (m) | |
| L3C16 | 3 | 16 | 0.02 | 0.01 |
| L3C16R | 3 | 16 | 0.01 | 0.01 |
| L4C16 | 4 | 16 | 0.02 | 0.01 |
| L5C16 | 5 | 16 | 0.02 | 0.01 |
| L3C20 | 3 | 20 | 0.02 | 0.01 |
| L3C24 | 3 | 24 | 0.02 | 0.01 |
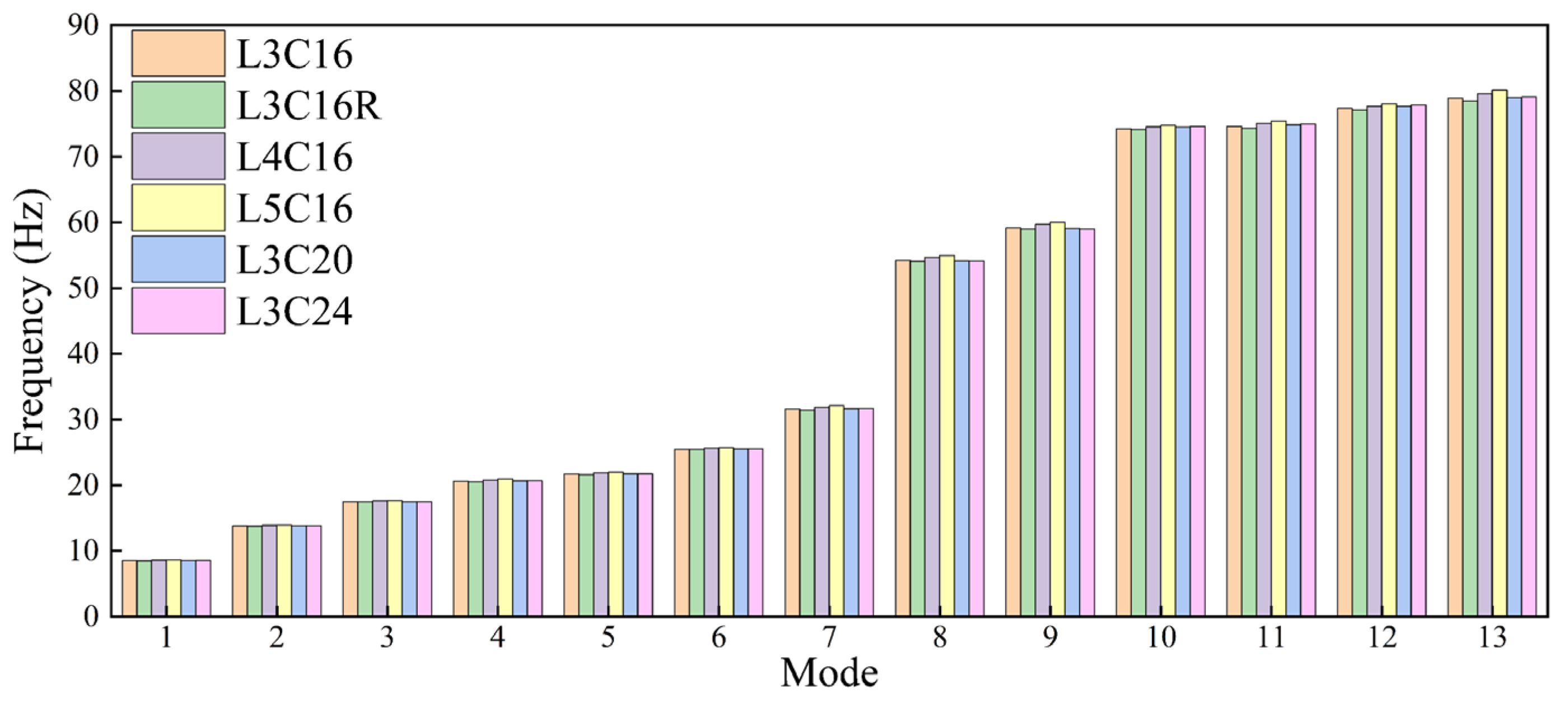
| Mode | Frequency (Hz) | Type |
|---|---|---|
| 1 | 8.520 | Out-of-plane |
| 2 | 13.825 | In-plane |
| 3 | 17.473 | In-plane |
| 4 | 20.628 | Out-of-plane |
| 5 | 21.707 | Out-of-plane |
| 6 | 25.497 | In-plane |
| 7 | 31.608 | Out-of-plane |
| 8 | 54.246 | In-plane |
| 9 | 59.186 | In-plane |
| 10 | 74.268 | In-plane |
| 11 | 74.622 | Out-of-plane |
| 12 | 77.364 | Out-of-plane |
| 13 | 78.933 | Out-of-plane |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Li, W.; Lin, S.; Han, F.; Zhou, X. Dynamic Response Analysis of a Subsea Rigid M-Shaped Jumper under Combined Internal and External Flows. J. Mar. Sci. Eng. 2024, 12, 1261. https://doi.org/10.3390/jmse12081261
Li G, Li W, Lin S, Han F, Zhou X. Dynamic Response Analysis of a Subsea Rigid M-Shaped Jumper under Combined Internal and External Flows. Journal of Marine Science and Engineering. 2024; 12(8):1261. https://doi.org/10.3390/jmse12081261
Chicago/Turabian StyleLi, Guangzhao, Wenhua Li, Shanying Lin, Fenghui Han, and Xingkun Zhou. 2024. "Dynamic Response Analysis of a Subsea Rigid M-Shaped Jumper under Combined Internal and External Flows" Journal of Marine Science and Engineering 12, no. 8: 1261. https://doi.org/10.3390/jmse12081261
APA StyleLi, G., Li, W., Lin, S., Han, F., & Zhou, X. (2024). Dynamic Response Analysis of a Subsea Rigid M-Shaped Jumper under Combined Internal and External Flows. Journal of Marine Science and Engineering, 12(8), 1261. https://doi.org/10.3390/jmse12081261






