Three-Dimensional Marine Magnetotelluric Parallel Forward Modeling in Conductive and Magnetic Anisotropic Medium Using Finite-Element Method Based on Secondary Field
Abstract
1. Introduction
2. Problem Formulation
2.1. Differential Equations
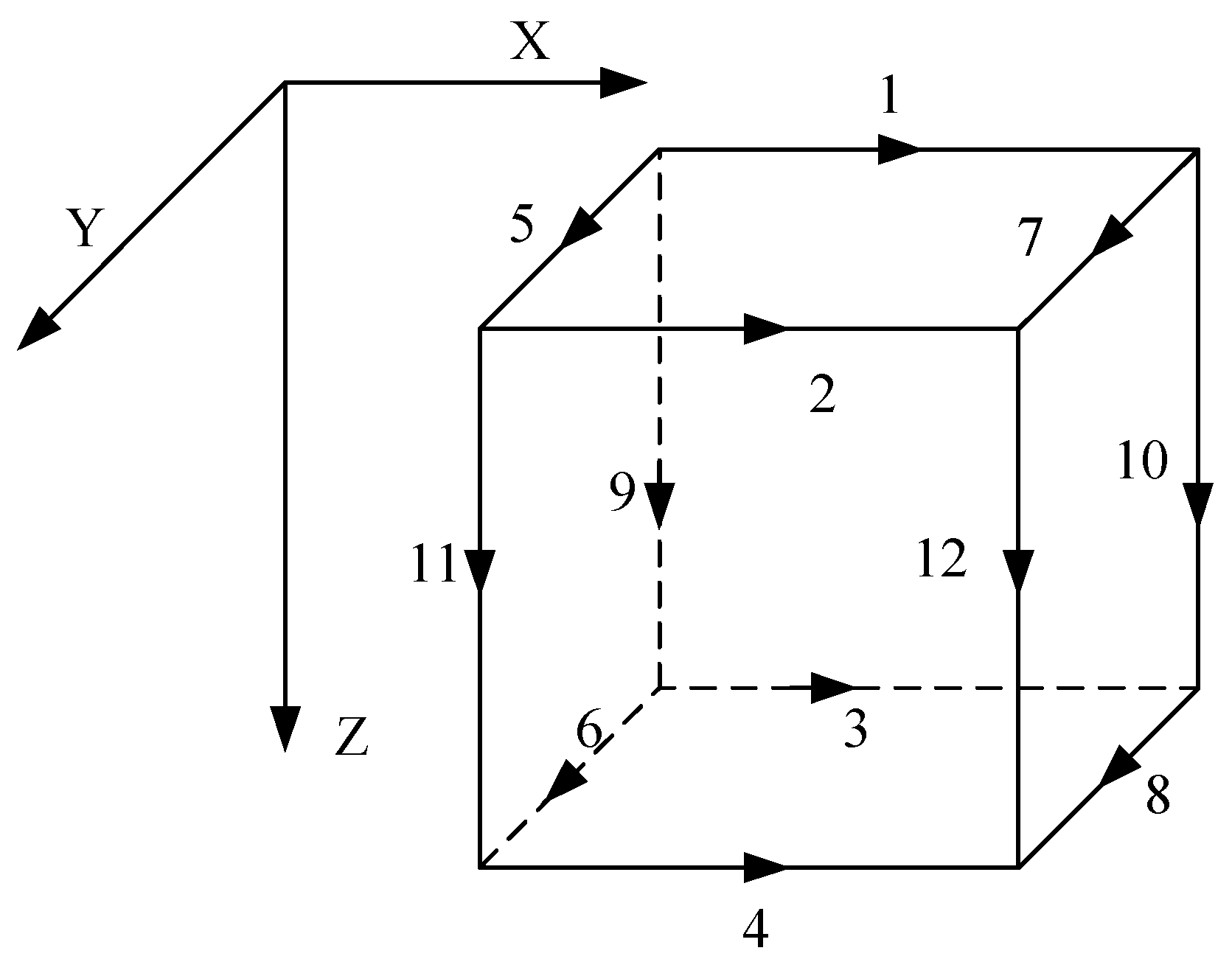
2.2. Finite Element Analysis
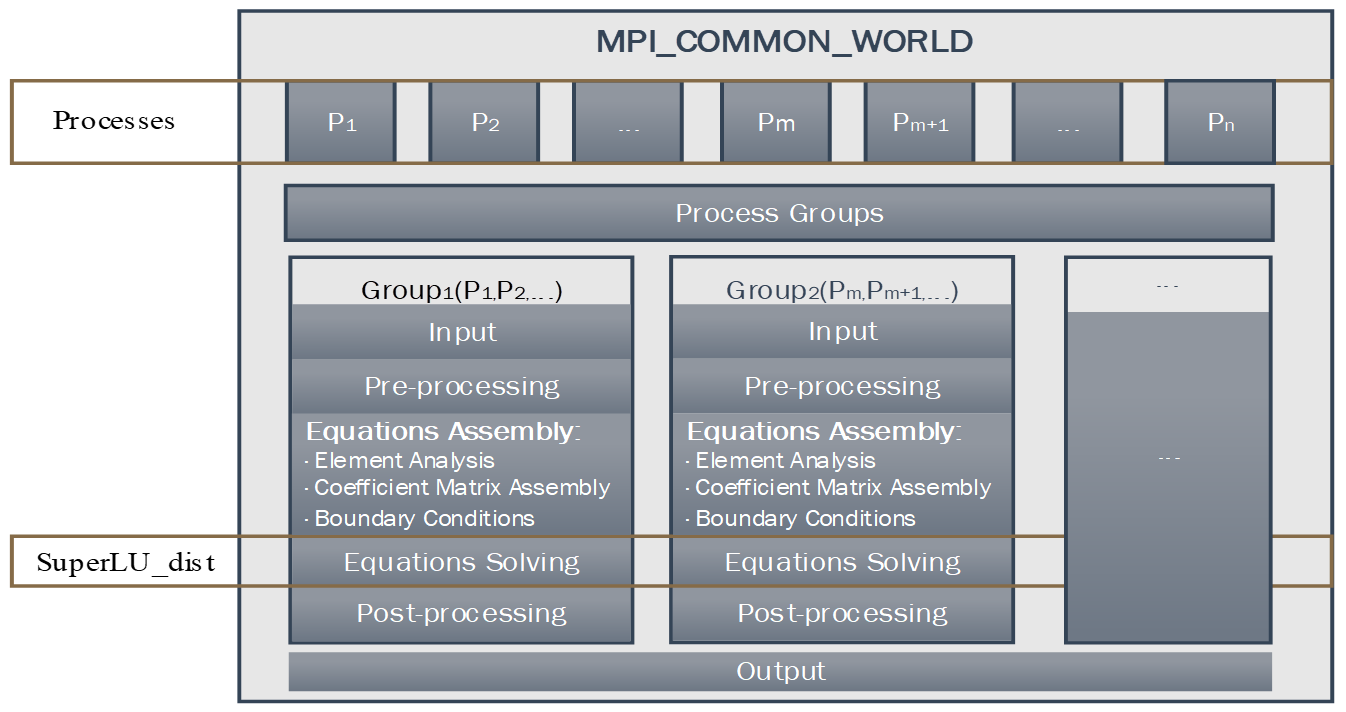
3. Multi-Level Parallel Algorithm
4. Numerical Results
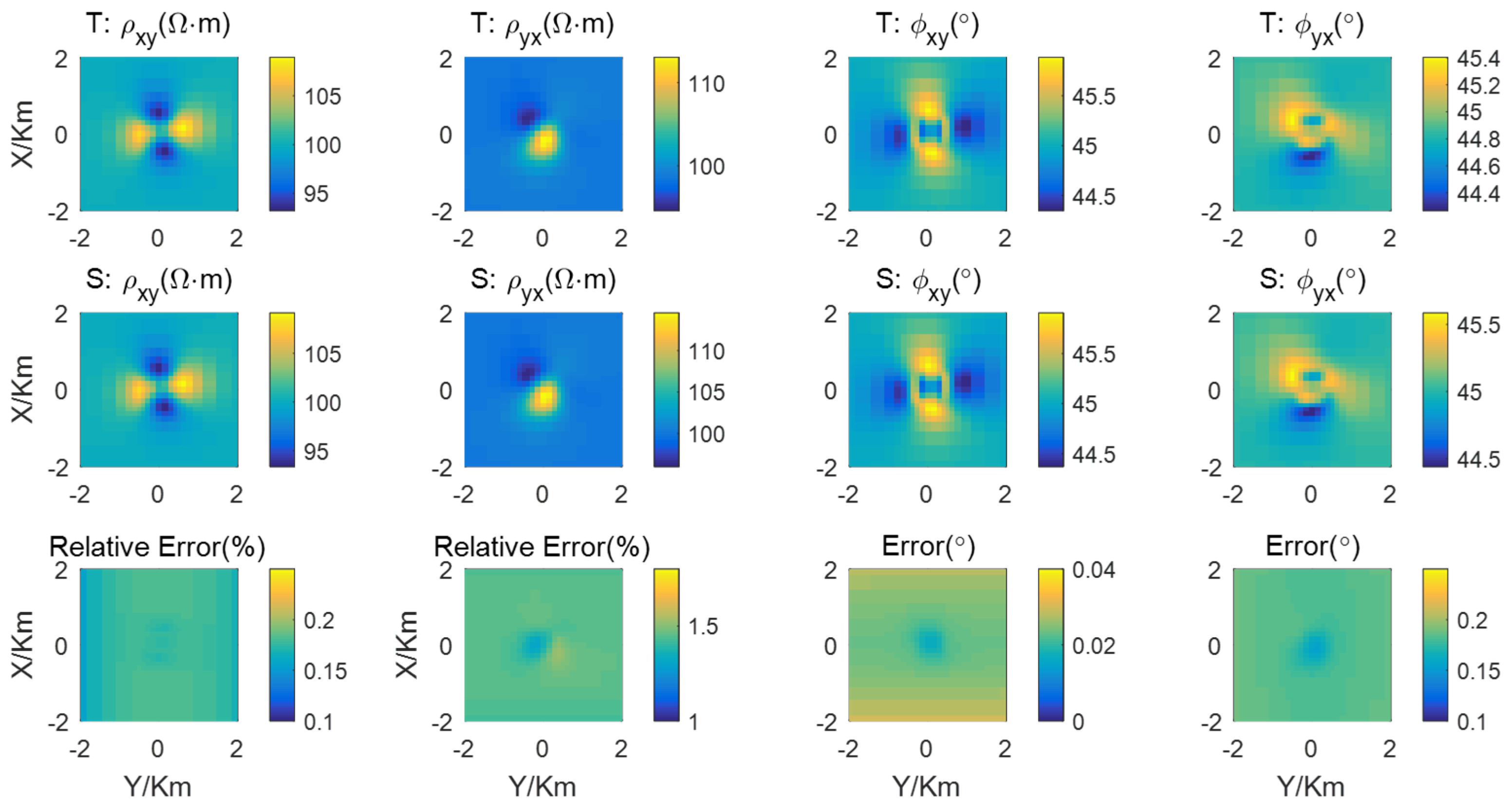
4.1. Accuracy Validation
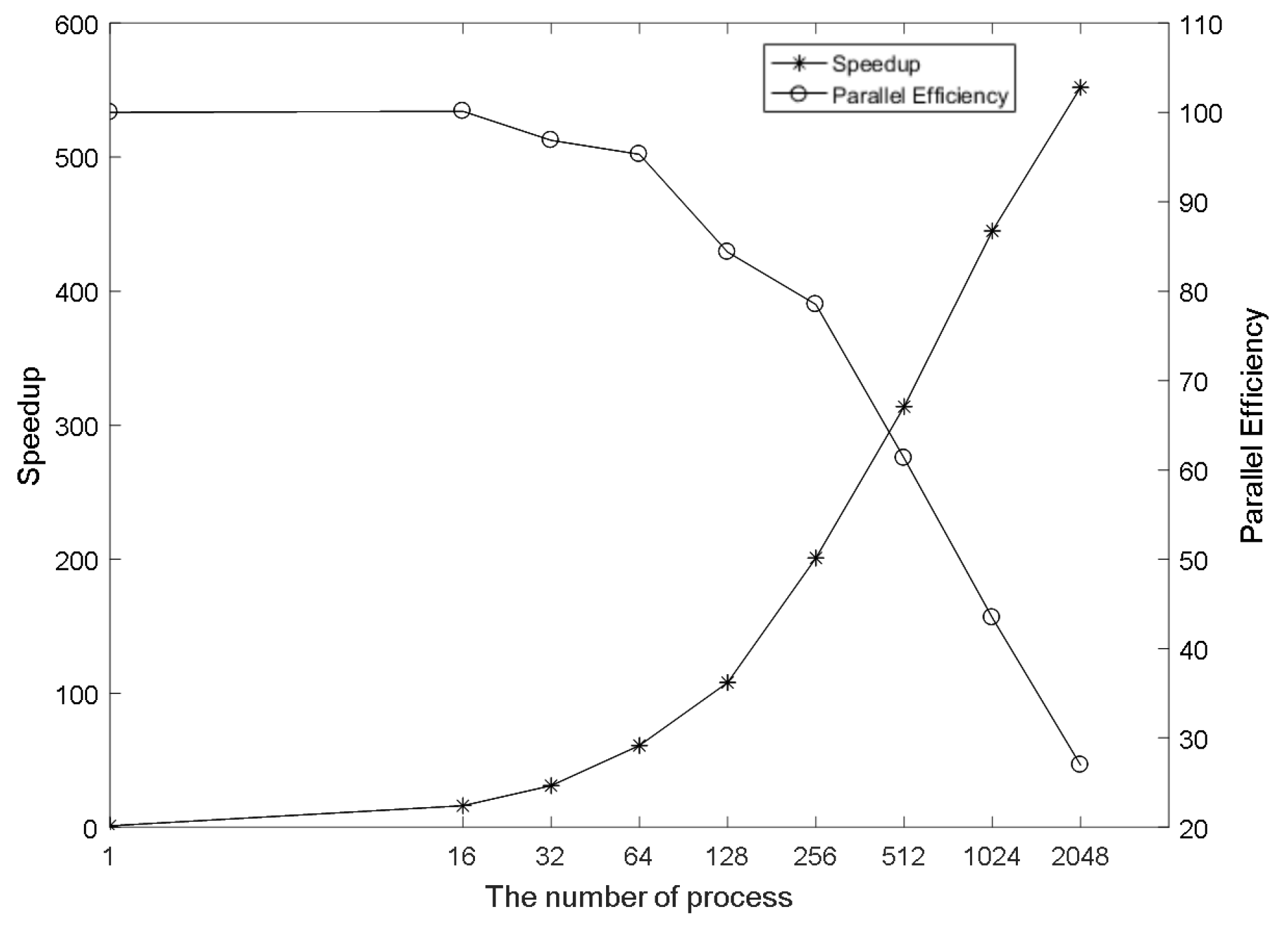
4.2. Scalability Test
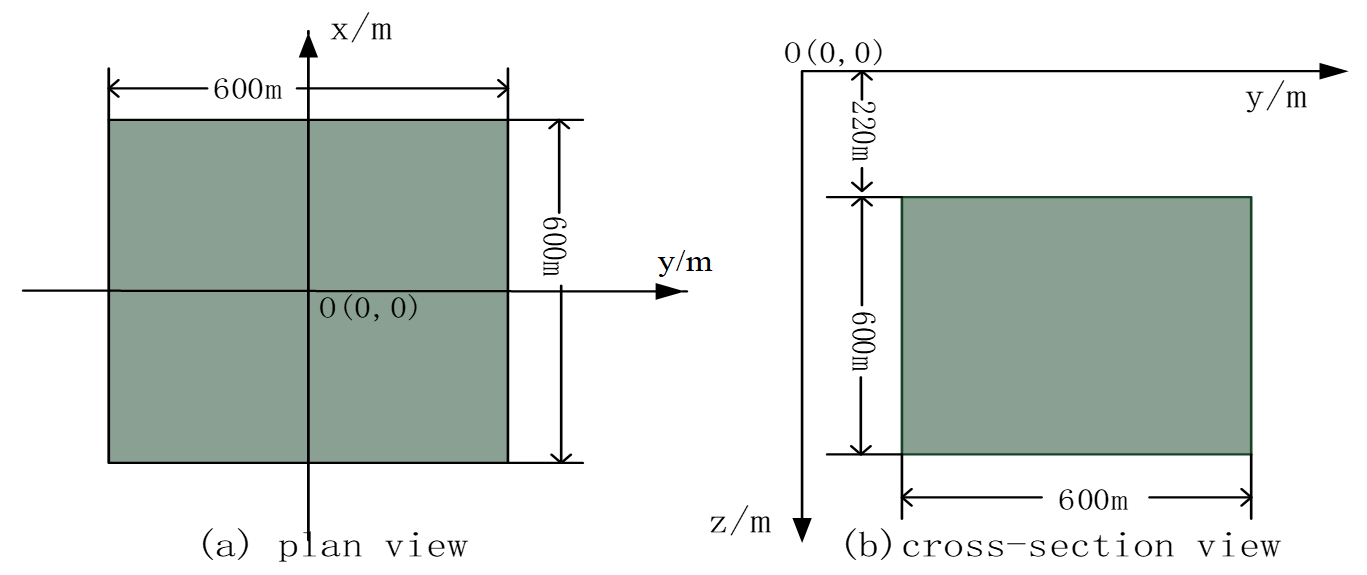
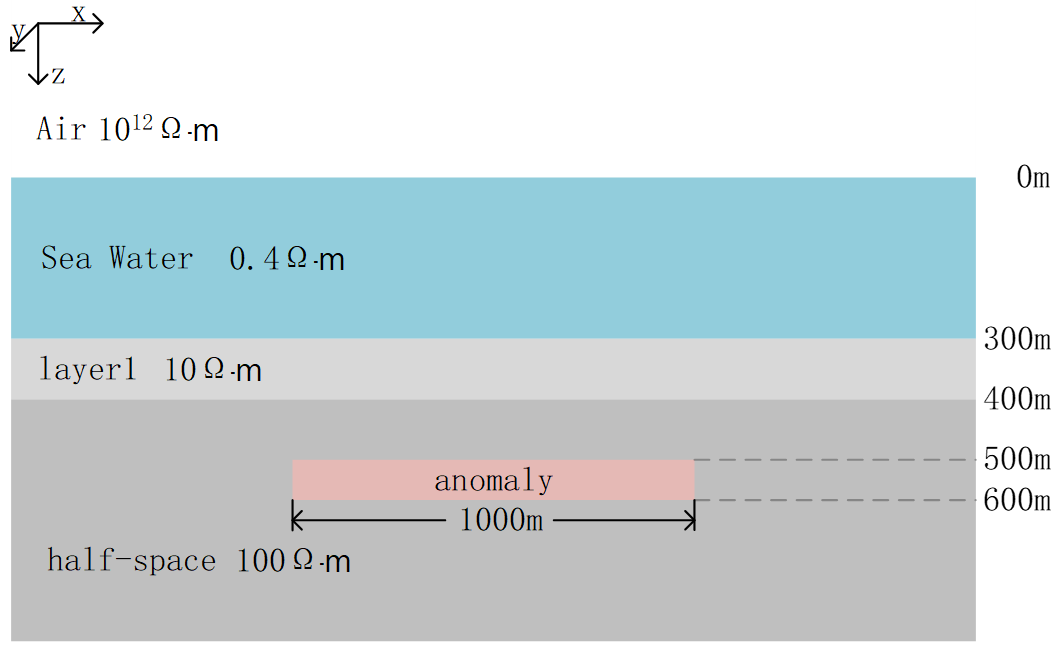
4.3. Typical Models
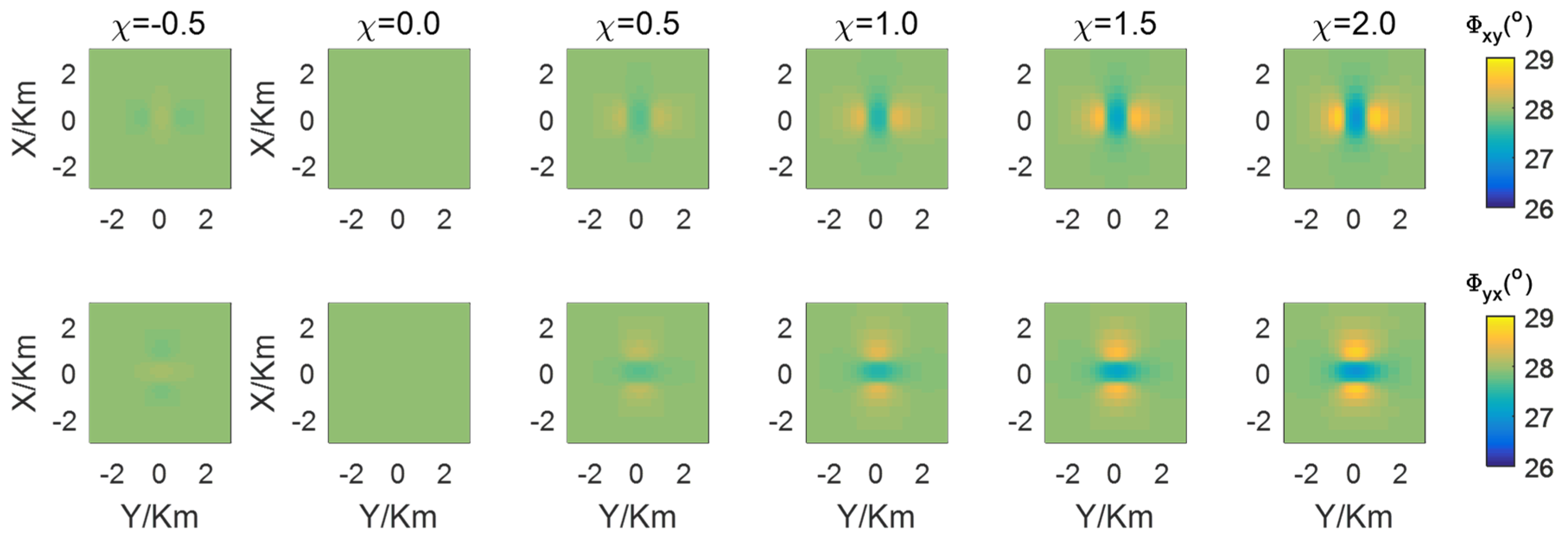
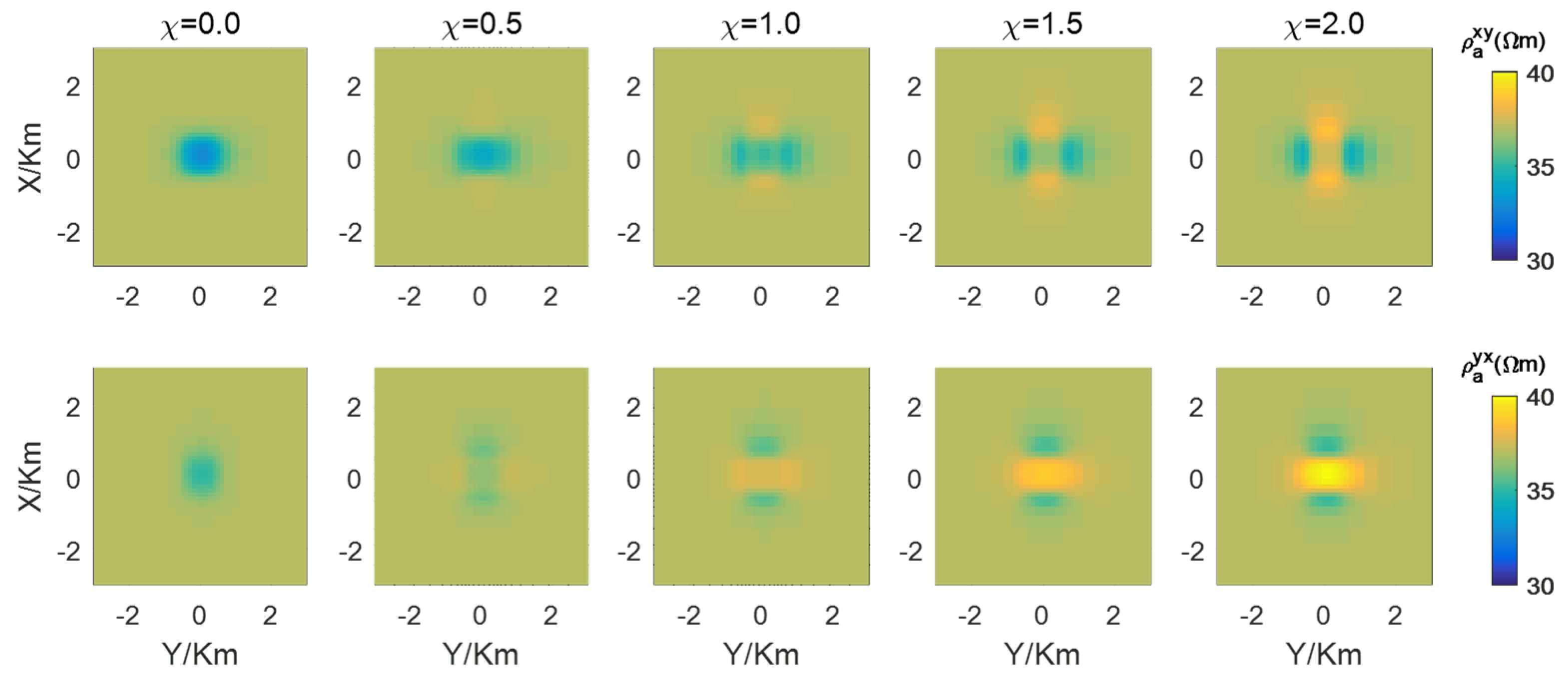
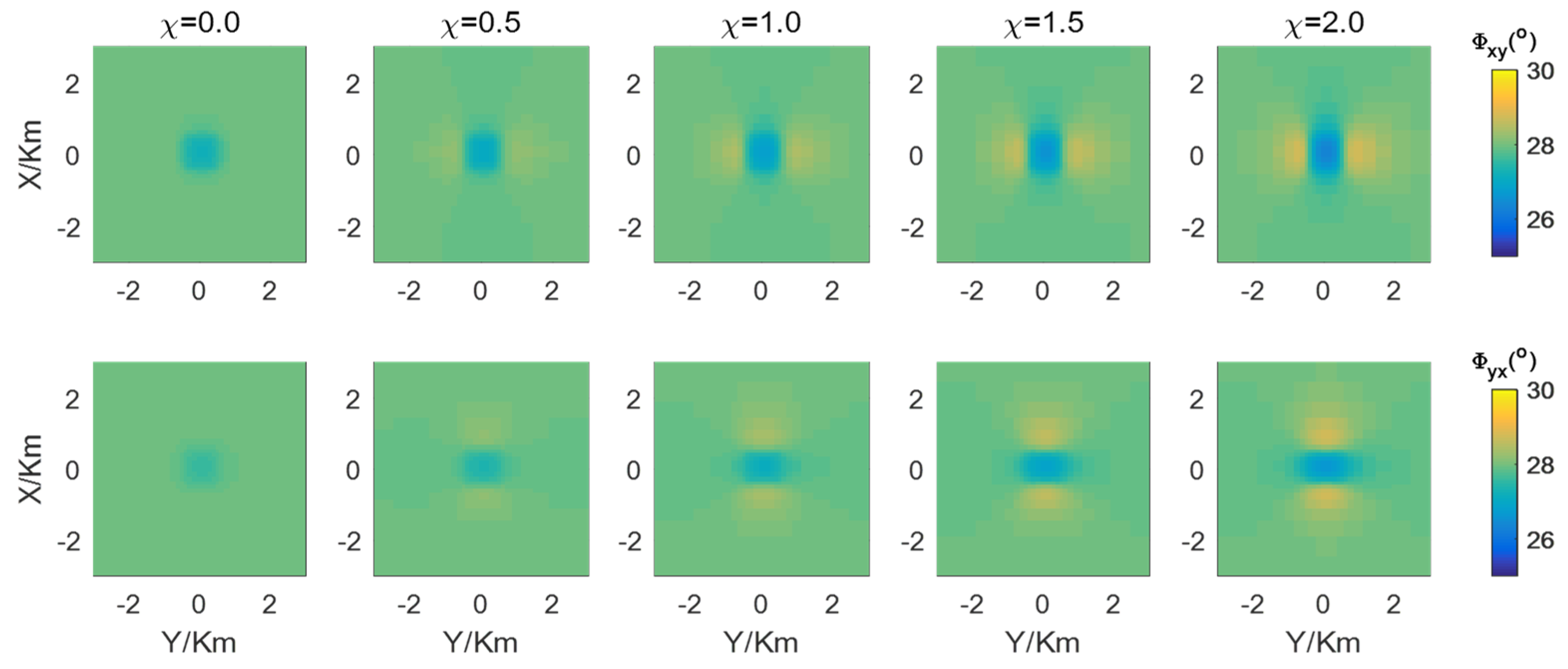
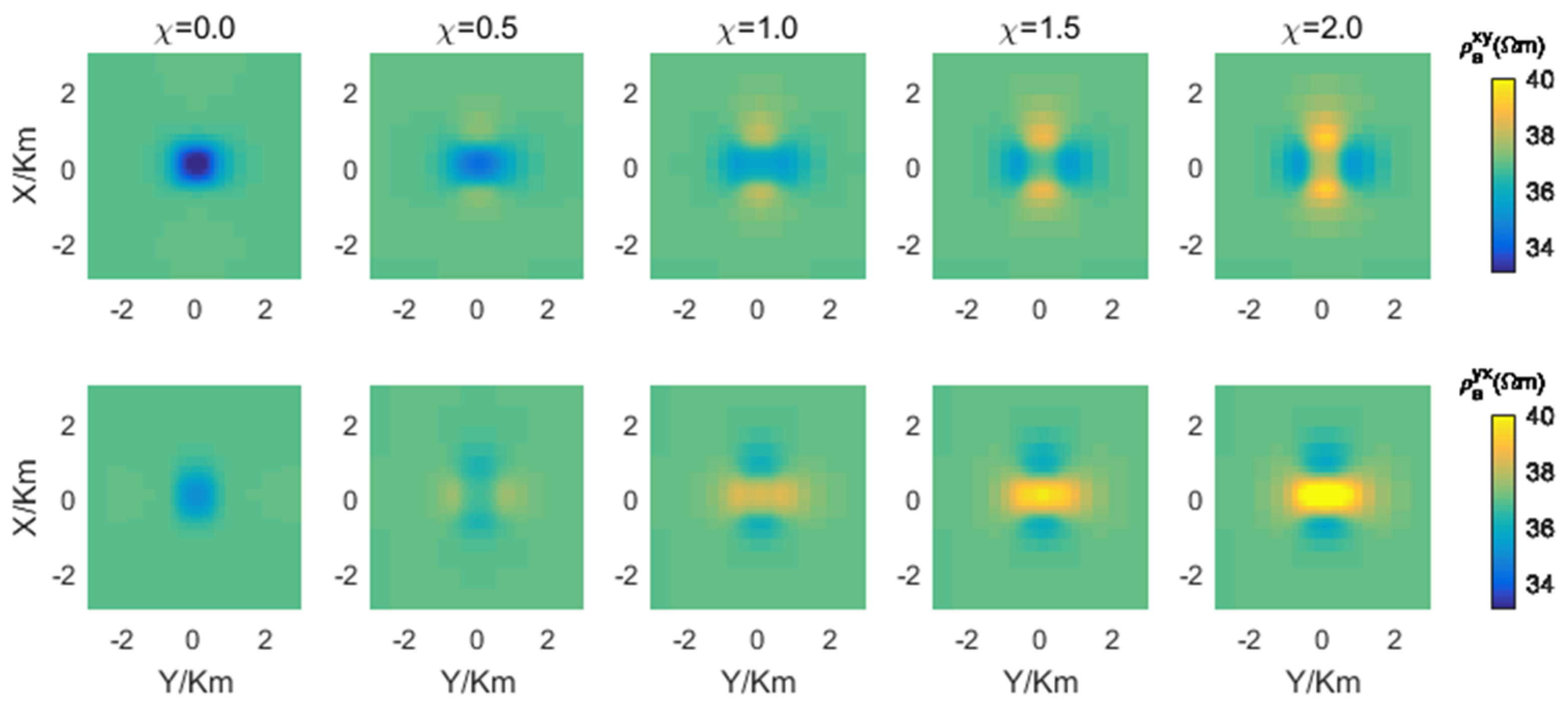
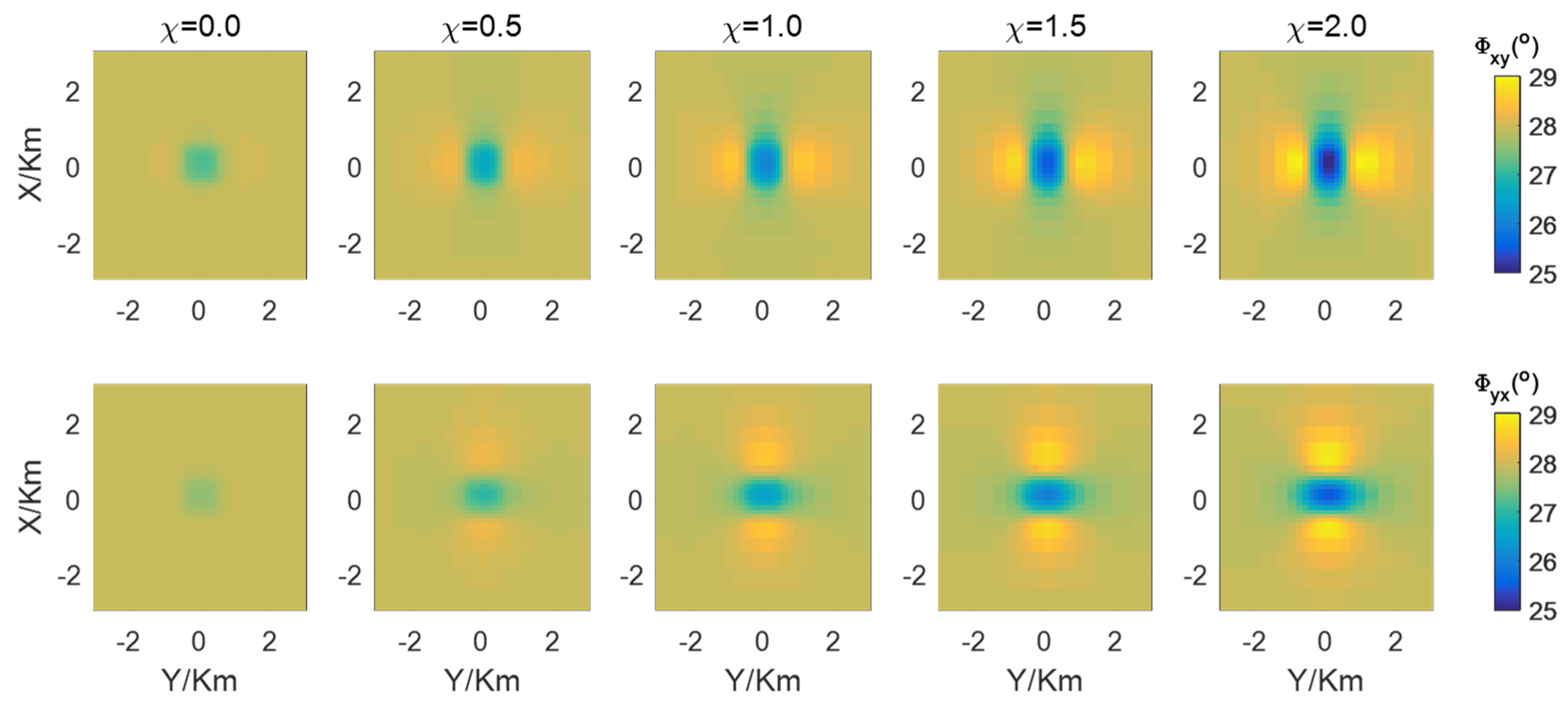
4.3.1. Cases with Single Anomaly
4.3.2. Case with Synthetic Anomalies
5. Analysis and Discussion
5.1. Accuracy Validation
5.2. Scalability Test
5.3. Typical Models
5.3.1. Cases with Single Anomaly
5.3.2. Case with Synthetic Anomalies
6. Conclusions
- (1)
- For a single conductive and magnetic anomaly, such as an anomaly with lower resistivity and higher susceptibility than the surrounding rocks, the apparent resistivities and phases can even be reversed due to the influence of susceptibility.
- (2)
- For synthetic anomalies, such as the combination of a conductive anomaly and a magnetic anomaly, the responses of the conductive anomaly can be affected or even covered by the influence of the magnetic anomaly.
- (3)
- The marine geological environment of practical MMT works is extremely intricate. It needs more attention for the influences of susceptibility, especially in magnetite-rich regions though non-zero susceptibility and conductive anisotropy complicate MMT’s responses.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. The Values of , , , and
- (1)
- The values of
- (2)
- The values of
- (3)
- The values of
- (4)
- The values of
References
- Zhdanov, M.S.; Wan, L.; Gribenko, A.; Čuma, M.; Key, K.; Constable, S. Large-scale 3D inversion of marine magnetotelluric data: Case study from the Gemini prospect, Gulf of Mexico. Geophysics 2011, 76, F77–F87. [Google Scholar] [CrossRef]
- Worzewski, T.; Jegen, M.; Kopp, H.; Brasse, H.; Castillo, W.T. Magnetotelluric image of the fluid cycle in the Costa Rican subduction zone. Nat. Geosci. 2011, 4, 108–111. [Google Scholar] [CrossRef]
- Castillo-Reyes, O.; Queralt, P.; Piñas-Varas, P.; Ledo, J.; Rojas, O. Electromagnetic Subsurface Imaging in the Presence of Metallic Structures: A Review of Numerical Strategies. Surv. Geophys. 2024, 34, 1–35. [Google Scholar] [CrossRef]
- Constable, S.; Key, K.; Lewis, L. Mapping offshore sedimentary structure using electromagnetic methods and terrain effects in marine magnetotelluric data. Geophys. J. Int. 2009, 176, 431–442. [Google Scholar] [CrossRef]
- Key, K.; Constable, S. Coast effect distortion of marine magnetotelluric data: Insights from a pilot study offshore northeastern Japan. Phys. Earth Planet. Inter. 2011, 184, 194–207. [Google Scholar] [CrossRef]
- Hansen, R.O.; Racic, L.; Grauch, V.J.S. Magnetic methods in near-surface geophysics. In Near-Surface Geophysics; Society of Exploration Geophysicists: Houston, TX, USA, 2005; pp. 151–176. [Google Scholar]
- Mickus, K. Magnetic Method. 2023. Available online: https://www.researchgate.net/publication/228994566_Magnetic_Method (accessed on 21 July 2024).
- Chen, L.; Xiao, T.; Liu, J.; Zheng, X.; Liu, J.; Wang, Y. One-dimensional magnetotelluric modeling in magnetic and resistive axial anisotropic media. Prog. Geophys. 2022, 37, 2373–2380. (In Chinese) [Google Scholar]
- Zhang, Z.; Oldenburg, D.W. Recovering magnetic susceptibility from electromagnetic data over a one-dimensional earth. Geophys. J. Int. 1997, 130, 422–434. [Google Scholar] [CrossRef][Green Version]
- Zhang, Z.; Oldenburg, D.W. Simultaneous reconstruction of 1-D susceptibility and conductivity from EM data. In SEG Technical Program Expanded Abstracts; Society of Exploration Geophysicists: Houston, TX, USA, 1996; pp. 241–244. [Google Scholar]
- Thomas, L. Electromagnetic sounding with susceptibility among the model parameters. Geophysics 1977, 42, 92–96. [Google Scholar] [CrossRef]
- Sasaki, Y.; Kim, J.H.; Cho, S.J. Multidimensional inversion of loop-loop frequency-domain EM data for resistivity and magnetic susceptibility. Geophysics 2010, 75, F213–F223. [Google Scholar] [CrossRef]
- Zhang, Z.; Xie, S.; Li, M.; Ma, X.; Li, W. Two-dimensional magnetotelluric inversion considering resistivity and magnetic permittivity. J. East China Univ. Technol. (Nat. Sci.) 2018, 41, 405–412. [Google Scholar]
- Pek, J.; Santos, F.A. Magnetotelluric impedances and parametric sensitivities for 1-D anisotropic layered media. Comput. Geosci. 2002, 28, 939–950. [Google Scholar] [CrossRef]
- Yin, C.; Maurer, H.M. Electromagnetic induction in a layered earth with arbitrary anisotropy. Geophysics 2001, 66, 1405–1416. [Google Scholar] [CrossRef]
- Li, Y.; Pek, J. Adaptive finite element modelling of two-dimensional magnetotelluric fields in general anisotropic media. Geophys. J. Int. 2008, 175, 942–954. [Google Scholar] [CrossRef]
- Weidelt, P.; Oristaglio, M.; Spies, B. 3-D conductivity models: Implications of electrical anisotropy. Three-Dimens. Electromagn. 1999, 7, 119–137. [Google Scholar]
- Xu, Z. Research on Three-Dimensional Magnetotelluric Modeling in General Anisotropic Media. Master’s Thesis, Ocean University of China, Qingdao, China, 2015. [Google Scholar]
- Xiao, T.; Liu, Y.; Wang, Y.; Fu, L.-Y. Three-dimensional magnetotelluric modeling in anisotropic media using edge-based finite element method. J. Appl. Geophys. 2018, 149, 1–9. [Google Scholar] [CrossRef]
- Liu, Y.; Xu, Z.; Li, Y. Adaptive finite element modelling of three-dimensional magnetotelluric fields in general anisotropic media. J. Appl. Geophys. 2018, 151, 113–124. [Google Scholar] [CrossRef]
- Xiao, T.; Huang, X.; Wang, Y. Three-dimensional magnetotelluric modelling in anisotropic media using the A-phi method. Explor. Geophys. 2019, 50, 31–41. [Google Scholar] [CrossRef]
- Xiao, T.; Huang, X.; Wang, Y. 3D MT modeling using the T–Ω method in general anisotropic media. J. Appl. Geophys. 2019, 160, 171–182. [Google Scholar] [CrossRef]
- Zhou, J.; Hu, X.; Xiao, T.; Cai, H.; Li, J.; Peng, R.; Long, Z. Three-dimensional edge-based finite element modeling of magnetotelluric data in anisotropic media with a divergence correction. J. Appl. Geophys. 2021, 189, 104324. [Google Scholar] [CrossRef]
- Bai, N.; Zhou, J.; Hu, X.; Han, B. 3D edge-based and nodal finite element modeling of magnetotelluric in general anisotropic media. Comput. Geosci. 2022, 158, 104975. [Google Scholar] [CrossRef]
- Xiao, T.; Wang, Y.; Huang, X.; He, G.; Liu, J. Magnetotelluric responses of three-dimensional conductive and magnetic anisotropic anomalies. Geophys. Prospect. 2020, 68, 1016–1040. [Google Scholar] [CrossRef]
- Yu, N.; Chen, Z.; Wu, X.; Kong, W.; Chen, H.; Li, T.; Feng, X. Unstructured Grid Finite Element Modeling of the Three-Dimensional Magnetotelluric Responses in a Model with Arbitrary Conductivity and Magnetic Susceptibility Anisotropies. IEEE Trans. Geosci. Remote Sens. 2024, 62, 1–13. [Google Scholar] [CrossRef]
- SuperLU: Home Page. Available online: https://www.nersc.gov (accessed on 21 July 2024).
- Cullity, B.D.; Graham, C.D. Introduction to Magnetic Materials; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Greiner, W. Classical Electrodynamics; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Jin, J.M. The Finite Element Method in Electromagnetics, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2002. [Google Scholar]
- Castillo-Reyes, O.; Modesto, D.; Queralt, P.; Marcuello, A.; Ledo, J.; Amor-Martin, A.; de la Puente, J.; García-Castillo, L.E. 3D magnetotelluric modeling using high-order tetrahedral Nédélec elements on massively parallel computing platforms. Comput. Geosci. 2022, 160, 105030. [Google Scholar] [CrossRef]
- Castillo-Reyes, O.; de la Puente, J.; Cela, J.M. PETGEM: A parallel code for 3D CSEM forward modeling using edge finite elements. Comput. Geosci. 2018, 119, 123–136. [Google Scholar] [CrossRef]





| Modes | Dofs | NoNs | Runtime (m) | Memory (GB) |
|---|---|---|---|---|
| Serial | / | / | 7015.20 | 1.22 |
| Parallel | 20,218,352 | 2,613,227,456 | 12.70 | 38.94 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Z.; Xiao, T.; Zhou, J.; Zhu, X.; Yang, B.; Gong, C.; Liu, J.; Wang, Y. Three-Dimensional Marine Magnetotelluric Parallel Forward Modeling in Conductive and Magnetic Anisotropic Medium Using Finite-Element Method Based on Secondary Field. J. Mar. Sci. Eng. 2024, 12, 1750. https://doi.org/10.3390/jmse12101750
Zhou Z, Xiao T, Zhou J, Zhu X, Yang B, Gong C, Liu J, Wang Y. Three-Dimensional Marine Magnetotelluric Parallel Forward Modeling in Conductive and Magnetic Anisotropic Medium Using Finite-Element Method Based on Secondary Field. Journal of Marine Science and Engineering. 2024; 12(10):1750. https://doi.org/10.3390/jmse12101750
Chicago/Turabian StyleZhou, Zongyi, Tiaojie Xiao, Junjun Zhou, Xiaoxiong Zhu, Bo Yang, Chunye Gong, Jie Liu, and Yun Wang. 2024. "Three-Dimensional Marine Magnetotelluric Parallel Forward Modeling in Conductive and Magnetic Anisotropic Medium Using Finite-Element Method Based on Secondary Field" Journal of Marine Science and Engineering 12, no. 10: 1750. https://doi.org/10.3390/jmse12101750
APA StyleZhou, Z., Xiao, T., Zhou, J., Zhu, X., Yang, B., Gong, C., Liu, J., & Wang, Y. (2024). Three-Dimensional Marine Magnetotelluric Parallel Forward Modeling in Conductive and Magnetic Anisotropic Medium Using Finite-Element Method Based on Secondary Field. Journal of Marine Science and Engineering, 12(10), 1750. https://doi.org/10.3390/jmse12101750







