Analysis of Stress–Strain Characteristics and Signal Coherence of Low-Specific-Speed Impeller Based on Fluid–Structure Interaction
Abstract
:1. Introduction
2. Numeral Calculations
2.1. Objective Pump Model
2.2. Objective Pump Model
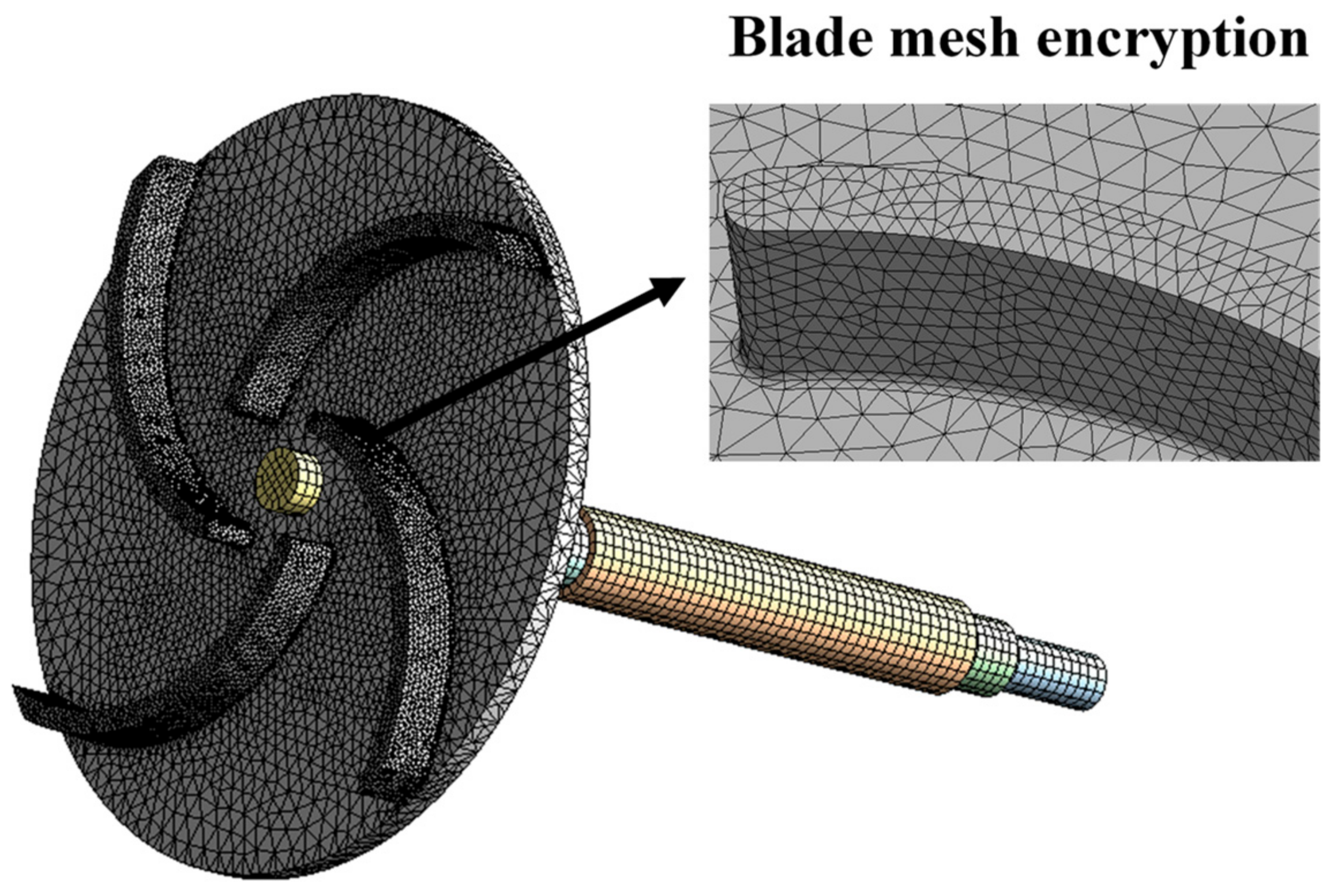
2.3. Meshing and Mesh Independence Verification
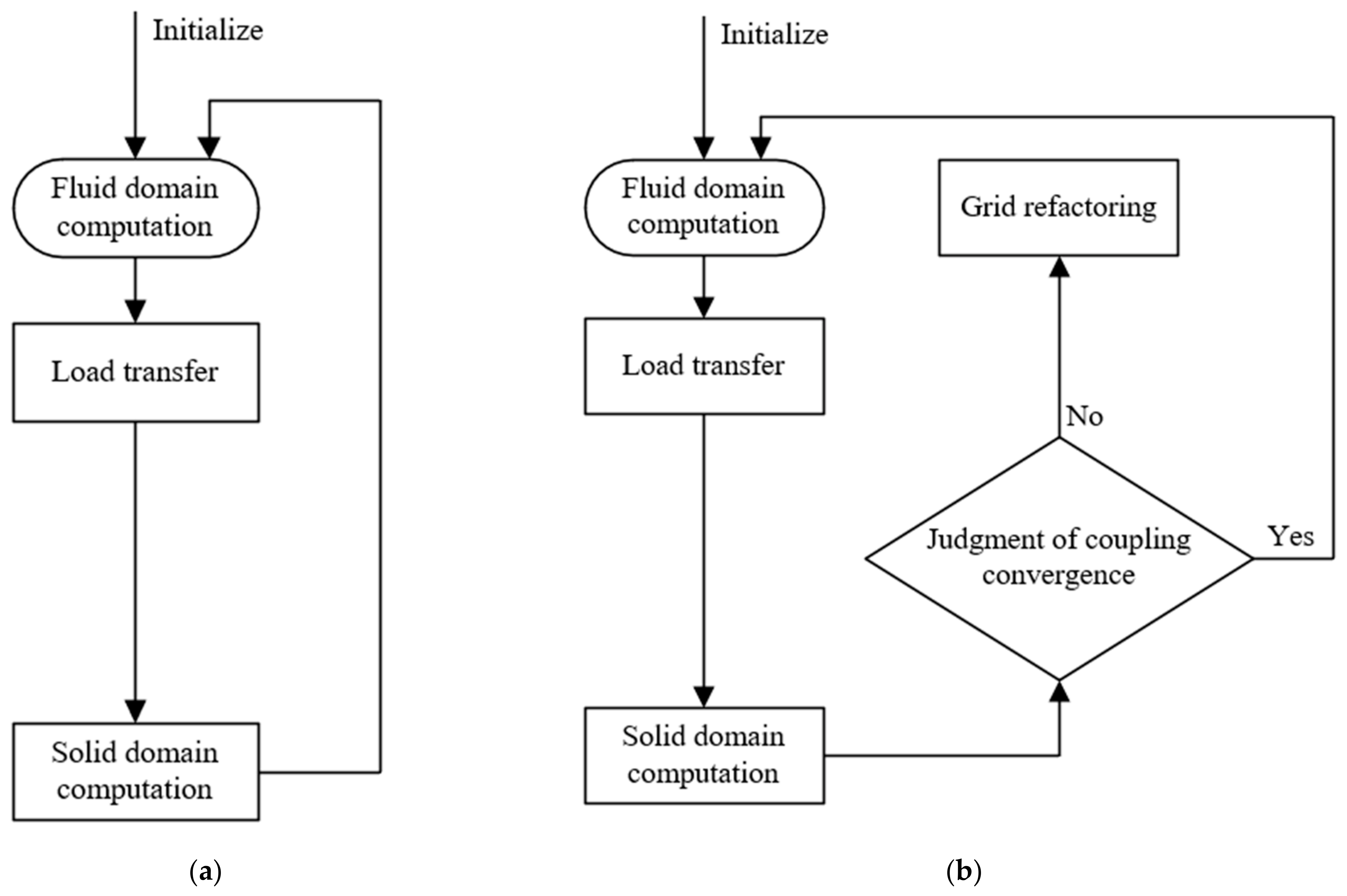
2.4. One-Way FSI
2.5. Two-Way FSI
2.6. Modal Analysis Methods
3. Result Analysis
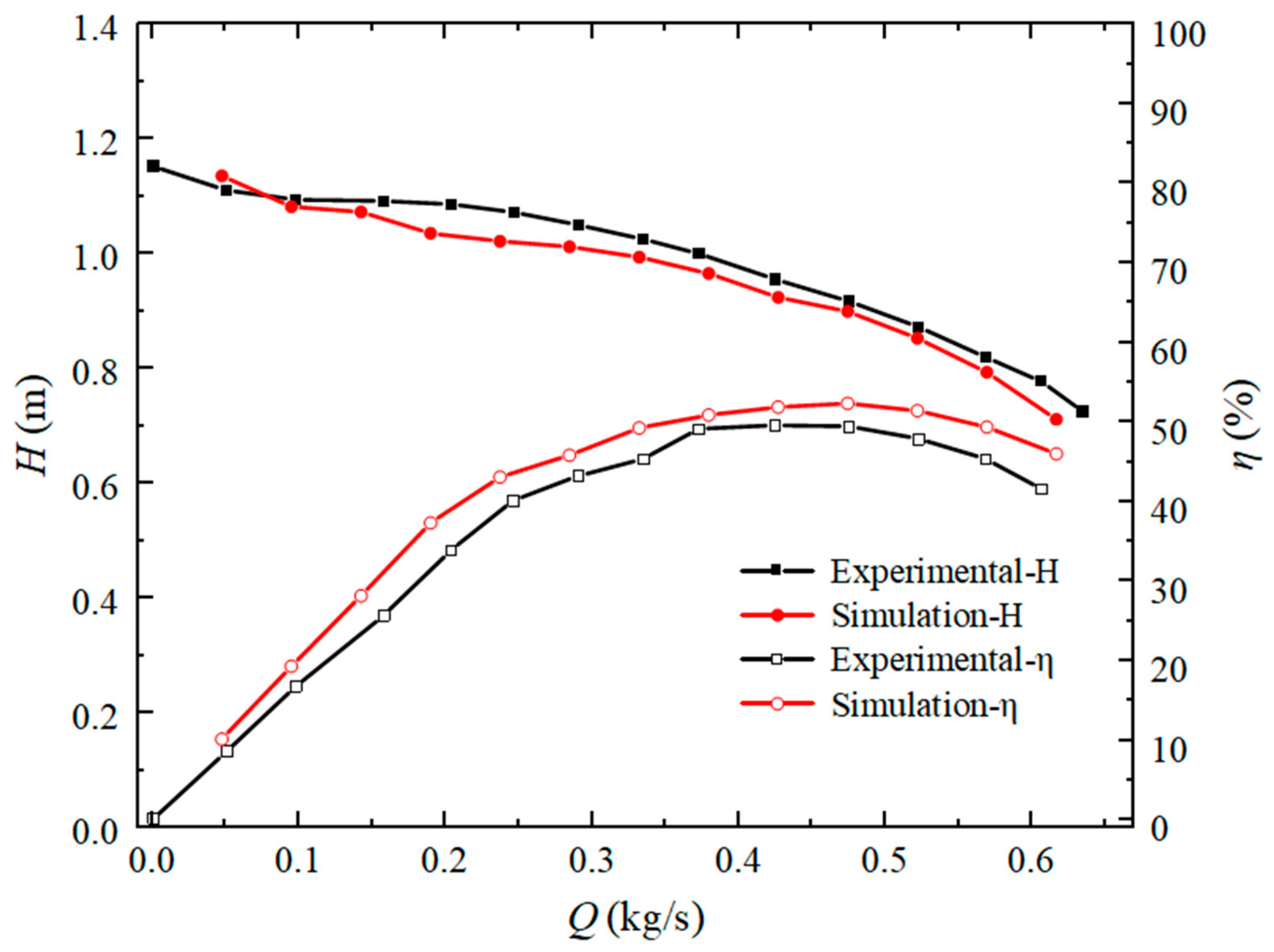
3.1. Analysis of External Characteristics
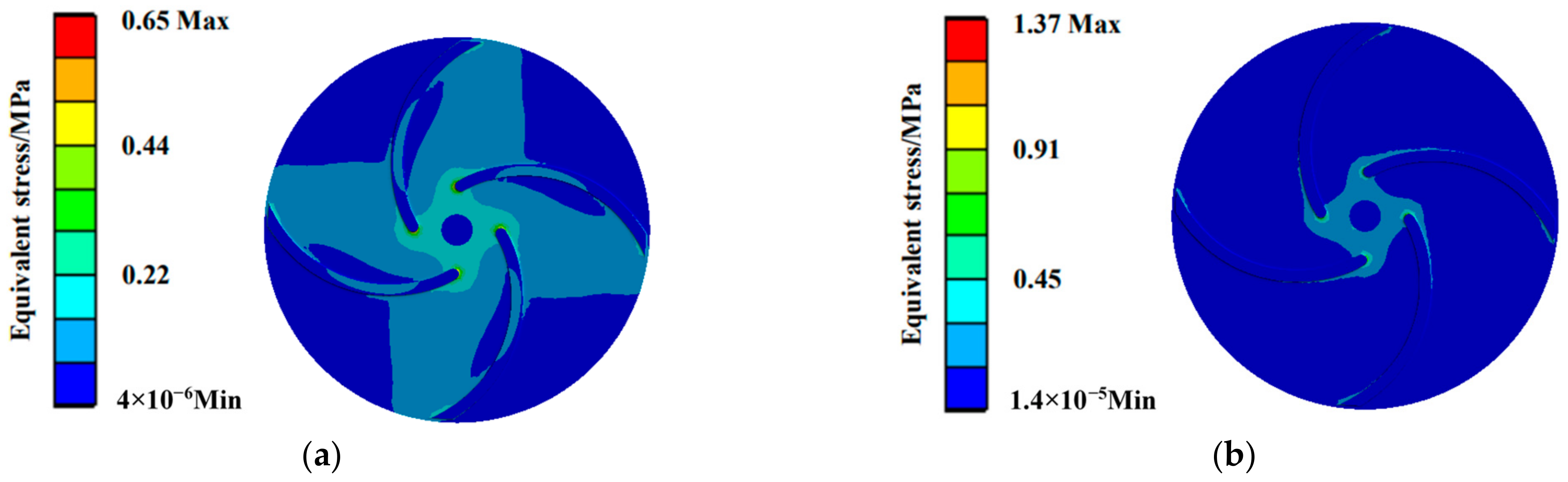
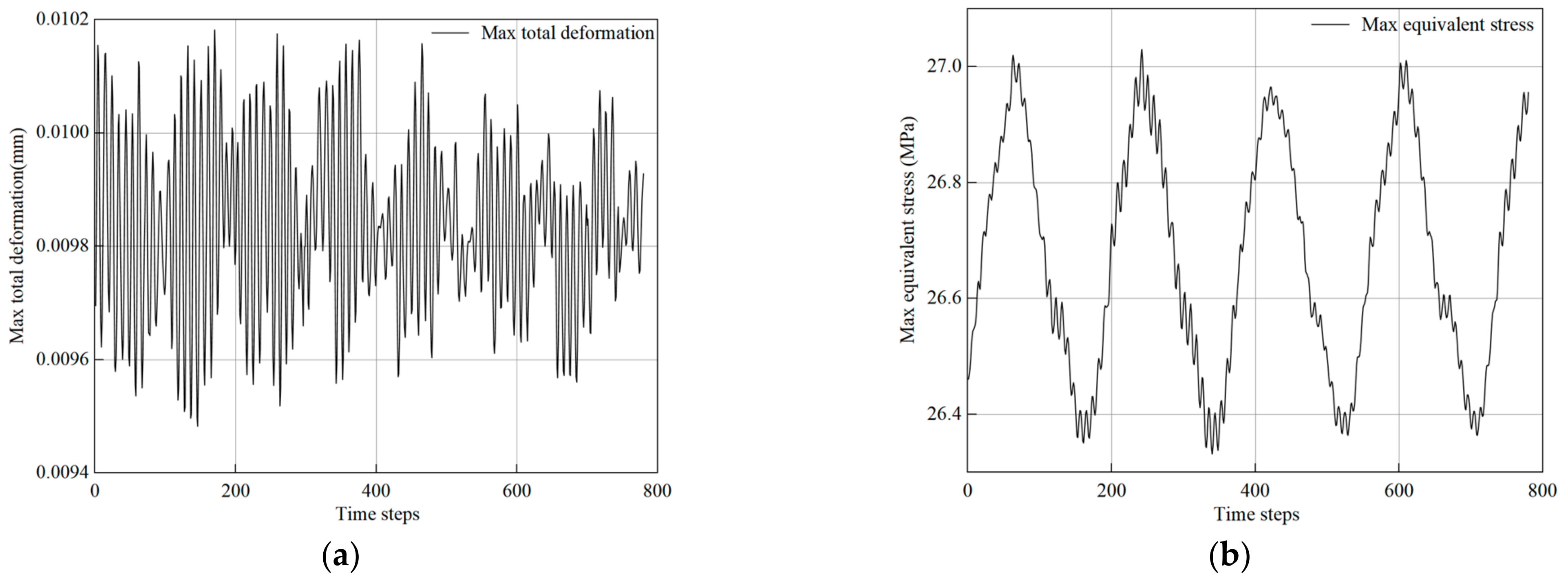
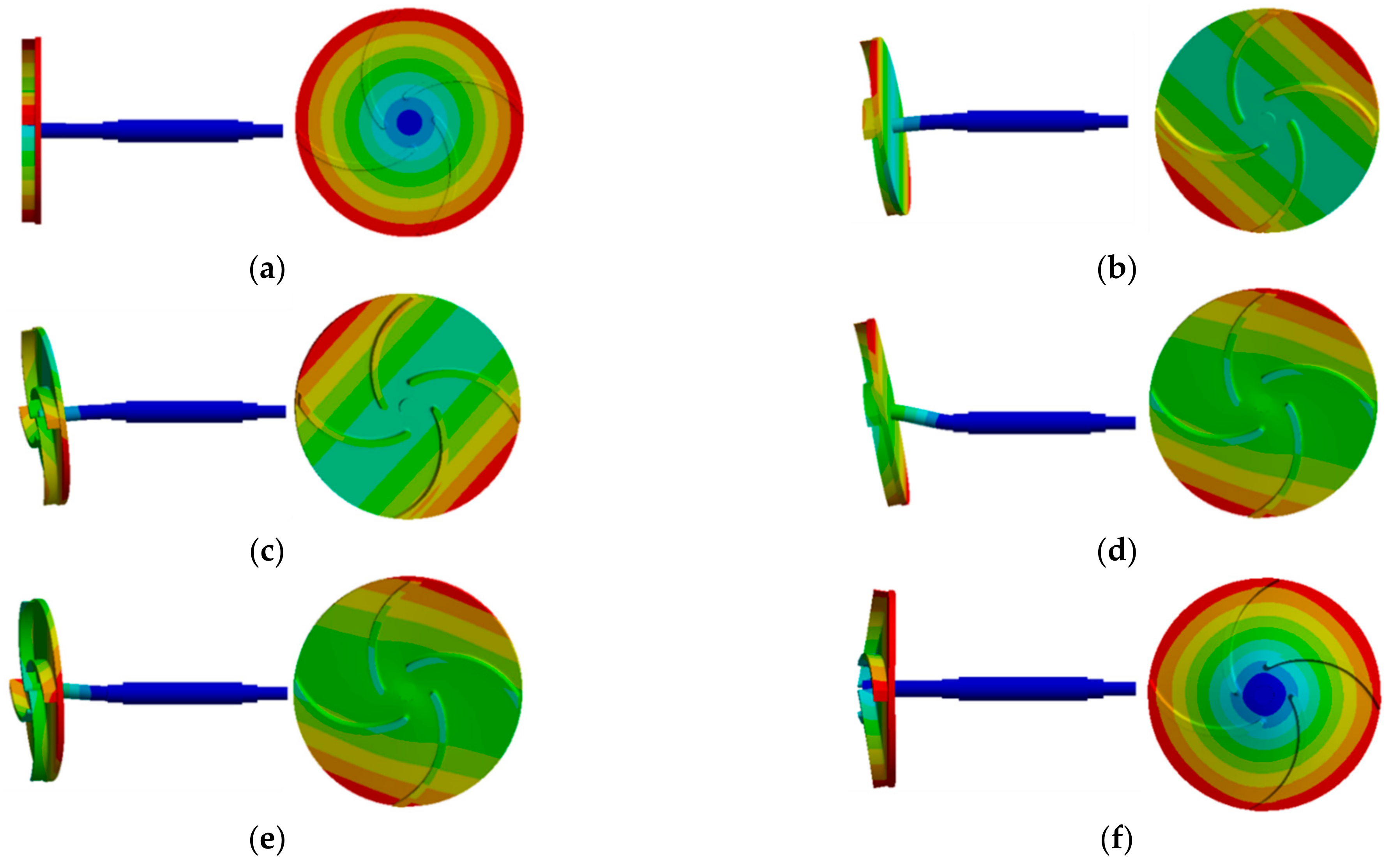
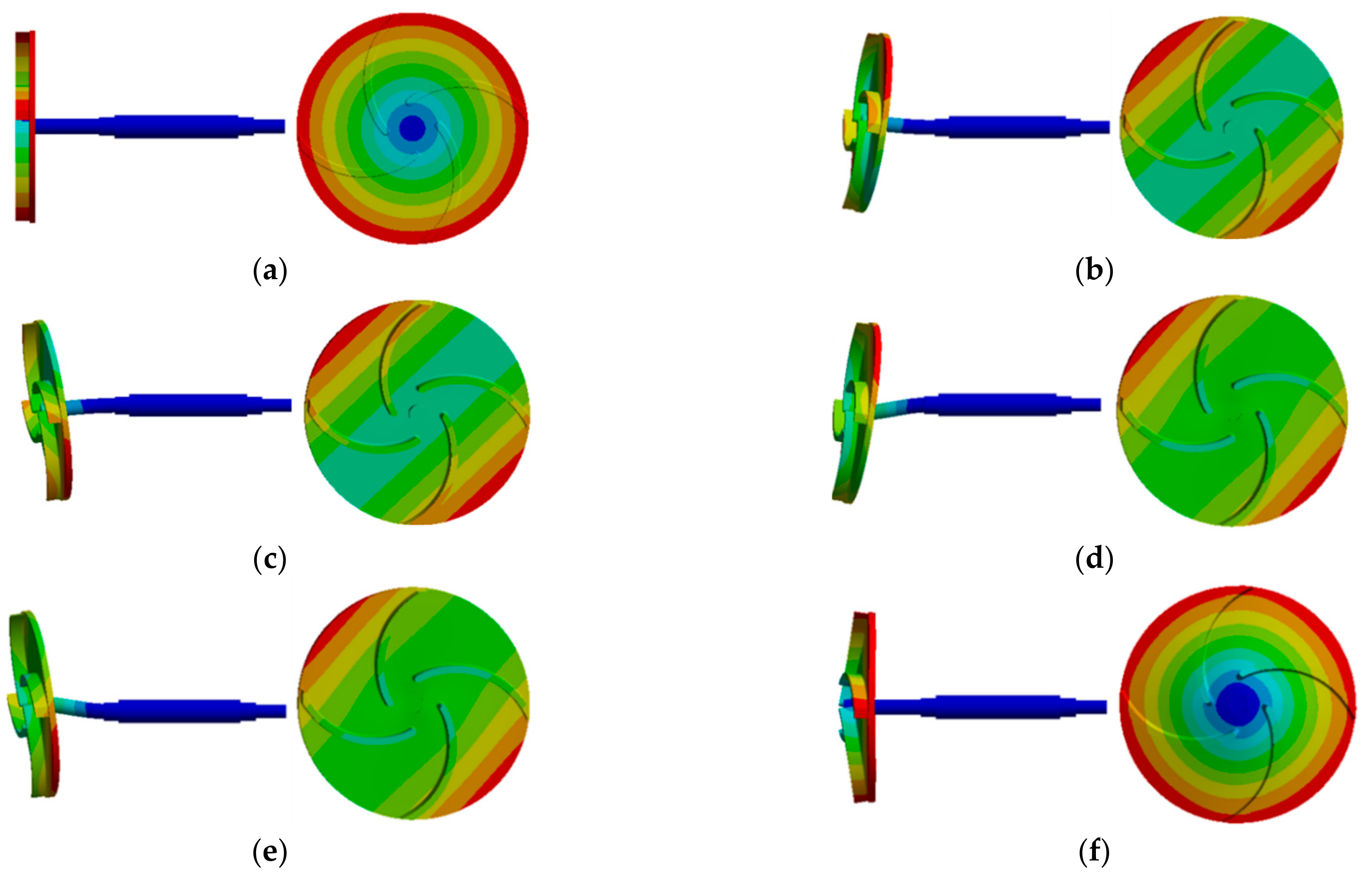
3.2. Influence of FSI on Flow Field and Structural Field
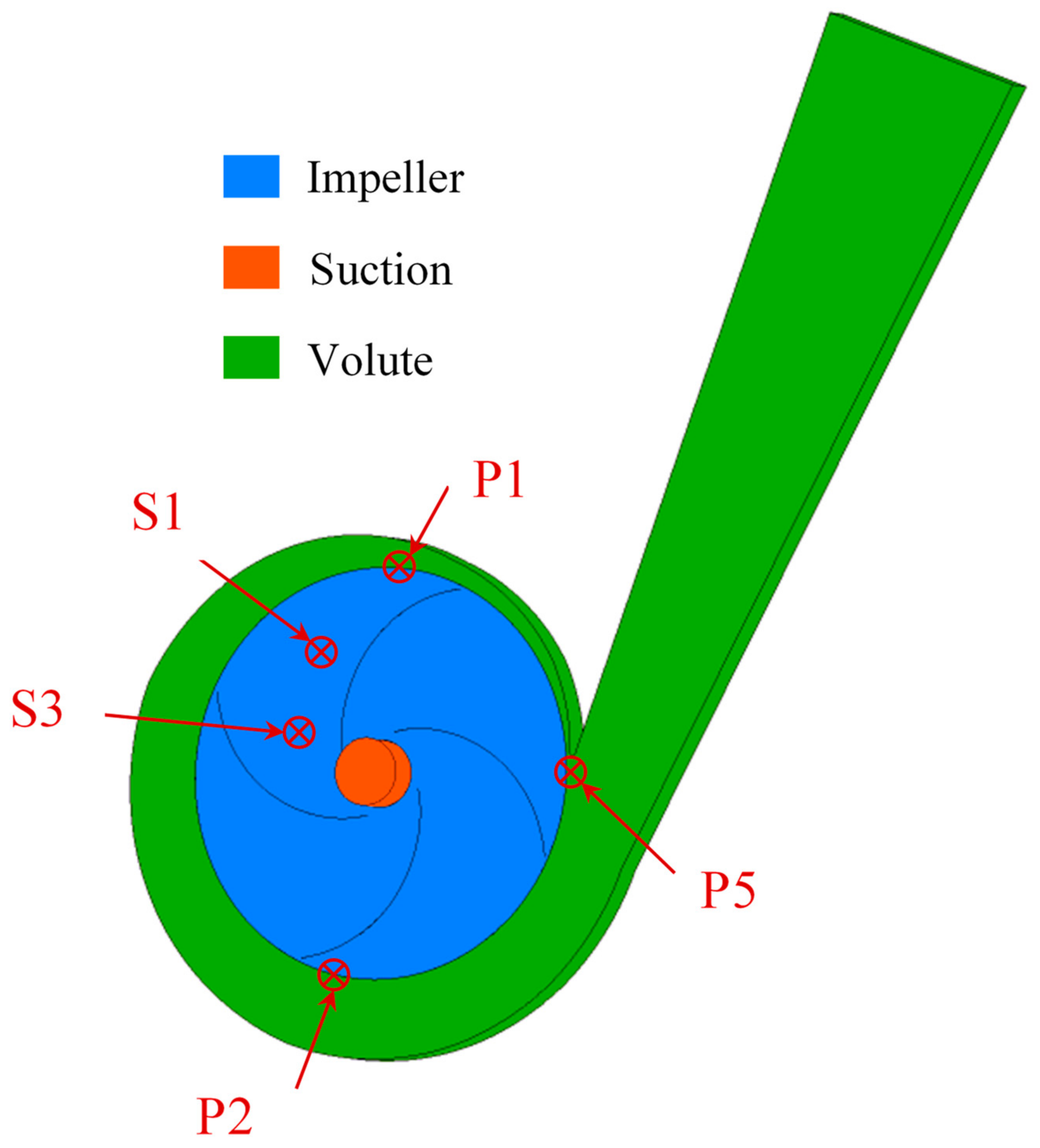
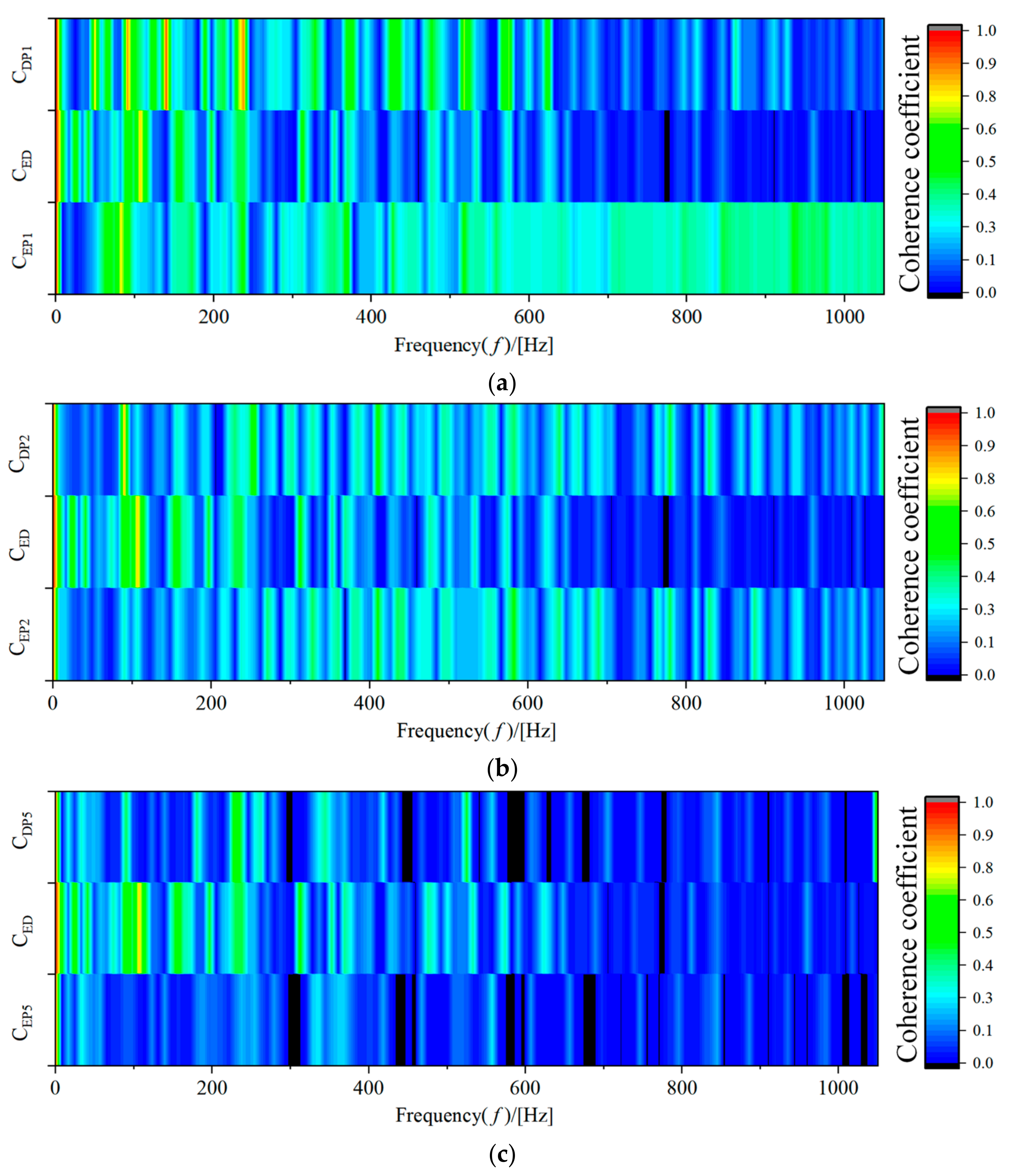
3.3. Coherence Analysis between Structural Field and Flow Field
3.4. FSI Modal Analysis
4. Conclusions
- (1)
- By employing the two-way FSI method, we successfully compute the flow characteristics of the centrifugal pump, providing a comprehensive revelation of the impact of the FSI on the internal flow structure. This contributes to a more comprehensive understanding of the performance and behavior of the pump. The FSI has a greater influence on the pressure distribution at the cut-water. This is because the prediction of the rotor–stator interaction at the cut-water between the impeller and the volute is more accurate after considering the FSI. Therefore, in designs and optimizations involving the cut-water region, it is necessary to pay attention to this location. Through the comparison of the external characteristics before and after the two-way FSI with the experimental values, it is found that the error between the predicted and experimental values of the external characteristics is smaller after the two-way FSI and that the prediction is more accurate.
- (2)
- Different coupling methods have a certain influence on the impeller stress and deformation. Using the two coupling methods, the maximum deformation is located at the outlet of the impeller, and the maximum equivalent stress is located at the connection between the leading edge of the impeller inlet and the impeller hub. This underscores that the critical region of force on the impeller of the low-specific-speed centrifugal pump experiences the maximum stress and may be an area that requires particular attention. The results of the two-way FSI are larger than those of the one-way FSI. However, considering that the two-way FSI requires a lot of computing resources, the results of the one-way FSI can be used when the deformation of the research object is not large. This balance can be found between computational efficiency and result accuracy. In the case of one-way coupling and uncoupling, the first six modal frequencies of the impeller are similar, but the fourth and fifth orders have a great influence, and the frequency difference is 1.39 Hz. This highlights the importance of selecting the appropriate coupling method at specific modal frequencies.
- (3)
- Through signal coherence analysis, we delved into the correlation between the flow field and the structural field, providing a perspective that offers a deeper understanding of the FSI mechanism. The pressure pulsation signals at the interface of the volute and the impeller exhibit high coherence with the signals of maximum equivalent stress and maximum deformation. The frequencies at which these coherence coefficients have peak values align with the rotational speed of the impeller and the blade passing frequency. This indicates that the rotor–stator interaction between the interface of the volute and the impeller leads to significant variations in the structural field performance. On the other hand, the pressure pulsation signals originating from the unstable flow structures within the flow path of the impeller show weaker coherence with the stress and strain signals in the structural field. At specific frequencies of approximately 93 Hz and 233 Hz, all monitoring points display strong coherence between the flow field signals and the maximum deformation signals. These frequencies correspond to twice and five times the blade passing frequency. These findings contribute to a better understanding of the interaction between the fluid and the structure in low-specific-speed centrifugal pumps, offering valuable insights for improved design and performance predictions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Fluid–structure interaction | FSI |
| Computational fluid dynamic | CFD |
| Particle image velocimetry | PIV |
| Shear-stress-transport | SST |
| Number of blades | Z |
| Specific speed | ns |
| Design rotation speed | nd |
| Design flow rate | Qd |
| Design head | Hd |
| Efficiency | η |
| Impeller torque | M |
| Impeller angular velocity | ω0 |
| Density | ρ |
| Dynamic viscosity | μ |
| Hamiltonian operator | ▽ |
| Kinematic viscosity | ν |
| Turbulent kinetic energy | k |
| Empirical coefficient | β* |
| Stiffness matrix | [K] |
| Displacement vector | {x} |
| Force vector | {F} |
| Elastic matrix | [B] |
| Strain matrix | [D] |
References
- Zhang, F.; Appiah, D.; Hong, F.; Zhang, J.; Yuan, S.; Adu-Poku, K.A.; Wei, X. Energy loss evaluation in a side channel pump under different wrapping angles using entropy production method. Int. Commun. Heat Mass Transf. 2020, 113, 104526. [Google Scholar] [CrossRef]
- Šavar, M.; Kozmar, H.; Sutlović, I. Improving centrifugal pump efficiency by impeller trimming. Desalination 2009, 249, 654–659. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, Y.; Hou, H.; Yuan, Z. Theory and application of two-dimension viscous hydraulic design of the ultra-low specific-speed centrifugal pump. Proc. Inst. Mech. Eng. Part A J. Power Energy 2020, 234, 58–71. [Google Scholar] [CrossRef]
- Zhang, Y.-L.; Zhu, Z.-C.; Li, W.-G. Experiments on transient performance of a low specific speed centrifugal pump with open impeller. Proc. Inst. Mech. Eng. Part A J. Power Energy 2016, 230, 648–659. [Google Scholar] [CrossRef]
- Choi, Y.-D.; Nishino, K.; Kurokawa, J.; Matsui, J. PIV measurement of internal flow characteristics of very low specific speed semi-open impeller. Exp. Fluids 2004, 37, 617–630. [Google Scholar] [CrossRef]
- Chabannes, L.; Štefan, D.; Rudolf, P. Effect of Splitter Blades on Performances of a Very Low Specific Speed Pump. Energies 2021, 14, 3785. [Google Scholar] [CrossRef]
- Liang, D.; Yuqi, Z.; Houlin, L.; Cui, D.; Vladimirovich, G.D.; Yong, W. The effect of front streamline wrapping angle variation in a super-low specific speed centrifugal pump. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 4301–4311. [Google Scholar] [CrossRef]
- Jafarzadeh, B.; Hajari, A.; Alishahi, M.; Akbari, M. The flow simulation of a low-specific-speed high-speed centrifugal pump. Appl. Math. Model. 2011, 35, 242–249. [Google Scholar] [CrossRef]
- Sakthivel, N.; Sugumaran, V.; Babudevasenapati, S. Vibration based fault diagnosis of monoblock centrifugal pump using decision tree. Expert Syst. Appl. 2010, 37, 4040–4049. [Google Scholar] [CrossRef]
- Yanhua, W.; Longlong, H.; Yong, L.; Jingsong, X. Comparative analysis of cycloid pump based on CFD and fluid structure in-teractions. Adv. Mech. Eng. 2020, 12, 1687814020973533. [Google Scholar] [CrossRef]
- Pellegri, M.; Manne, V.H.B.; Vacca, A. A simulation model of Gerotor pumps considering fluid–structure interaction effects: Formulation and validation. Mech. Syst. Signal Process. 2020, 140, 106720. [Google Scholar] [CrossRef]
- Adamkowski, A.; Henke, A.; Lewandowski, M. Resonance of torsional vibrations of centrifugal pump shafts due to cavitation erosion of pump impellers. Eng. Fail. Anal. 2016, 70, 56–72. [Google Scholar] [CrossRef]
- Ouyang, J.; Luo, Y.; Tao, R. Influence of blade leaning on hydraulic excitation and structural response of a reversible pump turbine. Proc. Inst. Mech. Eng. Part A J. Power Energy 2021, 236, 241–259. [Google Scholar] [CrossRef]
- Wang, J.; Wang, P.; Zhang, X.; Ruan, X.; Xu, Z.; Fu, X. Research of Modal Analysis for Impeller of Reactor Coolant Pump. Appl. Sci. 2019, 9, 4551. [Google Scholar] [CrossRef]
- Yin, T.; Pei, J.; Yuan, S.; Osman, M.K.; Wang, J.; Wang, W. FSI analysis of an impeller for a high-pressure booster pump for seawater desalination. J. Mech. Sci. Technol. 2017, 31, 5319–5328. [Google Scholar] [CrossRef]
- Matlakala, M.E.; Kallon, D.V.; Mogapi, K.E.; Mabelane, I.M.; Makgopa, D.M. Influence of Impeller Diameter on the Performance of Centrifugal pumps. IOP Conf. Ser. Mater. Sci. Eng. 2019, 655, 012009. [Google Scholar] [CrossRef]
- Mousmoulis, G.; Kassanos, I.; Aggidis, G.; Anagnostopoulos, I. Numerical simulation of the performance of a centrifugal pump with a semi-open impeller under normal and cavitating conditions. Appl. Math. Model. 2020, 89, 1814–1834. [Google Scholar] [CrossRef]
- Xiao, R.; Wang, Z.; Luo, Y. Dynamic stresses in a francis turbine runner based on FSI analysis. Tsinghua Sci. Technol. 2008, 13, 587–592. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, S.; Yin, G.; Guan, C. Fluid–structure interaction analysis of fluid pressure pulsation and structural vibration features in a vertical axial pump. Adv. Mech. Eng. 2019, 11, 1–19. [Google Scholar] [CrossRef]
- Afra, B.; Delouei, A.A.; Tarokh, A. Flow-Induced Locomotion of a Flexible Filament in the Wake of a Cylinder in Non-Newtonian Flows. Int. J. Mech. Sci. 2022, 234, 107693. [Google Scholar] [CrossRef]
- Delouei, A.A.; Karimnejad, S.; He, F. Direct Numerical Simulation of pulsating flow effect on the distribution of non-circular particles with increased levels of complexity: IB-LBM. Comput. Math. Appl. 2022, 121, 115–130. [Google Scholar] [CrossRef]
- Jiang, Y.; Yoshimura, S.; Imai, R.; Katsura, H.; Yoshida, T.; Kato, C. Quantitative evaluation of flow-induced structural vibration and noise in turbomachinery by full-scale weakly coupled simulation. J. Fluids Struct. 2007, 23, 531–544. [Google Scholar] [CrossRef]
- Li, W.; Ji, L.; Shi, W.; Zhou, L.; Jiang, X. FSI study of a mixed-flow pump impeller during startup. Eng. Comput. 2018, 35, 18–34. [Google Scholar] [CrossRef]
- Kato, C.; Yoshimura, S.; Yamade, Y.; Jiang, Y.Y.; Wang, H.; Imai, R.; Katsura, H.; Yoshida, T.; Takano, Y. Prediction of the noise from a multi-stage centrifugal pump. In Proceedings of the ASME 2005 Fluids Engineering Division Summer Meeting, Houston, TX, USA, 19–23 June 2005; pp. 1273–1280. [Google Scholar]
- MacPhee, D.W.; Beyene, A. Fluid–structure interaction analysis of a morphing vertical axis wind turbine. J. Fluids Struct. 2016, 60, 143–159. [Google Scholar] [CrossRef]
- Benra, F.K.; Dohmen, H.J.; Pei, J.; Schuster, S.; Wan, B. A comparison of one-way and two-way coupling methods for numerical analysis of FSIs. J. Appl. Math. 2011, 2011, 1–16. [Google Scholar] [CrossRef]
- Degroote, J. Partitioned Simulation of Fluid-Structure Interaction Coupling Black-Box Solvers with Quasi-Newton Techniques. Arch. Comput. Methods Eng. 2013, 20, 185–238. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Dhavalikar, S.; Awasare, S.; Joga, R.; Kar, A. Whipping response analysis by one way fluid structure interaction—A case study. Ocean Eng. 2015, 103, 10–20. [Google Scholar] [CrossRef]
- Tran, D.-M.; Liauzun, C. Frequency and Time Domain FSI Methods for Turbomachineries; Springer: Dordrecht, The Netherlands, 1990; pp. 397–408. [Google Scholar]
- Masoomi, M.; Mosavi, A. The one-way fsi method based on rans-fem for the open water test of a marine propeller at the dif-ferent loading conditions. J. Mar. Sci. Eng. 2021, 9, 351. [Google Scholar] [CrossRef]
- Procedure for Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008, 130, 1–4.
- Pei, J.; Meng, F.; Li, Y.; Yuan, S.; Chen, J. Fluid–structure coupling analysis of deformation and stress in impeller of an axial-flow pump with two-way passage. Adv. Mech. Eng. 2016, 8, 1687814016646266. [Google Scholar] [CrossRef]



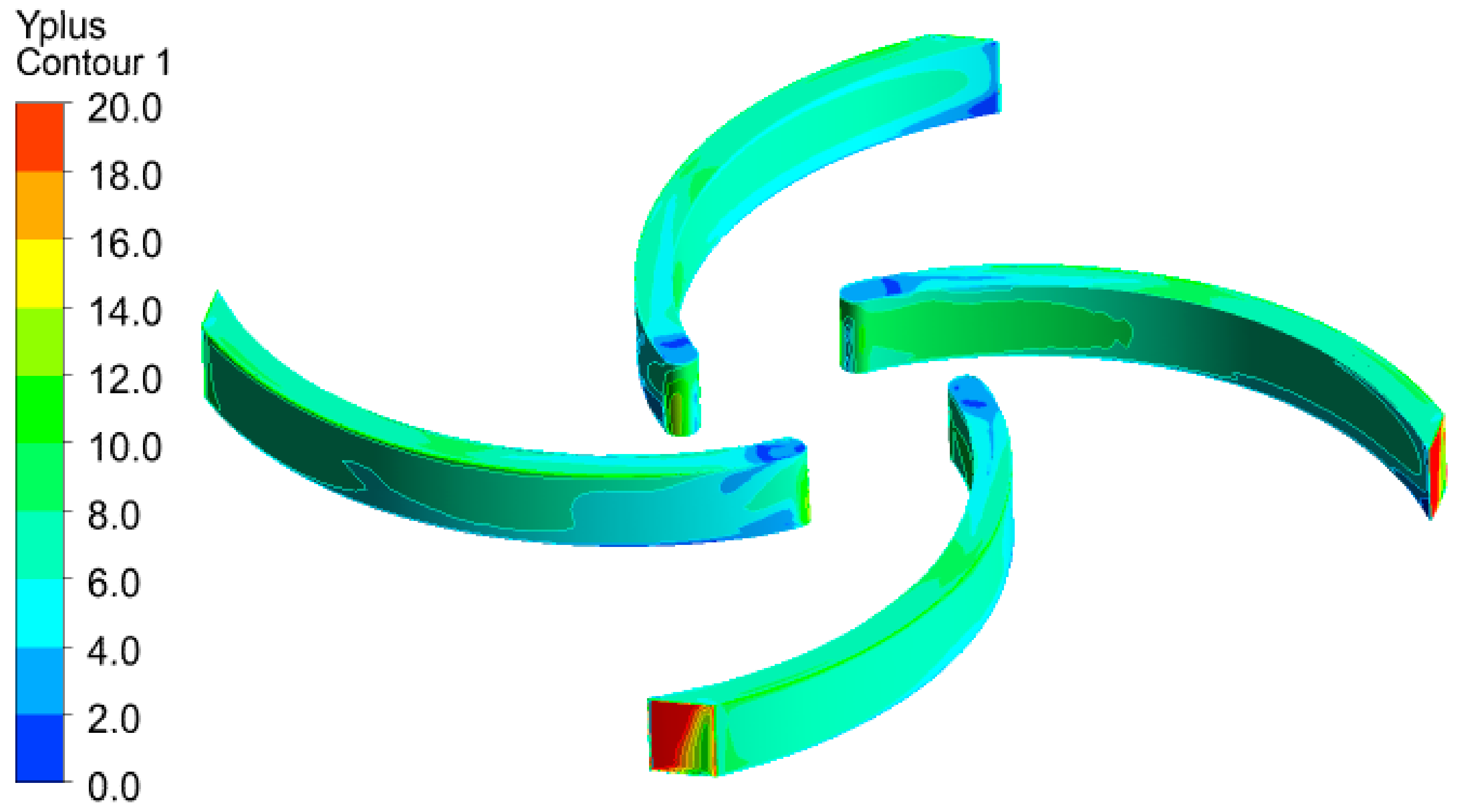









| Parameter | Numerical Value | Unit |
|---|---|---|
| Number of blades (Z) | 4 | [-] |
| Specific speed (ns) | 60.28 | [-] |
| Design rotation speed (nd) | 700 | r/min |
| Design flow rate (Qd) | 0.4745 | kg/s |
| Design head (Hd) | 0.899 | m |
| Mesh Scheme | Inlet | Impeller | Volute | Total |
|---|---|---|---|---|
| 1 | 408,677 | 1,547,700 | 553,876 | 2,510,253 |
| 2 | 222,608 | 1,127,191 | 315,098 | 1,664,897 |
| 3 | 244,675 | 823,320 | 267,567 | 1,335,562 |
| 4 | 157,589 | 690,370 | 208,674 | 1,056,633 |
| 5 | 145,243 | 478,456 | 273,945 | 897,644 |
| Order (n) | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| With prestress (Hz) | 232.93 | 275.27 | 275.71 | 841.90 | 843.09 | 1104.5 |
| Without prestress (Hz) | 232.92 | 275.98 | 276.41 | 840.51 | 841.70 | 1104.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qiao, F.; Sun, Y.; Zhu, D.; Fang, M.; Zhang, F.; Tao, R.; Xiao, R. Analysis of Stress–Strain Characteristics and Signal Coherence of Low-Specific-Speed Impeller Based on Fluid–Structure Interaction. J. Mar. Sci. Eng. 2024, 12, 2. https://doi.org/10.3390/jmse12010002
Qiao F, Sun Y, Zhu D, Fang M, Zhang F, Tao R, Xiao R. Analysis of Stress–Strain Characteristics and Signal Coherence of Low-Specific-Speed Impeller Based on Fluid–Structure Interaction. Journal of Marine Science and Engineering. 2024; 12(1):2. https://doi.org/10.3390/jmse12010002
Chicago/Turabian StyleQiao, Fengquan, Yi Sun, Di Zhu, Mingkun Fang, Fangfang Zhang, Ran Tao, and Ruofu Xiao. 2024. "Analysis of Stress–Strain Characteristics and Signal Coherence of Low-Specific-Speed Impeller Based on Fluid–Structure Interaction" Journal of Marine Science and Engineering 12, no. 1: 2. https://doi.org/10.3390/jmse12010002
APA StyleQiao, F., Sun, Y., Zhu, D., Fang, M., Zhang, F., Tao, R., & Xiao, R. (2024). Analysis of Stress–Strain Characteristics and Signal Coherence of Low-Specific-Speed Impeller Based on Fluid–Structure Interaction. Journal of Marine Science and Engineering, 12(1), 2. https://doi.org/10.3390/jmse12010002








