An Experimental Investigation into the Feasibility of a DC Hybrid Power Plant for a Northern Sea Route Ship
Abstract
:1. Introduction
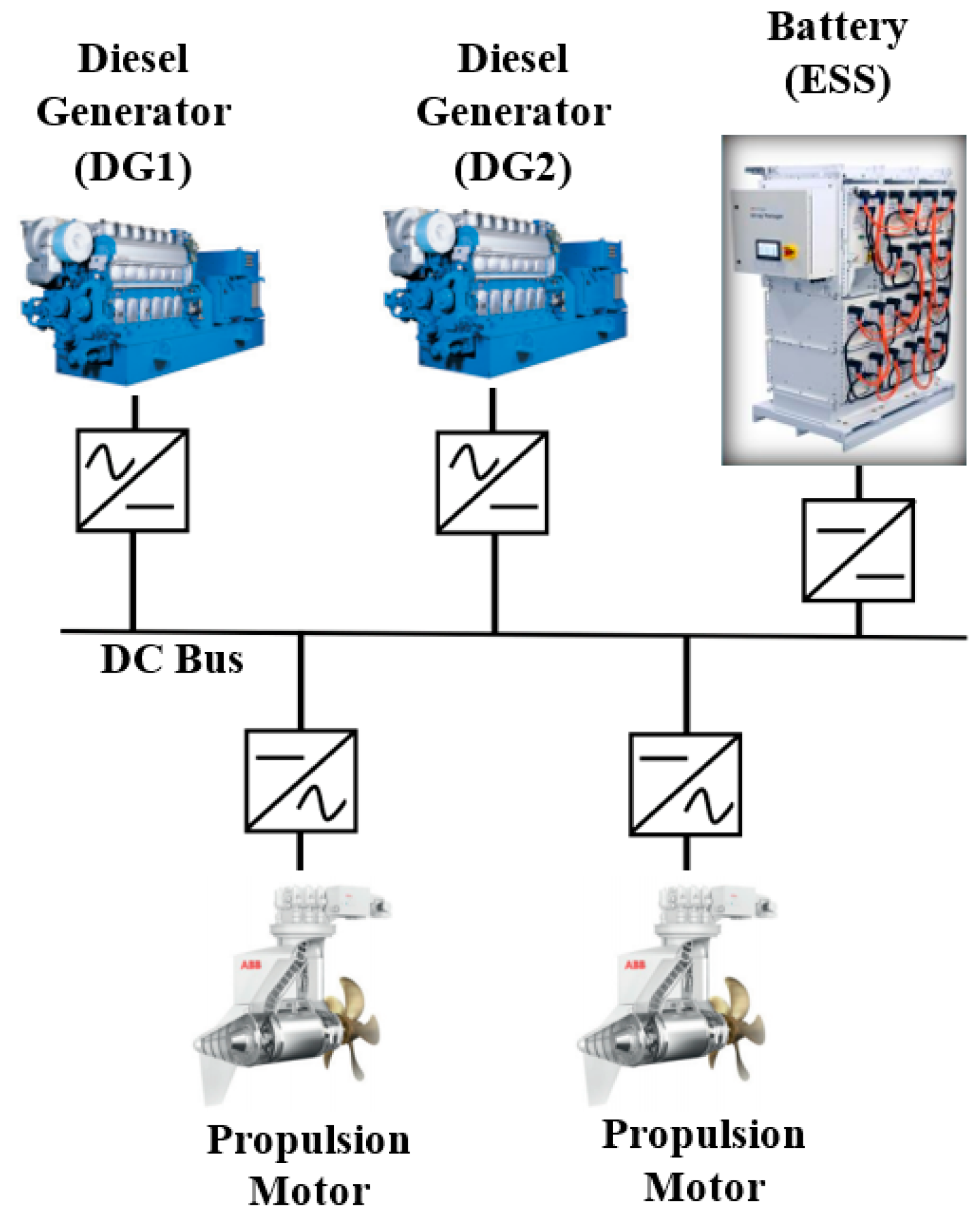
2. Modelling of the DC Hybrid Power System
2.1. Generator Sets
2.2. Lithium-Ion Battery
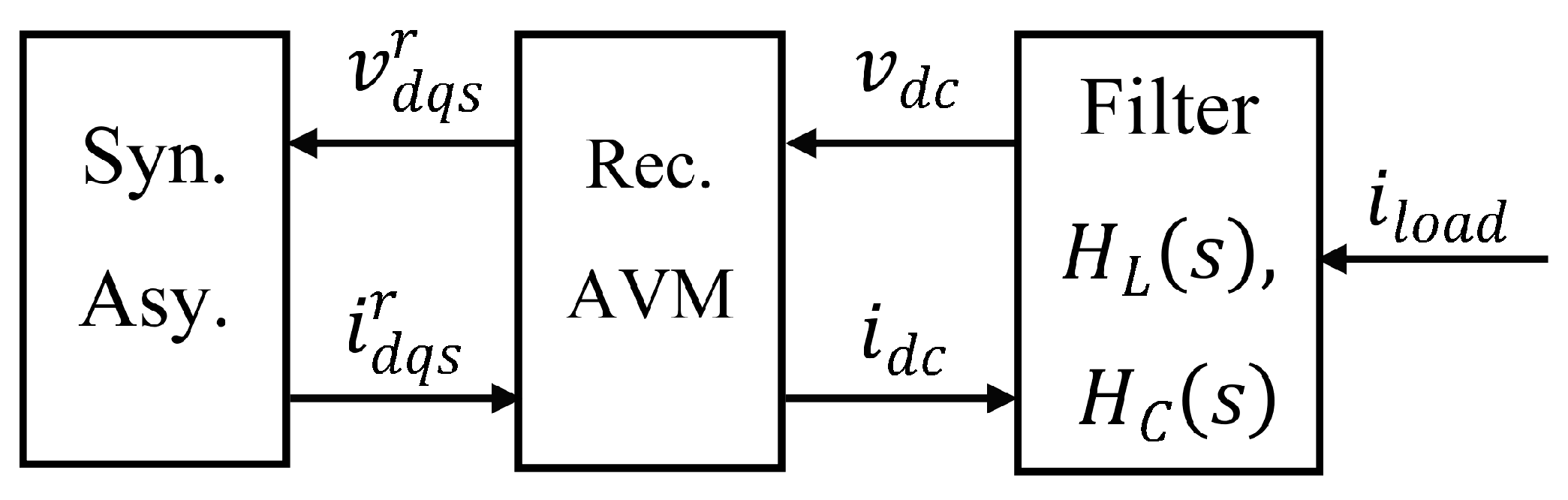
2.3. Average-Value-Model (AVM) Rectifier
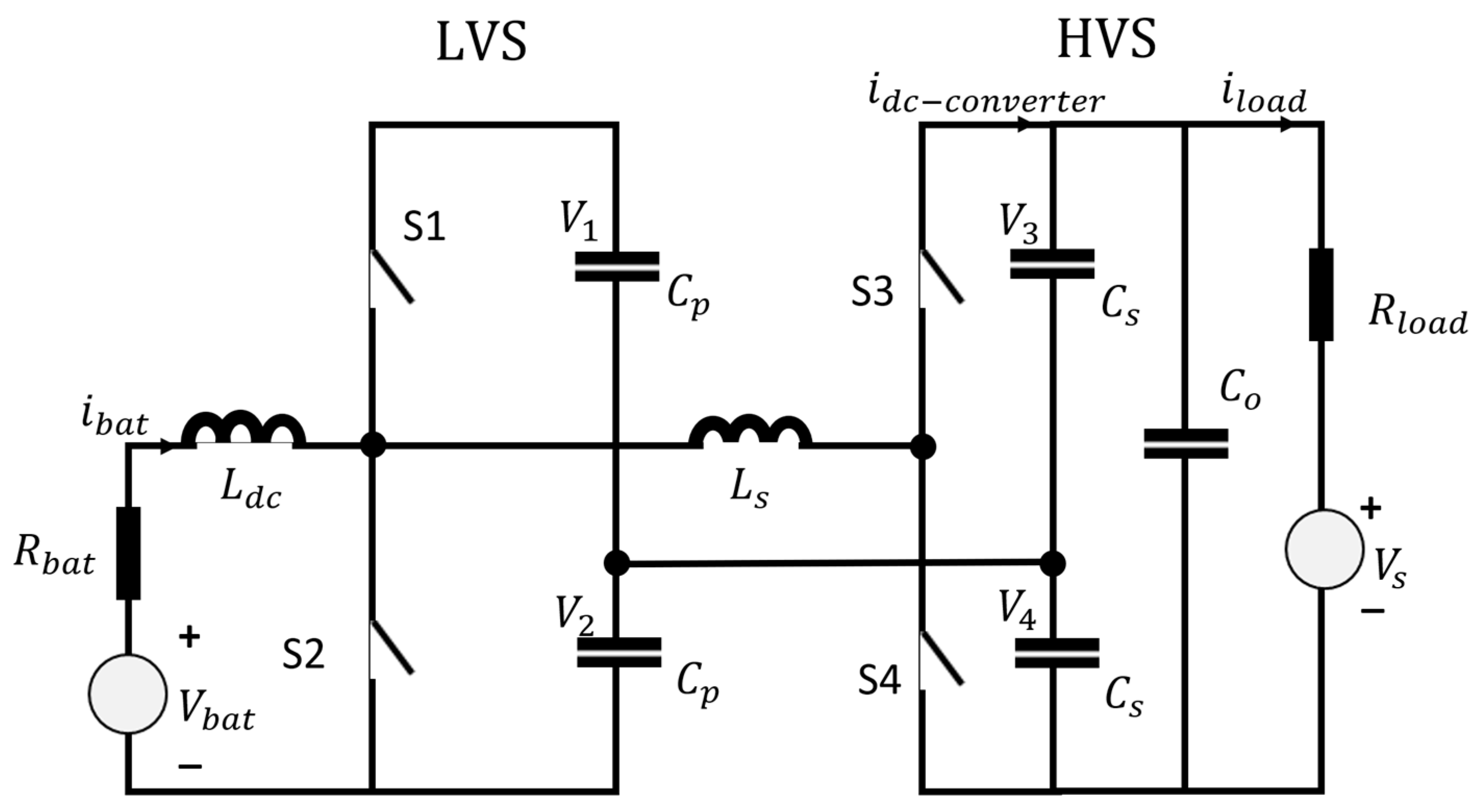
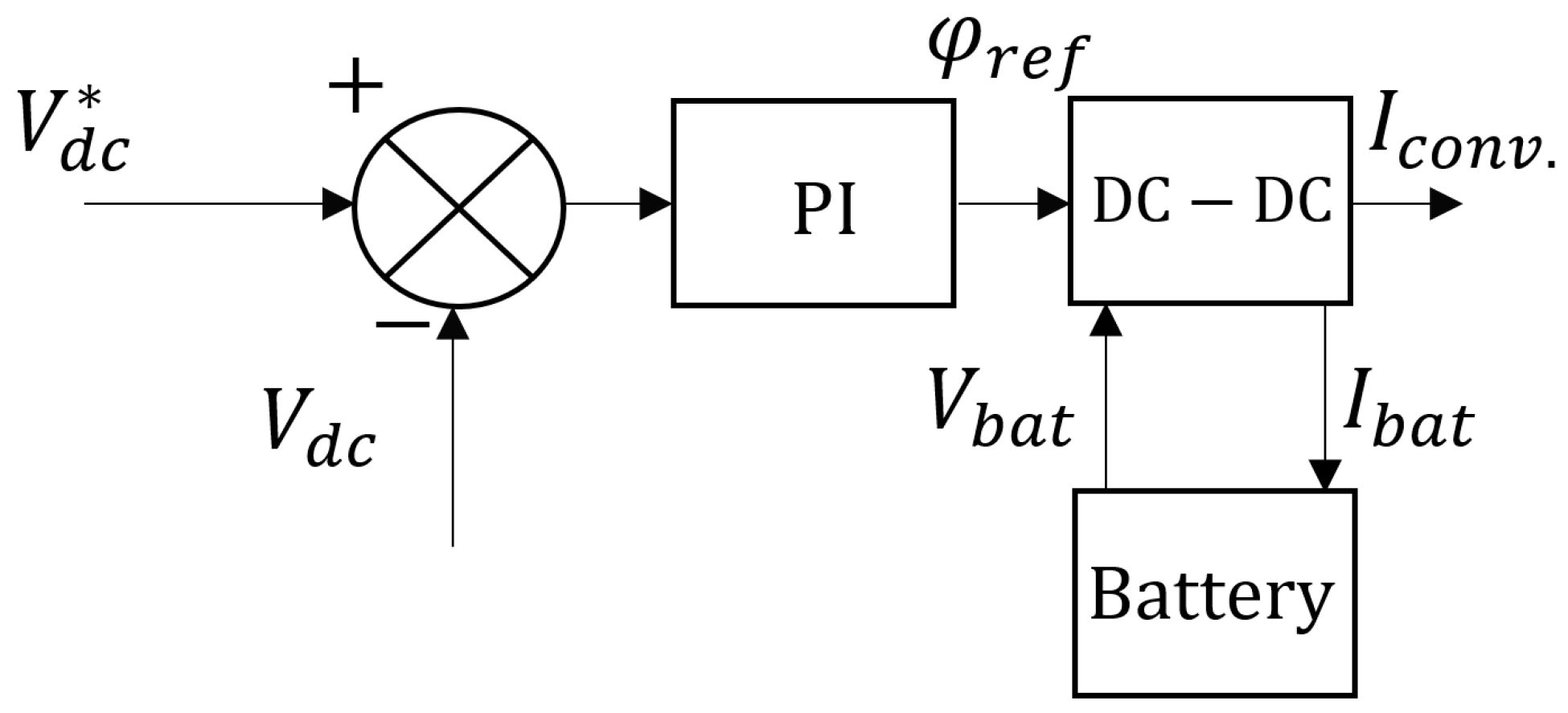
2.4. Bidirectional DC–DC Converter
3. Control Strategy and Optimisation Algorithm
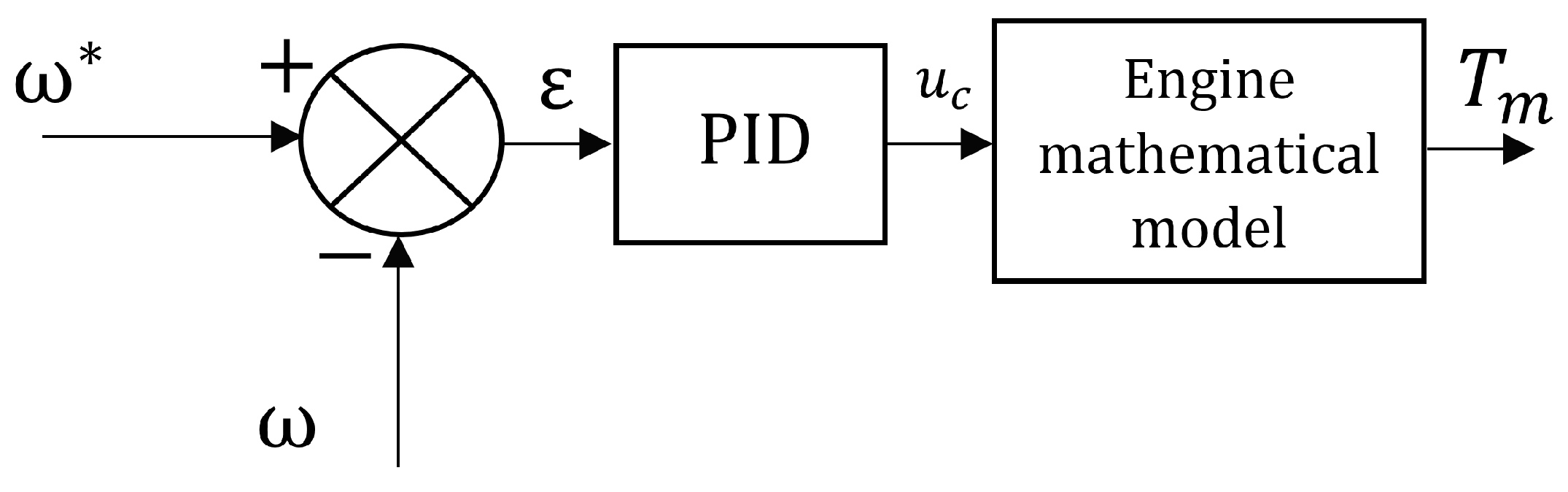
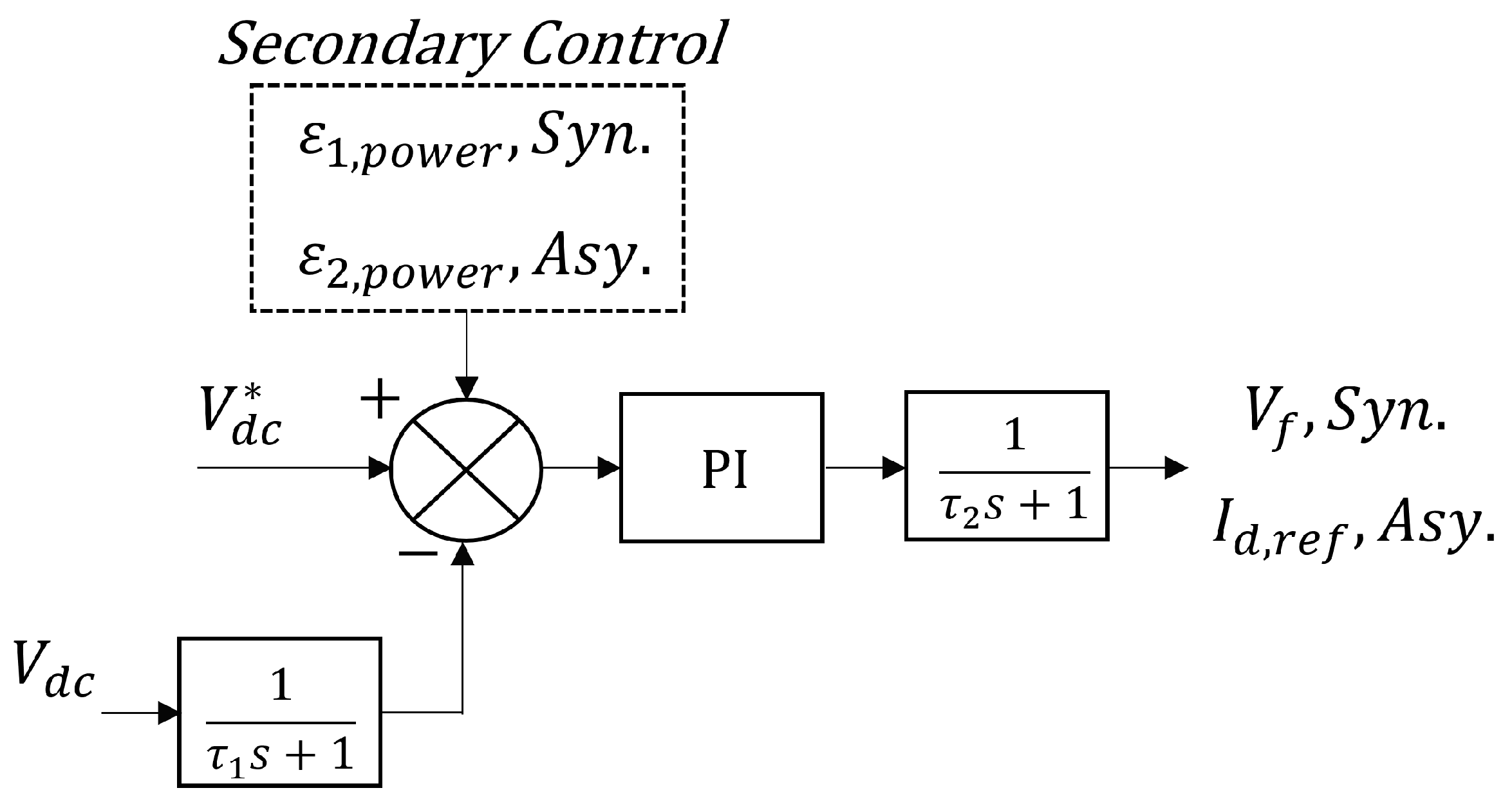
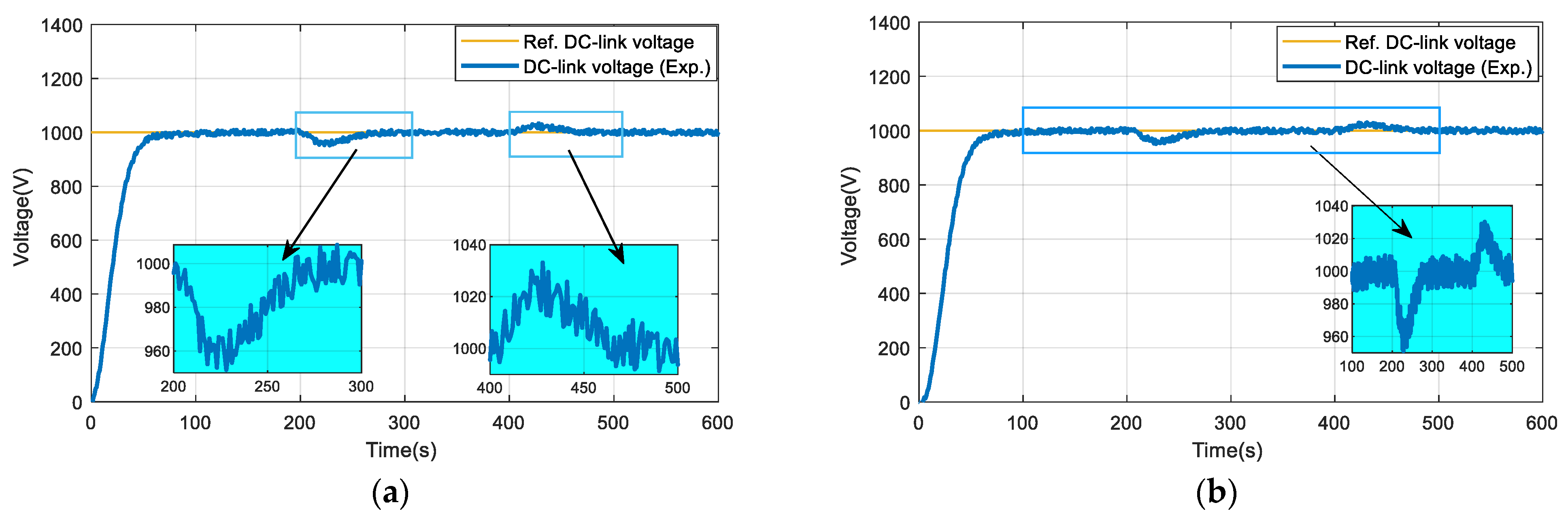
3.1. Voltage and Frequency Control
3.2. Energy and Power Management System
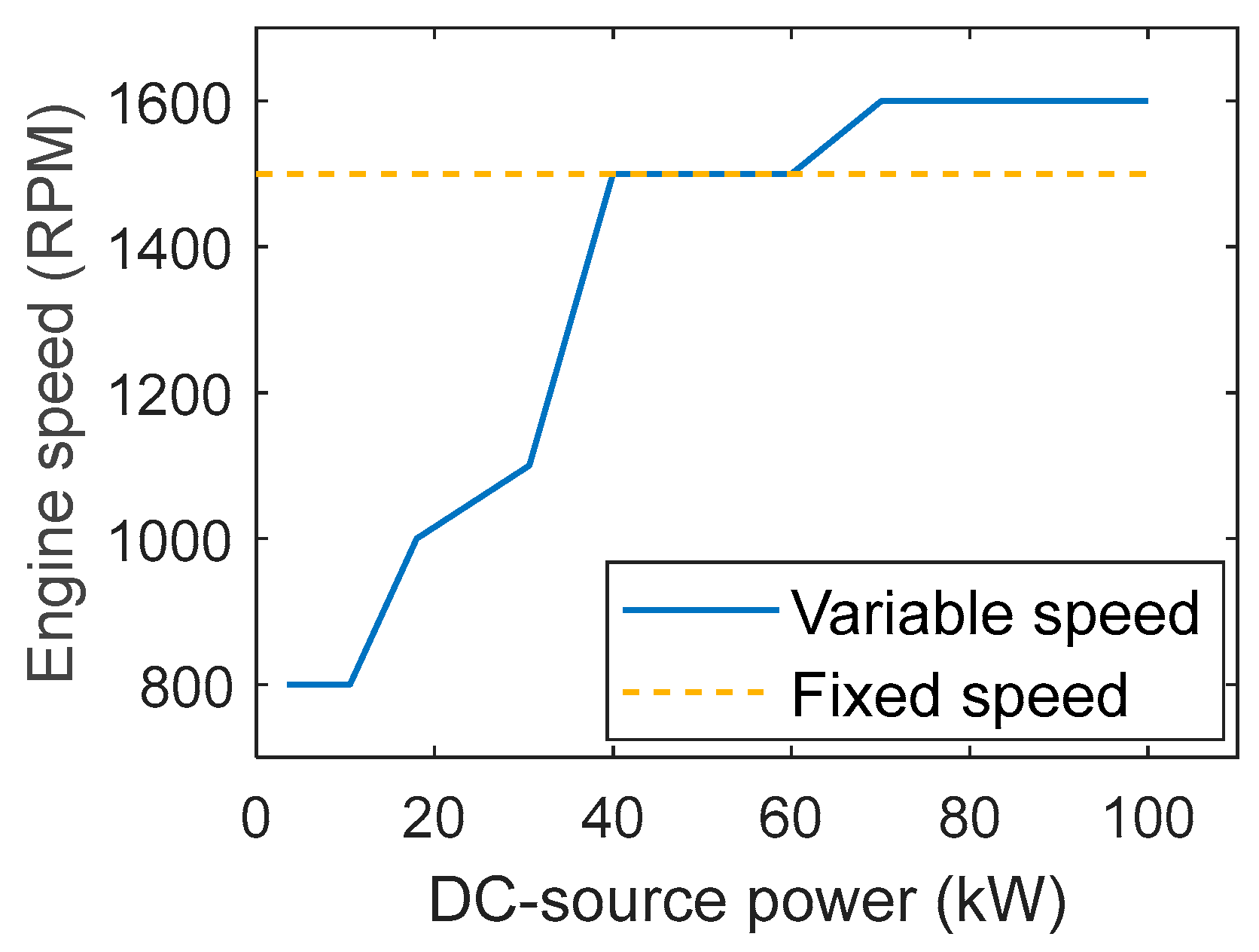
3.3. Efficiency Optimization Algorithm
- (1)
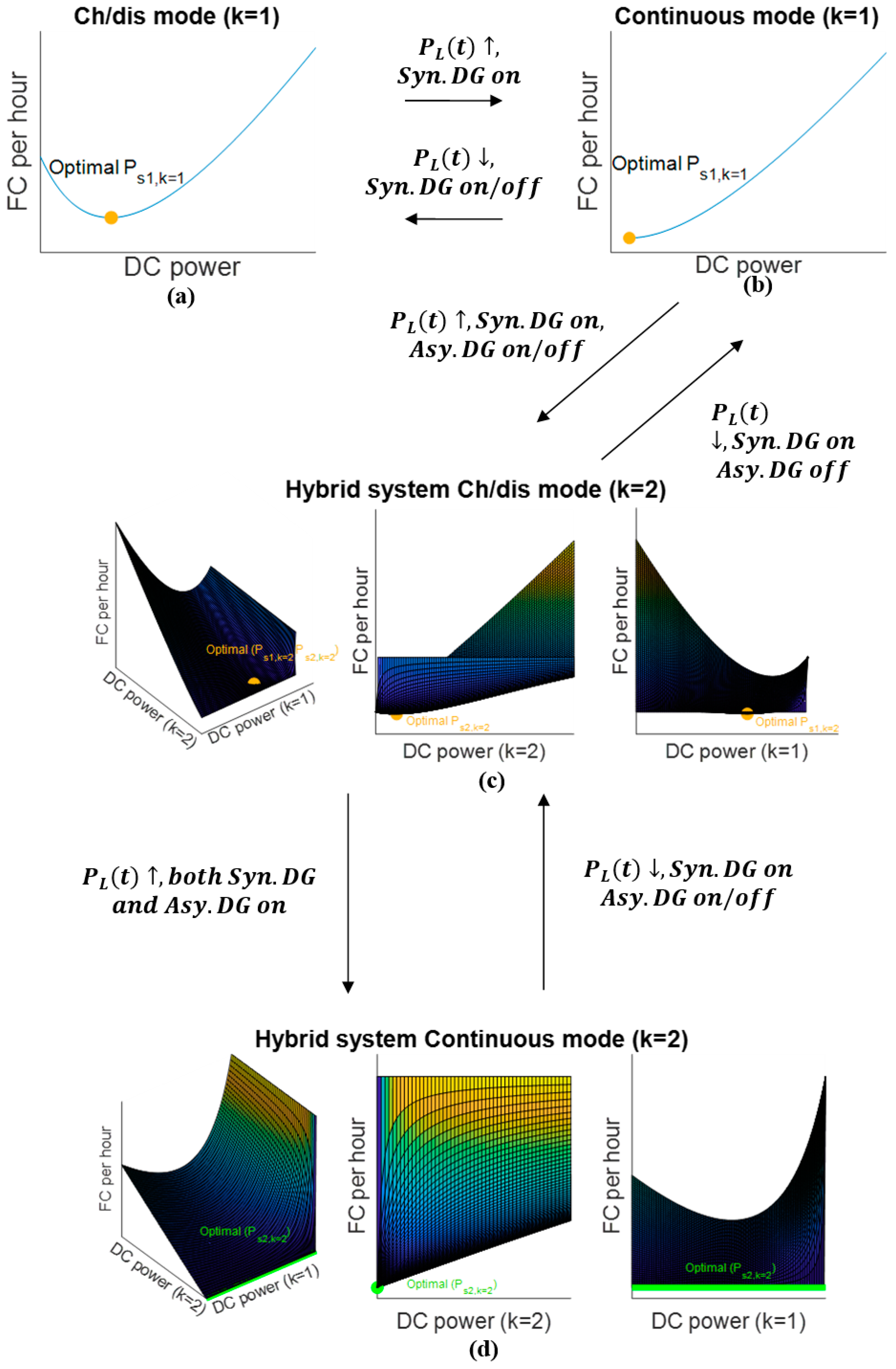
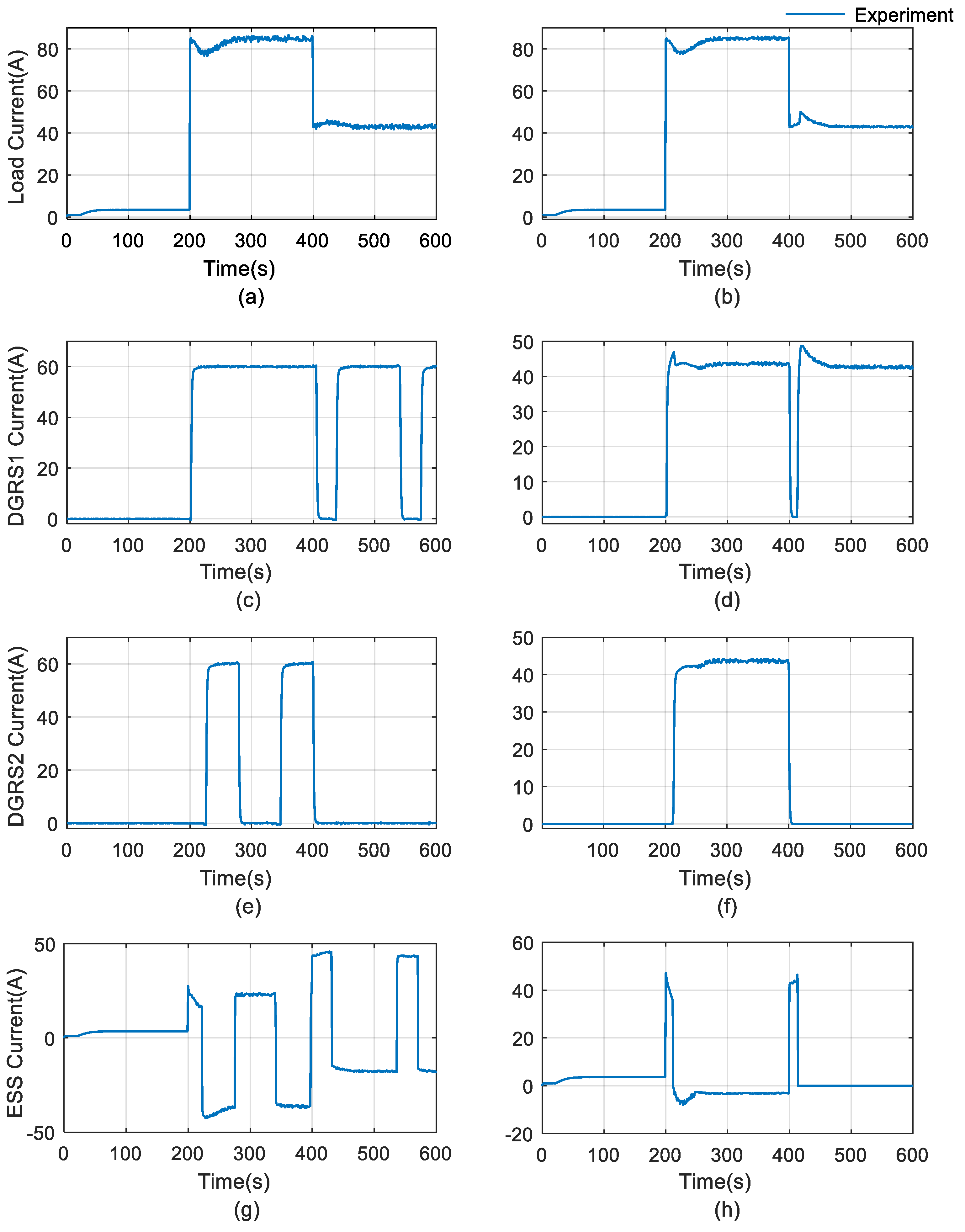
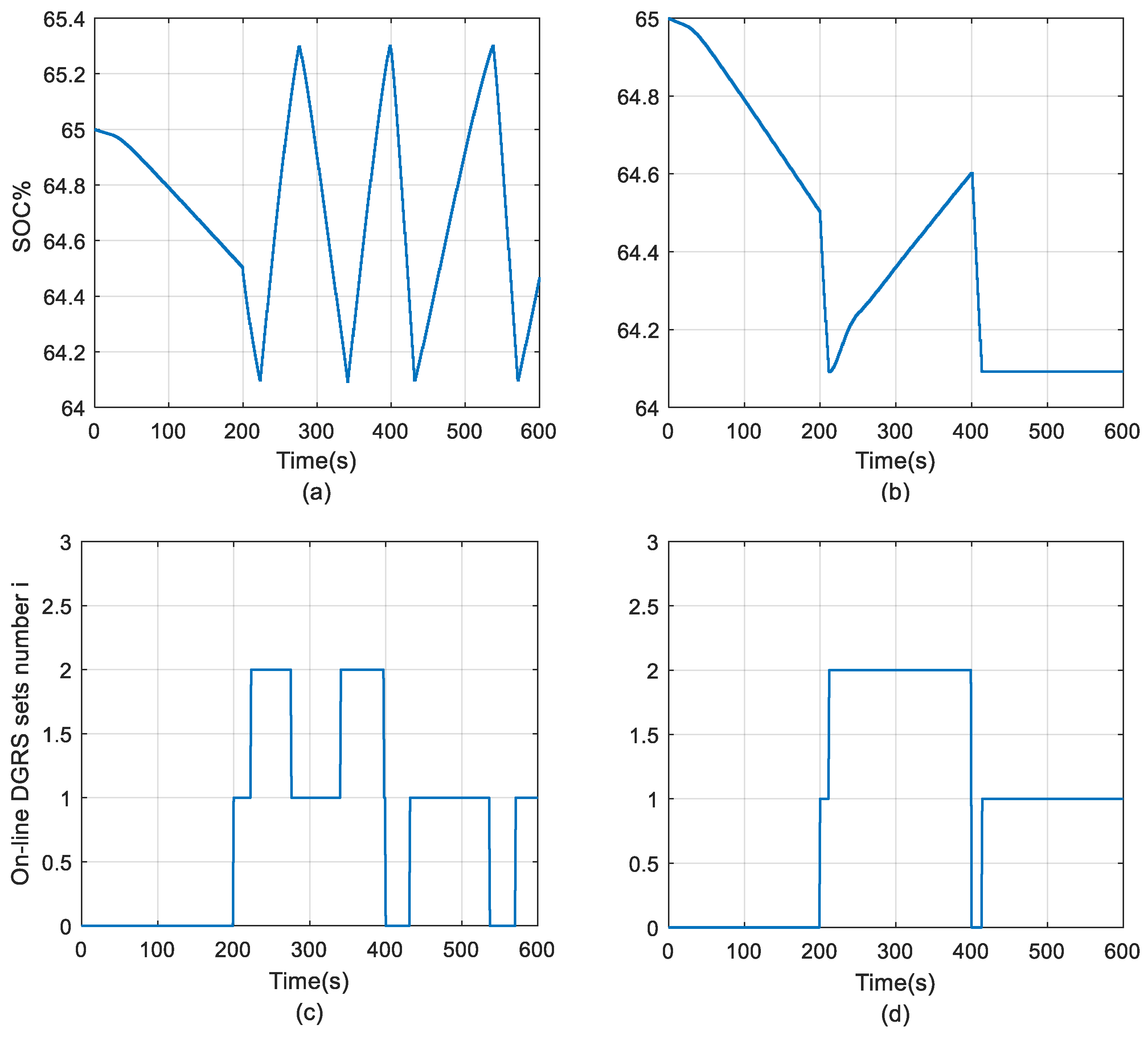
- Ch/dis mode (k = 1): This mode is applied to relatively light loading conditions. The ESS will start firstly to compensate the load power. When battery SOC% goes below , the DG1 will be powered up to balance the load and charge the battery. The DG2 will not be involved at this stage.
- (2)
- Continuous mode (k = 1): As increases, the duty cycle for the Syn. DGRS will rise; thus, the power from the Syn. DGRS available to charge the battery decreases. When the duty cycle reaches 1, the battery SOC% will be stable as no power flows into the battery. At this stage, the Syn. DGRS will work alone to balance the load power.
- (3)
- Ch/dis mode (k = 2): In this loading condition, the Asy. DGRS will be powered up to cooperate with the Syn. DGRS. The Syn. DGRS will always be kept on at this stage to match the high loading power. The Asy. DGRS will be involved only if the FC (fuel consumption) per hour of the Continuous mode (k = 1) Ch/dis mode (k = 2) , which means that the FC of one DGRS in operation is higher than that of two DGRS. The ESS will be charged/discharged according to the on/off state of the Asy. DGRS.
- (4)
- Continuous mode (k = 2): In the condition of Ch/dis mode (k = 2), with the increase in load power, , the system will be switched to the Continuous mode (k = 2) when the duty cycle gradually approaches 1. In this case, both the DG1 and DG2 are powered up and share the load power evenly. Simultaneously, the battery SOC% will be maintained at a steady level, as no power flows in/out of the ESS. This mode is applied to tackle the highest power-demand condition.
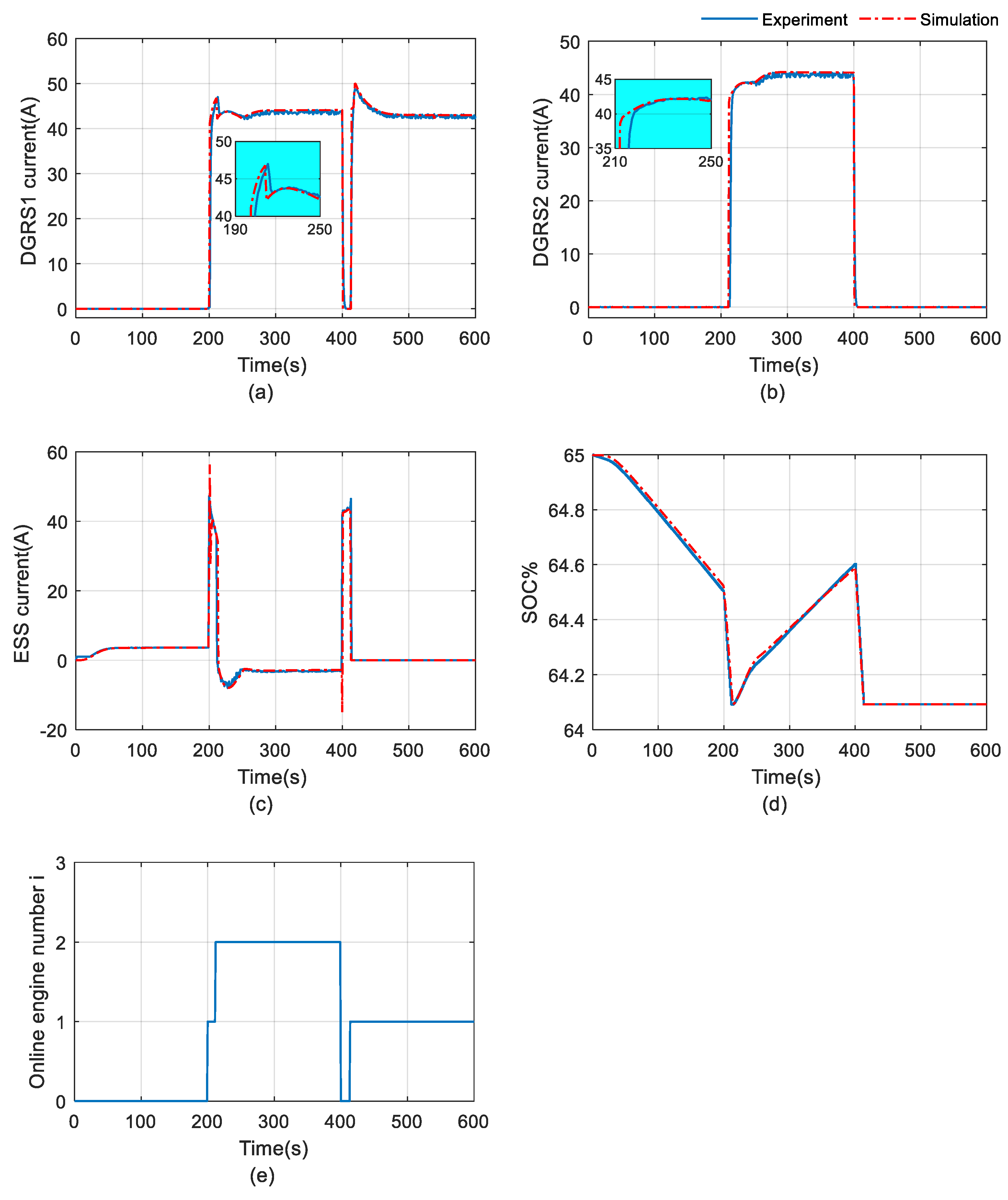
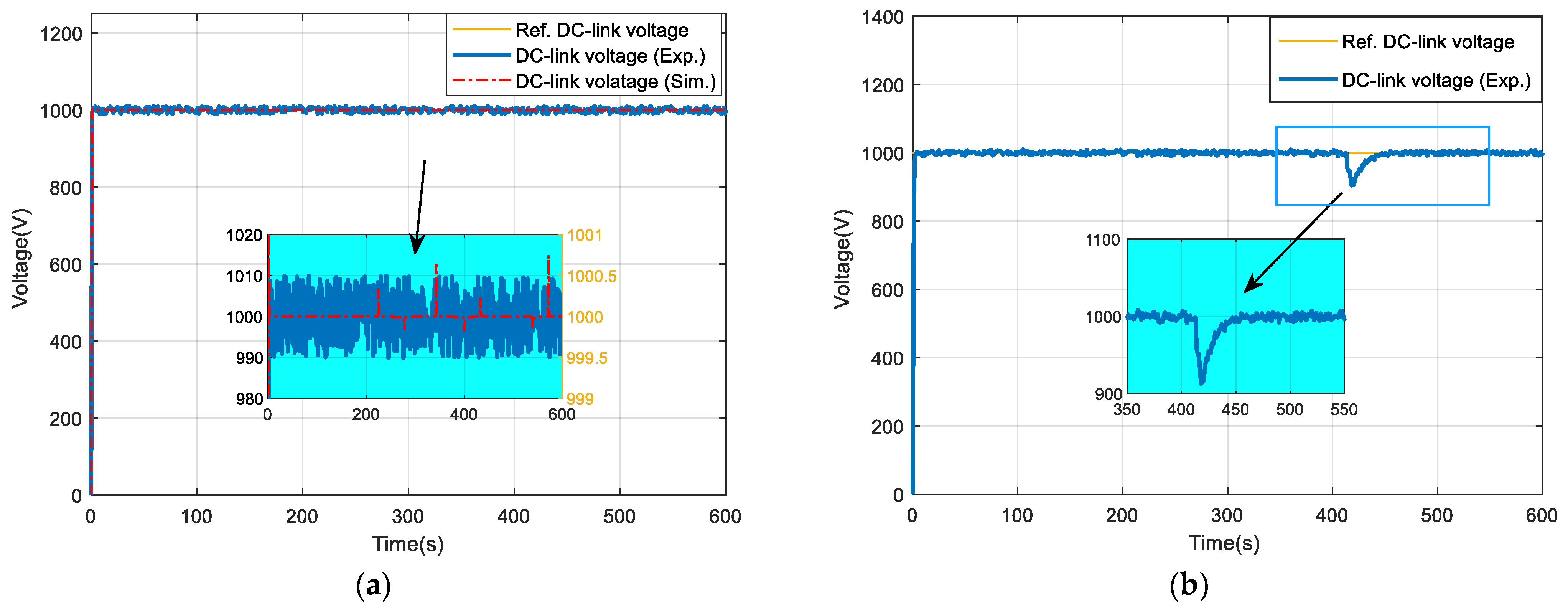
4. Simulation and Experimental Results in Optimisation Control
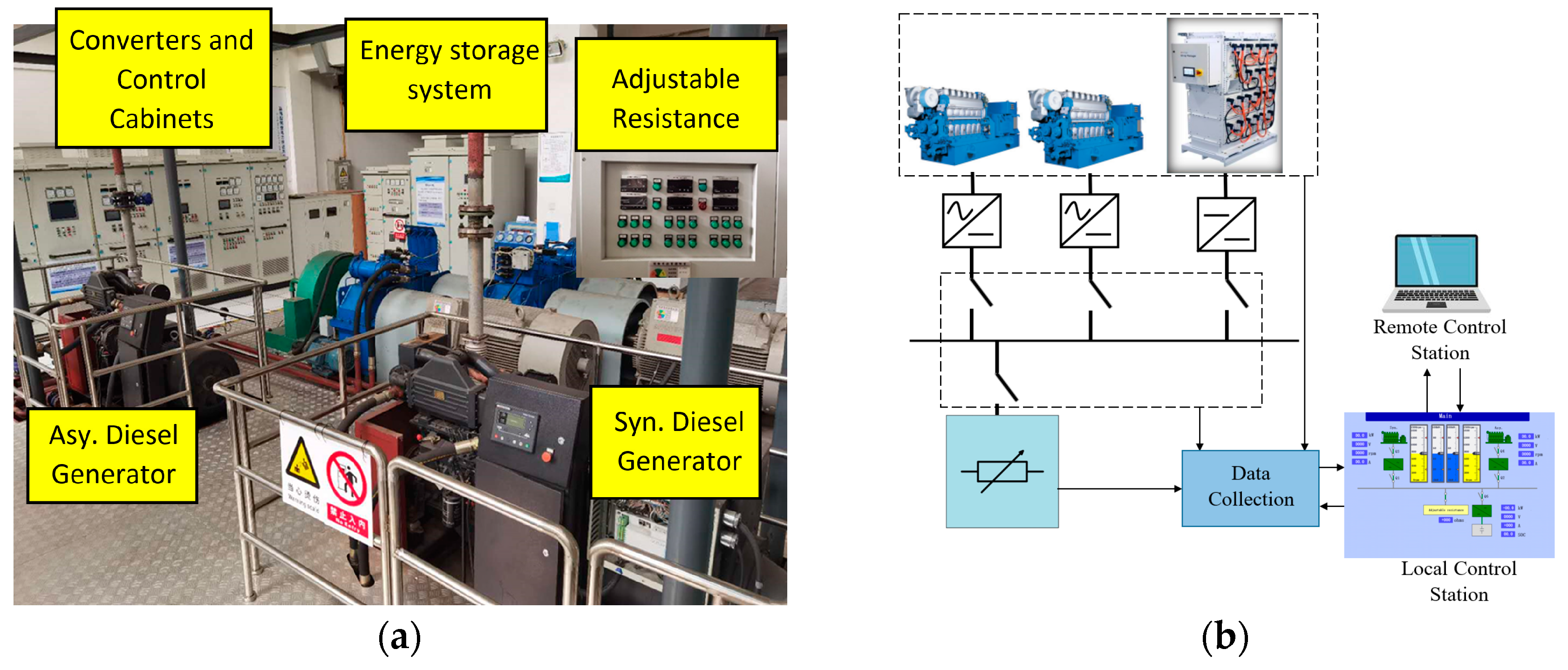
4.1. Simulation and Experimental Setups
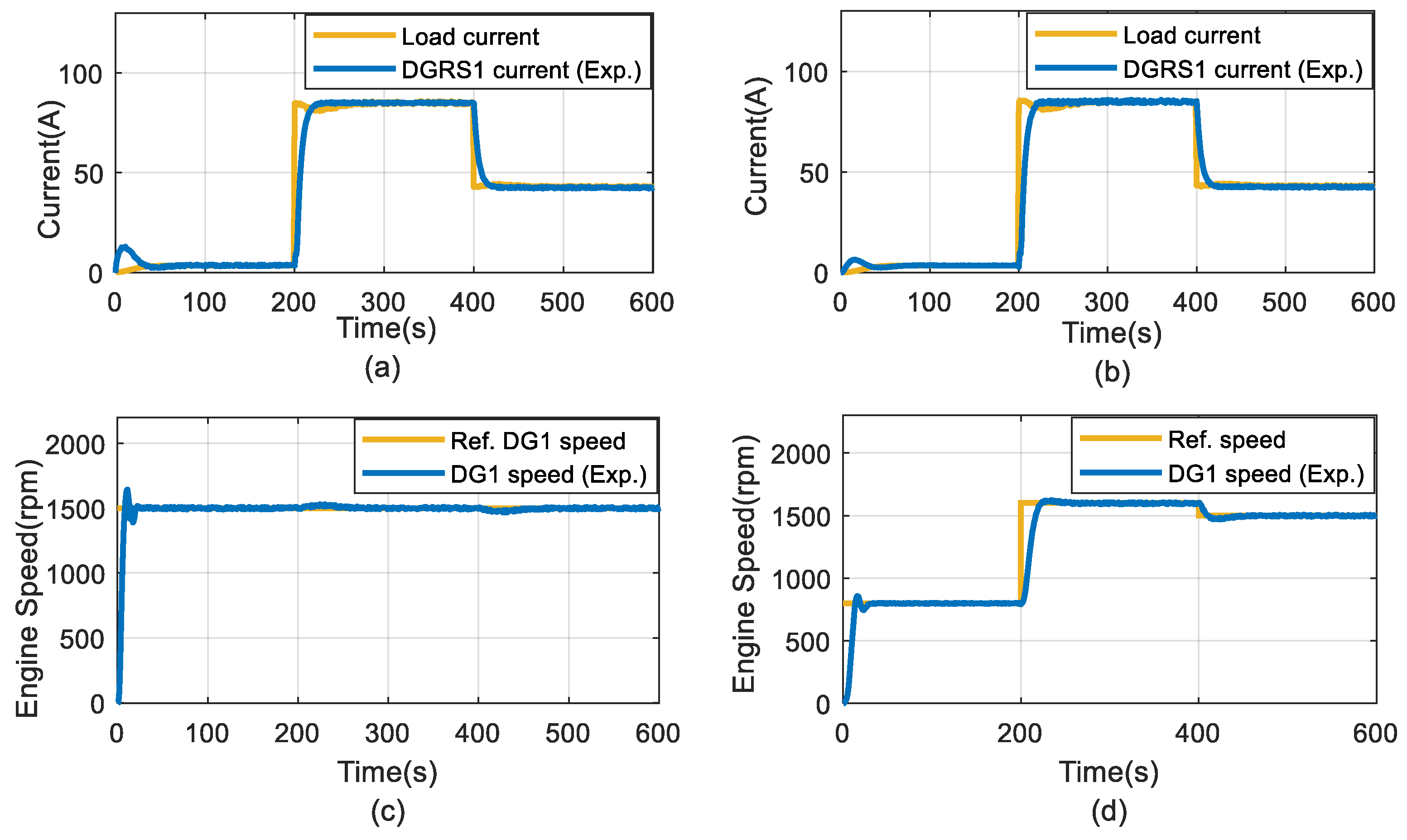
4.2. Simulation and Experimental Results
5. Comparison of Various System Configurations
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Exponential zone amplitude |
| B | Inverse of the exponential zone time constant |
| C | Fuel consumption per hour |
| Damping coefficient of rotor | |
| Duty cycles for the one and two active engine condition | |
| Battery constant voltage | |
| Field voltage vector | |
| Battery current | |
| DC current to DC bus | |
| DC current from converter | |
| Reference d-axis current | |
| Current vector | |
| Inertia of generator | |
| K | Polarisation constant |
| Actuator gain | |
| Control parameters for secondary controller | |
| Inductance on the low voltage side of DC–DC converter | |
| Adjustable inductance in converter | |
| Nitrogen Dioxide | |
| O3 | Ozone |
| Number of poles | |
| Reference power setpoint | |
| Actual power for diesel generator rectifier system | |
| Minimum diesel generator rectifier system power setpoint | |
| Optimal power setpoint of diesel generator rectifier system | |
| Reference power setpoint of diesel generator rectifier system | |
| Load power | |
| Power management system | |
| Output power | |
| DC-source powers for diesel generator rectifier system 1 and 2 | |
| Q | Battery capacity |
| R | Battery internal resistance |
| Resistance matrices | |
| Resistance in the load side of DC–DC converter | |
| SOC% | Battery state of charge |
| Battery state of charge maximal threshold | |
| Battery state of charge minimal threshold | |
| Time constant of the actuator | |
| Electric torque | |
| Mechanical torque | |
| Sample time of converter | |
| Voltage vector | |
| Average voltage on the Low Voltage Side | |
| Average voltage on the High Voltage Side | |
| Battery voltage | |
| DC-link voltage | |
| Reference DC-link voltage | |
| Excitation field voltage | |
| Voltage on the load side of DC–DC converter | |
| Leakage reactance matrices | |
| Coefficients for synchronous diesel generator rectifier system hourly fuel consumption | |
| Coefficients for hourly fuel consumption when two diesel generator rectifier sets work together | |
| Power error of diesel generator rectifier system | |
| Switching frequency of converter | |
| DC current from rectifier | |
| dq-axis components of stator winding phase current | |
| Load current | |
| Filtered current | |
| It | Actual battery charge |
| K | Number of diesel generator rectifier sets |
| Time-delay constant | |
| Control signal from the engine controller | |
| Permutation matrix group | |
| DC voltage of diesel generator rectifier system | |
| dq-axis components of stator winding phase voltage | |
| Integrated reactance | |
| Integrated dq reactance | |
| Speed error | |
| Control signal from the secondary controller | |
| DC–DC converter efficiency | |
| Parameters for the filters in voltage regulator | |
| Phase shift regulated by the adjustable inductance | |
| Reference phase shift | |
| Magnetic flux vector | |
| Magnetizing flux vector | |
| Actual speed | |
| Base speed | |
| Speed reference | |
| Rotor speed | |
| Speed matrix | |
| Slip matrices | |
| Power shift on reference power of diesel generator rectifier system by applying efficiency optimisation system |
Appendix A
| Synchronous generator: |
| Stator resistance = 0.382 Stator leakage reactance = 0.4222 |
| Base electrical angular speed = 3600 rpm |
| Field winding resistance = 0.112 Field winding reactance = 0.5768 |
| Damping dq-axis winding resistance = 14 , = 5.07 |
| Damping dq-axis winding reactance = 3.7209 , = 9.3871 |
| Asynchronous generator: |
| Stator resistance = 0.382 Stator leakage reactance = 0.4222 |
| Base electrical angular speed = 3600 rpm |
| Rotor winding resistance, = 0.11 Rotor reactance = 0.57 |
| Integrated reactance = 0.09 |
| Filter parameters: |
| Mechanical system: |
| Inertia moment J = 0.03 Damping coefficient D = 0.85 |
| Diesel engine parameters: |
| Actuator gain = Actuator constant |
| Time delay |
| Quadratic function coefficients of fuel consumption against DC source: |
| Li-ion Battery: |
Exponential zone amplitude A = 58.78 V = 65% |
| DC to DC converter: |
| F F H = 20,000 Hz Duty cycle D = 50% |
| DC-link capacitor: Ct = 0.006 F |
References
- Liu, M.; Kronbak, J. The potential economic viability of using the Northern Sea Route (NSR) as an alternative route between Asia and Europe. J. Transp. Geogr. 2010, 18, 434–444. [Google Scholar] [CrossRef]
- Aston, F. Rise of the icebreaker [Arctic Ocean vessels]. Eng. Technol. 2017, 12, 48–51. [Google Scholar] [CrossRef]
- Derwent, R.G.; Stevenson, D.S.; Doherty, R.M.; Collins, W.J.; Sanderson, M.G.; Johnson, C.E.; Cofala, J.; Mechler, R.; Amann, M.; Dentener, F.J. The contribution from shipping emissions to air quality and acid deposition in Europe. AMBIO A J. Hum. Environment 2005, 34, 54–59. [Google Scholar] [CrossRef]
- Eyring, V.; Köhler, H.W.; Lauer, A.; Lemper, B. Emissions from international shipping: 2. Impact of future technologies on scenarios until 2050. J. Geophys. Res. 2005, 110. [Google Scholar] [CrossRef]
- Acciaro, M.; Hoffmann, P.N.; Eide, M.S. The energy efficiency gap in maritime transport. J. Shipp. Ocean. Eng. 2013, 3, 1. [Google Scholar]
- European Commission. 2019 Annual Report on CO2 Emissions from Maritime Transport; European Commission: Brussels, Belgium, 2020. [Google Scholar]
- Gibson, M.; Murphy, A.J.; Pazouki, K. Evaluation of environmental performance indices for ships. Transp. Res. D Transp. Environ. 2019, 73, 152–161. [Google Scholar] [CrossRef]
- Schröder, C.; Reimer, N.; Jochmann, P. Environmental impact of exhaust emissions by Arctic shipping. AMBI 2017, 46, 400–409. [Google Scholar] [CrossRef]
- Riska, K. Design of ice breaking ships. Course Mater. NTNU 2011, 114. Available online: https://scholar.google.com/scholar?hl=en&as_sdt=0%2C5&q=Riska%2C+K.+Design+of+ice+breaking+ships&btnG= (accessed on 13 June 2023).
- Paine, L.P. Ships of the World: An Historical Encyclopedia, 2nd ed.; Houghton Mifflin: Boston, MA, USA, 1998. [Google Scholar]
- Geertsma, R.D.; Negenborn, R.R.; Visser, K.; Hopman, J.J. Design and control of hybrid power and propulsion systems for smart ships: A review of developments. Appl. Energy 2017, 194, 30–54. [Google Scholar]
- Satpathi, K.; Ukil, A.; Pou, J. Short-circuit fault management in DC electric ship propulsion system: Protection requirements, review of existing technologies and future research trends. IEEE Trans. Transp. Electrif. 2017, 4, 272–291. [Google Scholar] [CrossRef]
- Hodge, C.G.; Mattick, D.J. The electric warship then, now and later. In Proceedings of the 9th international naval engineering conference, Hamburg, Germany, 1–3 April 2008. [Google Scholar]
- Strategies for the Success of Nuclear-Powered Commercial Shipping. In Report Submitted to Connecticut Maritime Association. 2014. Available online: https://d1wqtxts1xzle7.cloudfront.net/36943308/Strategies_for_the_Success_of_Nuclear_Powered_Commercial_Shipping-libre.pdf?1426110055=&response-content-disposition=inline%3B+filename%3DStrategies_for_the_Success_of_Nuclear_Po.pdf&Expires=1692790493&Signature=fJSPfCz6dBIhufXGJnJ3jzCh-~hu2xRKwBuQ6USqEYHNpi5upxDfELGHEtbC1t6Zalgk2aH58LTVPl2avkhUEz0hBZWW-2WgKqsc8ppS6q64s4fd8ji6xGoJCAHDCkfQVHJuAorJ7sTjiorl~vVP0ejsmpk8MYXwO2tVlcb7JcmzYIBPDqck9HYtQsGFTYFKKW4ecm5h4oYZSKa-Y-EPqo2-PbtVV0KDKiecLpbcKWnQeKQ-fQJYWMF8m6CZXSSPFlBGKErUcyfj5Ep3ycpeRhef9SanwDQC5d0MLe6~~3qW4m83M5gc6RWqweCEaLhuAa29tHxz1rDBhm-kimMfyw__&Key-Pair-Id=APKAJLOHF5GGSLRBV4ZA (accessed on 21 February 2023).
- Çabukoglu, E.; Georges, G.; Küng, L.; Pareschi, G.; Boulouchos, K. Battery electric propulsion: An option for heavy-duty vehicles? Results from a Swiss case-study. Transp. Res. Part C Emerg. Technol. 2018, 88, 107–123. [Google Scholar] [CrossRef]
- ABS Advisory on Hybrid Electric Power Systems. Available online: https://ww2.eagle.org/content/dam/eagle/advisories-and-debriefs/ABS_Hybrid_Advisory_17033.pdf (accessed on 21 February 2023).
- Lindtjørn, J.O.; Wendt, F.; Gundersen, B.; Hansen, J.F. Demonstrating the benefits of advanced power systems and energy storage for DP vessels. In Proceedings of the Dynamic Positioning Conference, Houston, TX, USA, 14–15 October 2014. [Google Scholar]
- Ghimire, P.; Zadeh, M.; Pedersen, E.; Thorstensen, J. Dynamic Modeling, Simulation, and Testing of a Marine DC Hybrid Power System. IEEE Trans. Transp. Electrif. 2020, 7, 905–919. [Google Scholar] [CrossRef]
- Skjong, S.; Taskar, B.; Pedersen, E.; Steen, S. Simulation of a Hybrid Marine Propulsion System in Waves. In Proceedings of the 28th CIMAC World Congress, Helsinki, Finland, 6–10 June 2016. [Google Scholar]
- Navy DDG-1000 and DDG-51 Destroyer Programs: Background, Oversight issues, and Options for Congress. Available online: https://apps.dtic.mil/sti/pdfs/ADA500951.pdf (accessed on 21 February 2023).
- Haseltalab, A.; Botto, M.A.; Negenborn, R.R. Model Predictive DC Voltage Control for all-electric ships. Control. Eng. Pract. 2019, 90, 133–147. [Google Scholar] [CrossRef]
- Park, H.; Sun, J.; Pekarek, S.; Stone, P.; Opila, D.; Meyer, R.; Kolmanovsky, I.; DeCarlo, R. Real-time model predictive control for shipboard power management using the IPA-SQP approach. IEEE Trans Control Syst Technol. 2015, 23, 2129–2143. [Google Scholar] [CrossRef]
- Meral, M.E.; Çelík, D. A comprehensive survey on control strategies of distributed generation power systems under normal and abnormal conditions. Annu. Rev. Control. 2019, 47, 112–132. [Google Scholar] [CrossRef]
- Al-Falahi, M.D.; Jayasinghe, S.D.; Enshaei, H. Hybrid algorithm for optimal operation of hybrid energy systems in electric ferries. Energy 2019, 187, 115923. [Google Scholar] [CrossRef]
- Yuan, Y.; Zhang, T.; Shen, B.; Yan, X.; Long, T. A fuzzy logic energy management strategy for a photovoltaic/diesel/battery hybrid ship based on experimental database. Energies 2018, 11, 2211. [Google Scholar] [CrossRef]
- Bui, T.M.; Dinh, T.Q.; Marco, J.; Watts, C. An Energy Management Strategy for DC Hybrid Electric Propulsion System of Marine Vessels. In Proceedings of the 2018 5th International Conference on Control, Decision and Information Technologies (CoDIT), Thessaloniki, Greece, 10–13 April 2018. [Google Scholar]
- Jianyun, Z.; Li, C.; Lijuan, X.; Bin, W. Bi-objective optimal design of plug-in hybrid electric propulsion system for ships. Energy 2019, 177, 247–261. [Google Scholar] [CrossRef]
- Jatskevich, J.; Pekarek, S.D.; Davoudi, A. Parametric average-value model of synchronous machine-rectifier systems. IEEE Trans. Energy Convers. 2006, 21, 9–18. [Google Scholar] [CrossRef]
- Karrari, M.; Rosehart, W.; Malik, O.P. Comprehensive control strategy for a variable speed cage machine wind generation unit. IEEE Trans. Energy Convers. 2005, 20, 415–423. [Google Scholar] [CrossRef]
- Tremblay, O.; Dessaint, L.A. Experimental validation of a battery dynamic model for EV applications. World Electr. Veh. J. 2009, 3, 289–298. [Google Scholar] [CrossRef]
- Evangelou, S.A.; Shukla, A. Advances in the modelling and control of series hybrid electric vehicles. In Proceedings of the 2012 American Control Conference (ACC), Montreal, QC, Canada, 27–29 June 2012. [Google Scholar]
- Bassam, A.M.; Phillips, A.B.; Turnock, S.R.; Wilson, P.A. Development of a multi-scheme energy management strategy for a hybrid fuel cell driven passenger ship. Int. J. Hydrogen Energy 2017, 42, 623–635. [Google Scholar] [CrossRef]
- Shahab, A. Dynamic Average-Value Modeling of Doubly-Fed Induction Generator Wind Energy Conversion Systems. Master’ Thesis, University of Manitoba, Winnipeg, MB, Canada, 2013. [Google Scholar]
- Zahedi, B.; Norum, L.E. Modeling and simulation of all-electric ships with low-voltage DC hybrid power systems. IEEE Trans. Power Electron. 2012, 28, 4525–4537. [Google Scholar] [CrossRef]
- Li, H.; Peng, F.Z.; Lawler, J.S. A natural ZVS high-power bi-directional DC-DC converter with minimum number of devices. In Proceedings of the Conference Record of the 2001 IEEE Industry Applications Conference. 36th IAS Annual Meeting, Chicago, IL, USA, 30 September–4 October 2001. [Google Scholar]
- Guerrero, J.M.; Vasquez, J.C.; Matas, J.; De Vicuña, L.G.; Castilla, M. Hierarchical control of droop-controlled AC and DC microgrids—A general approach toward standardization. IEEE Trans. Ind. Electron. 2010, 58, 158–172. [Google Scholar] [CrossRef]
- Chua, L.W.; Tjahjowidodo, T.; Seet, G.G.; Chan, R. Implementation of optimisation-based power management for all-electric hybrid vessels. IEEE Access 2018, 6, 74339–74354. [Google Scholar] [CrossRef]
- Zhou, Y.; Pazouki, K.; Norman, R. The Modelling and Optimal Control of a Hybrid Propulsion System for an Ice-Capable Ship. In Proceedings of the 38th International Conference on Offshore Mechanics and Arctic Engineering, Glasgow, Scotland, UK, 9–14 June 2019. [Google Scholar]
- Kim, K.; Park, K.; Roh, G.; Chun, K. DC-grid system for ships: A study of benefits and technical considerations. J. Int. Marit. Saf. Environ. Aff. Shipp. 2018, 2, 1–12. [Google Scholar] [CrossRef]







| Equation (22) | ||
Battery Ch. | Battery Dis. | ||
Battery Ch. | Battery Dis. |
| Components | |
|---|---|
| Energy Storage System | Lithium-ion, 384 V, 38 kW, |
| DC-link Voltage | 1000 V |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Pazouki, K.; Norman, R.; Gao, H.; Lin, Z. An Experimental Investigation into the Feasibility of a DC Hybrid Power Plant for a Northern Sea Route Ship. J. Mar. Sci. Eng. 2023, 11, 1653. https://doi.org/10.3390/jmse11091653
Zhou Y, Pazouki K, Norman R, Gao H, Lin Z. An Experimental Investigation into the Feasibility of a DC Hybrid Power Plant for a Northern Sea Route Ship. Journal of Marine Science and Engineering. 2023; 11(9):1653. https://doi.org/10.3390/jmse11091653
Chicago/Turabian StyleZhou, Yi, Kayvan Pazouki, Rose Norman, Haibo Gao, and Zhiguo Lin. 2023. "An Experimental Investigation into the Feasibility of a DC Hybrid Power Plant for a Northern Sea Route Ship" Journal of Marine Science and Engineering 11, no. 9: 1653. https://doi.org/10.3390/jmse11091653
APA StyleZhou, Y., Pazouki, K., Norman, R., Gao, H., & Lin, Z. (2023). An Experimental Investigation into the Feasibility of a DC Hybrid Power Plant for a Northern Sea Route Ship. Journal of Marine Science and Engineering, 11(9), 1653. https://doi.org/10.3390/jmse11091653










