Softening/Hardening Damage Model and Numerical Implementation of Seabed Silt-Steel Interface in Yellow River Underwater Delta
Abstract
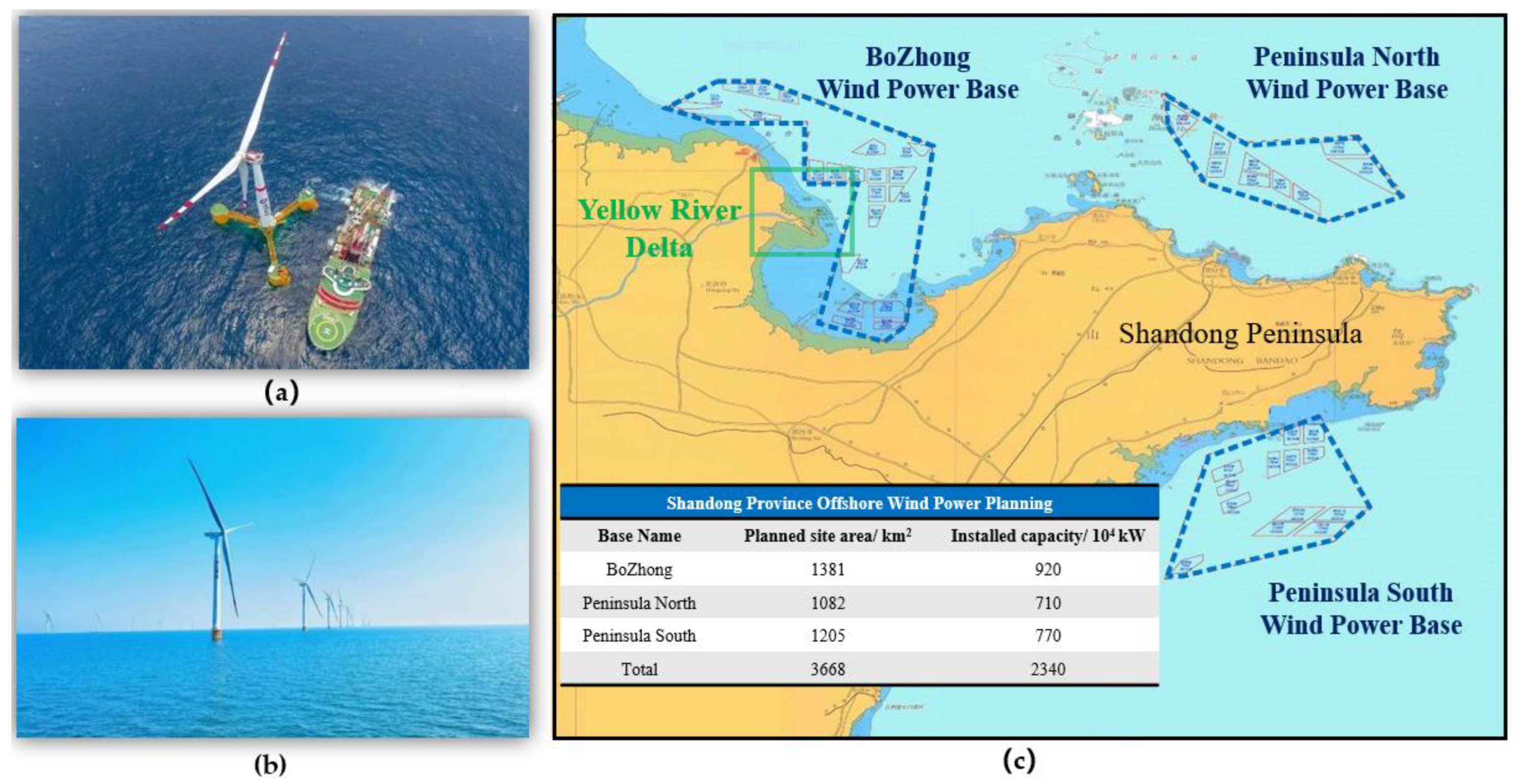
:1. Introduction
2. Softening/Hardening Damage Model
2.1. Assumption
- (1)
- Assuming the soil is isotropic and the structure is a fixed rigid body.
- (2)
- Divide the soil–structure interface into several microelements, assuming that there are only two states: undamaged (0) and damaged (1).
- (3)
- Assuming that all friction and contact on the soil–structure interface (between soil particles, between soil structure) are unevenly and randomly distributed, the shear strength and shear failure at the interface are also unevenly and randomly distributed.
- (4)
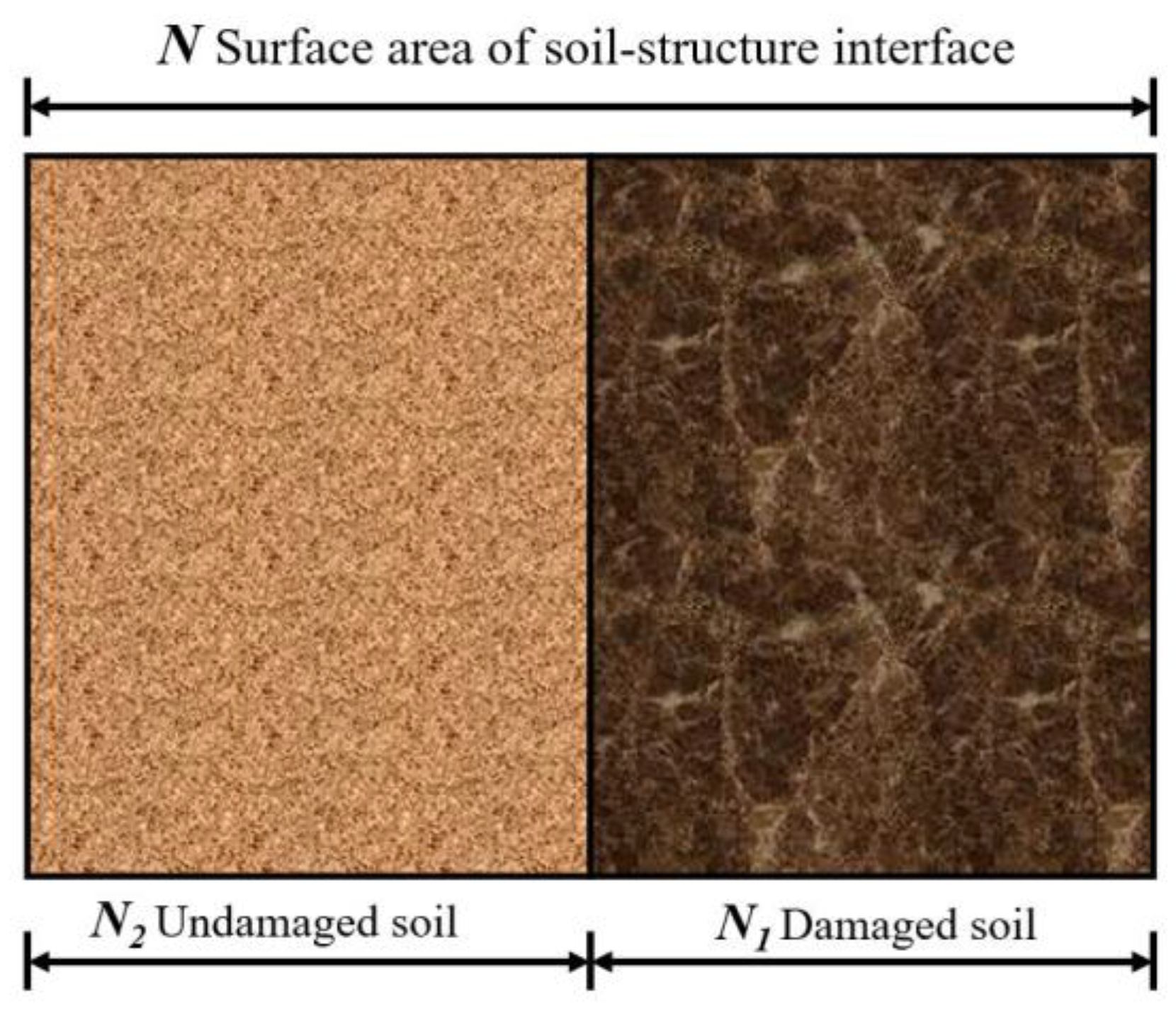
- Assuming that the damage process at the soil–structure interface is a continuous process that undergoes an evolution from undamaged to damage, the soil at the interface can be divided into two parts, damaged soil and undamaged soil (Figure 2), and the undamaged soil bears effective stress.
2.2. Model Derivation
2.3. Model Parameters
3. Interface Monotonic Shear Test
3.1. Test Equipment and Plan
3.2. Results and Analysis
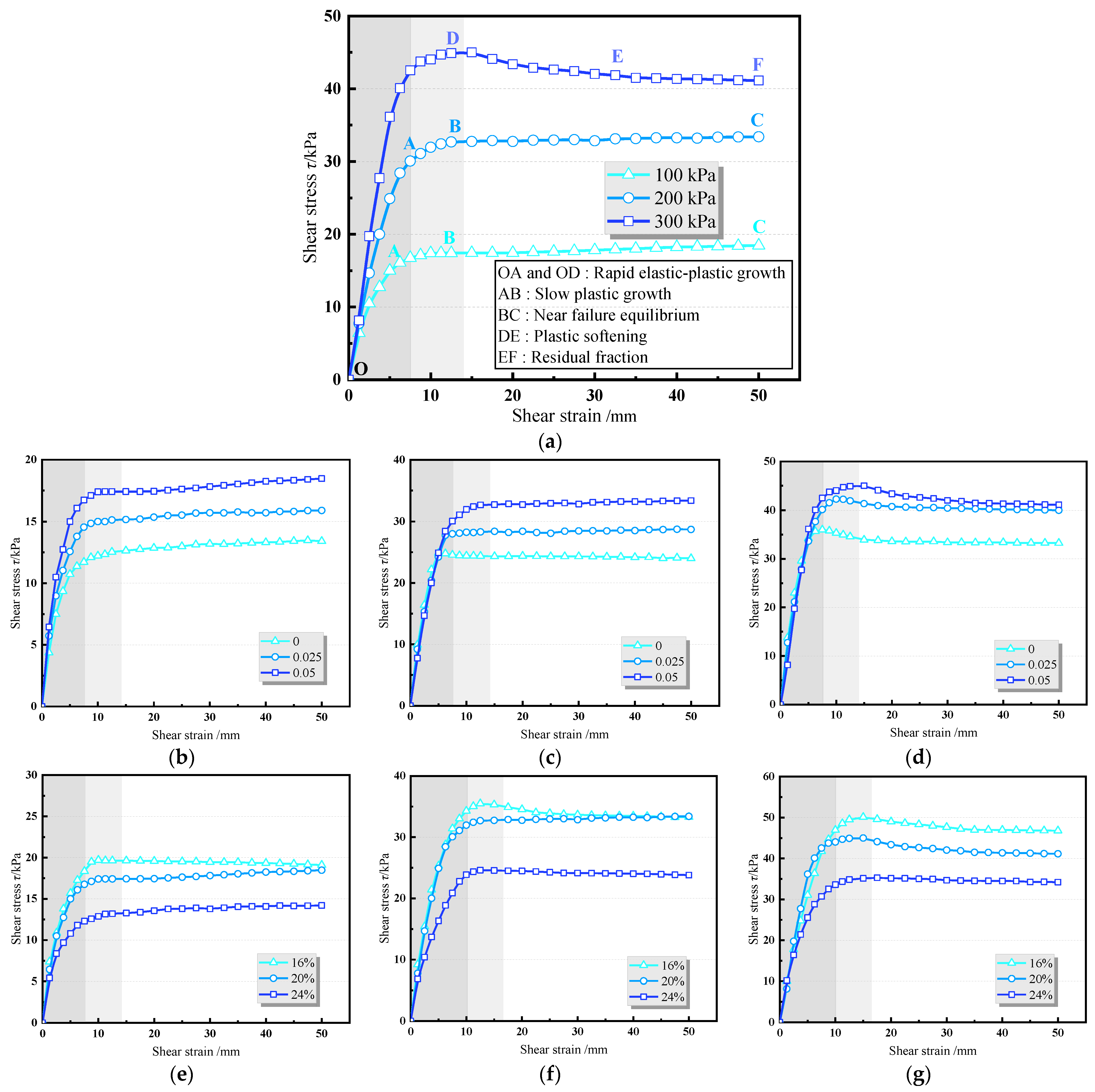
3.2.1. Stress-Strain Rule
- (1)
- Normal stress
- (2)
- Roughness
- (3)
- Water content
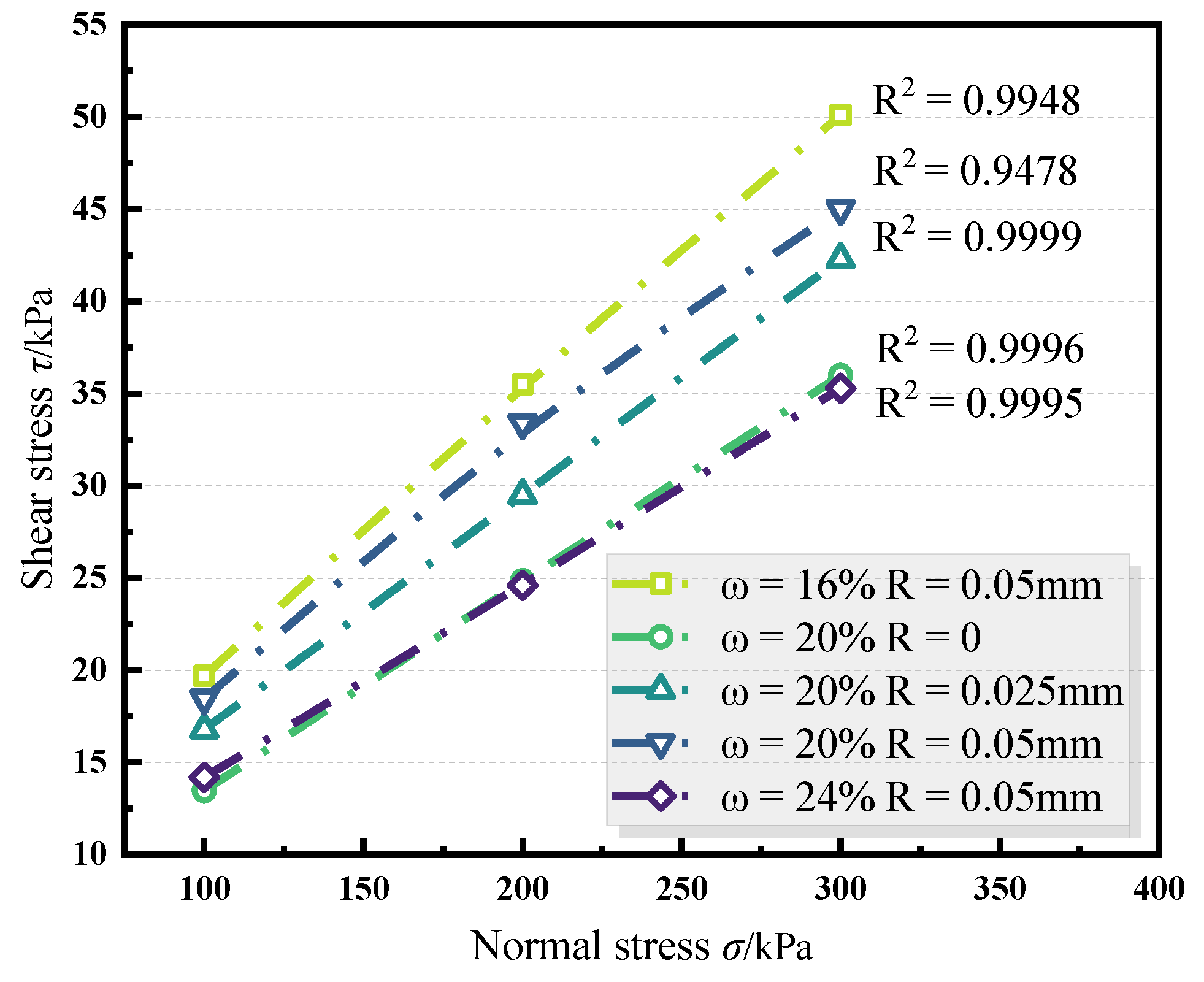
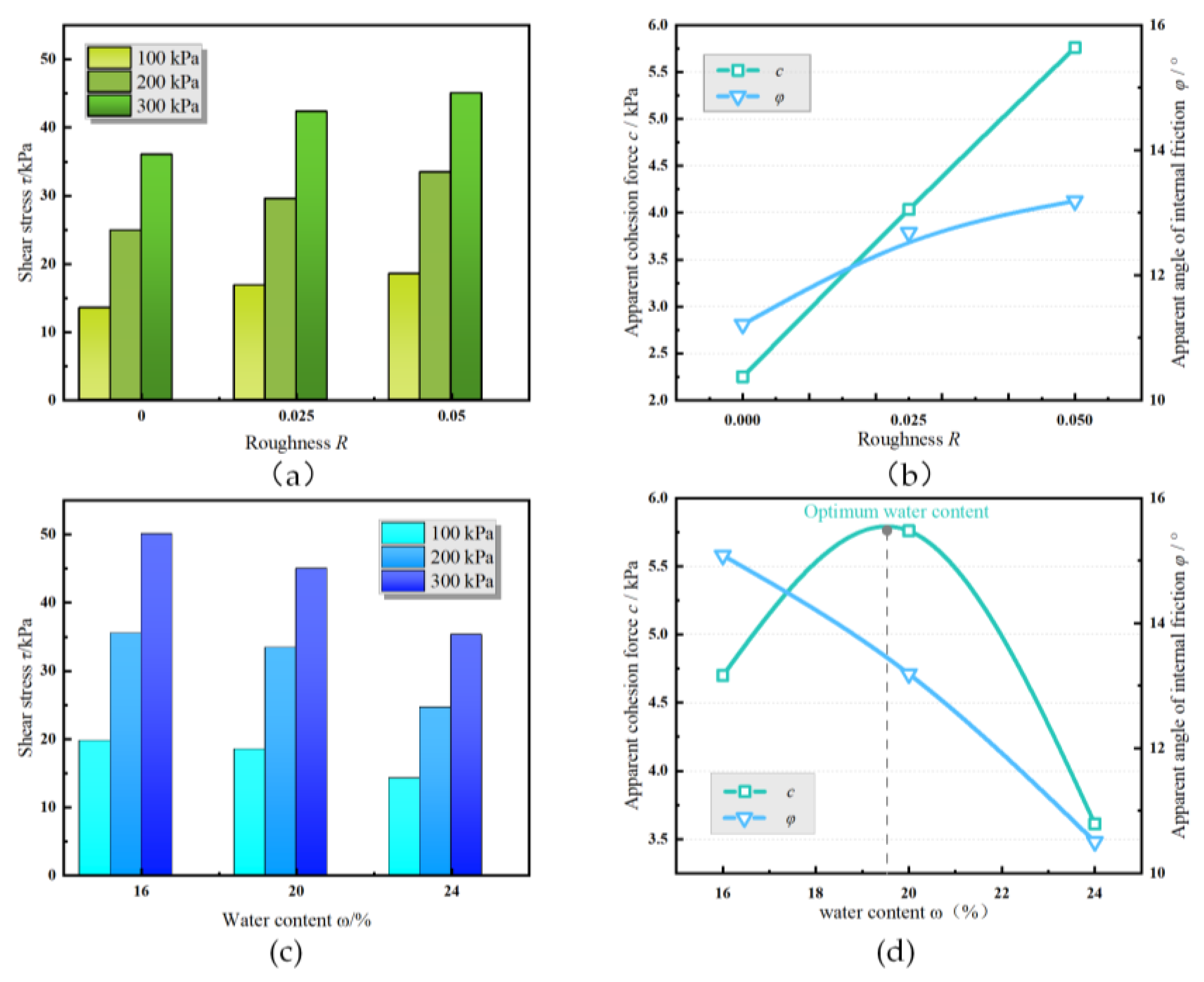
3.2.2. Interface Shear Strength
- (1)
- Roughness
- (2)
- Water content
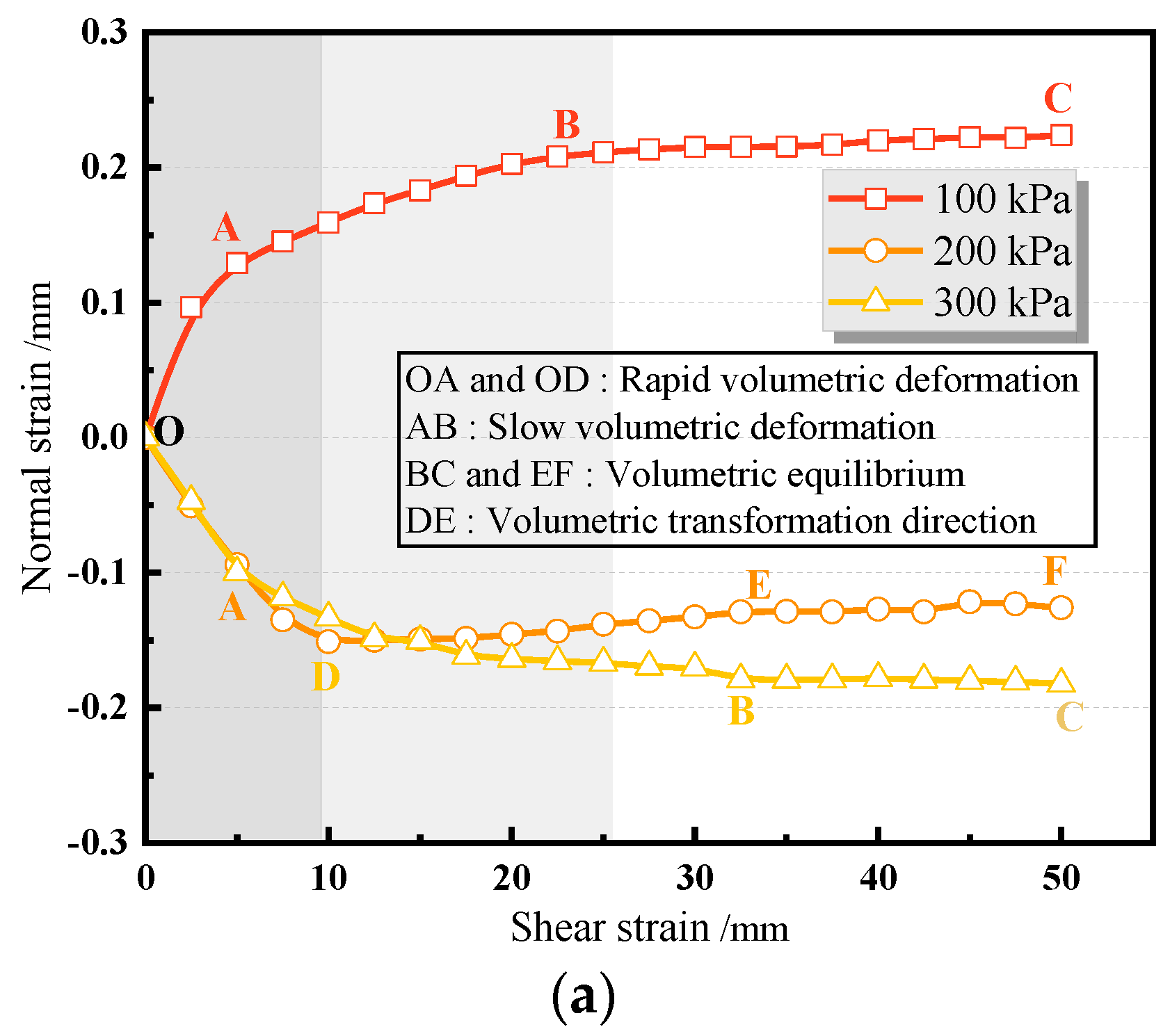
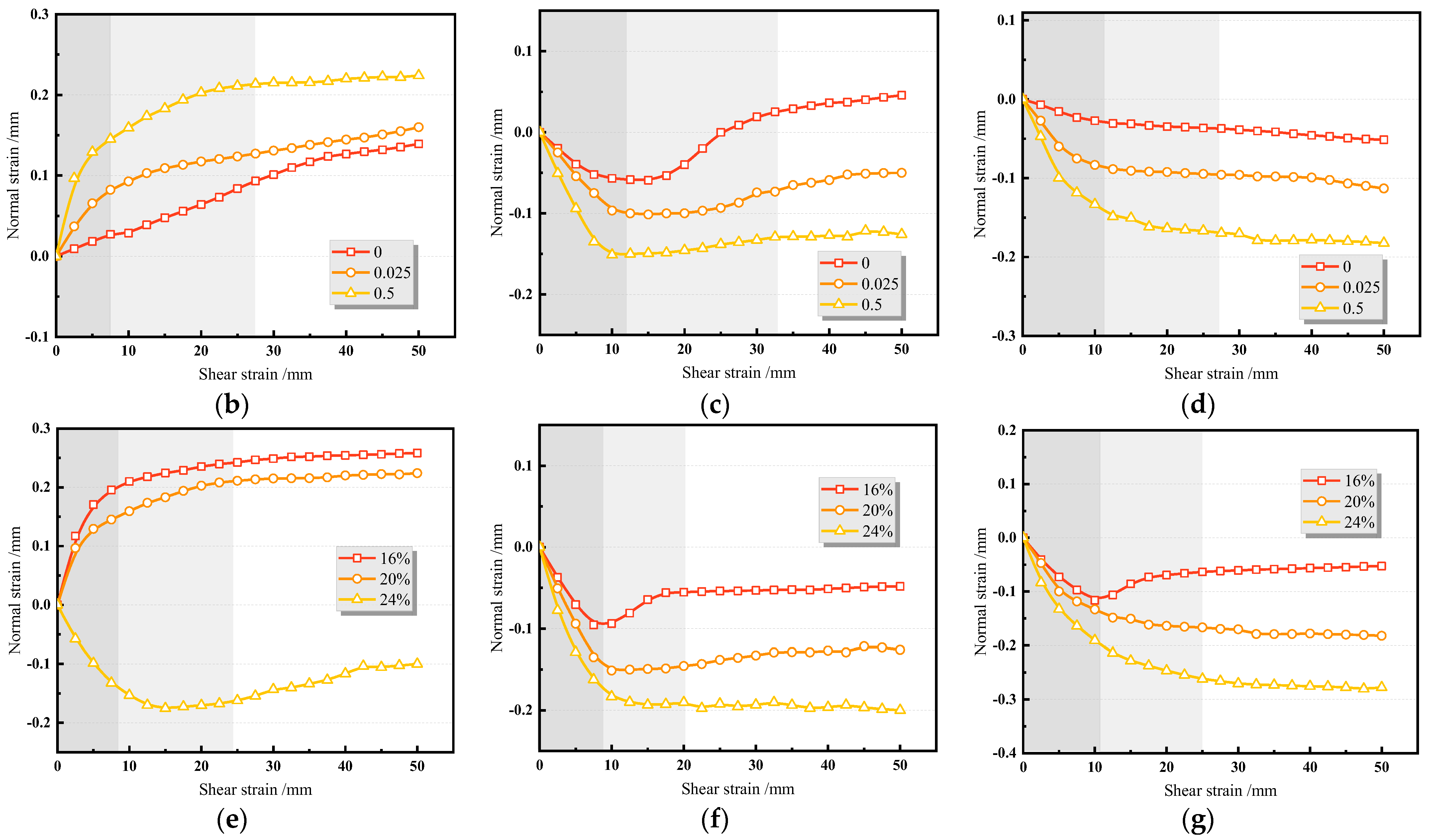
3.2.3. Volumetric Deformation Laws
- (1)
- Normal stress
- (2)
- Roughness
- (3)
- Water content
4. Model Validation and Numerical Implementation
4.1. Model Validation
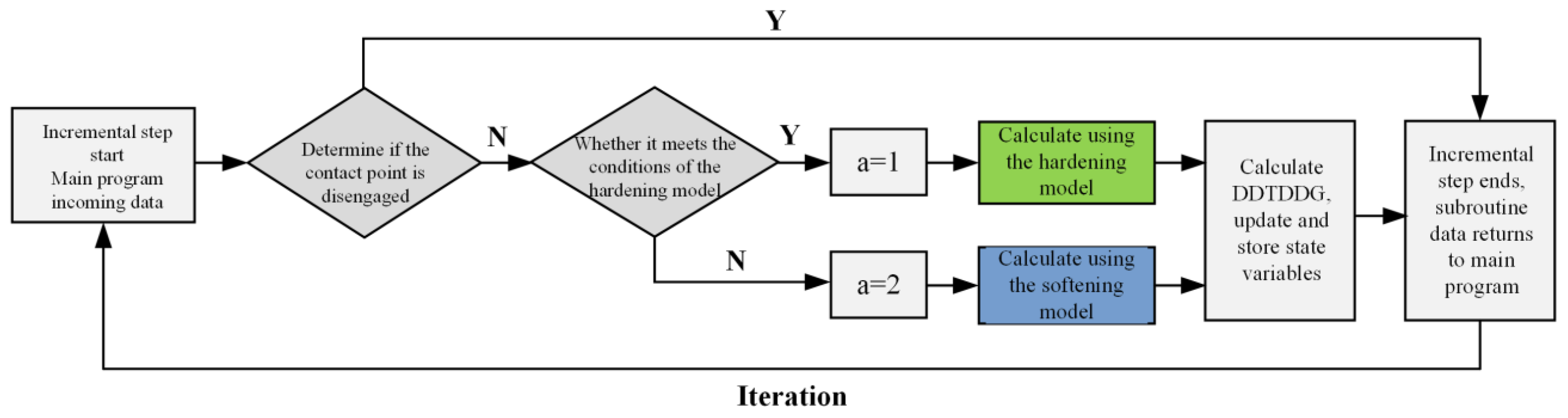
4.2. Numerical Implementation
4.2.1. Subroutine Programming
4.2.2. Subroutine Validation
5. Conclusions
- (1)
- Compared to the strain hardening, shear shrinkage deformation and strain softening, the shear expansion deformation behavior of coarse-grained soil and cohesive soil silt exhibits two characteristics: softening/hardening and shear shrinkage/expansion under different conditions. The maximum shear stress increases with normal stress, roughness, and water content increase. Roughness has a significant impact on cohesion. As roughness increases, the cohesion and internal friction angle increase 1.56 times and by 17.67%, respectively; water content mainly affects the internal friction angle. As the water content increases, the internal friction angle decreases, with a decrease of 30.37%. The cohesion shows a trend of increasing and then decreasing, with an increase of 22.6% and −59.5%, respectively, and reaches its peak near optimal water content.
- (2)
- The proposed shear damage model has fewer parameters and a clear physical meaning. The strength parameters are τmax, G0, G1, γm, τr, and the model parameters are m and n. The softening model, based on the classic rock damage model, can better simulate the stress–strain relationship of the silt–steel interface under high normal stress and low water content. In contrast, the hardening model based on the classic hyperbola model can better simulate the stress–strain relationship under low normal stress and high water content. After using the model parameters obtained from the test results, the theoretical model curve is in good agreement with the shear test curve, which proves that the proposed contact surface damage model is reasonable. However, the model parameters m and n are simultaneously affected by normal stress, roughness and water content, so they cannot be simply corrected by fitting curves. Considering the complexity of factors affecting the soil–structure interface in real environments, the model still needs further optimization and improvement.
- (3)
- The numerical simulation application of the damage model was achieved through the FRIC subroutine, providing a mathematical model basis for subsequent research on the stability analysis of offshore structures considering interface weakening effects.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Guo, X.; Fan, N.; Liu, Y.; Liu, X.; Wang, Z.; Xie, X.; Jia, Y. Deep seabed mining: Frontiers in engineering geology and environment. Int. J. Coal Sci. Technol. 2023, 10, 23. [Google Scholar] [CrossRef]
- Yu, P.; Liu, H.; Wang, Z.; Fu, J.; Zhang, H.; Wang, J.; Yang, Q. Development of urban underground space in coastal cities in China: A review. Deep. Undergr. Sci. Eng. 2023, 2, 148–172. [Google Scholar] [CrossRef]
- Yu, P.; Hu, R.; Yang, J.; Liu, H. Numerical investigation of local scour around USAF with different hydraulic conditions under currents and waves. Ocean Eng. 2020, 213, 107696. [Google Scholar] [CrossRef]
- Zhang, Y.; Feng, X.; Ding, C.; Liu, Y.; Liu, T. Study of cone penetration rate effects in the Yellow River Delta silty soils with different clay contents and state parameters. Ocean Eng. 2022, 250, 110982. [Google Scholar] [CrossRef]
- Ravera, E.; Laloui, L. Failure mechanism of fine-grained soil-structure interface for energy piles. Soils Found. 2022, 62, 101152. [Google Scholar] [CrossRef]
- Hashemi, A.; Sutman, M.; Medero, G.M. A review on the thermo-hydro-mechanical response of soil–structure interface for energy geostructures applications. Géoméch. Energy Environ. 2023, 33, 100439. [Google Scholar] [CrossRef]
- Liu, J.; Zou, D.; Kong, X. A three-dimensional state-dependent model of soil–structure interface for monotonic and cyclic loadings. Comput. Geotech. 2014, 61, 166–177. [Google Scholar] [CrossRef]
- Stutz, H.; Mašín, D.; Wuttke, F. Enhancement of a hypoplastic model for granular soil–structure interface behaviour. Acta Geotech. 2016, 11, 1249–1261. [Google Scholar] [CrossRef]
- Hosseinali, M.; Toufigh, V. A plasticity-based constitutive model for the behavior of soil-structure interfaces under cyclic loading. Transp. Geotech. 2018, 14, 41–51. [Google Scholar] [CrossRef]
- Saberi, M.; Annan, C.-D.; Konrad, J.-M. Three-dimensional constitutive model for cyclic behavior of soil-structure interfaces. Soil Dyn. Earthq. Eng. 2020, 134, 106162. [Google Scholar] [CrossRef]
- De-chun, L.U.; Lei, L.; Xin, W.; Xiu-li, D.U. Softening/Hardening constitutive model for soil-structure interface and numerical implementation. Eng. Mech. 2017, 34, 41–50. [Google Scholar] [CrossRef]
- Ajcinovic, K.R.D.; Silva, M.A.G. Statistical of the continuous damage theory. Int. J. Solids. Struct. 1982, 19, 551–562. [Google Scholar] [CrossRef]
- Hu, L.; Pu, J. Testing and Modeling of Soil-Structure Interface. J. Geotech. Geoenviron. 2004, 130, 851–860. [Google Scholar] [CrossRef]
- Long, Y.; Chen, J.; Zhang, J. Introduction and analysis of a strain-softening damage model for soil–structure interfaces considering shear thickness. KSCE J. Civ. Eng. 2017, 21, 2634–2640. [Google Scholar] [CrossRef]
- Zhao, L.; Yang, P.; Zhang, L.-C.; Wang, J. Cyclic direct shear behaviors of an artificial frozen soil-structure interface under constant normal stress and sub-zero temperature. Cold Reg. Sci. Technol. 2017, 133, 70–81. [Google Scholar] [CrossRef]
- Zhu, Z.W.; Guo, Z. Temperature damage and constitutive model of frozen soil under dynamic loading. Mech. Mater. 2016, 102, 108–116. [Google Scholar] [CrossRef]
- Chen, W.; Luo, Q.; Liu, J.; Wang, T.; Wang, L. Modeling of frozen soil-structure interface shear behavior by supervised deep learning. Cold Reg. Sci. Technol. 2022, 200, 10358. [Google Scholar] [CrossRef]
- Sun, K.; Tang, L.; Zhou, A.; Ling, X. An elastoplastic damage constitutive model for frozen soil based on the super/subloading yield surfaces. Comput. Geotech. 2020, 128, 103842. [Google Scholar] [CrossRef]
- Liu, L.; Ji, H.; Elsworth, D.; Zhi, S.; Lv, X.; Wang, T. Dual-damage constitutive model to define thermal damage in rock. Int. J. Rock Mech. Min. Sci. 2019, 126, 104185. [Google Scholar] [CrossRef]
- Rehman, Z.; Zhang, G. Three-dimensional elasto-plastic damage model for gravelly soil-structure interface considering the shear coupling effect. Compu. Geotech. 2021, 129, 103868. [Google Scholar] [CrossRef]
- Yang, Q.; Zheng, Z.; El Naggar, M.H.; Kong, G.; Zhang, S.; Yang, G.; Ren, Y. Experimental study of the interface evolution behavior and softening mechanism of structure-marine soil. Soils Found. 2023, 63, 101324. [Google Scholar] [CrossRef]
- Zhang, P.; Fei, K.; Dai, D. Modeling of granular soil-structure interface under monotonic and cyclic loading with the nonlinear approach. Comput. Geotech. 2023, 159, 105480. [Google Scholar] [CrossRef]
- Clough, G.W.; Duncan, J.M. Finite element analysis of retaining wall behavior. J. Soil. Mech. Found 1971, 97, 1657–1672. [Google Scholar] [CrossRef]
- Yu, P.; Dong, J.; Liu, H.; Xu, R.; Wang, R.; Xu, M.; Liu, H. Analysis of Cyclic Shear Stress–Displacement Mechanical Properties of Silt–Steel Interface in the Yellow River Delta. J. Mar. Sci. Eng. 2022, 10, 1704. [Google Scholar] [CrossRef]
- Lemaiter, J. A continuous damage mechanics model for ductile materials. J. Eng. Mater. Techno. 1985, 107, 83–89. [Google Scholar]
- Yu, P.; Dong, J.; Guan, Y.; Wang, Q.; Jia, S.; Xu, M.; Liu, H.; Yang, Q. Experimental Investigation on the Cyclic Shear Mechanical Characteristics and Dynamic Response of a Steel–Silt Interface in the Yellow River Delta. J. Mar. Sci. Eng. 2023, 11, 223. [Google Scholar] [CrossRef]
- Su, L.-J.; Zhou, W.-H.; Chen, W.-B.; Jie, X. Effects of relative roughness and mean particle size on the shear strength of sand-steel interface. Measurement 2018, 122, 339–346. [Google Scholar] [CrossRef]
- Abdulrahman, A.A.; Rayhani, M. Interface shear strength characteristics of steel piles in frozen clay under varying exposure temperature. Soils. Found. 2019, 59, 2110–2124. [Google Scholar] [CrossRef]
- Ma, X.; Lei, H.; Kang, X. Examination of interface roughness and particle morphology on granular soil–structure shearing behavior using DEM and 3D printing. Eng. Struct. 2023, 290, 116365. [Google Scholar] [CrossRef]
- Chusak, K. Effect of oil-contamination and water saturation on the bearing capacity and shear strength parameters of silty sandy soil. Eng. Geol. 2019, 257, 105138. [Google Scholar] [CrossRef]
- Wang, T.-L.; Wang, H.-H.; Hu, T.-F.; Song, H.-F. Experimental study on the mechanical properties of soil-structure interface under frozen conditions using an improved roughness algorithm. Cold Reg. Sci. Technol. 2019, 158, 62–68. [Google Scholar] [CrossRef]
- El Hariri, A.; Ahmed, A.E.E.; Kiss, P. Review on soil shear strength with loam sand soil results using direct shear test. J. Terramech. 2023, 107, 47–59. [Google Scholar] [CrossRef]
- Jiang, Q.; Yang, Y.; Yan, F.; Zhou, J.; Li, S.; Yang, B.; Zheng, H. Deformation and failure behaviours of rock-concrete interfaces with natural morphology under shear testing. Constr. Build. Mater. 2021, 293, 123468. [Google Scholar] [CrossRef]
- Timbolmas, C.; Bravo, R.; Rescalvo, F.J.; Villanueva, P.; Portela, M. Digital image correlation and numerical analysis of CFRP-poplar timber interface subjected to modified single shear test. Compos. Struct. 2023, 320, 117188. [Google Scholar] [CrossRef]
- Yang, W.; He, J.; Liu, L.; Yang, H. Testing the shearing creep of composite geomembranes-cushion interface and its empirical model. Soils Found. 2022, 62, 101236. [Google Scholar] [CrossRef]
- Guo, X.; Nian, T.; Fu, C.; Zheng, D. Numerical Investigation of the Landslide Cover Thickness Effect on the Drag Forces Acting on Submarine Pipelines. J. Waterw. Port Coast. Ocean Eng. 2023, 149, 04022032. [Google Scholar] [CrossRef]
- Yan, D.J. Numerical Simulation Analysis of Interfacial Mechanical Behavior of Concrete Structures Strengthened by CFRP Grid-Polymer Cement Mortar (PCM). Master’s Thesis, Chang an University, Xi’an, China, 2020. (In Chinese). [Google Scholar]
- Shi, F. Numerical Simulation of Interfacial Debonding between FRP and Concrete under Fatigue Loading. Master’s Thesis, China University of Petroleum, Beijing, China, 2021. (In Chinese). [Google Scholar]
- Wang, F.L. Experimental Study and Finite Element Simulation of Interfacial Bond Behavior of CFRP- Concrete under Cyclic Loading. Master’s Thesis, Qingdao University of Technology, Qingdao, China, 2021. (In Chinese). [Google Scholar]
- Wang, W.D.; Li, Y.H.; Wu, J.B. Pile-soil interface shear model of super long bored pile and its FEM simulation. Rock Soil Mech. 2012, 33, 3818–3824+3832. (In Chinese) [Google Scholar] [CrossRef]



| Group | Roughness R (mm) | Water Content ω (%) | Normal Stress σ (kPa) |
|---|---|---|---|
| 1 | 0.05 | 16 | 100 |
| 2 | 200 | ||
| 3 | 300 | ||
| 4 | 20 | 100 | |
| 5 | 200 | ||
| 6 | 300 | ||
| 7 | 24 | 100 | |
| 8 | 200 | ||
| 9 | 300 | ||
| 10 | 0 | 20 | 100 |
| 11 | 200 | ||
| 12 | 300 | ||
| 13 | 0.025 | 100 | |
| 14 | 200 | ||
| 15 | 300 |
| Test Conditions | τmax (kPa) | τr (kPa) | γm (mm) | m | n | |||
|---|---|---|---|---|---|---|---|---|
| Test Value | Theoretical Value | Test Value | Theoretical Value | Test Value | Theoretical Value | |||
| Hardening type | ||||||||
| σ = 100 kPa R = 0.05 mm ω = 20% | 18.47 | 18.61 | 0.4271 | 4.4132 × 10−3 | ||||
| σ = 200 kPa R = 0.05 mm ω = 20% | 33.43 | 33.53 | 0.3175 | 2.2541 × 10−3 | ||||
| σ = 100 kPa R = 0.025 mm ω = 20% | 15.92 | 16.53 | 0.5137 | 4.6871 × 10−3 | ||||
| σ = 100 kPa R = 0.05 mm ω = 24% | 14.21 | 13.71 | 0.5546 | 5.1247 × 10−3 | ||||
| Softening type | ||||||||
| σ = 200 kPa R = 0.05 mm ω = 16% | 35.53 | 35.01 | 33.28 | 33.01 | 12.4 | 13.6 | 0.2985 | 2.1187 × 10−3 |
| σ = 300 kPa R = 0.05 mm ω = 16% | 50.12 | 50.93 | 46.78 | 47.42 | 15.1 | 15.7 | 0.1782 | 1.7895 × 10−3 |
| σ = 300 kPa R = 0.05 mm ω = 20% | 45.08 | 46.61 | 41.14 | 42.33 | 12.6 | 14.3 | 0.2297 | 2.0891 × 10−3 |
| σ = 300 kPa R = 0.025 mm ω = 20% | 42.33 | 43.84 | 40.17 | 39.83 | 10.1 | 11.3 | 0.2457 | 2.1879 × 10−3 |
| Category | Elastic Modulus E (MPa) | Poisson’s Ratio v | Unit Weight γ (kN/m3) | Cohesion c (kPa) | Internal Friction Angle φ (°) |
|---|---|---|---|---|---|
| Rigid body | 2.1 × 105 | 0.3 | 78 | / | / |
| Soil mass | 3 | 0 | 8 | 12 | 20 |
| Category | G (MPa) | τr (kPa) | α (kPa) | m | n |
| Hardening | 23.13 | / | 15.45 | 0.4271 | 4.4132 × 10−3 |
| Softening | 74.89 | 40.13 | / | 0.2297 | 2.0891 × 10−3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, P.; Liu, H.; Geng, L.; Wang, S.; Yu, Y.; Zhu, C.; Yang, Q.; Liu, H.; Guan, Y. Softening/Hardening Damage Model and Numerical Implementation of Seabed Silt-Steel Interface in Yellow River Underwater Delta. J. Mar. Sci. Eng. 2023, 11, 1415. https://doi.org/10.3390/jmse11071415
Yu P, Liu H, Geng L, Wang S, Yu Y, Zhu C, Yang Q, Liu H, Guan Y. Softening/Hardening Damage Model and Numerical Implementation of Seabed Silt-Steel Interface in Yellow River Underwater Delta. Journal of Marine Science and Engineering. 2023; 11(7):1415. https://doi.org/10.3390/jmse11071415
Chicago/Turabian StyleYu, Peng, Honghua Liu, Lin Geng, Shuai Wang, Yang Yu, Chenghao Zhu, Qi Yang, Hongjun Liu, and Yong Guan. 2023. "Softening/Hardening Damage Model and Numerical Implementation of Seabed Silt-Steel Interface in Yellow River Underwater Delta" Journal of Marine Science and Engineering 11, no. 7: 1415. https://doi.org/10.3390/jmse11071415
APA StyleYu, P., Liu, H., Geng, L., Wang, S., Yu, Y., Zhu, C., Yang, Q., Liu, H., & Guan, Y. (2023). Softening/Hardening Damage Model and Numerical Implementation of Seabed Silt-Steel Interface in Yellow River Underwater Delta. Journal of Marine Science and Engineering, 11(7), 1415. https://doi.org/10.3390/jmse11071415






