An Unorthodox Arrangement of Boussinesq-Type Wave Equations for Accurate and Robust Numerical Treatment
Abstract
:1. Introduction
2. Governing Equations
2.1. Continuity Equation
2.2. Momentum Equation
2.3. Intermediate Velocity Variable
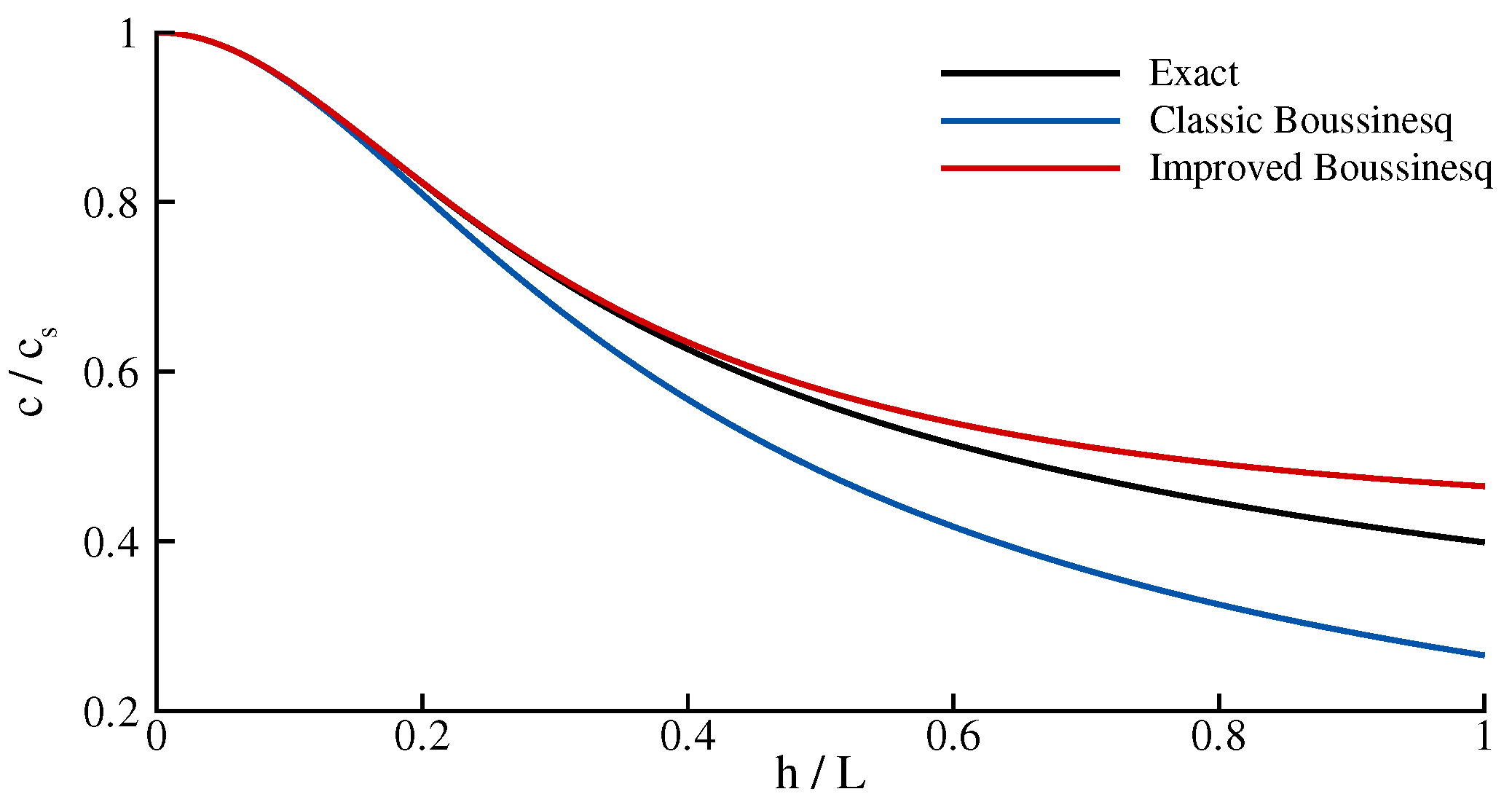
2.4. Linear Dispersion Relationship of Wave Model
3. Numerical Formulation
3.1. Finite Difference Discretization of Equations
3.2. Treatment of Boundaries
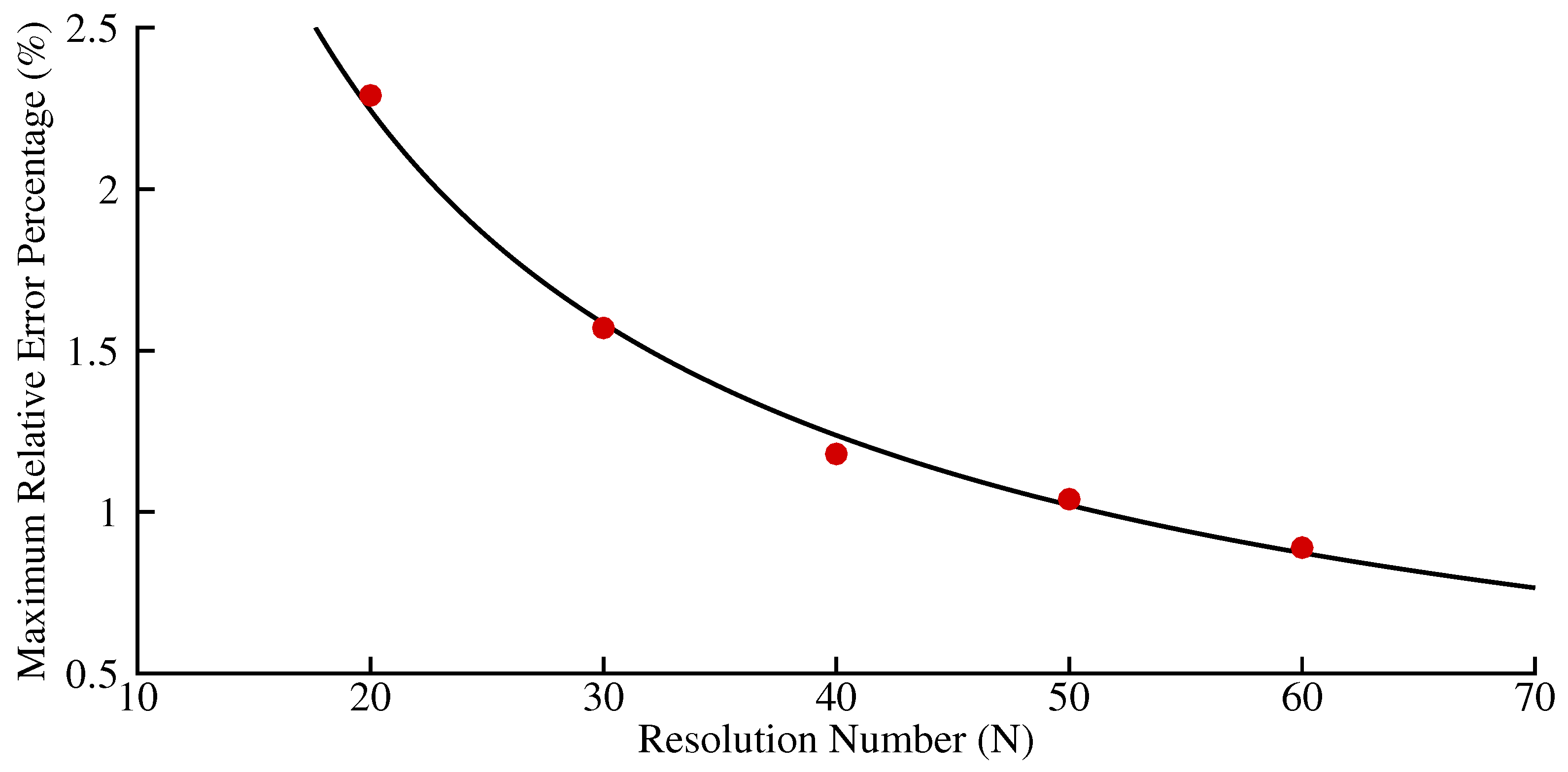
4. Simulations
4.1. Wave Propagation over Varying Depths
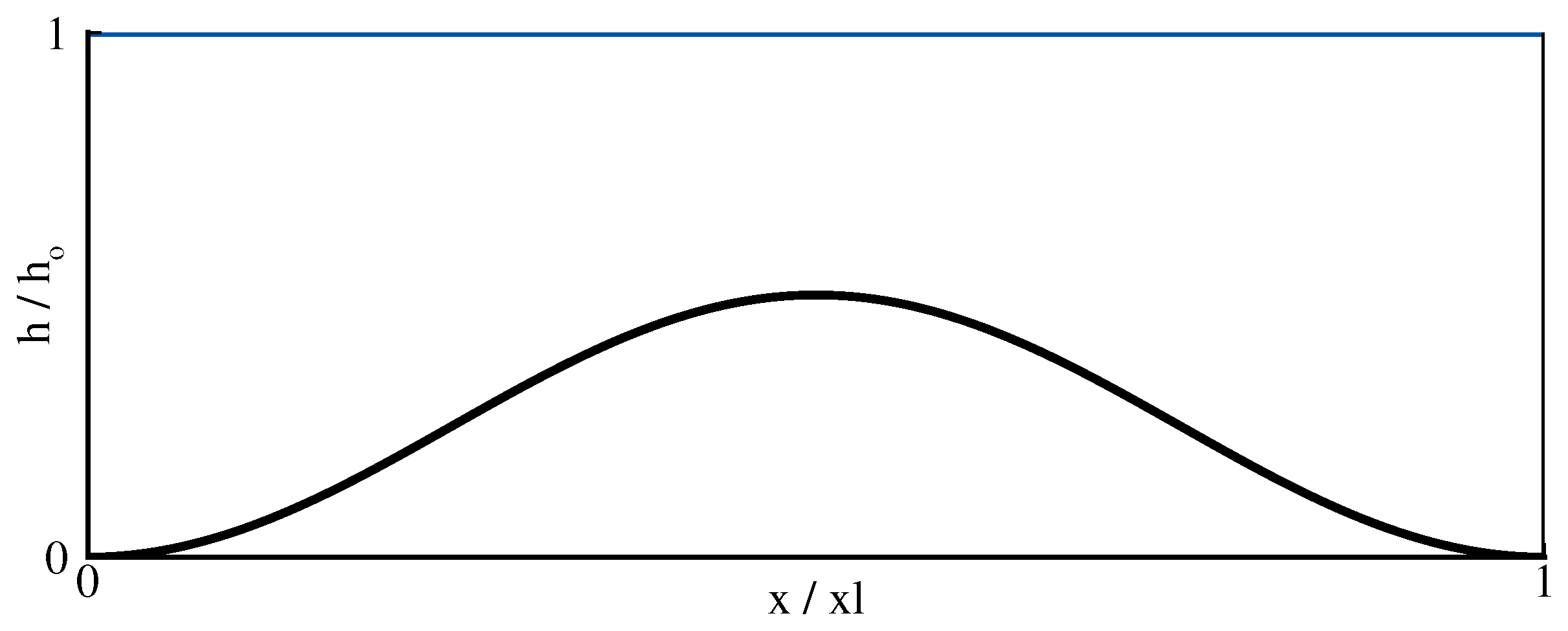
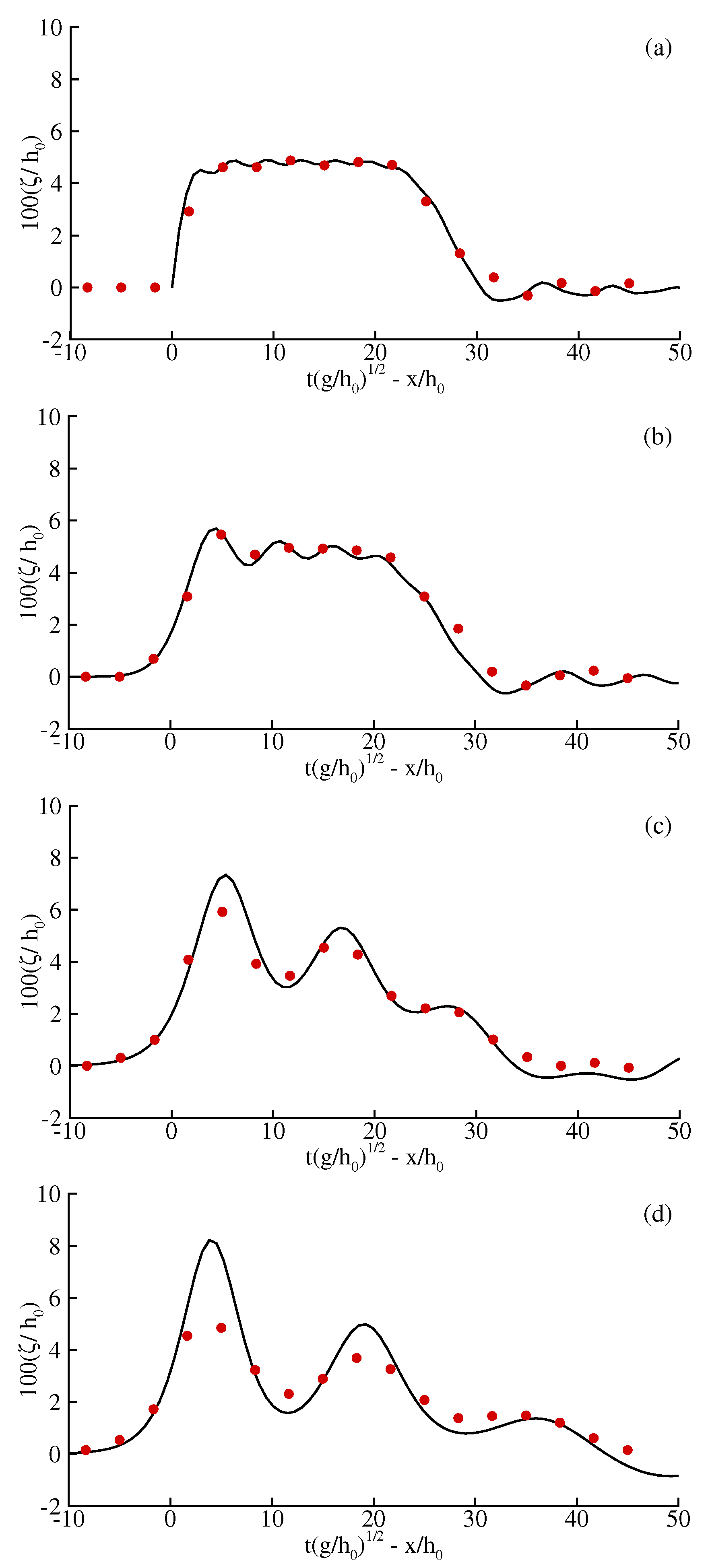
4.2. Waves Generated by Bottom Movement
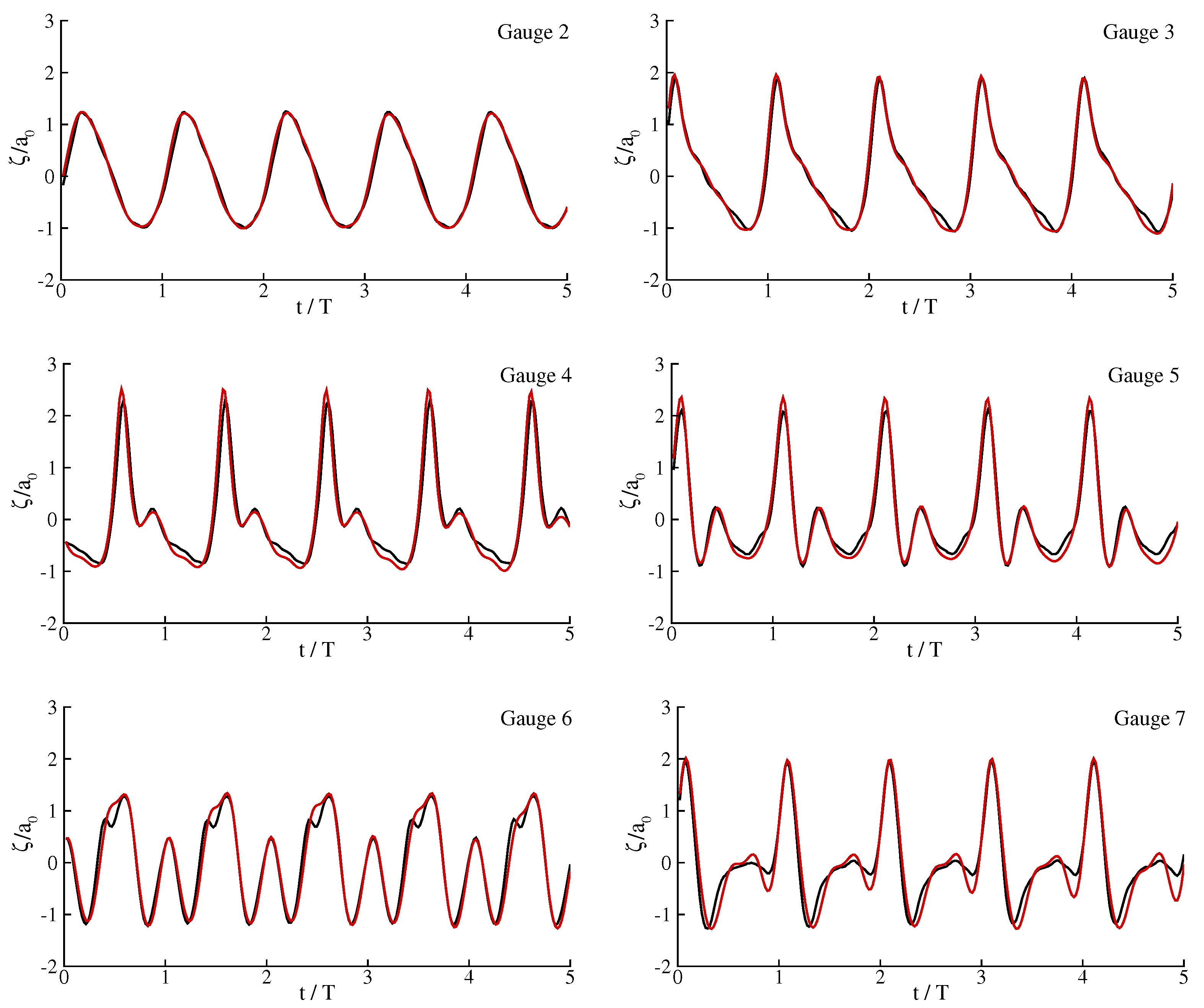
4.3. Nonlinear Wave Propagation over a Submerged Bar
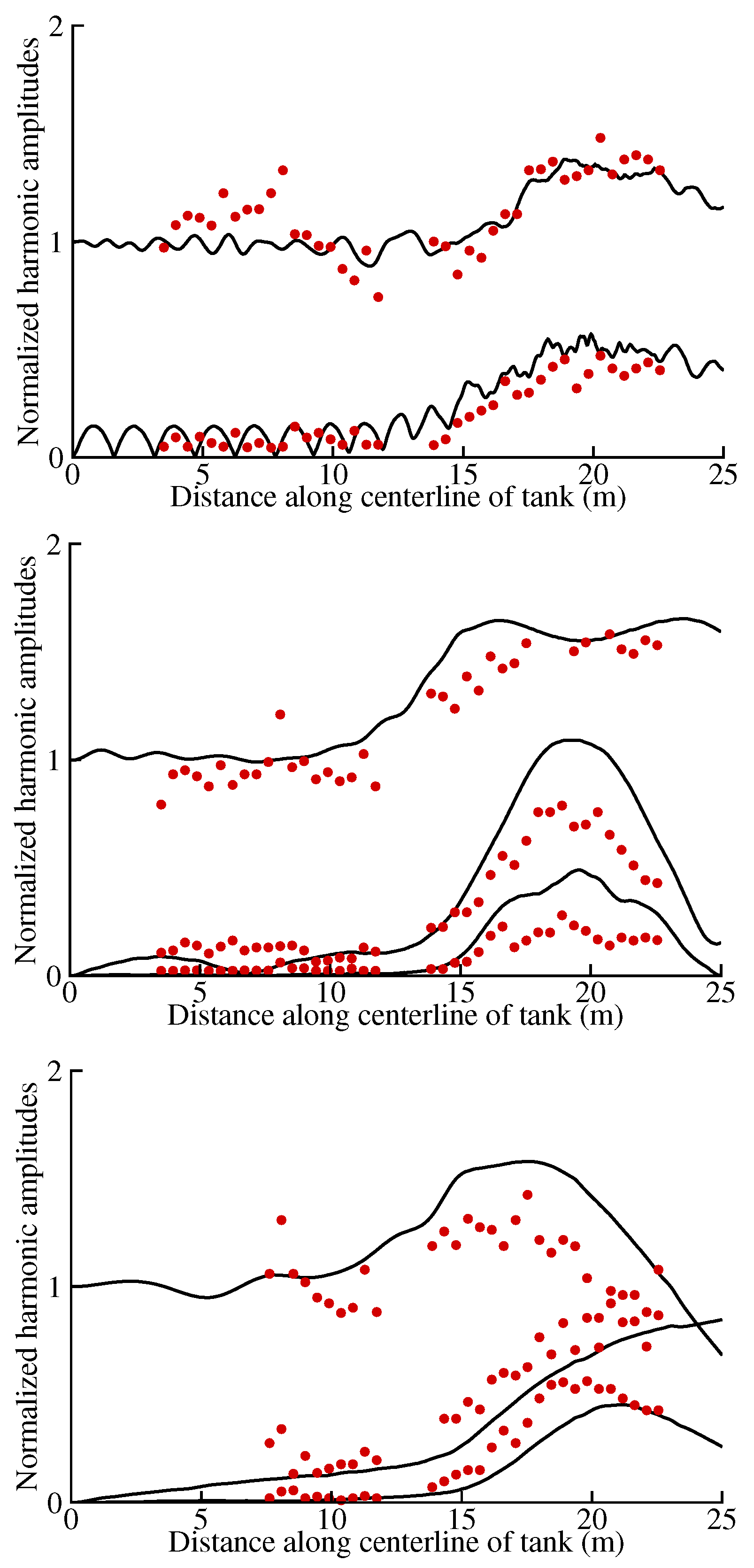
4.4. Nonlinear Wave Convergence over a Topographical Lens
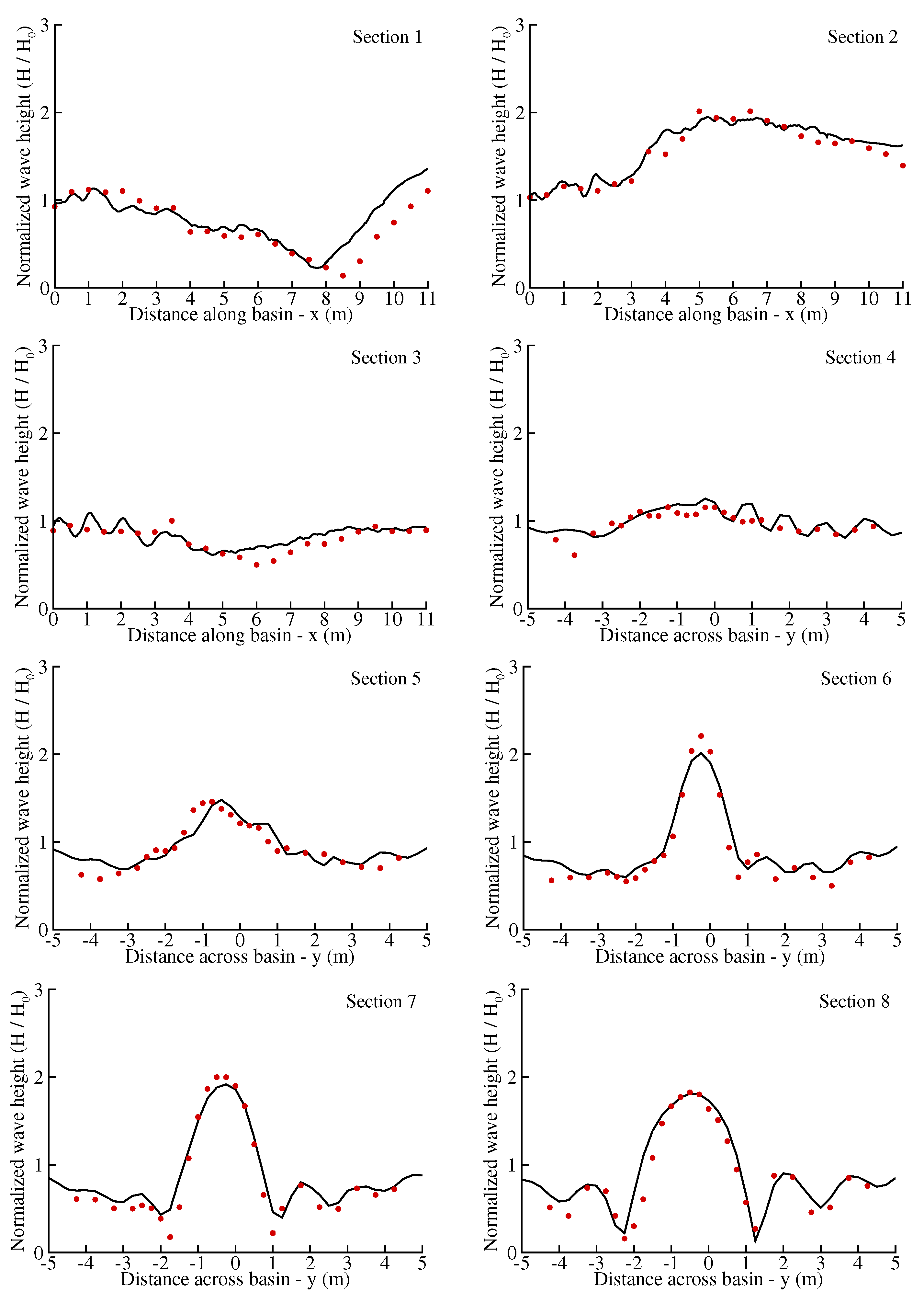
4.5. Combined Refraction–Diffraction over an Elliptic Shoal
5. Concluding Remarks
Funding
Data Availability Statement
Conflicts of Interest
References
- Witting, J.M. A unified model for the evolution of nonlinear water waves. J. Comput. Phys. 1984, 56, 203–236. [Google Scholar] [CrossRef]
- Madsen, P.A.; Murray, R.; Sørensen, O.R. A new form of the Boussinesq equations with improved linear dispersion characteristics. Coast. Eng. 1991, 15, 371–388. [Google Scholar] [CrossRef]
- Madsen, P.A.; Sørensen, O.R. A new form of the Boussinesq equations with improved linear dispersion characteristics: Part 2. A slowly-varying bathymetry. Coast. Eng. 1992, 18, 183–204. [Google Scholar] [CrossRef]
- Nwogu, O. Alternative form of Boussinesq equations for nearshore propagation. J. Waterw. Port Coast. Ocean Eng. 1993, 9, 618–638. [Google Scholar] [CrossRef]
- Beji, S.; Nadaoka, K. A formal derivation and numerical modelling of the improved Boussinesq equations for varying depth. Ocean Eng. 1996, 23, 691–704. [Google Scholar]
- Peregrine, D.H. Long waves on a beach. J. Fluid Mech. 1967, 27, 815–827. [Google Scholar] [CrossRef]
- Madsen, P.A.; Schäffer, H.A. Higher-order Boussinesq-type equations for surface gravity waves: Derivation and analysis. Phil. Trans. R. Soc. Lond. A 1998, 356, 3123–3184. [Google Scholar] [CrossRef]
- Karambas, T.V.; Memos, C.D. Boussinesq model for weakly nonlinear fully dispersive water waves. J. Waterw. Coast. Ocean Eng. 2009, 135, 187–199. [Google Scholar] [CrossRef]
- Memos, C.D.; Klonaris, G.T.; Chondros, M.K. On higher-order Boussinesq-type wave models. J. Waterw. Coast. Ocean Eng. 2016, 142, 04015011. [Google Scholar] [CrossRef]
- Klonaris, G.T.; Memos, C.D.; Drønen, N.K. High-order Boussinesq-type model for integrated nearshore dynamics. J. Waterw. Coast. Ocean Eng. 2016, 142, 04016010. [Google Scholar] [CrossRef]
- Walkley, M.; Berzins, M. A finite element method for the two-dimensional extended Boussinesq equations. Int. J. Numer. Methods Fluids 2002, 39, 865–885. [Google Scholar] [CrossRef]
- Zhan, J.M.; Li, Y.S.; Wai, O.W.H. An accurate finite difference scheme for Boussinesq equations. Int. J. Comput. Fluid Dyn. 2004, 18, 421–430. [Google Scholar] [CrossRef]
- Eskilsson, C.; Sherwin, S.J. Spectral/hp discontinuous Galerkin methods for modelling 2D Boussinesq equations. J. Comput. Phys. 2006, 212, 566–589. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhan, J.; Li, Y. High-order finite volume WENO schemes for Boussinesq modelling of nearshore wave processes. J. Hydraul. Res. 2016, 54, 646–662. [Google Scholar] [CrossRef]
- Bossi, U.; Engsig-Karup, A.P.; Eskilsson, C.; Ricchiuto, M. A spectral/hp element depth-integrated model for nonlinear wave-body interaction. Comput. Methods Appl. Mech. Eng. 2019, 348, 222–249. [Google Scholar] [CrossRef]
- Bayraktar, D.; Beji, S. Numerical simulation of waves generated by a moving pressure field. Ocean Eng. 2013, 59, 231–239. [Google Scholar] [CrossRef]
- David, C.G.; Roeber, V.; Goseberg, N.; Schlurmann, T. Generation and propagation of ship-borne waves -Solutions from a Boussinesq-type model. Coast. Eng. 2017, 127, 170–187. [Google Scholar] [CrossRef]
- Basco, D.R. A qualitative description of wave breaking. J. Waterw. Port Coast. Ocean Eng. 1985, 111, 171–187. [Google Scholar] [CrossRef]
- Kennedy, A.B.; Chen, Q.; Kirby, J.T.; Dalrymple, R.A. Boussinesq modeling of wave transformation, breaking and run-up. I: 1D. J. Waterw. Port Coast. Ocean Eng. 2000, 126, 39–47. [Google Scholar] [CrossRef]
- Chen, Q.; Kirby, J.T.; Dalrymple, R.A.; Kennedy, A.B.; Chawla, A. Boussinesq modeling of wave transformation, breaking and run-up. II: 2D. J. Waterw. Port Coast. Ocean Eng. 2000, 126, 48–56. [Google Scholar] [CrossRef]
- D’Alessandro, F.; Tomasicchio, G.R. The BCI criterion for the initiation of breaking process in Boussinesq type equations wave models. Coast. Eng. 2008, 55, 1174–1184. [Google Scholar] [CrossRef]
- Roeber, V.; Cheung, K.F.; Kobayashi, M.H. Shock-capturing Boussinesq-type model for nearshore wave processes. Coast. Eng. 2010, 57, 407–423. [Google Scholar] [CrossRef]
- Tissier, M.; Bonneton, P.; Marche, F.; Chazel, F.; Lannes, D. A new approach to handle wave breaking in fully non-linear Boussinesq models. Coast. Eng. 2012, 67, 54–66. [Google Scholar]
- Roeber, V.; Cheung, K.F. Boussinesq-type model for energetic breaking waves in fringing reef environments. Coast. Eng. 2012, 70, 1–20. [Google Scholar] [CrossRef]
- Fang, K.Z.; Liu, Z.B.; Zou, Z.L. Fully nonlinear modeling wave transformation over fringing reefs using shock-capturing Boussinesq model. J. Coast. Res. 2016, 32, 164–171. [Google Scholar] [CrossRef]
- Kaur, S.; Kumar, P.; Rajni. Non-linear periodic long waves based on Boussinesq equation for shallow water waves: A coupled FEM modeling. Ocean Eng. 2022, 245, 110469. [Google Scholar] [CrossRef]
- Watanabe, M.; Kan, H.; Toguchi, K.; Nakashima, Y.; Roeber, V.; Arikawa, T. Effect of the structural complexity of a coral reef on wave propagation: A case study from Komaka Island, Japan. Ocean Eng. 2023, 287, 115632. [Google Scholar]
- Beji, S. Improved Boussinesq-type equations for spatially and temporally varying bottom. Coast. Eng. J. 2018, 60, 318–326. [Google Scholar] [CrossRef]
- Beji, S. Note on conservation equations for nonlinear surface waves. Ocean Eng. 1998, 25, 607–613. [Google Scholar]
- Nadaoka, K.; Beji, S.; Nakagawa, Y. A fully dispersive and weakly nonlinear model for water waves. Proc. R. Soc. Lond. A 1997, 453, 303–319. [Google Scholar] [CrossRef]
- Abbott, M.B. Computational Hydraulics: Elements of the Theory of Free Surface Flows; Pitman: London, UK, 1979. [Google Scholar]
- Mei, C.C. The Applied Dynamics of Ocean Surface Waves; World Scientific: Singapore, 1989. [Google Scholar]
- Baker, G.S., Jr.; Graves-Morris, P. Padé Approximants; Cambridge University Press: Cambridge, UK, 1980. [Google Scholar]
- Bonnefoy, F.; Haudin, F.; Michel, G.; Semin, B.; Humbert, T.; Aumaître, S.; Berhanu, M.; Falcon, E. Observation of resonant interactions among surface gravity waves. J. Fluid Mech. 2016, 805, R3. [Google Scholar] [CrossRef]
- Stiassnie, M. On the strength of the weakly nonlinear theory for surface gravity waves. J. Fluid Mech. 2017, 810, 1–4. [Google Scholar] [CrossRef]
- Kantha, L.H.; Clayson, C.A. Numerical Models of Oceans and Oceanic Processes; Academic Press: San Diego, CA, USA, 2000. [Google Scholar]
- Simarro, G. Energy balance, wave shoaling and group celerity in Boussinesq-type wave propagation models. Ocean Model. 2013, 72, 74–79. [Google Scholar] [CrossRef]
- Simarro, G.; Orfila, A.; Galan, A. Linear shoaling in Boussinesq-type wave propagation models. Coast. Eng. 2013, 80, 100–106. [Google Scholar] [CrossRef]
- Hammack, J.L. A note on tsunamis: Their generation and propagation in an ocean of uniform depth. J. Fluid Mech. 1973, 60, 769–799. [Google Scholar] [CrossRef]
- Beji, S.; Battjes, J.A. Experimental investigation of nonlinear wave propagation over a bar. Coast. Eng. 1993, 19, 151–162. [Google Scholar] [CrossRef]
- Beji, S.; Battjes, J.A. Numerical simulation of nonlinear wave propagation over a bar. Coast. Eng. 1994, 23, 1–16. [Google Scholar] [CrossRef]
- Whalin, R.W. The Limit of Applicability of Linear Wave Refraction Theory in a Convergence Zone; Research Report H-71-3; U.S. Army Corps of Engineers, The Waterways Experiment Station: Vicksburg, MS, USA, 1971. [Google Scholar]
- Berkhoff, J.C.W.; Booy, N.; Radder, A.C. Verification of numerical wave propagation models for simple harmonic linear water waves. Coast. Eng. 1982, 6, 255–279. [Google Scholar] [CrossRef]
- Beji, S.; Nadaoka, K. Fully dispersive nonlinear water wave model in curvilinear coordinates. J. Comput. Phys. 2004, 198, 645–658. [Google Scholar] [CrossRef]
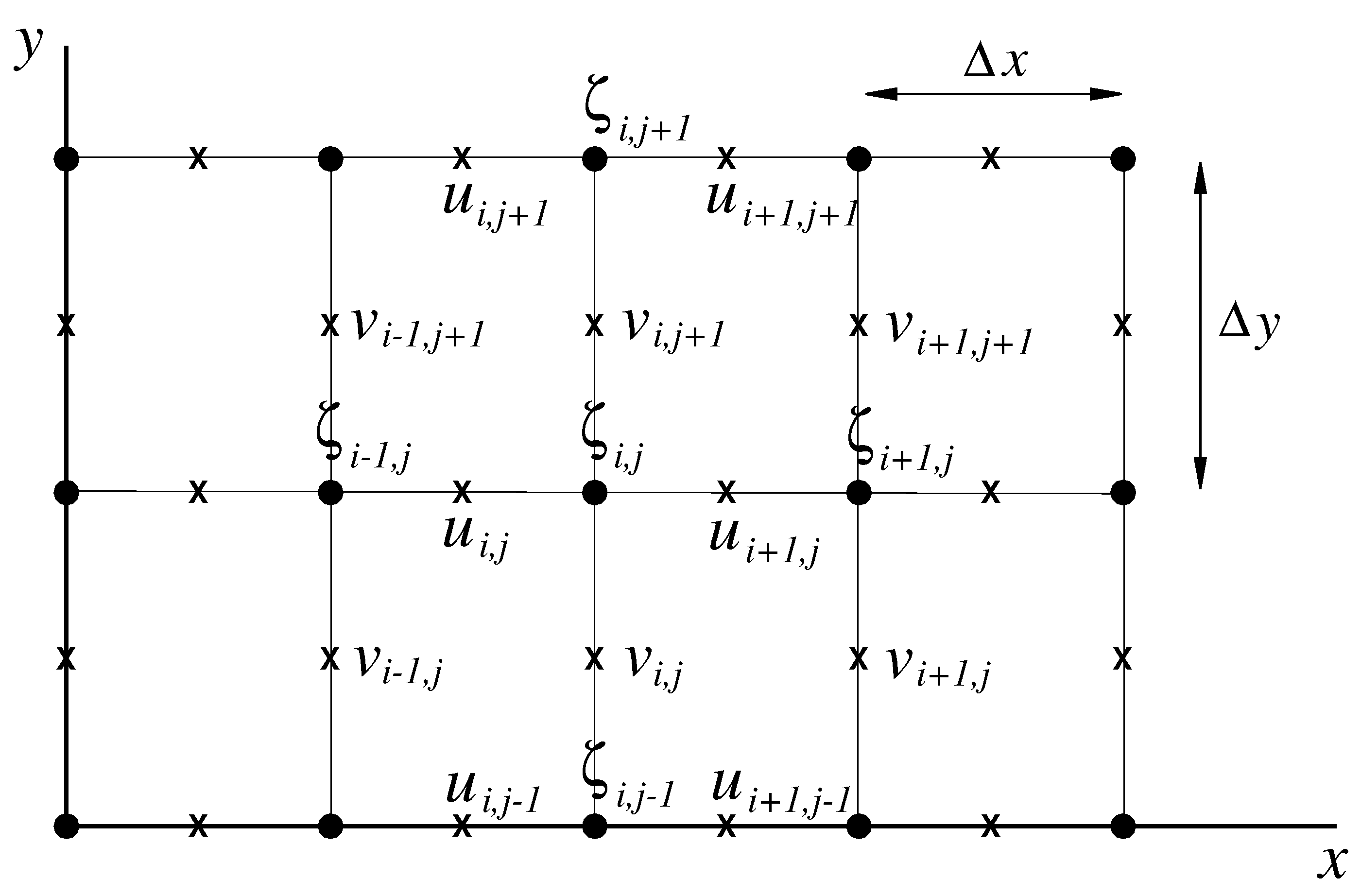

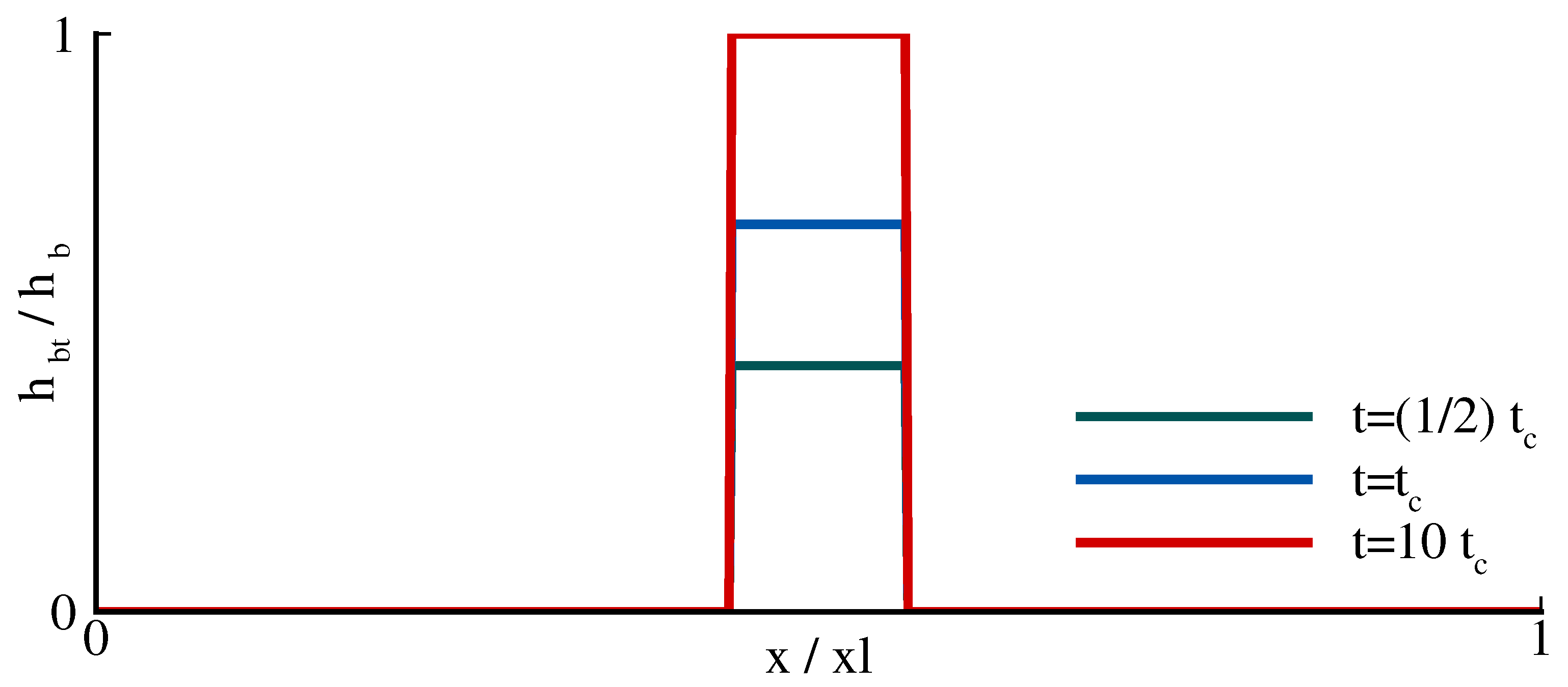


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Beji, S. An Unorthodox Arrangement of Boussinesq-Type Wave Equations for Accurate and Robust Numerical Treatment. J. Mar. Sci. Eng. 2023, 11, 1936. https://doi.org/10.3390/jmse11101936
Beji S. An Unorthodox Arrangement of Boussinesq-Type Wave Equations for Accurate and Robust Numerical Treatment. Journal of Marine Science and Engineering. 2023; 11(10):1936. https://doi.org/10.3390/jmse11101936
Chicago/Turabian StyleBeji, Serdar. 2023. "An Unorthodox Arrangement of Boussinesq-Type Wave Equations for Accurate and Robust Numerical Treatment" Journal of Marine Science and Engineering 11, no. 10: 1936. https://doi.org/10.3390/jmse11101936
APA StyleBeji, S. (2023). An Unorthodox Arrangement of Boussinesq-Type Wave Equations for Accurate and Robust Numerical Treatment. Journal of Marine Science and Engineering, 11(10), 1936. https://doi.org/10.3390/jmse11101936





