Study on the Resistance of a Large Pure Car Truck Carrier with Bulbous Bow and Transom Stern
Abstract
:1. Introduction
2. Model and Methods
2.1. Geometric Model
2.2. Ship Resistance
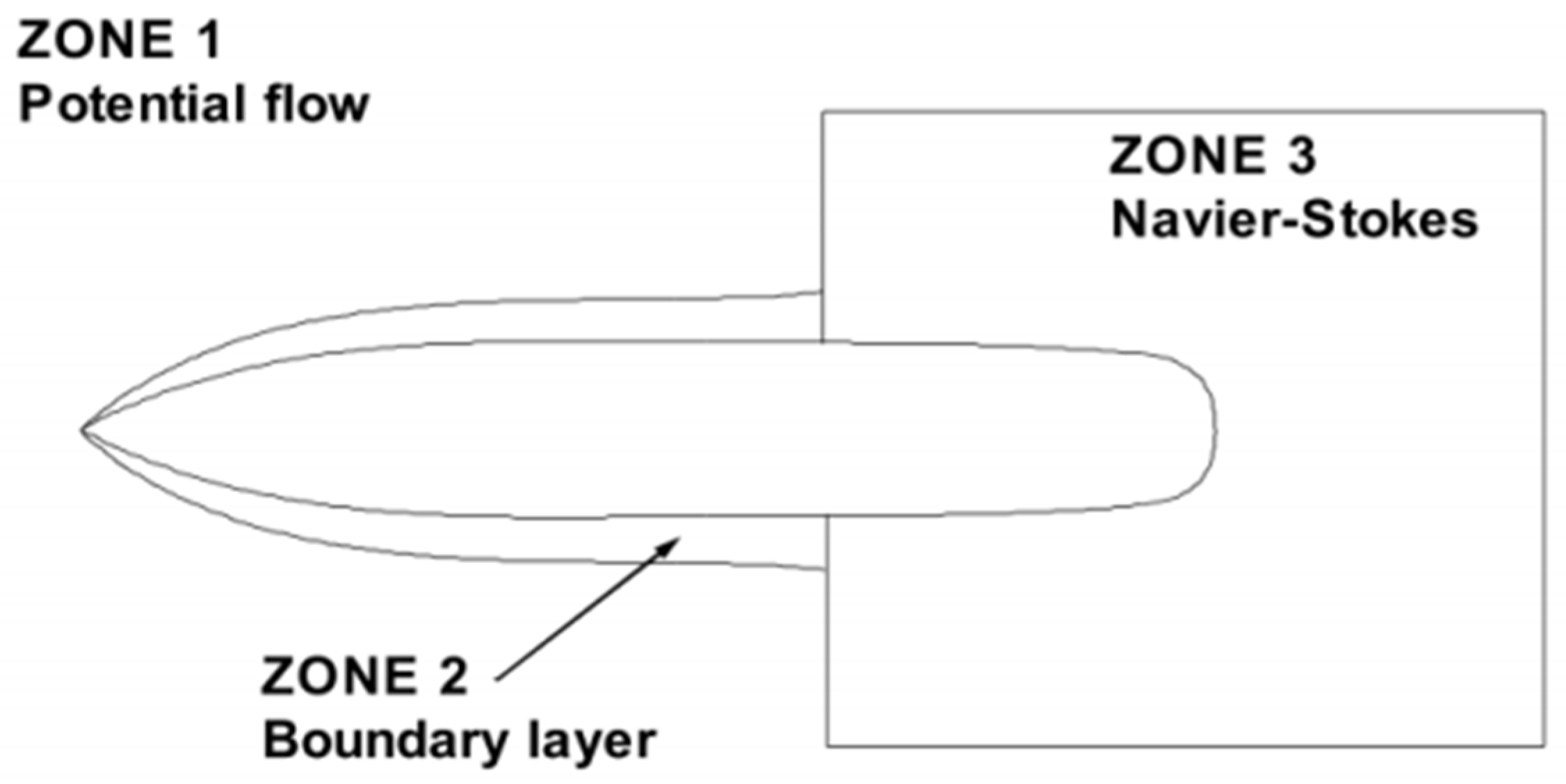
2.3. SHIPFLOW Solver
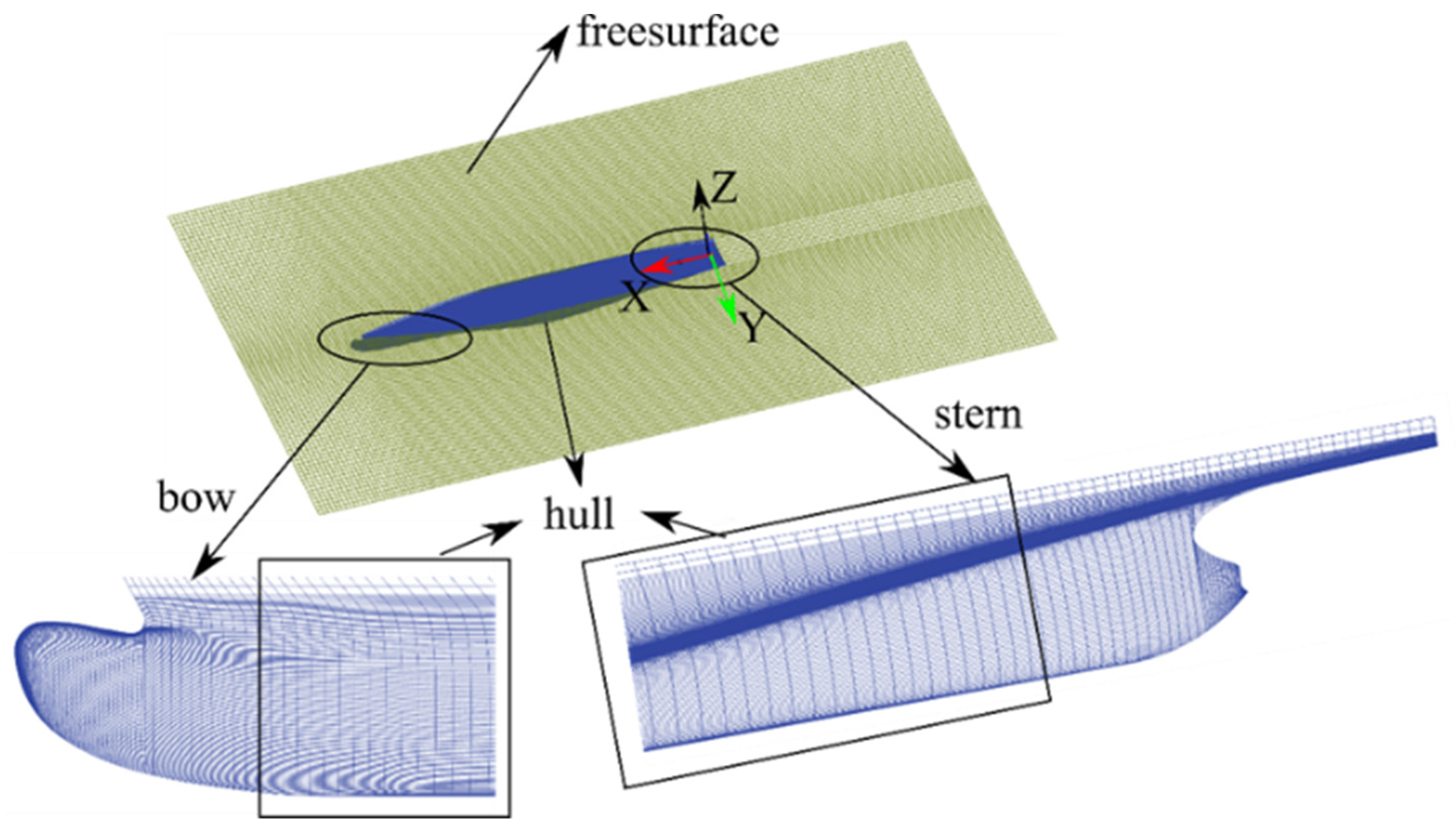
2.4. Grid Generation
2.5. Boundary Conditions
2.6. Experimental Measurements
3. Results and Discussion
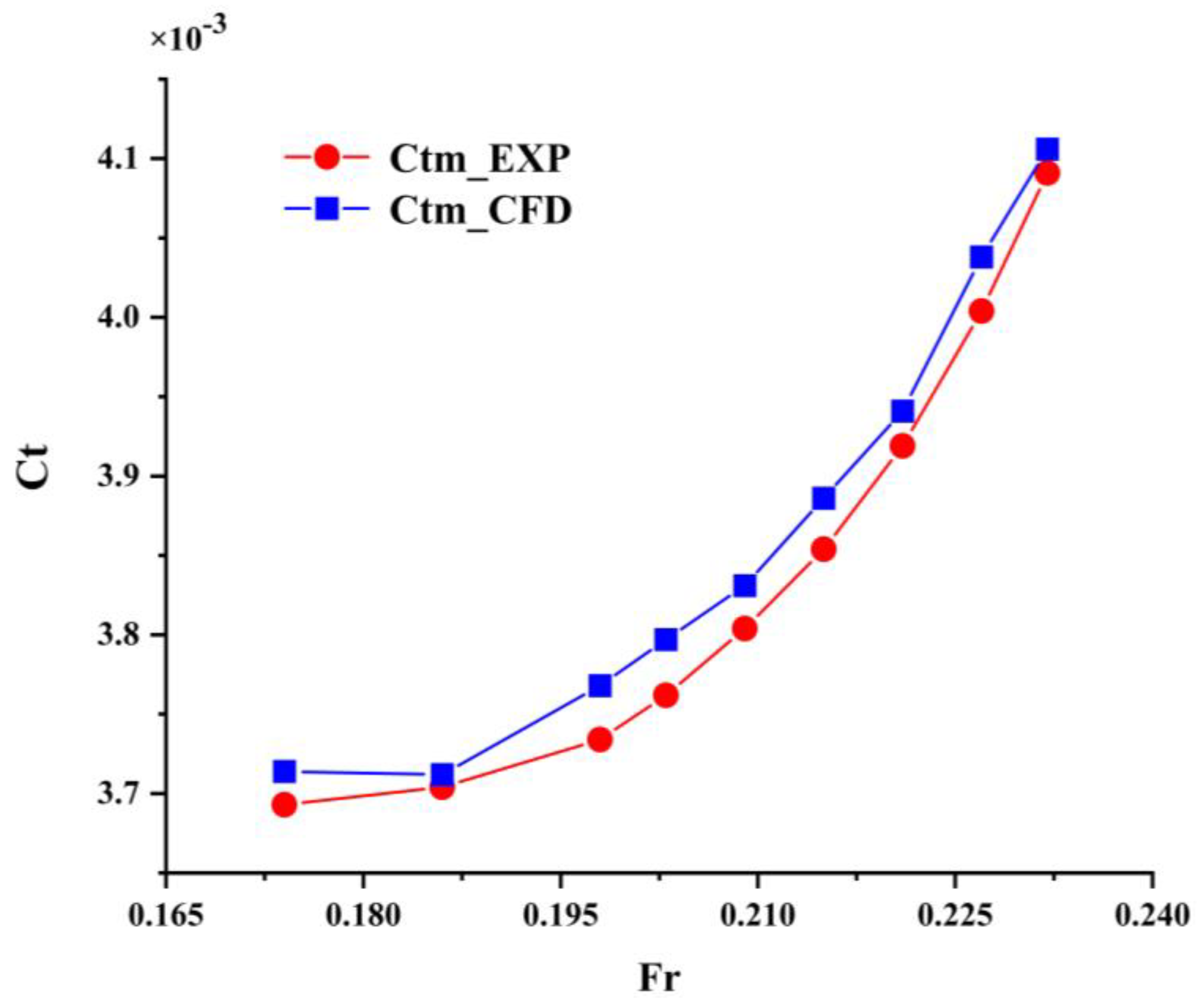
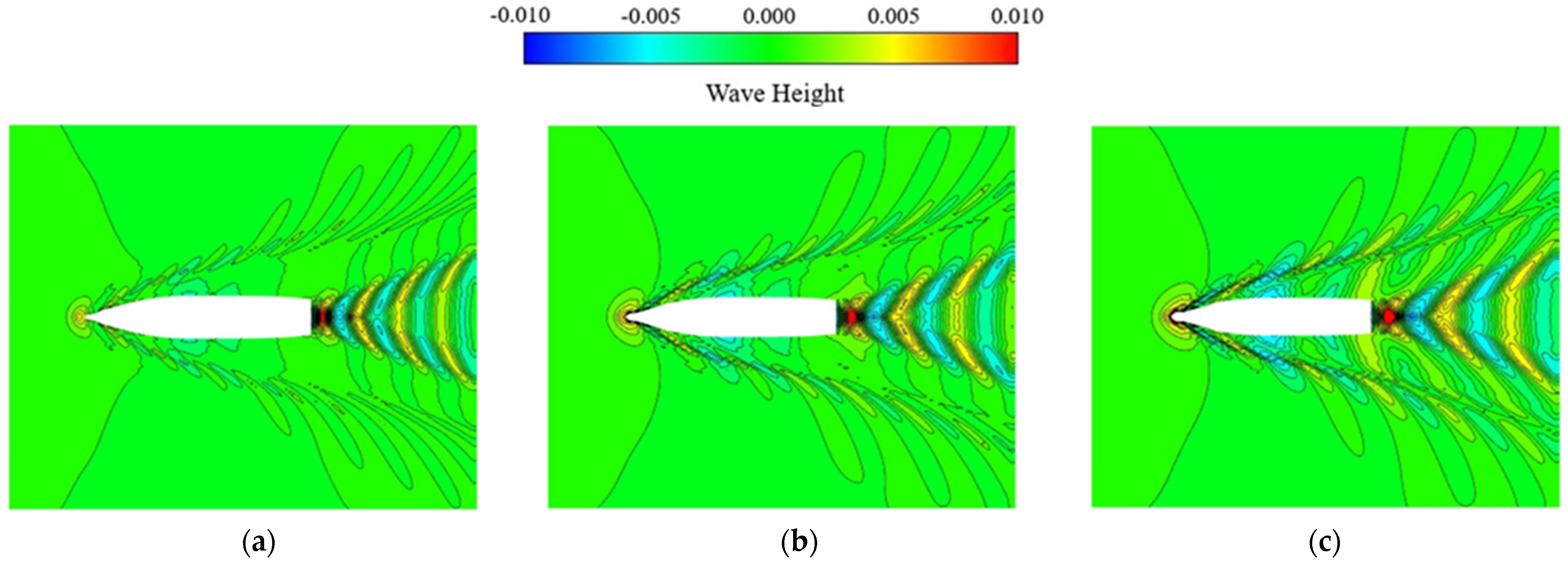
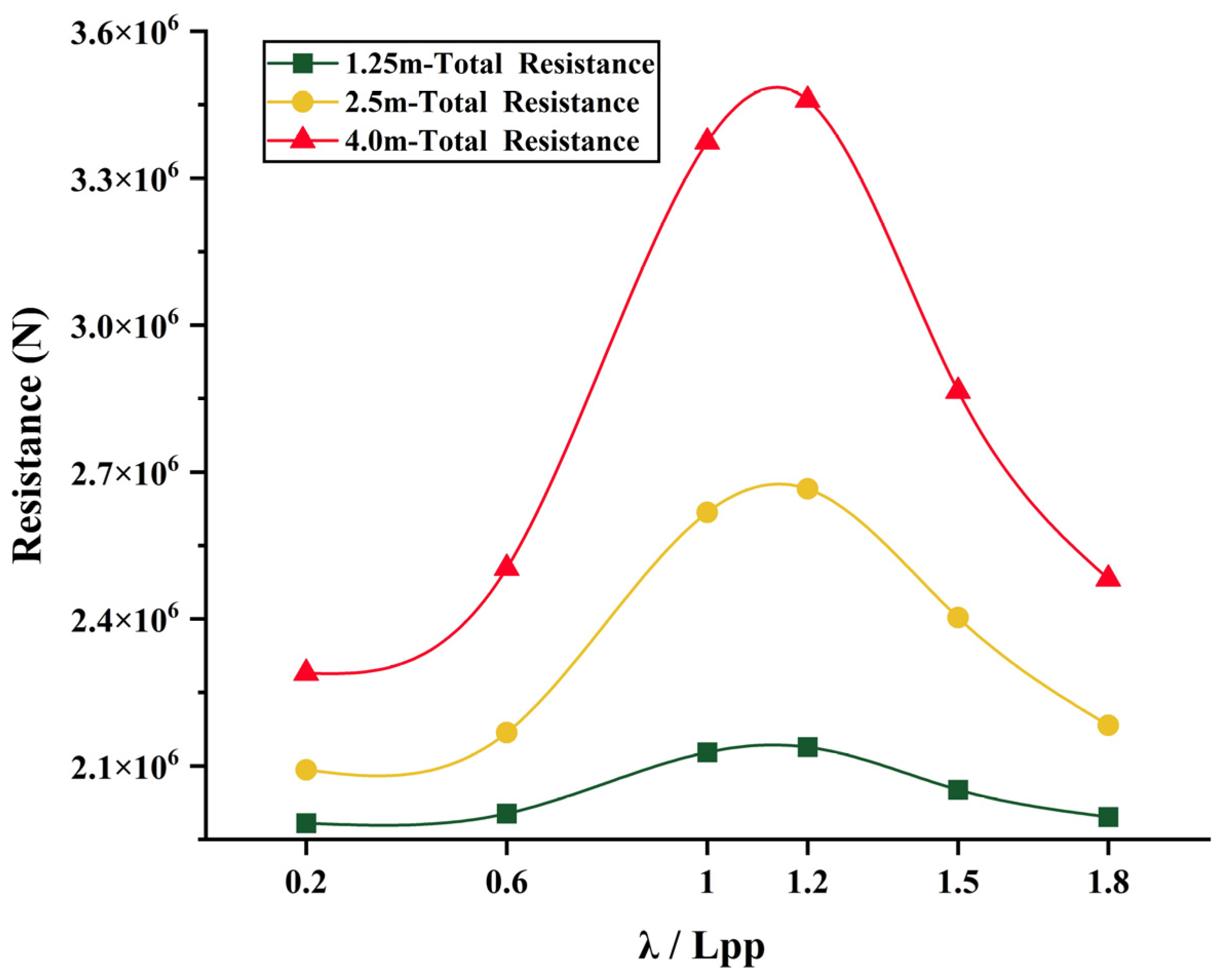
3.1. Total Resistance in Calm Water
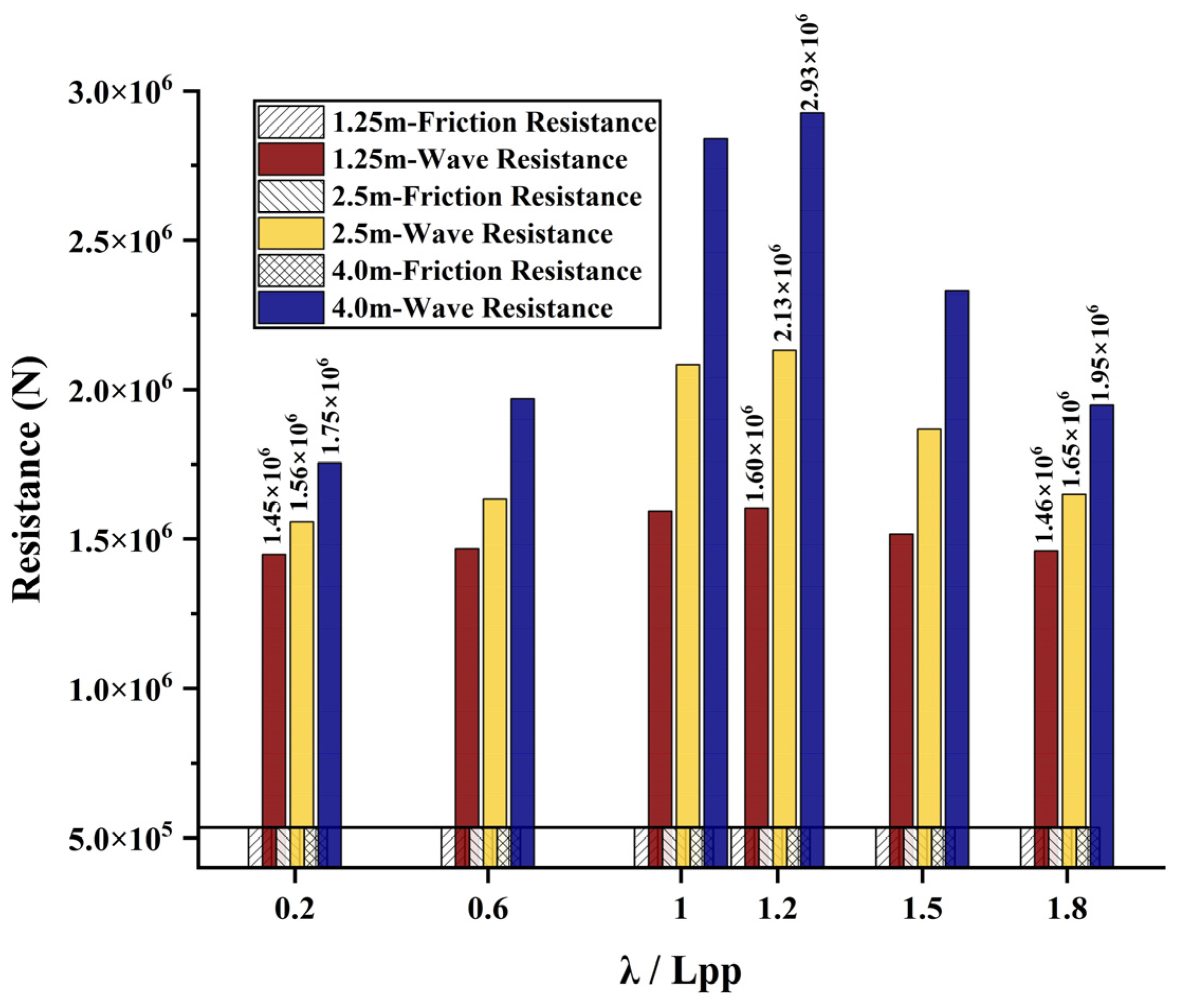
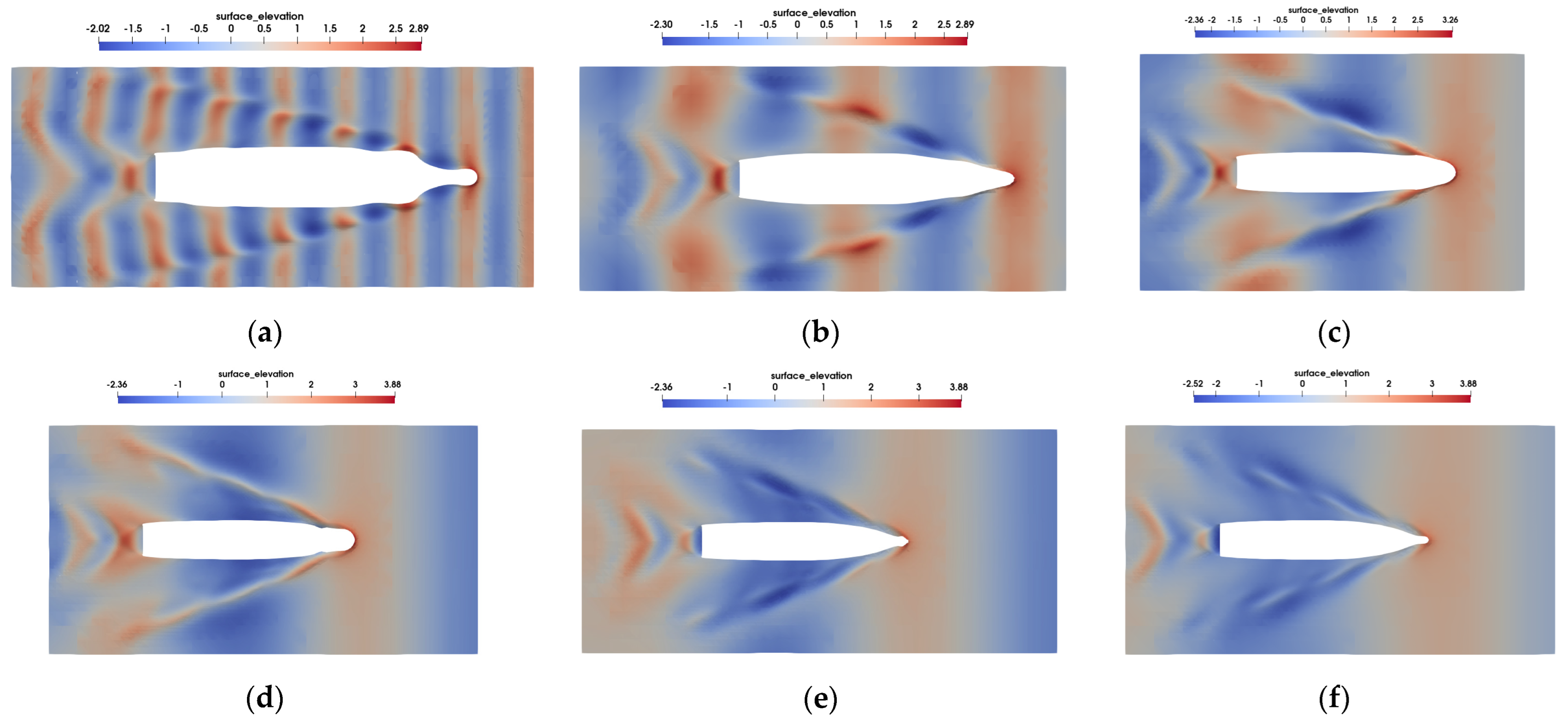
3.2. Ship Resistance under Regular Waves
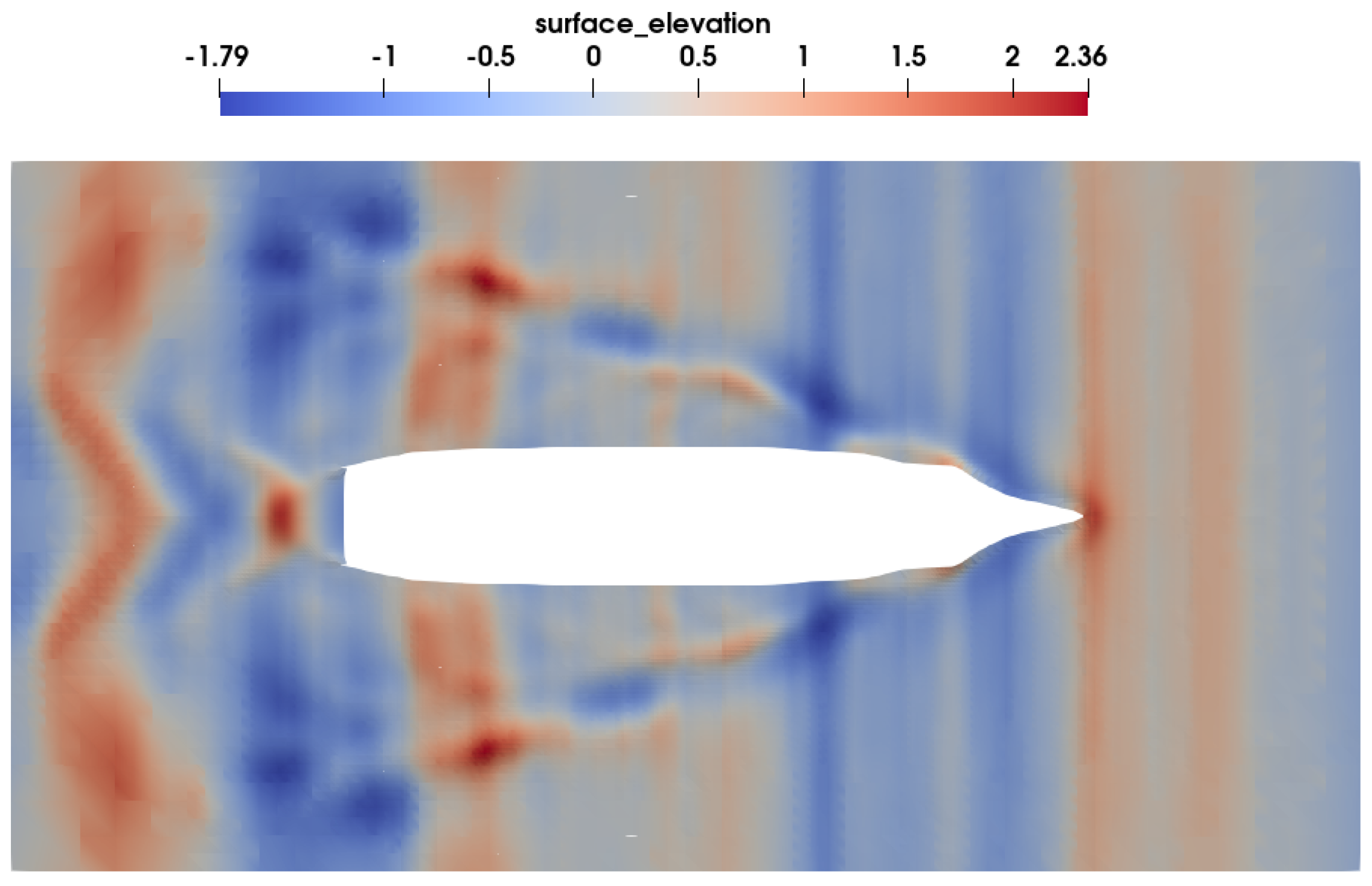
3.3. Ship Resistance in Irregular Waves under Three Different Sea States
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Park, D.M.; Lee, J.H.; Jung, Y.W.; Lee, J.; Kim, Y. Experimental and numerical studies on added resistance of ship in oblique sea conditions. Ocean Eng. 2019, 186, 106070. [Google Scholar] [CrossRef]
- Subbaiah, B.V.; Thampi, S.G.; Mustafa, V. Modelling and CFD analysis of traditional snake Boats of Kera-la. Aquat. Procedia 2015, 4, 481–491. [Google Scholar] [CrossRef]
- Ahmed, Y.M. Numerical simulation for the free surface flow around a complex ship hull form at different Froude Numbers. Alex. Eng. J. 2011, 50, 229–235. [Google Scholar] [CrossRef]
- Yu, J. Research on Calculation and Prediction for Ship Resistance Based on CFD Theory. Master’s Thesis, Shanghai Jiao Tong University, Shanghai, China, 2009. (In Chinese). [Google Scholar]
- Ahmed, Y.M. Viscous Resistance Prediction of a Tourist Submarine at Various Speeds. Int. Rev. Mech. Eng. IREME 2016, 10, 247–252. [Google Scholar] [CrossRef]
- Sun, J.; Tu, H.; Chen, Y.; Xie, D.; Zhou, J. A Study on Trim Optimization for a Container Ship Based on Effects due to Resistance. J. Ship Res. 2016, 60, 30–47. [Google Scholar] [CrossRef]
- Le, T.-H.; Vu, M.T.; Bich, V.N.; Phuong, N.K.; Ha, N.T.H.; Chuan, T.Q.; Tu, T.N. Numerical investigation on the effect of trim on ship resistance by RANSE method. Appl. Ocean Res. 2021, 111, 102642. [Google Scholar] [CrossRef]
- Dogrul, A.; Song, S.; Demirel, Y.K. Scale effect on ship resistance components and form factor. Ocean Eng. 2020, 209, 107428. [Google Scholar] [CrossRef]
- Abdelkhalek, H.; Han, D.F.; Gao, L.T.; Wang, Q. Numerical estimation of ship resistance using CFD with different turbulence model. Adv. Mater. Res. 2014, 1021, 209–213. [Google Scholar] [CrossRef]
- Peng, H. Numerical Computation of Multi-Hull Ship Resistance and Motion. Ph.D. Thesis, Dalhousie University, Halifax, Nova Scotia, 2001. [Google Scholar]
- Saha, G.K.; Miazee, M.A. Numerical and experimental study of resistance, sinkage and trim of a container ship. Procedia Eng. 2017, 194, 67–73. [Google Scholar] [CrossRef]
- Campbell, R.; Terziev, M.; Tezdogan, T.; Incecik, A. Computational fluid dynamics predictions of draught and trim variations on ship resistance in confined waters. Appl. Ocean Res. 2022, 126, 103301. [Google Scholar] [CrossRef]
- Feng, D.; Ye, B.; Zhang, Z.; Wang, X. Numerical Simulation of the Ship Resistance of KCS in Different Water Depths for Model-Scale and Full-Scale. J. Mar. Sci. Eng. 2020, 8, 745. [Google Scholar] [CrossRef]
- Ao, Y.; Li, Y.; Gong, J.; Li, S. An artificial intelligence-aided design (AIAD) of ship hull structures. J. Ocean Eng. Sci. 2021, 8, 15–32. [Google Scholar] [CrossRef]
- Yang, Y.; Tu, H.; Song, L.; Chen, L.; Xie, D.; Sun, J. Research on accurate prediction of the container ship resistance by RBFNN and other machine learning algorithms. J. Mar. Sci. Eng. 2021, 9, 376. [Google Scholar] [CrossRef]
- Cepowski, T. The use of a set of artificial neural networks to predict added resistance in head waves at the parametric ship design stage. Ocean Eng. 2023, 281, 114744. [Google Scholar] [CrossRef]
- SHIPFLOW; V6.02; Hangzhou Dianzi University: Hangzhou, China, 2016.
- Janson, C. Potential Flow Pane Methods for the Calculation of Free Surface Flows with Lift. Ph.D. Thesis, Chalmers University of Technology, Gothenburg, Sweden, 1997. [Google Scholar]
- Irannezhad, M.; Eslamdoost, A.; Kjellberg, M.; Bensow, R.E. Investigation of ship responses in regular head waves through a Fully Nonlinear Potential Flow approach. Ocean Eng. 2022, 246, 110410. [Google Scholar] [CrossRef]
- Dawson, C.W. A practical computer method for solving ship-wave problems. In Proceedings of the Second International Conference on Numerical Ship Hydrodynamics, Washington, DC, USA, 24–27 September 1977; pp. 30–38. [Google Scholar]
- Report of Tank Test; Vienna Model Basin Ltd.: Vienna, Austria, 2015; Volume 10.
- Miloh, T.; Landweber, L. Generalization of the Kelvin-Kirchhoff equations for the motion of a body through a fluid. Phys. Fluids 1981, 24, 6–9. [Google Scholar] [CrossRef]
- Ershkov, S.V.; Christianto, V.; Shamin, R.V.; Giniyatullin, A.R. About analytical ansatz to the solving procedure for Kelvin-Kirchhoff equations. Eur. J. Mech. B. Fluids 2020, 79, 87–91. [Google Scholar] [CrossRef]


| Items | Ship | Model |
|---|---|---|
| Length between perpendiculars, (m) | 187.25 | 6.87 |
| Breadth, B (m) | 36.45 | 1.34 |
| Draught, T (m) | 9.5 | 0.35 |
| Wetted surface, S (m2) | 7816 | 10.51 |
| Scale (λ) | 27.27 | 1 |
| Model basin dimensions | 180 m × 10 m × 5 m |
| Density of tank water | 1000 kg/m3 |
| Temp. of the tank water | 14.3 °C |
| Viscosity of tank water at 14.3 °C | 1.16030 × 10−6 m2/s |
| Material of model hull | Wood |
| Turbulence stimulation | Carborundum stripes |
| Instrumentation | Electronic dynamometer, load cell, ultrasonic probes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, X.; Xie, T.; Liu, Z.; Lai, X.; Pan, H.; Wang, C.; Leng, J.; Rahman, M.M. Study on the Resistance of a Large Pure Car Truck Carrier with Bulbous Bow and Transom Stern. J. Mar. Sci. Eng. 2023, 11, 1932. https://doi.org/10.3390/jmse11101932
Tian X, Xie T, Liu Z, Lai X, Pan H, Wang C, Leng J, Rahman MM. Study on the Resistance of a Large Pure Car Truck Carrier with Bulbous Bow and Transom Stern. Journal of Marine Science and Engineering. 2023; 11(10):1932. https://doi.org/10.3390/jmse11101932
Chicago/Turabian StyleTian, Xiaoqing, Tianwei Xie, Zhangming Liu, Xianghua Lai, Huachen Pan, Chizhong Wang, Jianxing Leng, and M. M. Rahman. 2023. "Study on the Resistance of a Large Pure Car Truck Carrier with Bulbous Bow and Transom Stern" Journal of Marine Science and Engineering 11, no. 10: 1932. https://doi.org/10.3390/jmse11101932
APA StyleTian, X., Xie, T., Liu, Z., Lai, X., Pan, H., Wang, C., Leng, J., & Rahman, M. M. (2023). Study on the Resistance of a Large Pure Car Truck Carrier with Bulbous Bow and Transom Stern. Journal of Marine Science and Engineering, 11(10), 1932. https://doi.org/10.3390/jmse11101932








