Multi-Scale Influence of Flexible Submerged Aquatic Vegetation (SAV) on Estuarine Hydrodynamics
Abstract
1. Introduction
1.1. Estuarine Hydrodynamics
1.2. Vegetation-Induced Bottom Friction
2. Methods
2.1. Study Site—Coos Bay Estuary

2.2. Numerical Model
2.3. Boundary Conditions
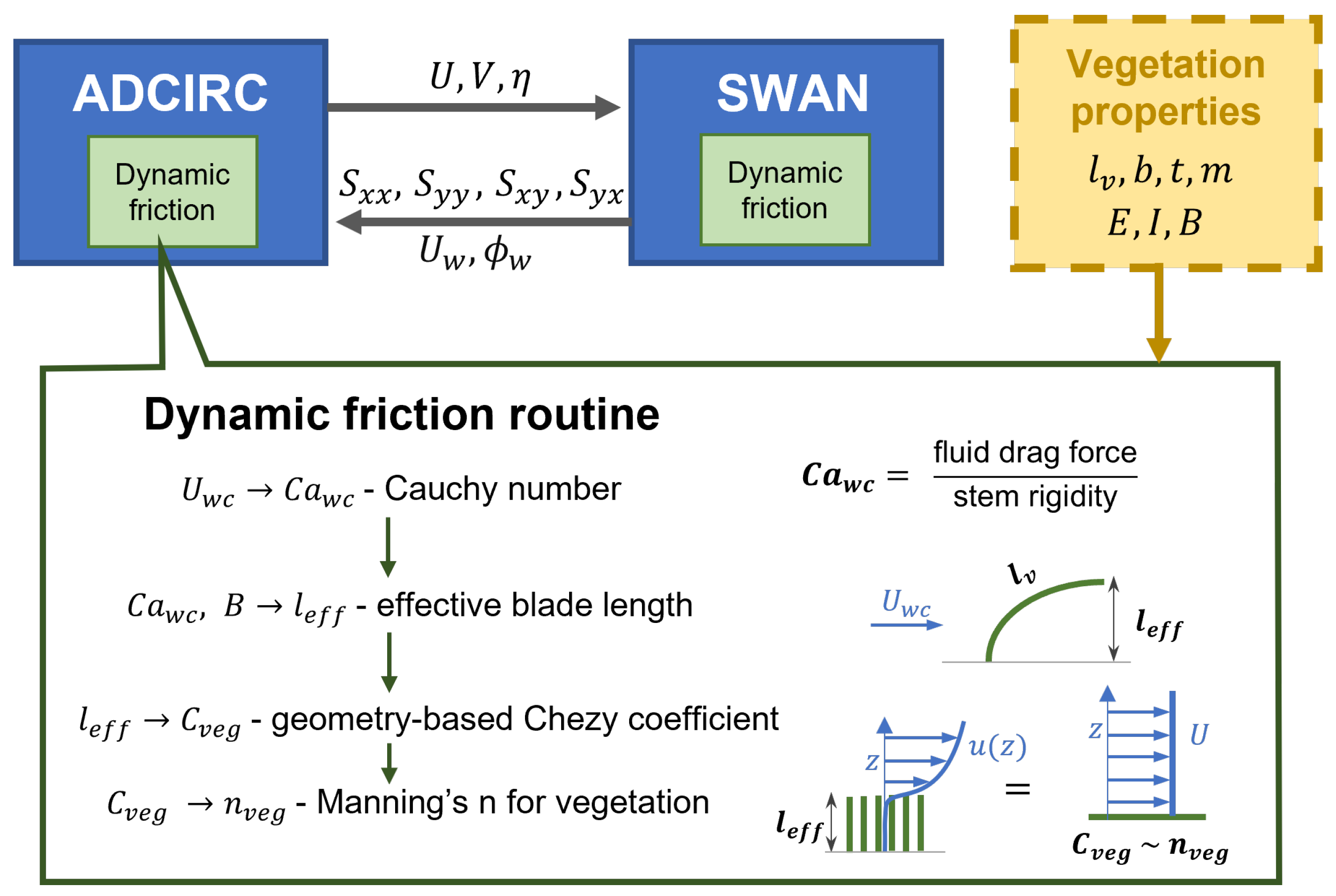
2.4. Dynamic Bottom Friction
2.5. Experimental Design
2.6. Analysis
2.6.1. Depth-Averaged Hydrodynamics
2.6.2. Sediment Fluxes
2.6.3. Circulation
2.6.4. Tidal Velocity and Duration Asymmetry
3. Results
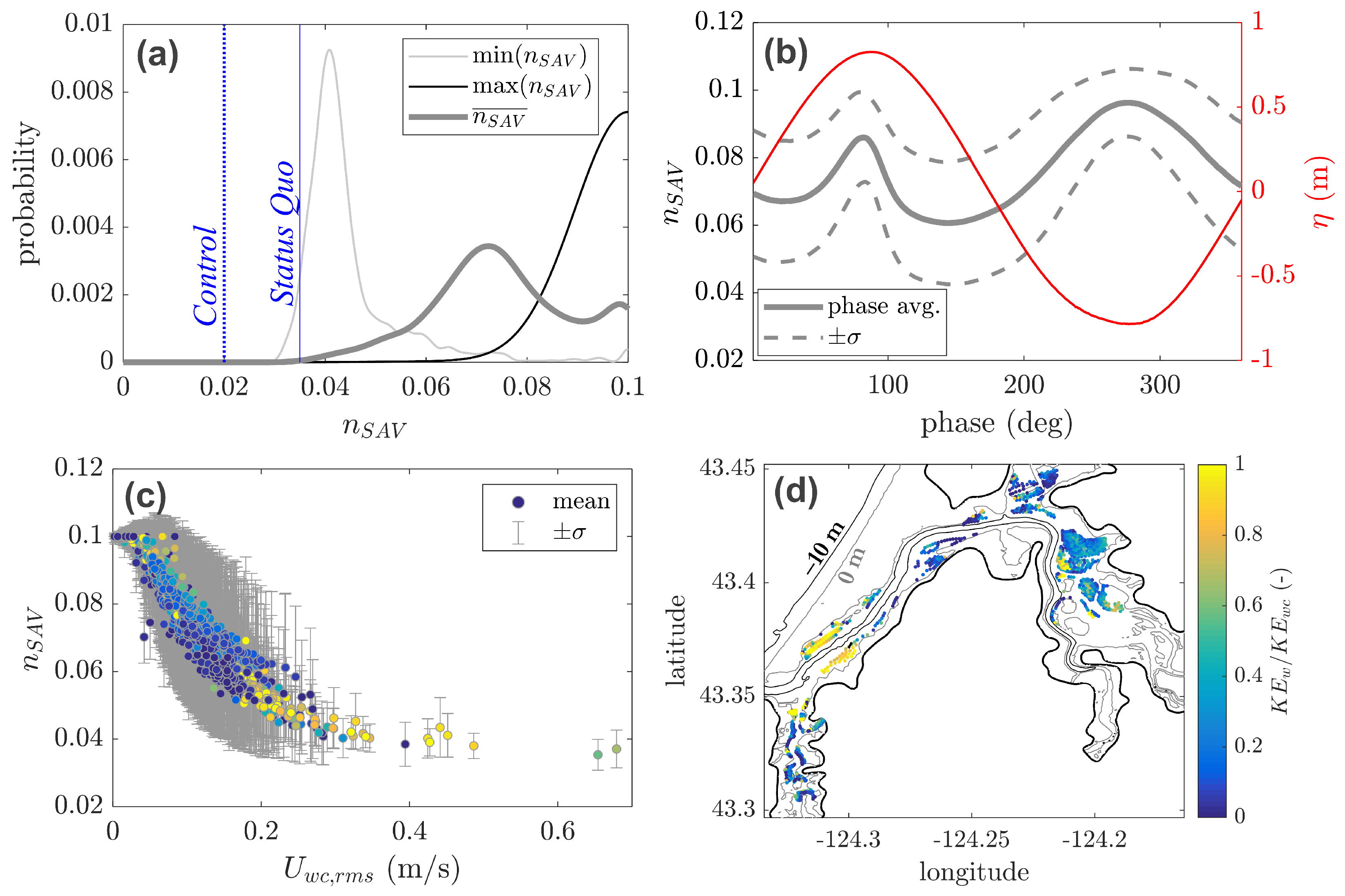
3.1. Characterization of Dynamic
3.2. Hydrodynamic and Sediment Transport Implications
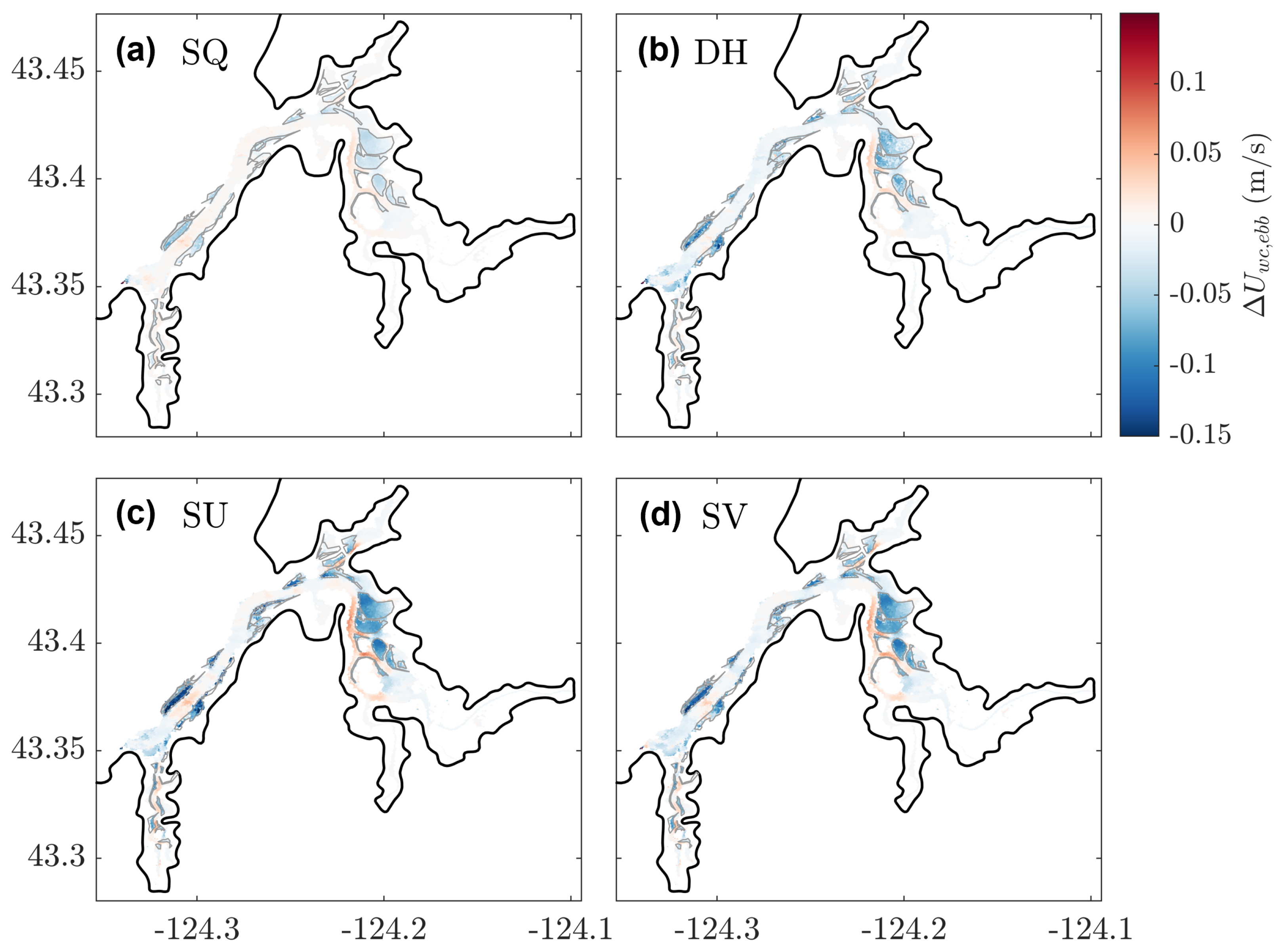
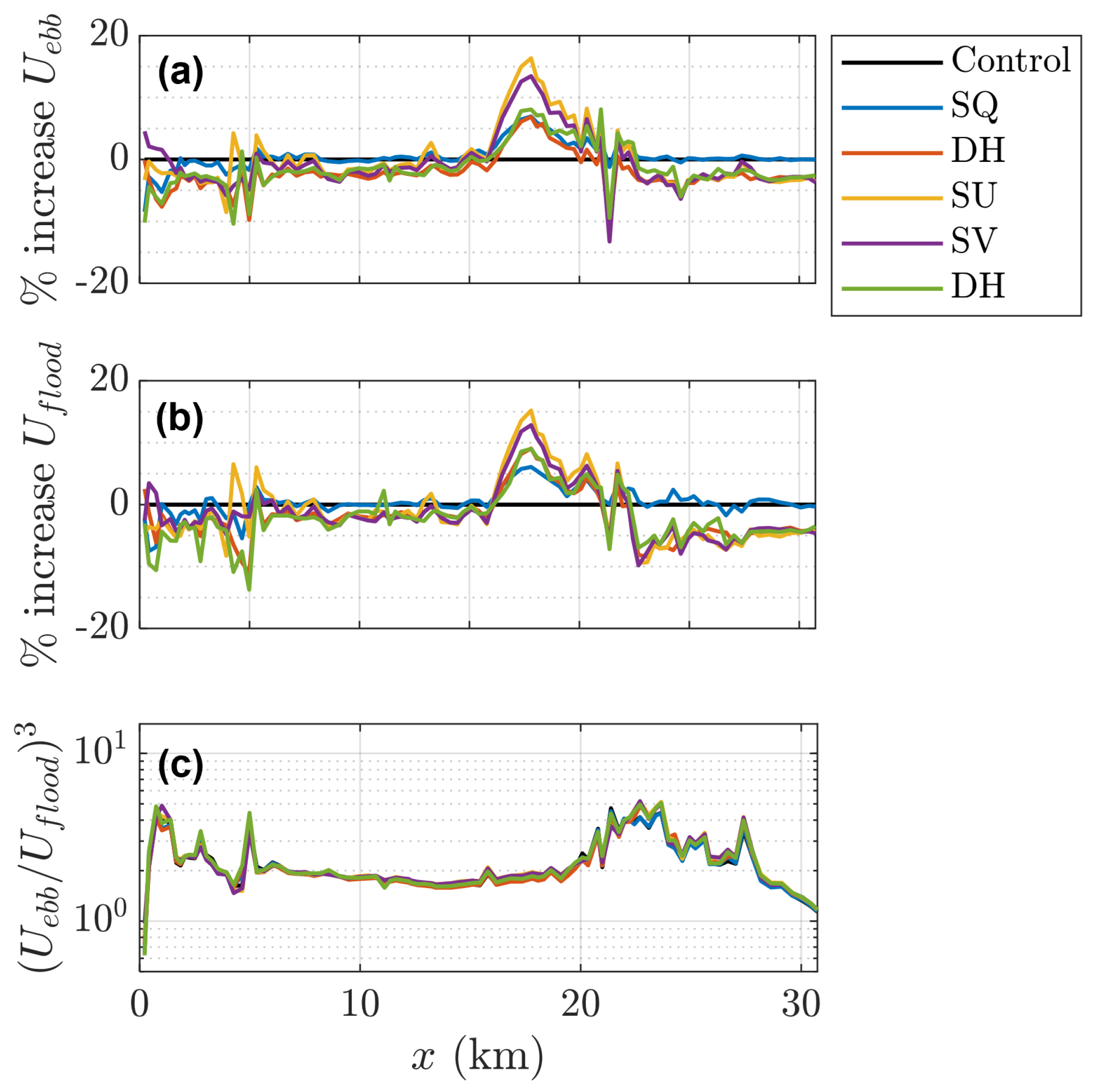
3.2.1. SAV Modification of Wave and Current Velocities
3.2.2. Sediment Fluxes
3.2.3. Circulation
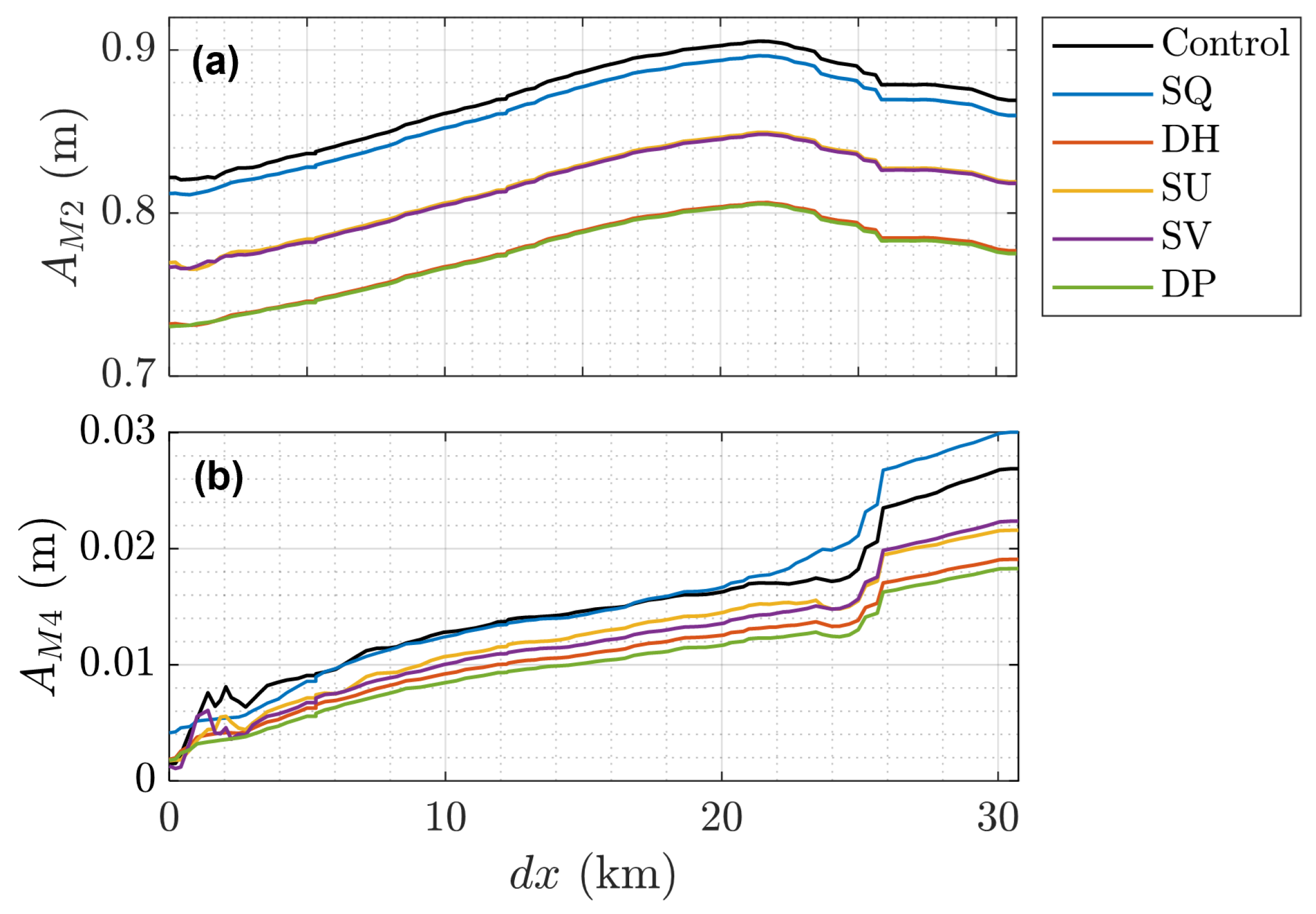
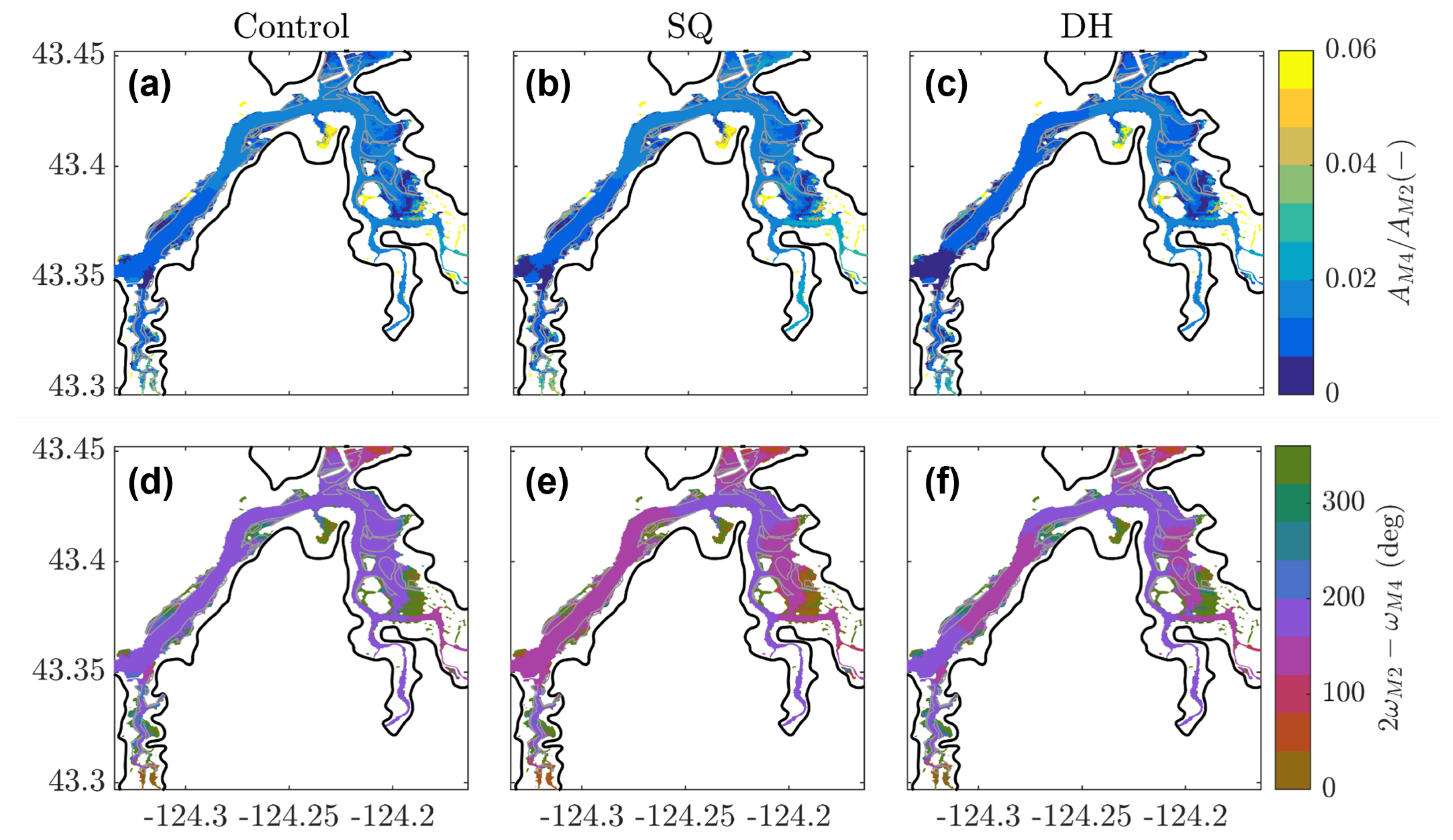
3.2.4. SAV Modification of Tidal Dynamics
4. Discussion
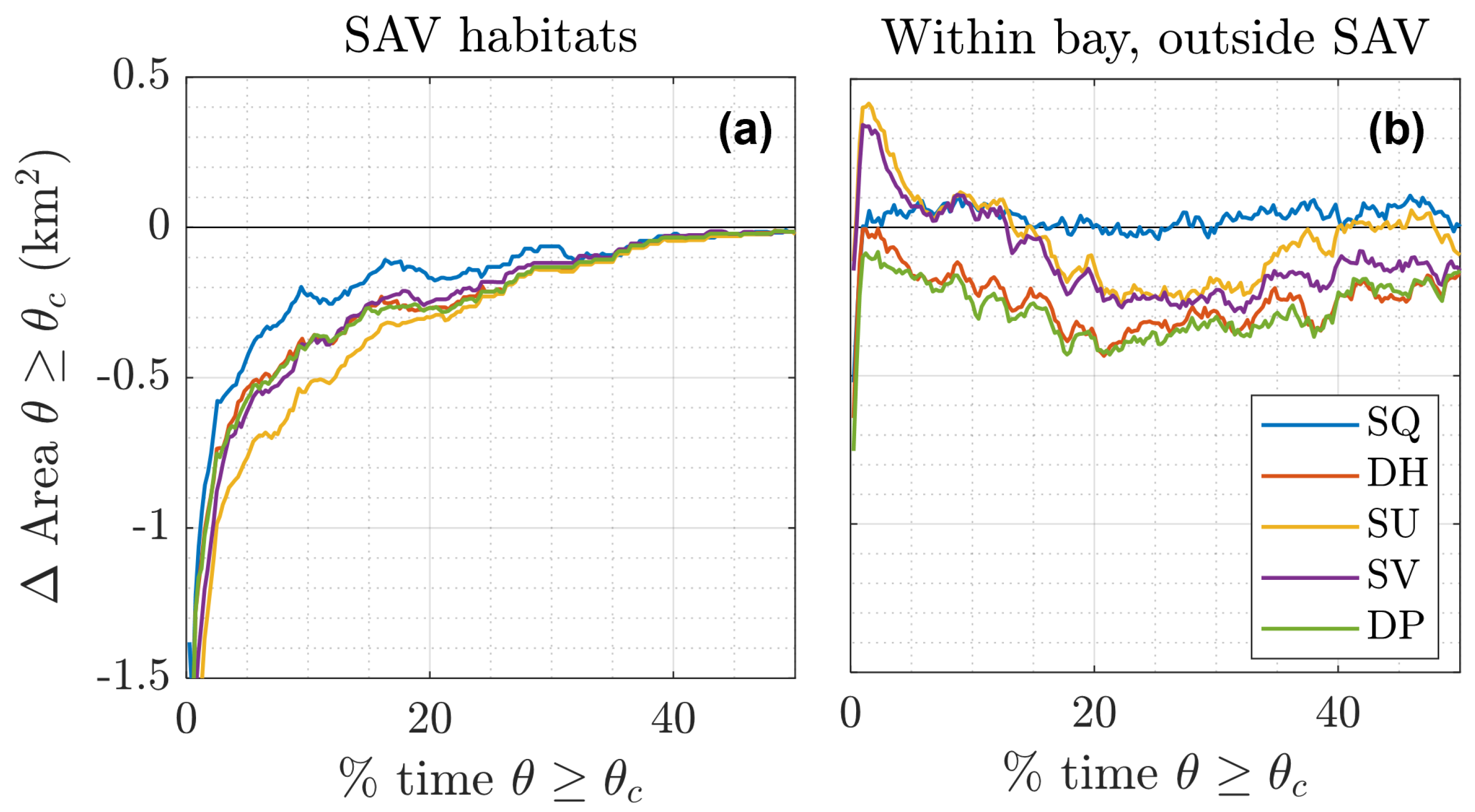
4.1. Spatial and Temporal Dependence of SAV Attenuation
4.2. Integrated Influence of SAV Attenuation
4.3. Relative Role of SAV in the CBE
4.4. Applicability to Other Systems
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Variable | Units | Value |
| Constants | |||
| Density of water | kg/m | 1000 | |
| Kinematic viscosity of water | m/s | 1.2 × 10 | |
| s | Specific gravity of sediment | - | 2.65 |
| g | Gravitational acceleration | m/s | 9.81 |
| Von Karman coefficient | - | 0.4 | |
| Vegetation drag coefficient | - | 1.0 | |
| b | Vegetation blade width | mm | 6.0 |
| t | Vegetation blade thickness | mm | 0.254 |
| Vegetation height | m | 0.75 | |
| m | Vegetation canopy density | blades/m | 1000 |
| B | Vegetation buoyancy parameter | - | 0.696 |
| I | Vegetation moment of inertia | 1/m | 2.65 × 10 |
| E | Vegetation elasticity | Pa | 2.09 × 10 |
| Variables | |||
| n | Manning’s coefficient | - | |
| C | Chezy coefficient | m/s | |
| Cauchy number | m/s | ||
| Bottom shear stress | Pa | ||
| Effective vegetation height | m | ||
| h | Water depth | m | |
| U | Current velocity (E-W) | m/s | |
| V | Current velocity (N-S) | m/s | |
| Nearbed wave orbital velocity | m/s | ||
| RMS wave velocity | m/s | ||
| Combined wave-current velocity | m/s | ||
| W | Wind speed | m/s | |
| Current propagation direction | ° | ||
| Wave propagation direction | ° | ||
| Peak wave propagation direction | ° | ||
| Wind direction | ° | ||
| A | Tidal constituent amplitude | m | |
| Tidal constituent phase | ° | ||
| Shields parameter | - | ||
| Critical Shields parameter | - | ||
| Median sediment grain size | mm | ||
| Dimensionless grain size | - | ||
| Wave friction factor | - | ||
| Roughness height | m | ||
| Wave friction coefficient | m/s |
References
- McLusky, D.; Elliott, M. The Estuarine Ecosystem: Ecology, Threats and Management; OUP Oxford: Oxford, UK, 2004. [Google Scholar]
- Buschman, F.; Van Der Vegt, M.; Hoitink, A.; Hoekstra, P. Water and suspended sediment division at a stratified tidal junction. J. Geophys. Res. Ocean. 2013, 118, 1459–1472. [Google Scholar] [CrossRef]
- Zhang, F.; Sun, J.; Lin, B.; Huang, G. Seasonal hydrodynamic interactions between tidal waves and river flows in the Yangtze Estuary. J. Mar. Syst. 2018, 186, 17–28. [Google Scholar] [CrossRef]
- Bryan, K.R.; Nardin, W.; Mullarney, J.C.; Fagherazzi, S. The role of cross-shore tidal dynamics in controlling intertidal sediment exchange in mangroves in Cù Lao Dung, Vietnam. Cont. Shelf Res. 2017, 147, 128–143. [Google Scholar] [CrossRef]
- Mazda, Y.; Wolanski, E.; King, B.; Sase, A.; Ohtsuka, D.; Magi, M. Drag force due to vegetation in mangrove swamps. Mangroves Salt Marshes 1997, 1, 193–199. [Google Scholar] [CrossRef]
- Temmerman, S.; Bouma, T.J.; Govers, G.; Wang, Z.; De Vries, M.; Herman, P. Impact of vegetation on flow routing and sedimentation patterns: Three-dimensional modeling for a tidal marsh. J. Geophys. Res. Earth Surf. 2005, 110. [Google Scholar] [CrossRef]
- Mazda, Y.; Kanazawa, N.; Wolanski, E. Tidal asymmetry in mangrove creeks. Hydrobiologia 1995, 295, 51–58. [Google Scholar] [CrossRef]
- Nardin, W.; Larsen, L.; Fagherazzi, S.; Wiberg, P. Tradeoffs among hydrodynamics, sediment fluxes and vegetation community in the Virginia Coast Reserve, USA. Estuar. Coast. Shelf Sci. 2018, 210, 98–108. [Google Scholar] [CrossRef]
- Savenije, H.H.; Toffolon, M.; Haas, J.; Veling, E.J. Analytical description of tidal dynamics in convergent estuaries. J. Geophys. Res. Ocean. 2008, 113, C10025. [Google Scholar] [CrossRef]
- Cook, S.; Lippmann, T. Tidal Energy Dissipation in Three Estuarine Environments; Coastal Dynamics: San Juan, Trinidad and Tobago, 2017; pp. 346–355. [Google Scholar]
- Du, J.; Shen, J.; Zhang, Y.J.; Ye, F.; Liu, Z.; Wang, Z.; Wang, Y.P.; Yu, X.; Sisson, M.; Wang, H.V. Tidal response to sea-level rise in different types of estuaries: The Importance of Length, Bathymetry, and Geometry. Geophys. Res. Lett. 2018, 45, 227–235. [Google Scholar] [CrossRef]
- Speer, P.; Aubrey, D. A study of non-linear tidal propagation in shallow inlet/estuarine systems Part II: Theory. Estuar. Coast. Shelf Sci. 1985, 21, 207–224. [Google Scholar] [CrossRef]
- Cook, S.E.; Lippmann, T.C.; Irish, J.D. Modeling nonlinear tidal evolution in an energetic estuary. Ocean Model. 2019, 136, 13–27. [Google Scholar] [CrossRef]
- Nielsen, P. Coastal Bottom Boundary Layers and Sediment Transport; World Scientific: Singapore, 1992; Volume 4. [Google Scholar]
- Wang, Z.; Jeuken, M.; Gerritsen, H.; De Vriend, H.; Kornman, B. Morphology and asymmetry of the vertical tide in the Westerschelde estuary. Cont. Shelf Res. 2002, 22, 2599–2609. [Google Scholar] [CrossRef]
- Guo, L.; Wang, Z.B.; Townend, I.; He, Q. Quantification of tidal asymmetry and its nonstationary variations. J. Geophys. Res. Ocean. 2019, 124, 773–787. [Google Scholar] [CrossRef]
- Ralston, D.K.; Talke, S.; Geyer, W.R.; Al-Zubaidi, H.A.; Sommerfield, C.K. Bigger tides, less flooding: Effects of dredging on barotropic dynamics in a highly modified estuary. J. Geophys. Res. Ocean. 2019, 124, 196–211. [Google Scholar] [CrossRef]
- Horrevoets, A.; Savenije, H.; Schuurman, J.; Graas, S. The influence of river discharge on tidal damping in alluvial estuaries. J. Hydrol. 2004, 294, 213–228. [Google Scholar] [CrossRef]
- Cai, H.; Savenije, H.; Toffolon, M. Linking the river to the estuary: Influence of river discharge on tidal damping. Hydrol. Earth Syst. Sci. 2014, 18, 287–304. [Google Scholar] [CrossRef]
- Snyder, R.; Sidjabat, M.; Filloux, J. A study of tides, setup and bottom friction in a shallow semi-enclosed basin. Part II: Tidal model and comparison with data. J. Phys. Oceanogr. 1979, 9, 170–188. [Google Scholar] [CrossRef][Green Version]
- Georgas, N. Large seasonal modulation of tides due to ice cover friction in a midlatitude estuary. J. Phys. Oceanogr. 2012, 42, 352–369. [Google Scholar] [CrossRef]
- Donatelli, C.; Ganju, N.K.; Kalra, T.S.; Fagherazzi, S.; Leonardi, N. Changes in hydrodynamics and wave energy as a result of seagrass decline along the shoreline of a microtidal back-barrier estuary. Adv. Water Resour. 2019, 128, 183–192. [Google Scholar] [CrossRef]
- Bos, A.R.; Bouma, T.J.; de Kort, G.L.; van Katwijk, M.M. Ecosystem engineering by annual intertidal seagrass beds: Sediment accretion and modification. Estuar. Coast. Shelf Sci. 2007, 74, 344–348. [Google Scholar] [CrossRef]
- Sherman, K.; DeBruyckere, L.A. Eelgrass Habitats on the U.S. West Coast: State of the Knowledge of Eelgrass Ecosystem Services and Eelgrass Extent; Technical Report; Pacific Marine and Estuarine Fish Habitat Partnership: Salem, OR, USA, 2018. [Google Scholar]
- Thom, A. Momentum absorption by vegetation. Q. J. R. Meteorol. Soc. 1971, 97, 414–428. [Google Scholar] [CrossRef]
- Luhar, M.; Nepf, H.M. From the blade scale to the reach scale: A characterization of aquatic vegetative drag. Adv. Water Resour. 2013, 51, 305–316. [Google Scholar] [CrossRef]
- Beudin, A.; Kalra, T.S.; Ganju, N.K.; Warner, J.C. Development of a coupled wave-flow-vegetation interaction model. Comput. Geosci. 2017, 100, 76–86. [Google Scholar] [CrossRef]
- Dalrymple, R.A.; Kirby, J.T.; Hwang, P.A. Wave diffraction due to areas of energy dissipation. J. Waterw. Port Coastal Ocean Eng. 1984, 110, 67–79. [Google Scholar] [CrossRef]
- Mendez, F.J.; Losada, I.J. An empirical model to estimate the propagation of random breaking and nonbreaking waves over vegetation fields. Coast. Eng. 2004, 51, 103–118. [Google Scholar] [CrossRef]
- Suzuki, T.; Zijlema, M.; Burger, B.; Meijer, M.C.; Narayan, S. Wave dissipation by vegetation with layer schematization in SWAN. Coast. Eng. 2012, 59, 64–71. [Google Scholar] [CrossRef]
- Kobayashi, N.; Raichle, A.W.; Asano, T. Wave attenuation by vegetation. J. Waterw. Port Coast. Ocean Eng. 1993, 119, 30–48. [Google Scholar] [CrossRef]
- Bradley, K.; Houser, C. Relative velocity of seagrass blades: Implications for wave attenuation in low-energy environments. J. Geophys. Res. Earth Surf. 2009, 114, F1. [Google Scholar] [CrossRef]
- Anderson, M.; Smith, J. Wave attenuation by flexible, idealized salt marsh vegetation. Coast. Eng. 2014, 83, 82–92. [Google Scholar] [CrossRef]
- Augustin, L.N.; Irish, J.L.; Lynett, P. Laboratory and numerical studies of wave damping by emergent and near-emergent wetland vegetation. Coast. Eng. 2009, 56, 332–340. [Google Scholar] [CrossRef]
- Jadhav, R.S.; Chen, Q.; Smith, J.M. Spectral distribution of wave energy dissipation by salt marsh vegetation. Coast. Eng. 2013, 77, 99–107. [Google Scholar] [CrossRef]
- Nepf, H.M. Drag, turbulence, and diffusion in flow through emergent vegetation. Water Resour. Res. 1999, 35, 479–489. [Google Scholar] [CrossRef]
- Losada, I.J.; Maza, M.; Lara, J.L. A new formulation for vegetation-induced damping under combined waves and currents. Coast. Eng. 2016, 107, 1–13. [Google Scholar] [CrossRef]
- Chen, H.; Ni, Y.; Li, Y.; Liu, F.; Ou, S.; Su, M.; Peng, Y.; Hu, Z.; Uijttewaal, W.; Suzuki, T. Deriving vegetation drag coefficients in combined wave-current flows by calibration and direct measurement methods. Adv. Water Resour. 2018, 122, 217–227. [Google Scholar] [CrossRef]
- Wu, W.C.; Cox, D.T. Effects of wave steepness and relative water depth on wave attenuation by emergent vegetation. Estuar. Coast. Shelf Sci. 2015, 164, 443–450. [Google Scholar] [CrossRef]
- Hu, K.; Chen, Q.; Wang, H. A numerical study of vegetation impact on reducing storm surge by wetlands in a semi-enclosed estuary. Coast. Eng. 2015, 95, 66–76. [Google Scholar] [CrossRef]
- Bunya, S.; Dietrich, J.C.; Westerink, J.; Ebersole, B.; Smith, J.; Atkinson, J.; Jensen, R.; Resio, D.; Luettich, R.; Dawson, C.; et al. A high-resolution coupled riverine flow, tide, wind, wind wave, and storm surge model for southern Louisiana and Mississippi. Part I: Model development and validation. Mon. Weather Rev. 2010, 138, 345–377. [Google Scholar] [CrossRef]
- Wiyono, R.U.A. Unstructured Mesh Tsunami Simulation Using FVCOM Considering the Fine Structure of Land Use. Ph.D. Thesis, Yokohama National University, Yokohama, Kanagawa, 2015. [Google Scholar]
- Nardin, W.; Locatelli, S.; Pasquarella, V.; Rulli, M.C.; Woodcock, C.E.; Fagherazzi, S. Dynamics of a fringe mangrove forest detected by Landsat images in the Mekong River Delta, Vietnam. Earth Surf. Process. Landf. 2016, 41, 2024–2037. [Google Scholar] [CrossRef]
- Nardin, W.; Lera, S.; Nienhuis, J. Effect of offshore waves and vegetation on the sediment budget in the Virginia Coast Reserve (VA). Earth Surf. Process. Landf. 2020, 45, 3055–3068. [Google Scholar] [CrossRef]
- Bandhoe, S. Modelling Interspecific Competition between the Salicornia and Spartina Species and Its Effect on the Bio-Geomorphological Development of Salt Marshes. Master’s Thesis, University of Twente, Enschede, The Netherlands, 2020. [Google Scholar]
- Medeiros, S.C.; Hagen, S.C.; Weishampel, J.F. Comparison of floodplain surface roughness parameters derived from land cover data and field measurements. J. Hydrol. 2012, 452, 139–149. [Google Scholar] [CrossRef]
- Kerr, P.; Martyr, R.; Donahue, A.; Hope, M.; Westerink, J.; Luettich, R., Jr.; Kennedy, A.; Dietrich, J.; Dawson, C.; Westerink, H. US IOOS coastal and ocean modeling testbed: Evaluation of tide, wave, and hurricane surge response sensitivities to mesh resolution and friction in the Gulf of Mexico. J. Geophys. Res. Ocean. 2013, 118, 4633–4661. [Google Scholar] [CrossRef]
- Passeri, D.; Hagen, S.C.; Smar, D.; Alimohammadi, N.; Risner, A.; White, R. Sensitivity of an ADCIRC tide and storm surge model to Manning’s n. Estuar. Coast. Model. 2011, 2013, 457–475. [Google Scholar]
- Abdolahpour, M.; Hambleton, M.; Ghisalberti, M. The wave-driven current in coastal canopies. J. Geophys. Res. Ocean. 2017, 122, 3660–3674. [Google Scholar] [CrossRef]
- Lei, J.; Nepf, H. Blade dynamics in combined waves and current. J. Fluids Struct. 2019, 87, 137–149. [Google Scholar] [CrossRef]
- van Veelen, T.J.; Fairchild, T.P.; Reeve, D.E.; Karunarathna, H. Experimental study on vegetation flexibility as control parameter for wave damping and velocity structure. Coast. Eng. 2020, 157, 103648. [Google Scholar] [CrossRef]
- Ashall, L.M.; Mulligan, R.P.; van Proosdij, D.; Poirier, E. Application and validation of a three-dimensional hydrodynamic model of a macrotidal salt marsh. Coast. Eng. 2016, 114, 35–46. [Google Scholar] [CrossRef]
- Fringer, O.B.; Dawson, C.N.; He, R.; Ralston, D.K.; Zhang, Y.J. The future of coastal and estuarine modeling: Findings from a workshop. Ocean Model. 2019, 143, 101458. [Google Scholar] [CrossRef]
- Rumrill, S. The Ecology of the South Slough Estuary: Site Profile of the South Slough National Estuarine Research Reserve; Technical Report; Oregon Department of State Lands: Salem, OR, USA, 2006.
- Eidam, E.; Sutherland, D.; Ralston, D.; Dye, B.; Conroy, T.; Schmitt, J.; Ruggiero, P.; Wood, J. Impacts of 150 years of shoreline and bathymetric change in the Coos Estuary, Oregon, USA. Estuar. Coasts 2020, 1–19. [Google Scholar] [CrossRef]
- Caldera, M. South Slough Adventures: Life on a Southern Oregon Estuary; South Coast Printing: Coos Bay, OR, USA, 1995. [Google Scholar]
- Eidam, E.; Sutherland, D.; Ralston, D.; Conroy, T.; Dye, B. Shifting sediment dynamics in the Coos Bay Estuary in response to 150 years of modification. J. Geophys. Res. Ocean. 2021, 126, e2020JC016771. [Google Scholar] [CrossRef]
- Hickey, B.M.; Banas, N.S. Oceanography of the US Pacific Northwest coastal ocean and estuaries with application to coastal ecology. Estuaries 2003, 26, 1010–1031. [Google Scholar] [CrossRef]
- Engle, J.M.; Miller, K. Distribution and morphology of eelgrass (Zostera marina L.) at the California Channel Islands. In Proceedings of the Sixth California Islands Symposium, Arcata, CA, USA, 7–11 November 2005; pp. 405–414. [Google Scholar]
- Parker, K.; Hill, D.; García-Medina, G.; Beamer, J. The effects of changing climate on estuarine water levels: A United States Pacific Northwest case study. Nat. Hazards Earth Syst. Sci. 2019, 19, 1601–1618. [Google Scholar] [CrossRef]
- Henry Lee, I.; Brown, C.A. Classification of Regional Patterns of Environmental Drivers and Benthic Habitats in Pacific Northwest Estuaries; Technical Report; US Environmental Protection Agency: Washington, DC, USA, 2009.
- Conroy, T.; Sutherland, D.A.; Ralston, D.K. Estuarine exchange flow variability in a seasonal, segmented estuary. J. Phys. Oceanogr. 2020, 50, 595–613. [Google Scholar] [CrossRef]
- Risley, J.; Stonewall, A.; Haluska, T. Estimating Flow-Duration and Low-Flow Frequency Statistics for Unregulated Streams in Oregon; Technical Report; U.S. Geological Survey: Washington, DC, USA, 2008.
- The StreamStats Program for Oregon. Available online: http://water.usgs.gov/osw/streamstats/colorado.html (accessed on 1 January 2020).
- National Weather Service. U.S. States and Territories. Available online: http://www.nws.noaa.gov/geodata/catalog/national/html/us_state.htm (accessed on 1 January 2022).
- Luettich, R.A.; Westerink, J.J.; Scheffner, N.W. ADCIRC: An Advanced Three-Dimensional Circulation Model for Shelves, Coasts, and Estuaries. Report 1, Theory and Methodology of ADCIRC-2DD1 and ADCIRC-3DL; Technical Report; US Army Engineer Waterways Experiment Station: Washington, DC, USA, 1992.
- Dietrich, J.; Westerink, J.; Kennedy, A.; Smith, J.; Jensen, R.; Zijlema, M.; Holthuijsen, L.; Dawson, C.; Luettich, R.; Powell, M.; et al. Hurricane Gustav (2008) waves and storm surge: Hindcast, synoptic analysis, and validation in southern Louisiana. Mon. Weather Rev. 2011, 139, 2488–2522. [Google Scholar] [CrossRef]
- Cheng, T.; Hill, D.; Read, W. The contributions to storm tides in Pacific northwest estuaries: Tillamook Bay, Oregon, and the December 2007 storm. J. Coast. Res. 2015, 31, 723–734. [Google Scholar] [CrossRef]
- Graham, L.; Butler, T.; Walsh, S.; Dawson, C.; Westerink, J.J. A measure-theoretic algorithm for estimating bottom friction in a coastal inlet: Case study of bay St. Louis during hurricane Gustav (2008). Mon. Weather Rev. 2017, 145, 929–954. [Google Scholar] [CrossRef]
- Garzon, J.L.; Ferreira, C.M. Storm surge modeling in large estuaries: Sensitivity analyses to parameters and physical processes in the Chesapeake Bay. J. Mar. Sci. Eng. 2016, 4, 45. [Google Scholar] [CrossRef]
- Akbar, M.K.; Kanjanda, S.; Musinguzi, A. Effect of bottom friction, wind drag coefficient, and meteorological forcing in hindcast of Hurricane Rita storm surge using SWAN+ ADCIRC model. J. Mar. Sci. Eng. 2017, 5, 38. [Google Scholar] [CrossRef]
- Egbert, G.D.; Erofeeva, S.Y. Efficient inverse modeling of barotropic ocean tides. J. Atmos. Ocean. Technol. 2002, 19, 183–204. [Google Scholar] [CrossRef]
- Roberts, K.J.; Pringle, W.J.; Westerink, J.J. OceanMesh2D 1.0: MATLAB-based software for two-dimensional unstructured mesh generation in coastal ocean modeling. Geosci. Model Dev. 2019, 12, 1847–1868. [Google Scholar] [CrossRef]
- Iowa State University Iowa Environmental Mesonet. Available online: https://mesonet.agron.iastate.edu/sites/site.php?station=OTH&network=OR_ASOS (accessed on 1 May 2021).
- RAWS USA Climate Archive. Available online: https://raws.dri.edu/ (accessed on 1 May 2021).
- Kalnay, E.; Kanamitsu, M.; Kistler, R.; Collins, W.; Deaven, D.; Gandin, L.; Iredell, M.; Saha, S.; White, G.; Woollen, J.; et al. The NCEP/NCAR 40-year reanalysis project. Bull. Am. Meteorol. Soc. 1996, 77, 437–472. [Google Scholar] [CrossRef]
- Baptist, M.; Babovic, V.; Rodríguez Uthurburu, J.; Keijzer, M.; Uittenbogaard, R.; Mynett, A.; Verwey, A. On inducing equations for vegetation resistance. J. Hydraul. Res. 2007, 45, 435–450. [Google Scholar] [CrossRef]
- Uittenbogaard, R. Modelling turbulence in vegetated aquatic flows. In Proceedings of the Riparian Forest Vegetated Channels Workshop, Trendo, Italy, 20–22 February 2003. [Google Scholar]
- Luhar, M.; Nepf, H.M. Flow-induced reconfiguration of buoyant and flexible aquatic vegetation. Limnol. Oceanogr. 2011, 56, 2003–2017. [Google Scholar] [CrossRef]
- Philips, R.C. The Ecology of Eelgrass Meadows in the Pacific Northwest: A Community Profile; Technical Report; US Fish and Wildflife Service: Washington, DC, USA, 1984.
- Gambi, M.C.; Nowell, A.R.; Jumars, P.A. Flume observations on flow dynamics in Zostera marina (eelgrass) beds. Mar. Ecol. Prog. Ser. 1990, 61, 159–169. [Google Scholar] [CrossRef]
- Thom, R.M.; Borde, A.B.; Rumrill, S.; Woodruff, D.L.; Williams, G.D.; Southard, J.A.; Sargeant, S.L. Factors influencing spatial and annual variability in eelgrass (Zostera marina L.) meadows in Willapa Bay, Washington, and Coos Bay, Oregon, estuaries. Estuaries 2003, 26, 1117–1129. [Google Scholar] [CrossRef]
- Lefebvre, A.; Thompson, C.; Amos, C. Influence of Zostera marina canopies on unidirectional flow, hydraulic roughness and sediment movement. Cont. Shelf Res. 2010, 30, 1783–1794. [Google Scholar] [CrossRef]
- Abdelrhman, M.A. Modeling coupling between eelgrass Zostera marina and water flow. Mar. Ecol. Prog. Ser. 2007, 338, 81–96. [Google Scholar] [CrossRef]
- Ruessink, B.G.; Michallet, H.; Abreu, T.; Sancho, F.; Van Der A, D.A.; Van Der Werf, J.J.; Silva, P.A. Observations of velocities, sand concentrations, and fluxes under velocity-asymmetric oscillatory flows. J. Geophys. Res. Ocean. 2011, 116, C3. [Google Scholar] [CrossRef]
- Hessing-Lewis, M.L.; Hacker, S.D.; Menge, B.A.; Rumrill, S.S. Context-dependent eelgrass–macroalgae interactions along an estuarine gradient in the Pacific Northwest, USA. Estuar. Coasts 2011, 34, 1169–1181. [Google Scholar] [CrossRef]
- Lacy, J.R.; Rubin, D.M.; Ikeda, H.; Mokudai, K.; Hanes, D.M. Bed forms created by simulated waves and currents in a large flume. J. Geophys. Res. Ocean. 2007, 112, C10. [Google Scholar] [CrossRef]
- Luhar, M.; Infantes, E.; Orfila, A.; Terrados, J.; Nepf, H.M. Field observations of wave-induced streaming through a submerged seagrass (Posidonia oceanica) meadow. J. Geophys. Res. Ocean. 2013, 118, 1955–1968. [Google Scholar] [CrossRef]
- Chow, V.T. Open Channel Hydraulics; McGraw Hill: New York, NY, USA, 1959. [Google Scholar]
- Bretschneider, C.L.; Agrawal, J.D.; Carmella, N.C.; Wohlmut, K.A. Hurricane Iniki, hindcast, winds, windwaves, swells, storm surge, and wave runup. In Proceedings of the American Society of Civil Engineers, Hurricanes of 1992 Technical Conference, Miami, FL, USA, 1–3 December 1993. [Google Scholar]
- Madsen, O.S.; Poon, Y.K.; Graber, H.C. Spectral wave attenuation by bottom friction: Theory. In Proceedings of the 21st International Conference on Coastal Engineering, Costa del Sol-Malaga, Spain, 20–25 June 1988; pp. 492–504. [Google Scholar]
- Baron-Hyppolite, C.; Lashley, C.H.; Garzon, J.; Miesse, T.; Ferreira, C.; Bricker, J.D. Comparison of Implicit and Explicit Vegetation Representations in SWAN Hindcasting Wave Dissipation by Coastal Wetlands in Chesapeake Bay. Geosciences 2019, 9, 8. [Google Scholar] [CrossRef]
- Moki, H.; Taguchi, K.; Nakagawa, Y.; Montani, S.; Kuwae, T. Spatial and seasonal impacts of submerged aquatic vegetation (SAV) drag force on hydrodynamics in shallow waters. J. Mar. Syst. 2020, 209, 103373. [Google Scholar] [CrossRef]
- Zhao, H.; Chen, Q. Modeling attenuation of storm surge over deformable vegetation: Parametric study. J. Eng. Mech. 2016, 142, 06016006. [Google Scholar] [CrossRef]
- Zhao, H.; Chen, Q. Modeling attenuation of storm surge over deformable vegetation: Methodology and verification. J. Eng. Mech. 2014, 140, 04014090. [Google Scholar] [CrossRef]
- Wickham, J.; Stehman, S.V.; Sorenson, D.G.; Gass, L.; Dewitz, J.A. Thematic accuracy assessment of the NLCD 2016 land cover for the conterminous United States. Remote Sens. Environ. 2021, 257, 112357. [Google Scholar] [CrossRef]
- Stark, J.; Plancke, Y.; Ides, S.; Meire, P.; Temmerman, S. Coastal flood protection by a combined nature-based and engineering approach: Modeling the effects of marsh geometry and surrounding dikes. Estuar. Coast. Shelf Sci. 2016, 175, 34–45. [Google Scholar] [CrossRef]
- Paquier, A.E.; Oudart, T.; Le Bouteiller, C.; Meulé, S.; Larroudé, P.; Dalrymple, R.A. 3D numerical simulation of seagrass movement under waves and currents with GPUSPH. Int. J. Sediment Res. 2020, 36, 711–722. [Google Scholar] [CrossRef]
- Soulsby, R. Dynamics of Marine Sands; T. Telford: London, UK, 1997. [Google Scholar]
- Masselink, G.H.M.; Knight, J. Introduction to Coastal Processes and Geomorphology; Taylor and Francis: London, UK, 2011. [Google Scholar]
- Edinger, J.E.; Buchak, E.M.; Kollubru, V.S. Modeling flushing and mixing in a deep estuary. Water Air Soil Pollut. 1998, 102, 345–353. [Google Scholar] [CrossRef]
- Wang, C.F.; Hsu, M.H.; Kuo, A.Y. Residence time of the Danshuei River estuary, Taiwan. Estuar. Coast. Shelf Sci. 2004, 60, 381–393. [Google Scholar] [CrossRef]
- Xu, D.; Xue, H.; Greenberg, D.A. A numerical study of the circulation and drifter trajectories in Cobscook Bay. Estuar. Coast. Model. 2005, 2006, 176–195. [Google Scholar]
- Hill, D.; Ciavola, S.; Etherington, L.; Klaar, M. Estimation of freshwater runoff into Glacier Bay, Alaska and incorporation into a tidal circulation model. Estuar. Coast. Shelf Sci. 2009, 82, 95–107. [Google Scholar] [CrossRef]
- Haase, A.T.; Eggleston, D.B.; Luettich, R.A.; Weaver, R.J.; Puckett, B.J. Estuarine circulation and predicted oyster larval dispersal among a network of reserves. Estuar. Coast. Shelf Sci. 2012, 101, 33–43. [Google Scholar] [CrossRef]
- Hofmann, E.E.; Hedström, K.S.; Moisan, J.R.; Haidvogel, D.B.; Mackas, D.L. Use of simulated drifter tracks to investigate general transport patterns and residence times in the Coastal Transition Zone. J. Geophys. Res. Ocean. 1991, 96, 15041–15052. [Google Scholar] [CrossRef]
- Wengrove, M.E.; Foster, D.L.; Lippmann, T.C.; de Schipper, M.A.; Calantoni, J. Observations of Bedform Migration and Bedload Sediment Transport in Combined Wave-Current Flows. J. Geophys. Res. Ocean. 2019, 124, 4572–4590. [Google Scholar] [CrossRef]
- Short, F.T.; Coles, R.G. (Eds.) Global Seagrass Research Methods; Elsevier: Amsterdam, The Netherlands, 2001. [Google Scholar]
- Orth, R.J.; Carruthers, T.J.B.; Dennison, W.C.; Duarte, C.M.; Fourqurean, J.W.; Heck, K.L.; Hughes, A.R.; Kendrick, G.A.; Kenworthy, W.J.; Olyarnik, S.; et al. A Global Crisis for Seagrass Ecosystems. BioScience 2006, 56, 987–996. [Google Scholar] [CrossRef]
- Kalra, T.S.; Aretxabaleta, A.; Seshadri, P.; Ganju, N.K.; Beudin, A. Sensitivity analysis of a coupled hydrodynamic-vegetation model using the effectively subsampled quadratures method (ESQM v5. 2). Geosci. Model Dev. 2017, 10, 4511–4523. [Google Scholar] [CrossRef]

| Value | Data Sources | Record Length | |
|---|---|---|---|
| Waves | JONSWAP | NOAA NDBC | 2005–2013 |
| (m) | 3.01 | ||
| (s) | 12.6 | ||
| (deg) | 275 | ||
| Wind | |||
| Daily (m/s) | 4.10 | NOAA NWS WRCC | 1996–2006 |
| Daily (m/s) | 3.86 | ISU IEM | 1949–Present |
| Mean () | 166 | NCEP NCARR | 1981–2010 |
| Streamflow (m/s) | USGS Streamstats | 1906–2005 | |
| Coos River | 86.6 | ||
| Isthmus Slough | 3.44 | ||
| Palouse Slough | 1.64 |
| Treatment | ID | Coverage Extent | Spatially Varying? | Time Varying? | Description |
|---|---|---|---|---|---|
| Control | − | none | N | N | Friction values derived from LULC classification [60,96] |
| Status Quo | SQ | historic [24] | N | N | given empirically derived value (0.035) from published LULC-to-Manning’s n conversion tables |
| Dynamic Historic | DH | historic | Y | Y | calculated from dynamic friction routine (Figure 2) |
| Dynamic Potential | DP | potential [55] | Y | Y | calculated from dynamic friction routine (Figure 2) |
| Static Varying | SV | historic | Y | N | at each SAV node equal to time-averaged value from DH simulation |
| Static Uniform | SU | historic | N | N | at each SAV node equal to time- and space-averaged value from DH simulation |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Holzenthal, E.R.; Hill, D.F.; Wengrove, M.E. Multi-Scale Influence of Flexible Submerged Aquatic Vegetation (SAV) on Estuarine Hydrodynamics. J. Mar. Sci. Eng. 2022, 10, 554. https://doi.org/10.3390/jmse10040554
Holzenthal ER, Hill DF, Wengrove ME. Multi-Scale Influence of Flexible Submerged Aquatic Vegetation (SAV) on Estuarine Hydrodynamics. Journal of Marine Science and Engineering. 2022; 10(4):554. https://doi.org/10.3390/jmse10040554
Chicago/Turabian StyleHolzenthal, Elizabeth R., David F. Hill, and Meagan E. Wengrove. 2022. "Multi-Scale Influence of Flexible Submerged Aquatic Vegetation (SAV) on Estuarine Hydrodynamics" Journal of Marine Science and Engineering 10, no. 4: 554. https://doi.org/10.3390/jmse10040554
APA StyleHolzenthal, E. R., Hill, D. F., & Wengrove, M. E. (2022). Multi-Scale Influence of Flexible Submerged Aquatic Vegetation (SAV) on Estuarine Hydrodynamics. Journal of Marine Science and Engineering, 10(4), 554. https://doi.org/10.3390/jmse10040554






