Numerical Investigation of Vortex Shedding from a 5:1 Rectangular Cylinder at Different Angles of Attack
Abstract
1. Introduction
2. Numerical Simulation Method
2.1. Governing Equations and Numerical Algorithm
2.2. Computational Domain and Boundary Conditions
2.3. Numerical Validation
3. Results and Discussion
3.1. Force Coefficients Characteristics
3.1.1. Time Histories of Force Coefficient
3.1.2. Relationships between Force Coefficients and Vortex Structure
3.2. Effects of AoA on Global Flow Characteristics
3.2.1. Time-Averaged Separation and Reattachment
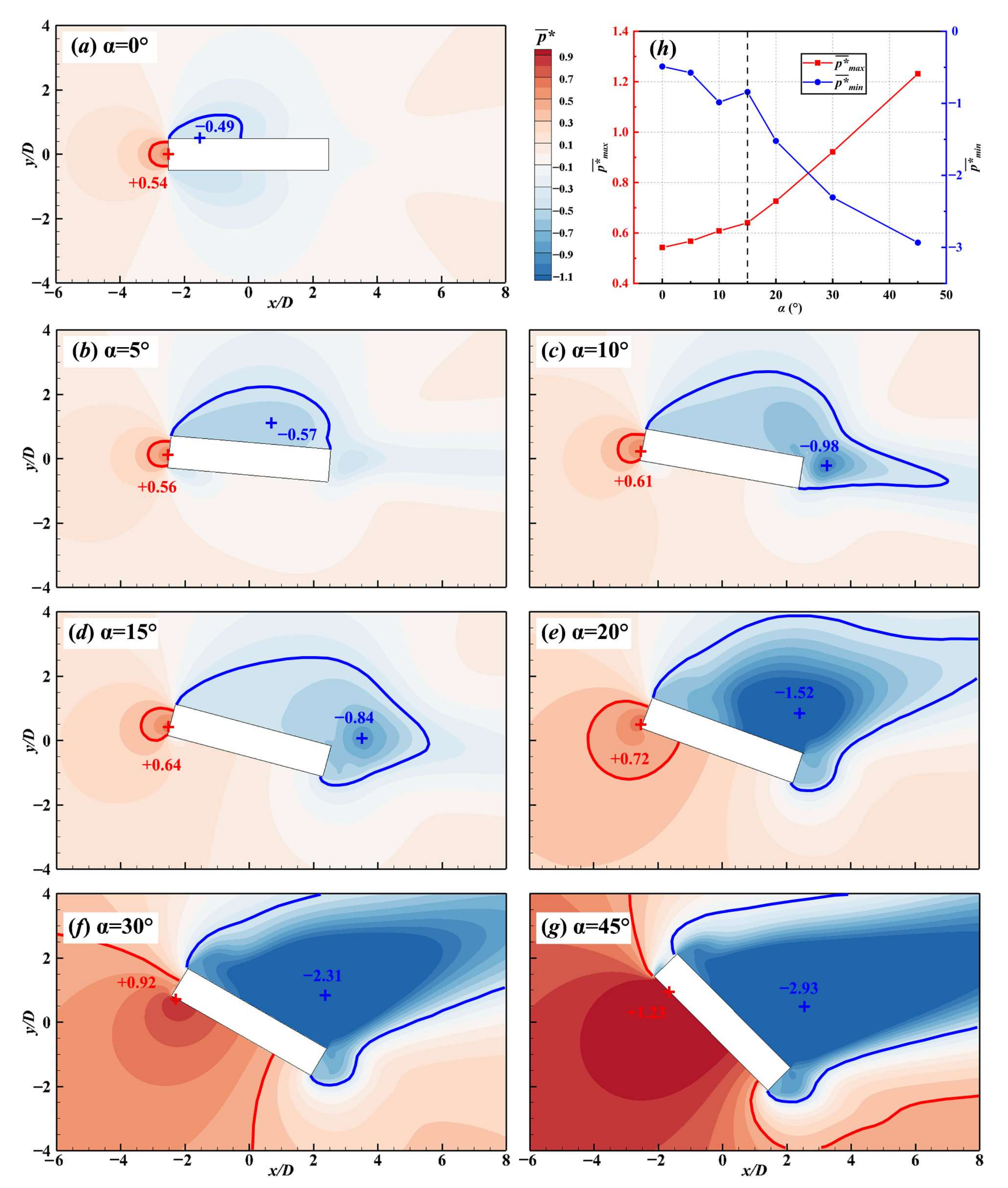
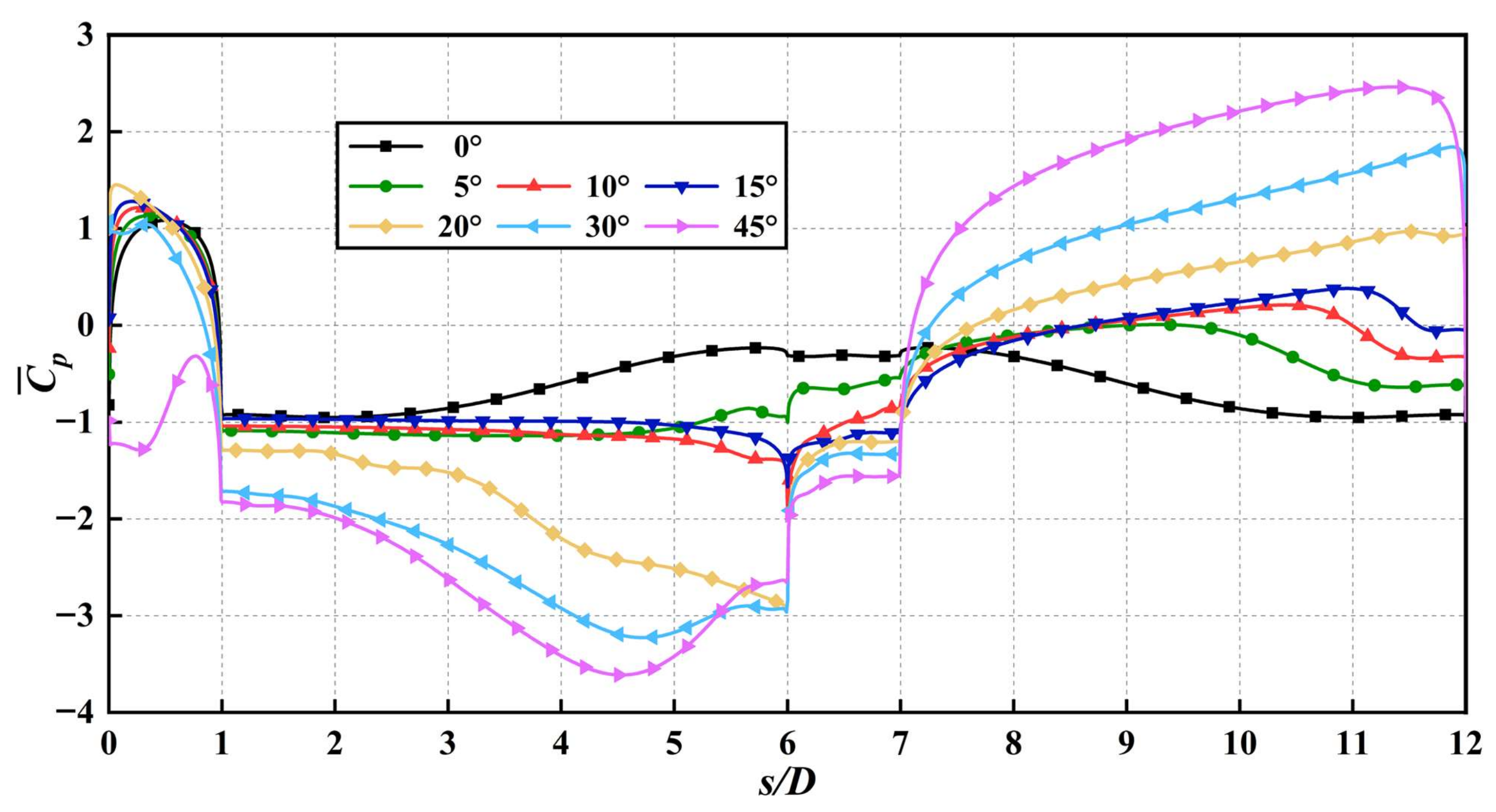
3.2.2. Pressure Distribution in the Flow Field
3.3. Effects of AoA on Local Flow Characteristics
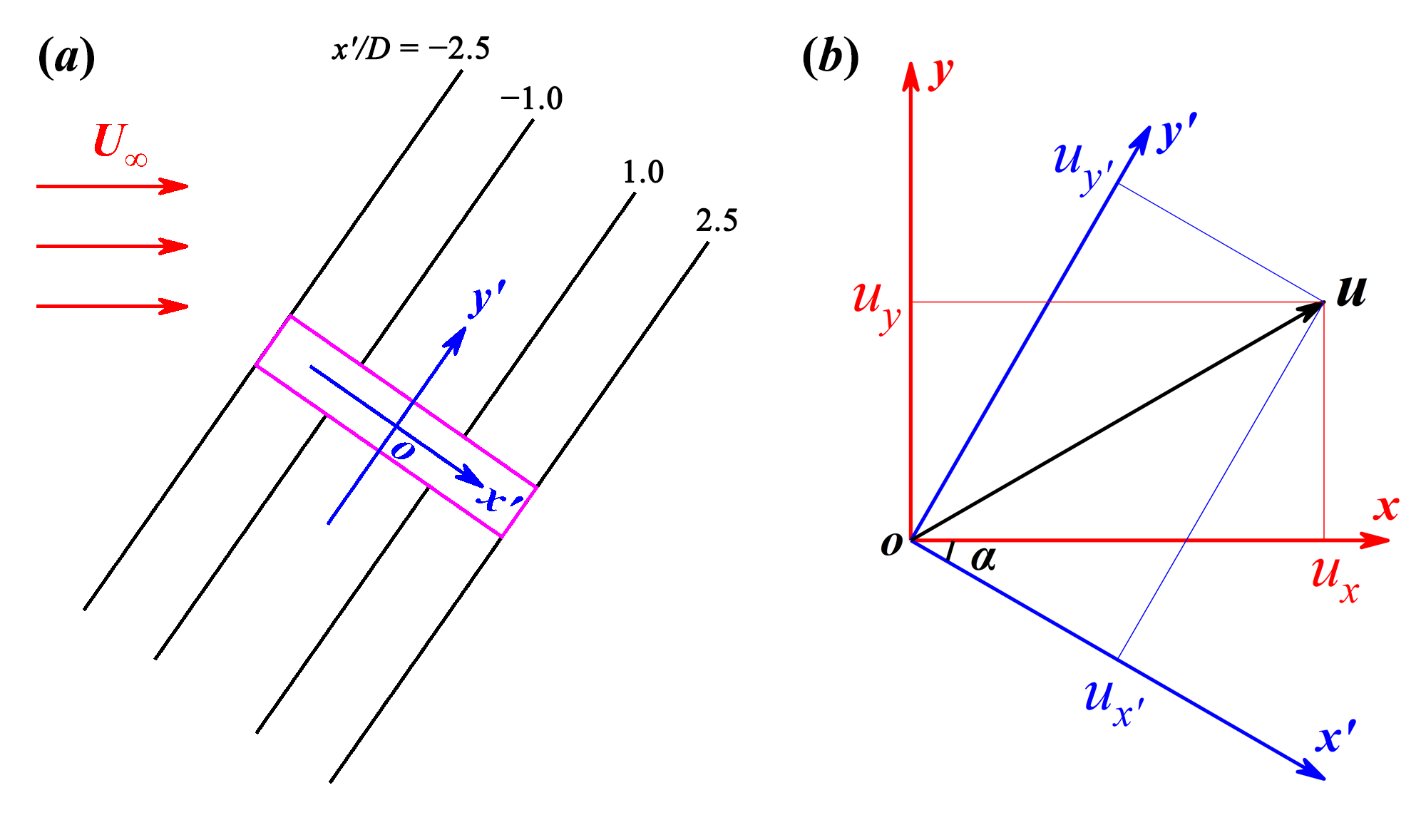
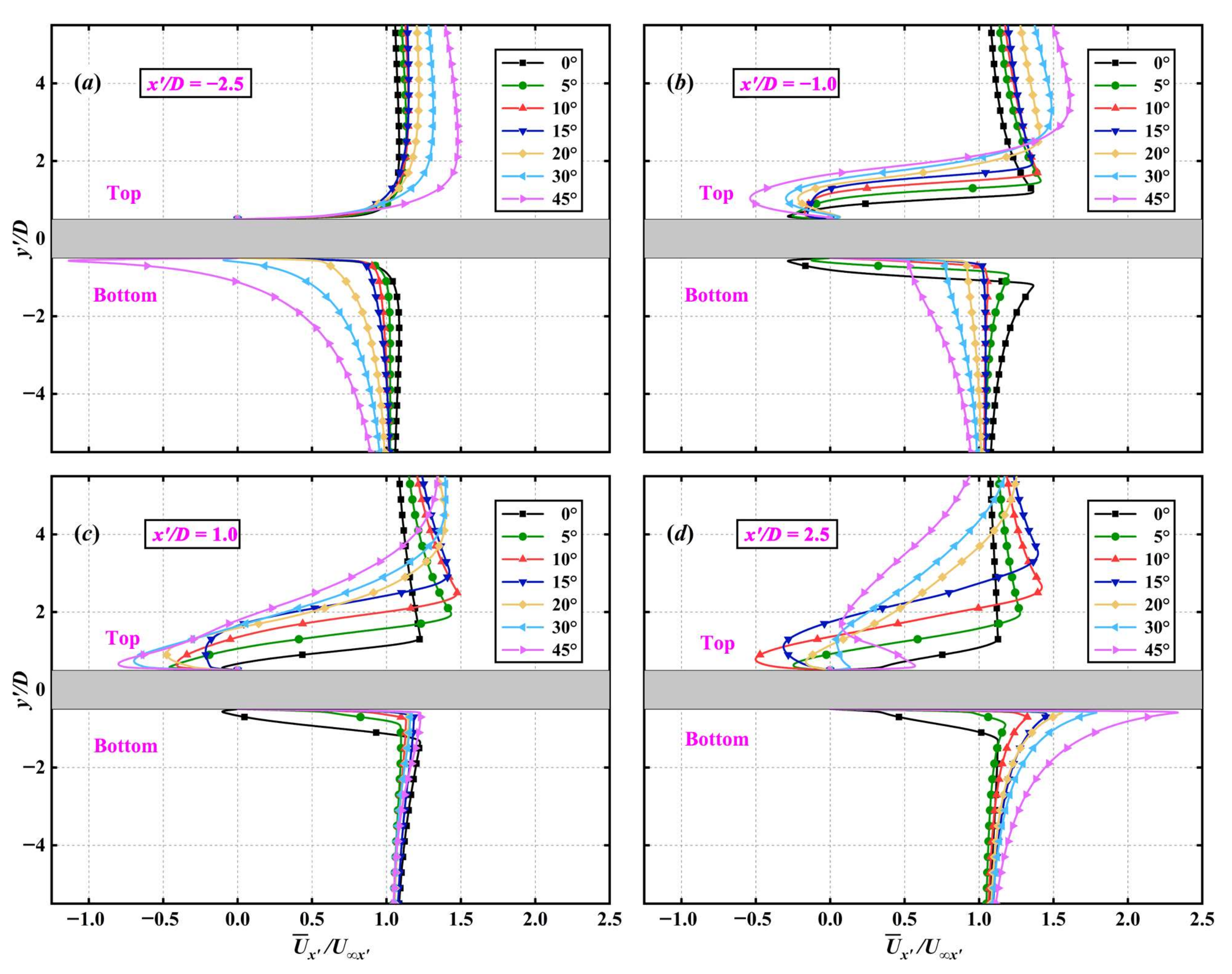
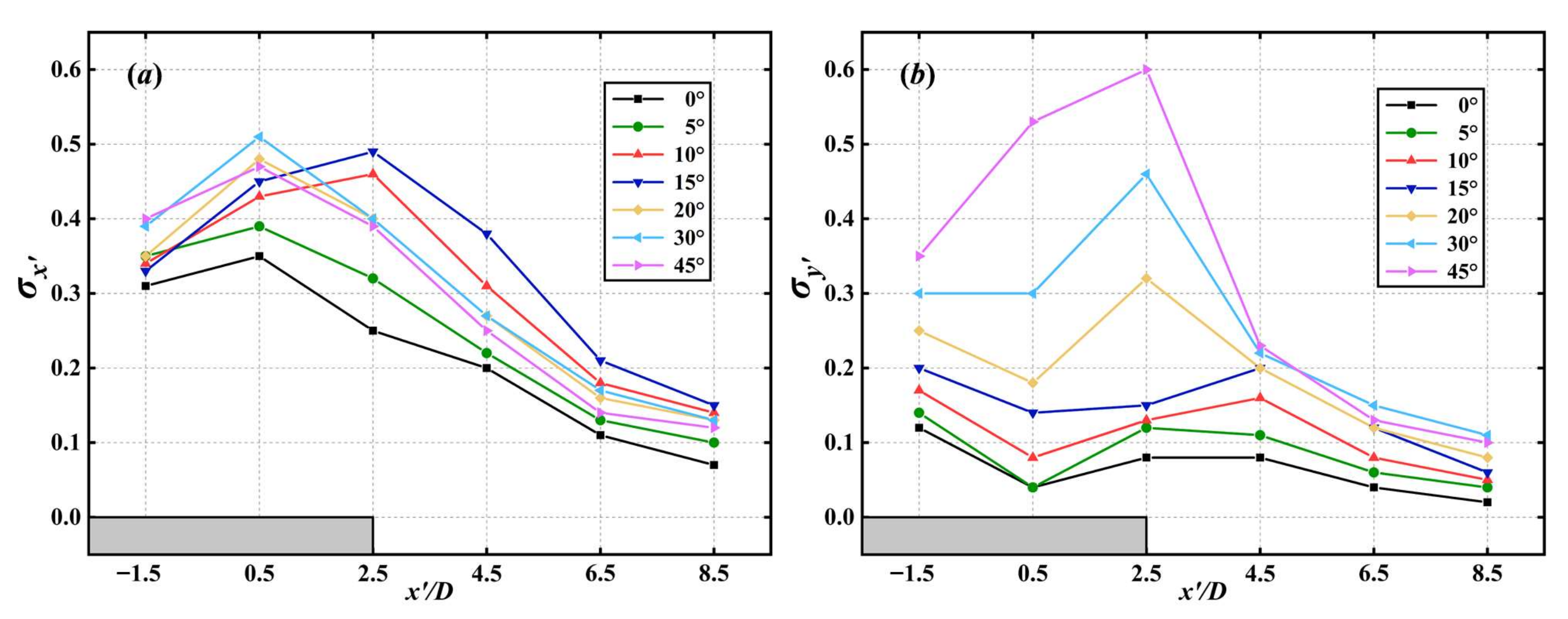
3.3.1. Mean and Fluctuating Velocity Distribution in the Flow Field
3.3.2. Pressure Coefficient Distribution over the Cylinder Surface
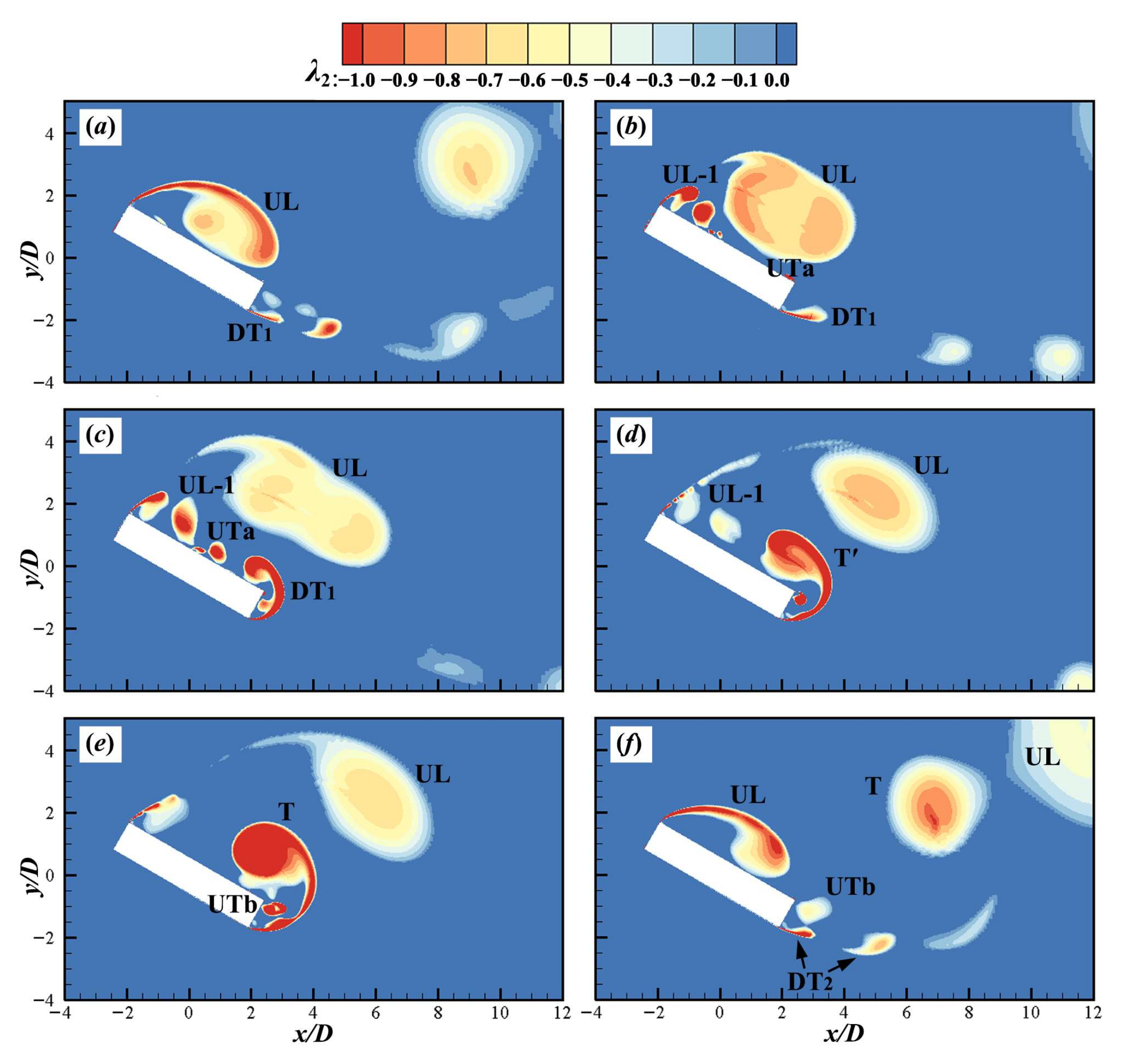
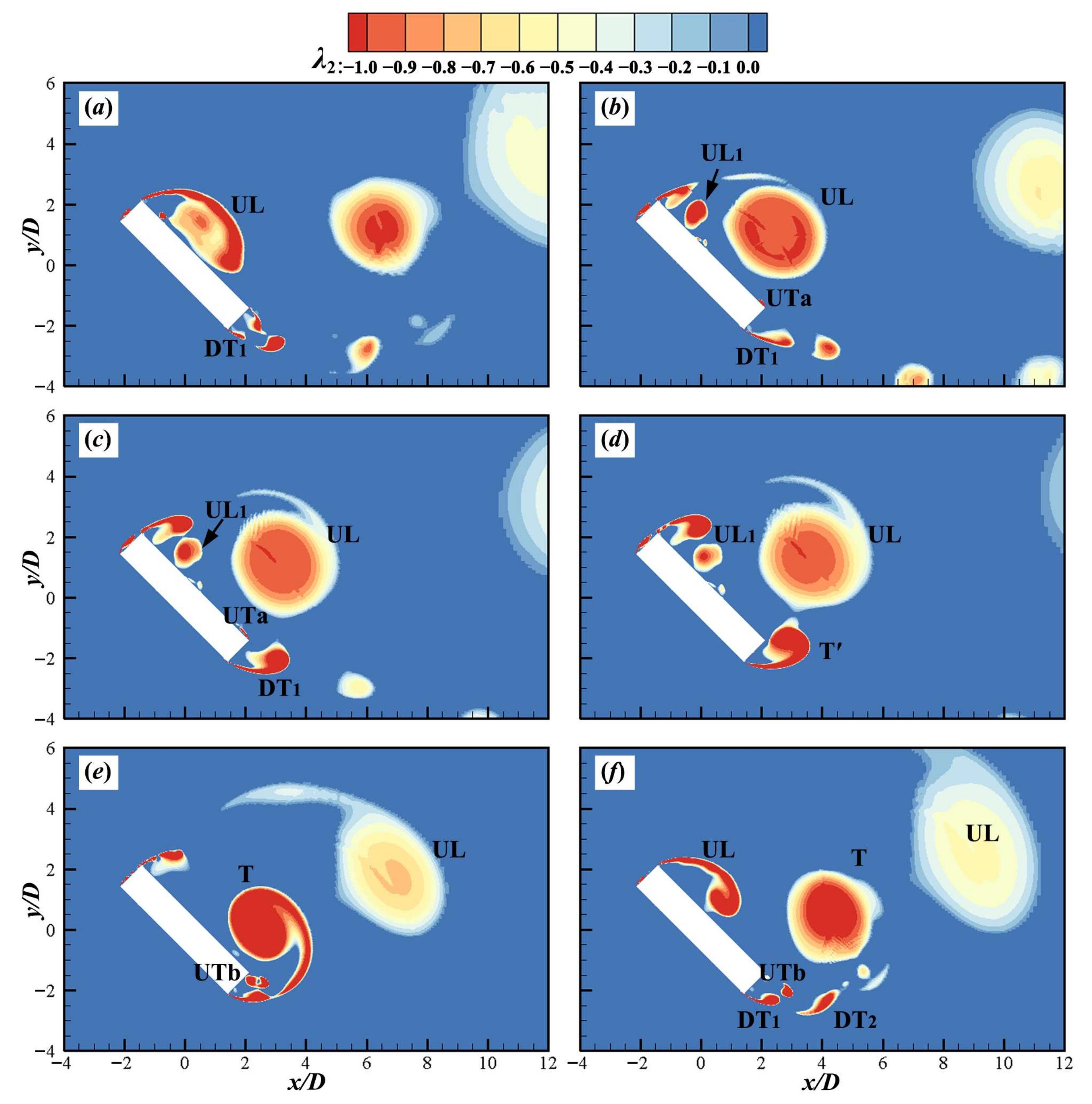
3.4. Vortex Shedding Modes
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bruno, L.; Fransos, D.; Coste, N.; Bosco, A. 3D flow around a rectangular cylinder: A computational study. J. Wind Eng. Ind. Aerod. 2010, 98, 263–276. [Google Scholar] [CrossRef]
- Bruno, L.; Coste, N.; Fransos, D. Simulated flow around a rectangular 5:1 cylinder: Spanwise discretisation effects and emerging flow features. J. Wind Eng. Ind. Aerod. 2012, 104, 203–215. [Google Scholar] [CrossRef]
- Schewe, G. Reynolds-number-effects in flow around a rectangular cylinder with aspect ratio 1:5. J. Fluid Struct. 2013, 39, 15–26. [Google Scholar] [CrossRef]
- Patruno, L.; Ricci, M.; De Miranda, S.; Ubertini, F. Numerical simulation of a 5:1 rectangular cylinder at non-null angles of attack. J. Wind Eng. Ind. Aerod. 2016, 151, 146–157. [Google Scholar] [CrossRef]
- Mannini, C.; Marra, A.M.; Pigolotti, L.; Bartoli, G. The effects of free-stream turbulence and angle of attack on the aerodynamics of a cylinder with rectangular 5:1 cross section. J. Wind Eng. Ind. Aerod. 2017, 161, 42–58. [Google Scholar] [CrossRef]
- Ricci, M.; Patruno, L.; de Miranda, S.; Ubertini, F. Flow field around a 5:1 rectangular cylinder using LES: Influence of inflow turbulence conditions, spanwise domain size and their interaction. Comput. Fluids 2017, 149, 181–193. [Google Scholar] [CrossRef]
- Wu, B.; Li, S.; Cao, S.; Yang, Q.; Zhang, L. Numerical investigation of the separated and reattaching flow over a 5:1 rectangular cylinder in streamwise sinusoidal flow. J. Wind Eng. Ind. Aerodyn. 2020, 198, 104120. [Google Scholar] [CrossRef]
- Wu, B.; Li, S.; Li, K.; Zhang, L. Numerical and experimental studies on the aerodynamics of a 5:1 rectangular cylinder at angles of attack. J. Wind Eng. Ind. Aerodyn. 2020, 199, 104097. [Google Scholar] [CrossRef]
- Lou, X.; Sun, C.; Jiang, H.; Zhu, H.; An, H.; Zhou, T. Three-Dimensional Direct Numerical Simulations of a Yawed Square Cylinder in Steady Flow. J. Mar. Sci. Eng. 2022, 10, 1128. [Google Scholar] [CrossRef]
- Li, D.; Yang, Q.; Ma, X.; Dai, G. Free Surface Characteristics of Flow around Two Side-by-Side Circular Cylinders. J. Mar. Sci. Eng. 2018, 6, 75. [Google Scholar] [CrossRef]
- Han, X.; Wang, J.; Zhou, B.; Zhang, G.; Tan, S.-K. Numerical Simulation of Flow Control around a Circular Cylinder by Installing a Wedge-Shaped Device Upstream. J. Mar. Sci. Eng. 2019, 7, 422. [Google Scholar] [CrossRef]
- Wang, W.; Mao, Z.; Tian, W.; Zhang, T. Numerical Investigation on Vortex-Induced Vibration Suppression of a Circular Cylinder with Axial-Slats. J. Mar. Sci. Eng. 2019, 7, 454. [Google Scholar] [CrossRef]
- Piran, F.; Karampour, H.; Woodfield, P. Numerical Simulation of Cross-Flow Vortex-Induced Vibration of Hexagonal Cylinders with Face and Corner Orientations at Low Reynolds Number. J. Mar. Sci. Eng. 2020, 8, 387. [Google Scholar] [CrossRef]
- Anwar, M.U.; Lashin, M.M.A.; Khan, N.B.; Munir, A.; Jameel, M.; Muhammad, R.; Guedri, K.; Galal, A.M. Effect of Variation in the Mass Ratio on Vortex-Induced Vibration of a Circular Cylinder in Crossflow Direction at Reynold Number = 104: A Numerical Study Using RANS Model. J. Mar. Sci. Eng. 2022, 10, 1126. [Google Scholar] [CrossRef]
- Nazvanova, A.; Yin, G.; Ong, M.C. Numerical Investigation of Flow around Two Tandem Cylinders in the Upper Transition Reynolds Number Regime Using Modal Analysis. J. Mar. Sci. Eng. 2022, 10, 1501. [Google Scholar] [CrossRef]
- Taheri, E.; Zhao, M.; Wu, H. Numerical Investigation of the Vibration of a Circular Cylinder in Oscillatory Flow in Oblique Directions. J. Mar. Sci. Eng. 2022, 10, 767. [Google Scholar] [CrossRef]
- Wang, T.; Yang, Q.; Tang, Y.; Shi, H.; Zhang, Q.; Wang, M.; Epikhin, A.; Britov, A. Spectral Analysis of Flow around Single and Two Crossing Circular Cylinders Arranged at 60 and 90 Degrees. J. Mar. Sci. Eng. 2022, 10, 811. [Google Scholar] [CrossRef]
- Bartoli, G.; Bruno, L.; Buresti, G.; Ricciardelli, F.; Salvetti, M.V.; Zasso, A. BARC Overview Document. 2008. Available online: http://www.aniv-iawe.org/barc (accessed on 17 July 2020).
- Bruno, L.; Salvetti, M.V.; Ricciardelli, F. Benchmark on the Aerodynamics of a Rectangular 5:1 Cylinder: An overview after the first four years of activity. J. Wind Eng. Ind. Aerodyn. 2014, 126, 87–106. [Google Scholar] [CrossRef]
- Álvareza, A.J.; Nietoa, F.; Nguyen, D.T.; Owen, J.S.; Hernándeza, S. 3D LES simulations of a static and vertically free-to-oscillate 4:1 rectangular cylinder: Effects of the grid resolution. J. Wind Eng. Ind. Aerodyn. 2019, 192, 31–44. [Google Scholar] [CrossRef]
- Zhang, Z.; Xu, F. Spanwise length and mesh resolution effects on simulated flow around a 5:1 rectangular cylinder. J. Wind Eng. Ind. Aerodyn. 2020, 202, 104186. [Google Scholar] [CrossRef]
- Tang, Y.; Hui, Y.; Li, K. LES study on variation of flow pattern around a 4:1 rectangular cylinder and corresponding wind load during VIV. J. Wind Eng. Ind. Aerodyn. 2022, 228, 105121. [Google Scholar] [CrossRef]
- Mannini, C.; Soda, A.; Voss, R.; Schewe, G. URANS and DES simulation of flow around a rectangular cylinder. New Res. Num. Exp. Fluid Mech. VI 2007, 96, 36–43. [Google Scholar]
- Mannini, C. Applicability of URANS and DES simulations of flow past rectangular cylinders and bridge sections. Computation 2015, 3, 479–508. [Google Scholar] [CrossRef]
- Bai, W.; Mingham, C.G.; Causon, D.M.; Qian, L. Detached eddy simulation of turbulent flow around square and circular cylinders on Cartesian cut cells. Ocean Eng. 2016, 117, 1–14. [Google Scholar] [CrossRef]
- Hong, F.; Xue, H.; Zhang, B. Improved detached-eddy simulation of the turbulent unsteady flow past a square cylinder. AIP Adv. 2020, 10, 125011. [Google Scholar] [CrossRef]
- Yousif, M.Z.; Lim, H. Improved delayed detached-eddy simulation and proper orthogonal decomposition analysis of turbulent wake behind a wall-mounted square cylinder. AIP Adv. 2021, 11, 045011. [Google Scholar] [CrossRef]
- Zhang, D. Comparison of Various Turbulence Models for Unsteady Flow around a Finite Circular Cylinder at Re = 20,000. IOP Conf. Ser. J. Phys. Conf. Ser. 2017, 910, 012027. [Google Scholar] [CrossRef]
- Nietoa, F.; Hargreavesb, D.M.; Owenb, J.S.; Hernándeza, S. On the applicability of 2D URANS and SST k-ω turbulence model to the fluid-structure interaction of rectangular cylinders. Eng. Appl. Comput. Fluid Mech. 2015, 9, 157–173. [Google Scholar]
- Gorle, J.M.R.; Chatellier, L.; Pons, F.; Ba, M. Flow and performance analysis of H-Darrieus hydroturbine in a confined flow: A computational and experimental study. J. Fluids Struct. 2016, 66, 382–402. [Google Scholar] [CrossRef]
- Gorle, J.M.R.; Chatellier, L.; Pons, F.; Ba, M. Modulated circulation control around the blades of a vertical axis hydrokinetic turbine for flow control and improved performance. Renew. Sustain. Energy Rev. 2019, 105, 363–377. [Google Scholar] [CrossRef]
- Mannini, C.; Šoda, A.; Schewe, G. Unsteady RANS modelling of flow past a rectangular cylinder: Investigation of Reynolds number effects. Comput. Fluids 2010, 39, 1609–1624. [Google Scholar] [CrossRef]
- Matsumoto, M.; Yagi, T.; Tamaki, H.; Tsubota, T. Vortex-induced vibration and its effect on torsional flutter instability in the case of B/D=4 rectangular cylinder. J. Wind Eng. Ind. Aerodyn. 2008, 96, 971–983. [Google Scholar] [CrossRef]
- Zhang, Q.; Liu, Y. Separated flow over blunt plates with different chord-to-thickness ratios: Unsteady behaviors and wall-pressure fluctuations. Exp. Therm. Fluid Sci. 2017, 84, 199–216. [Google Scholar] [CrossRef]
- Carassale, L. Flow-induced actions on cylinders in statistically-symmetric cross flow. Probab. Eng. Mech. 2009, 24, 288–299. [Google Scholar] [CrossRef]
- Menter, F.R. Zonal two equation k-ω turbulence models for aerodynamic flows. In Proceedings of the 23rd Fluid Dynamics, Plasmadynamics, and Lasers Conference, Orlando, FL, USA, 6–9 July 1993; Volume 93, p. 2906. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Menter, F.R.; Kuntz, M.; Langtry, R. Ten years of industrial experience with the SST turbulence model. Turbul. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- Zhang, D.; Cheng, L.; An, H.; Draper, S. Flow around a surface-mounted finite circular cylinder completely submerged within the bottom boundary layer. Eur. J. Mech. B-Fluid. 2021, 86, 169–197. [Google Scholar] [CrossRef]
- Bruno, L.; Salvetti, M.V. Benchmark on the Aerodynamics of a Rectangular 5:1 Cylinder (BARC): Description, Test Case Studies, Evaluation, and Best Practice Advice. 2017. Available online: http://www.kbwiki.ercoftac.org/w/index.php/Abstr:UFR_2-15 (accessed on 24 October 2020).
- Galli, F. Aerodynamic Behaviour of Bluff Line-like Structures: Experimental and Computational Approach. Master’s Thesis, Politecnico di Torino, Turin, Italy, 2005. [Google Scholar]
- Ribeiro, A.F.P. Unsteady RANS modelling of flow past a rectangular 5:1 cylinder: Investigation of edge sharpness effects. In Proceedings of the 13th International Conference on Wind Engineering, Amsterdam, The Netherlands, 10–15 July 2011. [Google Scholar]
- Grozescu, A.N.; Bruno, L.; Fransos, D.; Salvetti, M.V. Large-eddy simulations of a Benchmark on the Aerodynamics of a Rectangular 5:1 Cylinder. In Proceedings of the 20th Italian Conference on Theoretical and Applied Mechanics, Bologna, Italy, 12–15 September 2011; Available online: https://arpi.unipi.it/handle/11568/238156 (accessed on 24 October 2020).
- Grozescu, A.N.; Salvetti, M.V.; Camarri, S.; Buresti, G. Variational multiscale large-eddy simulations of the BARC flow configuration. In Proceedings of the 13th International Conference on Wind Engineering, Amsterdam, The Netherlands, 12–15 September 2011. [Google Scholar]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]


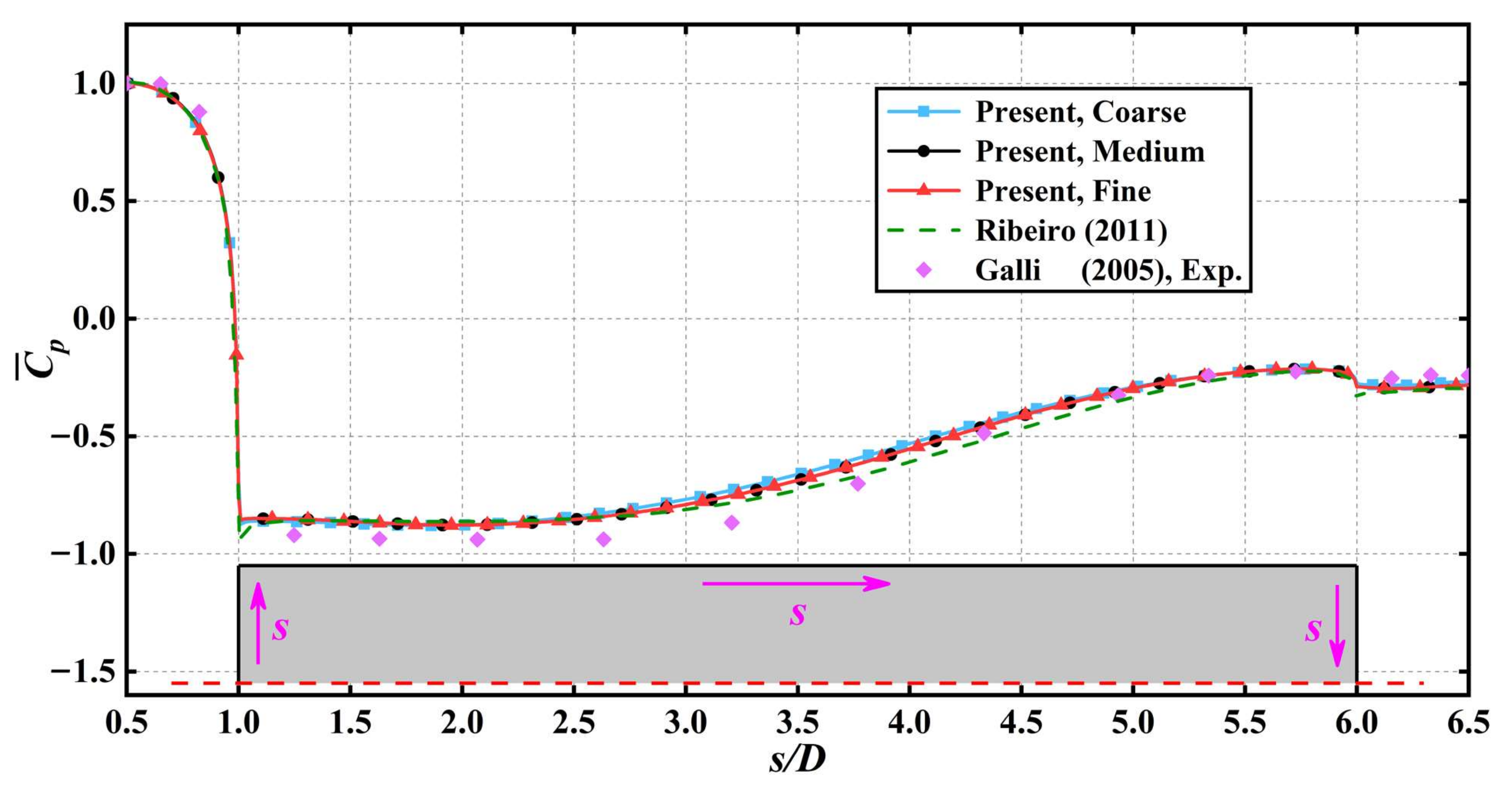

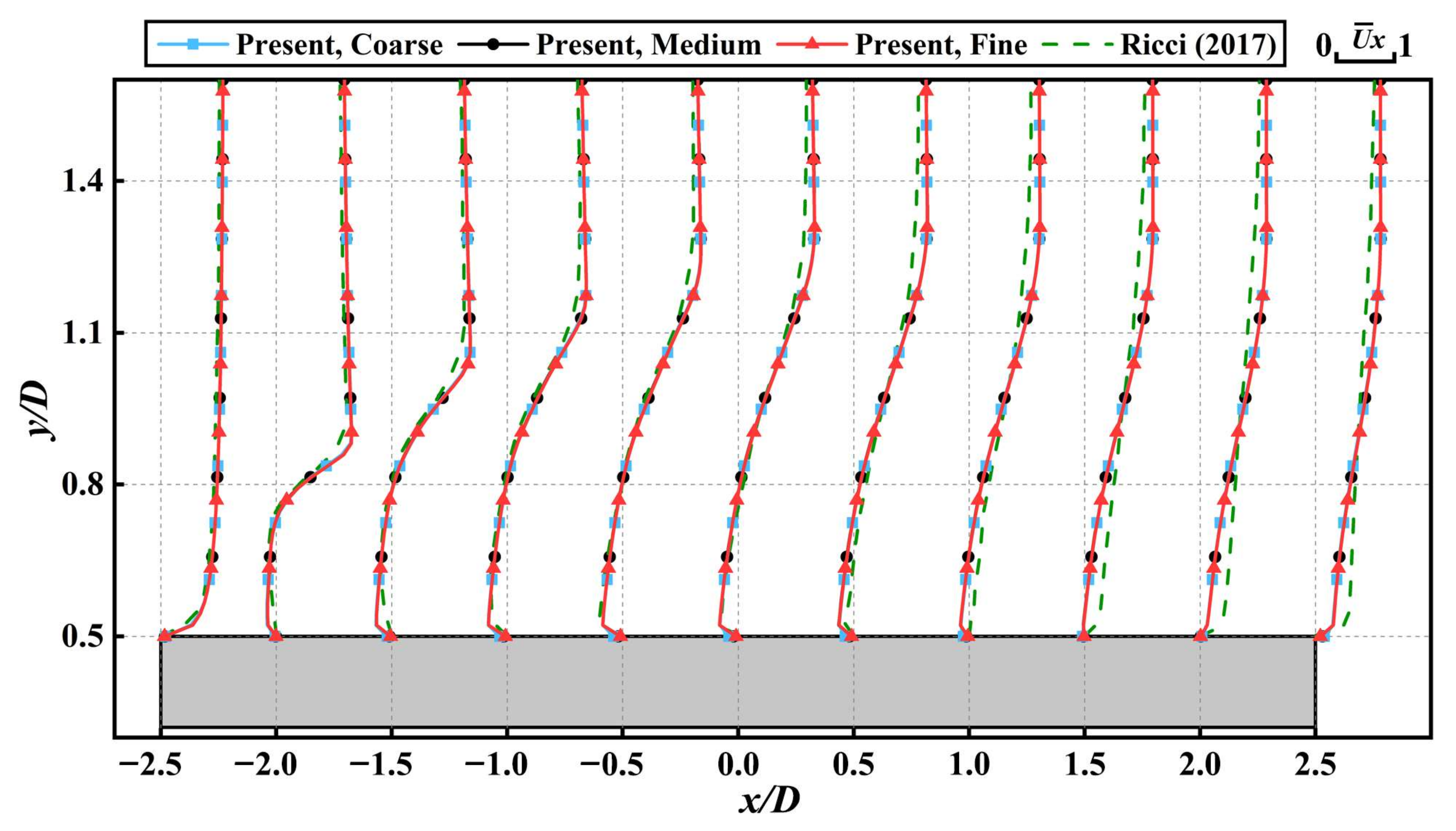

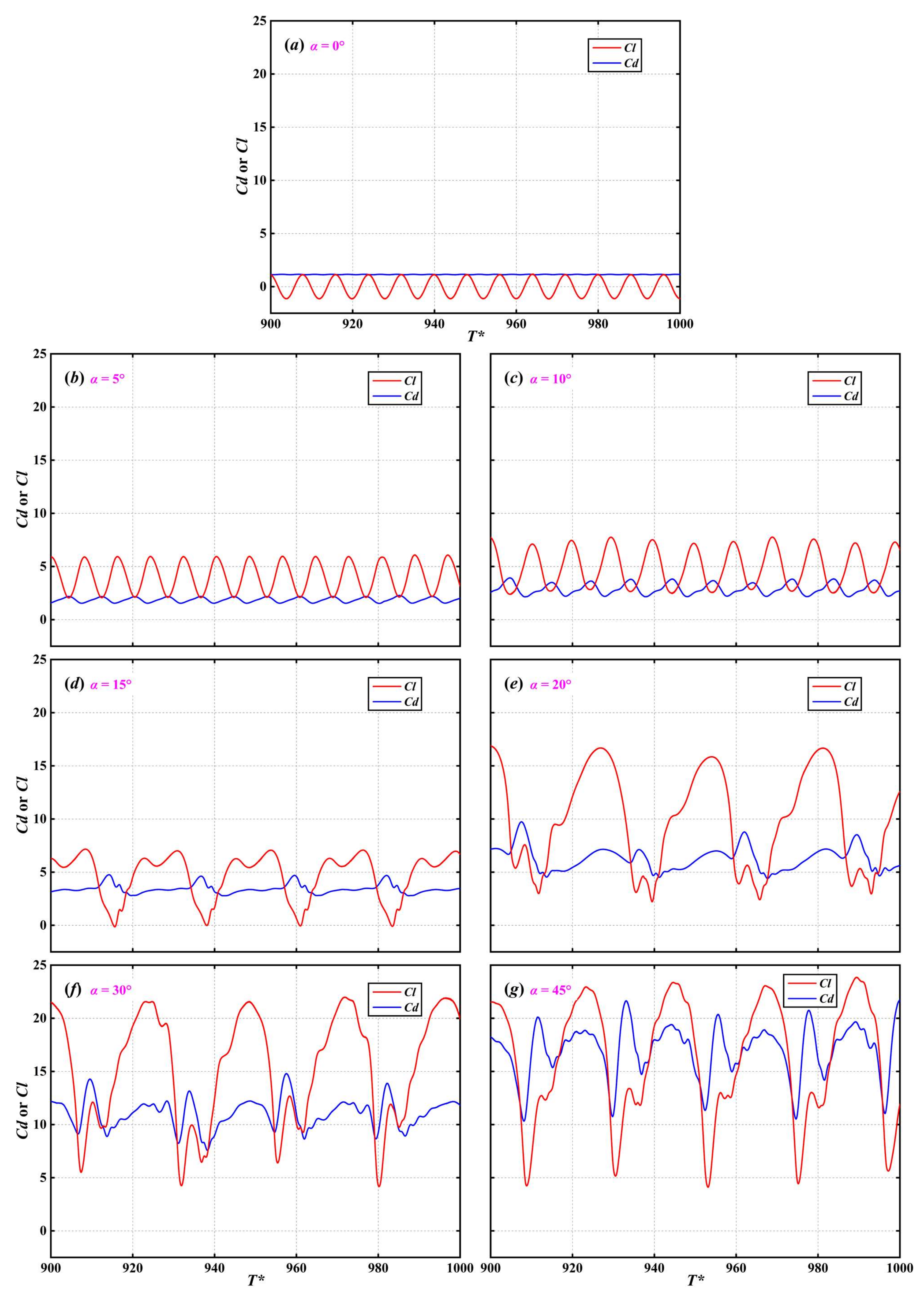



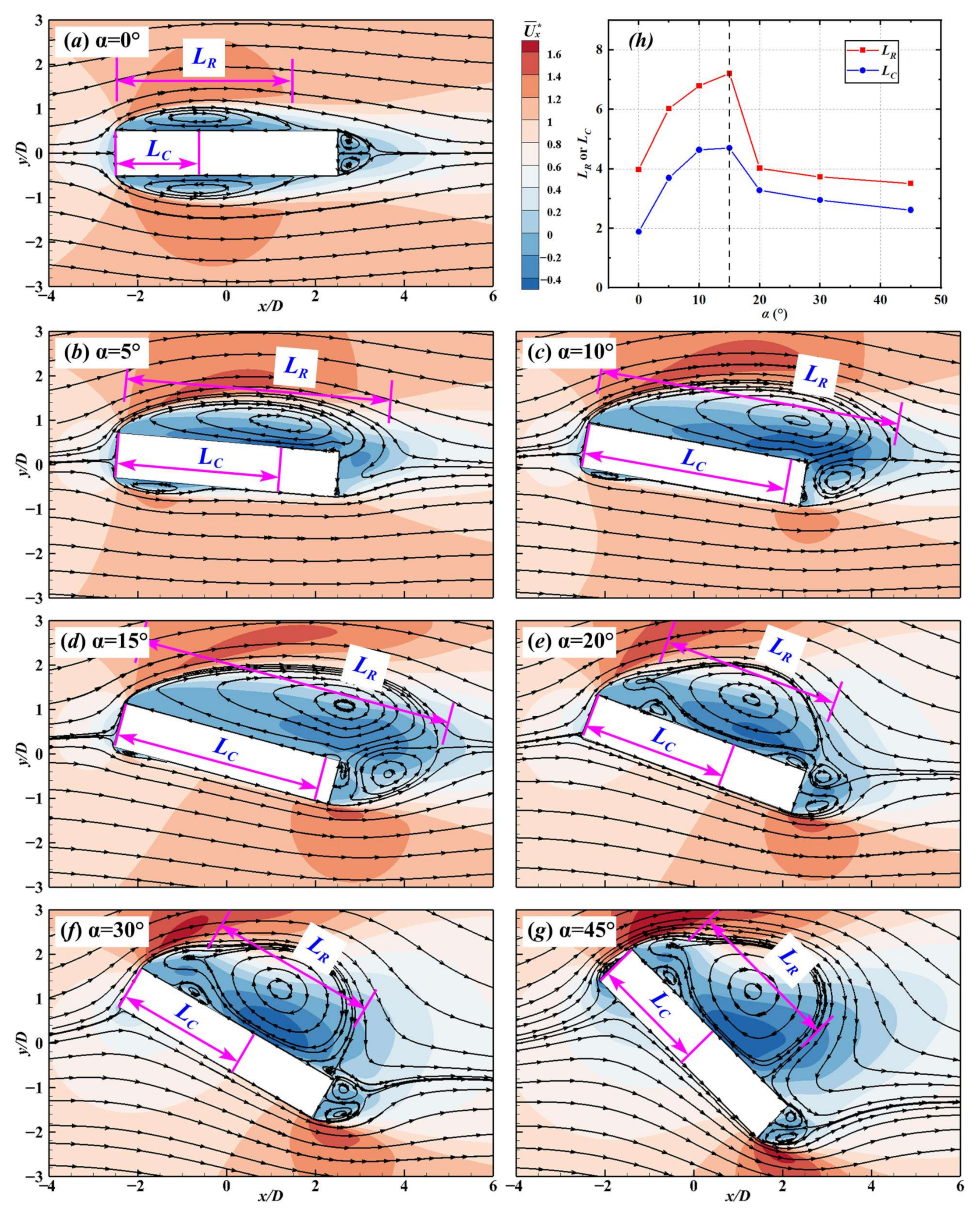
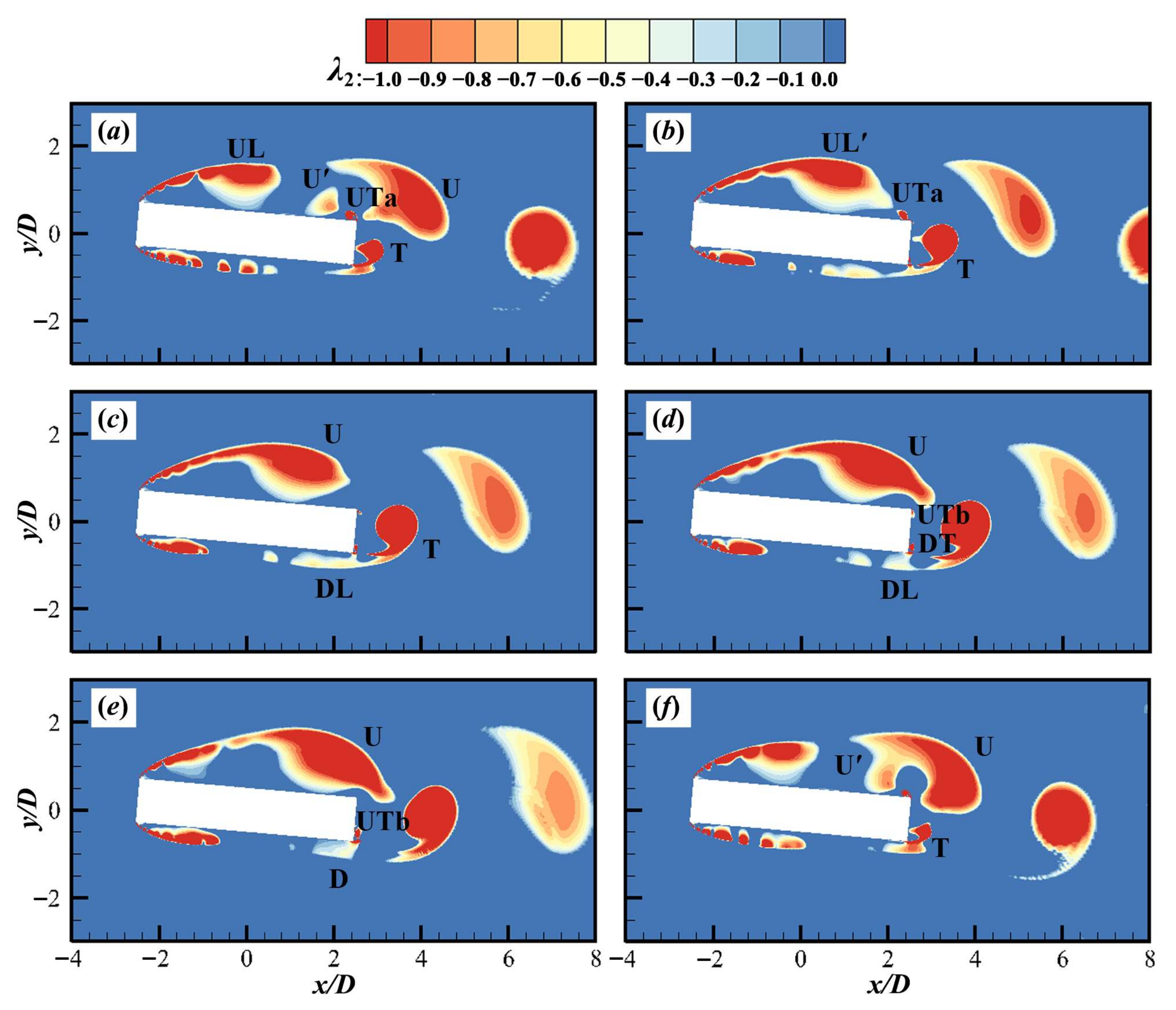
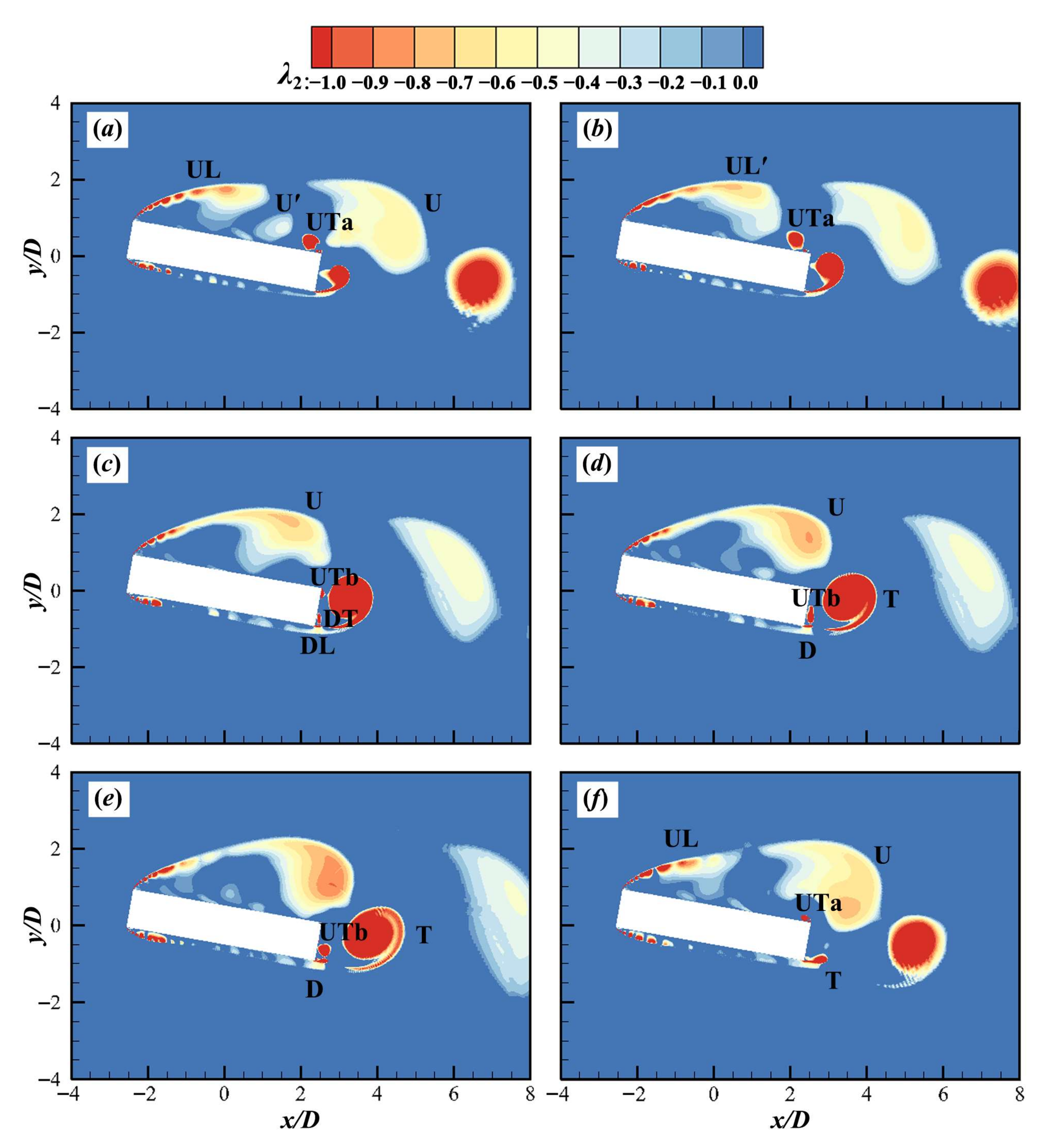
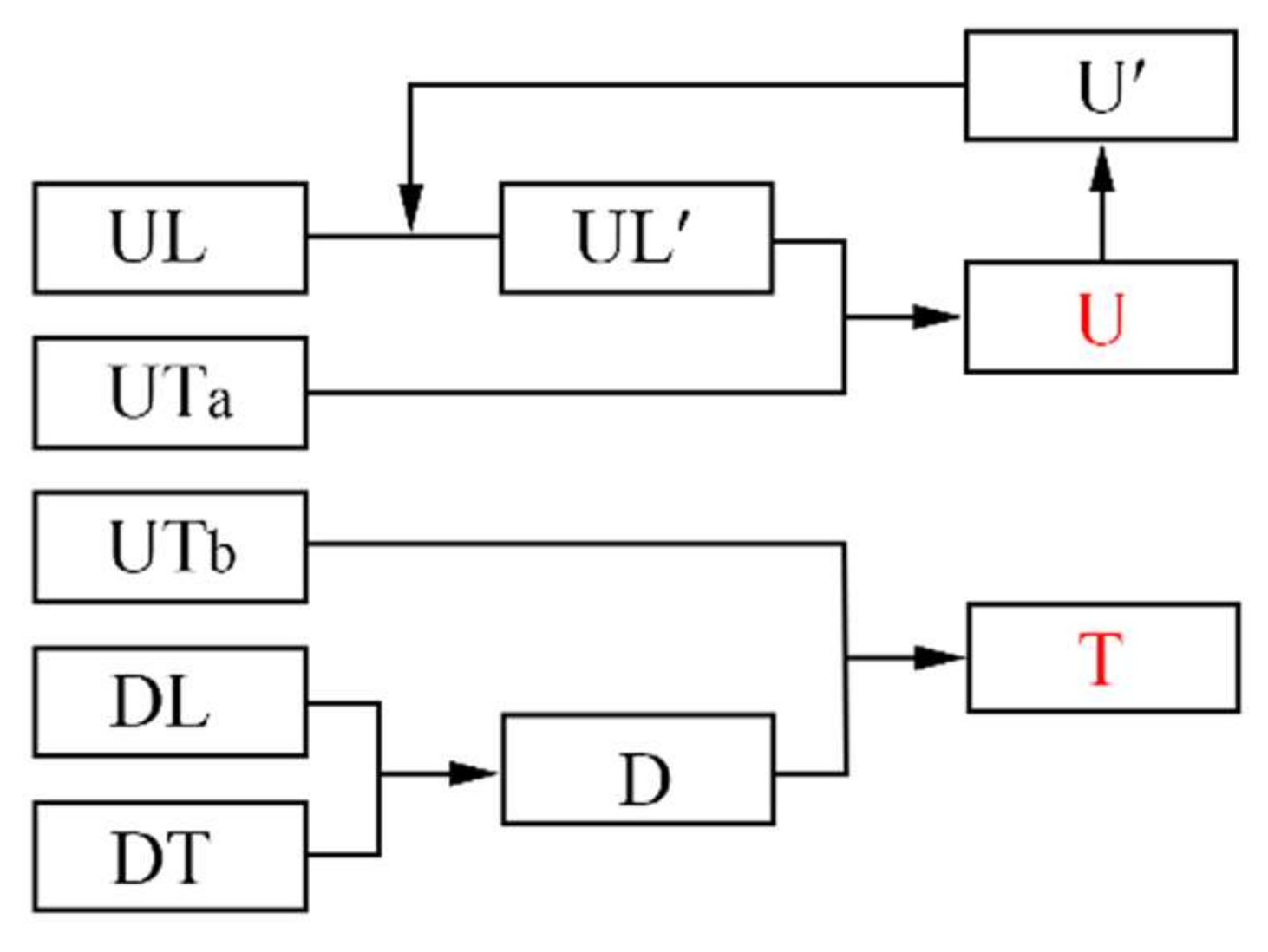
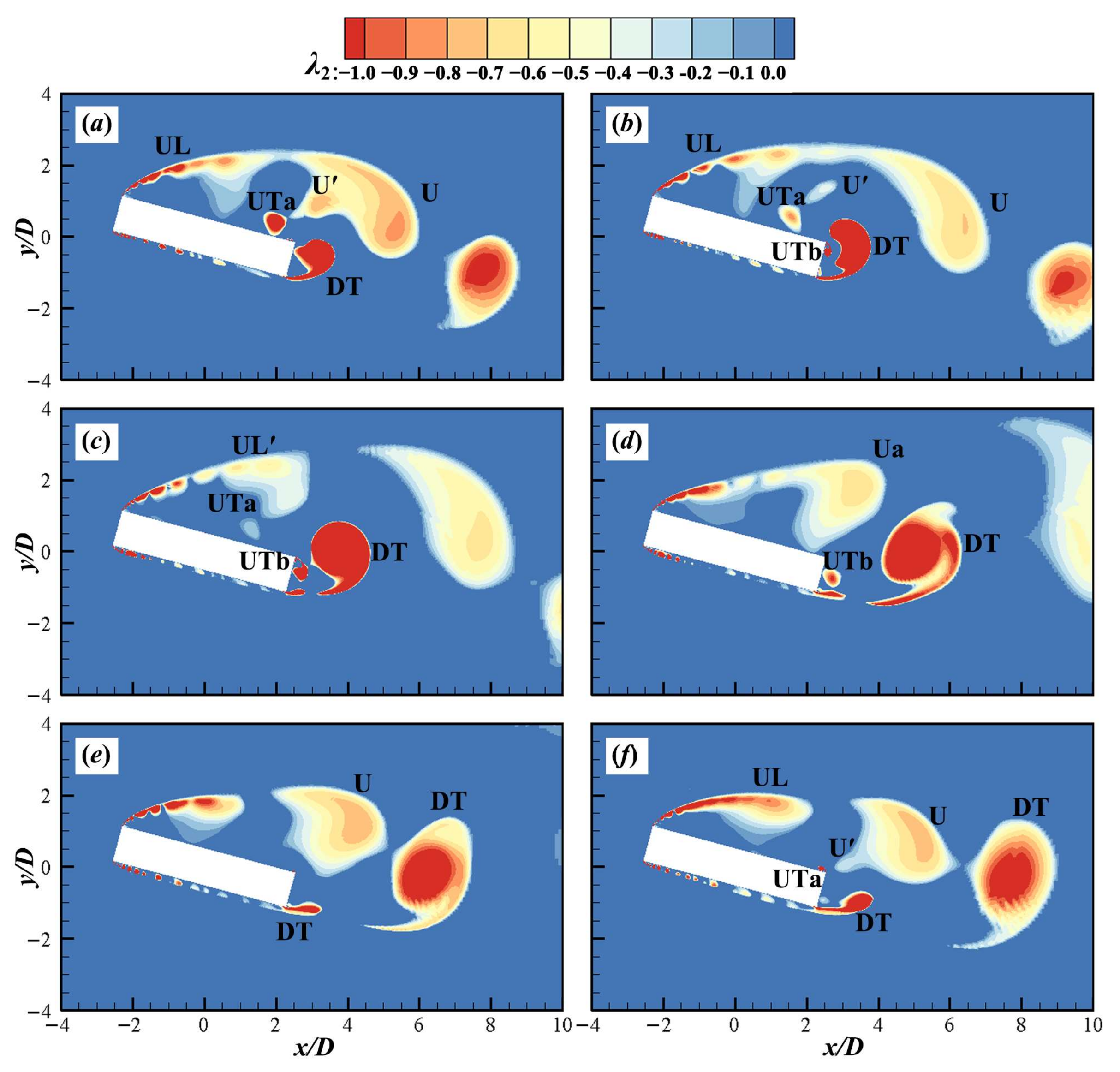




| Cases | Cells Number (105) | δ/D (10−3) | Δt* (10−4) | y+ | Wall Nodes | |
|---|---|---|---|---|---|---|
| Core Region | Total | |||||
| 0°-Coarse | 245,100 | 583,280 | 4 | 10 | ~2 | 1840 |
| 0°-Medium | 347,300 | 984,910 | 2 | 6 | ~1 | 2080 |
| 0°-Fine | 425,800 | 1,182,960 | 1 | 3 | ~0.65 | 2280 |
| 5° | 347,300 | 984,830 | 2 | 6 | ~1 | 2080 |
| 10°, 15°, 20° | 347,300 | 983,180 * | 2 | 5 | ~1 | 2080 |
| 30°, 45° | 347,300 | 982,050 * | 2 | 4 | ~1 | 2080 |
| Cases | St | |||
|---|---|---|---|---|
| α = 0°-Coarse | −0.01 | 0.825 | 1.146 | 0.123 |
| α = 0°-Medium | −0.006 | 0.827 | 1.145 | 0.122 |
| α = 0°-Fine | −0.006 | 0.821 | 1.143 | 0.121 |
| Bruno et al. [2] | −0.21 | / | 0.98 | 0.12 |
| Ribeiro et al. [42] | / | 0.9 | 1.17 | / |
| Case | Xc | Yc | Xr | Xs |
|---|---|---|---|---|
| α = 0°-Coarse | −0.68 | 0.81 | 1.71 | 3.24 |
| α = 0°-Medium | −0.65 | 0.81 | 1.69 | 3.24 |
| α = 0°-Fine | −0.63 | 0.80 | 1.69 | 3.24 |
| Grozescu et al. [43,44] | −0.88 | 0.78 | 1.63 | 3.3 |
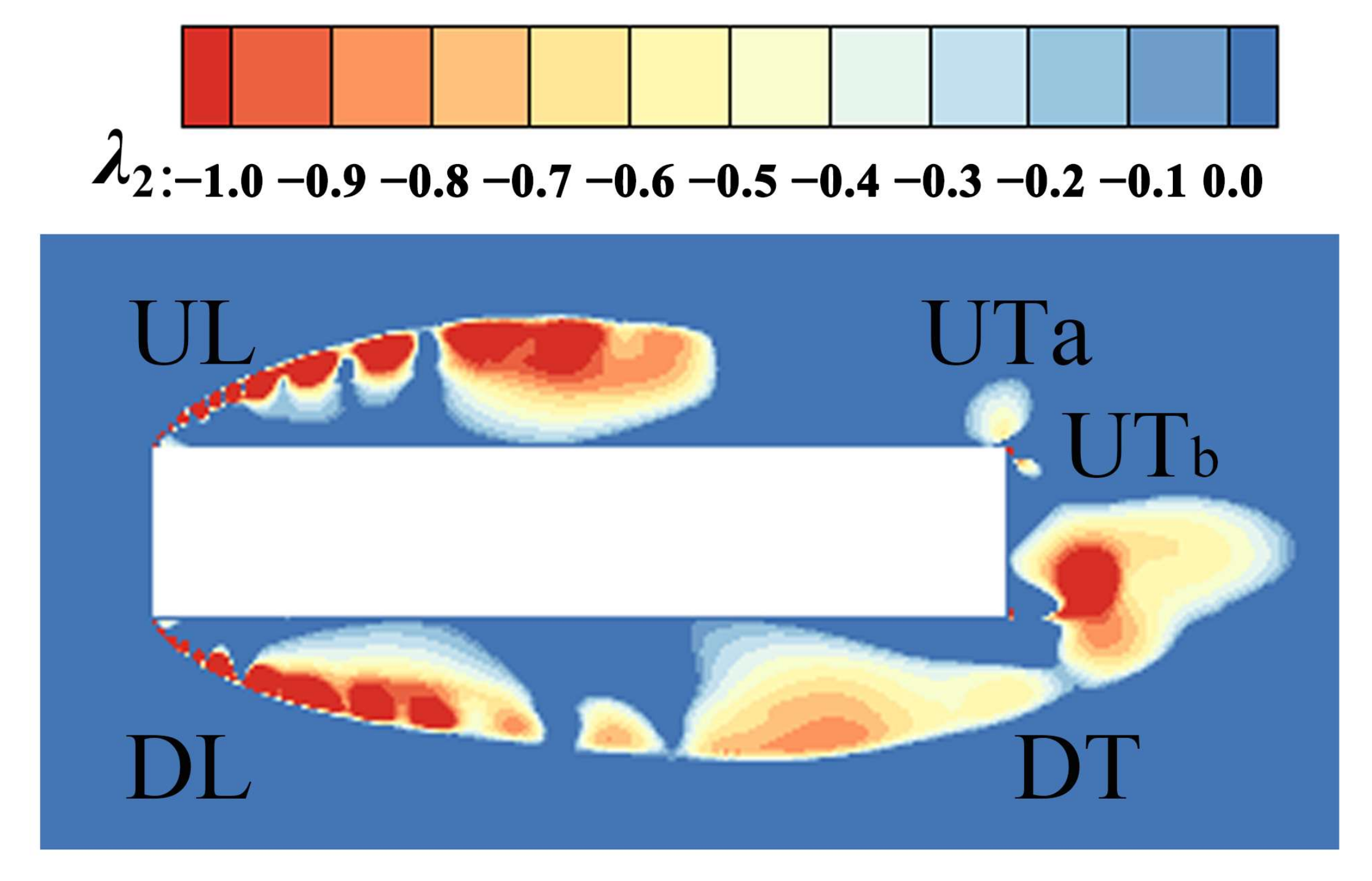
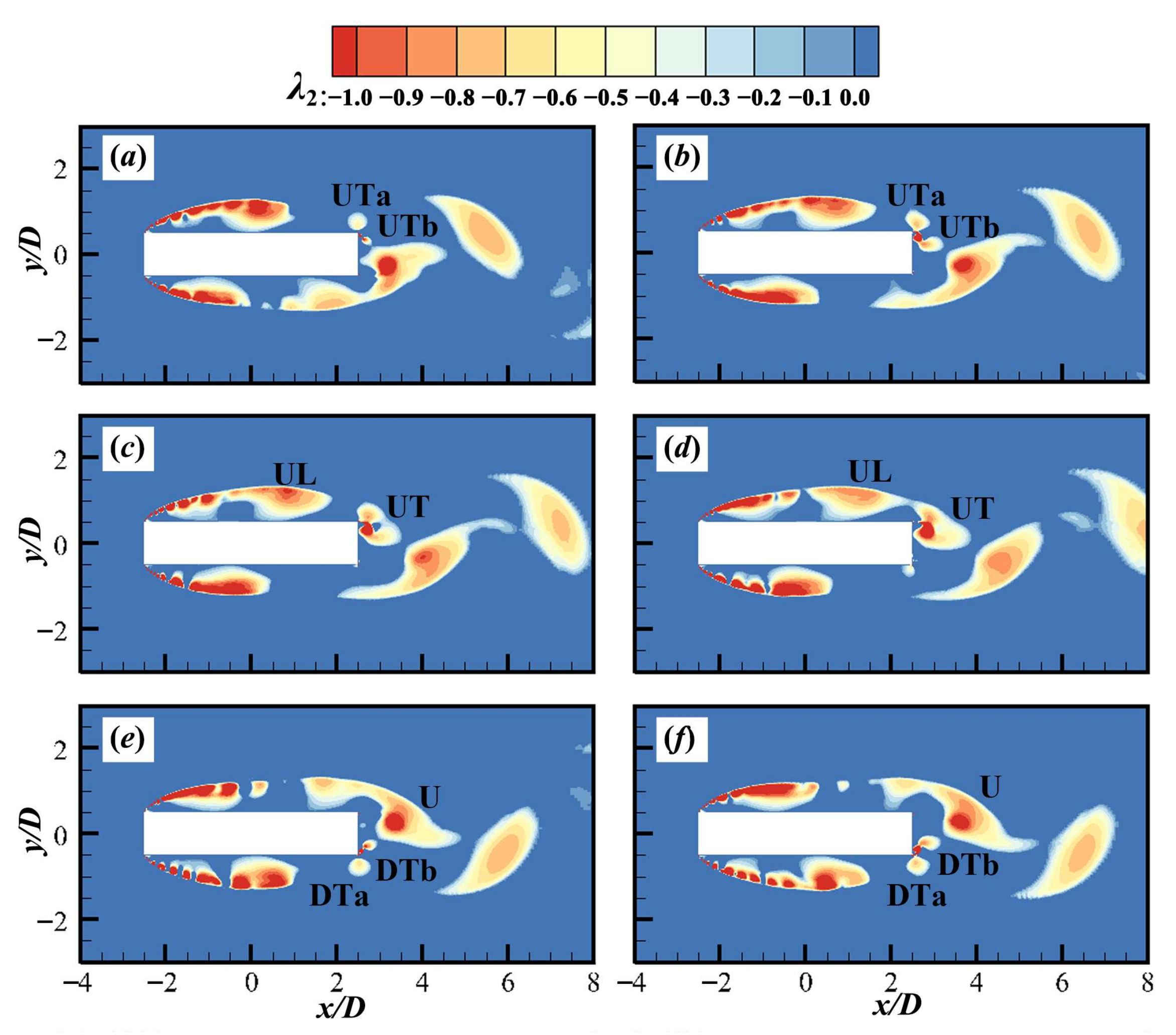
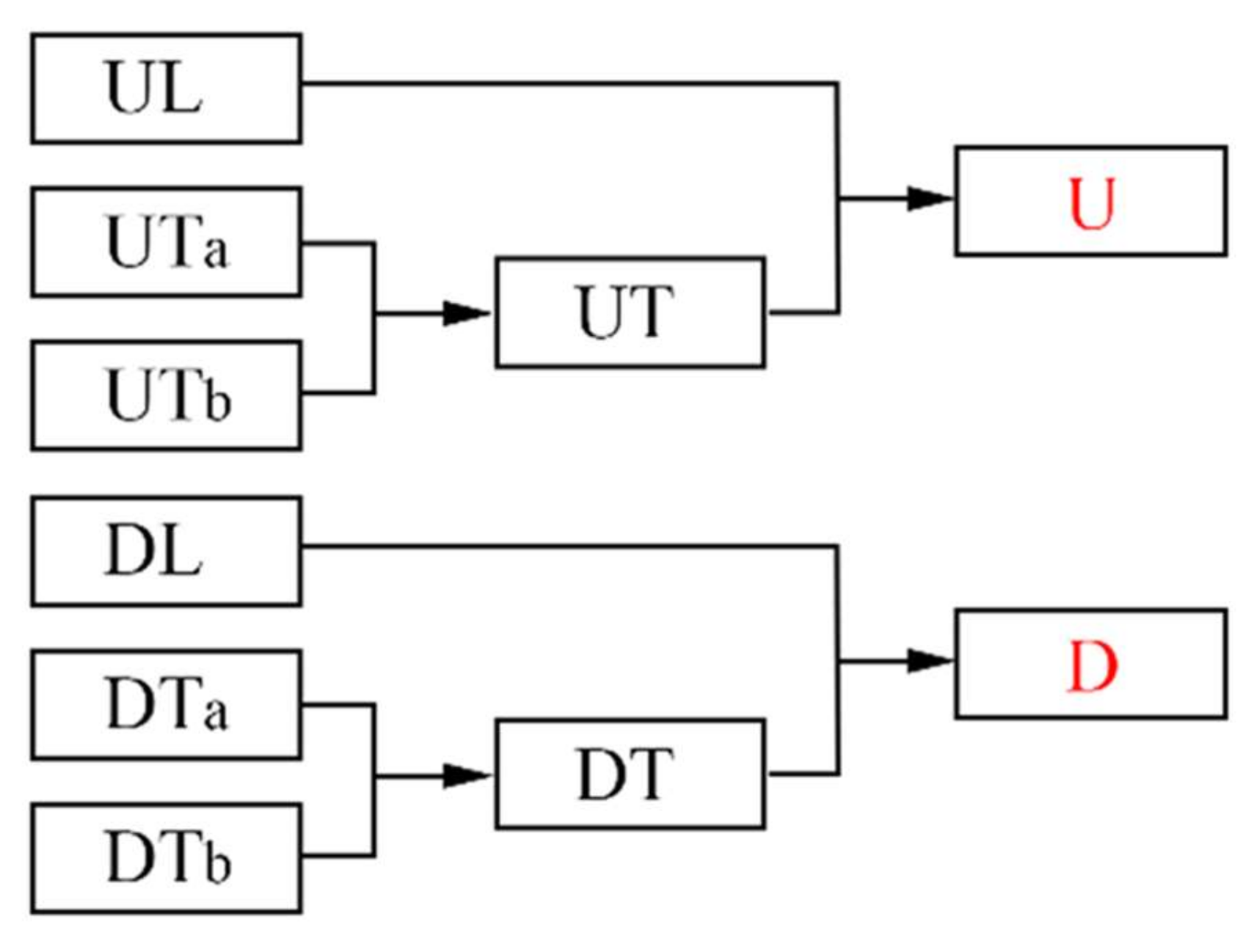
| α | Mode | UT Characteristic | UL Characteristic | Vortex Street Characteristic |
|---|---|---|---|---|
| 0° | U-D | UTa & UTb | The UL-vortex flaps slightly. | “1 + 1” Kármán vortex street |
| 5° | U-T | UTa & UL | ||
| 10° | UTb & DT | |||
| 15° | U-DT | UT & UL | ||
| 20° | UL-T-U-DT | UTa & DT | The UL-vortex flaps and generates a secondary vortex. | “2 + 2” Kármán vortex street |
| 30° | UL-T- UTb-DT | UTb & UL | ||
| 45° | UTa & DT |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, J.; Liu, Y.; Zhang, D.; Cao, Z.; Guo, Z. Numerical Investigation of Vortex Shedding from a 5:1 Rectangular Cylinder at Different Angles of Attack. J. Mar. Sci. Eng. 2022, 10, 1913. https://doi.org/10.3390/jmse10121913
Wu J, Liu Y, Zhang D, Cao Z, Guo Z. Numerical Investigation of Vortex Shedding from a 5:1 Rectangular Cylinder at Different Angles of Attack. Journal of Marine Science and Engineering. 2022; 10(12):1913. https://doi.org/10.3390/jmse10121913
Chicago/Turabian StyleWu, Jian, Yakun Liu, Di Zhang, Ze Cao, and Zijun Guo. 2022. "Numerical Investigation of Vortex Shedding from a 5:1 Rectangular Cylinder at Different Angles of Attack" Journal of Marine Science and Engineering 10, no. 12: 1913. https://doi.org/10.3390/jmse10121913
APA StyleWu, J., Liu, Y., Zhang, D., Cao, Z., & Guo, Z. (2022). Numerical Investigation of Vortex Shedding from a 5:1 Rectangular Cylinder at Different Angles of Attack. Journal of Marine Science and Engineering, 10(12), 1913. https://doi.org/10.3390/jmse10121913





