Risk Assessment of Wave Over-Topping Based on Smoothed-Particle Hydrodynamics Method
Abstract
1. Introduction
2. Models and Methods
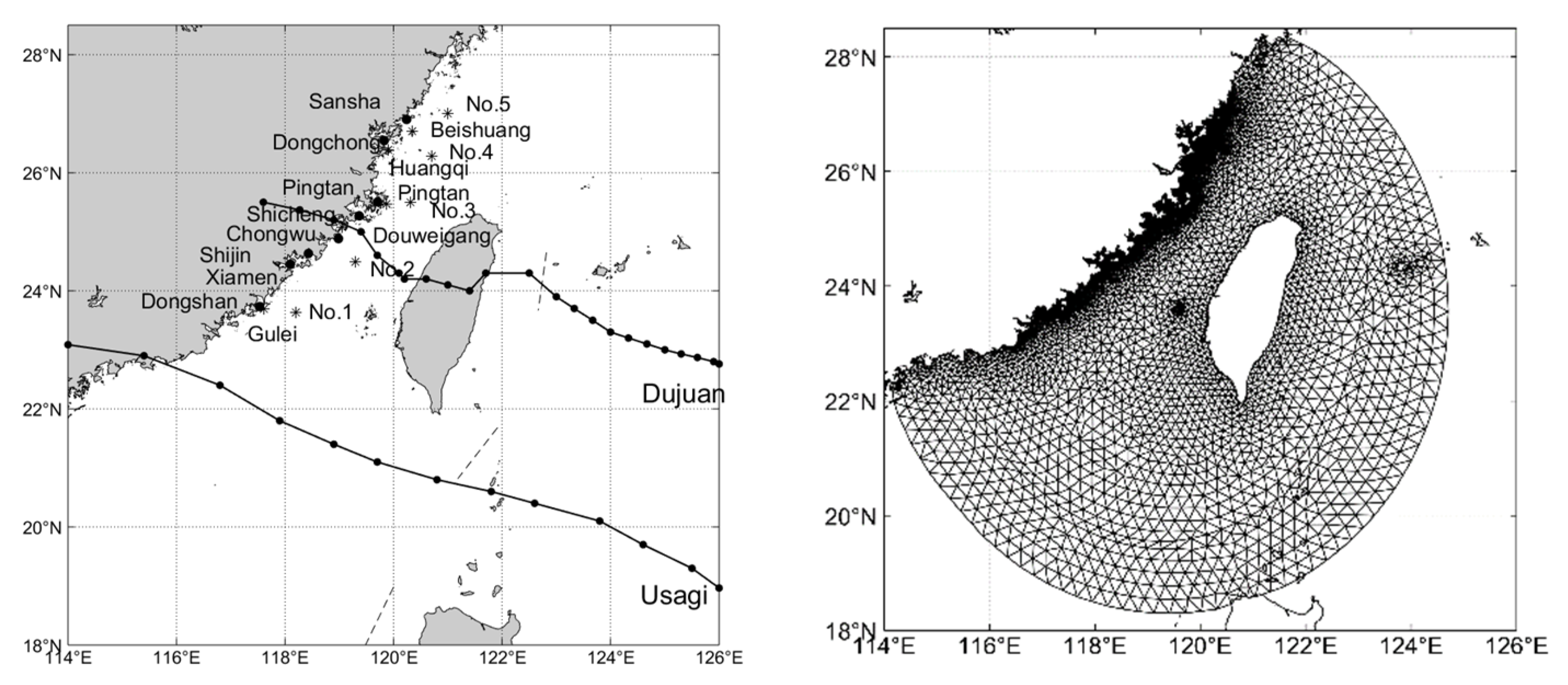
2.1. Study Area and Storm Cases
2.2. FETSWCM-SWAN Model
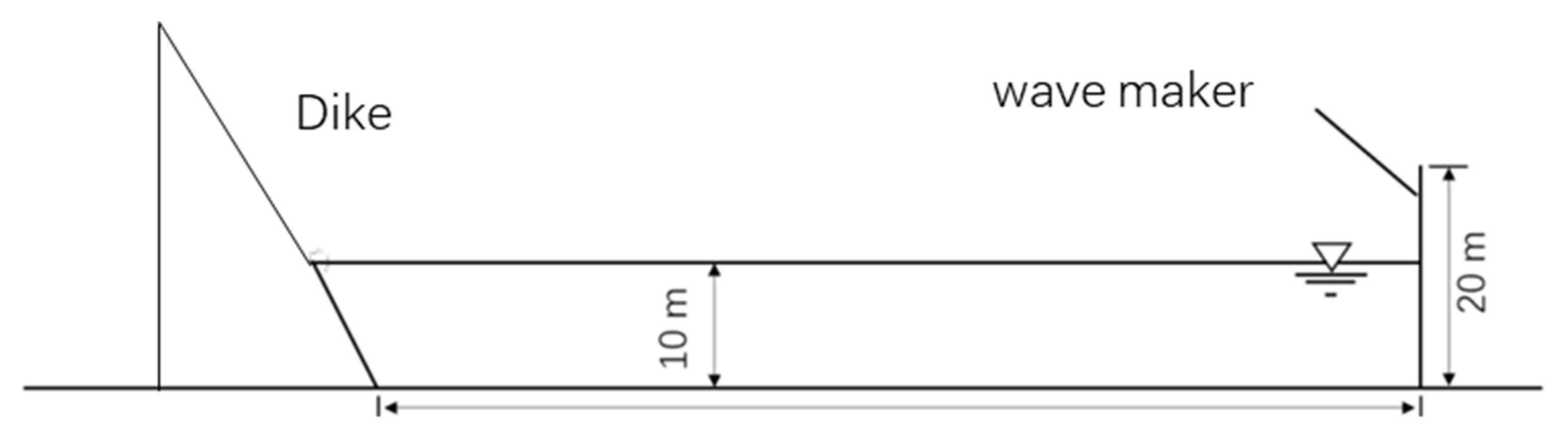
2.3. DualSPHysics Model
3. Results and Discussion
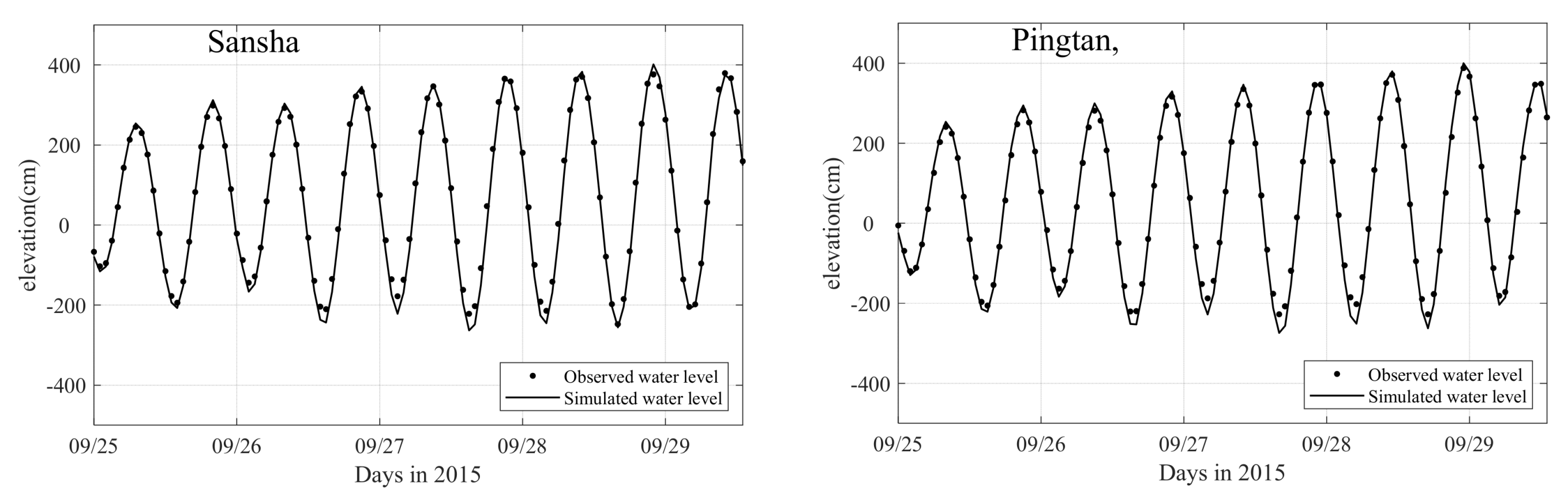
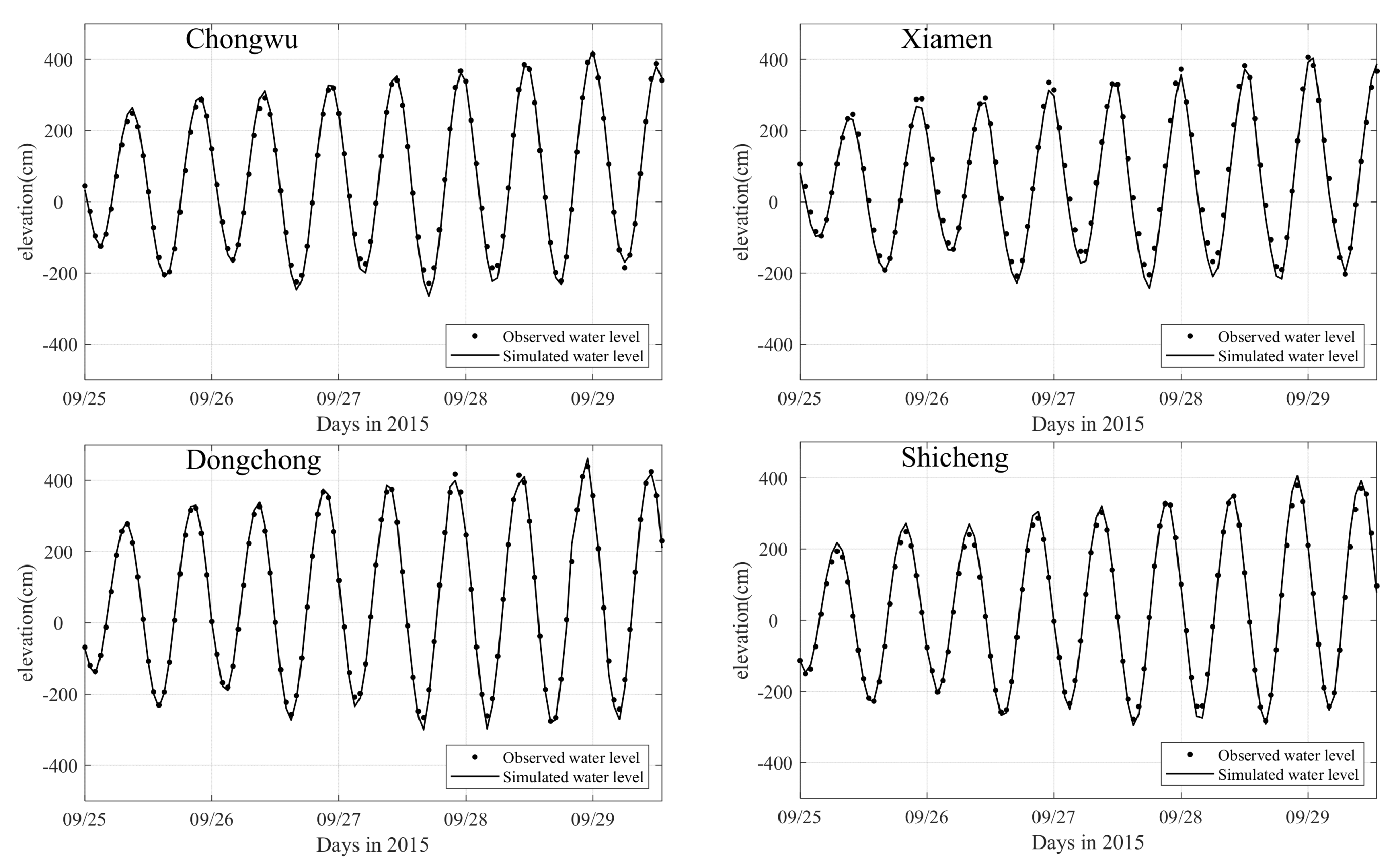
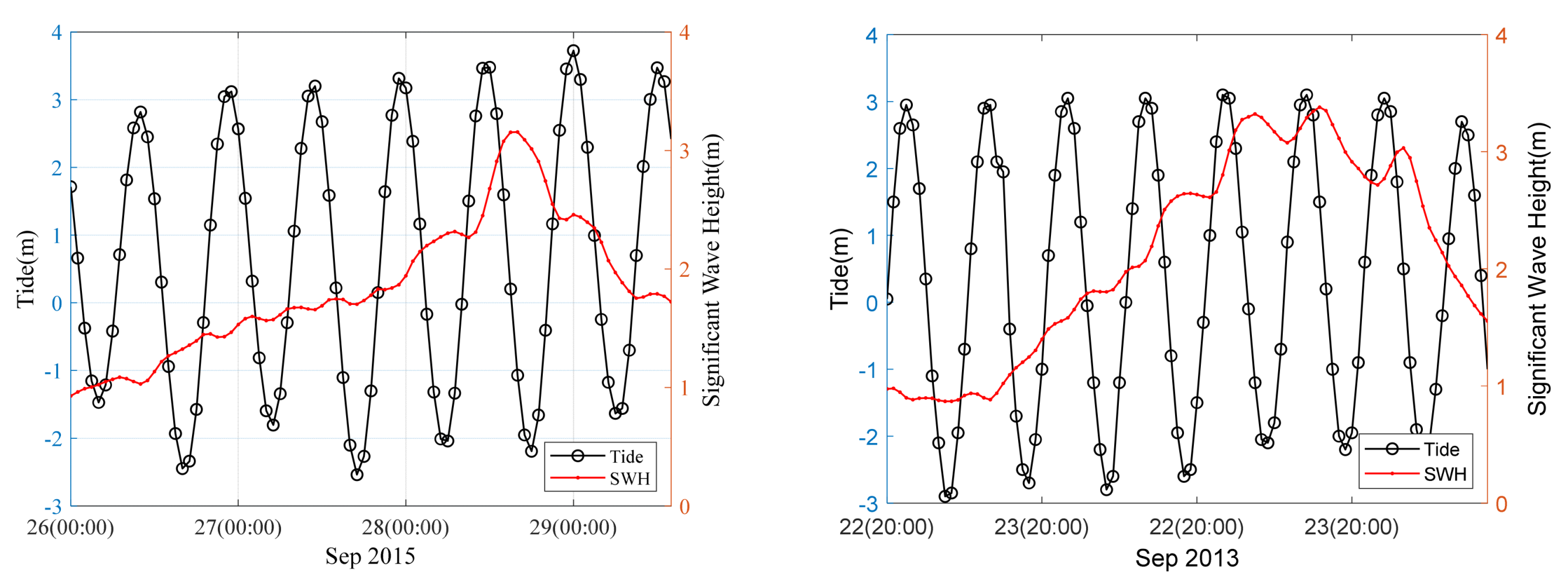
3.1. Water Level Simulation
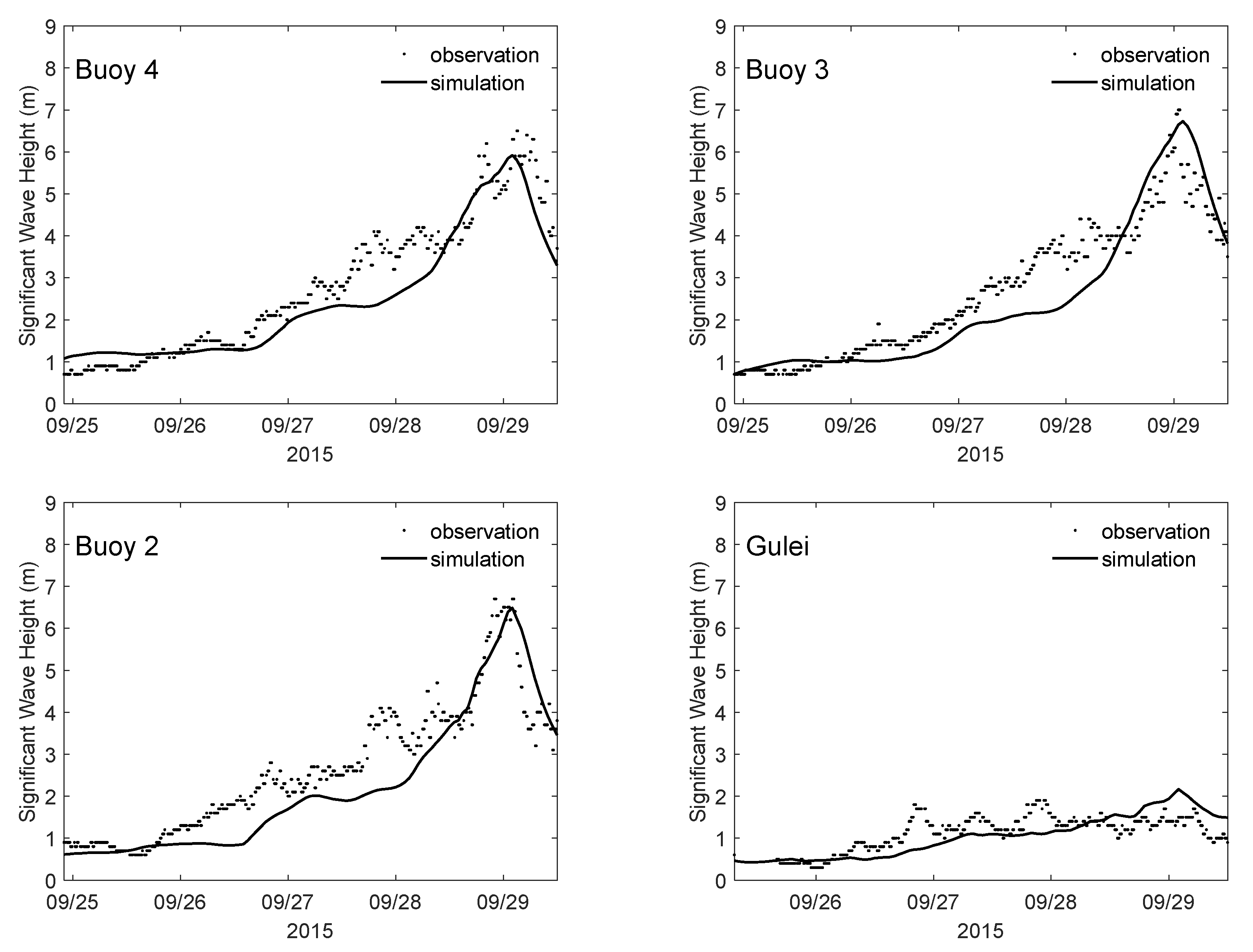
3.2. Wave Simulation
3.3. Typical Wave Run-Up Simulation
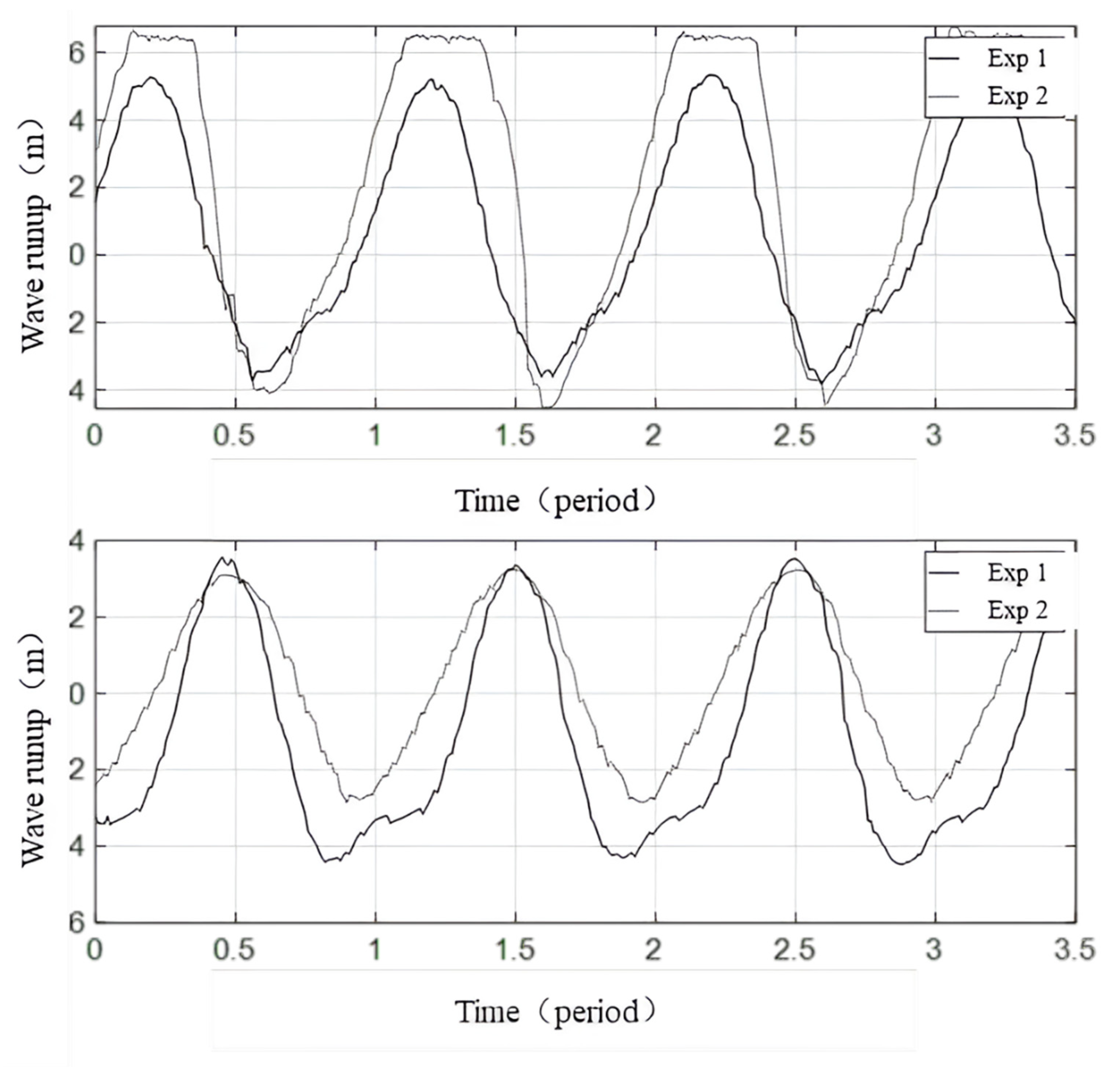
3.4. Storm Over-Topping Simulation
4. Conclusions
- (1)
- The FETSWCM-SWAN model is used to calculate the tidal level, wind, and waves over Fujian and Taiwan Strait during Typhoon Usagi in 2013 and Typhoon Dujuan in 2015. The simulation results are in good agreement with the wave heights recorded by buoys. The FETSWCM-SWAN model successfully reproduces wave oscillation due to the interaction of tide and wave.
- (2)
- The DualSPHysics model is used to study the wave climbing process of Dazuo Seawall in Chongwu, Fujian province. A numerical flume with the Active Wave Absorption System is used to simulate the wave run-ups at Dazuo Seawall under typical waves and typhoon waves. The simulation results show that the typical wave run-up height of Dazuo Seawall is lower than 2 m, and the design of the seawall meets the daily protection requirements. Under the extreme conditions of Typhoon Usagi, the wave run-up height reached 19.95 m, exceeding the designed protecting height of Dazuo Seawall, and over-topping occurred.
- (3)
- The typhoon process usually lasts 3–5 days. Based on an analysis of tide level and wave simulations three days before the landfall of the typhoons, eight sets of experiments were conducted. The results show that the risk of over-topping increases during high tide. The wave run-up height decreases as the water becomes deeper under the same wave conditions as a result of the ocean wave being stretched and shortened by the different propagation speeds at crest and trough.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Warner, J.C.; Perlin, N.; Skyllingstad, E.D. Using the model coupling toolkit to couple earth system models. Environ. Model. Softw. 2008, 23, 1240–1249. [Google Scholar] [CrossRef]
- Dietrich, J.C.; Zijlema, M.; Westerink, J.J.; Holthuijsen, L.H.; Dawson, C.; Luettich, R.A., Jr.; Stone, G.W. Modeling hurricane waves and storm surge using integrally-coupled, scalable computations. Coast. Eng. 2011, 58, 45–65. [Google Scholar] [CrossRef]
- Sebastian, A.; Proft, J.; Dietrich, J.C.; Du, W.; Bedient, P.B.; Dawson, C.N. Characterizing hurricane storm surge behavior in Galveston Bay using the SWAN + ADCIRC model. Coast. Eng. 2014, 88, 171–181. [Google Scholar] [CrossRef]
- Xie, D.M.; Zou, Q.P.; Cannon, J.W. Application of SWAN ADCIRC to tide-surge and wave simulation in Gulf of Maine during patriot’s day storm. Water Sci. Eng. 2016, 9, 33–41. [Google Scholar] [CrossRef]
- Qi, J.; Chen, C.; Beardsley, R.C.; Perrie, W.; Cowles, G.W.; Lai, Z. An unstructured-grid finite-volume surface wave model (FVCOM-SWAVE): Implementation, validations and applications. Ocean. Model. 2009, 28, 153–166. [Google Scholar] [CrossRef]
- Zhang, L.; Shang, S.; Zhang, F.; Xie, Y. Tide-surge-wave interaction in the Taiwan Strait during Typhoons Soudelor (2015) and Dujuan (2015). Appl. Sci. 2020, 10, 7382. [Google Scholar] [CrossRef]
- Saville, T., Jr. Wave run-up on shore structures. J. Waterw. Harb. Div. 1956, 82, 925-1–925-14. [Google Scholar] [CrossRef]
- Iwagaki, Y.; Sakai, T.; Tsuda, T.; Oka, Y. Wave refraction and wave height variation due to current. Bull. Disaster Prev. Res. Inst. 1977, 27, 73–91. [Google Scholar]
- Van Der Meer, J.W.; De Waal, J.P. Wave run-up and overtopping on coastal structures. In Proceedings of the 23rd International Conference, Venice, Italy, 4–9 October 1992. [Google Scholar]
- Duan, X.; Mao, P.; Mao, C. Research on the status of seawall along the southeast coast (Fujian Province). Ocean. Eng. 1996, 14, 41–49. (In Chinese) [Google Scholar]
- Hofland, B.; Diamantidou, E.; van Steeg, P.; Meys, P. Wave runup and wave overtopping measurements using a laser scanner. Coast. Eng. 2015, 106, 20–29. [Google Scholar] [CrossRef]
- Zhang, L.; Shang, S.; Zhang, F.; Xie, Y. A tide-storm surge-wave coupled inundation warning system for Fujian coast. Mar. Forecast. 2016, 33, 61–69. [Google Scholar]
- Tanizawa, K. The state of the art on numerical wave tank. In Proceedings of the 4th Osaka Colloquium on Seakeeping Performance of Ships, Osaka, Japan, 17–21 October 2000; Volume 2000, pp. 95–144. [Google Scholar]
- Chang, Y.; Liu, S.; He, X.; Li, S.; Wang, G. Semi-analytical Solid Boundary Conditions for Free Surface Flows. In Computer Graphics Forum; Wiley: Hoboken, NJ, USA, 2020; Volume 39, pp. 131–141. [Google Scholar]
- Winchenbach, R.; Akhunov, R.; Kolb, A. Semi-analytic boundary handling below particle resolution for smoothed particle hydrodynamics. ACM Trans. Graph. TOG 2020, 39, 1–17. [Google Scholar] [CrossRef]
- Suzuki, T.; Altomare, C.; Yasuda, T.; Verwaest, T. Characterization of overtopping waves on sea dikes with gentle and shallow foreshores. J. Mar. Sci. Eng. 2020, 8, 752. [Google Scholar] [CrossRef]
- Zhang, F.; Crespo, A.; Altomare, C.; Domínguez, J.; Marzeddu, A.; Shang, S.P.; Gómez-Gesteira, M. DualSPHysics: A numerical tool to simulate real breakwaters. J. Hydrodyn. 2018, 30, 95–105. [Google Scholar] [CrossRef]
- Zhang, F.; Zhang, L.; Xie, Y.; Wang, Z.; Shang, S. Smoothed Particle Hydrodynamics Simulation of a Mariculture Platform under Waves. Water 2021, 13, 2847. [Google Scholar] [CrossRef]
- Lu, X.Q.; Yu, H.; Ying, M.; Zhao, B.K.; Zhang, S.; Lin, L.M.; Bai, L.N.; Wan, R.J. Western North Pacific tropical cyclone database created by the China Meteorological Administration. Adv. Atmos. Sci. 2021, 38, 690–699. [Google Scholar] [CrossRef]
- Chen, D.W.; Shang, S.P.; Shang, S.L.; University, X. Remote Sensing Study of Sea Surface Wind Field around Taiwan Island During Typhoon Periods. J.-Xiamen Univ. Nat. Sci. 2007, 46, 141–145. [Google Scholar]
- Holland, G.J. An analytic model of the wind and pressure profiles in hurricanes. Mon. Weather. Rev. 1980, 108, 1212–1218. [Google Scholar] [CrossRef]
- Matsumoto, K.; Takanezawa, T.; Ooe, M. Ocean Tide Models Developed by Assimilating TOPEX/POSEIDON Altimeter Data into Hydrodynamical Model: A Global Model and a Regional Model Around Japan. J. Oceanogr. 2000, 56, 567–581. [Google Scholar] [CrossRef]
- Crespo, A.J.; Domínguez, J.M.; Rogers, B.D.; Gómez-Gesteira, M.; Longshaw, S.; Canelas, R.J.F.B.; García-Feal, O. DualSPHysics: Open-source parallel CFD solver based on Smoothed Particle Hydrodynamics (SPH). Comput. Phys. Commun. 2015, 187, 204–216. [Google Scholar] [CrossRef]
- Fiedler, J.W.; Young, A.P.; Ludka, B.C.; O’Reilly, W.C.; Henderson, C.; Merrifield, M.A.; Guza, R.T. Predicting site-specific storm wave run-up. Nat. Hazards 2020, 104, 493–517. [Google Scholar] [CrossRef]
- Bender, J.; Kugelstadt, T.; Weiler, M.; Koschier, D. Implicit frictional boundary handling for SPH. IEEE Trans. Vis. Comput. Graph. 2020, 26, 2982–2993. [Google Scholar] [CrossRef] [PubMed]
- Altomare, C.; Crespo, A.J.; Rogers, B.D.; Dominguez, J.M.; Gironella, X.; Gómez-Gesteira, M. Numerical modelling of armour block sea breakwater with smoothed particle hydrodynamics. Comput. Struct. 2014, 130, 34–45. [Google Scholar] [CrossRef]
- da Silva, P.G.; Coco, G.; Garnier, R.; Klein, A.H. On the prediction of runup, setup and swash on beaches. Earth-Sci. Rev. 2020, 204, 103148. [Google Scholar] [CrossRef]
- Di Luccio, D.; Benassai, G.; Budillon, G.; Mucerino, L.; Montella, R.; Pugliese Carratelli, E. Wave run-up prediction and observation in a micro-tidal beach. Nat. Hazards Earth Syst. Sci. 2018, 18, 2841–2857. [Google Scholar] [CrossRef]
- Altomare, C.; Crespo, A.J.; Domínguez, J.M.; Gómez-Gesteira, M.; Suzuki, T.; Verwaest, T. Applicability of Smoothed Particle Hydrodynamics for estimation of sea wave impact on coastal structures. Coast. Eng. 2015, 96, 1–12. [Google Scholar] [CrossRef]
- Wendland, H. Piecewiese polynomial, positive definite and compactly supported radial functions of minimal degree. Adv. Comput. Math. 1995, 4, 389–396. [Google Scholar] [CrossRef]
- Madsen, P.A.; Fuhrman, D.R.; Schäffer, H.A. On the solitary wave paradigm for tsunamis. J. Geophys. Res.-Ocean. 2008, 113, 1–22. [Google Scholar] [CrossRef]
- Crespo, A.J.C.; Gómez-Gesteira, M.; Dalrymple, R. Boundary conditions generated by dynamic particles in SPH methods. Comput. Mater. Contin. 2007, 5, 173–184. [Google Scholar]
- Schaffer, H.A.; Klopman, G. Review of multidirectional active wave absorption methods. J. Waterw. Port Coast. Ocean. Eng. 2000, 126, 88–97. [Google Scholar] [CrossRef]
- Leimkuhler, B.J.; Reich, S.; Skeel, R.D. Integration Methods for Molecular Dynamic IMA Volume in Mathematics and Its Application; Springer: New York, NY, USA, 1996. [Google Scholar]




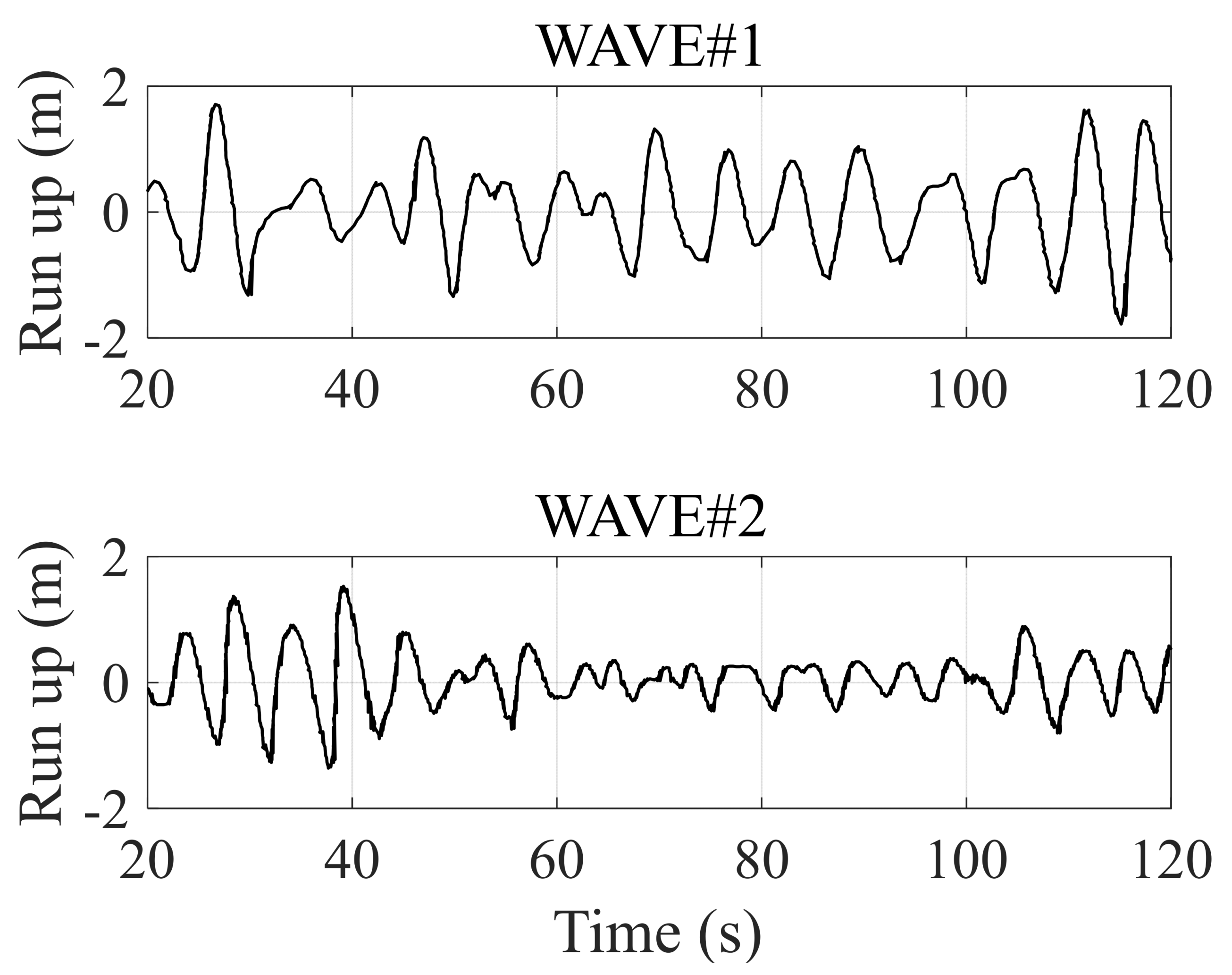

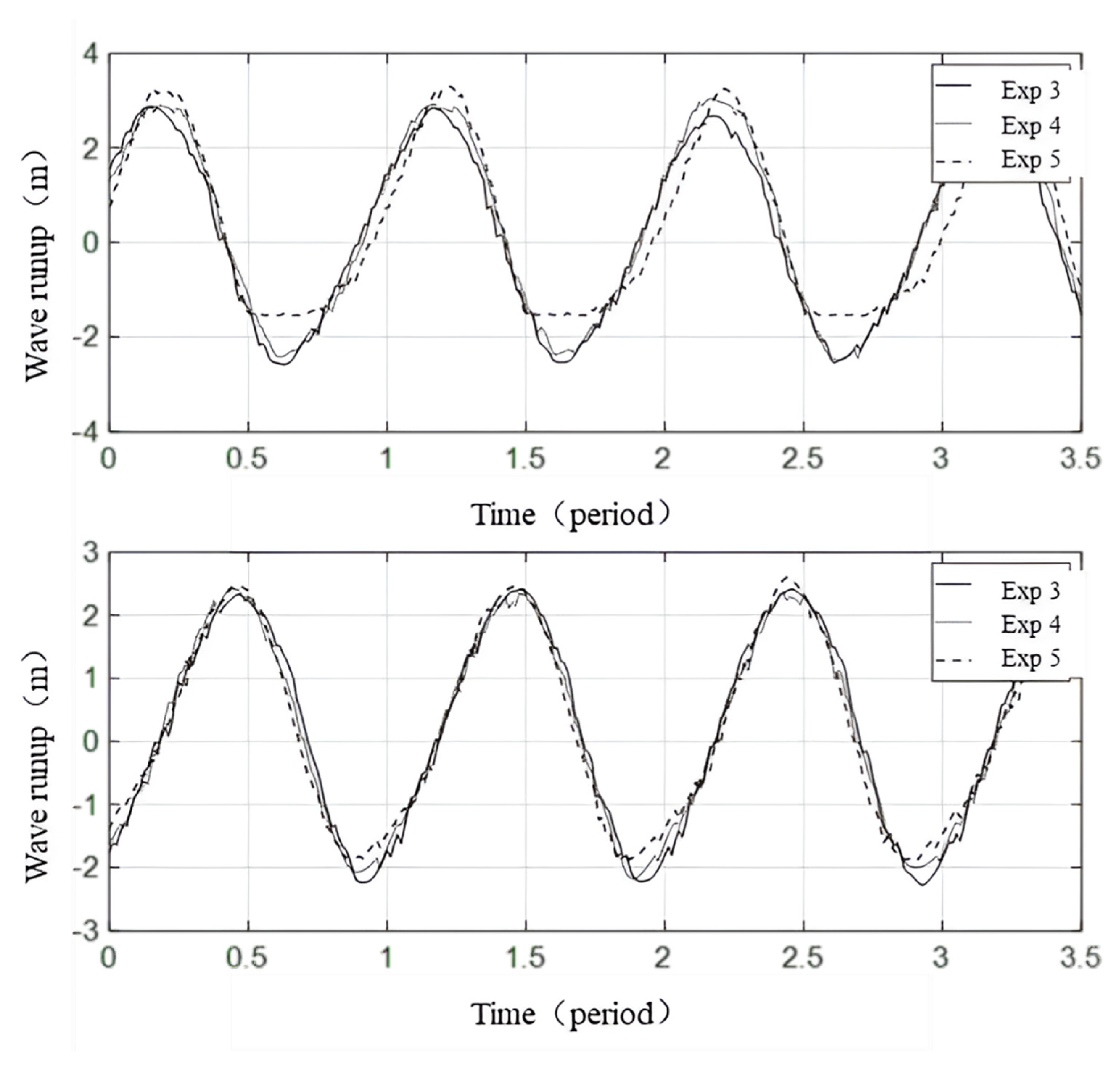
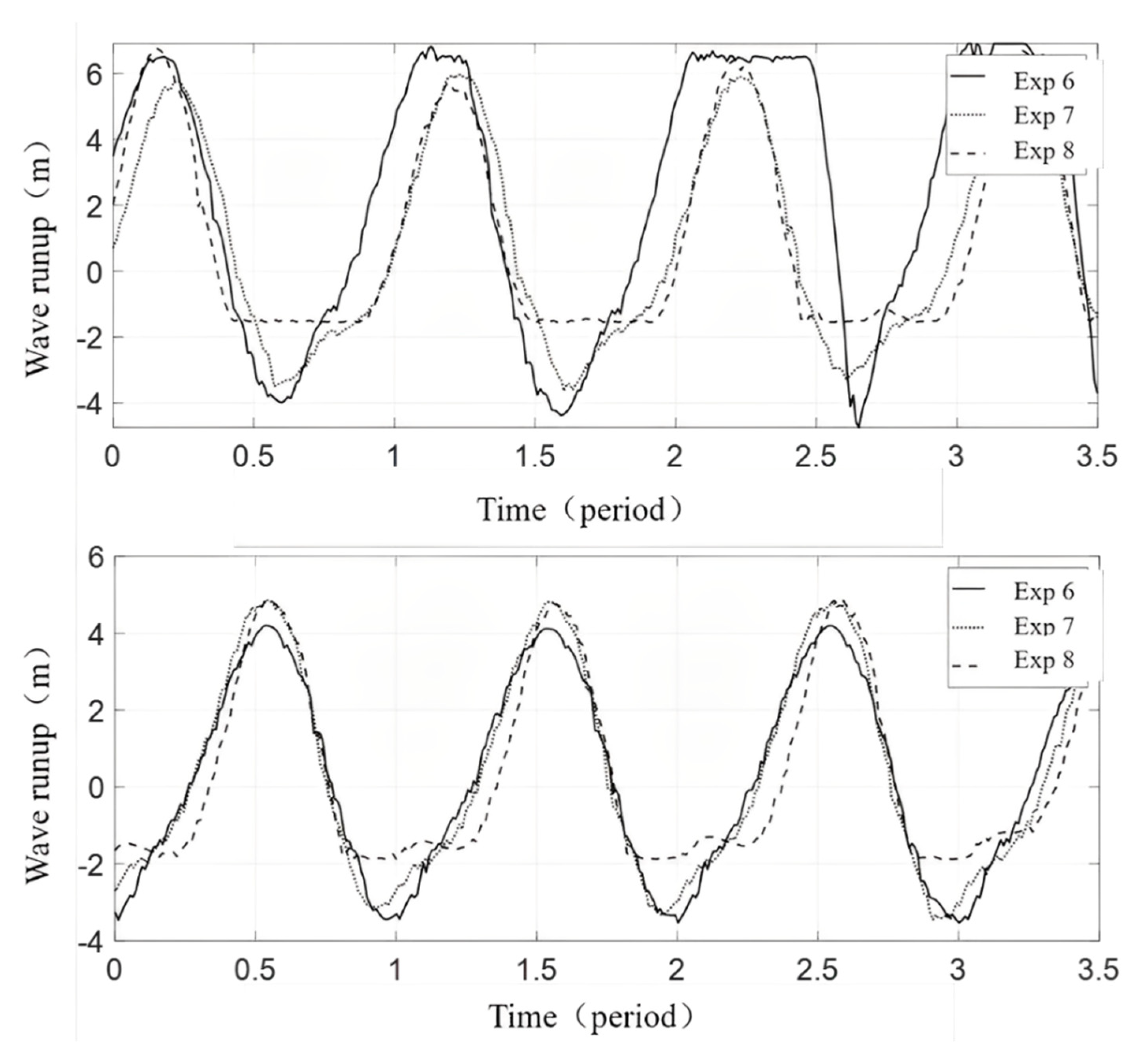
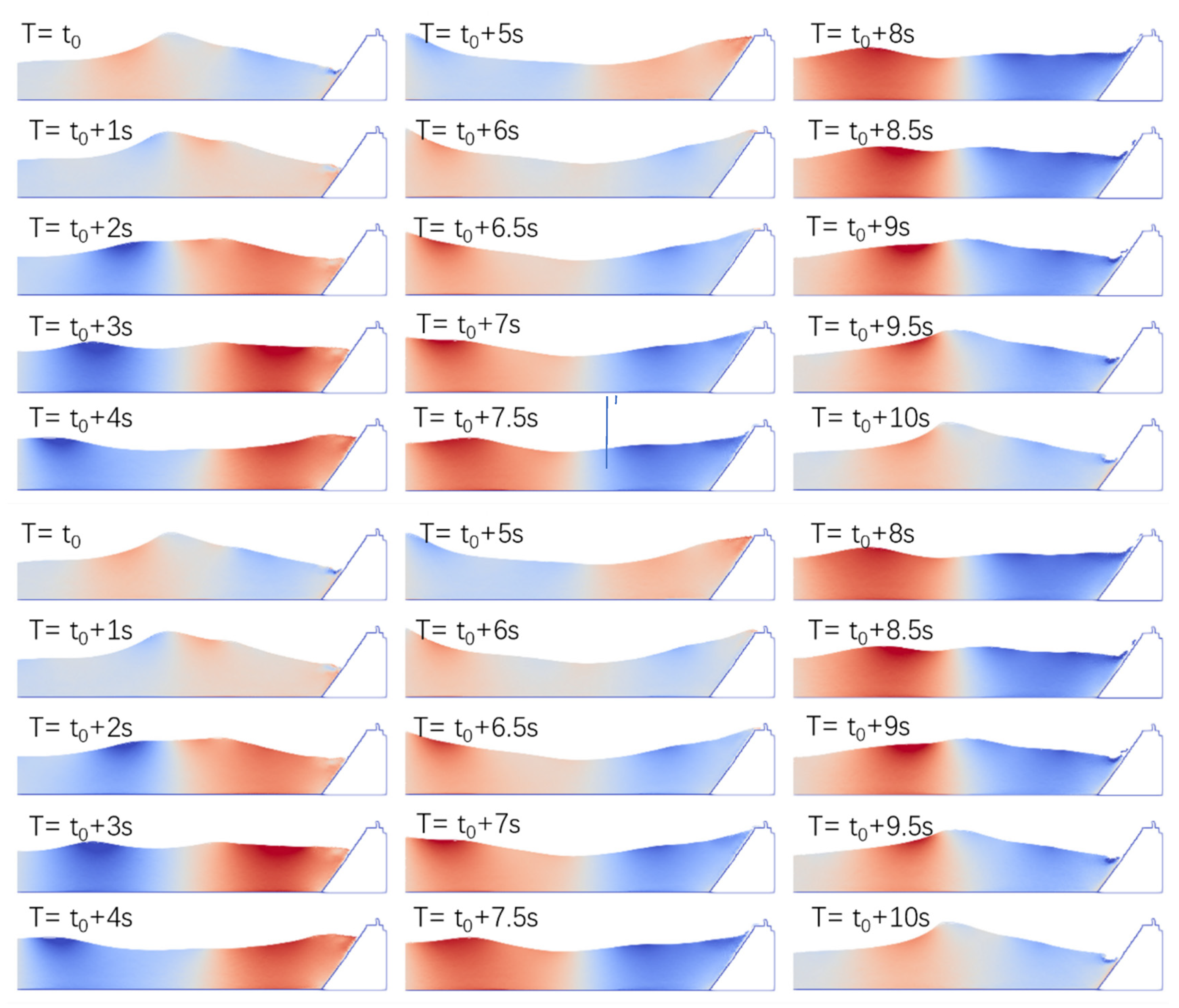
| Wave Height (m) | Wave Period (s) | Water Depth (m) | Wave Length (m) | Maximum Regular Wave Run-Up Height (m) | Maximum Irregular Wave Run-Up Height (m) | |
|---|---|---|---|---|---|---|
| Wave#1 | 1.24 | 7.03 | 10 | 45 | 1.87 | 1.89 |
| Wave#2 | 0.76 | 5.29 | 10 | 33 | 1.05 | 1.52 |
| Exp | Significant Wave Height (m) | Tide Level (m) | Wave Period (s) | Maximum Wave Run-Up (m) | Wave Run-Up Height (m) | Overtopping |
|---|---|---|---|---|---|---|
| 1 | 3.38 | 1.50 | 9.8 | 5.34 | 16.84 | no |
| 2 | 3.29 | 3.10 | 9.9 | 6.85 | 19.95 | yes |
| 3 | 2.33 | 3.05 | 8.4 | 3.13 | 16.18 | no |
| 4 | 2.33 | 0.36 | 8.4 | 3.10 | 13.46 | no |
| 5 | 2.33 | −2.45 | 8.4 | 3.30 | 10.85 | no |
| 6 | 3.38 | 3.05 | 9.8 | 6.90 | 19.95 | yes |
| 7 | 3.38 | 0.36 | 9.8 | 5.99 | 16.35 | no |
| 8 | 3.38 | −2.45 | 9.8 | 6.75 | 14.30 | no |
| Exp | Significant Wave Height (m) | Tide Level (m) | Wave Period (s) | Maximum Wave Run-Up (m) | Wave Run-Up Height (m) | Overtopping |
|---|---|---|---|---|---|---|
| 1 | 3.15 | −1.07 | 9.5 | 5.13 | 14.06 | no |
| 2 | 2.45 | 3.72 | 9.0 | 3.30 | 17.02 | no |
| 3 | 1.94 | 3.30 | 8.0 | 2.50 | 15.80 | no |
| 4 | 1.94 | 0.57 | 8.0 | 2.55 | 13.12 | no |
| 5 | 1.94 | −2.12 | 8.0 | 2.66 | 10.54 | no |
| 6 | 3.15 | 3.30 | 9.5 | 4.31 | 17.61 | no |
| 7 | 3.15 | 0.57 | 9.5 | 4.91 | 15.48 | no |
| 8 | 3.15 | −2.12 | 9.5 | 5.73 | 13.61 | no |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Zhang, L.; Xie, Y.; Zhong, Y.; Sun, Y.; Wang, Z.; Zhang, F.; Shang, S. Risk Assessment of Wave Over-Topping Based on Smoothed-Particle Hydrodynamics Method. J. Mar. Sci. Eng. 2022, 10, 1809. https://doi.org/10.3390/jmse10121809
Wang L, Zhang L, Xie Y, Zhong Y, Sun Y, Wang Z, Zhang F, Shang S. Risk Assessment of Wave Over-Topping Based on Smoothed-Particle Hydrodynamics Method. Journal of Marine Science and Engineering. 2022; 10(12):1809. https://doi.org/10.3390/jmse10121809
Chicago/Turabian StyleWang, Lei, Li Zhang, Yanshuang Xie, Yaozhao Zhong, Yuan Sun, Zhiyuan Wang, Feng Zhang, and Shaoping Shang. 2022. "Risk Assessment of Wave Over-Topping Based on Smoothed-Particle Hydrodynamics Method" Journal of Marine Science and Engineering 10, no. 12: 1809. https://doi.org/10.3390/jmse10121809
APA StyleWang, L., Zhang, L., Xie, Y., Zhong, Y., Sun, Y., Wang, Z., Zhang, F., & Shang, S. (2022). Risk Assessment of Wave Over-Topping Based on Smoothed-Particle Hydrodynamics Method. Journal of Marine Science and Engineering, 10(12), 1809. https://doi.org/10.3390/jmse10121809









