Optimizing Sulfur Fertilization for Enhanced Physiological Performance, Grain Filling Characteristics, and Grain Yield of High-Yielding Winter Wheat Under Drip Irrigation
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Site Description
2.2. Experimental Design
2.3. Measurement Items and Methods
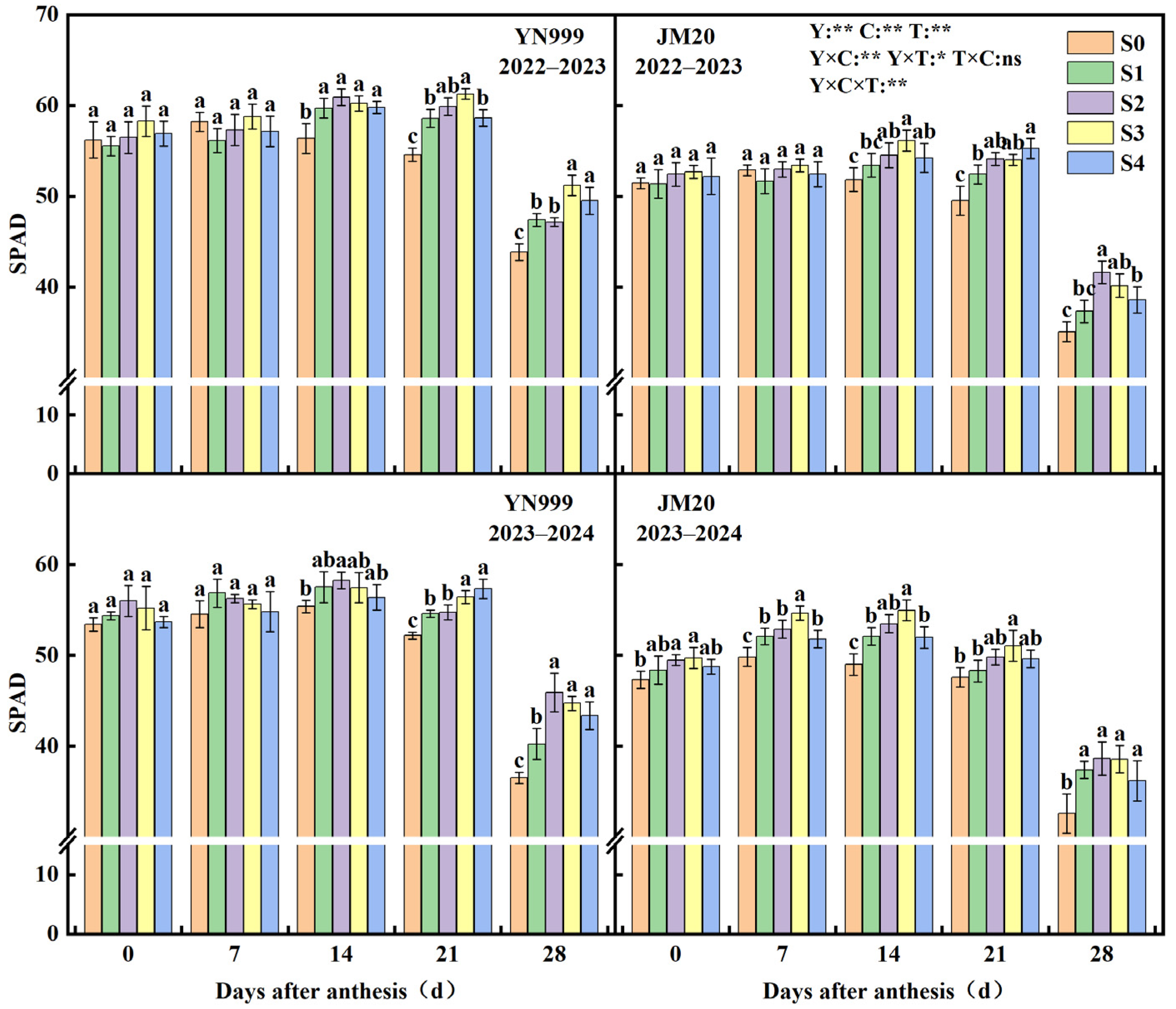
2.3.1. Relative Chlorophyll Content (SPAD)
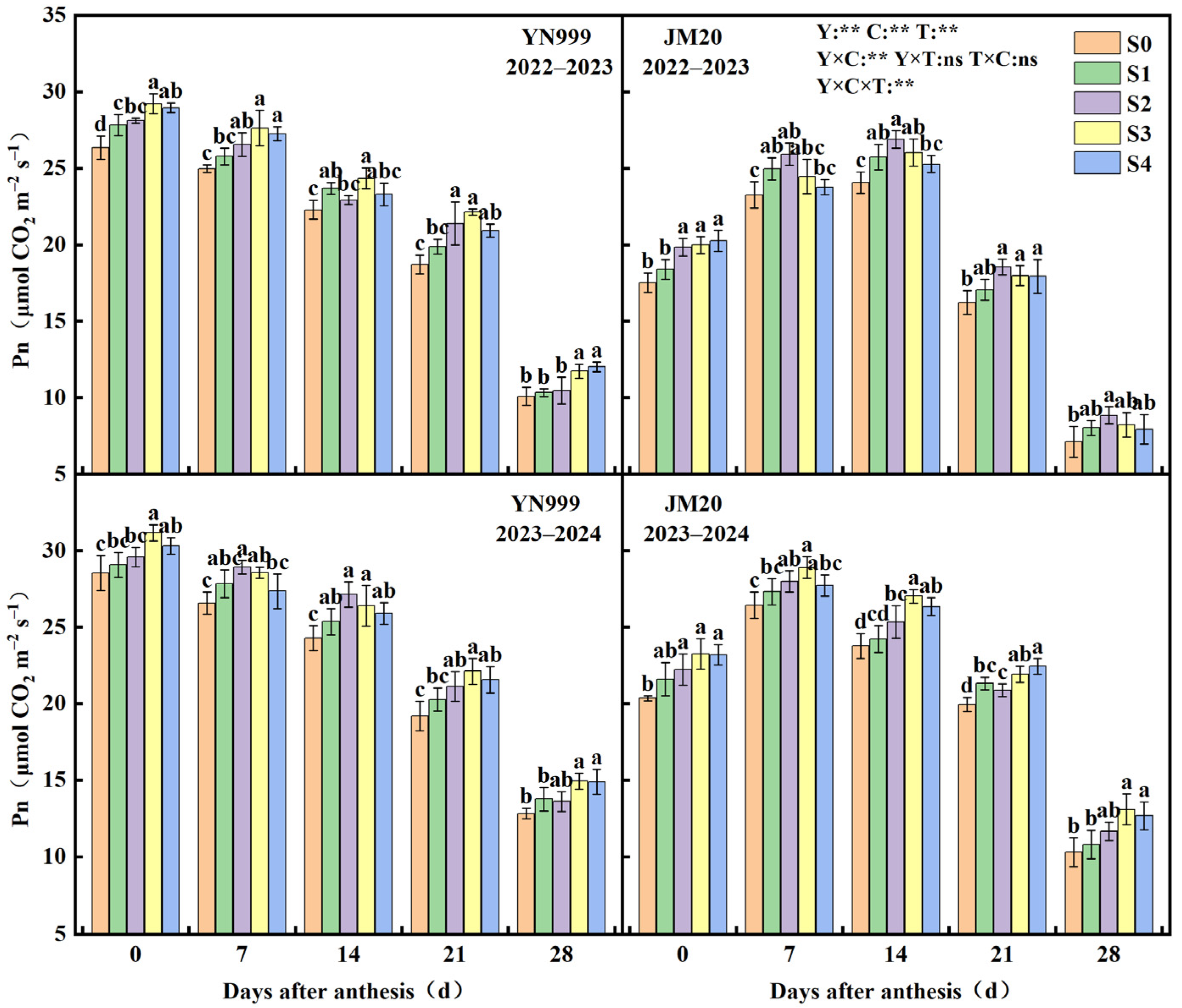
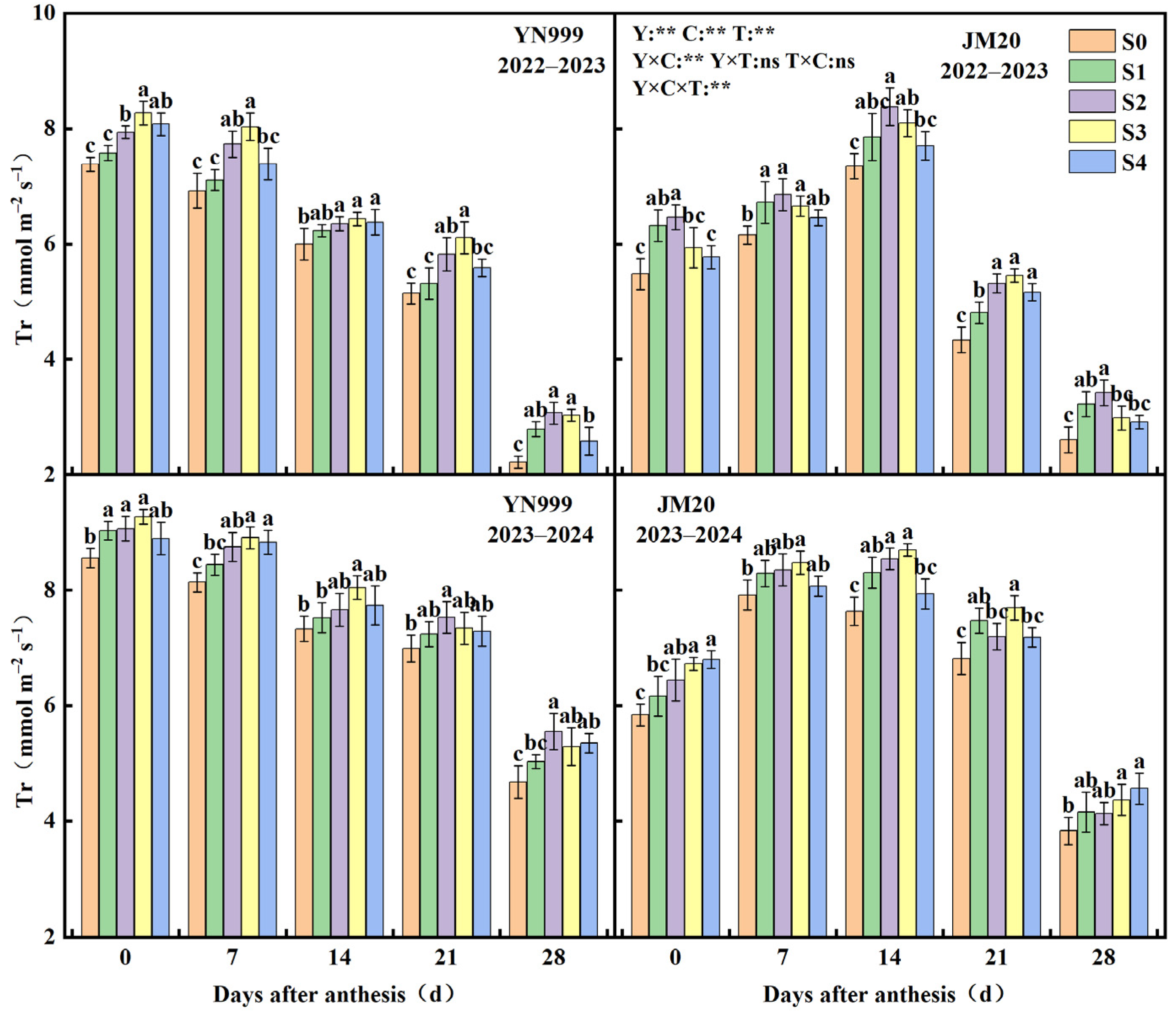
2.3.2. Photosynthetic Characteristics of Flag Leaves
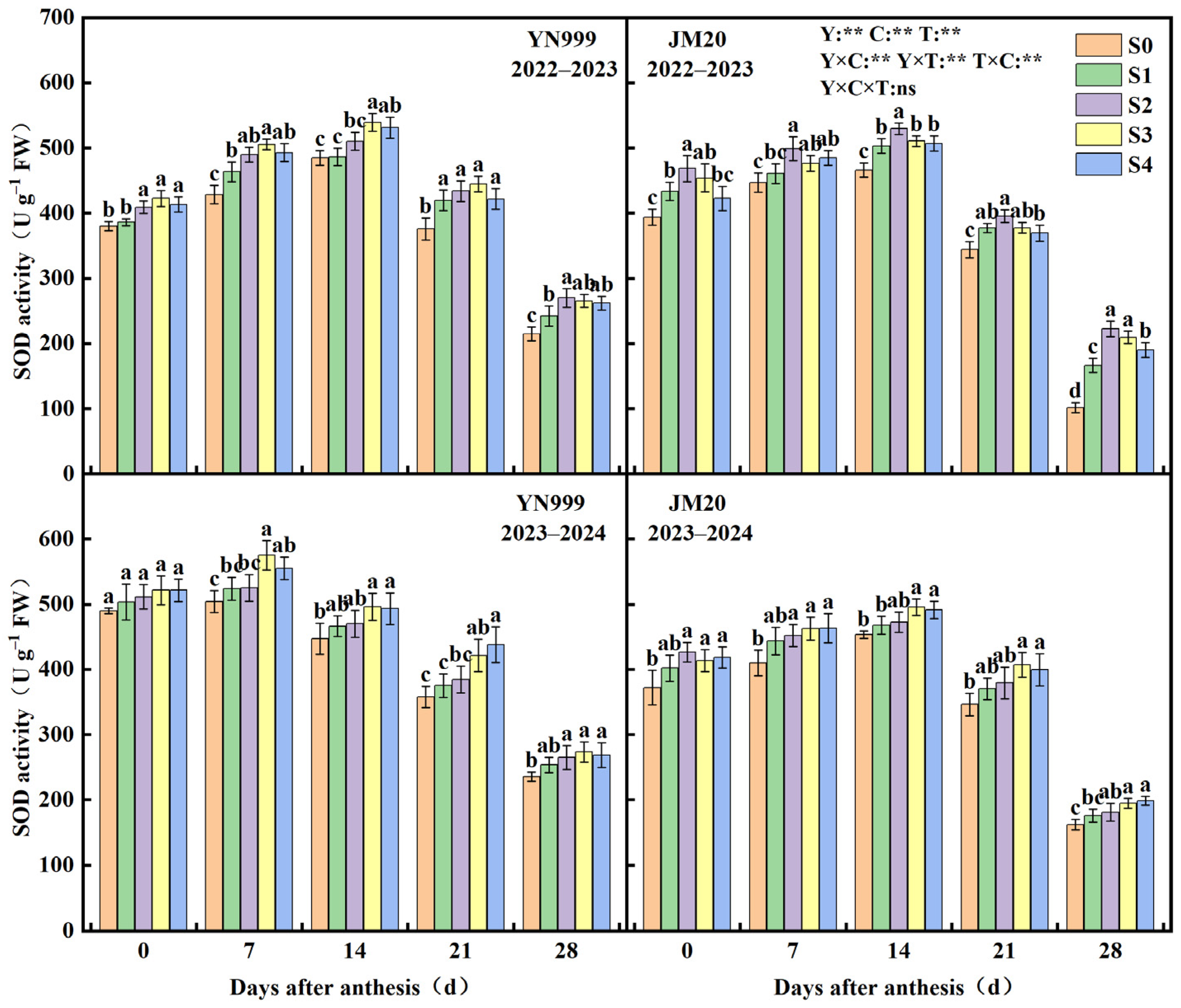
2.3.3. Senescence Characteristics of Flag Leaves
2.3.4. Grain Filling
2.3.5. Analysis of Yield and Yield Components
2.3.6. Data Analysis and Processing
3. Results
3.1. Effect of Sulfur Application on the SPAD of Winter Wheat Flag Leaves
3.2. Effect of Sulfur Application on the Photosynthetic Characteristics of Winter Wheat Flag Leaves
3.3. Effect of Sulfur Application on the Senescence Characteristics of Winter Wheat Flag Leaves
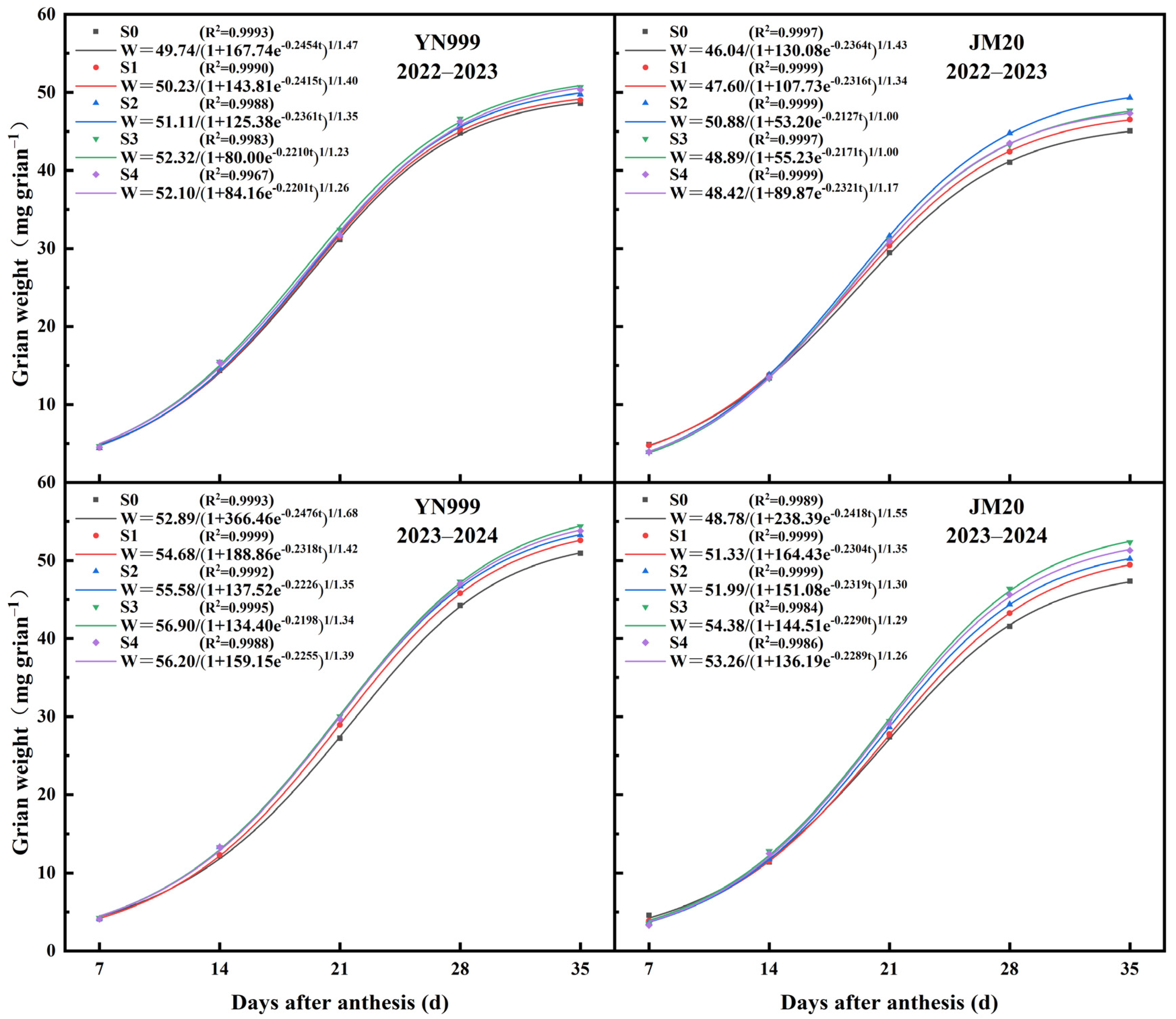
3.4. Effect of Sulfur Application on the Richards Grain Filling Curve and Fitting Equation in Winter Wheat
3.5. Effect of Sulfur Application on the Grain Filling Parameters of Wheat
3.6. Effect of Sulfur Application on the Grain Filling Characteristics of Winter Wheat at Different Grain Filling Stages
3.7. Effect of Sulfur Application on the Yield and Yield Components of Winter Wheat
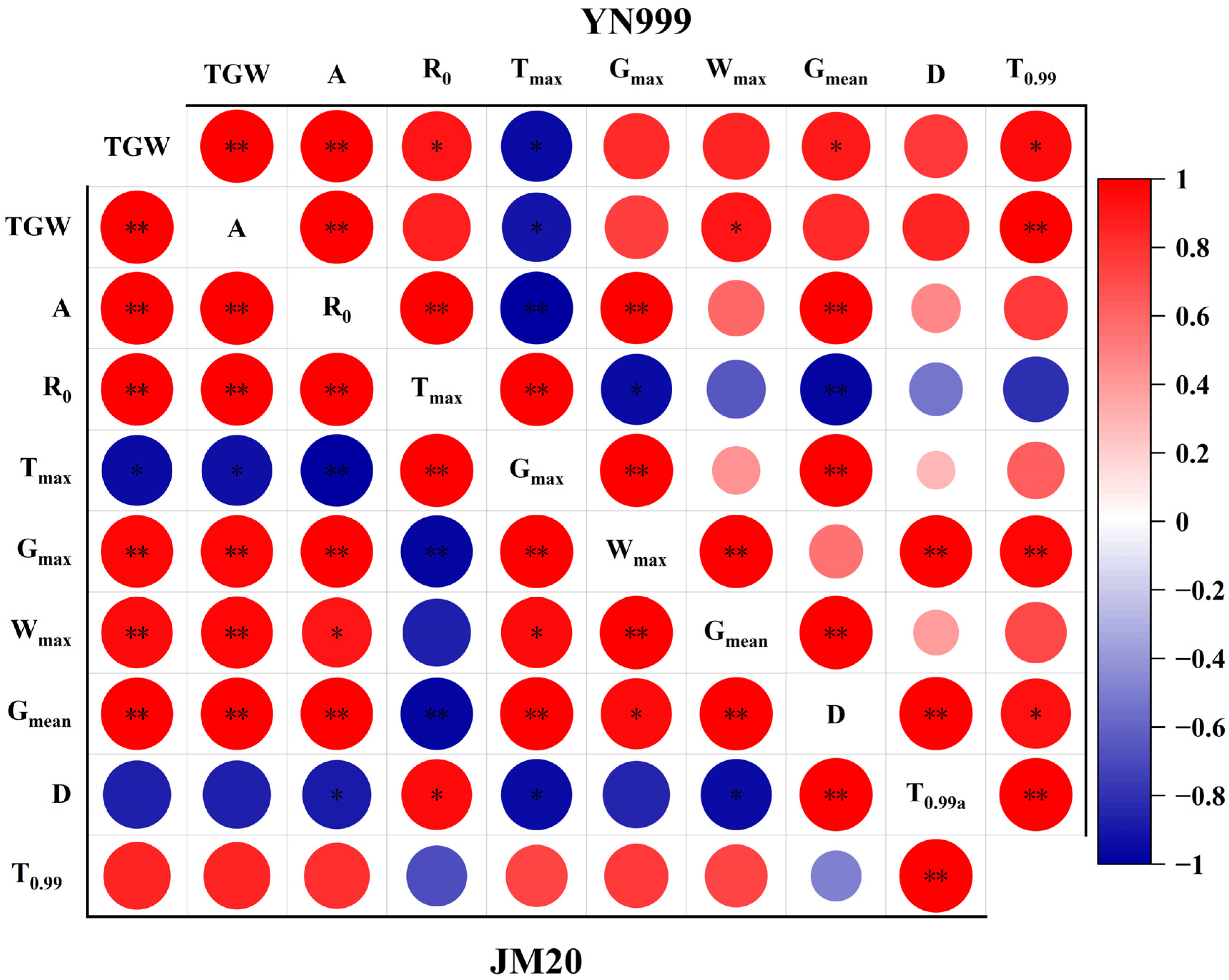
3.8. Correlation Analysis of SPAD, Photosynthetic Characteristics, Senescence Traits, and Yield
3.9. Correlation Analysis of Final Growth and Grain Filling Parameters with Grain Weight
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Hawkesford, M.J.; Araus, J.L.; Park, R.; Calderini, D.; Miralles, D.; Shen, T.M.; Zhang, J.P.; Parry, M.A.J. Prospects of doubling global wheat yields. Food Energy Secur. 2013, 2, 34–48. [Google Scholar] [CrossRef]
- He, J.N.; Shi, Y.; Zhao, J.Y.; Yu, Z.W. Strip rotary tillage with subsoiling increases winter wheat yield by alleviating leaf senescence and increasing grain filling. Crop J. 2020, 8, 327–340. [Google Scholar] [CrossRef]
- Rivera-Amado, C.; Molero, G.; Trujillo-Negrellos, E.; Reynolds, M.; Foulkes, J. Estimating organ contribution to grain filling and potential for source upregulation in wheat cultivars with a contrasting source–sink balance. Agronomy 2020, 10, 1527. [Google Scholar] [CrossRef]
- Baillot, N.; Girousse, C.; Allard, V.; Piquet-Pissaloux, A.; Gouis, J. Different grain-filling rates explain grain-weight differences along the wheat ear. PLoS ONE 2018, 13, e0209597. [Google Scholar] [CrossRef]
- Guo, Z.J.; Shi, Y.; Yu, Z.W.; Zhang, Y.L. Supplemental irrigation affected flag leaves senescence post-anthesis and grain yield of winter wheat in the Huang-Huai-Hai Plain of China. Field Crops Res. 2015, 180, 100–109. [Google Scholar] [CrossRef]
- Mu, H.; Jiang, D.; Wollenweber, B.; Dai, T.; Jing, Q.; Cao, W. Long-term low radiation decreases leaf photosynthesis, photochemical efficiency and grain yield in winter wheat. J. Agron. Crop Sci. 2010, 196, 38–47. [Google Scholar] [CrossRef]
- Liu, X.M.; Meng, Y.; Gu, W.R.; Tong, T.; Li, C.F.; Li, W.H. Plant Growth Regulators Application Improves Spring Maize Yield by Improving Net Photosynthesis and Grain Filling Rate. Int. J. Agric. Biol. 2019, 22, 1223–1230. [Google Scholar] [CrossRef]
- Saito, K. Sulfur Assimilatory Metabolism. The Long and Smelling Road. Plant Physiol. 2004, 136, 2443–2450. [Google Scholar] [CrossRef]
- Zenda, T.; Liu, S.; Dong, A.; Duan, H. Revisiting Sulphur—The Once Neglected Nutrient: It’s Roles in Plant Growth, Metabolism, Stress Tolerance and Crop Production. Agriculture 2021, 11, 626. [Google Scholar] [CrossRef]
- Roa, G.A.; Quintana-Obregón, E.A.; González-Renteria, M.; Ruiz Diaz, D.A. Increasing wheat protein and yield through sulfur fertilization and its relationship with nitrogen. Nitrogen 2024, 5, 553–571. [Google Scholar] [CrossRef]
- Sharma, R.K.; Cox, M.S.; Oglesby, C.; Dhillon, J.S. Revisiting the role of sulfur in crop production: A narrative review. J. Agr. Food Res. 2024, 15, 101013. [Google Scholar] [CrossRef]
- Scherer, H.W. Sulphur in crop production—Invited paper. Eur. J. Agron. 2001, 14, 81–111. [Google Scholar] [CrossRef]
- Narayan, O.P.; Kumar, P.; Yadav, B.; Dua, M.; Johri, A.K. Sulfur nutrition and its role in plant growth and development. Plant Signal. Behav. 2023, 18, 2030082. [Google Scholar] [CrossRef]
- Booali, S.; Zoufan, P.; Zare Bavani, M.R. Effect of biofertilizer containing Thiobacillus bacteria along with different levels of chemical sulfur fertilizer on growth response and photochemical efficiency of small radish plants (Raphanus sativus L. var. shushtari) under greenhouse conditions. Sci. Hortic. 2024, 327, 112835. [Google Scholar] [CrossRef]
- Domínguez, F.; Cejudo, F.J. Chloroplast dismantling in leaf senescence. J. Exp. Bot. 2021, 72, 5905–5918. [Google Scholar] [CrossRef]
- Tavanti, T.R.; Melo, A.; Moreira, L.D.K.; Sanchez, D.E.J.; Silva, R.D.; Silva, R.M.D.; Reis, A.R.D. Micronutrient fertilization enhances ROS scavenging system for alleviation of abiotic stresses in plants. Plant Physiol. Bioch. 2021, 160, 386–396. [Google Scholar] [CrossRef]
- Zhu, Y.; Tian, W.; Xie, Y.; Guo, T.C.; Wang, C.; Liu, N. Effects of Sulphur Ascorbic Acid and Glutathione Circulatory System in Flag Leaf of Winter Wheat. Acta Bot. Boreali-Occident. Sin. 2010, 30, 2191–2196. [Google Scholar]
- Mukwevho, E.; Ferreira, Z.; Ayeleso, A. Potential Role of Sulfur-Containing Antioxidant Systems in Highly Oxidative Environments. Molecules 2014, 19, 19376–19389. [Google Scholar] [CrossRef]
- Wrigley, C.W.; Du Cros, D.L.; Fullington, J.G.; Kasarda, D.D. Changes in polypeptide composition and grain quality due to sulfur deficiency in wheat. J. Cereal Sci. 1984, 2, 15–24. [Google Scholar] [CrossRef]
- Ju, X.-T.; Xing, G.-X.; Chen, X.-P.; Zhang, S.-L.; Zhang, L.-J.; Liu, X.-J.; Cui, Z.-L.; Yin, B.; Christie, P.; Zhu, Z.-L.; et al. Reducing environmental risk by improving N management in intensive Chinese agricultural systems. Proc. Natl. Acad. Sci. USA 2009, 106, 3041–3046. [Google Scholar] [CrossRef]
- Shahane, A.A.; Shivay, Y.S.; Prasanna, R.; Kumar, D. Nutrient removal by rice-wheat cropping system as influenced by crop establishment techniques and fertilization options in conjunction with microbial inoculation. Sci. Rep. 2020, 10, 21944. [Google Scholar] [CrossRef] [PubMed]
- David, M.B.; Gentry, L.E.; Mitchell, C.A. Riverine Response of Sulfate to Declining Atmospheric Sulfur Deposition in Agricultural Watersheds. J. Environ. Qual. 2016, 45, 1313–1319. [Google Scholar] [CrossRef]
- Kulczycki, G. The effect of elemental sulfur fertilization on plant yields and soil properties. Adv. Agron. 2021, 167, 105–181. [Google Scholar]
- Mandi, S.; Shivay, Y.S.; Prasanna, R.; Nayak, S.; Baral, K.; Reddy, K.S.; Borate, R.B. Insights into the Response of Elemental Sulfur Fertilization on Crop Yield and Nutritional Quality of Durum Wheat. J. Soil Sci. Plant Nutr. 2024, 24, 8306–8320. [Google Scholar] [CrossRef]
- Xie, Y.-X.; Zhang, H.; Zhu, Y.-J.; Zhao, L.; Yang, J.-H.; Cha, F.-N.; Liu, C.; Wang, C.-Y.; Guo, T.-C. Grain yield and water use of winter wheat as affected by water and sulfur supply in the North China Plain. J. Integr. Agric. 2017, 16, 614–625. [Google Scholar] [CrossRef]
- Hamani, A.K.M.; Abubakar, S.A.; Si, Z.Y.; Kama, R.; Gao, Y.; Duan, A.W. Responses of grain yield and water-nitrogen dynamic of drip-irrigated winter wheat (Triticum aestivum L.) to different nitrogen fertigation and water regimes in the North China Plain. Agric. Water Manag. 2023, 288, 108494. [Google Scholar] [CrossRef]
- Richards, F.J. A flexible growth function for empirical use. J. Exp. Bot. 1959, 10, 290–301. [Google Scholar] [CrossRef]
- Jiang, Q.; Du, Y.; Tian, X.; Wang, Q.; Xiong, R.; Xu, G.; Yan, C.; Ding, Y. Effect of panicle nitrogen on grain filling characteristics of high-yielding rice cultivars. Eur. J. Agron. 2016, 74, 185–192. [Google Scholar] [CrossRef]
- Hřivna, L.; Kotková, B.; Burešová, I. Effect of Sulphur Fertilization on Yield and Quality of Wheat Grain. Cereal Res. Commun. 2015, 43, 344–352. [Google Scholar] [CrossRef]
- Liu, Z.; Fu, X.Y.; Wang, S.; Ma, J.Y.; Li, D.X.; Li, R.Q. Effects of Sulfur Application Period on Yield and Physiological Characteristics of Strong-Gluten Wheat. Acta Agric. Boreali-Sin. 2024, 39, 102–109. [Google Scholar]
- Pireivatlou, A.S.; Aliyev, R.; Lalehloo, B.S. Grain filling rate and duration in bread wheat under irrigated and drought stressed conditions. J. Plant Physiol. Breed. 2011, 1, 69–86. [Google Scholar]
- Bolaños, J. Physiological bases for yield differences in selected maize cultivars from Central America. Field Crops Res. 1995, 42, 69–80. [Google Scholar] [CrossRef]
- Borrás, L.; Gambín, B.L. Trait dissection of maize kernel weight: Towards integrating hierarchical scales using a plant growth approach. Field Crops Res. 2010, 118, 1–12. [Google Scholar] [CrossRef]
- Liu, H.X.; Si, X.M.; Wang, Z.Y.; Cao, L.J.; Gao, L.F.; Zhou, X.L.; Wang, W.X.; Wang, K.; Jiao, C.Z.; Zhuang, L.; et al. TaTPP-7A positively feedback regulates grain filling and wheat grain yield through T6P-SnRK1 signalling pathway and sugar-ABA interaction. Plant Biotechnol. J. 2023, 21, 1159–1175. [Google Scholar] [CrossRef]
- Lv, X.K.; Han, J.; Liao, Y.C.; Liu, Y. Effect of phosphorus and potassium foliage application post-anthesis on grain filling and hormonal changes of wheat. Field Crops Res. 2017, 214, 83–93. [Google Scholar] [CrossRef]
- Shen, X.S.; Zhu, Y.J.; Guo, T.C.; Li, G.Q.; Qu, H.J. Effects of Sulphur Application on Characteristics of Grain Filling and Grain Yield of Winter Wheat Cultivar ‘Yumai 50’. Acta Bot. Boreali-Occident. Sin. 2007, 27, 1265–1269. [Google Scholar]
- Zhao, Y.X.; Li, N.; Zhou, F.; Li, X.-F.; Wang, L.-Q. Effects of N and S application on grain filling characteristics and yield of winter wheat. Chin. J. Appl. Ecol. 2014, 25, 1366–1372. [Google Scholar]
- Ouyang, X.; Jiang, G.; Liu, J.; Wang, H.; Wang, R. Effect of nitrogen reduction on the remobilization of post-anthesis assimilates to grain and grain-filling characteristics in a drip-irrigated spring wheat system. Crop Sci. 2023, 63, 293–305. [Google Scholar] [CrossRef]
- Wang, J.J.; Sun, X.; Hussain, S.; Yang, L.H.; Gao, S.S.; Zhang, P.; Chen, X.L.; Ren, X.L. Reduced nitrogen rate improves post-anthesis assimilates to grain and ameliorates grain-filling characteristics of winter wheat in dry land. Plant Soil 2024, 499, 91–112. [Google Scholar] [CrossRef]
- Shivay, Y.S.; Pooniya, V.; Prasad, R.; Pal, M.; Bansal, R. Sulphur-coated Urea as a Source of Sulphur and an Enhanced Efficiency of Nitrogen Fertilizer for Spring Wheat. Cereal Res. Commun. 2016, 44, 513–523. [Google Scholar] [CrossRef]
- Ullah, I.; Muhammad, D.; Mussarat, M. Effect of Various Nitrogen Sources at Various Sulfur Levels on Maize-Wheat Yield and N/S Uptake under Different Climatic Conditions. J. Plant Growth Regul. 2023, 42, 2073–2087. [Google Scholar] [CrossRef]
- Noor, H.; Yan, Z.Z.; Sun, P.J.; Zhang, L.M.; Ding, P.C.; Li, L.H.; Ren, A.X.; Sun, M.; Gao, Z.Q. Effects of Nitrogen on Photosynthetic Productivity and Yield Quality of Wheat (Triticum aestivum L.). Agronomy 2023, 13, 1448. [Google Scholar] [CrossRef]
- Xie, Y.; Zhu, Y.; Guo, T.C.; Wang, C.; Wang, Y.; Ma, D. Effects of sulphureous fertilization on photosynthetic and physiological characteristics and yields of winter wheat. J. Plant Nutr. Fertil. 2009, 15, 403–409. [Google Scholar]
- Tian, W.; Zhu, Y.; Guo, T.C.; Xie, Y.; Zhu, X.; Wang, F. Effects of irrigation and sulphur and their interactions on photosynthetic characteristics and yield of winter wheat. Agric. Res. Arid. Areas 2009, 27, 114–119. [Google Scholar]
- Vishniac, W.; Rose, I.A. Mechanism of chlorophyll action in photosynthesis. Nature 1958, 182, 1089–1090. [Google Scholar] [CrossRef]
- Zahid, A.; Ul Din, K.; Ahmad, M.; Hayat, U.; Zulfiqar, U.; Askri, S.M.H.; Anjum, M.Z.; Maqsood, M.F.; Aijaz, N.; Chaudhary, T.; et al. Exogenous application of sulfur-rich thiourea (STU) to alleviate the adverse effects of cobalt stress in wheat. BMC Plant Biol. 2024, 24, 126. [Google Scholar] [CrossRef]






| Treatment | N kg ha−1 | P kg ha−1 | K kg ha−1 | S kg ha−1 | |
|---|---|---|---|---|---|
| Basal | Topdressing | Basal | Basal | Topdressing | |
| S0 | 90 | 120 | 90 | 90 | 0 |
| S1 | 15 | ||||
| S2 | 30 | ||||
| S3 | 45 | ||||
| S4 | 60 | ||||
| Year | Cultivar | Treatment | R0 | Tmax (d) | Gmax (mg·grain−1·d−1) | Wmax (mg) | Gmean (mg·grain−1·d−1) | D (d) | T0.99 (d) |
|---|---|---|---|---|---|---|---|---|---|
| 2022–23 | YN999 | S0 | 0.1672 b | 19.30 a | 2.67 bc | 26.88 a | 1.76 b | 28.30 b | 38.04 b |
| S1 | 0.1725 ab | 19.18 a | 2.70 ab | 26.88 a | 1.78 a | 28.17 b | 38.20 b | ||
| S2 | 0.1750 ab | 19.18 a | 2.73 a | 27.14 a | 1.80 a | 28.39 b | 38.65 b | ||
| S3 | 0.1799 a | 18.90 b | 2.70 ab | 27.25 a | 1.79 a | 29.23 a | 39.70 a | ||
| S4 | 0.1754 ab | 19.11 ab | 2.66 c | 27.26 a | 1.76 b | 29.58 a | 39.98 a | ||
| JM20 | S0 | 0.1655 b | 19.09 a | 2.41 b | 24.76 ab | 1.59 b | 29.02 a | 38.49 b | |
| S1 | 0.1726 b | 18.94 ab | 2.50 b | 25.23 ab | 1.65 b | 28.76 a | 38.64 b | ||
| S2 | 0.2126 a | 18.66 bc | 2.70 a | 25.45 a | 1.80 a | 28.26 ab | 40.31 a | ||
| S3 | 0.2164 a | 18.47 c | 2.65 a | 24.49 b | 1.77 a | 27.65 ab | 39.57 ab | ||
| S4 | 0.1985 a | 18.70 bc | 2.67 a | 24.97 ab | 1.77 a | 27.31 b | 38.50 b | ||
| 2023–24 | YN999 | S0 | 0.1471 b | 21.74 a | 2.71 c | 29.43 a | 1.78 c | 29.77 bc | 40.30 b |
| S1 | 0.1643 a | 21.13 b | 2.82 ab | 29.38 a | 1.86 ab | 29.36 c | 40.78 ab | ||
| S2 | 0.1657 a | 20.79 a | 2.80 b | 29.50 a | 1.85 b | 30.06 ab | 41.43 ab | ||
| S3 | 0.1646 a | 20.98 a | 2.84 a | 30.15 a | 1.88 a | 30.35 a | 41.88 a | ||
| S4 | 0.1623 a | 21.02 a | 2.83 a | 30.03 a | 1.87 a | 30.07 ab | 41.39 ab | ||
| JM20 | S0 | 0.1560 b | 20.81 a | 2.53 c | 26.68 c | 1.66 d | 29.40 a | 39.83 a | |
| S1 | 0.1706 a | 20.84 a | 2.67 b | 27.27 bc | 1.76 c | 29.10 a | 40.79 a | ||
| S2 | 0.1795 a | 20.52 ab | 2.77 ab | 27.38 bc | 1.83 b | 28.43 a | 40.35 a | ||
| S3 | 0.1771 a | 20.60 ab | 2.86 a | 28.63 a | 1.89 a | 28.78 a | 40.67 a | ||
| S4 | 0.1820 a | 20.46 b | 2.83 a | 27.90 ab | 1.87 ab | 28.49 a | 40.53 a | ||
| ANOVA | Y | ** | ** | ** | ** | ** | ** | ** | |
| C | ** | ** | ** | ** | ** | ** | ns | ||
| T | ** | ** | ** | ** | ** | ns | ** | ||
| Y × C | ns | ns | ns | ns | ns | * | * | ||
| Y × T | * | ns | ** | ** | ** | ns | ns | ||
| T × C | ** | ns | ** | ns | ** | ** | ns | ||
| Y × C × T | * | ns | ns | * | ns | * | ns |
| Year | Cultivar | Treatment | Gradual Increase Period | Rapid Increase Period | Slow Increase Period | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Days (D) | MG1 (mg·grain−1·d−1) | RGC1 (%) | Days (D) | MG2 (mg·grain−1·d−1) | RGC2 (%) | Days (D) | MG3 (mg·grain−1·d−1) | RGC3 (%) | |||
| 2022–23 | YN999 | S0 | 13.41 a | 0.96 a | 26.03 a | 11.77 b | 2.35 bc | 55.61 c | 12.86 b | 0.67 ab | 17.37 c |
| S1 | 13.27 a | 0.96 a | 25.38 ab | 11.81 b | 2.38 ab | 55.91 bc | 13.12 b | 0.68 a | 17.71 bc | ||
| S2 | 13.20 ab | 0.96 a | 24.87 abc | 11.97 b | 2.40 a | 56.14 abc | 13.49 b | 0.68 a | 17.99 abc | ||
| S3 | 12.65 c | 0.98 a | 23.64 c | 12.50 a | 2.37 abc | 56.57 a | 14.55 a | 0.67 ab | 18.67 a | ||
| S4 | 12.80 bc | 0.97 a | 23.90 bc | 12.61 a | 2.34 c | 56.69 ab | 14.57 a | 0.66 b | 18.52 ab | ||
| JM20 | S0 | 13.03 a | 0.91 a | 25.74 a | 12.11 a | 2.12 b | 55.73 c | 13.35 c | 0.61 c | 17.53 c | |
| S1 | 12.92 a | 0.92 a | 24.96 ab | 12.11 a | 2.20 b | 56.10 bc | 13.61 bc | 0.63 bc | 17.95 bc | ||
| S2 | 12.46 a | 0.86 ab | 21.15 c | 12.41 a | 2.37 a | 57.72 a | 15.45 a | 0.66 a | 20.13 a | ||
| S3 | 12.41 a | 0.84 b | 21.25 c | 12.12 a | 2.33 a | 57.68 a | 15.05 ab | 0.65 ab | 20.07 a | ||
| S4 | 12.82 a | 0.87 ab | 23.00 bc | 11.76 a | 2.35 a | 56.97 ab | 13.92 bc | 0.66 a | 19.03 ab | ||
| 2023–24 | YN999 | S0 | 15.70 a | 0.94 a | 28.03 a | 12.08 c | 2.39 c | 54.64 b | 12.52 b | 0.69 b | 16.33 b |
| S1 | 15.01 ab | 0.94 a | 25.75 ab | 12.25 bc | 2.48 ab | 55.71 ab | 13.52 ab | 0.71 a | 17.54 ab | ||
| S2 | 14.45 b | 0.95 a | 24.83 b | 12.68 ab | 2.46 b | 56.16 a | 14.30 a | 0.70 ab | 18.01 a | ||
| S3 | 14.57 b | 0.97 a | 24.73 b | 12.82 a | 2.50 a | 56.21 a | 14.49 a | 0.71 a | 18.06 a | ||
| S4 | 14.71 b | 0.97 a | 25.27 b | 12.62 ab | 2.49 ab | 55.96 a | 14.06 a | 0.71 a | 17.77 a | ||
| JM20 | S0 | 14.75 a | 0.89 a | 26.82 a | 12.12 a | 2.22 c | 55.23 b | 12.96 b | 0.64 b | 16.95 b | |
| S1 | 14.71 a | 0.87 a | 24.88 b | 12.27 a | 2.35 b | 56.13 a | 13.81 a | 0.67 b | 17.98 ab | ||
| S2 | 14.49 a | 0.87 a | 24.31 b | 12.06 a | 2.43 ab | 56.39 a | 13.80 a | 0.69 ab | 18.30 a | ||
| S3 | 14.49 a | 0.91 a | 24.30 b | 12.21 a | 2.51 a | 56.40 a | 13.97 a | 0.71 a | 18.30 a | ||
| S4 | 14.39 a | 0.89 a | 23.98 b | 12.13 a | 2.48 a | 56.53 a | 14.00 a | 0.70 a | 18.49 a | ||
| ANOVA | Y | ** | ns | ** | ** | ** | ** | ns | ** | ** | |
| C | ** | ** | ** | * | ** | ** | ns | ** | ** | ||
| T | ** | ns | ** | * | ** | ** | ** | ** | ** | ||
| Y × C | ns | * | ns | * | ns | ns | ns | ns | ns | ||
| Y × T | ns | ** | ns | ns | ** | ns | ns | * | ns | ||
| T × C | ns | ** | ns | ** | ** | ns | ns | ** | ns | ||
| Y × C × T | ns | ** | ns | ns | ns | ns | ns | ns | ns | ||
| Year | Cultivar | Treatment | 1000-Grain Weight (g) | Number of Grains per Spike | Number of Spikes Hectare | Grain Yield (kg ha−1) |
|---|---|---|---|---|---|---|
| 2022–23 | YN999 | S0 | 48.28 c | 36.34 a | 590.01 a | 8875.31 c |
| S1 | 49.87 b | 36.17 a | 594.12 a | 9093.07 bc | ||
| S2 | 50.98 ab | 35.95 a | 590.09 a | 9159.54 abc | ||
| S3 | 52.06 a | 35.62 a | 598.61 a | 9458.36 a | ||
| S4 | 51.62 a | 35.43 a | 591.97 a | 9266.55 ab | ||
| JM20 | S0 | 45.12 c | 31.63 a | 623.97 b | 7422.63 c | |
| S1 | 46.53 bc | 32.89 a | 638.68 ab | 8498.10 ab | ||
| S2 | 49.43 a | 32.46 a | 645.44 a | 8778.31 a | ||
| S3 | 47.85 b | 32.31 a | 647.06 a | 8389.91 b | ||
| S4 | 47.54 b | 31.88 a | 636.92 ab | 8261.97 b | ||
| 2023–24 | YN999 | S0 | 52.08 c | 35.03 a | 628.05 a | 9248.85 c |
| S1 | 53.85 b | 35.27a | 641.20 a | 9933.97 b | ||
| S2 | 55.49 a | 35.68 a | 636.85 a | 10,472.82 ab | ||
| S3 | 56.05 a | 36.02 a | 645.07 a | 10,789.21 a | ||
| S4 | 55.72 a | 35.87 a | 634.52 a | 10,442.82 ab | ||
| JM20 | S0 | 48.38 c | 32.06 a | 672.20 b | 8774.36 c | |
| S1 | 50.67 b | 32.27 a | 668.10 b | 9227.62 bc | ||
| S2 | 51.98 ab | 33.23 a | 678.89 ab | 9998.72 a | ||
| S3 | 53.33 a | 32.85 a | 691.23 a | 10,262.66 a | ||
| S4 | 52.45 a | 32.22 a | 681.08 ab | 9598.83 ab | ||
| ANOVA | Y | ** | ns | ** | ** | |
| C | ** | ** | ** | ** | ||
| T | ** | * | ns | ** | ||
| Y × C | ns | * | ns | * | ||
| Y × T | ns | * | ns | ** | ||
| T × C | ns | ns | ns | ns | ||
| Y × C × T | ns | ns | ns | ns |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duan, H.; Li, W.; Jiang, Y.; Du, Y.; Zhao, L.; Jia, J.; Liu, S.; Zhao, C. Optimizing Sulfur Fertilization for Enhanced Physiological Performance, Grain Filling Characteristics, and Grain Yield of High-Yielding Winter Wheat Under Drip Irrigation. Agriculture 2025, 15, 1012. https://doi.org/10.3390/agriculture15091012
Duan H, Li W, Jiang Y, Du Y, Zhao L, Jia J, Liu S, Zhao C. Optimizing Sulfur Fertilization for Enhanced Physiological Performance, Grain Filling Characteristics, and Grain Yield of High-Yielding Winter Wheat Under Drip Irrigation. Agriculture. 2025; 15(9):1012. https://doi.org/10.3390/agriculture15091012
Chicago/Turabian StyleDuan, Hongxiao, Wenlu Li, Yulei Jiang, Yihang Du, Ludi Zhao, Jing Jia, Shanzhang Liu, and Changxing Zhao. 2025. "Optimizing Sulfur Fertilization for Enhanced Physiological Performance, Grain Filling Characteristics, and Grain Yield of High-Yielding Winter Wheat Under Drip Irrigation" Agriculture 15, no. 9: 1012. https://doi.org/10.3390/agriculture15091012
APA StyleDuan, H., Li, W., Jiang, Y., Du, Y., Zhao, L., Jia, J., Liu, S., & Zhao, C. (2025). Optimizing Sulfur Fertilization for Enhanced Physiological Performance, Grain Filling Characteristics, and Grain Yield of High-Yielding Winter Wheat Under Drip Irrigation. Agriculture, 15(9), 1012. https://doi.org/10.3390/agriculture15091012





