Research on Optimization of the Bulb Form of the Bulb Tubular Pump Device for a Low-Head Agricultural Irrigation Pumping Station
Abstract
:1. Introduction
2. Numerical Simulation
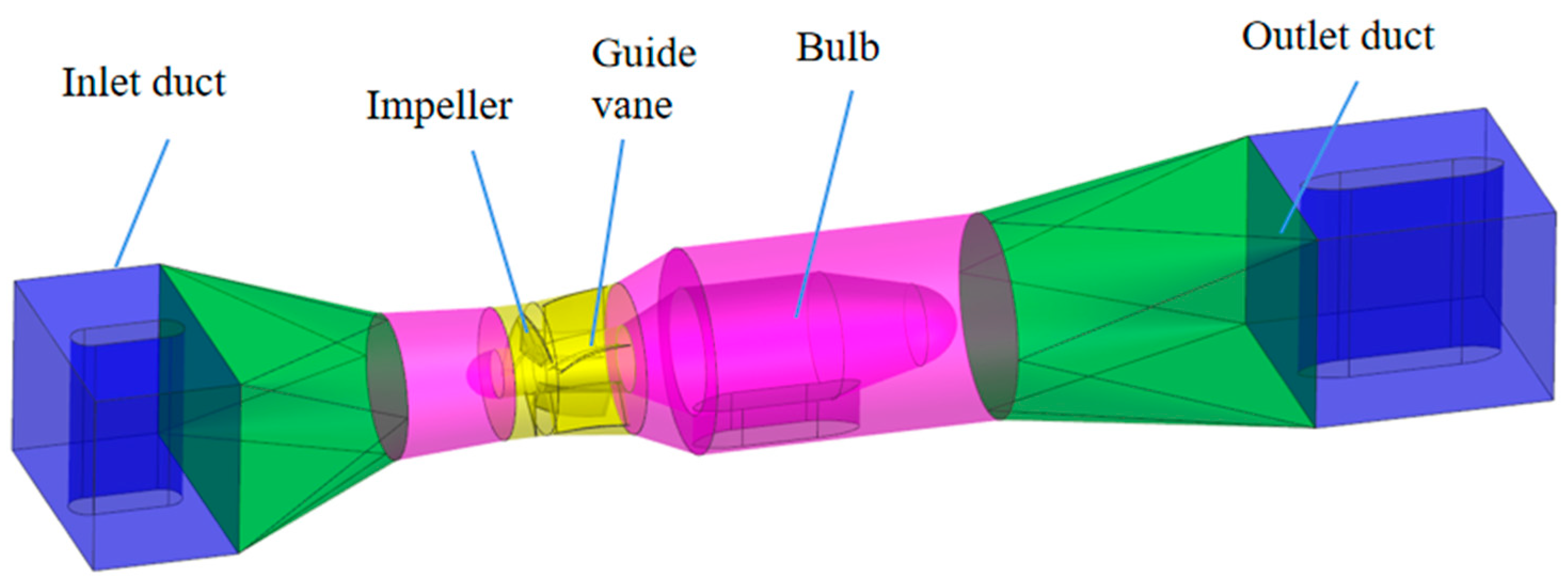
2.1. Three-Dimensional Geometric Model
2.2. Numerical Simulation
2.2.1. Calculation Method
2.2.2. Computational Grid Independence Analysis
2.2.3. Calculation Parameters and Boundary Condition Setting
2.2.4. Entropy Production Dissipation Theory
3. Analysis of Calculation Results
3.1. Analysis on Influencing Factors of Hydraulic Performance of Bulb Section
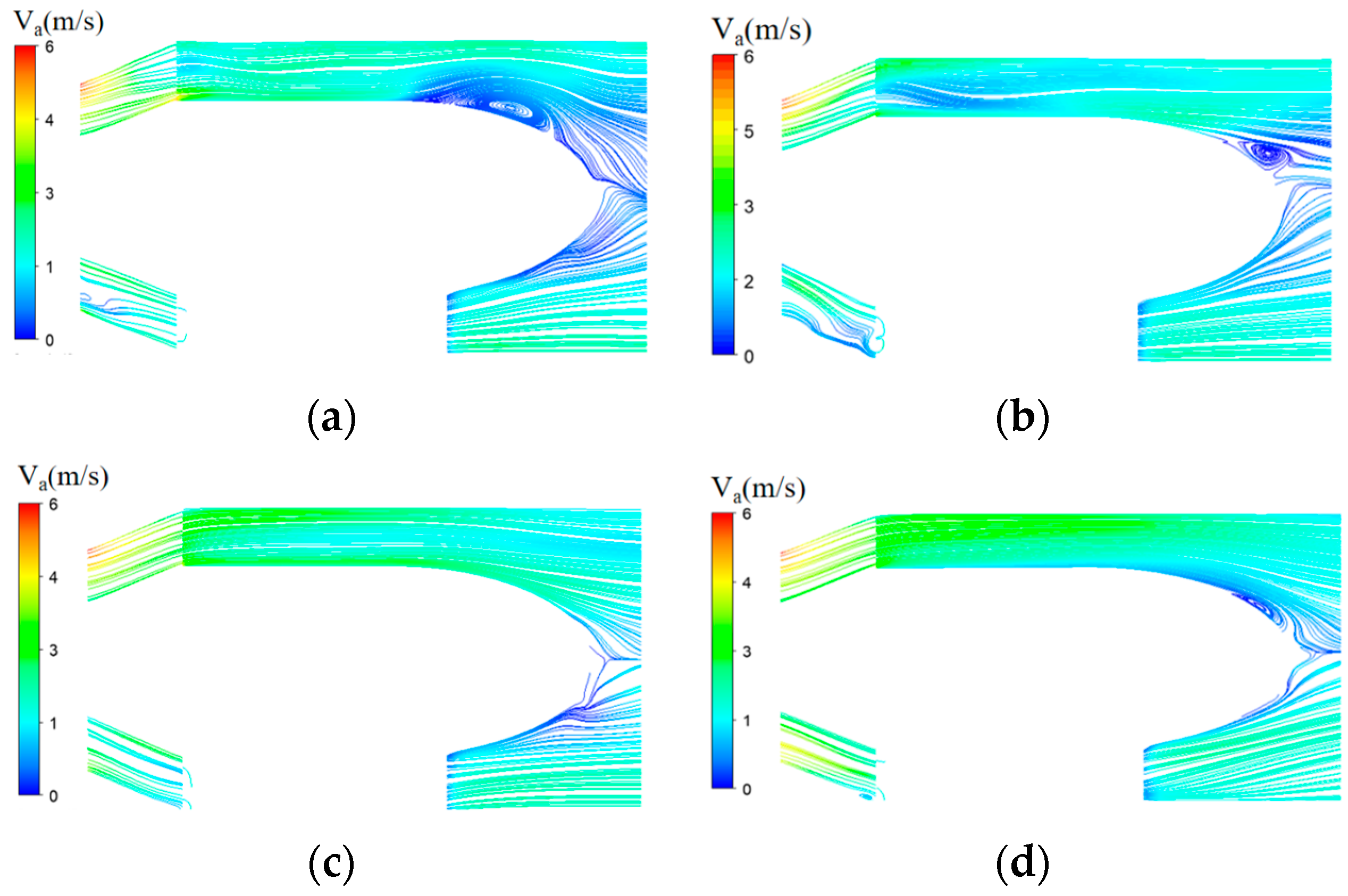
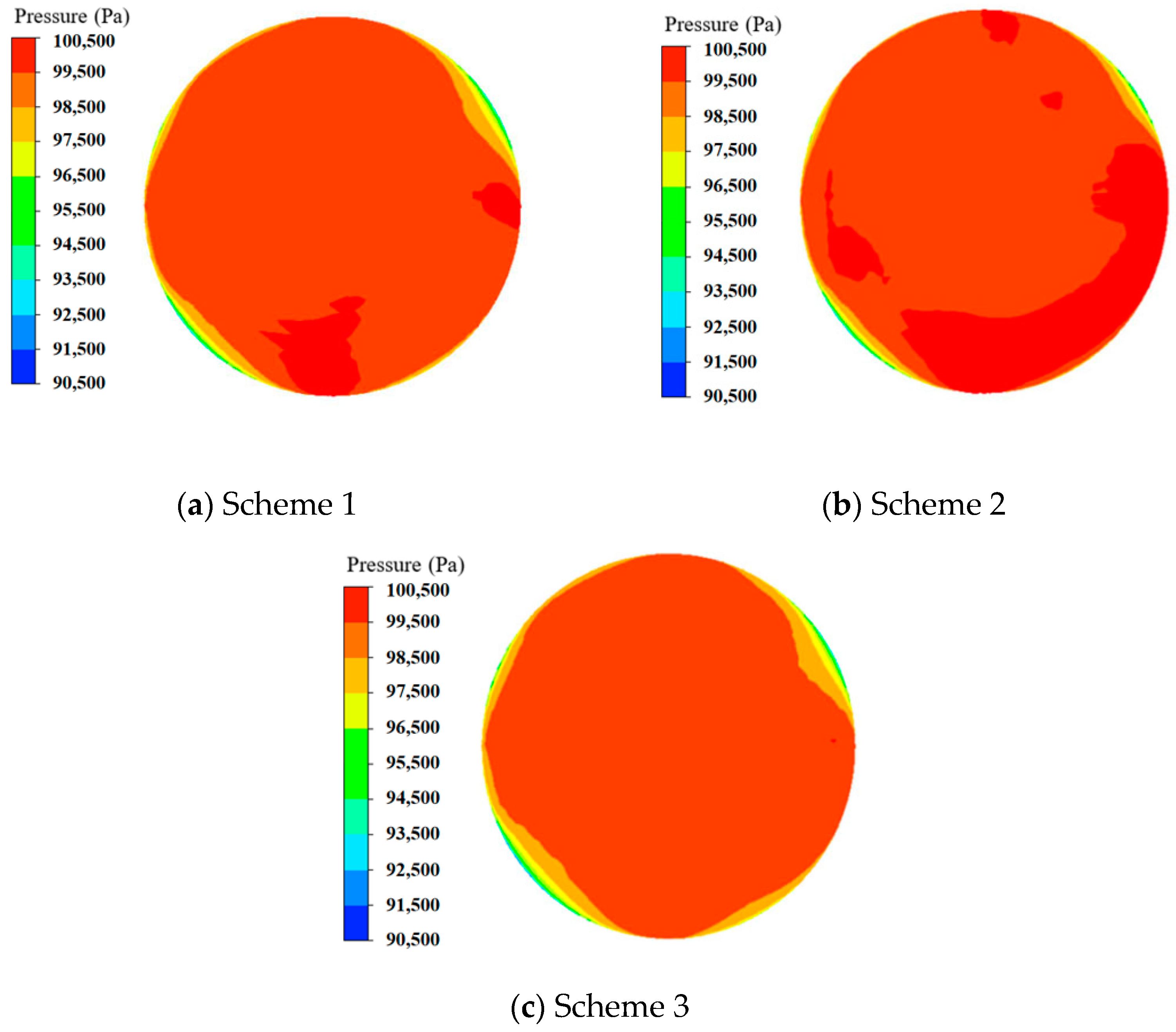
3.2. Influence of Bulb Tail Shape on Hydraulic Performance
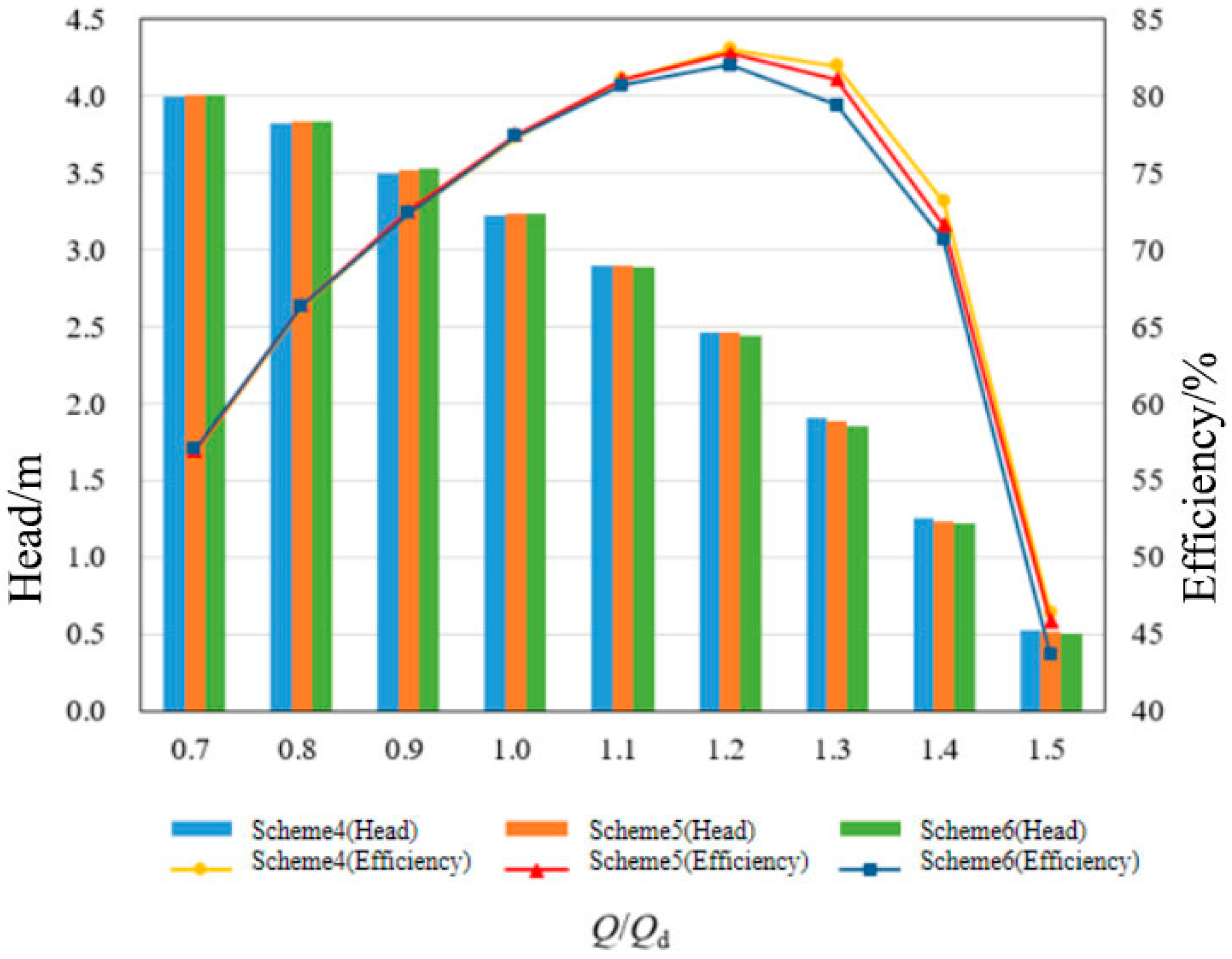
3.3. Influence of Bulb Ratio on Hydraulic Performance
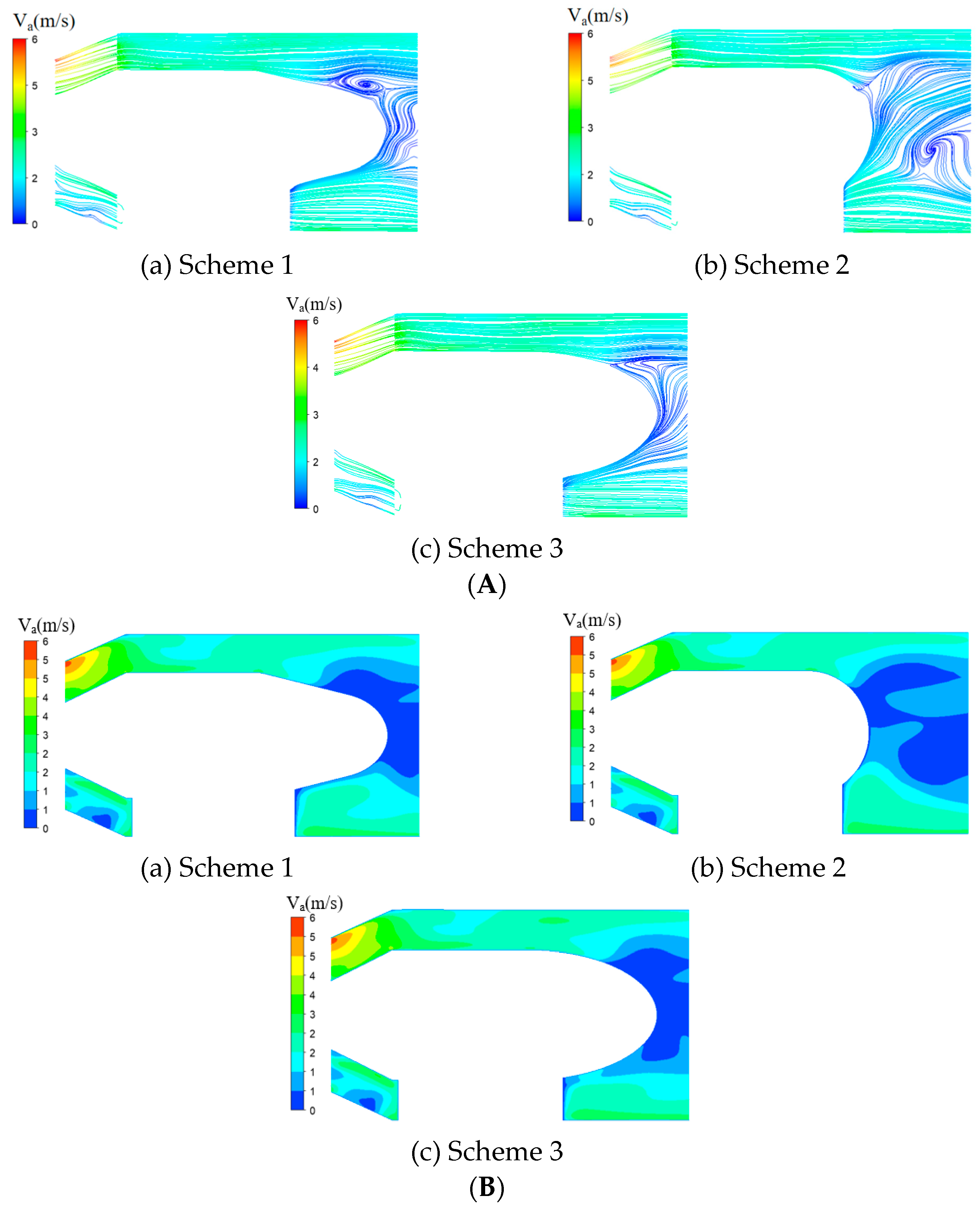
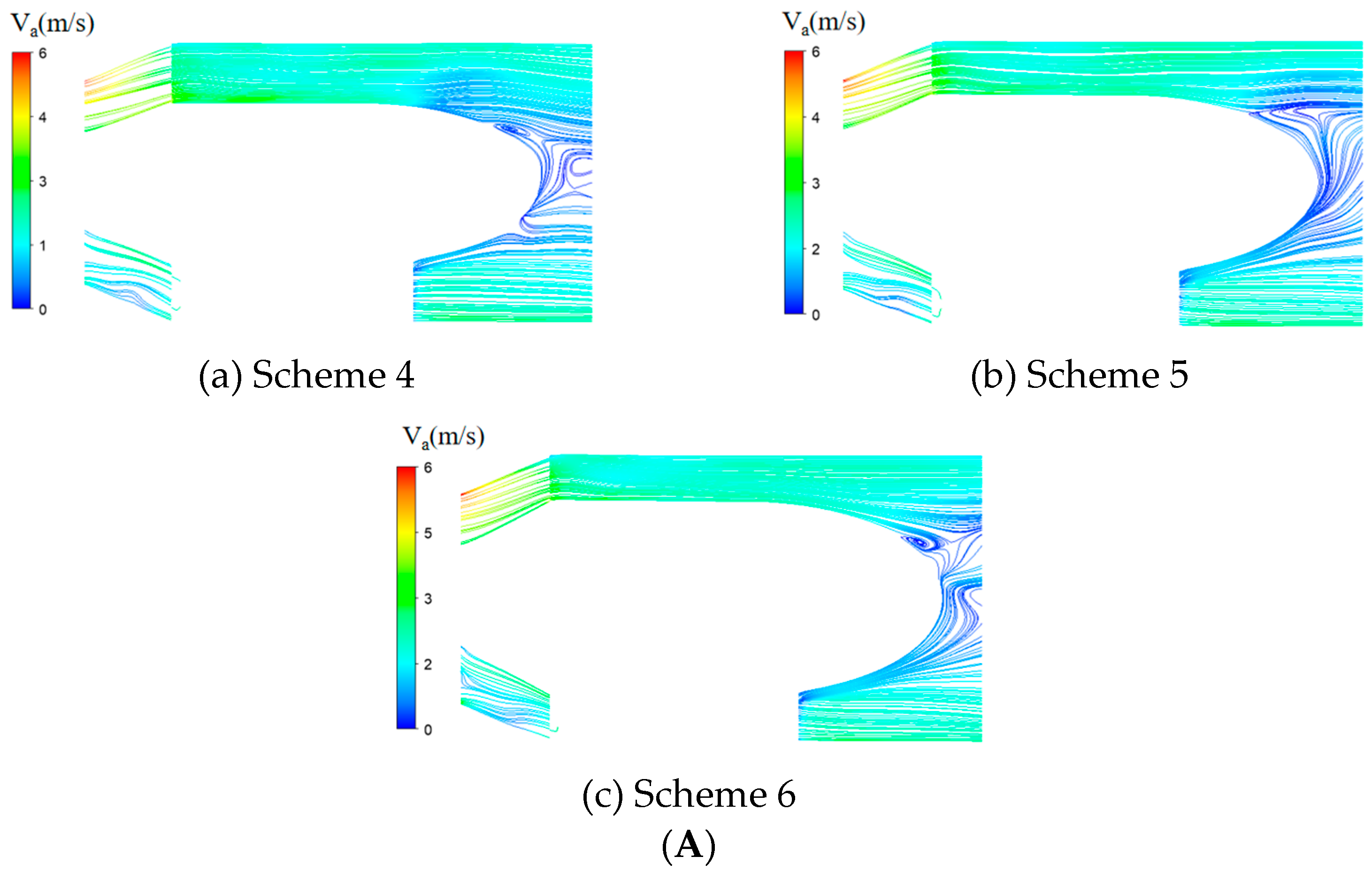
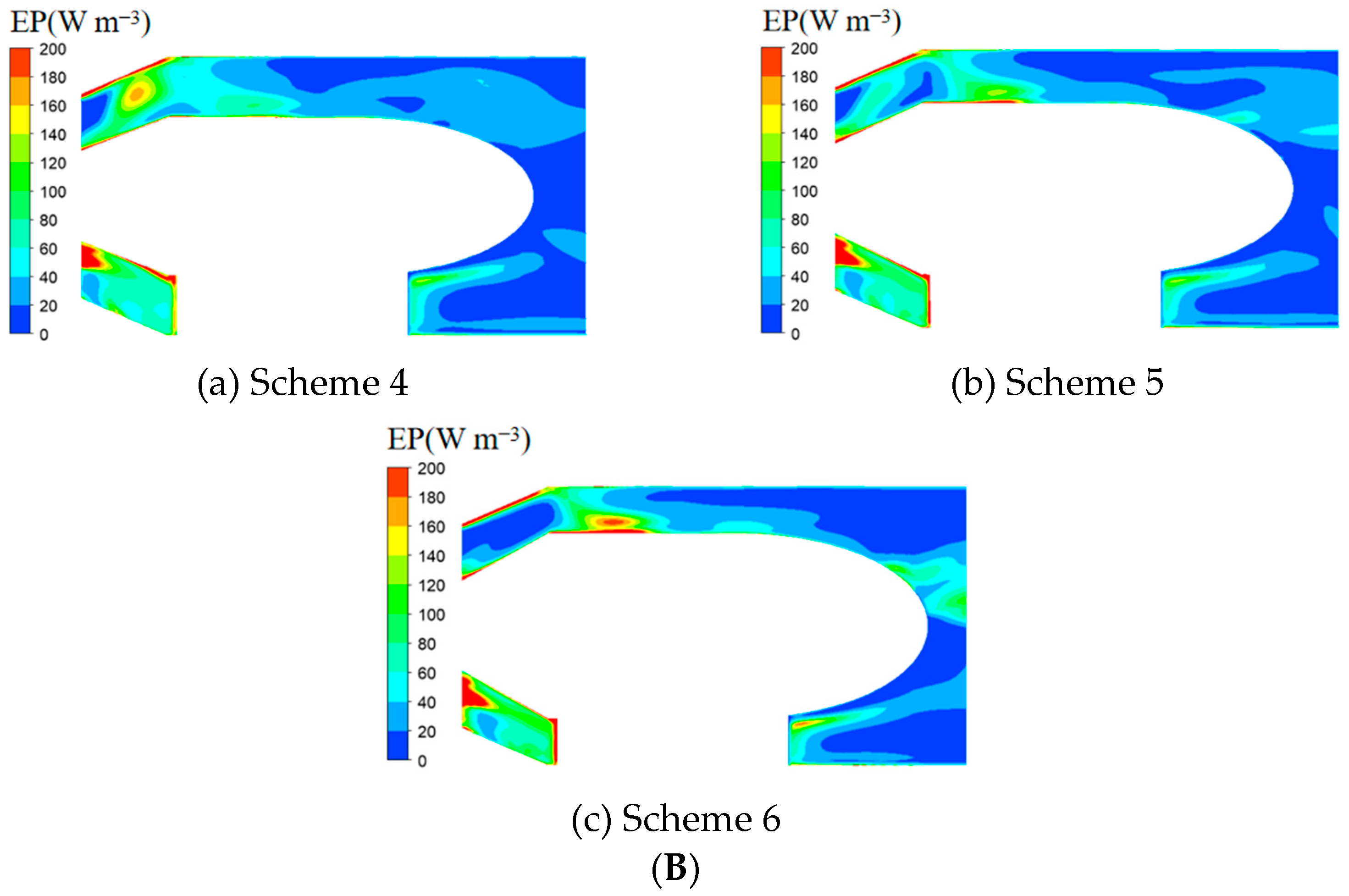
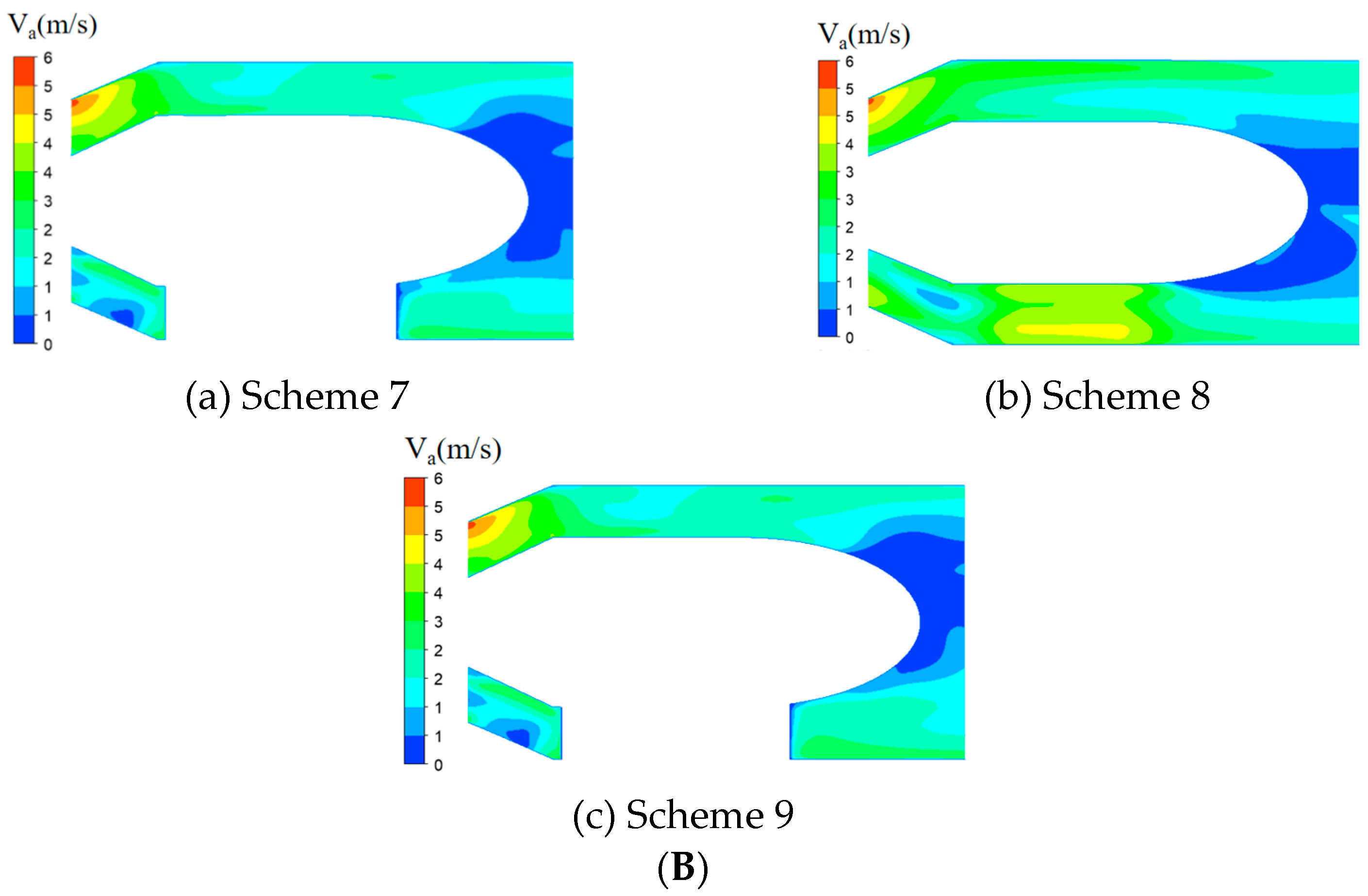
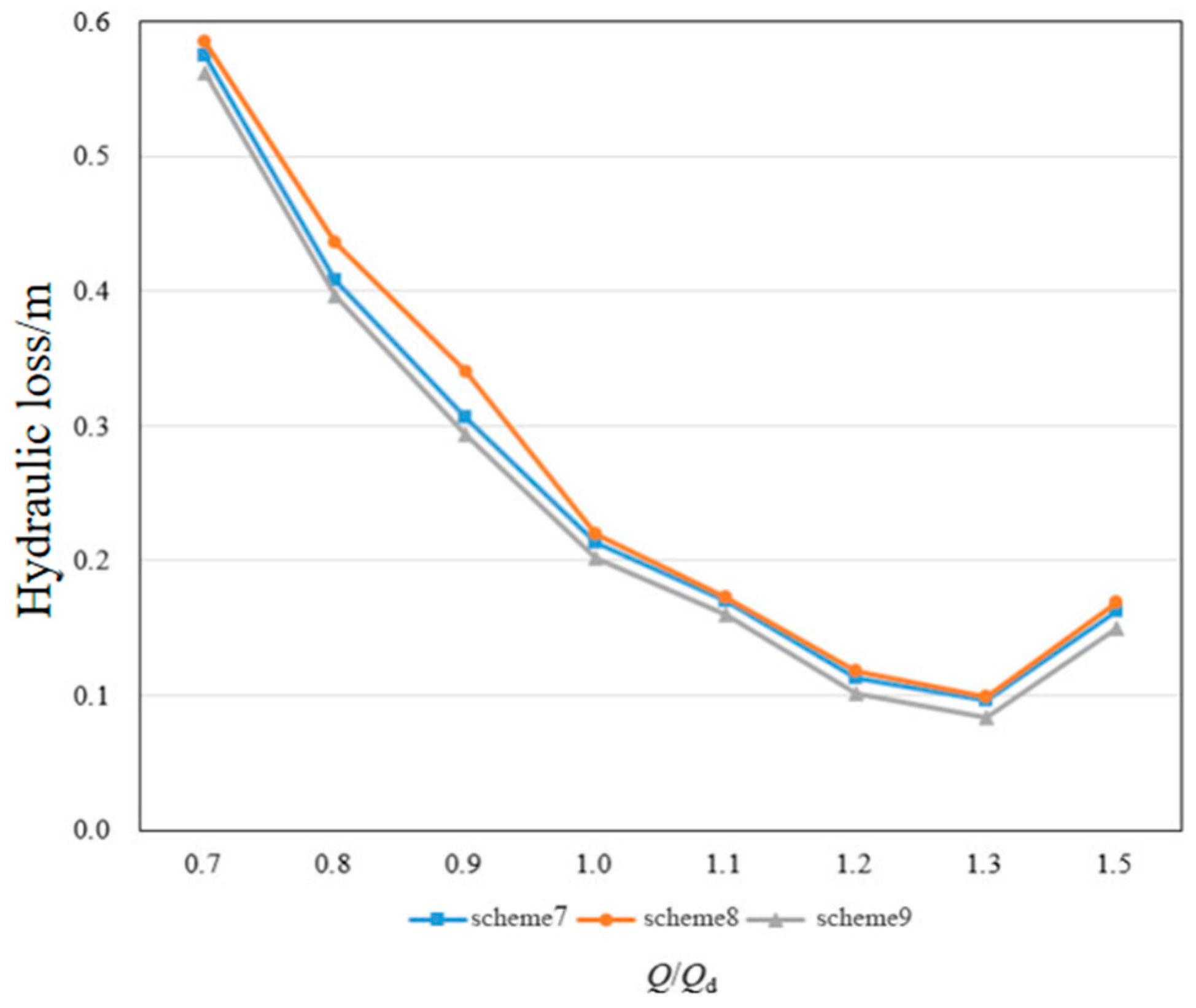
3.4. Influence of Support Shape on Hydraulic Performance
4. Conclusions
- (1)
- Because the bulb was in the key position of the flow channel, it affected the flow state in the flow channel and had a significant influence on the efficiency of the entire device. The hydraulic loss of the bulb part is about 40% of the overall hydraulic loss of the pump device, which accounts for the largest proportion of the entire pump device.
- (2)
- Different tail shapes of the bulb had different effects on the flow pattern and the performance of the entire device. The flow pattern distribution at the tail of the bulb was improved to a certain extent by using the elliptical bulb tail. This reduces the hydraulic loss of the bulb section and increases the efficiency of the device by about 5% compared to the hemisphere bulb structure.
- (3)
- The bulb ratio of the bulb with a semi-ellipsoid tail shape was small, the flow area was large, the flow pattern was smoother, and the bulb section had higher efficiency. Schemes 4 and 5 had a small tail entropy output value, small hydraulic loss, and high efficiency. Considering that the pump station was directly connected by the synchronous motor, we selected scheme 5 to facilitate the installation of the motor.
- (4)
- The bulb support is an important internal flow component of the tubular pump unit. A change in its shape had a significant influence on the flow pattern of the water in the pump unit and also on the hydraulic performance of the pump unit. Reasonable shape design of the support parts according to minimum resistance requirements effectively improved the flow pattern, reduced hydraulic losses, and improved the efficiency of the pump unit. Scheme 9’s supports had better hydraulic performance, improved the device efficiency, and achieved the optimization purpose. Therefore, we selected scheme 9 as the bulb optimization scheme. Its geometric characteristics and parameters were as follows: the shape of the bulb tail was oval, the bulb ratio was 0.96, and the shape of the supports was streamlined.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Li, W.; Huang, Y.; Ji, L.; Ma, L.; Agarwal, R.K.; Awais, M. Prediction model for energy conversion characteristics during transient processes in a mixed-flow pump. Energy 2023, 271, 127082. [Google Scholar] [CrossRef]
- Jiao, W.; Chen, H.; Cheng, L.; Zhang, B.; Gu, Y. Energy loss and pressure fluctuation characteristics of coastal two-way channel pumping stations under the ultra-low head condition. Energy 2023, 278, 127953. [Google Scholar] [CrossRef]
- Sun, L.; Pan, Q.; Zhang, D.; Zhao, R.; van Esch, B.B. Numerical study of the energy loss in the bulb tubular pump system focusing on the off-design conditions based on combined energy analysis methods. Energy 2022, 258, 124794. [Google Scholar] [CrossRef]
- Zhang, B.; Cheng, L.; Jiao, W.; Zhang, D. Experimental and statistical analysis of the flap gate energy loss and pressure fluctuation spatiotemporal characteristics of a mixed-flow pump device. Energy 2023, 272, 127117. [Google Scholar] [CrossRef]
- Jia, X.; Lv, H.; Zhu, Z. Research on the influence of impeller tip clearance on the internal flow loss of axial circulating pump under unpowered driven condition. ASME J. Fluids Eng. 2023, 145, 021202. [Google Scholar] [CrossRef]
- Zhang, B.; Yang, A.; Cheng, L.; Jiao, W.; Chen, Y.; Zhao, H. Spatial-temporal evolution and pressure fluctuation characteristics of the combined submerged vortex in a closed pump sump. Phys. Fluids 2023, 35, 065140. [Google Scholar]
- Jiao, W.; Chen, H.; Cheng, L.; Zhang, B.; Yang, Y.; Luo, C. Experimental study on flow evolution and pressure fluctuation characteristics of the underwater suction vortex of water jet propulsion pump unit in shallow water. Ocean. Eng. 2022, 266, 112569. [Google Scholar] [CrossRef]
- Song, X.J.; Luo, Y.Y.; Wang, Z.W. Numerical prediction of the influence of free surface vortex air- entrainment on pump unit performance. Ocean. Eng. 2022, 256, 111503. [Google Scholar] [CrossRef]
- Pan, Q.; Shi, W.; Zhang, D.; Van Esch, B.P. Analysis, Design, and Validation of a Vaned Diffuser for Improved Fish Friendliness. ASME J. Fluids Eng. 2022, 144, 051502. [Google Scholar] [CrossRef]
- Jiao, W.; Li, Z.; Cheng, L.; Wang, Y.; Zhang, B. Study on the Hydraulic and Energy Loss Characteristics of the Agricultural Pumping Station Caused by Hydraulic Structures. Agriculture 2022, 12, 1770. [Google Scholar] [CrossRef]
- Ji, L.; Li, W.; Shi, W.; Tian, F.; Agarwal, R. Effect of Blade Thickness on Rotating Stall of Mixed-Flow Pump Using Entropy Generation Analysis. Energy 2021, 236, 121381. [Google Scholar] [CrossRef]
- Zhou, J.R.; Cheng, L.; Liu, C.; Tang, F.P. Numerical simulation of bulb form of rear bulb penetration device. J. Disch. Irrig. Mech. Eng. 2011, 29, 72–76. [Google Scholar]
- Zhang, R.T.; Shan, H.C.; Bu, G.; Zhou, W.; Zhu, H.G.; Yao, L.B. Structural characteristics of bulb tubular pump for Phase I project of East Route of South-to-North Water Transfer. J. Drain. Irrig. Mech. Eng. 2016, 34, 774–782+789. [Google Scholar]
- Sun, Z.; Yu, J.; Tang, F. The Influence of Bulb Position on Hydraulic Performance of Submersible Tubular Pump Device. J. Mar. Sci. Eng. 2021, 9, 831. [Google Scholar] [CrossRef]
- Wang, Z.; Zheng, Y.; Wang, F.; Lin, Z.; Huan, C.; Li, Y. Experimental Study on Energy Consumption and Hydraulic Stability for Distributed Pumping System. Arab. J. Sci. Eng. 2014, 39, 6883–6894. [Google Scholar] [CrossRef]
- Zhang, X.; Tang, F.; Jiang, Y. Experimental and numerical study of reverse power generation in coastal axial flow pump system using coastal water. Ocean. Eng. 2023, 271, 113805. [Google Scholar] [CrossRef]
- Liu, A.; Zhang, C.; Zhang, Y.; Zhang, Y. Analysis of hydraulic characteristics of fluid multiplier fish pump based on CFD simulation. Ocean. Eng. 2023, 272, 113854. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, X.; Bai, L.; Yao, Y.; Wang, H.; Ji, L.; He, Z.; Song, X.; Zhou, L. Quantification and investigation of pressure fluctuation intensity in a multistage electric submersible pump. Phys. Fluids 2023, 35, 035122. [Google Scholar] [CrossRef]
- Yang, Y.; Li, W.; Shi, W.; Ping, Y.; Yang, Y.; Wang, L. Numerical investigation on the unstable flow at off-design condition in a mixed-flow pump. Proc. Inst. Mech. Eng. Part A J. Power Energy 2019, 233, 849–865. [Google Scholar] [CrossRef]
- Huang, R.; Wang, Y.; Du, T.; Luo, X.; Zhang, W.; Dai, Y. Mechanism analyses of the unsteady vortical cavitation behaviors for a waterjet pump in a non-uniform inflow. Ocean. Eng. 2021, 233, 108798. [Google Scholar] [CrossRef]
- Yang, Y.; Zhou, L.; Bai, L.; Xu, H.; Lv, W.; Shi, W.; Wang, H. Numerical Investigation of Tip Clearance Effects on the Performance and Flow Pattern within a Sewage Pump. J. Fluids Eng. Trans. ASME 2022, 144, 081202. [Google Scholar]
- Jiao, W.; Zhao, H.; Cheng, L.; Yang, Y.; Li, Z.; Wang, C. Nonlinear dynamic characteristics of suction-vortex-induced pressure fluctuations based on chaos theory for a water jet pump unit. Ocean. Eng. 2023, 268, 113429. [Google Scholar]
- Song, X.J.; Liu, C. Experiment study of the floor-attached vortices in pump sump using V3V. Renew. Energy 2021, 164, 752–766. [Google Scholar] [CrossRef]

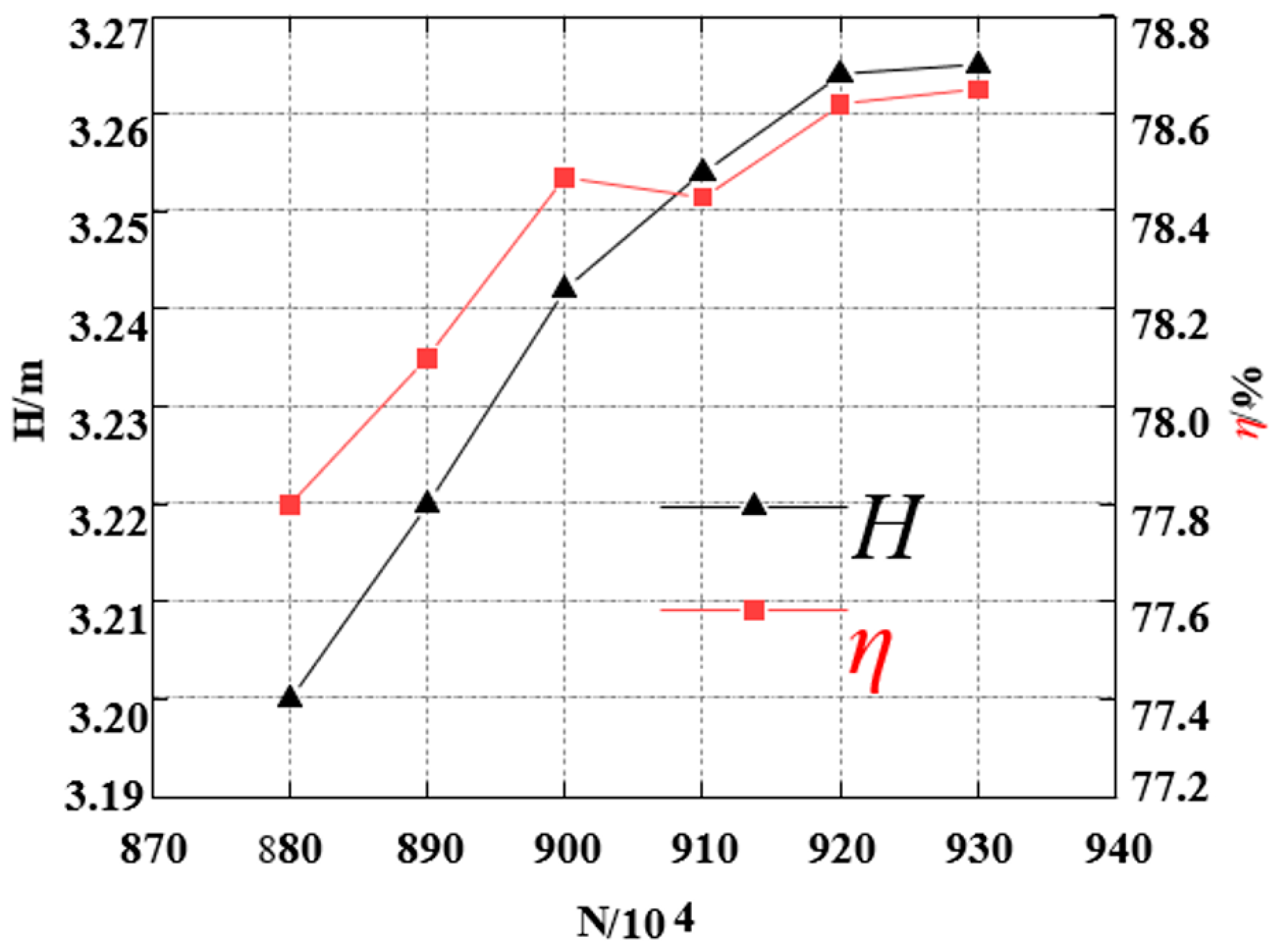




| Grid | Impeller Grid/Million | Guide Vane Grid/Million | Passage Inlet Grid/Million | Passage Outlet (Including Bulb) Grid/Million | Total Grid/Million |
|---|---|---|---|---|---|
| 1 | 1.40 | 1.80 | 2.60 | 3.00 | 8.80 |
| 2 | 1.50 | 1.80 | 2.60 | 3.00 | 8.90 |
| 3 | 1.60 | 1.80 | 2.60 | 3.00 | 9.00 |
| 4 | 1.70 | 1.80 | 2.60 | 3.00 | 9.10 |
| 5 | 1.80 | 1.80 | 2.60 | 3.00 | 9.20 |
| 6 | 1.90 | 1.80 | 2.60 | 3.00 | 9.30 |
| Flow Rate | Hydraulic Loss/M | Passage Inlet | Guide Vane | Bulb | Passage Outlet |
|---|---|---|---|---|---|
| 0.9Qd | 0.663 | 3.66 | 43.06 | 39.30 | 13.98 |
| 1.0Qd | 0.258 | 15.43 | 28.29 | 35.43 | 20.85 |
| 1.1Qd | 0.343 | 9.89 | 25.73 | 42.42 | 21.96 |
| 1.2Qd | 0.484 | 5.95 | 28.97 | 47.43 | 17.65 |
| Flow | 0.9Qd | 1.0Qd | 1.1Qd | 1.2Qd |
|---|---|---|---|---|
| Head/m | 3.56 | 3.27 | 2.89 | 2.38 |
| Efficiency/% | 73.87 | 78.75 | 82.17 | 83.28 |
| Scheme | Bulb Tail Form | Three-Dimensional Model |
|---|---|---|
| 1 | First straight-line progressive section, then semi-ellipsoid; tail contraction angle is 27 degrees |  |
| 2 | Hemisphere |  |
| 3 | Semi-ellipsoid | 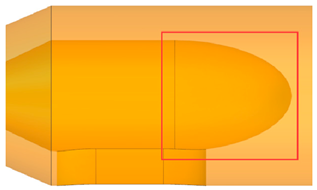 |
| Scheme | Three-Dimensional Diagram | |
|---|---|---|
| 4 | 0.88 |  |
| 5 | 0.96 |  |
| 6 | 1.04 |  |
| Scheme | Support Shape | Three-Dimensional Model |
|---|---|---|
| 7 | Original scheme |  |
| 8 | Split into two supports | 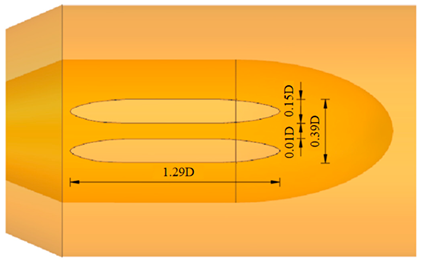 |
| 9 | Streamline design | 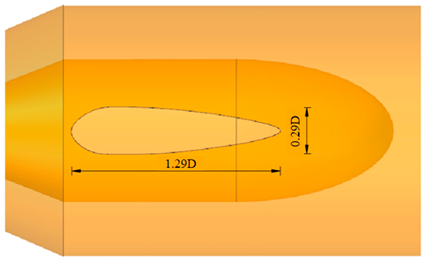 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Liu, J.; Wu, J.; Jiao, W.; Cheng, L.; Yuan, M. Research on Optimization of the Bulb Form of the Bulb Tubular Pump Device for a Low-Head Agricultural Irrigation Pumping Station. Agriculture 2023, 13, 1698. https://doi.org/10.3390/agriculture13091698
Zhang H, Liu J, Wu J, Jiao W, Cheng L, Yuan M. Research on Optimization of the Bulb Form of the Bulb Tubular Pump Device for a Low-Head Agricultural Irrigation Pumping Station. Agriculture. 2023; 13(9):1698. https://doi.org/10.3390/agriculture13091698
Chicago/Turabian StyleZhang, Hongyin, Jianlong Liu, Jinxin Wu, Weixuan Jiao, Li Cheng, and Mingbin Yuan. 2023. "Research on Optimization of the Bulb Form of the Bulb Tubular Pump Device for a Low-Head Agricultural Irrigation Pumping Station" Agriculture 13, no. 9: 1698. https://doi.org/10.3390/agriculture13091698
APA StyleZhang, H., Liu, J., Wu, J., Jiao, W., Cheng, L., & Yuan, M. (2023). Research on Optimization of the Bulb Form of the Bulb Tubular Pump Device for a Low-Head Agricultural Irrigation Pumping Station. Agriculture, 13(9), 1698. https://doi.org/10.3390/agriculture13091698










