Comparing the Carbon Storage Potential of Naturally Regenerated Tea Trees with Default New Zealand Carbon Look-Up Tables: A Case Study
Abstract
1. Introduction
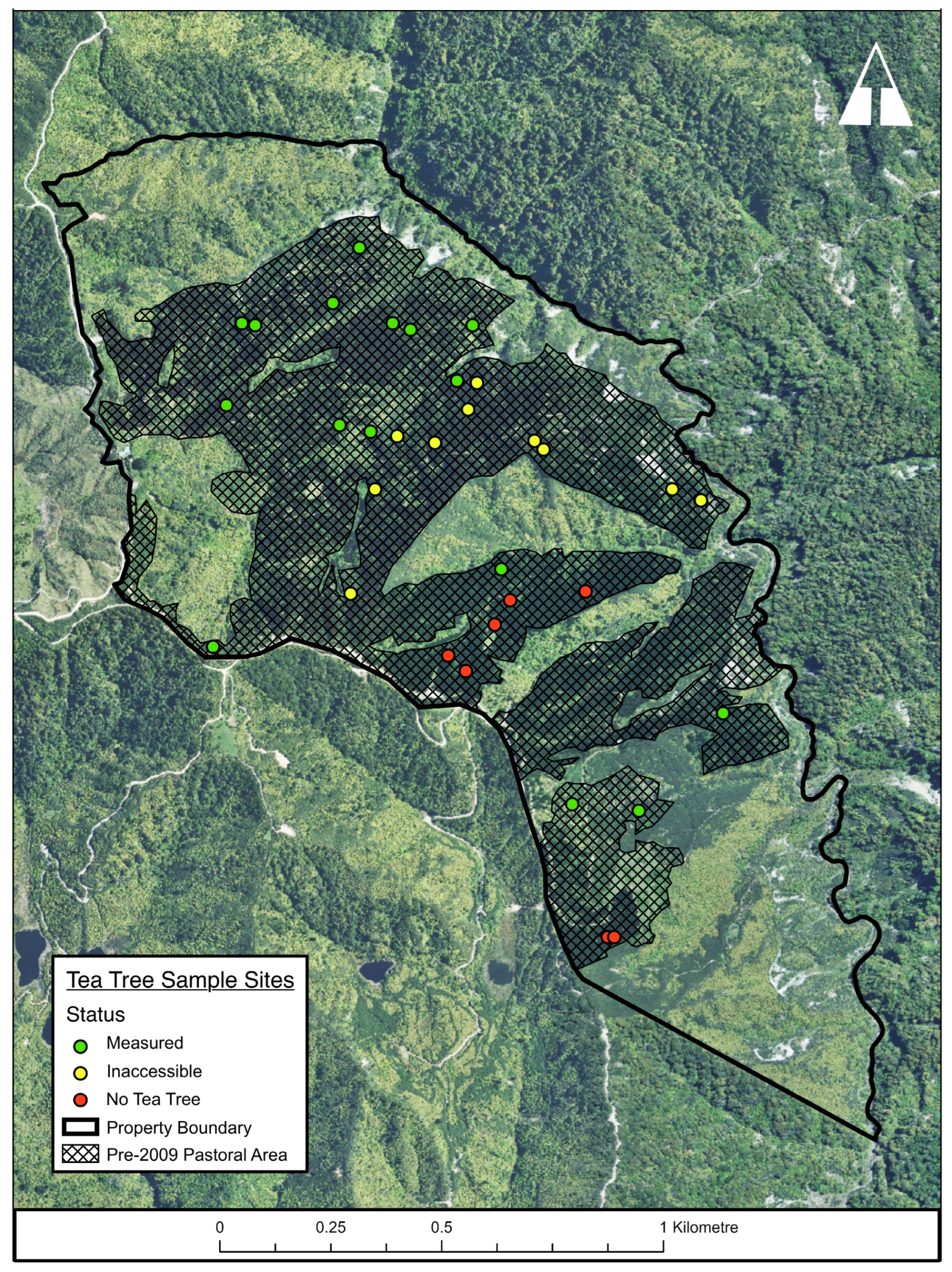
2. Materials and Methods
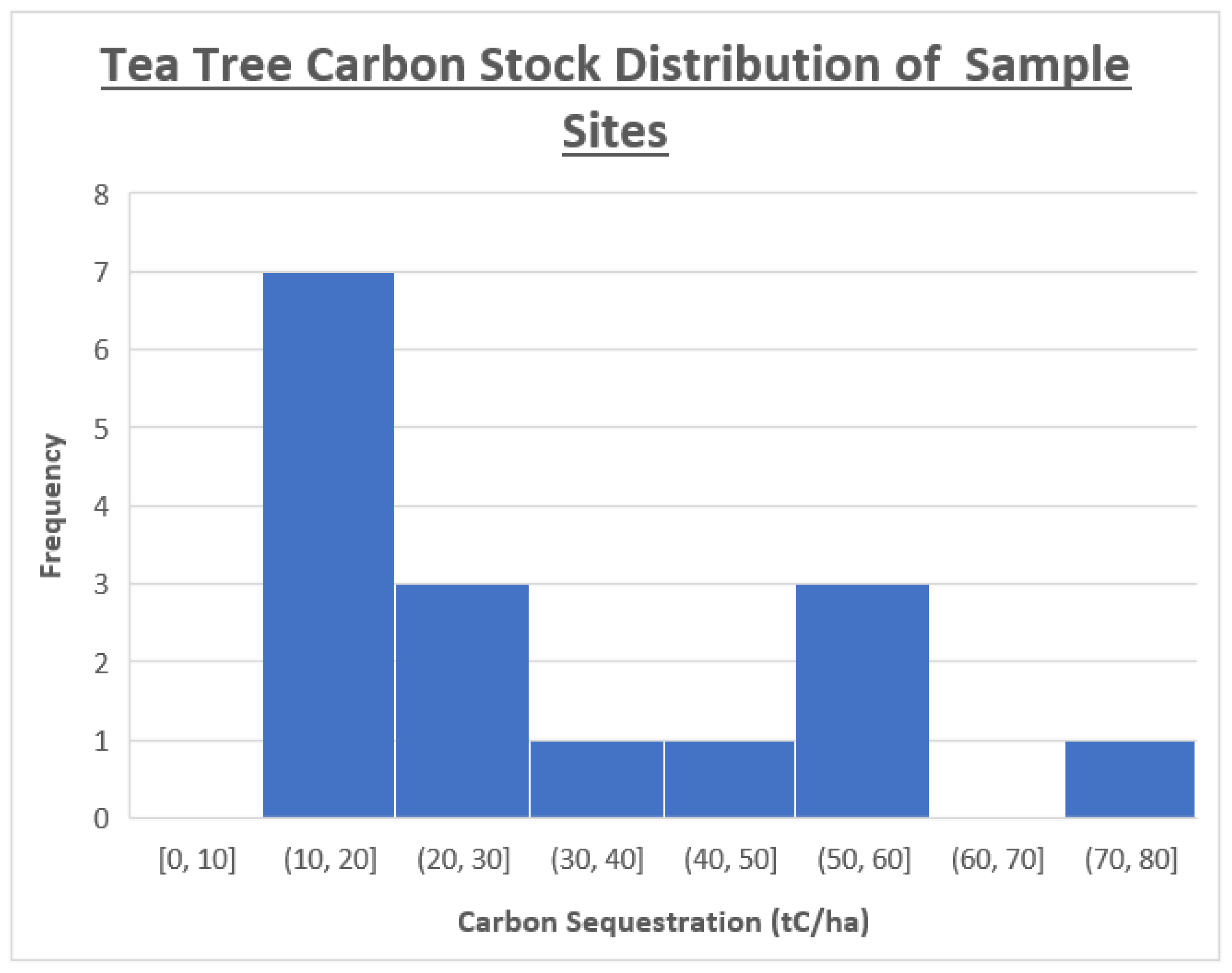
3. Results
4. Discussion
4.1. Inclusion of Unvegetated Land
4.2. Bimodal Distribution
4.3. Potential Sources of Bias
4.4. Review of Indigenous Forestry Category on the MPI Look-Up Tables
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fernandez, M.A.; Daigneault, A. The Paris Agreement and its impact on cattle and food sectors of New Zealand. N. Z. J. Agric. Res. 2016, 59, 436–443. [Google Scholar] [CrossRef]
- Fernandez, M.A.; Daigneault, A.J. Money does Grow on Trees: Impacts of the Paris Agreement on the New Zealand Economy. Clim. Chang. Econ. 2018, 9, 23. [Google Scholar] [CrossRef]
- Leahy, S.; Clark, H.; Reisinger, A. Challenges and Prospects for Agricultural Greenhouse Gas Mitigation Pathways Consistent with the Paris Agreement. Front. Sustain. Food Syst. 2020, 4, 8. [Google Scholar] [CrossRef]
- New Zealand Government. Climate Change Response Act 2002. 2919, Wellington. pp. 571p. Available online: https://faolex.fao.org/docs/pdf/nze80299.pdf (accessed on 22 February 2023).
- Wang, Y.; Sharp, B.; Poletti, S.; Nam, K.-M. Economic and land use impacts of net zero-emission target in New Zealand. Int. J. Urban Sci. 2022, 26, 291–308. [Google Scholar] [CrossRef]
- Leining, C.; Kerr, S.; Bruce-Brand, B. The New Zealand Emissions Trading Scheme: Critical review and future outlook for three design innovations. Clim. Policy 2020, 20, 246–264. [Google Scholar] [CrossRef]
- Climate Change Commission. Ināia Tonu Nei: A Low Emissions Future for AOTEAROA. 2021; Wellington; pp. 418p. Available online: https://www.climatecommission.govt.nz/public/Inaia-tonu-nei-a-low-emissions-future-for-Aotearoa/Inaia-tonu-nei-a-low-emissions-future-for-Aotearoa.pdf (accessed on 16 February 2023).
- Climate Change Commission. Progress Towards Agricultural Emissions Pricing. 2022; Wellington; pp. 25p. Available online: https://environment.govt.nz/assets/publications/Pricing-agricultural-emissions-report-under-section-215-of-the-CCRA.pdf (accessed on 16 February 2023).
- Evison, D. The New Zealand forestry sector’s experience in providing carbon sequestration services under the New Zealand Emissions Trading Scheme, 2008 to 2012. For. Policy Econ. 2017, 75, 89–94. [Google Scholar] [CrossRef]
- Kerr, S.; Anastasiadis, S.; Olssen, A.; Power, W.; Timar, L.; Zhang, W. Spatial and Temporal Responses to an Emissions Trading Scheme Covering Agriculture and Forestry: Simulation Results from New Zealand. Forests 2012, 3, 1133–1156. [Google Scholar] [CrossRef]
- Manley, B.; Maclaren, P. Potential impact of carbon trading on forest management in New Zealand. For. Policy Econ. 2012, 24, 35–40. [Google Scholar] [CrossRef]
- Kerr, S.; Ormsby, J.; White, D. Delinking the New Zealand Emissions Trading Scheme from the Kyoto Protocol: Comparing theory with practice. Clim. Policy 2021, 21, 792–803. [Google Scholar] [CrossRef]
- Diaz-Rainey, I.; Tulloch, D.J. Carbon pricing and system linking: Lessons from the New Zealand Emissions Trading Scheme. Energy Econ. 2018, 73, 66–79. [Google Scholar] [CrossRef]
- Dodd, M.B.; Rennie, G.; Kirschbaum, M.U.F.; Giltrap, D.L.; Smiley, D.; van der Weerden, T.J. Improving the economic and environmental performance of a New Zealand hill country farm catchment: 4. Greenhouse gas and carbon stock implications of land management change. N. Z. J. Agric. Res. 2021, 64, 540–564. [Google Scholar] [CrossRef]
- Ministry for Primary Industries. Carbon Look-Up Tables for Forestry in the Emissions Trading Scheme. 2017. Wellington. pp. 44p. Available online: https://www.bioenergy.org.nz/documents/resource/Carbon-look-up-tables-2017-ETS-guide.pdf (accessed on 17 February 2023).
- West, T.A.P.; Monge, J.J.; Dowling, L.J.; Wakelin, S.J.; Gibbs, H.K. Promotion of afforestation in New Zealand’s marginal agricultural lands through payments for environmental services. Ecosyst. Serv. 2020, 46, 10. [Google Scholar] [CrossRef]
- Beets, P.N.; Kimberley, M.O.; Oliver, G.R.; Pearce, S.H. The Application of Stem Analysis Methods to Estimate Carbon Sequestration in Arboreal Shrubs from a Single Measurement of Field Plots. Forests 2014, 5, 919–935. [Google Scholar] [CrossRef]
- Lambie, S.M.; Awatere, S.; Daigneault, A.; Kirschbaum, M.U.F.; Marden, M.; Soliman, T.; Spiekermann, R.I.; Walsh, P.J. Trade-offs between environmental and economic factors in conversion from exotic pine production to natural regeneration on erosion prone land. N. Z. J. For. Sci. 2021, 51, 19. [Google Scholar] [CrossRef] [PubMed]
- Beets, P.N.; Brandon, A.M.; Goulding, C.J.; Kimberley, M.O.; Paul, T.S.H.; Searles, N. The inventory of carbon stock in New Zealand’s post-1989 planted forest for reporting under the Kyoto protocol. For. Ecol. Manag. 2011, 262, 1119–1130. [Google Scholar] [CrossRef]
- Saunders, L.; Boffa Miskell Ltd. The Manuka and Kanuka Plantation Guide. 2017; pp. 86p. Available online: https://www.trc.govt.nz/assets/Documents/Guidelines/Land-infosheets/Manuka-plantation-guide-landcare-April2017.pdf (accessed on 13 February 2023).
- Morales, N.S.; Perry, G.L.W.; Burns, B.R. Fencing is not enough to reinstate regeneration: Evidence from a large fruited canopy tree Beilschmiedia tawa. For. Ecol. Manag. 2016, 376, 36–44. [Google Scholar] [CrossRef]
- Douglas, G.B.; Dodd, M.B.; Power, I.L. Potential of direct seeding for establishing native plants into pastoral land in New Zealand. N. Z. J. Ecol. 2007, 31, 143–153. Available online: http://www.newzealandecology.org/nzje/ (accessed on 25 March 2022).
- UMF Ltd. Quality Factors that Matter in Manuka Honey. 2023. Available online: https://www.umf.org.nz/unique-manuka-factor/umf-four-factor-quality-testing/on (accessed on 20 February 2023).
- Gordon, A.D.; Pont, D. Inventory estimates of stem volume using nine sampling methods in thinned Pinus radiata stands, New Zealand. N. Z. J. For. Sci. 2015, 45, 8. [Google Scholar] [CrossRef]
- Ucar, Z.; Bettinger, P.; Merry, K.; Siry, J.; Bowker, J.M.; Akbulut, R. A comparison of two sampling approaches for assessing the urban forest canopy cover from aerial photography. Urban For. Urban Green. 2016, 16, 221–230. [Google Scholar] [CrossRef]
- Scott, N.A.; White, J.D.; Townsend, J.A.; Whitehead, D.; Leathwick, J.R.; Hall, G.M.J.; Marden, M.; Rogers, G.N.D.; Watson, A.J.; Whaley, P.T. Carbon and nitrogen distribution and accumulation in a New Zealand scrubland ecosystem. Can. J. For. Res. 2000, 30, 1246–1255. [Google Scholar] [CrossRef]
- Ministry for Primary Industries. A Guide to the Field Measurement Approach for Forestry in the Emissions Trading Scheme. 2023; Wellington; pp. 94p. Available online: https://www.mpi.govt.nz/dmsdocument/3666-A-Guide-to-the-Field-Measurement-Approach-for-Forestry-in-the-Emissions-Trading-Scheme (accessed on 21 February 2023).
- Searle, E.B.; Chen, H.Y.H. Tree size thresholds produce biased estimates of forest biomass dynamics. For. Ecol. Manag. 2017, 400, 468–474. [Google Scholar] [CrossRef]
- Sullivan, M.J.P.; Lewis, S.L.; Hubau, W.; Qie, L.; Baker, T.R.; Banin, L.F.; Chave, J.; Cuni-Sanchez, A.; Feldpausch, T.R.; Lopez-Gonzalez, G.; et al. Field methods for sampling tree height for tropical forest biomass estimation. Methods Ecol. Evol. 2018, 9, 1179–1189. [Google Scholar] [CrossRef]
- Lambie, S.M.; Dando, J. Seasonal litterfall composition and carbon and nitrogen returns in New Zealand shrubland. Aust. J. Bot. 2019, 67, 610–616. [Google Scholar] [CrossRef]
- New Zealand Institute of Forestry Inc. Forestry Handbook, 4th ed.; Colley, M., Ed.; Design & Print Management Ltd.: Tauranga, New Zealand, 2005; 382p, Available online: https://nzif.org.nz/assets/NZIF-Handbook-HiRes.pdf (accessed on 20 February 2023).
- Beets, P.N.; Garrett, L.G. Carbon fraction of Pinus radiata biomass components within New Zealand. N. Z. J. For. Sci. 2018, 48, 14. [Google Scholar] [CrossRef]
- Marden, M.; Lambie, S.; Phillips, C. Potential effectiveness of low-density plantings of manuka (Leptospermum scoparium) as an erosion mitigation strategy in steeplands, northern Hawke’s Bay, New Zealand. N. Z. J. For. Sci. 2020, 50, 20. [Google Scholar] [CrossRef]
- Pedersen, R.O.; Skovsgaard, J.P. Impact of bias in predicted height on tree volume estimation: A case-study of intrinsic nonlinearity. Ecol. Model. 2009, 220, 2656–2664. [Google Scholar] [CrossRef]
- Tozer, K.; Douglas, G.; Dodd, M.; Muller, K. Vegetation Options for Increasing Resilience in Pastoral Hill Country. Front. Sustain. Food Syst. 2021, 5, 22. [Google Scholar] [CrossRef]
- Burns, B.R.; Floyd, C.G.; Smale, M.C.; Arnold, G.C. Effects of forest fragment management on vegetation condition and maintenance of canopy composition in a New Zealand pastoral landscape. Austral. Ecol. 2011, 36, 153–166. [Google Scholar] [CrossRef]
- Carver, T.; Kerr, S. Facilitating Carbon Offsets from Native Forests. 2017, pp. 6p. Available online: https://www.motu.org.nz/assets/Documents/our-work/environment-and-resources/climate-change-mitigation/emissions-trading/Native-Forest-Offset-Options-Exec-Summary.pdf (accessed on 22 February 2023).
- Funk, J.M.; Field, C.B.; Kerr, S.; Daigneault, A. Modeling the impact of carbon farming on land use in a New Zealand landscape. Environ. Sci. Policy 2014, 37, 1–10. [Google Scholar] [CrossRef]
- Norton, D.A.; Suryaningrum, F.; Buckley, H.L.; Case, B.S.; Cochrane, C.H.; Forbes, A.S.; Harcombe, M. Achieving win-win outcomes for pastoral farming and biodiversity conservation in New Zealand. N. Z. J. Ecol. 2020, 44, 9. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wilson, T.; Grafton, M.; Irwin, M. Comparing the Carbon Storage Potential of Naturally Regenerated Tea Trees with Default New Zealand Carbon Look-Up Tables: A Case Study. Agriculture 2023, 13, 856. https://doi.org/10.3390/agriculture13040856
Wilson T, Grafton M, Irwin M. Comparing the Carbon Storage Potential of Naturally Regenerated Tea Trees with Default New Zealand Carbon Look-Up Tables: A Case Study. Agriculture. 2023; 13(4):856. https://doi.org/10.3390/agriculture13040856
Chicago/Turabian StyleWilson, Thomas, Miles Grafton, and Matthew Irwin. 2023. "Comparing the Carbon Storage Potential of Naturally Regenerated Tea Trees with Default New Zealand Carbon Look-Up Tables: A Case Study" Agriculture 13, no. 4: 856. https://doi.org/10.3390/agriculture13040856
APA StyleWilson, T., Grafton, M., & Irwin, M. (2023). Comparing the Carbon Storage Potential of Naturally Regenerated Tea Trees with Default New Zealand Carbon Look-Up Tables: A Case Study. Agriculture, 13(4), 856. https://doi.org/10.3390/agriculture13040856






