Network Derivation of Liquid Junction Potentials in Single-Membrane System
Abstract
1. Introduction
2. Materials and Methods
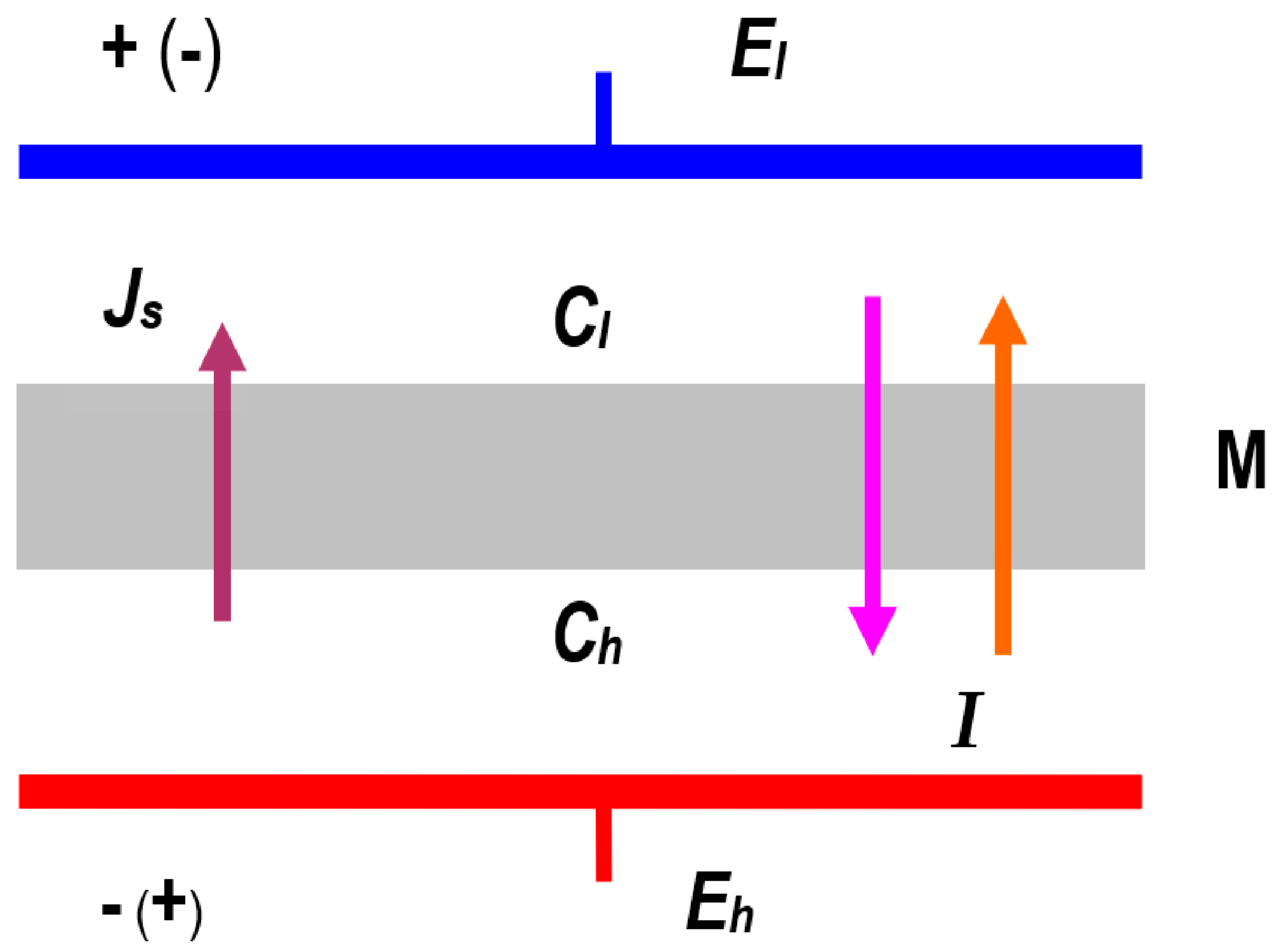
2.1. Membrane System
2.2. L Version of the Kedem–Katchalsky–Peusner Equations
2.3. Mathematical Model of Energy Conversion in the Membrane System
2.4. Biomembrane Characteristics
3. Results and Discussion
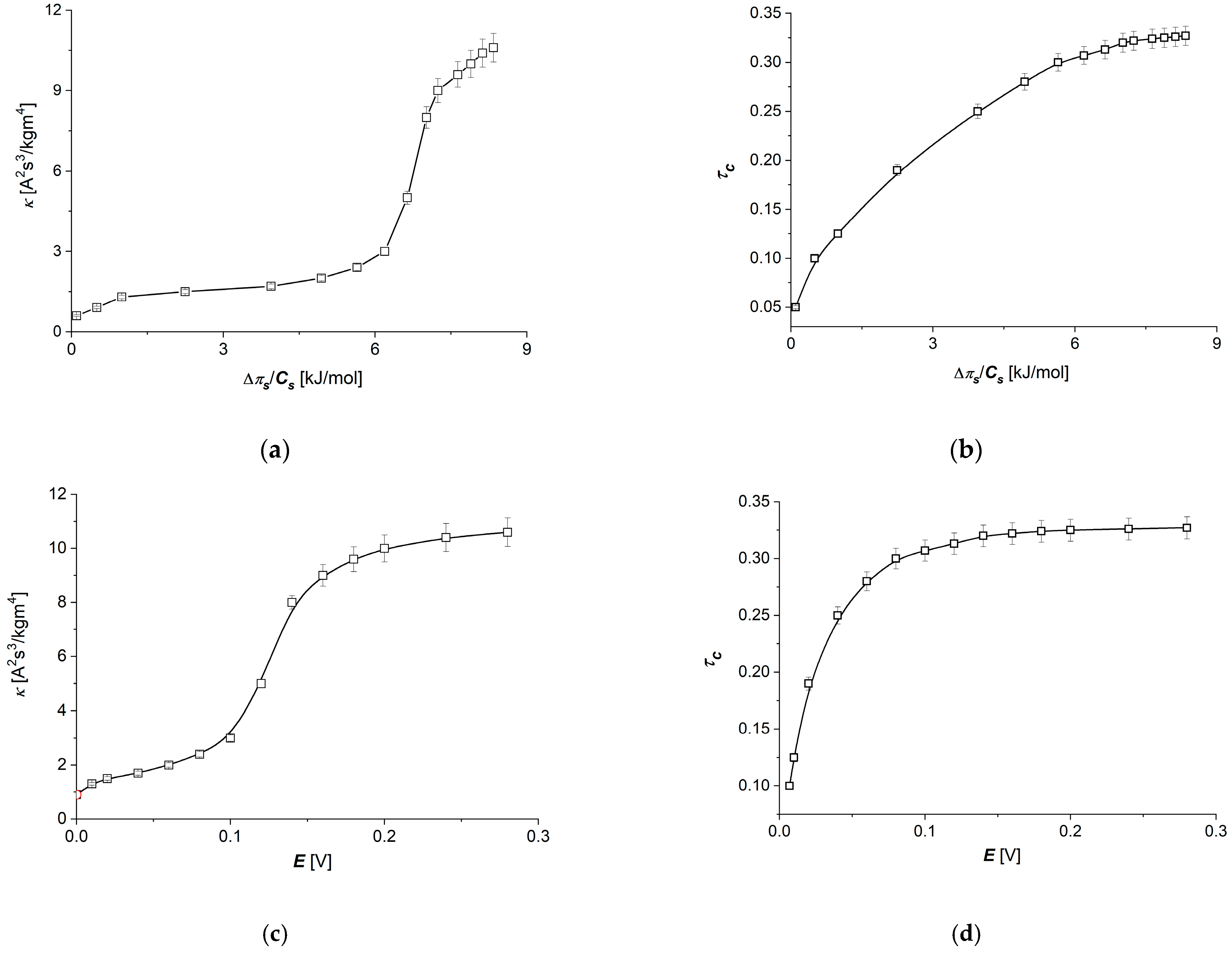
3.1. The Characteristics ) ( ∈ {1, 2}) and )
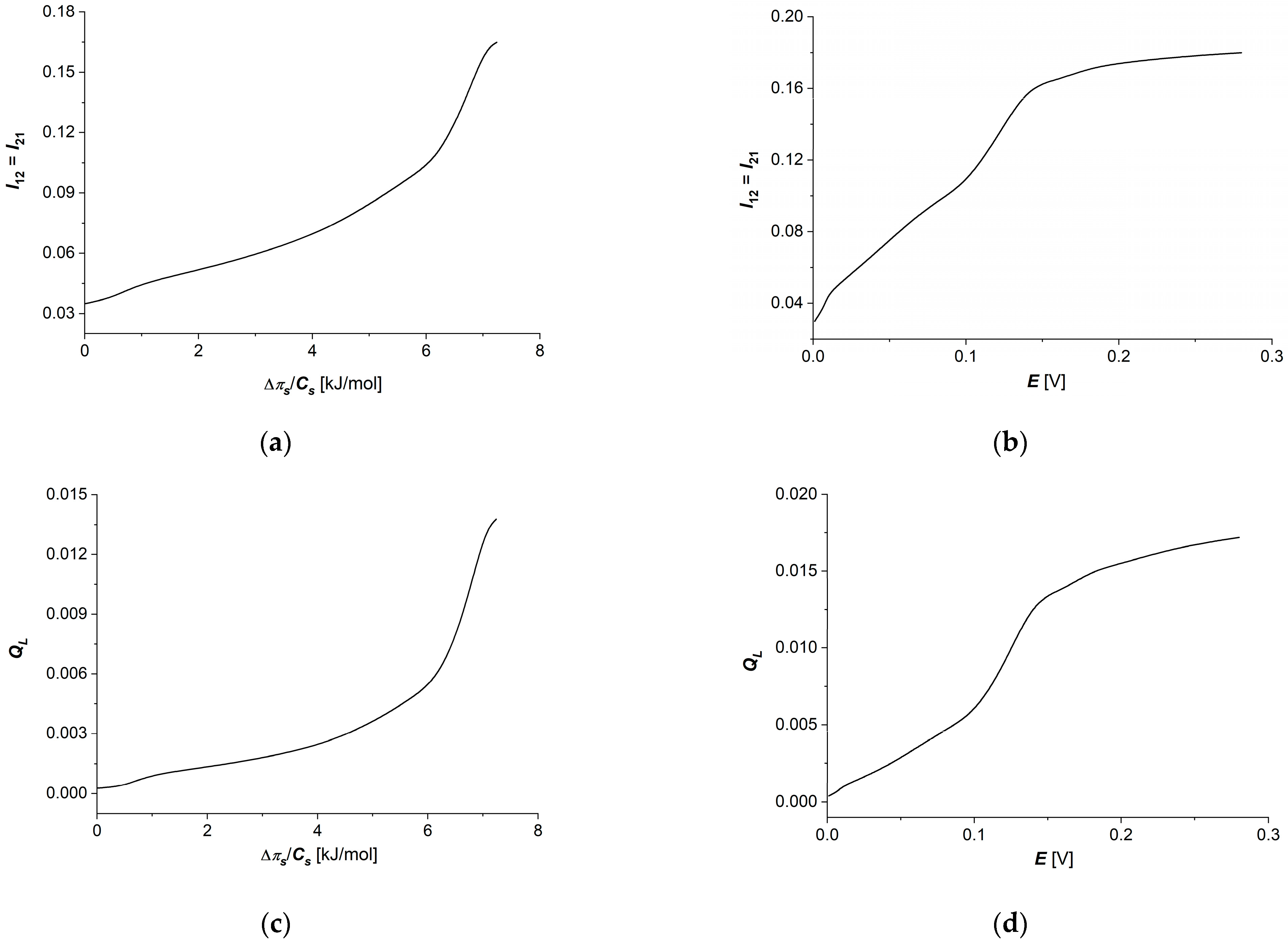
3.2. Characteristics , ( ∈ {1, 2, 3}) and
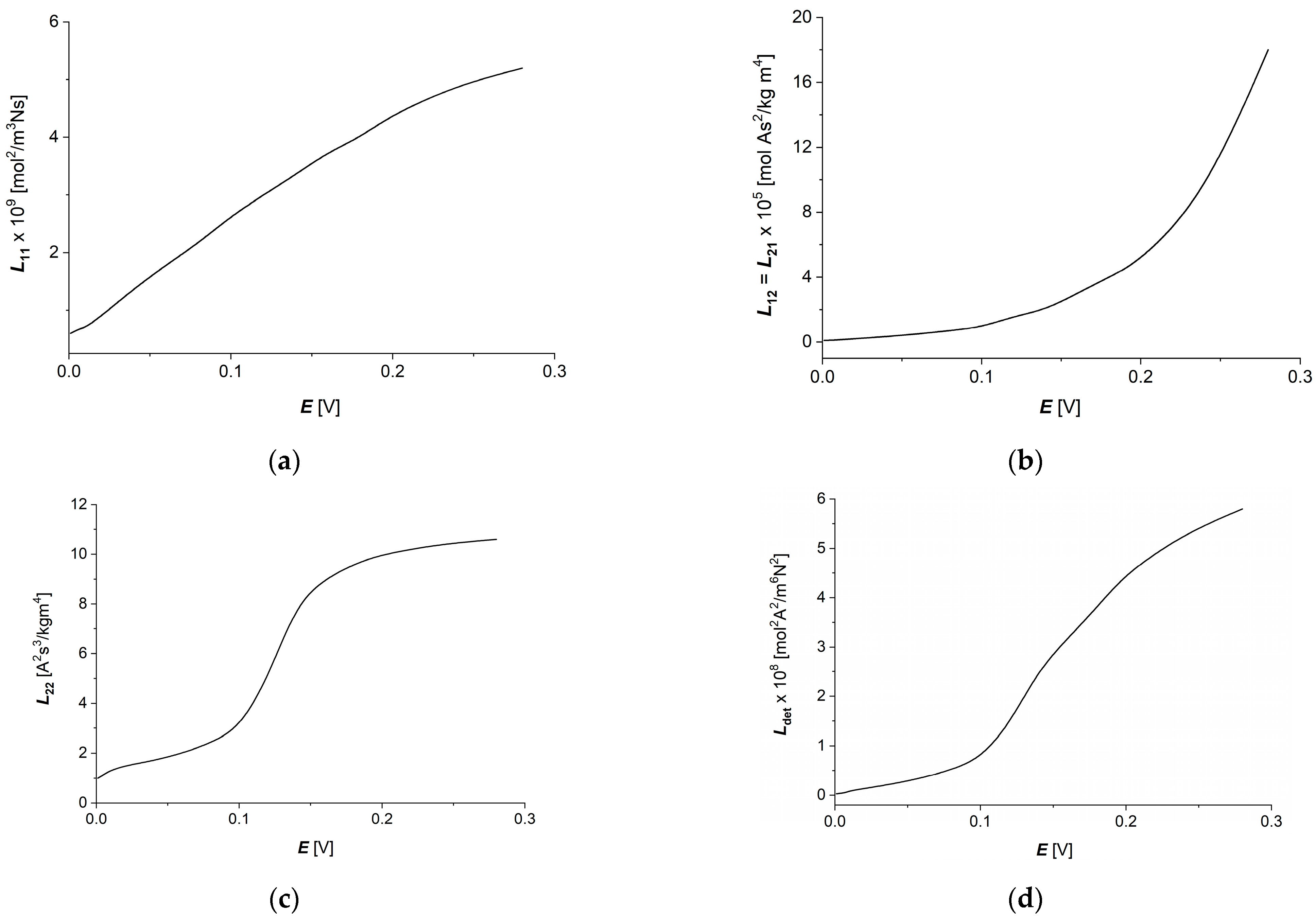
3.3. Characteristics and
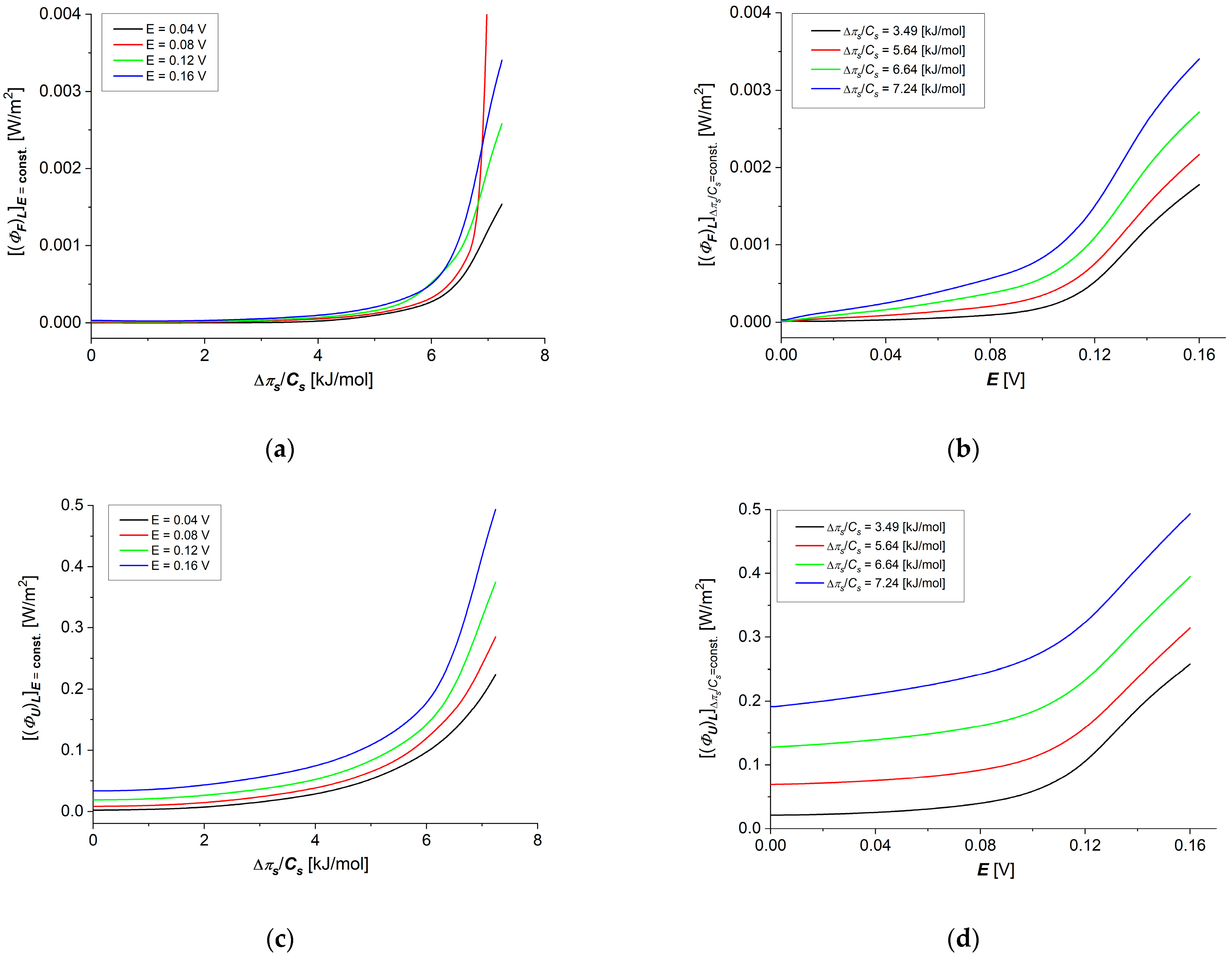
3.4. Characteristics , , and
4. Discussion
5. Conclusions
- The membrane transport of ions through an Ultra Flo 145 Dialyser membrane requires the extension of a linear model of membrane transport, for example, by forcing the dependence of the model’s transport coefficients on thermodynamic forces.
- Increases in thermodynamic forces ( or ) on the Ultra Flo 145 Dialyser membrane in the new model cause increases in all coefficients characterizing the membrane transport processes of ions and increases in energy conversion efficiency of membrane transport processes.
- Fluxes of free energy and dissipated energy also nonlinearly depend on the thermodynamic forces ( and ) used in the Ultra Flo 145 Dialyser membrane system with aqueous electrolytes solutions.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Baker, R. Membrane Technology and Application; John Wiley & Sons: New York, NY, USA, 2012. [Google Scholar]
- Radu, E.R.; Voicu, S.I.; Thakur, V.K. Polymeric membranes for biomedical applications. Polymers 2023, 15, 619. [Google Scholar] [CrossRef]
- Dorotkiewicz-Jach, A.; Markowitz, P.; Rachuna, J.; Arabski, M.; Drulis-Kawa, Z. The impact of agarose immobilization on the activity of lytic Pseudomonas araginosa phages combined with chemicals. Appl. Microbiol. Biotechnol. 2023, 107, 897–913. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Yu, L.; Zhang, X.-D.; Wang, Y.-H.; Yang, C.; Liu, X.; Wang, W.-P.; Zhang, Y.; Li, X.-T.; Li, G.; et al. A smart risk-responding polymer membrane for safer batteries. Sci. Adv. 2023, 9, eade5802. [Google Scholar] [CrossRef] [PubMed]
- Bolto, B.; Zhang, J.; Wu, X.; Xie, Z. A review on current development of membranes for oil removal from wastewaters. Membranes 2020, 10, 65. [Google Scholar] [CrossRef] [PubMed]
- Yahya, L.A.; Tobiszewski, M.; Kubica, P.; Koronkiewicz, S.; Vakh, C. Polymeric porous membranes as solid support and protective material in microextraction processes: A review. TrAC Trends Anal. Chem. 2024, 173, 117651. [Google Scholar] [CrossRef]
- Demirel, Y. Nonequilibrium Thermodynamics: Transport and Rate Processes in Physical, Chemical and Biological Systems, 2nd ed.; Elsevier: Amsterdam, The Netherlands; Heidelberg, Germany, 2007. [Google Scholar]
- Batko, K.M.; Ślęzak-Prochazka, I.; Ślęzak, A.; Bajdur, W.M.; Włodarczyk-Makuła, M. Management of energy conversion processes in membrane systems. Energies 2022, 15, 1661. [Google Scholar] [CrossRef]
- Katchalsky, A.; Curran, P.F. Nonequilibrium Thermodynamics in Biophysics; Harvard University Press: Cambridge, UK, 1965. [Google Scholar]
- Paynter, H. Analysis and Design of Engineering Systems; MIT: Cambrigde, MA, USA, 1961. [Google Scholar]
- Meixner, J. Thermodynamics of electrical networks and Onsager-Casimir reciprocal relation. J. Math. Phys. 1963, 4, 154–159. [Google Scholar] [CrossRef]
- Peusner, L. The Principles of Network Thermodynamics and Biophysical Applications. PhD Thesis, Harvard University, Cambridge, MA, USA, 1970. [Google Scholar]
- Oster, G.F.; Perelson, A.; Katchalsky, A. Network Thermodynamics. Nature 1971, 234, 393–399. [Google Scholar] [CrossRef]
- Peusner, L. Hierarchies of irreversible energy conversion systems: A network thermodynamic approach. I. Linear steady state without storage. J. Theor. Biol. 1983, 102, 7–39. [Google Scholar] [CrossRef]
- Peusner, L. Hierarchies of irreversible energy conversion systems. II. Network derivation of linear transport equations. J. Theor. Biol. 1985, 115, 319–335. [Google Scholar] [CrossRef]
- Peusner, L. Studies in Network Thermodynamics; Elsevier: Amsterdam, The Netherlands; New York, NY, USA, 1986. [Google Scholar]
- Batko, K.M.; Slezak-Prochazka, I.; Grzegorczyn, S.; Ślęzak, A. Membrane transport in concentration polarization conditions: Network thermodynamics model equations. J. Porous Media 2014, 17, 573–586. [Google Scholar] [CrossRef]
- Ślęzak, A.; Grzegorczyn, S.; Batko, K.M.; Bajdur, W.M.; Włodarczyk-Makuła, M. Applicability of the Lr form of the Kedem–Katchalsky–Peusner equations for membrane transport in water purification technology. Desalination Water Treat. 2020, 202, 48–260. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Mamedov, M.M. Phenomenological derivation of the Onsager reciprocal relations. Tech. Phys. Lett. 2003, 29, 676–678. [Google Scholar] [CrossRef]
- Filippov, A.N. A Cell model of an ion-exchange membrane. capillary-osmosis and reverse-osmosis coefficients. Colloid J. 2022, 84, 332–343. [Google Scholar] [CrossRef]
- Kedem, O.; Caplan, S.R. Degree of coupling and its relation to efficiency of energy conversion. Trans. Faraday Soc. 1965, 61, 1897–1911. [Google Scholar] [CrossRef]
- Caplan, S.R. Nonequilibrium thermodynamics and its application to bioenergetics. Curr. Top. Bioenerg. 1971, 4, 1–79. [Google Scholar] [CrossRef]
- Twardowski, Z.J. History of hemodialyzers’ designs. Hemodial. Int. 2008, 12, 173–210. [Google Scholar] [CrossRef]
- Grzegorczyn, S. Effects of Concentration Polarization of Flat Bacterial Cellulose Membranes; Silesian Medical Academy Press: Katowice, Poland, 2006. (In Polish) [Google Scholar]
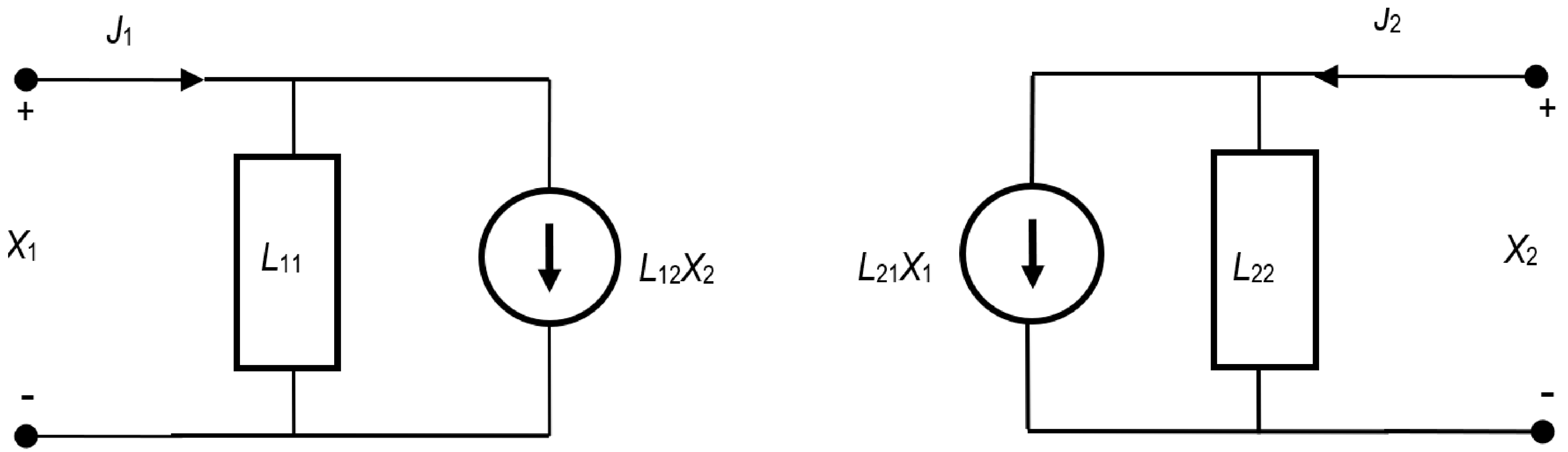





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ślęzak, A.; Grzegorczyn, S.M. Network Derivation of Liquid Junction Potentials in Single-Membrane System. Membranes 2024, 14, 140. https://doi.org/10.3390/membranes14060140
Ślęzak A, Grzegorczyn SM. Network Derivation of Liquid Junction Potentials in Single-Membrane System. Membranes. 2024; 14(6):140. https://doi.org/10.3390/membranes14060140
Chicago/Turabian StyleŚlęzak, Andrzej, and Sławomir M. Grzegorczyn. 2024. "Network Derivation of Liquid Junction Potentials in Single-Membrane System" Membranes 14, no. 6: 140. https://doi.org/10.3390/membranes14060140
APA StyleŚlęzak, A., & Grzegorczyn, S. M. (2024). Network Derivation of Liquid Junction Potentials in Single-Membrane System. Membranes, 14(6), 140. https://doi.org/10.3390/membranes14060140





