Design of a Reverse Electrodialysis Plant for Salinity Gradient Energy Extraction in a Coastal Wastewater Treatment Plant
Abstract
1. Introduction
2. Materials and Methods
2.1. Definition of the WWTP Sites Addressed
- (1)
- S1, WWTP of Comillas, located in the North of Spain (ES). This facility has a treatment capacity of 35,200 population equivalent (p.e.), and it is located in the coastal area of the municipality of Comillas, next to the Cantabrian Sea.
- (2)
- S2, WWTP of Ierapetra, located in Greece (GR). This medium-sized plant has a treatment capacity of 25,700 p.e. and is located on the warm coast of the Mediterranean Sea.
2.2. Techno-Economic Assessment
3. Results and Discussion
3.1. NPV Optimization
- S1 − ES − 4 EUR/m2 − winter (case 1)/summer (case 3) = 29/21 RUs
- S2 − GR − 4 EUR/m2 − winter (case 5)/summer (case 9) = 58/58 RUs
- S2 − GR − 10 EUR/m2 − winter (case 6)/summer (case 10) = 30/32 RUs
- S2 − EU − 4 EUR/m2 − winter (case 7)/summer (case 11) = 53/54 RUs
- S2 − EU − 10 EUR/m2 − winter (case 8)/summer (case 12) = 22/24 RUs
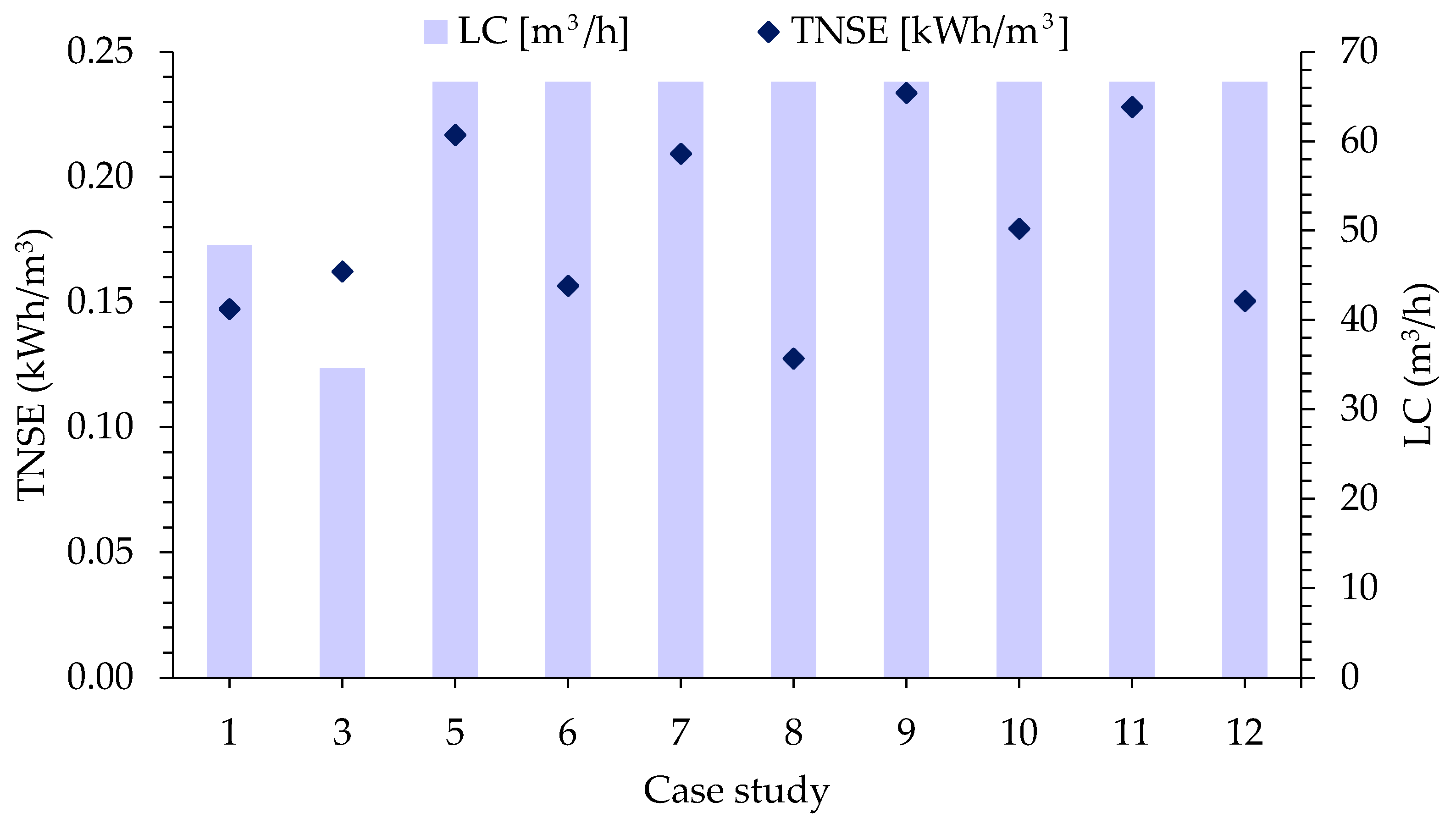
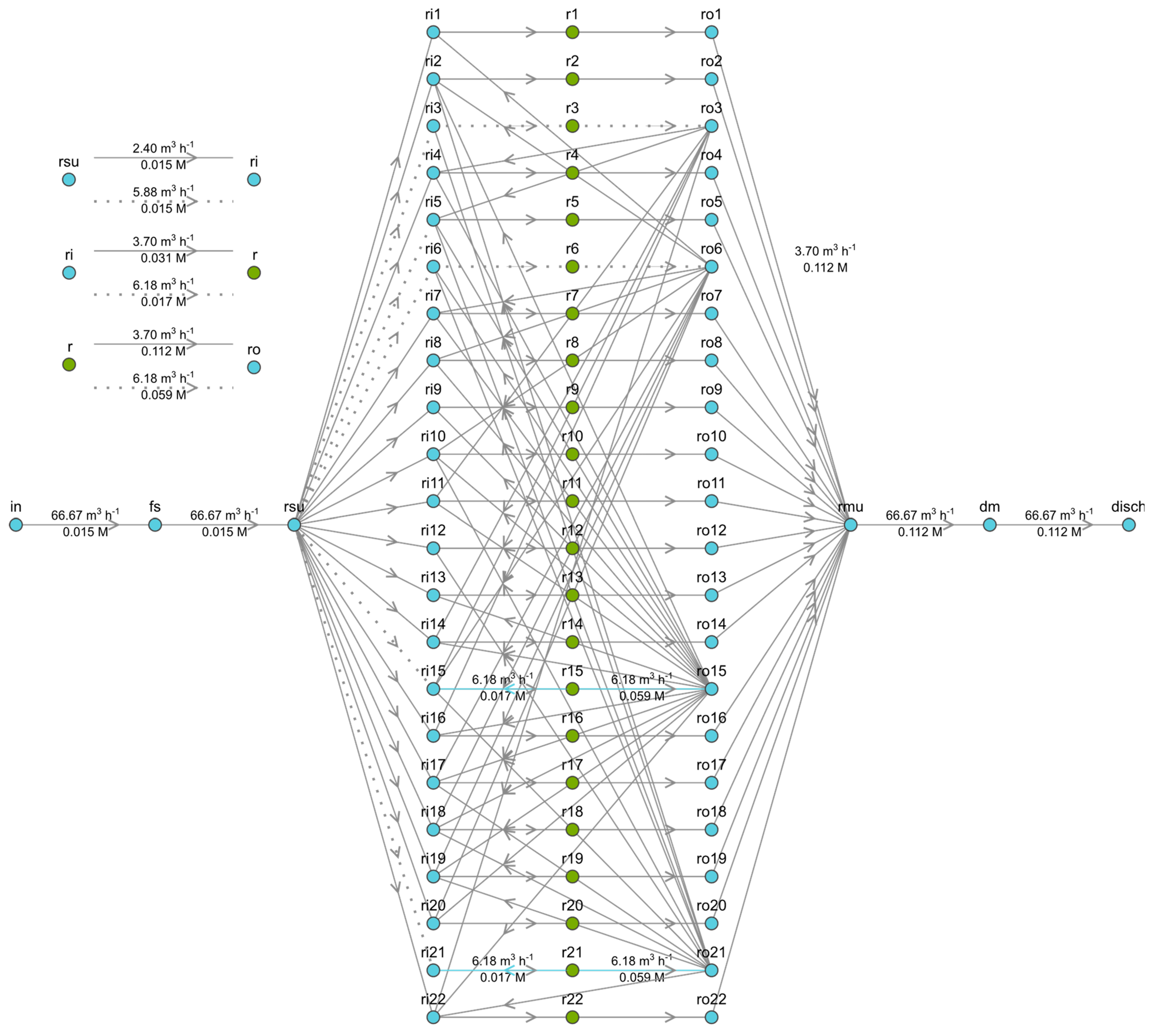
3.2. Optimal RU Configuration
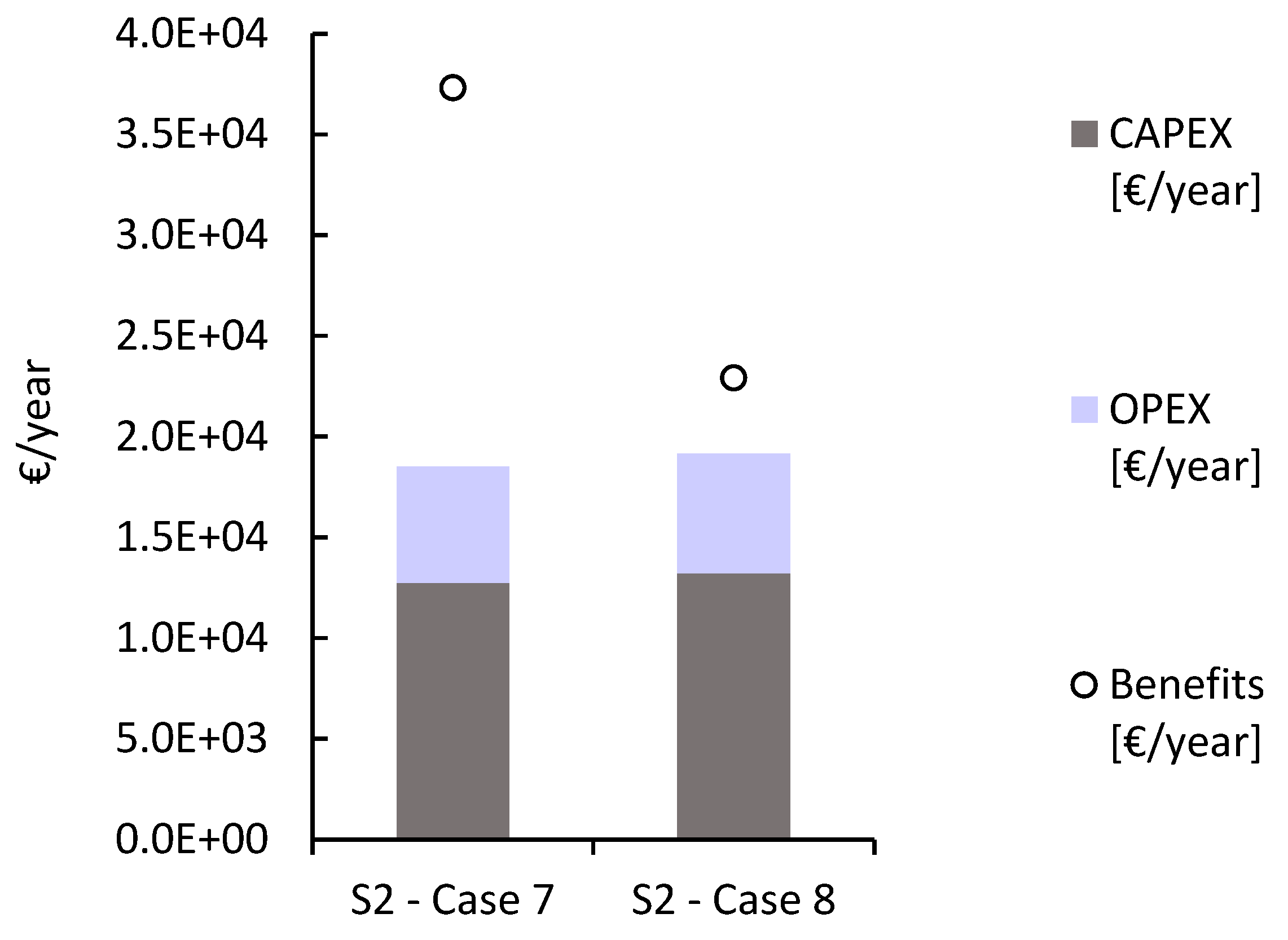
3.3. CAPEX/OPEX Analysis
3.4. Economic Comparison of SGE-RED on the Energy Market
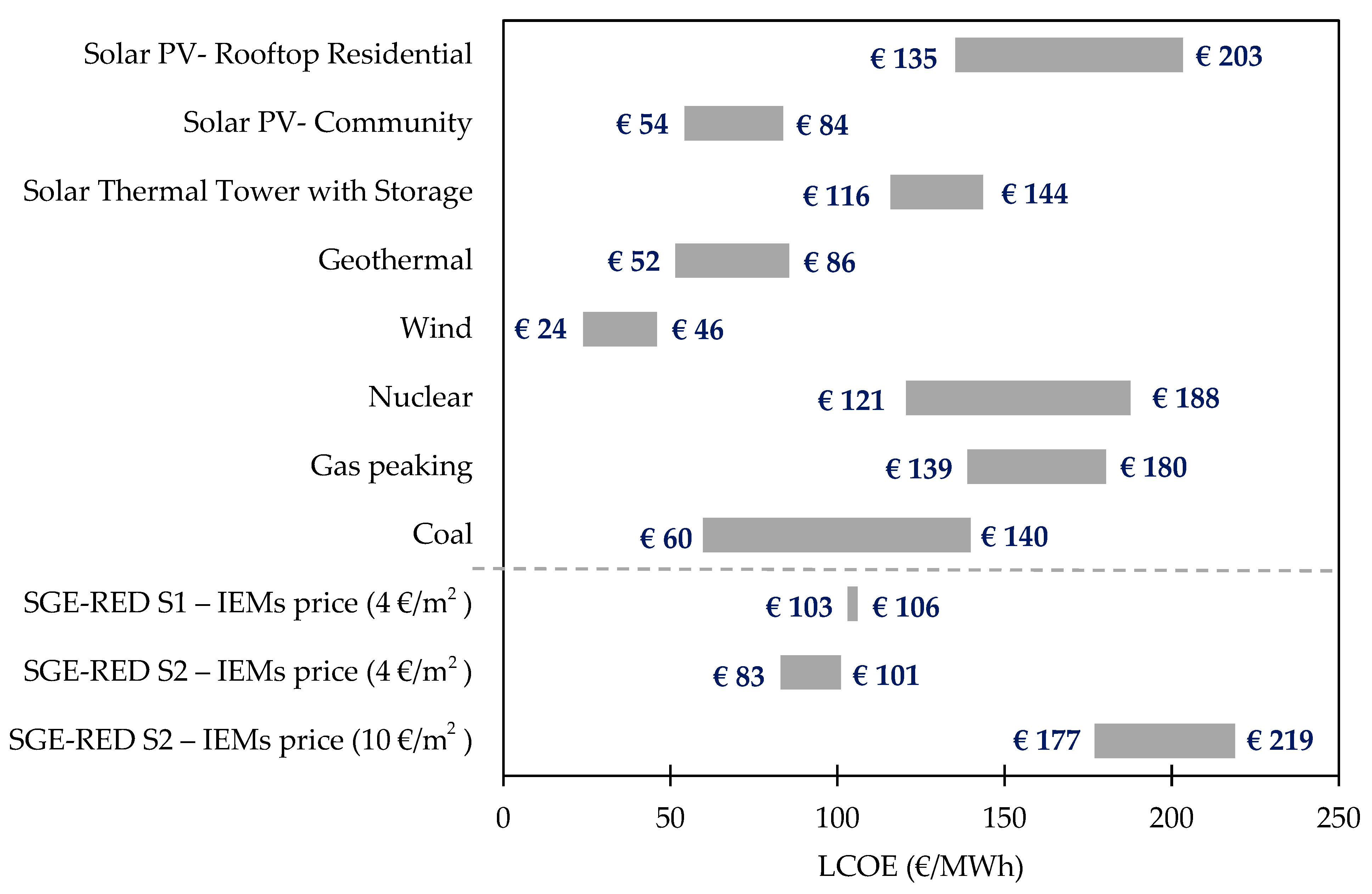
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Nomenclature | Description | Units |
| Am,r | Total IEM area stack | m2 |
| b | Width | m |
| CAPEX | Capital expenses | EUR/year |
| cm | IEM price | EUR/m2 |
| cp | Carbon price | EUR/tCO2e |
| CRF | Capital recovery factor | - |
| ef | Emission factor | kgCO2 eq/kWh |
| ep | Electricity price | EUR/kWh |
| L | Length | m |
| LCOE | Levelized Cost of Energy | EUR/MWh |
| LF | Load factor | % |
| LT | Plant lifetime | years |
| LTm | IEM lifetime | years |
| Ncp | Cell pairs | - |
| NPr | Net power per RED unit | kW |
| NPV | Net present value | EUR |
| OPEX | Operational expenses | EUR/year |
| QLC | LC flow rate | m3/h |
| r | Interest rate | % |
| TAC | Total annual cost | EUR/year |
| TNP | Total net power | kW |
| TNSE | Total Net Specific Energy | kWh/m3 |
| δsp | Spacer thickness | µm |
Abbreviations
| Abbreviations | Description |
| ES | Spain |
| GDP | Generalized disjunctive program |
| GR | Greece |
| HC | High-concentrated stream |
| IEM | Ion exchange membrane |
| LC | Low-concentrated stream |
| p.e. | Population equivalent |
| RED | Reverse electrodialysis |
| RU | RED units |
| S1 | Site 1, Comillas |
| S2 | Site 2, Ierapetra |
| SGE | Salinity gradient energy |
| TRL | Technology Readiness Level |
| UWWTP | Urban wastewater treatment plant |
| WWTP | Wastewater treatment plant |
References
- Tollefson, J. What the War in Ukraine Means for Energy, Climate and Food. Nature 2022, 604, 232–233. [Google Scholar] [CrossRef] [PubMed]
- International Renewable Energy Agency. World Energy Transitions Outlook 2022: 1.5 °C Pathway—Executive Summary; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2022; ISBN 9789292604295. [Google Scholar]
- COP26. COP26 Goals. Available online: https://ukcop26.org/ (accessed on 3 February 2022).
- International Energy Agency. Renewables 2021—Analysis and Forecast to 2026; IEA: Paris, France, 2021. [Google Scholar]
- Nyangchak, N. Emerging Green Industry toward Net-Zero Economy: A Systematic Review. J. Clean. Prod. 2022, 378, 134622. [Google Scholar] [CrossRef]
- Abascal, E.; Gómez-Coma, L.; Ortiz, I.; Ortiz, A. Global Diagnosis of Nitrate Pollution in Groundwater and Review of Removal Technologies. Sci. Total Environ. 2022, 810, 152233. [Google Scholar] [CrossRef] [PubMed]
- Karimidastenaei, Z.; Avellán, T.; Sadegh, M.; Kløve, B.; Haghighi, A.T. Unconventional Water Resources: Global Opportunities and Challenges. Sci. Total Environ. 2022, 827, 154429. [Google Scholar] [CrossRef]
- Fayiah, M.; Dong, S.; Singh, S.; Kwaku, E.A. A Review of Water–Energy Nexus Trend, Methods, Challenges and Future Prospects. Int. J. Energy Water Resour. 2020, 4, 91–107. [Google Scholar] [CrossRef]
- Micale, G.; Cipollina, A.; Tamburini, A. Salinity Gradient Energy. In Sustainable Energy from Salinity Gradients; Elsevier Inc.: Amsterdam, The Netherlands, 2016; pp. 1–17. ISBN 9780081003237. [Google Scholar]
- Yip, N.Y.; Brogioli, D.; Hamelers, H.V.M.; Nijmeijer, K. Salinity Gradients for Sustainable Energy: Primer, Progress, and Prospects. Environ. Sci. Technol. 2016, 50, 12072–12094. [Google Scholar] [CrossRef]
- Nijmeijer, K.; Metz, S. Chapter 5 Salinity Gradient Energy. Sustain. Sci. Eng. 2010, 2, 95–139. [Google Scholar]
- Alvarez-Silva, O.A.; Osorio, A.F.; Winter, C. Practical Global Salinity Gradient Energy Potential. Renew. Sustain. Energy Rev. 2016, 60, 1387–1395. [Google Scholar] [CrossRef]
- Rani, A.; Snyder, S.W.; Kim, H.; Lei, Z.; Pan, S.-Y. Pathways to a Net-Zero-Carbon Water Sector through Energy-Extracting Wastewater Technologies. NPJ Clean Water 2022, 5, 49. [Google Scholar] [CrossRef]
- Sampedro, T.; Tristán, C.; Gómez-Coma, L.; Rioyo, J.; Sainz, M.; Ortiz, I.; Ibañez, R. SWRO Concentrates for More Efficient Wastewater Reclamation. Desalination 2023, 545, 116156. [Google Scholar] [CrossRef]
- Gude, V.G. Desalination and Water Reuse to Address Global Water Scarcity. Rev. Environ. Sci. Biotechnol. 2017, 16, 591–609. [Google Scholar] [CrossRef]
- Food and Agriculture Organization of the United Nations AQUASTAT. Available online: https://tableau.apps.fao.org/views/ReviewDashboard-v1/special_groups_dashboard?%3Aembed=y&%3AisGuestRedirectFromVizportal=y (accessed on 25 October 2022).
- Plappally, A.K.; Lienhard, V.J.H. Energy Requirements for Water Production, Treatment, End Use, Reclamation, and Disposal. Renew. Sustain. Energy Rev. 2012, 16, 4818–4848. [Google Scholar] [CrossRef]
- Mei, Y.; Tang, C.Y. Recent Developments and Future Perspectives of Reverse Electrodialysis Technology: A Review. Desalination 2018, 425, 156–174. [Google Scholar] [CrossRef]
- Tian, H.; Wang, Y.; Pei, Y.; Crittenden, J.C. Unique Applications and Improvements of Reverse Electrodialysis: A Review and Outlook. Appl. Energy 2020, 262, 114482. [Google Scholar] [CrossRef]
- Güler, E.; Nijmeijer, K. Reverse Electrodialysis for Salinity Gradient Power Generation: Challenges and Future Perspectives. J. Membr. Sci. Res. 2018, 4, 108–110. [Google Scholar]
- Fontananova, E.; Messana, D.; Tufa, R.A.; Nicotera, I.; Kosma, V.; Curcio, E.; van Baak, W.; Drioli, E.; di Profio, G. Effect of Solution Concentration and Composition on the Electrochemical Properties of Ion Exchange Membranes for Energy Conversion. J. Power Sources 2017, 340, 282–293. [Google Scholar] [CrossRef]
- Mehdizadeh, S.; Yasukawa, M.; Abo, T.; Kuno, M.; Noguchi, Y.; Higa, M. The Effect of Feed Solution Temperature on the Power Output Performance of a Pilot-Scale Reverse Electrodialysis (RED) System with Different Intermediate Distance. Membranes 2019, 9, 73. [Google Scholar] [CrossRef]
- Chae, S.; Kim, H.; Gi, H.J.; Jang, J.; Higa, M.; Pishnamazi, M.; Choi, J.Y.; Chandula Walgama, R.; Bae, C.; Kim, I.S.; et al. Clean Power Generation from Salinity Gradient Using Reverse Electrodialysis Technologies: Recent Advances, Bottlenecks, and Future Direction. Chem. Eng. J. 2023, 452, 139482. [Google Scholar] [CrossRef]
- Abidin, M.N.Z.; Nasef, M.M.; Veerman, J. Towards the Development of New Generation of Ion Exchange Membranes for Reverse Electrodialysis: A Review. Desalination 2022, 537, 115854. [Google Scholar] [CrossRef]
- Gómez-Coma, L.; Ortiz-Martínez, V.M.; Fallanza, M.; Ortiz, A.; Ibañez, R.; Ortiz, I. Blue Energy for Sustainable Water Reclamation in WWTPs. J. Water Process Eng. 2020, 33, 101020. [Google Scholar] [CrossRef]
- Hossen, E.H.; Gobetz, Z.E.; Kingsbury, R.S.; Liu, F.; Palko, H.C.; Dubbs, L.L.; Coronell, O.; Call, D.F. Temporal Variation of Power Production via Reverse Electrodialysis Using Coastal North Carolina Waters and Its Correlation to Temperature and Conductivity. Desalination 2020, 491, 114562. [Google Scholar] [CrossRef]
- Ortiz-Martínez, V.M.; Gómez-Coma, L.; Tristán, C.; Pérez, G.; Fallanza, M.; Ortiz, A.; Ibañez, R.; Ortiz, I. A Comprehensive Study on the Effects of Operation Variables on Reverse Electrodialysis Performance. Desalination 2020, 482, 114389. [Google Scholar] [CrossRef]
- Pintossi, D.; Simões, C.; Saakes, M.; Borneman, Z.; Nijmeijer, K. Predicting Reverse Electrodialysis Performance in the Presence of Divalent Ions for Renewable Energy Generation. Energy Convers. Manag. 2021, 243, 114369. [Google Scholar] [CrossRef]
- la Cerva, M.L.; Liberto, M.D.; Gurreri, L.; Tamburini, A.; Cipollina, A.; Micale, G.; Ciofalo, M. Coupling CFD with a One-Dimensional Model to Predict the Performance of Reverse Electrodialysis Stacks. J. Memb. Sci. 2017, 541, 595–610. [Google Scholar] [CrossRef]
- Tristán, C.; Fallanza, M.; Ibáñez, R.; Ortiz, I.; Grossmann, I.E. A Generalized Disjunctive Programming Model for the Optimal Design of Reverse Electrodialysis Process for Salinity Gradient-Based Power Generation. Comput. Chem. Eng. 2023, 40, 108196. [Google Scholar] [CrossRef]
- Pontié, M.; Derauw, J.S.; Plantier, S.; Edouard, L.; Bailly, L. Seawater Desalination: Nanofiltration—A Substitute for Reverse Osmosis? Desalin. Water Treat. 2013, 51, 485–494. [Google Scholar] [CrossRef]
- Oren, Y.S.; Biesheuvel, P.M. Theory of Ion and Water Transport in Reverse Osmosis Membranes. Phys. Rev. Appl. 2017, 9, 024034. [Google Scholar] [CrossRef]
- Ortiz-Imedio, R.; Gomez-Coma, L.; Fallanza, M.; Ortiz, A.; Ibañez, R.; Ortiz, I. Comparative Performance of Salinity Gradient Power-Reverse Electrodialysis under Different Operating Conditions. Desalination 2019, 457, 8–21. [Google Scholar] [CrossRef]
- Tristán, C.; Fallanza, M.; Ibáñez, R.; Ortiz, I. Recovery of Salinity Gradient Energy in Desalination Plants by Reverse Electrodialysis. Desalination 2020, 496, 114699. [Google Scholar] [CrossRef]
- Tristán, C.; Fallanza, M.; Grossmann, I.; Ortiz, I.; Ibáñez, R. Generalized Disjunctive Programming Model for Optimization of Reverse Electrodialysis Process. In Proceedings of the IFAC-PapersOnLine; Elsevier: Amsterdam, The Netherlands, 2022; Volume 55, pp. 154–159. [Google Scholar]
- SBIR. STTR America’s Seed Fund Low-Cost Manufacturing of High-Performance Ion Exchange Membranes for Electrodialysis Using Initiated Chemical Vapor Deposition. Available online: https://beta.www.sbir.gov/awards/189321 (accessed on 15 February 2023).
- Campisi, G.; Cosenza, A.; Giacalone, F.; Randazzo, S.; Tamburini, A.; Micale, G. Desalination of Oilfield Produced Waters via Reverse Electrodialysis: A Techno-Economical Assessment. Desalination 2023, 548, 116289. [Google Scholar] [CrossRef]
- Daniilidis, A.; Herber, R.; Vermaas, D.A. Upscale Potential and Financial Feasibility of a Reverse Electrodialysis Power Plant. Appl. Energy 2014, 119, 257–265. [Google Scholar] [CrossRef]
- Eurostat Electricity Prices for Non-Household Consumers—Bi-Annual Data (from 2007 Onwards)(€/KWh) 1st Semester 2022—Band IB: 20 MWh < Consumption < 500 MWh; European Commission: Brussels, Belgium, 2022.
- Trading Economics EU Carbon Permits. Available online: https://tradingeconomics.com/commodity/carbon (accessed on 20 December 2022).
- Method IPCC 2013 Ecoinvent; Version 3.7.1; Market for Electricity, Medium Voltage; Ecoinvent: Zurich, Switzerland, 2020.
- IRENA. Renewable Power Generation Costs in 2021; IRENA: Masdar City, United Arab Emirates, 2021. [Google Scholar]
- Bazinet, L.; Geoffroy, T.R. Electrodialytic Processes: Market Overview, Membrane Phenomena, Recent Developments and Sustainable Strategies. Membranes 2020, 10, 221. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.; Yang, S.C.; Choi, J.; Kim, J.O.; Jeong, N. Optimization of the Number of Cell Pairs to Design Efficient Reverse Electrodialysis Stack. Desalination 2021, 497, 114676. [Google Scholar] [CrossRef]
- Ray; Douglas Lazard’s Levelized Cost of Energy Analysis; Version 15.0; Lazard: Hamilton, Bermuda, 2021.

| Scenario | S1. Comillas (Spain, ES) a | S2. Ierapetra (Greece, GR) b | ||
|---|---|---|---|---|
| Flow Rate (m3/day) | T (°C) | Flow Rate (m3/day) | T (°C) | |
| Winter season | 1161 | 14 | 1600 | 18 |
| Summer season | 832 | 20 | 1600 | 24 |
| Parameter | Value | Unit | Reference |
|---|---|---|---|
| Electricity price EU *, ep | 0.251 | EUR/kWh | [39] |
| Electricity price ES *, ep | 0.256 | EUR/kWh | [39] |
| Electricity price GR *, ep | 0.339 | EUR/kWh | [39] |
| Carbon price EU, cp | 78.16 | EUR/ton | [40] |
| Emission factor ES, ef | 0.374 | kgCO2 eq/kWh | [41] |
| Emission factor GR, ef | 0.813 | kgCO2 eq/kWh | [41] |
| Interest rate, r | 7.5 | % | [42] |
| Load factor, LF | 90 | % | [30] |
| IEM price, cm | 4–10 | EUR/m2 | [36,37] |
| IEM lifetime, LTm | 15 | years | - |
| Plant lifetime, LT | 30 | years | - |
| Parameter | Value | Unit |
|---|---|---|
| Total IEM area stack, Am,r | 2 × 0.175 | m2 |
| Cell pairs, Ncp | 1000 | - |
| Spacer thickness, δsp | 270 | µm |
| Width, b × length, L | 0.456 × 0.383 | m2 |
| Working Conditions | Optimal Results | ||||||
|---|---|---|---|---|---|---|---|
| Case | Scenario | Electricity Price (EUR/kWh) | IEM Price (EUR/m2) | RU | NPV (EUR) | TNP (kW) | |
| S1 | 1 | Winter Season | ES | 4 | 29 | 66,878 | 7.12 |
| 2 | 10 | 0 | ‒ | ‒ | |||
| 3 | Summer season | ES | 4 | 21 | 55,453 | 5.62 | |
| 4 | 10 | 0 | ‒ | ‒ | |||
| S2 | 5 | Winter Season | GR | 4 | 58 | 320,786 | 14.45 |
| 6 | 10 | 30 | 116,501 | 10.43 | |||
| 7 | EU | 4 | 53 | 204,720 | 13.95 | ||
| 8 | 10 | 22 | 40,723 | 8.50 | |||
| 9 | Summer season | GR | 4 | 58 | 361,535 | 15.57 | |
| 10 | 10 | 32 | 156,839 | 11.96 | |||
| 11 | EU | 4 | 54 | 237,634 | 15.20 | ||
| 12 | 10 | 24 | 69,302 | 10.03 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sampedro, T.; Tristán, C.; Gómez-Coma, L.; Fallanza, M.; Ortiz, I.; Ibañez, R. Design of a Reverse Electrodialysis Plant for Salinity Gradient Energy Extraction in a Coastal Wastewater Treatment Plant. Membranes 2023, 13, 546. https://doi.org/10.3390/membranes13060546
Sampedro T, Tristán C, Gómez-Coma L, Fallanza M, Ortiz I, Ibañez R. Design of a Reverse Electrodialysis Plant for Salinity Gradient Energy Extraction in a Coastal Wastewater Treatment Plant. Membranes. 2023; 13(6):546. https://doi.org/10.3390/membranes13060546
Chicago/Turabian StyleSampedro, Tamara, Carolina Tristán, Lucía Gómez-Coma, Marcos Fallanza, Inmaculada Ortiz, and Raquel Ibañez. 2023. "Design of a Reverse Electrodialysis Plant for Salinity Gradient Energy Extraction in a Coastal Wastewater Treatment Plant" Membranes 13, no. 6: 546. https://doi.org/10.3390/membranes13060546
APA StyleSampedro, T., Tristán, C., Gómez-Coma, L., Fallanza, M., Ortiz, I., & Ibañez, R. (2023). Design of a Reverse Electrodialysis Plant for Salinity Gradient Energy Extraction in a Coastal Wastewater Treatment Plant. Membranes, 13(6), 546. https://doi.org/10.3390/membranes13060546










