Understanding the Residence Time Distribution in a Transient Inline Spiking System: Modeling, Experiments, and Simulations
Abstract
1. Introduction
2. Experimental
2.1. Transient Inline Spiking System
2.2. Concentration Easurement
3. Modeling and Simulation
3.1. Modeling
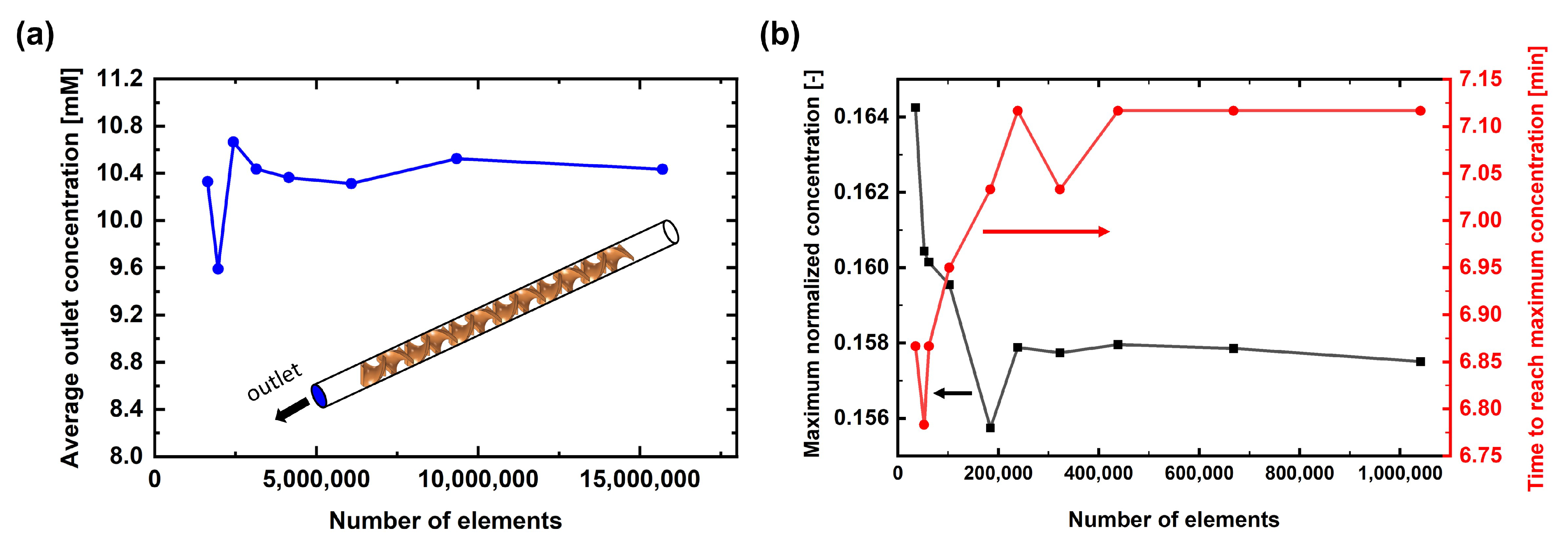
3.2. CFD Simulation
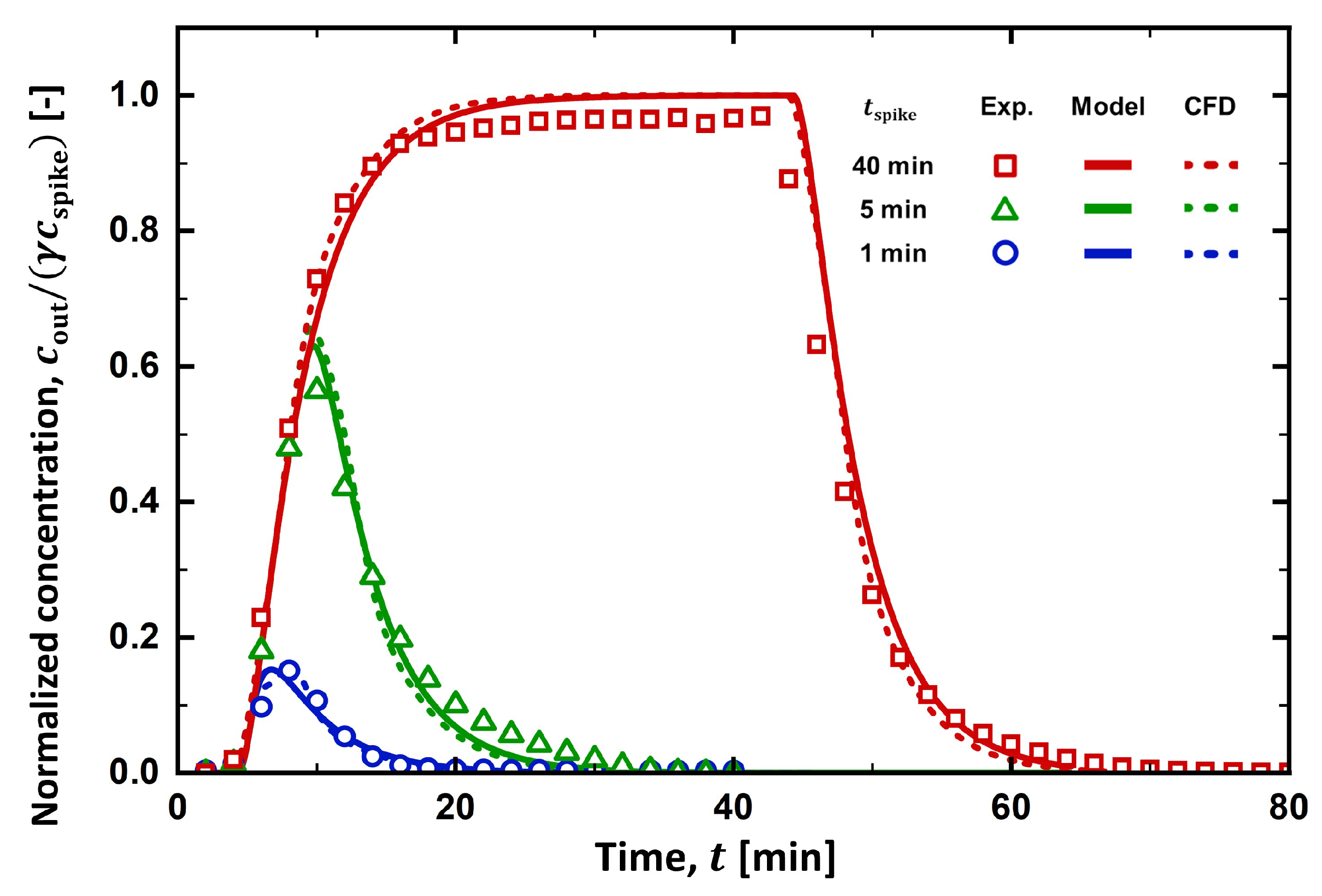
4. Results and Discussion
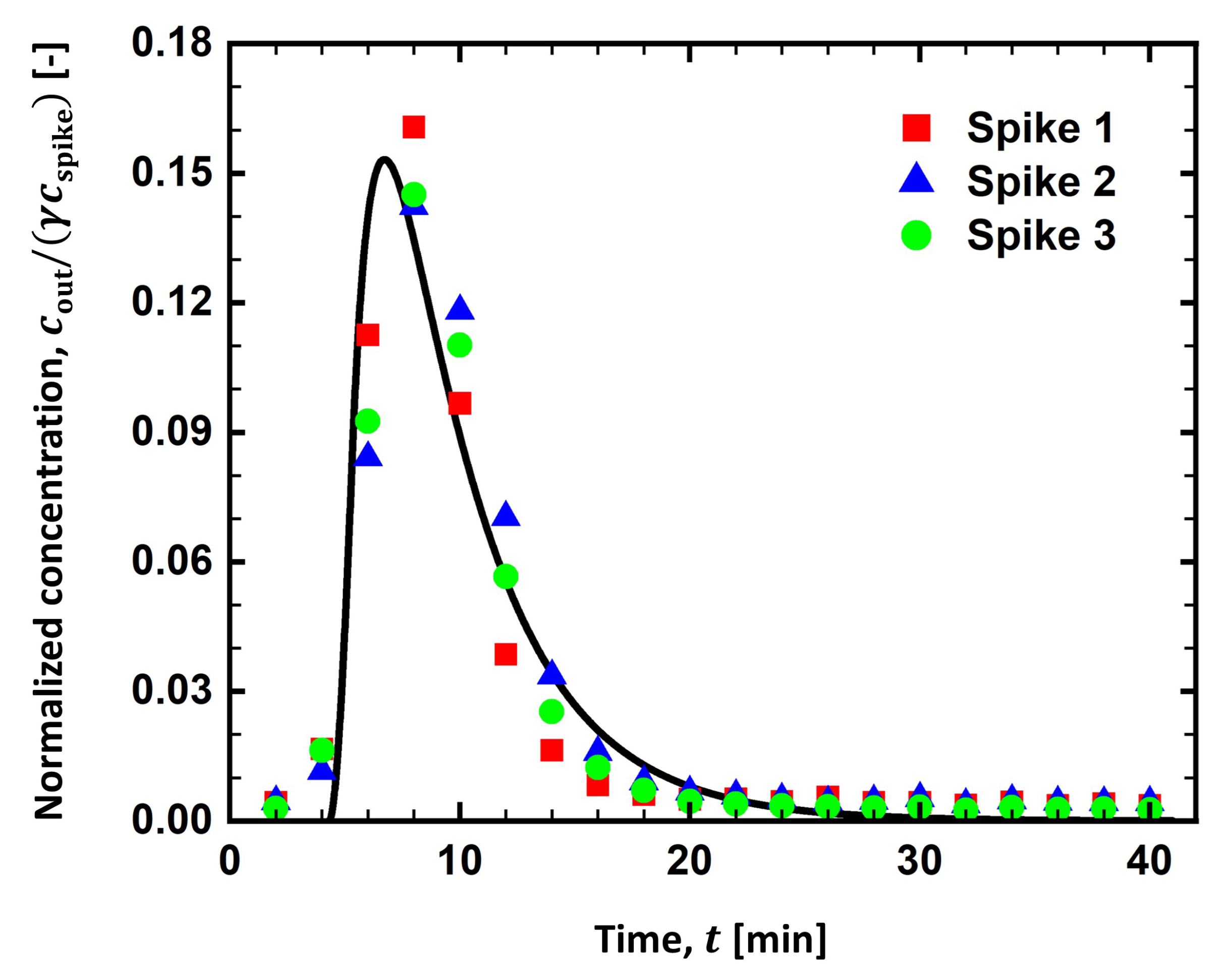
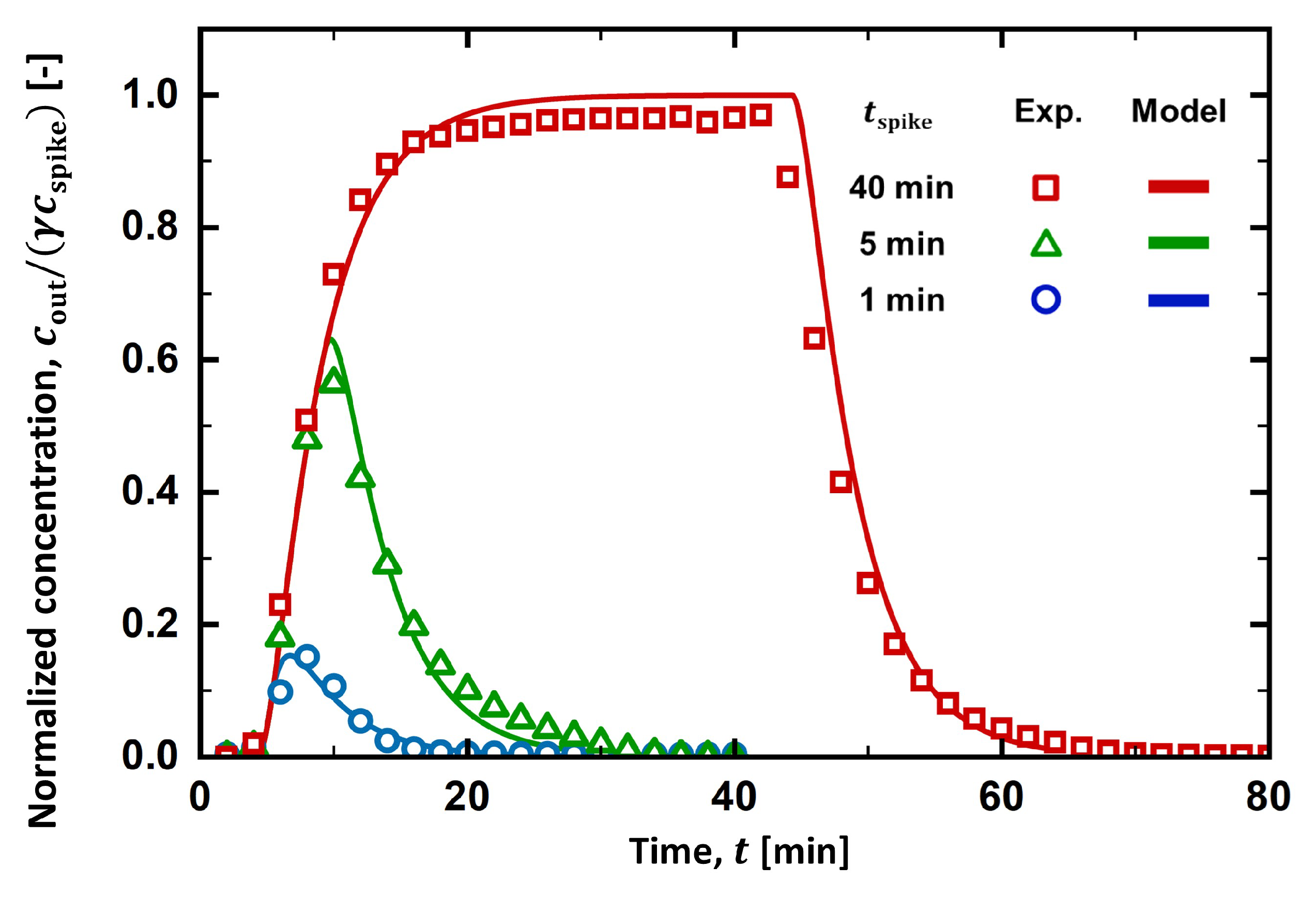
4.1. RTD Curve
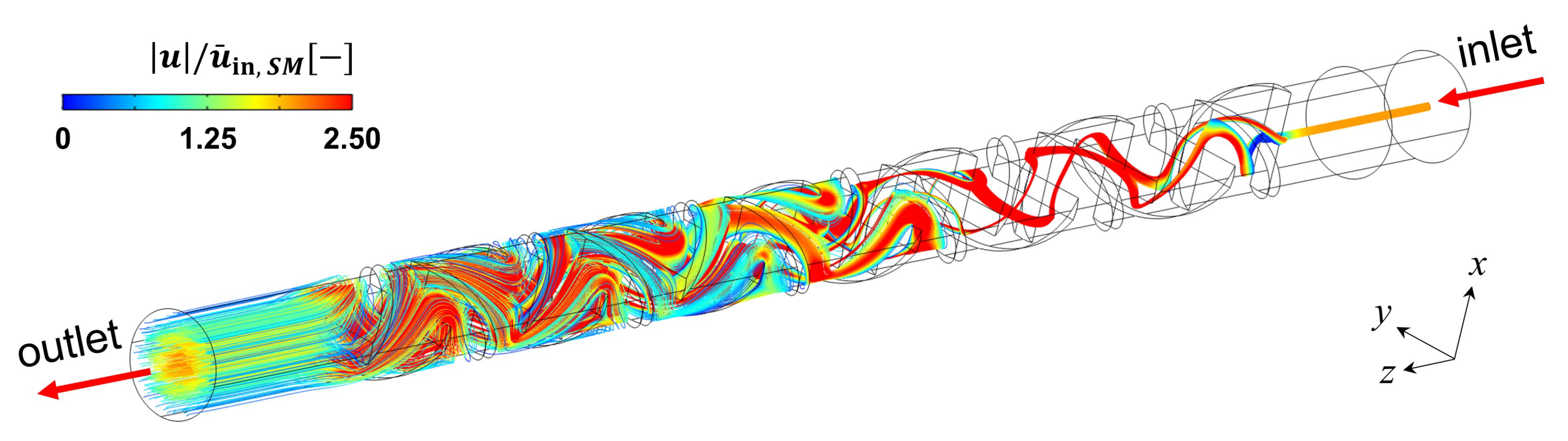
4.2. Static Mixer
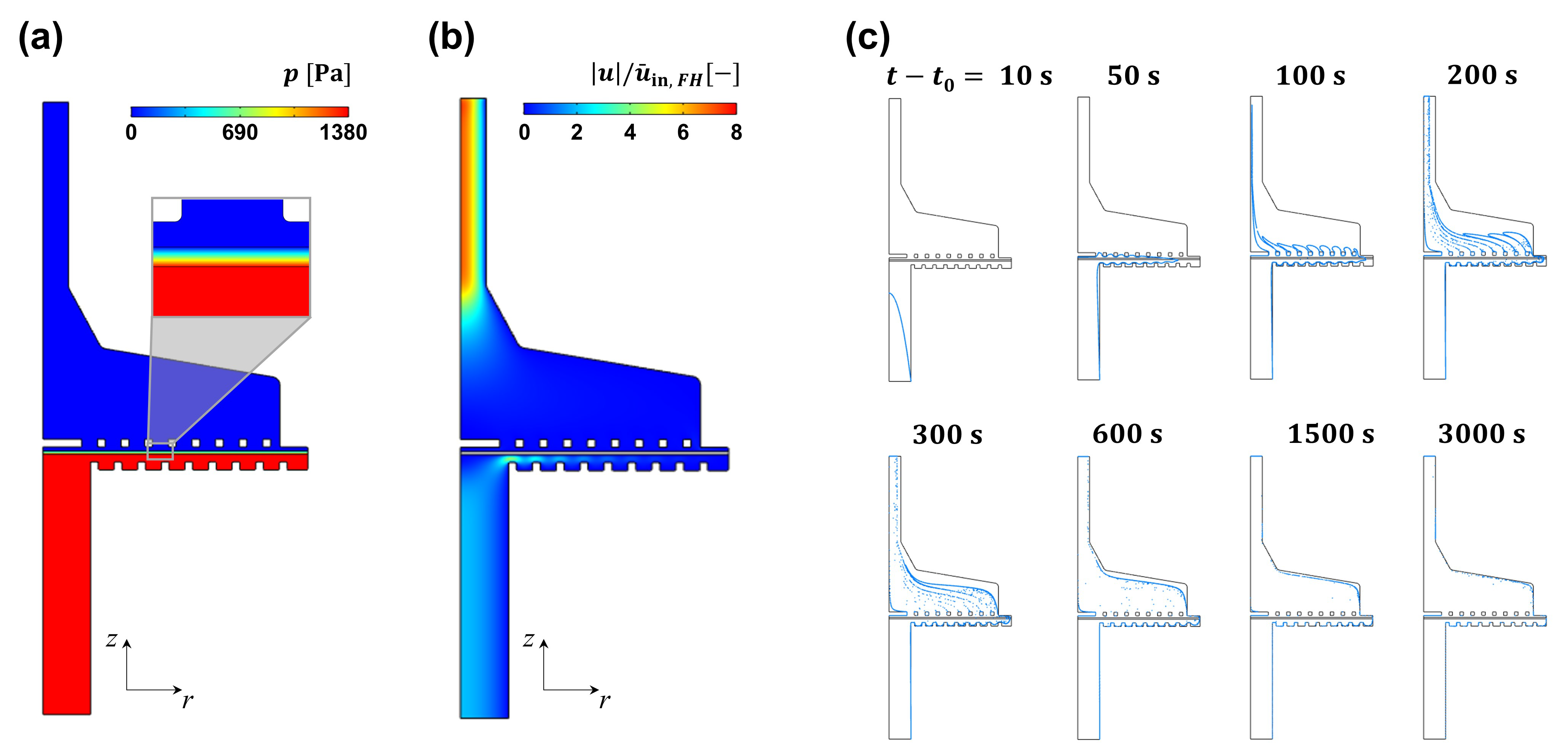
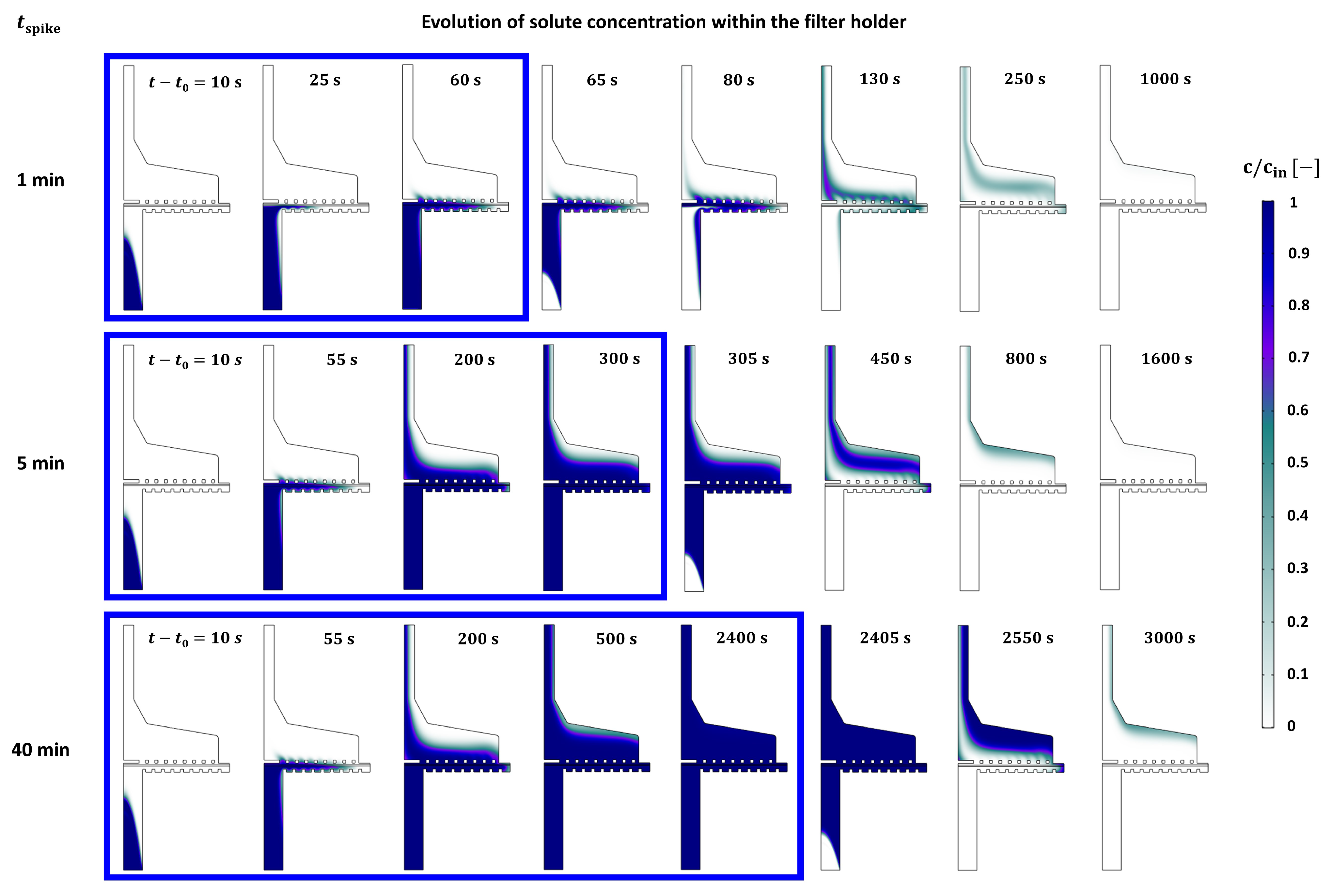
4.3. Filter Holder
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Gerstweiler, L.; Bi, J.; Middelberg, A.P.J. Continuous downstream bioprocessing for intensified manufacture of biopharmaceuticals and antibodies. Chem. Eng. Sci. 2021, 231, 116272. [Google Scholar] [CrossRef]
- Coffman, J.; Brower, M.; Connell-Crowley, L.; Deldari, S.; Farid, S.S.; Horowski, B.; Patil, U.; Pollard, D.; Qadan, M.; Rose, S.; et al. A common framework for integrated and continuous biomanufacturing. Biotechnol. Bioeng. 2021, 118, 1735–1749. [Google Scholar] [CrossRef] [PubMed]
- Khanal, O.; Lenhoff, A.M. Developments and opportunities in continuous biopharmaceutical manufacturing. mAbs 2021, 13, 1903664. [Google Scholar] [CrossRef] [PubMed]
- Zydney, A.L. Continuous downstream processing for high value biological products: A Review. Biotechnol. Bioeng. 2016, 113, 465–475. [Google Scholar] [CrossRef] [PubMed]
- Renate, K.; Reinhart, D. Advances in recombinant antibody manufacturing. Appl. Microbiol. Biotechnol. 2016, 100, 3451–3461. [Google Scholar] [CrossRef]
- Bohonak, D.M.; Mehta, U.; Weiss, E.R.; Voyta, G. Adapting virus filtration to enable intensified and continuous monoclonal antibody processing. Biotechnol. Prog. 2021, 37, e3088. [Google Scholar] [CrossRef]
- Johnson, S.A.; Chen, S.; Bolton, G.; Chen, Q.; Lute, S.; Fisher, J.; Brorson, K. Virus filtration: A review of current and future practices in bioprocessing. Biotechnol. Bioeng. 2022, 119, 743–761. [Google Scholar] [CrossRef]
- Lutz, H.; Chang, W.; Blandl, T.; Ramsey, G.; Parella, J.; Fisher, J.; Gefroh, E. Qualification of a novel inline spiking method for virus filter validation. Biotechnol. Prog. 2011, 27, 121–128. [Google Scholar] [CrossRef]
- Malakian, A.; Jung, S.Y.; Afzal, M.A.; Carbrello, C.; Giglia, S.; Johnson, M.; Miller, C.; Rayfield, W.; Boenitz, D.; Cetlin, D.; et al. Development of a transient inline spiking system for evaluating virus clearance in continuous bioprocessing—Proof of concept for virus filtration. Biotechnol. Bioeng. 2022, 119, 2134–2141. [Google Scholar] [CrossRef]
- David, L.; Waldschmidt, L.M.; Lobedann, M.; Schembecker, G. Simulation of pH level distribution inside a coiled flow inverter for continuous low pH viral inactivation. Biotechnol. Bioeng. 2020, 117, 429–437. [Google Scholar] [CrossRef]
- David, L.; Bayer, M.P.; Lobedann, M.; Schembecker, G. Simulation of continuous low pH viral inactivation inside a coiled flow inverter. Biotechnol. Bioeng. 2020, 117, 1048–1062. [Google Scholar] [CrossRef]
- Ghosh, P.; Vahedipour, K.; Lin, M.; Vogel, J.H.; Haynes, C.; von Lieres, E. Computational fluid dynamic simulation of axial and radial flow membrane chromatography: Mechanisms of non-ideality and validation of the zonal rate model. J. Chromatogr. A 2013, 1305, 114–122. [Google Scholar] [CrossRef]
- Ghosh, P.; Vahedipour, K.; Leuthold, M.; von Lieres, E. Model-based analysis and quantitative prediction of membrane chromatography: Extreme scale-up from 0.08 mL to 1200 mL. J. Chromatogr. A 2014, 1332, 8–13. [Google Scholar] [CrossRef]
- Jung, S.Y.; Nejatishahidein, N.; Kim, M.; Espah Borujeni, E.; Fernandez-Cerezo, L.; Roush, D.J.; Borhan, A.; Zydney, A.L. Quantitative interpretation of protein breakthrough curves in small-scale depth filter modules for bioprocessing. J. Membr. Sci. 2021, 627, 119217. [Google Scholar] [CrossRef]
- Out, D.J.P.; Los, J.M. Viscosity of aqueous solutions of univalent electrolytes from 5 to 95 °C. J. Solut. Chem. 1980, 9, 19–35. [Google Scholar] [CrossRef]
- Simion, A.I.; Grigoras, C.; Rosu, A.M.; Gavrilă, L. Mathematical modelling of density and viscosity of NaCl aqueous solutions. J. Agroaliment. Process. Technol. 2015, 21, 41–52. [Google Scholar]
- Kang, T.G.; Singh, M.K.; Anderson, P.D.; Meijer, H.E.H. A chaotic serpentine mixer efficient in the creeping flow regime: From design concept to optimization. Microfluid. Nanofluidics 2009, 7, 783. [Google Scholar] [CrossRef]
- Meijer, H.; Singh, M.; Anderson, P. On the performance of static mixers: A quantitative comparison. Prog. Polym. Sci. 2012, 37, 1333–1349. [Google Scholar] [CrossRef]
- Jung, S.Y.; Ahn, K.H.; Kang, T.G.; Park, G.T.; Kim, S.U. Chaotic mixing in a barrier-embedded partitioned pipe mixer. AIChE J. 2018, 64, 717–729. [Google Scholar] [CrossRef]
- Jung, S.Y.; Park, J.E.; Kang, T.G.; Park, J.D. Flow and mixing analysis of a thixotropic fluid in a barrier-embedded partitioned pipe mixer (BPPM): A numerical study. Int. J. Heat Mass Transf. 2022, 184, 122310. [Google Scholar] [CrossRef]
- Haynes, W. CRC Handbook of Chemistry and Physics; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Ottino, J.; Crighton, D. The Kinematics of Mixing: Stretching, Chaos, and Transport; Cambridge Texts in Applied Mathematics; Cambridge University Press: Cambridge, UK, 1989. [Google Scholar]
- Ghahfarokhi, N.J.; Bayareh, M. Numerical study of a novel spiral-type micromixer for low Reynolds number regime. Korea Aust. Rheol. 2021, 33, 333–342. [Google Scholar] [CrossRef]
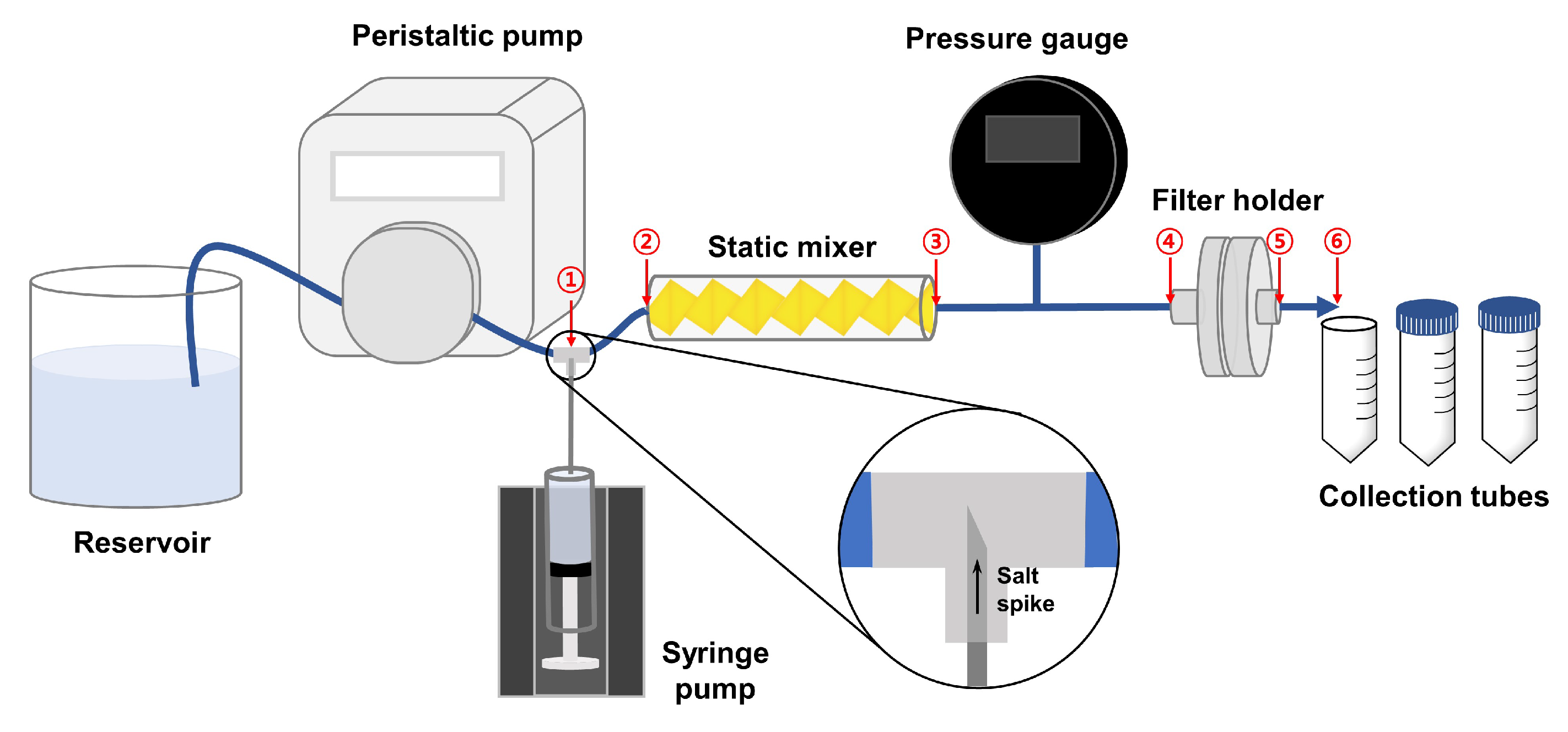
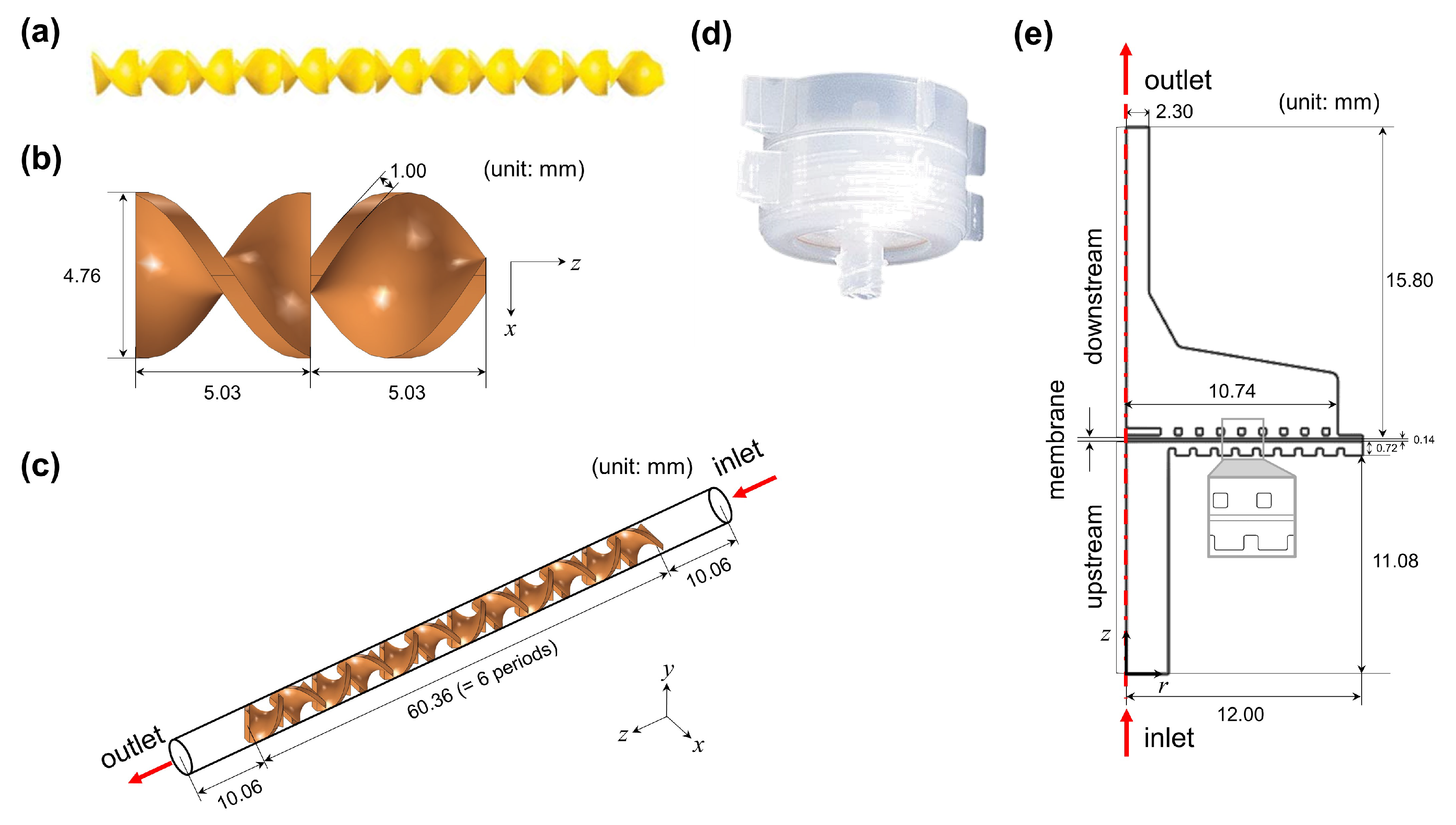

| No. | Interval | Holdup Time [min] | Description |
|---|---|---|---|
| 1 | ①–② | 0.98 ± 0.02 | Tubing (spiking station → static mixer) |
| 2 | ②–③ | 2.18 ± 0.29 | Static mixer |
| 3 | ③–④ | 0.98 ± 0.07 | Tubing (static mixer → filter holder) |
| 4 | ④–⑤ | 1.05 ± 0.57 | Filter holder |
| 5 | ⑤–⑥ | 0.34 ± 0.01 | Tubing (filter holder → collection tube) |
| Static Mixer (SM) Domain | |||
|---|---|---|---|
| Symbol | Value | Unit | Description |
| m/s | Average inlet velocity into the static mixer | ||
| 4.76 | mm | Diameter of the static mixer | |
| 0.337 | mm | Diameter of the syringe needle | |
| 10.06 | mm | Length of the periodic unit of the static mixer | |
| 1.00 | mm | Thickness of the static mixer | |
| Filter Holder (FH) domain | |||
| Symbol | Value | Unit | Description |
| m/s | Average inlet velocity into the filter holder | ||
| 4.3 | mm | Diameter of the inlet of the filter holder | |
| 2.3 | mm | Diameter of the outlet of the filter holder | |
| 24 | mm | Diameter of the membrane | |
| 1380 | Pa | Transmembrane pressure (TMP) | |
| 140 | μm | Membrane thickness | |
| m2 | Membrane permeability | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, M.; Wang, J.; Jung, S.Y. Understanding the Residence Time Distribution in a Transient Inline Spiking System: Modeling, Experiments, and Simulations. Membranes 2023, 13, 375. https://doi.org/10.3390/membranes13040375
Hwang M, Wang J, Jung SY. Understanding the Residence Time Distribution in a Transient Inline Spiking System: Modeling, Experiments, and Simulations. Membranes. 2023; 13(4):375. https://doi.org/10.3390/membranes13040375
Chicago/Turabian StyleHwang, Minsun, Junsuk Wang, and Seon Yeop Jung. 2023. "Understanding the Residence Time Distribution in a Transient Inline Spiking System: Modeling, Experiments, and Simulations" Membranes 13, no. 4: 375. https://doi.org/10.3390/membranes13040375
APA StyleHwang, M., Wang, J., & Jung, S. Y. (2023). Understanding the Residence Time Distribution in a Transient Inline Spiking System: Modeling, Experiments, and Simulations. Membranes, 13(4), 375. https://doi.org/10.3390/membranes13040375






