The Effect of the Rotating Disk Geometry on the Flow and Flux Enhancement in a Dynamic Filtration System
Abstract
1. Introduction
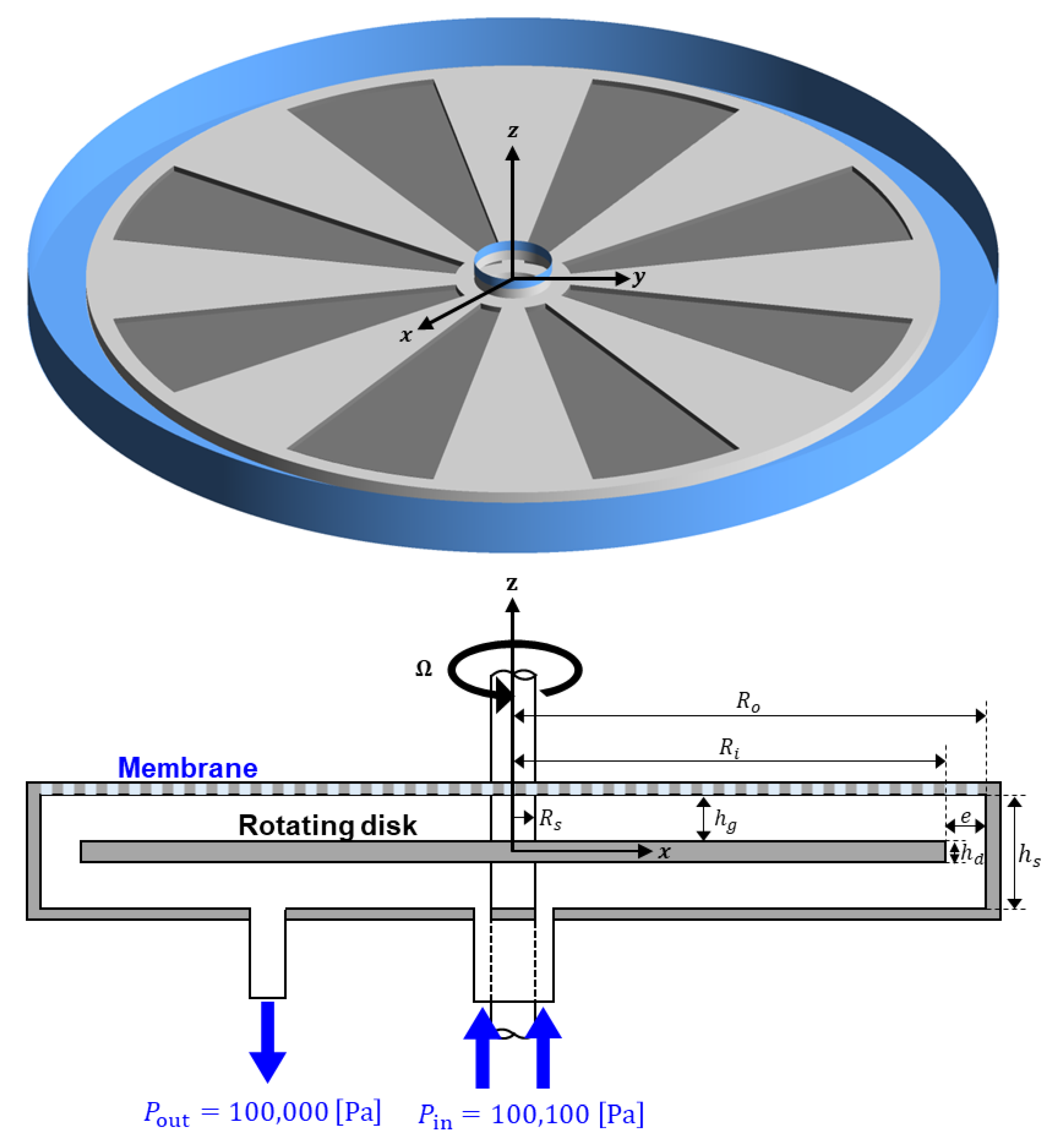
2. Problem Description
2.1. Dynamic Filtration Sytem and Operating Conditions
2.2. Modeling
2.3. Numerical Scheme
3. Results and Discussion
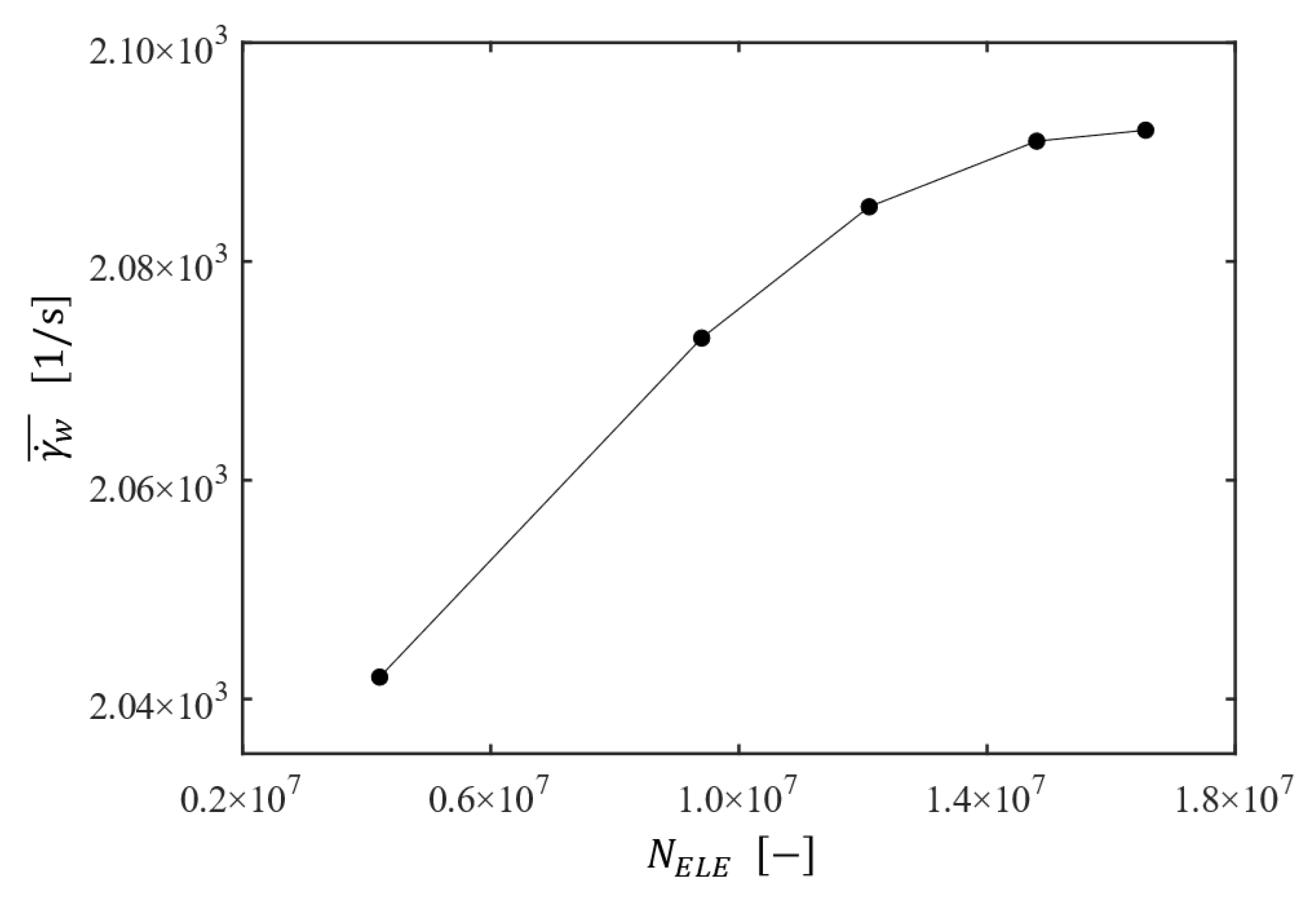
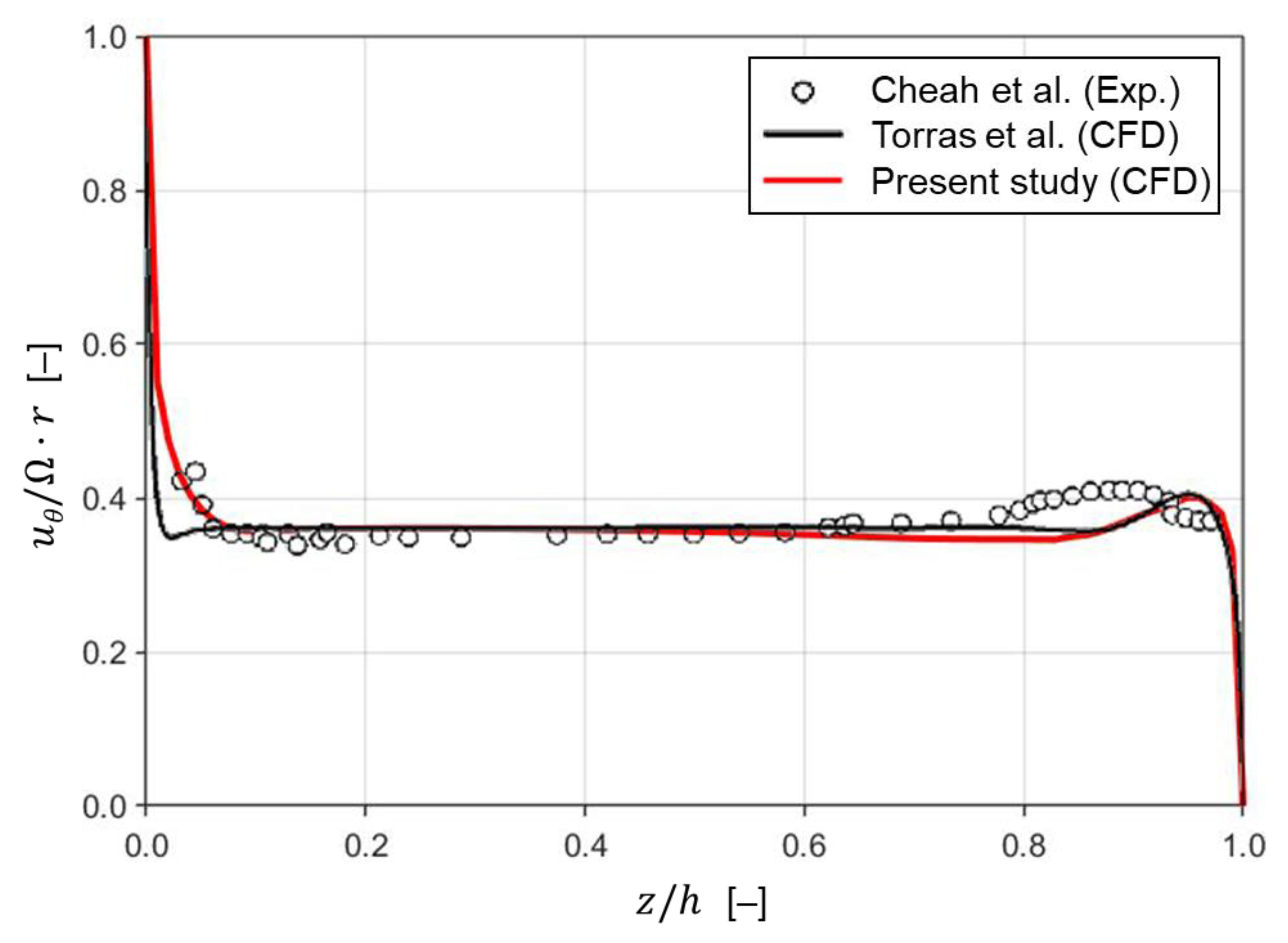
3.1. Mesh Convergence Test and Validation
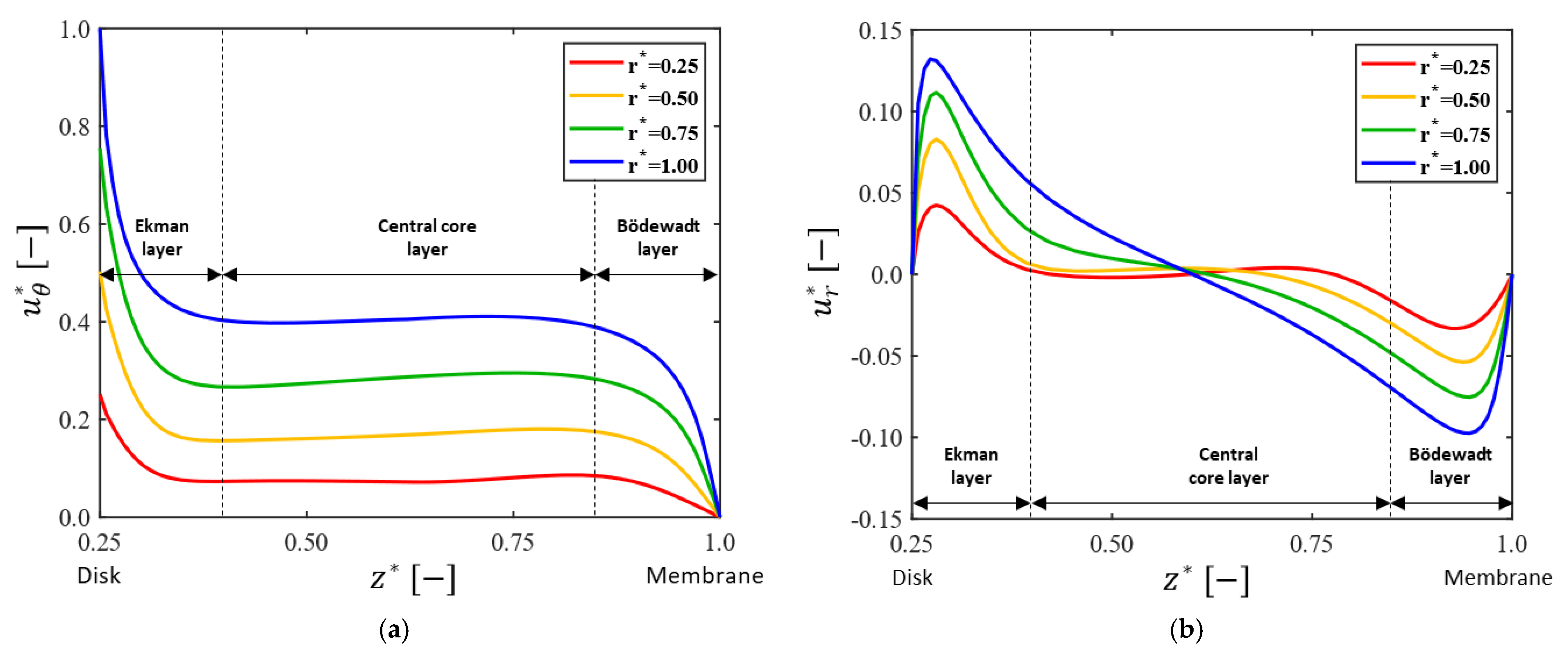
3.2. Flow Charactetistics
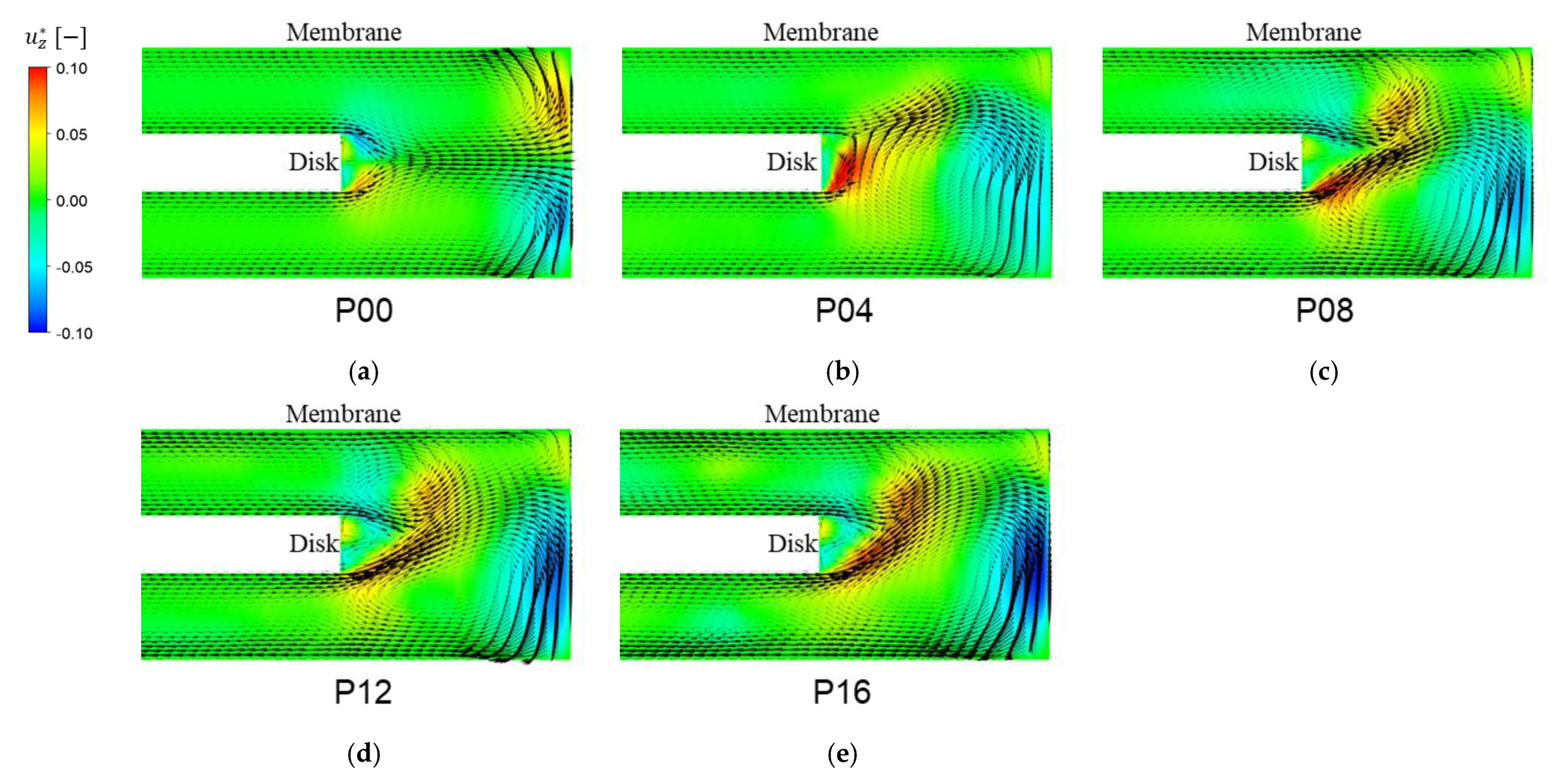
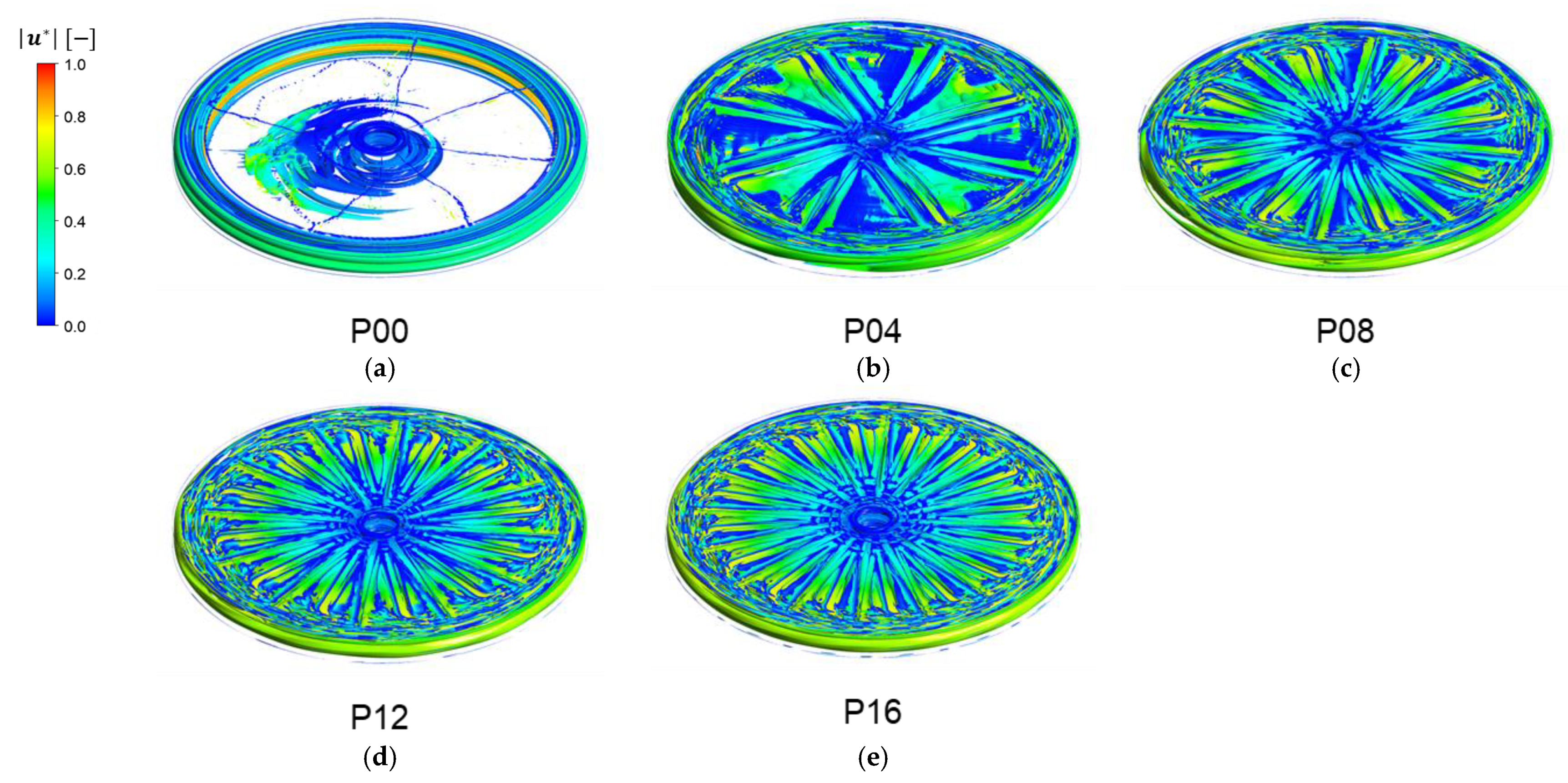
3.3. Vortex Structure
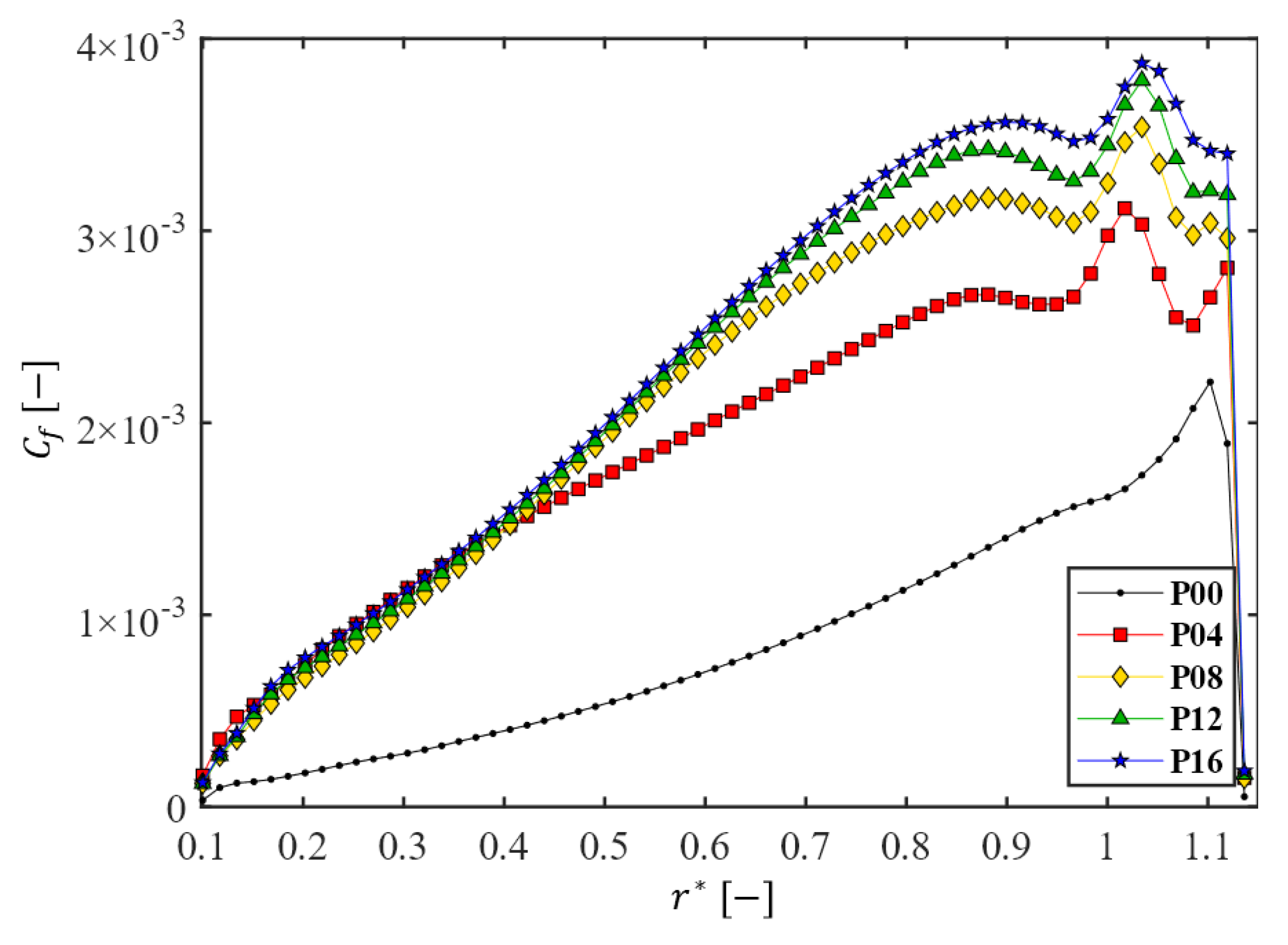
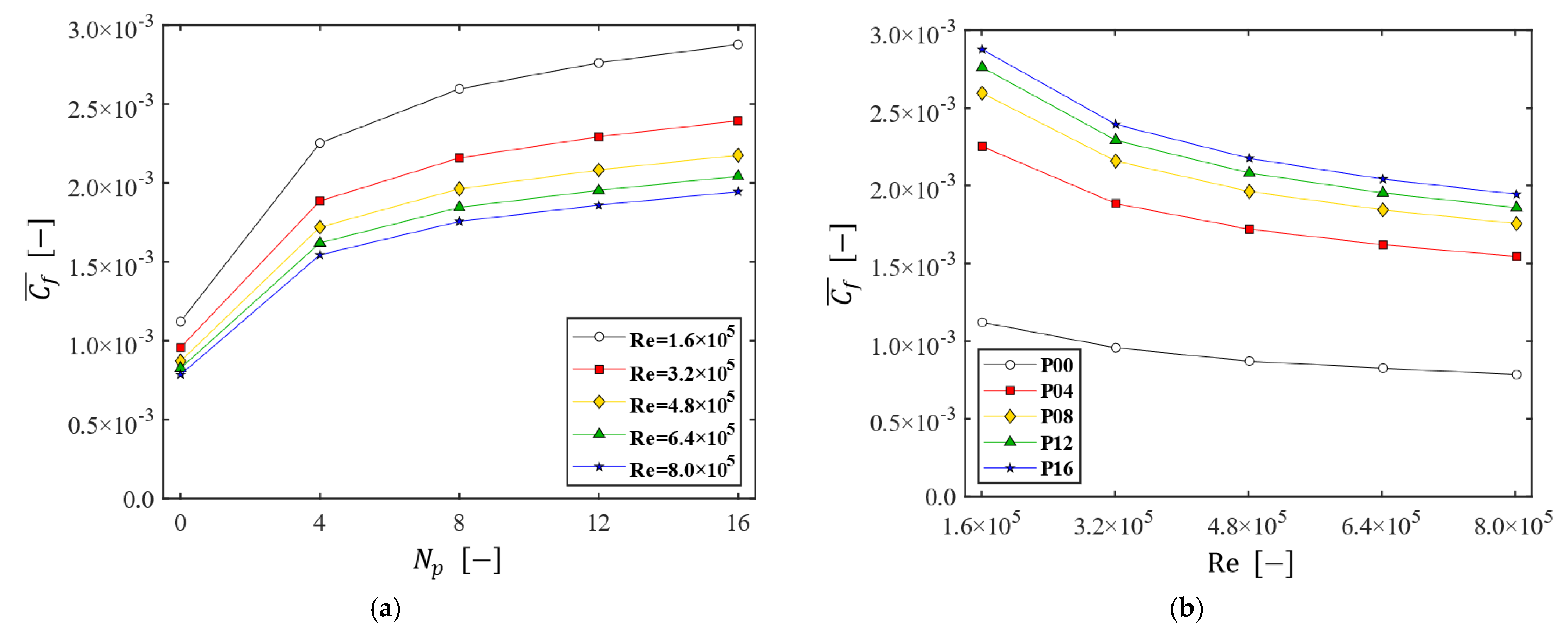
3.4. Shear Stress Distribution
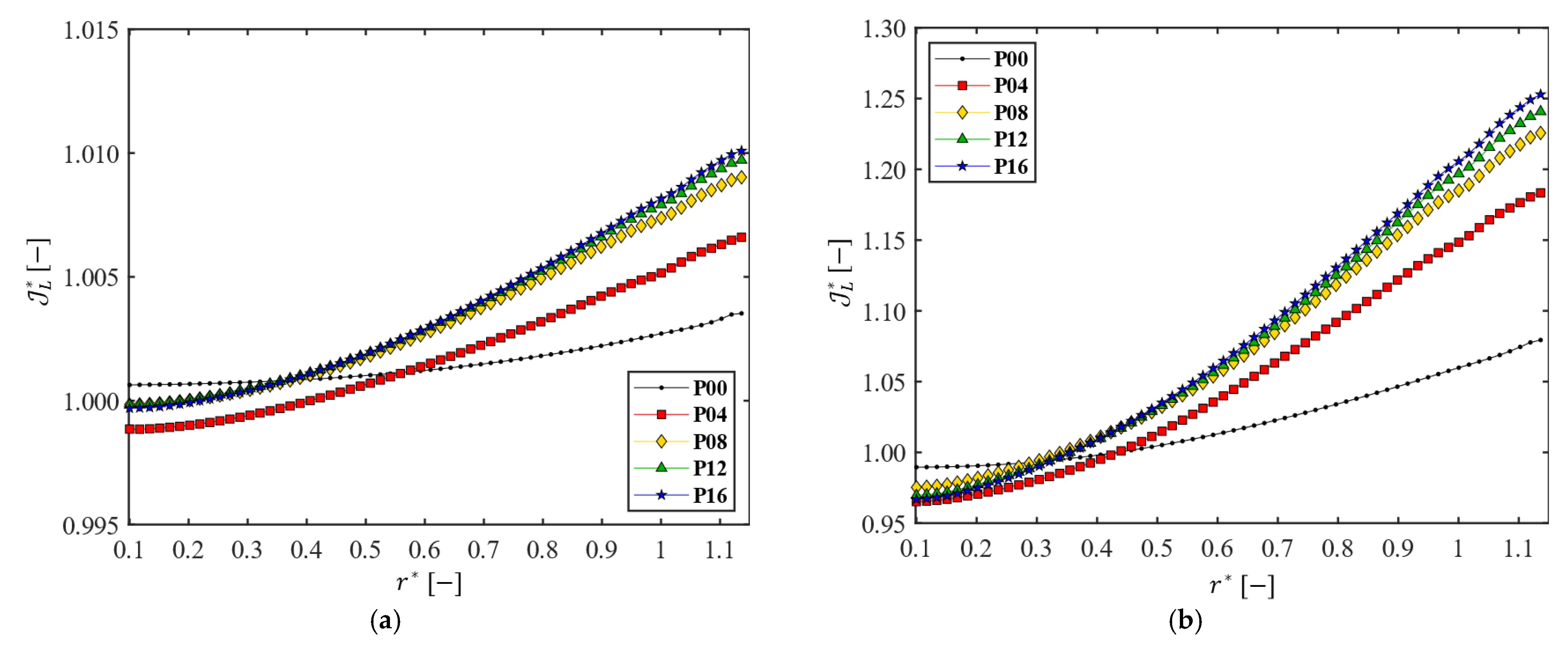
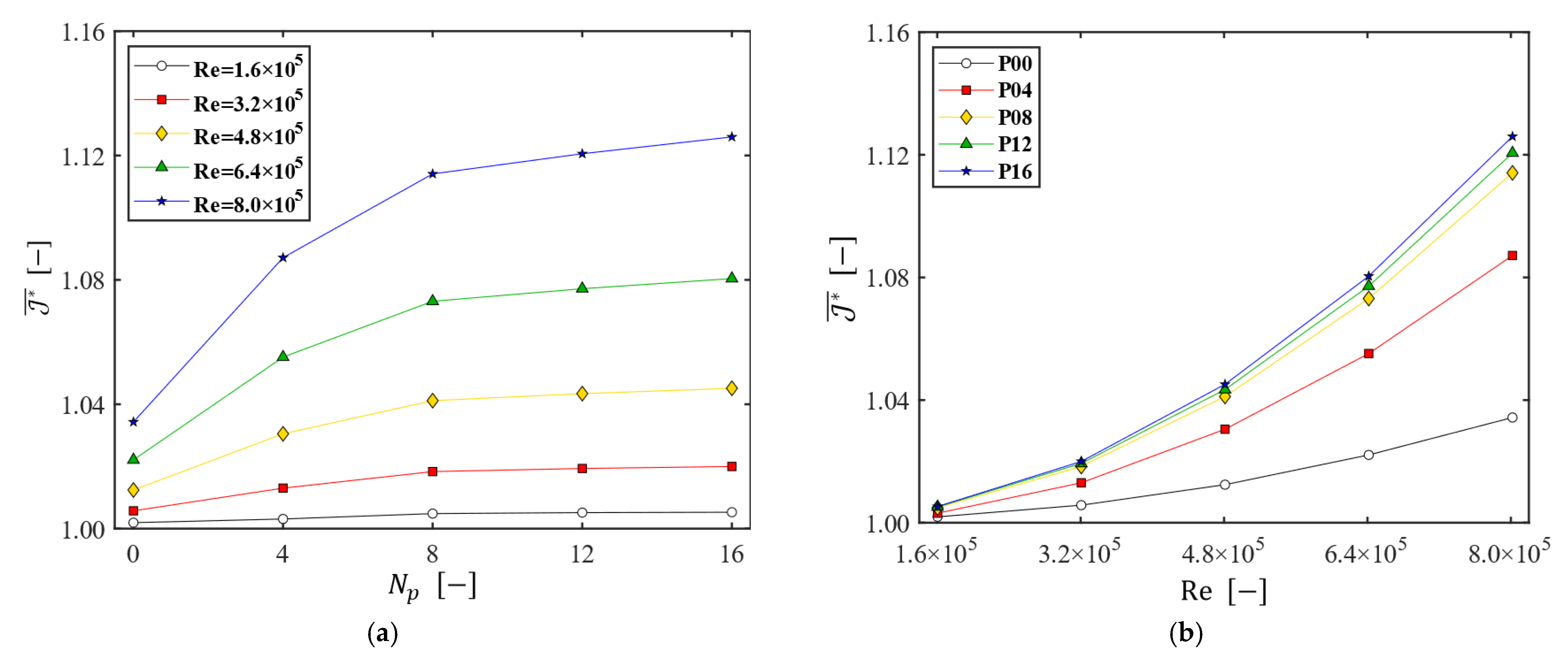
3.5. Filtration Performance
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| AMG | Algebraic multigrid |
| CFD | Computational fluid dynamics |
| CFF | Crossflow filtration |
| CP | Concentration polarization |
| DF | Aynamic filtration |
| MRF | Multiple reference frame |
| MSD | Multishift disk |
| RANS | Reynolds-averaged Navier-Stokes |
| RMS | Root mean square |
| SST | Shear stress transport |
| SWM | spiral-wound module |
| TMP | transmembrane pressure |
| List of Symbols | |
| Membrane area, m2 | |
| Pore radius of membrane, μm | |
| Friction coefficient | |
| Area-averaged friction coefficient | |
| Symmetric part of velocity gradient, 1/s | |
| Aspect ratio | |
| Gap from the x–axis to membrane, mm | |
| Axial gap, mm | |
| Permeate flux, m/s | |
| Reference permeate flux, m/s | |
| Dimensionless permeate flux | |
| Area-averaged dimensionless permeate flux | |
| Membrane permeability, m/s·Pa | |
| Turbulence kinetic energy, J/kg | |
| Membrane thickness, μm | |
| Number of elements | |
| Number of patterns on one side of a disk | |
| Outward unit normal vector | |
| Pressure, Pa | |
| Pressure at the inlet, Pa | |
| Pressure at the outlet, Pa | |
| Radius, mm | |
| Disk radius, mm | |
| Stator radius, mm | |
| Shaft radius, mm | |
| Re | Reynolds number |
| Radial distance from the z–axis, mm | |
| Dimensionless radius | |
| Time, s | |
| Reynolds stress tensor, Pa | |
| Linear velocity, m/s | |
| Velocity vector, m/s | |
| Relative velocity vector, m/s | |
| Circumferential velocity, m/s | |
| Radial velocity, m/s | |
| Axial velocity, m/s | |
| Dimensionless velocity vector | |
| Dimensionless circumferential velocity | |
| Dimensionless radial velocity | |
| Dimensionless axial velocity | |
| Antisymmetric part of velocity gradient, 1/s | |
| Dimensionless z–coordinate | |
| Greek Letters | |
| Inlet boundary | |
| Outlet boundary | |
| Membrane (permeable boundary) | |
| Disk surface | |
| Shaft surface | |
| Impermeable boundary | |
| Shear rate, 1/s | |
| Wall shear rate, 1/s | |
| Average wall shear rate, 1/s | |
| Difference | |
| Tortuosity | |
| Real eigenvalue | |
| Viscosity of fluid, Pa·s | |
| Density of fluid, kg/m3 | |
| Wall shear stress, Pa | |
| Dimensionless wall shear stress | |
| Porosity | |
| Angular velocity, 1/s | |
| Specific dissipation rate, 1/s |
References
- Cui, Z.; Jiang, Y.; Field, R. Fundamentals of Pressure-Driven Membrane Separation Processes. In Membrane Technology, 1st ed.; Cui, Z., Muralidhara, H., Eds.; Butterworth-Heinemann: Oxford, UK, 2010; Volume 1, pp. 1–18. [Google Scholar] [CrossRef]
- Sablani, S.; Goosen, M.; Al-Belushi, R.; Wilf, M. Concentration polarization in ultrafiltration and reverse osmosis: A critical review. Desalination 2001, 141, 269–289. [Google Scholar] [CrossRef]
- Zydney, A.L. Stagnant film model for concentration polarization in membrane systems. J. Membr. Sci. 1997, 130, 275–281. [Google Scholar] [CrossRef]
- Mariñas, B.J.; Urama, R.I. Modeling concentration-polarization in reverse osmosis spiral-wound elements. J. Environ. Eng. 1996, 122, 292–298. [Google Scholar] [CrossRef]
- Hoek, E.M.; Elimelech, M. Cake-enhanced concentration polarization: A new fouling mechanism for salt-rejecting membranes. Environ. Sci. Technol. 2003, 37, 5581–5588. [Google Scholar] [CrossRef] [PubMed]
- Porter, M.C. Concentration polarization with membrane ultrafiltration. Ind. Eng. Chem. Prod. Res. Dev. 1972, 11, 234–248. [Google Scholar] [CrossRef]
- She, Q.; Wang, R.; Fane, A.G.; Tang, C.Y. Membrane fouling in osmotically driven membrane processes: A review. J. Membr. Sci. 2016, 499, 201–233. [Google Scholar] [CrossRef]
- Tummons, E.; Han, Q.; Tanudjaja, H.J.; Hejase, C.A.; Chew, J.W.; Tarabara, V.V. Membrane fouling by emulsified oil: A review. Sep. Purif. Technol. 2020, 248, 116919. [Google Scholar] [CrossRef]
- AlSawaftah, N.; Abuwatfa, W.; Darwish, N.; Husseini, G.A. A Review on Membrane Biofouling: Prediction, Characterization, and Mitigation. Membranes 2022, 12, 1271. [Google Scholar] [CrossRef] [PubMed]
- Jung, S.Y.; Jung, H.I.; Kang, T.G.; Ahn, K.H. Fouling Mitigation in Crossflow Filtration Using Chaotic Advection: A Numerical Study. AIChE J. 2020, 66, e16792. [Google Scholar] [CrossRef]
- Baitalow, K.; Wypysek, D.; Leuthold, M.; Weisshaar, S.; Lölsberg, J.; Wessling, M. A Mini-Module with Built-in Spacers for High-Throughput Ultrafiltration. J. Memb. Sci. 2021, 637, 119602. [Google Scholar] [CrossRef]
- Chevarin, C.; Wang, X.; Bouyer, D.; Tarabara, V.; Chartier, T.; Ayral, A. CFD-guided patterning of tubular ceramic membrane surface by stereolithography: Optimizing morphology at the mesoscale for improved hydrodynamic control of membrane fouling. J. Membr. Sci. 2023, 672, 121435. [Google Scholar] [CrossRef]
- Lin, W.; Wang, Q.; Sun, L.; Wang, D.; Cabrera, J.; Li, D.; Hu, L.; Jiang, G.; Wang, X.M.; Huang, X. The Critical Role of Feed Spacer Channel Porosity in Membrane Biofouling: Insights and Implications. J. Memb. Sci. 2022, 649, 120395. [Google Scholar] [CrossRef]
- Lin, W.; Lei, J.; Wang, Q.; Wang, X.M.; Huang, X. Performance Enhancement of Spiral-Wound Reverse Osmosis Membrane Elements with Novel Diagonal-Flow Feed Channels. Desalination 2022, 523, 115447. [Google Scholar] [CrossRef]
- Lin, W.; Li, D.; Wang, Q.; Wang, X.M.; Huang, X. Dynamic Evolution of Membrane Biofouling in Feed Channels Affected by Spacer–Membrane Clearance and the Induced Hydrodynamic Conditions. J. Memb. Sci. 2023, 668, 121209. [Google Scholar] [CrossRef]
- Guan, H.; Lin, P.; Yu, S.; Hu, X.; Li, X.; Zhu, Z. Hydrodynamic effects of nonuniform feed spacer structures on energy loss and mass transfer in spiral wound module. J. Membr. Sci. 2023, 673, 121479. [Google Scholar] [CrossRef]
- Kroner, K.H.; Nissinen, V. Dynamic filtration of microbial suspensions using an axially rotating filter. J. Membr. Sci. 1988, 36, 85–100. [Google Scholar] [CrossRef]
- Holeschovsky, U.B.; Cooney, C.L. Quantitative description of ultrafiltration in a rotating filtration device. AIChE J. 1991, 37, 1219–1226. [Google Scholar] [CrossRef]
- Lee, S.S.; Burt, A.; Russotti, G.; Buckland, B. Microfiltration of recombinant yeast cells using a rotating disk dynamic filtration system. Biotechnol. Bioeng. 1995, 48, 386–400. [Google Scholar] [CrossRef] [PubMed]
- Ding, L.H.; Jaffrin, M.Y.; Mellal, M.; He, G. Investigation of performances of a multishaft disk (MSD) system with overlapping ceramic membranes in microfiltration of mineral suspensions. J. Membr. Sci. 2006, 276, 232–240. [Google Scholar] [CrossRef]
- He, G.; Ding, L.H.; Paullier, P.; Jaffrin, M.Y. Experimental study of a dynamic filtration system with overlapping ceramic membranes and non-permeating disks rotating at independent speeds. J. Membr. Sci. 2007, 300, 63–70. [Google Scholar] [CrossRef]
- Uppu, A.; Chaudhuri, A.; Das, S.P. Numerical modeling of particulate fouling and cake-enhanced concentration polarization in roto-dynamic reverse osmosis filtration systems. Desalination 2019, 468, 114053. [Google Scholar] [CrossRef]
- Uppu, A.; Chaudhuri, A.; Das, S.P.; Prakash, N. CFD modeling of gypsum scaling in cross-flow RO filters using moments of particle population balance. J. Environ. Chem. Eng. 2020, 8, 104151. [Google Scholar] [CrossRef]
- Prakash, N.; Chaudhuri, A.; Das, S.P. Numerical modelling and analysis of concentration polarization and scaling of gypsum over RO membrane during seawater desalination. Chem. Eng. Res. Des. 2023, 190, 497–507. [Google Scholar] [CrossRef]
- Jaffrin, M.Y. Dynamic shear-enhanced membrane filtration: A review of rotating disks, rotating membranes and vibrating systems. J. Membr. Sci. 2008, 324, 7–25. [Google Scholar] [CrossRef]
- Jaffrin, M.Y. Hydrodynamic techniques to enhance membrane filtration. Annu. Rev. Fluid Mech. 2012, 44, 77–96. [Google Scholar] [CrossRef]
- Cheng, M.; Xie, X.; Schmitz, P.; Fillaudeau, L. Extensive review about industrial and laboratory dynamic filtration modules: Scientific production, configurations and performances. Sep. Purif. Technol. 2021, 265, 118293. [Google Scholar] [CrossRef]
- Kim, K.; Jung, J.-Y.; Kwon, J.-H.; Yang, J.-W. Dynamic microfiltration with a perforated disk for effective harvesting of microalgae of microalgae. J. Membr. Sci. 2015, 475, 252–258. [Google Scholar] [CrossRef]
- Jogdand, A.; Chaudhuri, A. Modeling of concentration polarization and permeate flux variation in a roto-dynamic reverse osmosis filtration system. Desalination 2015, 375, 54–70. [Google Scholar] [CrossRef]
- Xie, X.; Le Men, C.; Dietrich, N.; Schmitz, P.; Fillaudeau, L. Local hydrodynamic investigation by PIV and CFD within a Dynamic filtration unit under laminar flow. Sep. Purif. Technol. 2018, 198, 38–51. [Google Scholar] [CrossRef]
- Torras, C.; Pallares, J.; Garcia-Valls, R.; Jaffrin, M.Y. Numerical simulation of the flow in a rotating disk filtration module. Desalination 2009, 235, 122–138. [Google Scholar] [CrossRef]
- Pinilla, A.; Berrio, J.C.; Guerrero, E.; Valdés, J.P.; Becerra, D.; Pico, P.; Vargas, L.; Madsen, S.; Bentzen, T.R.; Ratkovich, N. CFD modelling of the hydrodynamics in a filtration unit with rotating membranes. J. Water Proc. Eng. 2020, 36, 101368. [Google Scholar] [CrossRef]
- Childs, P.R. Rotating Flow, 1st ed.; Butterworth-Heinemann: Oxford, UK, 2010; pp. 1–15. [Google Scholar] [CrossRef]
- Launder, B.; Poncet, S.; Serre, E. Laminar, transitional, and turbulent flows in rotor-stator cavities. Annu. Rev. Fluid Mech. 2010, 42, 229–248. [Google Scholar] [CrossRef]
- Ekman, V.W. On the influence of the earth’s rotation on ocean-currents. Ark. Mat. Astr. Fys. 1905, 2, 1–52. [Google Scholar]
- Kármán, T.V. Über laminare und turbulente Reibung. J. Appl. Math. Mech. 1921, 1, 233–252. [Google Scholar] [CrossRef]
- Bödewadt, V.U. Die drehströmung über festem grunde. J. Appl. Math. Mech. 1940, 20, 241–253. [Google Scholar] [CrossRef]
- Batchelor, G.K. Note on a class of solutions of the Navier-Stokes equations representing steady rotationally-symmetric flow. Q. J. Mech. Appl. Math. 1951, 4, 29–41. [Google Scholar] [CrossRef]
- Stewartson, K. On the flow between two rotating coaxial disks. Math. Proc. Camb. Philos. Soc. 1953, 49, 333–341. [Google Scholar] [CrossRef]
- Zandbergen, P.J.; Dijkstra, D. Von Kármán swirling flows. Annu. Rev. Fluid Mech. 1987, 19, 465–491. [Google Scholar] [CrossRef]
- Daily, J.W.; Nece, R.E. Chamber dimension effects on induced flow and frictional resistance of enclosed rotating disks. J. Fluids Eng. 1960, 82, 217–230. [Google Scholar] [CrossRef]
- Chew, J.W.; Owen, J.M.; Pincombe, J.R. Numerical predictions for laminar source-sink flow in a rotating cylindrical cavity. J. Fluid Mech. 1984, 143, 451–466. [Google Scholar] [CrossRef]
- Chew, J.W. Development of a computer program for the prediction of flow in a rotating cavity. Int. J. Numer. Methods Fluids 1984, 4, 667–683. [Google Scholar] [CrossRef]
- Stroock, A.D.; Dertinger, S.K.W.; Ajdari, A.; Mezić, I.; Stone, H.A.; Whitesides, G.M. Chaotic mixer for microchannels. Science 2002, 295, 647–651. [Google Scholar] [CrossRef] [PubMed]
- Kang, T.G.; Singh, M.K.; Kwon, T.H.; Anderson, P.D. Chaotic mixing using periodic and aperiodic sequences of mixing protocols in a micromixer. Microfluid. Nanofluidics 2008, 4, 589–599. [Google Scholar] [CrossRef]
- Jang, H.K.; Kim, Y.J.; Woo, N.S.; Hwang, W.R. Tensorial Navier-slip boundary conditions for patterned surfaces for fluid mixing: Numerical simulations and experiments. AIChE J. 2016, 62, 4574–4585. [Google Scholar] [CrossRef]
- Jung, H.I.; Park, J.E.; Jung, S.Y.; Kang, T.G.; Ahn, K.H. Flow and mixing characteristics of a groove-embedded partitioned pipe mixer. Korea-Aust. Rheol. J. 2020, 32, 319–329. [Google Scholar] [CrossRef]
- Jung, S.Y.; Won, Y.-J.; Jang, J.H.; Yoo, J.H.; Ahn, K.H.; Lee, C.-H. Particle deposition on the patterned membrane surface: Simulation and experiments. Desalination 2015, 370, 17–24. [Google Scholar] [CrossRef]
- Jung, S.Y.; Ahn, K.H. Transport and deposition of colloidal particles on a patterned membrane surface: Effect of cross-flow velocity and the size ratio of particle to surface pattern. J. Membr. Sci. 2019, 572, 309–319. [Google Scholar] [CrossRef]
- Zhou, Z.; Ling, B.; Battiato, I.; Husson, S.M.; Ladner, D.A. Concentration polarization over reverse osmosis membranes with engineered surface features. J. Membr. Sci. 2021, 617, 118199. [Google Scholar] [CrossRef]
- Choi, W.; Lee, C.; Yoo, C.H.; Shin, M.G.; Lee, G.W.; Kim, T.-S.; Jung, H.W.; Lee, J.S.; Lee, J.-H. Structural tailoring of sharkskin-mimetic patterned reverse osmosis membranes for optimizing biofouling resistance. J. Membr. Sci. 2020, 595, 117602. [Google Scholar] [CrossRef]
- Malakian, A.; Zhou, Z.; Messick, L.; Spitzer, T.N.; Ladner, D.A.; Husson, S.M. Understanding the role of pattern geometry on nanofiltration threshold flux. Membranes 2020, 10, 445. [Google Scholar] [CrossRef]
- Kim, K.T.; Park, J.E.; Jung, S.Y.; Kang, T.G. Fouling Mitigation via Chaotic Advection in a Flat Membrane Module with a Patterned Surface. Membranes 2021, 11, 724. [Google Scholar] [CrossRef]
- Jung, S.Y.; Park, J.E.; Kang, T.G.; Ahn, K.H. Design optimization for a microfluidic crossflow filtration system incorporating a micromixer. Micromachines 2019, 10, 836. [Google Scholar] [CrossRef]
- Argyropoulos, C.D.; Markatos, N.C. Recent advances on the numerical modelling of turbulent flows. Appl. Math. Model. 2015, 39, 693–732. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Menter, F.R. Review of the shear-stress transport turbulence model experience from an industrial perspective. Int. J. Comput. Fluid Dyn. 2009, 23, 305–316. [Google Scholar] [CrossRef]
- Zadravec, M.; Basic, S.; Hribersek, M. The influence of rotating domain size in a rotating frame of reference approach for simulation of rotating impeller in a mixing vessel. J. Eng. Sci. Technol. 2007, 2, 126–138. [Google Scholar]
- Silva, P.A.S.F.; Tsoutsanis, P.; Antoniadis, A.F. Simple multiple reference frame for high-order solution of hovering rotors with and without ground effect. Aerosp. Sci. Technol. 2021, 111, 106518. [Google Scholar] [CrossRef]
- Cheah, S.C.; Iacovides, H.; Jackson, D.C.; Ji, H.; Launder, B.E. Experimental investigation of enclosed rotor-stator disk flows. Exp. Therm. Fluid Sci. 1994, 9, 445–455. [Google Scholar] [CrossRef]
- Bouzerar, R.; Jaffrin, M.Y.; Ding, L.; Paullier, P. Influence of geometry and angular velocity on performance of a rotating disk filter. AIChE J. 2000, 46, 257–265. [Google Scholar] [CrossRef]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Takata, K.; Tanida, K. Effect of UF Membrane Rotation on Filtration Performance Using High Concentration Latex Emulsion Solution. Membranes 2022, 12, 422. [Google Scholar] [CrossRef] [PubMed]
- Purkait, M.; Singh, R. Membrane Technology in Separation Science, 1st ed.; CRC Press: Boca Raton, FL, USA, 2017; pp. 53–54. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.E.; Kang, T.G.; Moon, H. The Effect of the Rotating Disk Geometry on the Flow and Flux Enhancement in a Dynamic Filtration System. Membranes 2023, 13, 291. https://doi.org/10.3390/membranes13030291
Park JE, Kang TG, Moon H. The Effect of the Rotating Disk Geometry on the Flow and Flux Enhancement in a Dynamic Filtration System. Membranes. 2023; 13(3):291. https://doi.org/10.3390/membranes13030291
Chicago/Turabian StylePark, Jo Eun, Tae Gon Kang, and Heejang Moon. 2023. "The Effect of the Rotating Disk Geometry on the Flow and Flux Enhancement in a Dynamic Filtration System" Membranes 13, no. 3: 291. https://doi.org/10.3390/membranes13030291
APA StylePark, J. E., Kang, T. G., & Moon, H. (2023). The Effect of the Rotating Disk Geometry on the Flow and Flux Enhancement in a Dynamic Filtration System. Membranes, 13(3), 291. https://doi.org/10.3390/membranes13030291






