Cake Layer Fouling Potential Characterization for Wastewater Reverse Osmosis via Gradient Filtration
Abstract
:1. Introduction
2. Materials and Methods
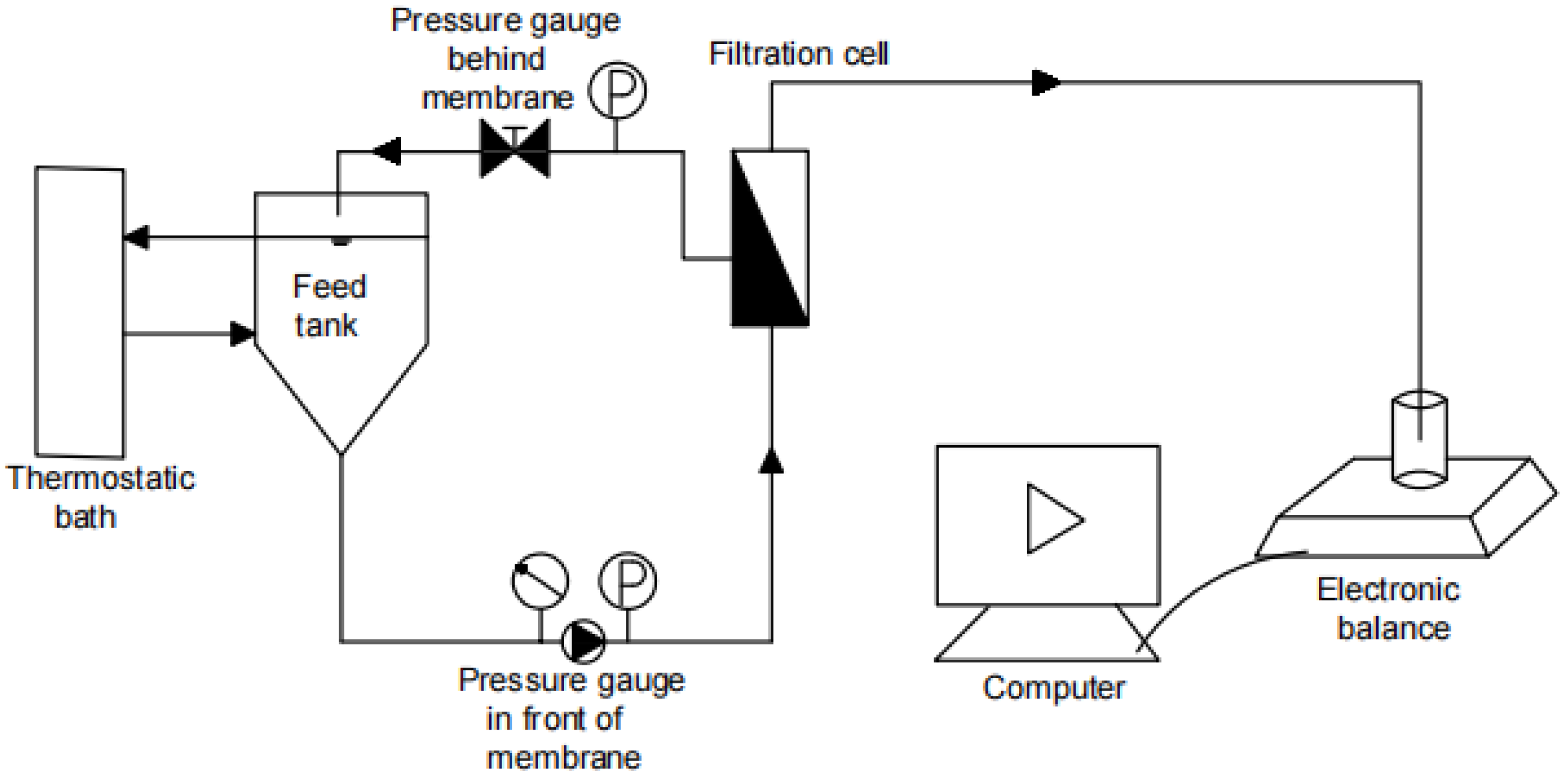
2.1. Experimental Setup for Gradient Filtration
2.2. Wastewater Sample and Analytical Method
2.3. Membrane Fouling Mechanism Analysis
3. Results and Discussion
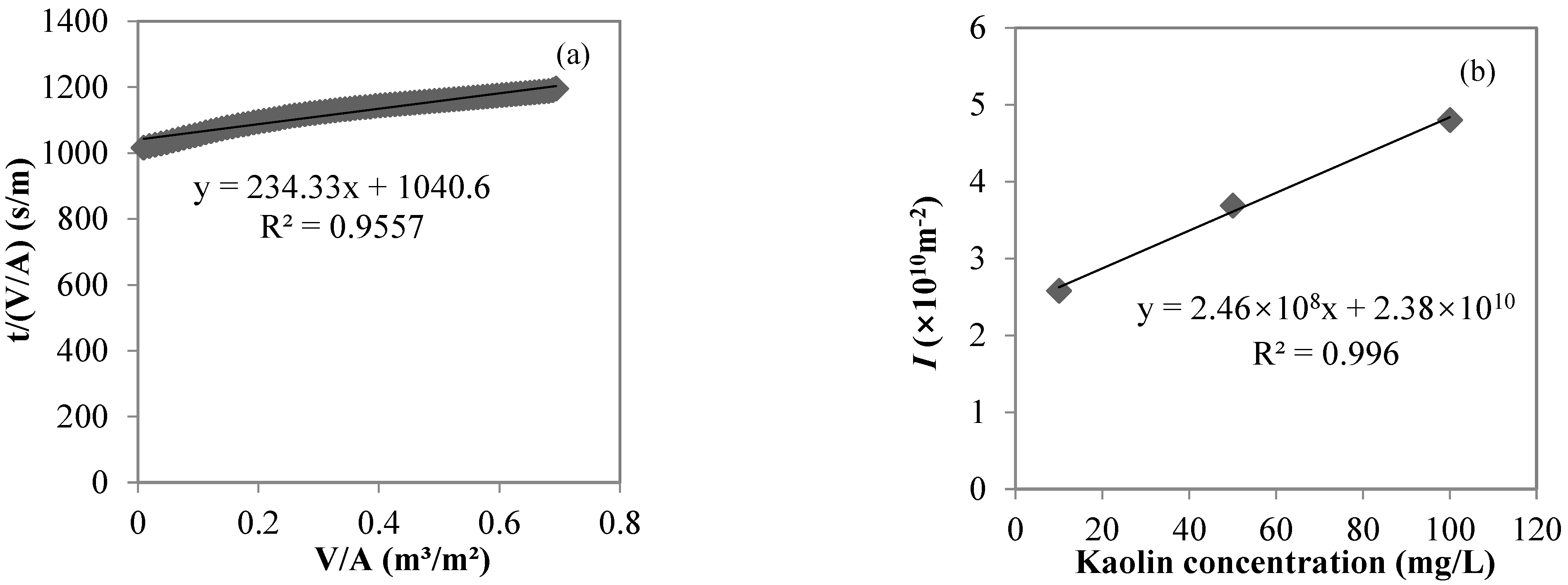
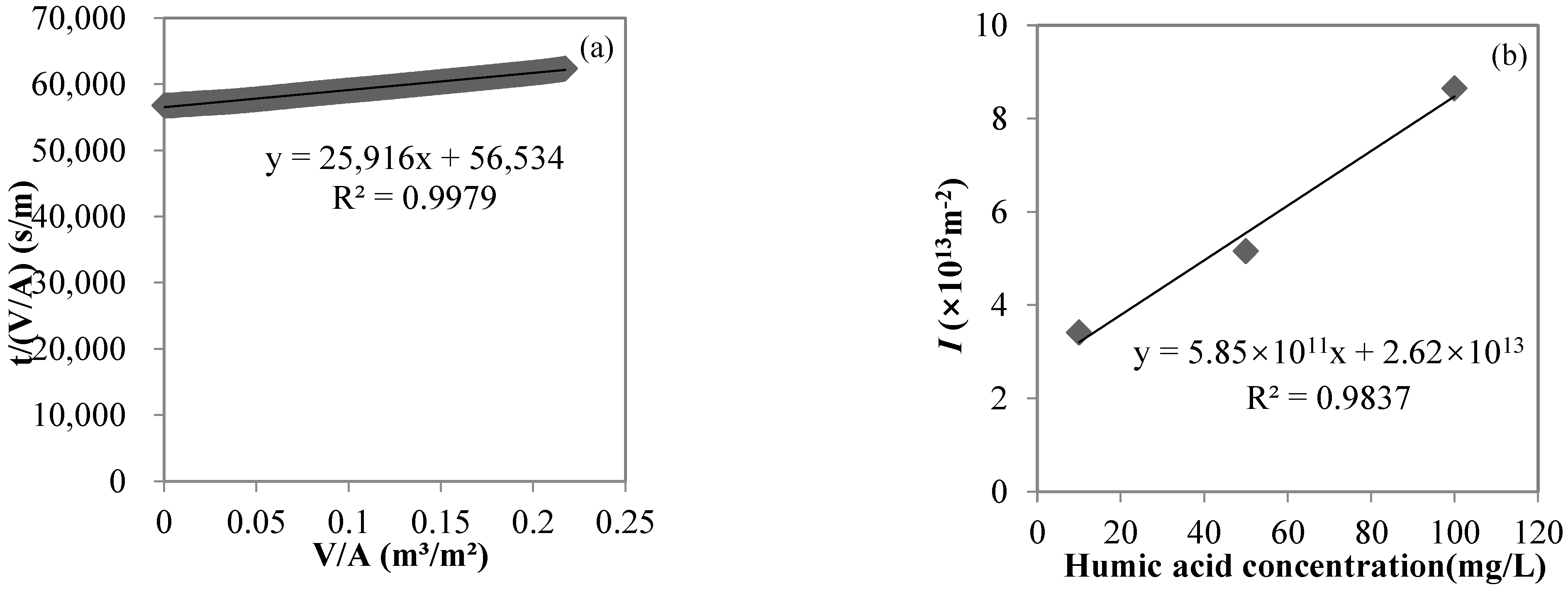
3.1. Response of Cake Layer Fouling Index, I, to Targeted Foulant Concentrations for Individual MF, UF, and NF Tests
3.2. Development of Cake Layer Fouling Potential Assessment Methodology in Gradient Filtration Based on SWW
3.2.1. Rejection Performance during the Gradient Filtration of SWW
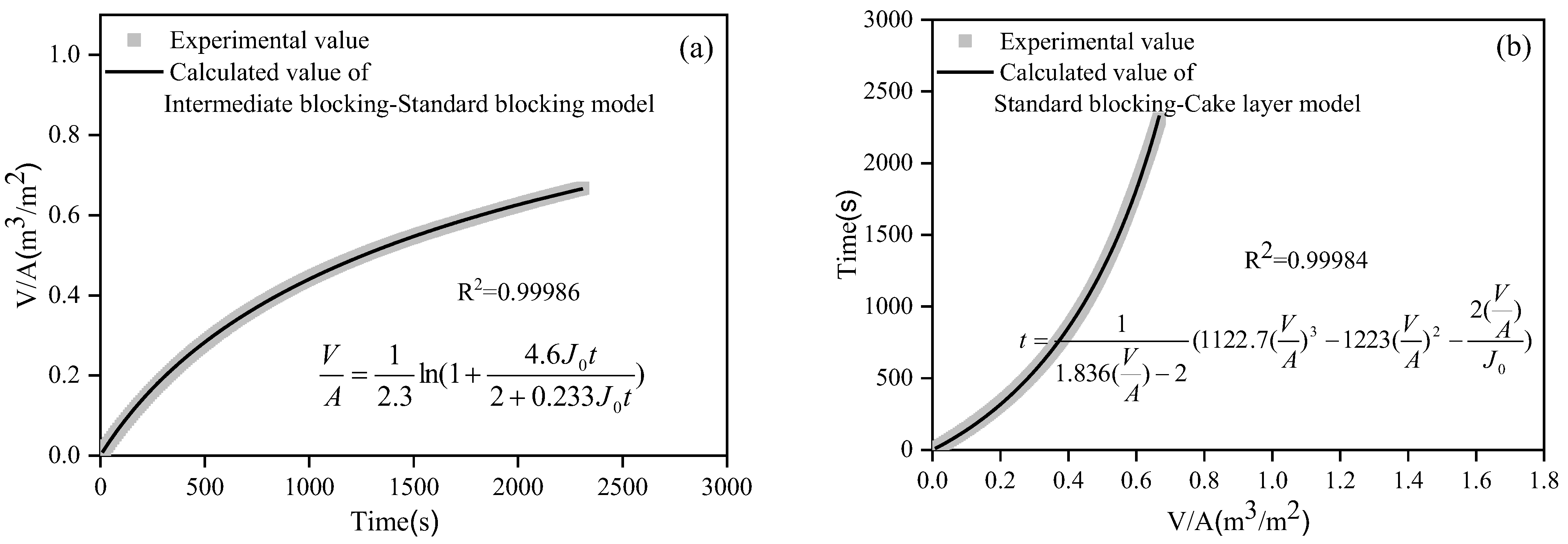
3.2.2. Membrane Fouling Mechanism Analysis in Gradient Filtration Based on Single Models
3.2.3. Membrane Fouling Mechanism Analysis in Gradient Filtration Based on Combined Models
3.2.4. Evaluation of Cake Layer Fouling Potential of SWW Based on Gradient Filtration
3.3. Cake Layer Fouling Potential Assessment via Gradient Filtration of RWW
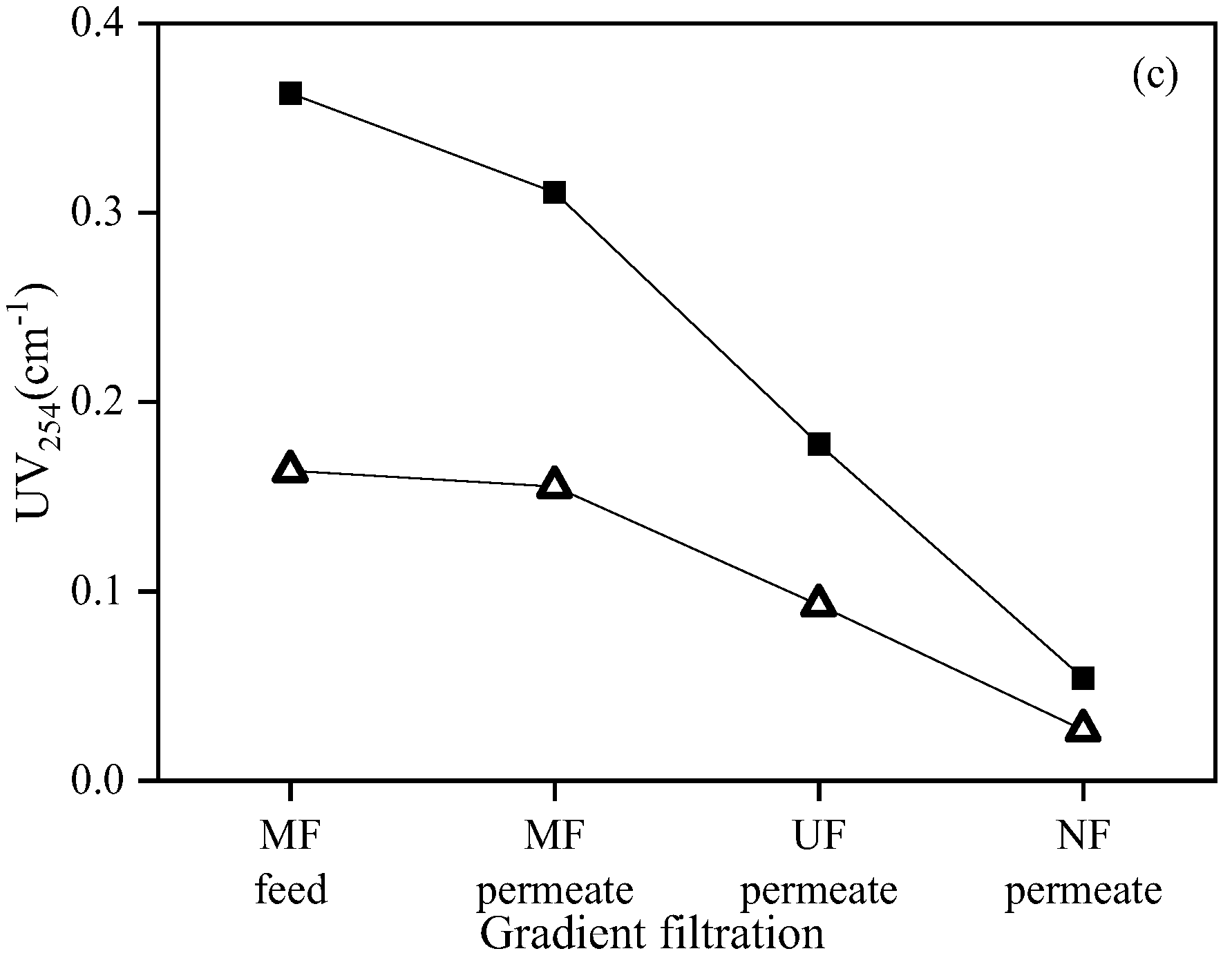
3.3.1. Rejection Performance in Gradient Filtration of RWW
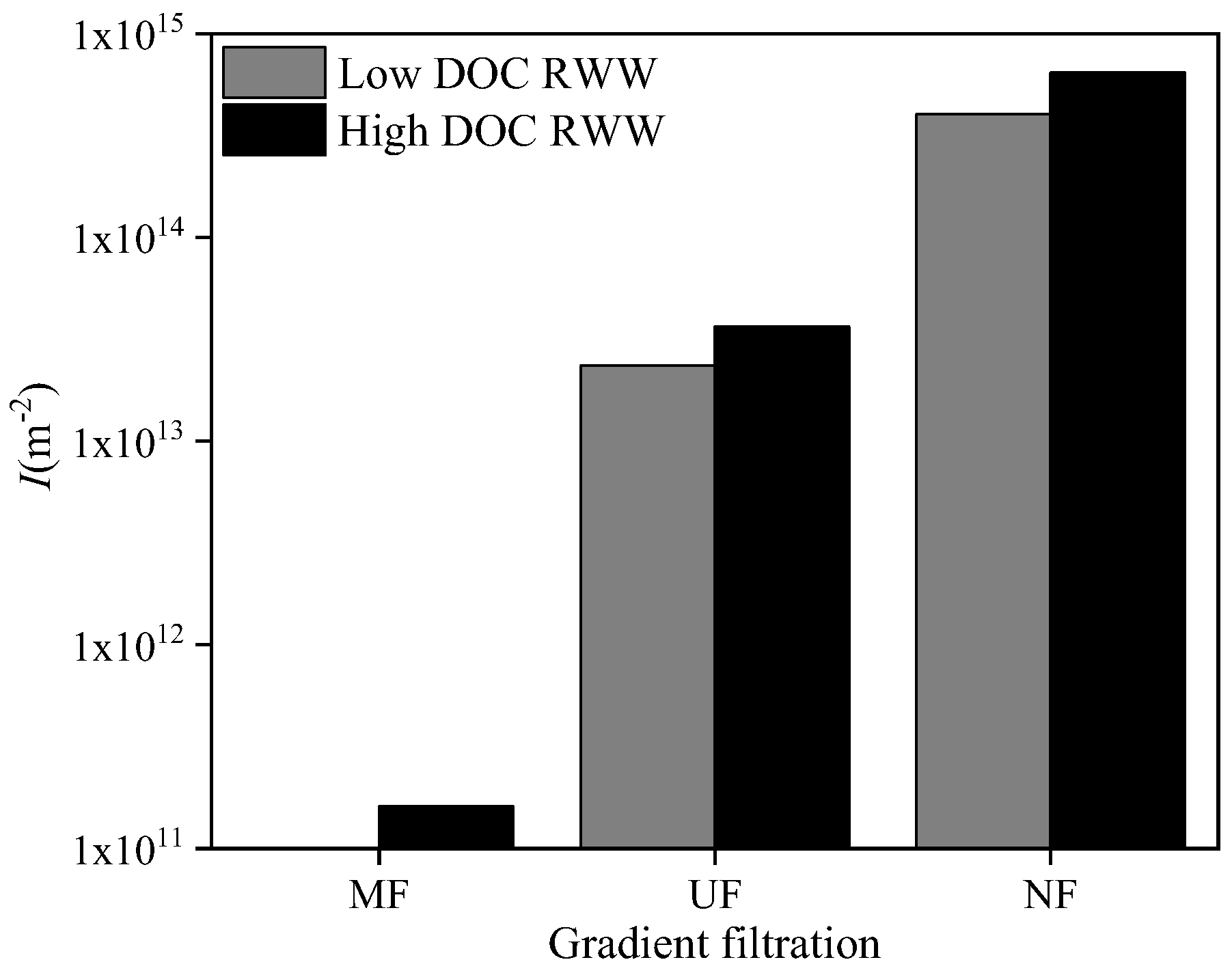
3.3.2. Membrane Fouling Mechanism Analysis and Cake Layer Fouling Potential Evaluation in Gradient Filtration of RWW
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Biesheuvel, P.M.; Porada, S.; Elimelech, M.; Dykstra, J.E. Tutorial review of reverse osmosis and electrodialysis. J. Membr. Sci. 2022, 647, 120221. [Google Scholar] [CrossRef]
- Moossa, B.; Trivedi, P.; Saleem, H.; Zaidi, S.J. Desalination in the GCC countries- a review. J. Clean. Prod. 2022, 357, 131717. [Google Scholar] [CrossRef]
- Park, J.; Lee, S. Desalination Technology in South Korea: A Comprehensive Review of Technology Trends and Future Outlook. Membranes 2022, 12, 204. [Google Scholar] [CrossRef] [PubMed]
- Ruan, G.L.; Wang, M.; An, Z.H.; Xu, G.R.; Ge, Y.H.; Zhao, H.L. Progress and Perspectives of Desalination in China. Membranes 2021, 11, 206. [Google Scholar] [CrossRef]
- Lin, S.S.; Zhao, H.Y.; Zhu, L.P.; He, T.; Chen, S.F.; Gao, C.J.; Zhang, L. Seawater desalination technology and engineering in China: A review. Desalination 2020, 498, 114728. [Google Scholar] [CrossRef]
- Zhang, Z.W.; Wu, Y.H.; Luo, L.W.; Li, G.L.; Li, Y.B.; Hu, H.Y. Application of disk tube reverse osmosis in wastewater treatment: A review. Sci. Total Environ. 2021, 792, 148291. [Google Scholar] [CrossRef]
- Jeffrey, P.; Yang, Z.; Judd, S.J. The status of potable water reuse implementation. Water Res. 2022, 214, 118198. [Google Scholar] [CrossRef]
- Yang, J.Q.; Monnot, M.; Ercolei, L.; Moulin, P. Membrane-Based Processes Used in Municipal Wastewater Treatment for Water Reuse: State-of-the-Art and Performance Analysis. Membranes 2020, 10, 131. [Google Scholar] [CrossRef]
- Jafari, M.; Vanoppen, M.; van Agtmaal, J.M.C.; Cornelissen, E.R.; Vrouwenvelder, J.S.; Verliefde, A.; van Loosdrecht, M.C.M.; Picioreanu, C. Cost of fouling in full-scale reverse osmosis and nanofiltration installations in the Netherlands. Desalination 2020, 500, 114865. [Google Scholar] [CrossRef]
- Jiang, S.X.; Li, Y.N.; Ladewig, B.P. A review of reverse osmosis membrane fouling and control strategies. Sci. Total Environ. 2017, 595, 567–583. [Google Scholar] [CrossRef]
- Matin, A.; Laoui, T.; Falath, W.; Farooque, M. Fouling control in reverse osmosis for water desalination & reuse: Current practices & emerging environment-friendly technologies. Sci. Total Environ. 2020, 765, 142721. [Google Scholar] [CrossRef]
- Qrenawi, L.I.; Abuhabib, A.A. A review on sources, types, mechanisms, characteristics, impacts and control strategies of fouling in RO membrane systems. Water Treat. 2020, 208, 43–69. [Google Scholar] [CrossRef]
- AlSawaftah, N.; Abuwatfa, W.; Darwish, N.; Husseini, G. A Comprehensive Review on Membrane Fouling: Mathematical Modelling, Prediction, Diagnosis, and Mitigation. Water 2021, 13, 1327. [Google Scholar] [CrossRef]
- Xu, H.; Xiao, K.; Wang, X.M.; Liang, S.; Wei, C.H.; Wen, X.H.; Huang, X. Outlining the Roles of Membrane-Foulant and Foulant-Foulant Interactions in Organic Fouling During Microfiltration and Ultrafiltration: A Mini-Review. Front. Chem. 2020, 8, 417. [Google Scholar] [CrossRef]
- Badruzzaman, M.; Voutchkov, N.; Weinrich, L.; Jacangelo, J.G. Selection of pretreatment technologies for seawater reverse osmosis plants: A review. Desalination 2019, 449, 78–91. [Google Scholar] [CrossRef]
- Abushaban, A.; Salinas-Rodriguez, S.G.; Pastorelli, D.; Schippers, J.C.; Mondal, S.; Goueli, S.; Kennedy, M.D. Assessing Pretreatment Effectiveness for Particulate, Organic and Biological Fouling in a Full-Scale SWRO Desalination Plant. Membranes 2021, 11, 167. [Google Scholar] [CrossRef]
- Sim, L.N.; Chong, T.H.; Taheri, A.H.; Sim, S.T.V.; Lai, L.; Krantz, W.B.; Fane, A.G. A review of fouling indices and monitoring techniques for reverse osmosis. Desalination 2018, 434, 169–188. [Google Scholar] [CrossRef]
- Jin, Y.; Lee, H.; Park, C.; Hong, S. ASTM Standard Modified Fouling Index for Seawater Reverse Osmosis Desalination Process: Status, Limitations, and Perspectives. Sep. Purif. Rev. 2020, 49, 55–67. [Google Scholar] [CrossRef]
- Abushaban, A.; Salinas-Rodriguez, S.G.; Philibert, M.; Le Bouille, L.; Necibi, M.C.; Chehbouni, A. Biofouling potential indicators to assess pretreatment and mitigate biofouling in SWRO membranes: A short review. Desalination 2022, 527, 115543. [Google Scholar] [CrossRef]
- D4189-07; Standard Test Method for Silt Density Index (SDI) of Water. ASTM International: West Conshohocken, PA, USA, 2014. [CrossRef]
- Alhadidi, A.; Blankert, B.; Kemperman, A.J.B.; Schurer, R.; Schippers, J.C.; Wessling, M.; van der Meer, W.G.J. Limitations, improvements and alternatives of the silt density index. Desalin Water Treat. 2013, 51, 1104–1113. [Google Scholar] [CrossRef]
- Yoo, H.Y.; Lee, Y.S.; Oh, H.K.; Kim, J.O. Silt density index as a fouling propensity parameter of various membrane materials using dissolved organic matter. J. Water Process Eng. 2021, 44, 102391. [Google Scholar] [CrossRef]
- Wei, C.H.; Laborie, S.; Ben Aim, R.; Amy, G. Full utilization of silt density index (SDI) measurements for seawater pre-treatment. J. Membr. Sci. 2012, 405, 212–218. [Google Scholar] [CrossRef]
- D8002-15; Standard Test Method for Modified Fouling Index (MFI-0.45) of Water. ASTM International: West Conshohocken, PA, USA, 2015. [CrossRef]
- Jin, Y.; Ju, Y.; Lee, H.; Hong, S. Fouling potential evaluation by cake fouling index: Theoretical development, measurements, and its implications for fouling mechanisms. J. Membr. Sci. 2015, 490, 57–64. [Google Scholar] [CrossRef]
- Boerlage, S.F.E.; Kennedy, M.; Aniye, M.P.; Schippers, J.C. Applications of the MFI-UF to measure and predict particulate fouling in RO systems. J. Membr. Sci. 2003, 220, 97–116. [Google Scholar] [CrossRef]
- Khirani, S.; Ben Aim, R.; Manero, M.H. Improving the measurement of the Modified Fouling Index using nanofiltration membranes (NF-MFI). Desalination 2006, 191, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Boerlage, S.F.E.; Kennedy, M.D.; Dickson, M.R.; El-Hodali, D.E.Y.; Schippers, J.C. The modified fouling index using ultrafiltration membranes (MFI-UF): Characterisation, filtration mechanisms and proposed reference membrane. J. Membr. Sci. 2002, 197, 1–21. [Google Scholar] [CrossRef]
- Salinas-Rodriguez, S.G.; Amy, G.L.; Schippers, J.C.; Kennedy, M.D. The Modified Fouling Index Ultrafiltration constant flux for assessing particulate/colloidal fouling of RO systems. Desalination 2015, 365, 79–91. [Google Scholar] [CrossRef] [Green Version]
- Sim, L.N.; Ye, Y.; Chen, V.; Fane, A.G. Crossflow Sampler Modified Fouling Index Ultrafiltration (CFS-MFIUF)-An alternative Fouling Index. J. Membr. Sci. 2010, 360, 174–184. [Google Scholar] [CrossRef]
- Ju, Y.; Hong, S. Nano-colloidal fouling mechanisms in seawater reverse osmosis process evaluated by cake resistance simulator-modified fouling index nanofiltration. Desalination 2014, 343, 88–96. [Google Scholar] [CrossRef]
- Yu, Y.; Lee, S.; Hong, K.; Hong, S. Evaluation of membrane fouling potential by multiple membrane array system (MMAS): Measurements and applications. J. Membr. Sci. 2010, 362, 279–288. [Google Scholar] [CrossRef]
- Ju, Y.; Hong, I.; Hong, S. Multiple MFI measurements for the evaluation of organic fouling in SWRO desalination. Desalination 2015, 365, 136–143. [Google Scholar] [CrossRef]
- Xu, P.; Drewes, J.E.; Kim, T.U.; Bellona, C.; Amy, G. Effect of membrane fouling on transport of organic contaminants in NF/RO membrane applications. J. Membr. Sci. 2006, 279, 165–175. [Google Scholar] [CrossRef]
- Bolton, G.; LaCasse, D.; Kuriyel, R. Combined models of membrane fouling: Development and application to microfiltration and ultrafiltration of biological fluids. J. Membr. Sci. 2006, 277, 75–84. [Google Scholar] [CrossRef]
- Huang, B.; Gu, H.K.; Xiao, K.; Qu, F.S.; Yu, H.R.; Wei, C.H. Fouling Mechanisms Analysis via Combined Fouling Models for Surface Water Ultrafiltration Process. Membranes 2020, 10, 149. [Google Scholar] [CrossRef]
- Li, R.H.; Gao, B.Y.; Wang, W.Y.; Yue, Q.Y.; Wang, Y. Floc properties and membrane fouling in coagulation/ultrafiltration process for the treatment of Xiaoqing River: The role of polymeric aluminum-polymer dual-coagulants. Chemosphere 2019, 243, 125391. [Google Scholar] [CrossRef]
- Xing, J.J.; Liang, H.; Xu, S.Q.; Chuah, C.J.; Luo, X.S.; Wang, T.Y.; Wang, J.L.; Li, G.B.; Snyder, S.A. Organic matter removal and membrane fouling mitigation during algae-rich surface water treatment by powdered activated carbon adsorption pretreatment: Enhanced by UV and UV/chlorine oxidation. Water Res. 2019, 159, 283–293. [Google Scholar] [CrossRef]
- Sun, J.Y.; Xiao, K.; Mo, Y.H.; Liang, P.; Shen, Y.X.; Zhu, N.W.; Huang, X. Seasonal characteristics of supernatant organics and its effect on membrane fouling in a full-scale membrane bioreactor. J. Membr. Sci. 2014, 453, 168–174. [Google Scholar] [CrossRef]
- Wei, C.H.; Amy, G. Sludge Water Characteristics Under Different Separation Methods from a Membrane Bioreactor. Sep. Sci. Technol. 2013, 48, 2540–2548. [Google Scholar] [CrossRef]







| Model Name | Equation * | Characteristic Parameters | Schematic Diagram |
|---|---|---|---|
| Cake layer | Kc (s/m2) |  | |
| Complete blocking | Kb (s−1) |  | |
| Intermediate blocking | Ki (m−1) |  | |
| Standard blocking | Ks (m−1) |  | |
| Complete blocking–Cake layer | Kc (s/m2), Kb (s−1) |  | |
| Intermediate blocking–Cake layer | Kc (s/m2), Ki (m−1) |  | |
| Complete blocking–Standard blocking | Kb (s−1), Ks (m−1) |  | |
| Intermediate blocking–Standard blocking | Ki (m−1), Ks (m−1) |  | |
| Standard blocking–Cake layer | Kc (s/m2), Ks (m−1) |  |
| Cake Layer | Standard Blocking | Intermediate Blocking | Complete Blocking | |||||
|---|---|---|---|---|---|---|---|---|
| Membrane | Low-Concentration SWW | High-Concentration SWW | Low-Concentration SWW | High-Concentration SWW | Low-Concentration SWW | High-Concentration SWW | Low-Concentration SWW | High-Concentration SWW |
| MF | 0.9494 | 0.9862 | 0.9995 | 0.9868 | 0.9958 | 0.9955 | 0.9691 | 0.8441 |
| UF | 0.9996 | 0.9771 | 0.9704 | 0.9419 | 0.9783 | 0.9739 | 0.8213 | 0.8116 |
| NF | 0.9529 | 0.8367 | 0.9776 | 0.8242 | 0.9786 | 0.7607 | 0.9698 | 0.8024 |
| Concentration | Gradient Filtration | Combined Model | Parameter Value | Nonlinear Fitting R2 |
|---|---|---|---|---|
| low-concentration SWW | MF | Intermediate blocking–Standard blocking | Ki = 2.30 m−1 Ks = 0.223 m−1 | 0.99986 |
| Standard blocking–Cake layer | Ks = 1.836 m−1 Kc = 1223 s/m2 | 0.99984 | ||
| UF | Intermediate blocking–Cake layer | Ki = 2.61 × 10−7 m−1 | 0.9994 | |
| Kc = 5.8 × 104 s/m2 | ||||
| NF | Intermediate blocking–Cake layer | Ki = 4.03 × 10−5 m−1 | 0.9988 | |
| Kc = 1.41 × 105 s/m2 | ||||
| high-concentration SWW | MF | Complete blocking–Cake layer | Kb = 0.0178 s−1 | 0.9946 |
| Kc = 2.89 × 104 s/m2 | ||||
| UF | Intermediate blocking–Cake layer | Ki = 1.74 × 10−7 m−1 | 0.9893 | |
| Kc = 1.03 × 105 s/m2 | ||||
| NF | Intermediate blocking–Cake layer | Ki = 1.85 × 10−11 m−1 | 0.995 | |
| Kc = 2.17 × 105 s/m2 |
| Cake Layer | Standard Blocking | Intermediate Blocking | Complete Blocking | |||||
|---|---|---|---|---|---|---|---|---|
| Membrane | Low-DOC RWW | High-DOC RWW | Low-DOC RWW | High-DOC RWW | Low-DOC RWW | High-DOC RWW | Low-DOC RWW | High-DOC RWW |
| MF | --- | 0.9986 | 0.9996 | 0.9934 | 0.9823 | 0.9913 | 0.9117 | 0.9644 |
| UF | 0.9748 | 0.9913 | 0.9518 | 0.9851 | 0.6943 | 0.804 | 0.8857 | 0.9207 |
| NF | 0.8930 | 0.968 | 0.8809 | 09811 | 0.8554 | 0.953 | 0.8824 | 0.9681 |
| Type | Gradient Filtration | Combined Model | Parameter Value | Nonlinear Fitting R2 |
|---|---|---|---|---|
| Low-DOC RWW | MF | Intermediate blocking–Standard blocking | Ki = 3.88 m−1, Ks = 0.70 m−1 | 0.9988 |
| UF | Intermediate blocking–Cake layer | Ki = 9.05 × 10−10 m−1 * | 0.9914 | |
| Kc = 2.36 × 105 s/m2 | ||||
| NF | Intermediate blocking–Cake layer | Ki = 2.36 × 10−6 m−1 * | 0.9913 | |
| Kc = 4.04 × 105 s/m2 | ||||
| High-DOC RWW | MF | Intermediate blocking–Cake layer | Ki = 0.0516 m−1, Kc = 3226 s/m2 | 0.99997 |
| Standard blocking–Cake layer | Ks = 0.468 m−1, Kc = 2532 s/m2 | 0.99996 | ||
| UF | Intermediate blocking–Cake layer | Ki = 2.24 × 10−11 m−1 | 0.9978 | |
| Kc = 3.67 × 105 s/m2 | ||||
| NF | Standard blocking–Cake layer | Ks = 5.97 m−1 | 0.9999 | |
| Kc = 6.47 × 105 s/m2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ouyang, R.; Huang, B.; Wei, C.-H.; Rong, H.; Yu, H.; Qu, F.; Xiao, K.; Huang, X. Cake Layer Fouling Potential Characterization for Wastewater Reverse Osmosis via Gradient Filtration. Membranes 2022, 12, 810. https://doi.org/10.3390/membranes12080810
Ouyang R, Huang B, Wei C-H, Rong H, Yu H, Qu F, Xiao K, Huang X. Cake Layer Fouling Potential Characterization for Wastewater Reverse Osmosis via Gradient Filtration. Membranes. 2022; 12(8):810. https://doi.org/10.3390/membranes12080810
Chicago/Turabian StyleOuyang, Rulu, Bin Huang, Chun-Hai Wei, Hongwei Rong, Huarong Yu, Fangshu Qu, Kang Xiao, and Xia Huang. 2022. "Cake Layer Fouling Potential Characterization for Wastewater Reverse Osmosis via Gradient Filtration" Membranes 12, no. 8: 810. https://doi.org/10.3390/membranes12080810
APA StyleOuyang, R., Huang, B., Wei, C.-H., Rong, H., Yu, H., Qu, F., Xiao, K., & Huang, X. (2022). Cake Layer Fouling Potential Characterization for Wastewater Reverse Osmosis via Gradient Filtration. Membranes, 12(8), 810. https://doi.org/10.3390/membranes12080810








