Knowledge and Technology Used in Capacitive Deionization of Water
Abstract
1. Introduction
2. Background of Research on Capacitive Deionization Method
3. Importance of the CDI Method
4. Geometric and Operational Methods for Testing and Evaluating a CDI Cell
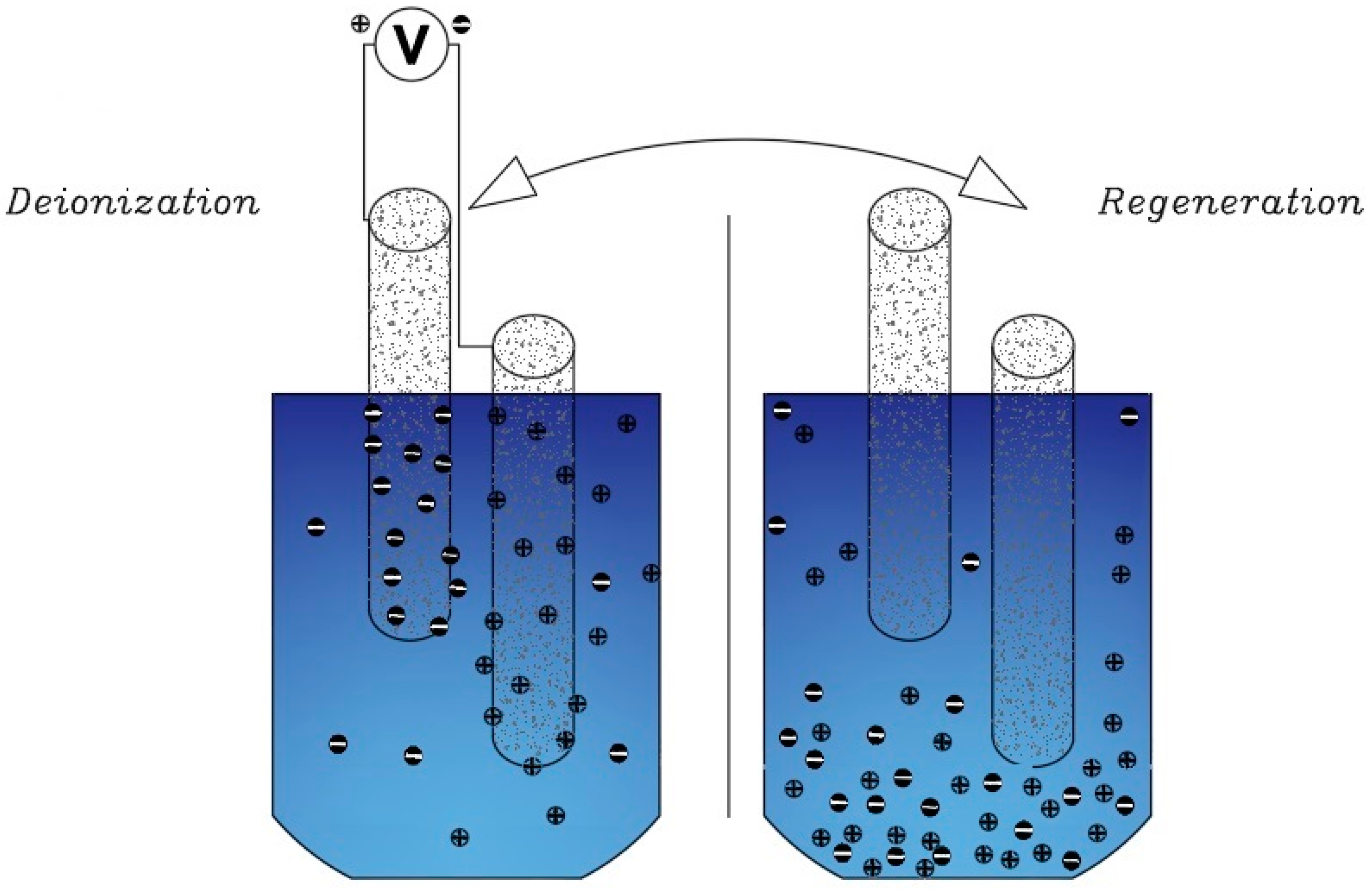
4.1. System Structure
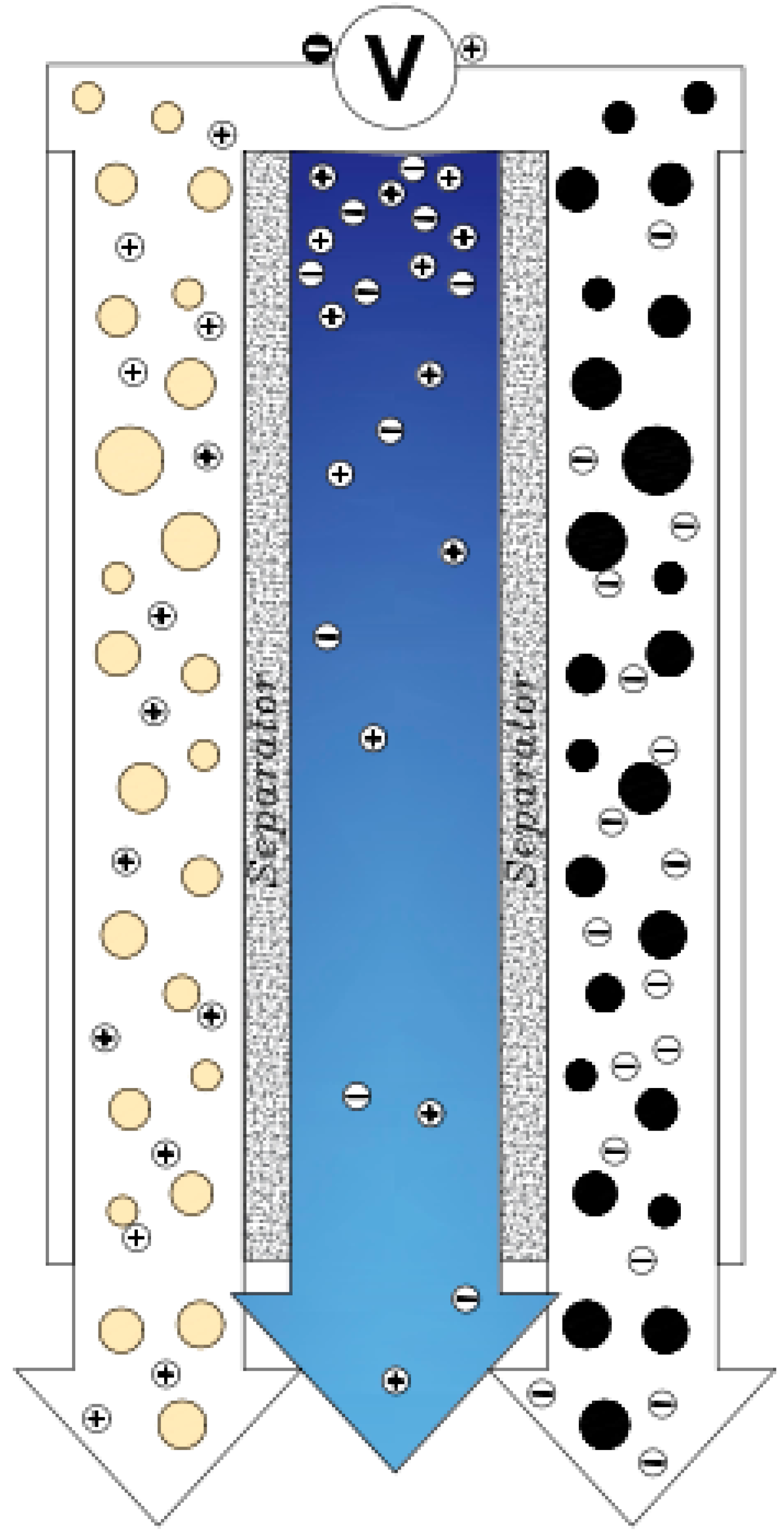
4.2. Designs Used in the Capacitive Method Based on Cell Construction with Two Electrodes
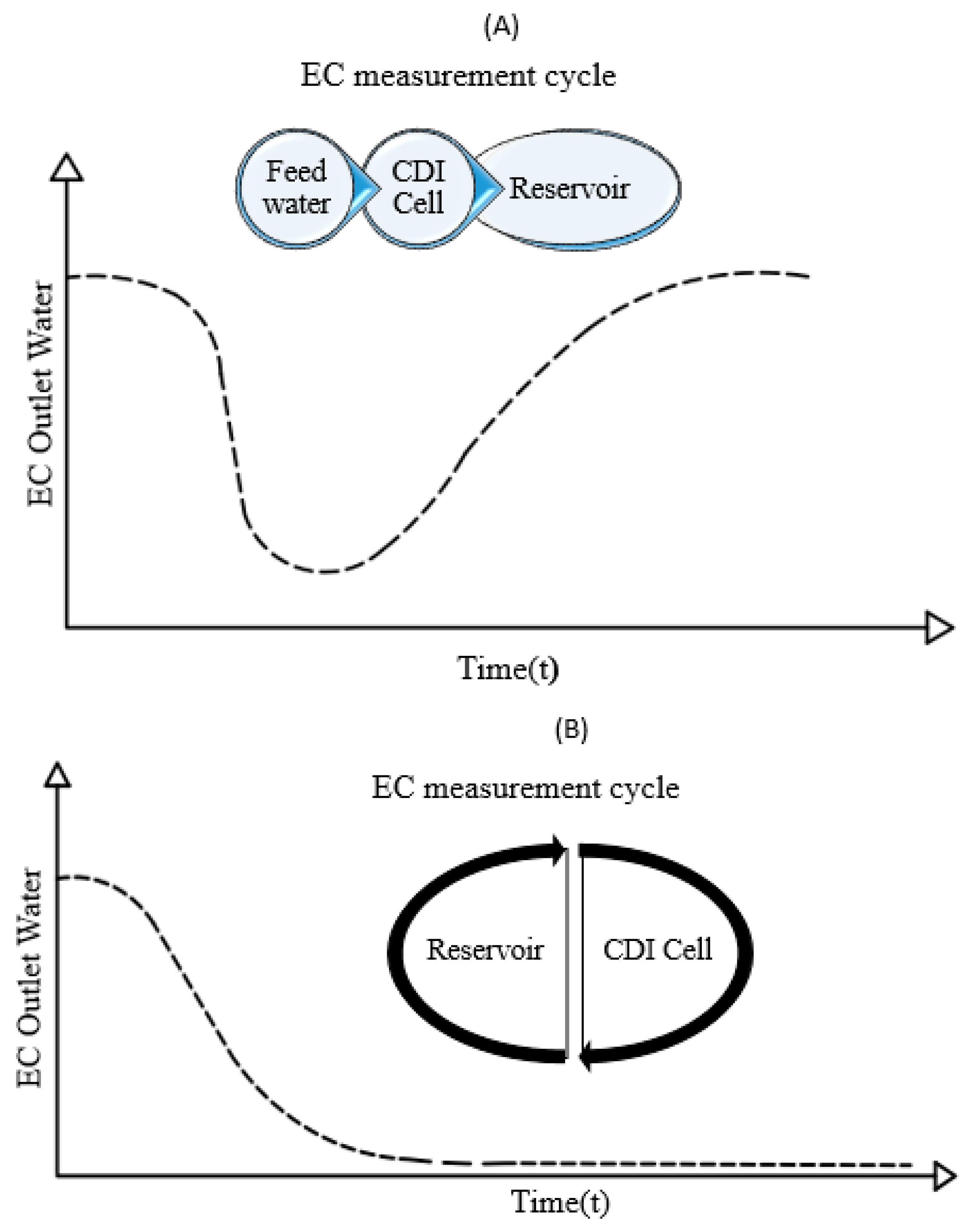
5. Method of Performing CDI Tests
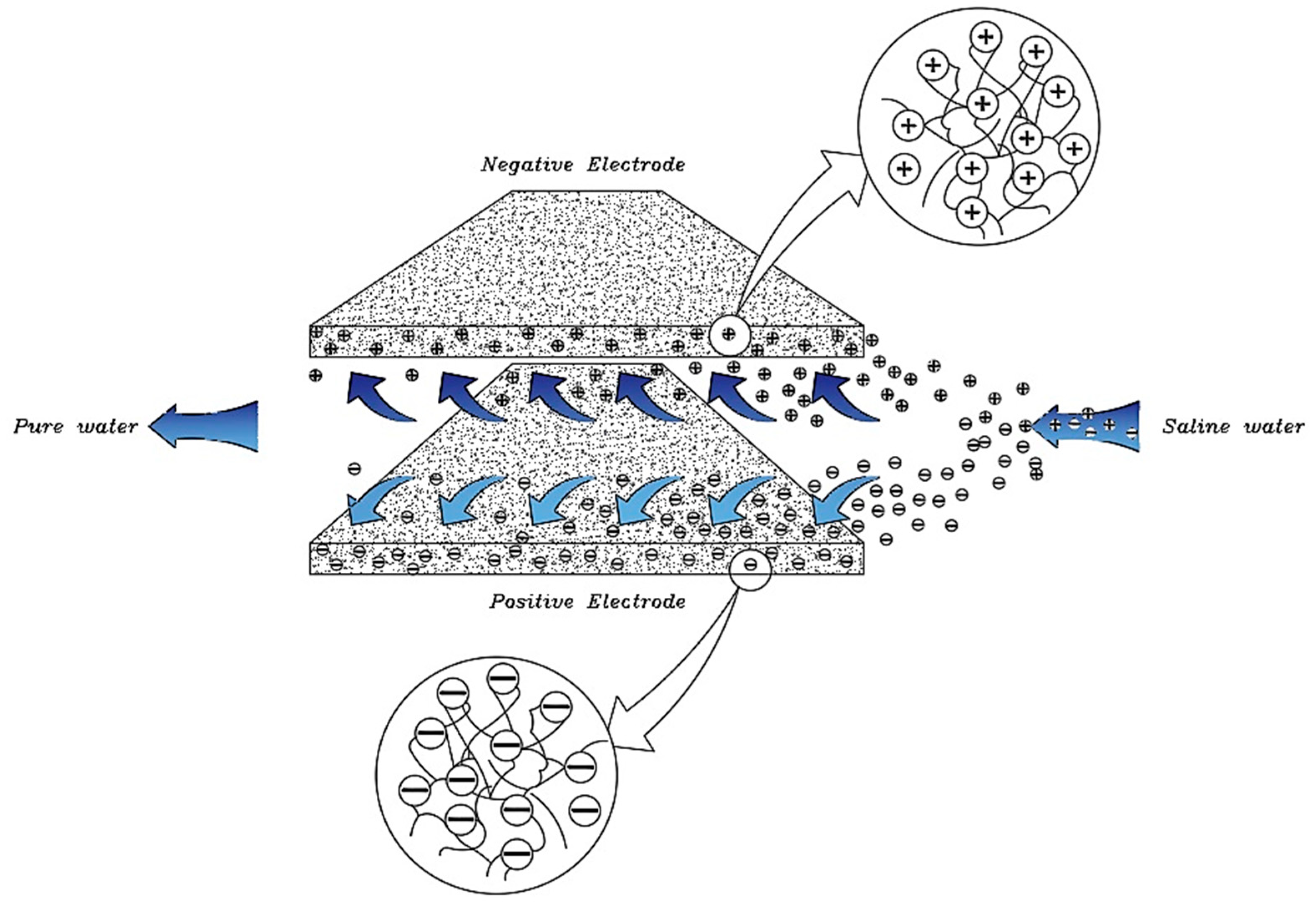
6. Theory and Dominant Principles Capacitive Deionization Method
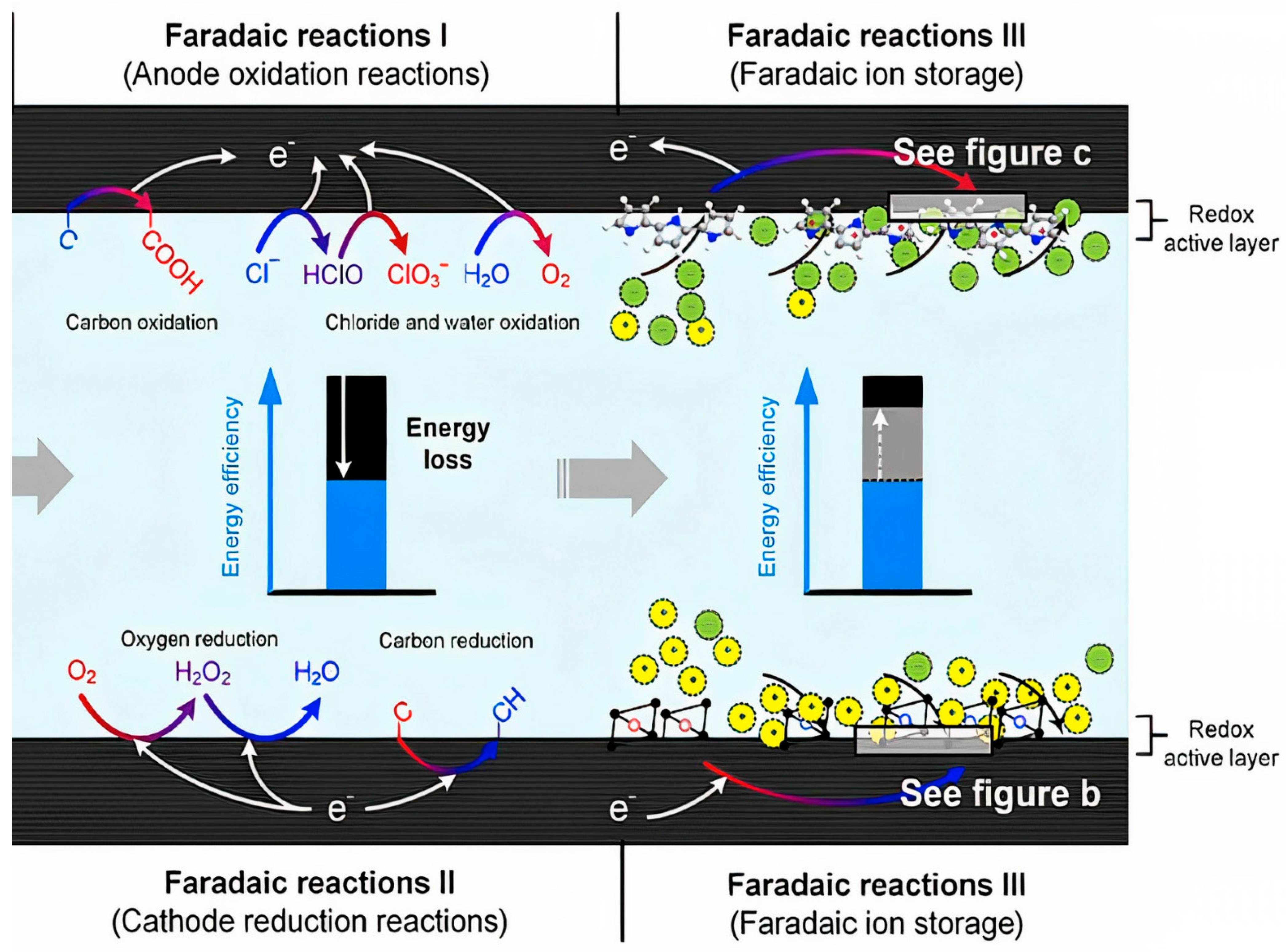
6.1. Electrochemical Reactions and Processes in the Relationship between Carbon and Electrolyte
6.2. Faraday Reactions
6.3. Non-Faraday Reactions
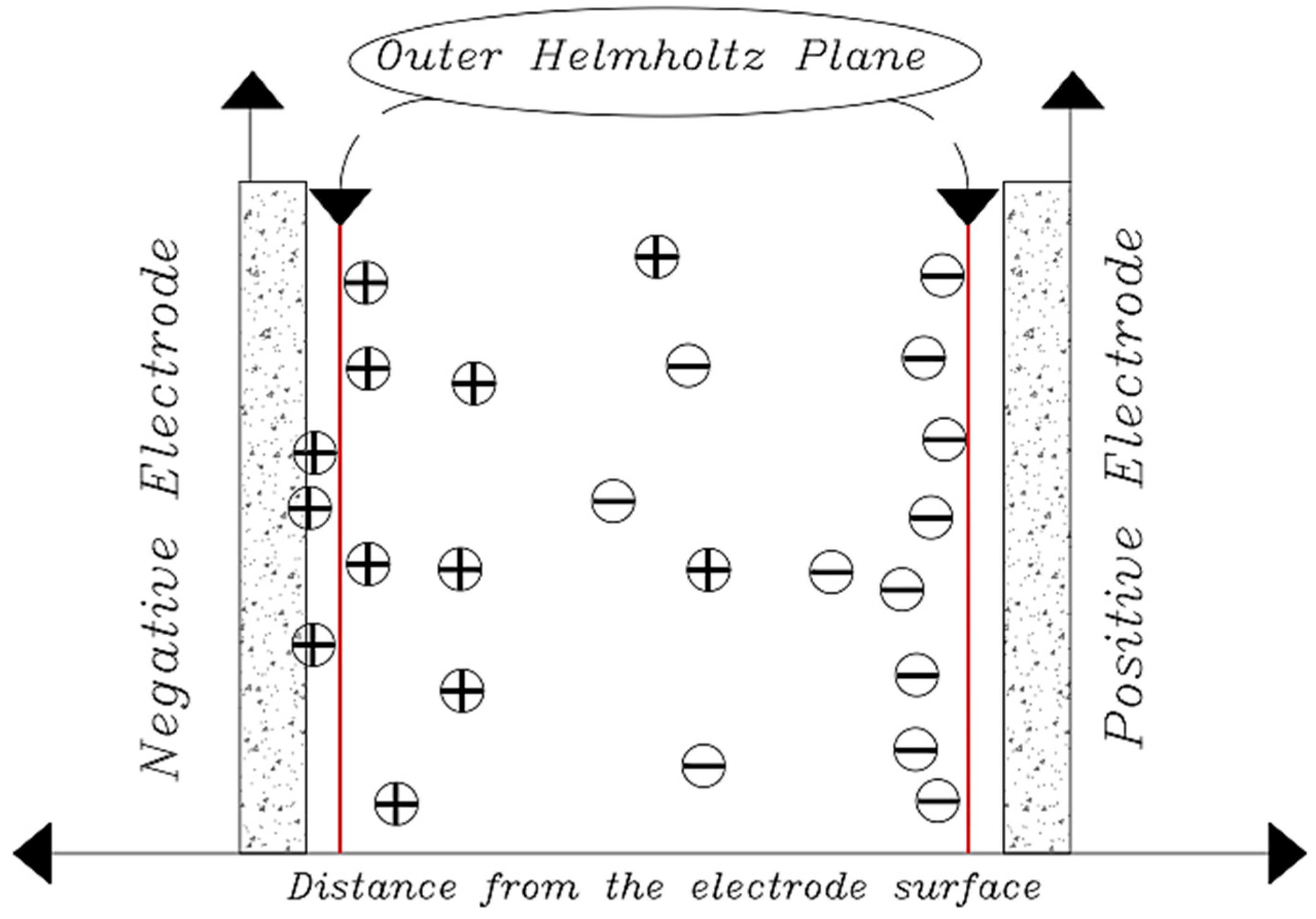
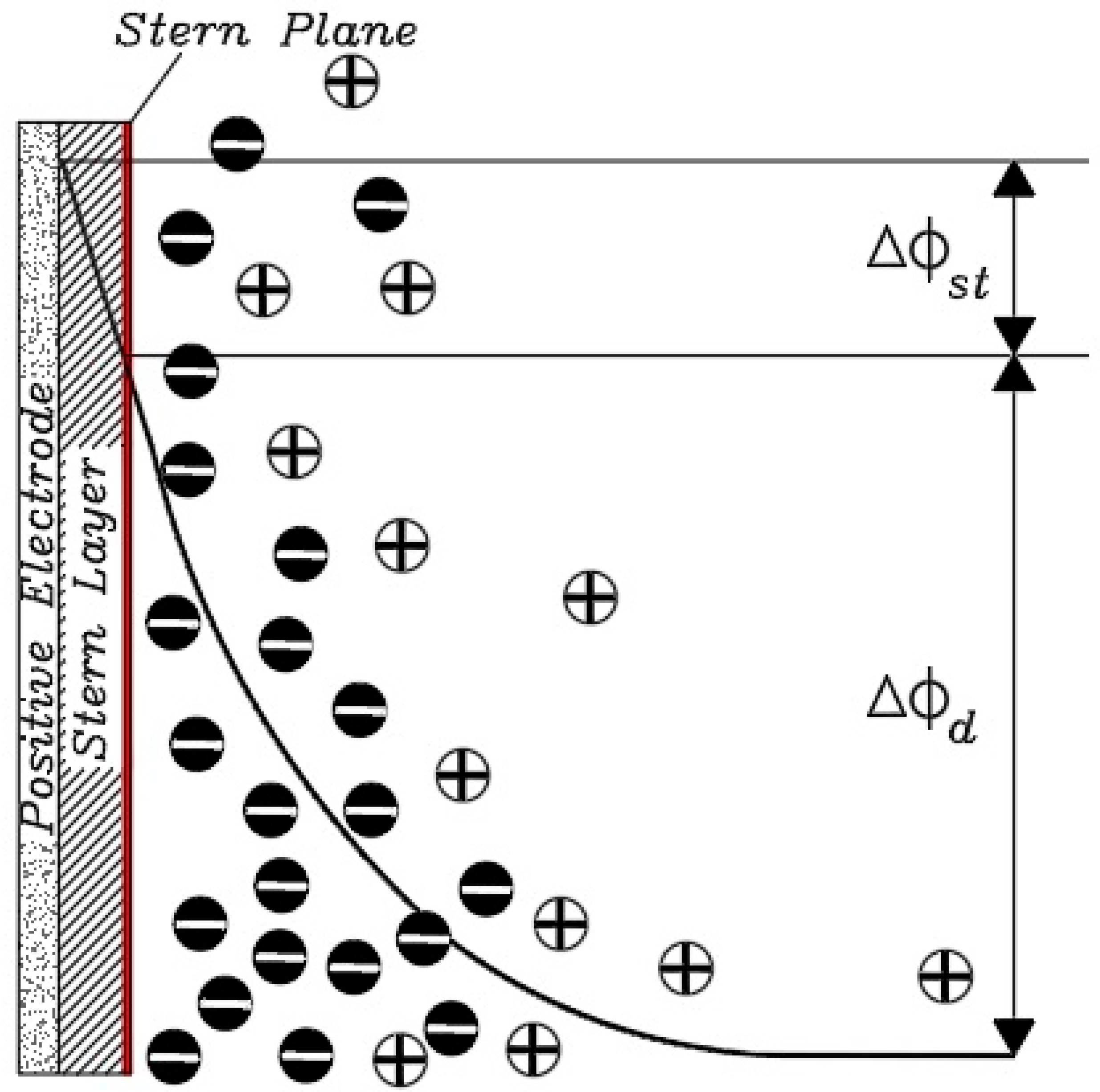
6.4. Electrical Double Layers Theory
6.5. Gouy–Chapman–Stern Theory
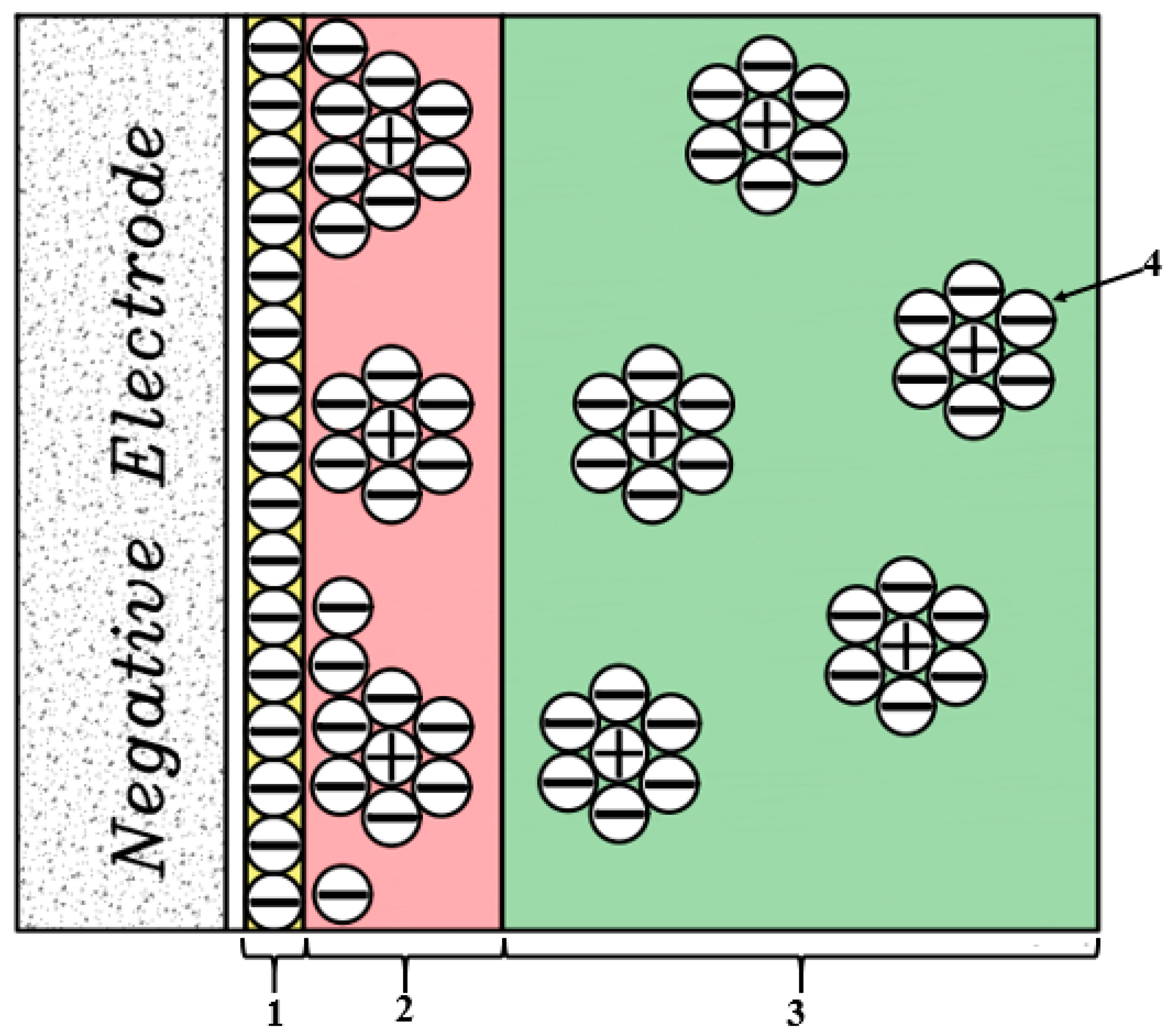
6.6. Modified Donnan Model for Porous Spaces with Overlap in the EDL
7. Standardization of Parameters and Criteria for Measuring the Performance of a Capacitive Deionization System
7.1. Salt Absorption Capacity
7.2. Average Salt Absorption Rate
7.3. Charging Efficiency
7.4. Current Efficiency
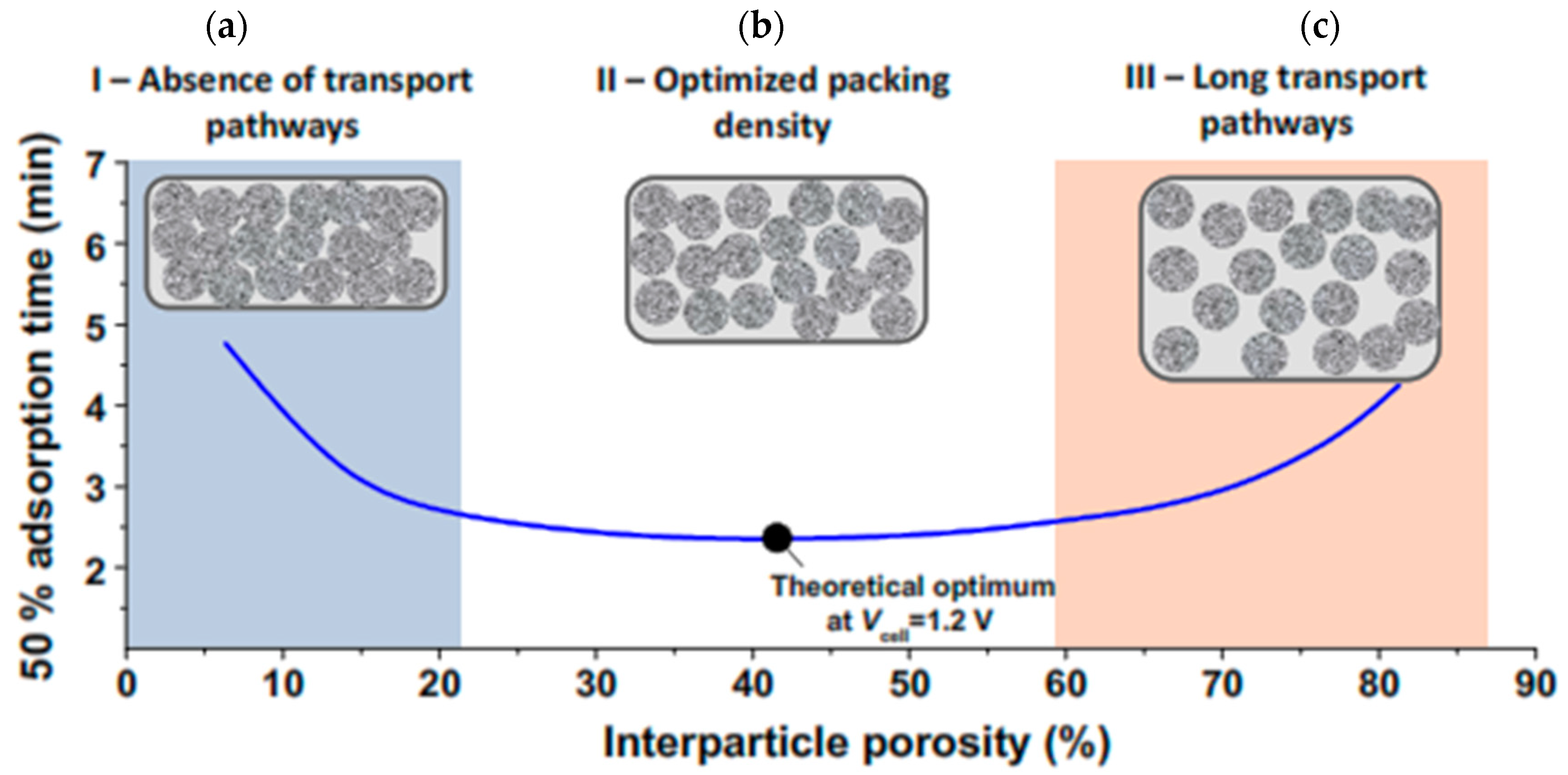
8. Materials Used in Fabricated CDI Cell Electrodes
- It has a high specific surface area in order to be able to absorb ions better, which leads to increasing the adsorption capacity of salt ions [99].
- Ability of fast movement of ion particles inside the porous space that can absorb many ions without restricting the kinetic movement of ions [165].
- High chemical stability in different voltage and pH ranges for system stability and long electrode life [167].
- High hydrophilicity behavior to ensure the utilization of the total porosity in the deionization process [117].
- Flexibility and formability to make electrodes with different shapes [12].
- Not harmful to the environment with the availability of a large amount of raw material [34].
- Low tendency to scaling and fouling.
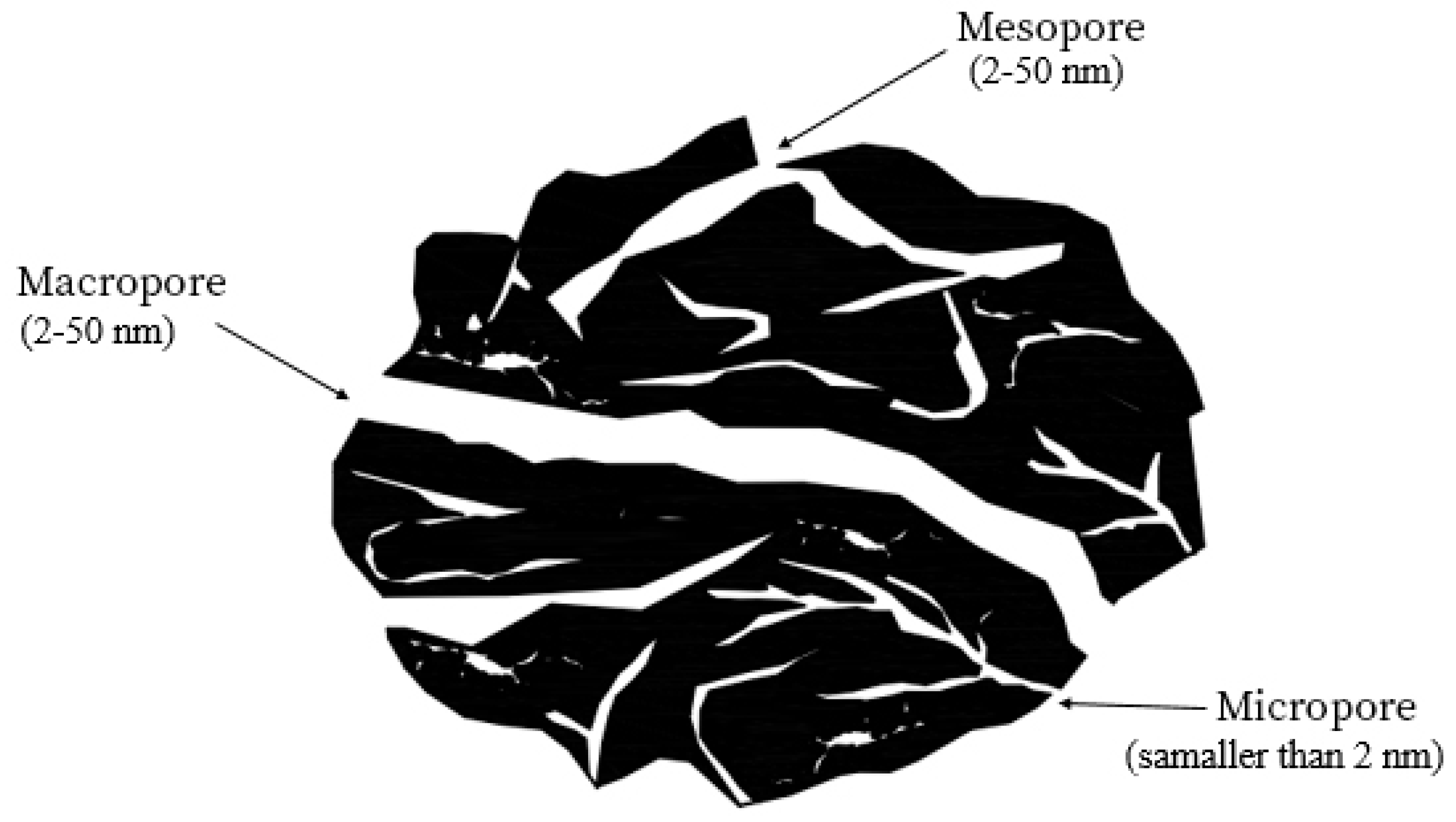
- Macropores with a size larger than 50 nm
- Mesopores with a size between 2 to 50 nm
- Micropores less than 2 nanometers in size, also known as nanopores [171].
8.1. Activated Carbon
8.2. Carbon Aerogel
8.3. Graphene
8.4. Ordered Mesoporous Activated Carbon
8.5. Carbide-Derived Carbons
8.6. Nanotube Carbon
8.7. Carbon Black
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gude, V.G. Desalination and sustainability—An appraisal and current perspective. Water Res. 2016, 89, 87–106. [Google Scholar] [CrossRef] [PubMed]
- Macedonio, F.; Drioli, E.; Gusev, A.A.; Bardow, A.; Semiat, R.; Kurihara, M. Efficient technologies for worldwide clean water supply. Chem. Eng. Process. Process Intensif. 2012, 51, 2–17. [Google Scholar] [CrossRef]
- Keyghobadei, Y. Modeling and Analysis of Combined Dehumidification-Dehumidification System and Low Capacity Gas Turbines; Shahrood University of Technology: Shahroud, Iran, 2016. [Google Scholar]
- Shannon, M.A.; Bohn, P.W.; Elimelech, M.; Georgiadis, J.G.; Mariñas, B.J.; Mayes, A.M. Science and technology for water purification in the coming decades. Nature 2008, 452, 301–310. [Google Scholar] [CrossRef]
- Łaskawiec, E.; Dudziak, M.; Wyczarska-Kokot, J. The application of membrane filtration for recovery of water from filtration bed backwashing stream. Desalin. Water Treat. 2018, 128, 89–95. [Google Scholar] [CrossRef]
- Mitko, K.; Turek, M.; Jaroszek, H.; Bernacka, E.; Sambor, M.; Skóra, P.; Dydo, P. Pilot studies on circular economy solution for the coal mining sector. Water Resour. Ind. 2021, 26, 100161. [Google Scholar] [CrossRef]
- Dehkhoda, A.M. Development and Characterization of Activated Biochar as Electrode Material for Capacitive Deionization. Ph.D. Thesis, University of British Columbia, Vancouver, BC, Canada, 2016. [Google Scholar]
- Demirer, O.N.; Naylor, R.M.; Rios Perez, C.A.; Wilkes, E.; Hidrovo, C. Energetic performance optimization of a capacitive deionization system operating with transient cycles and brackish water. Desalination 2013, 314, 130–138. [Google Scholar] [CrossRef]
- Zhang, J.; Hatzell, K.B.; Hatzell, M.C. A Combined Heat- and Power-Driven Membrane Capacitive Deionization System. Environ. Sci. Technol. Lett. 2017, 4, 470–474. [Google Scholar] [CrossRef]
- Mitko, K.; Turek, M. Membrane-Based Solutions for the Polish Coal Mining Industry. Membranes 2021, 11, 638. [Google Scholar] [CrossRef]
- Turek, M.; Mitko, K.; Laskowska, E.; Chorążewska, M.; Piotrowski, K.; Jakóbik-Kolon, A.; Dydo, P. Energy Consumption and Gypsum Scaling Assessment in a Hybrid Nanofiltration-Reverse Osmosis-Electrodialysis system. Chem. Eng. Technol. 2018, 41, 392–400. [Google Scholar] [CrossRef]
- Oren, Y. Capacitive Deionization (CDI) for Desalination and Water Treatment—Past, Present and Future (A Review). Desalination 2008, 228, 10–29. [Google Scholar] [CrossRef]
- Anderson, M.A.; Cudero, A.L.; Palma, J. Capacitive deionization as an electrochemical means of saving energy and delivering clean water. Comparison to present desalination practices: Will it compete? Electrochim. Acta 2010, 55, 3845–3856. [Google Scholar] [CrossRef]
- Welgemoed, T.J.; Schutte, C.F. Capacitive Deionization Technology™: An alternative desalination solution. Desalination 2005, 183, 327–340. [Google Scholar] [CrossRef]
- Farmer, J. Method and Apparatus for Capacitive Deionization, Electrochemical Purification and Regeneration of Electrodes. U.S. Patent No. 5,425,858, 20 June 1995. [Google Scholar]
- Wang, G.; Qian, B.; Dong, Q.; Yang, J.; Zhao, Z.; Qiu, J. Highly mesoporous activated carbon electrode for capacitive deionization. Sep. Purif. Technol. 2013, 103, 216–221. [Google Scholar] [CrossRef]
- Oh, H.-J.; Lee, J.-H.; Ahn, H.-J.; Jeong, Y.; Kim, Y.-J.; Chi, C.-S. Nanoporous activated carbon cloth for capacitive deionization of aqueous solution. Thin Solid Film. 2006, 515, 220–225. [Google Scholar] [CrossRef]
- Bouhadana, Y.; Ben-Tzion, M.; Soffer, A.; Aurbach, D. A control system for operating and investigating reactors: The demonstration of parasitic reactions in the water desalination by capacitive de-ionization. Desalination 2011, 268, 253–261. [Google Scholar] [CrossRef]
- Biesheuvel, P.M.; Zhao, R.; Porada, S.; van der Wal, A. Theory of membrane capacitive deionization including the effect of the electrode pore space. J. Colloid Interface Sci. 2011, 360, 239–248. [Google Scholar] [CrossRef]
- Zhao, R.; Biesheuvel, P.M.; van der Wal, A. Energy consumption and constant current operation in membrane capacitive deionization. Energy Environ. Sci. 2012, 5, 9520–9527. [Google Scholar] [CrossRef]
- Długołęcki, P.; van der Wal, A. Energy Recovery in Membrane Capacitive Deionization. Environ. Sci. Technol. 2013, 47, 4904–4910. [Google Scholar] [CrossRef]
- Suss, M.E.; Baumann, T.F.; Bourcier, W.L.; Spadaccini, C.M.; Rose, K.A.; Santiago, J.G.; Stadermann, M. Capacitive desalination with flow-through electrodes. Energy Environ. Sci. 2012, 5, 9511–9519. [Google Scholar] [CrossRef]
- Jeon, S.-I.; Park, H.-R.; Yeo, J.-G.; Yang, S.; Cho, C.H.; Han, M.H.; Kim, D.K. Desalination via a new membrane capacitive deionization process utilizing flow-electrodes. Energy Environ. Sci. 2013, 6, 1471–1475. [Google Scholar] [CrossRef]
- Buonomenna, M.G. Membrane processes for a sustainable industrial growth. RSC Adv. 2013, 3, 5694–5740. [Google Scholar] [CrossRef]
- Suss, M. Capacitive Water Desalination with Hierarchical Porous Electrodes; Stanford University: Stanford, CA, USA, 2013. [Google Scholar]
- Blair, J.W.; Murphy, G.W. Electrochemical Demineralization of Water with Porous Electrodes of Large Surface Area. In Saline Water Conversion; American Chemical Society: Washington, DC, USA, 1960; Volume 27, pp. 206–223. [Google Scholar]
- Zhao, R.; Satpradit, O.; Rijnaarts, H.H.M.; Biesheuvel, P.M.; Van der Wal, A. Optimization of salt adsorption rate in membrane capacitive deionization. Water Res. 2013, 47, 1941–1952. [Google Scholar] [CrossRef] [PubMed]
- Arnold, B.B.; Murphy, G.W. Studies on the electrochemistry of carbon and chemically-modified carbon surfaces. J. Phys. Chem. 1961, 65, 135–138. [Google Scholar] [CrossRef]
- Evans, S.; Hamilton, W.S. The mechanism of demineralization at carbon electrodes. J. Electrochem. Soc. 1966, 113, 1314. [Google Scholar] [CrossRef]
- Murphy, G.W.; Caudle, D.D. Mathematical theory of electrochemical demineralization in flowing systems. Electrochim. Acta 1967, 12, 1655–1664. [Google Scholar] [CrossRef]
- Reid, G.W. Field Operation of a 20 Gallons per Day Pilot Plant Unit for Electrochemical Desalination of Brackish Water; US Department of the Interior: Washington, DC, USA, 1968.
- Evans, S.; Accomazzo, M.; Accomazzo, J.E. Electrochemically Controlled Ion Exchange: I. Mechanism. J. Electrochem. Soc. 1969, 116, 307. [Google Scholar] [CrossRef]
- Murphy, G.W. Activated Carbon Used as Electrodes in Electrochemical Demineralization of Saline Water; AGRIS: Klidi, Greece, 1969. [Google Scholar]
- Porada, S.; Zhao, R.; van der Wal, A.; Presser, V.; Biesheuvel, P.M. Review on the science and technology of water desalination by capacitive deionization. Prog. Mater. Sci. 2013, 58, 1388–1442. [Google Scholar] [CrossRef]
- Johnson, A.M.; Venolia, W. The Electrosorb Process for Desalting Water; US Department of Interior: Washington, DC, USA, 1970.
- Zhao, R. Theory and Operation of Capacitive Deionization Systems; Wageningen University and Research: Wageningen, The Netherlands, 2013. [Google Scholar]
- Johnson, A.; Newman, J. Desalting by means of porous carbon electrodes. J. Electrochem. Soc. 1971, 118, 510. [Google Scholar] [CrossRef]
- Soffer, A.; Folman, M. The electrical double layer of high surface porous carbon electrode. J. Electroanal. Chem. Interfacial Electrochem. 1972, 38, 25–43. [Google Scholar] [CrossRef]
- Oren, Y.; Soffer, A. Electrochemical Parametric Pumping. J. Electrochem. Soc. 1978, 125, 869–875. [Google Scholar] [CrossRef]
- Oren, Y.; Soffer, A. Water desalting by means of electrochemical parametric pumping. J. Appl. Electrochem. 1983, 13, 473–487. [Google Scholar] [CrossRef]
- Biesheuvel, P.M.; Fu, Y.; Bazant, M.Z. Diffuse charge and Faradaic reactions in porous electrodes. Phys. Rev. E 2011, 83, 061507. [Google Scholar] [CrossRef] [PubMed]
- Tsouris, C.; Mayes, R.; Kiggans, J.; Sharma, K.; Yiacoumi, S.; De Paoli, D.; Dai, S. Mesoporous Carbon for Capacitive Deionization of Saline Water. Environ. Sci. Technol. 2011, 45, 10243–10249. [Google Scholar] [CrossRef] [PubMed]
- Farmer, J.C.; Fix, D.V.; Mack, G.V.; Pekala, R.W.; Poco, J.F. The Use of Capacitive Deionization with Carbon Aerogel Electrodes to Remove Inorganic Contaminants from Water; Lawrence Livermore National Lab.: Livermore, CA, USA, 1995. [Google Scholar]
- Farmer, J.C.; Fix, D.V.; Mack, G.V.; Pekala, R.W.; Poco, J.F. Capacitive deionization of NaCl and NaNO3 solutions with carbon aerogel electrodes. J. Electrochem. Soc. 1996, 143, 159. [Google Scholar] [CrossRef]
- Chmiola, J.; Yushin, G.; Gogotsi, Y.; Portet, C.; Simon, P.; Taberna, P.L. Anomalous increase in carbon capacitance at pore sizes less than 1 nanometer. Science 2006, 313, 1760–1763. [Google Scholar] [CrossRef]
- Jost, K.; Perez, C.R.; McDonough, J.K.; Presser, V.; Heon, M.; Dion, G.; Gogotsi, Y. Carbon coated textiles for flexible energy storage. Energy Environ. Sci. 2011, 4, 5060–5067. [Google Scholar] [CrossRef]
- Presser, V.; Heon, M.; Gogotsi, Y. Carbide-derived carbons–from porous networks to nanotubes and graphene. Adv. Funct. Mater. 2011, 21, 810–833. [Google Scholar] [CrossRef]
- Hung, K.; Masarapu, C.; Ko, T.; Wei, B. Wide-temperature range operation supercapacitors from nanostructured activated carbon fabric. J. Power Sources 2009, 193, 944–949. [Google Scholar] [CrossRef]
- Foo, K.Y.; Hameed, B.H. A short review of activated carbon assisted electrosorption process: An overview, current stage and future prospects. J. Hazard. Mater. 2009, 170, 552–559. [Google Scholar] [CrossRef]
- Wang, M.; Huang, Z.-H.; Wang, L.; Wang, M.-X.; Kang, F.; Hou, H. Electrospun ultrafine carbon fiber webs for electrochemical capacitive desalination. New J. Chem. 2010, 34, 1843–1845. [Google Scholar] [CrossRef]
- Farma, R.; Deraman, M.; Awitdrus, A.; Talib, I.A.; Taer, E.; Basri, N.H.; Manjunatha, J.G.; Ishak, M.M.; Dollah, B.N.M.; Hashmi, S.A. Preparation of highly porous binderless activated carbon electrodes from fibres of oil palm empty fruit bunches for application in supercapacitors. Bioresour. Technol. 2013, 132, 254–261. [Google Scholar] [CrossRef] [PubMed]
- Yang, C.-M.; Choi, W.-H.; Na, B.-K.; Cho, B.W.; Cho, W.I. Capacitive deionization of NaCl solution with carbon aerogel-silicagel composite electrodes. Desalination 2005, 174, 125–133. [Google Scholar] [CrossRef]
- Xu, P.; Drewes, J.E.; Heil, D.; Wang, G. Treatment of brackish produced water using carbon aerogel-based capacitive deionization technology. Water Res. 2008, 42, 2605–2617. [Google Scholar] [CrossRef]
- Zou, L.; Li, L.; Song, H.; Morris, G. Using mesoporous carbon electrodes for brackish water desalination. Water Res. 2008, 42, 2340–2348. [Google Scholar] [CrossRef]
- Corry, B. Designing Carbon Nanotube Membranes for Efficient Water Desalination. J. Phys. Chem. B 2008, 112, 1427–1434. [Google Scholar] [CrossRef] [PubMed]
- Gao, Y.; Pan, L.; Li, H.; Zhang, Y.; Zhang, Z.; Chen, Y.; Sun, Z. Electrosorption behavior of cations with carbon nanotubes and carbon nanofibres composite film electrodes. Thin Solid Film. 2009, 517, 1616–1619. [Google Scholar] [CrossRef]
- Porada, S.; Weinstein, L.; Dash, R.; van der Wal, A.; Bryjak, M.; Gogotsi, Y.; Biesheuvel, P.M. Water Desalination Using Capacitive Deionization with Microporous Carbon Electrodes. ACS Appl. Mater. Interfaces 2012, 4, 1194–1199. [Google Scholar] [CrossRef] [PubMed]
- Ryoo, M.-W.; Kim, J.-H.; Seo, G. Role of titania incorporated on activated carbon cloth for capacitive deionization of NaCl solution. J. Colloid Interface Sci. 2003, 264, 414–419. [Google Scholar] [CrossRef]
- Wang, G.; Dong, Q.; Ling, Z.; Pan, C.; Yu, C.; Qiu, J. Hierarchical activated carbon nanofiber webs with tuned structure fabricated by electrospinning for capacitive deionization. J. Mater. Chem. 2012, 22, 21819–21823. [Google Scholar] [CrossRef]
- Li, H.; Lu, T.; Pan, L.; Zhang, Y.; Sun, Z. Electrosorption behavior of graphene in NaCl solutions. J. Mater. Chem. 2009, 19, 6773–6779. [Google Scholar] [CrossRef]
- Sami, S.K.; Seo, J.Y.; Hyeon, S.-E.; Shershah, M.S.A.; Yoo, P.-J.; Chung, C.-H. Enhanced capacitive deionization performance by an rGO–SnO2 nanocomposite modified carbon felt electrode. RSC Adv. 2018, 8, 4182–4190. [Google Scholar] [CrossRef]
- Baroud, T.N. Design and Synthesis of Hierarchical Porous Carbons for Optimum Performance as Capacitive Deionization Electrodes. Ph.D. Thesis, Cornell University, Ithaca, NY, USA, 2018. [Google Scholar]
- Cohen, I.; Avraham, E.; Noked, M.; Soffer, A.; Aurbach, D. Enhanced Charge Efficiency in Capacitive Deionization Achieved by Surface-Treated Electrodes and by Means of a Third Electrode. J. Phys. Chem. C 2011, 115, 19856–19863. [Google Scholar] [CrossRef]
- Mitko, K.; Rosiński, A.; Turek, M. Energy consumption in membrane capacitive deionization and electrodialysis of low salinity water. Desalin. Water Treat. 2021, 214, 294–301. [Google Scholar] [CrossRef]
- Biesheuvel, P.M.; van der Wal, A. Membrane capacitive deionization. J. Membr. Sci. 2010, 346, 256–262. [Google Scholar] [CrossRef]
- Lee, J.-B.; Park, K.-K.; Eum, H.-M.; Lee, C.-W. Desalination of a thermal power plant wastewater by membrane capacitive deionization. Desalination 2006, 196, 125–134. [Google Scholar] [CrossRef]
- Zhao, R.; van Soestbergen, M.; Rijnaarts, H.H.; van der Wal, A.; Bazant, M.Z.; Biesheuvel, P.M. Time-dependent ion selectivity in capacitive charging of porous electrodes. J. Colloid Interface Sci. 2012, 384, 38–44. [Google Scholar] [CrossRef]
- Alkuran, M.; Orabi, M.; Scheinberg, N. Highly efficient Capacitive De-Ionization (CDI) water purification system using a buck-boost converter. In Proceedings of the 2008 Twenty-Third Annual IEEE Applied Power Electronics Conference and Exposition, Austin, TX, USA, 24–28 February 2008; pp. 1926–1930. [Google Scholar]
- Landon, J.; Gao, X.; Neathery, J.K.; Liu, K. Energy recovery in parallel capacitive deionization operations. ECS Trans. 2013, 53, 235. [Google Scholar] [CrossRef]
- Council, N.R. Desalination: A National Perspective; The National Academies Press: Washington, DC, USA, 2008; p. 312. [Google Scholar]
- Avlonitis, S.A.; Kouroumbas, K.; Vlachakis, N. Energy consumption and membrane replacement cost for seawater RO desalination plants. Desalination 2003, 157, 151–158. [Google Scholar] [CrossRef]
- Arras, W.; Ghaffour, N.; Hamou, A. Performance evaluation of BWRO desalination plant—A case study. Desalination 2009, 235, 170–178. [Google Scholar] [CrossRef]
- Semiat, R. Energy Issues in Desalination Processes. Environ. Sci. Technol. 2008, 42, 8193–8201. [Google Scholar] [CrossRef]
- Elimelech, M.; Phillip, W.A. The Future of Seawater Desalination: Energy, Technology, and the Environment. Science 2011, 333, 712–717. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J. Experimental Investigation of Combined Heat and Power Capacitive Deionization. Ph.D. Thesis, Georgia Institute of Technology, Atlanta, GA, USA, 2017. [Google Scholar]
- Al-Karaghouli, A.; Renne, D.; Kazmerski, L.L. Technical and economic assessment of photovoltaic-driven desalination systems. Renew. Energy 2010, 35, 323–328. [Google Scholar] [CrossRef]
- Ramadan, M.; Hassan, H.M.A.; Shahat, A.; Elshaarawy, R.F.M.; Allam, N.K. Ultrahigh performance of novel energy-efficient capacitive deionization electrodes based on 3D nanotubular composites. New J. Chem. 2018, 42, 3560–3567. [Google Scholar] [CrossRef]
- Suss, M.E.; Porada, S.; Sun, X.; Biesheuvel, P.M.; Yoon, J.; Presser, V. Water desalination via capacitive deionization: What is it and what can we expect from it? Energy Environ. Sci. 2015, 8, 2296–2319. [Google Scholar] [CrossRef]
- Li, H.; Gao, Y.; Pan, L.; Zhang, Y.; Chen, Y.; Sun, Z. Electrosorptive desalination by carbon nanotubes and nanofibres electrodes and ion-exchange membranes. Water Res. 2008, 42, 4923–4928. [Google Scholar] [CrossRef]
- Lim, J.-A.; Park, N.-S.; Park, J.-S.; Choi, J.-H. Fabrication and characterization of a porous carbon electrode for desalination of brackish water. Desalination 2009, 238, 37–42. [Google Scholar] [CrossRef]
- Porada, S.; Bryjak, M.; van der Wal, A.; Biesheuvel, P.M. Effect of electrode thickness variation on operation of capacitive deionization. Electrochim. Acta 2012, 75, 148–156. [Google Scholar] [CrossRef]
- Zhao, X.T.; Tian, H.; Zhu, M.; Tian, K.; Wang, J.; Kang, F.; Outlaw, R.A. Carbon nanosheets as the electrode material in supercapacitors. J. Power Sources 2009, 194, 1208–1212. [Google Scholar] [CrossRef]
- Avraham, E.; Bouhadana, Y.; Soffer, A.; Aurbach, D. Limitation of Charge Efficiency in Capacitive Deionization I. On the Behavior of Single Activated Carbon. J. Electrochem. Soc. 2009, 156, 95. [Google Scholar] [CrossRef]
- Kim, Y.-J.; Choi, J.-H. Enhanced desalination efficiency in capacitive deionization with an ion-selective membrane. Sep. Purif. Technol. 2010, 71, 70–75. [Google Scholar] [CrossRef]
- Yeo, J.-H.; Choi, J.-H. Enhancement of nitrate removal from a solution of mixed nitrate, chloride and sulfate ions using a nitrate-selective carbon electrode. Desalination 2013, 320, 10–16. [Google Scholar] [CrossRef]
- Kim, Y.-J.; Choi, J.-H. Selective removal of nitrate ion using a novel composite carbon electrode in capacitive deionization. Water Res. 2012, 46, 6033–6039. [Google Scholar] [CrossRef] [PubMed]
- Bourcier, W.L.; Aines, R.D.; Haslam, J.J.; Schaldach, C.M.; O’Brien, K.C.; Cussler, E. Deionization and Desalination Using Electrostatic Ion Pumping; Lawrence Livermore National Lab.: Livermore, CA, USA, 2011. [Google Scholar]
- Hatzell, K.B.; Iwama, E.; Ferris, A.; Daffos, B.; Urita, K.; Tzedakis, T.; Chauvet, F.; Taberna, P.-L.; Gogotsi, Y.; Simon, P. Capacitive deionization concept based on suspension electrodes without ion exchange membranes. Electrochem. Commun. 2014, 43, 18–21. [Google Scholar] [CrossRef]
- Doutre, A.G. Study of Carbon Based Electrodes in Capacitive Deionization for Desalination; University of Idaho: Moscow, ID, USA, 2017. [Google Scholar]
- Lee, J.H.; Lee, S.B.; Bae, G.N.; Jeon, K.S.; Yoon, J.U.; Ji, J.H.; Sung, J.H.; Lee, B.G.; Lee, J.H.; Yang, J.S.; et al. Exposure assessment of carbon nanotube manufacturing workplaces. Inhal. Toxicol. 2010, 22, 369–381. [Google Scholar] [CrossRef] [PubMed]
- Biesheuvel, P.M.; Fu, Y.; Bazant, M.Z. Electrochemistry and capacitive charging of porous electrodes in asymmetric multicomponent electrolytes. Russ. J. Electrochem. 2012, 48, 580–592. [Google Scholar] [CrossRef]
- Benefield, L.D.; Judkins, J.F.; Weand, B.L. Process Chemistry for Water and Wastewater Treatment; Prentice Hall: Hoboken, NJ, USA, 1982. [Google Scholar]
- Cooper, J.L. Activated Carbon Used as Electrodes in Electrochemical Demineralization of Saline Water. Ph.D. Thesis, The University of Oklahoma, Norman, OK, USA, 1968. [Google Scholar]
- Accomazzo, M.A.; Evans, S. Electrochemically Controlled Ion Exchange. J. Electrochem. Soc. 1969, 116, 309. [Google Scholar] [CrossRef][Green Version]
- Liu, Y.; Nie, C.; Liu, X.; Xu, X.; Sun, Z.; Pan, L. Review on carbon-based composite materials for capacitive deionization. RSC Adv. 2015, 5, 15205–15225. [Google Scholar] [CrossRef]
- Dykstra, J.E.; Zhao, R.; Biesheuvel, P.M.; van der Wal, A. Resistance identification and rational process design in Capacitive Deionization. Water Res. 2016, 88, 358–370. [Google Scholar] [CrossRef]
- He, F.; Biesheuvel, P.M.; Bazant, M.Z.; Hatton, T.A. Theory of water treatment by capacitive deionization with redox active porous electrodes. Water Res. 2018, 132, 282–291. [Google Scholar] [CrossRef]
- Zhang, C.; He, D.; Ma, J.; Tang, W.; Waite, T.D. Faradaic reactions in capacitive deionization (CDI)—Problems and possibilities: A review. Water Res. 2018, 128, 314–330. [Google Scholar] [CrossRef]
- Zhao, X.; Wei, H.; Zhao, H.; Wang, Y.; Tang, N. Electrode materials for capacitive deionization: A review. J. Electroanal. Chem. 2020, 873, 114416. [Google Scholar] [CrossRef]
- Maheshwari, K.; Agrawal, M. Advances in capacitive deionization as an effective technique for reverse osmosis reject stream treatment. J. Environ. Chem. Eng. 2020, 8, 104413. [Google Scholar] [CrossRef]
- Elisadiki, J.; King’ondu, C.K. Performance of ion intercalation materials in capacitive deionization/electrochemical deionization: A review. J. Electroanal. Chem. 2020, 878, 114588. [Google Scholar] [CrossRef]
- Sayed, E.T.; Al Radi, M.; Ahmad, A.; Abdelkareem, M.A.; Alawadhi, H.; Atieh, M.A.; Olabi, A.G. Faradic capacitive deionization (FCDI) for desalination and ion removal from wastewater. Chemosphere 2021, 275, 130001. [Google Scholar] [CrossRef]
- Yu, F.; Yang, Z.; Cheng, Y.; Xing, S.; Wang, Y.; Ma, J. A comprehensive review on flow-electrode capacitive deionization: Design, active material and environmental application. Sep. Purif. Technol. 2022, 281, 119870. [Google Scholar] [CrossRef]
- Bouhadana, Y.; Avraham, E.; Noked, M.; Ben-Tzion, M.; Soffer, A.; Aurbach, D. Capacitive Deionization of NaCl Solutions at Non-Steady-State Conditions: Inversion Functionality of the Carbon Electrodes. J. Phys. Chem. C 2011, 115, 16567–16573. [Google Scholar] [CrossRef]
- Avraham, E.; Noked, M.; Bouhadana, Y.; Soffer, A.; Aurbach, D. Limitations of charge efficiency in capacitive deionization processes III: The behavior of surface oxidized activated carbon electrodes. Electrochim. Acta 2010, 56, 441–447. [Google Scholar] [CrossRef]
- Ma, J.; He, C.; He, D.; Zhang, C.; Waite, T.D. Analysis of capacitive and electrodialytic contributions to water desalination by flow-electrode CDI. Water Res. 2018, 144, 296–303. [Google Scholar] [CrossRef]
- Cohen, I.; Avraham, E.; Bouhadana, Y.; Soffer, A.; Aurbach, D. The effect of the flow-regime, reversal of polarization, and oxygen on the long term stability in capacitive de-ionization processes. Electrochim. Acta 2015, 153, 106–114. [Google Scholar] [CrossRef]
- He, D.; Wong, C.E.; Tang, W.; Kovalsky, P.; Waite, T.D. Faradaic Reactions in Water Desalination by Batch-Mode Capacitive Deionization. Environ. Sci. Technol. Lett. 2016, 3, 222–226. [Google Scholar] [CrossRef]
- Lee, J.; Kim, S.; Kim, C.; Yoon, J.J.E.; Science, E. Hybrid capacitive deionization to enhance the desalination performance of capacitive techniques. Energy Environ. Sci. 2014, 7, 3683–3689. [Google Scholar] [CrossRef]
- Wouters, J.J.; Lado, J.J.; Tejedor-Tejedor, M.I.; Perez-Roa, R.; Anderson, M.A. Carbon fiber sheets coated with thin-films of SiO2 and γ-Al2O3 as electrodes in capacitive deionization: Relationship between properties of the oxide films and electrode performance. Electrochim. Acta 2013, 112, 763–773. [Google Scholar] [CrossRef]
- Smith, K.C. Theoretical evaluation of electrochemical cell architectures using cation intercalation electrodes for desalination. Electrochim. Acta 2017, 230, 333–341. [Google Scholar] [CrossRef]
- Su, X.; Hatton, T.A. Redox-electrodes for selective electrochemical separations. Adv. Colloid Interface Sci. 2017, 244, 6–20. [Google Scholar] [CrossRef] [PubMed]
- Tang, W.; He, D.; Zhang, C.; Kovalsky, P.; Waite, T.D. Comparison of Faradaic reactions in capacitive deionization (CDI) and membrane capacitive deionization (MCDI) water treatment processes. Water Res. 2017, 120, 229–237. [Google Scholar] [CrossRef] [PubMed]
- Holubowitch, N.; Omosebi, A.; Gao, X.; Landon, J.; Liu, K. Quasi-steady-state polarization reveals the interplay of capacitive and Faradaic processes in capacitive deionization. ChemElectroChem 2017, 4, 2404–2413. [Google Scholar] [CrossRef]
- Kornyshev, A.A. Double-Layer in Ionic Liquids: Paradigm Change? J. Phys. Chem. B 2007, 111, 5545–5557. [Google Scholar] [CrossRef]
- Silvester, D.S.; Jamil, R.; Doblinger, S.; Zhang, Y.; Atkin, R.; Li, H. Electrical Double Layer Structure in Ionic Liquids and Its Importance for Supercapacitor, Battery, Sensing, and Lubrication Applications. J. Phys. Chem. C 2021, 125, 13707–13720. [Google Scholar] [CrossRef]
- Luciano, M.A.; Ribeiro, H.; Bruch, G.E.; Silva, G.G. Efficiency of capacitive deionization using carbon materials based electrodes for water desalination. J. Electroanal. Chem. 2020, 859, 113840. [Google Scholar] [CrossRef]
- Bard, A.; Faulkner, L. Electrochemical Methods: Fundamentals and Applications; John Wiley & Sons: Hoboken, NJ, USA, 1980; Volume 2. [Google Scholar]
- Jia, B.; Zhang, W. Preparation and Application of Electrodes in Capacitive Deionization (CDI): A State-of-Art Review. Nanoscale Res. Lett. 2016, 11, 64. [Google Scholar] [CrossRef]
- Rieger, P.H. Electrode potentials. In Electrochemistry; Springer: Berlin/Heidelberg, Germany, 1994; pp. 1–58. [Google Scholar]
- Stojek, Z. The electrical double layer and its structure. In Electroanalytical Methods; Springer: Berlin/Heidelberg, Germany, 2010; pp. 3–9. [Google Scholar]
- Oren, Y.; Soffer, A. The electrical double layer of carbon and graphite electrodes: Part II. Fast and slow charging processes. J. Electroanal. Chem. Interfacial Electrochem. 1985, 186, 63–77. [Google Scholar] [CrossRef]
- Oren, Y.; Tobias, H.; Soffer, A. The electrical doublelayer of carbon and graphite electrodes: Part I. Dependence on electrolyte type and concentration. J. Electroanal. Chem. Interfacial Electrochem. 1984, 162, 87–99. [Google Scholar] [CrossRef]
- Oren, Y.; Soffer, A. The electrical double layer of carbon and graphite electrodes: Part III. Charge and dimensional changes at wide potential range. J. Electroanal. Chem. Interfacial Electrochem. 1986, 206, 101–114. [Google Scholar] [CrossRef]
- Kiliç, M.; Bazant, M.Z.; Ajdari, A. Steric effects in the dynamics of electrolytes at large applied voltages. II. Modified Poisson-Nernst-Planck equations. Phys. Rev. E 2007, 75, 021503. [Google Scholar] [CrossRef]
- Kilic, M.S.; Bazant, M.Z.; Ajdari, A. Steric effects in the dynamics of electrolytes at large applied voltages. I. Double-layer charging. Phys. Rev. E 2007, 75, 021502. [Google Scholar] [CrossRef] [PubMed]
- Yang, K.-L.; Ying, T.-Y.; Yiacoumi, S.; Tsouris, C.; Vittoratos, E.S. Electrosorption of Ions from Aqueous Solutions by Carbon Aerogel: An Electrical Double-Layer Model. Langmuir 2001, 17, 1961–1969. [Google Scholar] [CrossRef]
- Gustafsson, J.; Mikkola, P.; Jokinen, M.; Rosenholm, J.B. The influence of pH and NaCl on the zeta potential and rheology of anatase dispersions. Colloids Surf. A Physicochem. Eng. Asp. 2000, 175, 349–359. [Google Scholar] [CrossRef]
- Fedorov, M.V.; Kornyshev, A.A. Towards understanding the structure and capacitance of electrical double layer in ionic liquids. Electrochim. Acta 2008, 53, 6835–6840. [Google Scholar] [CrossRef]
- Zhao, R.; Biesheuvel, P.M.; Miedema, H.; Bruning, H.; van der Wal, A. Charge Efficiency: A Functional Tool to Probe the Double-Layer Structure Inside of Porous Electrodes and Application in the Modeling of Capacitive Deionization. J. Phys. Chem. Lett. 2010, 1, 205–210. [Google Scholar] [CrossRef]
- Bazant, M.Z.; Thornton, K.; Ajdari, A. Diffuse-charge dynamics in electrochemical systems. Phys. Rev. E 2004, 70, 021506. [Google Scholar] [CrossRef]
- Caudill, L.S. Pressure-Driven Stabilization of Capacitive Deionization. Master’s Thesis, University of Kentucky, Lexington, KY, USA, 2018. [Google Scholar]
- Kamran, K.K.; Van Soestbergen, M.; Pel, L. Electrokinetic Salt Removal from Porous Building Materials Using Ion Exchange Membranes. Transp. Porous Media 2012, 96, 221–235. [Google Scholar] [CrossRef]
- Arafat, H.A.; Franz, M.; Pinto, N.G. Effect of Salt on the Mechanism of Adsorption of Aromatics on Activated Carbon. Langmuir 1999, 15, 5997–6003. [Google Scholar] [CrossRef]
- Heldenbrand, A.M. Development of a Predictive and Mechanistic Model for Capacitive Deionization. Ph.D. Thesis, Rice University, Houston, TX, USA, 2015. [Google Scholar]
- Avraham, E.; Noked, M.; Cohen, I.; Soffer, A.; Aurbach, D. The dependence of the desalination performance in capacitive deionization processes on the electrodes PZC. J. Electrochem. Soc. 2011, 158, P168. [Google Scholar] [CrossRef]
- Gao, X.; Omosebi, A.; Landon, J.; Liu, K. Voltage-Based Stabilization of Microporous Carbon Electrodes for Inverted Capacitive Deionization. J. Phys. Chem. C 2018, 122, 1158–1168. [Google Scholar] [CrossRef]
- Qu, Y.; Baumann, T.F.; Santiago, J.G.; Stadermann, M. Characterization of Resistances of a Capacitive Deionization System. Environ. Sci. Technol. 2015, 49, 9699–9706. [Google Scholar] [CrossRef]
- Zornitta, R.L.; García-Mateos, F.J.; Lado, J.J.; Rodríguez-Mirasol, J.; Cordero, T.; Hammer, P.; Ruotolo, L.A.M. High-performance activated carbon from polyaniline for capacitive deionization. Carbon 2017, 123, 318–333. [Google Scholar] [CrossRef]
- Srimuk, P.; Ries, L.; Zeiger, M.; Fleischmann, S.; Jäckel, N.; Tolosa, A.; Krüner, B.; Aslan, M.; Presser, V. High performance stability of titania decorated carbon for desalination with capacitive deionization in oxygenated water. RSC Adv. 2016, 6, 106081–106089. [Google Scholar] [CrossRef]
- Stoller, M.D.; Ruoff, R.S. Best practice methods for determining an electrode material’s performance for ultracapacitors. Energy Environ. Sci. 2010, 3, 1294–1301. [Google Scholar] [CrossRef]
- Zhang, S.; Pan, N. Supercapacitors performance evaluation. Adv. Energy Mater. 2015, 5, 1401401. [Google Scholar] [CrossRef]
- Kim, T.; Yoon, J. CDI ragone plot as a functional tool to evaluate desalination performance in capacitive deionization. RSC Adv. 2015, 5, 1456–1461. [Google Scholar] [CrossRef]
- Biesheuvel, P.M.; Porada, S.; Levi, M.; Bazant, M.Z. Attractive forces in microporous carbon electrodes for capacitive deionization. J. Solid State Electrochem. 2014, 18, 1365–1376. [Google Scholar] [CrossRef]
- Kim, S.; Lee, J.; Kim, C.; Yoon, J. Na2FeP2O7 as a novel material for hybrid capacitive deionization. Electrochim. Acta 2016, 203, 265–271. [Google Scholar] [CrossRef]
- Yoon, H.; Lee, J.; Kim, S.; Yoon, J. Hybrid capacitive deionization with Ag coated carbon composite electrode. Desalination 2017, 422, 42–48. [Google Scholar] [CrossRef]
- Dai, K.; Shi, L.; Fang, J.; Zhang, D.; Yu, B. NaCl adsorption in multi-walled carbon nanotubes. Mater. Lett. 2005, 59, 1989–1992. [Google Scholar] [CrossRef]
- Nativ, P.; Badash, Y.; Gendel, Y. New insights into the mechanism of flow-electrode capacitive deionization. Electrochem. Commun. 2017, 76, 24–28. [Google Scholar] [CrossRef]
- Yue, Z.; Gao, T.; Li, H. Robust synthesis of carbon@ Na4Ti9O20 core-shell nanotubes for hybrid capacitive deionization with enhanced performance. Desalination 2019, 449, 69–77. [Google Scholar] [CrossRef]
- Arora, N.; Banat, F.; Bharath, G.; Alhseinat, E. Capacitive deionization of NaCl from saline solution using graphene/CNTs/ZnO NPs based electrodes. J. Phys. D Appl. Phys. 2019, 52, 12. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, T.; Lu, T.; Sun, Z.; Chua, D.H.; Pan, L. Nitrogen-doped porous carbon spheres for highly efficient capacitive deionization. Electrochim. Acta 2015, 158, 403–409. [Google Scholar] [CrossRef]
- Shen, J.; Li, Y.; Wang, C.; Luo, R.; Li, J.; Sun, X.; Shen, J.; Han, W.; Wang, L. Hollow ZIFs-derived nanoporous carbon for efficient capacitive deionization. Electrochim. Acta 2018, 273, 34–42. [Google Scholar] [CrossRef]
- Al-Enizi, A.M.; Abdel Hameed, R.M.; El-Halwany, M.M.; Bakrey, M.; Shaikh, S.F.; Yousef, A. Tungsten carbide@graphene nanoflakes: Preparation, characterization and electrochemical activity for capacitive deionization technology. J. Colloid Interface Sci. 2021, 581, 112–125. [Google Scholar] [CrossRef]
- Jin, J.; Li, M.; Tang, M.; Li, Y.; Liu, Y.; Cao, H.; Li, F. Tailorable Phase and Structural MnO2 as Electrode for Highly Efficient Hybrid Capacitive Desalination (HCDI). ChemRxiv 2019. [Google Scholar]
- Srimuk, P.; Kaasik, F.; Krüner, B.; Tolosa, A.; Fleischmann, S.; Jäckel, N.; Tekeli, M.C.; Aslan, M.; Suss, M.E.; Presser, V. MXene as a novel intercalation-type pseudocapacitive cathode and anode for capacitive deionization. J. Mater. Chem. A 2016, 4, 18265–18271. [Google Scholar] [CrossRef]
- Govindan, B.; Alhseinat, E.; Darawsheh, I.F.F.; Ismail, I.; Polychronopoulou, K.; Jaoude, M.A.; Arangadi, A.F.; Banat, F. Activated Carbon Derived from Phoenix dactylifera (Palm Tree) and Decorated with MnO2 Nanoparticles for Enhanced Hybrid Capacitive Deionization Electrodes. ChemistrySelect 2020, 5, 3248–3256. [Google Scholar] [CrossRef]
- Kondrat, S.; Wu, P.; Qiao, R.; Kornyshev, A.A. Accelerating charging dynamics in subnanometre pores. Nat. Mater. 2014, 13, 387–393. [Google Scholar] [CrossRef]
- Kim, T.; Yoo, H.D.; Oh, S.M.; Yoon, J. Potential Sweep Method to Evaluate Rate Capability in Capacitive Deionization. Electrochim. Acta 2014, 139, 374–380. [Google Scholar] [CrossRef]
- Kim, T.; Dykstra, J.E.; Porada, S.; van der Wal, A.; Yoon, J.; Biesheuvel, P.M. Enhanced charge efficiency and reduced energy use in capacitive deionization by increasing the discharge voltage. J. Colloid Interface Sci. 2015, 446, 317–326. [Google Scholar] [CrossRef]
- Huang, Y.; Chen, F.; Guo, L.; Yang, H.Y. Ultrahigh performance of a novel electrochemical deionization system based on a NaTi2(PO4)3/rGO nanocomposite. J. Mater. Chem. A 2017, 5, 18157–18165. [Google Scholar] [CrossRef]
- Avraham, E.; Noked, M.; Bouhadana, Y.; Soffer, A.; Aurbach, D. Limitations of Charge Efficiency in Capacitive Deionization. J. Electrochem. Soc. 2009, 156, P157. [Google Scholar] [CrossRef]
- Sufiani, O.; Tanaka, H.; Teshima, K.; Machunda, R.L.; Jande, Y.A.C. Enhanced electrosorption capacity of activated carbon electrodes for deionized water production through capacitive deionization. Sep. Purif. Technol. 2020, 247, 116998. [Google Scholar] [CrossRef]
- Gao, X.; Omosebi, A.; Landon, J.; Liu, K. Surface charge enhanced carbon electrodes for stable and efficient capacitive deionization using inverted adsorption–desorption behavior. Energy Environ. Sci. 2015, 8, 897–909. [Google Scholar] [CrossRef]
- Porada, S.; Weingarth, D.; Hamelers, H.V.M.; Bryjak, M.; Presser, V.; Biesheuvel, P.M. Carbon flow electrodes for continuous operation of capacitive deionization and capacitive mixing energy generation. J. Mater. Chem. A 2014, 2, 9313–9321. [Google Scholar] [CrossRef]
- Özkul, S. Investigation of Desalination Performance of Capacitive Deionization Technology. Master’s Thesis, Middle East Technical University, Ankara, Turkey, 2019. [Google Scholar]
- Gaikwad, M.S.; Balomajumder, C. Capacitive Deionization for Desalination Using Nanostructured Electrodes. Anal. Lett. 2016, 49, 1641–1655. [Google Scholar] [CrossRef]
- AlMarzooqi, F.A.; Al Ghaferi, A.A.; Saadat, I.; Hilal, N. Application of Capacitive Deionisation in water desalination: A review. Desalination 2014, 342, 3–15. [Google Scholar] [CrossRef]
- Chang, L.M.; Duan, X.Y.; Liu, W. Preparation and electrosorption desalination performance of activated carbon electrode with titania. Desalination 2011, 270, 285–290. [Google Scholar] [CrossRef]
- Machunda, R.L.; Jeon, H.; Lee, J.K.; Lee, J. Effects of acid treatment on the improvement of specific capacitance and deionization efficiency of porous carbon electrodes. Water Supply 2009, 9, 159–165. [Google Scholar] [CrossRef]
- Rouquerol, J.; Avnir, D.; Fairbridge, C.W.; Everett, D.H.; Haynes, J.M.; Pernicone, N.; Ramsay, J.D.F.; Sing, K.S.W.; Unger, K.K. Recommendations for the characterization of porous solids (Technical Report). J. Pure Appl. Chem. 1994, 66, 1739–1758. [Google Scholar] [CrossRef]
- Nikitin, A.; Gogotsi, Y. Nanostructured carbide-derived carbon. In Encyclopedia of Nanoscience and Nanotechnology; American Scientific Publishers: Valencia, CA, USA, 2004; Volume 7, pp. 553–574. [Google Scholar]
- Biesheuvel, P.M.; Bazant, M.Z. Nonlinear dynamics of capacitive charging and desalination by porous electrodes. Phys. Rev. E 2010, 81, 031502. [Google Scholar] [CrossRef]
- Dua, R. Advanced Carbon Materials for Environmental and Energy Applications. Ph.D. Thesis, King Abdullah University of Science and Technology, Thuwal, Saudi Arabia, 2014. [Google Scholar]
- Mutha, H.K. Characterization and Performance of Vertically-Aligned Carbon Nanotubes in Capacitive Deionization Systems. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2017. [Google Scholar]
- Ahmed, M.A.; Tewari, S. Capacitive deionization: Processes, materials and state of the technology. J. Electroanal. Chem. 2018, 813, 178–192. [Google Scholar] [CrossRef]
- Humplik, T.; Lee, J.; O’Hern, S.C.; Fellman, B.A.; Baig, M.A.; Hassan, S.F.; Atieh, M.A.; Rahman, F.; Laoui, T.; Karnik, R.; et al. Nanostructured materials for water desalination. Nanotechnology 2011, 22, 292001. [Google Scholar] [CrossRef]
- Bian, S.-W.; Zhao, Y.-P.; Xian, C.-Y. Porous MnO2 hollow spheres constructed by nanosheets and their application in electrochemical capacitors. Mater. Lett. 2013, 111, 75–77. [Google Scholar] [CrossRef]
- Zhang, D.; Shi, L.; Fang, J.; Dai, K.; Li, X. Preparation and desalination performance of multiwall carbon nanotubes. Mater. Chem. Phys. 2006, 97, 415–419. [Google Scholar] [CrossRef]
- Pan, S.-Y.; Snyder, S.W.; Lin, Y.J.; Chiang, P.-C. Electrokinetic desalination of brackish water and associated challenges in the water and energy nexus. Environ. Sci. Water Res. Technol. 2018, 4, 613–638. [Google Scholar] [CrossRef]
- Ma, X.; Chen, Y.-A.; Zhou, K.; Wu, P.-C.; Hou, C.-H. Enhanced desalination performance via mixed capacitive-Faradaic ion storage using RuO2-activated carbon composite electrodes. Electrochim. Acta 2019, 295, 769–777. [Google Scholar] [CrossRef]
- Chen, Z.; Xu, X.; Ding, Z.; Wang, K.; Sun, X.; Lu, T.; Konarova, M.; Eguchi, M.; Shapter, J.G.; Pan, L.; et al. Ti3C2 MXenes-derived NaTi2(PO4)3/MXene nanohybrid for fast and efficient hybrid capacitive deionization performance. Chem. Eng. J. 2021, 407, 127148. [Google Scholar] [CrossRef]
- Wang, K.; Liu, Y.; Ding, Z.; Li, Y.; Lu, T.; Pan, L. Metal–organic-frameworks-derived NaTi2(PO4)3/carbon composites for efficient hybrid capacitive deionization. J. Mater. Chem. A 2019, 7, 12126–12133. [Google Scholar] [CrossRef]
- Yang, S.; Kim, H.; Jeon, S.-I.; Choi, J.; Yeo, J.-G.; Park, H.-R.; Jin, J.; Kim, D.K. Analysis of the desalting performance of flow-electrode capacitive deionization under short-circuited closed cycle operation. Desalination 2017, 424, 110–121. [Google Scholar] [CrossRef]
- Kim, C.; Srimuk, P.; Lee, J.; Fleischmann, S.; Aslan, M.; Presser, V. Influence of pore structure and cell voltage of activated carbon cloth as a versatile electrode material for capacitive deionization. Carbon 2017, 122, 329–335. [Google Scholar] [CrossRef]
- Tang, K.; Chang, J.; Cao, H.; Su, C.; Li, Y.; Zhang, Z.; Zhang, Y. Macropore- and Micropore-Dominated Carbon Derived from Poly(vinyl alcohol) and Polyvinylpyrrolidone for Supercapacitor and Capacitive Deionization. ACS Sustain. Chem. Eng. 2017, 5, 11324–11333. [Google Scholar] [CrossRef]
- Hou, C.H.; Huang, C.Y.; Hu, C.Y. Application of capacitive deionization technology to the removal of sodium chloride from aqueous solutions. Int. J. Environ. Sci. Technol. 2013, 10, 753–760. [Google Scholar] [CrossRef]
- Feng, C.; Chen, Y.A.; Yu, C.P.; Hou, C.H. Highly porous activated carbon with multi-channeled structure derived from loofa sponge as a capacitive electrode material for the deionization of brackish water. Chemosphere 2018, 208, 285–293. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, X.; Wang, H.; Wu, X.; Shen, J. A positive-negative alternate adsorption effect for capacitive deionization in nano-porous carbon aerogel electrodes to enhance desalination capacity. Desalination 2019, 458, 45–53. [Google Scholar] [CrossRef]
- Kohli, D.K.; Singh, R.; Singh, M.K.; Singh, A.; Khardekar, R.K.; Ram Sankar, P.; Tiwari, P.; Gupta, P.K. Study of carbon aerogel-activated carbon composite electrodes for capacitive deionization application. Desalin. Water Treat. 2012, 49, 130–135. [Google Scholar] [CrossRef]
- Zafra, M.; Lavela, P.; Macías, C.; Rasines, G.; Tirado, J.L. Electrosorption of environmental concerning anions on a highly porous carbon aerogel. J. Electroanal. Chem. 2013, 708, 80–86. [Google Scholar] [CrossRef]
- Jia, B.; Zou, L. Wettability and its influence on graphene nansoheets as electrode material for capacitive deionization. Chem. Phys. Lett. 2012, 548, 23–28. [Google Scholar] [CrossRef]
- Abi Jaoude, M.; Alhseinat, E.; Polychronopoulou, K.; Bharath, G.; Darawsheh, I.F.F.; Anwer, S.; Baker, M.A.; Hinder, S.J.; Banat, F. Morphology-dependent electrochemical performance of MnO2 nanostructures on graphene towards efficient capacitive deionization. Electrochim. Acta 2020, 330, 135202. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, K.; Liu, L.; Wang, K.; Xiang, J.; Hou, D.; Wang, J. Titanium nitride nanoparticle embedded membrane for photothermal membrane distillation. Chemosphere 2020, 256, 127053. [Google Scholar] [CrossRef]
- Dai, K.; Shi, L.Y.; Zhang, D.S.; Fang, J.H. NaCl adsorption in multi-walled carbon nanotube/active carbon combination electrode. Chem. Eng. Sci. 2006, 61, 428–433. [Google Scholar] [CrossRef]
- El-Deen, A.G.; Barakat, N.A.; Kim, H.Y. Graphene wrapped MnO2-nanostructures as effective and stable electrode materials for capacitive deionization desalination technology. Desalination 2014, 344, 289–298. [Google Scholar] [CrossRef]
- Xu, X.; Pan, L.; Liu, Y.; Lu, T.; Sun, Z. Enhanced capacitive deionization performance of graphene by nitrogen doping. J. Colloid Interface Sci. 2015, 445, 143–150. [Google Scholar] [CrossRef]
- Sui, Z.; Meng, Q.; Zhang, X.; Ma, R.; Cao, B. Green synthesis of carbon nanotube–graphene hybrid aerogels and their use as versatile agents for water purification. J. Mater. Chem. 2012, 22, 8767–8771. [Google Scholar] [CrossRef]
- Yan, T.; Liu, J.; Lei, H.; Shi, L.; An, Z.; Park, H.S.; Zhang, D. Capacitive deionization of saline water using sandwich-like nitrogen-doped graphene composites via a self-assembling strategy. Environ. Sci. Nano 2018, 5, 2722–2730. [Google Scholar] [CrossRef]
- Li, L.; Zou, L.; Song, H.; Morris, G. Ordered mesoporous carbons synthesized by a modified sol–gel process for electrosorptive removal of sodium chloride. Carbon 2009, 47, 775–781. [Google Scholar] [CrossRef]
- Xu, X.; Tan, H.; Wang, Z.; Wang, C.; Pan, L.; Kaneti, Y.V.; Yang, T.; Yamauchi, Y. Extraordinary capacitive deionization performance of highly-ordered mesoporous carbon nano-polyhedra for brackish water desalination. Environ. Sci. Nano 2019, 6, 981–989. [Google Scholar] [CrossRef]
- Zhou, F.; Gao, T.; Luo, M.; Li, H. Heterostructured graphene@Na4Ti9O20 nanotubes for asymmetrical capacitive deionization with ultrahigh desalination capacity. Chem. Eng. J. 2018, 343, 1–15. [Google Scholar] [CrossRef]
- Wang, Z.; Dou, B.; Zheng, L.; Zhang, G.; Liu, Z.; Hao, Z. Effective desalination by capacitive deionization with functional graphene nanocomposite as novel electrode material. Desalination 2012, 299, 96–102. [Google Scholar] [CrossRef]
- Gu, X.; Yang, Y.; Hu, Y.; Hu, M.; Wang, C. Fabrication of Graphene-Based Xerogels for Removal of Heavy Metal Ions and Capacitive Deionization. ACS Sustain. Chem. Eng. 2015, 3, 1056–1065. [Google Scholar] [CrossRef]
- Zhang, D.; Wen, X.; Shi, L.; Yan, T.; Zhang, J. Enhanced capacitive deionization of graphene/mesoporous carbon composites. Nanoscale 2012, 4, 5440–5446. [Google Scholar] [CrossRef]
- El-Deen, A.G.; Boom, R.M.; Kim, H.Y.; Duan, H.; Chan-Park, M.B.; Choi, J.-H. Flexible 3D Nanoporous Graphene for Desalination and Bio-decontamination of Brackish Water via Asymmetric Capacitive Deionization. ACS Appl. Mater. Interfaces 2016, 8, 25313–25325. [Google Scholar] [CrossRef]
- Li, Z.; Song, B.; Wu, Z.; Lin, Z.; Yao, Y.; Moon, K.-S.; Wong, C.P. 3D porous graphene with ultrahigh surface area for microscale capacitive deionization. Nano Energy 2015, 11, 711–718. [Google Scholar] [CrossRef]
- Shi, W.; Li, H.; Cao, X.; Leong, Z.Y.; Zhang, J.; Chen, T.; Zhang, H.; Yang, H.Y. Ultrahigh Performance of Novel Capacitive Deionization Electrodes based on A Three-Dimensional Graphene Architecture with Nanopores. Sci. Rep. 2016, 6, 18966. [Google Scholar] [CrossRef]
- Evans, S.F.; Ivancevic, M.R.; Wilson, D.J.; Hood, Z.D.; Adhikari, S.P.; Naskar, A.K.; Tsouris, C.; Paranthaman, M.P. Carbon polyaniline capacitive deionization electrodes with stable cycle life. Desalination 2019, 464, 25–32. [Google Scholar] [CrossRef]
- Zhao, C.; Liu, G.; Sun, N.; Zhang, X.; Wang, G.; Zhang, Y.; Zhang, H.; Zhao, H. Biomass-derived N-doped porous carbon as electrode materials for Zn-air battery powered capacitive deionization. Chem. Eng. J. 2018, 334, 1270–1280. [Google Scholar] [CrossRef]
- Chao, L.; Liu, Z.; Zhang, G.; Song, X.; Lei, X.; Noyong, M.; Simon, U.; Chang, Z.; Sun, X. Enhancement of capacitive deionization capacity of hierarchical porous carbon. J. Mater. Chem. A 2015, 3, 12730–12737. [Google Scholar] [CrossRef]
- Arora, N.; Banat, F.; Alhseinat, E. Capacitive deionization performance of CNTs-Si-Ag based electrodes for the removal of heat stable salts from methyldiethanolamine (MDEA) solution in natural gas sweetening units. Chem. Eng. J. 2019, 356, 400–412. [Google Scholar] [CrossRef]
- Li, J.; Ji, B.; Jiang, R.; Zhang, P.; Chen, N.; Zhang, G.; Qu, L. Hierarchical hole-enhanced 3D graphene assembly for highly efficient capacitive deionization. Carbon 2018, 129, 95–103. [Google Scholar] [CrossRef]
- Chang, L.; Hang Hu, Y. 3D Channel-structured graphene as efficient electrodes for capacitive deionization. J. Colloid Interface Sci. 2019, 538, 420–425. [Google Scholar] [CrossRef]
- Liu, X.; Liu, H.; Mi, M.; Kong, W.; Ge, Y.; Hu, J. Technology, P. Nitrogen-doped hierarchical porous carbon aerogel for high-performance capacitive deionization. Sep. Purif. Technol. 2019, 224, 44–50. [Google Scholar] [CrossRef]
- Marsh, H.; Rodríguez-Reinoso, F. Preface. In Activated Carbon; Marsh, H., Rodríguez-Reinoso, F., Eds.; Elsevier Science Ltd.: Oxford, UK, 2006; pp. 15–16. [Google Scholar]
- Oladunni, J.; Zain, J.H.; Hai, A.; Banat, F.; Bharath, G.; Alhseinat, E. A comprehensive review on recently developed carbon based nanocomposites for capacitive deionization: From theory to practice. Sep. Purif. Technol. 2018, 207, 291–320. [Google Scholar] [CrossRef]
- Liu, P.; Yan, T.; Shi, L.; Park, H.S.; Chen, X.; Zhao, Z.; Zhang, D. Graphene-based materials for capacitive deionization. J. Mater. Chem. A 2017, 5, 13907–13943. [Google Scholar] [CrossRef]
- Ban, A.; Schafer, A.; Wendt, H. Fundamentals of electrosorption on activated carbon for wastewater treatment of industrial effluents. J. Appl. Electrochem. 1998, 28, 227–236. [Google Scholar] [CrossRef]
- Jung, C.-H.; Lee, H.-Y.; Moon, J.-K.; Won, H.-J.; Shul, Y.-G. Electrosorption of uranium ions on activated carbon fibers. J. Radioanal. Nucl. Chem. 2011, 287, 833–839. [Google Scholar] [CrossRef]
- Han, L.; Karthikeyan, K.G.; Anderson, M.A.; Wouters, J.J.; Gregory, K.B. Mechanistic insights into the use of oxide nanoparticles coated asymmetric electrodes for capacitive deionization. Electrochim. Acta 2013, 90, 573–581. [Google Scholar] [CrossRef]
- Myint, M.T.Z.; Dutta, J. Fabrication of zinc oxide nanorods modified activated carbon cloth electrode for desalination of brackish water using capacitive deionization approach. Desalination 2012, 305, 24–30. [Google Scholar] [CrossRef]
- Gabelich, C.J.; Tran, T.D.; Suffet, I.H.M. Electrosorption of Inorganic Salts from Aqueous Solution Using Carbon Aerogels. Environ. Sci. Technol. 2002, 36, 3010–3019. [Google Scholar] [CrossRef] [PubMed]
- Fattahi, H. Polymeric aerogels: Preparation, properties, and applications. Basparesh 2015, 5, 89–102. [Google Scholar]
- Kumar, R.; Sen Gupta, S.; Katiyar, S.; Raman, V.K.; Varigala, S.K.; Pradeep, T.; Sharma, A. Carbon aerogels through organo-inorganic co-assembly and their application in water desalination by capacitive deionization. Carbon 2016, 99, 375–383. [Google Scholar] [CrossRef]
- Macías, C.; Rasines, G.; Lavela, P.; Zafra, M.C.; Tirado, J.L.; Ania, C.O. Mn-Containing N-Doped Monolithic Carbon Aerogels with Enhanced Macroporosity as Electrodes for Capacitive Deionization. ACS Sustain. Chem. Eng. 2016, 4, 2487–2494. [Google Scholar] [CrossRef]
- Zafra, M.; Lavela, P.; Rasines, G.; Macías, C.; Tirado, J.; Ania, C.O. A novel method for metal oxide deposition on carbon aerogels with potential application in capacitive deionization of saline water. Electrochim. Acta 2014, 135, 208–216. [Google Scholar] [CrossRef]
- Zhu, G.; Wang, H.; Xu, H.; Zhang, L. Enhanced capacitive deionization by nitrogen-doped porous carbon nanofiber aerogel derived from bacterial-cellulose. J. Electroanal. Chem. 2018, 822, 81–88. [Google Scholar] [CrossRef]
- Luo, G.; Wang, Y.; Gao, L.; Zhang, D.; Lin, T. Graphene bonded carbon nanofiber aerogels with high capacitive deionization capability. Electrochim. Acta 2018, 260, 656–663. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Bandurin, D.; Torre, I.; Kumar, R.K.; Shalom, M.B.; Tomadin, A.; Principi, A.; Auton, G.; Khestanova, E.; Novoselov, K.; Grigorieva, I.V.; et al. Negative local resistance caused by viscous electron backflow in graphene. Science 2016, 351, 1055–1058. [Google Scholar] [CrossRef] [PubMed]
- Bonaccorso, F.; Colombo, L.; Yu, G.; Stoller, M.; Tozzini, V.; Ferrari, A.C.; Ruoff, R.S.; Pellegrini, V. Graphene, related two-dimensional crystals, and hybrid systems for energy conversion and storage. Science 2015, 347, 1246501. [Google Scholar] [CrossRef] [PubMed]
- El-Kady, M.F.; Shao, Y.; Kaner, R.B. Graphene for batteries, supercapacitors and beyond. Nat. Rev. Mater. 2016, 1, 16033. [Google Scholar] [CrossRef]
- Huang, Y.; Liang, J.; Chen, Y. An Overview of the Applications of Graphene-Based Materials in Supercapacitors. Small 2012, 8, 1805–1834. [Google Scholar] [CrossRef]
- Li, H.; Zou, L.; Pan, L.; Sun, Z. Using graphene nano-flakes as electrodes to remove ferric ions by capacitive deionization. Sep. Purif. Technol. 2010, 75, 8–14. [Google Scholar] [CrossRef]
- Lin, D.; Liu, Y.; Liang, Z.; Lee, H.-W.; Sun, J.; Wang, H.; Yan, K.; Xie, J.; Cui, Y. Layered reduced graphene oxide with nanoscale interlayer gaps as a stable host for lithium metal anodes. Nat. Nanotechnol. 2016, 11, 626–632. [Google Scholar] [CrossRef]
- Xu, C.; Xu, B.; Gu, Y.; Xiong, Z.; Sun, J.; Zhao, X.S. Graphene-based electrodes for electrochemical energy storage. Energy Environ. Sci. 2013, 6, 1388–1414. [Google Scholar] [CrossRef]
- Aghigh, A.; Alizadeh, V.; Wong, H.Y.; Islam, M.S.; Amin, N.; Zaman, M. Recent advances in utilization of graphene for filtration and desalination of water: A review. Desalination 2015, 365, 389–397. [Google Scholar] [CrossRef]
- Li, H.; Pan, L.; Lu, T.; Zhan, Y.; Nie, C.; Sun, Z. A comparative study on electrosorptive behavior of carbon nanotubes and graphene for capacitive deionization. J. Electroanal. Chem. 2011, 653, 40–44. [Google Scholar] [CrossRef]
- Dreyer, D.R.; Todd, A.D.; Bielawski, C.W. Harnessing the chemistry of graphene oxide. Chem. Soc. Rev. 2014, 43, 5288–5301. [Google Scholar] [CrossRef] [PubMed]
- Duan, H.; Yan, T.; An, Z.; Zhang, J.; Shi, L.; Zhang, D. Rapid construction of 3D foam-like carbon nanoarchitectures via a simple photochemical strategy for capacitive deionization. RSC Adv. 2017, 7, 39372–39382. [Google Scholar] [CrossRef]
- Liu, P.; Wang, H.; Yan, T.; Zhang, J.; Shi, L.; Zhang, D. Grafting sulfonic and amine functional groups on 3D graphene for improved capacitive deionization. J. Mater. Chem. A 2016, 4, 5303–5313. [Google Scholar] [CrossRef]
- Han, L.; Karthikeyan, K.G.; Anderson, M.A.; Gregory, K.B. Exploring the impact of pore size distribution on the performance of carbon electrodes for capacitive deionization. J. Colloid Interface Sci. 2014, 430, 93–99. [Google Scholar] [CrossRef] [PubMed]
- Tsai, Y.-C.; Doong, R.-A. Hierarchically ordered mesoporous carbons and silver nanoparticles as asymmetric electrodes for highly efficient capacitive deionization. Desalination 2016, 398, 171–179. [Google Scholar] [CrossRef]
- Zhai, Y.; Dou, Y.; Zhao, D.; Fulvio, P.F.; Mayes, R.T.; Dai, S. Carbon Materials for Chemical Capacitive Energy Storage. Adv. Mater. 2011, 23, 4828–4850. [Google Scholar] [CrossRef] [PubMed]
- Kyotani, T.; Chmiola, J.; Gogotsi, Y. Carbide derived carbon and templated carbons. Carbon Mater. Electrochem. Energy Storage Syst. 2009, 77À113. [Google Scholar]
- Aslan, M.; Zeiger, M.; Jäckel, N.; Grobelsek, I.; Weingarth, D.; Presser, V. Improved capacitive deionization performance of mixed hydrophobic/hydrophilic activated carbon electrodes. J. Phys. Condens. Matter Inst. Phys. J. 2016, 28, 114003. [Google Scholar] [CrossRef]
- Villar, I.; Roldan, S.; Ruiz, V.; Granda, M.; Blanco, C.; Menéndez, R.; Santamaría, R. Capacitive Deionization of NaCl Solutions with Modified Activated Carbon Electrodes. Energy Fuels 2010, 24, 3329–3333. [Google Scholar] [CrossRef]
- Iijima, S.; Ichihashi, T. Single-shell carbon nanotubes of 1-nm diameter. Nature 1993, 363, 603–605. [Google Scholar] [CrossRef]
- Cao, Z.; Wei, B. A perspective: Carbon nanotube macro-films for energy storage. Energy Environ. Sci. 2013, 6, 3183–3201. [Google Scholar] [CrossRef]
- Simon, P.; Gogotsi, Y. Materials for electrochemical capacitors. Nat. Mater. 2008, 7, 845–854. [Google Scholar] [CrossRef] [PubMed]
- Jiang, Q.; Qu, M.Z.; Zhou, G.M.; Zhang, B.L.; Yu, Z.L. A study of activated carbon nanotubes as electrochemical super capacitors electrode materials. Mater. Lett. 2002, 57, 988–991. [Google Scholar] [CrossRef]
- Frackowiak, E.; Delpeux, S.; Jurewicz, K.; Szostak, K.M.; Cazorla-Amorós, D.; Béguin, F. Enhanced capacitance of carbon nanotubes through chemical activation. Chem. Phys. Lett. 2002, 361, 35–41. [Google Scholar] [CrossRef]
- Wang, X.Z.; Li, M.G.; Chen, Y.W.; Cheng, R.M.; Huang, S.M.; Pan, L.K.; Sun, Z. Electrosorption of ions from aqueous solutions with carbon nanotubes and nanofibers composite film electrodes. Appl. Phys. Lett. 2006, 89, 053127. [Google Scholar] [CrossRef]
- Wang, L.; Wang, M.; Huang, Z.-H.; Cui, T.; Gui, X.; Kang, F.; Wang, K.; Wu, D. Capacitive deionization of NaCl solutions using carbon nanotube sponge electrodes. J. Mater. Chem. 2011, 21, 18295–18299. [Google Scholar] [CrossRef]
- Pandolfo, A.G.; Hollenkamp, A.F. Carbon properties and their role in supercapacitors. J. Power Sources 2006, 157, 11–27. [Google Scholar] [CrossRef]
- Mossad, M.; Zhang, W.; Zou, L. Using capacitive deionisation for inland brackish groundwater desalination in a remote location. Desalination 2013, 308, 154–160. [Google Scholar] [CrossRef]
- Kuhlbusch, T.A.J.; Crutzen, P.J. Toward a global estimate of black carbon in residues of vegetation fires representing a sink of atmospheric CO2 and a source of O2. Glob. Biogeochem. Cycles 1995, 9, 491–501. [Google Scholar] [CrossRef]
- Lima, M.C.F.S.; Zaida do Amparo, S.; Ribeiro, H.; Soares, A.L.; Viana, M.M.; Seara, L.M.; Paniago, R.M.; Silva, G.G.; Caliman, V. Aqueous suspensions of carbon black with ethylenediamine and polyacrylamide-modified surfaces: Applications for chemically enhanced oil recovery. Carbon 2016, 109, 290–299. [Google Scholar] [CrossRef]
- Donnet, J.-B. Carbon Black: Science and Technology; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Rasines, G.; Lavela, P.; Macías, C.; Zafra, M.C.; Tirado, J.L.; Ania, C.O. On the use of carbon black loaded nitrogen-doped carbon aerogel for the electrosorption of sodium chloride from saline water. Electrochim. Acta 2015, 170, 154–163. [Google Scholar] [CrossRef]
- Li, H.; Ma, Y.; Niu, R. Improved capacitive deionization performance by coupling TiO2 nanoparticles with carbon nanotubes. Sep. Purif. Technol. 2016, 171, 93–100. [Google Scholar] [CrossRef]
- Kinoshita, K. Carbon: Electrochemical and Physicochemical Properties; US Department of Energy: Washington, DC, USA, 1988.
- Beck, F.; Dolata, M.; Grivei, E.; Probst, N. Electrochemical supercapacitors based on industrial carbon blacks in aqueous H2SO4. J. Appl. Electrochem. 2001, 31, 845–853. [Google Scholar] [CrossRef]


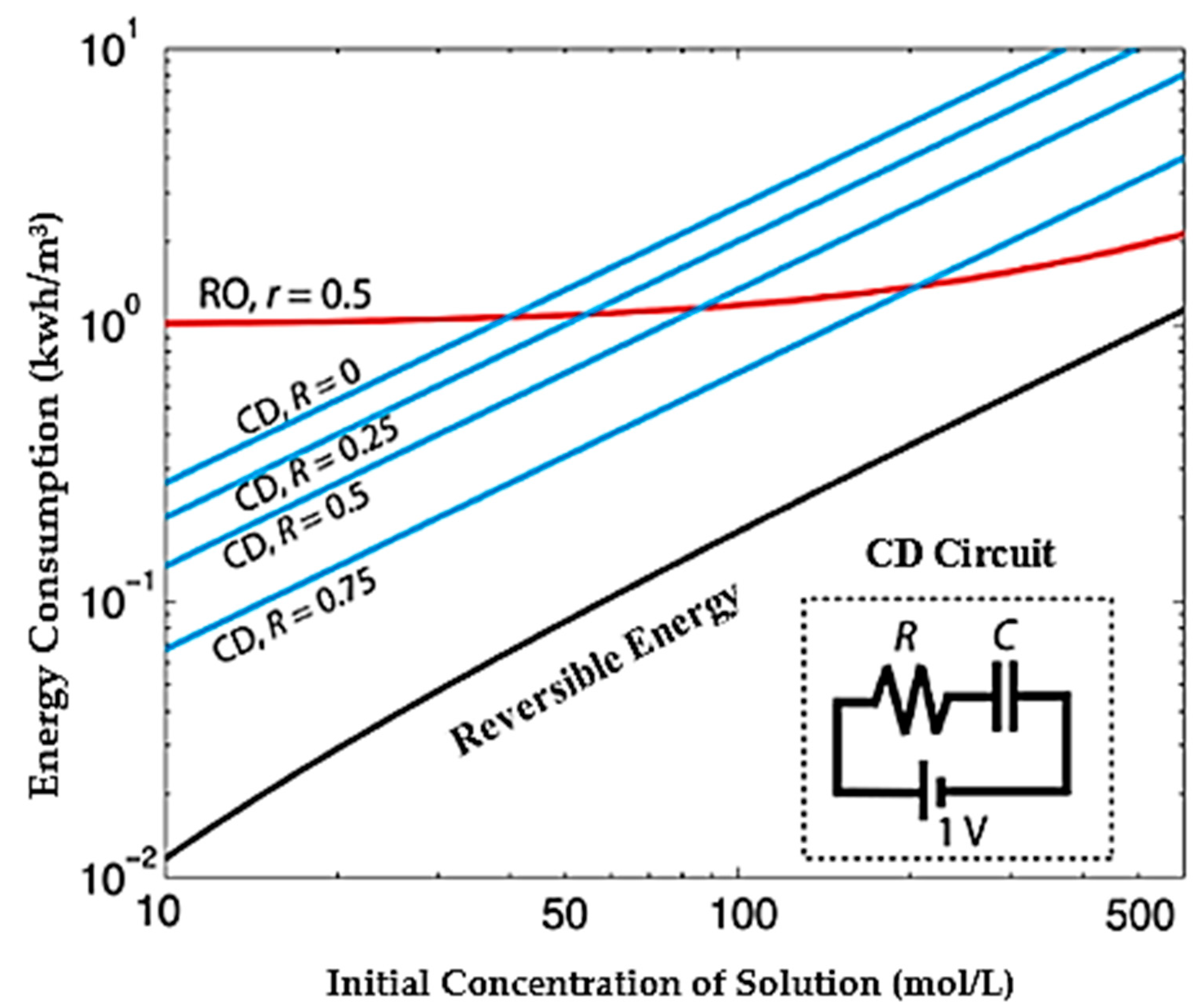
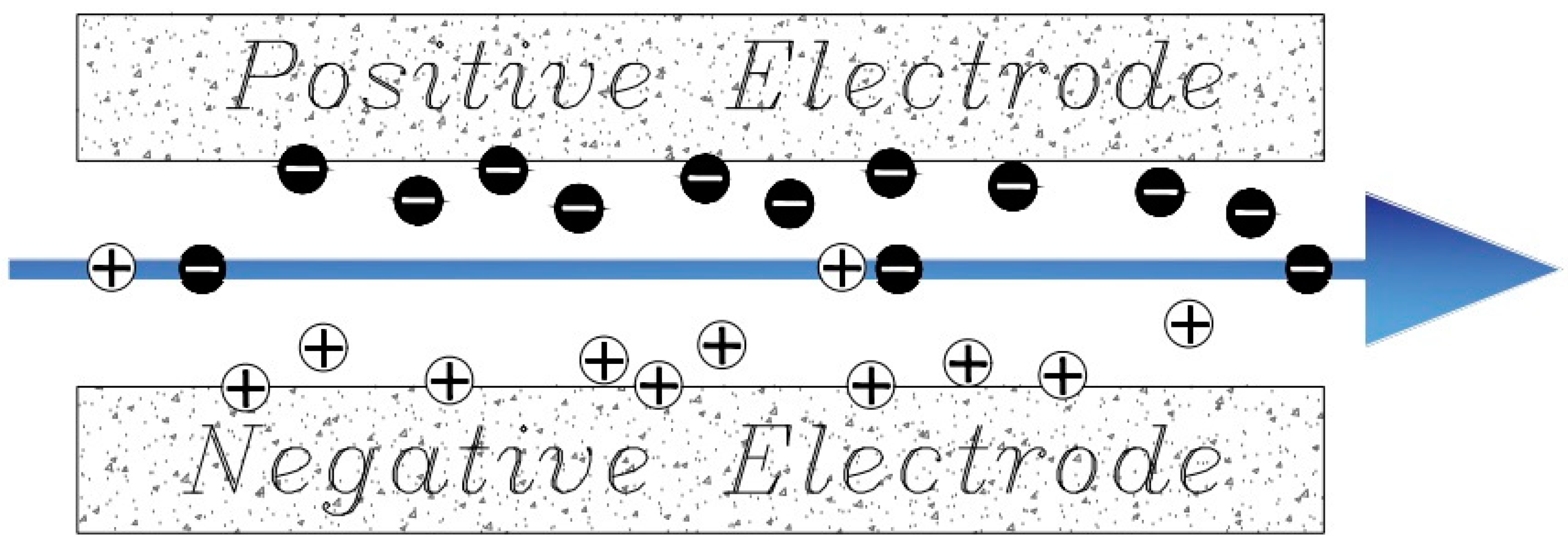

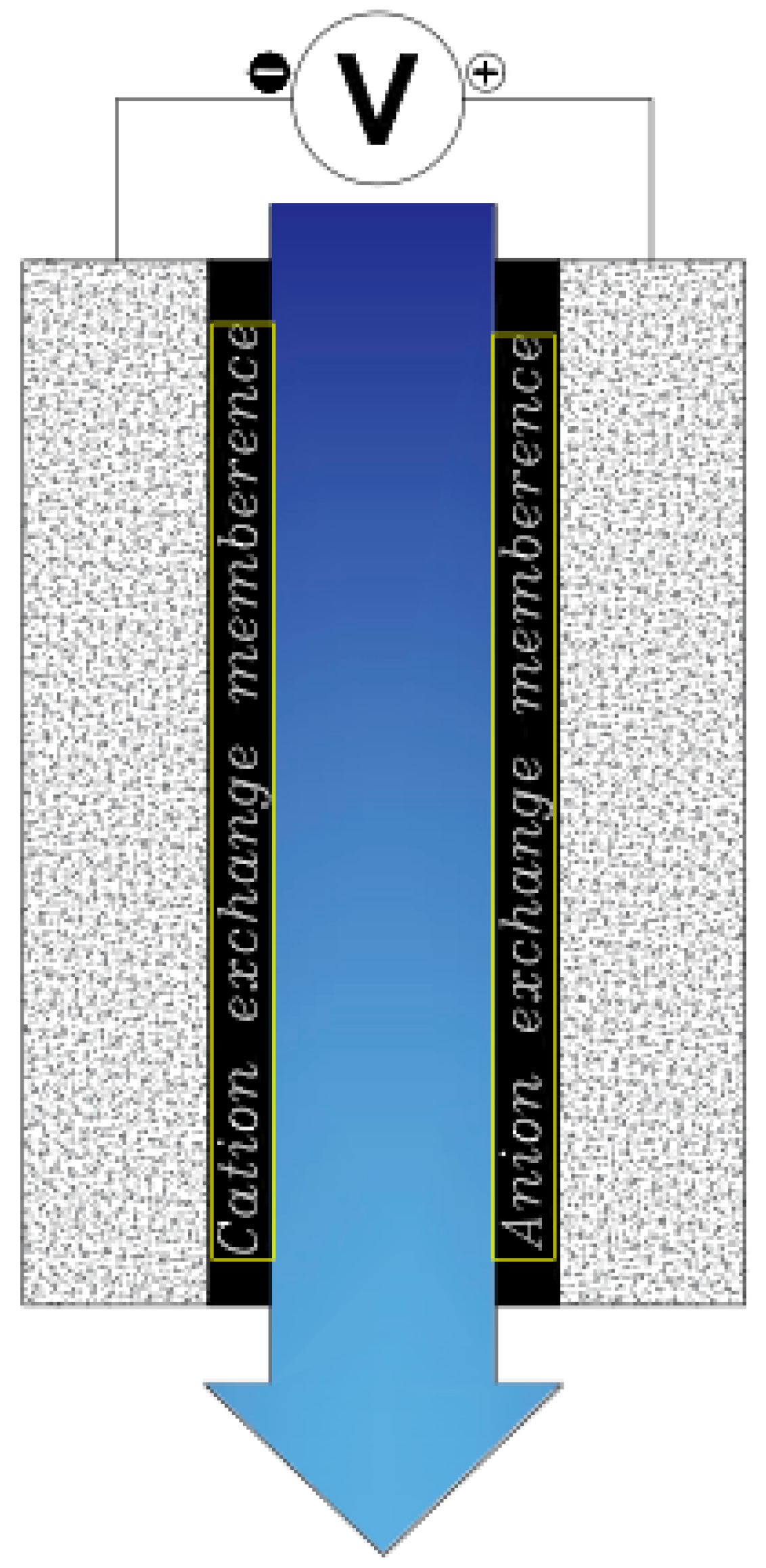
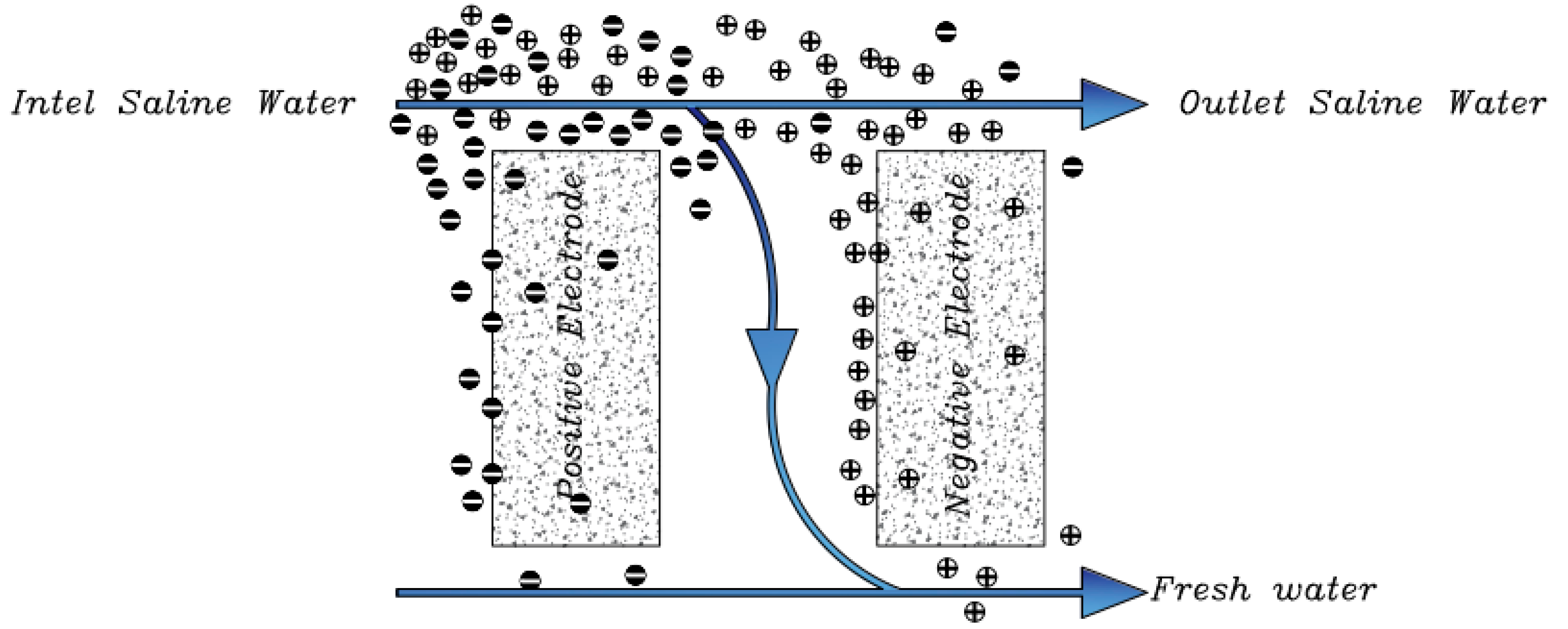
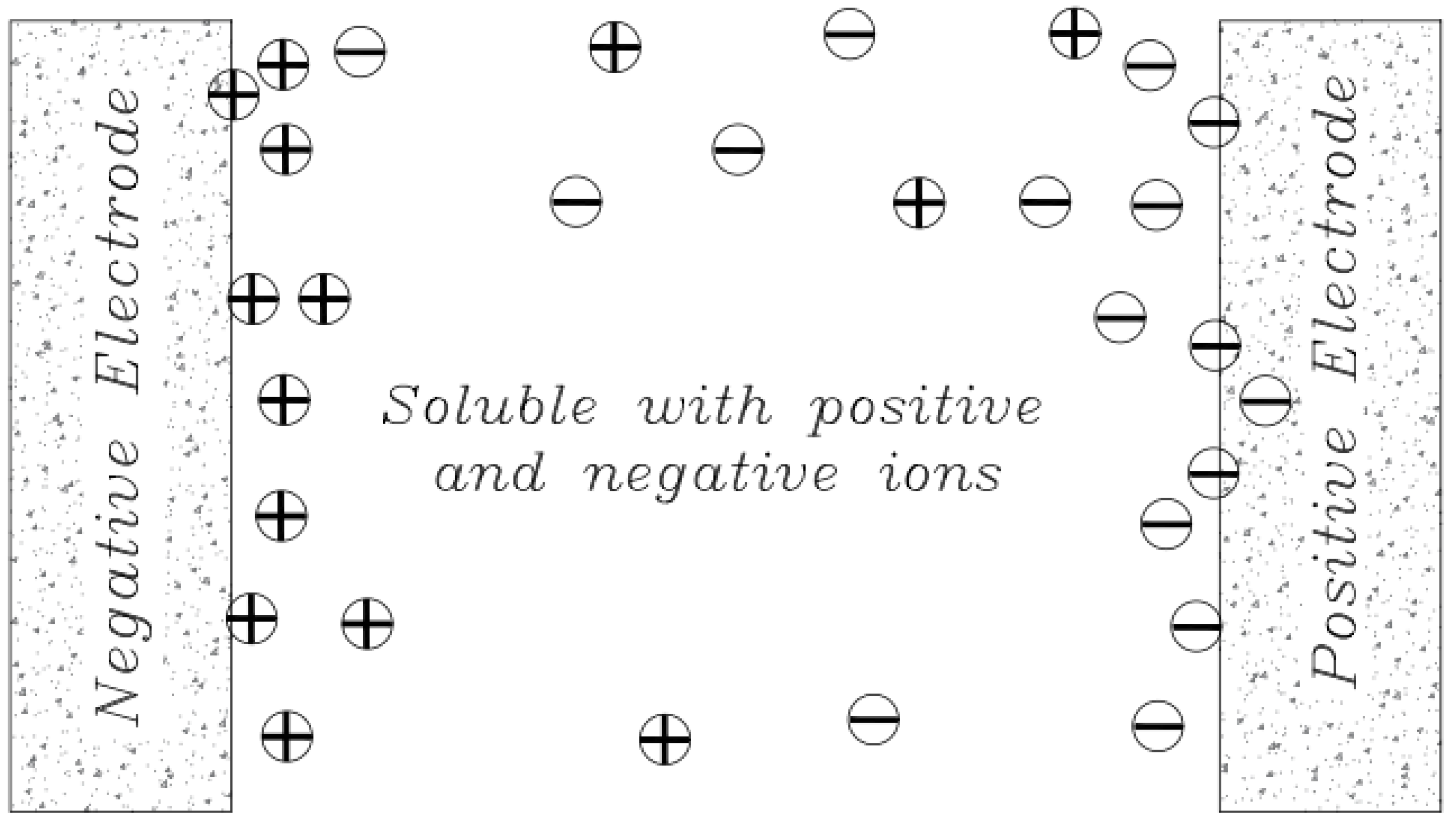




| Num. | Progress Process (Conceptual or Theory) | Year | Ref. |
|---|---|---|---|
| 1 | Electrochemical demineralization | 1960 | [26] |
| 2 | Electrochemistry of carbon | 1961 | [28] |
| 3 | The mechanism of demineralization at carbon electrodes | 1966 | [29] |
| 4 | Mathematical theory of electrochemical demineralization | 1967 | [30] |
| 5 | Activated carbon used in desalination | 1968 | [93] |
| 6 | Electrochemically controlled ion exchange | 1969 | [32] |
| 7 | Electrochemically controlled ion exchange | 1969 | [94] |
| 8 | Electrochemical desalination of brackish water | 1968 | [31] |
| 9 | Electric Double Layer theory (EDL) | 1970 | [35] |
| 10 | Porous carbon electrodes | 1971 | [37] |
| 11 | Four-action electrochemical parametric pumping cycles | 1978 | [39] |
| 12 | Carbon aerogel electrodes | 1995 | [43] |
| 13 | Membrane exchange in front of electrodes (MCDI) | 2006 | [66] |
| 14 | Time-dependent ion selectivity in porous electrodes | 2012 | [67] |
| 15 | Science of water desalination by capacitive deionization | 2013 | [34] |
| 16 | Water desalination via capacitive deionization | 2015 | [78] |
| 17 | Carbon-based composite materials | 2015 | [95] |
| 18 | Resistance in Capacitive Deionization | 2016 | [96] |
| 19 | Redox active porous electrodes in CDI | 2017 | [97] |
| 20 | Faradaic reactions in capacitive deionization | 2018 | [98] |
| 21 | Electrode materials for CDI | 2020 | [99] |
| 22 | CDI and RO desalination | 2020 | [100] |
| 23 | Ion intercalation materials in CDI | 2020 | [101] |
| 24 | Faradic capacitive deionization | 2021 | [102] |
| 25 | Flow-electrode capacitive deionization | 2022 | [103] |
| Type of Operation [Batch or Single Pass] | Initial Concentration of Feed Rate (mg/L) | Flow Rate (mL/min) | Applied Voltage (V) | Salt Adsorption Capacity (mg/g) | Electrode Material | Specific Capacitance/(F/g) | Ref. |
|---|---|---|---|---|---|---|---|
| Single pass | 5844 | Na | 1.2 | 30.2 | Activated carbon with Anion | 200 | [145] |
| Single pass | 584 | Na | 0.7 | 15.6 | Activated carbon | Na | [146] |
| Batch | 3000 | 30 | 1.7 | Na | Carbon nanotubes | 108/1 | [147] |
| Single pass | 4000 | 60 | 1.2 | 30 | NoritSX Ultra Activated Charcoal | Na | [148] |
| Batch | 1020 | 40 | 1.2 | 3 | carbon nanotubes and carbon nanofibers | Na | [79] |
| Batch | 1000 | Na | 1.4 | 66.15 | Activated carbon | 193.694 | [149] |
| Batch | 600 | 10 | 1.2 | 28.62 | Gr phene/CNTs/ZnO | 280 | [150] |
| Batch | 1000 | 50 | 1.2 | 14.91 | Nitrogen-doped porous carbonspheres | 290.74 | [151] |
| Batch | 500 | 25 | 1.2 | 15.31 | Herein, hollow ZIFs-derived nanoporous carbons | 243 | [152] |
| Single pass | 584 | 10 | 1.2 | 31.5 | sodium manganese oxide | 300 | [109] |
| Batch | 50 | 10 | 1.4 | 22.15 | Tungesten Carbide Graphene Nanoflakes | 580 | [153] |
| Batch | 500 | Na | 1.2 | 21.32 | Activated carbon | 246.6 | [154] |
| Single pass | 4000 | 22 | 1.2 | 13 | MXene | 132 | [155] |
| Batch | 600 | 10 | 1.2 | 17.8 | manganese oxide/ fabricated-AC | 388 | [156] |
| Electrode Material | Surface Area of Material (m2/g) | Specific Capacitance (F/g) | Flow Rate/(mL/min) | Applied Voltage (V) | Initial Concentration (mg/L) | Desalination Capacity (mg/g) | Ref. |
|---|---|---|---|---|---|---|---|
| activated carbon | 576 | 60.6 | - | 1.2 | 292 | 11.26 | [180] |
| activated carbon | 24.3 | - | 1.8 | 1000 | 128.6 | [181] | |
| activated carbon | 246.7 | 138.5 | - | 1.8 | 1000 | 167.4 | [182] |
| activated carbon | 1968 | - | - | 1.6 | 50 | 4.6 | [16] |
| activated carbon | - | - | - | 1.2 | 198.5 | 3.5 | [84] |
| activated carbon | - | 1.2 | 292 | 6.9 | [57] | ||
| activated carbon | - | 169.1 | - | 1.2 | 25 | 0.25 | [54] |
| activated carbon | - | - | 25 | 1.2 | 35 | 176.7 | [183] |
| Activated carbon cloth | 2794 | 125 | 1.2 | 292 | 16 | [184] | |
| Highly porous activated carbon | 2254 | 309 | 40 | 1.2 | 500 | 16.3 | [185] |
| Carbon aerogel | 460.34 | 74.23 | - | 1.2 | 400 | 270.59 | [186] |
| Carbon aerogel | - | - | - | 1.2 | 496 | 2.9 | [44] |
| Carbon aerogel | - | - | - | 1.5 | 3000 | 9.6 | [22] |
| Carbon aerogel | 113 | - | - | 1.3 | 2000 | 7 | [53] |
| Carbon aerogel | 910 | 83 | - | 1.2 | 200 | 5.62 | [187] |
| Carbon aerogel | - | - | 25 | 1.2 | 500 | 10.34 | [188] |
| Carbon aerogel- activated carbon composite | 1100 | 90 | - | 1.2 | 1000 | 17 | [189] |
| Aerogel activated CO2 | 1069 | 100.5 | 8 | 1.2 | 6200 | 11.8 | [190] |
| Graphene | - | - | - | 2 | 250 | 8.6 | [191] |
| Graphene | - | - | 40 | 2 | 3000 | 1.85 | [60] |
| Graphene | - | - | 10 | 1.2 | 100 | 29.5 | [192] |
| Graphene | 315.6 | 65 | - | 1.2 | 500 | 29.18 | [193] |
| Carbon nanotubes | 129.368 | - | - | 1.2 | 3000 | 1.734 | [194] |
| graphene/MnO2 | - | 292 | - | 1.2 | 70 | 5.01 | [195] |
| Graphene doped with nitrogen | 358.9 | 253.06 | - | 1.8 | 100 | 4.8 | [196] |
| Carbon nanotubes-graphenehybrid | 435 | - | - | 1.6 | 4000 | 79.4 | [197] |
| Nitrogen-Doped Graphene | 918 | 56.2 | - | 1.4 | 500 | 18.4 | [198] |
| Ordered Mesoporous Carbon | 1491 | 192 | - | 0.8 | 46 | 0.93 | [199] |
| Highly ordered mesoporous carbon nano-polyhedra | 750 | 130 | - | 1 | 116.8 | 14.58 | [200] |
| Graphene-Na4Ti9O20 nanotubes (AC/rGO@NTO) | 142.43 | 120.45 | - | 1.4 | 250 | 41.8 | [201] |
| Graphene nanocomposite | - | - | 20 | 1.2 | 55 | 2.5 | [202] |
| Graphene chitosan-Mn3 O4 (Gr- Cs- Mn3 O4 composite) | - | 190 | - | 1.6 | 300 | 12.7 | [203] |
| Graphene mesoporous carbon | 685.2 | 89.55 | 25 | 2 | 40 | 0.7 | [204] |
| 3D porous graphene | 680 | 70 | - | 1.4 | 300 | 14.32 | [205] |
| Graphene doped with nitrogen | 358.9 | 253.06 | - | 1.8 | 100 | 4.8 | [196] |
| Microporous graphene | 3513 | 20 | - | 2 | 74 | 11.86 | [206] |
| Nanoporous three-Dimensional Graphene | - | 200 | - | 1.6 | 500 | 17.1 | [207] |
| reduced graphene- carbon nanotubes aerogel | - | - | - | 1.6 | 4000 | 79.4 | [197] |
| Resorcinol-based MC-coated graphite | 488 | - | 30 | 1.2 | 5000 | 15.2 | [42] |
| carbon polymer composite | 952 | 168.2 | 30 | 1.2 | 1500 | 14.2 | [208] |
| Sulfonyl- N-doped porouscarbon | 844 | 215.3 | 15 | 1.2 | 40 | 15.5 | [209] |
| Hierarchically porous carbon | 2185.71 | - | 25 | 1.4 | 40 | 34.27 | [210] |
| Carbon nanotubes- Si-Ag | 77.907 | 149.1 | 1 | 0.8 | 210 | 21.5 | [211] |
| hole-rich graphene skeleton | - | 219.9 | 15 | 2 | 572 | 29.6 | [212] |
| three-dimensional channel-structured graphene | 711.9 | 207.4 | - | 2 | 295 | 9.6 | [213] |
| N-hierarchical porous CA | 2405 | 153 | 25 | 1.2 | 500 | 17.9 | [214] |
| nitrogen-doped porous carbon spheres | 1640 | 290.74 | 50 | 1.2 | 1000 | 14.91 | [151] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salari, K.; Zarafshan, P.; Khashehchi, M.; Chegini, G.; Etezadi, H.; Karami, H.; Szulżyk-Cieplak, J.; Łagód, G. Knowledge and Technology Used in Capacitive Deionization of Water. Membranes 2022, 12, 459. https://doi.org/10.3390/membranes12050459
Salari K, Zarafshan P, Khashehchi M, Chegini G, Etezadi H, Karami H, Szulżyk-Cieplak J, Łagód G. Knowledge and Technology Used in Capacitive Deionization of Water. Membranes. 2022; 12(5):459. https://doi.org/10.3390/membranes12050459
Chicago/Turabian StyleSalari, Kamran, Payam Zarafshan, Morteza Khashehchi, Gholamreza Chegini, Hamed Etezadi, Hamed Karami, Joanna Szulżyk-Cieplak, and Grzegorz Łagód. 2022. "Knowledge and Technology Used in Capacitive Deionization of Water" Membranes 12, no. 5: 459. https://doi.org/10.3390/membranes12050459
APA StyleSalari, K., Zarafshan, P., Khashehchi, M., Chegini, G., Etezadi, H., Karami, H., Szulżyk-Cieplak, J., & Łagód, G. (2022). Knowledge and Technology Used in Capacitive Deionization of Water. Membranes, 12(5), 459. https://doi.org/10.3390/membranes12050459










