The Influence of Mechanical Stress Micro Fields around Pores on the Strength of Elongated Etched Membrane
Abstract
1. Introduction
- (1)
- The reduction in the working section of a sample in a real single-axis, elongated cross-section, which is smaller than that of a normal cross-section because of pores.
- (2)
- The concentration of mechanical stresses on the pores, as on each defect of a solid structure, the local mechanical stress is more than a normal structure’s stress.
- (3)
- The interaction of elastic stress fields around closely spaced pores.
2. Materials and Methods
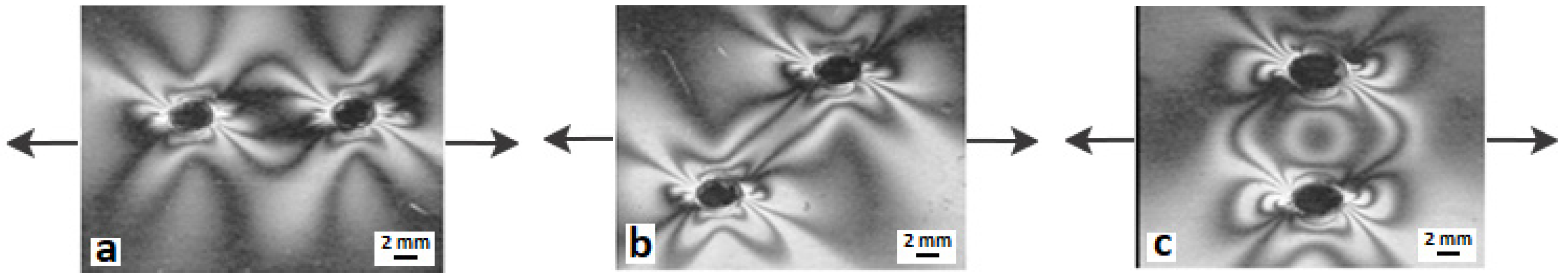
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Apel, P.Y.; Ivanov, O.M.; Lizunov, N.E.; Mamonova, T.I.; Nechaev, A.N.; Olejniczak, K.; Vacik, J.; Dmitriev, S.N. Production of Multi-, Oligo- and Single-Pore Membranes Using a Continuous Ion Beam. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2015, 365, 641–645. [Google Scholar] [CrossRef]
- Kravets, L.I.; Dmitriev, S.N.; Apel, P.Y. Production and Properties of Polypropylene Track Membranes. Collect. Czechoslov. Chem. Commun. 1997, 62, 752–760. [Google Scholar] [CrossRef]
- Worrel, L.; Morehouse, J.; Shimko, L.; Lloyd, D.; Lawler, D.; Freeman, B. Enhancement of Track-Etched Membrane Performance Via Stretching. Sep. Purif. Technol. 2007, 53, 71–80. [Google Scholar] [CrossRef]
- Hanks, P.L.; Kaczorowski, K.J.; Becker, E.B.; Lloyd, D.R. Modeling of Uni-Axial Stretching of Track-Etch Membranes. J. Membr. Sci. 2007, 305, 196–202. [Google Scholar] [CrossRef]
- Gumirova, V.N.; Abdurashidova, G.S.; Bedin, S.A.; Zabalueva, N.P.; Kuvaitseva, M.A.; Razumovskaya, I.V. Specific Features of the Fracture of Track Membranes and Related Polymer/Metal Composites Prepared by Template Synthesis. Phys. Solid State 2015, 57, 344–348. [Google Scholar] [CrossRef]
- Gumirova, V.N.; Bedin, S.A.; Abdurashidova, G.S.; Razumovskaya, I.V. The Influence of Pores in Track Etched Membranes and Prepared on Their Base Polymer/Metal Composites on Their Fracture Strength. Phys. Procedia 2015, 80, 148–150. [Google Scholar] [CrossRef][Green Version]
- Cristofari, F.; Piotrowski, B.; Pesci, R. Mechanical Properties of a Nanoporous Membrane Used in Implantable Medical Devices. Correlation between Experimental Characterization and 2d Numerical Simulation. J. Mech. Behav. Biomed. Mater. 2017, 74, 43–53. [Google Scholar] [CrossRef]
- Guo, L.; Wang, L.; Wang, Y. Stretched Homoporous Composite Membranes with Elliptic Nanopores for External-Energy-Free Ultrafiltration. Chem. Commun. 2016, 52, 6899–6902. [Google Scholar] [CrossRef] [PubMed]
- Shen, J.-W.; Matsumoto, H.; Maki, A.; Kuriyama, T.; Nemoto, T.; Koido, S.; Takeuchi, H. A Study on the Relationship between Microstructure and Mechanical Properties of Porous Polymer Films. Polymer 2020, 204, 122784. [Google Scholar] [CrossRef]
- Buczkowski, M.; Sartowska, B.; Wawszczak, D.; Starosta, W. Radiation Resistance of Track Etched Membranes. Radiat. Meas. 2001, 34, 597–599. [Google Scholar] [CrossRef]
- Apel, P. Track Etching Technique in Membrane Technology. Radiat. Meas. 2001, 34, 559–566. [Google Scholar] [CrossRef]
- Apel, P.Y.; Bobreshova, O.V.; Volkov, A.V.; Volkov, V.V.; Nikonenko, V.V.; Stenina, I.A.; Filippov, A.N.; Yampolskii, Y.P.; Yaroslavtsev, A.B. Prospects of Membrane Science Development. Membr. Membr. Technol. 2019, 1, 45–63. [Google Scholar] [CrossRef]
- Ma, T.; Jean-Marc, J.; Sebastien, B. Track-Etched Nanopore/Membrane: From Fundamental to Applica-tions. Small Methods 2020, 4, 2000366. [Google Scholar] [CrossRef]
- Wang, N.; Zhai, Y.; Yang, Y.; Yang, X.; Zhu, Z. Electrostatic assembly of superwetting porous nanofibrous membrane toward oil-in-water microemulsion separation. Chem. Eng. J. 2018, 354, 463–472. [Google Scholar] [CrossRef]
- Martin, C.R. Nanomaterials: A Membrane-Based Synthetic Approach. Science 1994, 266, 1961–1966. [Google Scholar] [CrossRef] [PubMed]
- Martin, C.R. Membrane-Based Synthesis of Nanomaterials. Chem. Mater. 1996, 8, 1739–1746. [Google Scholar] [CrossRef]
- Schönenberger, C.; van der Zande, B.M.I.; Fokkink, L.G.J.; Henny, M.; Schmid, C.; Krüger, M.; Bachtold, A.; Huber, R.; Birk, H.; Staufer, U. Template Synthesis of Nanowires in Porous Polycarbonate Membranes: Electrochemistry and Morphology. J. Phys. Chem. B 1997, 101, 5497–5505. [Google Scholar] [CrossRef]
- Pérez-Page, M.; Yu, E.; Li, J.; Rahman, M.; Dryden, D.M.; Vidu, R.; Stroeve, P. Template-based syntheses for shape controlled nanostructures. Adv. Colloid Interface Sci. 2016, 234, 51–79. [Google Scholar] [CrossRef] [PubMed]
- Mitrofanov, A.V.; Feshchenko, R.M. Angular Characteristics of Soft X-ray Transmission by Track Membranes with Large Pores. Bull. Lebedev Phys. Inst. 2022, 49, 169–173. [Google Scholar] [CrossRef]
- Bedin, S.A.; Rybalko, O.G.; Polyakov, N.B.; Zagorskii, D.L.; Razumovskaya, A.V.; Bondarenko, G.G.; Oleinikov, V.A. Metal Micro- and Nanowires Fabricated by Matrix Synthesis and Their Application in Mass Spectrometry. Inorg. Mater. Appl. Res. 2010, 1, 359–364. [Google Scholar] [CrossRef]
- Vilensky, A.I.; Apel, P.; Mchedlishvili, B.V. Dye Adsorption on Tracks and Pores of Track Membranes. Bull. Russ. Acad. Sci. Phys. 2006, 70, 1039–1042. [Google Scholar]
- Kulik, S.I.; Eremchev, I.Y.; Apel, P.Y.; Zagorski, D.L.; Naumov, A.V. Fluorescence Imaging for Ultrafiltration of Individual Nanoparticles from a Colloidal Solution in Track Membranes. J. Appl. Spectrosc. 2018, 85, 916–922. [Google Scholar] [CrossRef]
- Kozhina, E.P.; Andreev, S.N.; Tarakanov, V.P.; Bedin, S.A.; Doludenko, I.M.; Naumov, A.V. Study of Local Fields of Dendrite Nanostructures in Hot Spots Formed on Sers-Active Substrates Produced Via Template-Assisted Synthesis. Bull. Russ. Acad. Sci. Phys. 2021, 84, 1465–1468. [Google Scholar] [CrossRef]
- Kovalets, N.P.; Kozhina, E.P.; Razumovskaya, I.V.; Bedin, S.A.; Piryazev, A.A.; Grigoriev, Y.V.; Naumov, A.V. Toward Single-Molecule Surface-Enhanced Raman Scattering with Novel Type of Metasurfaces Synthesized by Crack-Stretching of Metallized Track-Etched Membranes. J. Chem. Phys. 2022, 156, 034902. [Google Scholar] [CrossRef]
- Kozhina, E.P.; Bedin, S.A.; Nechaeva, N.L.; Podoynitsyn, S.N.; Tarakanov, V.P.; Andreev, S.N.; Grigoriev, Y.V.; Naumov, A.V. Ag-Nanowire Bundles with Gap Hot Spots Synthesized in Track-Etched Membranes as Effective Sers-Substrates. Appl. Sci. 2021, 11, 1375. [Google Scholar] [CrossRef]
- Kozhina, E.; Kulesh, E.; Bedin, S.; Doludenko, I.; Piryazev, A.; Korolkov, I.; Kozlovskiy, A.; Zdorovets, M.; Rogachev, A.; Shumskaya, A. One-Dimensional Magneto-Optical Nanostructures: Template Synthesis, Structure, Properties, and Application in Spectroscopy Based on Plasmon Resonance. IEEE Magn. Lett. 2022, 13, 1–5. [Google Scholar] [CrossRef]
- Kuznetsov, E.V.; Lobanov, P.Y.; Manuylovich, I.S.; Meshkov, M.N.; Sidoryuk, O.E.; Skvortsov, L.A.; Stelmakh, M.F. Non-Destructive Control of Plastic Products by Means of Active Thermography with Pulse Laser Heating. Photonics 2014, 15, 428–442. [Google Scholar] [CrossRef]
- Kaan, K.; Dila, K.; Charles, M. Resistive-pulse Sensing of DNA with Polymeric Nanopore Sensor and Characterization of DNA Translocation. ChemNanoMat 2021, 8, e202100424. [Google Scholar]
- Park, S.E.; Shin, K.; Yang, J.H.; Park, B.K.; Kim, S.Y.; Kim, H.; Park, M.; Kim, K.J. Track-etched polyimide separator decorated with polyvinylpyrrolidone for self-assembling a robust protective layer on lithium-metal anode. Chem. Eng. J. 2022, 445, 136801. [Google Scholar] [CrossRef]
- Kuznetsov, N.V.; Bedin, S.A. Method for the Certification of Track Membranes Using Computer Vision. J. Surf. Investig. X-ray Synchrotron Neutron Tech. 2022, 16, 514–519. [Google Scholar] [CrossRef]
- Mokryakov, V.V. Application of the Multipole Method to the Problem on Two Close Holes. Mech. Solids 2007, 42, 771–785. [Google Scholar] [CrossRef]
- Altman, N.S. An Introduction to Kernel and Nearest-Neighbor Nonparametric Regression. Am. Stat. 1992, 46, 175–185. [Google Scholar]
- Barashenkov, V.S. Dispersion of Pores in Nuclear Filters. In JINR Communication 1-15; Joint Inst. for Nuclear Research: Dubna, Russia, 1977. [Google Scholar]
- Zienkiewicz, O.C.; Taylor, R.L. Finite Element Method, 5th ed.; Butterworth-Heinemann: Saddle River, NJ, USA, 2000. [Google Scholar]
- Marreddy, A.; Gerasimov, T.; De Lorenzis, L. A Review on Phase-Field Models of Brittle Fracture and a New Fast Hybrid Formulation. Comput. Mech. 2014, 55, 383–405. [Google Scholar]

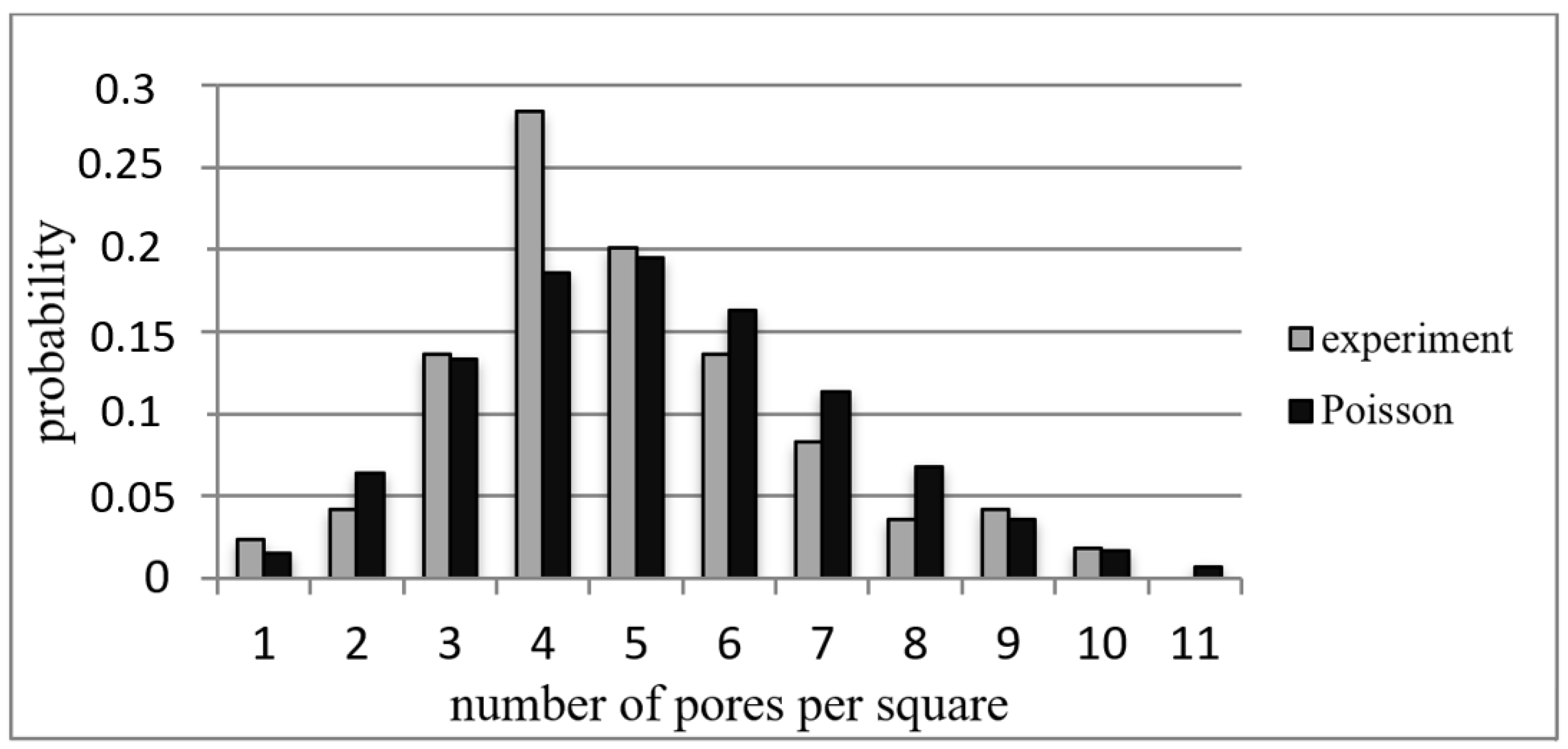
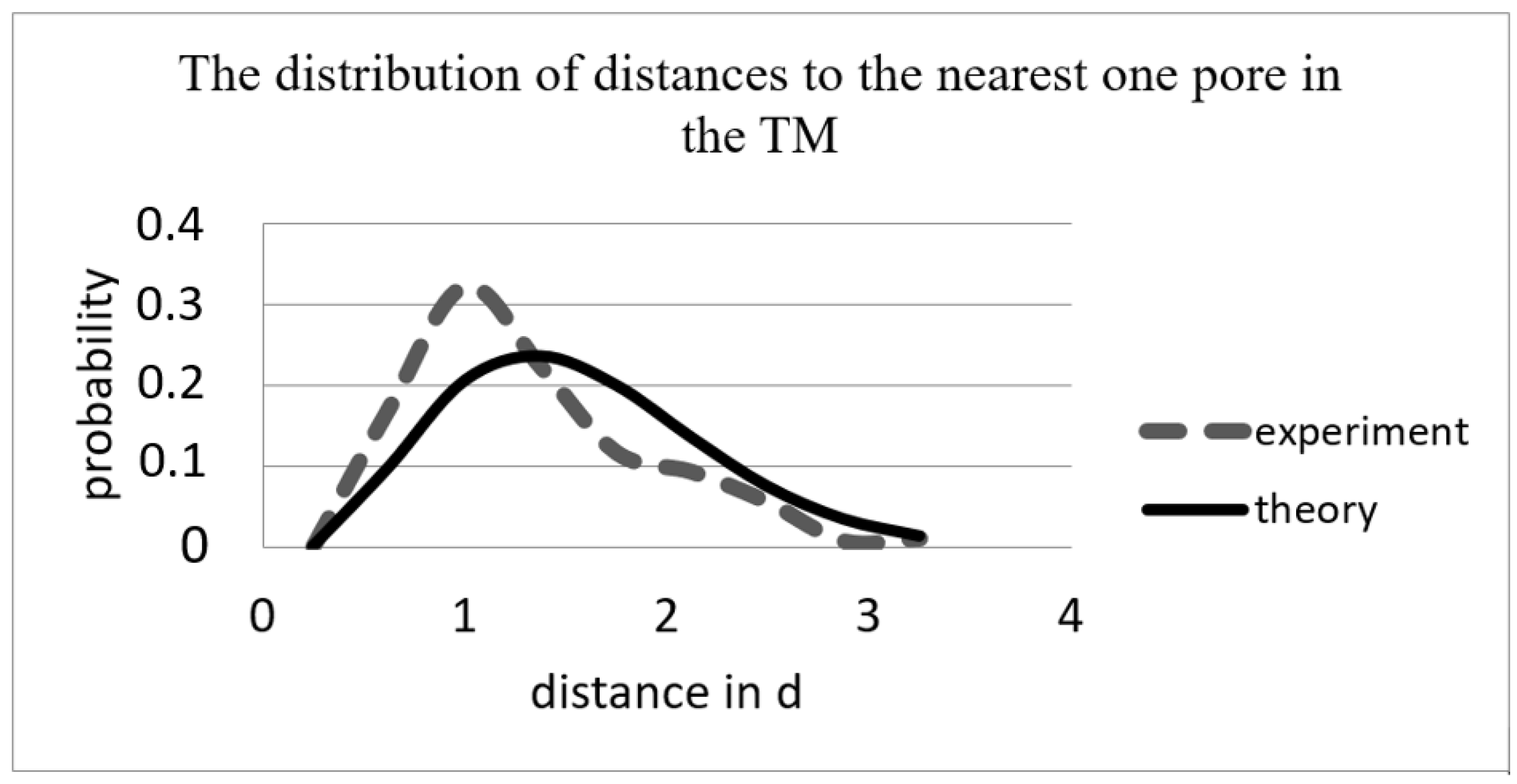
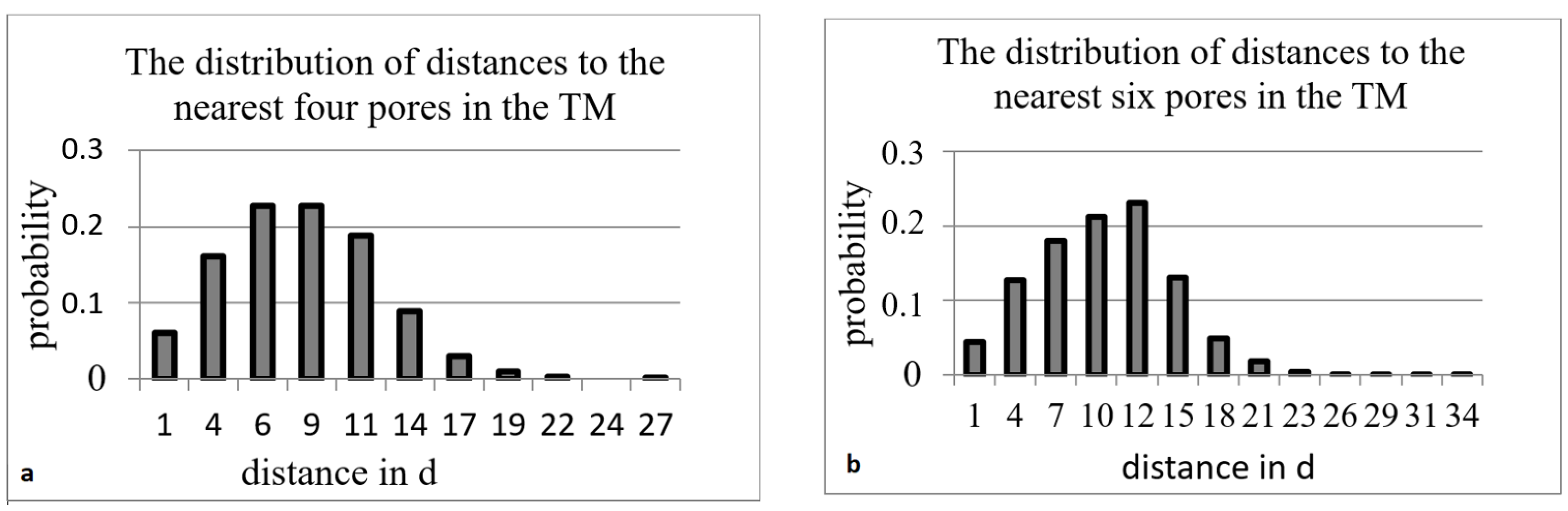

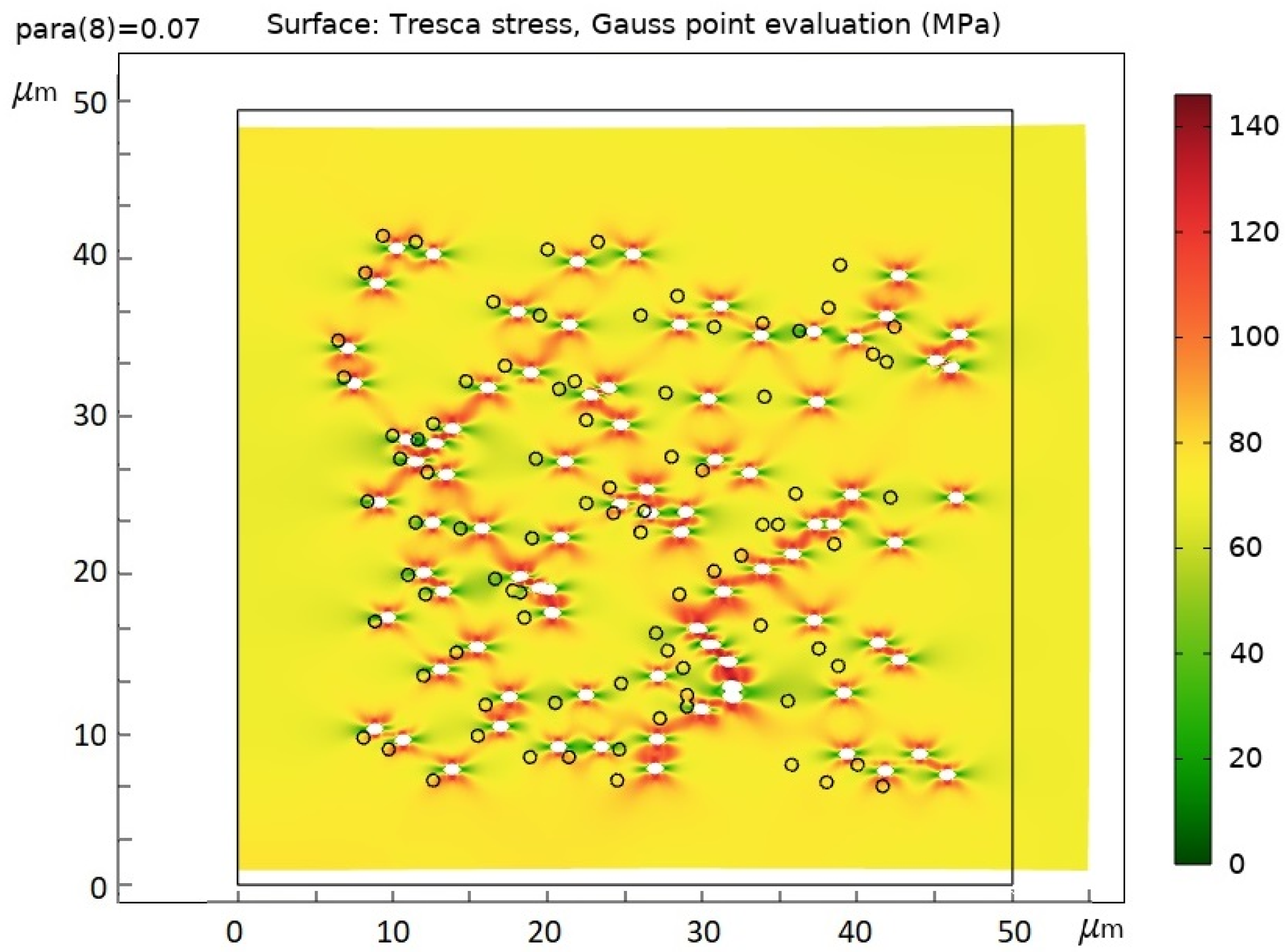

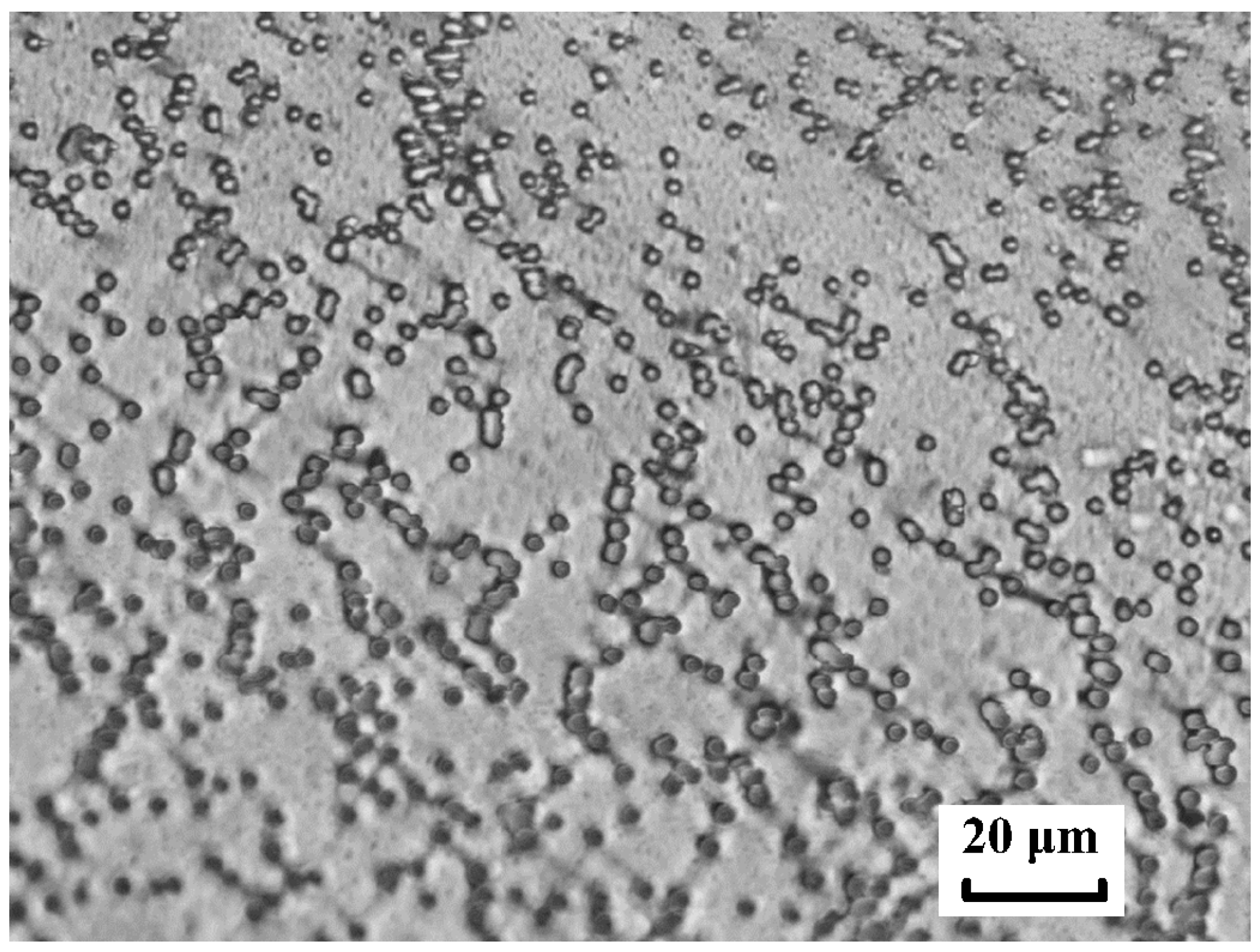
| Sample № | Etching Time, Min | Pore Diameter, μm | Film Thickness, μm |
|---|---|---|---|
| 1 | 30 | 0.22 | 11.4 ± 1.1 |
| 2 | 50 | 0.54 | 11.0 ± 1.1 |
| 3 | 70 | 0.84 | 10.7 ± 1.1 |
| 4 | 90 | 1.16 | 10.4 ± 1.0 |
| 5 | 120 | 1.60 | 10.0 ± 0.9 |
| 6 * | 0 | - | 12.0 ± 1.1 |
| Radiation Density, n, cm−2 | Pore Diameter, μm | Film Thickness, μm |
|---|---|---|
| 1.18 × 107 | 0.067 | 11.7 ± 0.2 |
| 1.18 × 107 | 0.105 | 11.7 ± 0.1 |
| 1.18 × 107 | 0.302 | 11.6 ± 0.2 |
| 1.18 × 107 | 0.487 | 11.4 ± 0.2 |
| 1.18 × 107 | 0.674 | 11.2 ± 0.2 |
| 1.18 × 107 | 1.221 | 10.8 ± 0.2 |
| 4.41 × 107 | 0.060 | 11.9 ± 0.2 |
| 4.41 × 107 | 0.158 | 11.9 ± 0.2 |
| 4.41 × 107 | 0.301 | 11.8 ± 0.2 |
| 4.41 × 107 | 0.772 | 11.2 ± 0.1 |
| 4.41 × 107 | 0.951 | 11.0 ± 0.2 |
| 106 | 4.0 | 10 ± 0.9 |
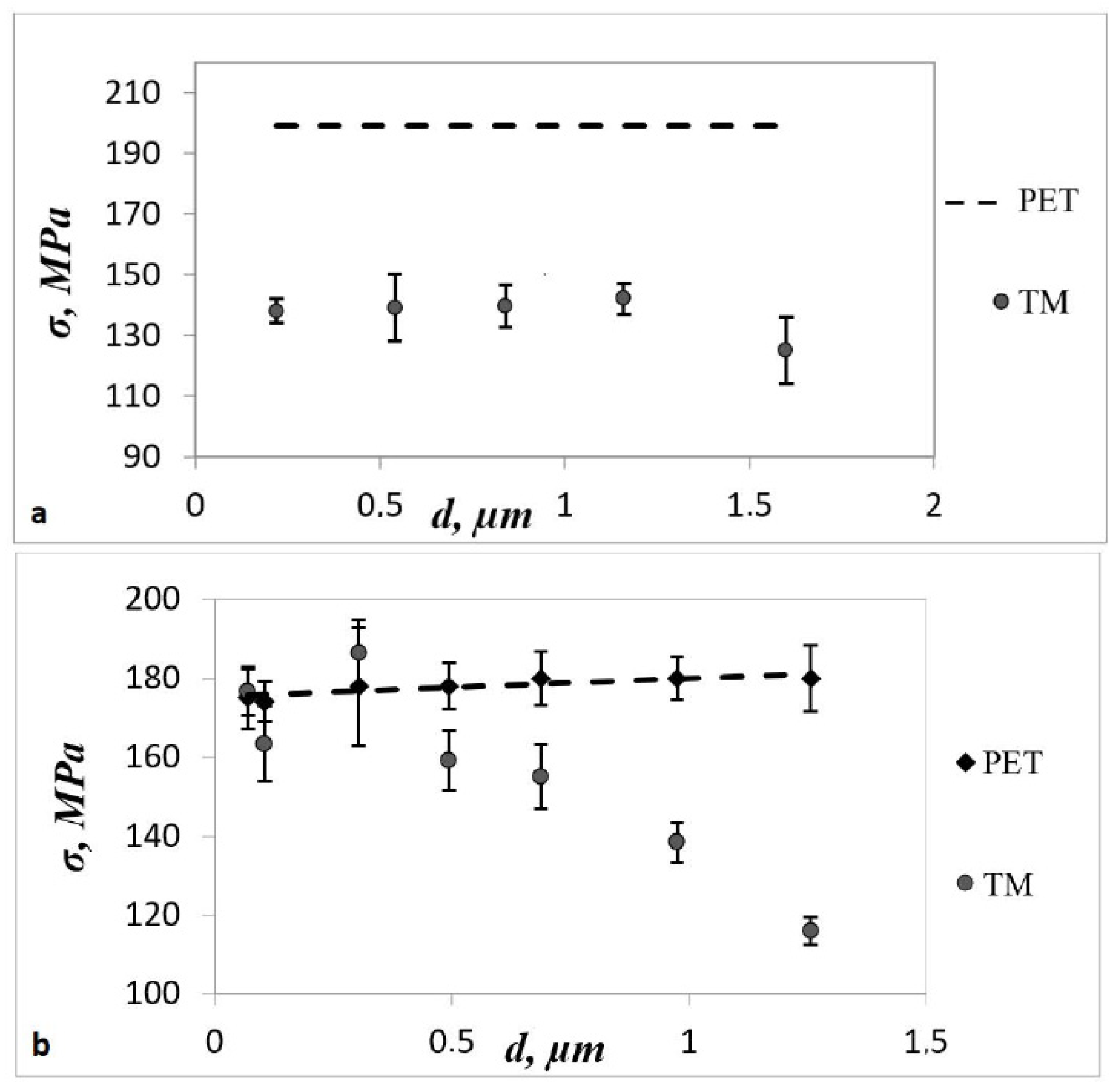
| Sample № | d, μm | Tensile Strength with Consideration of Reduced Working Section, σ, MPa | Conditional Yield Point with Consideration of Reduced Working Section, σT, MPa | Breaking Deformation ε, % | β |
|---|---|---|---|---|---|
| 1 | 0.22 | 138 ± 4 | 106 ± 3 | 19 ± 4 | 1.44 |
| 2 | 0.54 | 139 ± 11 | 111 ± 8 | 13 ± 1 | 1.43 |
| 3 | 0.84 | 140 ± 7 | 113 ± 6 | 11 ± 2 | 1.42 |
| 4 | 1.16 | 142 ± 5 | 114 ± 4 | 9 ± 2 | 1.40 |
| 5 | 1.60 | 125 ± 11 | 102 ± 7 | 5 ± 0.7 | 1.60 |
| 6 | 0 | 199 ± 9 | 106 ± 11 | 42 ± 2 | 1 |
| Sample № | d, μm | (For Regular Network) | (For Poisson Distribution) | (Calc. by SEM Images up to One Nearest Pore) | (Calc. by SEM Images up to 4 Nearest Pores) | (Calc. by SEM Images up to 6 Nearest Pores) |
|---|---|---|---|---|---|---|
| 1 | 0.22 | 23 | 11.5 ± 3.4 | 16.9 ± 9.7 | 19.8 ± 9.2 | 23.9 ± 10.8 |
| 2 | 0.54 | 9 | 4.5 ± 2.0 | 6.9 ± 3.9 | 8.1 ± 3.7 | 9.7 ± 4.4 |
| 3 | 0.84 | 6 | 3 ± 1.7 | 4.5 ± 2.5 | 5.2 ± 2.4 | 6.2 ± 2.8 |
| 4 | 1.16 | 4 | 2 ± 1.4 | 3.2 ± 1.8 | 3.8 ± 1.7 | 4.5 ± 2.0 |
| 5 | 1.60 | 3 | 1.5 ± 1.2 | 2.3 ± 1.3 | 2.7 ± 1.2 | 3.3 ± 1.5 |
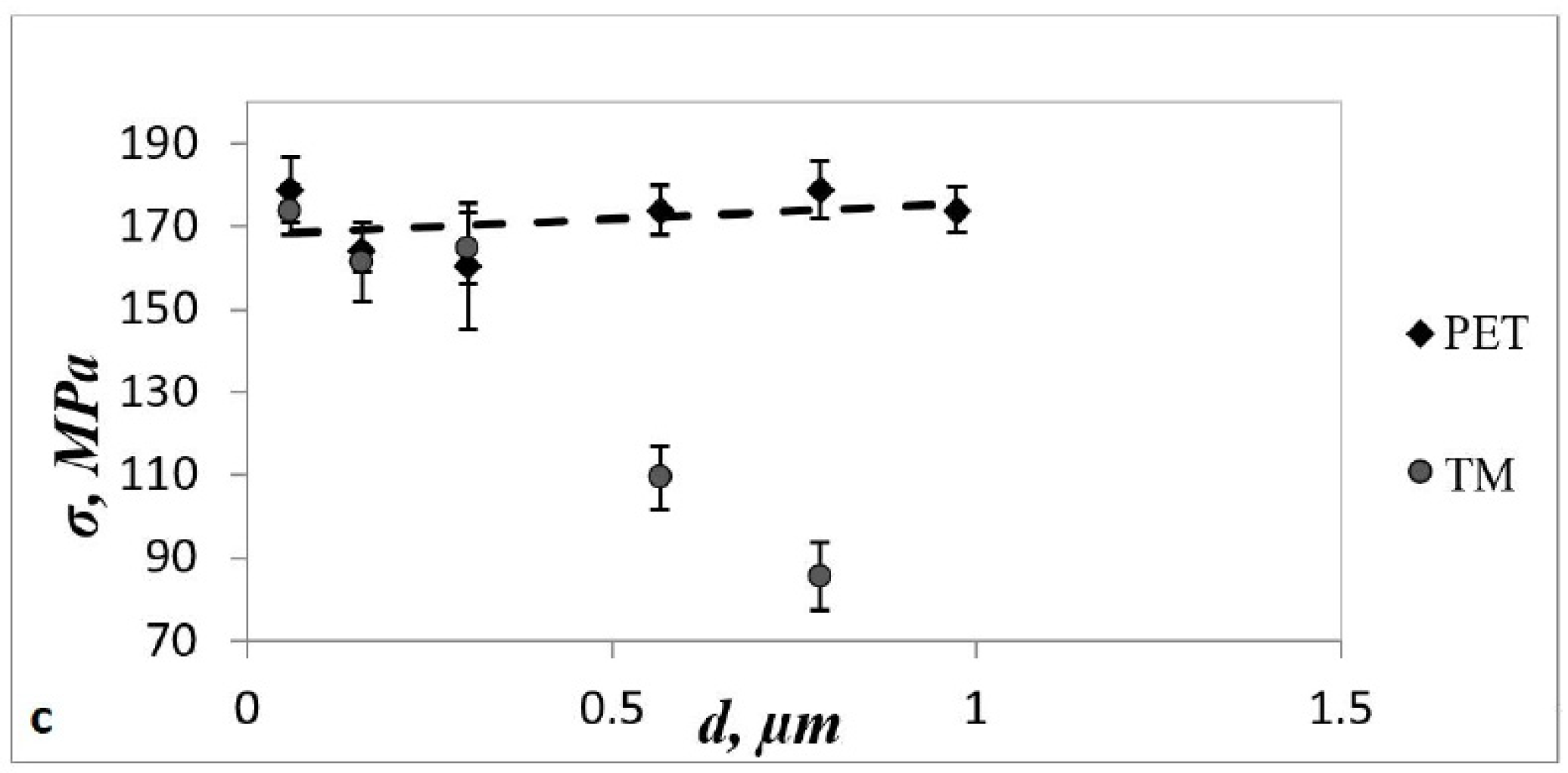
| Number of Holes | The Angle between Holes Relative to the Direction of Tension | Strength, σ, MPa | β |
|---|---|---|---|
| 0 | - | 190 ± 9 | 1 |
| 1 | - | 161 ± 8 | 1.2 |
| 2 | 0° | 128 ± 9 | 1.5 |
| 2 | 45° | 112 ± 6 | 1.7 |
| 2 | 90° | 118 ± 5 | 1.6 |
| d, μm | (calc. by SEM Images up to One Nearest Pore) | Fractal Dimension for TM | Fractal Dimension for a Model with Pores Distributed by Poisson’s Law | Fractal Dimension for a Model with Pores Distributed by Poisson’s Law |
|---|---|---|---|---|
| 0.22 | 16.9 ± 9.7 | 1.10 | 1–1.10 | 1.10 |
| 0.54 | 6.9 ± 3.9 | 1.25 | 1.30 | 1.27 |
| 0.84 | 4.5 ± 2.5 | 1.30 | 1.35 | 1.30 |
| 1.16 | 3.2 ± 1.8 | 1.40 | 1.40 | 1.34 |
| 1.60 | 2.3 ± 1.3 | 1.55 | 1.50 | 1.50 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gumirova, V.; Razumovskaya, I.; Apel, P.; Bedin, S.; Naumov, A. The Influence of Mechanical Stress Micro Fields around Pores on the Strength of Elongated Etched Membrane. Membranes 2022, 12, 1168. https://doi.org/10.3390/membranes12111168
Gumirova V, Razumovskaya I, Apel P, Bedin S, Naumov A. The Influence of Mechanical Stress Micro Fields around Pores on the Strength of Elongated Etched Membrane. Membranes. 2022; 12(11):1168. https://doi.org/10.3390/membranes12111168
Chicago/Turabian StyleGumirova, Venera, Irina Razumovskaya, Pavel Apel, Sergey Bedin, and Andrey Naumov. 2022. "The Influence of Mechanical Stress Micro Fields around Pores on the Strength of Elongated Etched Membrane" Membranes 12, no. 11: 1168. https://doi.org/10.3390/membranes12111168
APA StyleGumirova, V., Razumovskaya, I., Apel, P., Bedin, S., & Naumov, A. (2022). The Influence of Mechanical Stress Micro Fields around Pores on the Strength of Elongated Etched Membrane. Membranes, 12(11), 1168. https://doi.org/10.3390/membranes12111168






