Gradient NMR Method for Studies of Water Translational Diffusion in Plants
Abstract
:1. Introduction
2. Prerequisites for Applying Spin-Echo NMR Method to Studies of Water Condition and Transport in Plants
2.1. The Prehistory of the Problem of the Condition of Water in the Cells
2.2. The Main Structures That Determine the Transport of Water in Plant Tissues
3. The Development of Gradient NMR from the Constant Gradient Method to Pulse Method
3.1. Constant Gradient Method
3.2. Pulse Gradient Method
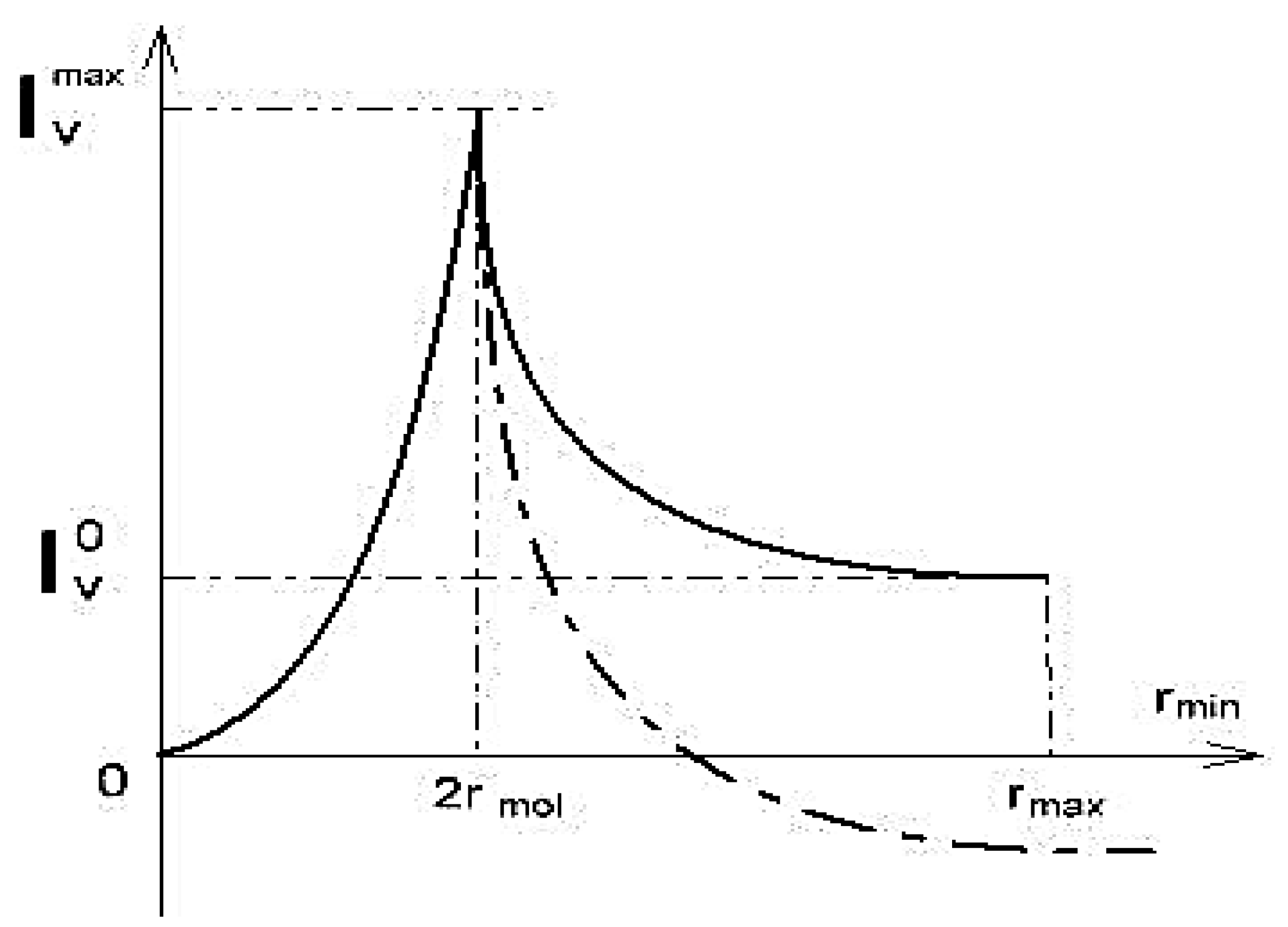
4. Restricted Diffusion
5. Stages of Development of Gradient NMR Technique
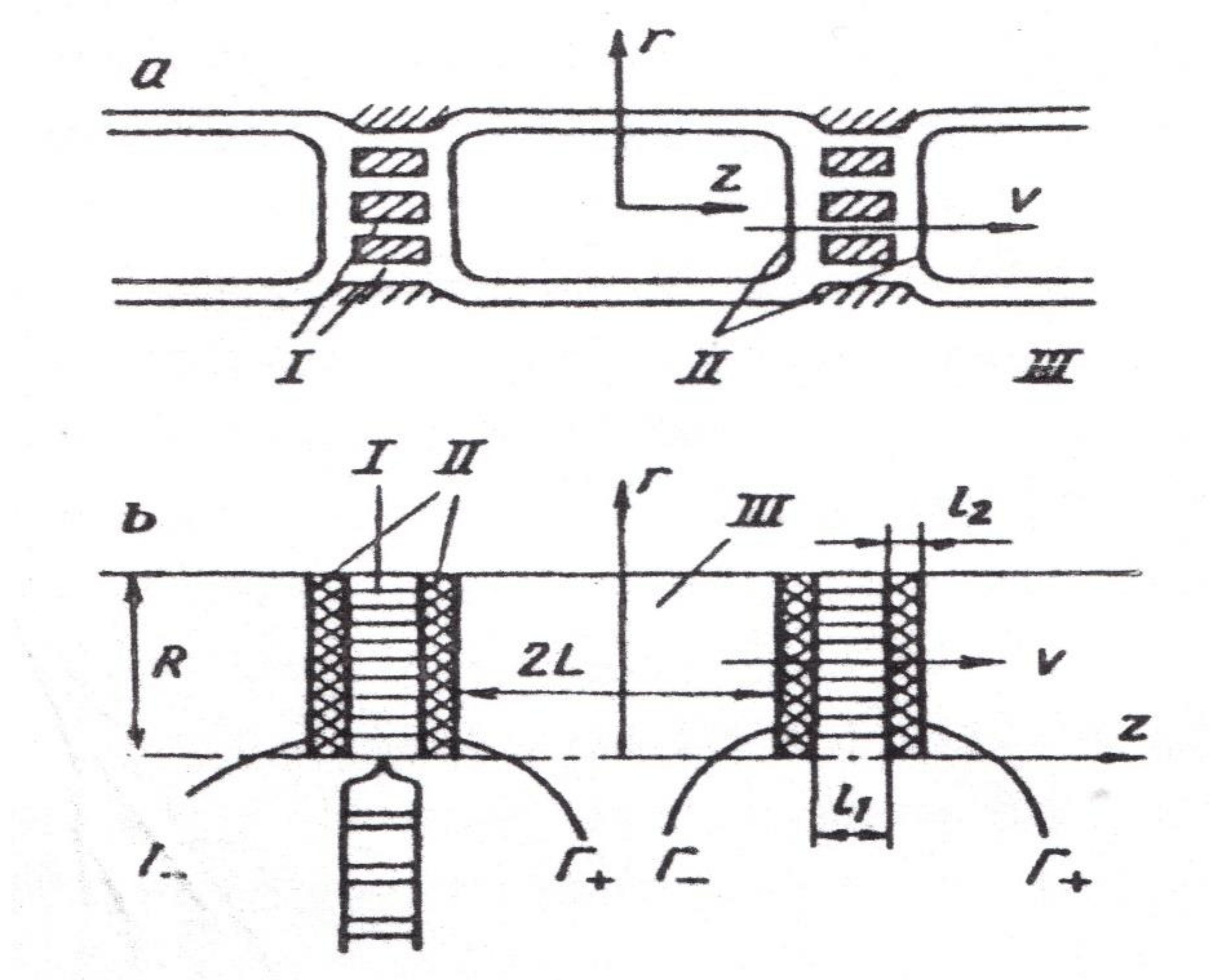
6. Methodological Approaches to Selective Measurements of Diffusion
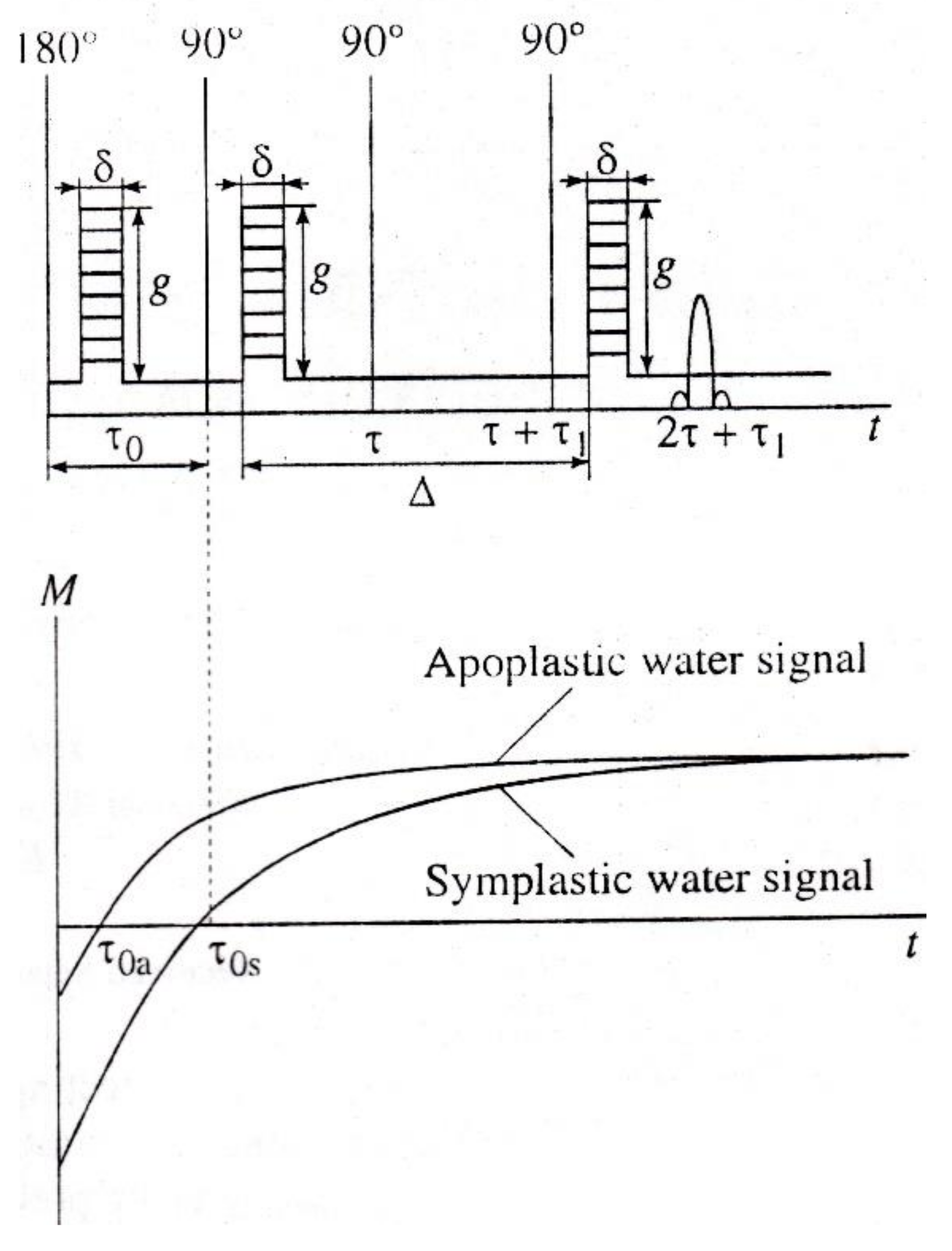
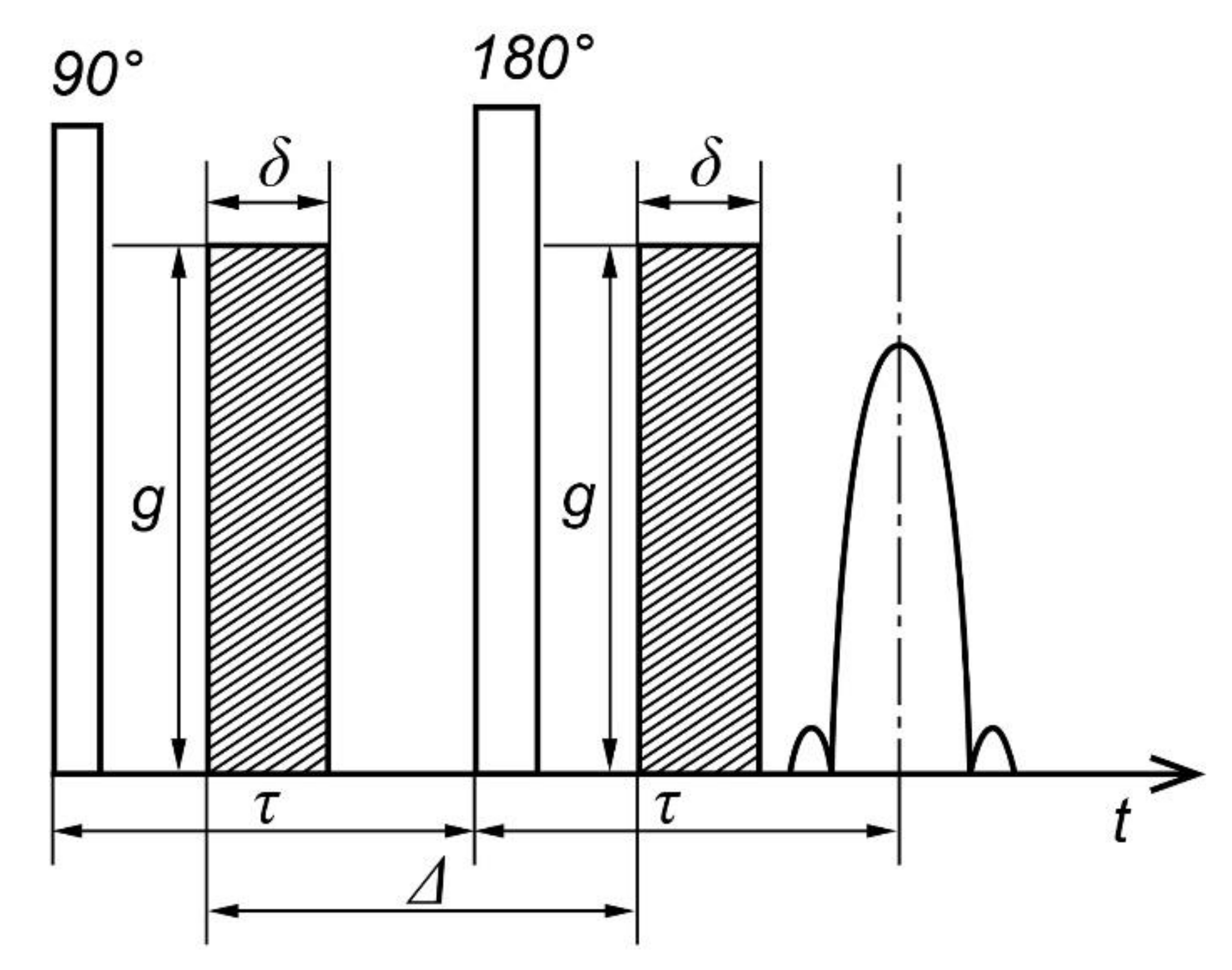
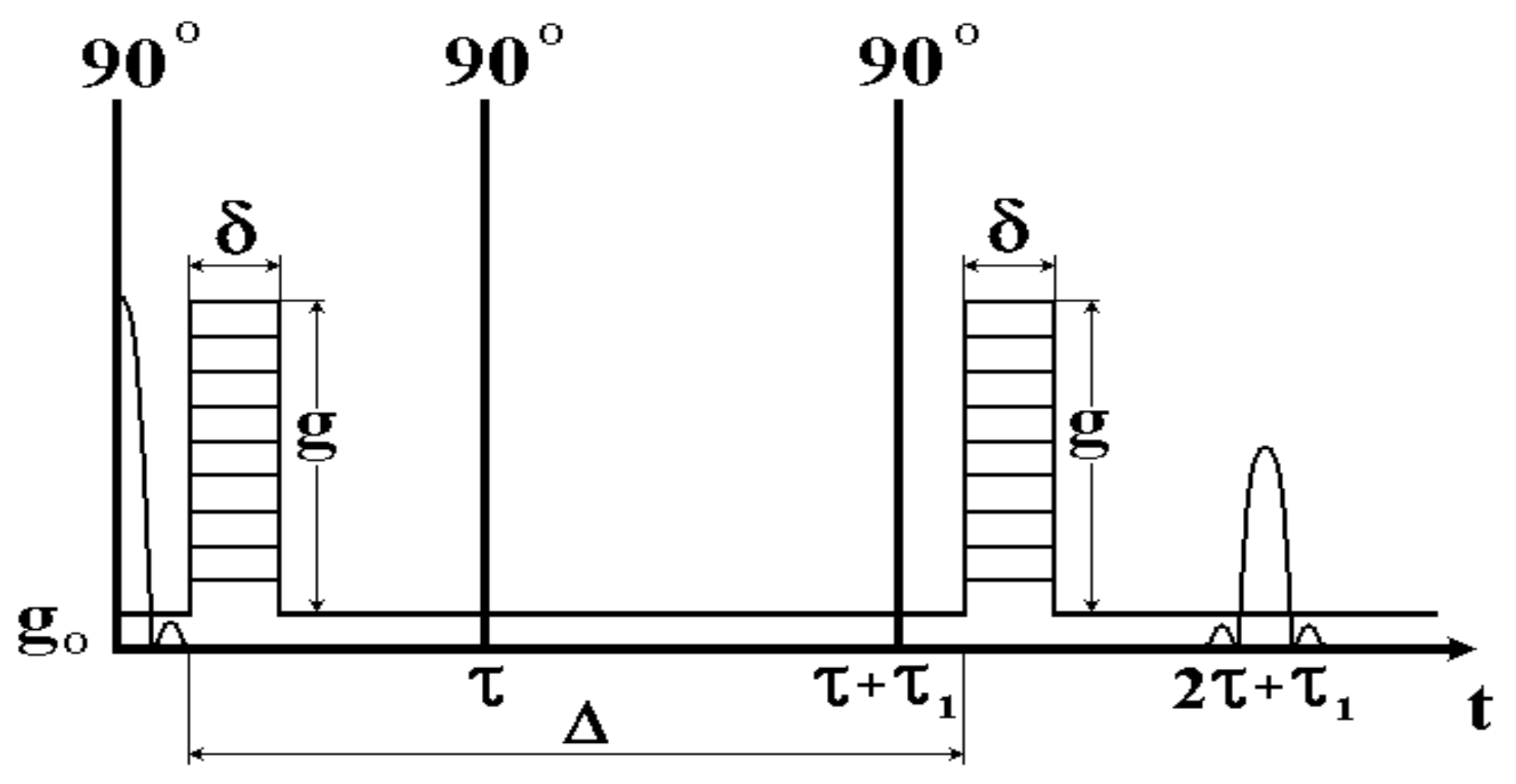
7. Special Features of Experimental Studies of Biological Objects
8. Gradient NMR in the Studies of Diffusion Transfer of Water through Different Pathways in the Root of Plants
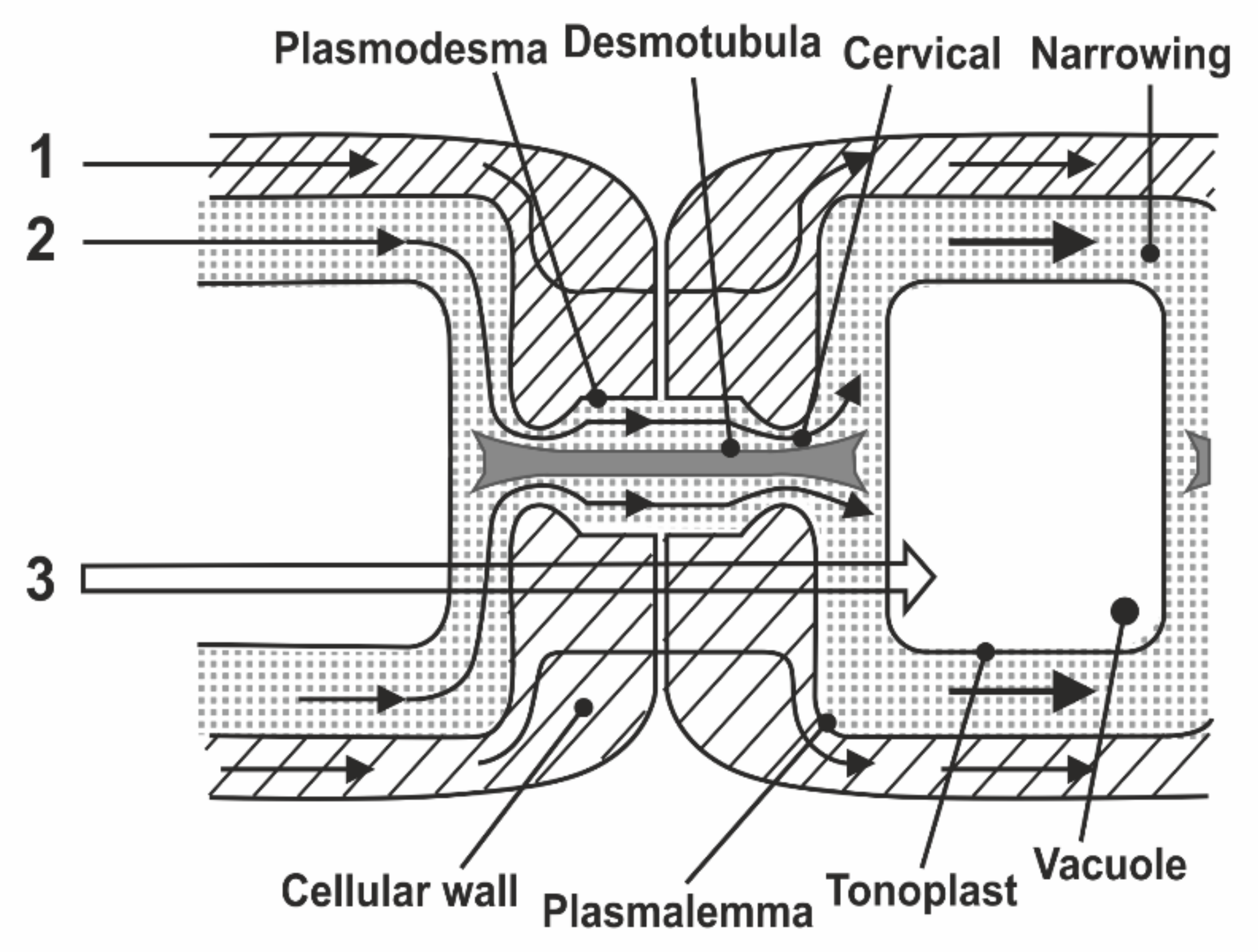
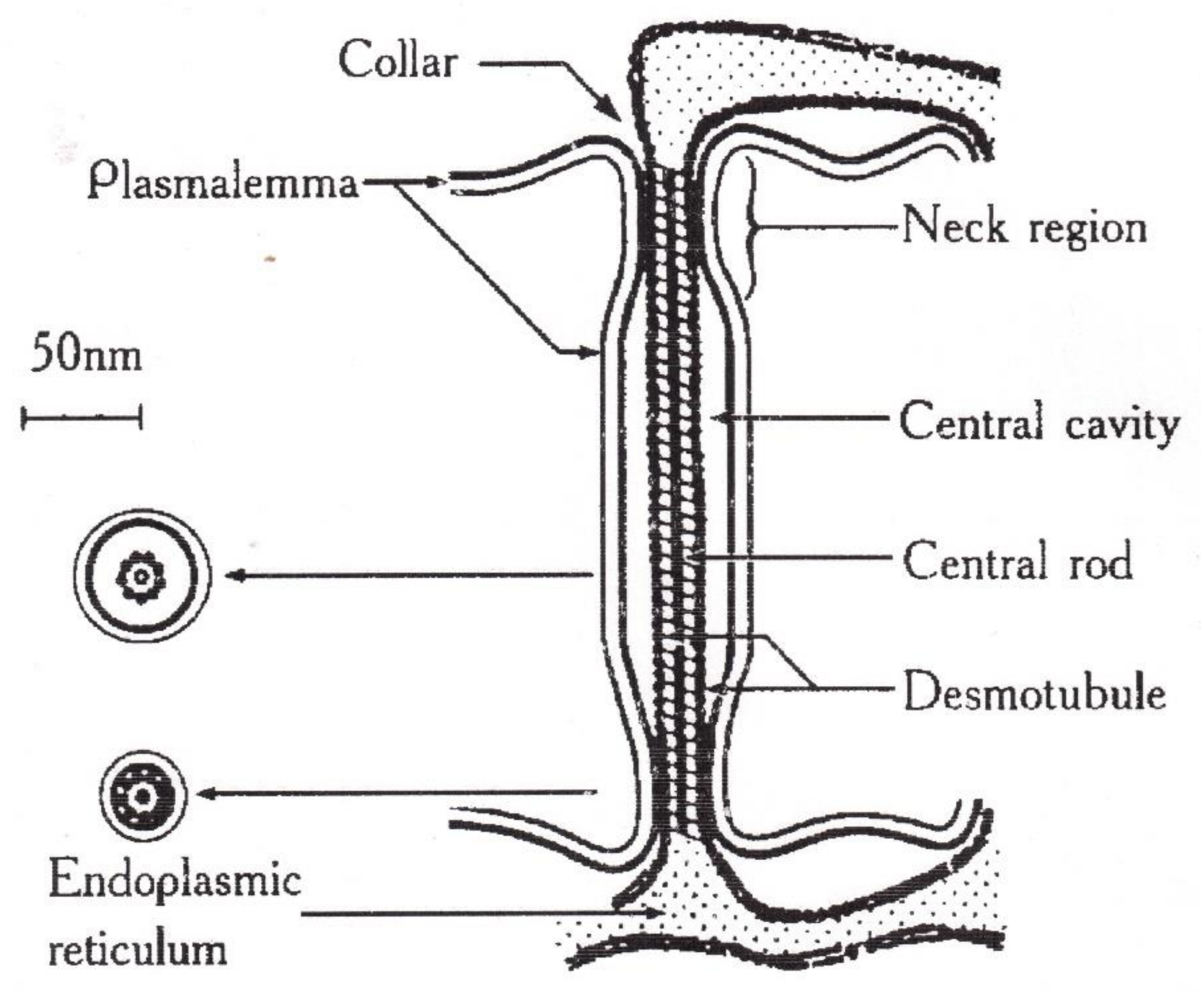
8.1. Transfer of Water through Cytoplasmic Symplast through Plasmodesmata
8.2. Controversial Model of Vacuolar Symplast Transport
8.3. Apoplast Transfer
8.4. Transmembrane Water Transfer through Aquaporins in the Studies of Aquaporin Permeability of Membranes
9. Gradient NMR in the Studies of Water Transport in Root Segments and Roots of Intact Plants When the Concentration of the Main Air Components Changes
9.1. Growth and Transfer of Water in the Root Segment
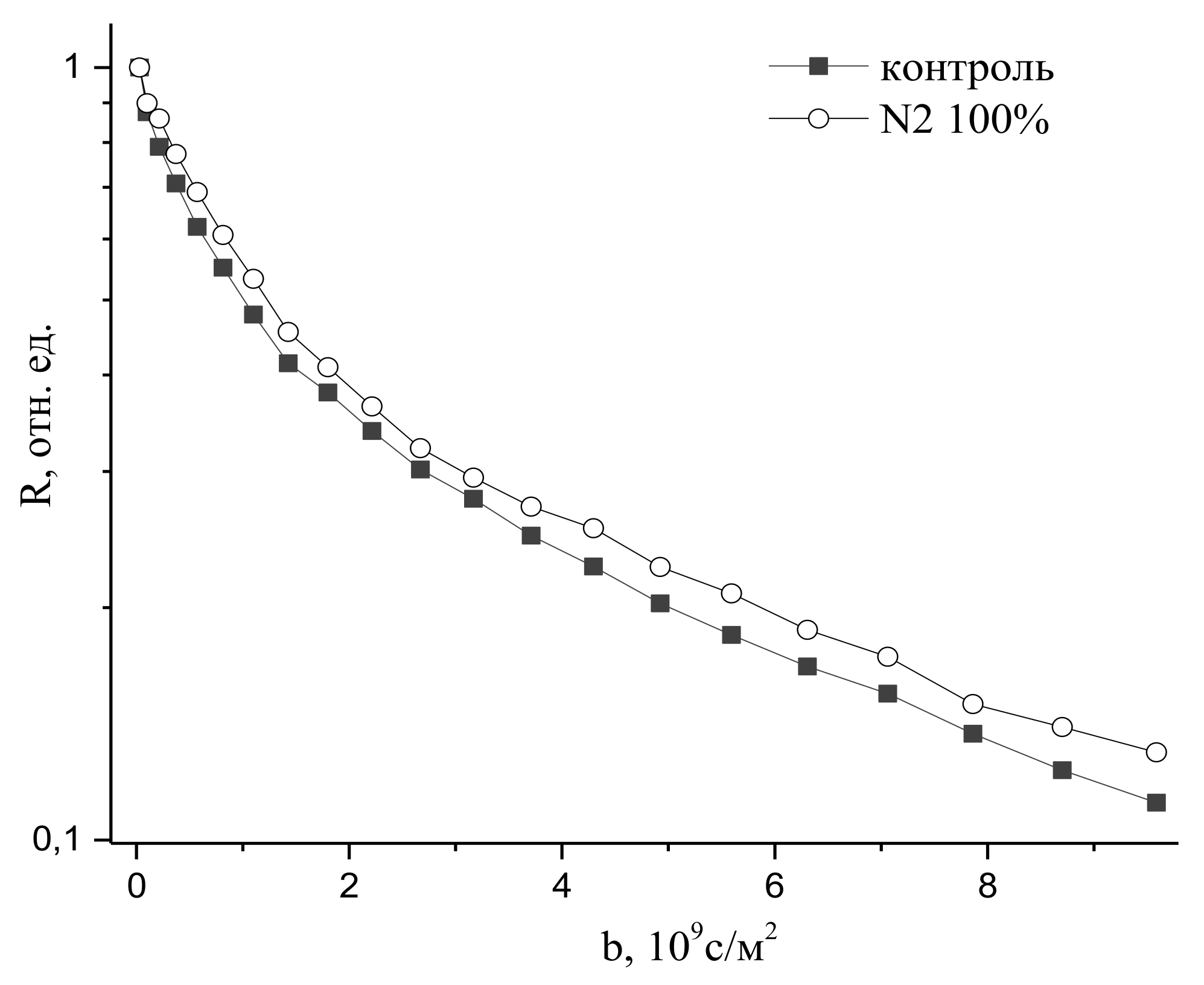
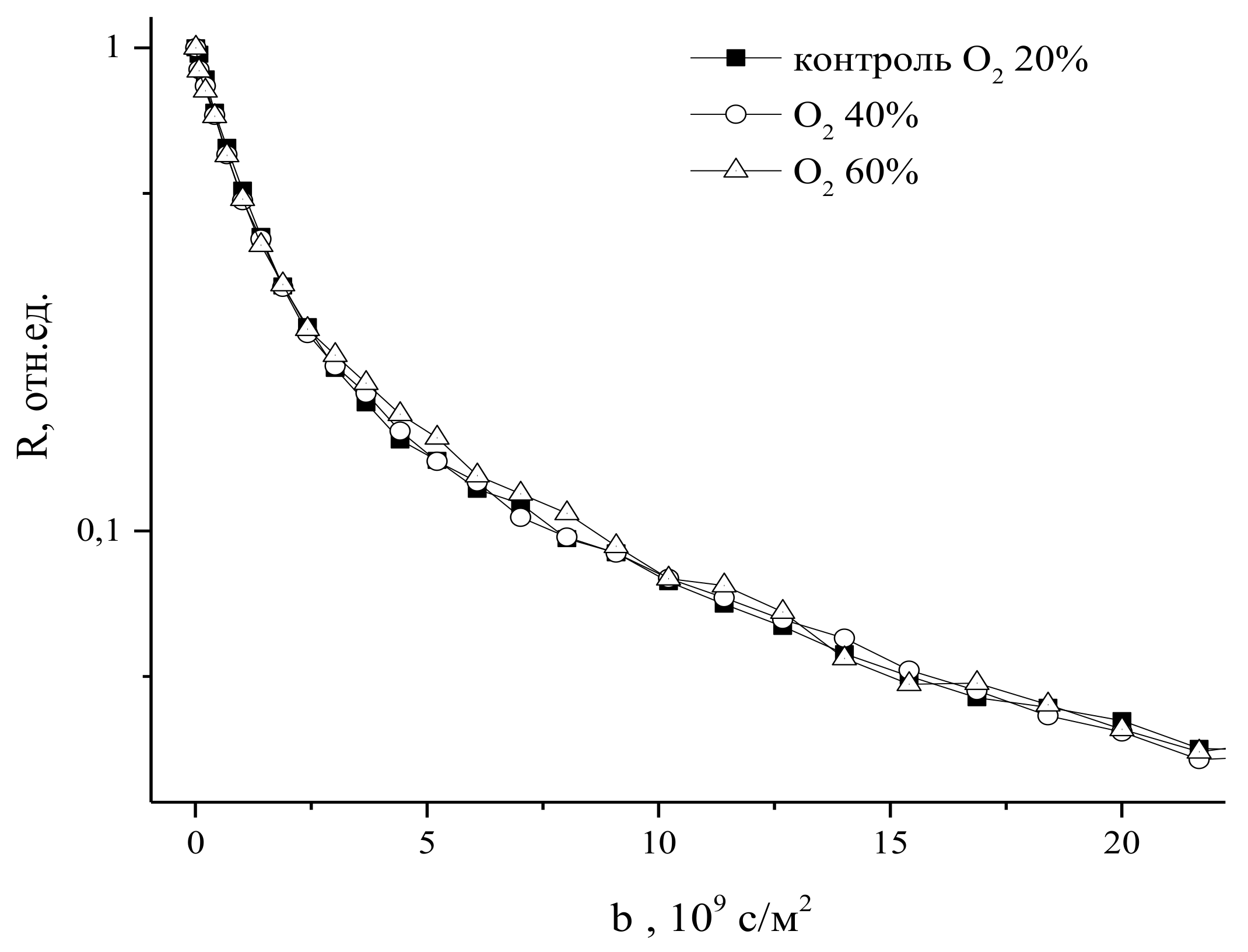
9.2. The Influence of Changes in the Concentration of Atmospheric Gases, Namely, Carbon Dioxide, Oxygen, and Nitrogen, in the Leaf Zone of a Plant on the Transfer of Water in the Root
10. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ling, G.N. Life at the Cell and Below-Cell Level: The Hidden History of a Fundamental Revolution in Biology; Pacific Press: New York, NY, USA, 2001. [Google Scholar]
- Mal’tsev, N.A.; Miftakhutdinova, F.G.; Fedotov, V.D. Characteristics of the condition of water in living plant tissues using the pulse method of nuclear magnetic resonance. In Voprosi Vodoobmena Kulturnix Rastenii (Issues of Water Exchange of Cultivated Plants); Kazan Gos. University: Kazan, Russia, 1965; pp. 20–28. [Google Scholar]
- Mal’tsev, N.A.; Abetsedarskaya, L.A.; Ivoilov, N.G. On the Structural Control. of the State of Water in Isolated Animal Tissues (Research Using the Nuclear Spin Echo Method); Collection of Articles. Biophysics of Cells; Nauka: Moscow, Russia, 1965; pp. 45–52. [Google Scholar]
- Аbetsedarskaya, L.A.; Miftakhutdinova, F.G.; Fedotov, V.D. On the state of water in living tissues (the results of studies by the NMR-spin echo method. Biophysics 1968, 13, 630–636. (In Russian) [Google Scholar]
- Anisimov, A.V.; Ratković, S. Transport Vody v Rasteniyakh: Issledovanie Impul’snym Metodom YaMR [Water Transport in Plants: Determination by Pulsed NMR]; Nauka: Moscow, Russia, 1992; 144p. (In Russian) [Google Scholar]
- Steudle, E. Water transport across roots. Plant Soil 1994, 167, 79–90. [Google Scholar] [CrossRef]
- Steudle, E.; Peterson, C.A. How does water get through roots? J. Exp. Bot. 1998, 49, 775–788. [Google Scholar] [CrossRef]
- Нahn, E.L. Spin echoes. Phys. Rev. 1950, 80, 580–584. [Google Scholar]
- Bloch, F. Nuclear induction. Phys. Rev. 1946, 70, 460–474. [Google Scholar] [CrossRef]
- Abraham, A. The Principles of Nuclear Magnetism; Oxford University Press: London, UK, 1961; 599p. [Google Scholar]
- Callaghan, P.T.; Stepishnik, J. Generalized analysis of motion using magnetic field gradients. In Advances in Magnetic and Optical Resonance; Warren, W.S., Ed.; Academic Press: San Diego, CA, USA, 1996; Volume 19, pp. 332–389. [Google Scholar]
- Das, T.P.; Saha, A.K. Mathematical Analysis of the Hahn Spin-Echo Experiment. Phys. Rev. 1954, 93, 749–755. [Google Scholar] [CrossRef]
- Torrey, H.C. Nuclear spin relaxation by translational diffusion. Phys. Rev. 1953, 92, 962–969. [Google Scholar] [CrossRef]
- Douglass, D.C.; McCall, D.W. Diffusion in Paraffin. Phys. Chem. 1958, 62, 1102–1107. [Google Scholar] [CrossRef]
- Ghosh, S.K.; Sinha, S.K. Nuclear spin-echoes and molecular self-diffusion in liquids Indian. J. Phys. 1960, 34, 339–357. [Google Scholar]
- Woessner, D.E. Seld-Diffusion measurements in liquids by the spin-echo technique. Rev. Sci. Instrum. 1960, 31, 1146–1153. [Google Scholar] [CrossRef]
- Woessner, D.E. Effects of diffusion in nuclear magnetic resonance spin-echo experiments. J. Chem. Phys. 1961, 34, 2057–2061. [Google Scholar] [CrossRef]
- Hurlimann, M.D.; Helmer, K.G.; de Swiet, T.M.; Sen, P.N.; Sotak, C.H. Spin Echoes in a Constant Gradient and in the Presence of Simple Restriction. J. Magn. Res. Ser. A 1995, 113, 260–266. [Google Scholar] [CrossRef]
- Appel, M.; Fleischer, G.; Fujara, F. Investigation of Polymer Dynamics in Polymer Solutions and Melts over Space Scales below 100 nm with Field-Gradient NMR. Visok. Soedin. (High. Mol. Compd.) 1993, 35, 1913–1917. [Google Scholar]
- Chang, I.; Fujara, F.; Geil, B.; Hinze, G.; Sillescu, H.; Tolle, A. New perspectives of NMR in ultrahigh static magnetic field gradients. J. Non-Cryst. Solids 1994, 172–174 Pt 2, 674–681. [Google Scholar] [CrossRef]
- Stejskal, E.O.; Tanner, J.E. Spin diffusion measurements: Spin echoes in the presence of a time-dependent field gradient. J. Chem. Phys. 1965, 42, 288–292. [Google Scholar] [CrossRef] [Green Version]
- Tanner, J.E. Use of the stimulated echo in NMR diffusion studies. J. Chem. Phys. 1970, 52, 2523–2526. [Google Scholar] [CrossRef]
- Maklakov, A.I.; Skirda, V.D.; Fatkullin, N.F. Samodiffuziya v Rastvorakh i Rasplavakh Polimerov (Self-Diffusion in Solutions and Melts of Polymers); Soloviev, U., Chalykh, A., Eds.; Kazan University Press: Kazan, Russia, 1987; p. 224. [Google Scholar]
- Karger, J. Zur Massbarkeit von Diffusionkoeffizienten in Zweiphase System mit Hilfe der Methode der Gepulsten Feld gradienten. Ann. Phys. Ser. 1B 1969, 24, 1–7. [Google Scholar]
- Levitt, D.G. Dynamics of a Single-File Pore: Non-Fickian Behavior. Phys. Rev. 1976, 8, 3050–3053. [Google Scholar] [CrossRef]
- Velikanov, G.A.; Volkov, V.Y. Diffusion of water through narrow pores of membranes. Biophysics 1978, 23, 813–816. [Google Scholar]
- Tanner, J.E.; Stejskal, E.O. Restricted self-diffusion of protons in colloidal systems by the pulse-gradient spin-echo method. J. Chem. Phys. 1968, 49, 1768–1777. [Google Scholar] [CrossRef]
- Cooper, R.L.; Chang, D.B.; Young, A.C.; Martin, C.J.; Ancker-Johnson, B. Restricted diffusion in biological systems. Biophys. J. 1974, 14, 161–177. [Google Scholar] [CrossRef] [Green Version]
- Robertson, B. Spin-Echo Decay of Spins Diffusing in a Bounded Region. Phys. Rev. 1966, 151, 273–277. [Google Scholar] [CrossRef]
- Chizmadzhev, Y.A.; Markin, V.S.; Tarasevich, M.R.; Chirkov, Y.T. Makrokinetika Protsessov v Poristykh Sredakh (Macrokinetics of Processes in Porous Media); Nauka: Moscow, Russia, 1971; p. 363. [Google Scholar]
- Volkov, V.Y.; Bashirov, F.I.; Dautov, R.A.; Saykin, K.S.; Nagibarov, V.R. Study of Spatially Limited Self-Diffusion of a Molecule by the Pulse Method of Nuclear Magnetic Resonance; Nekotorie Voproci Fiziki Gidkosti; Izd. KGPI: Kazan, Russia, 1976; Volume 158, pp. 65–88. [Google Scholar]
- Crick, F. Diffusion in embryogenesis. Nature 1970, 225, 420–422. [Google Scholar] [CrossRef]
- Robards, A.W.; Clarkin, D.T. The role of plasmadesmata in the transport of water and nutrients across roots. In Intercellular Communication in Plants: Studies on Plasmadesmata; Gunning, B.E.S., Robards, A.W., Eds.; Springer: Berlin, Germany, 1976; pp. 181–199. [Google Scholar]
- Fleisher, G.; Skirda, V.D.; Werner, A. NMR–investigation of restricted self-diffusion of oil in rape seeds. Eur. Biophys. J. 1990, 19, 1–6. [Google Scholar] [CrossRef]
- Skirda, V.D.; Valiullin, R.R. Scaling approach to the analysis of the time dependence of the effective diffusion coefficients in porous and polymer systems. Struct. Dyn. Mol. Syst. 1996, 2, 64–69. [Google Scholar]
- Valiullin, R.; Skirda, V. Time dependent self-diffusion coefficient of molecules in porous media. J. Chem. Phys. 2001, 114, 452–458. [Google Scholar] [CrossRef]
- Fatkullin, N.F. On the theory of diffusion attenuation of the spin echo signal in media with random obstacles. JETF 1990, 98, 2030–2037. [Google Scholar]
- Mitra, P.P.; Sen, P.N. Effects of microgeometry and surface relaxation on NMR pulsed-field-gradient experiments: Simple pore geometry. Phys. Rev. B 1992, 45, 143–156. [Google Scholar] [CrossRef]
- Cho, C.H.; Hong, Y.S.; Kang, K.; Volkov, V.I.; Skirda, V.; Lee, C.Y.J.; Lee, C.H. Water self-diffusion in Chlorella sp. studied by pulse field gradient NMR. Magn. Reson. Imaging 2003, 21, 1009–1017. [Google Scholar] [CrossRef]
- Anderson, W.A. Electrical Current Shims for Correcting Magnetic Fields. Rev. Sci. Instrum. 1961, 32, 241–250. [Google Scholar] [CrossRef]
- Tanner, J.E. Pulsed field gradients for NMR spin-echo diffusion measurements. Rev. Sci. Instrum. 1965, 36, 1086–1087. [Google Scholar] [CrossRef]
- Anisimov, A.V.; Velikanov, G.A.; Fedotov, V.D. Equipment to the installation of spin echo nuclear magnetic resonance to create a pulsed magnetic field gradient. Pribory i Technika Eksperimenta 1975, 4, 114–116. [Google Scholar]
- Matson, J.B. A precision Current Pulse Generator for NMR Self-Diffusion Measurements by the Pulsed Gradient Technique. Rev. Sci. Instrum. 1972, 43, 1504–1508. [Google Scholar] [CrossRef]
- Skirda, V.D.; Sevryugin, V.A.; Sundukov, V.I. Precision stabilizer of the integral value of current pulses in an inductive load. Prib. Tech. Eksperimenta 1984, 6, 122–125. [Google Scholar]
- Sevryugin, V.A.; Skirda, V.D. NMR sensor of a relaxometer with a coil of a pulsed magnetic field gradient. Prib. Tech. Eksperimenta 1982, 5, 112–113. [Google Scholar]
- Webster, D.S.; Marsden, K.N. Improved Apparatus for the NMR Measurement of Self-Diffusion Coefficients Using Pulsed Field Gradients. Rev. Sci. Instrum. 1974, 45, 1232–1234. [Google Scholar] [CrossRef]
- Anisimov, A.V.; Evarestov, A.S.; Zuikov, V.A. Program of a pulsed magnetic field gradient for studying adsorbed molecules by the spin echo method. Prib. Tech. Eksperimenta 1978, 1, 132–134. [Google Scholar]
- Anisimov, A.V.; Evarestov, A.S.; Ionenko, I.F.; Gusev, N.A. [Pulsed NMR method for estimation of intercellular water transport on symplasm. Dokl. Akad. Nauk SSSR 1983, 271, 1246–1249. (In Russian) [Google Scholar]
- Groβ, B.; Kosfeld, R.A. Anwendung der spin-echo methode bei der messung der selbsdiffusion. Messtechnik 1969, 77, 171–177. [Google Scholar]
- Anisimov, A.V.; Mishagina, E.A.; Dautova, N.R. Simple Modifications to the NMR PGSE Technique for Investigations in the Regime of Short Diffusion Observation Times. Concepts Magn. Reson. Part B 2008, 33, 216–219. [Google Scholar] [CrossRef]
- Anisimov, A.V.; Ionenko, I.F.; Romanov, A.V. Spin-echoNMR study of the translational water diffusion selectively along the apoplast and the cytoplasmic and vacuolar symplasts of plants. Biophysics 2004, 49, 891–896. (In Russian) [Google Scholar]
- Anisimov, A.V.; Vorob’ev, V.N.; Zholkevich, V.N. Vklad aktomiozinovogo motora vo vnutrikletochnyu perenos vodi Elodea canadensis (Contribution of the actomiosin motor to intracellular transport of water at Elodea canadensis). Dokl. Akad. Nauk SSSR 2003, 392, 823–824. [Google Scholar]
- Vorob’ev, V.N.; Anisimov, A.V.; Dautova, N.R. Contribution of the actomyosin motor to the temperature-dependent translational diffusion of water by cytoplasmic streaming in Elodea canadensis cells. Protoplasma 2004, 224, 195–199. [Google Scholar] [CrossRef] [PubMed]
- Maklakov, A.I.; Skirda, V.D.; Fatkullin, N.F. Self-diffusion in Polimer Systems. In Encyclopedia of Fluid Mechanics. 9. Polymer Flow Enginering; Cheremisinoff, N.P., Ed.; Gulf Publishing Company: Houston, TX, USA, 1990; Volume 22, pp. 705–746. [Google Scholar]
- Couvreur, V.; Faget, M.; Lobet, G.; Javaux, M.; Chaumont, F.; Draye, X. Going with the Flow: Multiscale Insights into the Composite Nature of Water Transport in Roots. Plant. Physiol. 2018, 178, 1689–1703. [Google Scholar] [CrossRef] [Green Version]
- Robards, A.W. The ultrastructure of plasmodesmate. Protoplasma 1971, 72, 315–323. [Google Scholar] [CrossRef]
- Lopez-Saens, J.F.; Gimener-Martina, G.G.; Risueno, M.C. Fine Structure of the plasmodesms. Protoplasma 1966, 61, 81–84. [Google Scholar] [CrossRef]
- Anisimov, A.V.; Egorov, A.G. Plasmodesmata as a modulator of osmotic water flows in plants. Plant. Physiol. 2002, 49, 758–766. [Google Scholar]
- Anderson, J.L.; Malone, D.M. Mechanism of Osmotic Flow in Porous Membranes. Biophys. J. 1974, 14, 957–982. [Google Scholar] [CrossRef] [Green Version]
- Oparka, A.; Prior, M. Direct evidence for pressure-generated closure of plasmodesmata. Plant. Sci. 1992, 9, 741–750. [Google Scholar] [CrossRef]
- Bacic, G.; Ratkovic, S. Water exchange in plant tissue studied by proton NMR in presence of paramagnetic centers. Biophys. J. 1984, 45, 767–776. [Google Scholar] [CrossRef] [Green Version]
- Usov, V.Y.; Churin, A.A.; Belyanin, M.L.; Borodin, O.Y.; Filimonov, V.D.; Bezlepkin, A.I. Poluchenie i doklinicheskoe issledovanie kompleksa MN-trans-1,2-diaminociklogeksan -N,N,N′,N′-tetraacetata (ciklomanga)kak paramagnitnogo kontrastnogo preparata dliy magnito-rezonansnoi spektroskopii. Meditsinskaya Vizualizatsija 2009, 5, 121–132. (In Russian) [Google Scholar]
- Carr, D.H.; Brown, J.; Bydder, G.M.; Steiner, R.E.; Weinmann, H.J.; Speck, U.; Hall, A.S.; Young, I.R. GadoliniumDTPA as a contrast agent in MPI: Initial clinical experience in 20 patients. Am. J. Radiol. 1984, 143, 215–224. [Google Scholar]
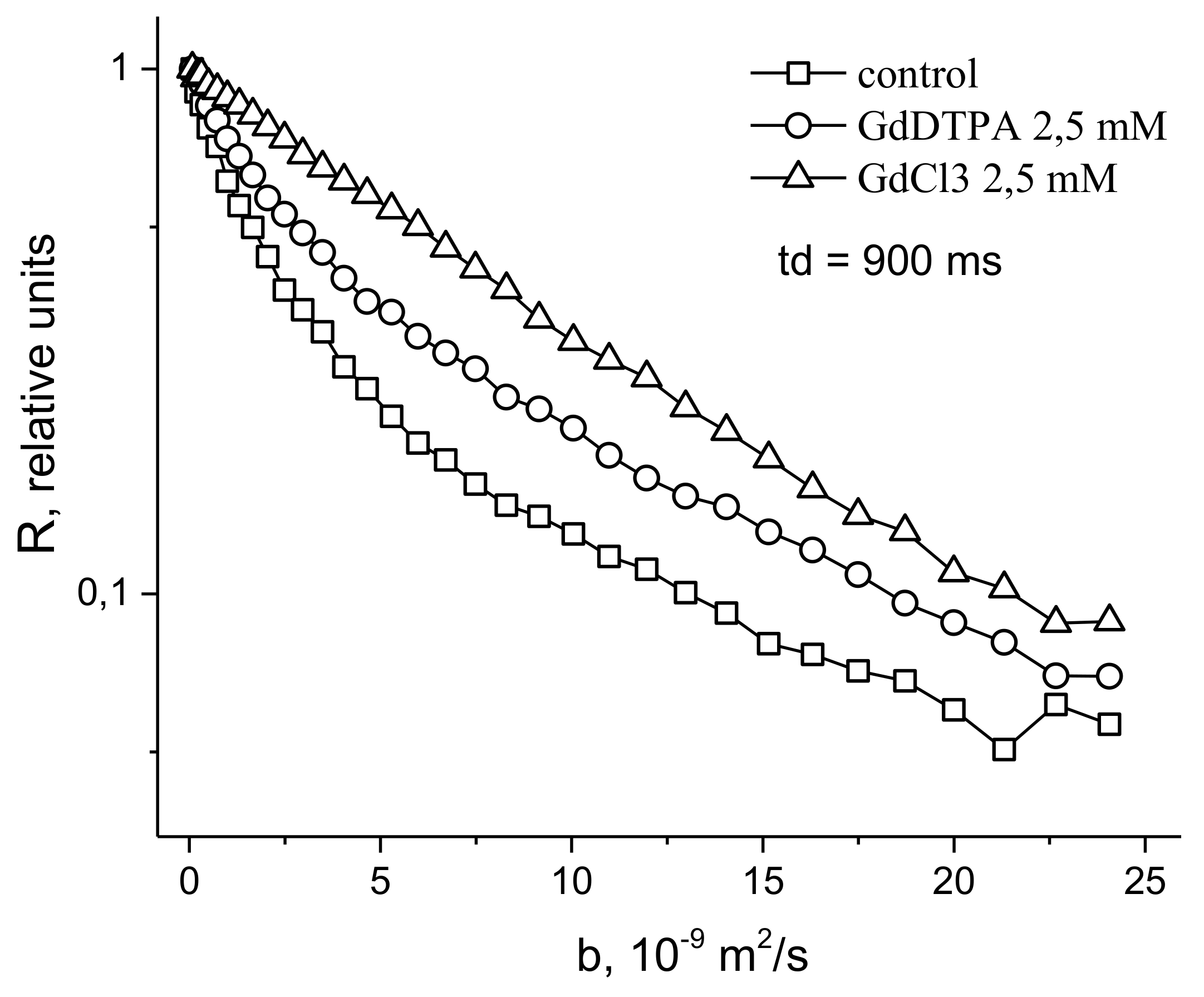
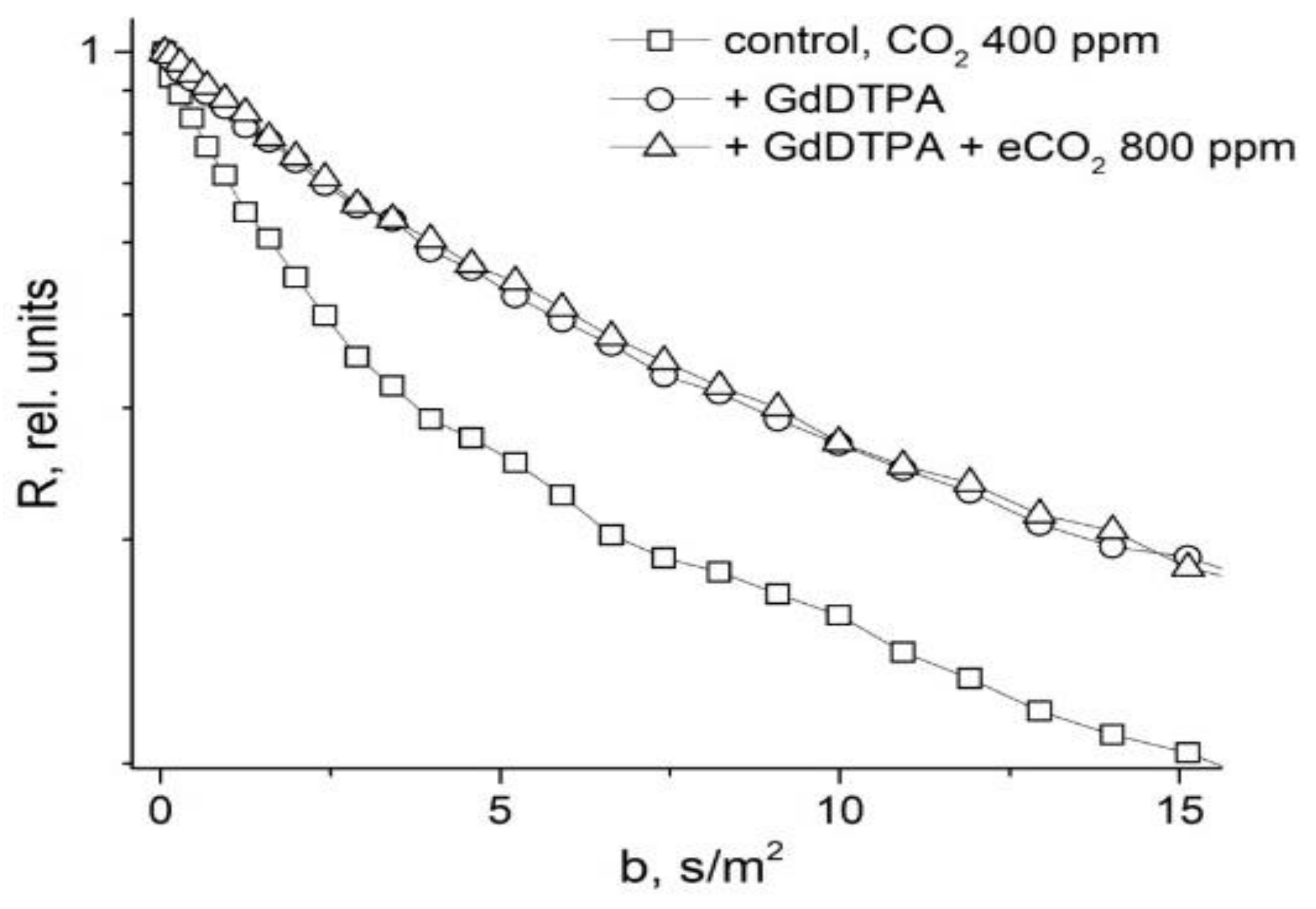
- Anisimov, A.V.; Suslov, M.A. Estimating the MRI contrasting agents effect on water permeability of plant cell membranes using the 1H NMR gradient technique. Appl. Magn. Reson. 2021, 52, 235–246. [Google Scholar] [CrossRef]
- Anisimov, A.V.; Egorov, A.G. Estimation of the axial water flow rate from the symplast of the plant cell chain from the value of the quasi-diffusion coefficient. Biophysics 1992, 37, 335–339. (In Russian) [Google Scholar]
- Slavik, B. Methods of Studying Plant. Water Relations; Ecological Studies Series; Springer: Berlin/Heidelberg, Germany, 1974; p. 235. [Google Scholar]
- Gamalei, Y.V. Precise organization of plants. Russ. J. Plant. Physiol. 1997, 44, 819–846. [Google Scholar]
- Gamalei, Y.V. Transportnaya Sistema Sosudistykh Rastenii (Transport System in Vascular Plants); St. Petersburg Gos. University: St. Petersburg, Russia, 2004. [Google Scholar]
- Overall, R.L.; Wolfe, J.; Gunning, B.E.S. Intercellular communication in Azolla roots. I. Ultrastructure of plasmodesmata. Protoplasma 1982, 111, 134–150. [Google Scholar] [CrossRef]
- Velikanov, G.A.; Volobueva, O.V.; Khokhlova, L.P. Self-diffusion of water inside vacuolar and cytoplasmic Self-diffusion of water inside vacuolar and cytoplasmic symplast. Dokl. Akad. Nauk. 1999, 368, 420–422. [Google Scholar]
- Velikanov, G.A.; Volobueva, O.V.; Khokhlova, L.P. Studying channels by Plasmodesm according to the Pulse NMR method. Russ. J. Plant. Physiol. 2001, 48, 375–383. [Google Scholar] [CrossRef]
- Velikanov, G.A. Vacuolar symplast and methodological approach to monitoring water self-diffusion between vacuoles of contacting root cells. Russ. J. Plant. Physiol. 2007, 54, 683–692. [Google Scholar] [CrossRef]
- Velikanov, G.A.; Volobueva, O.V.; Belova, L.P.; Gaponenko, E.M. Vacuolar Symplast as a Regulated Pathway for Water Flows in Plants. Russ. J. Plant. Physiol. 2005, 52, 326–331. [Google Scholar] [CrossRef]
- Velikanov, G.A.; Belova, L.P. Regulation of Water Permeability of Vacuolar Symplast. Russ. J. Plant. Physiol. 2005, 52, 758–764. [Google Scholar] [CrossRef]
- Andreev, I.M. Is there a vacuolar symplast in plants? (Comment to the works of Velikanova, G.A. with co-authors published in the “Russian Journal of Plant Physiology”). Russ. J. Plant. Physiol. 2008, 55, 931–933. [Google Scholar] [CrossRef]
- Оrtega, J. Plant cell growth in tissue. Plant. Physiol. 2010, 154, 1244–1253. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tyerman, S.D.; Bohnert, H.J.; Maurel, S.; Steudle, E.; Smith, J.A.C. Plant aquaporins: Their molecular biology, biophysics and significance for plant water relations. J. Exp. Bot. 1999, 50, 1055–1071. [Google Scholar] [CrossRef] [Green Version]
- Javot, H.; Maurel, C. The role of aquaporins in root water uptake. Ann. Bot. 2002, 90, 301–313. [Google Scholar] [CrossRef] [Green Version]
- Chaumont, F.; Barrieu, F.; Wojcik, E.; Chrispeels, M.J.; Jung, R. Aquaporins constitute a large and highly divergent protein family in maize. Plant. Physiol. 2001, 12, 1206–1215. [Google Scholar] [CrossRef] [Green Version]
- Zhang, W.H.; Tyerman, S.D. Inhibition of water channels by HgCl2 in intact wheat root cells. Plant. Physiol. 1999, 120, 849–858. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wan, X.; Zwiazek, J.J. Mercuric chloride effects on root water transport in aspen seedlings. Plant. Physiol. 1999, 121, 939–946. [Google Scholar] [CrossRef] [Green Version]
- Ionenko, I.F.; Anisimov, A.V.; Karimova, F.G. Water transport in maize roots under the influence of mercuric chloride and water stress: A role of water channels. Biol. Plant. 2006, 50, 74–80. [Google Scholar] [CrossRef]
- Ionenko, I.F.; Dautova, N.R.; Anisimov, A.V. Early changes of water diffusional transfer in maize roots under the influence of water stress. Environ. Exp. Bot. 2012, 76, 16–23. [Google Scholar] [CrossRef]
- Van As, H.; Van Vliet, P.A.; Schaafsma, T.J. 1H spin-echo nuclear magnetic resonance in plant tissue. The effect of Mn(II) and water content in wheat leaves. Biophys. J. 1980, 32, 1043–1051. [Google Scholar] [CrossRef] [Green Version]
- Van Dusschoten, D.; De Jager, P.A.; Van As, H. Extracting diffusion constants from echo-time-dependent PFG NMR data using relaxation-time information. J. Magn. Reson. 1995, 116, 22–28. [Google Scholar] [CrossRef]
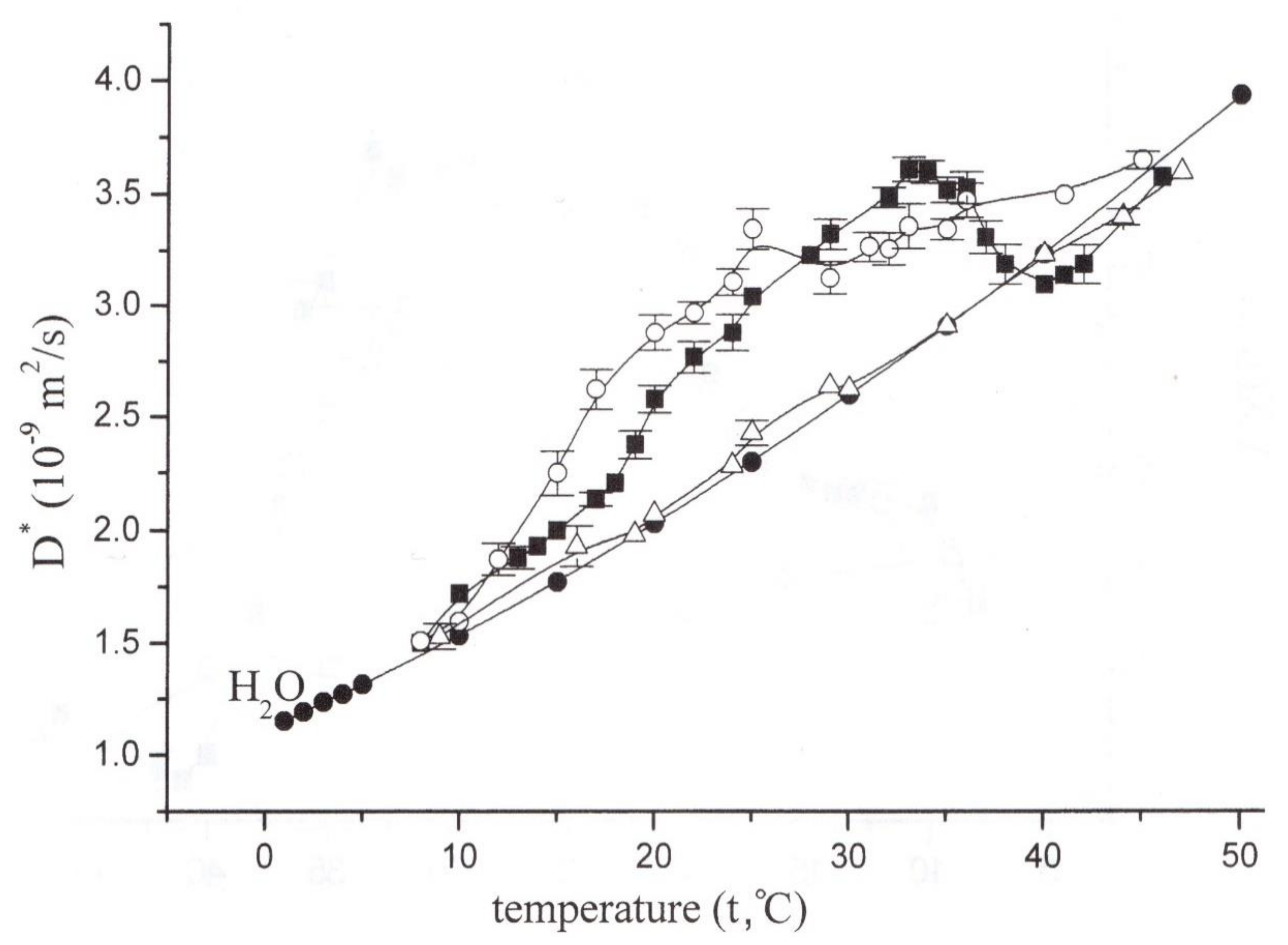
- Ionenko, I.F.; Anisimov, A.V.; Dautova, N.R. Effect of temperature on water transport through aquaporins. Biol. Plant. 2010, 54, 488–494. [Google Scholar] [CrossRef]
- Hertel, A.; Steudle, E. The function of water channels in Chara: The temperature dependence of water and solute flows provides evidence for composite membrane transport and for a slippage of small organic solutes across water channels. Planta 1997, 202, 324–335. [Google Scholar] [CrossRef]
- Henzler, T.; Steudle, E. Reversible closing of water channels in Chara internodes provides evidence for a composite transport model of the plasma membrane. J. Exp. Bot. 1995, 46, 199–209. [Google Scholar] [CrossRef] [Green Version]
- Kamiya, N. Physical and Chemical basis of cytoplasmic streaming. Annu. Rev. Plant. Physiol. 1981, 32, 205–235. [Google Scholar] [CrossRef] [Green Version]
- Tominaga, M.; Yokota, E.; Sonobe, S.; Simmen, T. Mechanism of inhibition of cytoplasmic streaming by a myosin inhibitor 2,3-butanedione monoxim. Protoplasma 2000, 213, 46–54. [Google Scholar] [CrossRef]
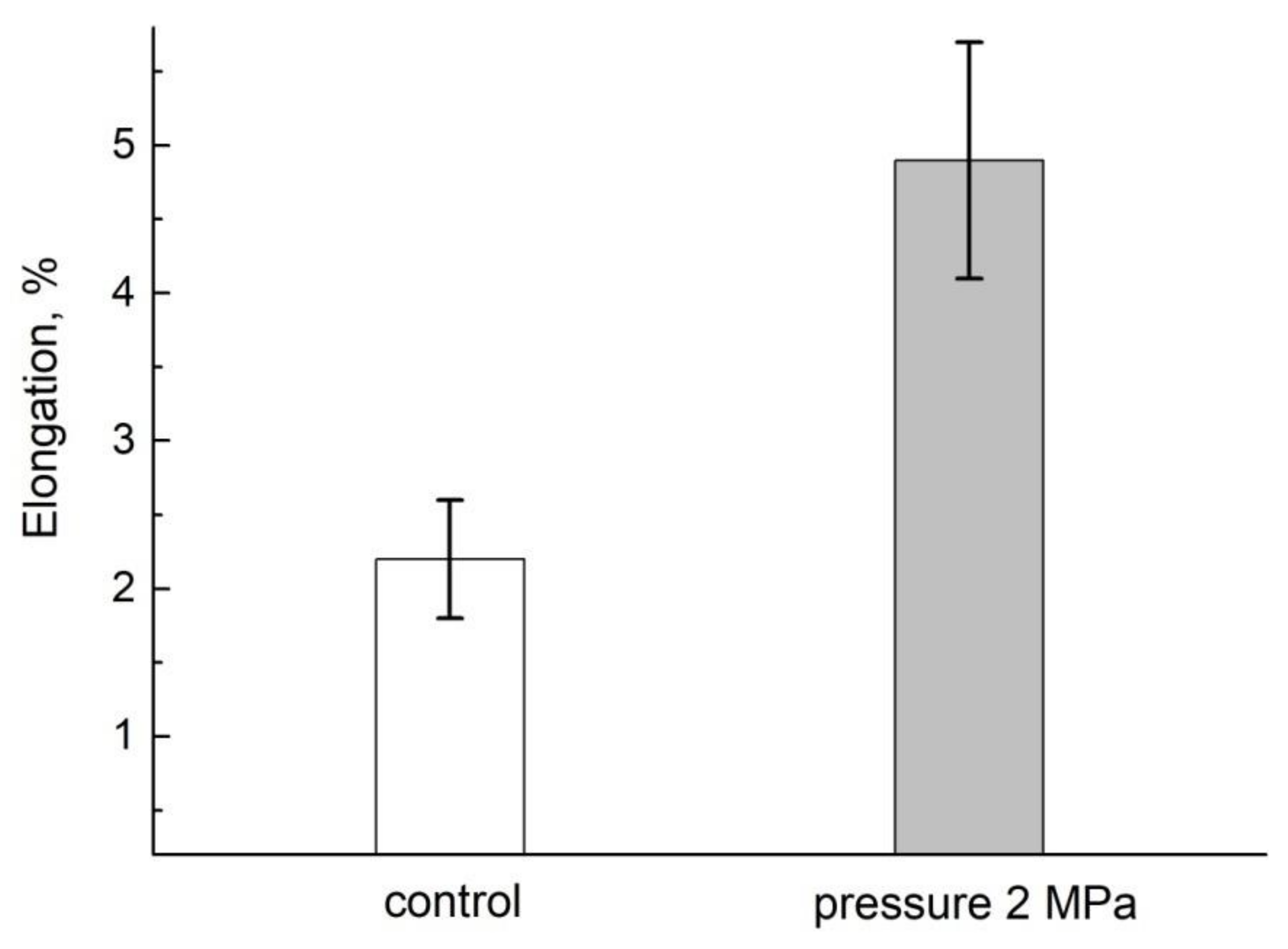
- Anisimov, A.V.; Suslov, M.A.; Alyab’ev, A.Y. Symplasmic Transport of Water along the Root Depends on Pressure. Russ. J. Plant. Physiol. 2014, 61, 512–520. [Google Scholar] [CrossRef]
- Pritchard, J.; Barlow, P.W.; Adam, J.S.; Tomos, A.D. Biophysics of the inhibition of the growth of maize roots by lowered temperature. Plant. Physiol. 1990, 93, 222–230. [Google Scholar] [CrossRef] [Green Version]
- Franks, P.J.; Buckley, T.N.; Shope, J.C.; Mott, K.A. Guard cell volume and pressure measured concurrently by confocal microscopy and the cell pressure probe. Plant. Physiol. 2001, 125, 1577–1584. [Google Scholar] [CrossRef] [Green Version]
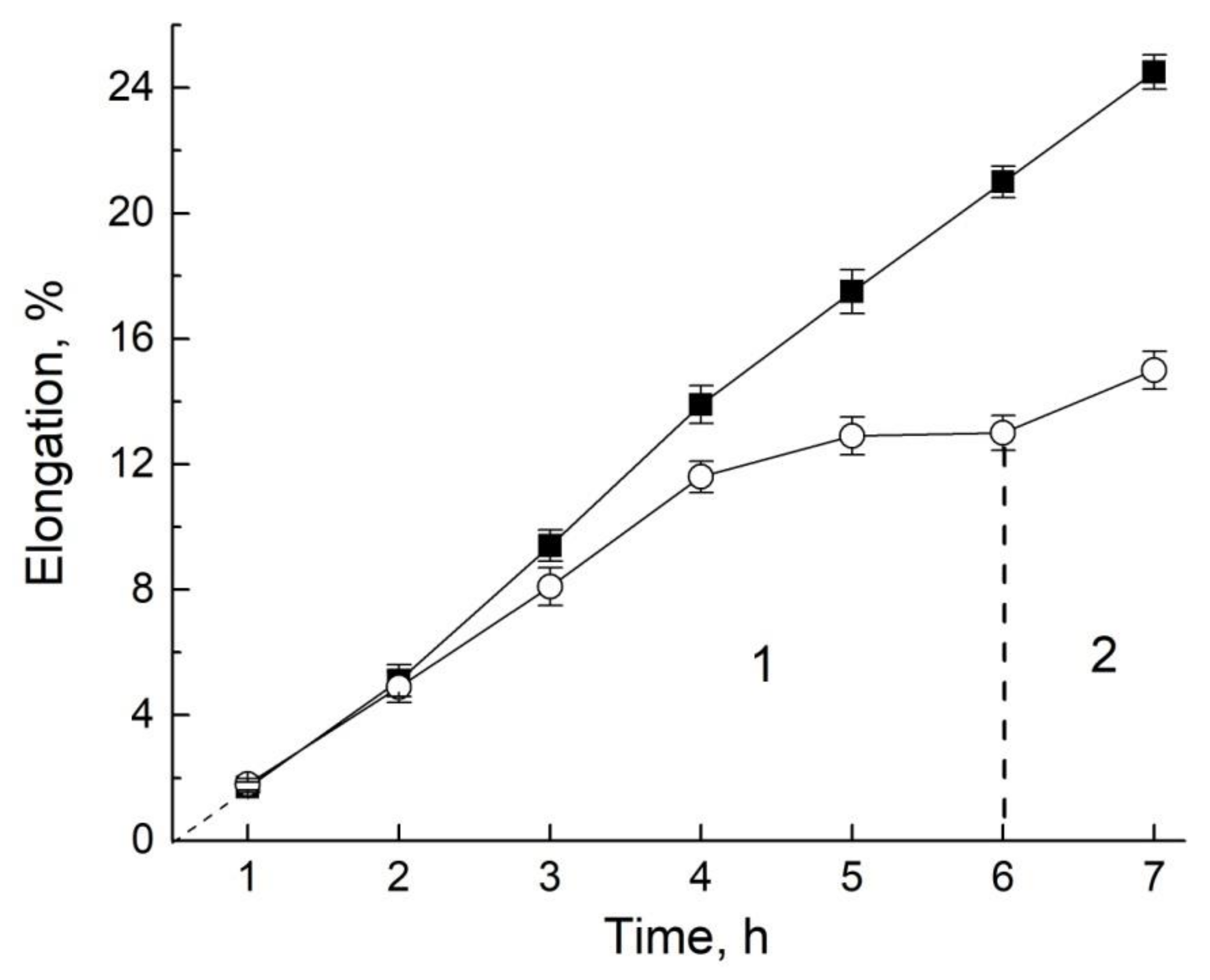
- Anisimov, A.V.; Dautova, N.R.; Suslov, M.A. Growth function and intercellular water transfer in excised roots. Protoplasma 2019, 256, 1425–1432. [Google Scholar] [CrossRef] [PubMed]
- Gong, X.; Li, J.; Lu, H.; Wan, R.; Li, J.; Hu, J.; Fang, H. A Charge-driven molecular water pump. Nat. Nanotechnol. 2007, 2, 709–712. [Google Scholar] [CrossRef]
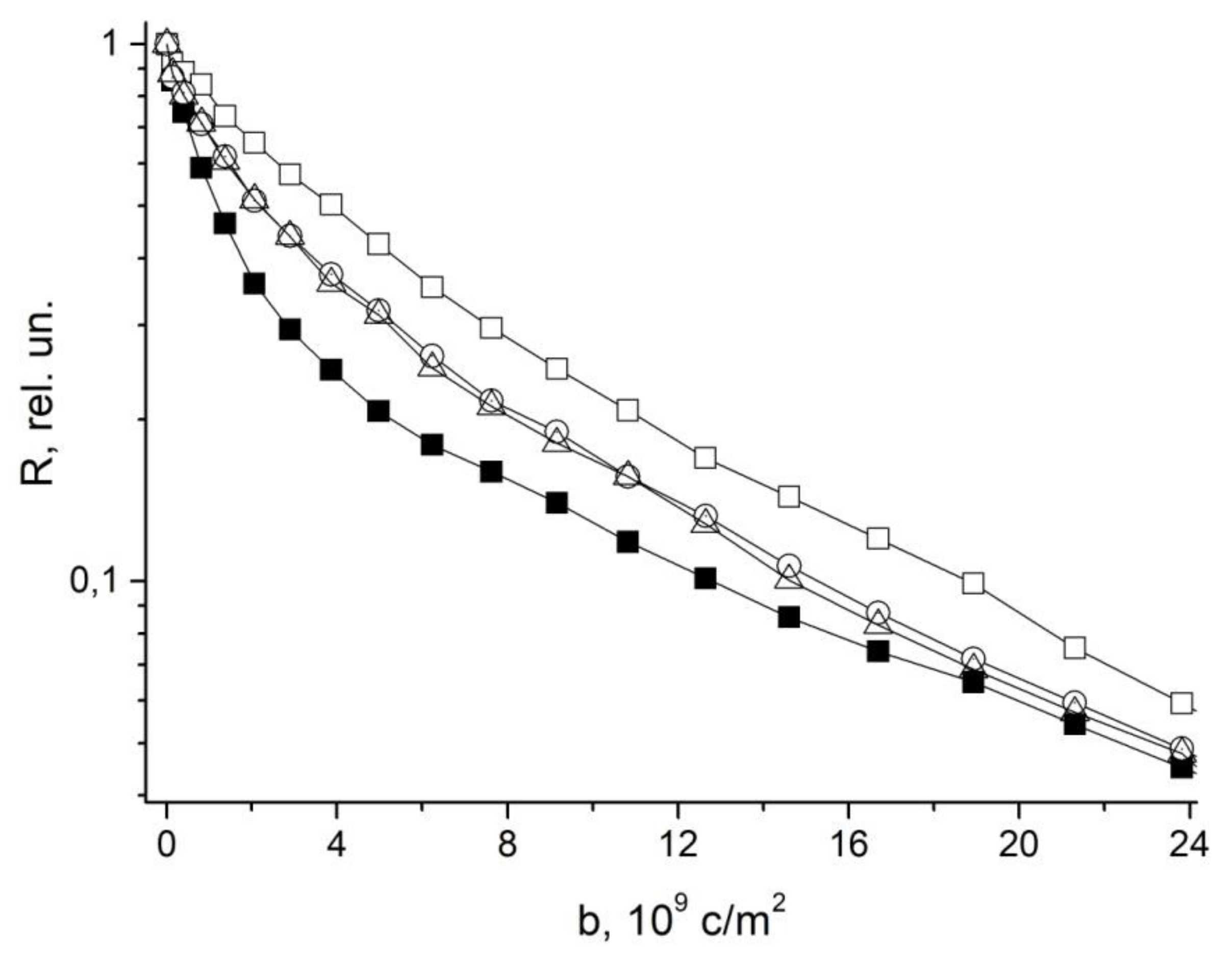
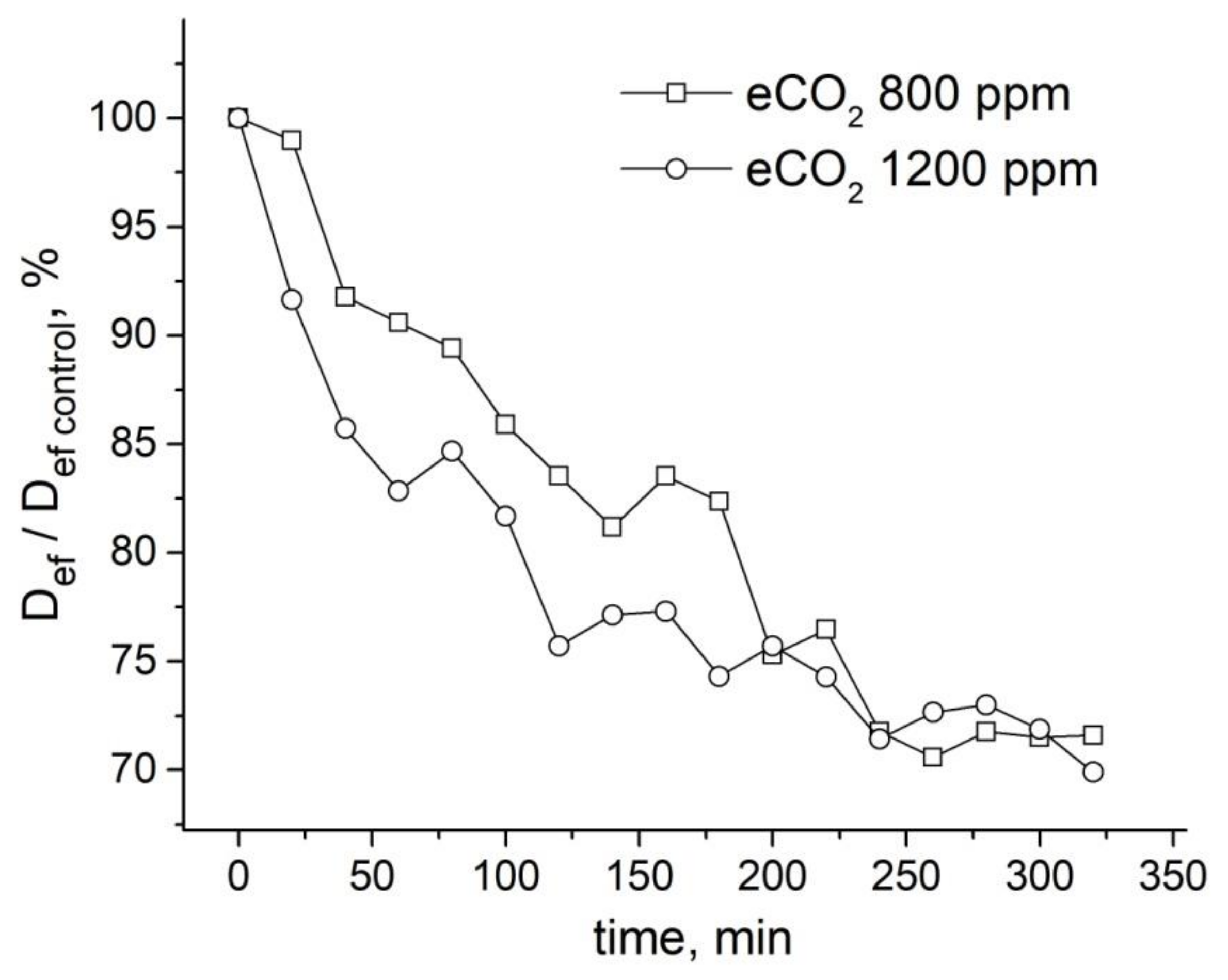
- Suslov, M.A. Dynamics of intercellular water transfer in the roots of intact Zea mays L. plants under elevated concentrations of atmospheric CO2. Plant. Physiol. Biochem. 2020, 151, 516–525. [Google Scholar] [CrossRef] [PubMed]
- Zaghdoud, C.; Carvajal, M.; Ferchichi, A.; Carmen Martínez-Ballesta, M. Water balance and N-metabolism in broccoli (Brassica oleracea L. var. Italica) plants depending on nitrogen source under salt stress and elevated CO2. Sci. Total Environ. 2016, 571, 763–771. [Google Scholar] [CrossRef]
- Maurel, C.; Simonneau, T.; Sutka, M. The significance of roots as hydraulic rheostats. J. Exp. Bot. 2010, 61, 3191–3198. [Google Scholar] [CrossRef] [PubMed] [Green Version]


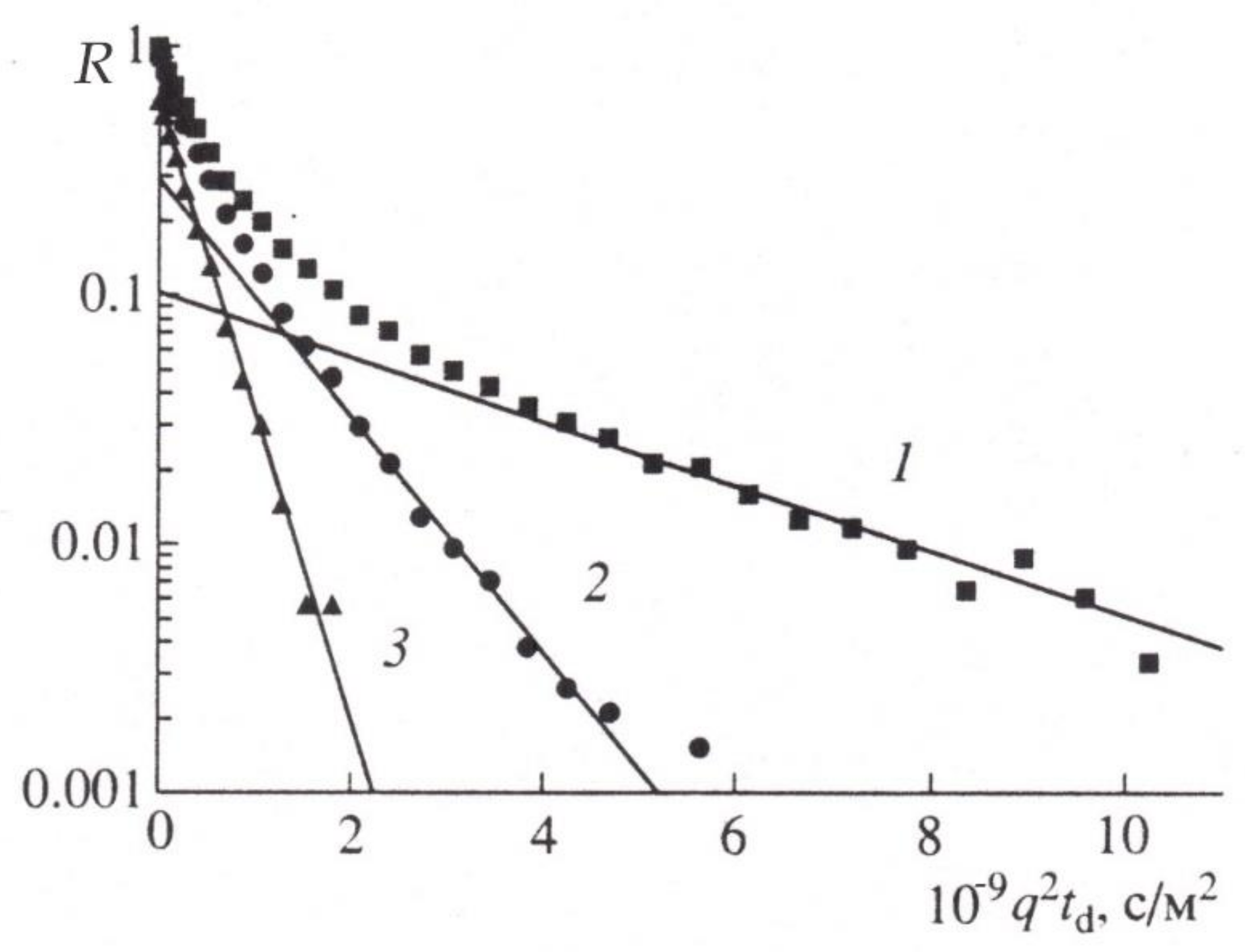

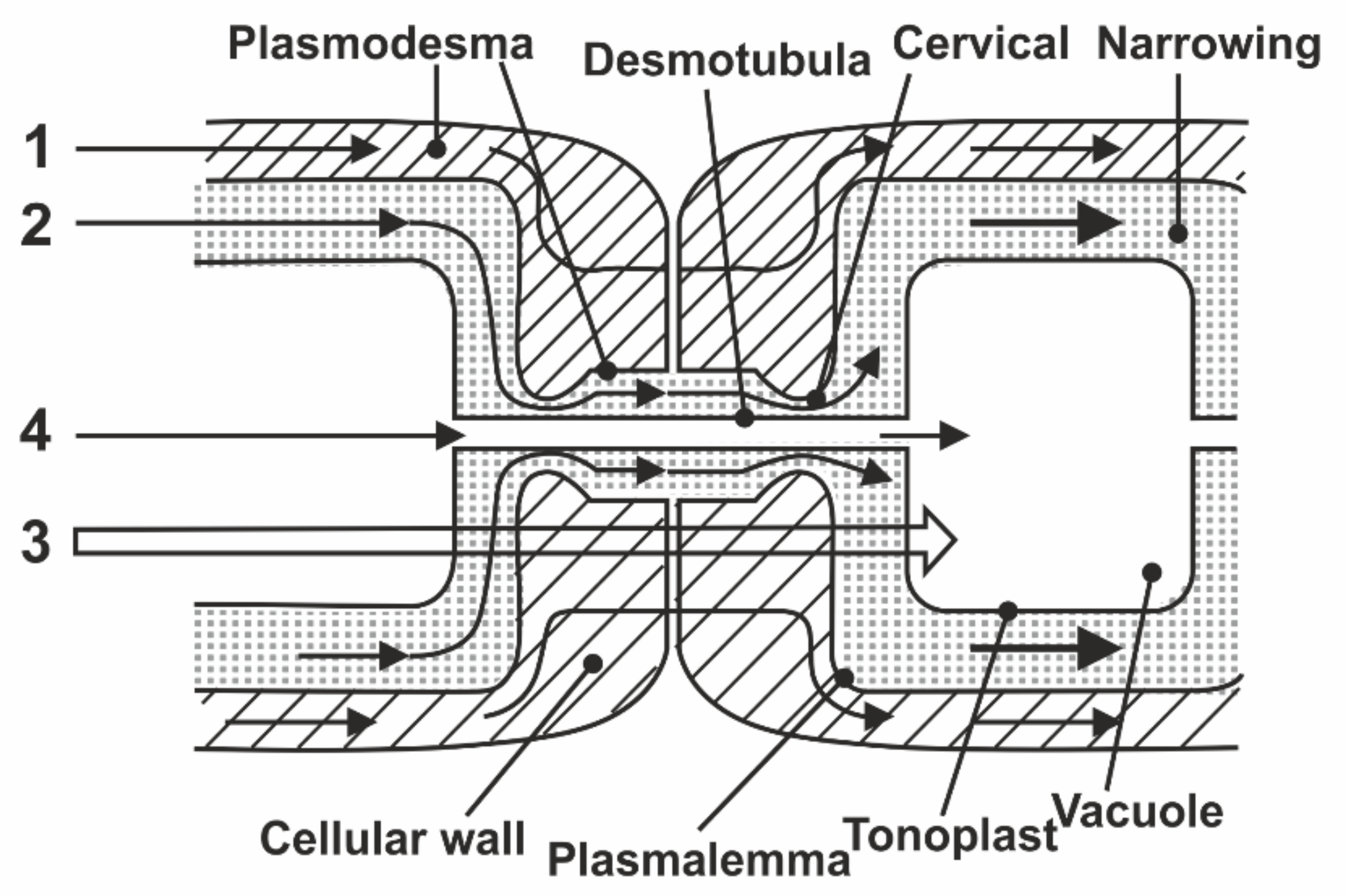
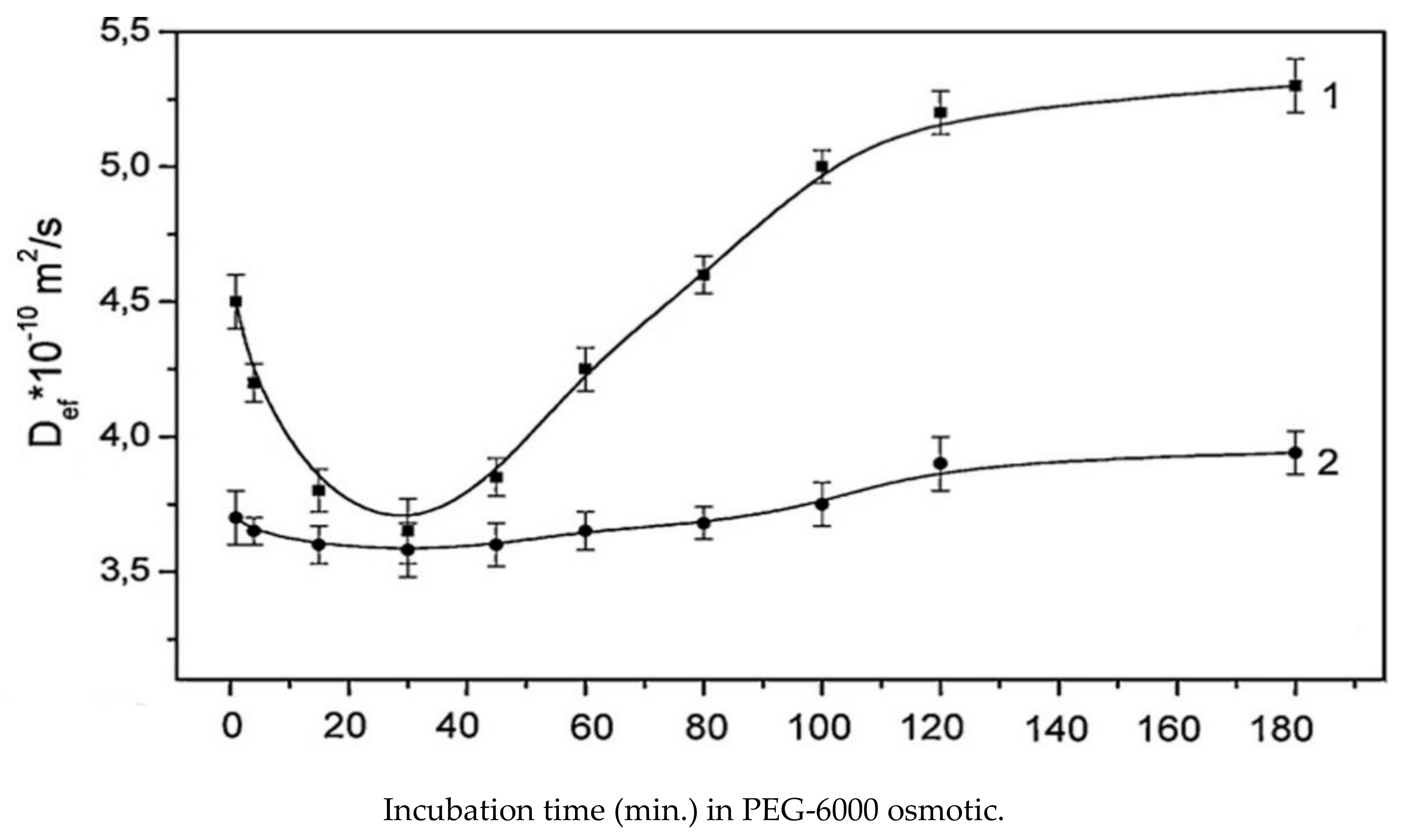
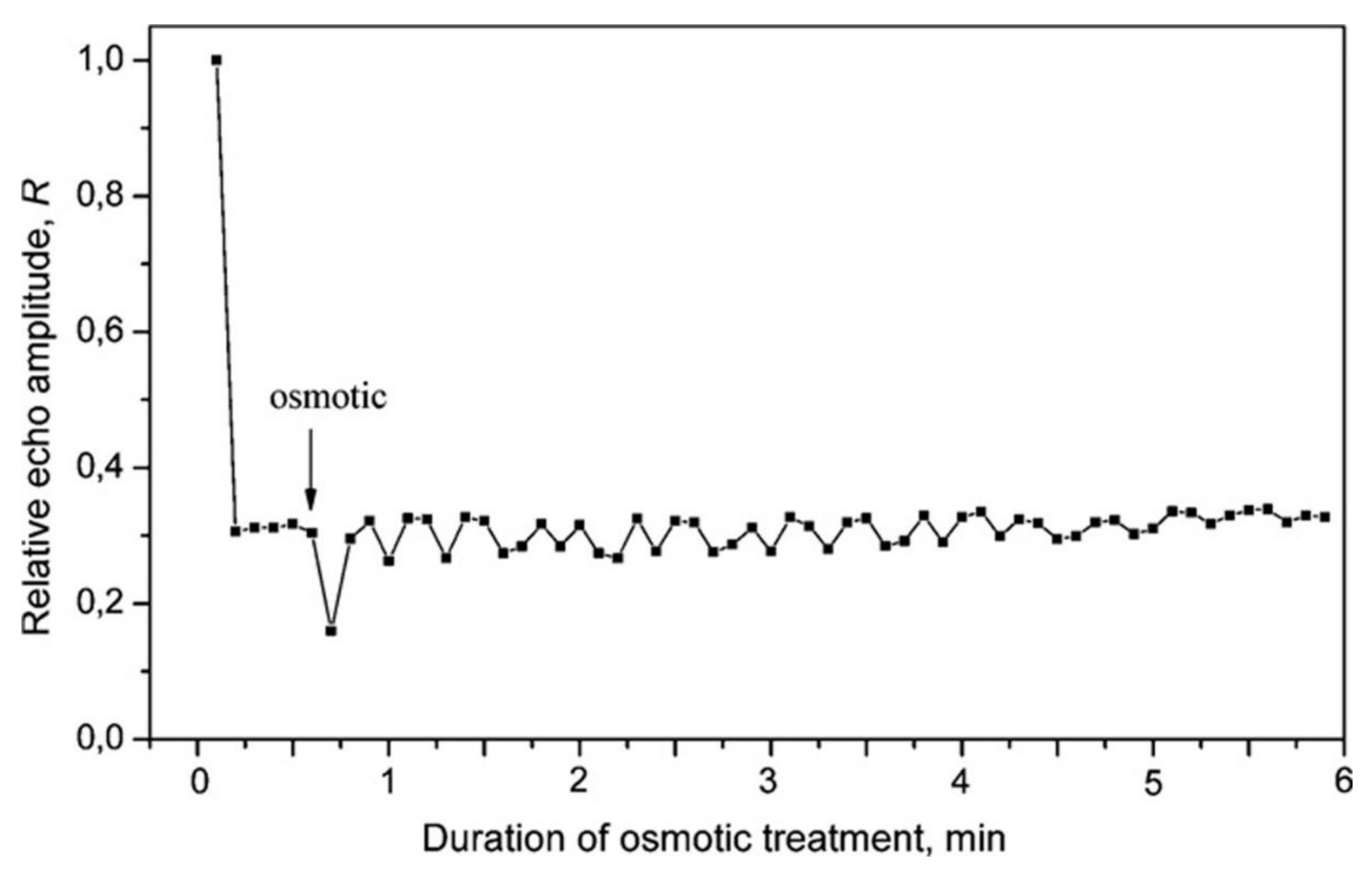


| Sample Treatment | Water Permeability, m/s |
|---|---|
| Control | (6.3 ± 0.3) × 10−5 |
| Excision of leaf zone | (5.7 ± 0,2) × 10−5 |
| Excision of meristem zone | (4.9 ± 0.25) × 10−5 |
| Fragmentation of segments into 3 mm long sections | (2.7 ± 0.3) × 10−5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anisimov, A. Gradient NMR Method for Studies of Water Translational Diffusion in Plants. Membranes 2021, 11, 487. https://doi.org/10.3390/membranes11070487
Anisimov A. Gradient NMR Method for Studies of Water Translational Diffusion in Plants. Membranes. 2021; 11(7):487. https://doi.org/10.3390/membranes11070487
Chicago/Turabian StyleAnisimov, Alexander. 2021. "Gradient NMR Method for Studies of Water Translational Diffusion in Plants" Membranes 11, no. 7: 487. https://doi.org/10.3390/membranes11070487
APA StyleAnisimov, A. (2021). Gradient NMR Method for Studies of Water Translational Diffusion in Plants. Membranes, 11(7), 487. https://doi.org/10.3390/membranes11070487





