Characterizing the Structure and Interactions of Model Lipid Membranes Using Electrophysiology
Abstract
1. Introduction
2. Model Membranes: Manufactures and Resulting Properties
2.1. Liposomes
2.2. Pore-Spanning Membranes
2.3. Solid Supported Membranes
2.4. Membranes Formed at The Interface of Immiscible Fluids
2.4.1. Droplet on Hydrogel Bilayers
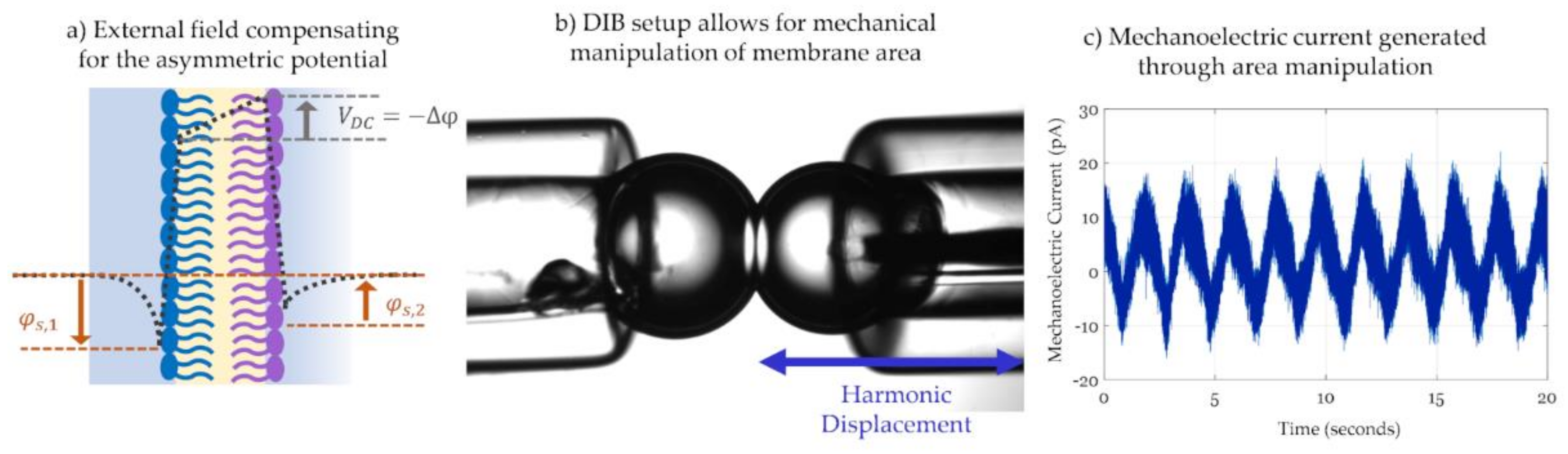
2.4.2. Droplet Interface Bilayers
3. Electrophysiological Methods for Characterizing Lipid Membranes
3.1. Conductance Measurements
3.2. Electrowetting and Electrocompression-Based Techniques
3.2.1. Dynamic Membrane Capacitance in Response to an Electric Field
3.2.2. Membrane Current Analysis and Attenuation Techniques
3.3. Electroimpedance Spectroscopy
4. Summary of Methods for Formation and Characterization
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lodish, H.; Berk, A. Molecular Cell Biology; Macmillan: New York, NY, USA, 2008; Volume 3. [Google Scholar]
- Grecco, H.E.; Schmick, M.; Bastiaens, P.I.J.C. Signaling from the living plasma membrane. Cell 2011, 144, 897–909. [Google Scholar] [CrossRef]
- Honigmann, A.; Pralle, A.J. Compartmentalization of the cell membrane. J. Mol. Biol. 2016, 428, 4739–4748. [Google Scholar] [CrossRef] [PubMed]
- Rothstein, A. Compartmentalization of the cell surface of yeast in relation to metabolic activities. Discuss. Faraday Soc. 1956, 21, 229–238. [Google Scholar] [CrossRef]
- Levental, I.; Levental, K.; Simons, K. The Differentiation of the Plasma Membrane; Federation of American Societies for Experimental Biology: Rockville, MD, USA, 2013. [Google Scholar]
- Sundelacruz, S.; Levin, M.; Kaplan, D.L. Role of membrane potential in the regulation of cell proliferation and differentiation. Stem Cell Rev. Rep. 2009, 5, 231–246. [Google Scholar] [CrossRef] [PubMed]
- Corre, I.; Niaudet, C.; Paris, F.J. Plasma membrane signaling induced by ionizing radiation. Mutat. Res. Rev. Mutat. Res. 2010, 704, 61–67. [Google Scholar] [CrossRef]
- Nagasawa, H.; Cremesti, A.; Kolesnick, R.; Fuks, Z.; Little, J.B. Involvement of membrane signaling in the bystander effect in irradiated cells. Cancer Res. 2002, 62, 2531–2534. [Google Scholar] [PubMed]
- Sharma, A.; Sharma, U.S. Liposomes in drug delivery: Progress and limitations. Int. J. Pharm. 1997, 154, 123–140. [Google Scholar] [CrossRef]
- Landon, C.D.; Park, J.-Y.; Needham, D.; Dewhirst, M.W. Nanoscale drug delivery and hyperthermia: The materials design and preclinical and clinical testing of low temperature-sensitive liposomes used in combination with mild hyperthermia in the treatment of local cancer. Open Nanomed. J. 2011, 3, 38. [Google Scholar] [CrossRef] [PubMed]
- Kamiya, K.J. Development of artificial cell models using microfluidic technology and synthetic biology. Micromachines 2020, 11, 559. [Google Scholar] [CrossRef]
- Bhattacharya, A.; Brea, R.J.; Niederholtmeyer, H.; Devaraj, N.K. A minimal biochemical route towards de novo formation of synthetic phospholipid membranes. Nat. Commun. 2019, 10, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Tien, H.; Ottova, A. Supported planar lipid bilayers (s-BLMs) as electrochemical biosensors. Electrochim. Acta 1998, 43, 3587–3610. [Google Scholar] [CrossRef]
- Castellana, E.T.; Cremer, P.S. Solid supported lipid bilayers: From biophysical studies to sensor design. Surf. Sci. Rep. 2006, 61, 429–444. [Google Scholar] [CrossRef]
- Jacobson, K.; Sheets, E.; Simson, R. Revisiting the fluid mosaic model of membranes. Science 1995, 268, 1441–1442. [Google Scholar] [CrossRef] [PubMed]
- Singer, S.J.; Nicolson, G.L. The Fluid Mosaic Model of the Structure of Cell Membranes. Science 1972, 175, 720–731. [Google Scholar] [CrossRef]
- Simons, K.; Vaz, W.L. Model Systems, Lipid Rafts, and Cell Membranes. Annu. Rev. Biophys. Biomol. Struct. 2004, 33, 269–295. [Google Scholar] [CrossRef] [PubMed]
- Fox, C.F. The Structure of Cell Membranes. Sci. Am. 1972, 226, 30–38. [Google Scholar] [CrossRef]
- Peetla, C.; Jin, S.; Weimer, J.; Elegbede, A.; Labhasetwar, V. Biomechanics and Thermodynamics of Nanoparticle Interactions with Plasma and Endosomal Membrane Lipids in Cellular Uptake and Endosomal Escape. Langmuir 2014, 30, 7522–7532. [Google Scholar] [CrossRef] [PubMed]
- Peetla, C.; Bhave, R.; Vijayaraghavalu, S.; Stine, A.; Kooijman, E.; Labhasetwar, V. Drug Resistance in Breast Cancer Cells: Biophysical Characterization of and Doxorubicin Interactions with Membrane Lipids. Mol. Pharm. 2010, 7, 2334–2348. [Google Scholar] [CrossRef]
- Wu, M.; Maier, E.; Benz, R.; Hancock, R.E. Mechanism of interaction of different classes of cationic antimicrobial peptides with planar bilayers and with the cytoplasmic membrane of Escherichia coli. Biochemistry 1999, 38, 7235–7242. [Google Scholar] [CrossRef]
- Soblosky, L.; Ramamoorthy, A.; Chen, Z. Membrane interaction of antimicrobial peptides using E. coli lipid extract as model bacterial cell membranes and SFG spectroscopy. Chem. Phys. Lipids 2015, 187, 20–33. [Google Scholar] [CrossRef] [PubMed]
- Wood, W.G.; Igbavboa, U.; Müller, W.E.; Eckert, G.P. Cholesterol asymmetry in synaptic plasma membranes. J. Neurochem. 2011, 116, 684–689. [Google Scholar] [CrossRef] [PubMed]
- Simons, K.; Toomre, D. Lipid rafts and signal transduction. Nat. Rev. Mol. Cell Biol. 2000, 1, 31–39. [Google Scholar] [CrossRef]
- Marquardt, D.; Geier, B.; Pabst, G. Asymmetric Lipid Membranes: Towards More Realistic Model Systems. Membreme 2015, 5, 180–196. [Google Scholar] [CrossRef]
- Aktas, M.; Danne, L.; Möller, P.; Narberhaus, F. Membrane lipids in Agrobacterium tumefaciens: Biosynthetic pathways and importance for pathogenesis. Front. Plant Sci. 2014, 5, 109. [Google Scholar] [CrossRef]
- Israelachvili, J.N.; Mitchell, D.; Ninham, B.W. Theory of self-assembly of lipid bilayers and vesicles. Biochim. Biophys. Acta Biomembr. 1977, 470, 185–201. [Google Scholar] [CrossRef]
- Goldman, D.E. Potential, impedance, and rectification in membranes. J. Gen. Physiol. 1943, 27, 37–60. [Google Scholar] [CrossRef] [PubMed]
- Hamill, O.P.; Marty, A.; Neher, E.; Sakmann, B.; Sigworth, F.J. Improved patch-clamp techniques for high-resolution current recording from cells and cell-free membrane patches. Pflügers Arch. Eur. J. Physiol. 1981, 391, 85–100. [Google Scholar] [CrossRef]
- Grewer, C.; Gameiro, A.; Mager, T.; Fendler, K. Electrophysiological Characterization of Membrane Transport Proteins. Annu. Rev. Biophys. 2013, 42, 95–120. [Google Scholar] [CrossRef] [PubMed]
- Mukherjee, P.; Nathamgari, S.S.P.; Kessler, J.A.; Espinosa, H.D. Combined Numerical and Experimental Investigation of Localized Electroporation-Based Cell Transfection and Sampling. ACS Nano 2018, 12, 12118–12128. [Google Scholar] [CrossRef]
- Siontorou, C.G.; Nikoleli, G.-P.; Nikolelis, D.P.; Karapetis, S.K. Artificial Lipid Membranes: Past, Present, and Future. Membreme 2017, 7, 38. [Google Scholar] [CrossRef]
- Khan, M.S.; Dosoky, N.S.; Williams, J.D. Engineering Lipid Bilayer Membranes for Protein Studies. Int. J. Mol. Sci. 2013, 14, 21561–21597. [Google Scholar] [CrossRef]
- Wu, L.; Jiang, X. Recent developments in methodology employed to study the interactions between nanomaterials and model lipid membranes. Anal. Bioanal. Chem. 2016, 408, 2743–2758. [Google Scholar] [CrossRef]
- Rascol, E.; Devoisselle, J.-M.; Chopineau, J. The relevance of membrane models to understand nanoparticles–cell membrane interactions. Nanoscale 2016, 8, 4780–4798. [Google Scholar] [CrossRef]
- Chatterjee, S.N.; Agarwal, S. Liposomes as membrane model for study of lipid peroxidation. Free Radic. Biol. Med. 1988, 4, 51–72. [Google Scholar] [CrossRef]
- Angelova, M.I.; Dimitrov, D.S. Liposome electroformation. Faraday Discuss. Chem. Soc. 1986, 81, 303–311. [Google Scholar] [CrossRef]
- Angelova, M.; Dimitrov, D.S. A mechanism of liposome electroformation. In Trends in Colloid and Interface Science II; Springer: Berlin/Heidelberg, Germany, 1988; pp. 59–67. [Google Scholar]
- Collins, M.D.; Gordon, S.E. Giant liposome preparation for imaging and patch-clamp electrophysiology. J. Vis. Exp. 2013, 76, e50227. [Google Scholar] [CrossRef] [PubMed]
- Pautot, S.; Frisken, B.J.; Weitz, D.A. Production of Unilamellar Vesicles Using an Inverted Emulsion. Langmuir 2003, 19, 2870–2879. [Google Scholar] [CrossRef]
- Ohno, M.; Hamada, T.; Takiguchi, K.; Homma, M. Dynamic Behavior of Giant Liposomes at Desired Osmotic Pressures. Langmuir 2009, 25, 11680–11685. [Google Scholar] [CrossRef] [PubMed]
- Ip, T.; Li, Q.; Brooks, N.; Elani, Y. Manufacture of multi-layered artificial cell membranes through sequential bilayer deposition on emulsion templates. ChemBioChem 2021. [Google Scholar] [CrossRef]
- Stachowiak, J.C.; Richmond, D.L.; Li, T.H.; Liu, A.P.; Parekh, S.H.; Fletcher, D.A. Unilamellar vesicle formation and encapsulation by microfluidic jetting. Proc. Natl. Acad. Sci. USA 2008, 105, 4697–4702. [Google Scholar] [CrossRef]
- Kamiya, K.; Kawano, R.; Osaki, T.; Akiyoshi, K.; Takeuchi, S. Cell-sized asymmetric lipid vesicles facilitate the investigation of asymmetric membranes. Nat. Chem. 2016, 8, 881–889. [Google Scholar] [CrossRef] [PubMed]
- Milardi, D.; Gazit, E.; Radford, S.E.; Xu, Y.; Gallardo, R.U.; Caflisch, A.; Westermark, G.T.; Westermark, P.; La Rosa, C.; Ramamoorthy, A. Proteostasis of Islet Amyloid Polypeptide: A Molecular Perspective of Risk Factors and Protective Strategies for Type II Diabetes. Chem. Rev. 2021, 121, 1845–1893. [Google Scholar] [CrossRef]
- Elani, Y.; Gee, A.; Law, R.V.; Ces, O. Engineering multi-compartment vesicle networks. Chem. Sci. 2013, 4, 3332–3338. [Google Scholar] [CrossRef]
- Elani, Y.; Purushothaman, S.; Booth, P.J.; Seddon, J.M.; Brooks, N.J.; Law, R.V.; Ces, O. Measurements of the effect of membrane asymmetry on the mechanical properties of lipid bilayers. Chem. Commun. 2015, 51, 6976–6979. [Google Scholar] [CrossRef]
- Karamdad, K.; Hindley, J.W.; Bolognesi, G.; Friddin, M.S.; Law, R.V.; Brooks, N.J.; Ces, O.; Elani, Y. Engineering thermoresponsive phase separated vesicles formed via emulsion phase transfer as a content-release platform. Chem. Sci. 2018, 9, 4851–4858. [Google Scholar] [CrossRef]
- Nasr, G.; Greige-Gerges, H.; Elaissari, A.; Khreich, N. Liposomal membrane permeability assessment by fluorescence techniques: Main permeabilizing agents, applications and challenges. Int. J. Pharm. 2020, 580, 119198. [Google Scholar] [CrossRef] [PubMed]
- Papahadjopoulos, D.; Nir, S.; Ohki, S. Permeability properties of phospholipid membranes: Effect of cholesterol and temperature. Biochim. Biophys. Acta Biomembr. 1972, 266, 561–583. [Google Scholar] [CrossRef]
- Fernandez-Puente, L.; Bivas, I.; Mitov, M.D.; Méléard, P. Temperature and Chain Length Effects on Bending Elasticity of Phosphatidylcholine Bilayers. Europhys. Lett. 1994, 28, 181–186. [Google Scholar] [CrossRef]
- Chaurasia, A.K.; Rukangu, A.M.; Philen, M.K.; Seidel, G.D.; Freeman, E.C. Evaluation of bending modulus of lipid bilayers using undulation and orientation analysis. Phys. Rev. E 2018, 97, 032421. [Google Scholar] [CrossRef]
- Schulz, M.; Olubummo, A.; Binder, W.H. Beyond the lipid-bilayer: Interaction of polymers and nanoparticles with membranes. Soft Matter 2012, 8, 4849–4864. [Google Scholar] [CrossRef]
- Zhang, S.; Nelson, A.; Beales, P.A. Freezing or Wrapping: The Role of Particle Size in the Mechanism of Nanoparticle–Biomembrane Interaction. Langmuir 2012, 28, 12831–12837. [Google Scholar] [CrossRef] [PubMed]
- Chen, K.L.; Bothun, G.D. Nanoparticles Meet Cell Membranes: Probing Nonspecific Interactions Using Model Membranes; ACS Publications: Washington, DC, USA, 2014. [Google Scholar]
- Strobl, F.G.; Seitz, F.; Westerhausen, C.; Reller, A.; Torrano, A.A.; Bräuchle, C.; Wixforth, A.; Schneider, M.F. Intake of silica nanoparticles by giant lipid vesicles: Influence of particle size and thermodynamic membrane state. Beilstein J. Nanotechnol. 2014, 5, 2468–2478. [Google Scholar] [CrossRef]
- Koning, G.A.; Eggermont, A.M.; Lindner, L.H.; Ten Hagen, T.L. Hyperthermia and thermosensitive liposomes for improved delivery of chemotherapeutic drugs to solid tumors. Pharm. Res. 2010, 27, 1750–1754. [Google Scholar] [CrossRef]
- Mayhew, E. Liposomes in Cancer. In “Liposome Letters”, AD Bangham; Academic Press: London, UK, 1983. [Google Scholar]
- Chen, Z.-J.; Yang, S.-C.; Liu, X.-L.; Gao, Y.; Dong, X.; Lai, X.; Zhu, M.-H.; Feng, H.-Y.; Zhu, X.-D.; Lu, Q.; et al. Nanobowl-Supported Liposomes Improve Drug Loading and Delivery. Nano Lett. 2020, 20, 4177–4187. [Google Scholar] [CrossRef] [PubMed]
- Kumar, B.; Pandey, M.; Pottoo, F.H.; Fayaz, F.; Sharma, A.; Sahoo, P. Liposomes: Novel Drug Delivery Approach for Targeting Parkinson’s Disease. Curr. Pharm. Des. 2020, 26, 4721–4737. [Google Scholar] [CrossRef] [PubMed]
- Kim, C.H.; Lee, S.G.; Kang, M.J.; Lee, S.; Choi, Y.W. Surface modification of lipid-based nanocarriers for cancer cell-specific drug targeting. J. Pharm. Investig. 2017, 47, 203–227. [Google Scholar] [CrossRef]
- Mady, M.M.; Fathy, M.M.; Youssef, T.; Khalil, W.M. Biophysical characterization of gold nanoparticles-loaded liposomes. Phys. Med. 2012, 28, 288–295. [Google Scholar] [CrossRef]
- Jølck, R.I.; Feldborg, L.N.; Andersen, S.; Moghimi, S.M.; Andresen, T.L. Engineering liposomes and nanoparticles for biological targeting. In Biofunctionalization of Polymers and their Applications; Springer: Berlin/Heidelberg, Germany, 2010; pp. 251–280. [Google Scholar]
- Tai, L.-A.; Tsai, P.-J.; Wang, Y.-C.; Lo, L.-W.; Yang, C.-S.; Wang, Y.-J. Thermosensitive liposomes entrapping iron oxide nanoparticles for controllable drug release. Nanotechnology 2009, 20, 135101. [Google Scholar] [CrossRef] [PubMed]
- Faizi, H.A.; Dimova, R.; Vlahovska, P.M. Viscosity of fluid membranes measured from vesicle deformation. arXiv 2021, arXiv:2103.02106. [Google Scholar]
- Correa, N.M.; Schelly, Z.A. Dynamics of Electroporation of Synthetic Liposomes Studied Using a Pore-Mediated Reaction, Ag++ Br-→ AgBr. J. Phys. Chem. B 1998, 102, 9319–9322. [Google Scholar] [CrossRef]
- Kolahdouz, E.M.; Salac, D. Dynamics of three-dimensional vesicles in dc electric fields. Phys. Rev. E 2015, 92, 012302. [Google Scholar] [CrossRef]
- Nomura, T.; Cox, C.D.; Bavi, N.; Sokabe, M.; Martinac, B. Unidirectional incorporation of a bacterial mechanosensitive channel into liposomal membranes. FASEB J. 2015, 29, 4334–4345. [Google Scholar] [CrossRef] [PubMed]
- Mueller, P.; Rudin, D.O.; Tien, H.T.; Wescott, W.C. Reconstitution of Cell Membrane Structure in vitro and its Transformation into an Excitable System. Nat. Cell Biol. 1962, 194, 979–980. [Google Scholar] [CrossRef]
- Montal, M.; Mueller, P. Formation of bimolecular membranes from lipid monolayers and a study of their electrical properties. Proc. Natl. Acad. Sci. USA 1972, 69, 3561–3566. [Google Scholar] [CrossRef] [PubMed]
- Fuks, B.; Homblé, F. Permeability and electrical properties of planar lipid membranes from thylakoid lipids. Biophys. J. 1994, 66, 1404–1414. [Google Scholar] [CrossRef][Green Version]
- Beltramo, P.J.; Scheidegger, L.; Vermant, J. Toward Realistic Large-Area Cell Membrane Mimics: Excluding Oil, Controlling Composition, and Including Ion Channels. Langmuir 2018, 34, 5880–5888. [Google Scholar] [CrossRef]
- Gross, L.C.; Heron, A.J.; Baca, S.C.; Wallace, M.I. Determining membrane capacitance by dynamic control of droplet interface bilayer area. Langmuir 2011, 27, 14335–14342. [Google Scholar] [CrossRef]
- Batishchev, O.V.; Indenbom, A.V. Alkylated glass partition allows formation of solvent-free lipid bilayer by Montal–Mueller technique. Bioelectrochemistry 2008, 74, 22–25. [Google Scholar] [CrossRef]
- White, S.H. Temperature-dependent structural changes in planar bilayer membranes: Solvent “freeze-out”. Biochim. Biophys. Acta 1974, 356, 8–16. [Google Scholar] [CrossRef]
- White, S.J. Formation of” solvent-free” black lipid bilayer membranes from glyceryl monooleate dispersed in squalene. Biophys. J. 1978, 23, 337–347. [Google Scholar] [CrossRef]
- Neher, E. Asymmetric membranes resulting from the fusion of two black lipid bilayers. Biochim. Biophys. Acta Biomembr. 1974, 373, 327–336. [Google Scholar] [CrossRef]
- Wiese, A.; Reiners, J.; Brandenburg, K.; Kawahara, K.; Zähringer, U.; Seydel, U. Planar asymmetric lipid bilayers of glycosphingolipid or lipopolysaccharide on one side and phospholipids on the other: Membrane potential, porin function, and complement activation. Biophys. J. 1996, 70, 321–329. [Google Scholar] [CrossRef]
- Van Gelder, P.; Dumas, F.; Winterhalter, M. Understanding the function of bacterial outer membrane channels by reconstitution into black lipid membranes. Biophys. Chem. 2000, 85, 153–167. [Google Scholar] [CrossRef]
- Sun, Y.; Zang, X.; Sun, Y.; Wang, L.; Gao, Z.J.C. Lipid membranes supported by planar porous substrates. Chem. Phys. Lipids 2020, 228, 104893. [Google Scholar] [CrossRef] [PubMed]
- Beltramo, P.J.; Van Hooghten, R.; Vermant, J. Millimeter-area, free standing, phospholipid bilayers. Soft Matter 2016, 12, 4324–4331. [Google Scholar] [CrossRef] [PubMed]
- Liu, P.; Zabala-Ferrera, O.; Beltramo, P.J. Fabrication and electromechanical characterization of free-standing asymmetric membranes. Biophys. J. 2021. [Google Scholar] [CrossRef] [PubMed]
- Jeon, T.-J.; Malmstadt, N.; Poulos, J.L.; Schmidt, J.J. Black lipid membranes stabilized through substrate conjugation to a hydrogel. Biointerphases 2008, 3, FA96–FA100. [Google Scholar] [CrossRef]
- Gomez-Lagunas, F.; Pena, A.; Lièvano, A.; Darszon, A. Incorporation of ionic channels from yeast plasma membranes into black lipid membranes. Biophys. J. 1989, 56, 115–119. [Google Scholar] [CrossRef]
- Geng, J.; Kim, K.; Zhang, J.; Escalada, A.; Tunuguntla, R.; Comolli, L.R.; Allen, F.I.; Shnyrova, A.V.; Cho, K.R.; Munoz, D.; et al. Stochastic transport through carbon nanotubes in lipid bilayers and live cell membranes. Nat. Cell Biol. 2014, 514, 612–615. [Google Scholar] [CrossRef]
- Groves, J.T.; Ulman, N.; Boxer, S.G. Micropatterning Fluid Lipid Bilayers on Solid Supports. Science 1997, 275, 651–653. [Google Scholar] [CrossRef] [PubMed]
- Su, H.; Liu, H.-Y.; Pappa, A.-M.; Hidalgo, T.C.; Cavassin, P.; Inal, S.; Owens, R.M.; Daniel, S. Facile Generation of Biomimetic-Supported Lipid Bilayers on Conducting Polymer Surfaces for Membrane Biosensing. ACS Appl. Mater. Interfaces 2019, 11, 43799–43810. [Google Scholar] [CrossRef] [PubMed]
- Tamm, L.K.; McConnell, H.M. Supported phospholipid bilayers. Biophys. J. 1985, 47, 105–113. [Google Scholar] [CrossRef]
- Reimhult, E.; Höök, A.F.; Kasemo, B. Intact Vesicle Adsorption and Supported Biomembrane Formation from Vesicles in Solution: Influence of Surface Chemistry, Vesicle Size, Temperature, and Osmotic Pressure†. Langmuir 2003, 19, 1681–1691. [Google Scholar] [CrossRef]
- Kalb, E.; Frey, S.; Tamm, L.K. Formation of supported planar bilayers by fusion of vesicles to supported phospholipid monolayers. Biochim. Biophys. Acta Biomembr. 1992, 1103, 307–316. [Google Scholar] [CrossRef]
- Zasadzinski, J.; Helm, C.; Longo, M.; Weisenhorn, A.; Gould, S.; Hansma, P. Atomic force microscopy of hydrated phosphatidylethanolamine bilayers. Biophys. J. 1991, 59, 755–760. [Google Scholar] [CrossRef]
- Egawa, H.; Furusawa, K. Liposome Adhesion on Mica Surface Studied by Atomic Force Microscopy. Langmuir 1999, 15, 1660–1666. [Google Scholar] [CrossRef]
- Bunjes, N.; Schmidt, E.K.; Jonczyk, A.; Rippmann, F.; Beyer, D.; Ringsdorf, H.; Gräber, P.; Knoll, W.; Naumann, R. Thiopeptide-Supported Lipid Layers on Solid Substrates. Langmuir 1997, 13, 6188–6194. [Google Scholar] [CrossRef]
- Lahiri, J.; Kalal, P.; Frutos, A.G.; Jonas, S.J.; Schaeffler, R. Method for Fabricating Supported Bilayer Lipid Membranes on Gold. Langmuir 2000, 16, 7805–7810. [Google Scholar] [CrossRef]
- Salamon, Z.; Wang, Y.; Tollin, G.; Macleod, H. Assembly and molecular organization of self-assembled lipid bilayers on solid substrates monitored by surface plasmon resonance spectroscopy. Biochim. Biophys. Acta Biomembr. 1994, 1195, 267–275. [Google Scholar] [CrossRef]
- Lagerholm, B.C.; Starr, T.E.; Volovyk, Z.N.; Thompson, N.L. Rebinding of IgE Fabs at Haptenated Planar Membranes: Measurement by Total Internal Reflection with Fluorescence Photobleaching Recovery. Biochemistry 2000, 39, 2042–2051. [Google Scholar] [CrossRef]
- Cremer, P.S.; Boxer, S.G. Formation and spreading of lipid bilayers on planar glass supports. J. Phys. Chem. B 1999, 103, 2554–2559. [Google Scholar] [CrossRef]
- Hillebrandt, H.; Wiegand, G.; Tanaka, M.; Sackmann, E. High Electric Resistance Polymer/Lipid Composite Films on Indium− Tin− Oxide Electrodes. Langmuir 1999, 15, 8451–8459. [Google Scholar] [CrossRef]
- Gritsch, S.; Nollert, P.; Jähnig, F.; Sackmann, E. Impedance Spectroscopy of Porin and Gramicidin Pores Reconstituted into Supported Lipid Bilayers on Indium−Tin-Oxide Electrodes. Langmuir 1998, 14, 3118–3125. [Google Scholar] [CrossRef]
- Johnson, S.; Bayerl, T.; McDermott, D.; Adam, G.; Rennie, A.; Thomas, R.; Sackmann, E. Structure of an adsorbed dimyristoylphosphatidylcholine bilayer measured with specular reflection of neutrons. Biophys. J. 1991, 59, 289–294. [Google Scholar] [CrossRef]
- Kühner, M.; Tampe, R.; Sackmann, E. Lipid mono- and bilayer supported on polymer films: Composite polymer-lipid films on solid substrates. Biophys. J. 1994, 67, 217–226. [Google Scholar] [CrossRef]
- Sackmann, E.; Tanaka, M.J. Supported membranes on soft polymer cushions: Fabrication, characterization and applications. Trends Biotechnol. 2000, 18, 58–64. [Google Scholar] [CrossRef]
- Wagner, M.L.; Tamm, L.K. Tethered polymer-supported planar lipid bilayers for reconstitution of integral membrane proteins: Silane-polyethyleneglycol-lipid as a cushion and covalent linker. Biophys. J. 2000, 79, 1400–1414. [Google Scholar] [CrossRef]
- Renner, L.; Pompe, T.; Lemaitre, R.; Drechsel, D.; Werner, C. Controlled enhancement of transmembrane enzyme activity in polymer cushioned supported bilayer membranes. Soft Matter 2010, 6, 5382–5389. [Google Scholar] [CrossRef]
- Wong, J.; Majewski, J.; Seitz, M.; Park, C.; Israelachvili, J.; Smith, G. Polymer-Cushioned Bilayers. I. A Structural Study of Various Preparation Methods Using Neutron Reflectometry. Biophys. J. 1999, 77, 1445–1457. [Google Scholar] [CrossRef]
- Cornell, B.A.; Braach-Maksvytis, V.L.B.; King, L.G.; Osman, P.D.J.; Raguse, B.; Wieczorek, L.; Pace, R.J. A biosensor that uses ion-channel switches. Nat. Cell Biol. 1997, 387, 580–583. [Google Scholar] [CrossRef] [PubMed]
- Rossi, C.; Chopineau, J. Biomimetic tethered lipid membranes designed for membrane-protein interaction studies. Eur. Biophys. J. 2007, 36, 955–965. [Google Scholar] [CrossRef]
- Majewski, J.; Wong, J.; Park, C.; Seitz, M.; Israelachvili, J.; Smith, G. Structural Studies of Polymer-Cushioned Lipid Bilayers. Biophys. J. 1998, 75, 2363–2367. [Google Scholar] [CrossRef]
- Zhang, L.; Longo, M.L.; Stroeve, P. Mobile Phospholipid Bilayers Supported on a Polyion/Alkylthiol Layer Pair. Langmuir 2000, 16, 5093–5099. [Google Scholar] [CrossRef]
- Naumann, C.A.; Prucker, O.; Lehmann, T.; Rühe, J.; Knoll, W.; Frank, C.W. The Polymer-Supported Phospholipid Bilayer: Tethering as a New Approach to Substrate−Membrane Stabilization. Biomacromolecules 2001, 3, 27–35. [Google Scholar] [CrossRef]
- Vockenroth, I.K.; Ohm, C.; Robertson, J.W.F.; McGillivray, D.J.; Lösche, M.; Köper, I. Stable insulating tethered bilayer lipid membranes. Biointerphases 2008, 3, FA68–FA73. [Google Scholar] [CrossRef] [PubMed]
- Vockenroth, I.K.; Fine, D.; Dodobalapur, A.; Jenkins, A.T.A.; Köper, I. Tethered bilayer lipid membranes with giga-ohm resistances. Electrochem. Commun. 2008, 10, 323–328. [Google Scholar] [CrossRef]
- Andersson, M.; Keizer, H.M.; Zhu, C.; Fine, D.; Dodabalapur, A.; Duran, R.S. Detection of Single Ion Channel Activity on a Chip Using Tethered Bilayer Membranes. Langmuir 2007, 23, 2924–2927. [Google Scholar] [CrossRef]
- Becucci, L.; D’Amico, M.; Cinotti, S.; Daniele, S.; Guidelli, R. Tethered bilayer lipid micromembranes for single-channel recording: The role of adsorbed and partially fused lipid vesicles. Phys. Chem. Chem. Phys. 2011, 13, 13341–13348. [Google Scholar] [CrossRef]
- Zhang, Y.; Inal, S.; Hsia, C.; Ferro, M.; Ferro, M.; Daniel, S.; Owens, R.M. Supported Lipid Bilayer Assembly on PEDOT:PSS Films and Transistors. Adv. Funct. Mater. 2016, 26, 7304–7313. [Google Scholar] [CrossRef]
- Tanaka, M.; Sackmann, E. Polymer-supported membranes as models of the cell surface. Nat. Cell Biol. 2005, 437, 656–663. [Google Scholar] [CrossRef] [PubMed]
- Stelzle, M.; Weissmueller, G.; Sackmann, E. On the application of supported bilayers as receptive layers for biosensors with electrical detection. J. Phys. Chem. 1993, 97, 2974–2981. [Google Scholar] [CrossRef]
- Kibrom, A.; Roskamp, R.F.; Jonas, U.; Menges, B.; Knoll, W.; Paulsen, H.; Naumann, R.L.C. Hydrogel-supported protein-tethered bilayer lipid membranes: A new approach toward polymer-supported lipid membranes. Soft Matter 2011, 7, 237–246. [Google Scholar] [CrossRef]
- Ren, X.; Kumbur, E.C.; Zhou, J.G.; Noh, M.; Chong, P.L.-G. Stability of free-standing tetraether planar membranes in microchips. J. Membr. Sci. 2017, 540, 27–34. [Google Scholar] [CrossRef]
- Coronado, R.; Latorre, R. Phospholipid bilayers made from monolayers on patch-clamp pipettes. Biophys. J. 1983, 43, 231–236. [Google Scholar] [CrossRef]
- Matsuno, Y.; Osono, C.; Hirano, A.; Sugawara, M. Single-Channel Recordings of Gramicidin at Agarose-Supported Bilayer Lipid Membranes Formed by the Tip-Dip and Painting Methods. Anal. Sci. 2004, 20, 1217–1221. [Google Scholar] [CrossRef]
- Shoji, K.; Kawano, R.; White, R.J. Recessed Ag/AgCl Microelectrode-Supported Lipid Bilayer for Nanopore Sensing. Anal. Chem. 2020, 92, 10856–10862. [Google Scholar] [CrossRef]
- Okuno, D.; Hirano, M.; Yokota, H.; Onishi, Y.; Ichinose, J.; Ide, T. A Simple Method for Ion Channel Recordings Using Fine Gold Electrode. Anal. Sci. 2016, 32, 1353–1357. [Google Scholar] [CrossRef] [PubMed]
- Shoji, K.; Kawano, R.; White, R.J. Spatially Resolved Chemical Detection with a Nanoneedle-Probe-Supported Biological Nanopore. ACS Nano 2019, 13, 2606–2614. [Google Scholar] [CrossRef]
- Hirano, M.; Yamamoto, D.; Asakura, M.; Hayakawa, T.; Mise, S.; Matsumoto, A.; Ide, T. A Lipid Bilayer Formed on a Hydrogel Bead for Single Ion Channel Recordings. Micromachines 2020, 11, 1070. [Google Scholar] [CrossRef]
- Challita, E.J.; Freeman, E.C. Hydrogel Microelectrodes for the Rapid, Reliable, and Repeatable Characterization of Lipid Membranes. Langmuir 2018, 34, 15166–15173. [Google Scholar] [CrossRef]
- Tsofina, L.M.; Liberman, E.A.; Babakov, A.V. Production of Bimolecular Protein-Lipid Membranes in Aqueous Solution. Nat. Cell Biol. 1966, 212, 681–683. [Google Scholar] [CrossRef]
- Tien, H.T. Bilayer Lipid Membranes (BLM): Theory and Practice; M. Dekker: New York, NY, USA, 1974. [Google Scholar]
- Seddon, J.; Templer, R. Polymorphism of lipid-water systems. In Handbook of Biological Physics; Elsevier: Amsterdam, The Netherlands, 1995; Volume 1, pp. 97–160. [Google Scholar]
- Thompson, J.R.; Heron, A.J.; Santoso, Y.; Wallace, M.I. Enhanced stability and fluidity in droplet on hydrogel bilayers for measuring membrane protein diffusion. Nano Lett. 2007, 7, 3875–3878. [Google Scholar] [CrossRef] [PubMed]
- Heron, A.J.; Thompson, J.R.; Mason, A.E.; Wallace, M.I. Direct detection of membrane channels from gels using water-in-oil droplet bilayers. J. Am. Chem. Soc. 2007, 129, 16042–16047. [Google Scholar] [CrossRef]
- Leptihn, S.; Castell, O.K.; Cronin, B.; Lee, E.-H.; Gross, L.C.M.; Marshall, D.P.; Thompson, J.R.; Holden, M.A.; Wallace, M.I. Constructing droplet interface bilayers from the contact of aqueous droplets in oil. Nat. Protoc. 2013, 8, 1048–1057. [Google Scholar] [CrossRef]
- Bayley, H.; Cronin, B.; Heron, A.; Holden, M.A.; Hwang, W.L.; Syeda, R.; Thompson, J.; Wallace, M. Droplet interface bilayers. Mol. Biosyst. 2008, 4, 1191–1208. [Google Scholar] [CrossRef]
- Funakoshi, K.; Suzuki, H.; Takeuchi, S. Lipid bilayer formation by contacting monolayers in a microfluidic device for membrane protein analysis. Anal. Chem. 2006, 78, 8169–8174. [Google Scholar] [CrossRef]
- Poulin, P.; Bibette, J. Adhesion of water droplets in organic solvent. Langmuir 1998, 14, 6341–6343. [Google Scholar] [CrossRef]
- El-Beyrouthy, J.; Makhoul-Mansour, M.M.; Taylor, G.; Sarles, S.A.; Freeman, E.C. A new approach for investigating the response of lipid membranes to electrocompression by coupling droplet mechanics and membrane biophysics. J. Royal Soc. Interface 2019, 16, 20190652. [Google Scholar] [CrossRef] [PubMed]
- Taylor, G.J.; Venkatesan, G.A.; Collier, C.P.; Sarles, S.A. Direct in situ measurement of specific capacitance, monolayer tension, and bilayer tension in a droplet interface bilayer. Soft Matter 2015, 11, 7592–7605. [Google Scholar] [CrossRef] [PubMed]
- Taylor, G.; Nguyen, M.-A.; Koner, S.; Freeman, E.; Collier, C.P.; Sarles, S.A. Electrophysiological interrogation of asymmetric droplet interface bilayers reveals surface-bound alamethicin induces lipid flip-flop. Biochim. Biophys. Acta Biomembr. 2018, 1861, 335–343. [Google Scholar] [CrossRef] [PubMed]
- Makhoul-Mansour, M.; Zhao, W.; Gay, N.; O’Connor, C.; Najem, J.S.; Mao, L.; Freeman, E.C. Ferrofluid-Based Droplet Interface Bilayer Networks. Langmuir 2017, 33, 13000–13007. [Google Scholar] [CrossRef]
- Makhoul-Mansour, M.M.; Freeman, E.C. Droplet-Based Membranous Soft Materials. Langmuir 2021, 37, 3231–3247. [Google Scholar] [CrossRef] [PubMed]
- Korner, J.L.; Stephenson, E.B.; Elvira, K.S. A bespoke microfluidic pharmacokinetic compartment model for drug absorption using artificial cell membranes. Lab Chip 2020, 20, 1898–1906. [Google Scholar] [CrossRef]
- Berry, J.D.; Neeson, M.J.; Dagastine, R.R.; Chan, D.Y.; Tabor, R.F. Measurement of surface and interfacial tension using pendant drop tensiometry. J. Colloid Interface Sci. 2015, 454, 226–237. [Google Scholar] [CrossRef]
- Rofeh, J.; Theogarajan, L. Instantaneous tension measurements in droplet interface bilayers using an inexpensive, integrated pendant drop camera. Soft Matter 2020, 16, 4484–4493. [Google Scholar] [CrossRef]
- Schoch, P.; Sargent, D.F.; Schwyzer, R. Capacitance and conductance as tools for the measurement of asymmetric surface potentials and energy barriers of lipid bilayer membranes. J. Membr. Biol. 1979, 46, 71–89. [Google Scholar] [CrossRef] [PubMed]
- Alvarez, O.; Latorre, R. Voltage-dependent capacitance in lipid bilayers made from monolayers. Biophys. J. 1978, 21, 1–17. [Google Scholar] [CrossRef]
- Cevc, G. Membrane electrostatics. Biochim. Biophys. Acta Rev. Biomembr. 1990, 1031, 311–382. [Google Scholar] [CrossRef]
- Huang, W.; Levitt, D. Theoretical calculation of the dielectric constant of a bilayer membrane. Biophys. J. 1977, 17, 111–128. [Google Scholar] [CrossRef]
- Toyama, S.; Nakamura, A.; Toda, F. Measurement of voltage dependence of capacitance of planar bilayer lipid membrane with a patch clamp amplifier. Biophys. J. 1991, 59, 939–944. [Google Scholar] [CrossRef][Green Version]
- Muller, N.; Birkhahn, R.H. Investigation of micelle structure by fluorine magnetic resonance. I. Sodium 10, 10, 10-trifluorocaprate and related compounds. J. Phys. Chem. 1967, 71, 957–962. [Google Scholar] [CrossRef]
- Sekiya, Y.; Shimizu, K.; Kitahashi, Y.; Ohyama, A.; Kawamura, I.; Kawano, R. Electrophysiological Analysis of Membrane Disruption by Bombinin and Its Isomer Using the Lipid Bilayer System. ACS Appl. Bio Mater. 2019, 2, 1542–1548. [Google Scholar] [CrossRef]
- Cherny, V.; Sokolov, V.S.; Abidor, I.G. 330-Determination of surface charge of bilayer lipid membranes. Bioelectrochemistry Bioenerg. 1980, 7, 413–420. [Google Scholar] [CrossRef]
- Brockman, H. Dipole potential of lipid membranes. Chem. Phys. Lipids 1994, 73, 57–79. [Google Scholar] [CrossRef]
- Clarke, R.J. The dipole potential of phospholipid membranes and methods for its detection. Adv. Colloid Interface Sci. 2001, 89, 263–281. [Google Scholar] [CrossRef]
- Wang, L. Measurements and implications of the membrane dipole potential. Annu. Rev. Biochem. 2012, 81, 615–635. [Google Scholar] [CrossRef]
- Makhoul-Mansour, M.M.; El-Beyrouthy, J.B.; Mumme, H.L.; Freeman, E.C. Photopolymerized microdomains in both lipid leaflets establish diffusive transport pathways across biomimetic membranes. Soft Matter 2019, 15, 8718–8727. [Google Scholar] [CrossRef] [PubMed]
- Bergeron, V. Forces and structure in thin liquid soap films. J. Physics Condens. Matter 1999, 11, R215–R238. [Google Scholar] [CrossRef]
- Dey, R.; DasGupta, S.; Chakraborty, S. Electrowetting of sessile drops on soft dielectric elastomer films. Microfluid. Nanofluidics 2017, 21. [Google Scholar] [CrossRef]
- Najem, J.S.; Dunlap, M.D.; Rowe, I.D.; Freeman, E.C.; Grant, J.W.; Sukharev, S.; Leo, D.J. Activation of bacterial channel MscL in mechanically stimulated droplet interface bilayers. Sci. Rep. 2015, 5, 13726. [Google Scholar] [CrossRef]
- Moghaddam, M.M.; Aghamollaei, H.; Kooshki, H.; Barjini, K.A.; Mirnejad, R.; Choopani, A. The development of antimicrobial peptides as an approach to prevention of antibiotic resistance. Rev. Med. Microbiol. 2015, 26, 98–110. [Google Scholar] [CrossRef]
- Jenssen, H.; Hamill, P.; Hancock, R.E. Peptide antimicrobial agents. Clin. Microbiol. Rev. 2006, 19, 491–511. [Google Scholar] [CrossRef]
- Hancock, R.E.; Rozek, A. Role of membranes in the activities of antimicrobial cationic peptides. FEMS Microbiol. Lett. 2002, 206, 143–149. [Google Scholar] [CrossRef] [PubMed]
- Christensen, B.; Fink, J.; Merrifield, R.B.; Mauzerall, D. Channel-forming properties of cecropins and related model compounds incorporated into planar lipid membranes. Proc. Natl. Acad. Sci. USA 1988, 85, 5072–5076. [Google Scholar] [CrossRef]
- Kagan, B.L.; Selsted, M.E.; Ganz, T.; Lehrer, R.I. Antimicrobial defensin peptides form voltage-dependent ion-permeable channels in planar lipid bilayer membranes. Proc. Natl. Acad. Sci. USA 1990, 87, 210–214. [Google Scholar] [CrossRef] [PubMed]
- Coronado, R.; Rosenberg, R.L.; Miller, C. Ionic selectivity, saturation, and block in a K+-selective channel from sarcoplasmic reticulum. J. Gen. Physiol. 1980, 76, 425–446. [Google Scholar] [CrossRef]
- Ashrafuzzaman; Andersen, O.S.; McElhaney, R.N. The antimicrobial peptide gramicidin S permeabilizes phospholipid bilayer membranes without forming discrete ion channels. Biochim. Biophys. Acta Biomembr. 2008, 1778, 2814–2822. [Google Scholar] [CrossRef]
- Michalak, Z.; Muzzio, M.; Milianta, P.J.; Giacomini, R.; Lee, S. Effect of monoglyceride structure and cholesterol content on water permeability of the droplet bilayer. Langmuir 2013, 29, 15919–15925. [Google Scholar] [CrossRef] [PubMed]
- Milianta, P.J.; Muzzio, M.; Denver, J.; Cawley, G.; Lee, S. Water Permeability across Symmetric and Asymmetric Droplet Interface Bilayers: Interaction of Cholesterol Sulfate with DPhPC. Langmuir 2015, 31, 12187–12196. [Google Scholar] [CrossRef]
- Mansour, M.M.; Challita, E.; Chaurasia, A.; Leo, D.; Sukharev, S.; Freeman, E. A skin-inspired soft material with directional mechanosensation. Bioinspiration Biomim. 2021. [Google Scholar]
- de Planque, M.R.; Aghdaei, S.; Roose, T.; Morgan, H. Electrophysiological characterization of membrane disruption by nanoparticles. ACS Nano 2011, 5, 3599–3606. [Google Scholar] [CrossRef] [PubMed]
- Punnamaraju, S.; You, H.; Steckl, A.J. Triggered Release of Molecules across Droplet Interface Bilayer Lipid Membranes Using Photopolymerizable Lipids. Langmuir 2012, 28, 7657–7664. [Google Scholar] [CrossRef]
- Quilliet, C.; Berge, B. Electrowetting: A recent outbreak. Curr. Opin. Colloid Interface Sci. 2001, 6, 34–39. [Google Scholar] [CrossRef]
- Mugele, F.; Baret, J.-C. Electrowetting: From basics to applications. J. Phys. Condens. Matter 2005, 17, R705–R774. [Google Scholar] [CrossRef]
- Trick, J.L.; Song, C.; Wallace, E.J.; Sansom, M.S.P. Voltage Gating of a Biomimetic Nanopore: Electrowetting of a Hydrophobic Barrier. ACS Nano 2017, 11, 1840–1847. [Google Scholar] [CrossRef] [PubMed]
- Martel, A.; Cross, B. Handling of artificial membranes using electrowetting-actuated droplets on a microfluidic device combined with integrated pA-measurements. Biomicrofluidics 2012, 6, 012813. [Google Scholar] [CrossRef] [PubMed]
- Fan, S.-K.; Chen, C.-W.; Lin, Y.-Y.; Chen, L.-C.; Tseng, F.-G.; Pan, R.-L. Formation of suspended bilayer lipid membrane between electrowetting-driven encapsulated droplets. Biomicrofluidics 2014, 8, 052006. [Google Scholar] [CrossRef]
- Sarles, S.A.; Leo, D.J.J.L.o.a.C. Physical encapsulation of droplet interface bilayers for durable, portable biomolecular networks. Lab Chip 2010, 10, 710–717. [Google Scholar] [CrossRef]
- Sarles, S.A.; Leo, D.J. Tailored current—voltage relationships of droplet-interface bilayers using biomolecules and external feedback control. Lab Chip 2009, 20, 1233–1247. [Google Scholar] [CrossRef]
- Kancharala, A.; Freeman, E.; Philen, M. A comprehensive flexoelectric model for droplet interface bilayers acting as sensors and energy harvesters. Smart Mater. Struct. 2016, 25, 104007. [Google Scholar] [CrossRef]
- Punnamaraju, S.; Steckl, A.J. Voltage Control of Droplet Interface Bilayer Lipid Membrane Dimensions. Langmuir 2011, 27, 618–626. [Google Scholar] [CrossRef] [PubMed]
- Lin, S.-Y.; Chen, L.-J.; Xyu, J.-W.; Wang, W.-J. An examination on the accuracy of interfacial tension measurement from pendant drop profiles. Langmuir 1995, 11, 4159–4166. [Google Scholar] [CrossRef]
- Najem, J.S.; Hasan, S.; Williams, R.S.; Weiss, R.J.; Rose, G.S.; Taylor, G.J.; Sarles, S.A.; Collier, C.P. Dynamical nonlinear memory capacitance in biomimetic membranes. Nat. Commun. 2019, 10, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Sokolov, V.; Kuz’min, V.J. Measurement of the difference in the surface potentials of bilayer membranes from the second harmonic of the capacitance current. Biophysics 1980, 25, 174–177. [Google Scholar]
- Pohl, E.E.; Peterson, U.; Sun, J.; Pohl, P. Changes of intrinsic membrane potentials induced by flip-flop of long-chain fatty acids. Biochemistry 2000, 39, 1834–1839. [Google Scholar] [CrossRef]
- Sokolov, V.; Gavrilchik, A.; Kulagina, A.; Meshkov, I.; Pohl, P.; Gorbunova, Y. Voltage-sensitive styryl dyes as singlet oxygen targets on the surface of bilayer lipid membrane. J. Photochem. Photobiol. B Biol. 2016, 161, 162–169. [Google Scholar] [CrossRef] [PubMed]
- Passechnik, V.I.; Sokolov, V.S. Estimation of electrochrome dyes position in the bilayer through the 2nd harmonic of capacitive current. Bioelectrochemistry 2002, 55, 47–51. [Google Scholar] [CrossRef]
- Mirsky, V.M. Ultrathin Electrochemical Chemo-and Biosensors: Technology and Performance; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013; Volume 2. [Google Scholar]
- Passechnik, V.I. Estimates of the intramembrane field through the harmonics of capacitive current in inhomogeneous bilayer lipid membranes. Bioelectrochemistry 2001, 54, 63–73. [Google Scholar] [CrossRef]
- El-Beyrouthy, J.; Freeman, E.C. Rapid and Real-Time Measurement of Membrane Potential Through Intramembrane Field Compensation. In Proceedings of the Smart Materials, Adaptive Structures and Intelligent Systems, Online, 15 September 2020; p. V001T006A008. [Google Scholar]
- Freeman, E.C.; Najem, J.S.; Sukharev, S.; Philen, M.K.; Leo, D.J. The mechanoelectrical response of droplet interface bilayer membranes. Soft Matter 2016, 12, 3021–3031. [Google Scholar] [CrossRef] [PubMed]
- Najem, J.S.; Sukharev, S.; Leo, D.J. The Effect of Transmembrane Potential on the Gating of MscL Channels in Droplet Interface Bilayers. In Smart Materials, Adaptive Structures and Intelligent Systems; American Society of Mechanical Engineers: New York, NY, USA, 2016; p. V002T006A012. [Google Scholar] [CrossRef]
- Coster, H.G.; Chilcott, T.C.; Coster, A.C. Impedance spectroscopy of interfaces, membranes and ultrastructures. Bioelectrochemistry Bioenerg. 1996, 40, 79–98. [Google Scholar] [CrossRef]
- Newman, J.; Thomas-Alyea, K.E. Electrochemical Systems; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Chang, B.Y.; Park, S.M. Electrochemical impedance spectroscopy. Annu. Rev. Anal. Chem. 2010, 3, 207–229. [Google Scholar] [CrossRef] [PubMed]
- Römer, W.; Steinem, C. Impedance analysis and single-channel recordings on nano-black lipid membranes based on porous alumina. Biophys. J. 2004, 86, 955–965. [Google Scholar] [CrossRef]
- Coster, H.; Smith, J. The molecular organization of bimolecular lipid membranes. A study of the low frequency Maxwell-Wagner impedance dispersion. Biochim. Biophys. Acta Biomembr. 1974, 373, 151–164. [Google Scholar] [CrossRef]
- Raila, T.; Ambrulevičius, F.; Penkauskas, T.; Jankunec, M.; Meškauskas, T.; Vanderah, D.J.; Valincius, G. Clusters of protein pores in phospholipid bilayer membranes can be identified and characterized by electrochemical impedance spectroscopy. Electrochim. Acta 2020, 364, 137179. [Google Scholar] [CrossRef]
- Wu, Z.; Tang, J.; Cheng, Z.; Yang, X.; Wang, E. Ion channel behavior of supported bilayer lipid membranes on a glassy carbon electrode. Anal. Chem. 2000, 72, 6030–6033. [Google Scholar] [CrossRef]
- Steinem, C.; Janshoff, A.; Ulrich, W.-P.; Sieber, M.; Galla, H.-J. Impedance analysis of supported lipid bilayer membranes: A scrutiny of different preparation techniques. Biochim. Biophys. Acta Biomembr. 1996, 1279, 169–180. [Google Scholar] [CrossRef]
- Valincius, G.; Meškauskas, T.; Ivanauskas, F. Electrochemical Impedance Spectroscopy of Tethered Bilayer Membranes. Langmuir 2012, 28, 977–990. [Google Scholar] [CrossRef]
- Alobeedallah, H.; Cornell, B.; Coster, H. The Effect of Cholesterol on the Dielectric Structure of Lipid Bilayers. J. Membr. Biol. 2018, 251, 153–161. [Google Scholar] [CrossRef]
- Alobeedallah, H.; Cornell, B.; Coster, H. The effect of benzyl alcohol on the dielectric structure of lipid bilayers. J. Membr. Biol. 2016, 249, 833–844. [Google Scholar] [CrossRef] [PubMed]
- Terrettaz, S.; Mayer, M.; Vogel, H. Highly Electrically Insulating Tethered Lipid Bilayers for Probing the Function of Ion Channel Proteins. Langmuir 2003, 19, 5567–5569. [Google Scholar] [CrossRef]
- Karolis, C.; Coster, H.G.; Chilcott, T.C.; Barrow, K.D. Differential effects of cholesterol and oxidised-cholesterol in egg lecithin bilayers. Biochim. Biophys. Acta Biomembr. 1998, 1368, 247–255. [Google Scholar] [CrossRef]
- Korman, C.E.; Megens, M.; Ajo-Franklin, C.M.; Horsley, D.A. Nanopore-Spanning Lipid Bilayers on Silicon Nitride Membranes That Seal and Selectively Transport Ions. Langmuir 2013, 29, 4421–4425. [Google Scholar] [CrossRef] [PubMed]
- Sarangi, N.K.; Stalcup, A.; Keyes, T.E. The Impact of Membrane Composition and Co-Drug Synergistic Effects on Vancomycin Association with Model Membranes from Electrochemical Impedance Spectroscopy. ChemElectroChem 2020, 7, 4535–4542. [Google Scholar] [CrossRef]
- Fujino, Y.; Nakamura, R.; Han, H.-W.; Yamashita, I.; Shimizu, T.; Shingubara, S.; Ito, T. Electrochemical impedance spectroscopy study of liposome adsorption and rupture on self-assembled monolayer: Effect of surface charge. J. Electroanal. Chem. 2020, 878, 114572. [Google Scholar] [CrossRef]
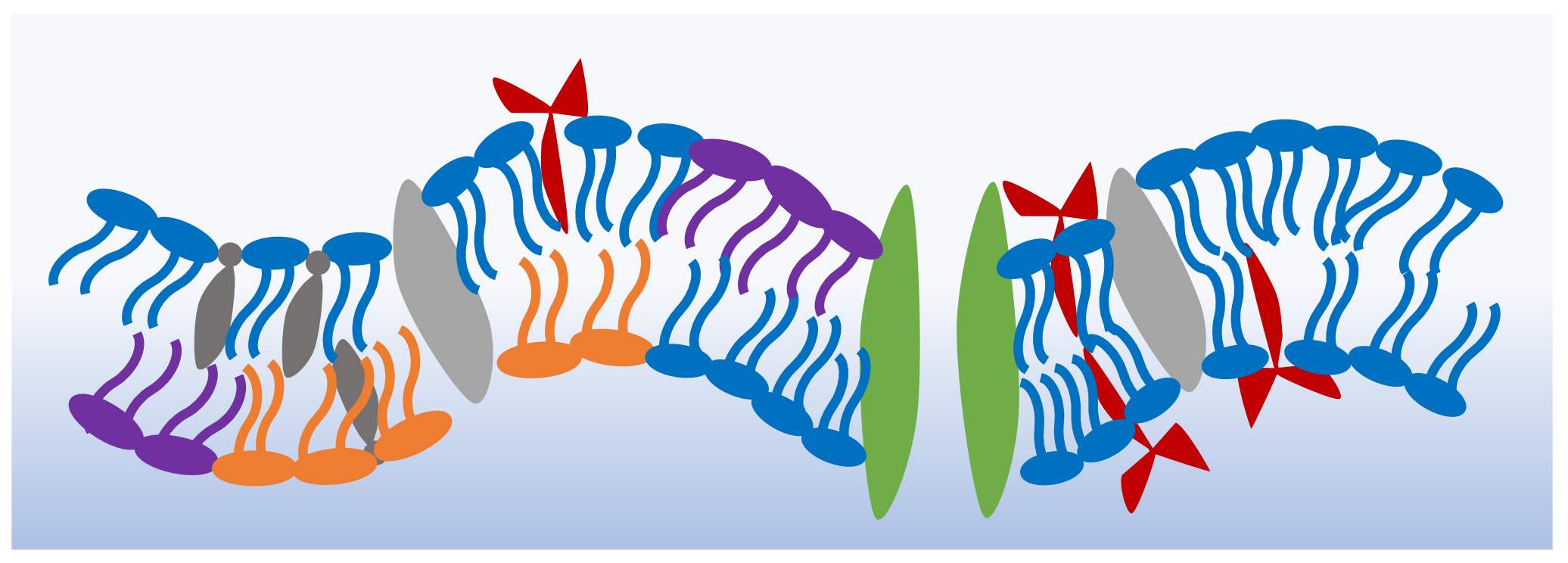
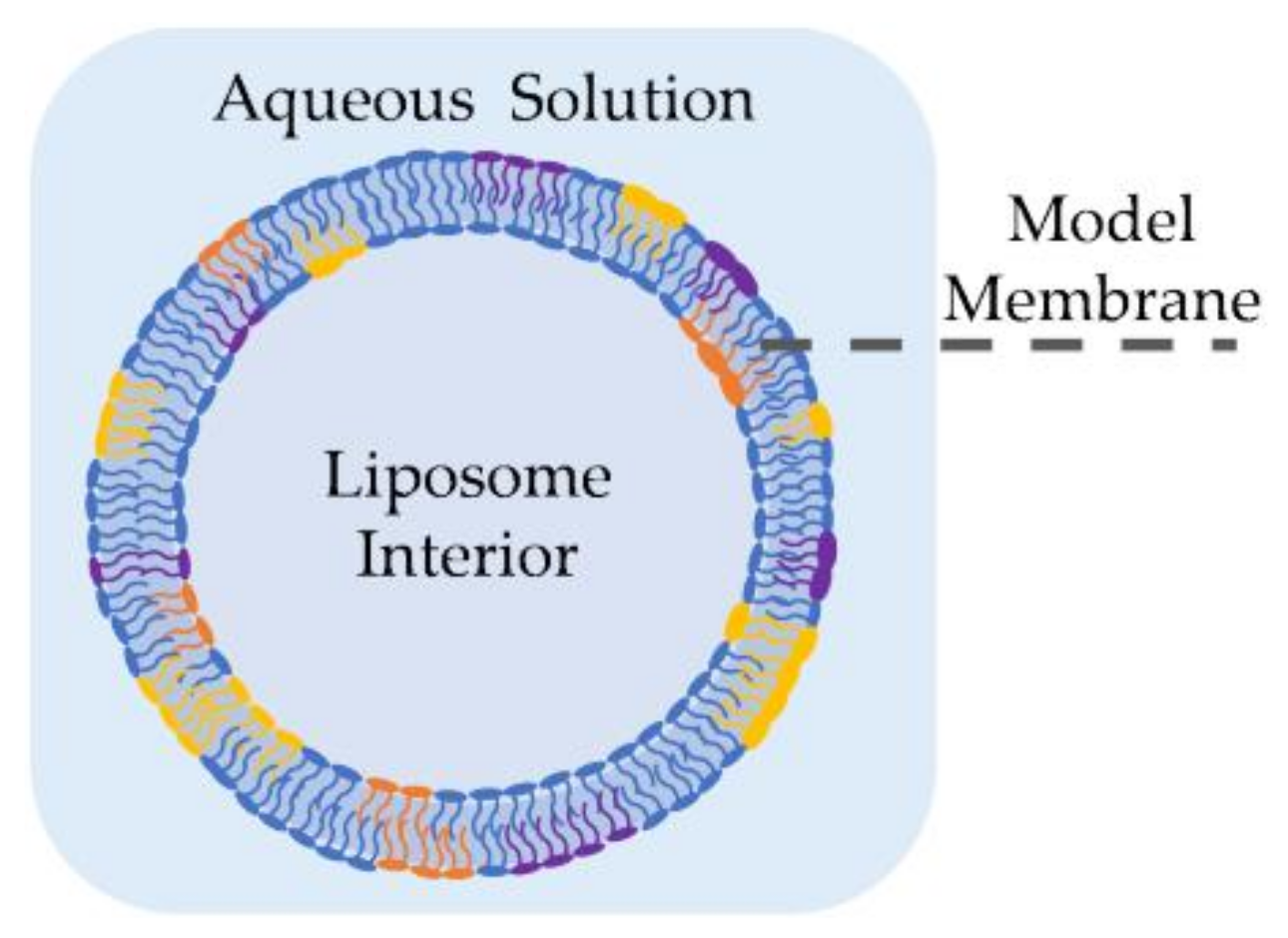
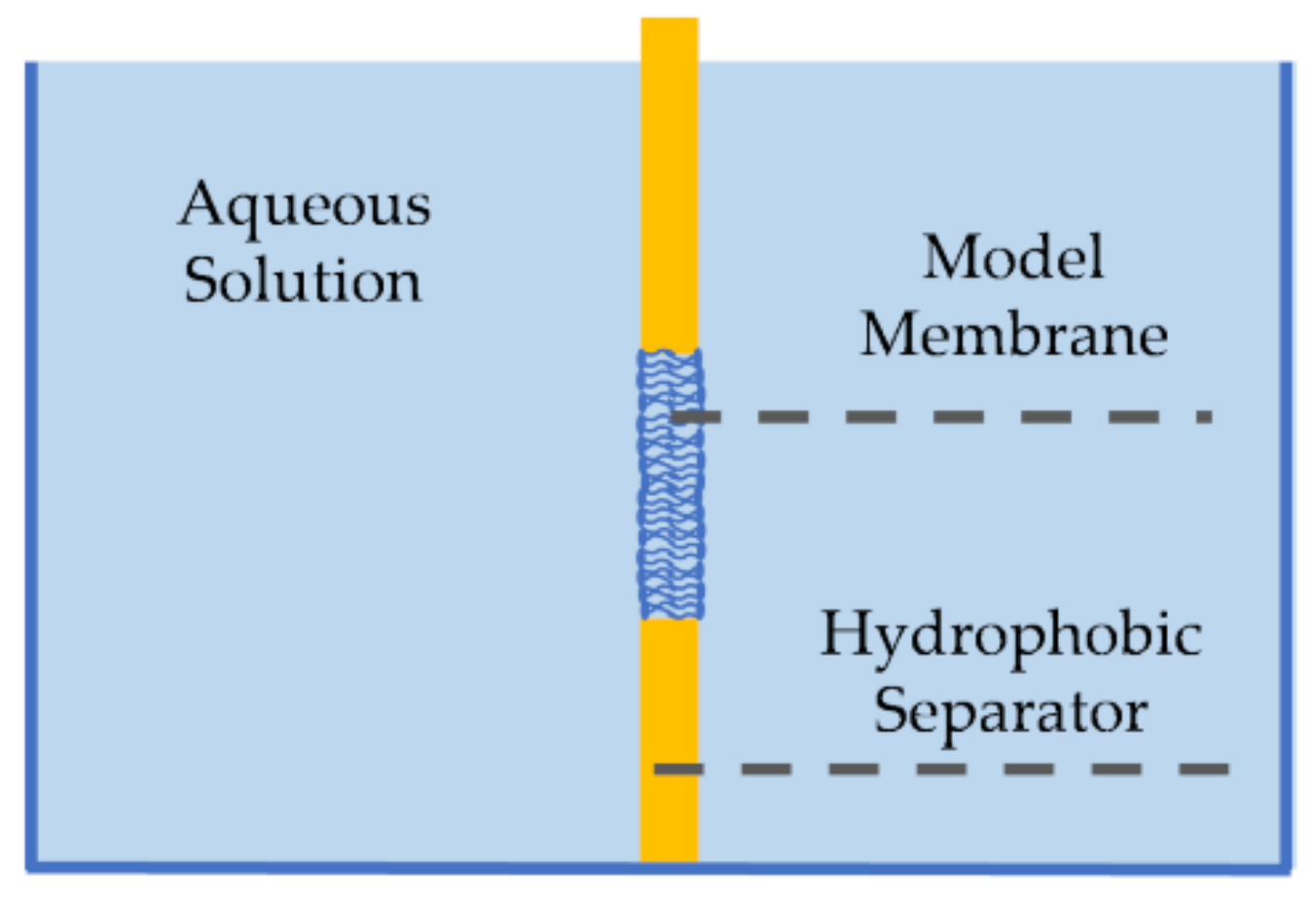
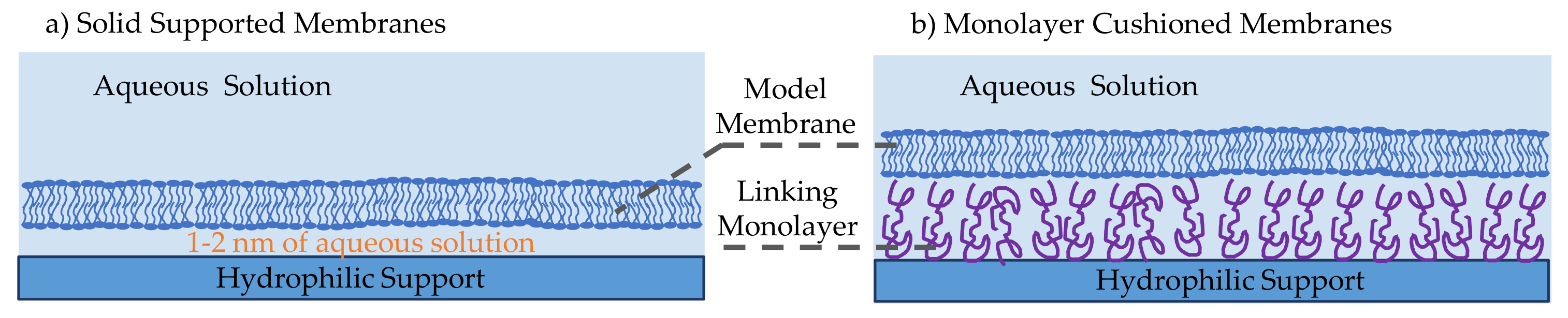
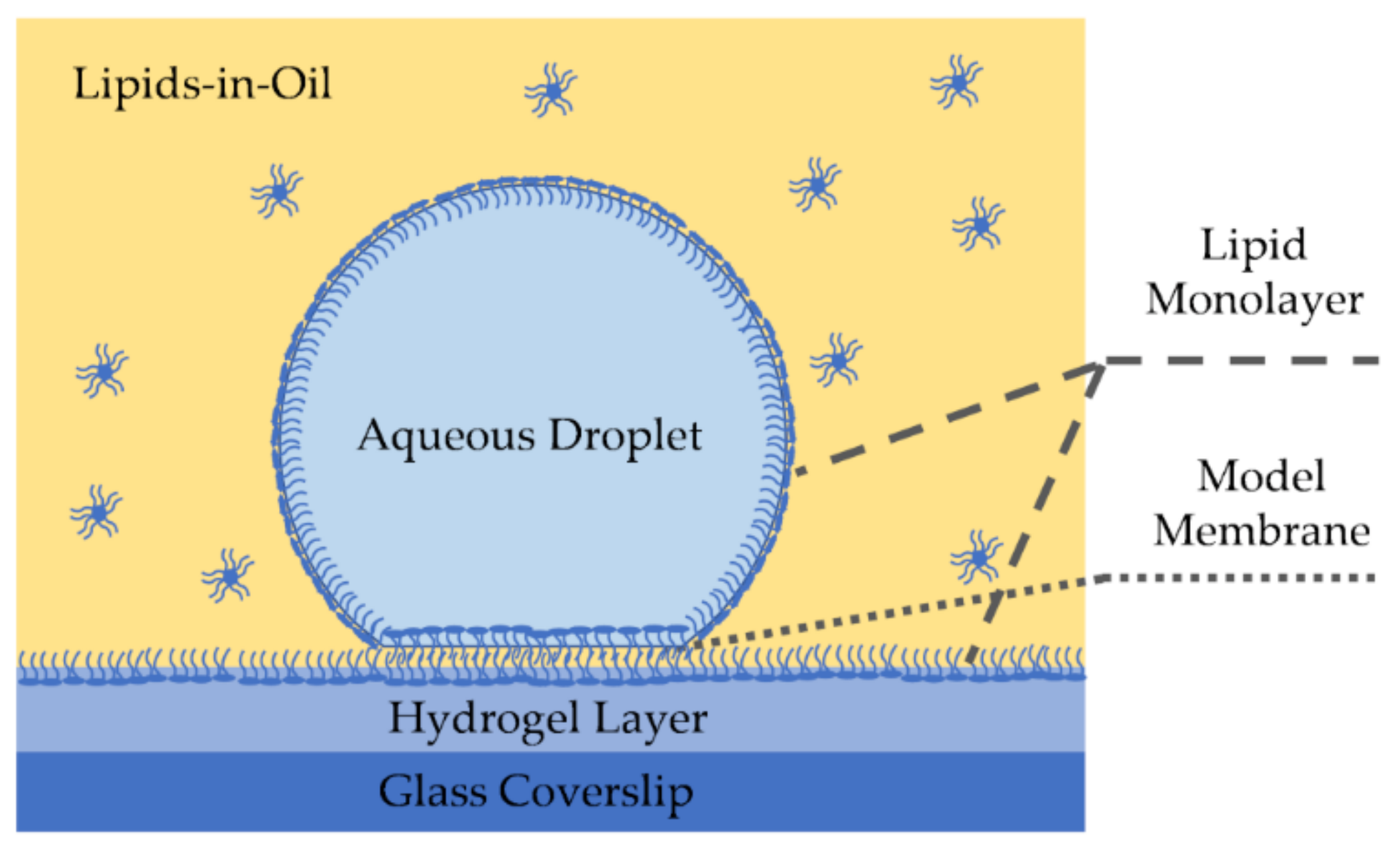
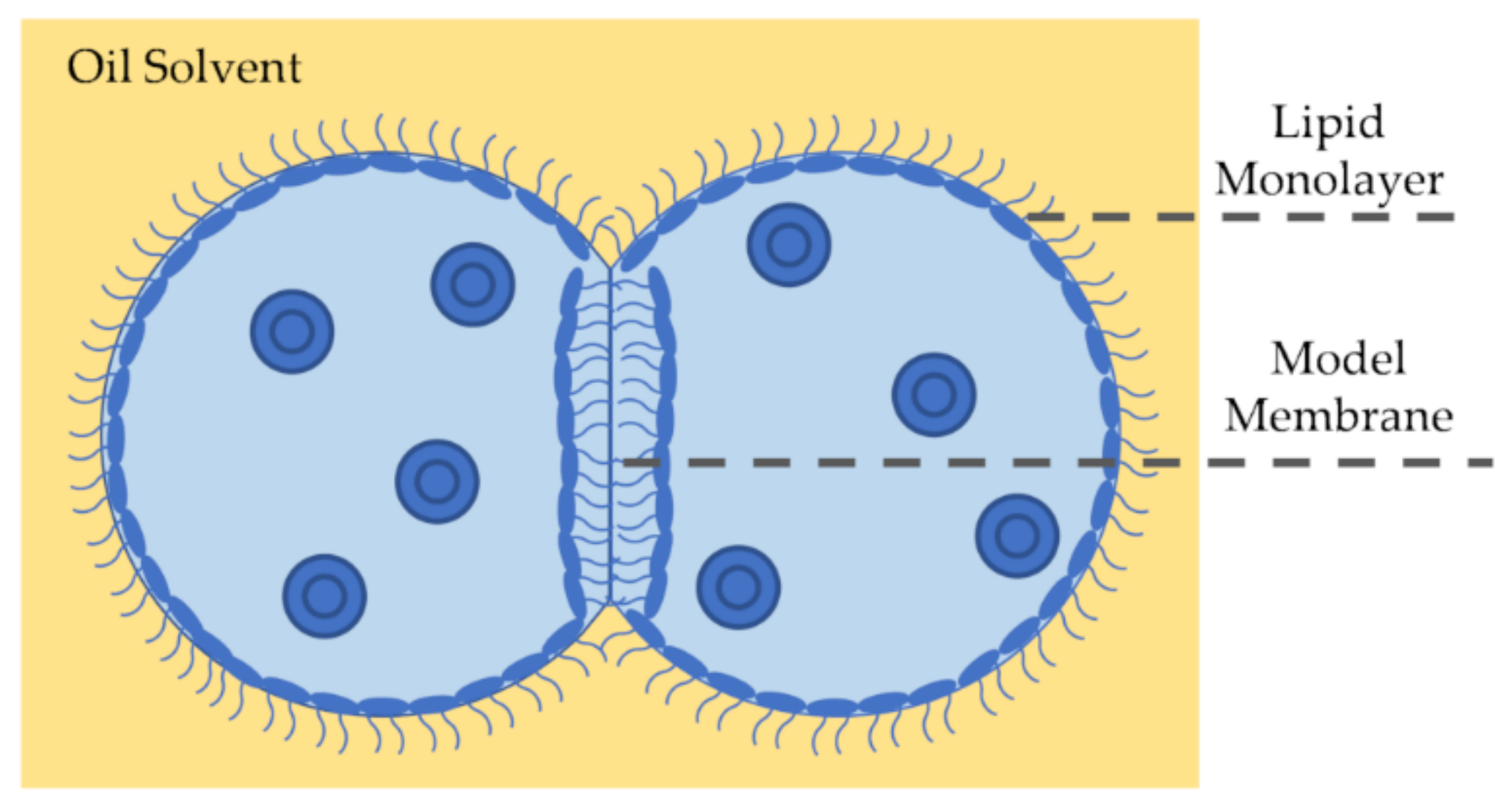


| Model Membrane | 2.1. Liposomes | 2.2. Pore-Spanning Membranes | 2.3. Solid Supported Membranes | 2.4. Emulsion-Based Membranes | |
| 2.4.1. Droplet on Hydrogel Bilayers | 2.4.2. Droplet Interface Bilayers | ||||
| Description | Lipid vesicles formed in an aqueous environment | Lipid bilayer formed at the orifice of a solid separator between two aqueous baths | Lipid bilayer formed on a solid support submerged in an aqueous solution | Lipid bilayer formed at a droplet-hydrogel interface | Lipid bilayer formed at a droplet-droplet interface |
| Manufacturing Techniques | Electroformation Phase Transfer Microfluidic Jets | Solvent painting Monolayers folding | Langmuir-Blodgett/ Langmuir-Schaefer Vesicle fusion | Microfluidic droplet deposition and manipulation in oil reservoirs | |
| Advantages | Similar in geometry and dimensions to natural membranes | Well-packed, high impedance membranes Isolate transverse properties | Mechanically robust, stable, and long-lasting membranes | Full membrane area visualization | Direct tension measurements Simple formation of asymmetric membranes |
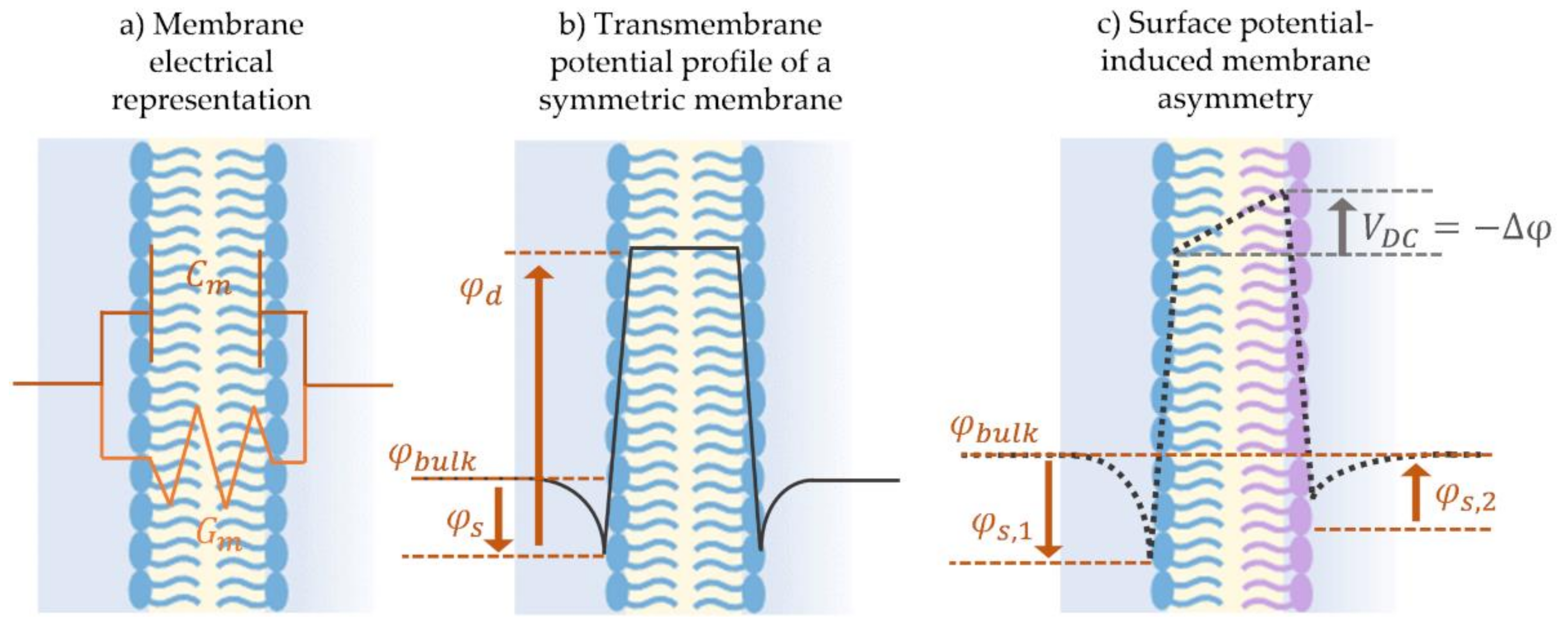
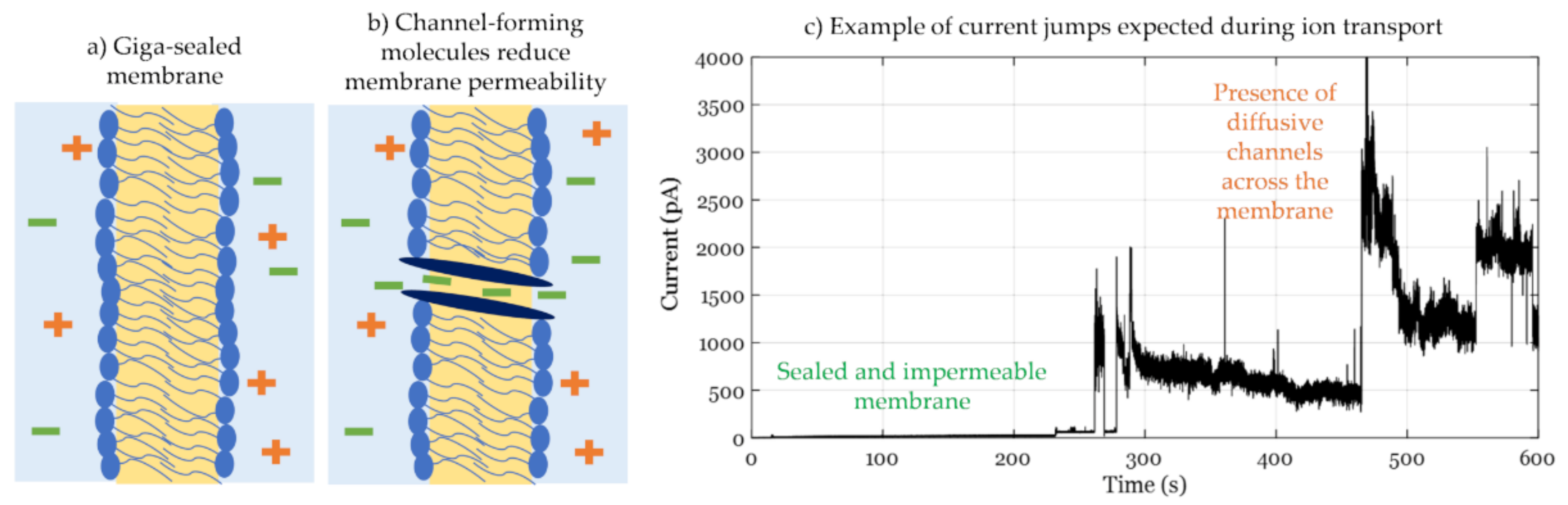
| Electrophysiology Technique | 3.1. Conductance Measurements | 3.2. Electrowetting and Electrocompression | 3.3. Electroimpedance Spectroscopy | |
| 3.2.1. Dynamic Capacitance | 3.2.2. Current Attenuation | |||
| Fundamental Equation | ||||
| Experimental Approach | Applying constant DC voltage and tracking the current | Applying step-DC voltage and calculating the equilibrium capacitance | Attenuating the current harmonics through varying the applied voltage. | Generating Bode or Nyquist plots and comparing them to the expected model circuit |
| Common Applications | Measuring channel-forming mechanisms of disruptive agents | Calculating membrane potential, dielectric thickness, and monolayer surface tension | Measuring membrane potential and rigidity Detecting intramembrane dynamics | Detection and localization of molecular adsorption and sensor platforms |
| Experimental Requirements | High base membrane resistance | Sufficient equilibrium time between voltage steps | Highly compressible membrane for enhancing measurements | High signal frequencies and compatible equipment. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
El-Beyrouthy, J.; Freeman, E. Characterizing the Structure and Interactions of Model Lipid Membranes Using Electrophysiology. Membranes 2021, 11, 319. https://doi.org/10.3390/membranes11050319
El-Beyrouthy J, Freeman E. Characterizing the Structure and Interactions of Model Lipid Membranes Using Electrophysiology. Membranes. 2021; 11(5):319. https://doi.org/10.3390/membranes11050319
Chicago/Turabian StyleEl-Beyrouthy, Joyce, and Eric Freeman. 2021. "Characterizing the Structure and Interactions of Model Lipid Membranes Using Electrophysiology" Membranes 11, no. 5: 319. https://doi.org/10.3390/membranes11050319
APA StyleEl-Beyrouthy, J., & Freeman, E. (2021). Characterizing the Structure and Interactions of Model Lipid Membranes Using Electrophysiology. Membranes, 11(5), 319. https://doi.org/10.3390/membranes11050319






