Numerical Modelling Assisted Design of a Compact Ultrafiltration (UF) Flat Sheet Membrane Module
Abstract
1. Introduction
2. Materials and Methods
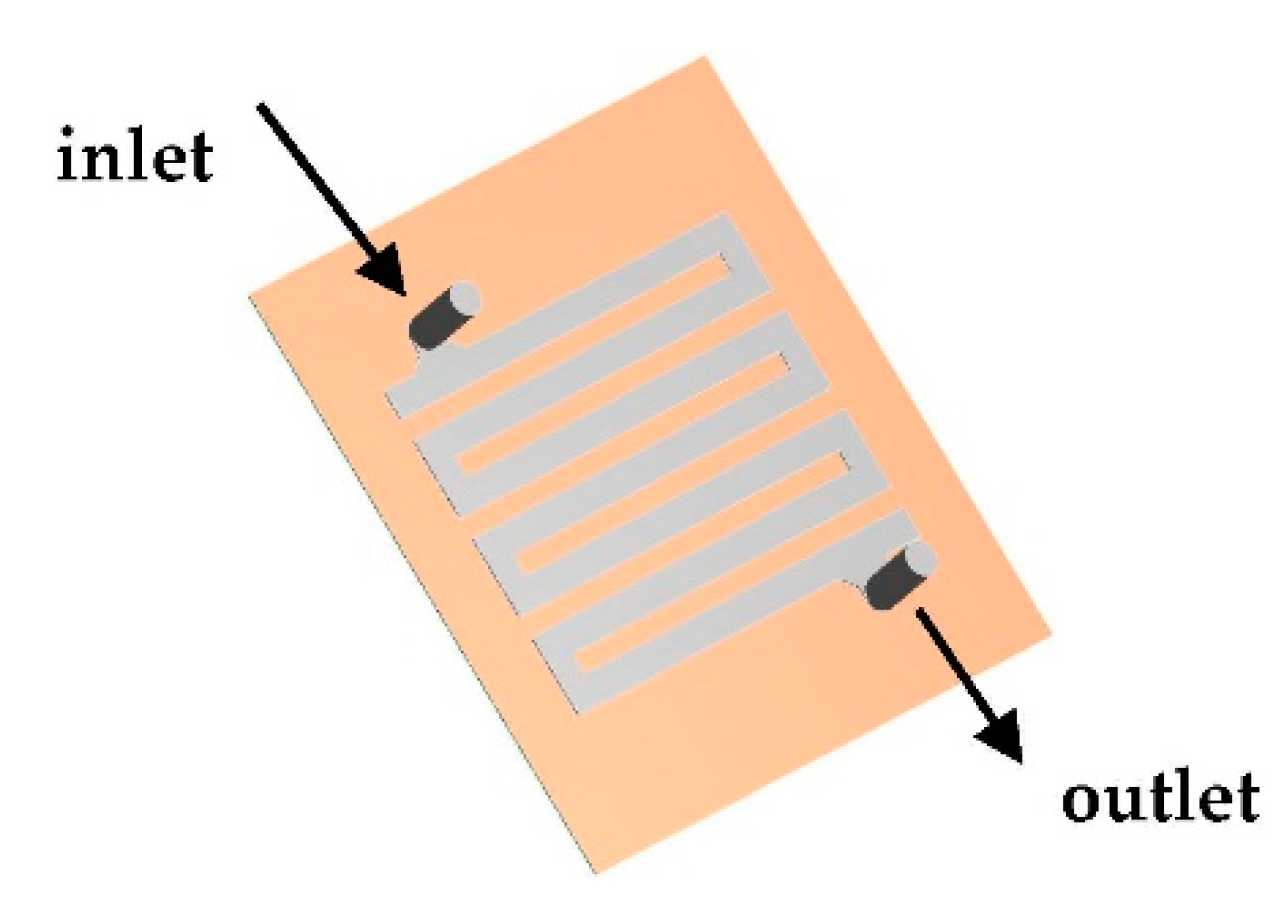
2.1. Proposed Module Design and Fabrication
Design Dimensions and Variables
2.2. Development of the CFD Modelling
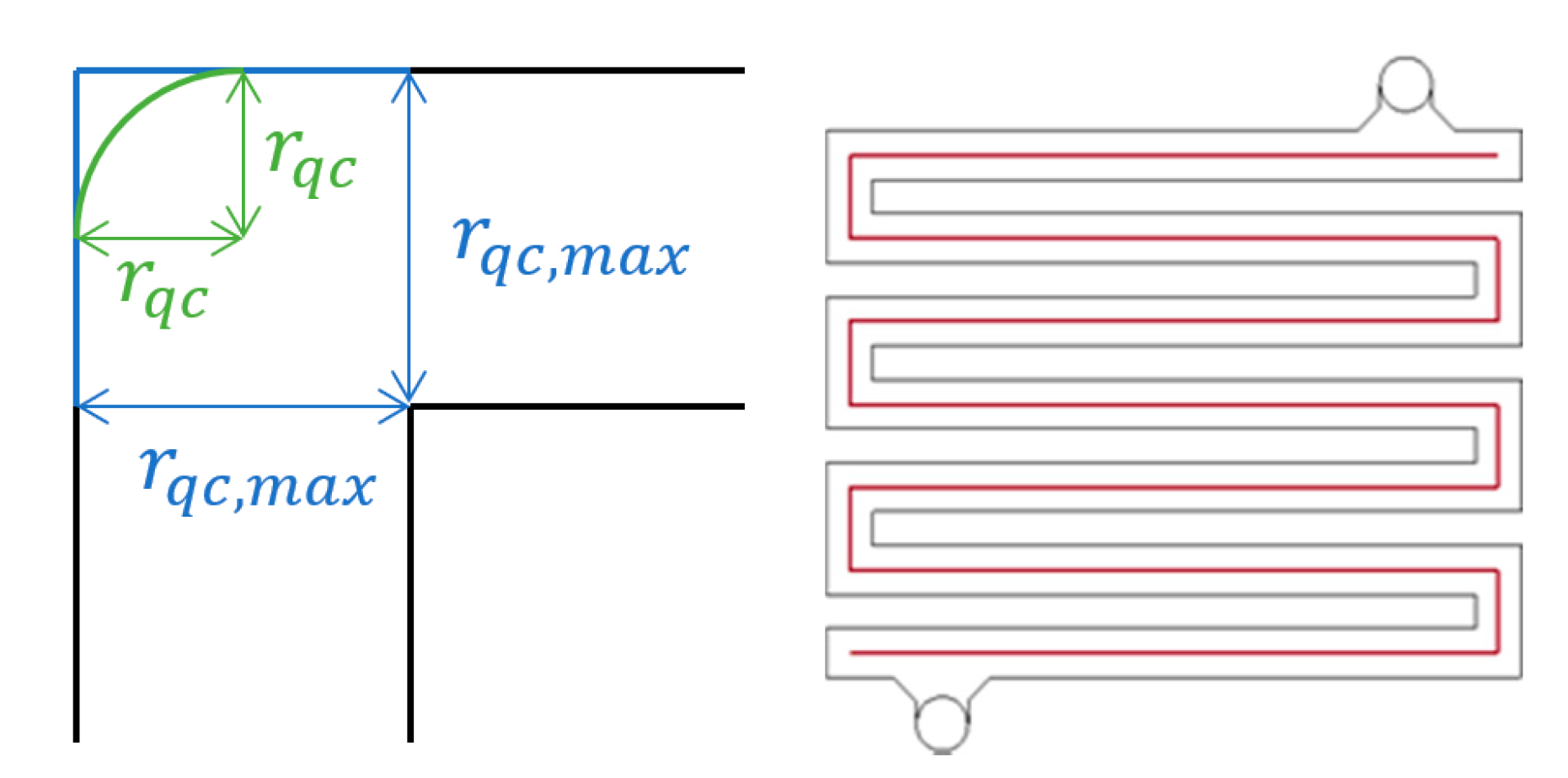
2.2.1. Geometry and Grid Creation
2.2.2. Governing Equations
2.2.3. Solution Method
3. Results and Discussion
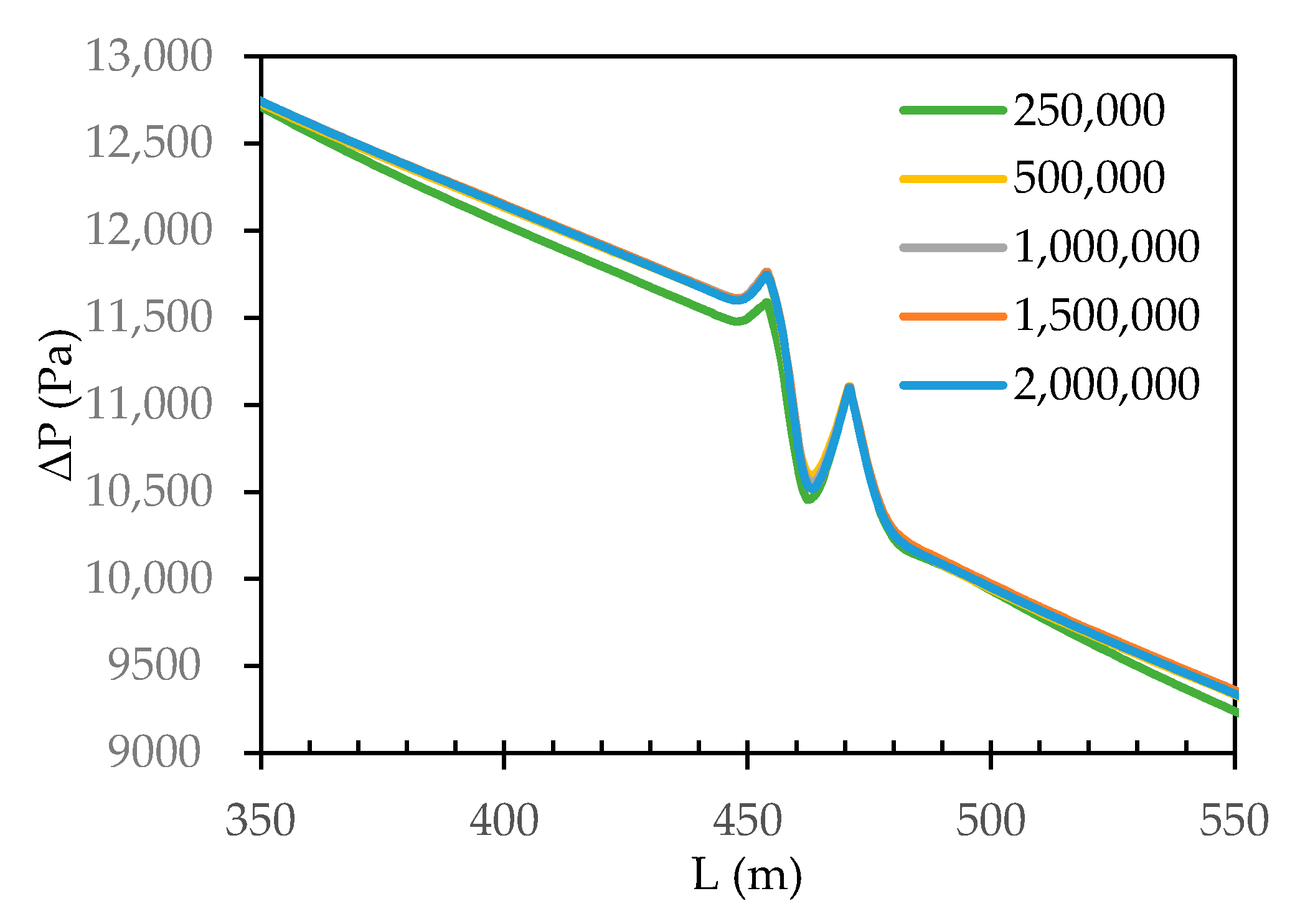
3.1. Grid Sensitivity Analysis
3.1.1. Grid Cell Count Quality
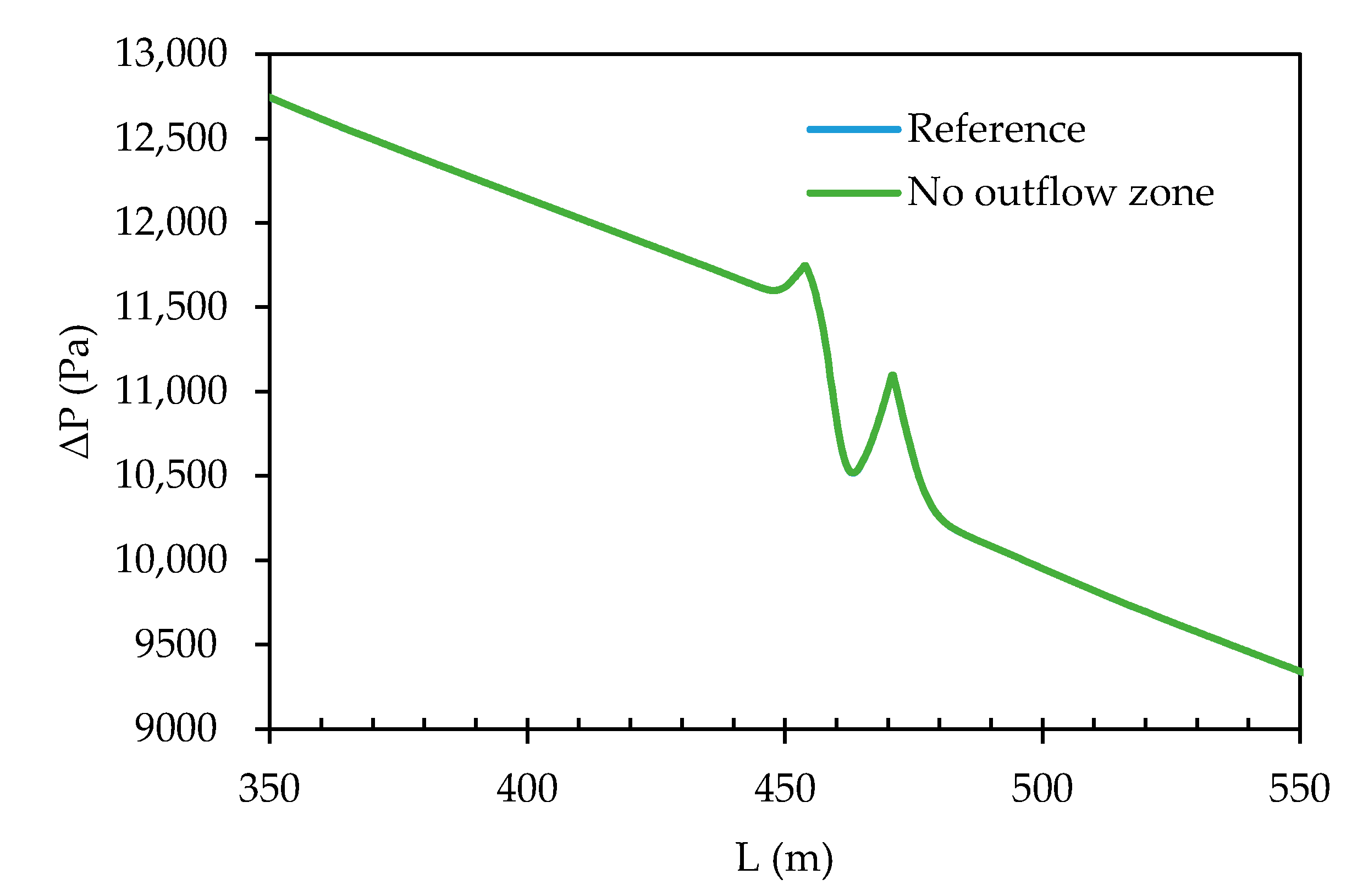
3.1.2. Inclusion of an Outflow Zone
3.2. Parametric Sensitivity Study on Geometrical Variables
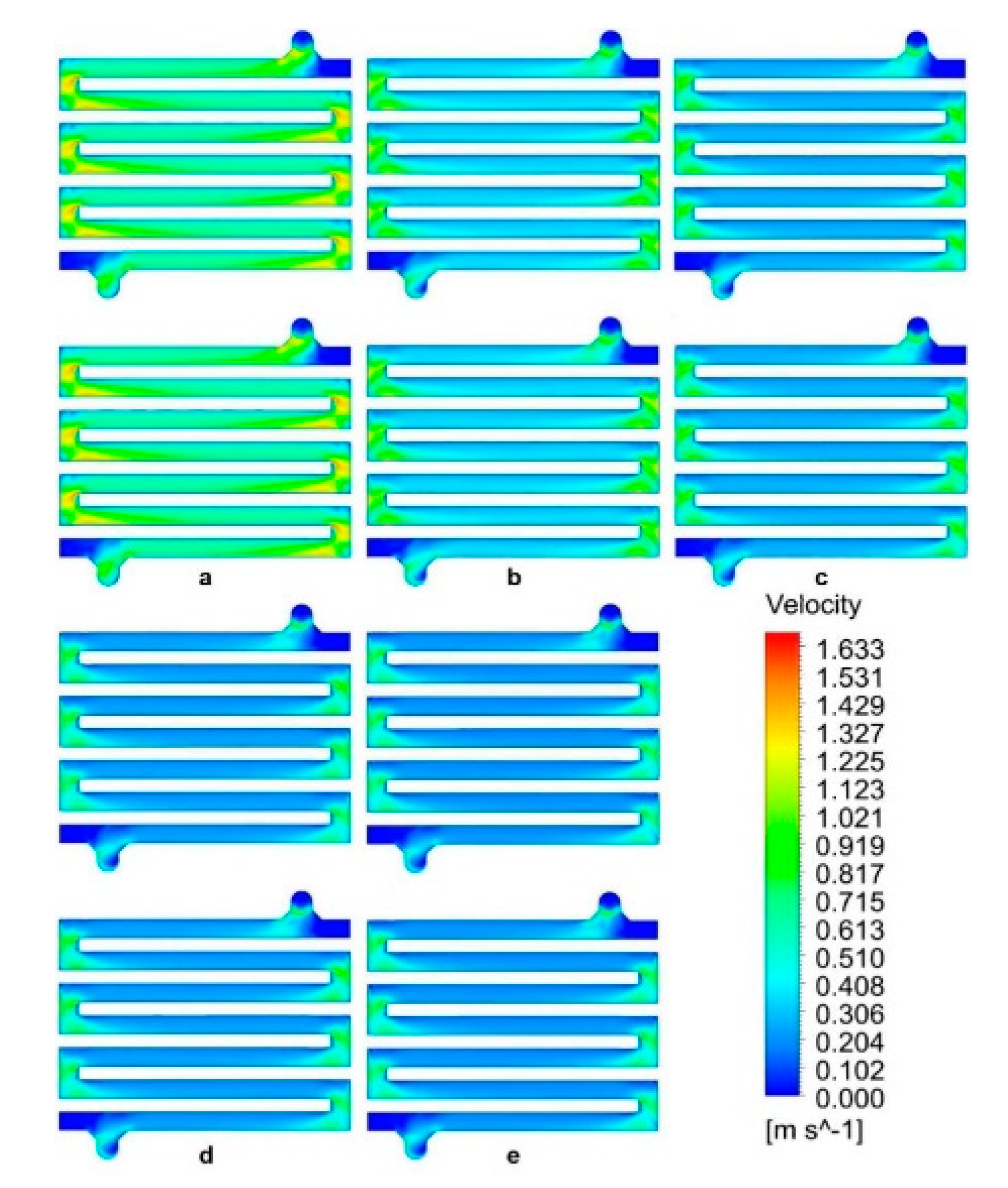
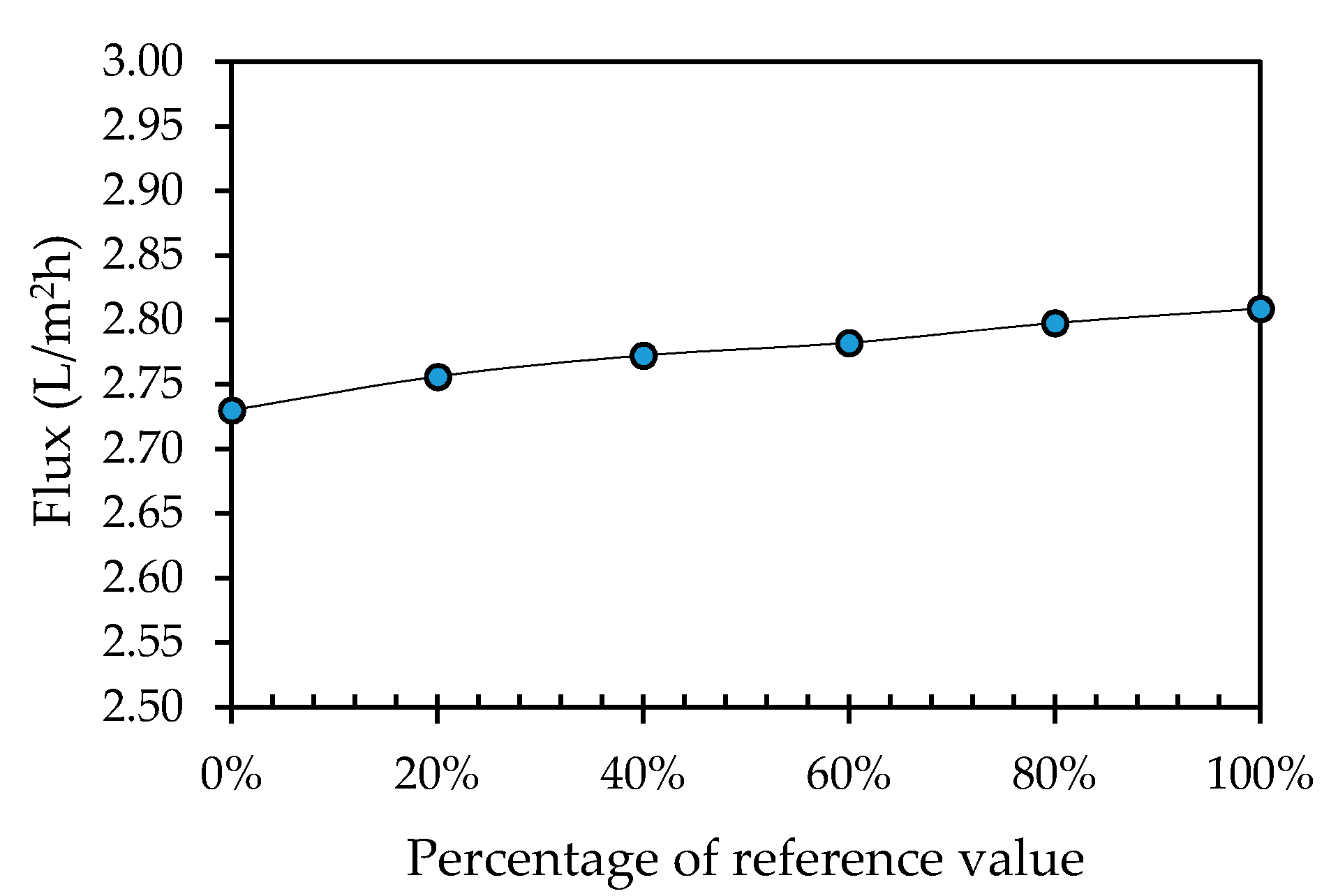
3.2.1. Spacer Thickness (Inflow Zone) and the Outflow Zone
3.2.2. Spacer Curviness
3.2.3. Inlet and Outlet Pipe Length
3.2.4. Inlet and Outlet Pipe Diameter
3.3. Sensitivity Analysis on Membrane Properties and Operating Conditions
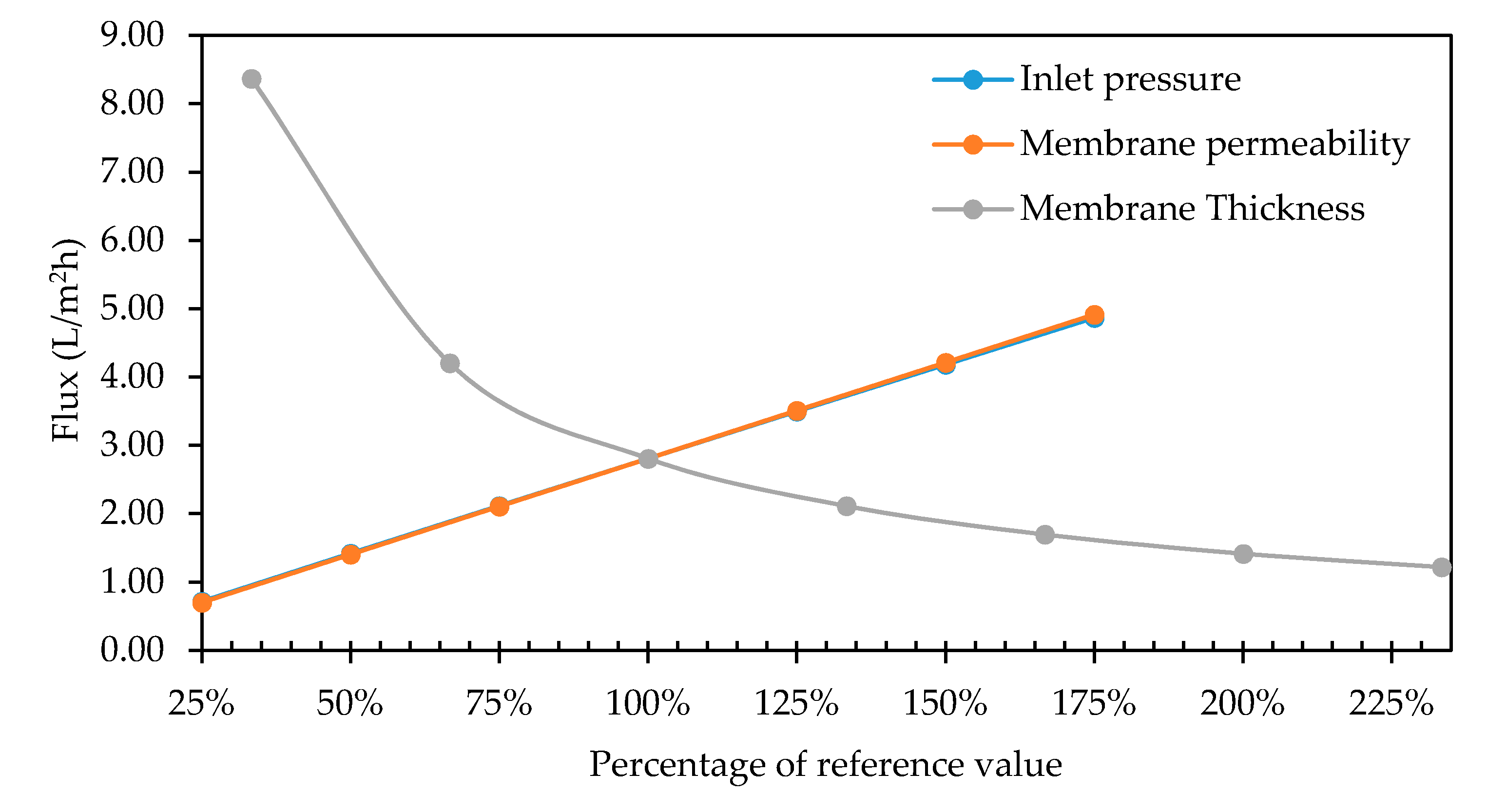
3.3.1. Membrane Permeability
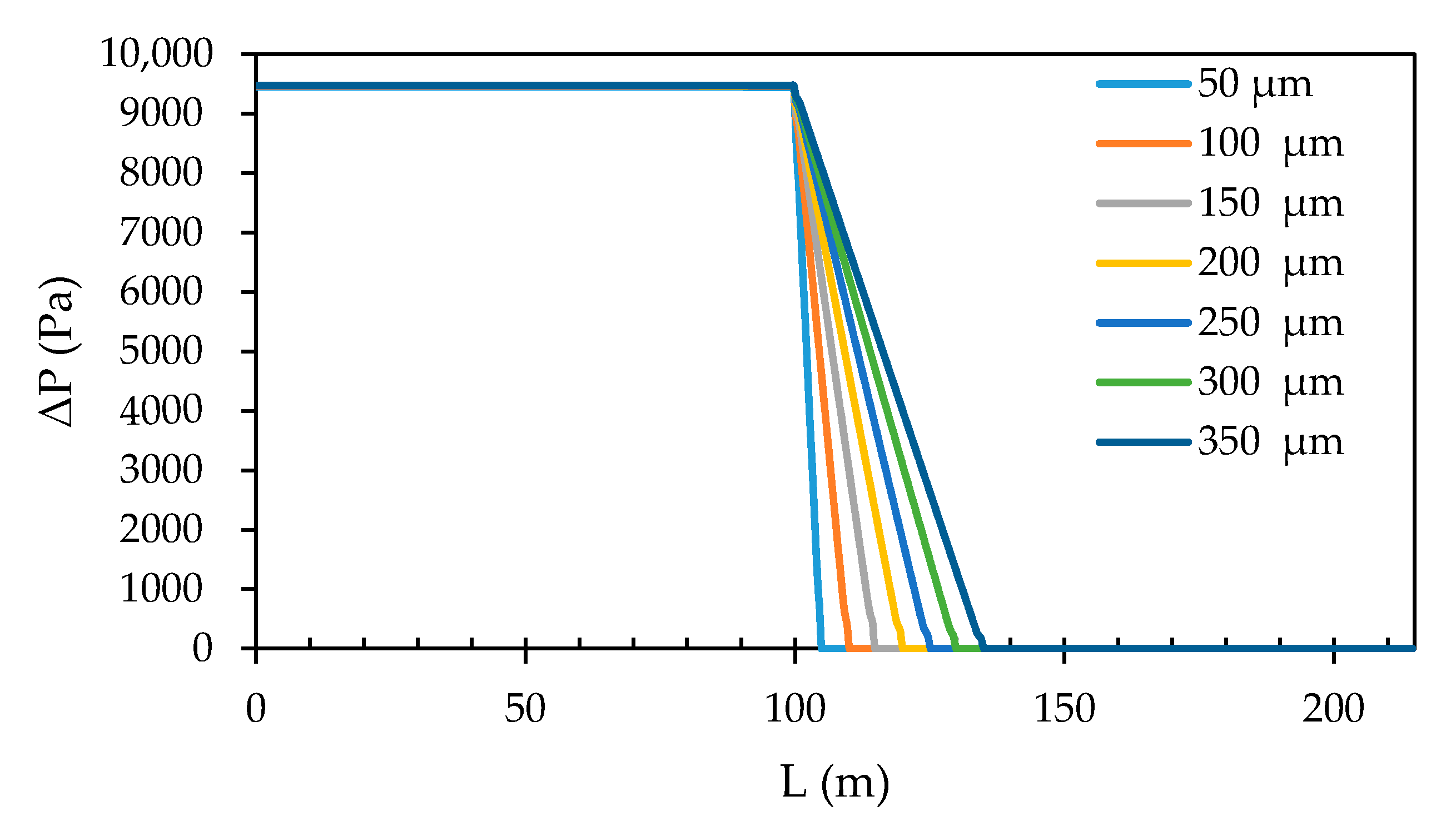
3.3.2. Membrane Thickness
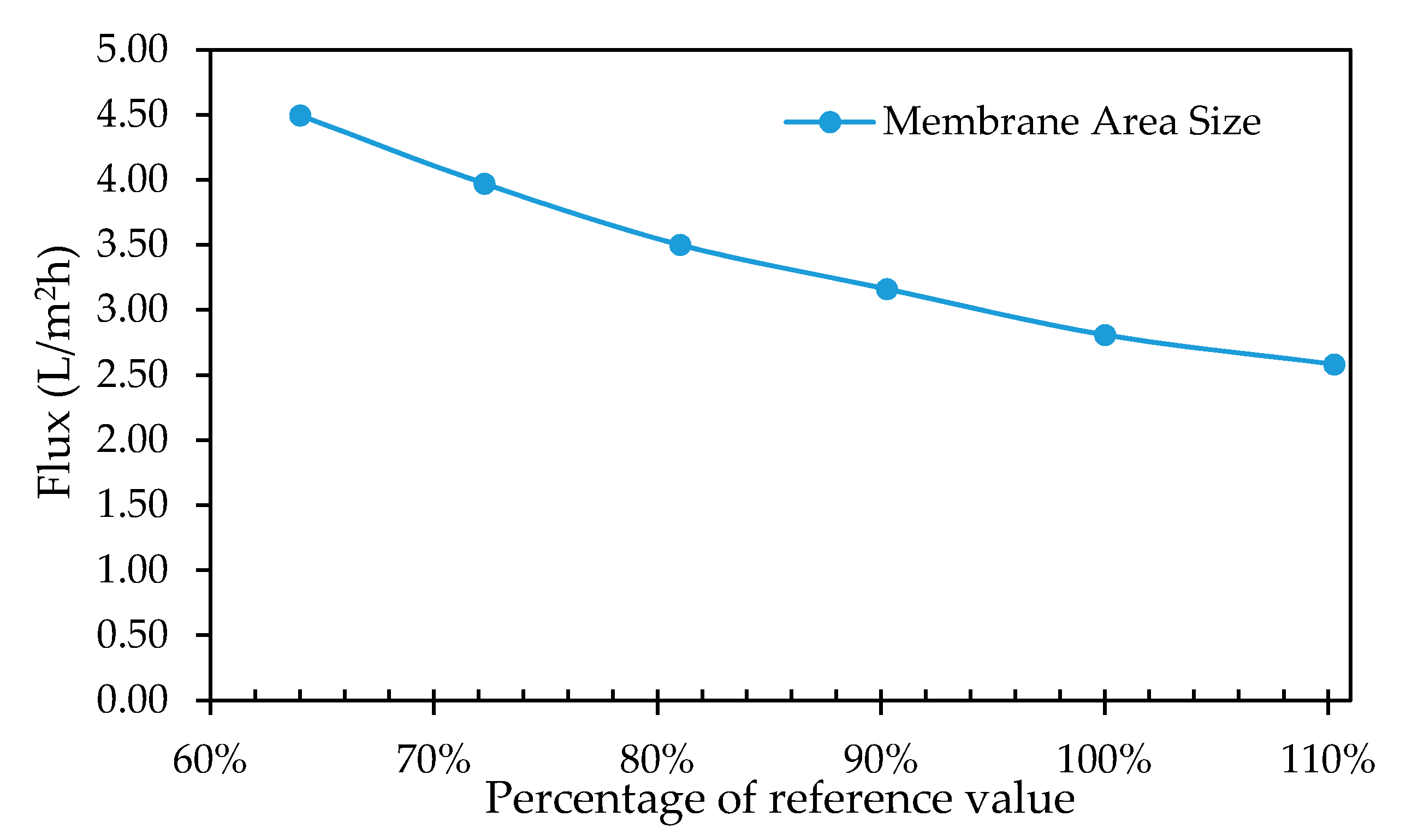
3.3.3. Membrane Surface Area
3.3.4. Inlet Pressure
3.3.5. Overview of the Parametric Sensitivity Analysis
4. Conclusions
- The numerical simulation solution could predict permeate flux with a reasonable error. The pressure distribution upon the membrane was found to depend on the fluid flow pattern on the membrane.
- A parametric analysis on configuration variables was carried out to determine the optimum design variables. The spacer geometry (tortuous spacer) with reasonable curviness was found to impact the permeating conditions and thus, may be used to produce a high and stable water quality over a long-time frame due to the lower associated pressure drop, leading to lower operational costs. The inlet diameter also showed a significant influence on the pressure drop within the system.
- The sensitivity analysis on membrane properties and operating conditions revealed that the inlet pressure, membrane permeability, membrane thickness and membrane area have a significant impact on the total permeate flux.
- The membrane area size provided useful information for optimization purposes: by reducing the membrane area to the size of the spacer, more, smaller membranes can be combined to obtain a higher flux.
Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Watkins, K. Human Development Report 2006-Beyond Scarcity: Power, Poverty and the Global Water Crisis. UNDP Human Development Reports 2006; United Nations Development Programme: New York, NY, USA, 2006. [Google Scholar]
- Arnal, J.; García-Fayos, B.; Sancho, M.; Verdú, G.; Lora, J. Design and installation of a decentralized drinking water system based on ultrafiltration in Mozambique. Desalination 2010, 250, 613–617. [Google Scholar] [CrossRef]
- Stats, S.A. Sustainable Development Goals: Baseline Report 2017; Statistics South Africa: Pretoria, South Africa, 2017. [Google Scholar]
- Statistics South Africa. Sustainable Development Goals: Indicator Baseline Report, Stats SA. 2017. Available online: http://www.statssa.gov.za/MDG/SDG_Baseline_Report_2017.pdf (accessed on 18 August 2019).
- Stats, S.A. Statistics South Africa Census 2011 Statistical Release—P0301.4/Statistics. 2012. Available online: http://www.statssa.gov.za/Publications/P03014/P030142011.Pdf (accessed on 19 August 2019).
- Molelekwa, G.F.; Mukhola, M.S.; Van Der Bruggen, B.; Luis, P. Preliminary Studies on Membrane Filtration for the Production of Potable Water: A Case of Tshaanda Rural Village in South Africa. PLoS ONE 2014, 9, e105057. [Google Scholar] [CrossRef]
- Caro, J. Basic Principles of Membrane Technology. Z. Phys. Chem. 1998, 203, 263. [Google Scholar] [CrossRef]
- Li, D.; Wang, R.; Chung, T.-S. Fabrication of lab-scale hollow fiber membrane modules with high packing density. Sep. Purif. Technol. 2004, 40, 15–30. [Google Scholar] [CrossRef]
- Fane, A.G.; Chang, S.; Chardon, E. Submerged hollow fibre membrane module—Design options and operational considerations. Desalination 2002, 146, 231–236. [Google Scholar] [CrossRef]
- Buer, T.; Cumin, J. MBR module design and operation. Desalination 2010, 250, 1073–1077. [Google Scholar] [CrossRef]
- Marriott, J.; Sørensen, E.; Bogle, I. Detailed mathematical modelling of membrane modules. Comput. Aided Chem. Eng. 2000, 8, 523–528. [Google Scholar] [CrossRef]
- Marriott, J.; Sørensen, E. A general approach to modelling membrane modules. Chem. Eng. Sci. 2003, 58, 4975–4990. [Google Scholar] [CrossRef]
- Wan, C.F.; Yang, T.; Lipscomb, G.G.; Stookey, D.J.; Chung, T.-S. Design and fabrication of hollow fiber membrane modules. J. Membr. Sci. 2017, 538, 96–107. [Google Scholar] [CrossRef]
- Kostoglou, M.; Karabelas, A.J. Comprehensive simulation of flat-sheet membrane element performance in steady state desalination. Desalination 2013, 316, 91–102. [Google Scholar] [CrossRef]
- Yang, X.; Wang, R.; Fane, A.G.; Tang, C.Y.; Wenten, I.G. Membrane module design and dynamic shear-induced techniques to enhance liquid separation by hollow fiber modules: A review. Desalin. Water Treat. 2013, 51, 3604–3627. [Google Scholar] [CrossRef]
- Oka, P.; Khadem, N.; Bérubé, P. Operation of passive membrane systems for drinking water treatment. Water Res. 2017, 115, 287–296. [Google Scholar] [CrossRef] [PubMed]
- Jacobs, P.E.; Pillay, V.L. The Development of Small-Scale Ultrafiltration Systems for Potable. 2004. Available online: http://www.wrc.org.za/wp-content/uploads/mdocs/1070-1-041.pdf (accessed on 7 March 2019).
- Swartz, C. Guidebook for the Selection of Small Water Treatment Systems for Potable Water Supply to Small Communities. 2007. Available online: http://www.wrc.org.za/wp-content/uploads/mdocs/TT319-07.pdf (accessed on 19 August 2019).
- Peter-Varbanets, M.; Zurbrügg, C.; Swartz, C.; Pronk, W. Decentralized systems for potable water and the potential of membrane technology. Water Res. 2009, 43, 245–265. [Google Scholar] [CrossRef]
- Boulestreau, M.; Hoa, E.; Peter-Verbanets, M.; Pronk, W.; Rajagopaul, R.; Lesjean, B. Operation of gravity-driven ultrafiltration prototype for decentralised water supply. Desalin. Water Treat. 2012, 42, 125–130. [Google Scholar] [CrossRef]
- Pronk, W.; Ding, A.; Morgenroth, E.; Derlon, N.; Desmond, P.; Burkhardt, M.; Wu, B.; Fane, A.G. Gravity-driven membrane filtration for water and wastewater treatment: A review. Water Res. 2019, 149, 553–565. [Google Scholar] [CrossRef]
- Baker, R.W. Membrane Technology and Applications—Richard W. Baker—Google Books. 2004. Available online: https://books.google.co.za/books/about/Membrane_Technology_and_Applications.html?id=FhtBKUq4rL8C&printsec=frontcover&source=kp_read_button&redir_esc=y#v=onepage&q&f=false (accessed on 21 August 2020).
- Gu, B.; Kim, D.; Kim, J.; Yang, D.R. Mathematical model of flat sheet membrane modules for FO process: Plate-and-frame module and spiral-wound module. J. Membr. Sci. 2011, 379, 403–415. [Google Scholar] [CrossRef]
- Jardón-Pérez, L.E.; González-Rivera, C.; Ramirez-Argaez, M.A.; Dutta, A. Numerical Modeling of Equal and Differentiated Gas Injection in Ladles: Effect on Mixing Time and Slag Eye. Processes 2020, 8, 917. [Google Scholar] [CrossRef]
- Hoang, Q.N.; Ramírez-Argáez, M.A.; Conejo, A.N.; Blanpain, B.; Dutta, D. Numerical Modeling of Liquid—Liquid Mass Transfer and the Influence of Mixing in Gas-Stirred Ladles. JOM 2018, 70, 2109–2118. [Google Scholar] [CrossRef]
- Laurent, J.; Samstag, R.W.; Ducoste, J.M.; Griborio, A.; Nopens, I.; Batstone, D.J.; Wicks, J.D.; Saunders, S.; Potier, O. A protocol for the use of computational fluid dynamics as a supportive tool for wastewater treatment plant modelling. Water Sci. Technol. 2014, 70, 1575–1584. [Google Scholar] [CrossRef]
- Samstag, R.W.; Ducoste, J.J.; Griborio, A.; Nopens, I.; Batstone, D.J.; Wicks, J.D.; Saunders, S.; Wicklein, E.A.; Kenny, G.; Laurent, J. CFD for wastewater treatment: An overview. Water Sci. Technol. 2016, 74, 549–563. [Google Scholar] [CrossRef]
- Zhang, J.; Huck, P.M.; Anderson, W.B.; Stubley, G.D. A Computational Fluid Dynamics Based Integrated Disinfection Design Approach for Improvement of Full-scale Ozone Contactor Performance. Ozone Sci. Eng. 2007, 29, 451–460. [Google Scholar] [CrossRef]
- Meister, M.; Winkler, D.; Rezavand, M.; Rauch, W. Integrating hydrodynamics and biokinetics in wastewater treatment modelling by using smoothed particle hydrodynamics. Comput. Chem. Eng. 2017, 99, 1–12. [Google Scholar] [CrossRef]
- Goula, A.M.; Kostoglou, M.; Karapantsios, T.D.; Zouboulis, A.I. A CFD methodology for the design of sedimentation tanks in potable water treatment. Chem. Eng. J. 2008, 140, 110–121. [Google Scholar] [CrossRef]
- Fimbres-Weihs, G.; Wiley, D.E. Review of 3D CFD modeling of flow and mass transfer in narrow spacer-filled channels in membrane modules. Chem. Eng. Process. Process Intensif. 2010, 49, 759–781. [Google Scholar] [CrossRef]
- Glucina, K.; Derekx, Q.; Langlais, C.; Laîné, J.-M. Use of advanced CFD tool to characterize hydrodynamic of commercial UF membrane module. Desalin. Water Treat. 2009, 9, 253–258. [Google Scholar] [CrossRef]
- Cortés-Juan, F.; Balannec, B.; Renouard, T. CFD-assisted design improvement of a bench-scale nanofiltration cell. Sep. Purif. Technol. 2011, 82, 177–184. [Google Scholar] [CrossRef]
- Haddadi, B.; Jordan, C.; Miltner, M.; Harasek, M. Membrane modeling using CFD: Combined evaluation of mass transfer and geometrical influences in 1D and 3D. J. Membr. Sci. 2018, 563, 199–209. [Google Scholar] [CrossRef]
- Keir, G.; Jegatheesan, V. A review of computational fluid dynamics applications in pressure-driven membrane filtration. Rev. Environ. Sci. Bio/Technol. 2014, 13, 183–201. [Google Scholar] [CrossRef]
- Completo, C.; Semiao, V.; Geraldes, V. Efficient CFD-based method for designing cross-flow nanofiltration small devices. J. Membr. Sci. 2016, 500, 190–202. [Google Scholar] [CrossRef]
- Saeed, A.; Vuthaluru, R.; Vuthaluru, H.B. Investigations into the effects of mass transport and flow dynamics of spacer filled membrane modules using CFD. Chem. Eng. Res. Des. 2015, 93, 79–99. [Google Scholar] [CrossRef]
- Parvareh, A.; Rahimi, M.; Madaeni, S.; Alsairafi, A. Experimental and CFD Study on the Role of Fluid Flow Pattern on Membrane Permeate Flux. Chin. J. Chem. Eng. 2011, 19, 18–25. [Google Scholar] [CrossRef]
- Ren, J.; Chowdhury, M.R.; Xia, L.; Ma, C.; Bollas, G.M.; McCutcheon, J. A computational fluid dynamics model to predict performance of hollow fiber membrane modules in forward osmosis. J. Membr. Sci. 2020, 603, 117973. [Google Scholar] [CrossRef]
- Bucs, S.S.; Linares, R.V.; Marston, J.; Radu, A.I.; Vrouwenvelder, J.S.; Picioreanu, C. Experimental and numerical characterization of the water flow in spacer-filled channels of spiral-wound membranes. Water Res. 2015, 87, 299–310. [Google Scholar] [CrossRef] [PubMed]
- Lotfiyan, H.; Ashtiani, F.Z.; Fouladitajar, A.; Armand, S.B. Computational fluid dynamics modeling and experimental studies of oil-in-water emulsion microfiltration in a flat sheet membrane using Eulerian approach. J. Membr. Sci. 2014, 472, 1–9. [Google Scholar] [CrossRef]
- Wang, J.; Gao, X.; Ji, G.; Gu, X. CFD simulation of hollow fiber supported NaA zeolite membrane modules. Sep. Purif. Technol. 2019, 213, 1–10. [Google Scholar] [CrossRef]
- Saeed, A.; Vuthaluru, R.; Yang, Y.; Vuthaluru, H.B. Effect of feed spacer arrangement on flow dynamics through spacer filled membranes. Desalination 2012, 285, 163–169. [Google Scholar] [CrossRef]
- Feron, P.; Van Heuven, J.; Akkerhuis, J.; Van Der Welle, R. Design and development of a membrane testcell with uniform mass transfer: Application to characterisation of high flux gas separation membranes. J. Membr. Sci. 1993, 80, 157–164. [Google Scholar] [CrossRef]
- Darcovich, K.; Dal-Cin, M.; Ballèvre, S.; Wavelet, J.-P. CFD-assisted thin channel membrane characterization module design. J. Membr. Sci. 1997, 124, 181–193. [Google Scholar] [CrossRef][Green Version]
- Tarabara, V.V.; Wiesner, M.R. Computational fluid dynamics modeling of the flow in a laboratory membrane filtration cell operated at low recoveries. Chem. Eng. Sci. 2003, 58, 239–246. [Google Scholar] [CrossRef]
- Balannec, B.; Cortès-Juan, F.; Renouard, T. Design Improvement of a Bench-scale Nanofiltration Device by CFD, Comsol.Ch. 2010. Available online: https://www.comsol.ch/paper/download/63180/balannec_paper.pdf (accessed on 21 August 2020).
- Da Costa, A.; Fane, A.; Wiley, D. Spacer characterization and pressure drop modelling in spacer-filled channels for ultrafiltration. J. Membr. Sci. 1994, 87, 79–98. [Google Scholar] [CrossRef]
- Gu, B.; Adjiman, C.S.; Xu, X.Y. The effect of feed spacer geometry on membrane performance and concentration polarisation based on 3D CFD simulations. J. Membr. Sci. 2017, 527, 78–91. [Google Scholar] [CrossRef]
- Kawachale, N.; Kirpalani, D.M.; Kumar, A. A mass transport and hydrodynamic evaluation of membrane separation cell. Chem. Eng. Process. Process Intensif. 2010, 49, 680–688. [Google Scholar] [CrossRef][Green Version]
- Ranade, V.V.; Kumar, A. Fluid dynamics of spacer filled rectangular and curvilinear channels. J. Membr. Sci. 2006, 271, 1–15. [Google Scholar] [CrossRef]
- Wiley, D.E.; Fletcher, D.F. Techniques for computational fluid dynamics modelling of flow in membrane channels. J. Membr. Sci. 2003, 211, 127–137. [Google Scholar] [CrossRef]
- Vinchurkar, S.; Longest, P.W. Evaluation of hexahedral, prismatic and hybrid mesh styles for simulating respiratory aerosol dynamics. Comput. Fluids 2008, 37, 317–331. [Google Scholar] [CrossRef]
- Bracci, M.; Tarini, M.; Pietroni, N.; Livesu, M.; Cignoni, P. HexaLab.net: An online viewer for hexahedral meshes. Comput. Des. 2019, 110, 24–36. [Google Scholar] [CrossRef]
- Blažek, J. Governing Equations; Elsevier BV: Amsterdam, The Netherlands, 2015; pp. 7–27. [Google Scholar]
- Vasquez, S. A Phase Coupled Method for Solving Multiphase Problems on Unstructured Mesh. In Proceedings of the 2000 ASME Fluids Engineering Division Summer Meeting, Boston, MA, USA, 11–15 June 2000. [Google Scholar]
- Singh, R.; Purkait, M.K. Microfiltration Membranes. In Membrane Separation Principles and Applications; Elsevier: Amsterdam, The Netherlands, 2019; pp. 111–146. [Google Scholar]
- Schwinge, J. Characterization of a zigzag spacer for ultrafiltration. J. Membr. Sci. 2000, 172, 19–31. [Google Scholar] [CrossRef]






| Parameter | Value | ||||||
|---|---|---|---|---|---|---|---|
| Intrinsic Permeability (10−17 m2) | 1.042 | 2.084 | 3.125 | 4.167 1 | 5.209 | 6.251 | 7.292 |
| Membrane thickness (µm) | 50 | 100 | 150 1 | 200 | 250 | 300 | 350 |
| Inlet pressure (bar) | 0.05 | 0.10 | 0.15 | 0.20 1 | 0.25 | 0.30 | 0.35 |
| Parameter | Value | ||||||
|---|---|---|---|---|---|---|---|
| Spacer thickness (mm) | 0.5 | 1 2 | 1.5 | 2.0 | 2.5 | 3.0 | |
| Spacer curviness (%) | 0 2 | 20 | 40 | 60 | 80 | 100 | |
| Inlet length (mm) | 10 | 15 | 20 | 25 2 | 30 | 35 | 40 |
| Outlet length (mm) | 10 | 15 | 20 | 25 2 | 30 | 35 | 40 |
| Inlet diameter (mm) | 5 | 6 | 7 | 8 | 9 | 10 | 11 2 |
| Outlet diameter (mm) | 5 | 6 | 7 | 8 | 9 | 10 | 11 2 |
| Parameter | Permeate Flux | Transmembrane Pressure |
|---|---|---|
| Spacer curviness | ↓ | ↓ |
| Thickness of the spacer | ↓↓ | ↓↓ |
| Thickness of the outflow zone | – | – |
| Length of the inlet pipe | – | – |
| Length of the outlet pipe | – | – |
| Diameter of the inlet pipe | ↑ | ↑ |
| Diameter of the outlet pipe | ↓↓↓ | ↓↓↓ |
| Inlet pressure | ↑↑↑↑ | ↑↑↑↑ |
| Membrane permeability | ↑↑↑↑ | ↓ |
| Membrane thickness | ↓↓↓↓ | ↑ |
| Membrane area size | ↓↓↓↓ | – |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bopape, M.F.; Van Geel, T.; Dutta, A.; Van der Bruggen, B.; Onyango, M.S. Numerical Modelling Assisted Design of a Compact Ultrafiltration (UF) Flat Sheet Membrane Module. Membranes 2021, 11, 54. https://doi.org/10.3390/membranes11010054
Bopape MF, Van Geel T, Dutta A, Van der Bruggen B, Onyango MS. Numerical Modelling Assisted Design of a Compact Ultrafiltration (UF) Flat Sheet Membrane Module. Membranes. 2021; 11(1):54. https://doi.org/10.3390/membranes11010054
Chicago/Turabian StyleBopape, Mokgadi F, Tim Van Geel, Abhishek Dutta, Bart Van der Bruggen, and Maurice Stephen Onyango. 2021. "Numerical Modelling Assisted Design of a Compact Ultrafiltration (UF) Flat Sheet Membrane Module" Membranes 11, no. 1: 54. https://doi.org/10.3390/membranes11010054
APA StyleBopape, M. F., Van Geel, T., Dutta, A., Van der Bruggen, B., & Onyango, M. S. (2021). Numerical Modelling Assisted Design of a Compact Ultrafiltration (UF) Flat Sheet Membrane Module. Membranes, 11(1), 54. https://doi.org/10.3390/membranes11010054







