Theoretical Framework for Characterizing Strain-Dependent Dynamic Soil Properties
Abstract
:1. Introduction
2. Explicit Formulas to Describe Nonlinear-Hysteretic Response
2.1. Constitutive Model: Stress-Strain Relation
2.2. Backbone Curve
2.3. Modulus Degradation
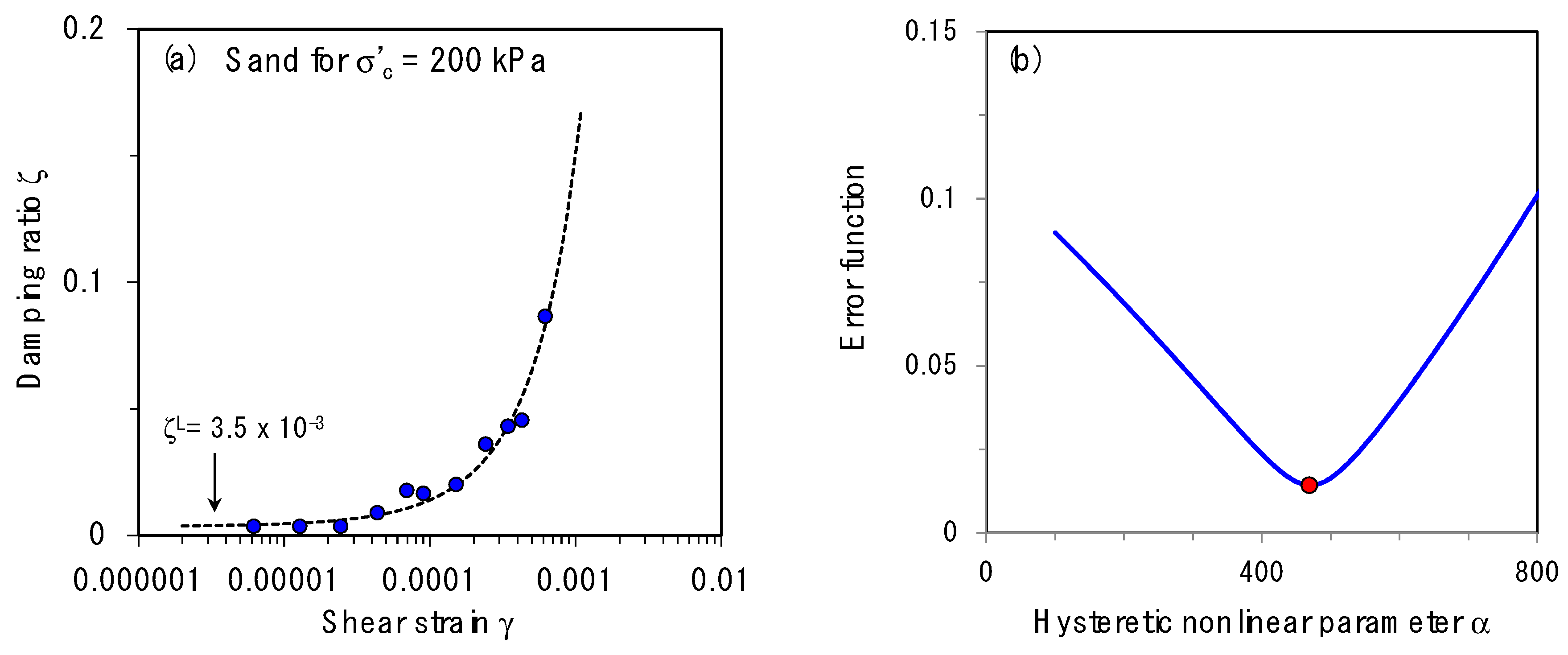
2.4. Damping Ratio
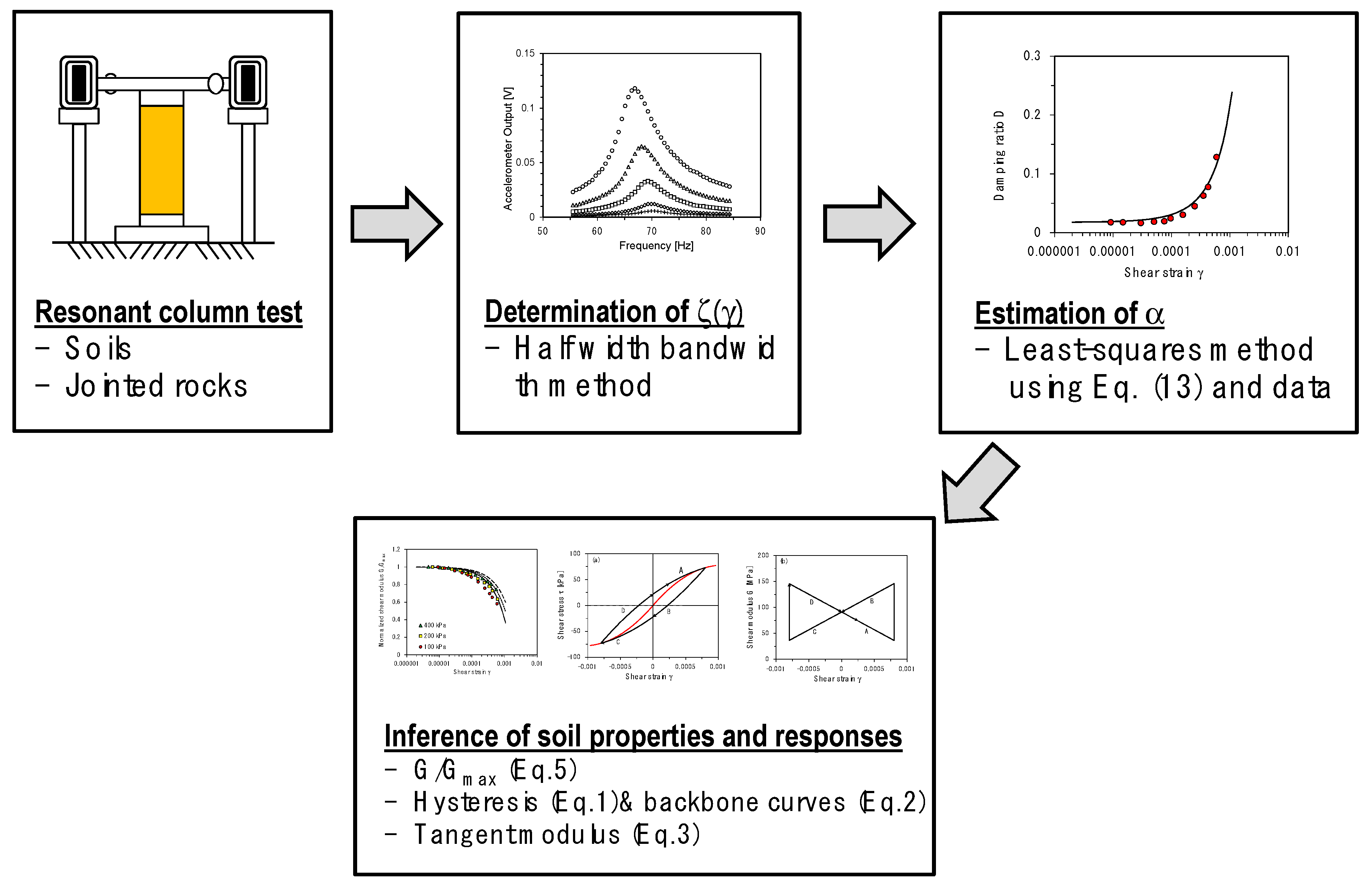
3. Results and Analysis
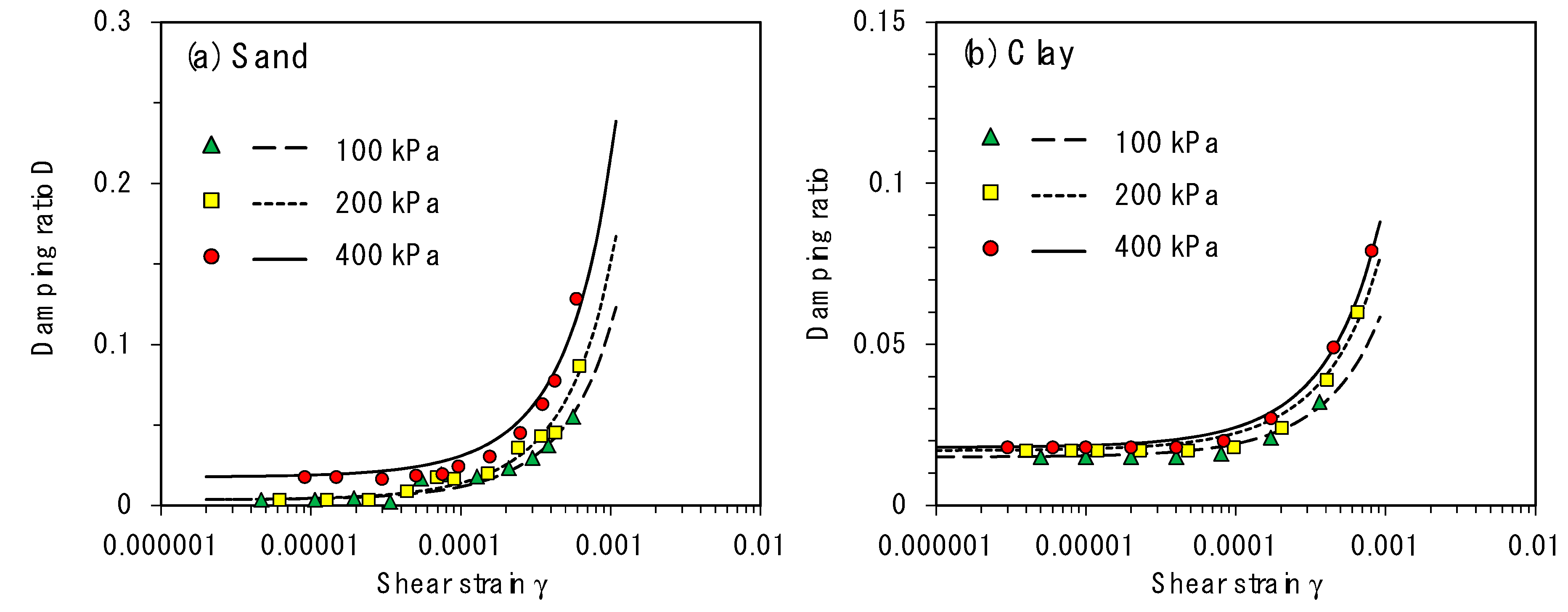
3.1. Examples
3.2. Correlation: Hysteretic Parameter and Confinement Stress
3.3. Comments: Shear Strain Range
4. Conclusions
- The constitutive model derives explicit formulas to describe the hysteretic nonlinear response. Note that all other models derived from this study model depend on the hysteretic nonlinear parameter α.
- The 36 damping ratio data sets measured from resonant column test were compared with the proposed model for different confinement stress levels and soils. It shows the inverse relation between the α parameter and confinement stress and higher α values for sand.
- The data analysis reveals that the model is valid for αγ < 1, and thus the explicit formula can be used to simulate the ground motion within intermediate strain range.
Funding
Conflicts of Interest
References
- Kramer, S.L. Geotechnical Earthquake Engineering; PrenticeHall: Englewood Cliffs, NJ, USA, 1996. [Google Scholar]
- Yamamuro, J.A.; Kaliakin, V.N. Soil Constitutive Models: Evaluation, Selection, and Calibration; Geotechnical Special Publication No. 128; American Society of Civil Engineering: Reston, VA, USA, 2005. [Google Scholar]
- Brinkgreve, R.B. Selection of Soil Models and Parameters for Geotechnical Engineering Application; Geotechnical Special Publication No.128; American Society of Civil Engineering: Reston, VA, USA, 2005; pp. 69–98. [Google Scholar]
- Kondner, R.L. Hyperbolic stress-strain response: Cohesive soils. J. Soil Mech. Found. Div. 1963, 89, 115–143. [Google Scholar]
- Duncan, J.M.; Chang, C.Y. Non-linear analysis of stress and strain in soils. J. Soil Mech. Found. Div. 1970, 96, 1629–1653. [Google Scholar]
- Darendeli, M.B. Development of a New Family of Normalized Modulus Reduction and Material Damping Curves. Ph.D. Thesis, University of Texas at Austin, Austin, TX, USA, 2001. [Google Scholar]
- Ramberg, W.; Osgood, W.R. Description of Stress-Strain Curves by Three Parameters; Technical Note No. 902; National Advisory Committee for Aeronautics: Washington, DC, USA, 1943.
- Hardin, B.O.; Drnecivh, V.P. Shear modulus and damping in soils: Design equation and curve. J. Soil Mech. Found. Div. 1972, 98, 667–692. [Google Scholar]
- Zhang, J.; Andrus, R.D.; Juang, C.H. Normalized shear modulus and material damping ratio relationships. J. Geotech. Geoenviron. Eng. 2005, 131, 453–464. [Google Scholar] [CrossRef]
- Assimaki, D.; Kausel, E.; Whittle, A. Model for dynamic shear modulus and damping for granular soils. J. Geotech. Geoenviron. Eng. 2000, 126, 859–869. [Google Scholar] [CrossRef]
- Pyke, R. Nonlinear soil models for irregular cyclic loadings. J. Geotech. Eng. 1979, 105, 715–725. [Google Scholar]
- Chiang, D.-Y. The generalized masing models for deteriorating hysteresis and cyclic plasticity. Appl. Math. Model. 1999, 23, 847–863. [Google Scholar] [CrossRef]
- Muravskii, G. On description of hysteretic behaviour of materials. Int. J. Solids Struct. 2005, 42, 2625–2644. [Google Scholar] [CrossRef]
- Iwan, W.D. A distributed-element model for hysteresis and its steady-state dynamic response. J. Appl. Mech. 1966, 33, 893–900. [Google Scholar] [CrossRef]
- Bolton, M.D.; Wilson, J.M.R. An experimental and theoretical comparison between static and dynamic torsional soil tests. Géotechnique 1989, 39, 585–599. [Google Scholar] [CrossRef]
- Matasović, N.; Vucetic, M. Cyclic characterization of liquefiable sands. J. Geotech. Eng. 1993, 119, 1805–1822. [Google Scholar] [CrossRef]
- Hashash, Y.M.A.; Park, D. Non-linear one-dimensional seismic ground motion propagation in the mississippi embayment. Eng. Geol. 2001, 62, 185–206. [Google Scholar] [CrossRef]
- Chong, S.-H. Soil dynamic constitutive model for characterizing the nonlinear-hysteretic response. Appl. Sci. 2017, 7, 1110. [Google Scholar] [CrossRef]
- McCall, K.R.; Guyer, R.A. Equation of state and wave propagation in hysteretic nonlinear elastic materials. J. Geophys. Res. Solid Earth 1994, 99, 23887–23897. [Google Scholar] [CrossRef]
- Guyer, R.A.; McCall, K.R.; Boitnott, G.N. Hysteresis, discrete memory, and nonlinear wave propagation in rock: A new paradigm. Phys. Rev. Lett. 1995, 74, 3491–3494. [Google Scholar] [CrossRef]
- Guyer, R.A.; Johnson, P.A. Nonlinear mesoscopic elasticity: Evidence for a new class of materials. Phys. Today 1999, 52, 30–36. [Google Scholar] [CrossRef]
- Guyer, R.A.; McCall, K.R.; Boitnott, G.N.; Hilbert, L.B.; Plona, T.J. Quantitative implementation of preisach-mayergoyz space to find static and dynamic elastic moduli in rock. J. Geophys. Res. Solid Earth 1997, 102, 5281–5293. [Google Scholar] [CrossRef]
- Van Den Abeele, K.E.; Johnson, P.A.; Guyer, R.A.; McCall, K.R. On the quasi-analytic treatment of hysteretic nonlinear response in elastic wave propagation. J. Acoust. Soc. Am. 1997, 101, 1885–1898. [Google Scholar] [CrossRef]
- Cabaret, J.; Béquin, P.; Theocharis, G.; Andreev, V.; Gusev, V.E.; Tournat, V. Nonlinear hysteretic torsional waves. Phys. Rev. Lett. 2015, 115, 054301. [Google Scholar] [CrossRef]
- Guyer, R.A.; Johnson, P.A. Nonlinear Mesoscopic Elasticity: The Complex Behaviour of Rocks, Soil, Concrete; John Wiley & Sons: Hoboken, NY, USA, 2009. [Google Scholar]
- Chong, S.-H.; Kim, J.-Y. Nonlinear vibration analysis of the resonant column test of granular materials. J. Sound Vib. 2017, 393, 216–228. [Google Scholar] [CrossRef]
- Chong, S.-H. The Effect of Subsurface Mass Loss on the Response of Shallow Foundations; Georgia Institute of Technology: Atlanta, GA, USA, 2014. [Google Scholar]
- Chong, S.-H.; Santamarina, J.C. Soil compressibility models for a wide stress range. J. Geotech. Geoenviron. Eng. 2016, 142, 06016003. [Google Scholar] [CrossRef]
- Kim, D.-S. Deformational Characteristics of Soils at Small to Intermediate Strains from Cyclic Tests. Ph.D. Thesis, University of Texas at Austin, Austin, TX, USA, 1991. [Google Scholar]
- Lu, Z. The phase shift method for studying nonlinear acoustics in a soil. Acta Acust. United Acust. 2007, 93, 542–554. [Google Scholar]
- Guyer, R.A.; TenCate, J.; Johnson, P. Hysteresis and the dynamic elasticity of consolidated granular materials. Phys. Rev. Lett. 1999, 82, 3280–3283. [Google Scholar] [CrossRef]
- Aleshin, V.; Van Den Abeele, K. Friction in unconforming grain contacts as a mechanism for tensorial stress–strain hysteresis. J. Mech. Phys. Solids 2007, 55, 765–787. [Google Scholar] [CrossRef]



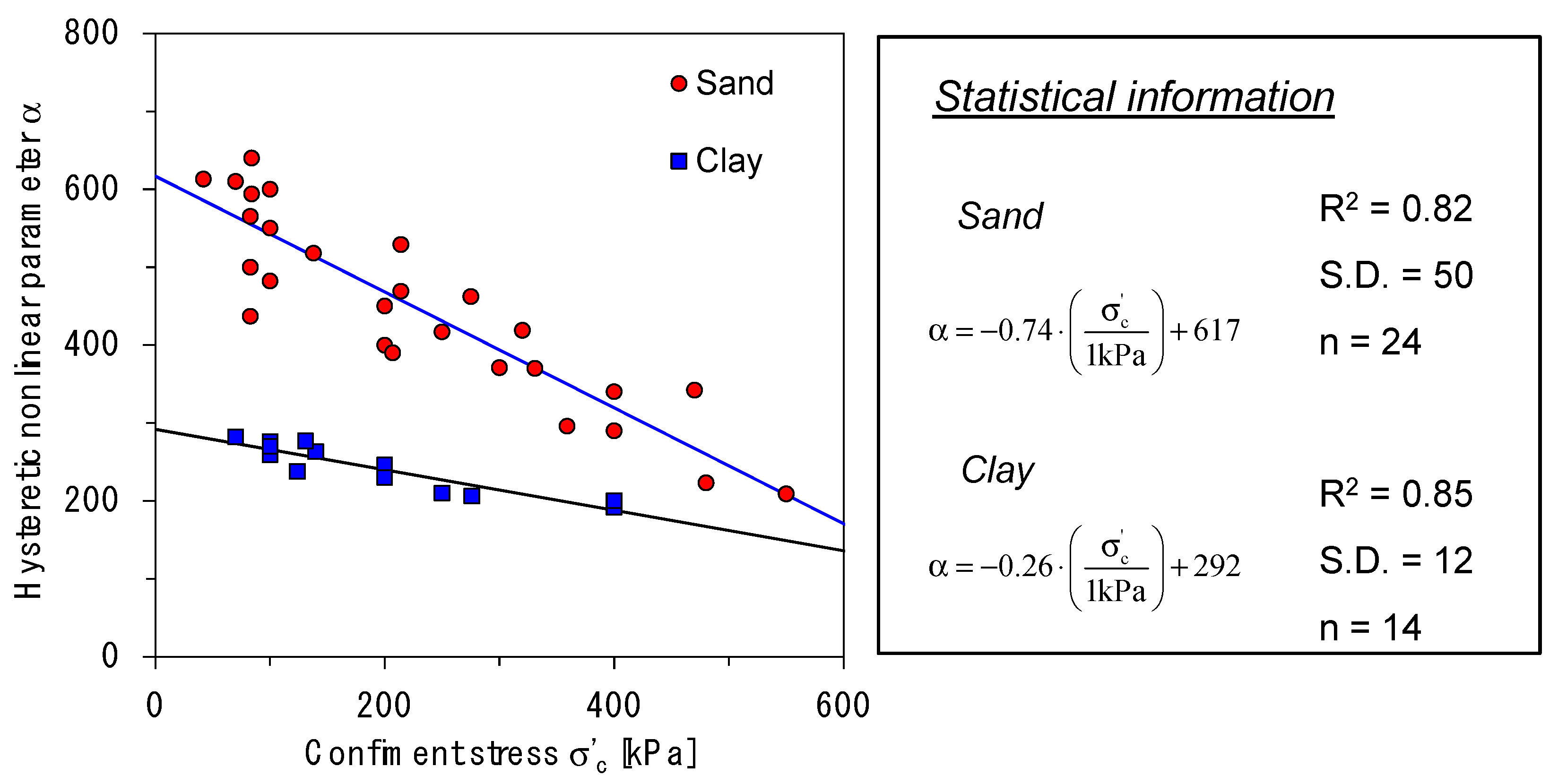
| Sand | Clay | ||||||
|---|---|---|---|---|---|---|---|
| # | σ’c [kPa] | α [ ] | References | # | σ’c [kPa] | α [ ] | References |
| 1 | 42 | 613 | [9] | 1 | 100 | 276 | [29] |
| 2 | 83 | 565 | 2 | 200 | 246 | ||
| 3 | 214 | 469 | 3 | 400 | 192 | ||
| 4 | 300 | 371 | 4 | 124 | 238 | ||
| 5 | 84 | 594 | 5 | 140 | 263 | ||
| 6 | 84 | 640 | 6 | 131 | 277 | ||
| 7 | 331 | 370 | 7 | 70 | 282 | [9] | |
| 8 | 359 | 296 | 8 | 276 | 206 | ||
| 9 | 214 | 529 | 9 | 250 | 210 | ||
| 10 | 470 | 342 | 10 | 100 | 260 | ||
| 11 | 275 | 462 | 11 | 100 | 259 | ||
| 12 | 250 | 417 | 12 | 100 | 270 | ||
| 13 | 320 | 419 | 13 | 200 | 230 | ||
| 14 | 480 | 223 | 14 | 400 | 200 | ||
| 15 | 550 | 209 | |||||
| 16 | 100 | 590 | [29] | ||||
| 17 | 200 | 469 | |||||
| 18 | 400 | 370 | |||||
| 19 | 70 | 610 | |||||
| 20 | 138 | 518 | |||||
| 21 | 207 | 390 | |||||
| 22 | 83 | 437 | |||||
| 23 | 83 | 500 | |||||
| 24 | 100 | 482 | |||||
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chong, S.-H. Theoretical Framework for Characterizing Strain-Dependent Dynamic Soil Properties. Appl. Sci. 2019, 9, 1897. https://doi.org/10.3390/app9091897
Chong S-H. Theoretical Framework for Characterizing Strain-Dependent Dynamic Soil Properties. Applied Sciences. 2019; 9(9):1897. https://doi.org/10.3390/app9091897
Chicago/Turabian StyleChong, Song-Hun. 2019. "Theoretical Framework for Characterizing Strain-Dependent Dynamic Soil Properties" Applied Sciences 9, no. 9: 1897. https://doi.org/10.3390/app9091897
APA StyleChong, S.-H. (2019). Theoretical Framework for Characterizing Strain-Dependent Dynamic Soil Properties. Applied Sciences, 9(9), 1897. https://doi.org/10.3390/app9091897





