Probing Globular Protein Self-Assembling Dynamics by Heterodyne Transient Grating Experiments
Abstract
1. Introduction
2. Experimental Methods
2.1. Sample Preparation
2.2. Transient Grating Experiments
2.3. Data Collection
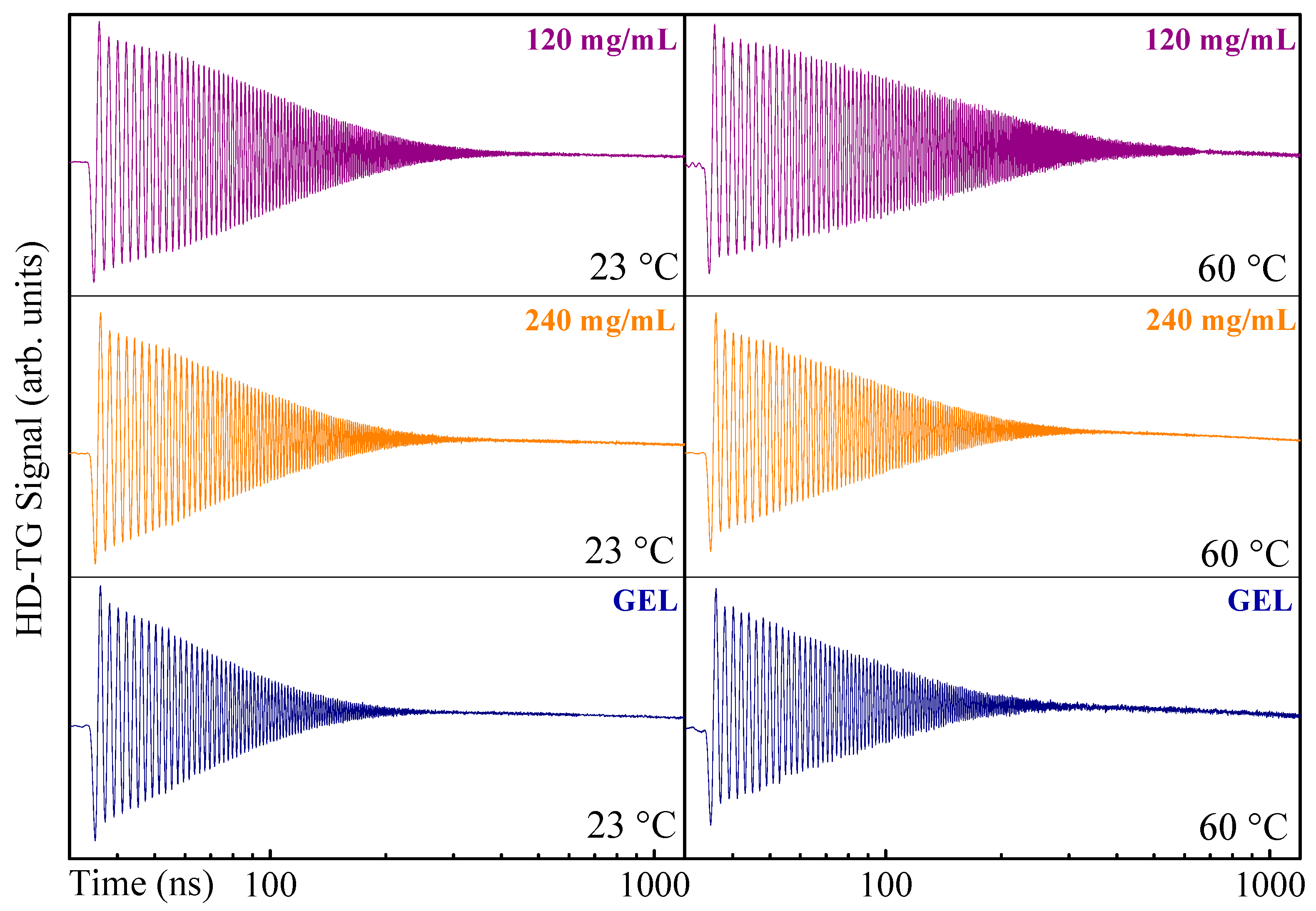
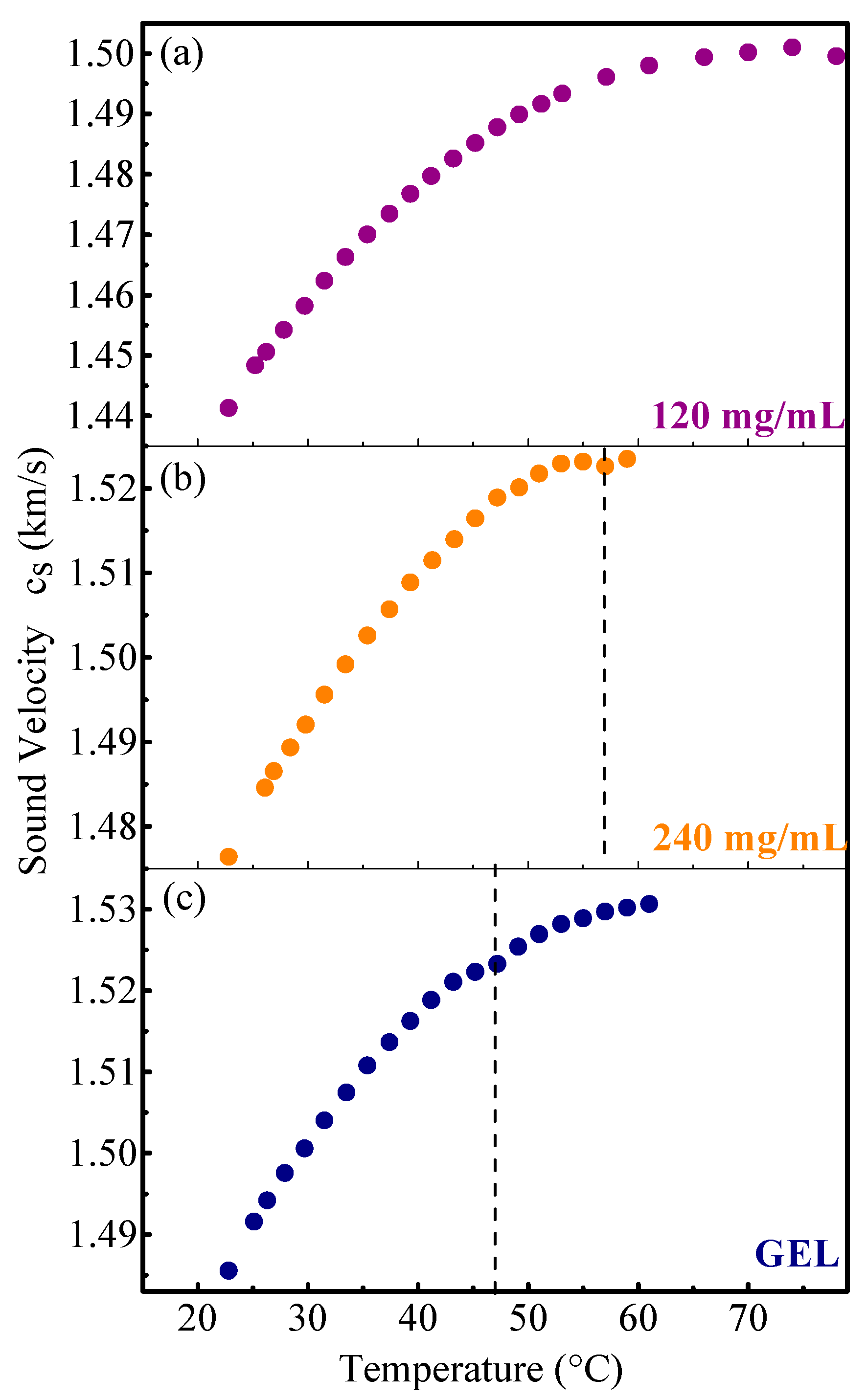
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dobson, C.M. Principles of protein folding, misfolding and aggregation. Semin. Cell Dev. Biol. 2004, 15, 3–16. [Google Scholar] [CrossRef] [PubMed]
- Stradner, A.; Sedgwick, H.; Cardinaux, F.; Poon, W.C.; Egelhaaf, S.U.; Schurtenberger, P. Equilibrium cluster formation in concentrated protein solutions and colloids. Nature 2004, 432, 492–495. [Google Scholar] [CrossRef] [PubMed]
- Porcar, L.; Falus, P.; Chen, W.R.; Faraone, A.; Fratini, E.; Hong, K.L.; Baglioni, P.; Liu, Y. Formation of the Dynamic Clusters in Concentrated Lysozyme Protein Solutions. J. Phys. Chem. Lett. 2010, 1, 126–129. [Google Scholar] [CrossRef]
- Sassi, P.; Onori, G.; Giugliarelli, A.; Paolantoni, M.; Cinelli, S.; Morresi, A. Conformational changes in the unfolding process of lysozyme in water and ethanol/water solutions. J. Mol. Liquids 2011, 159, 112–116. [Google Scholar] [CrossRef]
- Giugliarelli, A.; Tarpani, L.; Latterini, L.; Morresi, A.; Paolantoni, M.; Sassi, P. Spectroscopic and Microscopic Studies of Aggregation and Fibrillation of Lysozyme in Water/Ethanol Solutions. J. Phys. Chem. B 2015, 119, 13009–13017. [Google Scholar] [CrossRef] [PubMed]
- Yan, H.; Frielinghaus, H.; Nykanen, A.; Ruokolainen, J.; Saiani, A.; Miller, A.F. Thermoreversible lysozyme hydrogels: Properties and an insight into the gelation pathway. Soft Matter 2008, 4, 1313–1325. [Google Scholar] [CrossRef]
- Sassi, P.; Giugliarelli, A.; Paolantoni, M.; Morresi, A.; Onori, G. Unfolding and aggregation of lysozyme: A thermodynamic and kinetic study by FTIR spectroscopy. Biophys. Chem. 2011, 158, 46–53. [Google Scholar] [CrossRef]
- Yan, C.; Pochan, D.J. Rheological properties of peptide-based hydrogels for biomedical and other applications. Chem. Soc. Rev. 2010, 39, 3528–3540. [Google Scholar] [CrossRef]
- Jonker, A.M.; Lowik, D.W.P.M.; van Hest, J.C.M. Peptide- and Protein-Based Hydrogels. Chem. Mater. 2012, 24, 759–773. [Google Scholar] [CrossRef]
- Yan, H.; Saiani, A.; Gough, J.E.; Miller, A.F. Thermoreversible protein hydrogel as cell scaffold. Biomacromolecules 2006, 7, 2776–2782. [Google Scholar] [CrossRef]
- Khoury, L.R.; Nowitzke, J.; Shmilovich, K.; Popa, I. Study of Biomechanical Properties of Protein-Based Hydrogels Using Force-Clamp Rheometry. Macromolecules 2018, 51, 1441–1452. [Google Scholar] [CrossRef]
- Sahoo, S.; Ouyang, H.; Goh, J.C.; Tay, T.E.; Toh, S.L. Characterization of a novel polymeric scaffold for potential application in tendon/ligament tissue engineering. Tissue Eng. 2006, 12, 91–99. [Google Scholar] [CrossRef] [PubMed]
- Yan, H.; Nykanen, A.; Ruokolainen, J.; Farrar, D.; Gough, J.E.; Saiani, A.; Miller, A.F. Thermo-reversible protein fibrillar hydrogels as cell scaffolds. Faraday Discuss. 2008, 139, 71–84. [Google Scholar] [CrossRef] [PubMed]
- Catalini, S. Studio Spettroscopico dei Processi di Aggregazione e Gelificazione del Lisozima in Soluzione Acida. Master Thesis, Dip. di Chimica, Biologia e Biotecnologie, Università di Perugia, Perugia, Italy, 2016. [Google Scholar]
- Raccosta, S.; Manno, M.; Bulone, D.; Giacomazza, D.; Militello, V.; Martorana, V.; San Biagio, P.L. Irreversible gelation of thermally unfolded proteins: Structural and mechanical properties of lysozyme aggregates. Eur. Biophys. J. 2010, 39, 1007. [Google Scholar] [CrossRef] [PubMed]
- Bryant, C.M.; McClements, D.J. Ultrasonic spectroscopy study of relaxation and scattering in whey protein solutions. J. Sci. Food Agric. 1999, 79, 1754–1760. [Google Scholar] [CrossRef]
- Corredig, M.; Alexander, M.; Dalgleish, D.G. The application of ultrasonic spectroscopy to the study of the gelation of milk components. Food Res. Int. 2004, 37, 557–565. [Google Scholar] [CrossRef]
- Parker, N.G.; Povey, M.J.W. Ultrasonic study of the gelation of gelatin: Phase diagram, hysteresis and kinetics. Food Hydrocoll. 2012, 26, 99–107. [Google Scholar] [CrossRef]
- Lamanna, L.; Rizzi, F.; Demitri, C.; Pisanello, M.; Scarpa, E.; Qualtieri, A.; Sannino, A.; De Vittorio, M. Determination of absorption and structural properties of cellulose-based hydrogel via ultrasonic pulse-echo time-of-flight approach. Cellulose 2018, 25, 4331–4343. [Google Scholar] [CrossRef]
- Meng, Z.; Thakur, T.; Chitrakar, C.; Jaiswal, M.K.; Gaharwar, A.K.; Yakovlev, V.V. Assessment of Local Heterogeneity in Mechanical Properties of Nanostructured Hydrogel Networks. ACS Nano 2017, 11, 7690–7696. [Google Scholar] [CrossRef]
- Bottari, C.; Comez, L.; Corezzi, S.; D’Amico, F.; Gessini, A.; Mele, A.; Punta, C.; Melone, L.; Pugliese, A.; Masciovecchio, C.; et al. Correlation between collective and molecular dynamics in pH-responsive cyclodextrin-based hydrogels. Phys. Chem. Chem. Phys. PCCP 2017, 19, 22555–22563. [Google Scholar] [CrossRef]
- Pavlovskaya, G.; Mcclements, D.J.; Povey, M.J.W. Ultrasonic Investigation of Aqueous-Solutions of a Globular Protein. Food Hydrocoll. 1992, 6, 253–262. [Google Scholar] [CrossRef]
- O’Brien, W.D., Jr.; Dunn, F. Ultrasonic absorption mechanisms in aqueous solutions of bovine hemoglobin. J. Phys. Chem. 1972, 76, 528–533. [Google Scholar] [CrossRef] [PubMed]
- Cho, K.C.; Leung, W.P.; Mok, H.Y.; Choy, C.L. Ultrasonic absorption in myoglobin and other globular proteins. Biochim. Biophys. Acta 1985, 830, 36–44. [Google Scholar] [CrossRef]
- Kanda, H.; Ookubo, N.; Nakajima, H.; Suzuki, Y.; Minato, M. Ultrasonic absorption in aqueous solution of lysozyme. Biopolymers 1976, 15, 785–795. [Google Scholar] [CrossRef] [PubMed]
- Schwarz, G. On the Kinetics of the Helix-Coil Transition of Polypeptides in Solution. J. Mol. Biol. 1965, 11, 64–77. [Google Scholar] [CrossRef]
- Kessler, L.W.; Dunn, F. Ultrasonic investigation of the conformal changes of bovine serum albumin in aqueous solution. J. Phys. Chem. 1969, 73, 4256–4263. [Google Scholar] [CrossRef]
- Taschin, A.; Eramo, R.; Bartolini, P.; Torre, R. Transient Grating Experiments in Glass-Former Liquids, A Unique Tool to Investigate Complex Dynamics. In Time-Resolved Spectroscopy in Complex Liquids; Torre, R., Ed.; Springer: Boston, MA, USA, 2008. [Google Scholar] [CrossRef]
- Taschin, A.; Cucini, R.; Bartolini, P.; Torre, R. Does there exist an anomalous sound dispersion in supercooled water? Philos. Mag. 2011, 91, 1796–1800. [Google Scholar] [CrossRef]
- Taschin, A.; Bartolini, P.; Eramo, R.; Torre, R. Supercooled water relaxation dynamics probed with heterodyne transient grating experiments. Phys. Rev. E 2006, 74, 031502. [Google Scholar] [CrossRef]
- Pratesi, G.; Bartolini, P.; Senatra, D.; Ricci, M.; Righini, R.; Barocchi, F.; Torre, R. Experimental studies of the ortho-toluidine glass transition. Phys. Rev. E 2003, 67, 021505. [Google Scholar] [CrossRef]
- Pick, R.M.; Dreyfus, C.; Azzimani, a.; Gupta, R.; Torre, R.; Taschin, a.; Franosch, T. Heterodyne detected transient gratings in supercooled molecular liquids. Eur. Phys. J. B 2004, 39, 169–197. [Google Scholar] [CrossRef]
- Amirkhani, M.; Taschin, A.; Cucini, R.; Bartolini, P.; Leporini, D.; Torre, R. Polymer thermal and acoustic properties using heterodyne detected transient grating technique. J. Polym. Sci. Part B Polym. Phys. 2011, 49, 685–690. [Google Scholar] [CrossRef]
- Malfanti, I.; Taschin, a.; Bartolini, P.; Bonello, B.; Torre, R. Propagation of acoustic surface waves on a phononic surface investigated by transient reflecting grating spectroscopy. J. Mech. Phys. Solids 2011, 59, 2370–2381. [Google Scholar] [CrossRef]
- Taschin, A.; Cucini, R.; Bartolini, P.; Torre, R. Temperature of maximum density of water in hydrophilic confinement measured by transient grating spectroscopy. EPL (Europhys. Lett.) 2010, 92, 26005. [Google Scholar] [CrossRef]
- Plazanet, M.; Bartolini, P.; Torre, R.; Petrillo, C.; Sacchetti, F. Structure and acoustic properties of hydrated nafion membranes. J. Phys. Chem. B 2009, 113, 10121–10127. [Google Scholar] [CrossRef] [PubMed]
- Cucini, R.; Taschin, a.; Bartolini, P.; Torre, R. Acoustic, thermal and flow processes in a water filled nanoporous glass by time-resolved optical spectroscopy. J. Mech. Phys. Solids 2010, 58, 1302–1317. [Google Scholar] [CrossRef]
- Taschin, A.; Cucini, R.; Bartolini, P.; Torre, R. Acoustic phenomena in porous media studied by transient grating spectroscopy: A critical test of the Biot theory. EPL (Europhys. Lett.) 2008, 81, 58003. [Google Scholar] [CrossRef]
- Taschin, A.; Bartolini, P.; Sánchez-Ferrer, A.; Mezzenga, R.; Mrzel, A.; Torre, R. Investigation of Relaxation Processes in Nanocomposites by Transient Grating Experiments. Mater. Sci. Forum 2012, 714, 79–83. [Google Scholar] [CrossRef]
- Cucini, R.; Taschin, a.; Bartolini, P.; Torre, R. Acoustic phenomena and hydrodynamic flow in a water filled nano-porous glass studied by transient grating spectroscopy. J. Phys. Conf. Ser. 2010, 214, 012032. [Google Scholar] [CrossRef]
- Azzimani, A.; Dreyfus, C.; Pick, R.; Bartolini, P.; Taschin, a.; Torre, R. Analysis of a heterodyne-detected transient-grating experiment on a molecular supercooled liquid. I. Basic formulation of the problem. Phys. Rev. E 2007, 76, 011509. [Google Scholar] [CrossRef] [PubMed]
- Wegge, R.; Richter, M.; Span, R. Speed of sound measurements in deuterium oxide (D2O) over the temperature range from (278.2 to 353.2) K at pressures up to 20 MPa. Fluid Phase Equilibria 2016, 418, 175–180. [Google Scholar] [CrossRef]
- Matheus, S.; Friess, W.; Mahler, H.C. FTIR and nDSC as analytical tools for high-concentration protein formulations. Pharm Res 2006, 23, 1350–1363. [Google Scholar] [CrossRef] [PubMed]
- Zou, Y.; Li, Y.; Hao, W.; Hu, X.; Ma, G. Parallel beta-sheet fibril and antiparallel beta-sheet oligomer: New insights into amyloid formation of hen egg white lysozyme under heat and acidic condition from FTIR spectroscopy. J. Phys. Chem. B 2013, 117, 4003–4013. [Google Scholar] [CrossRef]
- Giugliarelli, A.; Paolantoni, M.; Morresi, A.; Sassi, P. Denaturation and preservation of globular proteins: The role of DMSO. J. Phys. Chem. B 2012, 116, 13361–13367. [Google Scholar] [CrossRef] [PubMed]
- Corredig, M.; Dalgleish, D.G. Effect of temperature and pH on the interactions of whey proteins with casein micelles in skim milk. Food Res. Int. 1996, 29, 49–55. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Catalini, S.; Taschin, A.; Bartolini, P.; Foggi, P.; Torre, R. Probing Globular Protein Self-Assembling Dynamics by Heterodyne Transient Grating Experiments. Appl. Sci. 2019, 9, 405. https://doi.org/10.3390/app9030405
Catalini S, Taschin A, Bartolini P, Foggi P, Torre R. Probing Globular Protein Self-Assembling Dynamics by Heterodyne Transient Grating Experiments. Applied Sciences. 2019; 9(3):405. https://doi.org/10.3390/app9030405
Chicago/Turabian StyleCatalini, Sara, Andrea Taschin, Paolo Bartolini, Paolo Foggi, and Renato Torre. 2019. "Probing Globular Protein Self-Assembling Dynamics by Heterodyne Transient Grating Experiments" Applied Sciences 9, no. 3: 405. https://doi.org/10.3390/app9030405
APA StyleCatalini, S., Taschin, A., Bartolini, P., Foggi, P., & Torre, R. (2019). Probing Globular Protein Self-Assembling Dynamics by Heterodyne Transient Grating Experiments. Applied Sciences, 9(3), 405. https://doi.org/10.3390/app9030405





