An Approximate Analytic Solution to a Non-Linear ODE for Air Jet Velocity Decay through Tree Crops Using Piecewise Linear Emulations and Rectangle Functions
Abstract
:Featured Application
Abstract
1. Introduction
- (a)
- The foliage is uniformly distributed in space, and subsequently, the LAD (leaf area density) is constant.
- (b)
- The forward motion of the sprayer is ignored since its velocity is one order of magnitude lower than the air velocity from fan exit, and no effect of travel speed on spray deposition in the canopy was experimentally observed [9].
- (c)
- The inverse relationship between the drag coefficient and air velocity is considered.
2. Mathematical Modeling of the Air Jet Velocity in the Canopy
2.1. Previous Results
- (a)
- Foliage is uniformly distributed in space,
- (b)
- Forward motion of the sprayer is ignored.
2.2. Linearization of the Non-Linear ODE
2.3. Solution of the Linear ODE Series and Their Combination
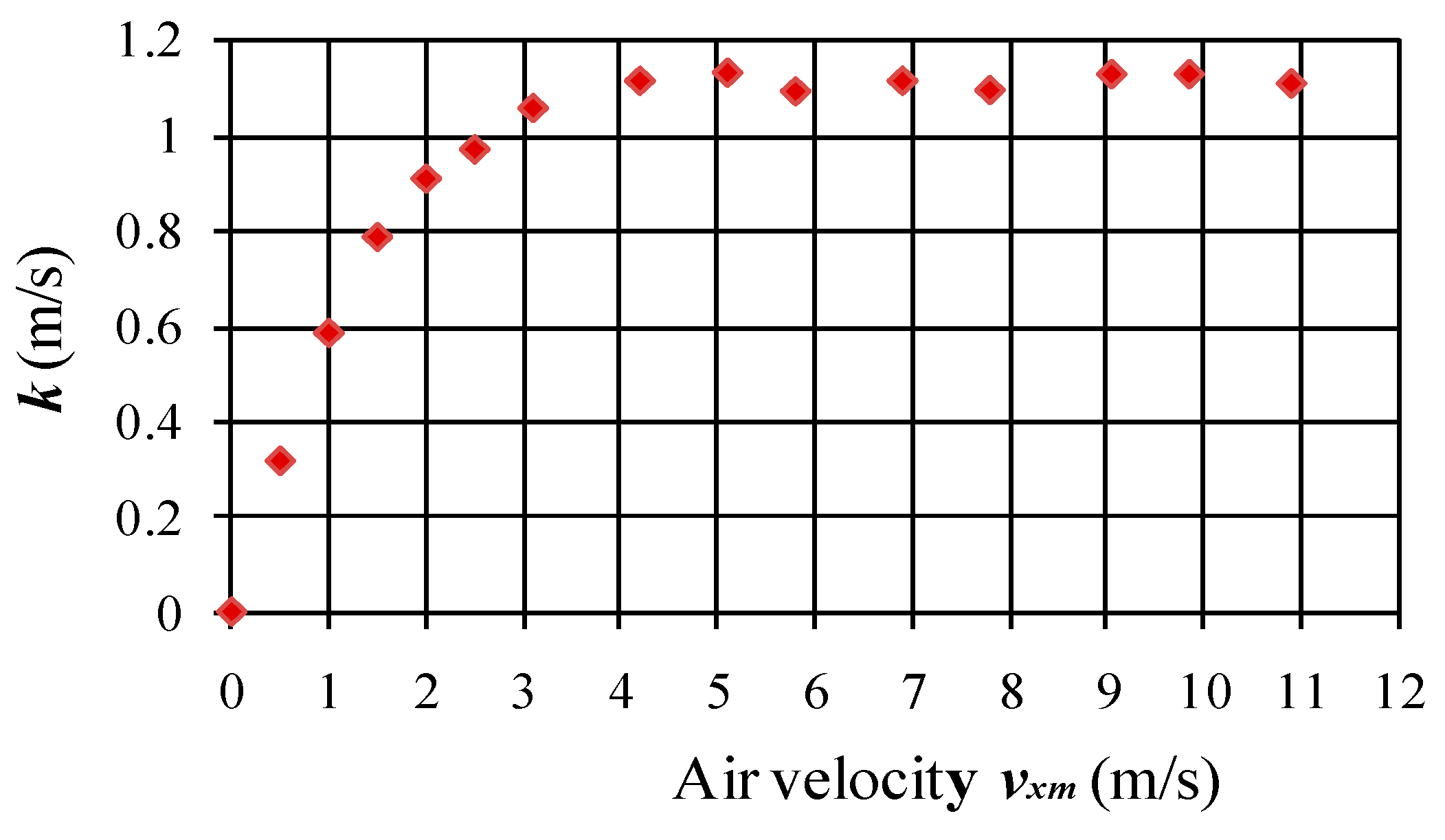
3. Experimental Evaluation
4. Results
5. Conclusions
Funding
Conflicts of Interest
References
- Marucco, P.; Tamagnone, M.; Balsari, P. Study of air velocity adjustment to maximise spray deposition in peach orchards. Agric. Eng. Int. CIGR J. 2008, X. May, manuscript ALNARP 08 009. 1–13. [Google Scholar]
- Pezzi, F.; Rondelli, V. The Performance of an Air-assisted Sprayer operating in Vines. J. Agric. Eng. Res. 2000, 76, 331–340. [Google Scholar] [CrossRef]
- Salcedo, R.; Garcera, C.; Granell, R.; Molto, E.; Chueca, P. Description of the airflow produced by an air assisted sprayer during pesticide applications to citrus. Span. J. Agric. Res. 2015, 13, e0208. [Google Scholar] [CrossRef] [Green Version]
- Salcedo, R.; Granel, R.; Palau, G.; Vallet, A.; Garcera, C.; Chueca, P.; Molto, E. Design and validation of a 2D CFD model of the airflow produced by an airblast sprayer during pesticide treatments of citrus. Comput. Electron. Agric. 2015, 116, 150–161. [Google Scholar] [CrossRef]
- Sun, C.; Qiu, W.; Ding, W.; Gu, J. Parameter optimization and experiment of air-assisted spraying on pear trees. Trans. Chin. Soc. Agric. Eng. 2015, 31, 30–38. [Google Scholar] [CrossRef]
- Cerruto, E.; Balsari, P.; Oggero, G.; Friso, D.; Guarella, A.; Raffaelli, W. Operator safety during pesticide application in greenhouses: A survey on Italian situation. Acta Hortic. 2008, 801, 1504–1514. [Google Scholar] [CrossRef]
- Holownicki, R.; Doruchowski, G.; Godyn, A.; Swiechowski, W. Variation of spray deposit and loss with air-jet directions applied in orchards. J. Agric. Eng. Res. 2000, 77, 129–136. [Google Scholar] [CrossRef]
- Friso, D.; Baldoin, C.; Pezzi, F. Mathematical modeling of the dynamics of air jet crossing the canopy of tree crops during pesticide application. Appl. Math. Sci. 2015, 9, 1281–1296. [Google Scholar] [CrossRef]
- Pascuzzi, S.; Cerruto, E. Spray deposition in “tendone” vineyards when using a pneumatic electrostatic sprayer. Crop Prot. 2015, 68, 1–11. [Google Scholar] [CrossRef]
- Vollsinger, S.; Mitchell, S.J.; Byrne, K.E.; Novak, M.D.; Rudnicki, M. Wind tunnel measurements of crown streamlining and drag relationships for several hardwood species. Can. J. For. Res. 2005, 35, 1238–1249. [Google Scholar] [CrossRef]
- Chapman, J.A.; Wilson, B.N.; Gulliver, J.S. Drag force parameters of rigid and flexible vegetal elements. Water Resour. Res. 2015, 51, 3292–3302. [Google Scholar] [CrossRef]
- Schütz, P.; Bode, M.; Gafiichuk, V.V. Transition from stationary to traveling localized patterns in a two-dimensional reaction-diffusion system. Phys. Rev. E 1995, 52, 4465–4473. [Google Scholar] [CrossRef]
- Zemskov, E.P.; Loskutov, A.Y. Traveling waves in a piecewise-linear reaction-diffusion model of excitable medium. Biophysics 2009, 54, 631–636. [Google Scholar] [CrossRef]
- Theodorakis, S. Piecewise linear emulator of the non linear Schroedinger equation and the resulting analytic solutions for Bose-Einstein condensates. Phys. Rev. E 2003, 67, 066701. [Google Scholar] [CrossRef]
- Theodorakis, S. Piecewise analytic approximation for Bose-Einstein condensates in isotropic harmonic traps. Phys. Rev. A 2004, 70, 1–5. [Google Scholar] [CrossRef]
- Zemskov, E.P.; Zykov, S.V.; Kassner, K.; Müller, S.C. Stability of travelling fronts in a piecewise-linear reaction-diffusion system. Nonlinearity 2000, 13, 2063–2076. [Google Scholar] [CrossRef]
- Tsatsarelis, C. Amortissement interne-externe des rameaux des arbres. Riv. Ing. Agric. 1979, 2, 17–25. (In French) [Google Scholar]
| n° Linear Piecewise n | Δvx (m/s) | a | b (m/s) | vxin (m/s) | xin (m) | vxen (m/s) |
|---|---|---|---|---|---|---|
| 1 | ≥3 | 0 | 1.10 | 7.70 | 0.71 | 3.00 |
| 2 | 3 ÷ 2 | 0.18 | 0.56 | 3.00 | 1.35 | 2.00 |
| 3 | 2 ÷ 1 | 0.33 | 0.26 | 2.00 | 1.63 | 1.00 |
| 4 | 1 ÷ 0 | 0.59 | 0 | 1.00 | 1.95 | 0 |
| Geometrical Data | Value |
|---|---|
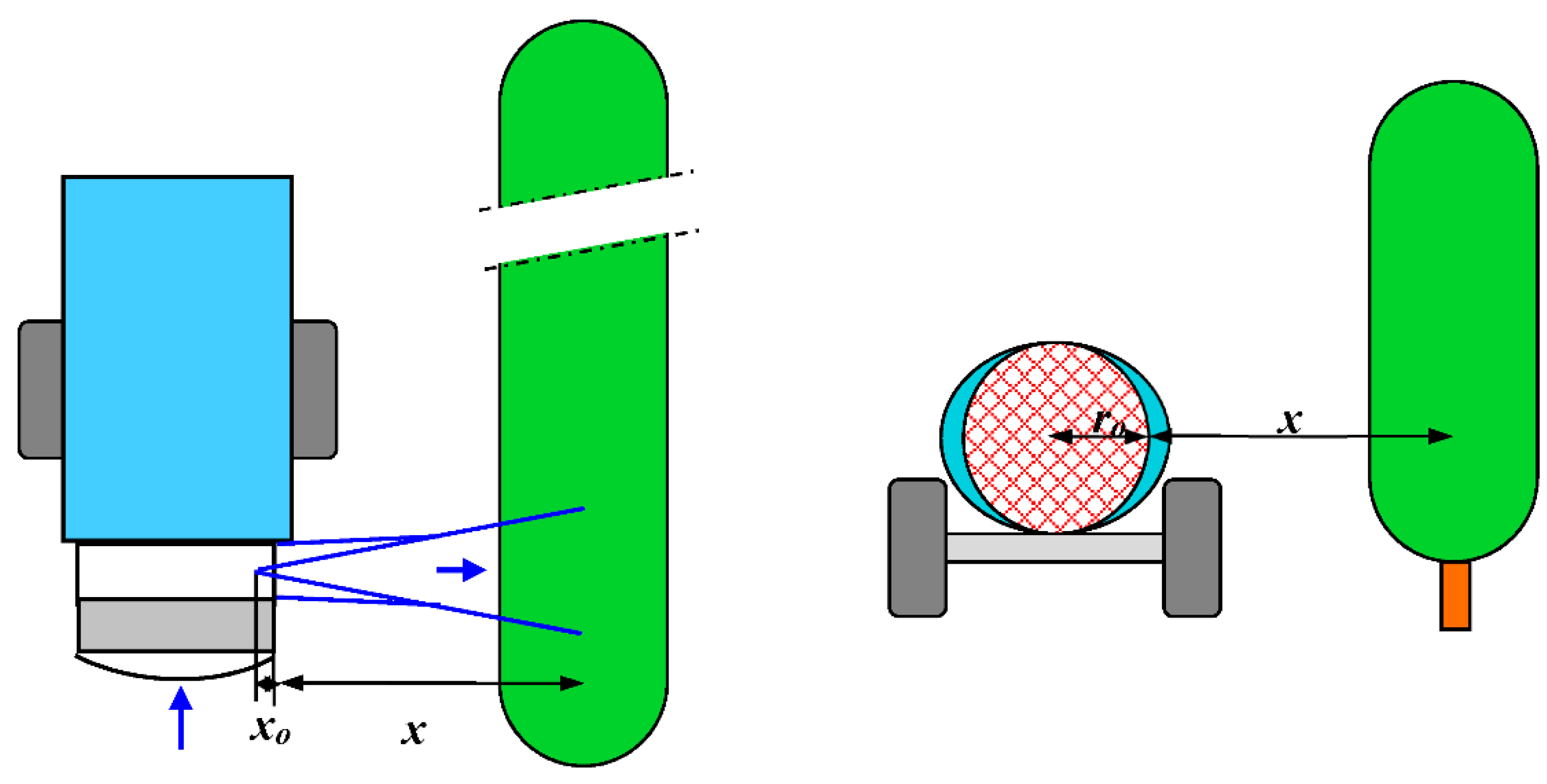
| ro (m) | 0.47 |
| bo(m) | 0.075 |
| xo (m) | –0.085 |
| xt (m) | 0.373 |
| xi (m) | 0.71 |
| xe (m) | 1.94 |
| vxi (m/s) | 7.70 |
| vxe (m/s) experim. | 0.98 |
| S.D. of vxe | 0.25 |
| ρl (s−1) | 11.1 |
| vxe (m/s) Equation (10) | 1.05 |
| vxe (m/s) Equation (11) | 0.96 |
| n° Linear Piecewise n | Δvx (m/s) | a | b (m/s) | vxin (m/s) | xin (m) | vxen (m/s) |
|---|---|---|---|---|---|---|
| 1 | ≥2 | 0 | 1.10 | 7.70 | 0.71 | 2.00 |
| 2 | 2 ÷ 0 | 0.55 | 0 | 2.00 | 1.59 | 0 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Friso, D. An Approximate Analytic Solution to a Non-Linear ODE for Air Jet Velocity Decay through Tree Crops Using Piecewise Linear Emulations and Rectangle Functions. Appl. Sci. 2019, 9, 5440. https://doi.org/10.3390/app9245440
Friso D. An Approximate Analytic Solution to a Non-Linear ODE for Air Jet Velocity Decay through Tree Crops Using Piecewise Linear Emulations and Rectangle Functions. Applied Sciences. 2019; 9(24):5440. https://doi.org/10.3390/app9245440
Chicago/Turabian StyleFriso, Dario. 2019. "An Approximate Analytic Solution to a Non-Linear ODE for Air Jet Velocity Decay through Tree Crops Using Piecewise Linear Emulations and Rectangle Functions" Applied Sciences 9, no. 24: 5440. https://doi.org/10.3390/app9245440
APA StyleFriso, D. (2019). An Approximate Analytic Solution to a Non-Linear ODE for Air Jet Velocity Decay through Tree Crops Using Piecewise Linear Emulations and Rectangle Functions. Applied Sciences, 9(24), 5440. https://doi.org/10.3390/app9245440




