Analysis of Nonlinear Dynamic Characteristics of a Mechanical-Electromagnetic Vibration System with Rubbing
Abstract
1. Introduction
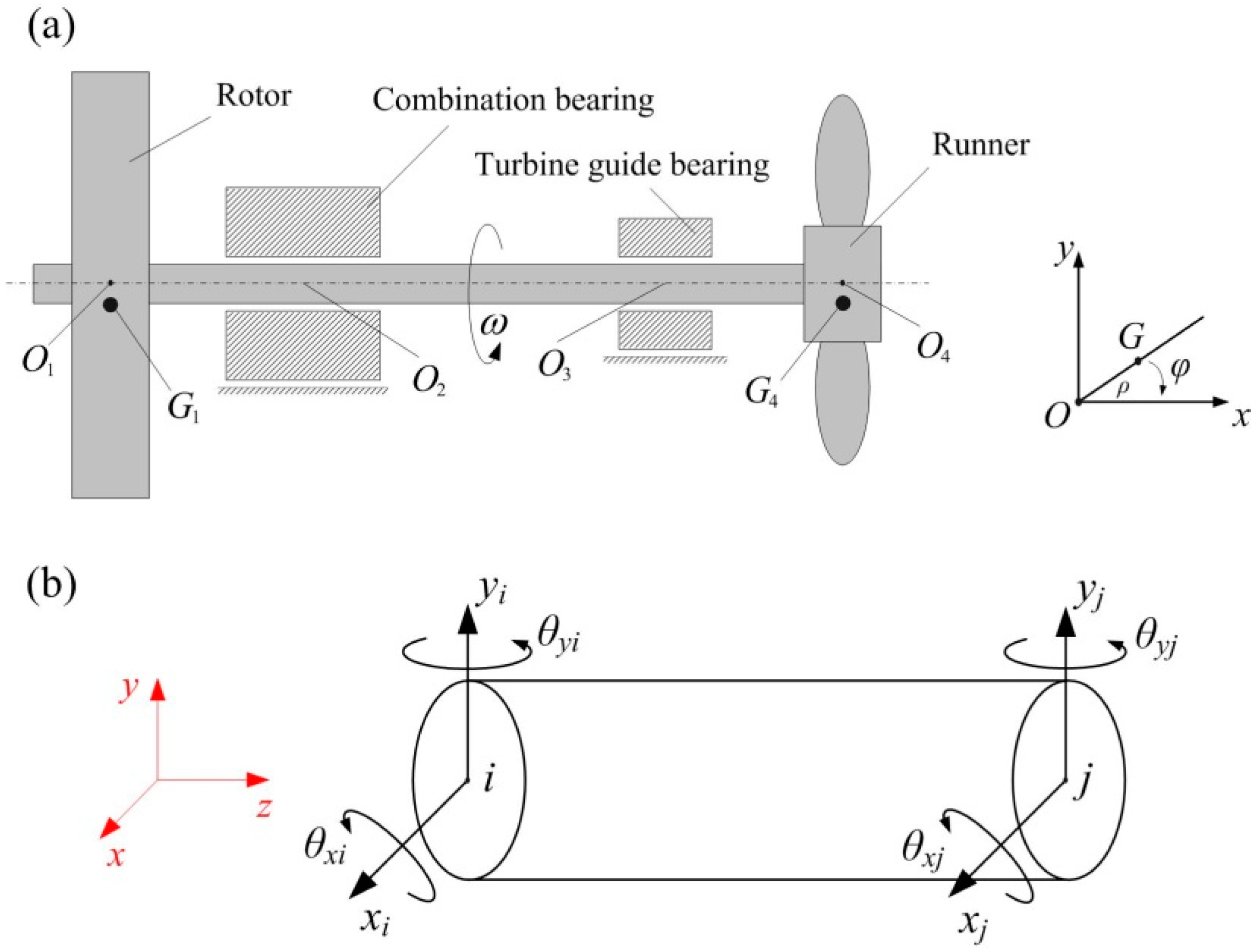
2. The Dynamic Model of the RBRS
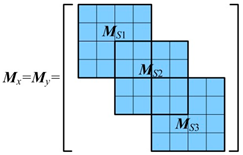
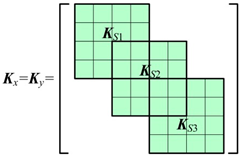
3. Nonlinear Dynamic Analysis of the RBRS
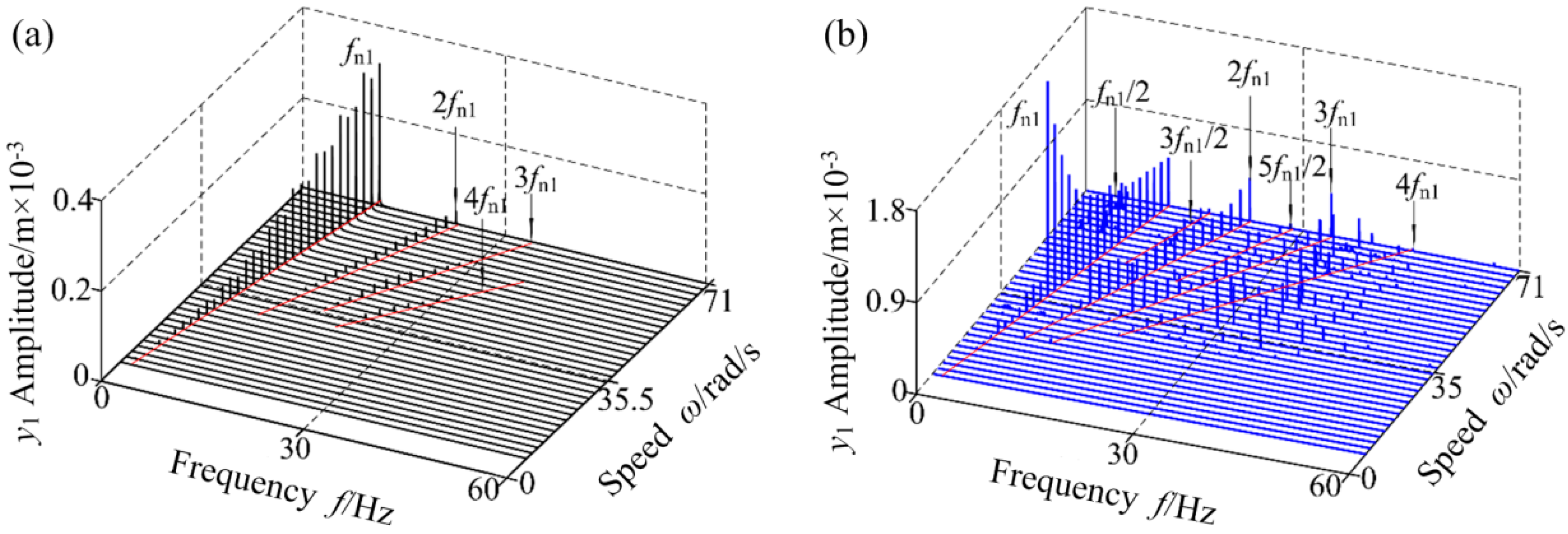
3.1. Model Verification
3.2. Influence of Excitation Current on Dynamic Characteristics of RBRS at Variable Speed
3.3. Effect of Radical Stiffness on Dynamic Characteristics of RBRS at Variable Speed
3.4. Influence of Friction Coefficient on Dynamic Characteristics of RBRS under Variable Speed
4. Conclusions
- (1)
- For the RBRS coupling system, with the change of parameters, there are many kinds of motion states, including periodic-n, quasi-periodic, and chaotic motion. At the same time, the coupling system has intermittent chaos many times, and Hopf, saddle-node, period-doubling, and inverse period-doubling bifurcation appear alternately. The dynamic properties of the coupling system caused by different nonlinear factors are interactional.
- (2)
- When the excitation current of the generator Ij is small, the dynamic behavior of the system is relatively simple. As the rotational speed increases, the system transits from short-term periodic motion, chaotic motion, and quasi-periodic motion to periodic motion, successively undergoing saddle-node bifurcation, period-doubling bifurcation, inverse period-doubling bifurcation, and Hopf bifurcation. The increase of the excitation current Ij can inhibit the response amplitude of the system to some extent, but it cannot inhibit the motion state of the system, which makes the motion state of the system more complex, the chaotic motion wider, and the jump discontinuity enhanced. At higher speeds, the number of high-order bifurcation increases as the excitation current Ij increases.
- (3)
- Due to the influence of the radial stiffness kr and the friction coefficient, the coupled system shows more complex nonlinear behavior. With the increase of radial stiffness kr, the motion complexity of the coupling system increases, the chaotic region increases, the response amplitude increases, and the vibration intensity increases. At the same time, in the high-speed region, the periodic motion region becomes narrow and tends to disappear. With the increase of the friction coefficient, the chaotic region increases first and then decreases, the complex motion and periodic motion alternate frequently, and the response amplitude increases gradually.
Author Contributions
Funding
Conflicts of Interest
References
- Ma, Z.Y.; Dong, Y.X. Dynamics of Water Turbine Generator Set; University of Technology: Dalian, China, 2003. [Google Scholar]
- Wen, B.C. Nonlinear Vibration of Engineering; Science: Beijing, China, 2007. [Google Scholar]
- Chu, F.; Lu, W. Experimental observation of nonlinear vibrations in a rub-impact rotor system. J. Sound Vib. 2005, 283, 621–643. [Google Scholar] [CrossRef]
- Patel, T.H.; Darpe, A.K. Coupled bending-torsional vibration analysis of rotor with rub and crack. J. Sound Vib. 2009, 326, 740–752. [Google Scholar] [CrossRef]
- Cao, J.; Ma, C.; Jiang, Z.; Liu, S. Nonlinear dynamic analysis of fractional order rub-impact rotor system. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1443–1463. [Google Scholar] [CrossRef]
- Huang, Z.; Zhou, J.; Yang, M.; Zhang, Y. Vibration characteristics of a hydraulic generator unit rotor system with parallel misalignment and rub-impact. Arch. Appl. Mech. 2011, 81, 829–838. [Google Scholar] [CrossRef]
- Ma, H.; Shi, C.; Han, Q.K.; Wen, B.C. Fixed-point rubbing fault characteristic analysis of a rotor system based on contact theory. Mech. Syst. Signal Process. 2013, 38, 137–153. [Google Scholar] [CrossRef]
- Khanlo, H.M.; Ghayour, M.; Ziaei-Rad, S. The effects of lateral-torsional coupling on the nonlinear dynamic behavior of a rotating continuous flexible shaft-disk system with rub-impact. Commun. Nonlinear Sci. Numer. Simul. 2013, 18, 1524–1538. [Google Scholar] [CrossRef]
- Jacquet-Richardet, G.; Torkhani, M.; Cartraud, P.; Thouverez, F.; Nouri Baranger, T.; Herran, M.; Gibert, C.; Baguet, S.; Almeida, P.; Peletan, L. Rotor to stator contacts in turbomachines. Review and application. Mech. Syst. Signal Process. 2013, 40, 401–420. [Google Scholar] [CrossRef]
- Lu, W.; Chu, F. Radial and torsional vibration characteristics of a rub rotor. Nonlinear Dyn. 2014, 76, 529–549. [Google Scholar] [CrossRef]
- Ma, H.; Zhao, Q.; Zhao, X.; Han, Q.K.; Wen, B.C. Dynamic characteristics analysis of a rotor-stator system under different rubbing forms. Appl. Math. Modell. 2015, 39, 2392–2408. [Google Scholar] [CrossRef]
- Xiang, L.; Gao, X.; Hu, A. Nonlinear dynamics of an asymmetric rotor-bearing system with coupling faults of crack and rub-impact under oil-film forces. Nonlinear Dyn. 2016, 86, 1057–1067. [Google Scholar] [CrossRef]
- Ma, H.; Lu, Y.; Wu, Z.; Tai, X.; Wen, B.C. Vibration response analysis of a rotational shaft-disk-blade system with blade-tip rubbing. Int. J. Mech. Sci. 2016, 107, 110–125. [Google Scholar] [CrossRef]
- Ma, H.; Yin, F.; Tai, X.; Wang, D.; Wen, B.C. Vibration response analysis caused by rubbing between rotating blade and casing. J. Mech. Sci. Technol. 2016, 30, 1983–1995. [Google Scholar] [CrossRef]
- Mokhtar, M.A.; Kamalakar Darpe, A.; Gupta, K. Investigations on bending-torsional vibrations of rotor during rotor-stator rub using Lagrange multiplier method. J. Sound Vib. 2017, 401, 94–113. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, D.; Wang, D.; Jiang, G. Response analysis of a dual-disc rotor system with multi-unbalances-multi-fixed-point rubbing faults. Nonlinear Dyn. 2017, 87, 109–125. [Google Scholar] [CrossRef]
- Yang, Y.; Cao, D.; Wang, D. Investigation of dynamic characteristics of a rotor system with surface coatings. Mech. Syst. Signal Process. 2017, 84, 469–484. [Google Scholar] [CrossRef]
- Yang, B.S.; Kim, Y.H.; Son, B.G. Instability and Imbalance Response of Large Induction Motor Rotor by Unbalanced Magnetic Pull. J. Vib. Control 2004, 10, 447–460. [Google Scholar] [CrossRef]
- Gustavsson, R.K.; Aidanpää, J.O. The influence of nonlinear magnetic pull on hydropower generator rotors. J. Sound Vib. 2006, 297, 551–562. [Google Scholar] [CrossRef]
- Perers, R.; Lundin, U.; Leijon, M. Saturation Effects on Unbalanced Magnetic Pull in a Hydroelectric Generator with an Eccentric Rotor. IEEE Trans. Magn. 2007, 43, 3884–3890. [Google Scholar] [CrossRef]
- Lundström, N.L.P.; Aidanpää, J.O. Dynamic consequences of electromagnetic pull due to deviations in generator shape. J. Sound Vib. 2007, 301, 207–225. [Google Scholar] [CrossRef]
- Calleecharan, Y.; Aidanpää, J.O. Dynamics of a hydropower generator subjected to unbalanced magnetic pull. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2011, 225, 2076–2088. [Google Scholar] [CrossRef]
- Xu, Y.; Li, Z. Computational Model for Investigating the Influence of Unbalanced Magnetic Pull on the Radial Vibration of Large Hydro-Turbine Generators. J. Vib. Acoust. 2012, 134, 051013. [Google Scholar] [CrossRef]
- Zeng, Y.; Zhang, L.; Guo, Y.; Qian, J.; Zhang, C. The generalized Hamiltonian model for the shafting transient analysis of the hydro turbine generating sets. Nonlinear Dyn. 2014, 76, 1921–1933. [Google Scholar] [CrossRef]
- Xu, B.; Chen, D.; Zhang, H.; Zhou, R. Dynamic analysis and modeling of a novel fractional-order hydro-turbine-generator unit. Nonlinear Dyn. 2015, 81, 1263–1274. [Google Scholar] [CrossRef]
- Xu, X.; Han, Q.; Chu, F. Nonlinear vibration of a generator rotor with unbalanced magnetic pull considering both dynamic and static eccentricities. Arch. Appl. Mech. 2016, 86, 1521–1536. [Google Scholar] [CrossRef]
- Xiang, C.; Liu, F.; Liu, H.; Han, L.; Zhang, X. Nonlinear dynamic behaviors of permanent magnet synchronous motors in electric vehicles caused by unbalanced magnetic pull. J. Sound Vib. 2016, 371, 277–294. [Google Scholar] [CrossRef]
- Li, R.; Li, C.; Peng, X.; Wei, W.; Sciubba, E. Electromagnetic Vibration Simulation of a 250-MW Large Hydropower Generator with Rotor Eccentricity and Rotor Deformation. Energies 2017, 10, 2155. [Google Scholar] [CrossRef]
- Yan, D.; Zhuang, K.; Xu, B.; Chen, D.; Mei, R.; Wu, C.; Wang, X. Excitation Current Analysis of a Hydropower Station Model Considering Complex Water Diversion Pipes. J. Energy Eng. 2017, 143, 04017012. [Google Scholar] [CrossRef]
- Bathe, K.J.; Wilson, E.L. Numericalmethodsin Finite Elementanalysis; Prentice-Hall Inc.: Upper Saddle River, NJ, USA, 1976. [Google Scholar]
- Zhou, J.Z.; Zhang, Y.C.; Li, C.S. Dynamic Problems and Fault Diagnosis Principles Methods of Hydroelectric Generating Set; Huazhong University of Science and Technology: Wuhan, China, 2013. [Google Scholar]



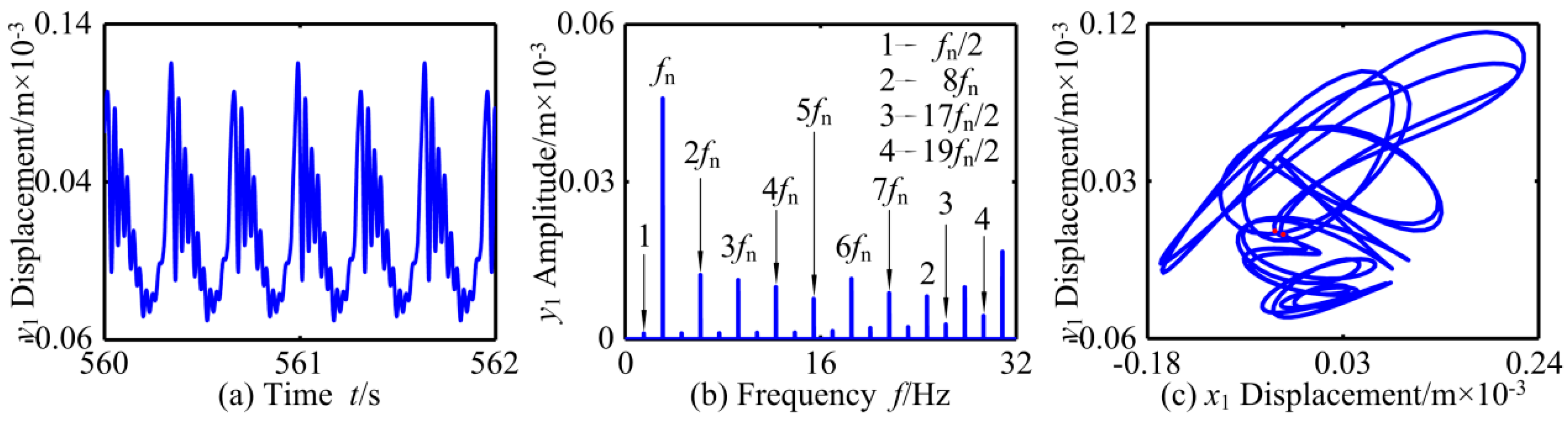
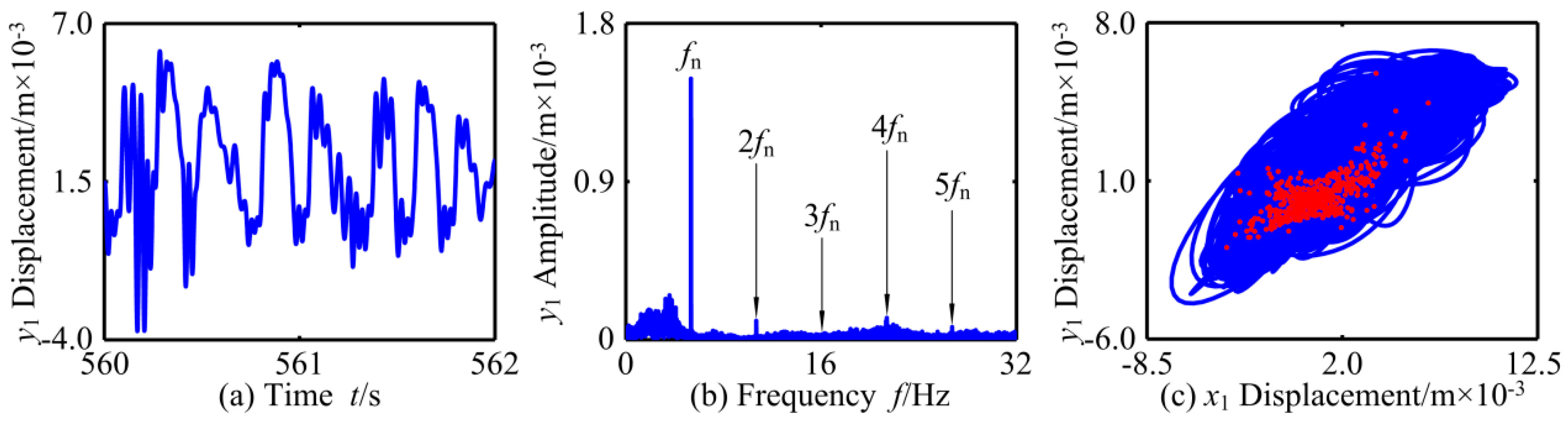
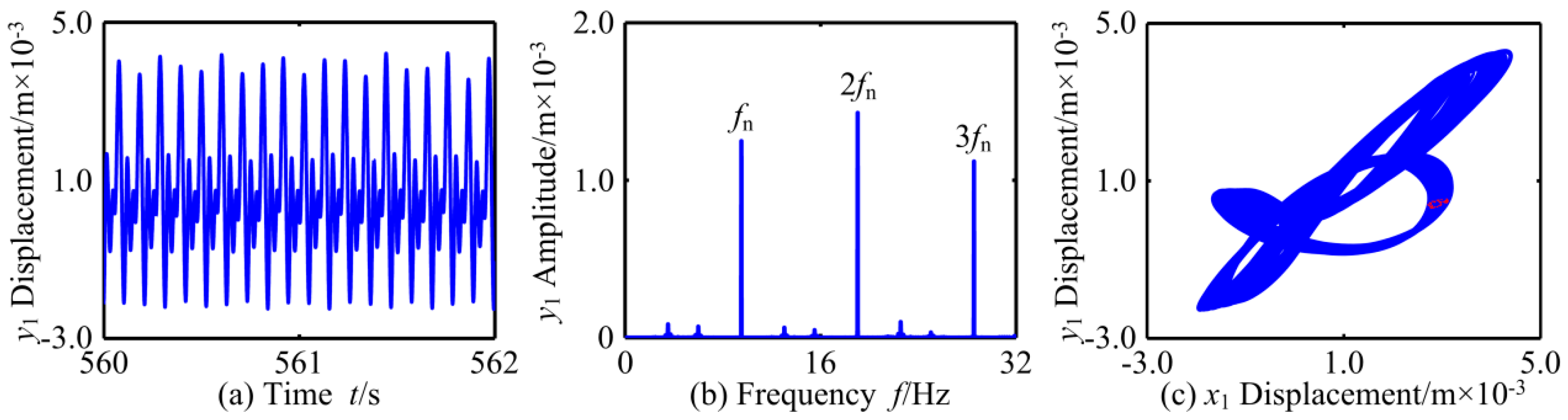

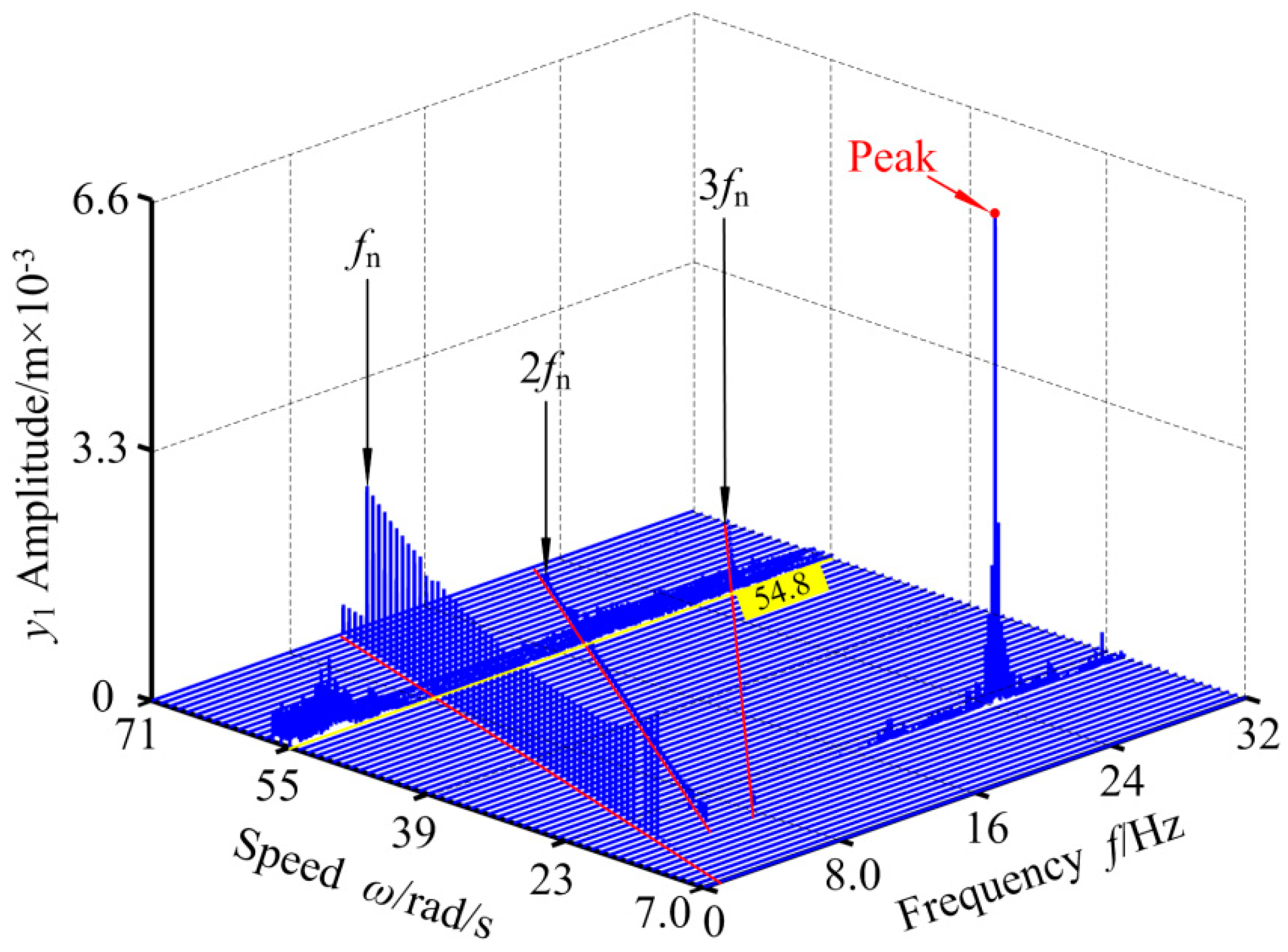
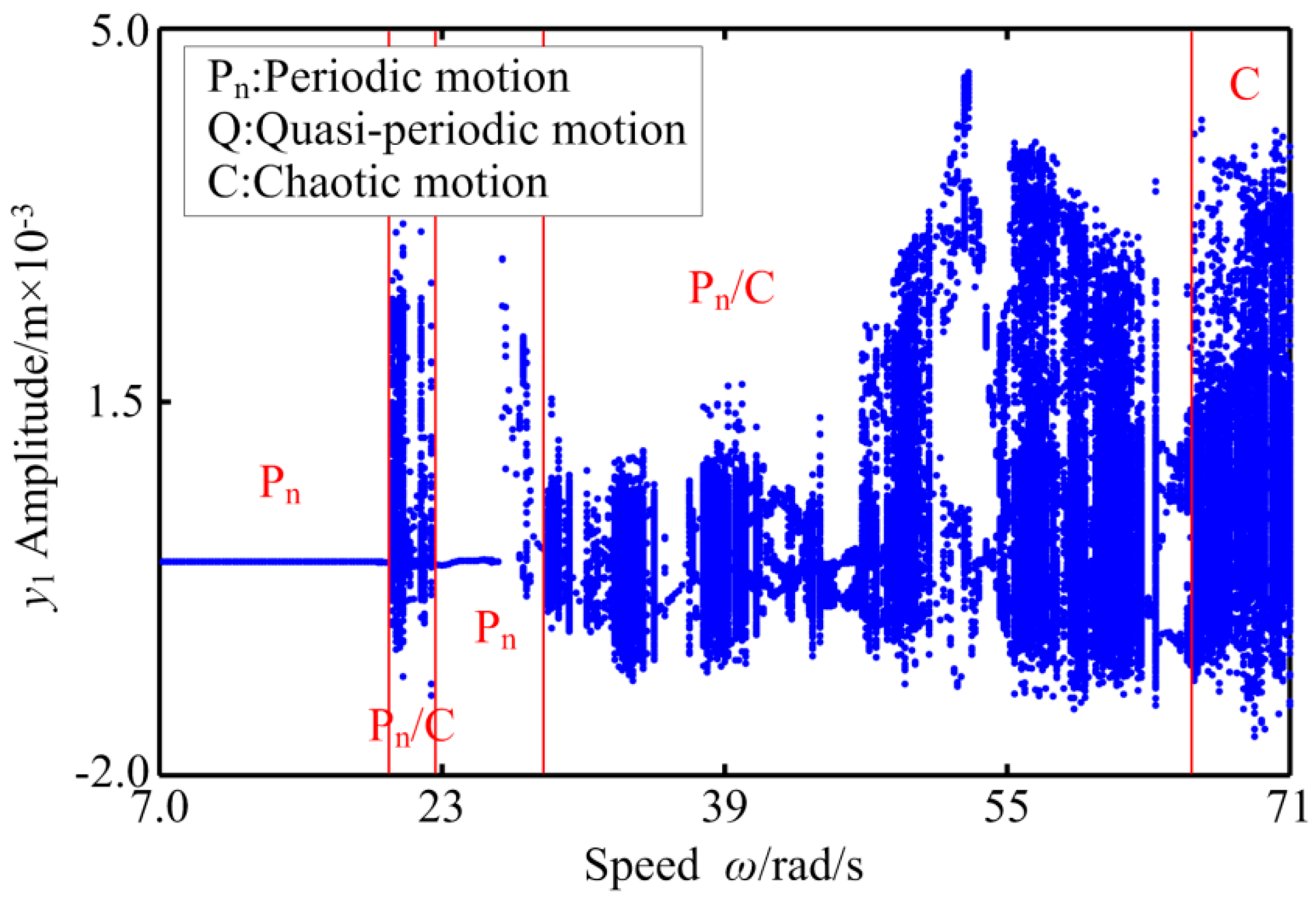

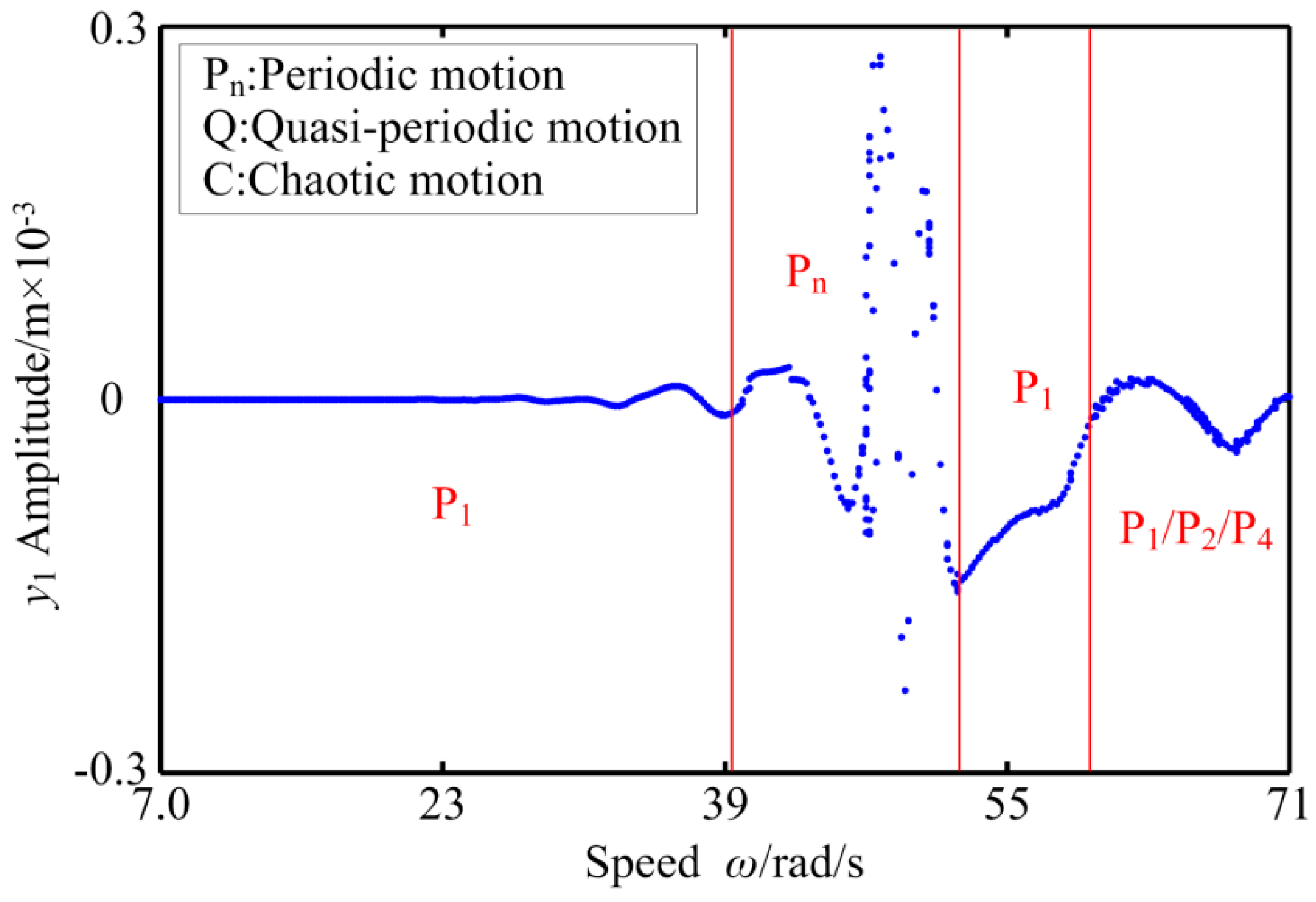
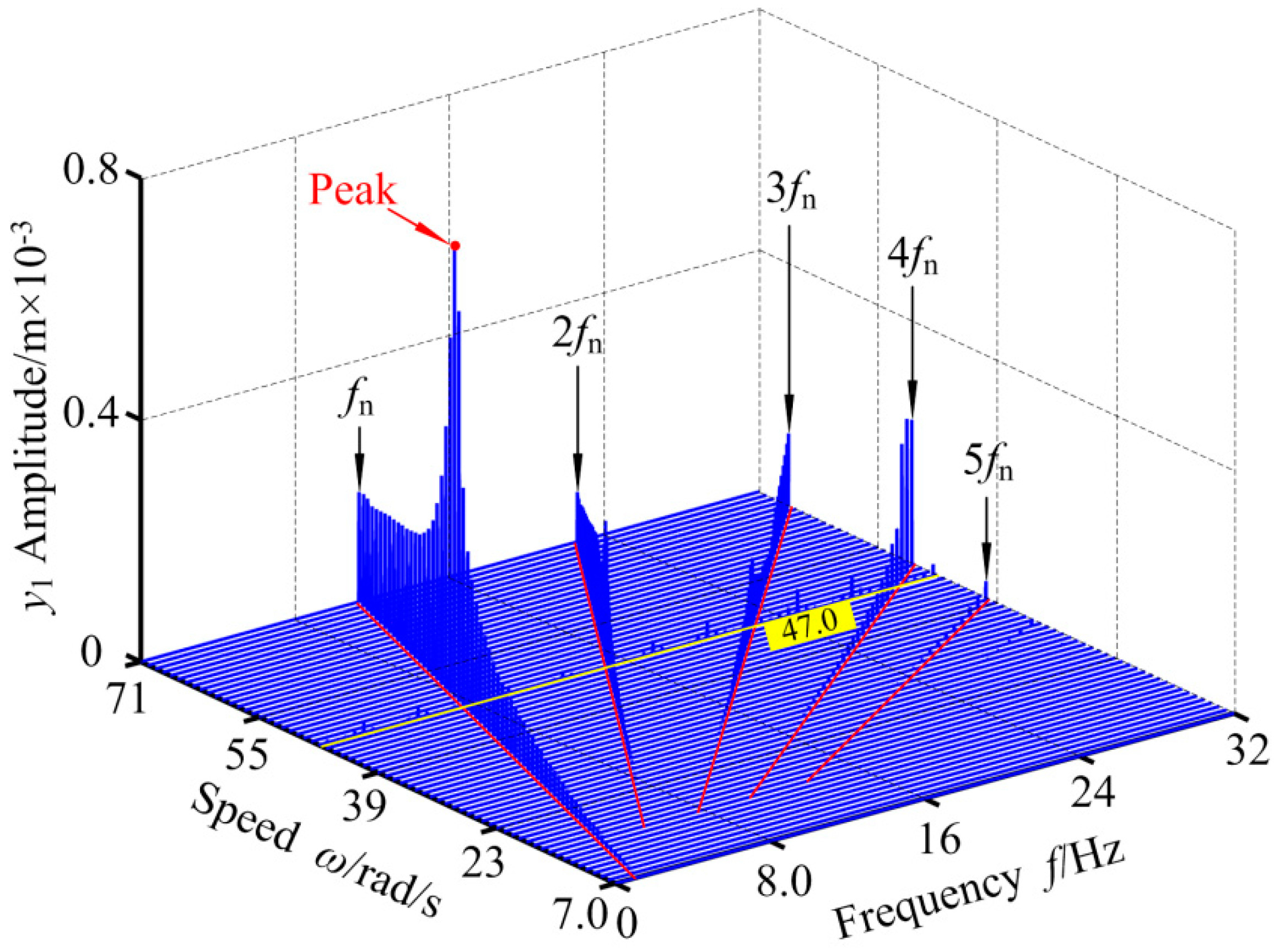


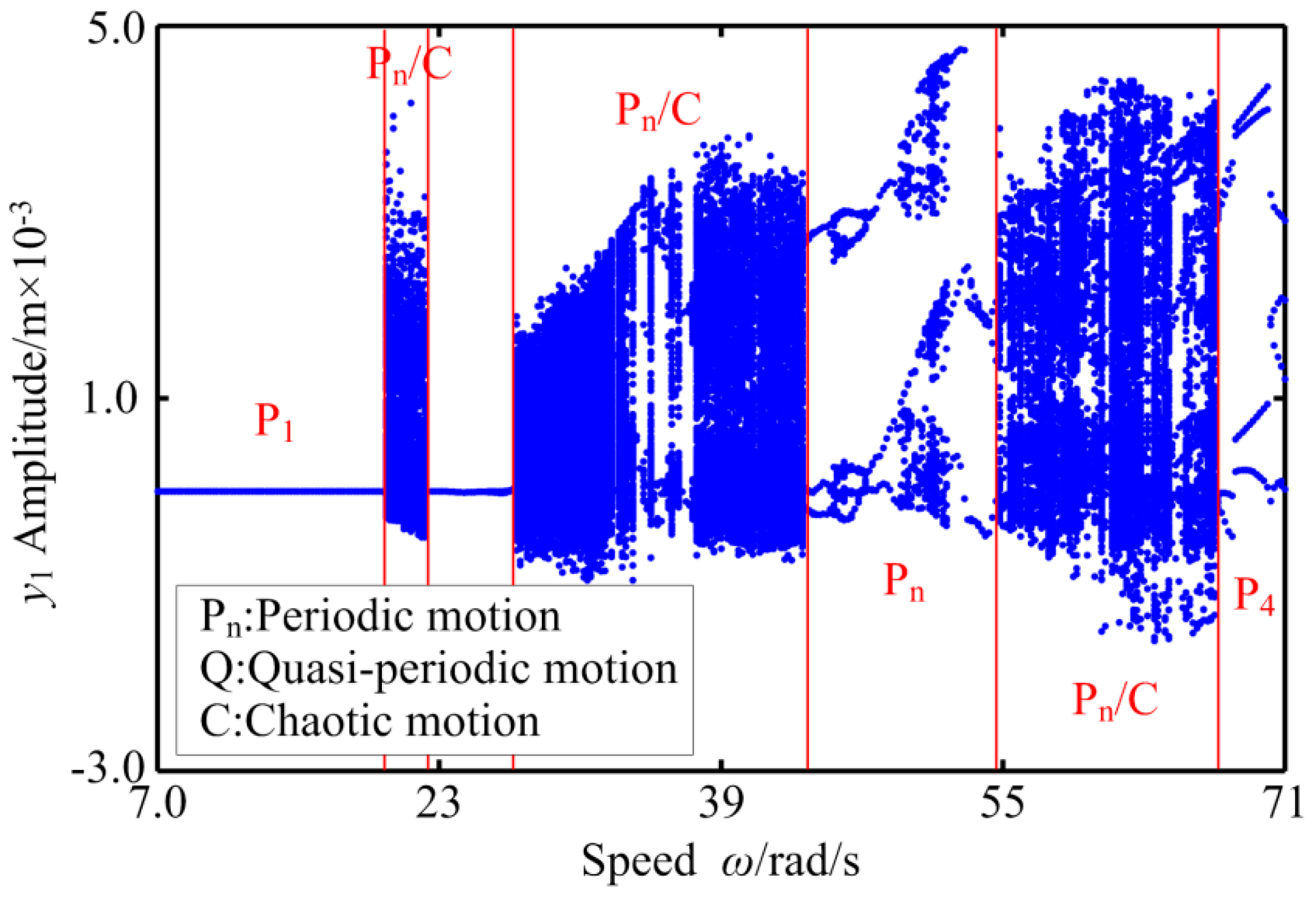
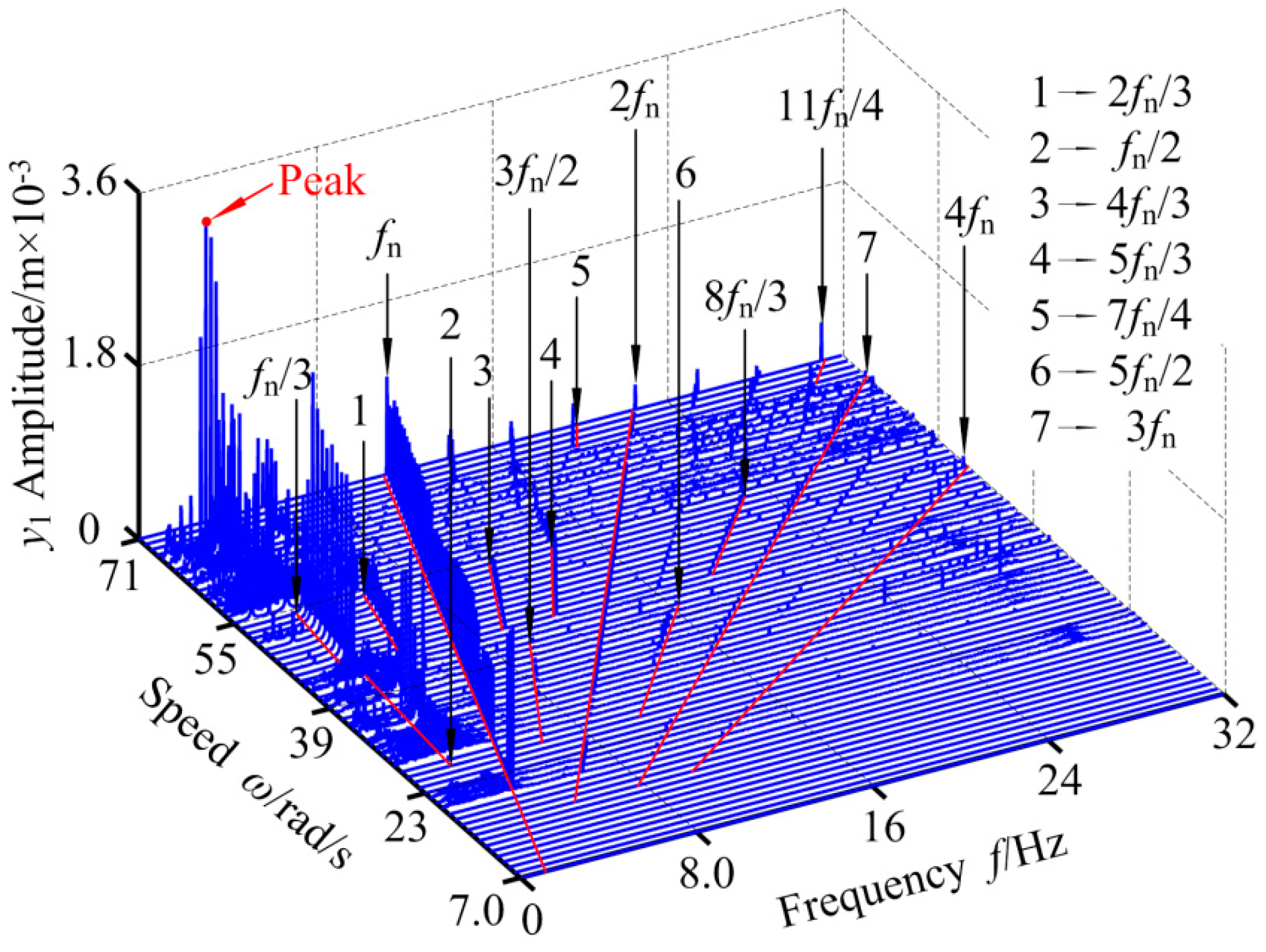
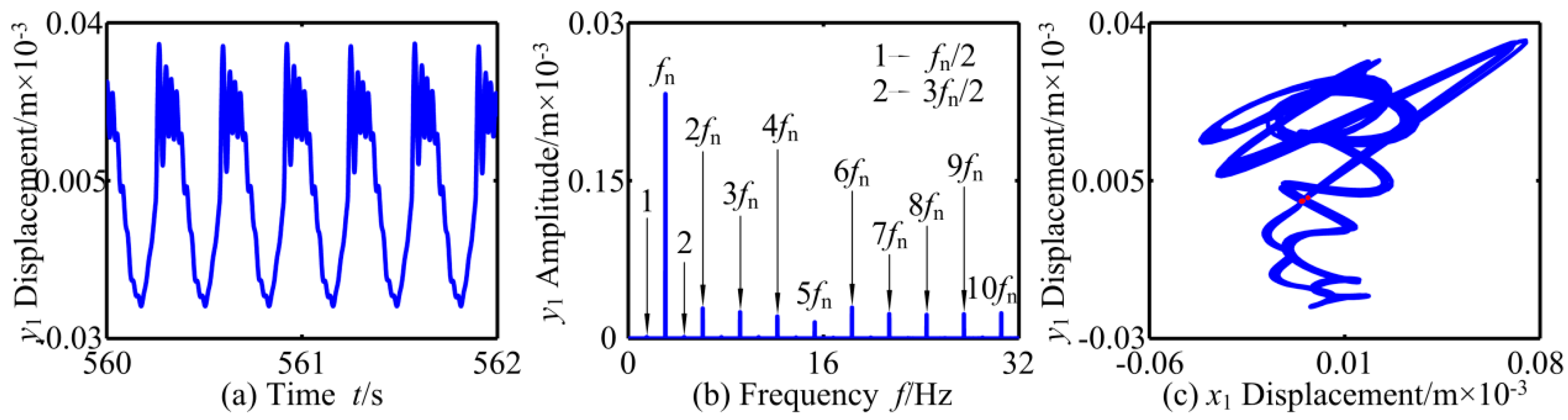

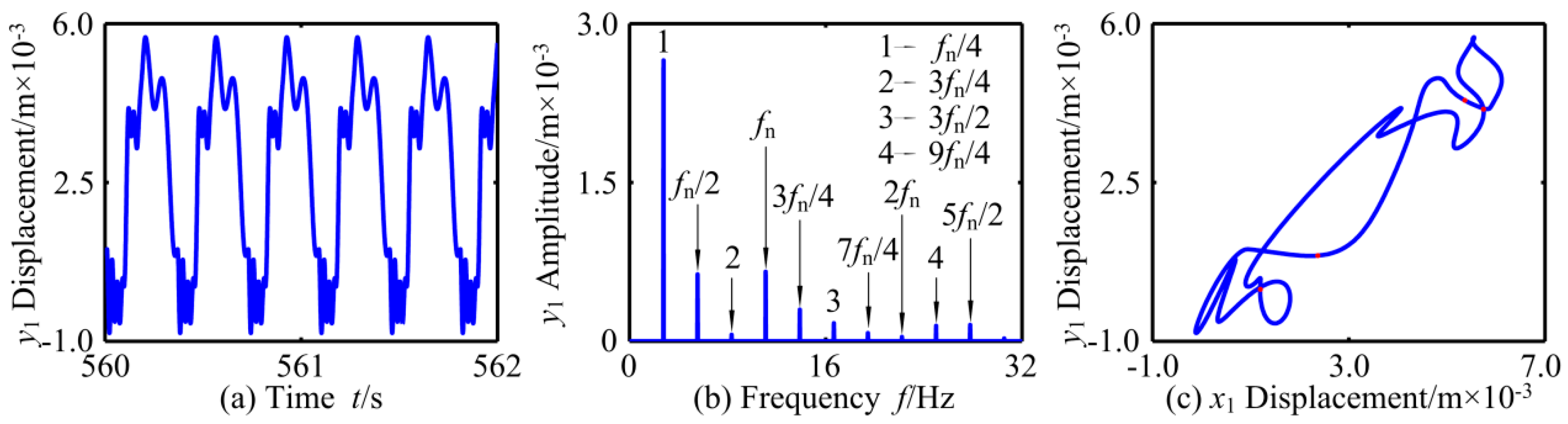
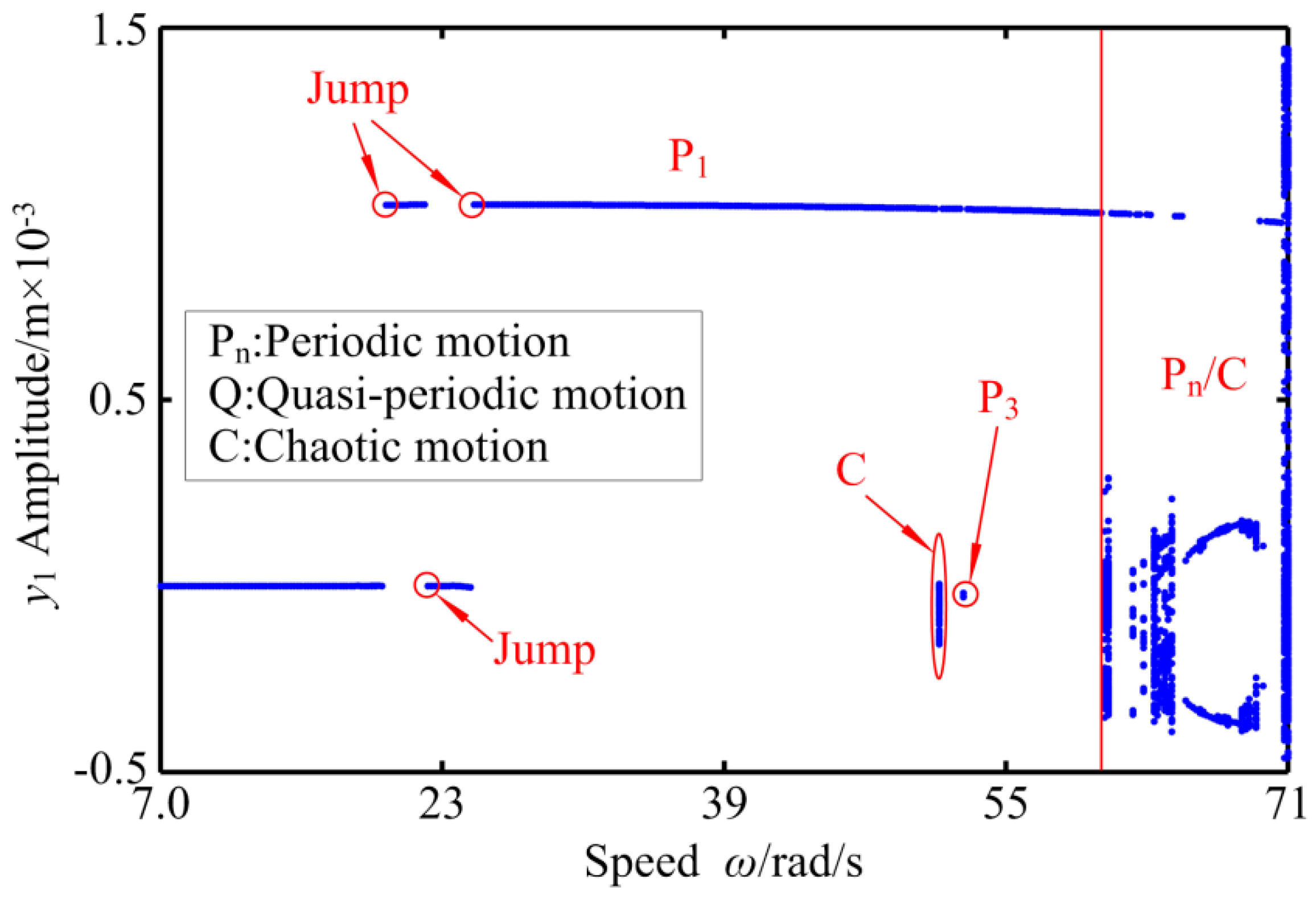
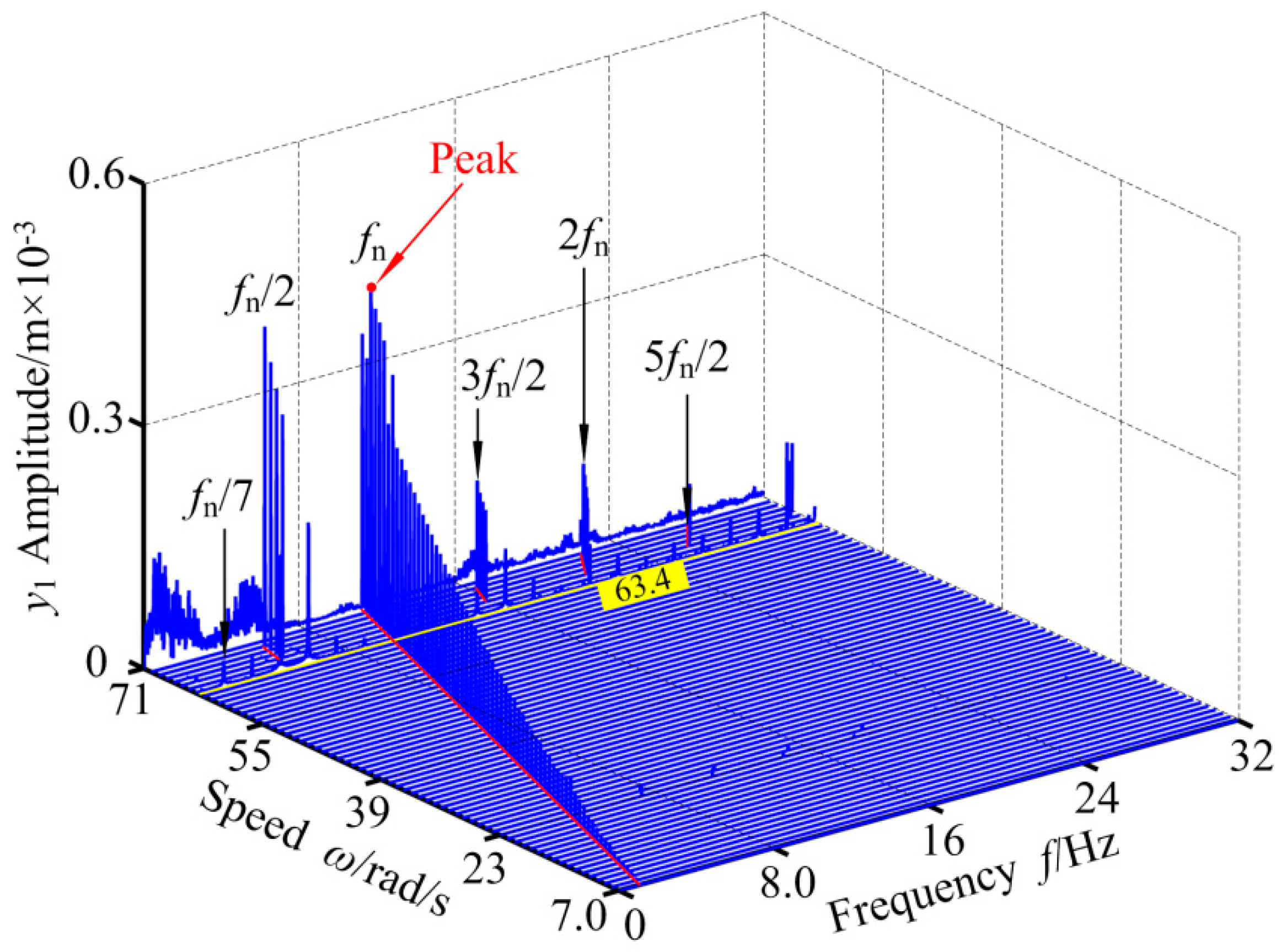
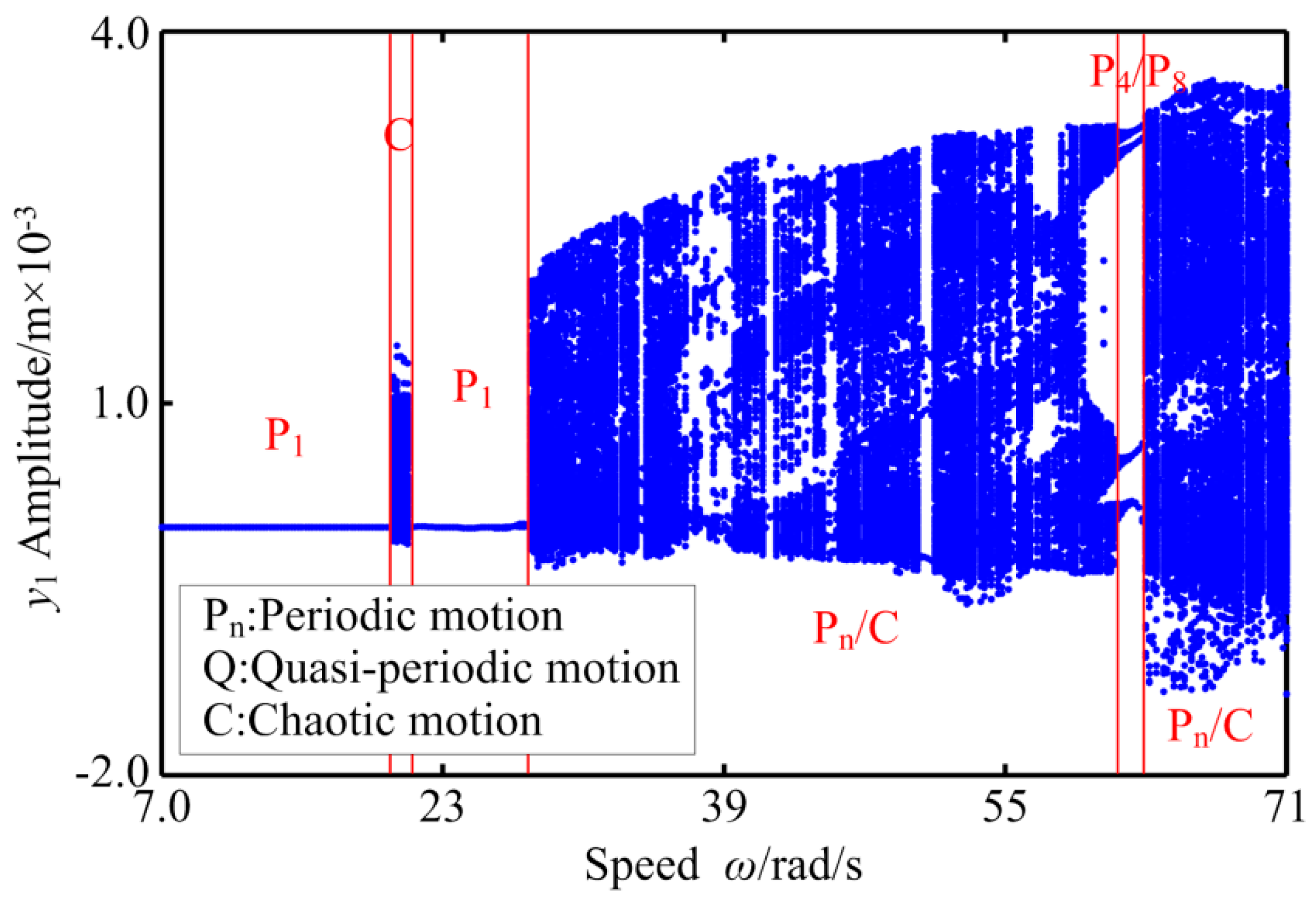
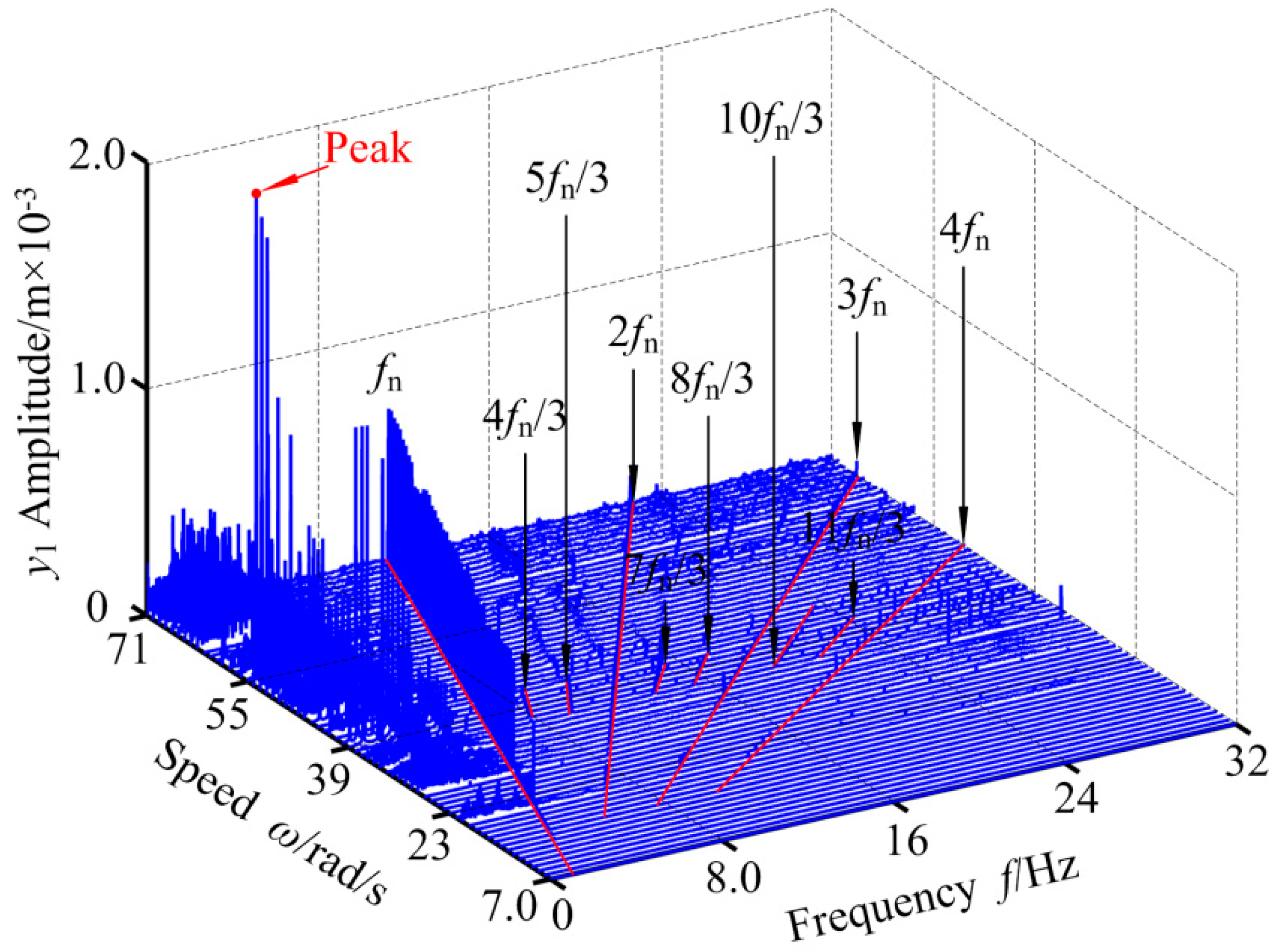

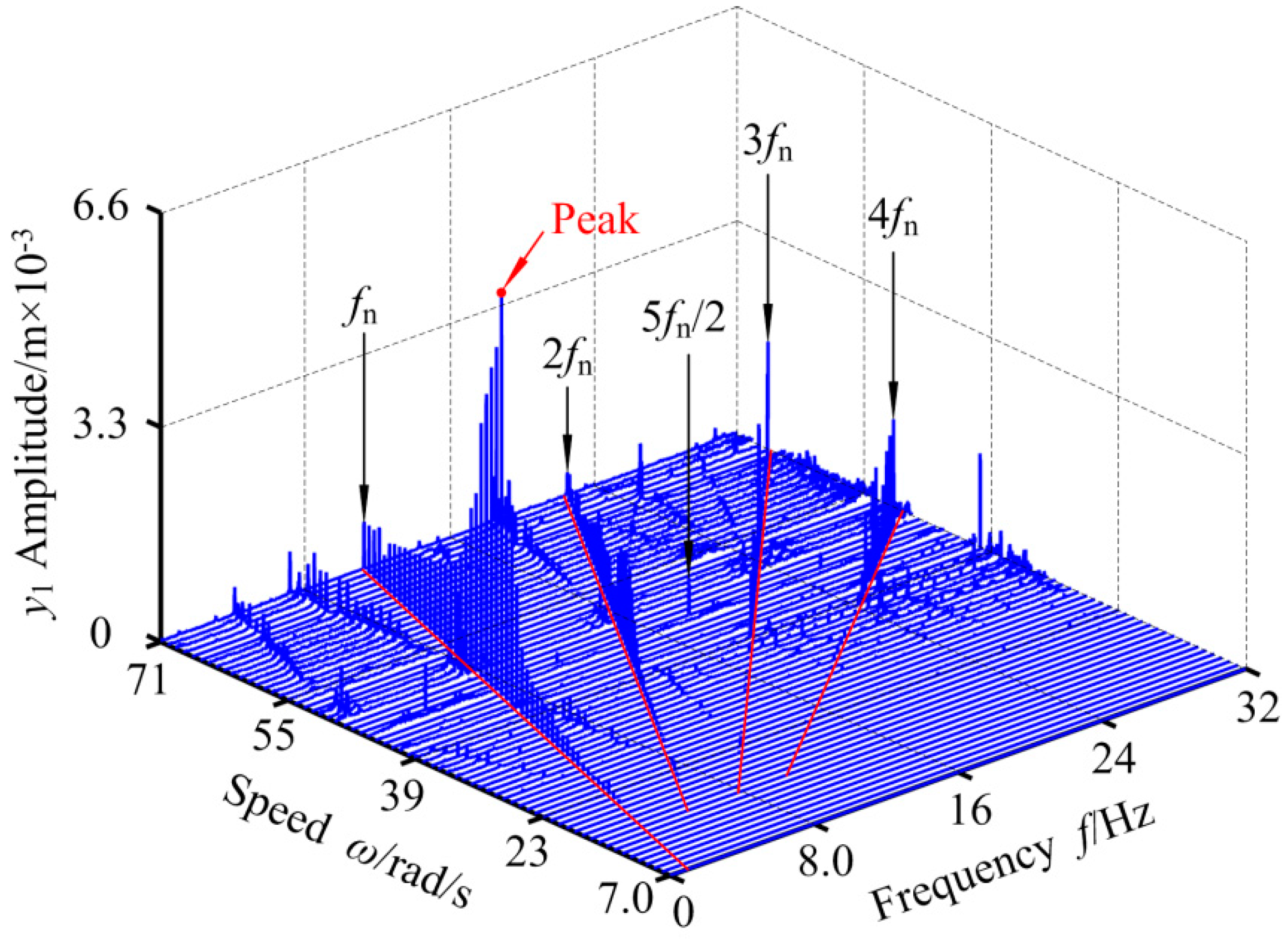

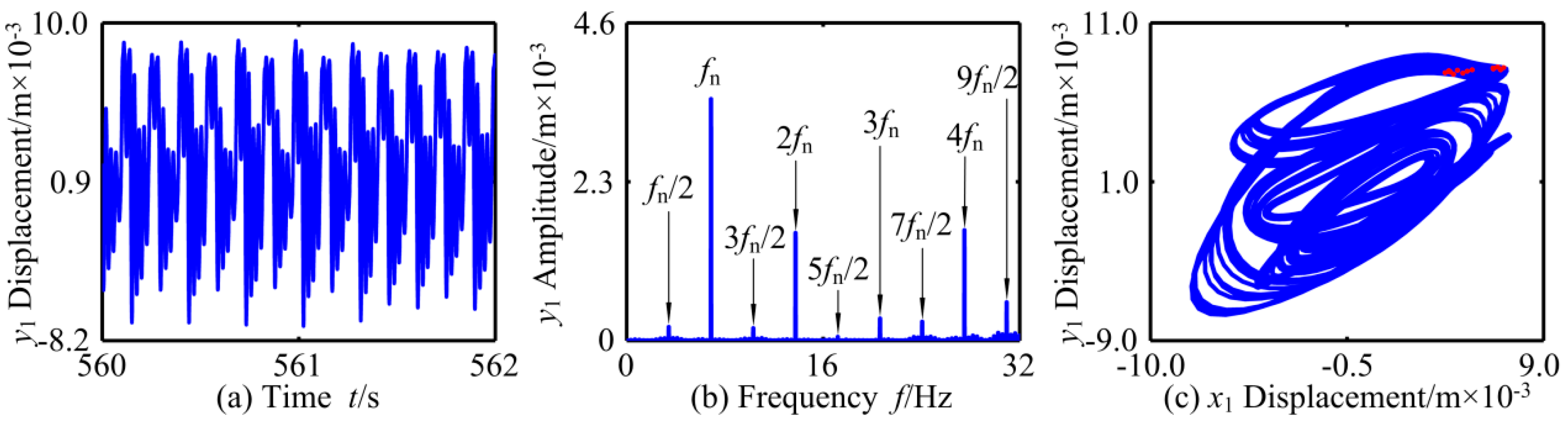
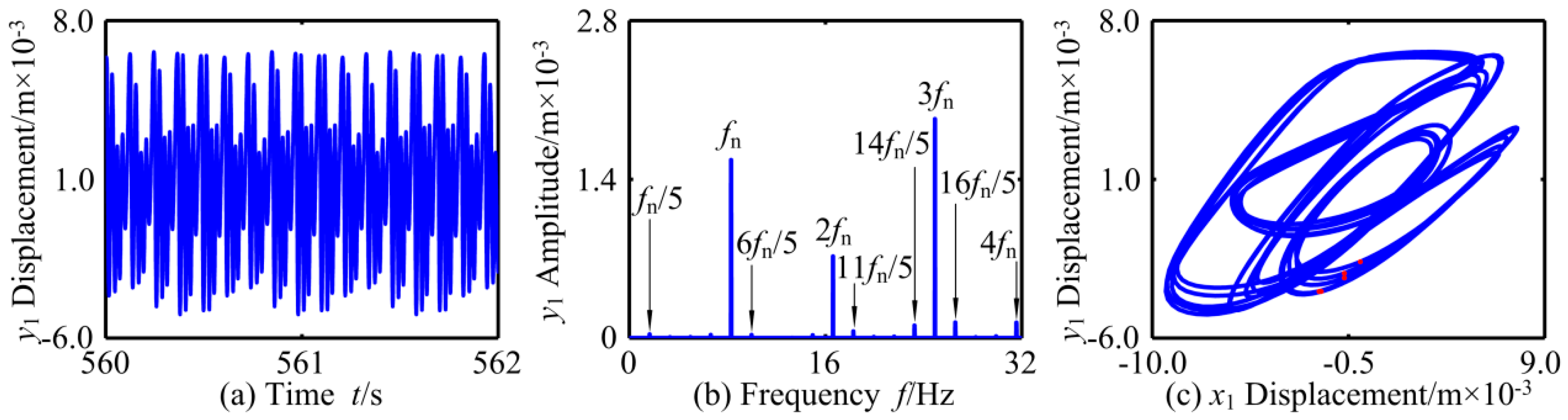
| Parameters | Value |
|---|---|
| m1, m2 (kg) | 5.7 × 104, 5.2225 × 104 |
| kz2x, kz3x (N/m) | 1.44 × 108, 0.34 × 108 |
| kz2y, kz3y (N/m) | 3.71 × 108, 1.92 × 108 |
| cz2x, cz3x (N·s/m) | 6.5 × 103, 3 × 103 |
| cz2y, cz3y (N·s/m) | 6.5 × 103, 3 × 103 |
| ξ1, ξ2 | 0.03, 0.03 |
| lμ0 | 4π × 10−7 |
| α | 1.3 |
| Kj | 5.1 |
| Kw (N/cm) | 1.63 × 104 |
| Rotating Speed (rad/s) | Reference [31] | This Paper | ||
|---|---|---|---|---|
| Main Frequency Feature | Rubbing | Main Frequency Feature | Rubbing | |
| 7 ≤ ω < 23 | fn1 | 0 | fn1 | 0 |
| 23 ≤ ω < 59 | fn1, 2fn1, 3fn1, 4fn1, 5fn1, unobvious frequency demultiplication components | 1 | fn1, 2fn1, 3fn1, 4fn1, 5fn1, fn1/2, 3fn1/2, 5fn1/2, 7fn1/2, continuous frequency components | 1 |
| 59 ≤ ω ≤ 71 | fn1, 2fn1 | 0 | fn1, 2fn1, 3fn1, 4fn1, 5fn1, fn1/2, 3fn1/2, 5fn1/2, 7fn1/2, fn1/4, 3fn14, 5fn1/4, 7fn1/4, continuous frequency components | 1 |
| Excitation Current Ij (A) | Rotating Speed ω (rad/s) | Main Frequency Feature (m: Positive Integer) |
|---|---|---|
| Ij = 700 | 7.0 ≤ ω ≤ 15.6 | fn |
| 15.6 < ω ≤ 20 | fn, 2fn, 3fn, 4fn,…… | |
| 20 < ω ≤ 21.2 | fn, 2fn, 3fn, 4fn,…, continuous frequency components | |
| 21.2 < ω ≤ 54.8 | fn, 2fn, 3fn, 4fn,…… | |
| 54.8 < ω ≤ 57.6 | fn/4, fn/3, fn, 2fn, 3fn, continuous frequency components | |
| 57.6 < ω ≤ 71 | fn, 2fn, 3fn | |
| Ij = 1000 | 7.0 ≤ ω ≤ 16.2 | fn |
| 16.2 < ω ≤ 18.6 | fn, 2fn, 3fn, 4fn,…… | |
| 18.6 < ω ≤ 19.8 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 19.8 < ω ≤ 23.6 | fn, 2fn, 3fn, 4fn,…, continuous frequency components | |
| 23.6 < ω ≤ 24.6 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 24.6 < ω ≤ 42.4 | fn, 2fn, 3fn, 4fn,…, continuous frequency components | |
| 42.4 < ω ≤ 42.8 | fn, 2fn, 3fn, 4fn,…… | |
| 42.8 < ω ≤ 54.6 | fn/2, fn, 3fn/2, 2fn, 5fn/2, 3fn, 7fn/2, 4fn, continuous frequency components | |
| 54.6 < ω ≤ 59.6 | fn, 2fn, 3fn, continuous frequency components | |
| 59.6 < ω ≤ 71 | fn, 2fn, 3fn, mfn/3 | |
| Ij = 1200 | 7.0 ≤ ω ≤ 15.4 | fn |
| 15.4 < ω ≤ 18.8 | fn, 2fn, 3fn, 4fn,…… | |
| 18.8 < ω ≤ 20 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 20 < ω ≤ 22.4 | fn, 2fn, 3fn, 4fn,…, continuous frequency components | |
| 22.4 < ω ≤ 27.2 | fn, 2fn, 3fn, 4fn,…… | |
| 27.2 < ω ≤ 28.8 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 28.8 < ω ≤ 65.2 | fn, 2fn, 3fn, 4fn,…, mfn/4, continuous frequency components | |
| 65.2 < ω ≤ 71 | fn, 2fn, 3fn, continuous frequency components |
| Excitation Current kr (N/m) | Rotating Speed ω (rad/s) | Main Frequency Feature (m: Positive Integer) |
|---|---|---|
| kr = 1 × 108 | 7.0 ≤ ω ≤ 15.4 | fn |
| 15.4 < ω ≤ 39.6 | fn, 2fn, 3fn, 4fn, 5fn,…… | |
| 39.6 < ω ≤ 52.2 | fn, 2fn, 3fn; mfn/2, mfn/3, and mfn/4 alternate | |
| 52.2 < ω ≤ 59.8 | fn, 2fn, 3fn, 4fn,…… | |
| 59.8 < ω ≤ 71 | fn, 2fn, 3fn; mfn/2 and mfn/4 alternate | |
| kr = 2.6 × 108 | 7.0 < ω ≤ 15.8 | fn |
| 15.8 < ω ≤ 29.6 | fn, 2fn, 3fn, 4fn,…… | |
| 29.6 < ω ≤ 32.6 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 32.6 < ω ≤ 35.8 | fn, 2fn, 3fn, 4fn,…… | |
| 35.8 < ω ≤ 40.2 | fn, 2fn, 3fn, 4fn,…; mfn/18, mfn/23, and mfn/27 alternate | |
| 40.2 < ω ≤ 47.8 | fn, 2fn, 3fn, 4fn,…, mfn/7 | |
| 47.8 < ω ≤ 51.6 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 51.6 < ω ≤ 60.4 | fn, 2fn, 3fn, 4fn,…… | |
| 60.4 < ω ≤ 66.8 | fn, 2fn, 3fn, 4fn,…, first mfn/2, then mfn/3 | |
| 66.8 < ω ≤ 71 | fn, 2fn, 3fn, mfn/7 | |
| kr = 4 × 108 | 7.0 < ω ≤ 15.4 | fn |
| 15.4 < ω ≤ 18.8 | fn, 2fn, 3fn, 4fn,…… | |
| 18.8 < ω ≤ 22.2 | fn, 2fn, 3fn, 4fn,…, mfn/2, continuous frequency components | |
| 22.2 < ω ≤ 25.6 | fn, 2fn, 3fn, 4fn,…… | |
| 25.6 < ω ≤ 43.6 | fn, 2fn, 3fn, 4fn,…; mfn/2, mfn/4, and mfn/6 alternate; continuous frequency components | |
| 43.6 < ω ≤ 54.6 | fn, 2fn, 3fn, 4fn,…; mfn/3, mfn/6, and mfn/12 alternate | |
| 54.6 < ω ≤ 67.4 | fn, 2fn, 3fn, 4fn,…; mfn/4, mfn/6, and mfn/14 alternate; continuous frequency components | |
| 67.4 < ω ≤ 71 | fn, 2fn, 3fn,…, mfn/4 |
| Excitation Current μ | Rotating Speed ω (rad/s) | Main Frequency Feature (m: Positive Integer) |
|---|---|---|
| μ = 0.05 | 7.0 < ω ≤ 15.4 | fn |
| 15.4 < ω ≤ 60.4 | fn, 2fn, 3fn, 4fn, 5fn,……(where: ω = 51.2 (fn, 2fn, 3fn, 4fn,…, continuous frequency components); ω = 52.6 (fn, 2fn, 3fn, 4fn,…, mfn/3)) | |
| 60.4 < ω ≤ 70.6 | fn, 2fn, 3fn, 4fn,…; mfn/2, mfn/5 and mfn/7 alternate; continuous frequency components | |
| 70.6 < ω ≤ 71.0 | fn, 2fn, 3fn,…, continuous frequency components | |
| μ = 0.3 | 7.0 < ω ≤ 15.6 | fn |
| 15.6 < ω ≤ 18.2 | fn, 2fn, 3fn, 4fn,…… | |
| 18.2 < ω ≤ 20.0 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 20.0 < ω ≤ 21.0 | fn, 2fn, 3fn,…, continuous frequency components | |
| 21.0 < ω ≤ 25.8 | fn, 2fn, 3fn, 4fn,…… | |
| 25.8 < ω ≤27.8 | fn, 2fn, 3fn, 4fn,…; mfn/2 and mfn/4 alternate | |
| 27.8 < ω ≤ 61.4 | fn, 2fn, 3fn, 4fn,…; mfn/3, mfn/4 and mfn/6 alternate; continuous frequency components | |
| 61.4 < ω ≤ 63.0 | fn, 2fn, 3fn, 4fn,…,first mfn/8,then mfn/4 | |
| 63.0 < ω ≤ 71 | fn, 2fn, 3fn,…, mfn/3, continuous frequency components | |
| μ = 0.78 | 7.0 < ω ≤ 15.4 | fn |
| 15.4 < ω ≤ 21.8 | fn, 2fn, 3fn, 4fn,…, mfn/2 | |
| 21.8 < ω ≤ 24.8 | fn, 2fn, 3fn, 4fn,…, mfn/3 and mfn/4, continuous frequency components | |
| 24.8 < ω ≤ 48.2 | fn, 2fn, 3fn, 4fn,…; mfn/2, mfn/3 and mfn/5 alternate; continuous frequency components | |
| 48.2 < ω ≤ 51.6 | fn, 2fn, 3fn,…, continuous frequency components | |
| 51.6 < ω ≤ 71 | fn, 2fn, 3fn, 4fn,…; mfn/3, mfn/7 and mfn/12 alternate; continuous frequency components |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Huang, Z.; Li, H.; Song, G. Analysis of Nonlinear Dynamic Characteristics of a Mechanical-Electromagnetic Vibration System with Rubbing. Appl. Sci. 2019, 9, 4612. https://doi.org/10.3390/app9214612
Li Y, Huang Z, Li H, Song G. Analysis of Nonlinear Dynamic Characteristics of a Mechanical-Electromagnetic Vibration System with Rubbing. Applied Sciences. 2019; 9(21):4612. https://doi.org/10.3390/app9214612
Chicago/Turabian StyleLi, Yiming, Zhilong Huang, He Li, and Guiqiu Song. 2019. "Analysis of Nonlinear Dynamic Characteristics of a Mechanical-Electromagnetic Vibration System with Rubbing" Applied Sciences 9, no. 21: 4612. https://doi.org/10.3390/app9214612
APA StyleLi, Y., Huang, Z., Li, H., & Song, G. (2019). Analysis of Nonlinear Dynamic Characteristics of a Mechanical-Electromagnetic Vibration System with Rubbing. Applied Sciences, 9(21), 4612. https://doi.org/10.3390/app9214612




