Simplified Regional Prediction Model of Long-Term Trend for Critical Frequency of Ionospheric F2 Region over East Asia
Abstract
1. Introduction
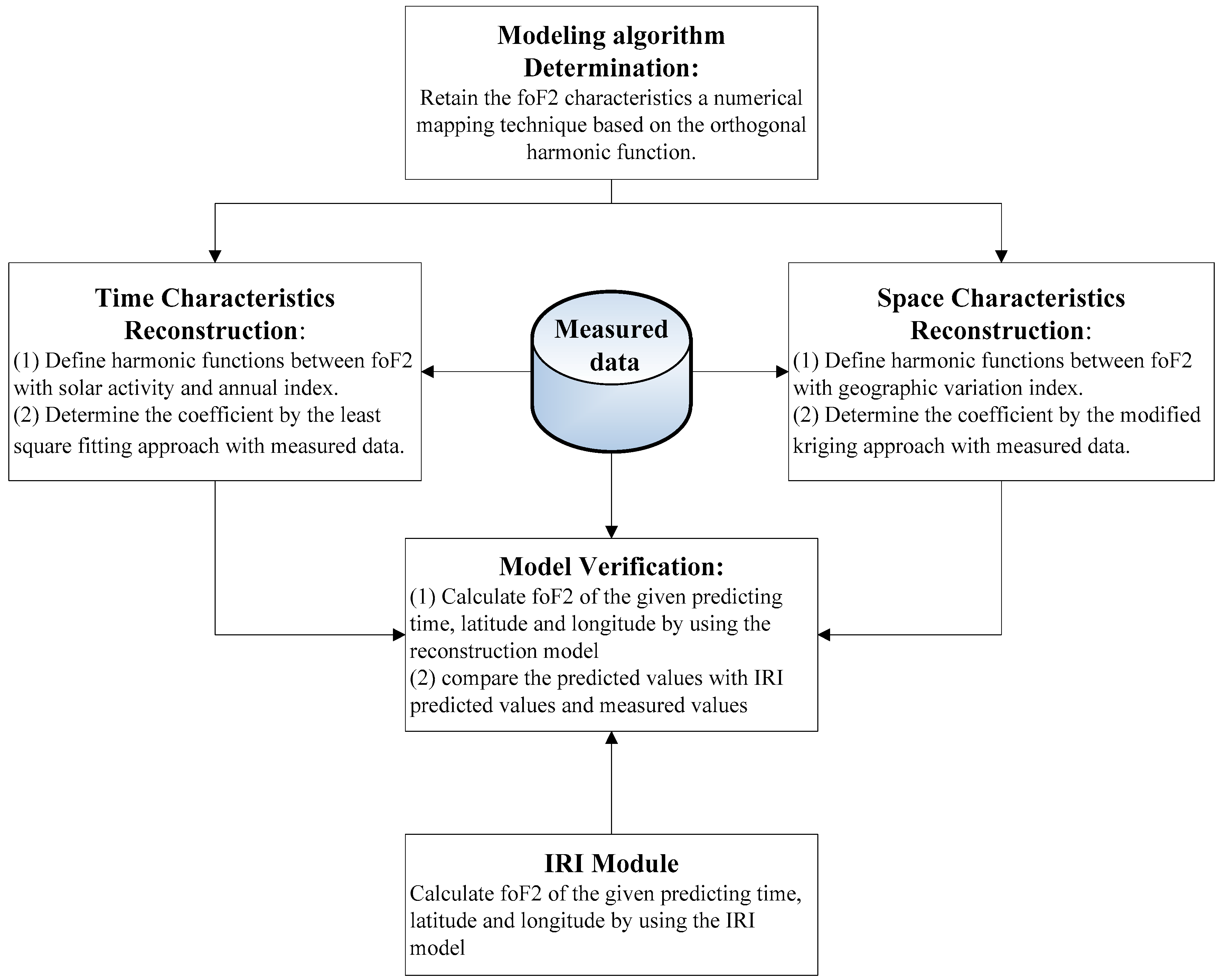
2. Modeling Method
2.1. Base Algorithm
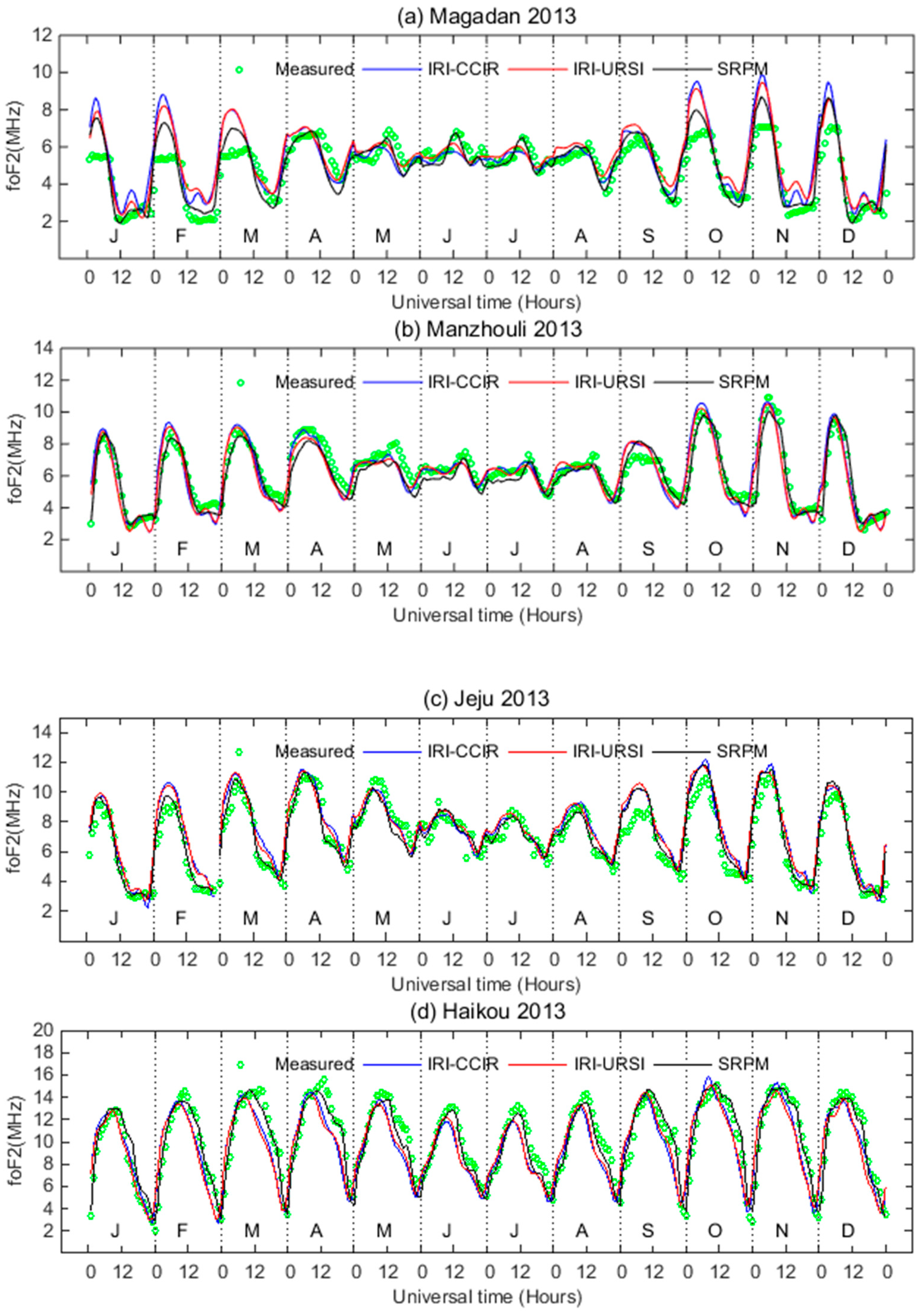
2.2. Model Verification
2.3. Dataset
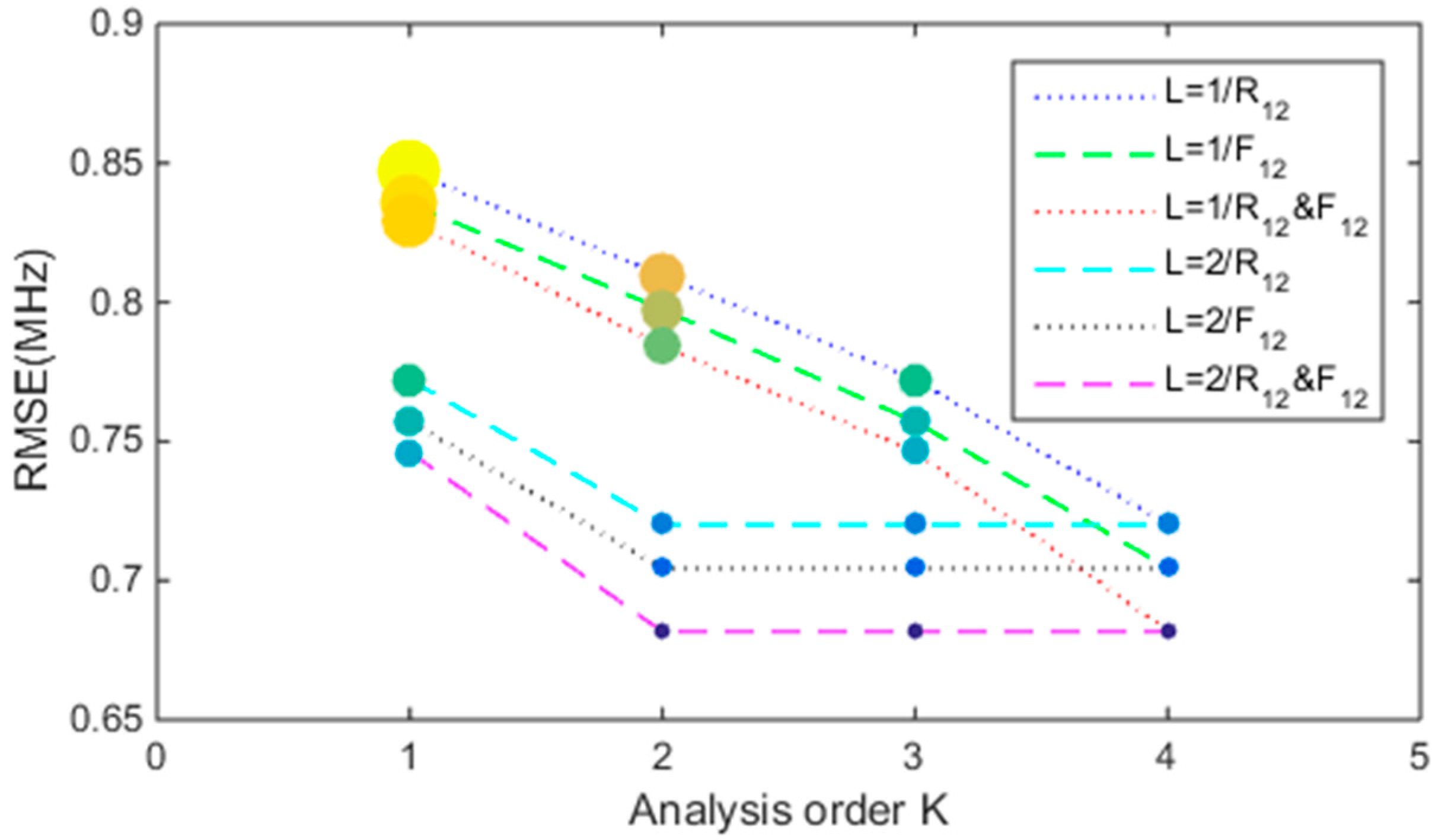
3. Model Reconstruction
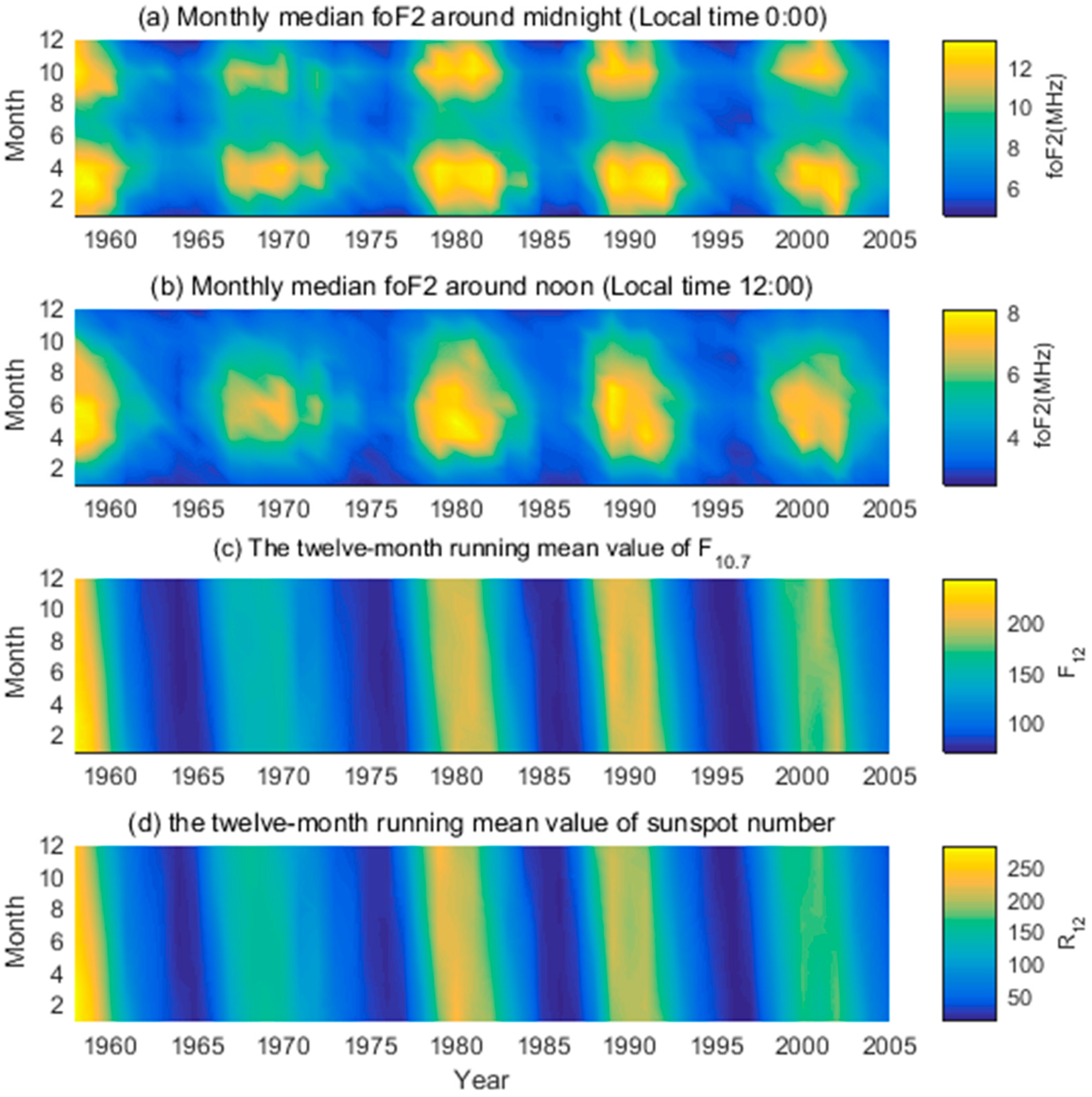
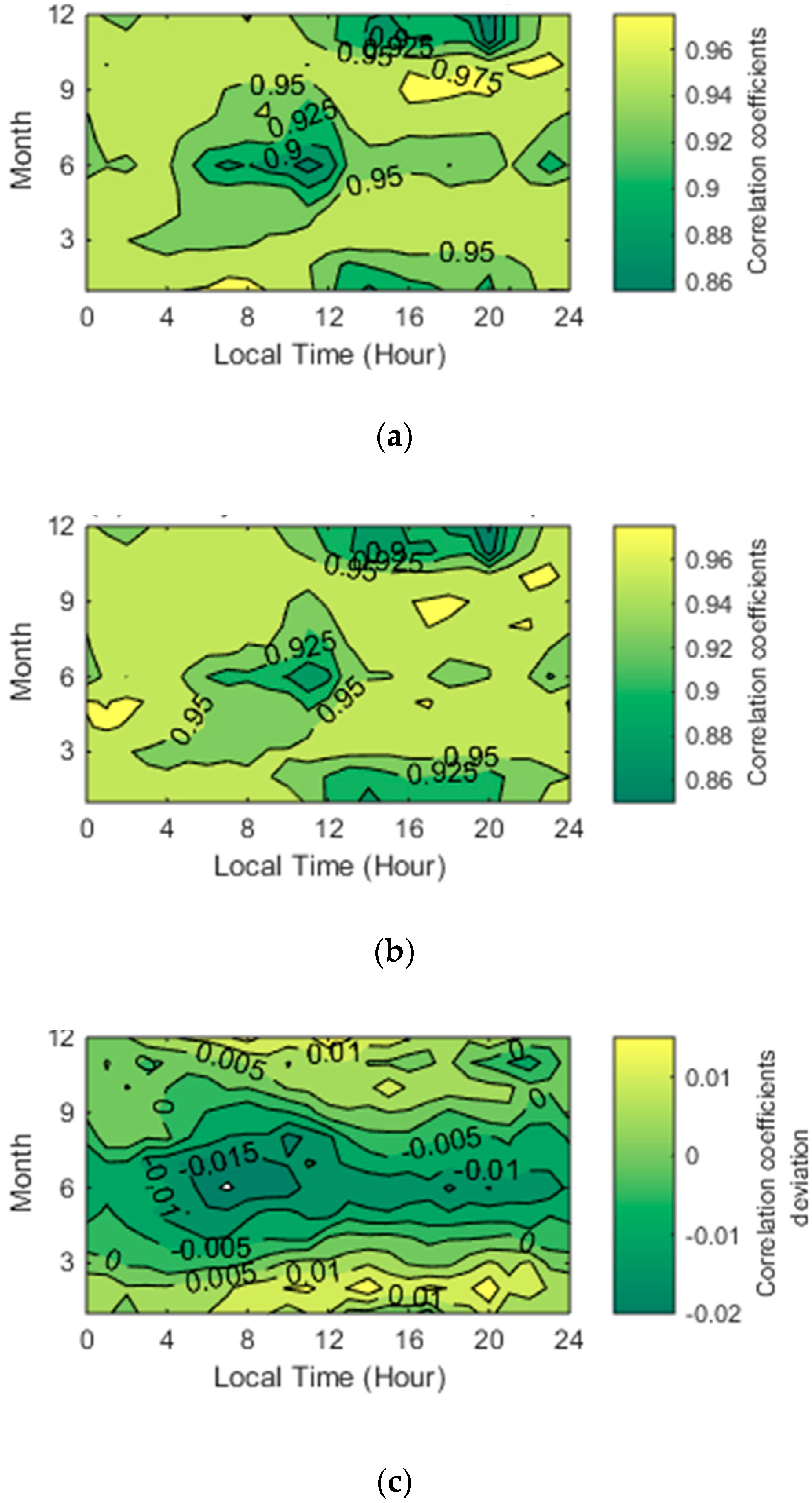
3.1. Temporal Characteristics Reconstruction
3.2. Spatial Characteristics Reconstruction
4. Discussions
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Yan, Z.; Wang, G.; Tian, G.; Li, W.; Su, D.; Rahman, T. The HF Channel EM Parameters Estimation Under a Complex Environment Using the Modified IRI and IGRF Model. IEEE Trans. Antenn. Propag. 2011, 59, 1778–1783. [Google Scholar] [CrossRef]
- Sezen, U.; Sahin, O.; Arikan, F.; Arikan, O. Estimation of hmF2 and foF2 Communication Parameters of Ionosphere Layer Using GPS Data and IRI-Plas Model. IEEE Trans. Antenn. Propag. 2013, 61, 5264–5273. [Google Scholar] [CrossRef]
- Mitch, R.H.; Psiaki, M.L.; Tong, D.M. Local ionosphere model estimation from dual-frequency global navigation satellite system observables. Radio Sci. 2013, 48, 671–684. [Google Scholar] [CrossRef]
- Wang, J.; Feng, X.Z.; Zhao, H.M.; Fu, W.; Ji, S.Y.; Wang, P. Refined study of HF frequency prediction method in China region. Chin. J. Geophys. Chin. Ed. 2013, 56, 1797–1808. [Google Scholar] [CrossRef]
- Yao, M.; Zhao, Z.; Chen, G.; Yang, G.; Su, F.; Li, S.; Zhang, X. Comparison of radar waveforms for a low-power vertical-incidence ionosonde. IEEE Geosci. Remote Sens. 2013, 7, 636–640. [Google Scholar] [CrossRef]
- Jiang, C.; Yang, G.; Zhao, Z.; Zhang, Y.; Zhu, P.; Sun, H. An automatic scaling technique for obtaining F2 parameters and F1 critical frequency from vertical incidence ionograms. Radio Sci. 2013, 48, 739–751. [Google Scholar] [CrossRef]
- Bullett, T.; Mabie, J. Vertical and oblique ionosphere sounding during the 21 August 2017 solar eclipse. Geophys. Res. Lett. 2018, 45, 3690–3697. [Google Scholar] [CrossRef]
- Pietrella, M.; Perrone, L. Instantaneous space-weighted ionospheric regional model for instantaneous mapping of the critical frequency of the F2 layer in the European region. Radio Sci. 2005, 40, RS1005. [Google Scholar] [CrossRef]
- Angling, M.J.; Shaw, J.; Shukla, A.K.; Cannon, P.S. Development of an HF selection tool based on the electron density assimilative model near-real-time ionosphere. Radio Sci. 2009, 44, 1–11. [Google Scholar] [CrossRef]
- Chen, C.H.; Lin, C.; Chen, W.H.; Matsuo, T. Modeling the ionospheric prereversal enhancement by using coupled thermosphere-ionosphere data assimilation. Geophys. Res. Lett. 2017, 44, 1652–1659. [Google Scholar] [CrossRef]
- Ikubanni, S.O.; Adeniyi, J.O. Relationship between ionospheric F2-layer critical frequency, F10.7, and F10.7P around African EIA trough. Adv. Space Res. 2017, 59, 1014–1022. [Google Scholar] [CrossRef]
- Jones, W.B.; Gallet, R.M. Representation of diurnal and geographic variations of ionospheric data by numerical methods. J. Res. Natl. Bur. Stand. 1962, 66D, 419–438. [Google Scholar] [CrossRef]
- Jones, W.B.; Gallet, R.M. The representation of diurnal and geographic variations of ionospheric data by numerical methods. Telecomm. J. 1965, 32, 18–28. [Google Scholar] [CrossRef]
- International Telecommunication Union. Recommendation ITU-R P.1239-3. In ITU-R Reference Ionospheric Characteristics; ITU: Geneva, Switzerland, 2012. [Google Scholar]
- Bilitza, D. International Reference Ionosphere: IRI-90; National Space Science Data Center: Greenbelt, MD, USA, 1990; pp. 52–65. [Google Scholar]
- Rawer, K.; Lincoln, J.V.; Conkright, R.O. International Reference Ionosphere—IRI 79. In Report UAG-82; World Data Center A for Solar-Terrestrial Physics: Boulder, CO, USA, 1981. [Google Scholar]
- Bilitza, D. International Reference Ionosphere 2000. Radio Sci. 2001, 36, 261–275. [Google Scholar] [CrossRef]
- Bilitza, D.; Reinisch, B.W. International Reference Ionosphere 2007: Improvements and new parameters. Adv. Space Res. 2008, 4, 599–609. [Google Scholar] [CrossRef]
- Bilitza, D.; Altadill, D.; Zhang, Y.; Mertens, C.; Truhlik, V. The International reference ionosphere 2012—A model of international collaboration. Space Weather 2014, 4, 689–721. [Google Scholar] [CrossRef]
- Bilitza, D.; Altadill, D.; Truhlik, V.; Shubin, V.; Galkin, I.; Reinisch, B.; Huang, X. International Reference Ionosphere 2016: From ionospheric climate to real-time weather predictions. Space Weather 2017, 15, 418–429. [Google Scholar] [CrossRef]
- Fernandez, J.R.; Mertens, C.J.; Bilitza, D.; Xu, X.; Russell, J.M.; Mlynczak, M.G. Feasibility of developing an ionospheric E-region electron density storm model using TIMED/SABER measurements. Adv. Space Res. 2010, 46, 1070–1077. [Google Scholar] [CrossRef]
- Oyeyemi, E.O.; Poole, A.W.V.; McKinnell, L.A. On the global model for foF2 using neural networks. Radio Sci. 2005, 40, RS6011. [Google Scholar] [CrossRef]
- Ercha, A.; Zhang, D.; Xiao, Z.; Hao, Y.; Ridley, A.J.; Moldwin, M. Modeling ionospheric foF2 by using empirical orthogonal function analysis. Ann. Geophys. 2011, 29, 1501–1515. [Google Scholar] [CrossRef]
- Jiang, C.; Zhou, C.; Yang, G.; Lan, T.; Yang, G.; Zhao, Z.; Zhu, P.; Sun, H.; Xiao, C. Comparison of the Kriging and neural network methods for modeling foF2 maps over North China region. Adv. Space Res. 2015, 56, 38–46. [Google Scholar] [CrossRef]
- Perna, L.; Pezzopane, M.; Pietrella, M.; Zolesi, B.; Cander, L.R. An updating of the SIRM model. Adv. Space Res. 2017, 60, 1249–1259. [Google Scholar] [CrossRef]
- Zolesi, B.; Cander, L.R. Advances in regional ionospheric mapping over Europe. Ann. Geophys. 1998, 41, 827–842. [Google Scholar]
- Francechi, G.D.; Zolesi, B. Regional ionospheric mapping and modeling over Antarctica. Ann Geophys. 1998, 41, 813–818. [Google Scholar]
- Dabas, R.S.; Sharma, K.; Das, R.M.; Sethi, N.K.; Pillai, K.G.M.; Mishra, A.K. Ionospheric modeling for shortand long-term predictions of F region parameters over Indian zone. J. Geophys. Res. 2008, 113, A03306. [Google Scholar] [CrossRef]
- Cao, H.; Sun, X. A new method of predicting the ionospheric F2 layer in the Asia oceania Region. Chin. J. Space Sci. 2009, 29, 502–507. [Google Scholar] [CrossRef]
- Ercha, A.; Zhang, D.; Ridley, A.J.; Xiao, Z.; Hao, Y. A global model: Empirical orthogonal function analysis of total electron content 1999–2009 data. J. Geophys. Res. 2012, 117, A03328. [Google Scholar] [CrossRef]
- Athieno, R.; Jayachandran, P.T.; Themens, D.R.; Danskin, D.W. Comparison of observed and predicted MUF (3000) F2 in the polar cap region. Radio Sci. 2015, 50, 509–517. [Google Scholar] [CrossRef]
- Zolesi, B.; Belehaki, A.; Tsagouri, I.; Cander, L.R. Real-time updating of the Simplified Ionospheric Regional Model for operational applications. Radio Sci. 2004, 39, 1–12. [Google Scholar] [CrossRef]
- Nandi, S.; Bandyopadhyay, B. Study of low-latitude ionosphere over Indian region using simultaneous algebraic reconstruction technique. Adv. Space Res. 2015, 55, 545–553. [Google Scholar] [CrossRef]
- Zhang, M.; Liu, C.; Wan, W.; Liu, L.; Ning, B. A global model of the ionospheric F2 peak height based on EOF analysis. Ann. Geophys. 2009, 27, 3203–3212. [Google Scholar] [CrossRef]
- Pearson, K. On lines and planes of closest fit to systems of points in space. Edinb. Dublin Philos. Mag. J. Sci. 1901, 2, 559–572. [Google Scholar] [CrossRef]
- Dvinskikh, N.I. Expansion for ionospheric characteristics in empirical orthogonal functions. Adv. Space Res. 1988, 8, 179–181. [Google Scholar] [CrossRef]
- Singer, W.; Dvinskikh, N.I. Comparison of empirical models of ionospheric characteristics developed by means of different mapping methods. Adv. Space Res. 1991, 11, 3–6. [Google Scholar] [CrossRef]
- Bibl, K. 60 years of ionospheric measurements and studies. Adv. Radio Sci. 2004, 2, 265–268. [Google Scholar] [CrossRef][Green Version]
- Reinisch, B.W.; Galkin, I.A.; Khmyrov, G.M.; Kozlov, A.V.; Bibl, K.; Lisysyan, I.A.; Cheney, G.P.; Huang, X.; Kitrosser, D.F.; Paznukhov, V.V.; et al. New digisonde for research and monitoring applications. Radio Sci. 2009, 44, RS0A24. [Google Scholar] [CrossRef]
- Wang, J.; Ji, S.; Wang, H.; Lu, D. Method for determining the critical frequency and propagation factor at the path midpoint from maximum usable frequency and its propagation delay based on oblique sounder. Chin. J. Space Sci. 2014, 34, 160–167. [Google Scholar] [CrossRef]
- Lan, J.; Ning, B.; Zhu, Z.; Hu, L.; Sun, W.; Li, G. Development of agile digital ionosonde and its preliminary observation. Chin. J. Space Sci. 2019, 39, 167–177. [Google Scholar] [CrossRef]
- Zhang, X.F.; Le, G.M.; Zhang, Y.X. Phase relation between the relative sunspot number and solar 10.7 cm flux. Chinese. Sci. Bull. 2012, 57, 2078–2082. [Google Scholar] [CrossRef]
- Purushottam, B.; Kamal, K.; Suresh, K.D.; Pramod, K.P.; Malik, A.W.; Prakash, K.; Roshni, A. Characteristic of ionospheric foF2 and solar indices during the 23rd solar cycle over high latitude station, Syowa, Antarctica. Am. J. Clim. Chang. 2015, 4, 408–416. [Google Scholar] [CrossRef][Green Version]
- Qian, L.; Burns, A.G.; Solomon, S.C.; Wang, W. Annual/semiannual variation of the ionosphere. Geophys. Res. Lett. 2013, 40, 1928–1933. [Google Scholar] [CrossRef]
- Fang, T.W.; Akmaev, R.; Fuller, R.T.; Wu, F.; Maruyama, N.; Millward, G. Longitudinal and day-to-day variability in the ionosphere from lower atmosphere tidal forcing. Geophys. Res. Lett. 2013, 40, 2523–2528. [Google Scholar] [CrossRef]
- Shepard, D. A two-dimensional interpolation function for regularly spaced data. In Proceedings of the ACM 68 Proceedings of the 1968 23rd ACM National Conference, Princeton, NJ, USA, 27–29 August 1968; pp. 517–524. [Google Scholar] [CrossRef]
- Pradipta, R.; Valladares, C.E.; Carter, B.A.; Doherty, P.H. Interhemispheric propagation and interactions of auroral traveling ionospheric disturbances near the equator. J. Geophys. Res. Space Phys. 2016, 121, 2462–2474. [Google Scholar] [CrossRef]
- Briggs, I.C. Machine contouring using minimum curvature. Geophysics 1974, 39, 39–48. [Google Scholar] [CrossRef]
- Hardy, R.L.; Nelson, S.A. A multiquadric biharmonic representation and approximation of disturbing potential. Geophys. Res. Lett. 1986, 13, 18–21. [Google Scholar] [CrossRef]
- Sandwell, D.T. Biharmonic Spline Interpolation of GEOS-3 and SEASAT Altimeter Data. Geophys. Res. Lett. 1987, 14, 139–142. [Google Scholar] [CrossRef]
- Zolesi, B.; Cander, L.R.; De Franceschi, G. Simplified ionospheric regional model for telecommunication applications. Radio Sci. 1993, 28, 603–612. [Google Scholar] [CrossRef]
- Zolesi, B.; Cander, L.R.; De Franceschi, G. On the potential applicability of the simplified ionospheric regional model to different midlatitude areas. Radio Sci. 1996, 31, 547–552. [Google Scholar] [CrossRef]
- Stanislawska, I.; Juchnikowski, G.; Cander, L.R. Kriging method for instantaneous mapping at low and equatorial latitudes. Adv. Space. Res. 1996, 18, 845–853. [Google Scholar] [CrossRef]
- Consultative Committee of International Radio. CCIRAtlas of Ionospheric Characteristics; Report 340-6; International Telecommunication Union: Geneva, Switzerland, 1991. [Google Scholar]
- Bates, A.P.; Khalid, Z.; Kennedy, R.A. Slepian Spatial-Spectral Concentration Problem on the Sphere: Analytical Formulation for Limited Colatitude–Longitude Spatial Region. IEEE Trans. Signal Process. 2016, 65, 1527–1537. [Google Scholar] [CrossRef]
- Deng, X.; Tang, Z. Moving surface spline interpolation based on green’s function. Math. Geosci. 2011, 43, 663–680. [Google Scholar] [CrossRef]
- Smith, M.J.; Cesnik, C.E.S.; Hodges, D.H. Evaluation of some data transfer algorithms for noncontiguous meshes. J. Aerosp. Eng. 2000, 13, 52–58. [Google Scholar] [CrossRef]
- De Boer, A.; van Zuijlen, A.H.; Bijl, H. Radial Basis Functions for Interface Interpolation and Mesh Deformation. Adv. Comput. Methods Sci. Eng. 2010, 71, 143–178. [Google Scholar] [CrossRef]
- Olivier, D. Comparing splines and kriging. Comput. Geosci. 1984, 10, 327–338. [Google Scholar]
- Danilov, A.D.; Konstantinova, A.V. Relationship between foF2 trends and geographic and geomagnetic coordinates. Geomagn. Aeron. 2014, 54, 348–354. [Google Scholar] [CrossRef]
- VanZandt, T.E.; Clark, W.L.; Warnock, J.M. Magnetic Apex Coordinates: A magnetic coordinate system for the ionospheric F2 layer. J. Geophys. Res. 1972, 77, 2406–2411. [Google Scholar] [CrossRef]
- Gustafsson, G.; Papitashvili, N.E.; Papitashvili, V.O. A revised corrected geomagnetic coordinate system for Epochs 1985 and 1990. J. Atmos. Terr. Phys. 1992, 54, 1609–1631. [Google Scholar] [CrossRef]
- Rawer, K. Geophysics III. In Encyclopedia of Physics; Springer: New York, NY, USA, 1984; Volume 7, pp. 389–391. [Google Scholar]
- Emmert, J.T.; Richmond, A.D.; Drob, D.P. A computationally compact representation of Magnetic-Apex and Quasi-Dipole coordinates with smooth base vectors. J. Geophys. Res. 2010, 115, A08322. [Google Scholar] [CrossRef]

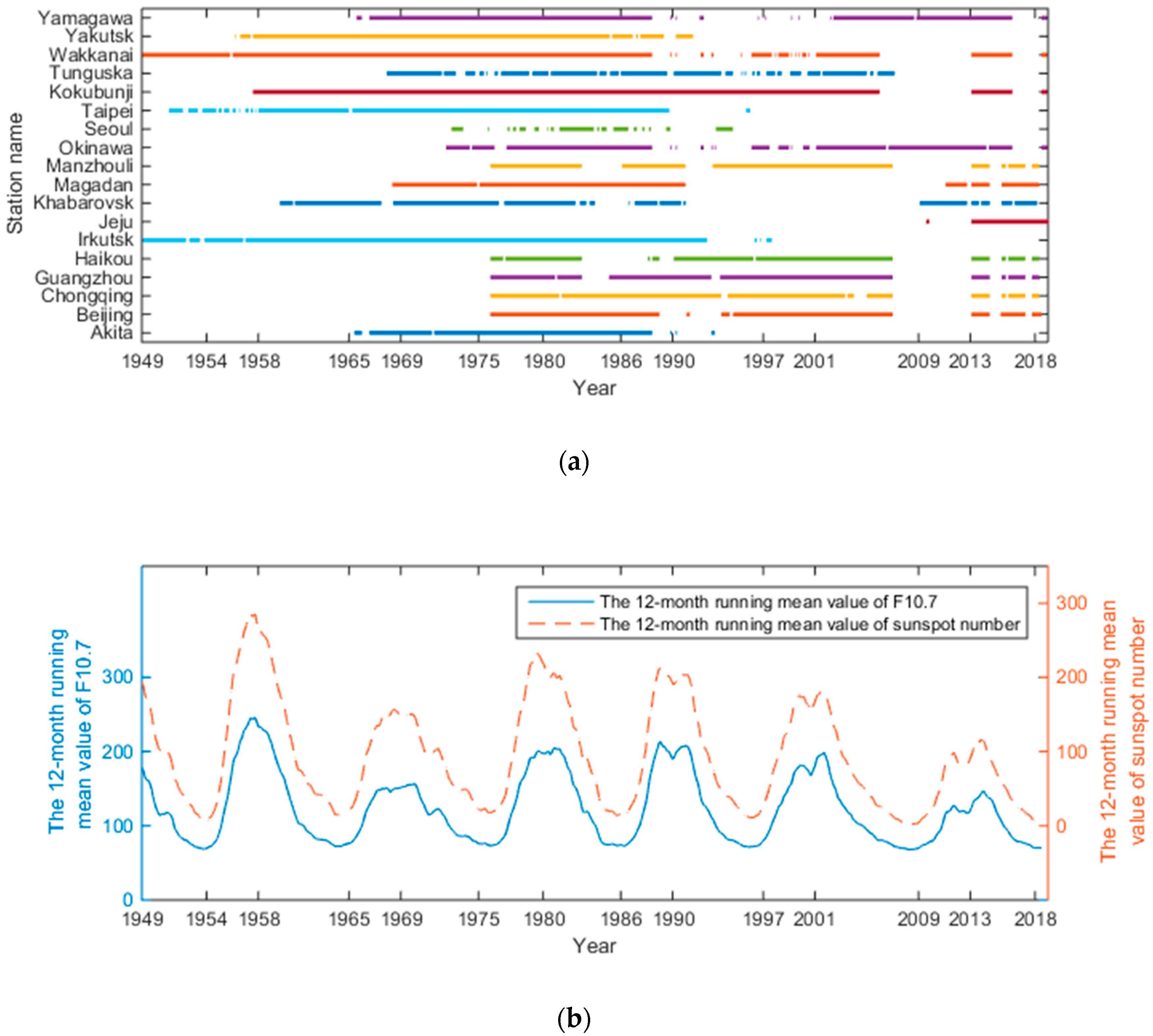




| Station Label | Station Name | Geog. Lon. (oE) | Geog. Lat. (oN) | Geomag. Lon. (oE) | Geomag. Lat. (oN) | Geomag. Dip Lat. (oN) | Modify Geomag. Dip Lat. (oN) | Data Volume (Entries) |
|---|---|---|---|---|---|---|---|---|
| 1 | Akita | 39.70 | 140.10 | 205.33 | 29.01 | 53.67 | 46.88 | 6683 |
| 2 | Beijing | 40.00 | 116.30 | 184.60 | 28.04 | 57.28 | 48.80 | 8772 |
| 3 | Chongqing | 29.50 | 106.40 | 175.80 | 17.54 | 42.56 | 38.52 | 9551 |
| 4 | Guangzhou | 23.10 | 113.40 | 182.25 | 11.11 | 31.44 | 29.77 | 9239 |
| 5 | Haikou | 20.00 | 110.30 | 179.34 | 8.00 | 25.07 | 24.30 | 8213 |
| 6 | Irkutsk | 52.50 | 104.00 | 174.40 | 40.57 | 71.24 | 57.89 | 12,720 |
| 7 | Jeju | 33.50 | 126.50 | 193.90 | 21.89 | 47.86 | 42.45 | 1771 |
| 8 | Khabarovsk | 48.50 | 135.10 | 199.90 | 37.36 | 63.78 | 53.83 | 10,002 |
| 9 | Magadan | 60.00 | 151.00 | 210.09 | 50.13 | 71.46 | 60.45 | 8219 |
| 10 | Manzhouli | 49.60 | 117.50 | 185.32 | 37.66 | 67.45 | 55.64 | 8589 |
| 11 | Okinawa | 26.30 | 127.80 | 195.54 | 14.77 | 36.84 | 34.18 | 10,167 |
| 12 | Seoul | 37.20 | 126.60 | 193.74 | 25.59 | 52.73 | 45.88 | 3120 |
| 13 | Taipei | 25.00 | 121.50 | 189.77 | 13.19 | 35.01 | 32.70 | 10,824 |
| 14 | Tokyo | 35.70 | 139.50 | 205.31 | 24.99 | 48.91 | 43.45 | 15,046 |
| 15 | Tunguska | 61.60 | 90.00 | 164.56 | 50.18 | 78.38 | 63.25 | 9480 |
| 16 | Wakkanai | 45.40 | 141.70 | 205.89 | 34.82 | 59.61 | 51.15 | 15,271 |
| 17 | Yakutsk | 62.00 | 129.60 | 193.60 | 50.46 | 75.88 | 62.64 | 9840 |
| 18 | Yamagawa | 31.20 | 130.60 | 197.76 | 19.83 | 44.11 | 39.77 | 11,167 |
| Statistical Analysis Item | RMSE (MHz) | Relative RMSE (%) | Percent Difference between CCIR and SRPM (%) | Percent Difference between CCIR and SRPM (%) | |||||
|---|---|---|---|---|---|---|---|---|---|
| IRI–CCIR | IRI–URSI | SRPM | IRI–CCIR | IRI–URSI | SRPM | ||||
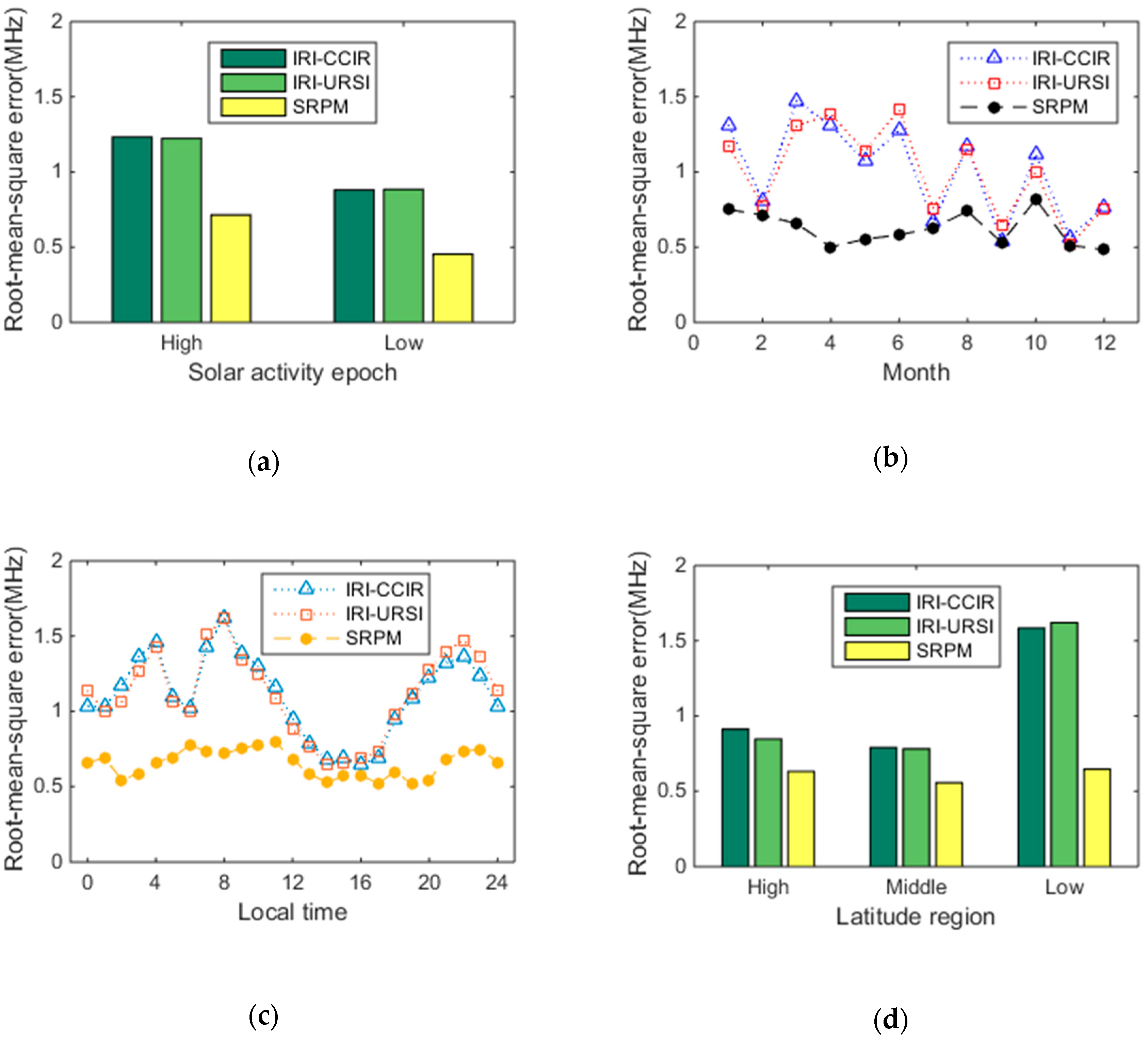
| solar activity epochs | high | 1.23 | 1.22 | 0.71 | 22.12 | 22.06 | 12.38 | 9.74 | 9.68 |
| low | 0.88 | 0.89 | 0.45 | 19.04 | 20.02 | 11.22 | 7.82 | 8.80 | |
| seasons | spring | 1.29 | 1.28 | 0.57 | 25.32 | 26.01 | 11.92 | 13.39 | 14.09 |
| summer | 1.07 | 1.14 | 0.65 | 18.76 | 20.03 | 13.48 | 5.28 | 6.55 | |
| autumn | 0.79 | 0.75 | 0.64 | 12.05 | 11.88 | 11.14 | 0.91 | 0.74 | |
| winter | 0.99 | 0.92 | 0.66 | 24.97 | 23.46 | 14.77 | 10.20 | 8.69 | |
| local time sectors | midnight (22:00–2:00) | 1.21 | 1.29 | 0.70 | 17.23 | 19.11 | 10.84 | 6.38 | 8.27 |
| sunrise (5:00–9:00) | 1.34 | 1.35 | 0.72 | 33.84 | 33.72 | 18.69 | 15.15 | 15.03 | |
| noon (10:00–14:00) | 1.14 | 1.09 | 0.72 | 16.46 | 15.34 | 10.56 | 5.90 | 4.78 | |
| sunset (16:00–20:00) | 0.83 | 0.85 | 0.56 | 11.03 | 11.87 | 8.77 | 2.26 | 3.10 | |
| latitude regions | high (≥60°N) | 0.91 | 0.85 | 0.63 | 24.21 | 23.43 | 16.27 | 7.93 | 7.16 |
| middle (30°N–60°N) | 0.79 | 0.78 | 0.56 | 16.47 | 16.11 | 10.43 | 6.03 | 5.67 | |
| low (≤30°N) | 1.58 | 1.62 | 0.65 | 23.98 | 26.60 | 8.71 | 15.27 | 17.89 | |
| average | 1.08 | 1.08 | 0.63 | 20.42 | 20.74 | 12.25 | 8.17 | 8.49 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Bai, H.; Huang, X.; Cao, Y.; Chen, Q.; Ma, J. Simplified Regional Prediction Model of Long-Term Trend for Critical Frequency of Ionospheric F2 Region over East Asia. Appl. Sci. 2019, 9, 3219. https://doi.org/10.3390/app9163219
Wang J, Bai H, Huang X, Cao Y, Chen Q, Ma J. Simplified Regional Prediction Model of Long-Term Trend for Critical Frequency of Ionospheric F2 Region over East Asia. Applied Sciences. 2019; 9(16):3219. https://doi.org/10.3390/app9163219
Chicago/Turabian StyleWang, Jian, Hongmei Bai, Xiangdong Huang, Yuebin Cao, Qiang Chen, and Jianguo Ma. 2019. "Simplified Regional Prediction Model of Long-Term Trend for Critical Frequency of Ionospheric F2 Region over East Asia" Applied Sciences 9, no. 16: 3219. https://doi.org/10.3390/app9163219
APA StyleWang, J., Bai, H., Huang, X., Cao, Y., Chen, Q., & Ma, J. (2019). Simplified Regional Prediction Model of Long-Term Trend for Critical Frequency of Ionospheric F2 Region over East Asia. Applied Sciences, 9(16), 3219. https://doi.org/10.3390/app9163219






