Light Transmission in Fog: The Influence of Wavelength on the Extinction Coefficient
Abstract
:Featured Application
Abstract
1. Introduction
- the real droplet size distribution (DSD) of fog;
- two fog types;
- the meteorological optical range (MOR), denoted by V, from 15 m to 175 m;
- and wavelengths from 350 nm to 2450 nm.
2. Theory and Definitions: Light and Fog
2.1. Fog Definition
2.2. Different Kinds of Fog
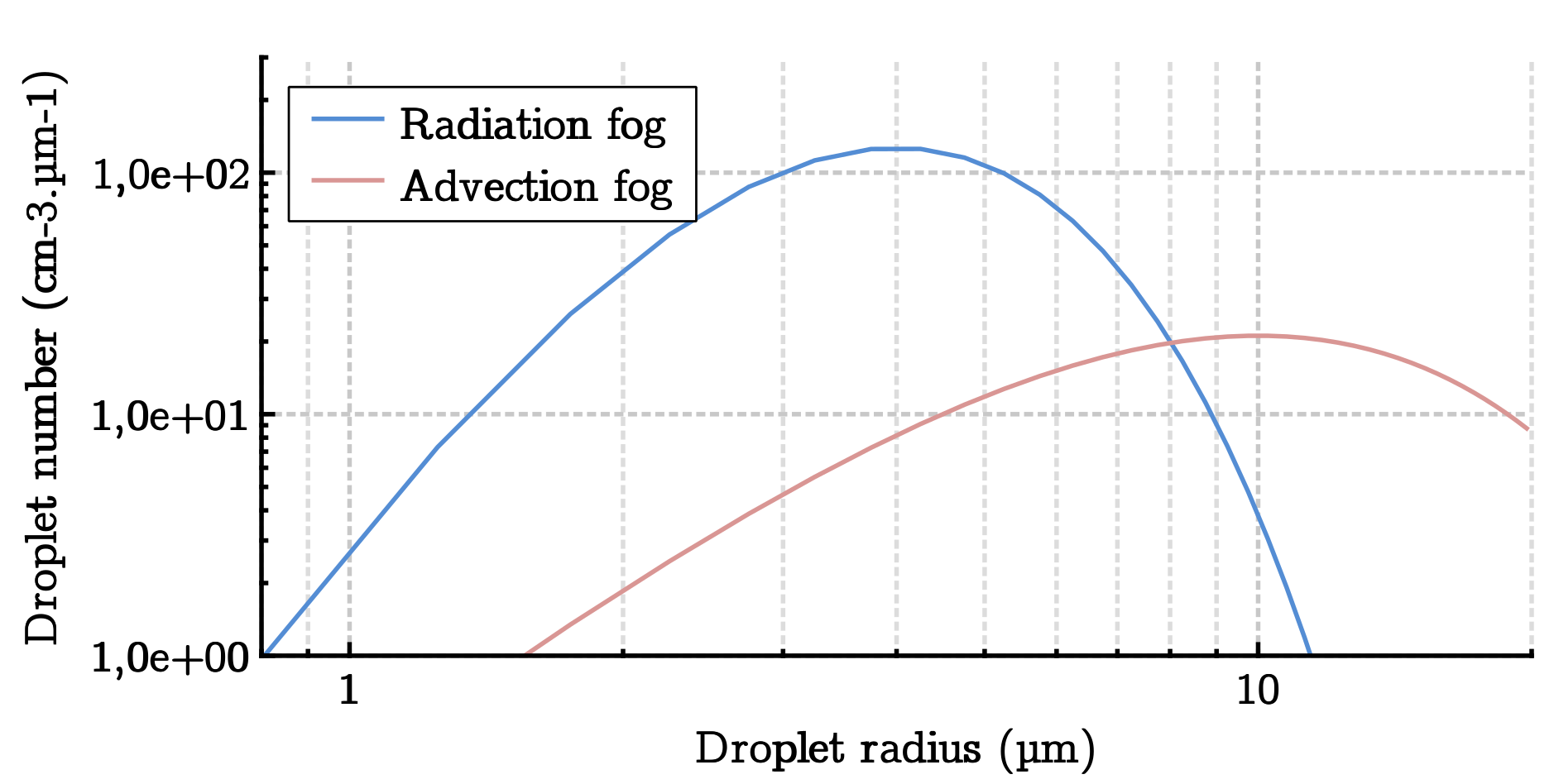
- Radiation fog forms overnight or early in the morning. This type of fog generally dissipates in the morning. It frequently flows into valleys or low-lying areas. It may then also be qualified as “continental fog”. Radiation fog is composed of small droplets, with diameters distributed around a mode between one and 10 microns.
- Advection fog forms when a moist warm air mass is pushed over a relatively cold surface. This type of fog can last all day. Advection fog may form over oceans and move to continents where the ground is colder. It may then also be qualified as “maritime fog”. Advection fog is composed of large droplets, with diameters distributed around a mode between 10 and 20 microns.
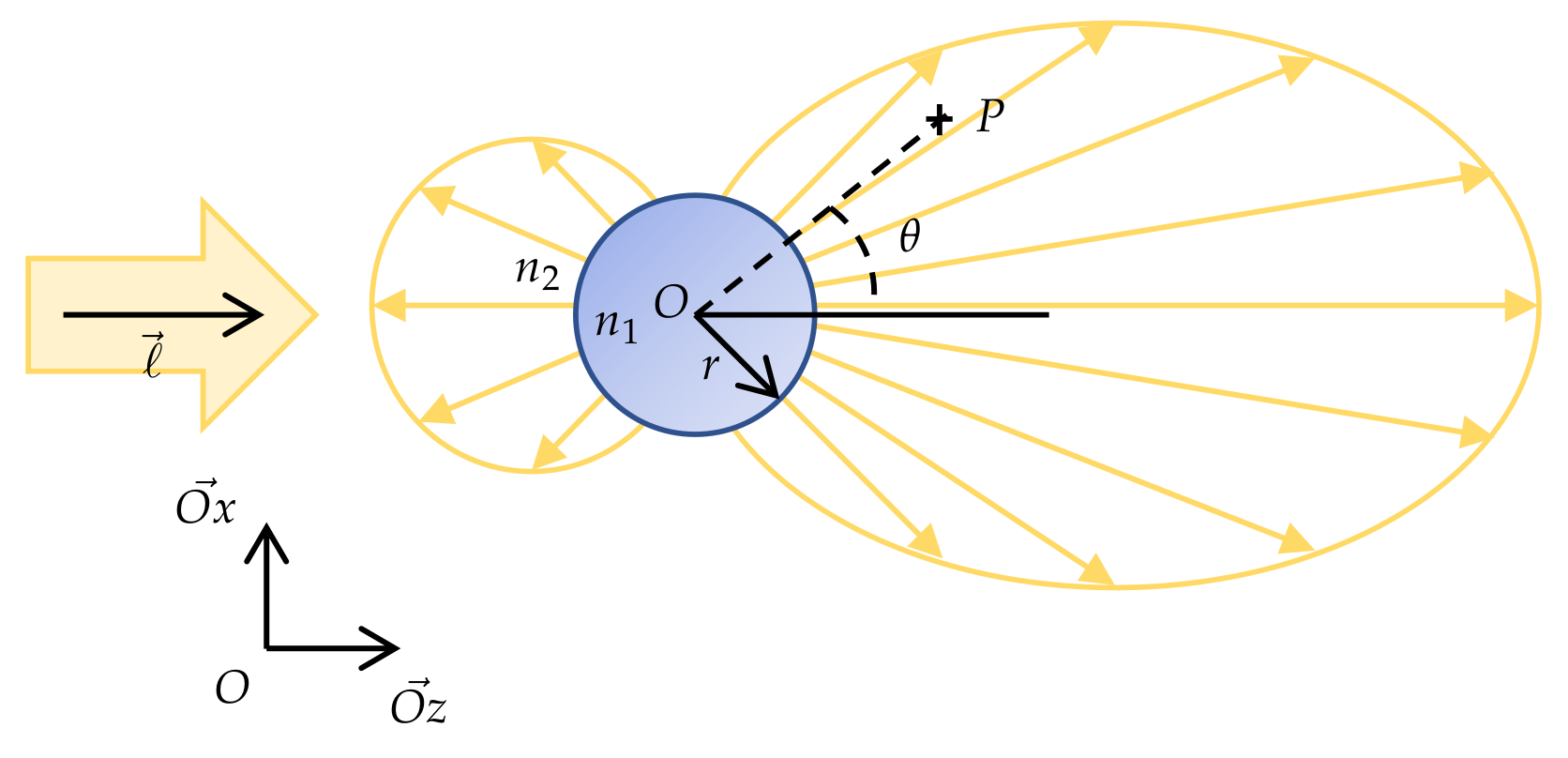
2.3. Light Attenuation Due to Fog
- a monochromatic incident light;
- a spherical, homogeneous, isotropic particle of radius r, of refractive index ;
- non-absorbing dispersion host environment of refractive index ;
- a low concentration (single scattering). This assumption will be discussed further, compared with the MODTRAN model, which includes multiple scattering.
3. Materials and Methods
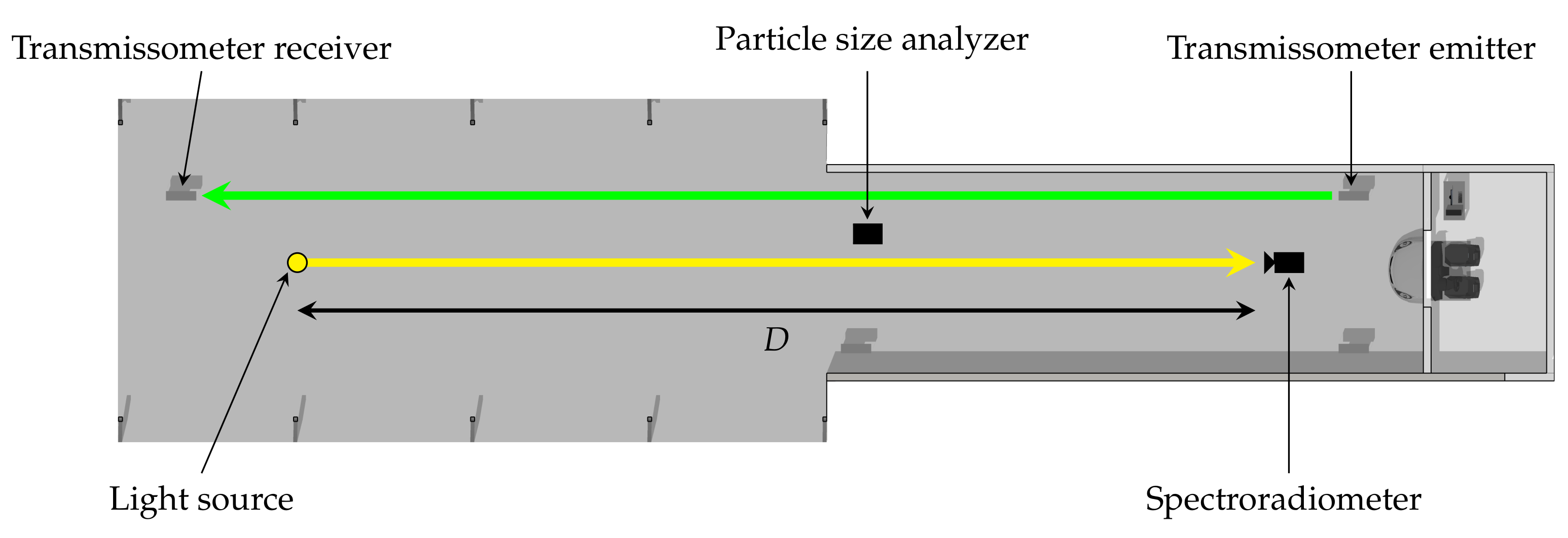
3.1. Pavin BP: A Fog and Rain Facility
3.2. Methodology
- a Spectral Evolution PSR+3500 spectroradiometer measuring radiance for each wavelength from 360–2450 nm. Radiance measurements were grouped into 10-nm packets;
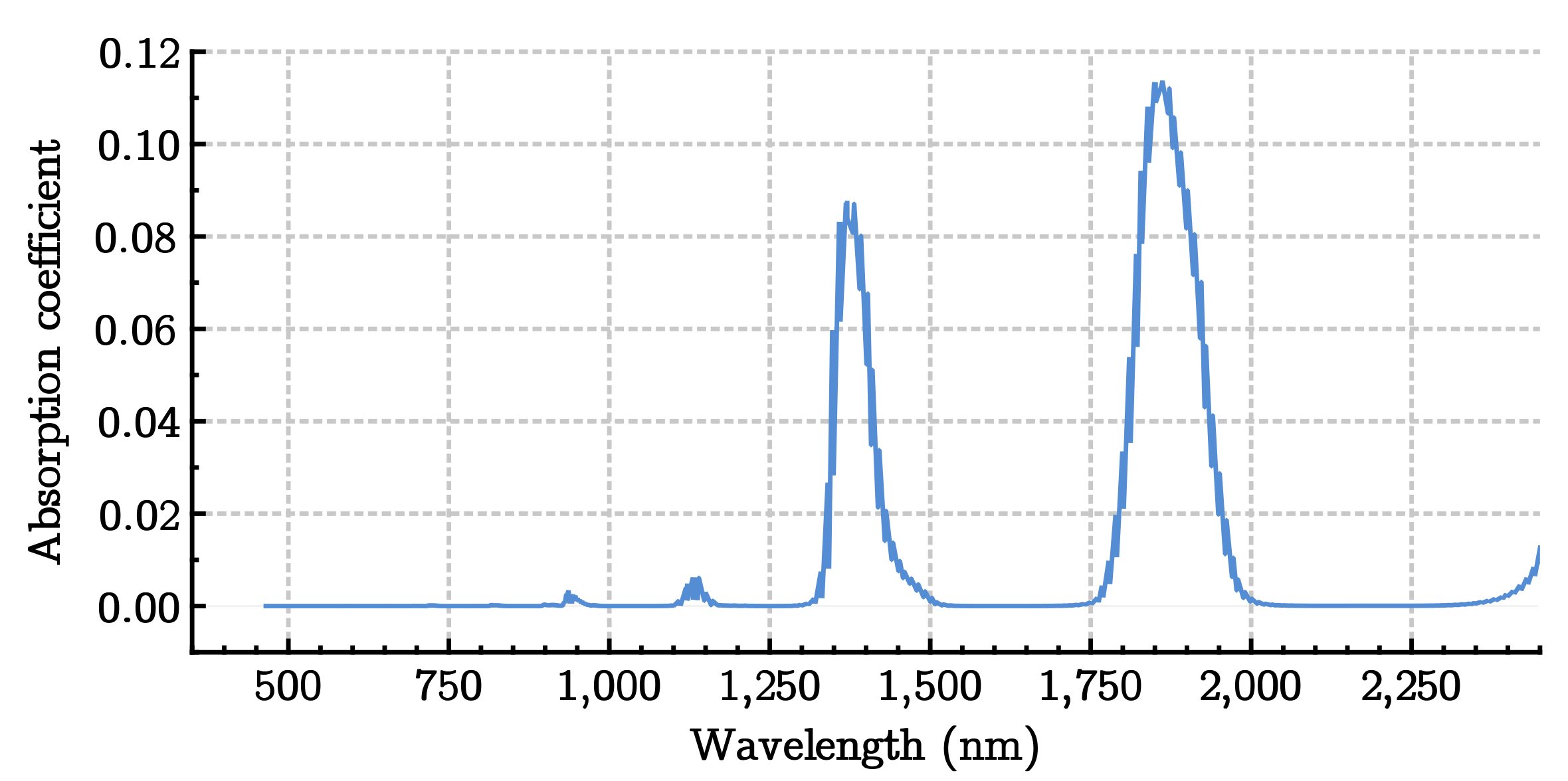
- a continuous spectrum light source. The light source chosen was a 400-W halogen lamp whose characteristics were measured. This source was stable over time, and its relative spectrum was measured and is shown in Figure 4. The halogen source was similar to a black body whose temperature was estimated at 2905 K by optimization; this value was used in the MODTRAN model;
- a PALAS WELAS 2100 PSA measuring the DSD of fog over the range of 0.3–17 m. This PSA identified the number of drops in 60 classes in this range;
- a Degreane Horizon TR30 transmissometer measuring the MOR throughout the measurements.
- a reference measurement was made with the spectroradiometer with no fog (for an MOR greater than 1000 m, according to the WMO standard [10]);
- the fog was produced in the facility until it reached an MOR of 10 m (very dense fog). The minimum value of 10 m was chosen because this was the limit of the transmissometer measurement;
- the production of fog was stopped, and measurements were then made continuously as the fog dissipated from 10 m–1000 m. The measurements included that of the MOR using the transmissometer, the radiance received by the spectroradiometer, and the DSD measured by the PSA;
- to obtain enough data, a second complete dissipation was reproduced using the same protocol.
4. Extinction Coefficient Results
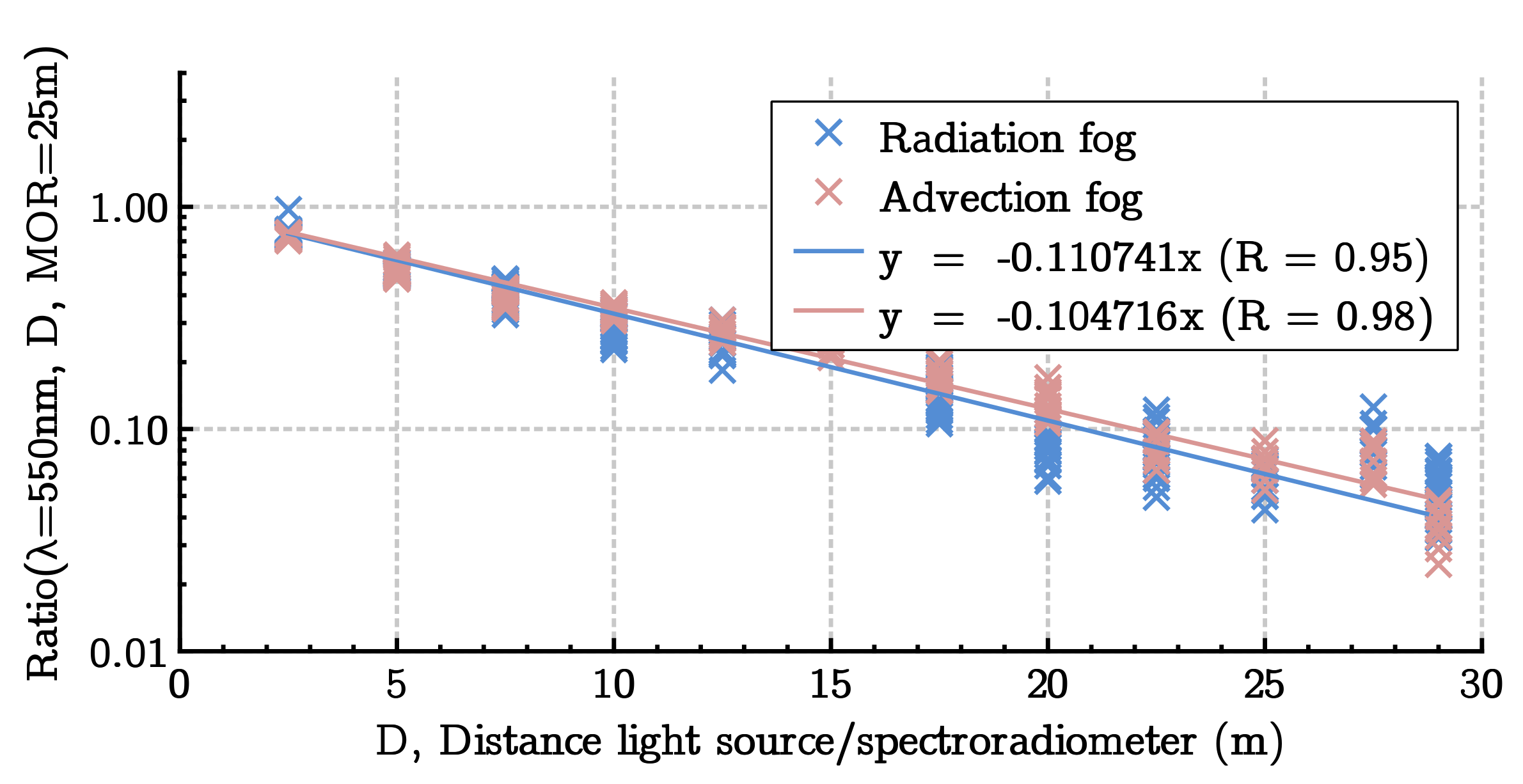
4.1. Estimation of the Extinction Coefficient
- spectroradiometer/light source distance D,
- fog type (advection or radiation),
- MOR (temporal resolution of one second),
- DSD (temporal resolution of one second),
- and radiance for each wavelength (temporal resolution of a few seconds).
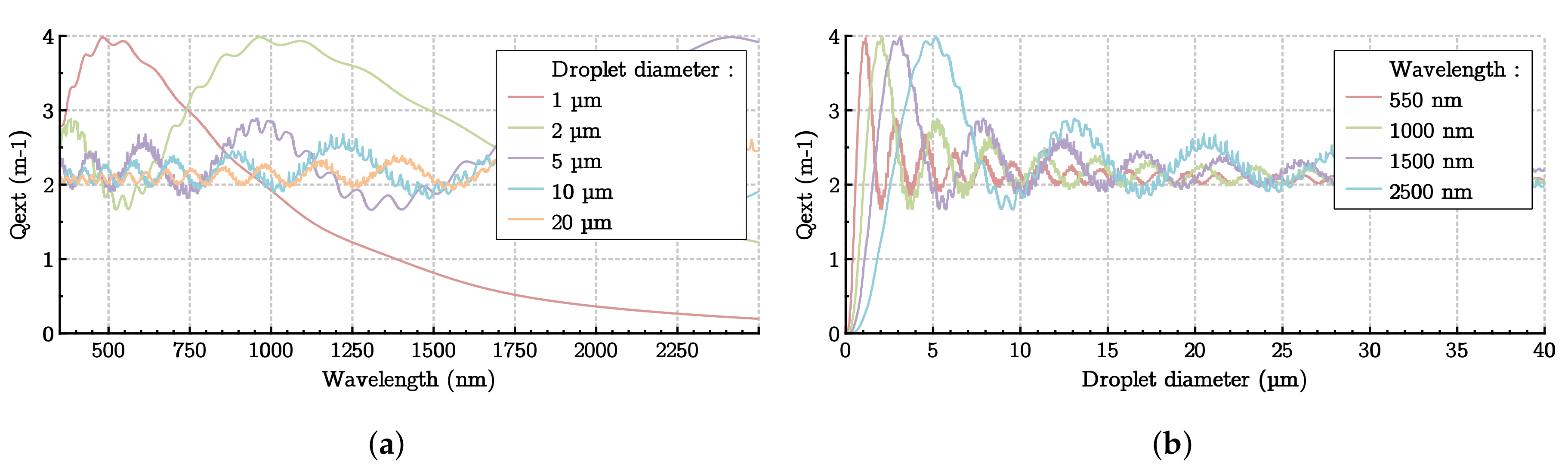
4.2. Analysis of the Extinction Coefficient as a Function of Wavelength
5. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| MOR | Meteorological optical range |
| DSD | Droplet size distribution |
| WMO | World Meteorological Organisation |
| FSO | Free space optics |
| TRL | Technology readiness levels |
| SWIR | Short wave infrared |
| ADAS | Advance driving assistance systems |
| MODTRAN | Moderate resolution atmospheric transmission |
| LOS | Line-of-sight |
References
- Kutila, M.; Pyykönen, P.; Holzhüter, H.; Colomb, M.; Duthon, P. Automotive LiDAR performance verification in fog and rain. In Proceedings of the 2018 21st International Conference on Intelligent Transportation Systems (ITSC), Maui, HI, USA, 4–7 November 2018. [Google Scholar]
- Arnulf, A.; Bricard, J.; Curé, E.; Véret, C. Transmission by Haze and Fog in the Spectral Region 035 to 10 Microns. J. Opt. Soc. Am. 1957, 47, 491. [Google Scholar] [CrossRef]
- Shah, S.; Mughal, S.; Memon, S. Theoretical and empirical based extinction coefficients for fog attenuation in terms of visibility at 850 nm. In Proceedings of the 2015 International Conference on Emerging Technologies, ICET 2015, Peshawar, Pakistan, 19–20 December 2015; pp. 3–6. [Google Scholar] [CrossRef]
- Al Naboulsi, M. Fog attenuation prediction for optical and infrared waves. Opt. Eng. 2004, 43, 319. [Google Scholar] [CrossRef]
- Grabner, M.; Kvicera, V. Multiple scattering in rain and fog on free-space optical links. J. Light. Technol. 2014, 32, 513–520. [Google Scholar] [CrossRef]
- Ijaz, M.; Ghassemlooy, Z.; Le-Minh, H.; Zvanovec, S.; Perez, J.; Pesek, J.; Fiser, O. Experimental validation of fog models for FSO under laboratory controlled conditions. In Proceedings of the IEEE International Symposium on Personal, Indoor and Mobile Radio Communications, PIMRC, London, UK, 8–11 September 2013; pp. 19–23. [Google Scholar] [CrossRef]
- Ijaz, M.; Ghassemlooy, Z.; Rajbhandari, S.; Le Minh, H.; Perez, J.; Gholami, A. Comparison of 830 nm and 1550 nm based free space optical communications link under controlled fog conditions. In Proceedings of the 2012 8th International Symposium on Communication Systems, Networks and Digital Signal Processing, CSNDSP 2012, Poznan, Poland, 18–20 July 2012. [Google Scholar] [CrossRef]
- Hergert, W.; Wriedt, T. The Mie Theory: Basics and Applications; Springer: Berlin/Heidelberg, Germany, 2012; Volume 169. [Google Scholar]
- Berk, A.; Anderson, G.P.; Acharya, P.K.; Bernstein, L.S.; Fox, M.; Adler-Golden, S.M.; Chetwynd, J.H.; Hoke, M.L.; Lewis, P.E. MODTRAN5: A Reformulated Atmospheric Band Model with Auxiliary Species and Practical Multiple Scattering Options. In Proceedings of the Remote Sensing of Clouds and the Atmosphere IX, Maspalomas, Spain, 13–16 September 2004. [Google Scholar]
- World Meteorological Organization. Guide to Meteorological Instruments and Methods of Observation; 2014 Edition Updated in 2017; WMO-No. 8; World Meteorological Organization: Geneva, Switzerland, 2014; p. 1163. [Google Scholar]
- Shettle, E.P.; Fenn, R.W. Models for the aerosols of the lower atmosphere and the effects of humidity variations on their optical properties. Environ. Res. Pap. 1979, 676, 89. [Google Scholar]
- Mason, J. The physics of radiation fog. J. Meteorol. Soc. Jpn. Ser. II 1982, 60, 486–499. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Klett, J.D. Microphysics of Clouds and Precipitation: Reprinted 1980; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Howell, J.R.; Menguc, M.P.; Siegel, R. Thermal Radiation Heat Transfer; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Middleton, W.E.K. Vision through the Atmosphere; University of Toronto Press: Toronto, ON, Canada, 1952. [Google Scholar]
- Mätzler, C. MATLAB Functions for Mie Scattering and Absorption; Version 2; Research Report No. 2002-11; University of Bern: Bern, Switzerland, 2002; p. 24. [Google Scholar]
- Colomb, M.; Hirech, K.; André, P.; Boreux, J.; Lacôte, P.; Dufour, J. An innovative artificial fog production device improved in the European project “FOG”. Atmos. Res. 2008, 87, 242–251. [Google Scholar] [CrossRef]




| MOR, V (m) | Number of Spectroradiometer Measurements | Number of PSA Measurements | ||
|---|---|---|---|---|
| Radiation Fog | Advection Fog | Radiation Fog | Advection Fog | |
| 15 | 360 | 238 | 2198 | 1427 |
| 20 | 240 | 206 | 1399 | 1185 |
| 30 | 241 | 121 | 1354 | 688 |
| 40 | 214 | 96 | 1057 | 567 |
| 50 | 141 | 100 | 732 | 519 |
| 70 | 76 | 90 | 320 | 480 |
| 100 | 57 | 34 | 202 | 176 |
| 125 | 64 | 24 | 266 | 133 |
| 150 | 58 | 30 | 235 | 127 |
| 175 | 54 | 27 | 250 | 112 |
| Ref (>1000 m) | 1028 | 957 | 0 | 0 |
| Total | 2533 | 1923 | 8013 | 5414 |
| MOR (m) | Radiation Fog | Advection Fog | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 360 | 550 | 1000 | 2000 | 2400 | 360 | 550 | 1000 | 2000 | 2400 | ||
| 15 | NA | 0.97 | 0.97 | 0.77 | NA | 0.96 | 0.98 | 0.98 | 0.64 | NA | |
| 20 | 0.91 | 0.98 | 0.98 | 0.99 | 0.96 | 0.96 | 0.98 | 0.98 | 0.98 | 0.66 | |
| 30 | 0.94 | 0.96 | 0.97 | 0.98 | 0.97 | 0.97 | 0.98 | 0.99 | 0.99 | 0.98 | |
| 40 | 0.93 | 0.94 | 0.96 | 0.97 | 0.96 | 0.97 | 0.99 | 0.99 | 0.99 | 0.98 | |
| 50 | 0.90 | 0.90 | 0.93 | 0.93 | 0.93 | 0.94 | 0.97 | 0.97 | 0.98 | 0.98 | |
| 70 | 0.84 | 0.84 | 0.87 | 0.88 | 0.87 | 0.93 | 0.96 | 0.97 | 0.97 | 0.97 | |
| 100 | 0.76 | 0.79 | 0.81 | 0.79 | 0.76 | 0.80 | 0.92 | 0.94 | 0.96 | 0.95 | |
| 125 | 0.49 | 0.50 | 0.67 | 0.78 | 0.78 | 0.69 | 0.85 | 0.88 | 0.92 | 0.91 | |
| 150 | 0.73 | 0.70 | 0.72 | 0.67 | 0.64 | 0.64 | 0.83 | 0.87 | 0.91 | 0.91 | |
| 175 | 0.71 | 0.70 | 0.74 | 0.67 | 0.66 | 0.61 | 0.80 | 0.89 | 0.91 | 0.88 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duthon, P.; Colomb, M.; Bernardin, F. Light Transmission in Fog: The Influence of Wavelength on the Extinction Coefficient. Appl. Sci. 2019, 9, 2843. https://doi.org/10.3390/app9142843
Duthon P, Colomb M, Bernardin F. Light Transmission in Fog: The Influence of Wavelength on the Extinction Coefficient. Applied Sciences. 2019; 9(14):2843. https://doi.org/10.3390/app9142843
Chicago/Turabian StyleDuthon, Pierre, Michèle Colomb, and Frédéric Bernardin. 2019. "Light Transmission in Fog: The Influence of Wavelength on the Extinction Coefficient" Applied Sciences 9, no. 14: 2843. https://doi.org/10.3390/app9142843
APA StyleDuthon, P., Colomb, M., & Bernardin, F. (2019). Light Transmission in Fog: The Influence of Wavelength on the Extinction Coefficient. Applied Sciences, 9(14), 2843. https://doi.org/10.3390/app9142843







