A Proposal for Evading the Measurement Uncertainty in Classical and Quantum Computing: Application to a Resonant Tunneling Diode and a Mach-Zehnder Interferometer
Abstract
1. Introduction
2. Quantum Uncertainty: The Problem and the Solution
2.1. The Problem
2.2. The Solution
- Condition 1: We enlarge the original quantum electron device in order to accommodate a large number of electrons simultaneously. Then, the many-particle wave function that defines this N electrons at time is:where the wave function is the single electron wave function that corresponds to the i-th electron prepared under the same conditions that we have used to prepare the wave function in the original quantum electron device.Strictly speaking, the condition is inaccessible in a practical scenario. We will see numerically in the following sections that a finite number of electrons is enough to drastically reduce the quantum noise. Identically, if the number of transport electrons in the original (unmodified) quantum electron device is already larger than one, the solution proposed here is still perfectly valid. See Appendix A for a generalization of the present protocol to more than one electron in the unmodified electron device. Finally, as can be seen in Equation (1), we assume a many-particle wave function of non-interacting electrons. This is obviously an approximation in realistic quantum devices since these electrons will suffer exchange and Coulomb interactions. In Appendix D, we test our protocol under the exchange symmetry for two electrons. Under the assumption of an initial negligible overlap of the wavepackets our protocol does not deviate from the actual result. These issues will be further elaborated in the practical implementation of this protocol in next two section.We mention that some (small) variation in the preparation of the state , ,… forming is allowed. For example, the time delay between the injection of different single electron wave packets can vary. Also the central position of the wave packets along the lateral dimension of the device can be different. Similarities between different wave packets have to be enough to justify that the probability distribution of the values of the current is identical for all single electron wave packets.
- Condition 2: We substitute the measuring apparatus associated with the single particle operator with a new measuring apparatus whose associated many-body operator is:where acts only on the quantum state and is the identity operator in the small Hilbert space of each degree of freedom. Notice the presence of the factor in the denominator of the operator .In next section, we will show the physical soundness of the many-particle operator in Equation (2) for typical semiconductor electron device technology.
3. Application to Classical Computing Device: Resonant Tunneling Diode
3.1. Implementation of Condition 1 and Condition 2
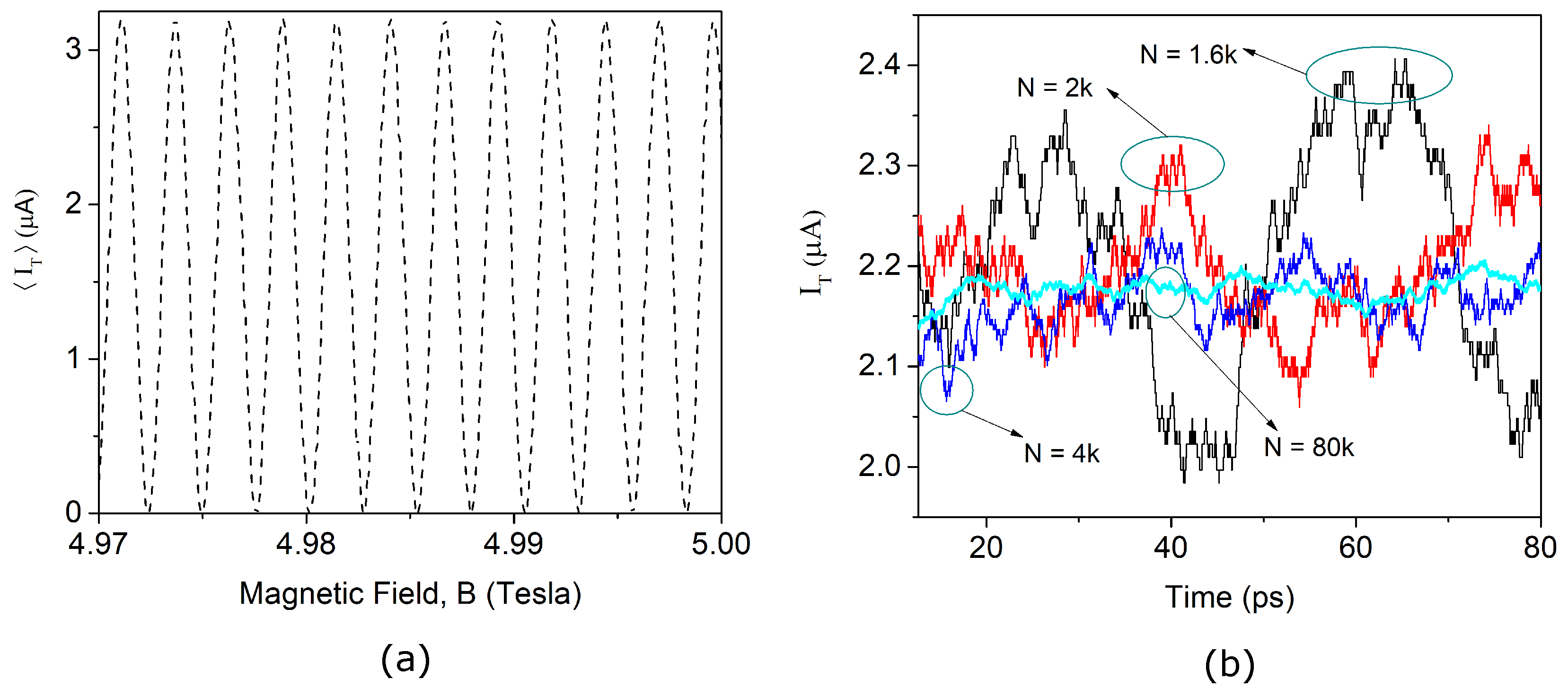
3.2. Numerical Results
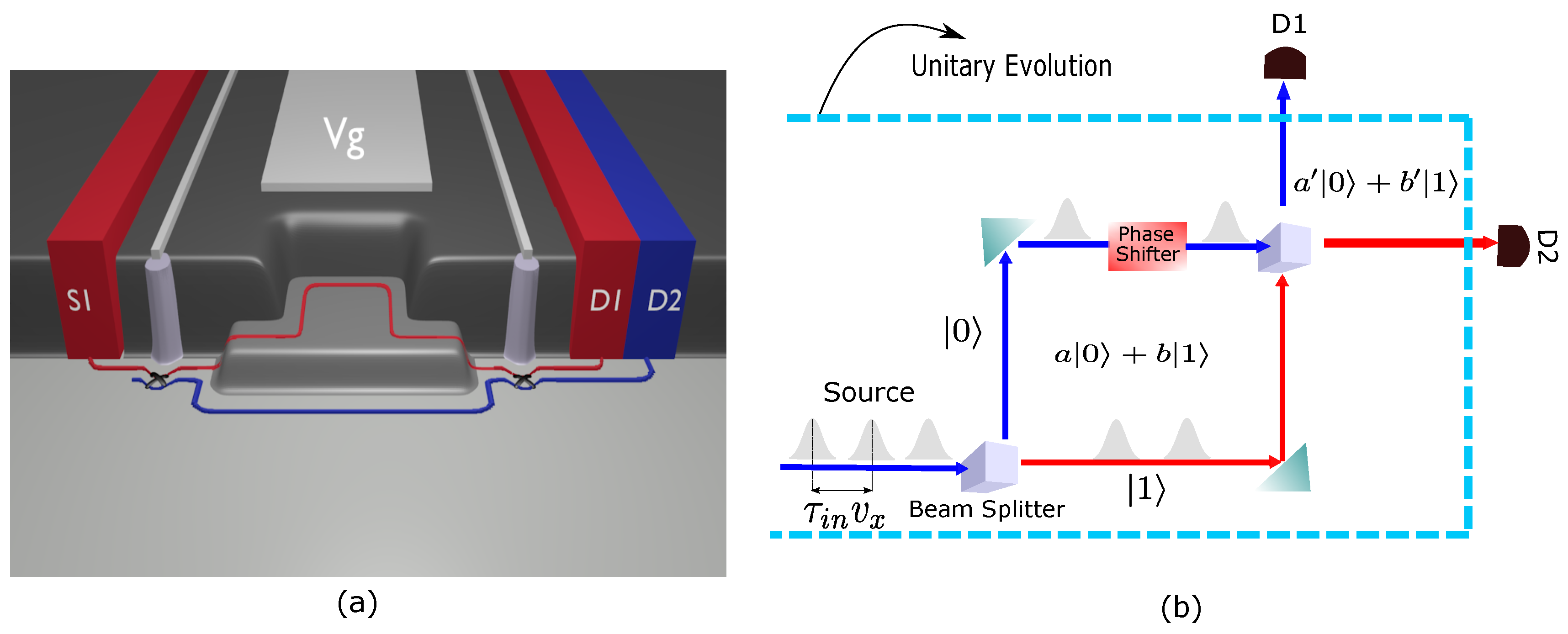
4. Application to Quantum Computing Devices: Mach-Zehnder Interferometer
4.1. Implementation of Condition 1 and Condition 2
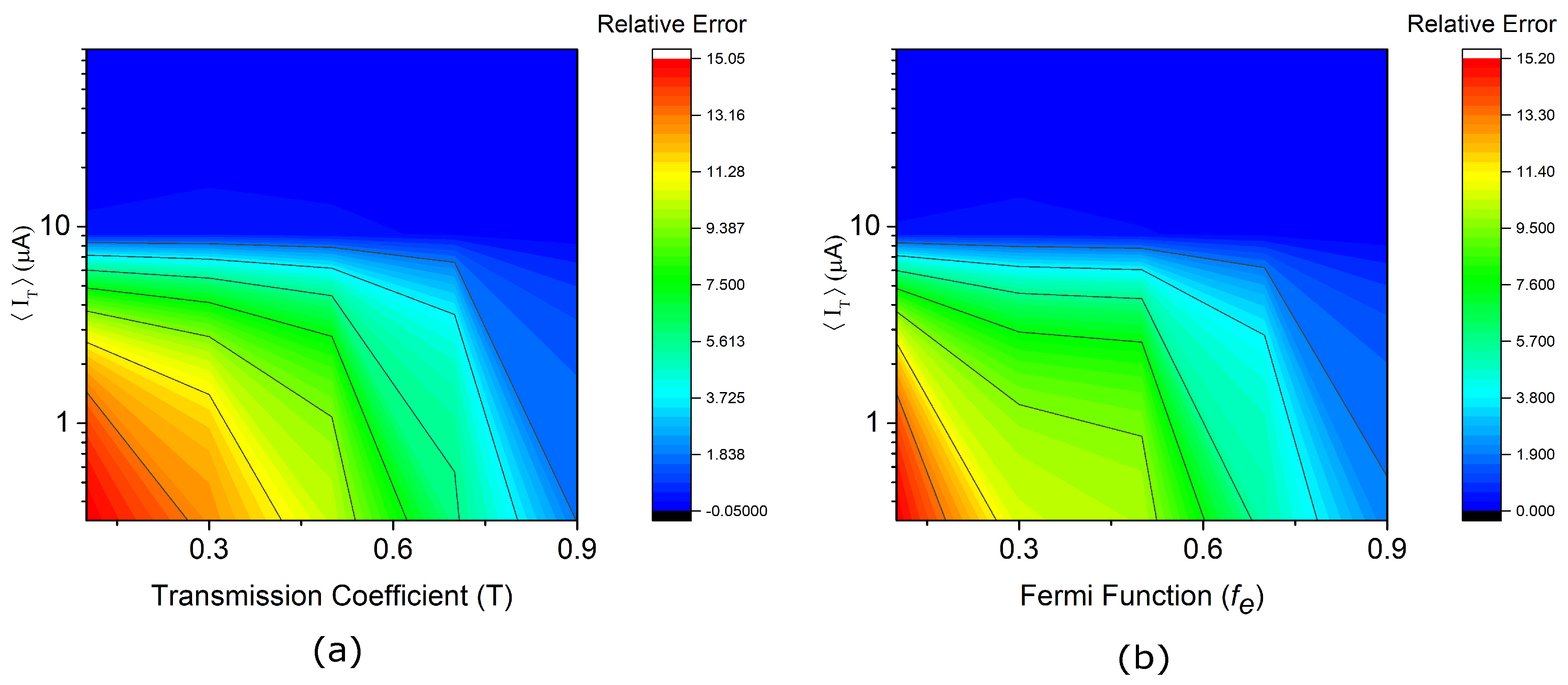
4.2. Numerical Results
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Appendix A. Generalization to an Unmodified Quantum Device with Many Electrons
Appendix B. The Injection Time
Appendix C. Description of the Current Signal and Condition 2
Appendix C.1. On the Assumption of a Large Lateral Area in the Active Region
Appendix C.2. On the Assumption of an Instantaneous Screening Time in the Metallic Contacts
Appendix D. Effects of Exchange Symmetry on the Total Current Many Body Operator
Appendix E. The Ontological Meaning of the Total Measured Current and the Classical Central Limit Theorem
References
- Le, H.Q.; Van Norstrand, J.A.; Thompto, B.W.; Moreira, J.E.; Nguyen, D.Q.; Hrusecky, D.; Kroener, M. IBM POWER9 processor core. IBM J. Res. Dev. 2018, 62, 2:1–2:12. [Google Scholar] [CrossRef]
- Cory, C.D.; Datta, S. Nanoscale transistors—Just around the gate? Science 2013, 341, 140–141. [Google Scholar]
- Nielsen, M.A.; Chuang, I.L. Quantum computation. In Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Boixo, S.; Isakov, S.V.; Smelyanskiy, V.N.; Babbush, R.; Ding, N.; Jiang, Z.; Neven, H. Characterizing quantum supremacy in near-term devices. Nat. Phys. 2018, 14, 595. [Google Scholar] [CrossRef]
- Classical and quantum computers are vying for superiority. Nature 2018, 564, 302. [CrossRef] [PubMed]
- Tang, A.E. Quantum-inspired classical algorithm for recommendation systems. arXiv 2018, arXiv:1807.04271. [Google Scholar]
- Deutsch, D. Uncertainty in quantum measurements. Phys. Rev. Lett. 1983, 50, 631. [Google Scholar] [CrossRef]
- Marian, D.; Zanghì, N.; Oriols, X. Weak values from displacement currents in multiterminal electron devices. Phys. Rev. Lett. 2016, 116, 110404. [Google Scholar] [CrossRef] [PubMed]
- Zhan, Z.; Kuang, X.; Colomés, E.; Pandey, D.; Yuam, S.; Oriols, X. Time-dependent quantum Monte Carlo simulation of electron devices with two-dimensional Dirac materials: A genuine terahertz signature for graphene. Phys. Rev. B 2019, 99, 155412. [Google Scholar] [CrossRef]
- Oriols, X.; Benseny, A. Conditions for the classicality of the center of mass of many-particle quantum states. New J. Phys. 2017, 19, 063031. [Google Scholar] [CrossRef]
- Lloyd, S.; Slotine, J.J.E. Quantum feedback with weak measurements. Phys. Rev. A 2000, 62, 012307. [Google Scholar] [CrossRef]
- Hou, Z.; Tang, J.F.; Shang, J.; Zhu, H.; Li, J.; Yuan, Y.; Wu, K.D.; Xiang, G.Y.; Li, C.F.; Guo, G.C. Deterministic realization of collective measurements via photonic quantum walks. Nat. Commun. 2018, 9, 1414. [Google Scholar] [CrossRef] [PubMed]
- Encomendero, J.; Faria, F.A.; Islam, S.M.; Protasenko, V.; Rouvimov, S.; Sensale-Rodriguez, B.; Xing, H.G. New tunneling features in polar III-nitride resonant tunneling diodes. Phys. Rev. X 2017, 7, 041017. [Google Scholar] [CrossRef]
- Gaskell, J.; Eaves, L.; Novoselov, K.S.; Mishchenko, A.; Geim, A.K.; Fromhold, T.M.; Greenaway, M.T. Graphene-hexagonal boron nitride resonant tunneling diodes as high-frequency oscillators. Appl. Phys. Lett. 2015, 107, 103105. [Google Scholar] [CrossRef]
- Avedillo, M.J.; Quintana, J.M.; Roldán, H.P. Increased Logic Functionality of Clocked Series-Connected RTDS. IEEE Trans. Nanotechnol. 2006, 5, 606–611. [Google Scholar] [CrossRef]
- Park, J.; Lee, J.; Yang, K. A 24-GHz Low-Power RTD-Based ON-OFF Keying Oscillator with an RTD Pair Configuration. IEEE Microwave Wirel. Comp. Lett. 2018, 28, 521–523. [Google Scholar] [CrossRef]
- Pandey, D.; Albareda, G.; Oriols, X. Measured and unmeasured properties of quantum systems. arXiv 2018, arXiv:1812.10257. [Google Scholar]
- Bertoni, A.; Bordone, P.; Brunetti, R.; Jacoboni, C.; Reggiani, S. Quantum Logic Gates based on Coherent Electron Transport in Quantum Wires. Phys. Rev. Lett. 2000, 84, 5912. [Google Scholar] [CrossRef] [PubMed]
- Buscemi, F.; Bordone, P.; Bertoni, A. Carrier-carrier entanglement and transport resonances in semiconductor quantum dots. Phys. Rev. B 2007, 76, 195317. [Google Scholar] [CrossRef]
- Buscemi, F.; Bordone, P.; Bertoni, A. Quantum teleportation of electrons in quantum wires with surface acoustic waves. Phys. Rev. B 2010, 81, 045312. [Google Scholar] [CrossRef]
- Lanting, T.; Przybysz, A.J.; Smirnov, A.Y.; Spedalieri, F.M.; Amin, M.H.; Berkley, A.J.; Dickson, N. Entanglement in a Quantum Annealing Processor. Phys. Rev. X 2014, 4, 021041. [Google Scholar] [CrossRef]
- Debnath, S.; Linke, N.M.; Figgatt, C.; Landsman, K.A.; Wright, K.; Monroe, C. Demonstration of a small programmable quantum computer with atomic qubits. Nature 2016, 536, 63–66. [Google Scholar] [CrossRef] [PubMed]
- Büttiker, M. Absence of backscattering in the quantum Hall effect in multiprobe conductors. Phys. Rev. B 1988, 38, 9375. [Google Scholar] [CrossRef]
- Roulleau, P.; Portier, F.; Roche, P.; Cavanna, A.; Faini, G.; Gennser, U.; Mailly, D. Direct Measurement of the Coherence Length of Edge States in the Integer Quantum Hall Regime. Phys. Rev. Lett. 2008, 100, 126802. [Google Scholar] [CrossRef] [PubMed]
- Venturelli, D.; Giovannetti, V.; Taddei, F.; Fazio, R.; Feinberg, D.; Usaj, G.; Balseiro, C.A. Edge channel mixing induced by potential steps in an integer quantum Hall system. Phys. Rev. B 2011, 83, 075315. [Google Scholar] [CrossRef]
- Beggi, A.; Bordone, P.; Buscemi, F.; Bertoni, A. Time-dependent simulation and analytical modelling of electronic Mach–Zehnder interferometry with edge-states wave packets. J. Phys.: Condens. Matter 2015, 27, 475301. [Google Scholar] [CrossRef] [PubMed]
- Deviatov, E.V.; Lorke, A. Experimental realization of a Fabry-Perot-type interferometer by copropagating edge states in the quantum Hall regime. Phys. Rev. B 2008, 77, 161302. [Google Scholar] [CrossRef]
- Neder, I.; Ofek, N.; Chung, Y.; Heiblum, M.; Mahalu, D.; Umansky, V. Interference between two indistinguishable electrons from independent sources. Nature 2007, 448, 333–337. [Google Scholar] [CrossRef]
- Bocquillon, E.; Freulon, V.; Berroir, J.M.; Degiovanni, P.; Plaçais, B.; Cavanna, A.; Jin, Y.; Fève, G. Coherence and Indistinguishability of Single Electrons Emitted by Independent Sources. Science 2013, 339, 1054–1057. [Google Scholar] [CrossRef]
- Weisz, E.; Choi, H.K.; Sivan, I.; Heiblum, M.; Gefen, Y.; Mahalu, D.; Umansky, V. An electronic quantum eraser. Science 2014, 3444, 1363–1366. [Google Scholar] [CrossRef]
- Giovannetti, V.; Taddei, F.; Frustaglia, D.; Fazio, R. Multichannel architecture for electronic quantum Hall interferometry. Phys. Rev. B 2008, 77, 155320. [Google Scholar] [CrossRef]
- Karmakar, B.; Venturelli, D.; Chirolli, L.; Giovannetti, V.; Fazio, R.; Roddaro, S.; Pellegrini, V. Nanoscale Mach-Zehnder interferometer with spin-resolved quantum Hall edge states. Phys. Rev. B 2015, 92, 195303. [Google Scholar] [CrossRef]
- Bellentani, L.; Beggi, A.; Bordone, P.; Bertoni, A. Dynamics of copropagating edge states in a multichannel Mach-Zender interferometer. J. Phys.: Conf. Ser. 2017, 906, 012027. [Google Scholar] [CrossRef]
- Bellentani, L.; Beggi, A.; Bordone, P.; Bertoni, A. Dynamics and Hall-edge-state mixing of localized electrons in a two-channel Mach-Zehnder interferometer. Phys. Rev. B 2018, 97, 205419. [Google Scholar] [CrossRef]
- Ji, Y.; Chung, Y.; Sprinzak, D.; Heiblum, M.; Mahalu, D.; Shtrikman, H. An electronic Mach–Zehnder interferometer. Nature 2003, 422, 415. [Google Scholar] [CrossRef] [PubMed]
- Bird, J.P.; Ishibashi, K.; Stopa, M.; Aoyagi, Y.; Sugano, T. Coulomb blockade of the Aharonov-Bohm effect in GaAs/AlxGa1 − x As quantum dots. Phys. Rev. B 1994, 50, 14983–14990. [Google Scholar] [CrossRef]
- Marian, D.; Colomés, E.; Oriols, X. Time-dependent exchange and tunneling: Detection at the same place of two electrons emitted simultaneously from different sources. J. Phys.: Condens. Matter 2015, 27, 245302. [Google Scholar] [CrossRef]
- Bellentani, L.; Bordone, P.; Oriols, X.; Bertoni, A. Coulomb and exchange interaction effects on the exact two-electron dynamics in the Hong-Ou-Mandel interferometer based on Hall edge states. arXiv 2019, arXiv:1903.02581. [Google Scholar]
- Oriols, X. Non-universal conductance quantization for long quantum wires: The role of the exchange interaction. Nanotechnology 2004, 15, S167. [Google Scholar] [CrossRef]
- Oriols, X.; Mompart, J. Applied Bohmian Mechanics: From Nanoscale Systems to Cosmology; CRC Press: Boca Raton, FL, USA, 2012. [Google Scholar]
- Albareda, G.; Traversa, F.L.; Benali, A.; Oriols, X. Computation of quantum electrical currents through the Ramo–Shockley–Pellegrini theorem with trajectories. Fluctuat. Noise Lett. 2012, 11, 1242008. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pandey, D.; Bellentani, L.; Villani, M.; Albareda, G.; Bordone, P.; Bertoni, A.; Oriols, X. A Proposal for Evading the Measurement Uncertainty in Classical and Quantum Computing: Application to a Resonant Tunneling Diode and a Mach-Zehnder Interferometer. Appl. Sci. 2019, 9, 2300. https://doi.org/10.3390/app9112300
Pandey D, Bellentani L, Villani M, Albareda G, Bordone P, Bertoni A, Oriols X. A Proposal for Evading the Measurement Uncertainty in Classical and Quantum Computing: Application to a Resonant Tunneling Diode and a Mach-Zehnder Interferometer. Applied Sciences. 2019; 9(11):2300. https://doi.org/10.3390/app9112300
Chicago/Turabian StylePandey, Devashish, Laura Bellentani, Matteo Villani, Guillermo Albareda, Paolo Bordone, Andrea Bertoni, and Xavier Oriols. 2019. "A Proposal for Evading the Measurement Uncertainty in Classical and Quantum Computing: Application to a Resonant Tunneling Diode and a Mach-Zehnder Interferometer" Applied Sciences 9, no. 11: 2300. https://doi.org/10.3390/app9112300
APA StylePandey, D., Bellentani, L., Villani, M., Albareda, G., Bordone, P., Bertoni, A., & Oriols, X. (2019). A Proposal for Evading the Measurement Uncertainty in Classical and Quantum Computing: Application to a Resonant Tunneling Diode and a Mach-Zehnder Interferometer. Applied Sciences, 9(11), 2300. https://doi.org/10.3390/app9112300





