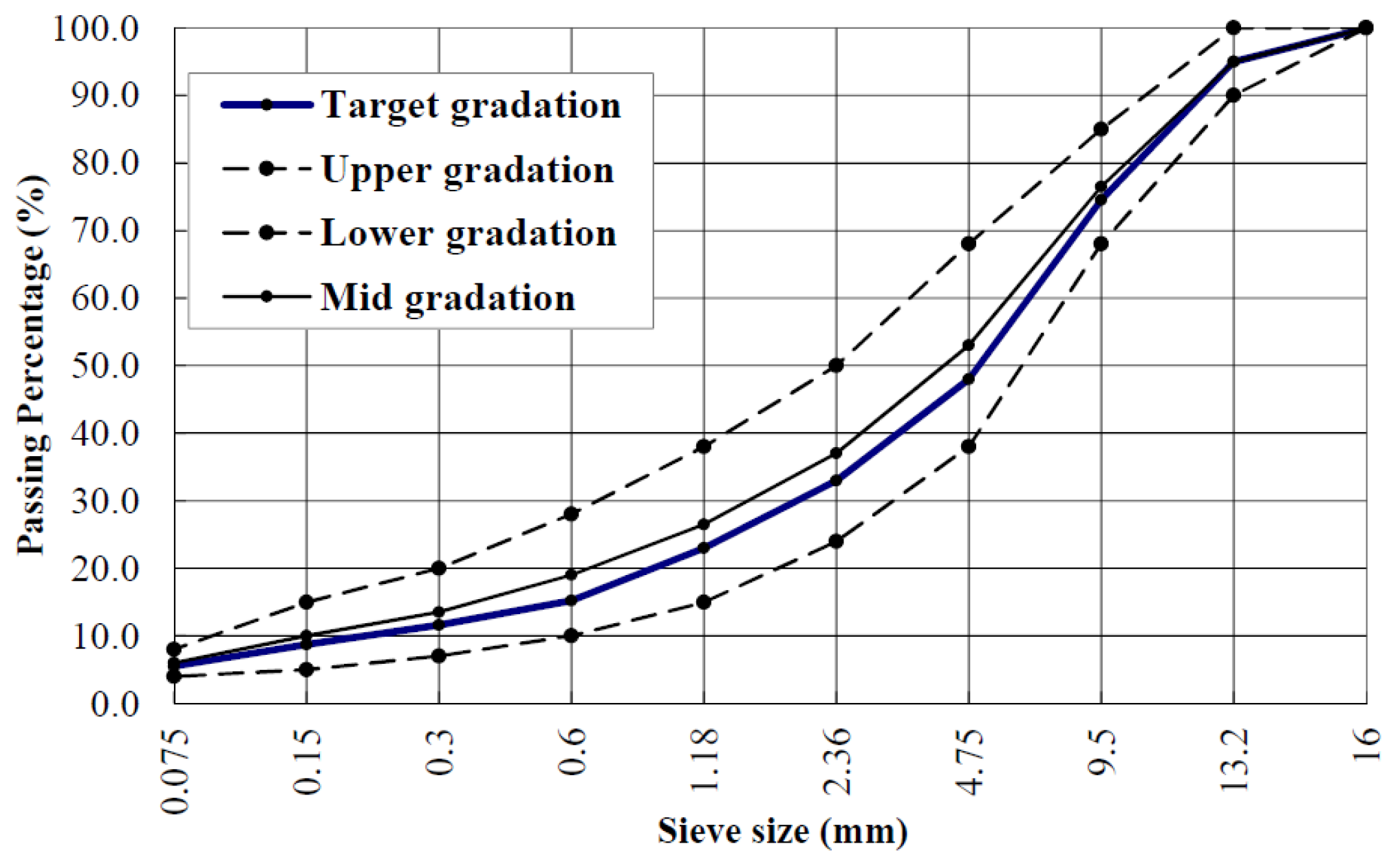
3.1. The Establishment of the Decay Model for Moduli
The conventional
S-N fatigue Equation is widely used to analyze the fatigue performance of asphalt mixtures [
26,
27]. Chaboche [
28] defined the traditional
S-N fatigue Equation as:
or
where,
Nf is fatigue life,
t is stress ratio,
σ is stress level.
K, and
n are the material parameters of asphalt mixtures.
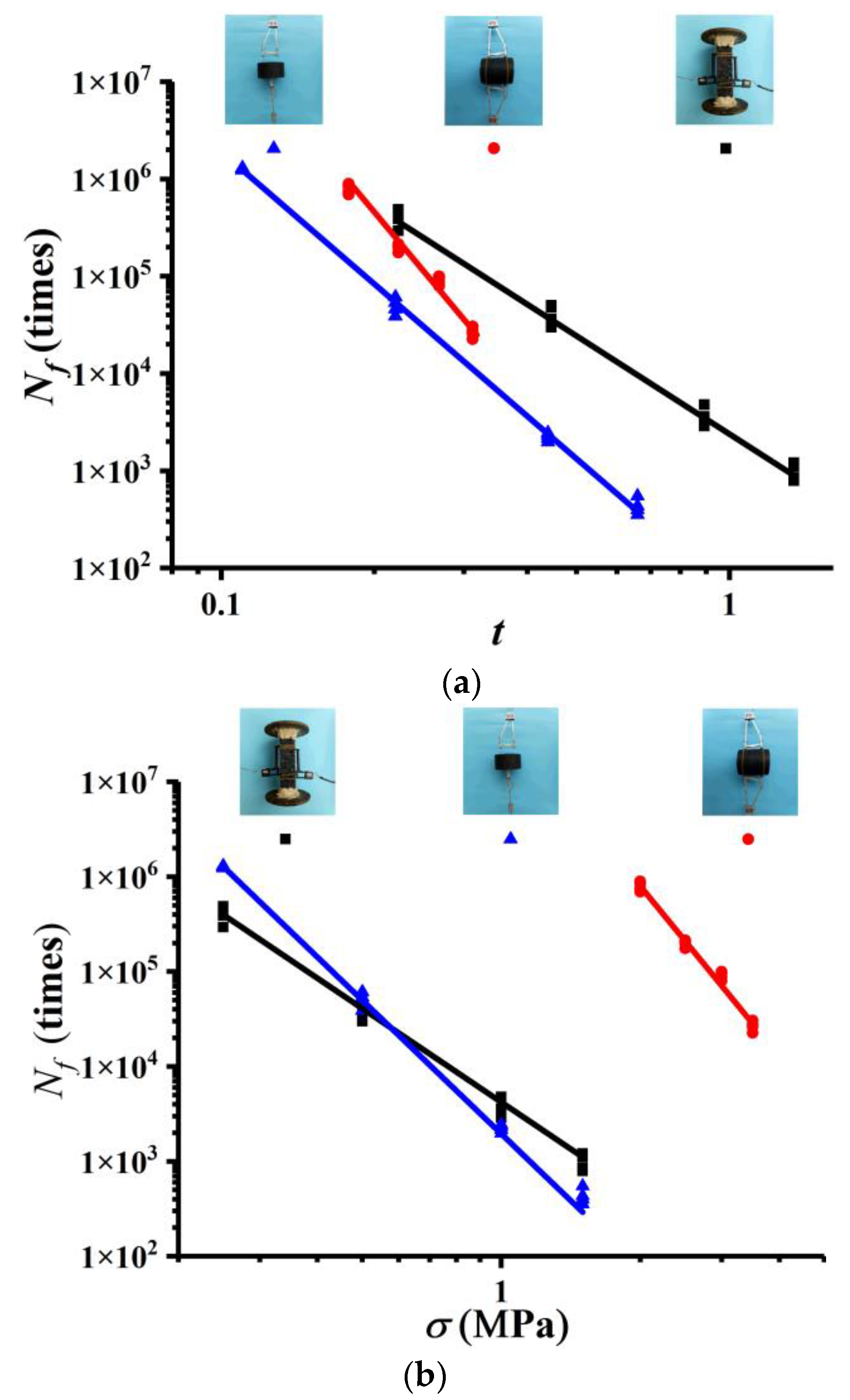
According to Equations (1) and (2), the fatigue curves characterized by stress ratios and stress levels were shown in
Figure 4a,b, respectively.
From
Figure 4, it can be observed that the
S-N fatigue curves of asphalt mixtures show great difference under different stress conditions. It is difficult to evaluate the fatigue performance of asphalt mixtures in different test methods [
7]. Given that, in this paper, the nonlinear fatigue damage model was implemented to simulate the moduli decay patterns. Defining the moduli as the damage variable, the damage model based on the moduli decay was established.
The damage variable could be expressed, as shown in Equation (3).
where,
D(
N) is damage variable,
E(
N) is modulus in loading cycle
Nf the specimen, and
E0 is the initial value of modulus.
Chaboche [
28] established another fatigue damage model. As shown in Equation (4).
where,
Nf is the fatigue life,
N is the loading cycles, and
α and
γ are the material parameters related to the stress.
Based on Equations (3) and (4), in this paper, Equation (5) is deduced as the decay Equation of the moduli for asphalt mixtures.
The fitting parameters are replaced by m, n, where, , .
Equation (5) can be simplified as:
3.4. Analysis of the Fitting Results of Fatigue Tests under Different Stress Levels
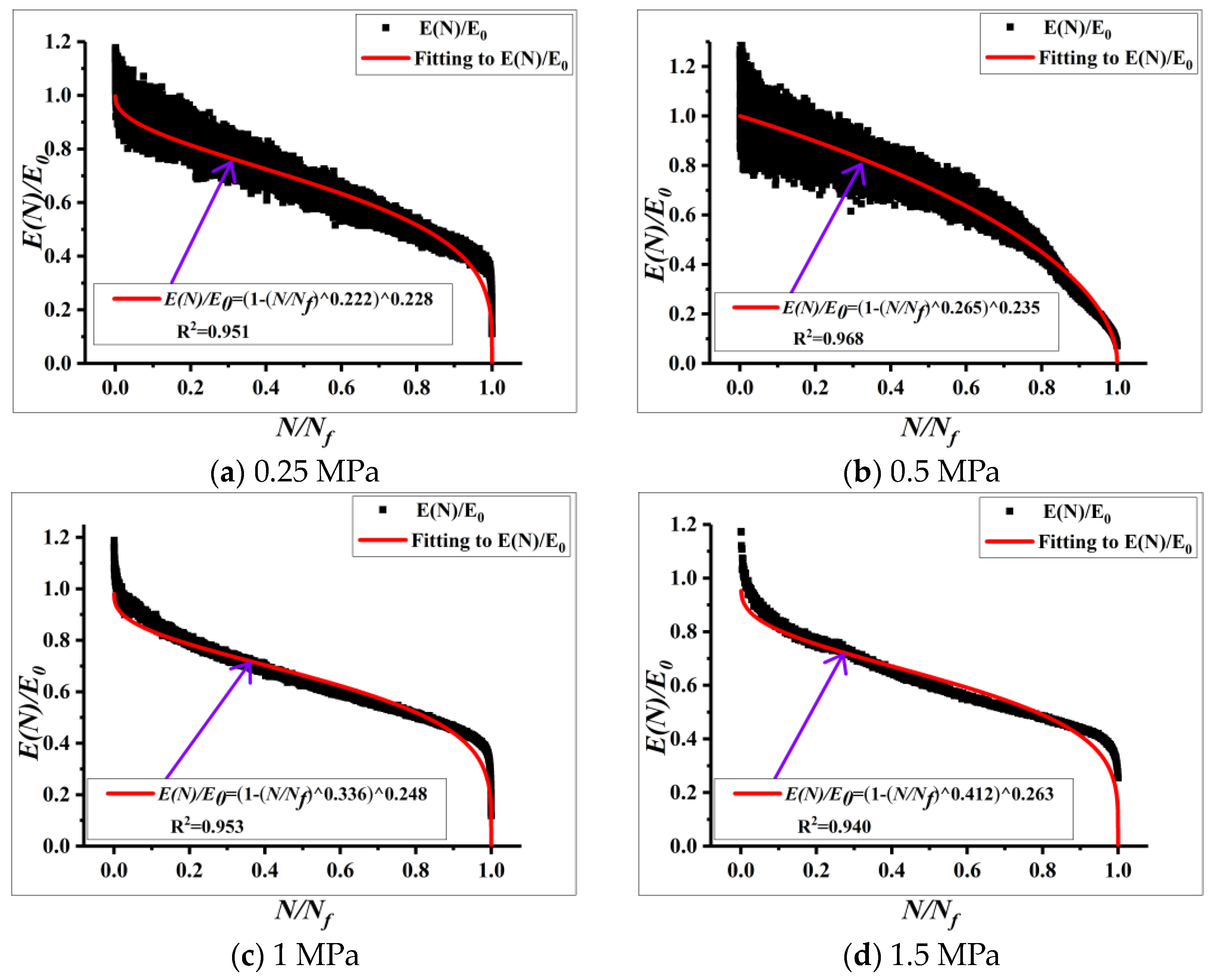
In order to compare the moduli decay pattern under the same stress state, the tensile moduli and compression moduli were compared, respectively. Real-time ratio
E(
N)/
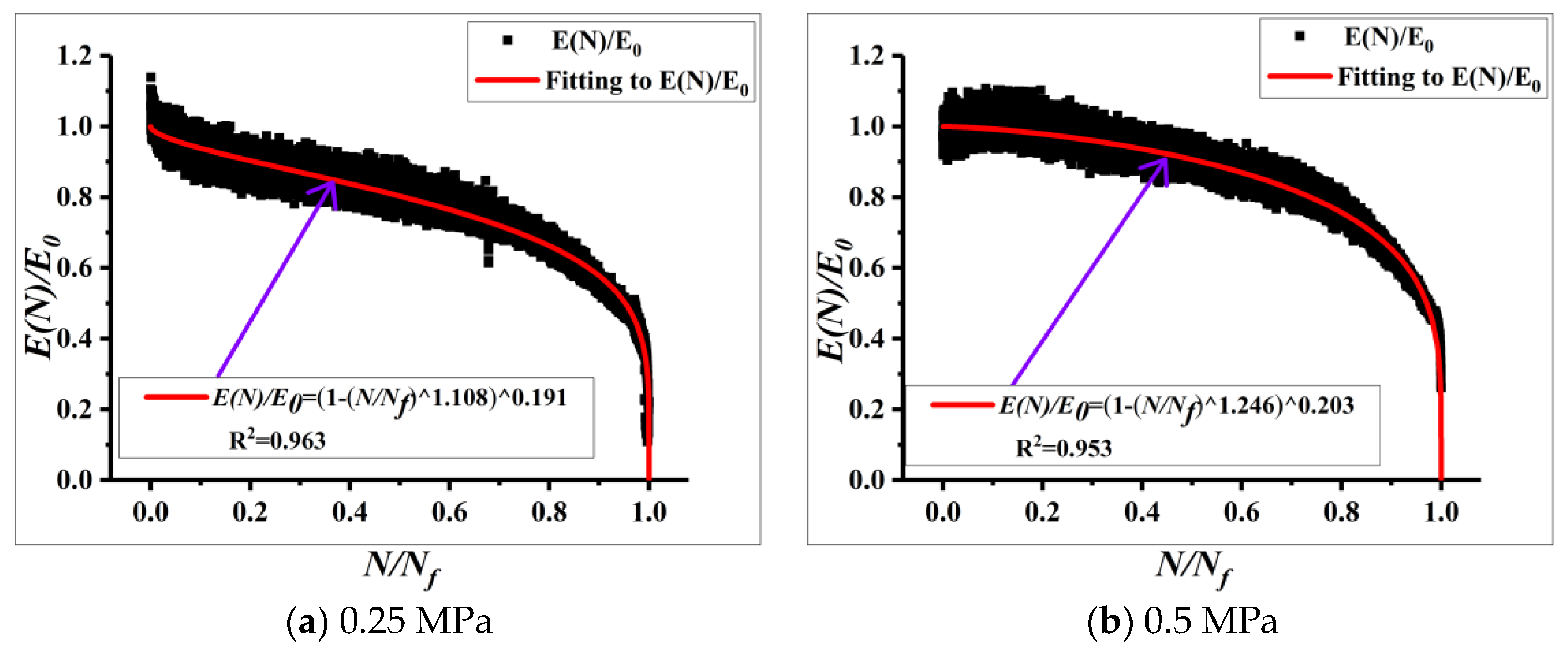
E0 for tensile moduli, as measured by direct tensile test and indirect tensile tests, was fitted with the cycle ratio by Equation (6). The fitting results of tensile moduli from the direct tensile and the indirect tensile fatigue tests in different stress levels are shown in
Figure 5 and
Figure 6, respectively.
It can be noticed from
Figure 5 and
Figure 6 that the decay patterns of tensile moduli that were obtained from these two test methods are similar. There are three stages: migration stage, steady stage, and destructive stage, and the whole process is nonlinear.
However, the decay rates of different stress state are different, which are caused by different fatigue resistance of asphalt. In the direct tensile test, the specimen is within a uniform tensile condition. The main factors determining its fatigue properties are the cohesion of the asphalt mortar (the adhesion of the aggregate with the asphalt and its internal friction based on the aggregate gradation). The effect of intrusion between aggregates is relatively weak [
30]. During the indirect tensile tests, the transverse tensile fatigue properties mainly depend on the adhesion (between asphalt mortar and aggregates) and the internal frictional resistance.
Figure 5 and
Figure 6 reflect the decay pattern of different stress levels under different stress states. In order to compare the decay pattern more obviously, this paper compared the decay pattern of tensile moduli under the same stress level. The parameters of the fitted curves for tensile stress were shown in the
Table 10:
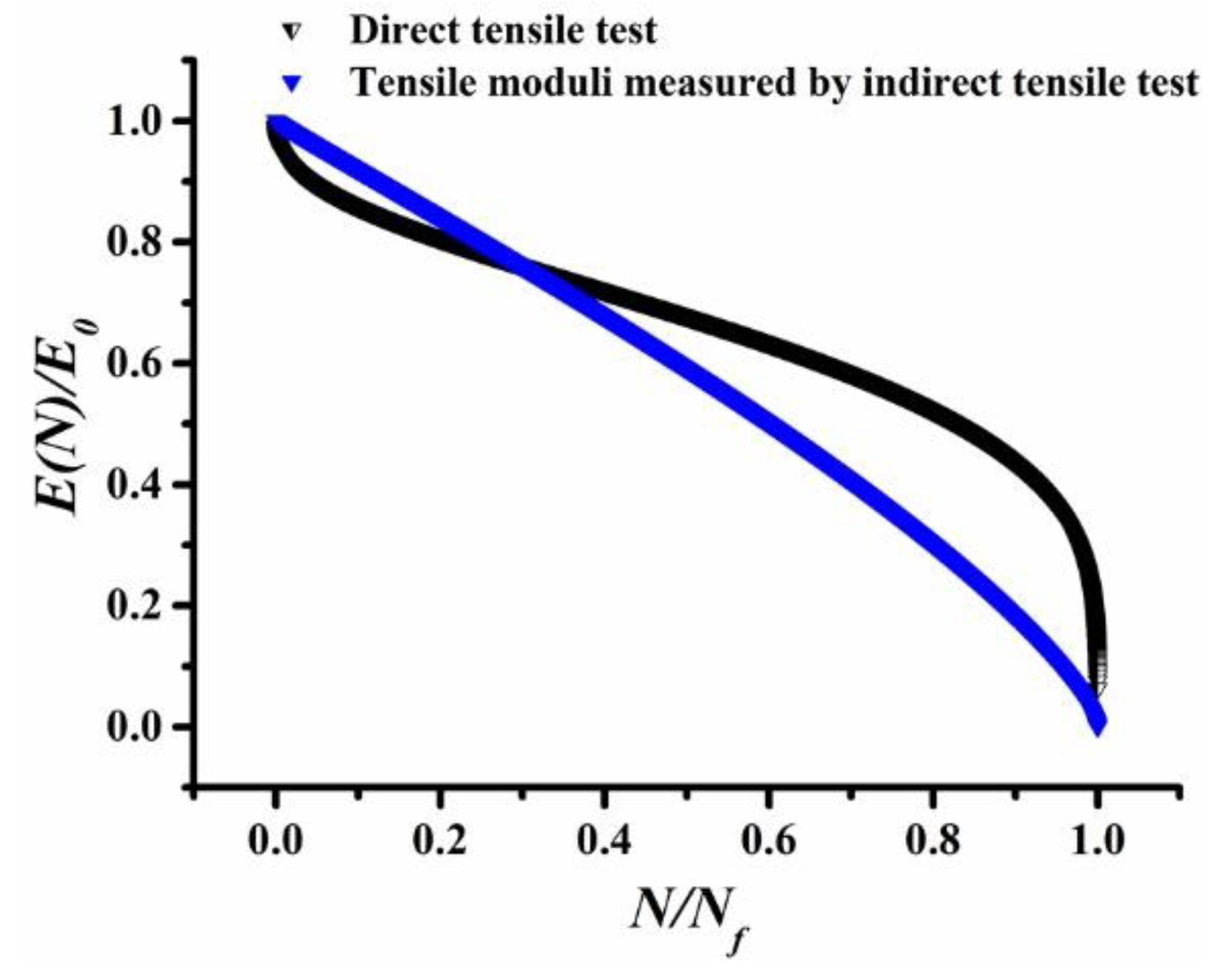
The tensile moduli measured by indirect tensile test and direct tensile under the same stress level 1 MPa were compared. As shown in
Figure 7:
In order to compare the decay pattern more clearly, the decay Equation is simplified.
Equation (6) is simplify to
, then,
where,
is the ratio of the modulus of the material to the initial modulus of the undamaged state after loading the specimen to N,
is the ratio of the loading cycles to the fatigue life,
m and
n are the material parameters related to the stress,
is the first derivative of
, and
is the two derivative of
.
While substituting parameters under the stress level of 1 MPa into Equation (8), the inflection point can be calculated, then the tangent line at the inflection point position can be adopted to compare the tangent slope. The result is as follows:
It can be noticed from
Figure 7 and
Table 11 that the decay patterns of tensile moduli, as measured by the indirect tensile test and the direct tensile test are nonlinear. The moduli parameters are different. The inflection point of direct tensile test is 0.381. The decay rate of direct tensile moduli decreases gradually before the inflection, while it increases after the inflection point. The inflection point of tensile moduli, as measured by the indirect tensile test is 0.114. In addition, the decay rate shows an increasing trend. There are three stages of migration stage, steady stage and destructive stage in the decay curves of the direct tensile tests and the indirect tensile tests. The decay rate of the direct tensile moduli is more quickly than that of the indirect tensile moduli during the migration state, but it is contrary during the steady stage. In addition, the decay rate of the direct tensile moduli is quicker than that of the indirect tensile moduli at the destructive stage.
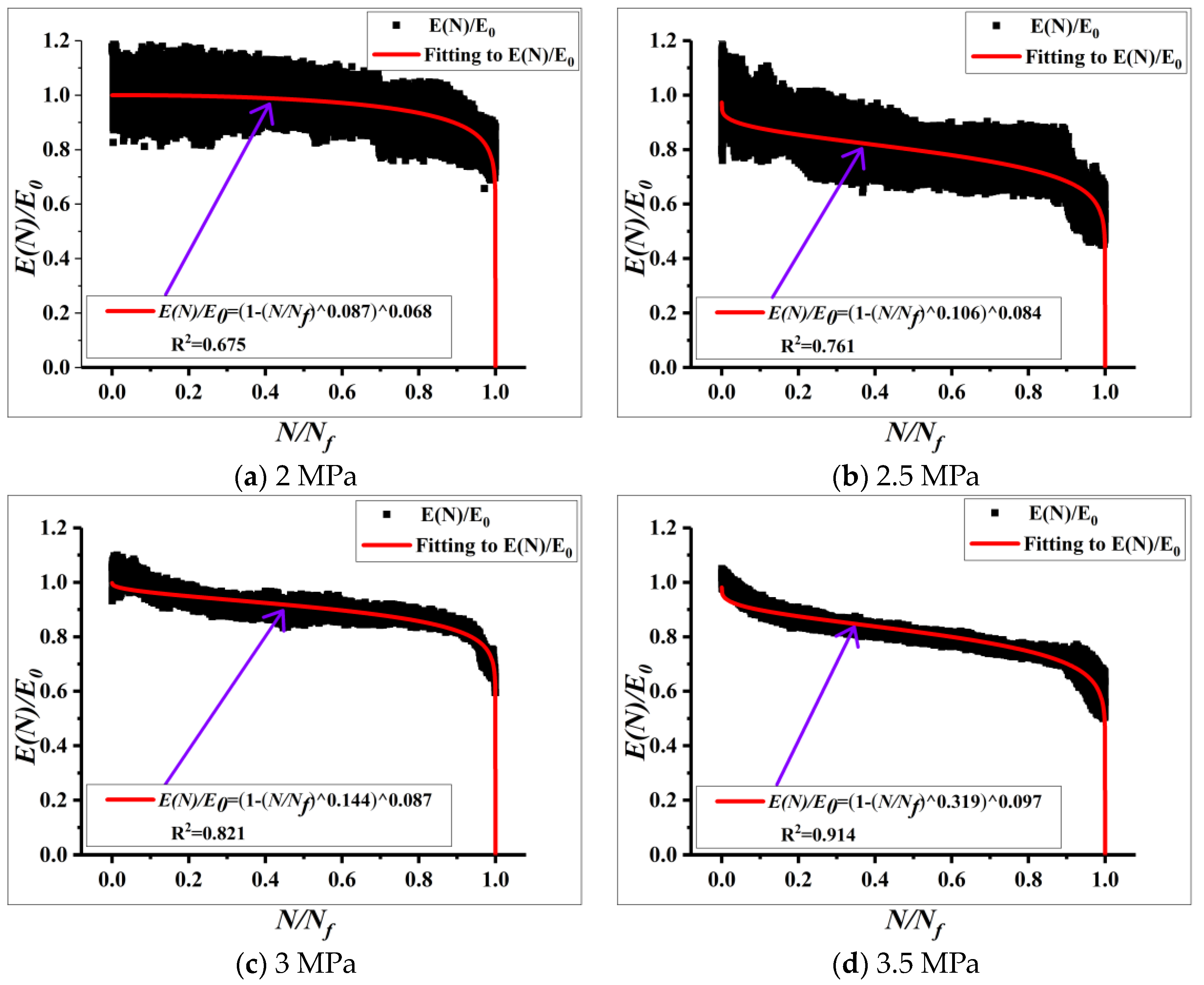
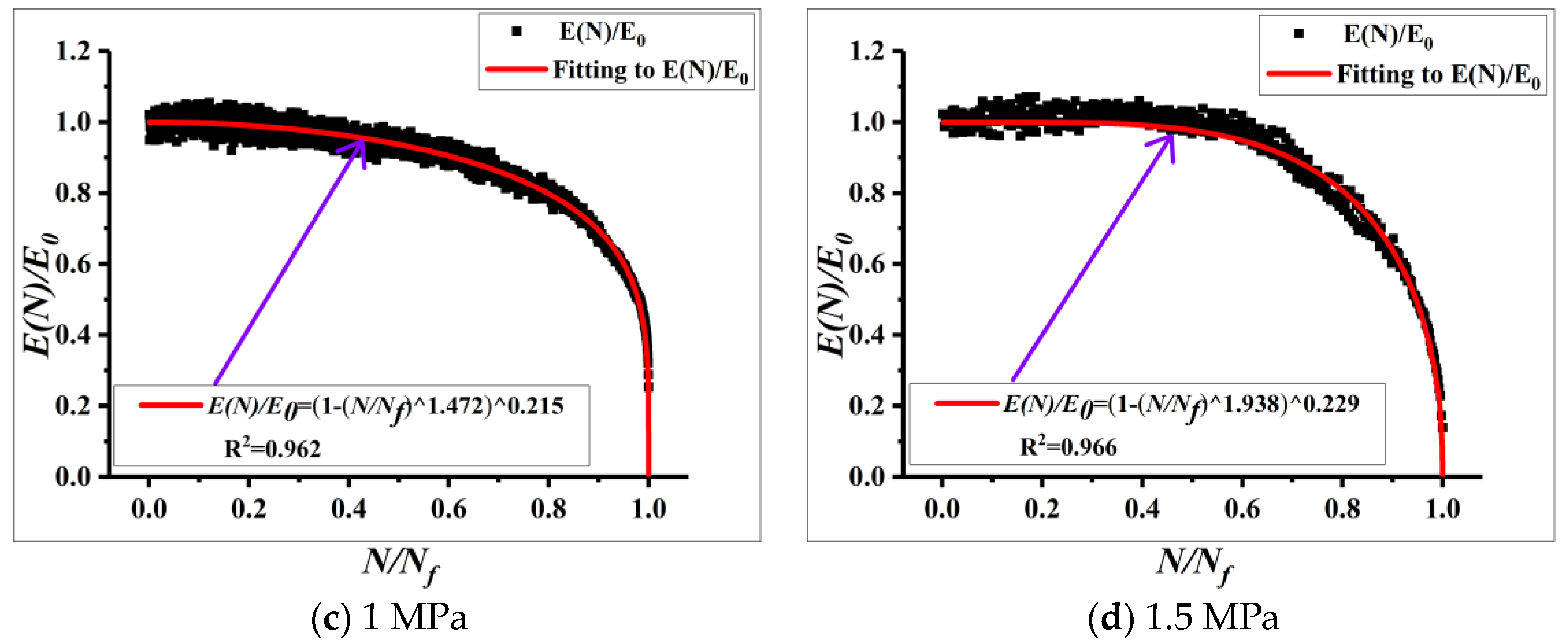
Similarly, the decay patterns of the compression moduli were fitted as shown in
Figure 8 and
Figure 9, respectively.
From
Figure 8 and
Figure 9, it can be observed that the decay patterns of compression moduli also exist three stages of migration stage, steady stage, and destructive stage, and the patterns are nonlinear. But, the decay rates are different under different stress state.
For uniaxial compression test, the specimen is in a compression stress state. The skeleton structure, composition form, and friction coefficient of aggregates determine the inter-particle frictional resistance. The main factors determining its fatigue properties are the cohesion of the aggregate particles [
31]. In the indirect tensile test, fatigue properties mainly depend on the interlocking effect of aggregate when the specimens are under the compression state [
32]. In summary, when the materials are in a different stress state, the factors that determine the fatigue properties are different.
In order to compare the decay pattern more obviously, this paper compared the decay pattern of compression moduli under same stress level. The parameters of the fitted curves for different stress levels are shown in
Table 12:
It is difficult to compare the moduli decay patterns. In this paper, the parameters
m and
n of compression moduli were fitted with stress levels. The fitting results were shown in Equations (9) and (10).
As there are four stress level (0.25, 0.5, 1, 1.5) of compression moduli, as measured by the indirect tensile test. Taking
σ = 1 MPa into Equations (9) and (10), respectively, then the parameters
m and
n of uniaxial compression moduli under 1 MPa can be obtained, as shown in
Table 13.
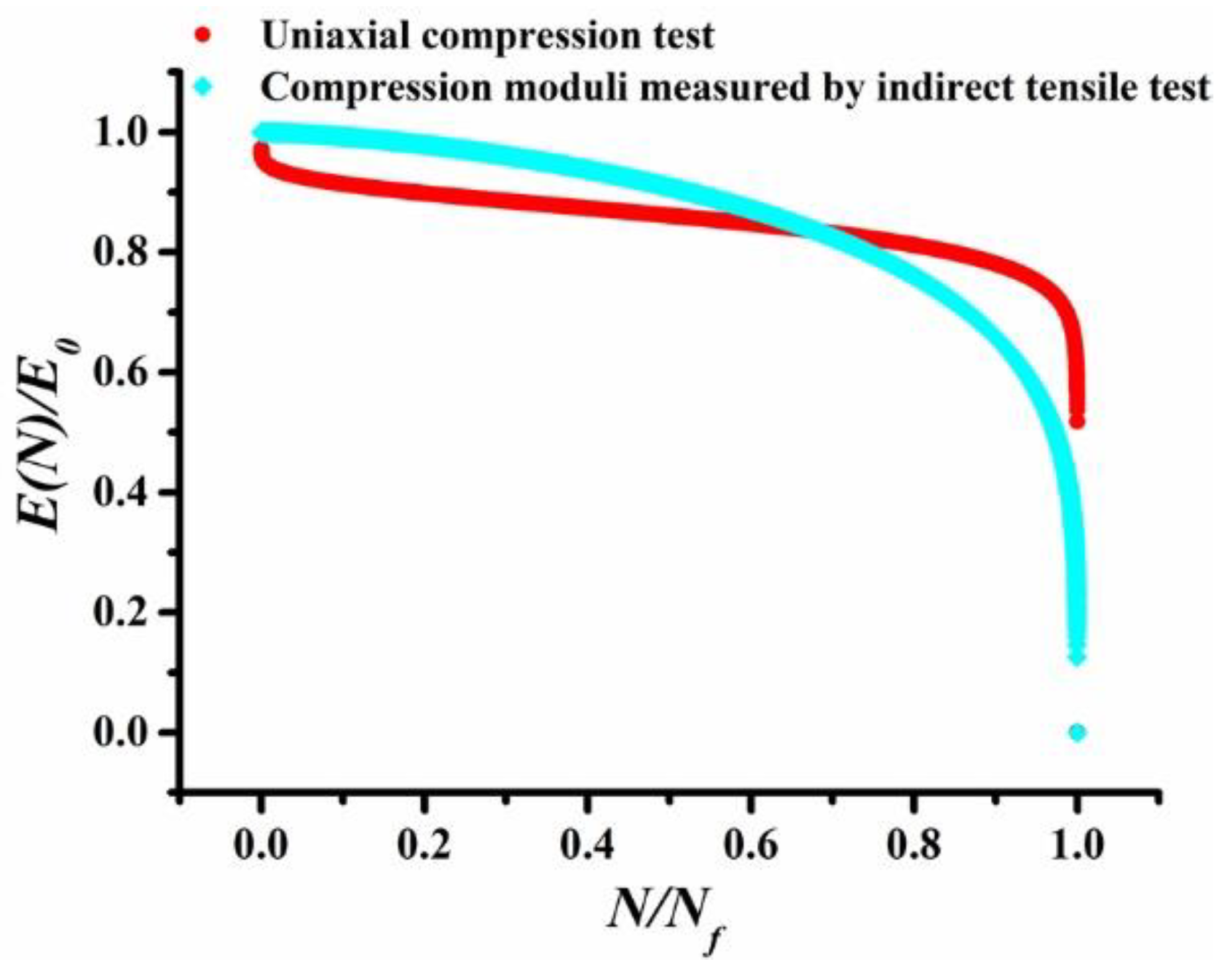
The compression moduli measured by indirect tensile test and uniaxial compression moduli under 1 MPa stress level were compared, as shown in
Figure 10. The decay patterns of the two compression moduli are experienced in three stages: migration stage, steady stage, and destructive stage, and the whole process is nonlinear.
In order to compare the decay rate, substituting the parameters in
Table 13 into Equation (8), the inflection point of the decay curve can be calculated. Then, the tangent line at the inflection point position can be implemented to compare the tangent slope. The result is as follows:
From
Figure 10 and
Table 14, it can be notified that the compression moduli decay pattern of uniaxial compression and indirect tensile similarly exist three stages of migration stage, steady stage, and destructive stage, and the patterns are nonlinear, too. The inflection point of uniaxial compression moduli is 0.370. The decay rate decreases before the inflection point, while it increases after the inflection point. There is no inflection point of indirect tensile moduli. In order to compare the decay rate on same position, the tangency point of indirect test is also taken as 0.37. It can be observed that the tangent slope of indirect test is larger than the uniaxial compression test on tangency point position. During the migration stage, the decay rate of uniaxial compression is faster than that of the indirect tensile. During the steady stage, the decay rate of uniaxial compression is slower than that of the indirect tensile. The decay rate of uniaxial compression is also faster than that of the indirect tensile during the destructive stage.
As the stress level of tensile moduli and compression moduli all is 1 MPa, the decay pattern of tensile moduli and compression moduli were compared simultaneously. The values of
m and
n in
Table 10 and
Table 13 were substituted into Equation (6). Then, the fatigue moduli decay patterns were shown in
Figure 11.
From
Figure 11, it can be observed that the fatigue moduli decay curves exist in the three stages of migration stage, steady stage, and destructive stage. The fatigue damage characteristics of asphalt mixtures are non-linear.
However, on one hand, the decay curves of fatigue moduli under different stress levels are quite different. In one-dimensional stress states, the tensile moduli and compression moduli are also quite different. In two-dimensional stress states, the difference is obvious between the compression moduli and tensile moduli, which were both obtained from the indirect tensile test. On the other hand, the direct tensile moduli are similar to the tensile moduli obtained from the indirect tensile test. The compression moduli of uniaxial compression test are similar to the compression moduli that were obtained from the indirect tensile test.
In addition, it can be found from
Table 11 and
Table 14 that the inflection point of tensile moduli measured by the indirect tensile is the smallest and that of the direct tensile are the maximum. At the tangent point, the uniaxial compression has the lowest moduli decay slope and the tensile moduli, as measured by the indirect tensile test, has the largest moduli decay slope. It can be concluded that the decay rate of the tensile moduli measured by the indirect tensile test is faster than the uniaxial compression moduli at the tangent point during the course of the fatigue tests.
It also can be observed that the position of the decay curve for the compression moduli is higher than that of the tensile moduli at the same cycle ratio state. It can be concluded that the decay rate of the tensile moduli is faster than that of the compression moduli during the course of the fatigue tests, which indicates that the tensile failure is the main reason of the fatigue damage for asphalt mixture.