1. Introduction
The exoskeleton is a human-robot interaction system that enhances the operator strength in various environments. It has been widely used in rehabilitation, medical, haptic interaction and power-assisted fields. The power-assisted upper-limb exoskeleton acts as a power amplifier to assist the user in performing tasks that are impossible or difficult to accomplish on human power alone, which is mainly used in rehabilitation and material handling and other fields. In industrial applications, the movement of the upper-limb exoskeleton used for material handling is in front of the body, and the weight of load is heavy [
1]. Therefore, it requires a light structure and stronger power than those of the rehabilitation exoskeletons. The existing exoskeleton with anthropomorphic structure can complete material handling [
2,
3,
4,
5,
6]. However, it has many joints, corresponding drivers and other components; thus, the exoskeleton has a large volume and a complex structure, and its own weight is relatively heavy. Hence, in industrial applications, it is necessary to ensure that it has a heavy load capacity and light self-weight, which is difficult for structural design.
Professor Amami Kanou of the University of Tsukuba in Japan developed a HAL-5 exoskeleton robot that weighted 21-kg [
7,
8]. This robot can help rescuers, porters and other physically demanding staff and can also help disabled people and elderly people take care of themselves. Galina Ivanova and Sergey Bulavintsev of Korea University of Technology and Education developed a 7-DOF upper-limb exoskeleton that can assist the human shoulder and elbow joints to complete daily activities [
9]. Joel C. Perry and Jacob Rosen of the University of Washington designed an exoskeleton called CADEN-7 with seven active DOFs [
10,
11,
12]. It can imitate the actions of 95% of healthy arms and is mainly used for rehabilitation, virtual reality simulation and power assistance. Professor Yamamoto of the Kanagawa Institute of Technology in Japan developed a wearable exoskeleton [
13,
14]. It can increase a person’s power by 0.5–1 times and provides help for nurses to help them move patients. R.A.R.C. Gopura and Kazuo Kiguchi of the University of Saga in Japan developed a 6-DOF upper extremity exoskeleton called SUEFUL-6, which is used primarily to assist with the daily activities of the upper-limbs [
15]. F. Martinez and I. Retolaza in Spain designed the 5-DOF upper-limb exoskeleton IKO, which is mainly used to provide help in performing daily activities [
16]. Wenbin Chen and Caihua Xiong of Huazhong University of Science and Technology in China developed a 10-DOF upper-limb exoskeleton prototype, which is mainly used to assist the body in daily activities. The shoulder joint contains 6-DOF, and the elbow and wrist joints each contain 4-DOF [
17]. F. Xiao et al. of Hefei University of Technology designed a 6-DOF cable-driven upper limb exoskeleton (CABexo) based on epicyclic gear trains. This exoskeleton has a parallel mechanical structure to the traditional serial structure, but is stiffer and has a stronger carrying capacity. Comparisons between CABexo and some of the existing exoskeleton systems show that it is able to meet the movement needs of most disabled and elderly individuals [
18]. These power-assisted exoskeletons with anthropomorphic structures fit well with the human arm in the course of work and can provide help to a user in daily life. Although they are lighter and less bulky, they have less joint torque and limited load capacity and cannot be used in an industrial setting.
The Raytheon Company developed the XOS2 exoskeleton; the upper-limb portion has 5-DOF, including 3-DOF shoulders and 2-DOF elbows. The exoskeleton can perform lateral-lifting, raising-up, pull down and other actions. It can amplify user strength and endurance and allow them, for example, to lift 200-lb loads repeatedly without tiring [
2,
3]. Massimo Bergamasco of the Scuola Superiore Sant’Anna in Italy developed a type of whole-body exoskeleton called the Body Extender system with a weight of 160-kg [
4,
5,
6]. The upper-limb of the exoskeleton has 5-DOF (including a crawl DOF). It can amplify the power of the human body 3–20 times, and each arm can lift a 50-kg load. These power-assisted exoskeletons with anthropomorphic structures have large joint torque and heavy load capacity. However, due to their complex structure and heavy weight, they are not suitable for industrial applications.
Lee et al. [
1] of the Hanyang University developed an upper-limb exoskeleton for material handling, which is called the Hanyang University EXoskeleton Assistive Robot (HEXAR). It has 3-DOF, including 2-DOF shoulders and 1-DOF elbow, and is actuated by motors. The exoskeleton is used to handling heavy loads in industrial settings by the front side of the operator body.
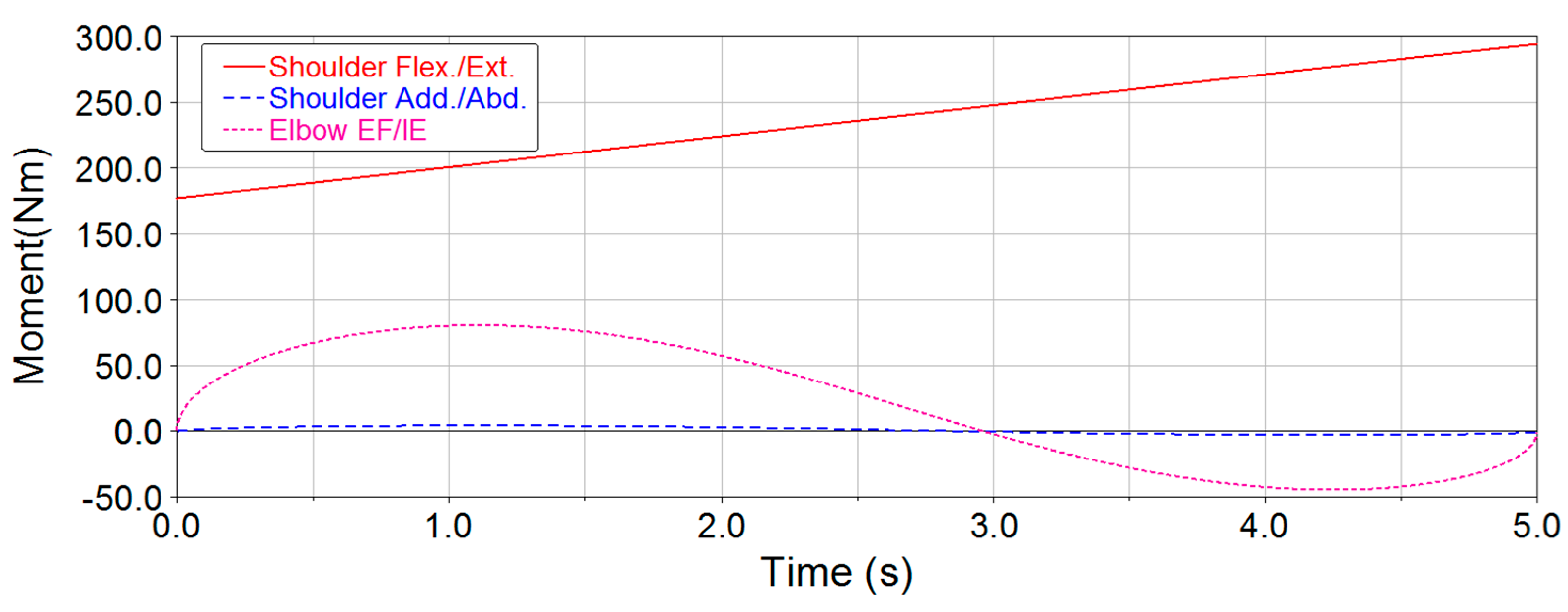
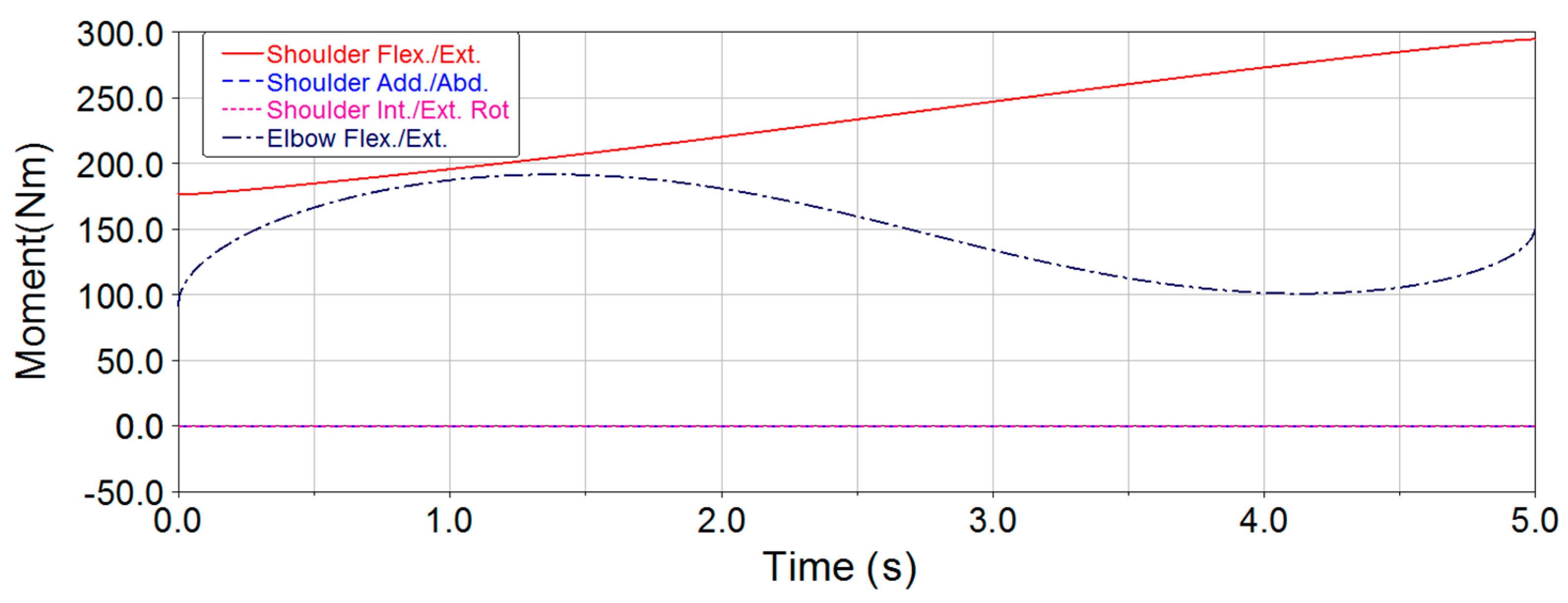
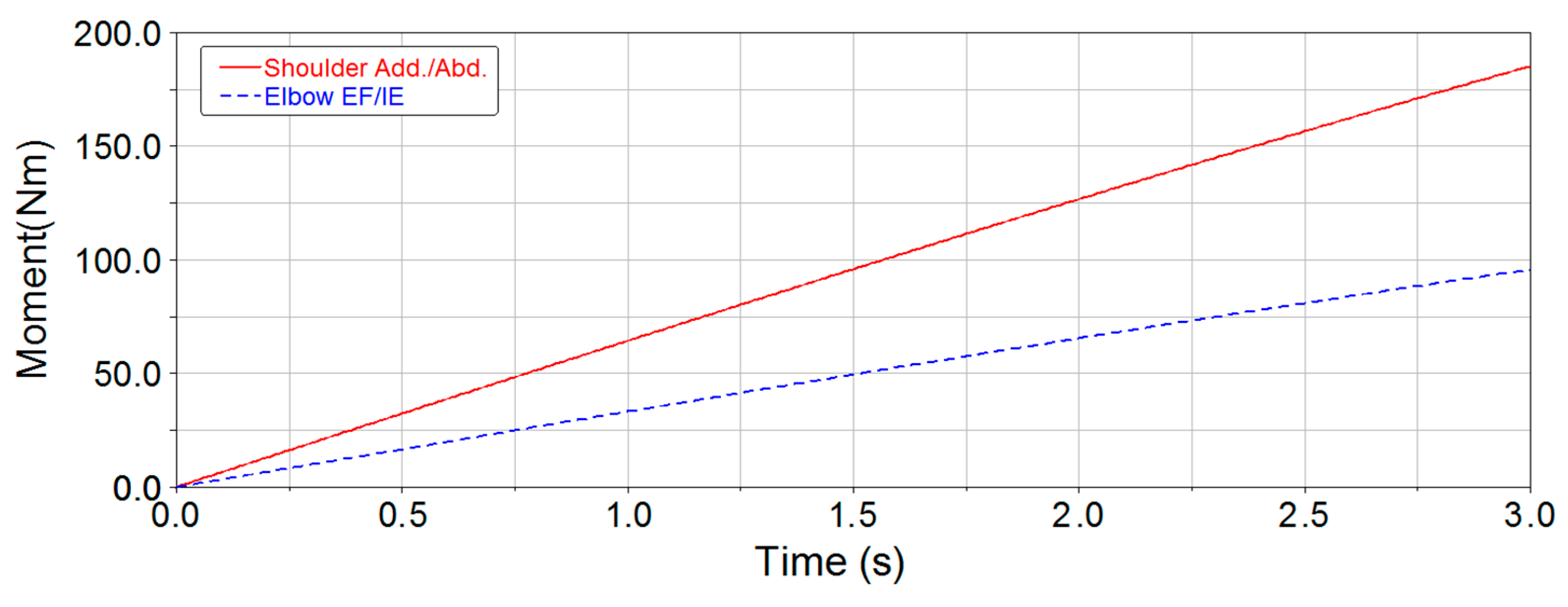
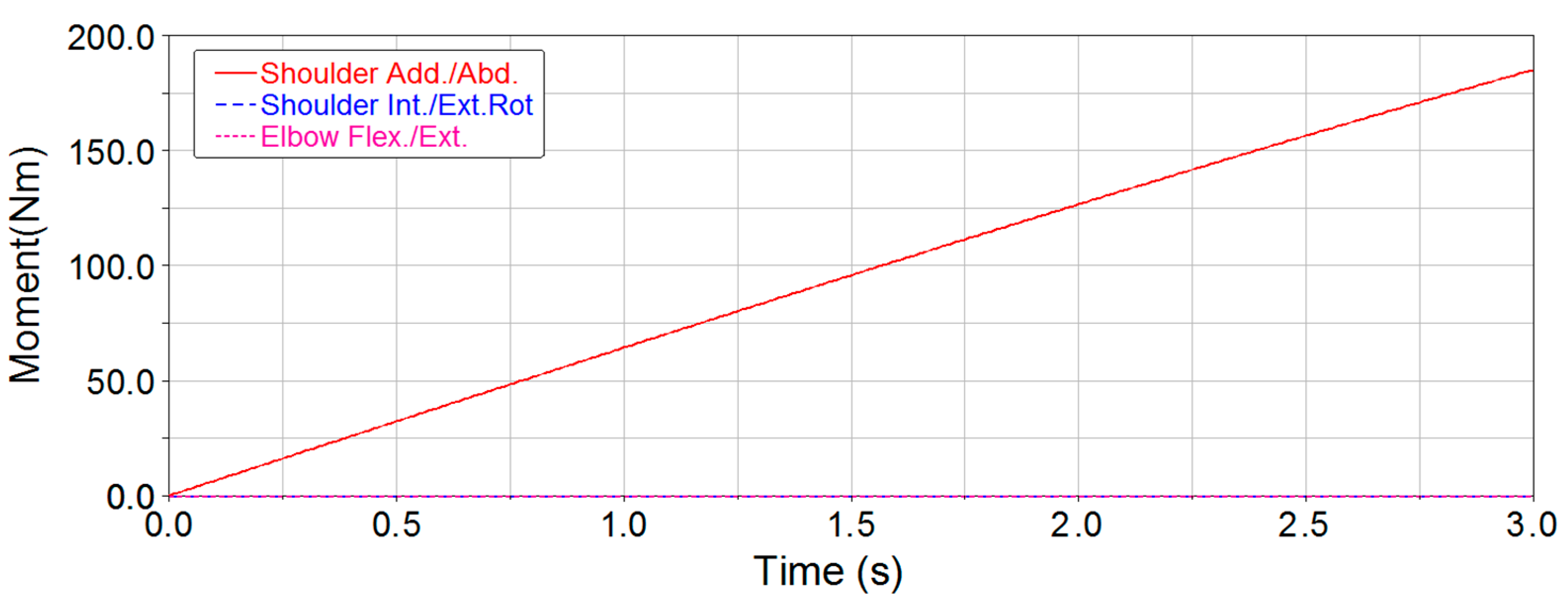
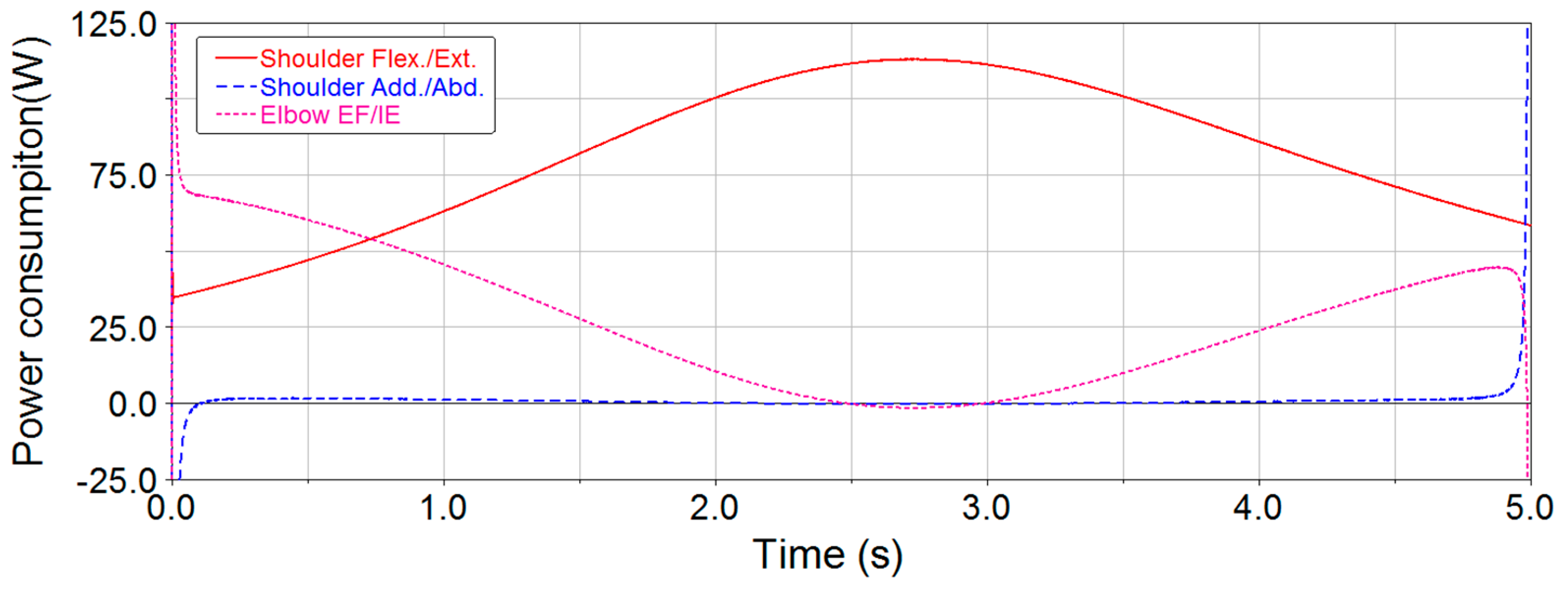
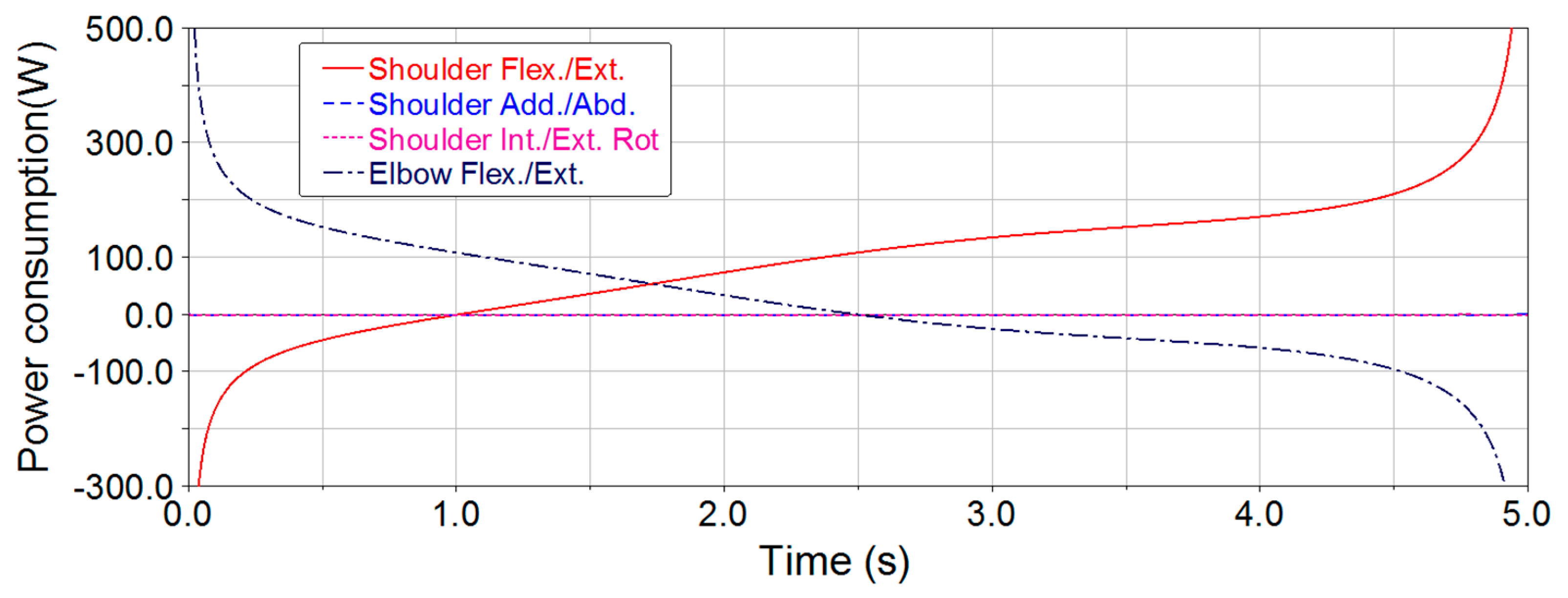
When carrying loads in front of the body, HEXAR’s assistive principle is the same as the XOS2 developed by Raytheon and the Body Extender system developed by Santa Ana University of Italy. They are all assisted by the anthropomorphic shoulder flexion/extension and elbow flexion/extension. The torque generated by a load on the elbow joint is entirely driven by the elbow flexion/extension actuator, which makes the elbow actuator larger and heavy, and the volume and weight of the upper-limb exoskeleton can hardly be reduced.
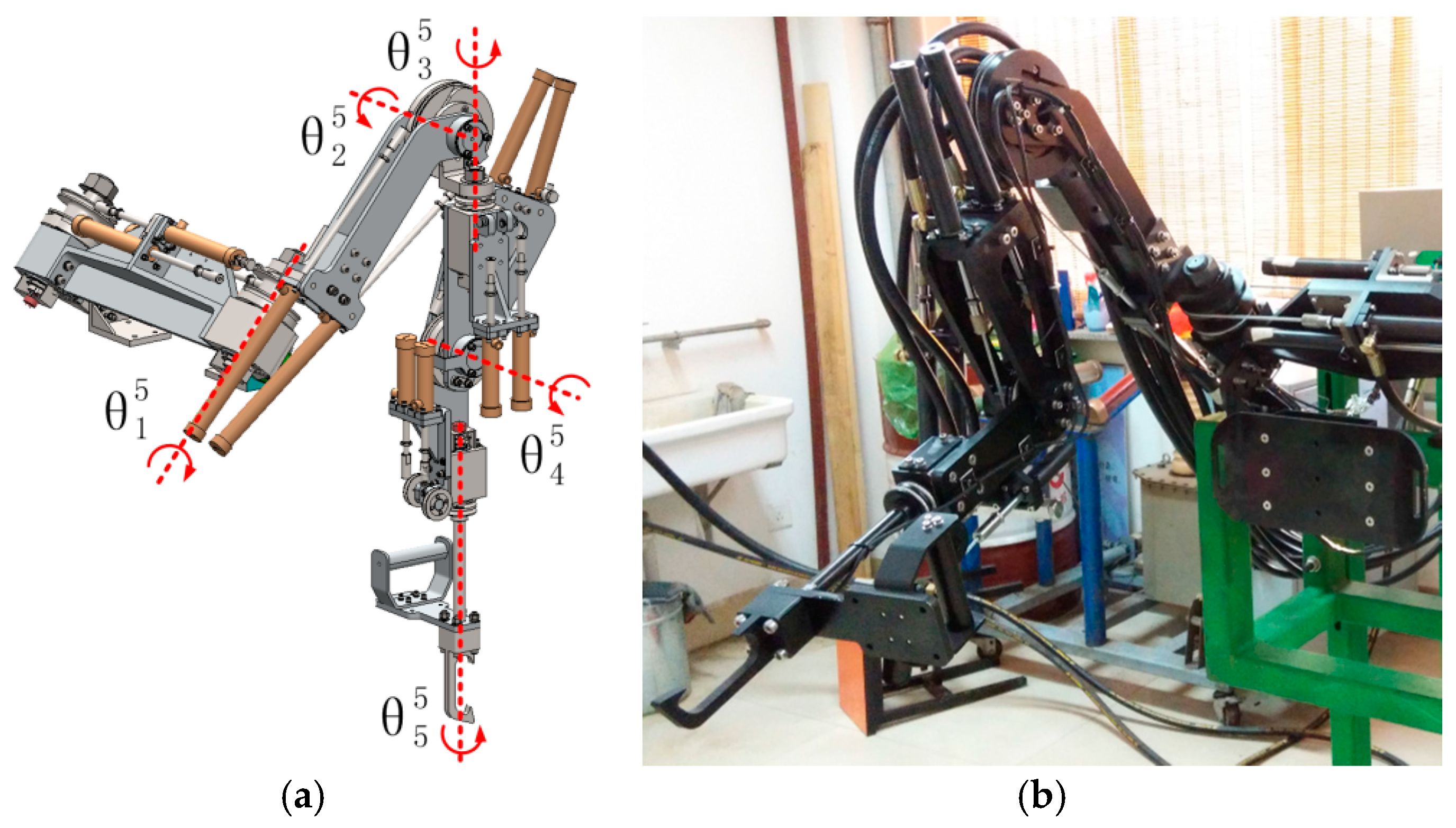
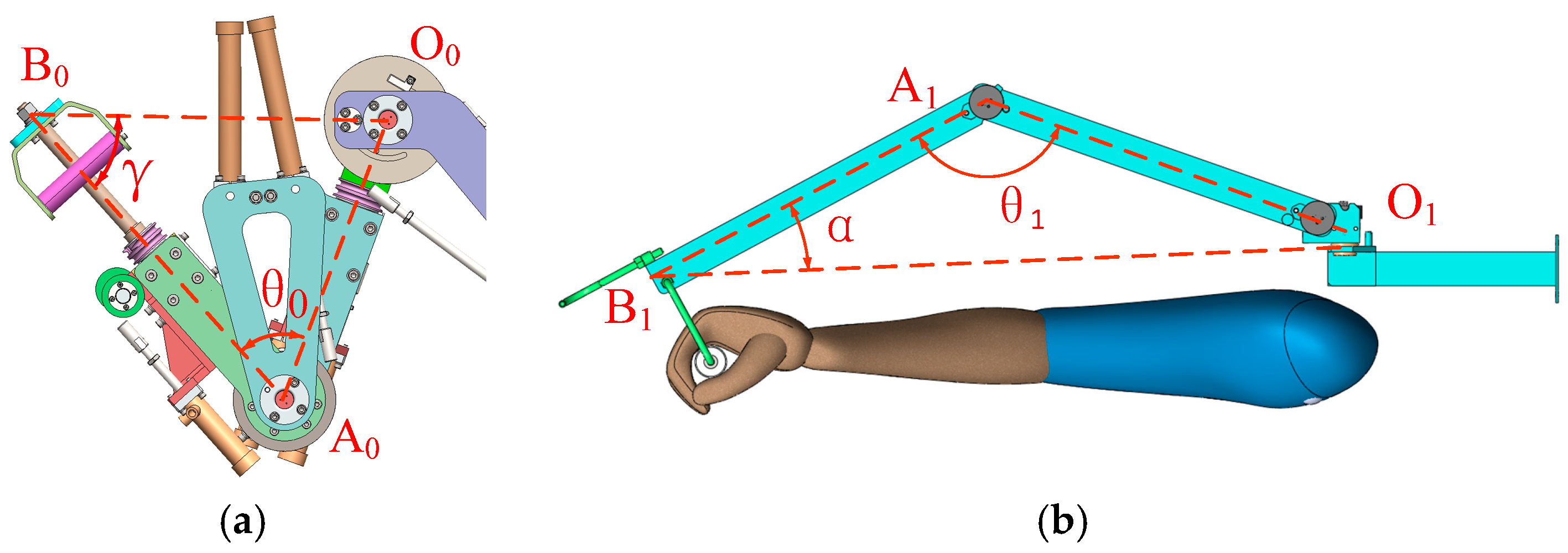
Many of the existing power-assisted exoskeletons adopt an anthropomorphic structure similar to that of the human body, with few considerations for non-anthropomorphic structures. In this article, a typical anthropomorphic 5-DOF power-assisted upper-limb exoskeleton for material handling was designed based on the upper-limb structure of the human body. On this basis, the joints were analyzed and optimized to reduce the self-weight and ensure the load capacity and the workspace. Then, a non-anthropomorphic 3-DOF upper-limb exoskeleton applied in an industrial setting was proposed, which internally rotated the traditional humanoid elbow joint 90°. The shoulder was modeled as a 2-DOF joint, and the elbow was modeled as a 1-DOF joint. The proposed exoskeleton can improve the force status of the elbow, reduce the required torque and power consumption of the elbow joint when carrying the load in industrial settings, and thus reduce the size and weight of the elbow joint actuator.
The paper is structured as follows.
Section 2 gives the principle of the 5-DOF upper-limb exoskeleton and the 3-DOF upper-limb exoskeleton. The differences and the singularity are analyzed. In
Section 3, the forward and inverse kinematics analysis based on workspace is presented.
Section 4 illustrates the dynamic analysis of the joint torque and power consumption.
Section 5 presents the experimental results of the exoskeleton prototypes. In
Section 6, the authors draw conclusions and provide directions for future work.
3. Kinematic Analysis
In order to verify whether the end-effector of the 3-DOF upper-limb exoskeleton can reach all points within the workspace of the 5-DOF upper-limb exoskeleton, the 5-DOF upper-limb exoskeleton and the 3-DOF upper-limb exoskeleton are analyzed with forward and inverse kinematics methods, respectively.
3.1. Forward Kinematics Analysis of the 5-DOF Upper-Limb Exoskeleton
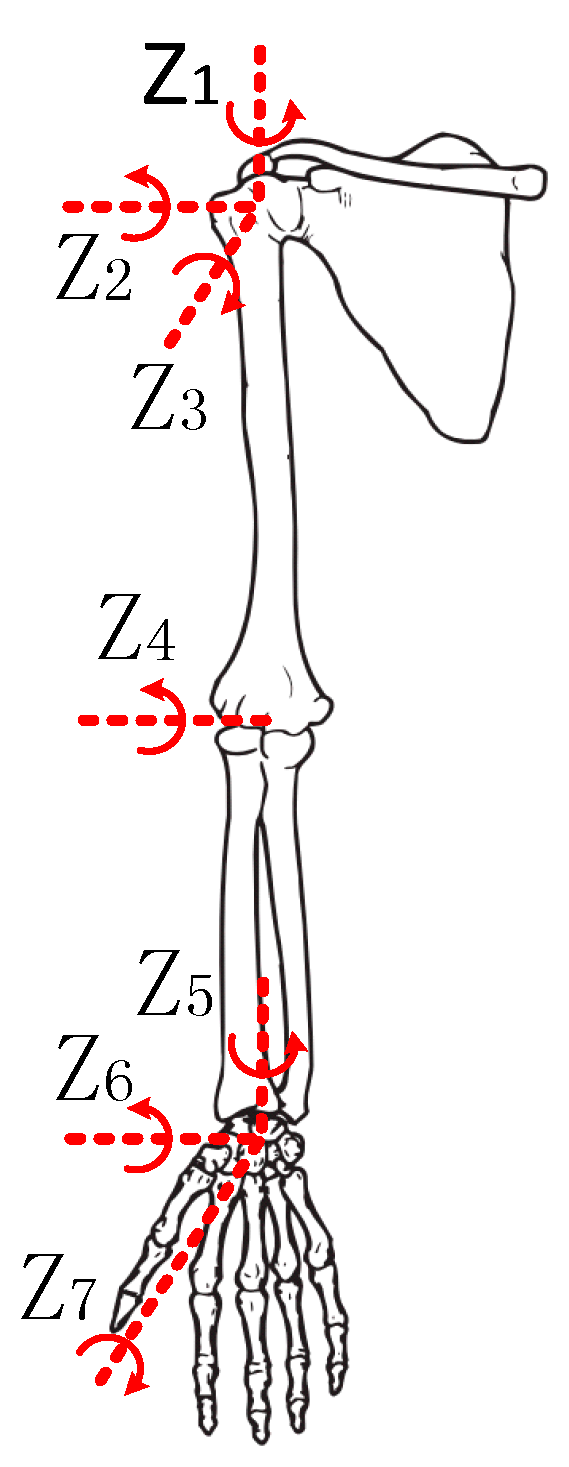
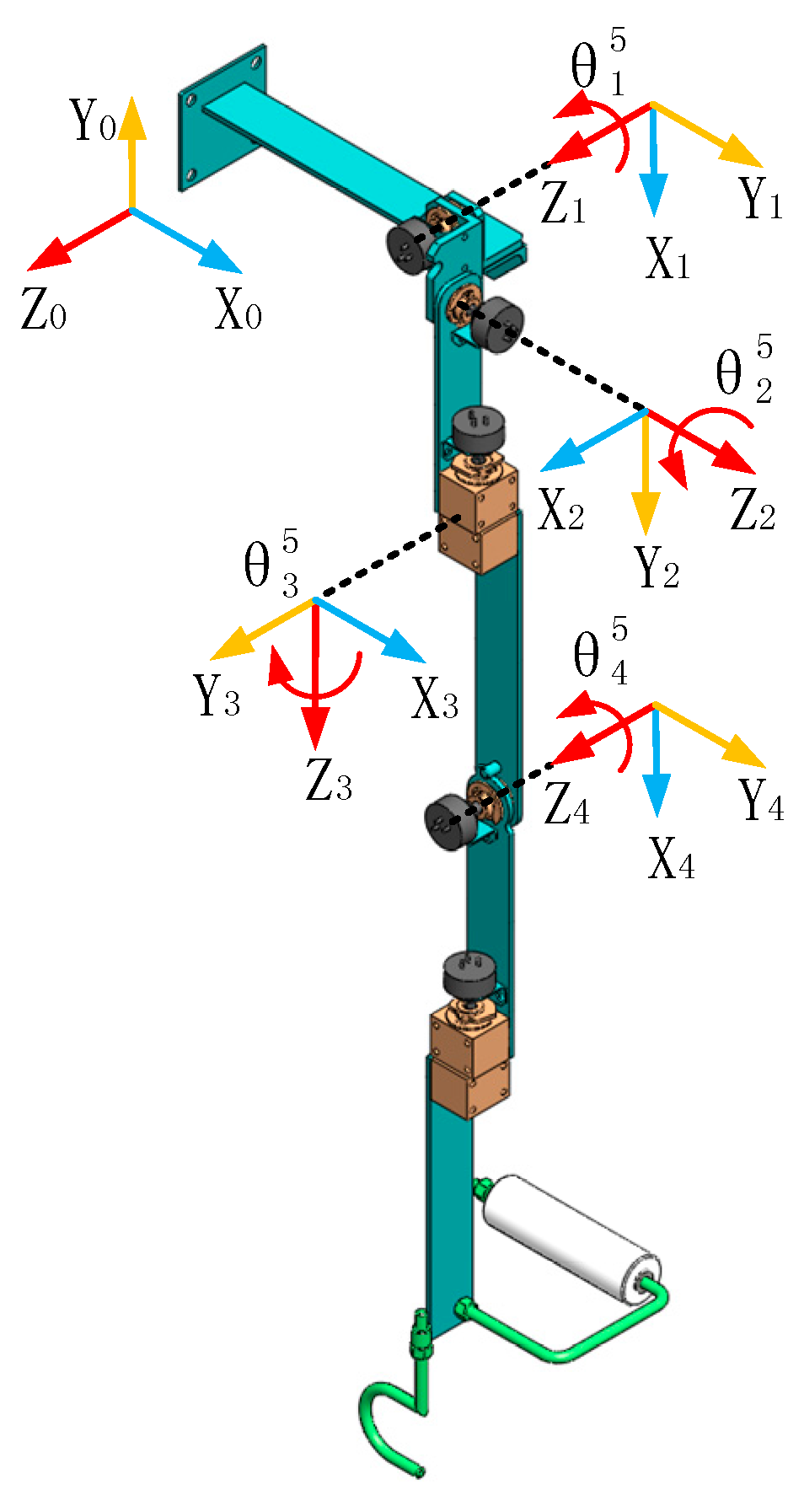
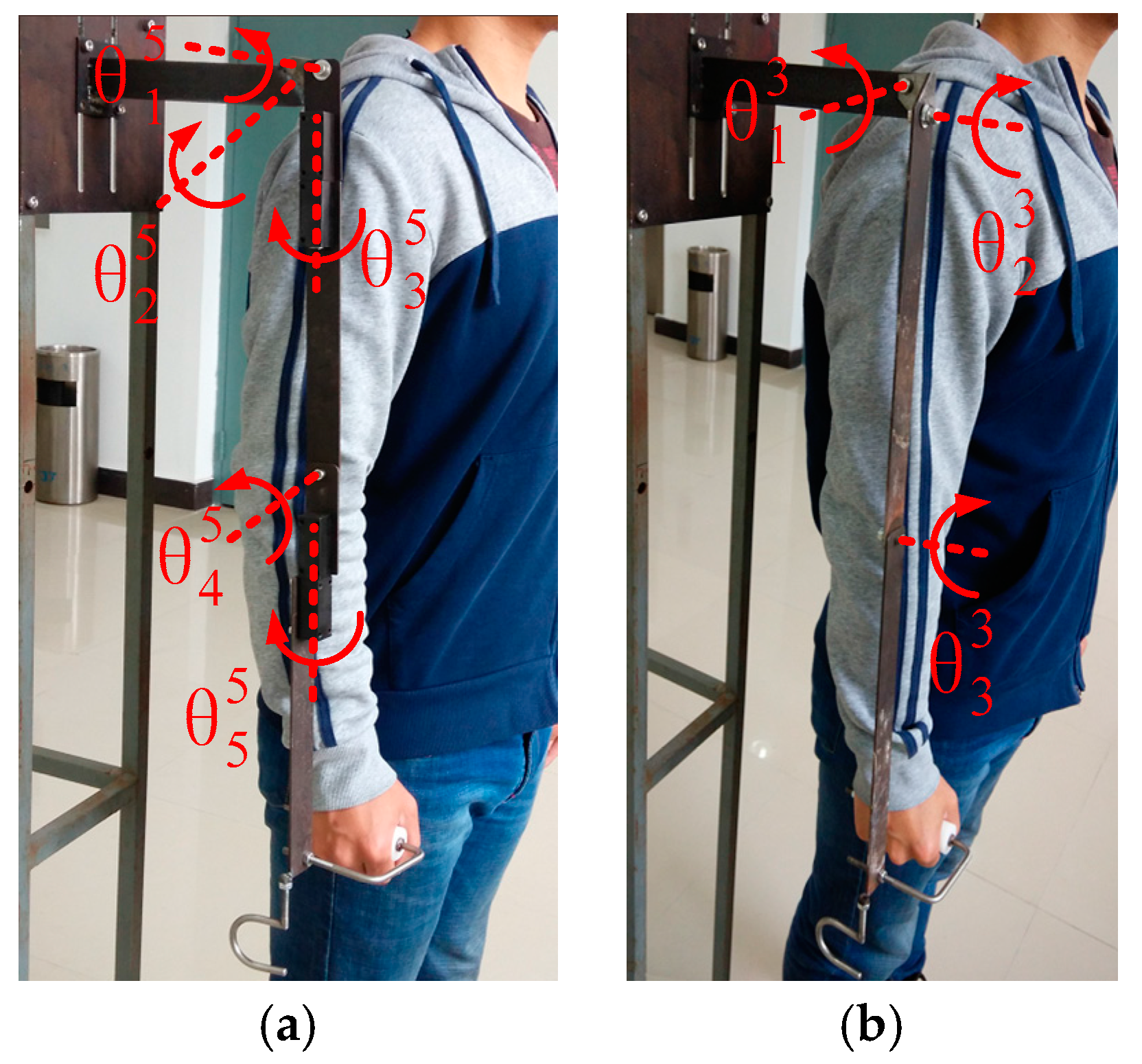
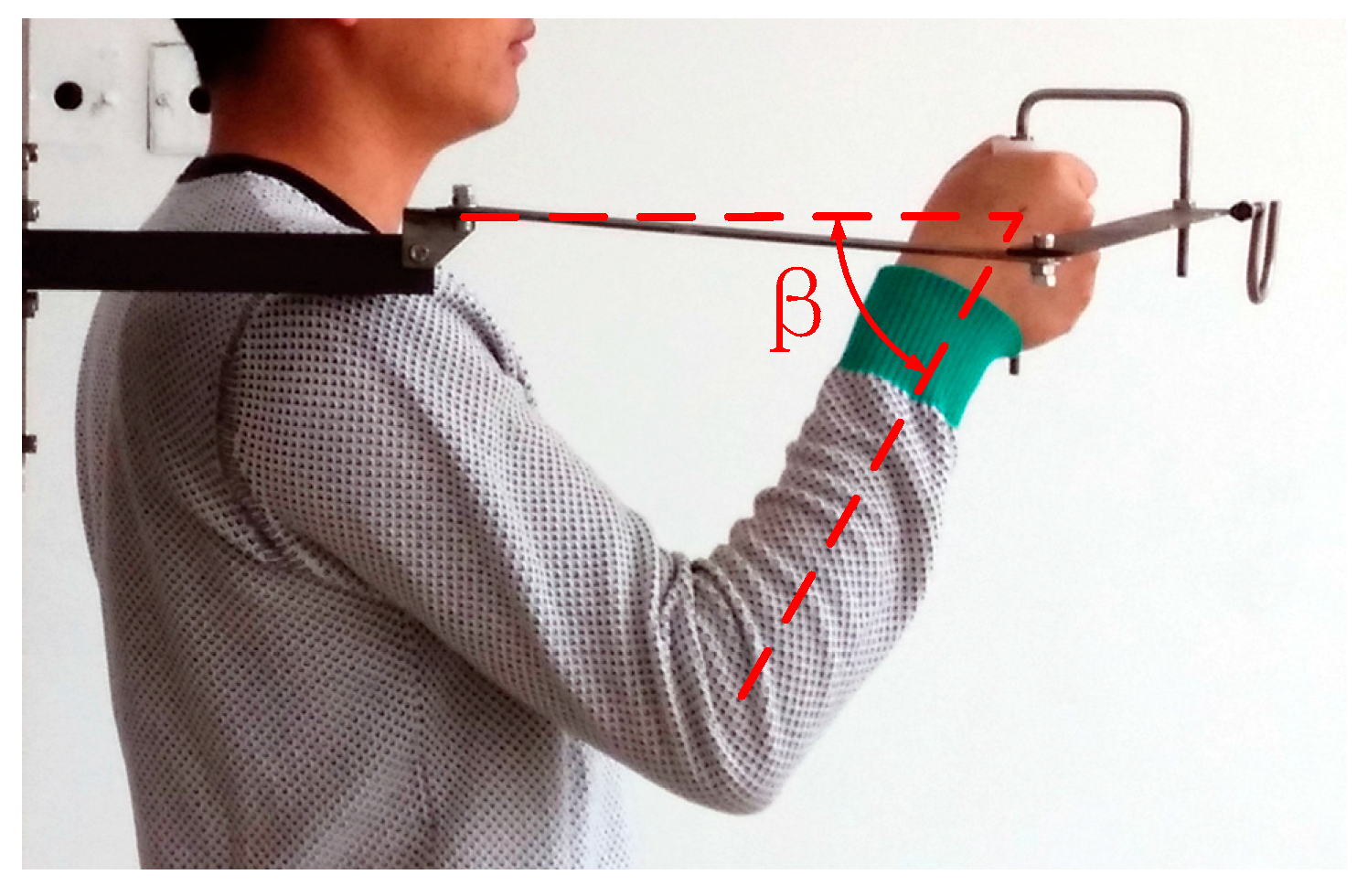
Elbow pronation/supination
has no effect on the size of the workspace, so it is considered a fixed constraint in the kinematics modeling. In order to simplify the configuration, the tilt of the abduction/adduction
axis of the shoulder joint is removed, and the flexion/extension
range of the shoulder joint is changed accordingly. Then, the kinematic model is obtained, as shown in
Figure 6. The kinematics of the 5-DOF upper-limb exoskeleton is analyzed with the Denavit-Hartenberg parameters (DH), where 0 is the initial coordinate. The DH parameters are listed in
Table 5.
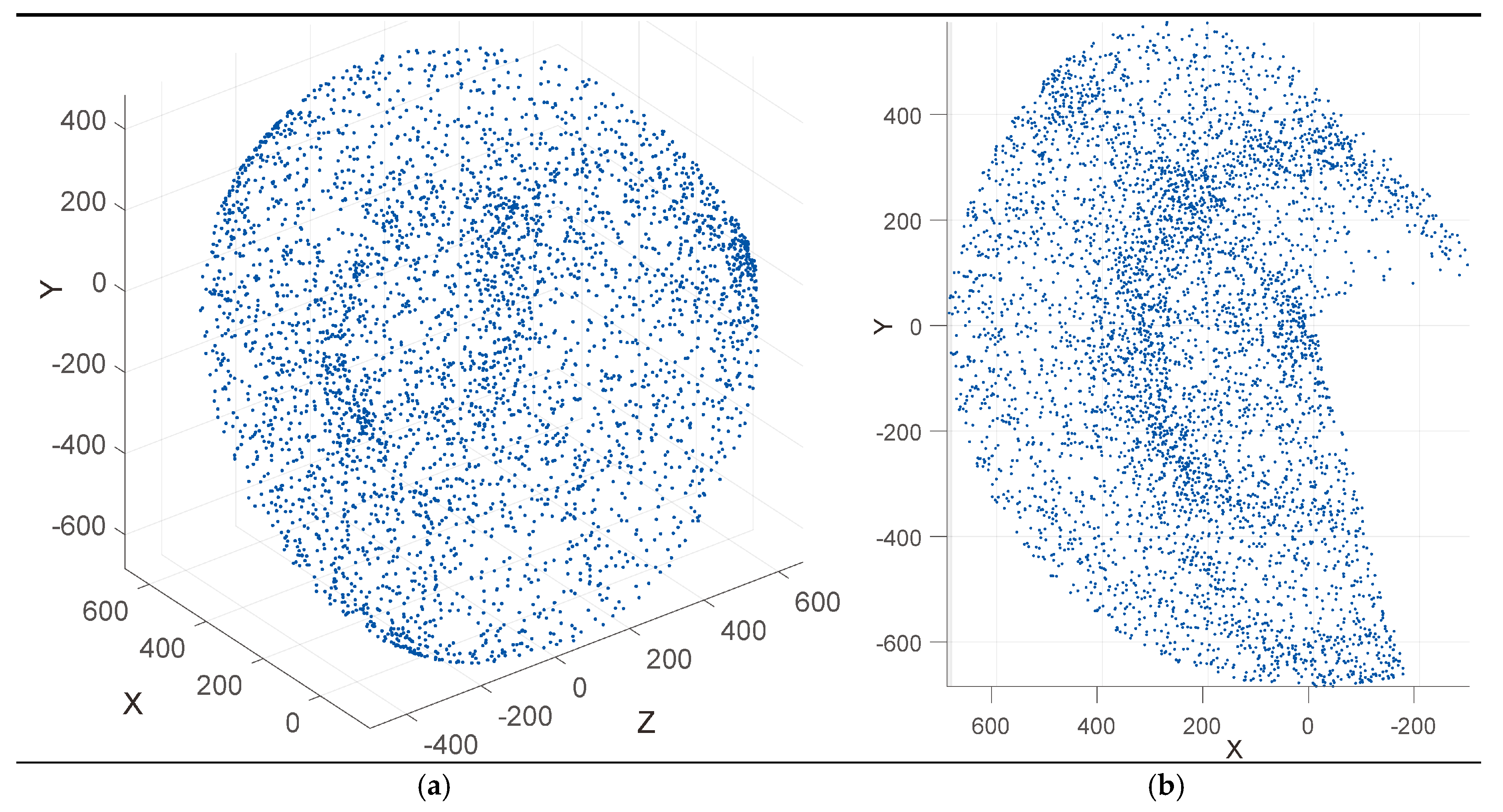
The workspace of the 5-DOF upper-limb exoskeleton is shown in
Figure 7, obtained by forward kinematics according to the motion range in
Table 2. Here, the x-axis is in the same direction as the exoskeleton, and point (0,0,0) is the center of shoulder flexion/extension.
3.2. Inverse Kinematics Analysis of the 3-DOF Upper-Limb Exoskeleton
Given an arbitrary end-effector position of the 5-DOF upper-limb exoskeleton in the workspace, the corresponding joint angles of the 3-DOF upper-limb exoskeleton can be calculated using inverse kinematics. In case the obtained angles are in the motion range of the corresponding joint, the 3-DOF upper-limb exoskeleton can reach the position of the 5-DOF upper-limb exoskeleton in the workspace, i.e., the given position is in the workspace of the 3-DOF upper-limb exoskeleton.
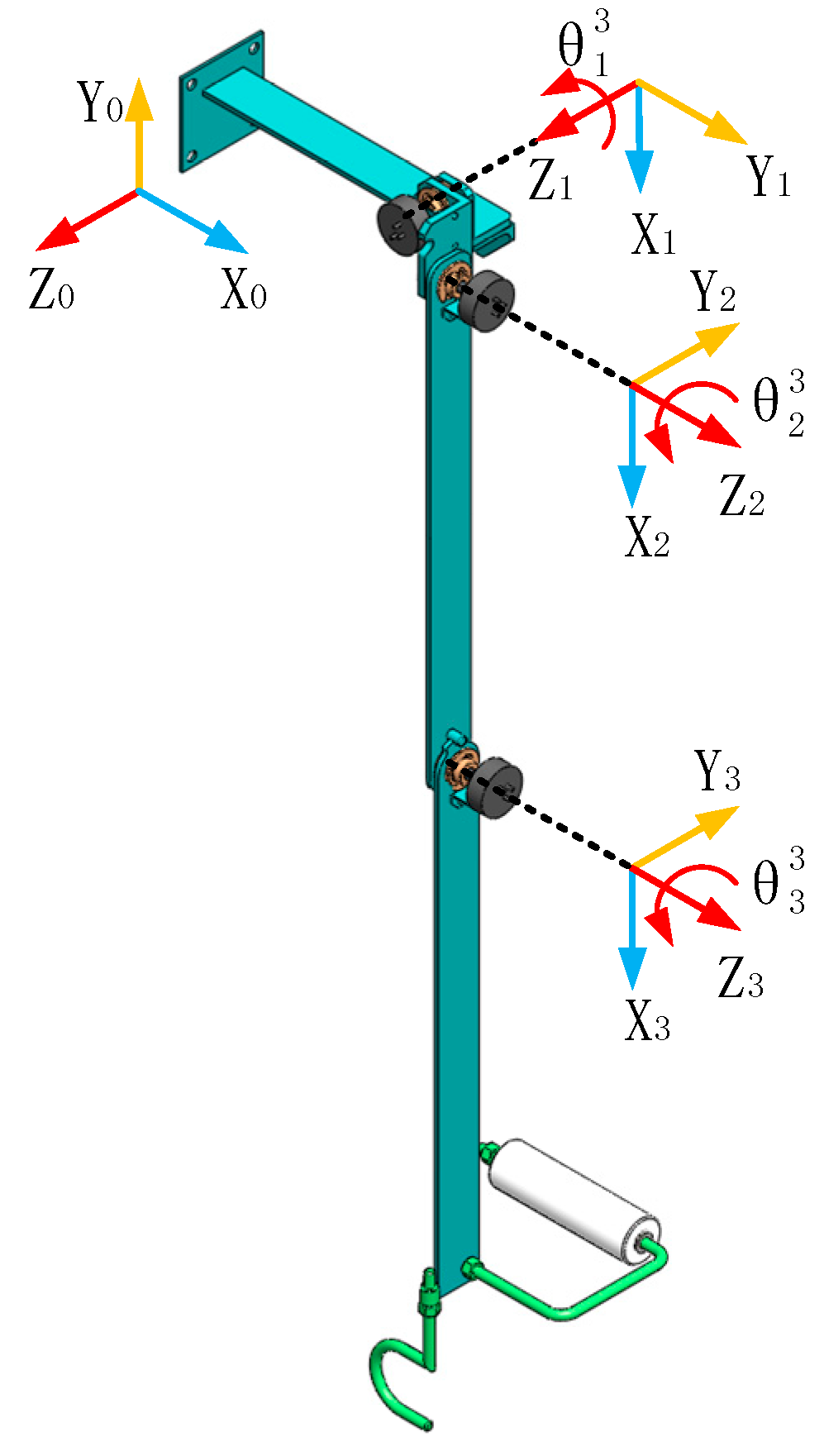
According to the previous results, the kinematics of the 3-DOF upper-limb exoskeleton are analyzed with the Denavit-Hartenberg parameters (DH), where 0 is the initial coordinate, as shown in
Figure 3. The DH parameters are listed in
Table 6.
The extrema of
,
and
obtained by inverse kinematics are listed in
Table 7 [
26]. The range of listed extremum
shown in
Table 7 is
greater than the range of elbow external-flexion/internal-extension
shown in
Table 4. The motion range of the elbow joint actuator in the 5-DOF upper-limb exoskeleton reaches
. It is feasible to reference this parameter as the motion range of the elbow external-flexion/internal-extension
, thus
derived from inverse kinematics meets the motion range of the 3-DOF upper-limb exoskeleton.
Based on the motion range of the 3-DOF upper-limb exoskeleton in
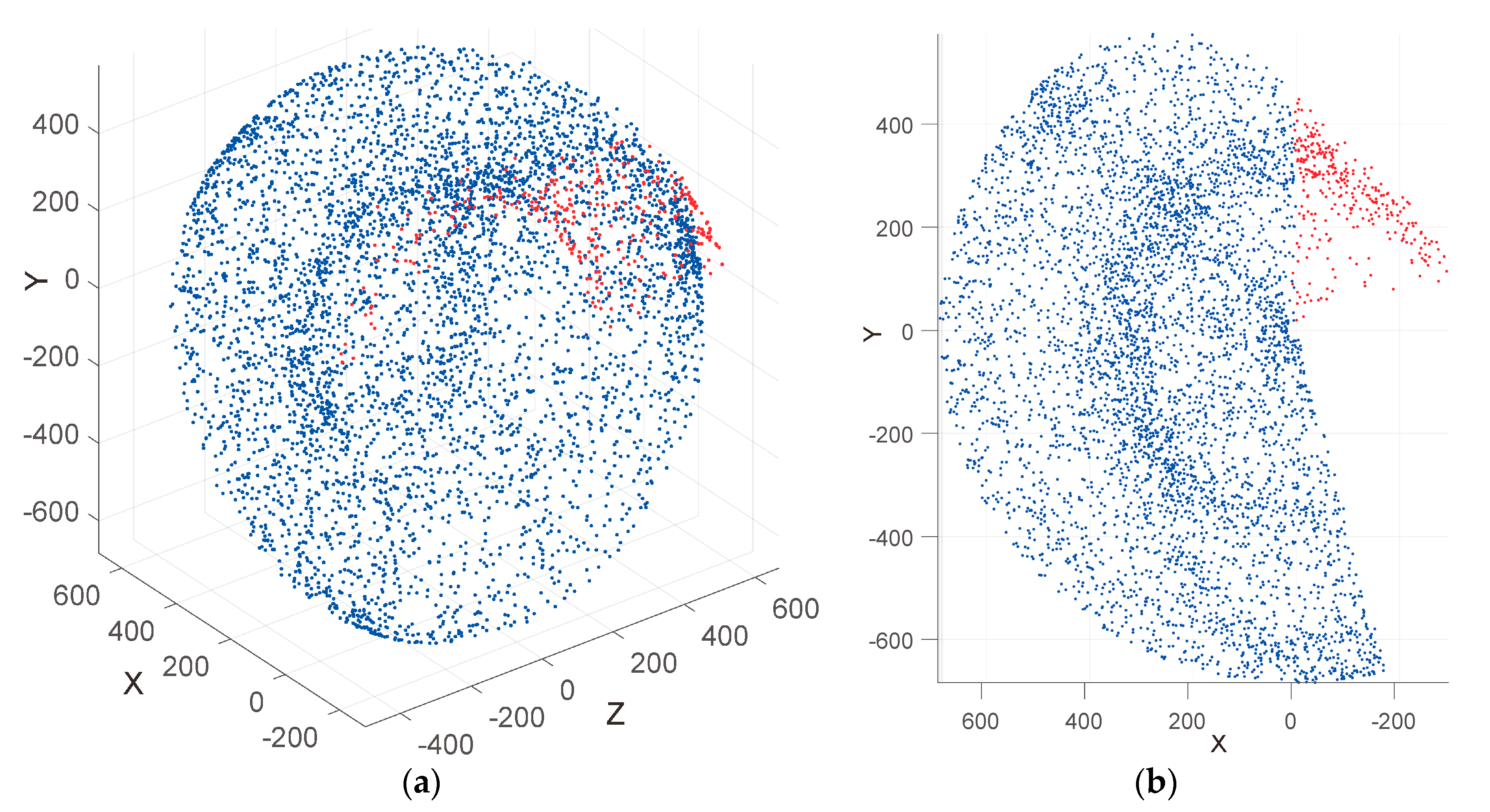
Table 4, the workspace of the 5-DOF upper-limb is divided into two parts: Part of the workspace that the 3-DOF upper-limb exoskeleton can reach called valid workspace; and part of the workspace that the 3-DOF upper-limb exoskeleton cannot reach called invalid workspace. The inverse kinematics workspace consists of valid workspace and invalid workspace (
Figure 8). The area consisting of the blue points is the valid workspace, and the area consisting of the red points is the invalid workspace. Here, the x-axis is in the same direction as the exoskeleton, and point (0,0,0) is the center of shoulder flexion/extension.
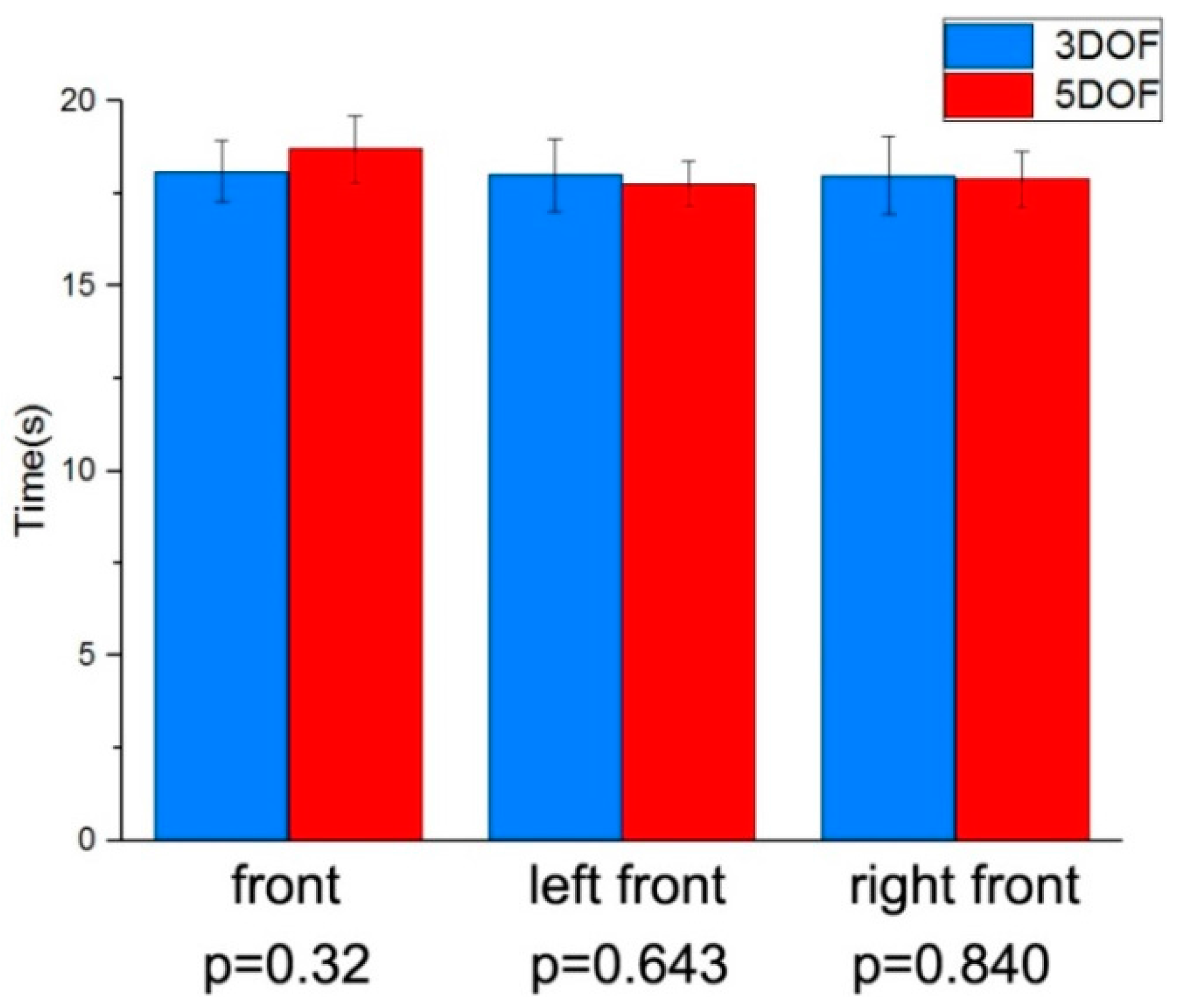
A total of 5001 points are placed in the inverse kinematics workspace, with 4621 points placed in the valid workspace, accounting for approximately 92.4%, and 380 points placed in the invalid workspace, accounting for approximately 7.6%. The invalid workspace is located behind the coronal plane of the shoulder joint. The end-effector of the 5-DOF upper-limb exoskeleton will not reach this area, while the valid workspace is the actual workspace of the 5-DOF upper-limb exoskeleton when working. The existence of invalid workspace does not affect the accessibility of the 3-DOF upper-limb exoskeleton, i.e., the 3-DOF upper-limb exoskeleton can reach all points in the actual workspace of the 5-DOF upper-limb exoskeleton.