Theoretical Assessment of DC/DC Power Converters’ Basic Topologies. A Common Static Model

Featured Application

DC/DC converters design and teaching.

Abstract

By the application of well-known circuit analysis techniques, this paper develops an intuitive approach to model the steady state regime of the three DC/DC power converters’ basic topologies (buck, boost and buck-boost). The developed approach can be considered new, realistic, accurate, general and practical. The approach is new because it is not present in the literature; realistic because it considers the main non-idealities of the different passive and active components that make up the converters; accurate because its theoretical results fit properly to those obtained in actual converters; general because it is valid for the three basic topologies; and practical because its applicability is easy and immediate from the data sheets of the converters’ components (no measurements are needed). The developed model transforms a complex system with strong non-idealities in the form of distributed parameters, in a simple and intuitive scheme of concentrated parameters (just three), which accurately reflects the actual behavior of the three basic converters’ topologies. The characteristic parameters of the model and its main relationships are determined analytically. The quality of the developed approach has been tested in the paper and can be considered excellent.

1. Introduction

The simplified study of allegedly ideal (no losses) DC/DC converters is enough for multiple applications. However, the current need for greater efficiency in generators and power conditioners demands precise models that take non-idealities and, therefore, efficiency losses into account. Bearing this aim in mind, the present work is focused on the search for a general static model of DC/DC power converters (applicable to the three basic configurations: buck, boost and buck-boost). This model is to be analytic (demanding no measurements), intuitive, easy-to-use and realistic, so that the theoretical and experimental results match.

The popularity of DC/DC power converters is growing quickly. One of the causes behind this growth may be that these converters are an essential part of renewable energy-based electric generator systems, which are a topical issue [1,2,3,4,5]. For instance, photovoltaic generators and fuel cells generate unregulated electric power at its output. However, they must be connected to loads with strict voltage requirements or be part of hybrid systems that share buses with necessarily-regulated voltages [6,7,8,9]. DC/DC converters are the power conditioners that enable the aforementioned generators and others to provide regulated power output. Therefore, in most facilities, DC/DC converters are a post-generator mandatory stage. The foregoing means that the overall efficiency of the system up to the regulated-voltage output is the product of the generator and converter efficiencies [10,11,12]. Thus, it is of great interest to know the DC/DC converter losses and, from them, its efficiency. Moreover, new power system topologies to improve the energy efficiency are being developed [13,14,15].

DC/DC modeling methods have been traditionally divided into two main groups [16]: analytical and numerical. The former are a consequence of the application of physic-mathematical relationships to circuits. They are usually easy to interpret; however, they often lead to such mathematical complexity that renders them impractical. This is the reason for their usual simplification, which makes them inaccurate for many applications. Numerical methods, mainly based on measurements, provide precision; however they may require long running time and provide poor physical interpretation, thus hindering designers’ work.

The low frequency characterization of DC/DC converters was carried out in 1972. The works [17,18] can be considered the earliest references to DC/DC converter modeling by means of average continuous time analytical techniques. The result was an equivalent circuit obtained by two circuits averaging in each commutation interval. In this decade, some other works studied the modeling of DC/DC converters from an analytic viewpoint, yet now from a discrete perspective [19,20]. DC/DC converters modeling in discontinuous conduction mode also arises in the 1970s [21].

In the 1980s, with the aim of increasing commutation frequency, the topologies of resonant and quasi-resonant converters, as well as their models, became relevant [22,23].

It is in the 1990s when the search for a circuit-oriented modeling methodology led to the suggestion of the use of PWM-Switch [24], a three-terminal structure that contains the active and passive switch (diode) of most converters. However, this methodology has no detection criteria in the model regarding its change of conduction, which makes it difficult to use. Later, a general average state model based on state-variable representation by means of Fourier series was proposed [25]. This method enables natural inclusion of resonant converters. Reference [26] proposes the application of this method to both resonant and PWM converters, substituting switches by dependent sources. Although a generic method, it is not applicable to the converter discontinuous conduction mode, as they include no conduction-mode detection mechanism.

At the beginning of the 21st century, a systematic method (applicable to all conduction modes) to obtain circuit-oriented average models for multiple-output DC/DC converters is presented [27]. From here, it seems to be noted that there are no recent major developments over basic DC/DC converter modeling, probably because the current modeling approaches have been very well complemented with accurate simulations. Actually at the present time, it seems that interest is mostly focused on frequency analysis [28], and in the control-oriented models [29,30], with emphasis on stability analysis [31,32,33]

In any case and from our view, it is suitable and formal (for analytical studies and demonstrations and of course in higher education, where students learn DC/DC converter theory from the three basic topologies) to have accurate analytical models that match the DC/DC converters’ actual behavior.

With this aim, the authors of the present work have tried to develop a new realistic static model of concentrated parameters, which it is aimed to be intuitive and useful, and faithfully reflecting the actual operation of the three basic DC/DC converters.

In order to contribute in this field, this work presents a new model of DC/DC converter, where losses are shaped as electrical components (they are the model parameters), which allows, based on the usual circuit theory, the obtaining of useful and easy-to-use mathematical relationships. The developed model allows accuracy in theoretical calculations and reliable simulations, as well as previous steps to successful experimental implementations, as their final results may be guaranteed in advance. Even more, the developed model is the result of an intuitive approach and very easy to understand and interpret, which can facilitate its use and understanding DC/DC converters’ actual operation.

The proposed model is based on the well-known transformer theory. Just as a transformer can be used for coupling impedances in AC (by controlling the ratio between the number of turns in its primary and secondary), a DC/DC converter can be used to match resistances in DC (controlling its duty cycle δ, i.e., the relationship between the time interval in which its switch is on, T_ON, regarding the switching period T). This means that for the coupling DC generator → DC/DC converter → load, if δ control is made adaptive (under generator and/or load demand), then the resistance of the generator and the load can be matched so that the DC/DC converter allows maximum power transfer from generator to load. Moreover, in the case of renewable power sources, which are subjected to constant fluctuations as a result of environmental changes, the DC/DC converter enables the generator to work at maximum power. Ideally speaking, the relationships that allow load adaptation by DC/DC converters according to their δ are well-known [34,35]. The problem lies in the fact that these expressions are approximate and in many operating conditions undergo deviations that can make them practically inapplicable, since DC/DC power converters are strongly non-ideal systems. Therefore, the theoretical results expected as a consequence of the application of the expressions commonly found in literature are usually very far from experimental reality. This requires that reliable experimental implementation demands a previous step: careful and exhaustive simulations.

From here arises the challenge in finding a truly useful model, which provides close-to-experimentation results. It should also be simple, intuitive, easy to use and really general (i.e., valid for the three basic converter types: boost, buck and buck-boost). Figure 1 shows the proposed model, which is remarkably simple, as it comprises an ideal DC/DC converter formed by two voltage gain (A_Vi)-dependent sources: a current source in its input and a voltage source in its output. At the ideal converter input is connected an equivalent circuit consisting of the generator (V_g) and a series resistor (R_X), which is aimed at concentrating all the losses of interest in the actual converter originated by the parasitic resistances of its components (active and passive). Of course, R_X can be added to the generator internal resistance.

At the ideal converter output, a source (V_X) and the load are connected. V_X concentrates the losses in the actual converter due to the threshold voltage of its diode. Currents and voltages in the model are given by their mean values.

In this paper, and according to the model shown in Figure 1, the values of R_X, V_X and A_Vi are calculated for each basic topology (i.e., boost, buck and buck-boost). From here, input resistance, voltage gain and actual efficiency for each of these three topologies are found. Finally, model quality is assessed.

The present paper is structured as follows: Section 2 is devoted to setting the conditions for the developed model and its parameters. The parameter values for each basic topology are calculated in Section 3, Section 4 and Section 5: boost, buck and buck-boost respectively. Section 6 is devoted to comparing the results of the developed model regarding actual converters. In Section 7 the results are discussed, the fruit of which becomes clear with the excellent behavior of the model, practically like an actual converter. Finally, the paper ends with some conclusions.

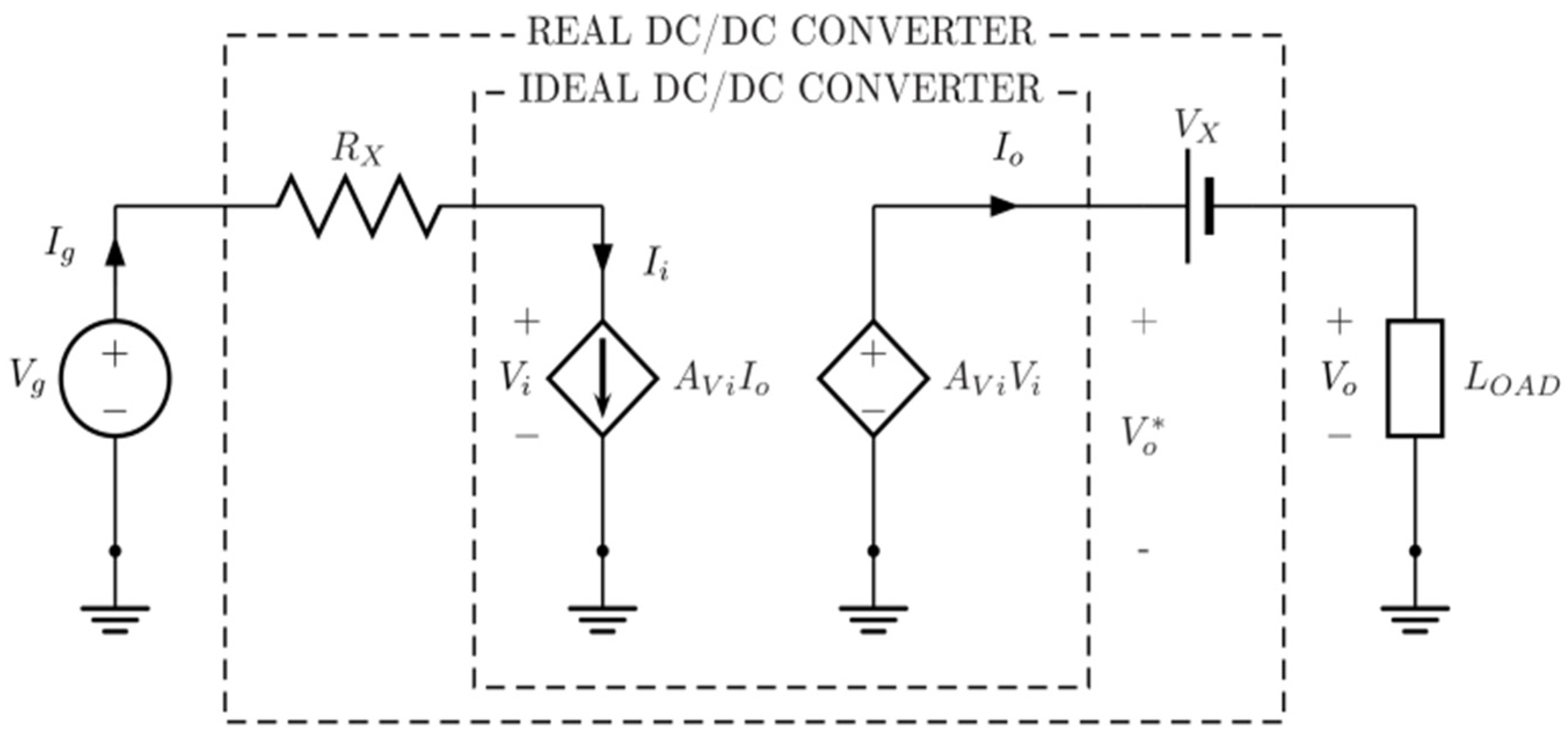
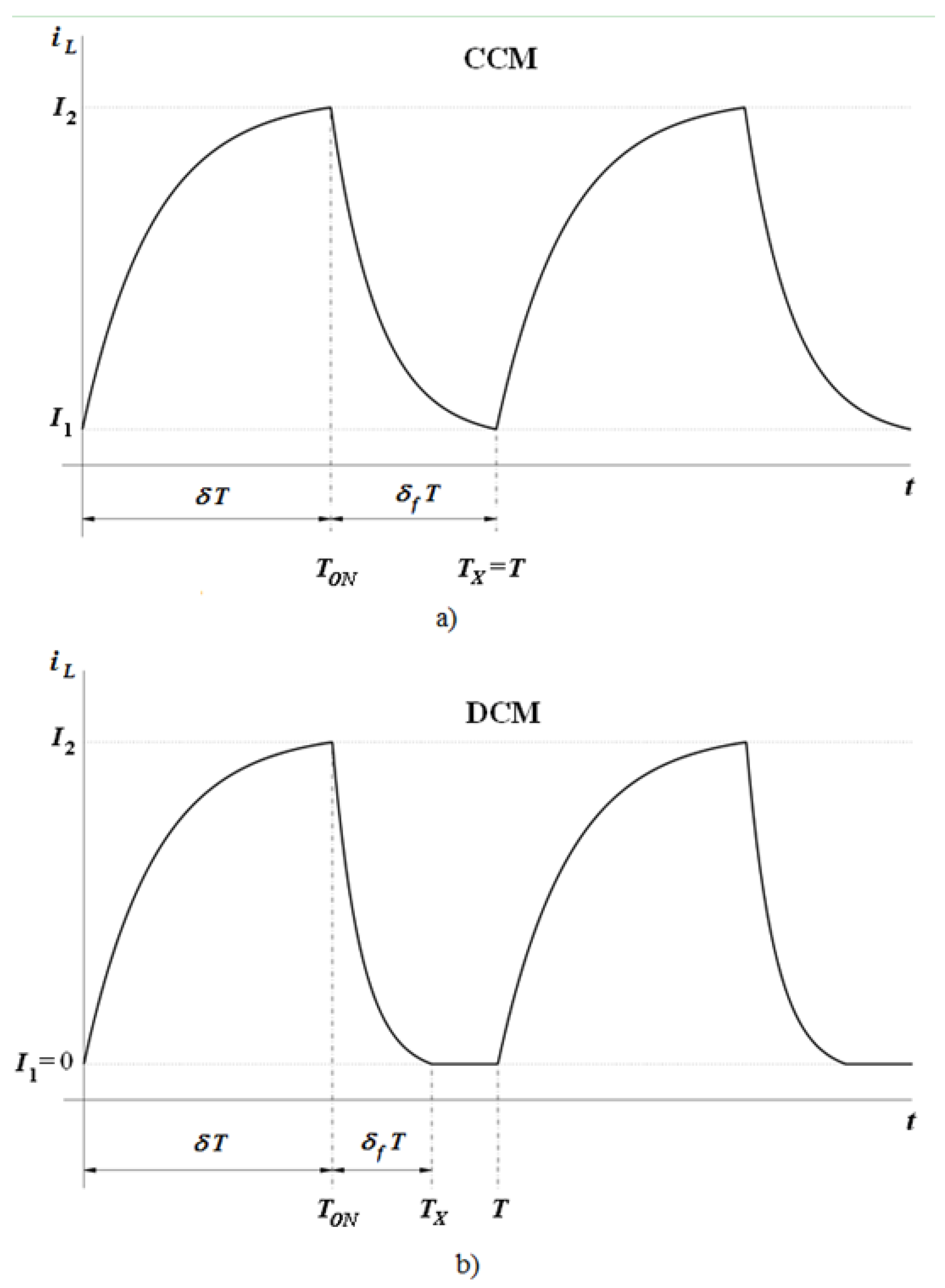
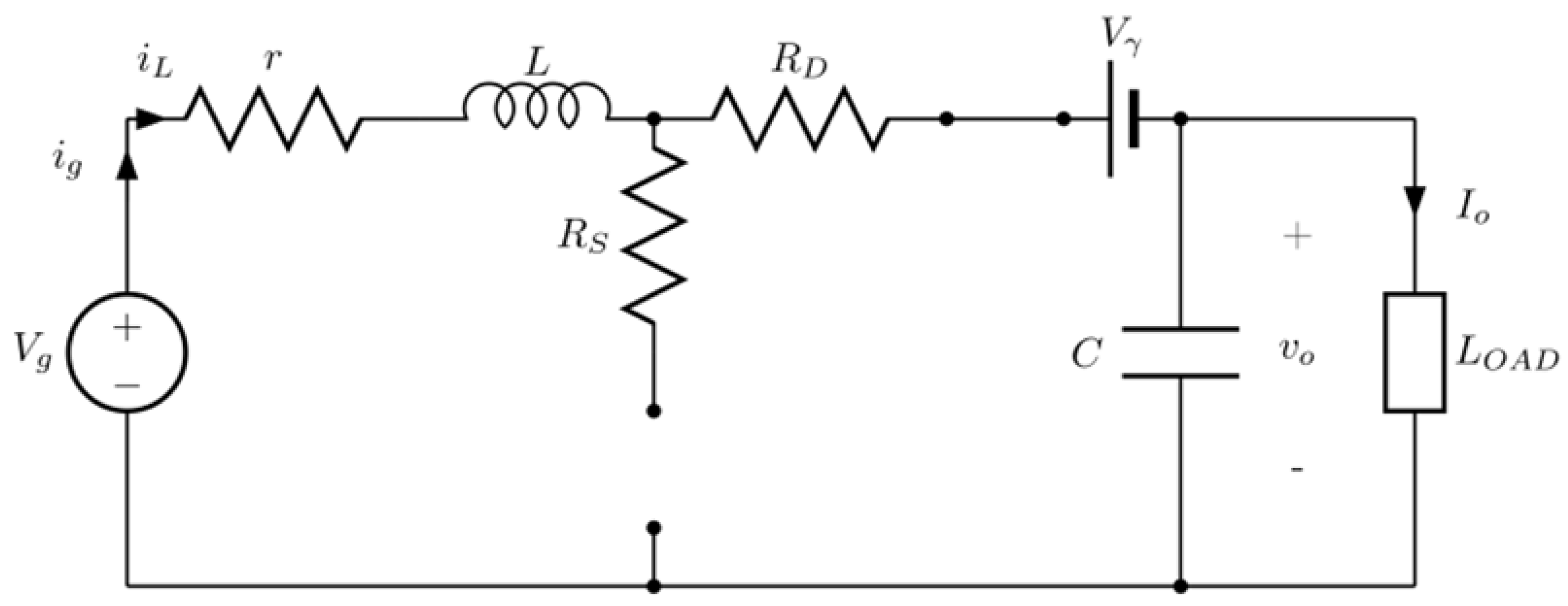
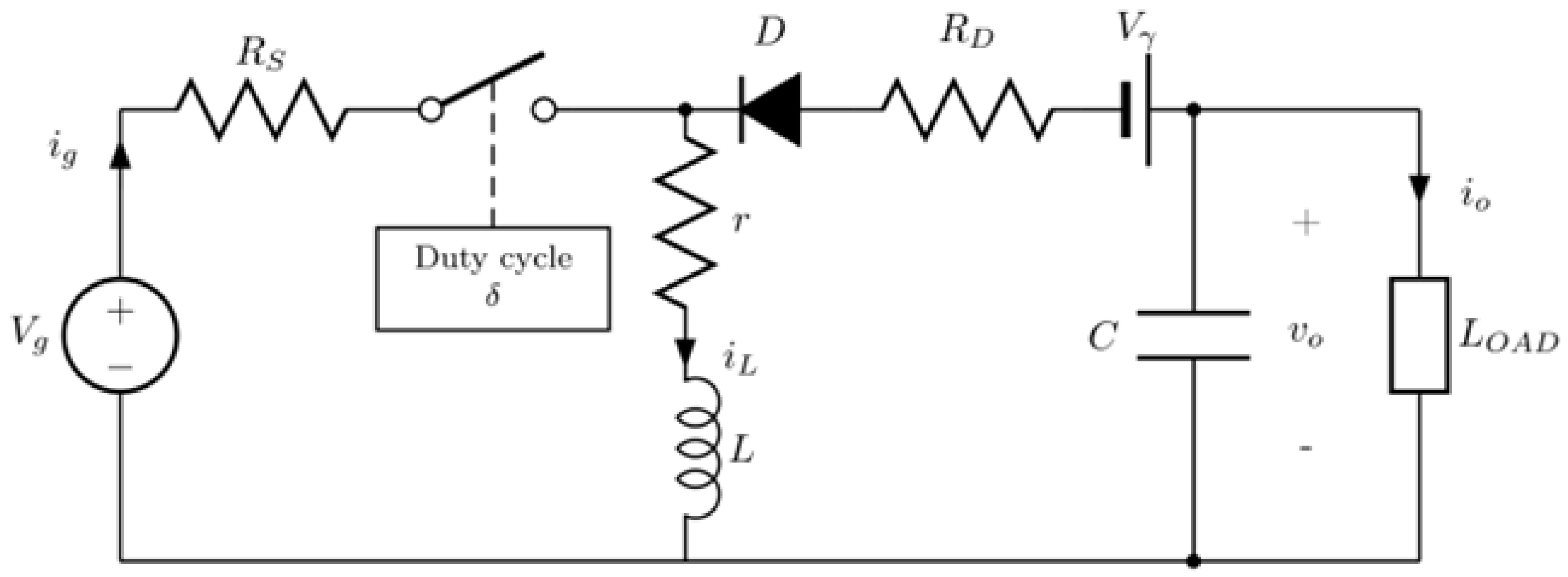
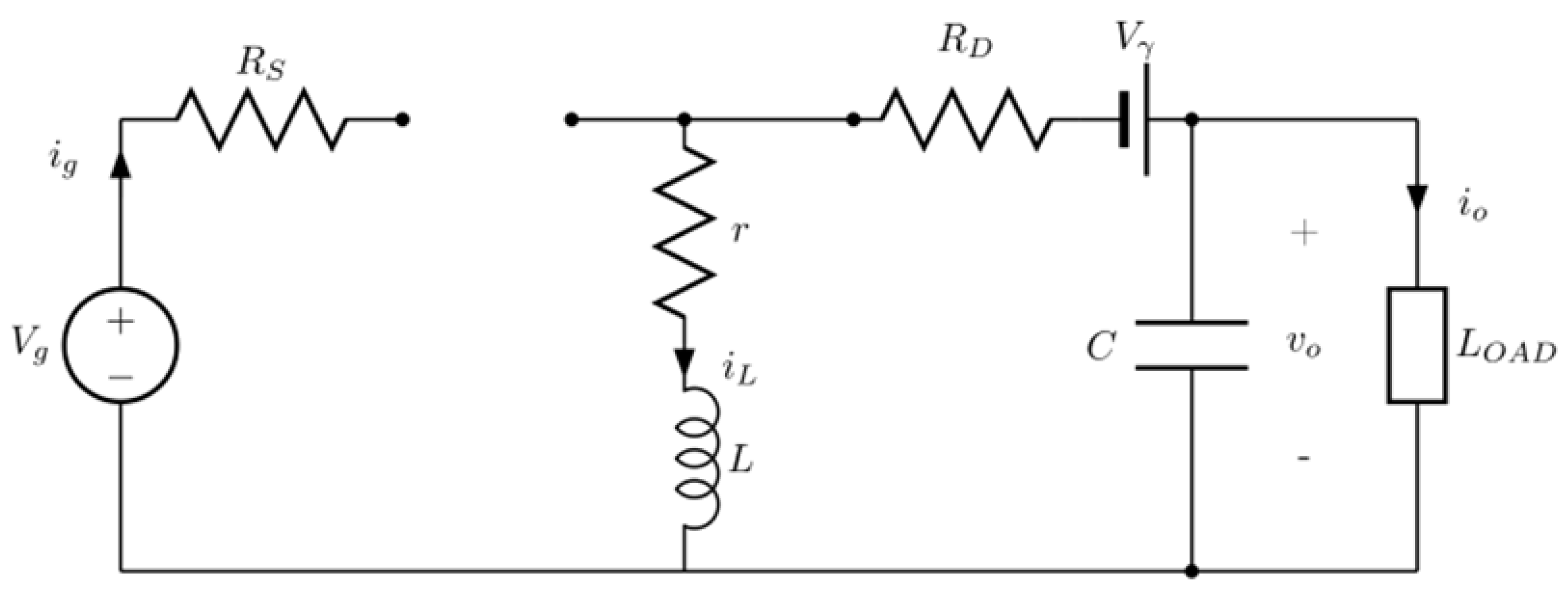
2. Operating Modes of a DC/DC Converter. Definition of Parameters

Next, in order to make the paper self-contained, the two operating modes (depending on current i_L through its inductor) of every DC/DC converter regardless of its topology are explained briefly. In addition, some parameters of interest will also be defined. The operating modes are:

Continuous conduction mode (CCM): current always flows through the inductor (see Figure 2a).

Discontinuous conduction mode (DCM): current flows at controlled intervals through the inductor (see Figure 2b).

Note in Figure 2 that the current through the converter inductor is periodical with period T. This current in turn depends on the current supplied $\left(i_g\right)$ by the generator connected to the converter. The following parameters are defined (see Figure 1, Figure 2 and Figure 3).

$$\mathrm{Switching} \mathrm{period} : T.$$

(1)

$$\mathrm{Switching} \mathrm{frequency} : f=1/T.$$

(2)

$$\mathrm{Duty} \mathrm{cycle} : \delta =T_{ON}/T=T_{ON}f.$$

(3)

$$\mathrm{Rise} \mathrm{time} \left(i_L\right): T_{ON}=\delta T=\delta /f.$$

(4)

$$\mathrm{Fall} \mathrm{time} : T_X-T_{ON}={\delta }_{f}T={\delta }_f/f.$$

(5)

$$\mathrm{Current} \mathrm{rise} : \Delta I=I_2-I_1.$$

(6)

$$\mathrm{Voltage} \mathrm{rise} : \Delta V=V_g-V_o.$$

(7)

$$\mathrm{Ideal} \mathrm{voltage} \mathrm{rise} : \Delta V^{*}=V_g-V_o^{*}=V_g- (V_0+V_X) .$$

(8)

Figure 2 shows that, in CCM, T_X = T, so:

$${{\delta }_f|}_{CCM}=\frac{T_X-T_{ON}}{T}=\frac{T-\delta T}{T}=1-\delta .$$

(9)

However, in DCM, T_X < T, so:

$${{\delta }_f|}_{DCM}=\frac{T_X-T_{ON}}{T}=\frac{T_X-\delta T}{T}=\frac{T_X}{T}-\delta <1-\delta .$$

(10)

In the following study, with the aim of making currents and voltages independent from time, these will be characterized according to their mean value (indicated by a capital letter). Thus, for I_g (Figure 1 and Figure 2) during a switching period:

$$I_g=\frac{1}{T}{\displaystyle \underset{0}{\overset{T}{\int }}{i}_g dt}=\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_g dt}+\frac{1}{T}{\displaystyle \underset{T_{ON}}{\overset{T_X}{\int }}{i}_g dt}+\frac{1}{T}{\displaystyle \underset{T_X}{\overset{T}{\int }}{i}_g dt}.$$

(11)

Both in CCM and DCM the third summing of (11) is null (Figure 2). Finally, the following hypothesis will be assumed: converters always operate in steady state regime (constant input voltage and fixed duty cycle according its operating conditions), the capacitor value C is to be assumed large enough and its losses negligible (very low equivalent series resistance, ESR). This allows consideration of a practically constant output voltage $V_0$ (Figure 3).

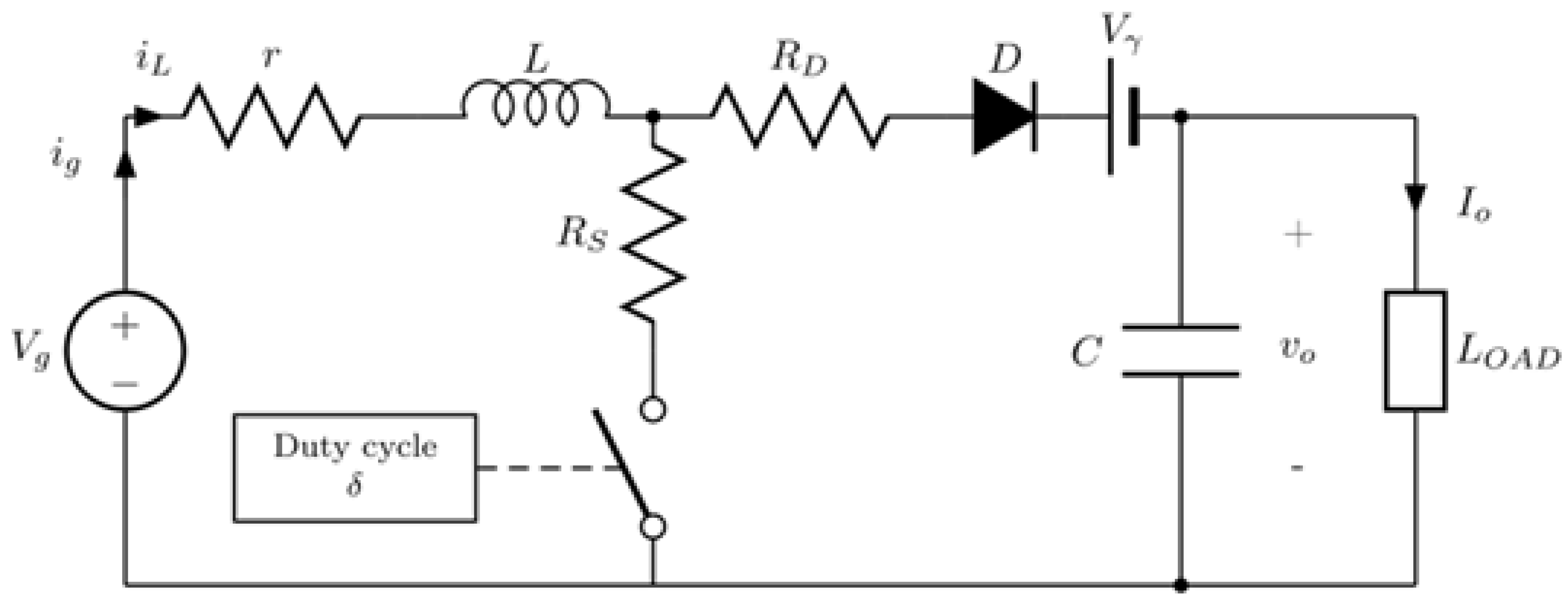
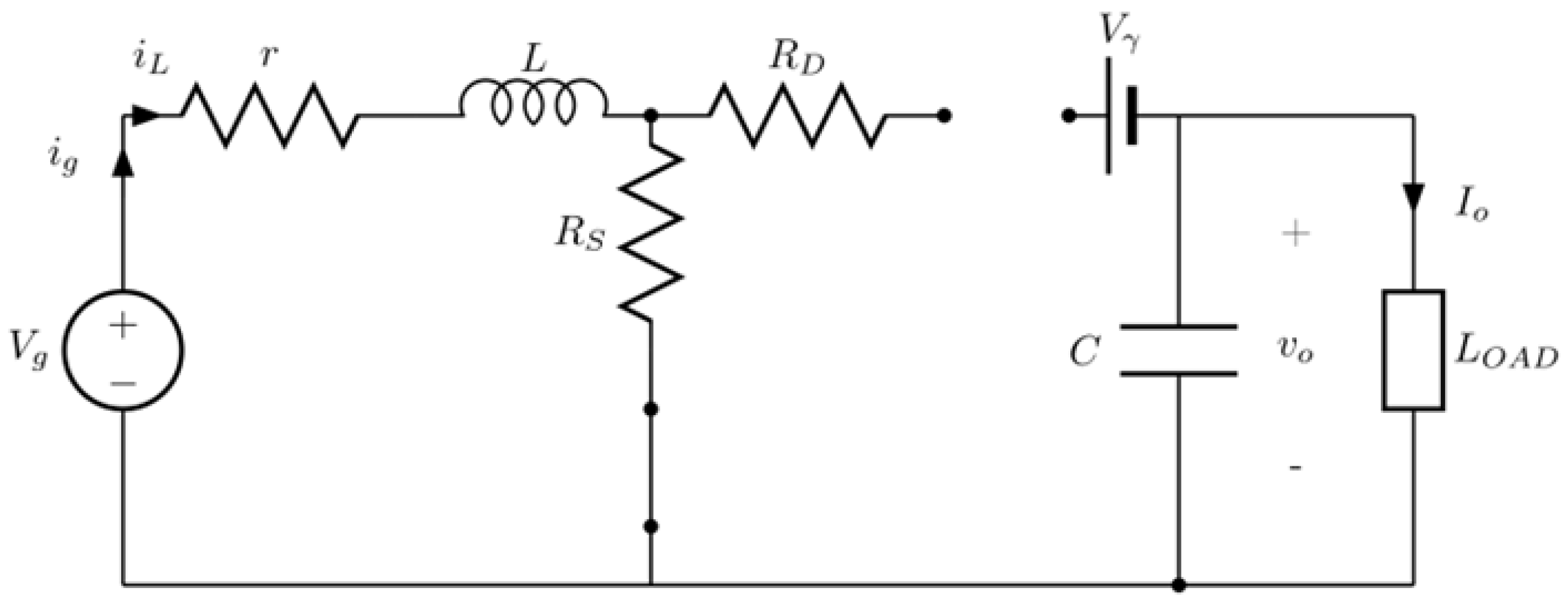
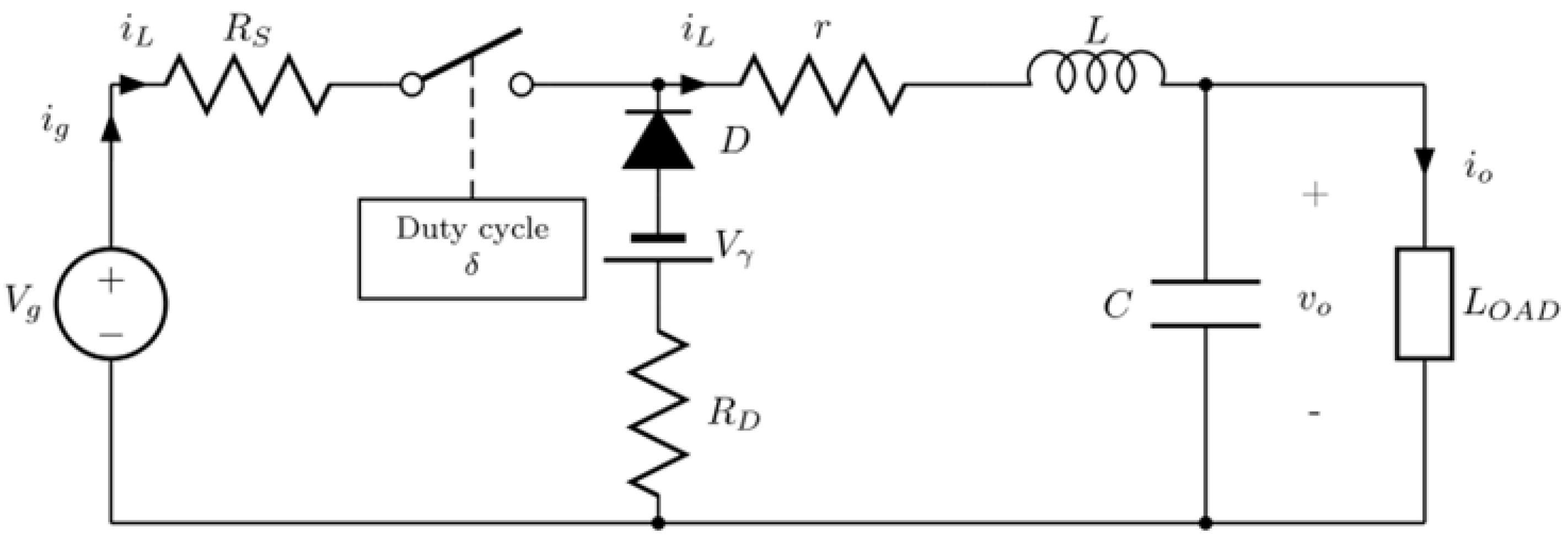
3. Boost Converter

Figure 3 shows a boost converter that includes its main non-idealities (losses). Thus, r stands for the internal inductor resistance and R_S for the power switch resistance in “on” state (closed). The leakage resistance of the power switch in “off” state (open) is so large that it can be considered an open circuit. R_D is the forward (on) resistance of the freewheeling diode (the leakage resistance in off condition is so large that it can be considered an open circuit) and V_γ is its threshold voltage. The capacitor is considered an ideal element, as its series resistance has a very low value and its leakage resistance is very large (for capacitors of certain quality) whereby it can be neglected in parallel with the load.

3.1. Determination of the Generator-Supplied Current

In Figure 3, the mean current supplied by the generator within a given time period T (see Figure 2 and (11)) is:

$$I_g=\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_L dt}+\frac{1}{T}{\displaystyle \underset{T_{ON}}{\overset{T_X}{\int }}{i}_L dt}$$

(12)

Next we will analyze the behavior of the converter in the time intervals considered in (12).

0 ≤ t ≤ T_ON

In this time interval, current through the inductor grows, the diode is reverse biased and therefore its branch is disconnected. Figure 4 shows the equivalent circuit.

From Figure 4 the following is deduced:

$$V_g=\left(r+R_S\right)i_L+L\frac{d{i}_L}{dt}\cdot$$

(13)

Solving it for i_L and integrating the result into (12):

$$\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_L dt}=\frac{1}{T}\left({\displaystyle \underset{0}{\overset{T_{ON}}{\int }}\frac{V_g}{r+R_S}dt}-\frac{L}{r+R_S}{\displaystyle \underset{I_1}{\overset{I_2}{\int }}d{i}_L}\right)=\frac{V_g}{r+R_S}\delta -\frac{f\cdot L}{r+R_S}\Delta I,$$

(14)

where $\delta$ and ΔI are given by (3) and (6) respectively. On the other hand, from (13), (4) and Figure 2 it follows that:

$${\displaystyle \underset{I_1}{\overset{I_2}{\int }}\frac{d{i}_L}{V_g-\left(r+R_S\right)i_L}}={\displaystyle \underset{0}{\overset{\delta T}{\int }}\frac{dt}{L}}\cdot$$

(15)

By a simple variable change in the left-hand integral:

$$\ln \left(\frac{V_g-\left(r+R_S\right)I_2}{V_g-\left(r+R_S\right)I_1}\right)=-\frac{r+R_S}{f\cdot L}\delta =-{\gamma }_1,$$

(16)

where

$${\gamma }_1=\frac{r+R_S}{f\cdot L}\delta =\frac{\delta }{k_1},$$

(17)

and

$$k_1=\frac{f\cdot L}{r+R_S}\cdot$$

(18)

Finally, by solving (16) for I₁:

$$I_1=\frac{V_g}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2 e^{{\gamma }_1}.$$

(19)

T_ON ≤ t ≤ T_X

In this time interval, the diode branch is connected to the generator. Figure 5 shows the equivalent circuit. Figure 2a shows how current through the inductor drops exponentially (up to t = T_X) in this time interval. At this point, if the current reaches 0 (DCM), it then keeps this value until new switch conduction (see Figure 2b).

From Figure 5 the following is deduced:

$$V_g-\left(V_o+V_{\gamma }\right)=\left(r+R_D\right)i_L+L\frac{d{i}_L}{dt}\cdot$$

(20)

By solving it for i_L and integrating the result into (12):

$$\frac{1}{T}{\displaystyle \underset{T_{ON}}{\overset{T_X}{\int }}{i}_L dt}=\frac{1}{T}\left({\displaystyle \underset{T_{ON}}{\overset{T_X}{\int }}\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}dt}-\frac{L}{r+R_D}{\displaystyle \underset{I_2}{\overset{I_1}{\int }}d{i}_L}\right)=\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}{\delta }_f+\frac{f\cdot L}{r+R_D}\Delta I.$$

(21)

where ${\delta }_f$ is given by (5). On the other hand, from (20) and Figure 2 it follows that:

$${\displaystyle \underset{I_2}{\overset{I_1}{\int }}\frac{d{i}_L}{V_g-\left(V_o+V_{\gamma }\right)-\left(r+R_D\right)i_L}}={\displaystyle \underset{T_{ON}}{\overset{T_X}{\int }}\frac{dt}{L}}\cdot$$

(22)

Now, by solving (22) as (15):

$$\ln \left(\frac{V_g-\left(V_o+V_{\gamma }\right)-\left(r+R_D\right)I_1}{V_g-\left(V_o+V_{\gamma }\right)-\left(r+R_D\right)I_2}\right)=-\frac{r+R_D}{f\cdot L}{\delta }_f=-{\gamma }_2,$$

(23)

where

$${\gamma }_2=\frac{r+R_D}{f\cdot L}{\delta }_f=\frac{{\delta }_f}{k_2},$$

(24)

and

$$k_2=\frac{f\cdot L}{r+R_D}\cdot$$

(25)

Finally, by solving (23) for I₂:

$$I_2=\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}\left(1-e^{{\gamma }_2}\right)+I_1{e}^{{\gamma }_2}.$$

(26)

The complete solution of (12) can be found by adding (14) and (21). However, both depend on ΔI, so this value must be calculated previously. For this, the equation system comprising (19), (26) and (6) is suggested:

$$I_1=\frac{V_g}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2 e^{{\gamma }_1}.$$

(27a)

$$I_2=\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}\left(1-e^{{\gamma }_2}\right)+I_1 e^{{\gamma }_2}.$$

(27b)

$$\Delta I=I_2-I_1.$$

(27c)

By solving (27c) for I₁ and substituting it in (27a):

$$I_2=\frac{V_g}{r+R_S}+\frac{\Delta I}{1-e^{{\gamma }_1}}\cdot$$

(28)

An analogous procedure in (27c) and (27b) leads to:

$$I_1=\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}-\frac{\Delta I}{1-e^{{\gamma }_2}}\cdot$$

(29)

Subtracting (29) from (28) and operating:

$$\Delta I\left(-1+\frac{1}{1-e^{{\gamma }_1}}+\frac{1}{1-e^{{\gamma }_2}}\right)=\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}-\frac{V_g}{r+R_S}\cdot$$

(30)

Now, multiplying the numerator and denominator in the left-side of (30) by $-e^{-{\gamma }_2}$ and solving for ΔI:

$$\Delta I=\beta \left(\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}-\frac{V_g}{r+R_S}\right),$$

(31)

where

$$\beta =\frac{\left(1-e^{{\gamma }_1}\right)\left(1-e^{-{\gamma }_2}\right)}{e^{{\gamma }_1}-e^{-{\gamma }_2}}\cdot$$

(32)

Now (12) can be solved. Thus, from (14) and (21):

$$I_g=\frac{V_g}{r+R_S}\delta -\frac{f\cdot L}{r+R_S}\Delta I+\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}{\delta }_f+\frac{f\cdot L}{r+R_D}\Delta I.$$

(33)

Substituting in this equation coefficients k₁ and k₂ given by (18) and (25) respectively:

$$I_g=\frac{V_g}{r+R_S}\delta +\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}{\delta }_f+k\Delta I,$$

(34)

where

$$k=k_2-k_1.$$

(35)

Substituting now (31) in (34), the following is obtained:

$$I_g=\frac{V_g}{r+R_S}\left(\delta -k\beta \right)+\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}\left({\delta }_f+k\beta \right).$$

(36)

Finally, substituting $\Delta V^{*}$ in the previous equation by its value in (8), it is finally obtained that:

$$I_g=\left(\frac{\delta -k\beta }{r+R_S}+\frac{{\delta }_f+k\beta }{r+R_D}\right)V_g-\frac{{\delta }_f+k\beta }{r+R_D}\left(V_o+V_{\gamma }\right)=\left(\frac{\delta -k\beta }{r+R_S}+\frac{{\delta }_f+k\beta }{r+R_D}\right)V_g-\frac{{\delta }_f+k\beta }{r+R_D}{V}_o-\frac{{\delta }_f+k\beta }{r+R_D}{V}_{\gamma }.$$

(37)

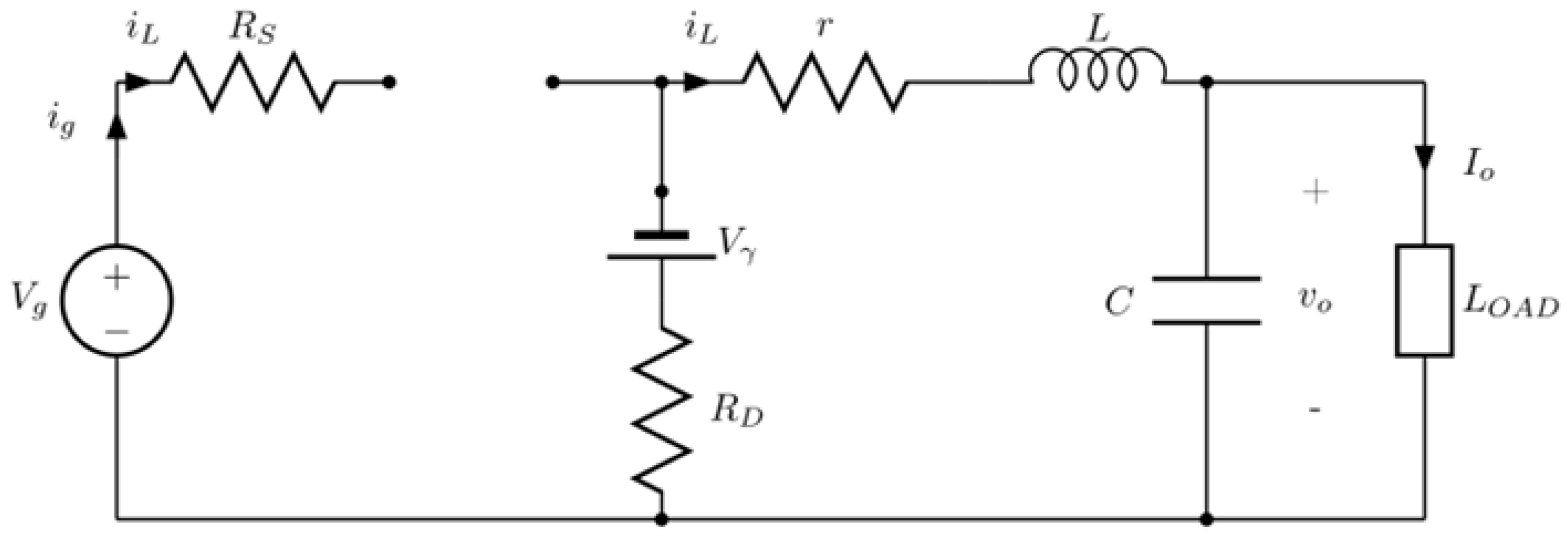
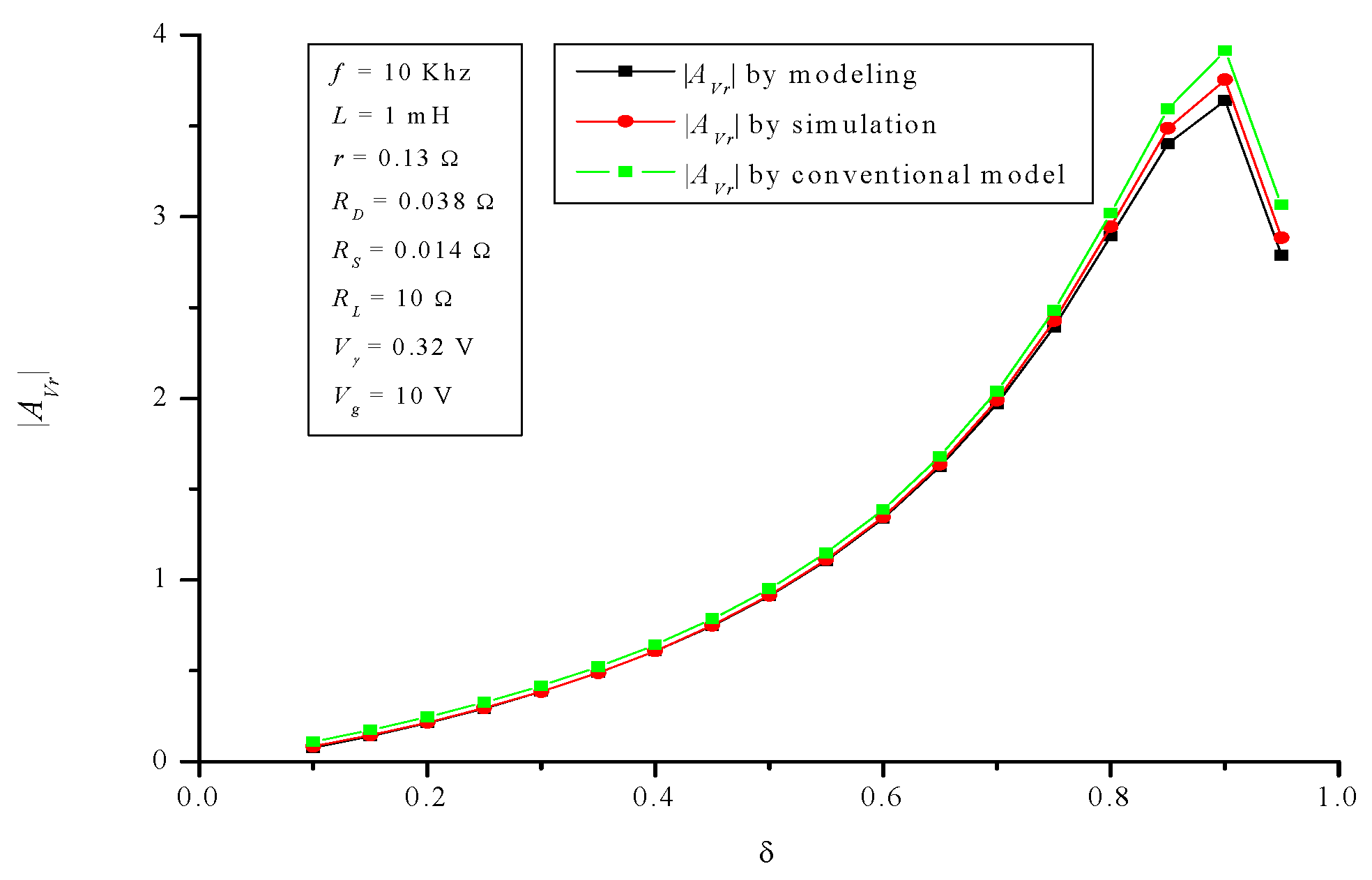
3.2. Loss Resistance, Loss Voltage and Voltage Gain Determination

At the input of the ideal converter (Figure 1):

$$P_i=V_i{I}_i=A_{Vi}{V}_i I_o,$$

(38)

while at the output:

$$P_o^{*}=V_o^{*}{I}_o=A_{Vi}{V}_i I_o.$$

(39)

Of course, (38) and (39) show that in an ideal converter $P_i=P_o^{*}$, so the efficiency $\eta =P_o^{*}/P_i$ is 1. At the input of the actual converter (Figure 1):

$$V_g=I_g{R}_X+V_i=A_{Vi} I_o R_X+\frac{V_o^{*}}{A_{Vi}}=A_{Vi} I_o R_X+\frac{V_o+V_X}{A_{Vi}}\cdot$$

(40)

Multiplying the previous equation by $A_{Vi},$ and considering $R_L=V_o/I_o$ the load resistance connected to the converter,

$$A_{Vi}{V}_g=A_{Vi}{}^2 V_o\frac{R_X}{R_L}+V_o+V_X=V_o\left(1+A_{Vi}{}^2\frac{R_X}{R_L}\right)+V_X.$$

(41)

From here the output voltage V_o is given by

$$V_o=\frac{ A_{Vi}-\frac{V_X}{V_g}}{\left(1+A_{Vi}{}^2\frac{R_X}{R_L}\right)}{V}_g=A_{Vr}{V}_g ,$$

(42)

where A_Vr is the converter actual voltage gain. That is:

$$A_{Vr}=\frac{V_o}{V_g}=\frac{A_{Vi}-\frac{V_X}{V_g}}{1+A_{Vi}{}^2\frac{R_X}{R_L}}\cdot$$

(43)

At this point, we are interested in identifying the parameters R_X, V_X and A_Vi of the model of Figure 1. From it:

$$I_g=I_i=\frac{V_g-V_i}{R_X}=\frac{V_g}{R_X}-\frac{V_i}{R_X}=\frac{V_g}{R_X}-\frac{V_o^{*}}{A_{Vi} R_X}=\frac{V_g}{R_X}-\frac{V_o}{A_{Vi} R_X}-\frac{V_X}{A_{Vi} R_X}\cdot$$

(44)

The previous equation is then compared to (37) to determine that:

$$R_X={\left(\frac{\delta -k\beta }{r+R_S}+\frac{{\delta }_f+k\beta }{r+R_D}\right)}^{ -1},$$

(45)

$$V_X=V_{\gamma },$$

(46)

and

$$A_{Vi}=1+\frac{r+R_D}{r+R_S}\cdot \frac{\delta -k\beta }{{\delta }_f+k\beta }\cdot$$

(47)

Equations (45)–(47), respectively, allow obtaining the practical values (from V_γ, R_D and R_S given by the manufacturers of the converter components) of the model parameters (Figure 1) of a generic and real boost converter.

Then, writing Equation (45) as:

$$\frac{1}{R_X}=\frac{1}{R_{X1}}+\frac{1}{R_{X2}}=\frac{\delta -k\beta }{r+R_S}+\frac{{\delta }_f+k\beta }{r+R_D},$$

(48)

the total loss resistance R_X can be obtained as shows Figure 6.

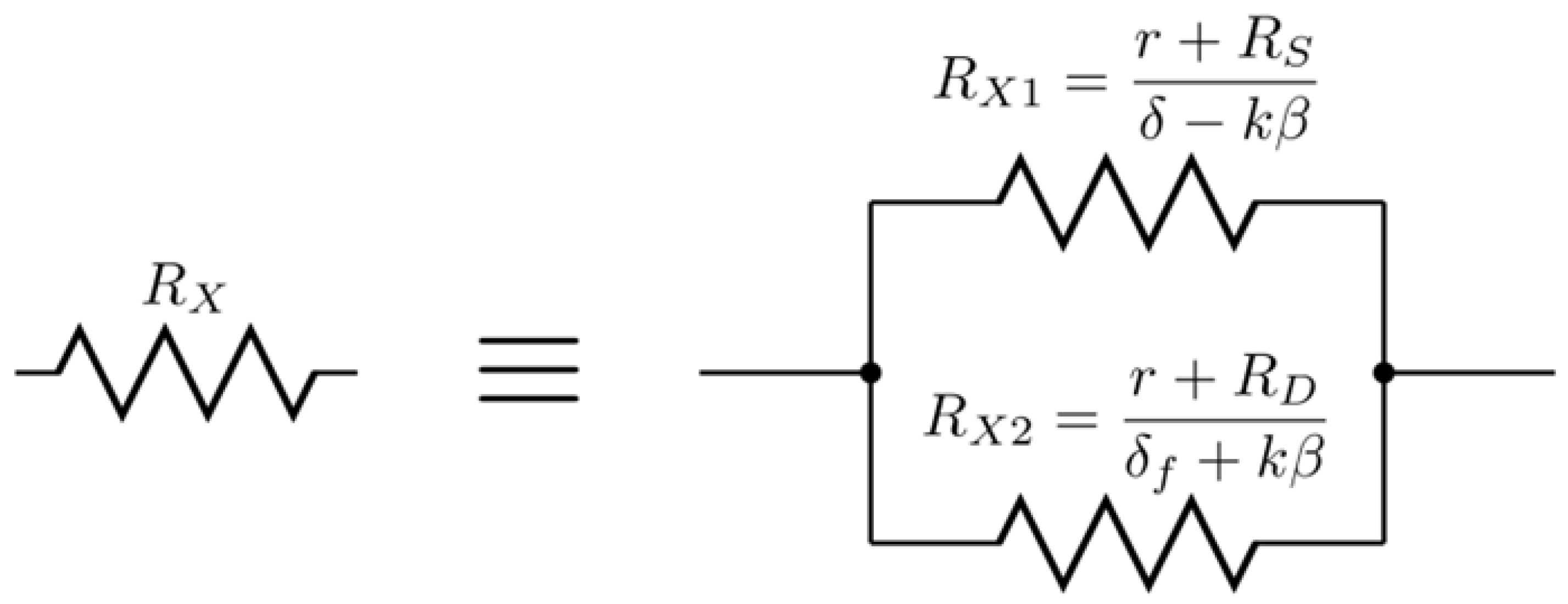
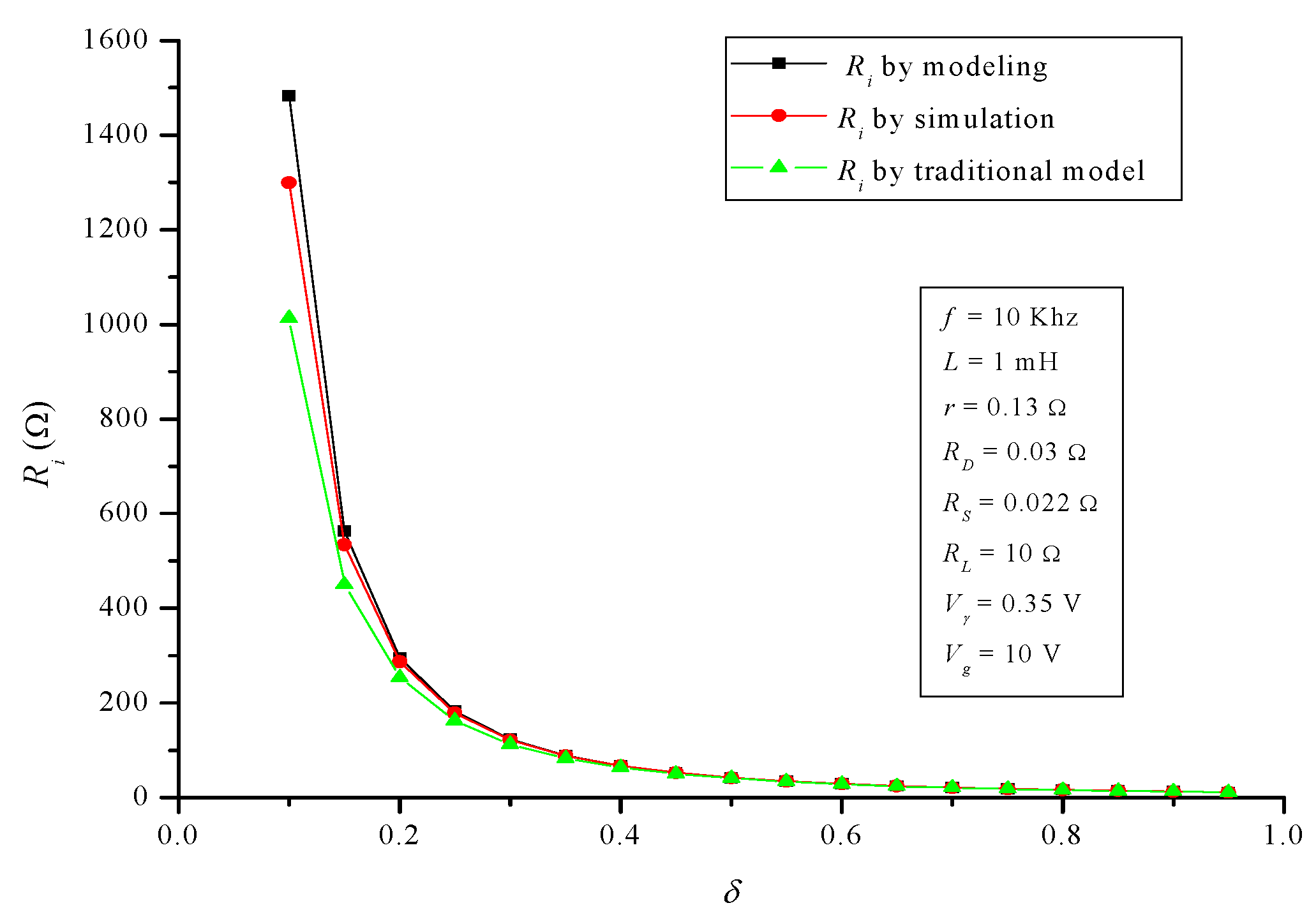
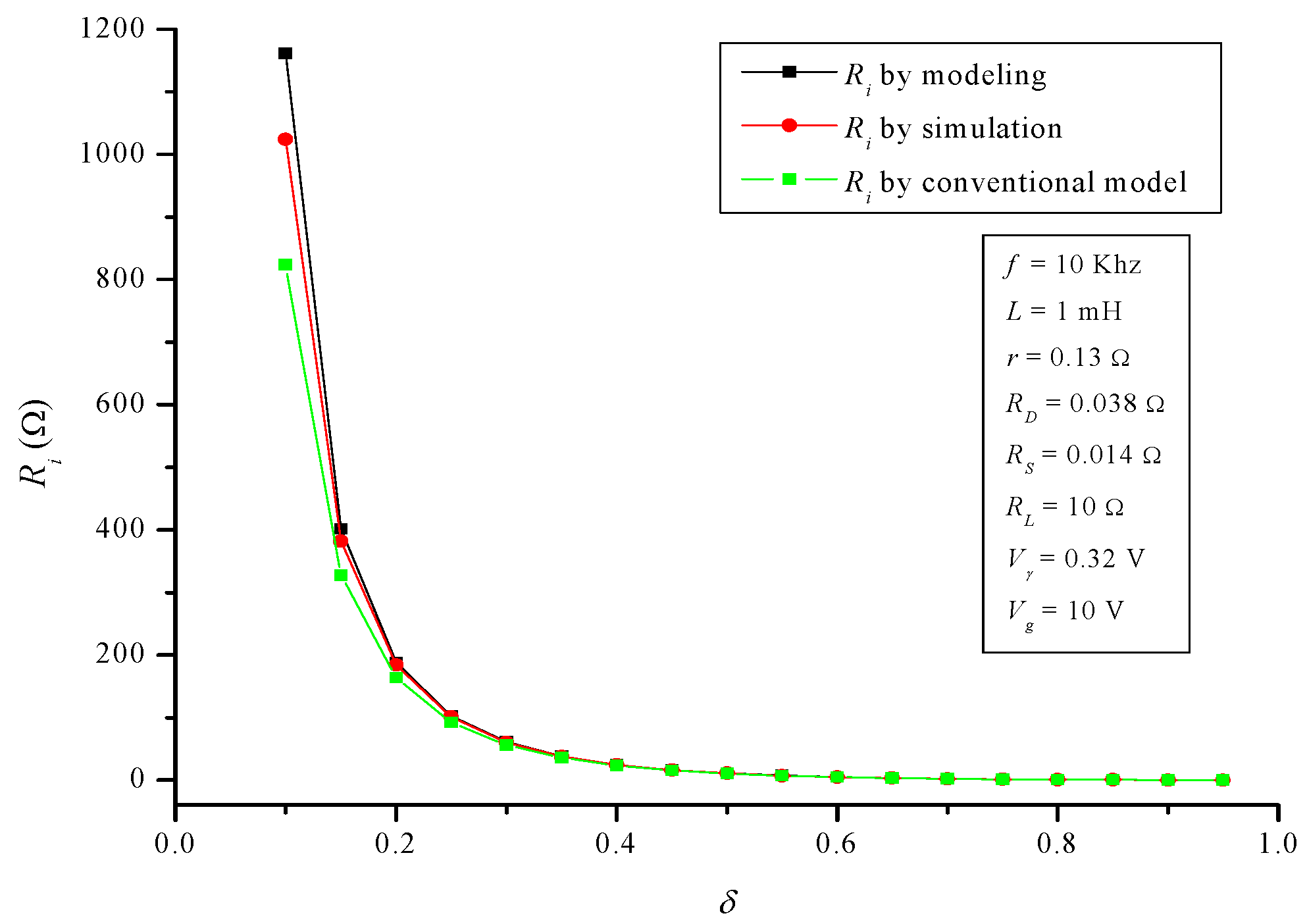
3.3. Input Resistance

This parameter is very important when the purpose of the converter is to match the generator output and load resistances. This is a typical application to achieve maximum power transfer from the generator to load. Then, from Figure 1, (40) and considering the load R_L,

$$R_i=\frac{V_g}{I_g}=R_X+\frac{V_o^{*}}{A_{Vi}{I}_g}=R_X+\frac{V_o}{A_{Vi}{I}_g}+\frac{V_X}{A_{Vi}{I}_g}=R_X+\frac{1}{A_{Vi}{}^2}\frac{V_o}{I_o}+\frac{V_X}{A_{Vi}\frac{V_g}{R_i}}=R_X+\frac{1}{A_{Vi}{}^2}{R}_L+\frac{R_i{V}_X}{A_{Vi}{V}_g}\cdot$$

(49)

Now, by solving for R_i,

$$R_i=\frac{R_X+\frac{1}{A_{Vi}{}^2}{R}_L}{1-\frac{V_X}{A_{Vi}{V}_g}}\cdot$$

(50)

where R_X, V_X and A_Vi are given by (45)–(47) respectively. Equation (50) can be approximated taking into account that usually $\left|A_{Vi}{V}_g\right|\gg \left|V_X\right|$, wherewith,

$$R_i=R_X+\frac{1}{A_{Vi}{}^2}{R}_L,$$

(51)

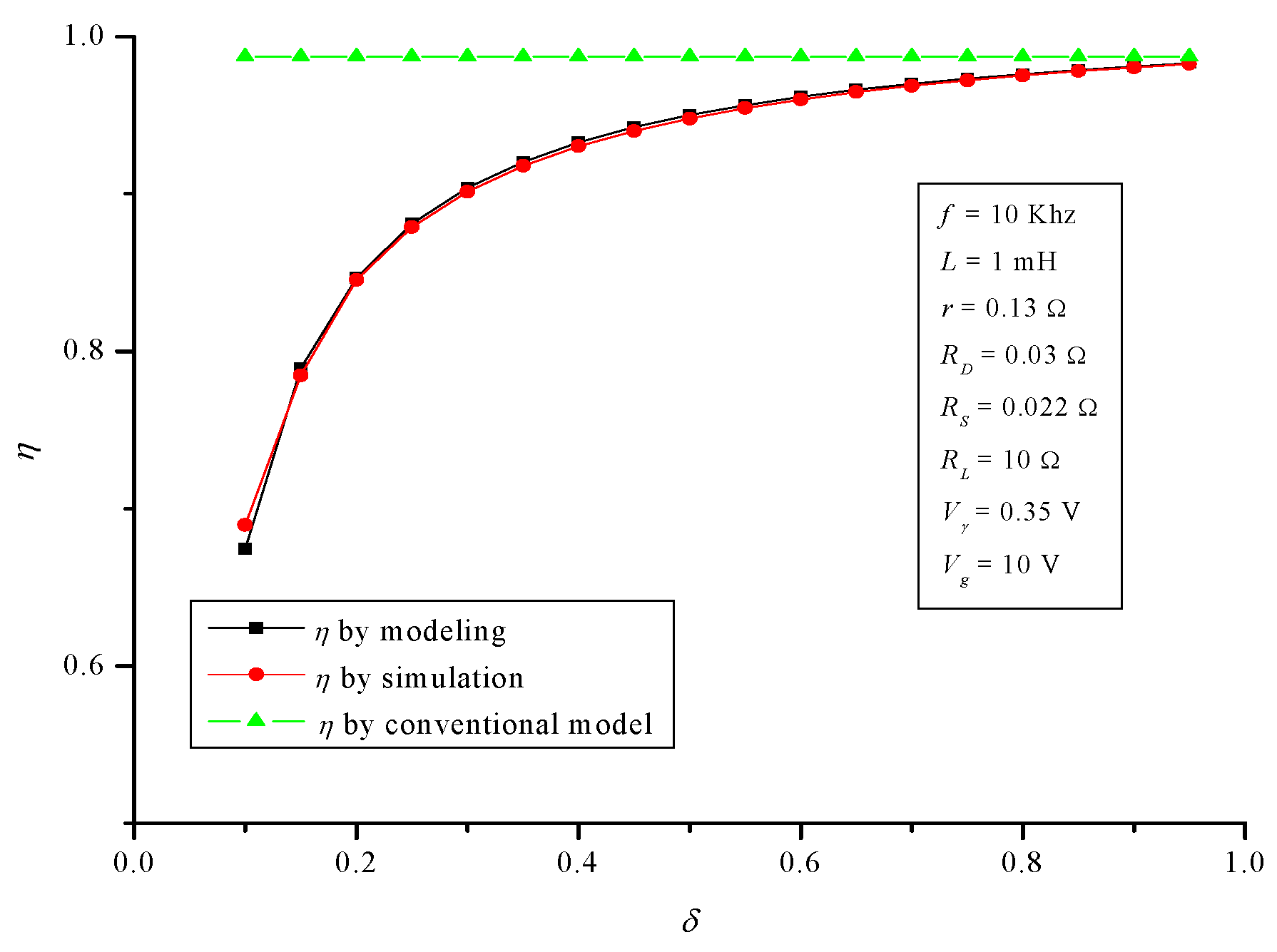
3.4. Efficiency

Efficiency η is defined as the power ratio in the load and that supplied by the generator (Figure 1):

$$\eta =\frac{P_o}{P_g}=\frac{V_o{I}_o}{V_g{I}_g}=\frac{A_{Vr}}{A_{Vi}}\cdot$$

(52)

Now, substituting A_Vr by its value given in (43):

$$\eta =\frac{1-\frac{V_X}{A_{Vi}{V}_g}}{1+A_{Vi}{}^2\frac{R_X}{R_L}}\cdot$$

(53)

where R_X, V_X and A_Vi are given by (45)–(47) respectively.

3.5. Conventional Approximate Analysis

It is interesting to show how from the exact expressions of R_X and A_Vi, (45) and (47) respectively, it is easy to get their common idealized CCM values [24,36,37,38,39]:

$${R_X|}_{CCM}=r,$$

(28)

and

$${A_{Vi}|}_{CCM}=\frac{1}{1-\delta }\cdot$$

(55)

Let us assume that at the working frequency ${\gamma }_1,{\gamma }_2\ll 1$ (see (17) and (24)). Under this premise, $e^{{\gamma }_1}\simeq 1+{\gamma }_1$ and $e^{-{\gamma }_2}\simeq 1-{\gamma }_2$. Substituting these values in (32):

$${\beta |}_{{}_{{\gamma }_1,{\gamma }_2\ll 1}}\simeq -\frac{{\gamma }_1{\gamma }_2}{{\gamma }_1+{\gamma }_2}\cdot$$

(56)

Keep in mind that being ${\gamma }_1,{\gamma }_2\ll 1$, $\beta \to 0$. On the other hand, according to (18), (25), and (35):

$$k=k_2-k_1=f\cdot L\left[\frac{1}{r+R_D}-\frac{1}{r+R_S}\right]\cdot$$

(57)

It is rather common that $\left(r+R_D\right)\simeq \left(r+R_S\right),$ which leads to $k\to 0$. Thus, $k\beta \to 0$. Them from (45):

$$R_X\simeq \frac{1}{\frac{\delta }{r+R_S}+\frac{{\delta }_f}{r+R_D}}\cdot$$

(58)

Usually $r\gg R_D$ and $r\gg R_S$, so (58) can be written as:

$$R_X\simeq \frac{r}{\delta +{\delta }_f}\cdot$$

(59)

From (47) and taking into account again that $\left(r+R_D\right)\simeq \left(r+R_S\right)$ and $k\beta \to 0,$ A_Vi can be approximated to:

$$A_{Vi}\simeq 1+\frac{\delta }{{\delta }_f}\cdot$$

(60)

In CCM $\left({\delta }_f=1-\delta \right)$, wherewith (59) and (60) lead to (54) and (55) respectively, which are the usual simplifications in the literature.

Analogously from (51) and with (59) and (60) in CCM,

$${R_i|}_{CCM}\simeq r+{\left(1-\delta \right)}^2{R}_L$$

(61)

Finally, from (53), (46) and with (59) and (60) in CCM,

$${\eta |}_{CCM}\simeq \frac{1}{1+\frac{1}{{\left(1-\delta \right)}^2}\cdot \frac{r}{R_L}}=\frac{{\left(1-\delta \right)}^2{R}_L}{{\left(1-\delta \right)}^2{R}_L+r}\cdot$$

(62)

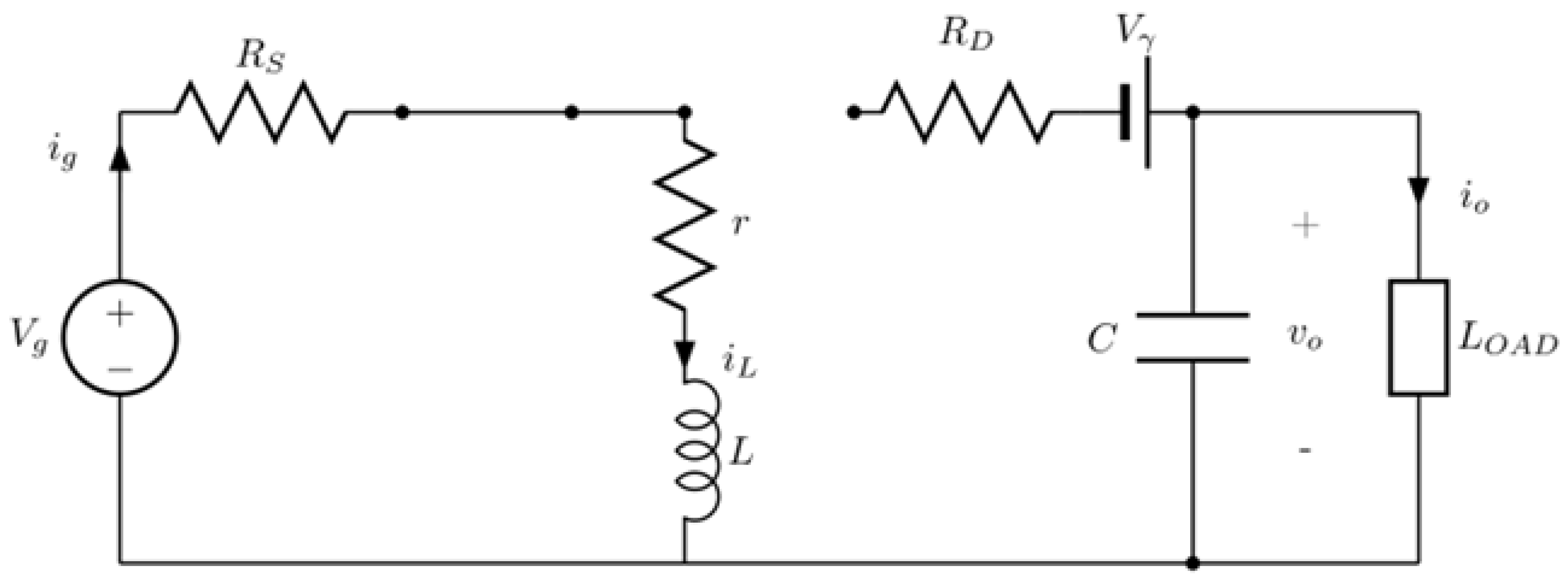
3.6. Discontinuous Conduction Mode (DCM)

In DCM (see Figure 2b), (27) will be written as:

$$\begin{array}{cc}0=\frac{V_g}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2 e^{{\gamma }_1}.\hfill & \hfill \left(\mathrm{a}\right)\\ I_2=\frac{V_g-\left(V_o+V_{\gamma }\right)}{r+R_D}\left(1-e^{{\gamma }_2}\right).\hfill & \hfill \left(\mathrm{b}\right)\\ \Delta I=I_2.\hfill & \hfill \left(\mathrm{c}\right)\end{array}$$

(63)

By solving (63a) for I₂ and substituting the result in (63b),

$${{\gamma }_2|}_{DCM}=\ln \left[1-\frac{V_g}{V_g-\left(V_o+V_{\gamma }\right)}\cdot \frac{r+R_D}{r+R_S}\left(1-e^{-{\gamma }_1}\right)\right].$$

(64)

From ${\gamma }_2$ given by (24), it is easy to get the duty cycle value $\left({\delta }_{f-crit}\right)$ that sets the boundary between DCM and CCM:

$${\delta }_{f-crit}=k_2\cdot \ln \left[1-\frac{V_g}{V_g-\left(V_o+V_{\gamma }\right)}\cdot \frac{k_1}{k_2}\left(1-e^{-{\gamma }_1}\right)\right].$$

(65)

4. Buck Converter

Figure 7 shows a buck converter with its main non-idealities.

4.1. Determination of Generator-Supplied Current

We are going to analyze the behavior of the converter in the time intervals considered in (11). Now, in both operation modes, the mean current supplied by the generator within a given time period T (see Figure 2 and (11)) is

$$I_g=\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_g dt}=\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_L dt}.$$

(66)

A similar analysis to Section 3 will then be carried out.

0 ≤ t ≤ T_ON

In this time interval current through the inductor grows, the diode is reversing biased and its branch is disconnected. Figure 8 shows the equivalent circuit.

Reasoning in Figure 8 like from (13) to (14), but now for $\Delta V=V_g-V_0$,

$$I_g=\frac{\Delta V}{r+R_S}\delta -\frac{f\cdot L}{r+R_S}\Delta I.$$

(67)

Now, operating like from (15) to (19) it comes to

$$I_1=\frac{\Delta V}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2 e^{{\gamma }_1}.$$

(68)

T_ON ≤ t ≤ T_X

Now the generator branch is disconnected. Figure 9 shows the equivalent circuit.

Reasoning on Figure 9 like from (20) to (21),

$$\frac{1}{T}{\displaystyle \underset{T_{ON}}{\overset{T_X}{\int }}{i}_L dt}=-\frac{V_0+V_{\gamma }}{r+R_D}{\delta }_f+\frac{f\cdot L}{r+R_D}\Delta I.$$

(69)

Now, operating like from (22) to (26),

$$I_2=-\frac{V_0+V_{\gamma }}{r+R_D}\left(1-e^{{\gamma }_2}\right)+I_1 e^{{\gamma }_2}.$$

(70)

At this point, as in the step to (27), the equation system formed by (68), (70), and (6) is suggested:

$$I_1=\frac{\Delta V}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2{e}^{{\gamma }_1}.$$

(71a)

$$I_2=-\frac{V_0+V_{\gamma }}{r+R_D}\left(1-e^{{\gamma }_2}\right)+I_1 e^{{\gamma }_2}.$$

(71b)

$$\Delta I=I_2-I_1.$$

(71c)

Solving (71) in the same way that from (27) to (31):

$$\Delta I=-\beta \left(\frac{V_0+V_{\gamma }}{r+R_D}+\frac{\Delta V}{r+R_S}\right),$$

(72)

with β given in (32).

Now we can calculate the current supplied by the generator in the interval that “sees” the load. Therefore, substituting (72) into (67),

$$I_g=\frac{\Delta V}{r+R_S}\delta +\frac{f\cdot L}{r+R_S}\beta \left(\frac{\Delta V}{r+R_S}+\frac{V_0+V_{\gamma }}{r+R_D}\right)\cdot$$

(73)

Taking into account k₁ and k₂ values from (18) and (25) respectively, ΔV from (7) and considering (35),

$$I_g=\frac{V_g}{r+R_S}\left(\delta +k_1\beta \right)-\frac{V_o}{r+R_S}\left(\delta -k\beta \right)+\frac{k_2\beta V_{\gamma }}{r+R_S}.$$

(74)

4.2. Loss Resistance, Loss Voltage and Voltage Gain Determination

With the aim of identifying R_X, V_X and A_Vi according to the converter parameters given in Figure 1, we proceeded as follows. Comparing (44) and (74) the following is obtained:

$$R_X=\frac{r+R_S}{\delta +k_1\beta },$$

(75)

$$V_X=\left[\frac{k_2\beta }{k\beta -\delta }\right]V_{\gamma },$$

(76)

and

$$A_{Vi}=\frac{\delta +k_1\beta }{\delta -k\beta }\cdot$$

(77)

Substituting (75)–(77) in (43), it is immediate to calculate the converter actual voltage gain, A_vr.

4.3. Input Resistance

It is immediate, simply replace R_X, V_X and A_Vi from (75)–(77), respectively, in (50).

$$R_i=\frac{\frac{r+R_S}{\delta +k_1 \beta }+{\left(\frac{\delta -k\beta }{\delta +k_1 \beta }\right)}^2{R}_L}{1+\frac{k_2 \beta }{\delta +k_1 \beta }\frac{V_{\gamma }}{V_g}}.$$

(78)

4.4. Efficiency

Again it is immediate, simply replace R_X, V_X and A_Vi from (75) to (77), respectively, in (53).

$$\eta =\frac{1+\frac{k_2 \beta }{\delta +k_1 \beta }\cdot \frac{V_{\gamma }}{V_g}}{1+\frac{\delta +k_1 \beta }{{\left(\delta -k\beta \right)}^2}\frac{r+R_S}{R_L}}\cdot$$

(79)

4.5. Conventional Approximate Analysis

Assume that at the working frequency, β can be approximated like in (56), whereby

$${k_1 \beta |}_{{\gamma }_1,{\gamma }_2\ll 1}\simeq -k_1\frac{{\gamma }_1 {\gamma }_2}{{\gamma }_1+{\gamma }_2}=-k_1\frac{\frac{\delta }{k_1}\cdot \frac{{\delta }_f}{k_2}}{\frac{\delta }{k_1}+\frac{{\delta }_f}{k_2}}=-\frac{\frac{\delta {\delta }_f}{k_2}}{\frac{\delta }{k_1}+\frac{{\delta }_f}{k_2}}\cdot$$

(80)

As in Section 3.5 (reasoning after (57)), under the commonest operating conditions $k_1\cong k_2$ and $k\to 0,$ you have to

$$k_1\beta \simeq -\frac{\delta {\delta }_f}{\delta +{\delta }_f}\cdot$$

(81)

Then, (75) and (77) can be approximated respectively to:

$$R_X\simeq \frac{\delta +{\delta }_f}{{\delta }^2}\left(r+R_S\right)\simeq \frac{\delta +{\delta }_f}{{\delta }^2}r,$$

(82)

and

$$A_{Vi}\simeq 1-\frac{{\delta }_f}{\delta +{\delta }_f}\cdot$$

(83)

Finally, regarding (76), considering in this order: (25), (56) with (17) and (24), and (35) with (18), it can be rewritten as:

$$V_X\simeq \frac{{\delta }_f}{\delta +{\delta }_f}{V}_{\gamma }$$

(84)

If the converter works in CCM $\left({\delta }_f=1-\delta \right),$ (82) and (83) lead to the literature common expressions [24,36,37,38,39]:

$${R_X|}_{CCM}\simeq \frac{1}{{\delta }^2}\left(r+R_S\right)\simeq \frac{r}{{\delta }^2},$$

(85)

and

$${A_{Vi}|}_{CCM}\simeq \delta .$$

(86)

On the other hand, substituting (82) and (83) in (51):

$$R_i\simeq \frac{\delta +{\delta }_f}{{\delta }^2}r+{\left(\frac{\delta +{\delta }_f}{\delta }\right)}^2{R}_L,$$

(87)

that for CCM condition $\left({\delta }_f=1-\delta \right)$ leads to:

$${R_i|}_{CCM}\simeq \frac{r+R_L}{{\delta }^2}\cdot$$

(88)

Similarly, (84) can be written in CCM as:

$$V_X\cong \left(1-\delta \right)V_{\gamma }.$$

(89)

Regarding approximate efficiency determination, it is enough to substitute (82)–(84) in (53) with the condition that $\left|A_{Vi}{V}_g\right|\gg \left|V_{\gamma }\right|$:

$$\eta \simeq \frac{1}{1+\frac{r}{\left(\delta +{\delta }_f\right)R_L}}\cdot$$

(90)

This efficiency for CCM condition can be written as:

$${\eta |}_{CCM}\simeq \frac{1}{1+\frac{r}{R_L}}\cdot$$

(91)

4.6. Discontinuous Conduction Mode (DCM)

For this mode, (71) is expressed as follows:

$$0=\frac{\Delta V}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2{e}^{{\gamma }_1}.$$

(92a)

$$I_2=-\frac{V_0+V_{\gamma }}{r+R_D}\left(1-e^{{\gamma }_2}\right).$$

(92b)

$$\Delta I=I_2.$$

(92c)

By solving (92a) for I₂ and substituting the result in (92b),

$${{\gamma }_2|}_{DCM}=\ln \left[1+\frac{\Delta V}{V_0+V_{\gamma }}\cdot \frac{r+R_D}{r+R_S}\left(1-e^{-{\gamma }_1}\right)\right],$$

(93)

Finally, from ${\gamma }_2$ value given by (24), the duty cycle value $\left({\delta }_{f-crit}\right)$ that sets the boundary between DCM and CCM can be calculated from (93) as

$${\delta }_{f-crit}=k_2\cdot \ln \left[1+\frac{\Delta V}{V_0+V_{\gamma }}\cdot \frac{k_1}{k_2}\left(1-e^{-{\gamma }_1}\right)\right].$$

(94)

where k₁ and k₂ are given by (18) and (25) respectively.

5. Buck-Boost Converter

Figure 10 shows a buck-boost converter that includes its main non-idealities (losses).

5.1. Determination of Generator-Supplied Current

In Figure 10, the mean current supplied by the generator within a given time period T (see Figure 2 and (11)) is:

$$I_g=\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_g dt}=\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_L dt}.$$

(95)

Next we will proceed like in the previous sections.

0 ≤ t ≤ T_ON

In this time interval the diode branch is disconnected. Figure 11 shows the equivalent circuit.

Reasoning in Figure 11 for V_g as was done from (13) to (14):

$$I_g=\frac{1}{T}{\displaystyle \underset{0}{\overset{T_{ON}}{\int }}{i}_L dt}=\frac{V_g}{r+R_S}\delta -\frac{f\cdot L}{r+R_S}\Delta I.$$

(96)

Equation (96) is analogous to (14), wherewith and as might be expected, (97) is also (19):

$$I_1=\frac{V_g}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2 e^{{\gamma }_1}.$$

(97)

T_ON ≤ t ≤ T_X

Now the generator branch is disconnected. Figure 12 shows the equivalent circuit.

Reasoning on Figure 12 like from (20) to (21),

$$\frac{1}{T}{\displaystyle \underset{T_{ON}}{\overset{T_X}{\int }}{i}_L dt}=\frac{V_o-V_{\gamma }}{r+R_D}{\delta }_f+\frac{f\cdot L}{r+R_D}\Delta I.$$

(98)

Now, operating like from (22) to (26),

$$I_2=\frac{V_0-V_{\gamma }}{r+R_D}\left(1-e^{{\gamma }_2}\right)+I_1 e^{{\gamma }_2}.$$

(99)

As we did in (27), It is now suggests the equation system comprising (97), (99) and (6):

$$I_1=\frac{V_g}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2 e^{{\gamma }_1}.$$

(100a)

$$I_2=\frac{V_0-V_{\gamma }}{r+R_D}\left(1-e^{{\gamma }_2}\right)+I_1 e^{{\gamma }_2}.$$

(100b)

$$\Delta I=I_2-I_1.$$

(100c)

By solving (100) in the same way that from (27) to (31):

$$\Delta I=\beta \left(\frac{V_0-V_{\gamma }}{r+R_D}-\frac{V_g}{r+R_S}\right),$$

(101)

with β given in (32).

Now we can calculate the current supplied by the generator. So substituting (101) into (96),

$$I_g=\frac{V_g}{r+R_S}\delta -\frac{f\cdot L}{r+R_S}\beta \left(\frac{V_0-V_{\gamma }}{r+R_D}-\frac{V_g}{r+R_S}\right)\cdot$$

(102)

Taking into account k₁ and k₂ values from (18) and (25) respectively,

$$I_g=\frac{V_g}{r+R_S}\left(\delta +k_1 \beta \right)-\frac{ V_0}{r+R_S}{k}_2 \beta +\frac{V_{\gamma }}{r+R_S}{k}_2 \beta$$

(103)

5.2. Loss Resistance, Loss Voltage and Voltage Gain Determination

With the aim of identifying R_X, V_X and A_Vi according to the converter parameters given in Figure 1, we are going to proceed as follows. Comparing (44) and (103), the following is obtained:

$$R_X=\frac{r+R_S}{\delta +k_1 \beta },$$

(104)

$$V_X=-V_{\gamma },$$

(105)

and

$$A_{Vi}=\frac{\delta +k_1 \beta }{k_2 \beta }\cdot$$

(106)

5.3. Input Resistance

Substituting R_X, V_X and A_Vi from (104)–(106), respectively, in (50):

$$R_i=\frac{\frac{r+R_S}{\delta +k_1\beta }+{\left(\frac{k_2\beta }{\delta +k_1\beta }\right)}^2{R}_L}{1+\frac{k_2\beta }{\delta +k_1\beta }\cdot \frac{V_{\gamma }}{V_g}}.$$

(107)

5.4. Efficiency

Substituting R_X, V_X and A_Vi from (104)–(106), respectively, in (53):

$$\eta =\frac{1+\frac{k_2 \beta }{\delta +k_1 \beta }\cdot \frac{V_{\gamma }}{V_g}}{1+\frac{\delta +k_1\beta }{{\left(k_2\beta \right)}^2}\cdot \frac{r+R_S}{R_L}}\cdot$$

(108)

5.5. Conventional Approximate Analysis

Assume that at the working frequency β is (56), so $k_1\beta$ is (81). This allows writing (104) as

$$R_X\simeq \frac{\delta +{\delta }_f}{{\delta }^2}\left(r+R_S\right)\simeq \frac{\delta +{\delta }_f}{{\delta }^2}r.$$

(109)

Again, taking into account in (106) that $k_1\beta$ is (81) and the fact that $k_1\cong k_2,$

$$A_{Vi}\simeq -\frac{\delta }{{\delta }_f}\cdot$$

(110)

If the converter works in CCM $\left({\delta }_f=1-\delta \right),$ (109) and (110) leads to the common expressions in literature [24,36,37,38,39]:

$${R_X|}_{CCM}\simeq \frac{r}{{\delta }^2},$$

(111)

and

$${A_{Vi}|}_{CCM}\simeq -\frac{\delta }{1-\delta }\cdot$$

(112)

On the other hand, substituting (111) and (112) in (50):

$$R_i\simeq \frac{\delta +{\delta }_f}{{\delta }^2}r+\frac{{\delta }_f^2}{{\delta }^2}{R}_L,$$

(113)

that for CCM condition can be written as

$${R_i|}_{CCM}\simeq \frac{r+{\left(1-\delta \right)}^2{R}_L}{{\delta }^2}\cdot$$

(114)

Regarding approximate efficiency determination, it is enough to substitute (105), (109) and (110) in (53) with the condition that $\left|A_{Vi}{V}_g\right|\gg \left|V_{\gamma }\right|$:

$$\eta \simeq \frac{1}{1+\frac{\delta +{\delta }_f}{{\delta }_f^2}\cdot \frac{r}{R_L}},$$

(115)

that for CCM is expressed as follows:

$${\eta |}_{CCM}\simeq \frac{1}{1+\frac{1}{{\left(1-\delta \right)}^2}\frac{r}{R_L}}\cdot$$

(116)

5.6. Discontinuous Conduction Mode (DCM)

For this mode, (100) can be written as follows:

$$0=\frac{V_g}{r+R_S}\left(1-e^{{\gamma }_1}\right)+I_2 e^{{\gamma }_1}.$$

(117a)

$$I_2=\frac{V_0-V_{\gamma }}{r+R_D}\left(1-e^{{\gamma }_2}\right).$$

(117b)

$$\Delta I=I_2.$$

(117c)

By solving (117a) for I₂ and substituting the result in (117b),

$${{\gamma }_2|}_{DCM}=\ln \left[1-\frac{V_g}{V_0-V_{\gamma }}\cdot \frac{r+R_D}{r+R_S}\left(1-e^{-{\gamma }_1}\right)\right].$$

(118)

Matching this result with (24) and taking into account (25), the duty cycle value $\left({\delta }_{f-crit}\right)$ that sets the boundary between DCM and CCM is

$${\delta }_{f-crit}=k_2\cdot \ln \left[1-\frac{V_g}{V_0-V_{\gamma }}\cdot \frac{k_1}{k_2}\left(1-e^{-{\gamma }_1}\right)\right].$$

(119)

6. Results

Now we are going to show the suitability of the developments carried out. For that, the results obtained by the theoretical study (developed model) are going to be compared with the results obtained by actual converters. In order to have a broader perspective and it be able to show in a clear way the quality of the developed model, it will be also included in the comparison with the results by the conventional model used in the literature [24,36,37,38,39]. Note, see for example (61) or (62), that the conventional model only considers a non-ideality (losses), specifically the internal inductor resistance r.

Figure 13, Figure 14 and Figure 15 show the PSpice simulation circuits for the three DC/DC power converters’ basic topologies analyzed. For simulations we have used commercial diodes and switches. This allows working with actual values of V_γ, R_D and R_S. Regarding r, it has been obtained by measuring a 1 mH inductor made in the laboratory. For example, 10 V has been chosen for V_g and 20 or 10 Ω (depending on the characteristics of the active components) for R_L. 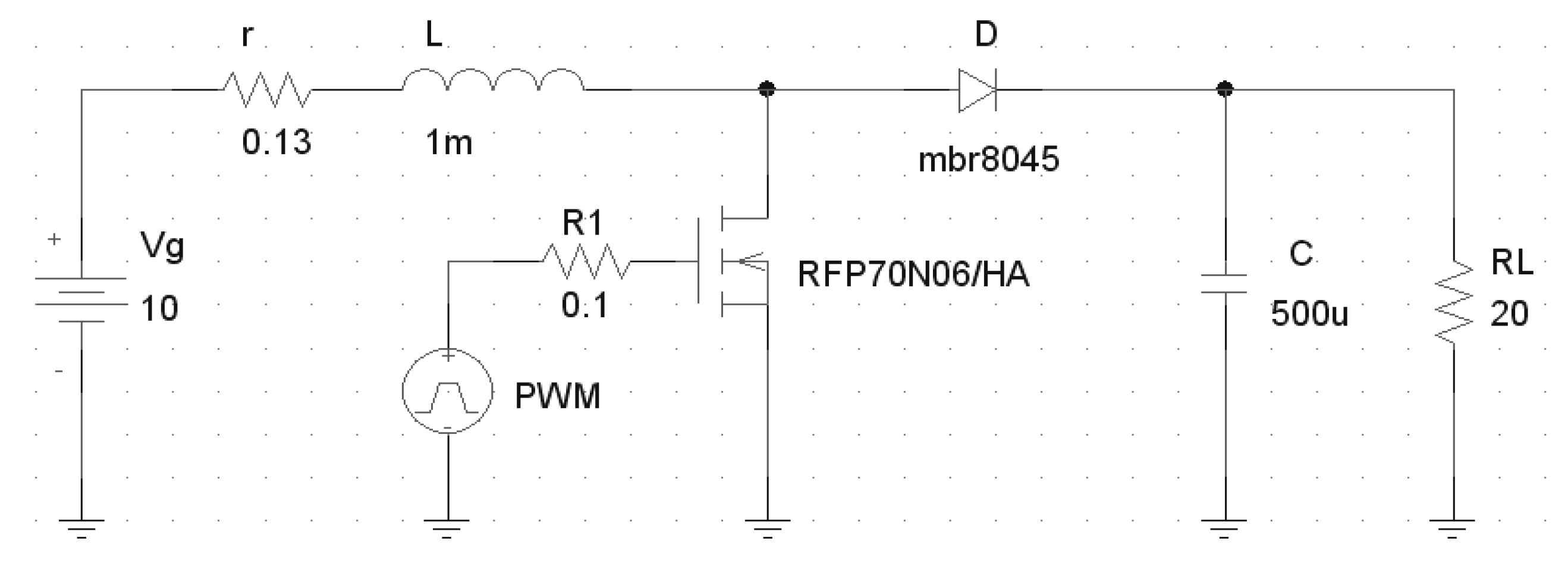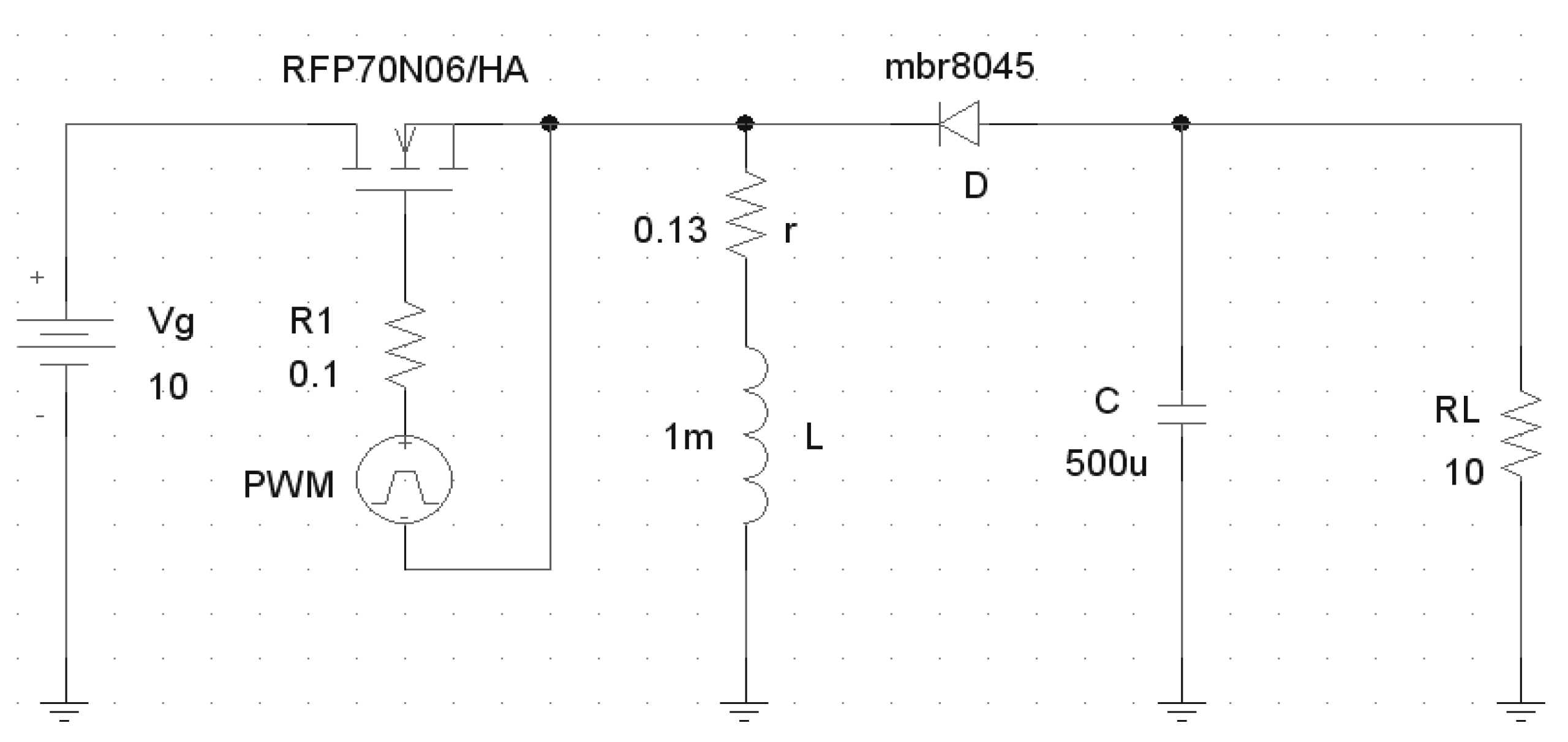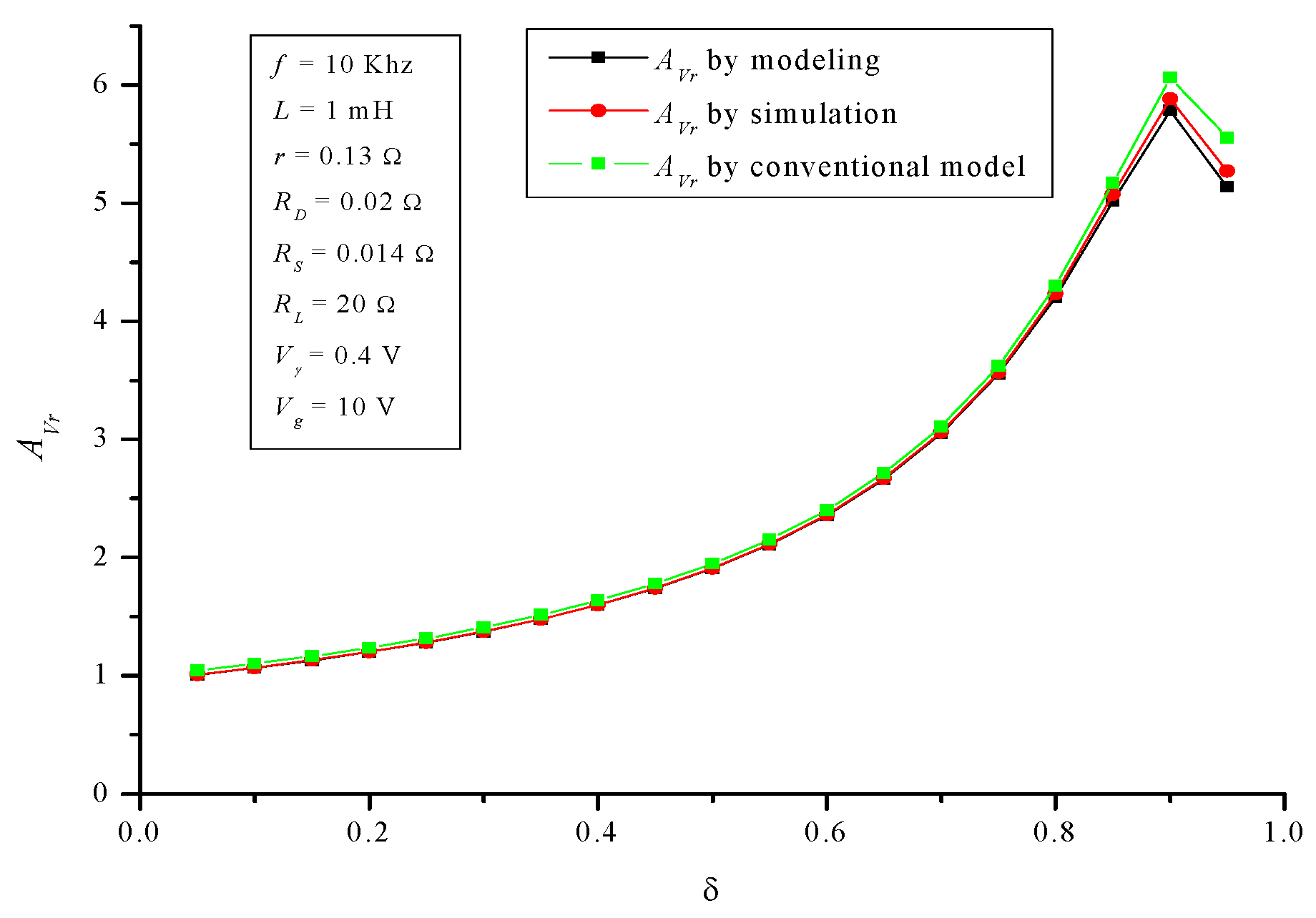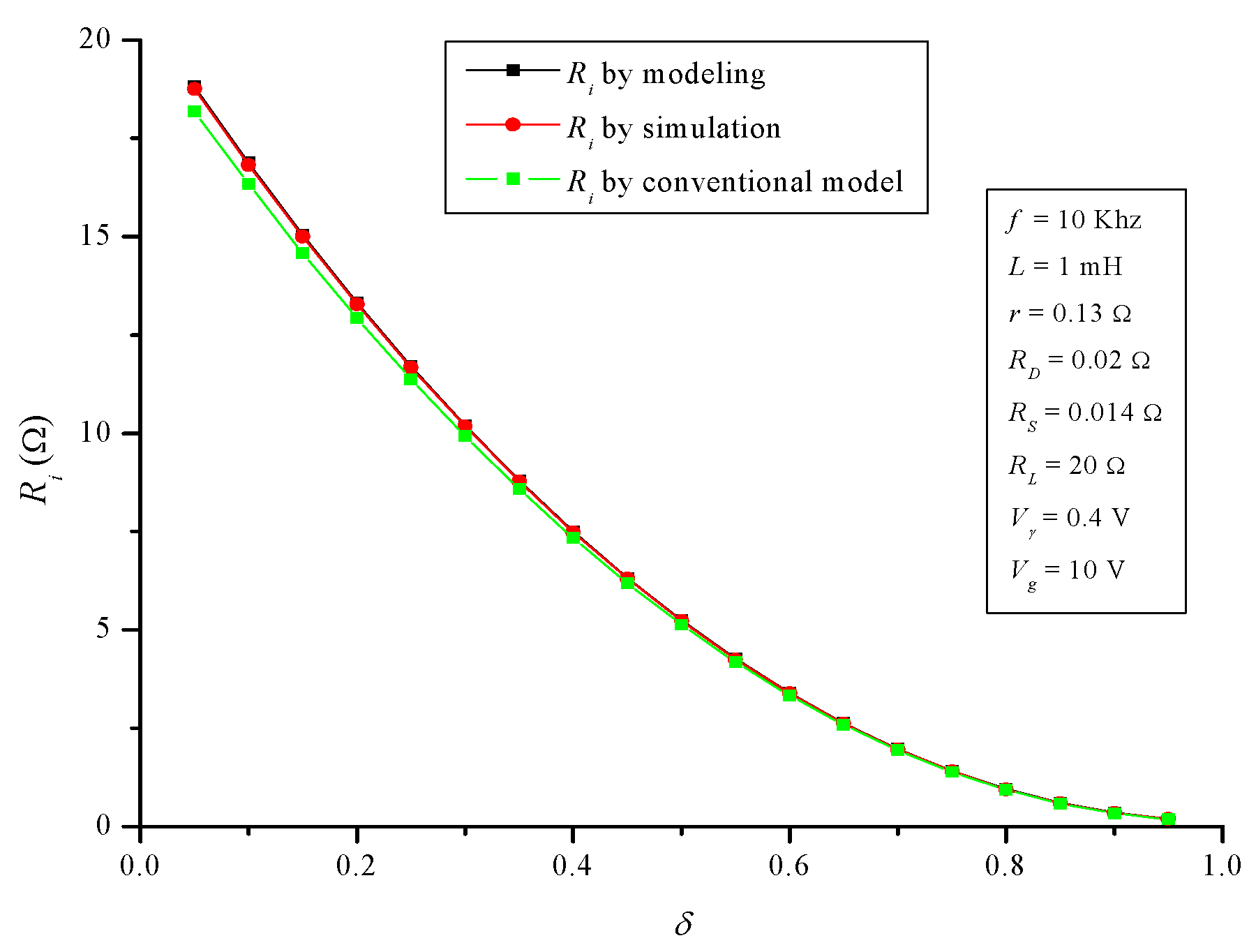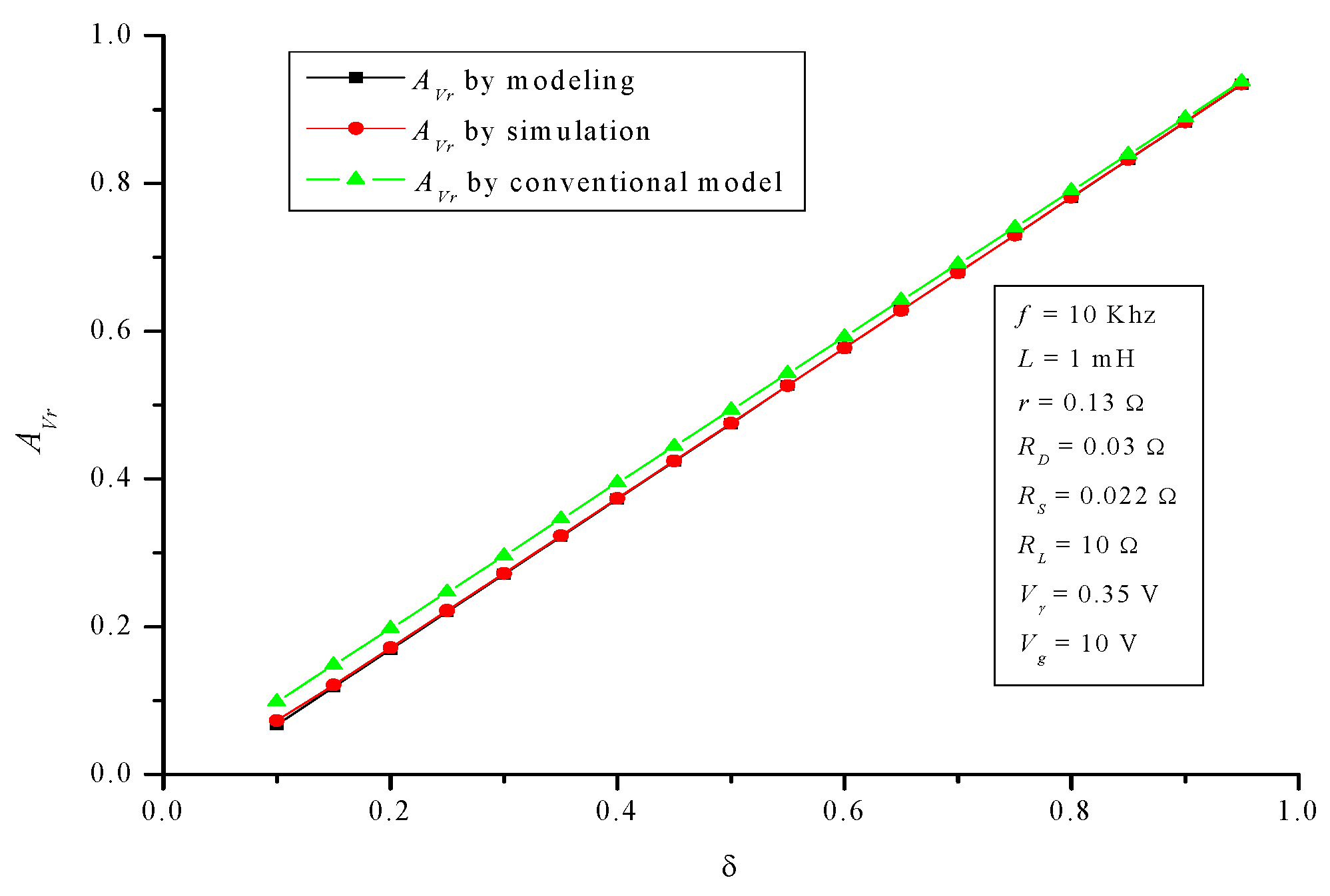Finally, we have used f = 10 kHz because it is usual in DC/DC power converters.

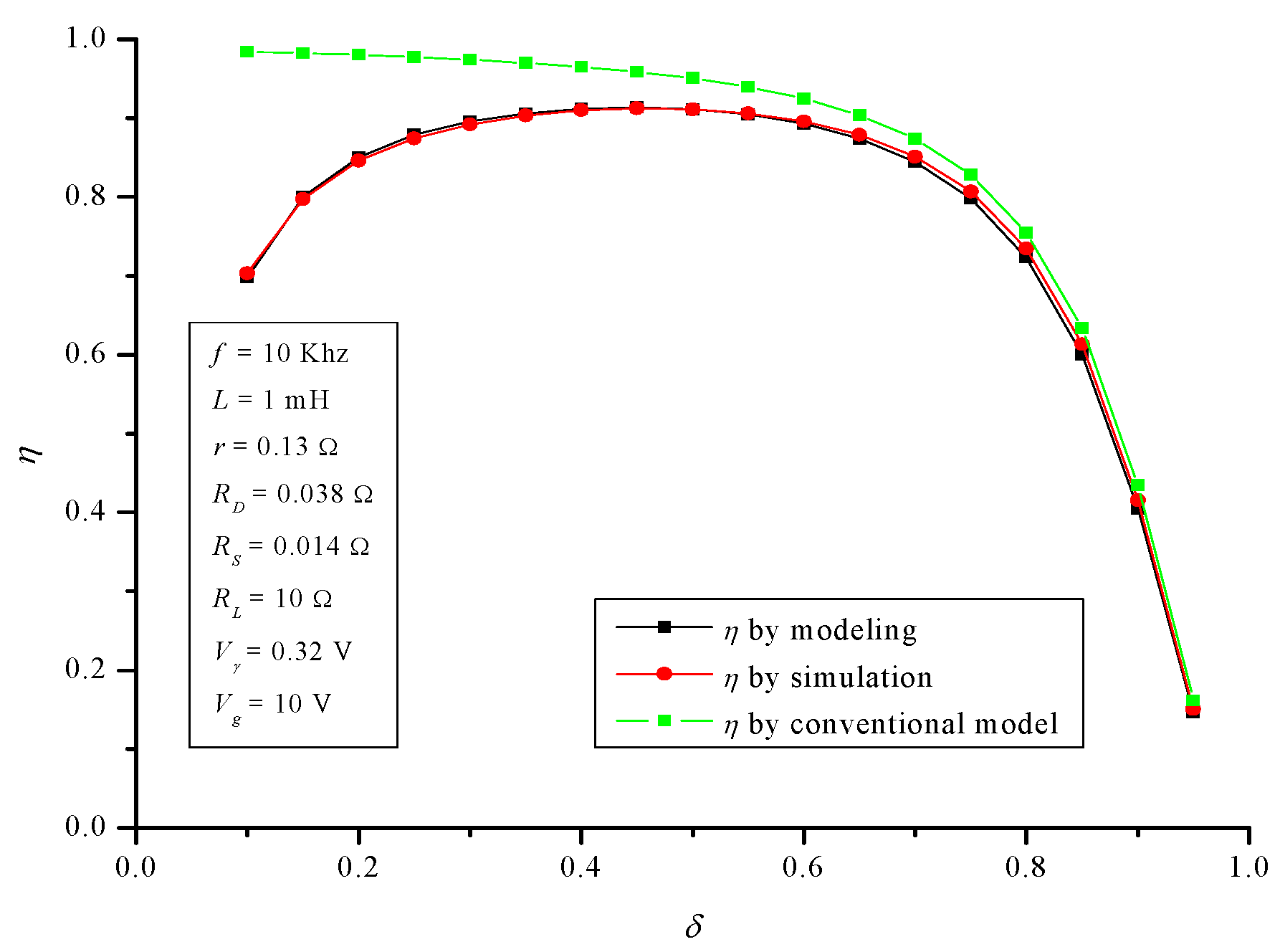
6.1. Boost Converter

Figure 16 shows the model-predicted A_Vr (introducing (45)–(47), with their respective values, into (43)) and the actual obtained by simulation (by the circuit of Figure 13).

Regarding input resistance, Figure 17 shows the model-predicted (introducing (45)–(47), with their respective values, into (50)), and by simulation.

Finally, regarding efficiency, Figure 18 shows model-predicted (introducing (45)–(47), with their respective values, into (53)), and by simulation.

6.2. Buck Converter

Following the same procedure as for the boost converter, Figure 19, Figure 20 and Figure 21 show the behavior of A_Vr, R_i and η.

6.3. Buck-Boost Converter

Figure 22, Figure 23 and Figure 24 show the behavior of A_Vr, R_i and η.

From the results shown in Figure 16, Figure 17, Figure 18, Figure 19, Figure 20, Figure 21, Figure 22, Figure 23 and Figure 24,

Table 1. NMAE% for the developed and conventional models regarding the actual converters.

Model	Converter	AVr	Ri	h
Developed	Boost	0.45%	0.66%	0.30%
	Buck	0.73%	1.61%	0.31%
	Buck-boost	1.4%	1.98%	0.84%
Conventional	Boost	2.93%	2.19%	2.69%
	Buck	7.10%	5.39%	8.09%
	Buck-boost	7.57%	5.87%	8.65%

summarizes the normalized mean absolute percent error (NMAE%) for the developed and conventional models regarding the actual converters.

Finally,

Table 2. Summary of the parameters for each DC/DC converter (see Figure 1).

Parameter	Boost	Buck	Buck-boost
RX	$\frac{1}{\frac{\delta -k\beta }{r+R_S}+\frac{{\delta }_f+k\beta }{r+R_D}}$	$\frac{r+R_S}{\delta +k_1\beta }$	$\frac{r+R_S}{\delta +k_1\beta }$
VX	$V_{\gamma }$	$\left[\frac{k_2\beta }{k\beta -\delta }\right]V_{\gamma }$	$-V_{\gamma }$
AVi	$1+\frac{r+R_D}{r+R_S}\cdot \frac{\delta -k\beta }{{\delta }_f+k\beta }$	$\frac{\delta +k_1\beta }{\delta -k\beta }$	$\frac{\delta +k_1\beta }{k_2\beta }$
δf-crit	$k_2\ln \left[1-\frac{V_g}{\Delta V-V_{\gamma }}\theta \right]$	$k_2\ln \left[1+\frac{\Delta V}{V_0+V_{\gamma }}\theta \right]$	$k_2\ln \left[1-\frac{V_g}{V_0-V_{\gamma }}\theta \right]$
where: $k_1=\frac{f\cdot L}{r+R_S}; k_2=\frac{f\cdot L}{r+R_D}; k=k_2-k_1; \beta =\frac{\left(1-e^{{\gamma }_1}\right)\cdot \left(1-e^{-{\gamma }_2}\right)}{e^{{\gamma }_1}-e^{-{\gamma }_2}}; {\gamma }_1=\frac{\delta }{k_1}; {\gamma }_2=\frac{{\delta }_f}{k_2}; {\delta }_f=\frac{T_X-T_{ON}}{T}; \Delta V=V_g-V_o; \theta =\frac{k_1}{k_2}\left(1-e^{-{\gamma }_1}\right).$
For all converters: $A_{Vr}=\frac{V_o}{V_g}=\frac{A_{Vi}-\frac{V_X}{V_g}}{1+A_{Vi}{}^2\frac{R_X}{R_L}};$$R_i=\frac{R_X+\frac{1}{A_{Vi}{}^2}{R}_L}{1-\frac{V_X}{A_{Vi}{V}_g}};$$\eta =\frac{1-\frac{V_X}{A_{Vi}{V}_g}}{1+A_{Vi}{}^2\frac{R_X}{R_L}}$

summarizes the obtained parameters of the developed model.

7. Discussion

Now we are going to discuss the results of the previous section and of the whole paper in general. As we are going to demonstrate next, the quality of the developed model (Figure 1) is excellent for the three DC/DC power converters’ basic topologies.

First of all it is important to discuss the way to obtain in the literature [24,36,37,38,39] the conventional expressions (practically ideal, h = 1, except for taking into account the internal inductor resistance r) for A_Vr, R_i and h. Authors arrive at these expressions from approximations from the beginning, specifically considering that the current through r is constant and equal to a mean value. In the developed model, you get to the same expressions (Section 3.5, Section 4.5 and Section 5.5), but without previous approximations and by the resolving of exact equations.

Analyzing Figure 16 it is easy to note how the developed model fits the voltage gain (A_Vr) of the actual boost converter. In fact, as you can see in Table 1, the (NMAE%) is negligible, only 0.45%. Figure 16 shows up the great influence of its non-idealities on an actual boost converter operation. Ideally, a boost converter presents no upper limit in the gain (55), so it can take anyone above 1. However, in the actual case, as it is well known, and the developed model is able to show, the gain is drastically modified. Although the conventional model error is also small (2.93%), it is 6.5 times greater than the developed model.

Continuing the boost converter and regarding its input resistance, Figure 17 shows again the quality of the model, NMAE% is very small, only 0,66% (2.19% in the conventional model). Finally, regarding efficiency, Figure 18 shows that NMAE is again negligible, 0.3%. Here is where the difference among the quality of the developed model and the conventional one is more notable, specifically the error of the latter is 9 times greater (2.69%). Knowing the actual efficiency of a converter is very important; in fact, in most facilities, DC/DC converters are a post-generator mandatory stage. The foregoing means that the overall efficiency of the system up to the regulated-voltage output is the product of the generator and converter efficiency. Thus, it is of great interest to know the actual DC/DC converter losses and, from them, its true efficiency.

Now, regarding the buck converter, Figure 19, Figure 20 and Figure 21 show the behavior of A_Vr, R_i and η. For the developed model, NMAE% is again negligible: 0.73%, 1.61% and 0.31% respectively. However, for the conventional model, NMAE% is 7.10%, 5.39% and 8.09% respectively. Again the differences are notable, and especially regarding efficiency, where the error is much worse than in the buck converter, specifically 26 times greater.

Finally, regarding buck-boost converter, Figure 22, Figure 23 and Figure 24 show the behavior of A_Vr, R_i and η. For the developed model, NMAE% is once again negligible: 1.4%, 1.98% and 0.84% respectively. In the case of the conventional model, NMAE% is: 7.57%, 5.87% and 8.65% respectively. Now, the efficiency error is more than 10 times greater in the conventional model regarding the developed one.

Going back to Section 3.5, Section 4.5 and Section 5.5 that attend to the idealizations, it is important to point out that in the converter literature, and also in converter teaching, it is very common to work with idealized expressions, which are of common use for students, teachers and postgraduate engineers. The developed model shows how it is possible to go in an easy way from exact expressions to idealized ones.

Continuing with the above and in view of the development carried out in Section 3, Section 4 and Section 5 of this paper, it may seem that the complexity of the modeling method may be quite high. However, in the opinion of the authors, this is not true for four reasons: (1) the approach is very intuitive; (2) the laws of circuit theory that have been applied are very simple and known; (3) the mathematical analysis used is within the scope of any student of engineering degree; and (4) the methodology and steps are identical for the three converter topologies. Moreover, one might think that for practical converters, the difference between the results of the accurate analysis carried out in this paper and the conventional approximate analysis is too small to justify the extra analytical effort (the results are shown in Table 1). Well, this can be true in some cases (especially in converters that work in a small range of their duty cycle), but not always, and in any case, the use of the developed model is very easy in practice, because it does not require any mathematical development. It is enough to apply directly to Figure 1 the parameter values that have been developed in the paper, depending on the converter, which has been summarized in Table 2. The parameter values can be obtained immediately from the data sheets of the converter components (no measurements are needed).

Finally, regarding the capacitor value C in the three topologies, it has been assumed to be large enough and with negligible losses (very low equivalent series resistance, ESR). This has allowed the consideration of a practically constant output voltage $V_0$. The ESR is inversely proportional to the capacity and today, for typical capacities of C in power converters applications, it is easy to find capacitors with ESR < 0.010 Ω.

8. Conclusions

An intuitive approach to model the steady state regime of the three DC/DC power converters’ basic topologies (buck, boost and buck-boost) has been developed in this work. This approach is new because it is not present in the literature. Besides, the developed model is common (it is suitable for the three basic topologies), realistic (it includes the main non-idealities (losses) of the active and passive components of the three topologies), accurate (the behavior of the real converter and its model are very close) and practical (it demands no measurements and just needs to have the data provided by the manufacturers’ data sheets of the converter components).

The developed model transforms a complex system with strong non-idealities in the form of distributed parameters, into a simple and intuitive scheme of concentrated parameters, which accurately reflect the actual behavior of the converters components.

The quality of the developed model has been checked by two independent lines. The first has been to probe how the relationships developed in this work can lead to the approximations commonly used in literature. The second has demonstrated how the behavior of the developed model matches the behavior of an actual converter in any of the studied basic structures. Moreover, the developed model has been subjected to a battery of comparatives for the three types of basic converters regarding the conventional model used until now in the literature. The results are summarized in Table 1 and the conclusion can be that the quality of the developed model is excellent and it far exceeds the conventional model.

As a future work that in fact it is already running, authors of this manuscript are working to try to extend the approach presented in this paper to other DC/DC converters topologies.