Front-End Light Source for aWaveform-Controlled High-Contrast Few-Cycle Laser System for High-Repetition Rate Relativistic Optics
Abstract
:1. Introduction
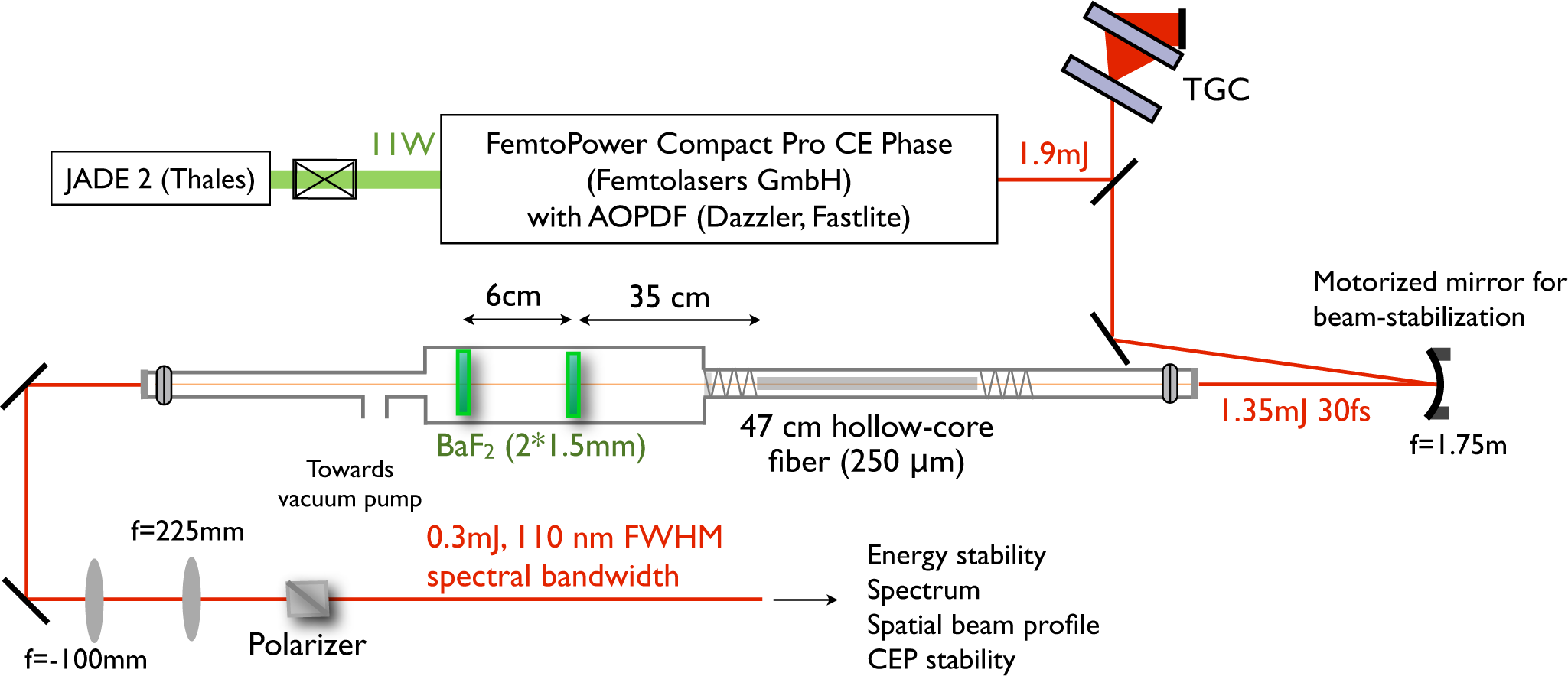
2. Experimental Setup
3. Characterization of Front-End Light Source Performance
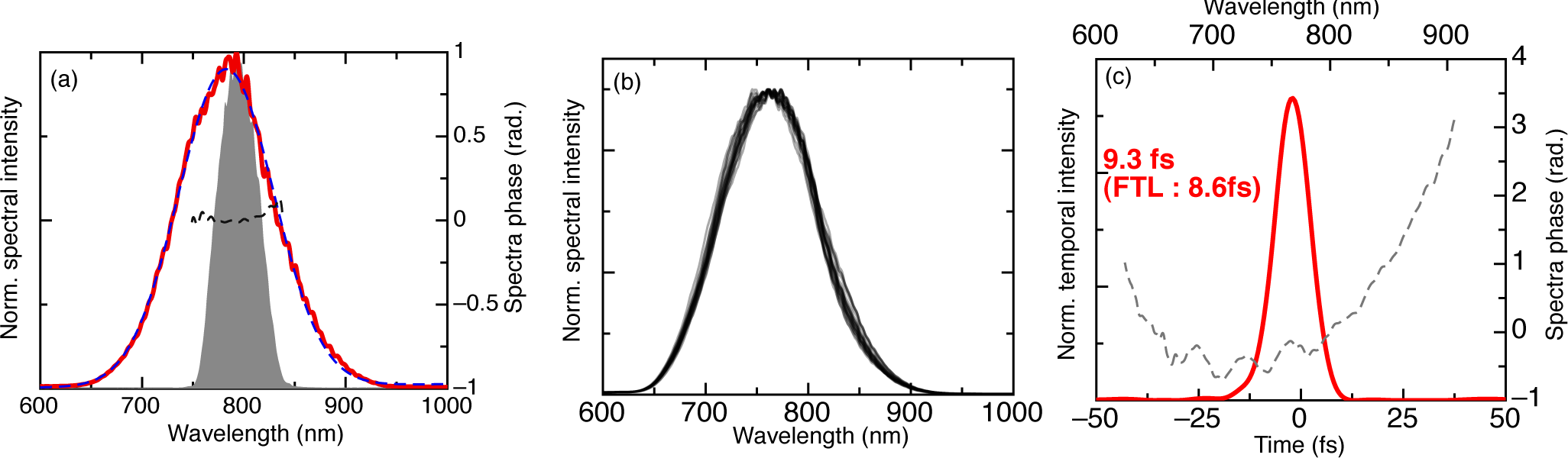
3.1. Spectro-Temporal Characterization
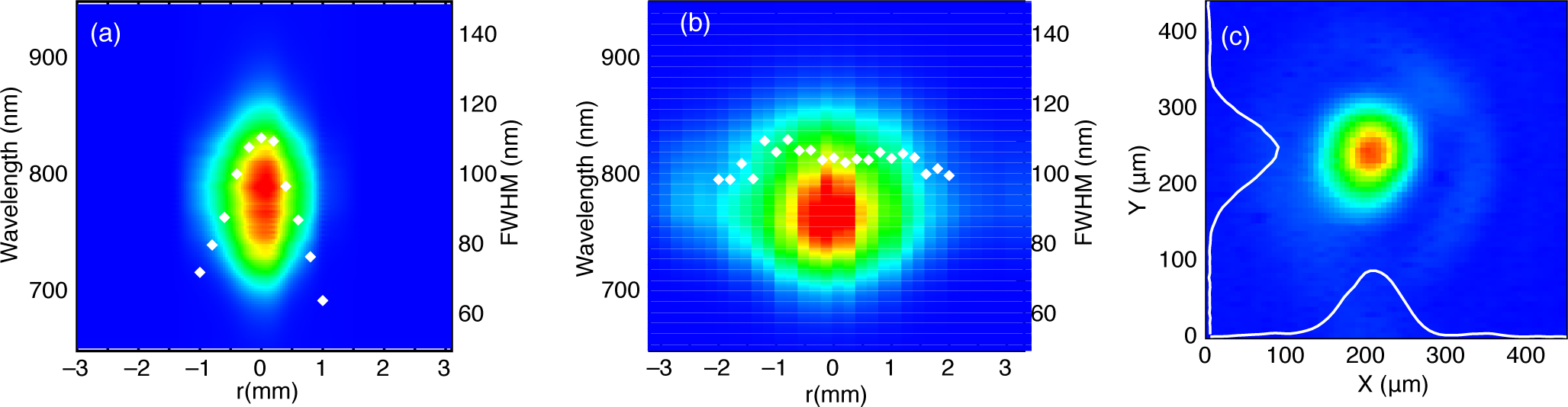
3.2. Spectro-Spatial Characterization
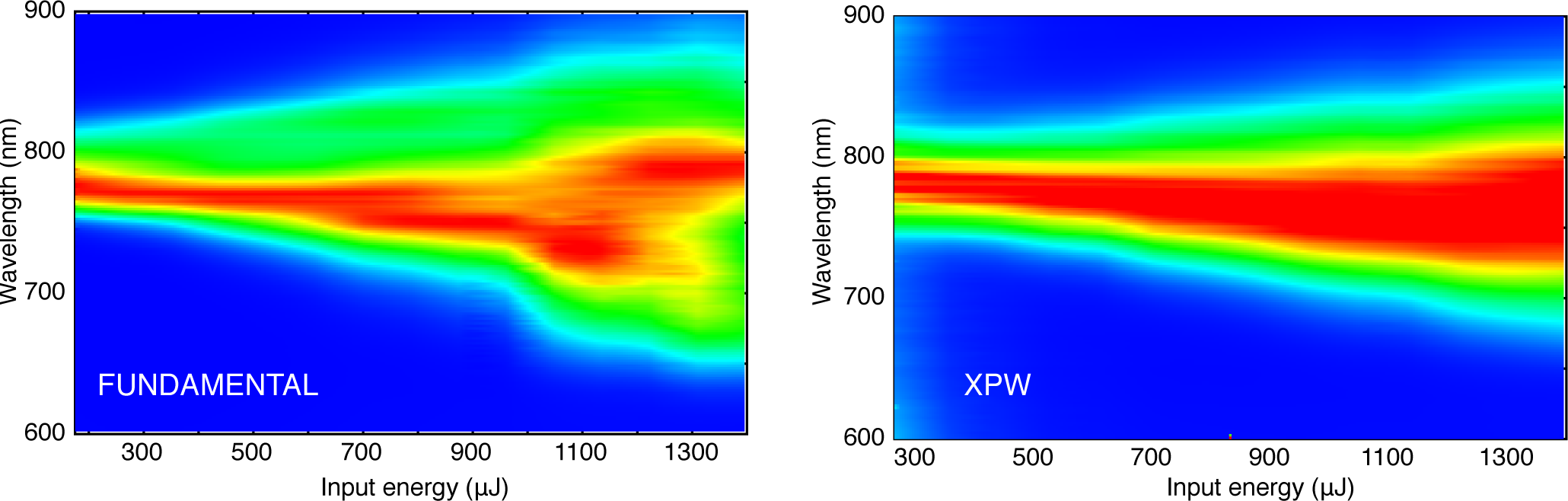
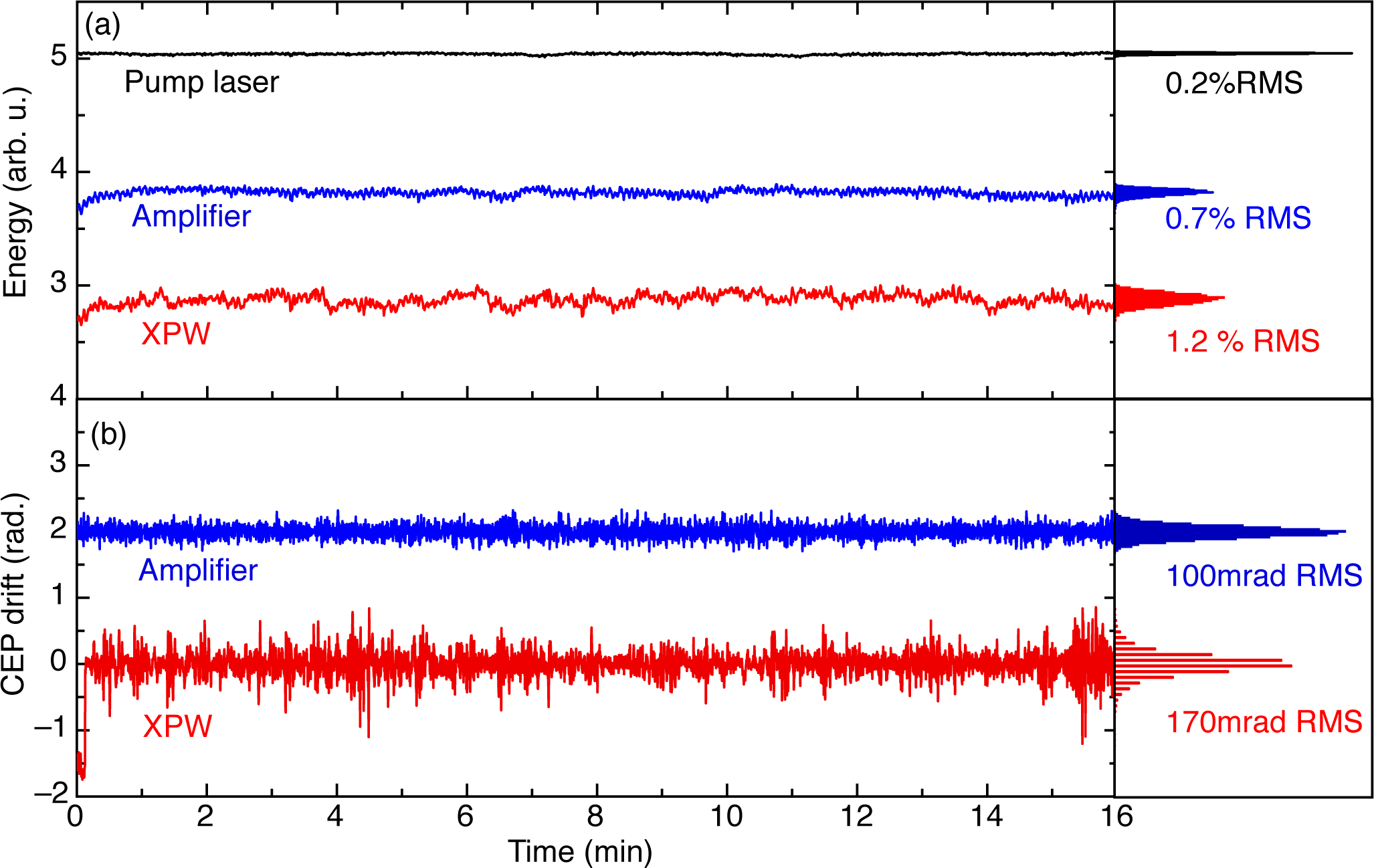
4. Fidelity of the Front-End
5. Conclusions
Acknowledgments
References
- Krausz, F.; Ivanov, M. Attosecond physics. Rev. Mod. Phys. 2009, 81, 163–234. [Google Scholar]
- Schmid, K.; Veisz, L.; Tavella, F.; Benavides, S.; Tautz, R.; Herrmann, D.; Buck, A.; Hidding, B.; Marcinkevicius, A.; Schramm, U.; et al. Few-cycle laser-driven electron acceleration. Phys. Rev. Lett. 2009, 102. [Google Scholar] [CrossRef]
- Buck, A.; Nicolai, M.; Schmid, K.; Sears, C.M.S.; Savert, A.; Mikhailova, J.M.; Krausz, F.; Kaulza, M.C.; Veisz, L. Real-time observation of laser-driven electron acceleration. Nat. Phys. 2011, 7, 543–548. [Google Scholar]
- Heissler, P.; Hörlein, R.; Mikhailova, J.M.; Waldecker, L.; Tzallas, P.; Buck, A.; Schmid, K.; Sears, C.M.S.; Krausz, F.; Veisz, L.; et al. Few-cycle driven relativistically oscillating plasma mirrors: A source of intense isolated attosecond pulses. Phys. Rev. Lett. 2012, 108, 235003. [Google Scholar]
- Borot, A.; Malvache, A.; Chen, X.; Jullien, A.; Geindre, J.-P.; Audebert, P.; Mourou, G.; Quéré, F.; Lopez-Martens, R. Attosecond control of collective electron motion in plasmas. Nat. Phys. 2012, 8. [Google Scholar] [CrossRef]
- Wheeler, J.A.; Borot, A.; Monchoce, S.; Vincenti, H.; Ricci, A.; Malvache, A.; Lopez-Martens, R.; Quere, F. Attosecond lighthouses from plasma mirrors. Nat. Photonics 2013, in press. [Google Scholar]
- Veltcheva, M.; Borot, A.; Thaury, C.; Malvache, A.; Lefebvre, E.; Flacco, A.; Lopez-Martens, R.; Malka, V. Brunel-dominated proton acceleration with a few-cycle laser pulse. Phys. Rev. Lett. 2012, 108, 075004. [Google Scholar]
- Chen, X.; Malvache, A.; Ricci, A.; Jullien, A.; Lopez-Martens, R. Efficient hollow fiber compression scheme for generating multi-mJ, carrier-envelope phase stable, sub-5 fs pulses. Laser Phys 2011, 21, 1–4. [Google Scholar]
- Doumy, G.; Quere, F.; Gobert, O.; Perdrix, M.; Martin, P.; Audebert, P.; Gauthier, J.C.; Geindre, J.-P.; Wittmann, T. Complete characterization of a plasma mirror for the production of high-contrast ultraintense laser pulse. Phys. Rev. E. 2004, 69, 026402. [Google Scholar]
- Mikhailova, J.M.; Buck, A.; Borot, A.; Schmid, K.; Sears, C.; Tsakiris, G.D.; Krausz, F.; Veisz, L. Ultra-high-contrast few-cycle pulses for multipetawatt-class laser technology. Opt. Lett. 2011, 36, 3145–3147. [Google Scholar]
- Kalashnikov, M.P.; Risse, E.; Schönnagel, H.; Sandner, W. Double chirped-pulse-amplification laser: A way to clean pulses temporally. Opt. Lett. 2005, 30, 923–925. [Google Scholar]
- Jullien, A.; Albert, O.; Burgy, F.; Hamoniaux, G.; Rousseau, J.-P.; Chambaret, J.-P.; Augé-Rochereau, F.; Chériaux, G.; Etchepare, J.; Minkovski, N.; et al. 10−10 temporal contrast for femtosecond ultraintense lasers by cross-polarized wave generation. Opt. Lett. 2005, 30, 920–922. [Google Scholar]
- Minkovski, N.; Petrov, G.I.; Saltiel, S.M.; Albert, O.; Etchepare, J. Nonlinear polarization rotation and orthogonal polarization generation experienced in a single-beam configuration. J. Opt. Soc. Am. B. 2004, 21, 1659–1664. [Google Scholar]
- Chvykov, V.; Rousseau, P.; Reed, S.; Kalinchenko, G.; Yanovsky, V. Generation of 1011 contrast 50 TW laser pulses. Opt. Lett. 2006, 31, 1456. [Google Scholar]
- Flacco, A.; Sylla, F.; Veltcheva, M.; Carrié, M.; Nuter, R.; Lefebvre, E.; Batani, D.; Malka, V. Dependence on pulse duration and foil thickness in high-contrast-laser proton acceleration. Phys. Rev. E. 2010, 81, 036405. [Google Scholar]
- Plateau, G.R.; Matlis, N.H.; Albert, O.; Toth, C.; Geddes, C.G.R.; Schroeder, C.B.; van Tilborg, J.; Esarey, E.; Leemans, W.P. Optimization of Thz Radiation Generation From a Laser Wakefield Accelerator, Proceedings of the AIP Conference Proceedings, Santa Cruz, CA, USA, 27 uly–2 August 2008.
- Leemans, W.P.; Simon-Boisson, C. The BELLA System and Facility, Proceedings of the Conference of the International Committee on Ultra-High Intensity Lasers, Mamaia, Romania, 16–21 September 2012.
- Ricci, A.; Jullien, A.; Forget, N.; Crozatier, V.; Tournois, P.; Lopez-Martens, R. Grism compressor for carrier-envelope phase-stable millijoule-energy chirped pulse amplifier lasers featuring bulk material stretcher. Opt. Lett. 2012, 37, 1196–1198. [Google Scholar]
- Osvay, K.; Canova, L.; Durfee, C.; Kovács, A.P.; Börzsönyi, A.; Albert, O.; Martens, R.L. Preservation of the carrier envelope phase during cross-polarized wave generation. Opt. Express. 2009, 17, 22358–22365. [Google Scholar]
- Bohman, S.; Suda, A.; Kanai, T.; Yamaguchi, S.; Midorikawa, K. Generation of 5.0 fs, 5.0 mJ pulses at 1 kHz using hollow-fiber pulse compression. Opt. Lett. 2010, 35, 1887–1889. [Google Scholar]
- Canova, L.; Chen, X.; Trisorio, A.; Jullien, A.; Assion, A.; Tempea, G.; Forget, N.; Oksenhendler, T.; Lopez-Martens, R. Carrier-envelope phase stabilization and control using a transmission grating compressor and an aopdf. Opt. Lett. 2009, 34, 1333–1335. [Google Scholar]
- Moulet, A.; Grabielle, S.; Cornaggia, C.; Forget, N.; Oksenhendler, T. Single-shot, high-dynamic-range measurement of sub-15 fs pulses by self-referenced spectral interferometry. Opt. Lett. 2010, 35, 3856–3858. [Google Scholar]
- Canova, L.; Albert, O.; Forget, N.; Mercier, B.; Kourtev, S.; Minkovski, N.; Saltiel, S.; Lopez-Martens, R. Influence of spectral phase on cross-polarized wave generation with short femtosecond pulses. App. Phys. B. 2008, 93, 443–453. [Google Scholar]
- Ramirez, L.P.; Papadopoulos, D.N.; Pellegrina, A.; Georges, P.; Druon, F.; Monot, P.; Ricci, A.; Jullien, A.; Chen, X.; Rousseau, J.P.; et al. Efficient cross polarized wave generation for compact, energy-scalable, ultrashort laser sources. Opt. Express. 2011, 19, 93–98. [Google Scholar]
- Jullien, A.; Albert, O.; Chériaux, G.; Etchepare, J.; Kourtev, S.; Minkovski, N.; Saltiel, S.M. A two crystal arrangement to fight efficiency saturation in cross-polarized wave generation. Opt. Express. 2006, 14, 2760–2769. [Google Scholar]
- Jullien, A.; Kourtev, S.; Albert, O.; Cheriaux, G.; Etchepare, J.; Minkovski, N.; Saltiel, S. Highly efficient temporal cleaner for femtosecond pulses based on cross-polarized wave generation in a dual crystal scheme. App. Phys. B. 2006, 84, 409–414. [Google Scholar]
- Jullien, A.; Rousseau, J.-P.; Mercier, B.; Antonucci, L.; Albert, O.; Chériaux, G.; Kourtev, S.; Minkovski, N.; Saltiel, S.M. Highly efficient nonlinear filter for femtosecond pulse contrast enhancement and pulse shortening. Opt. Lett. 2008, 33, 2353–2355. [Google Scholar]
- Jullien, A.; Durfee, C.G.; Trisorio, A.; Canova, L.; Rousseau, J.P.; Mercier, B.; Antonucci, L.; Cheriaux, G.; Albert, O.; Lopez-Martens, R. Nonlinear spectral cleaning of few-cycle pulses via cross-polarized wave (xpw) generation. App. Phys. B. 2009, 96, 293. [Google Scholar]
- Jullien, A.; Chen, X.; Ricci, A.; Rousseau, J.P.; Lopez-Martens, R.; Ramirez, L.P.; Papadopoulos, D.; Pellegrina, A.; Druon, F.; Georges, P. High-fidelity front-end for high-power, high temporal quality few-cycle lasers. App. Phys. B. 2011, 102, 769. [Google Scholar]
- Adams, D.E.; Planchon, T.A.; Squier, J.A.; Durfee, C.G. Spatiotemporal dynamics of cross-polarized wave generation. Opt. Lett. 2010, 35, 1115–1117. [Google Scholar]
- Grabielle, S.; Moulet, A.; Forget, N.; Crozatier, V.; Coudreau, S.; Gobert, O.; Cornaggia, C.; Oksenhendler, T. Sub-12fs Pulses Characterization by Self-Referenced Spectral Interferometry, Proceedings of The European Conference on Lasers and Electro-Optics, Munich, Germany, 22 May 2011.
- Adams, D.E.; Planchon, T.A.; Hrin, A.; Squier, J.A.; Durfee, C.G. Characterization of coupled nonlinear spatiospectral phase following an ultrafast self-focusing interaction. Opt. Lett. 2009, 34, 1294–1296. [Google Scholar]
- Ricci, A.; Ramirez, L.P.; Papadopoulos, D.; Pellegrina, A.; Georges, P.; Druon, F.; Monot, P.; Jullien, A.; Chen, X.; Rousseau, J.-P.; et al. High Energy and Efficient Cross Polarized Wave Generation for High Contrast Ultrashort Laser Sources, Proceedings of The European Conference on Lasers and Electro-Optics, Baltimore, MD, USA, 1–6 May 2011.
© 2013 by the authors; licensee MDPI, Basel, Switzerland This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Ricci, A.; Jullien, A.; Rousseau, J.-P.; Lopez-Martens, R. Front-End Light Source for aWaveform-Controlled High-Contrast Few-Cycle Laser System for High-Repetition Rate Relativistic Optics. Appl. Sci. 2013, 3, 314-324. https://doi.org/10.3390/app3010314
Ricci A, Jullien A, Rousseau J-P, Lopez-Martens R. Front-End Light Source for aWaveform-Controlled High-Contrast Few-Cycle Laser System for High-Repetition Rate Relativistic Optics. Applied Sciences. 2013; 3(1):314-324. https://doi.org/10.3390/app3010314
Chicago/Turabian StyleRicci, Aurélien, Aurélie Jullien, Jean-Philippe Rousseau, and Rodrigo Lopez-Martens. 2013. "Front-End Light Source for aWaveform-Controlled High-Contrast Few-Cycle Laser System for High-Repetition Rate Relativistic Optics" Applied Sciences 3, no. 1: 314-324. https://doi.org/10.3390/app3010314
APA StyleRicci, A., Jullien, A., Rousseau, J.-P., & Lopez-Martens, R. (2013). Front-End Light Source for aWaveform-Controlled High-Contrast Few-Cycle Laser System for High-Repetition Rate Relativistic Optics. Applied Sciences, 3(1), 314-324. https://doi.org/10.3390/app3010314




