Phase Space Insights: Wigner Functions for Qubits and Beyond
Abstract
1. Introduction
2. Wigner Function for a Spin S
3. A Menagerie of Wigner Functions
4. Generalized Wigner Function
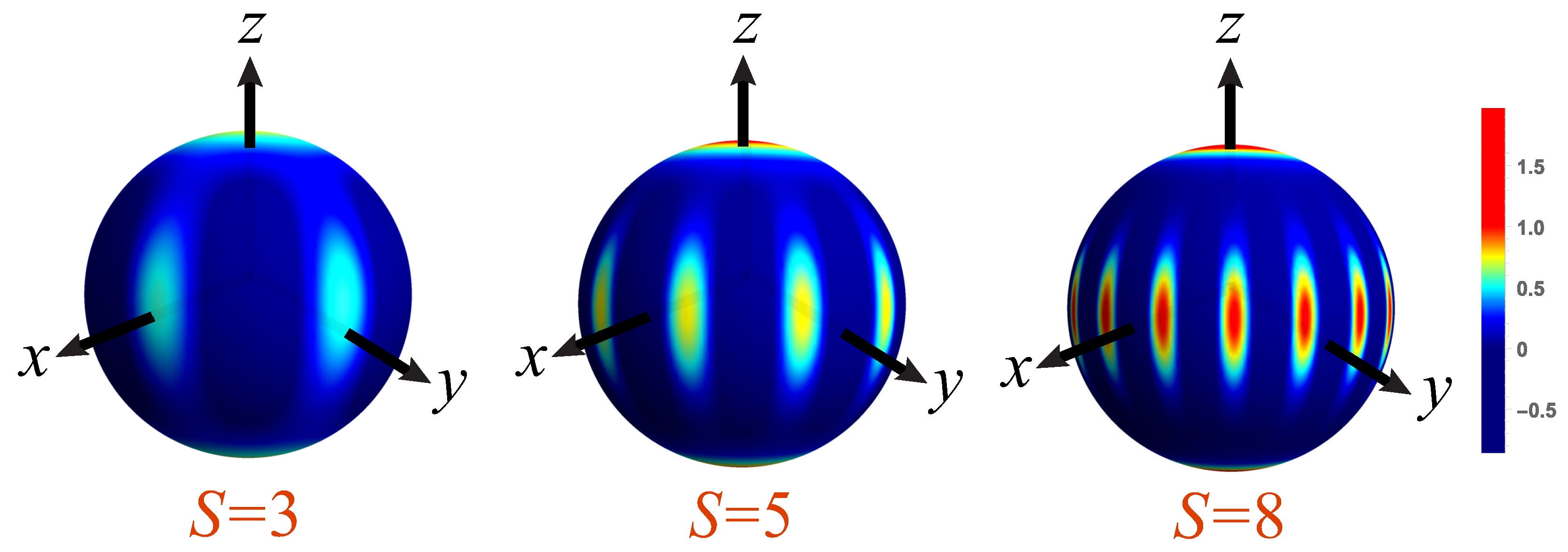
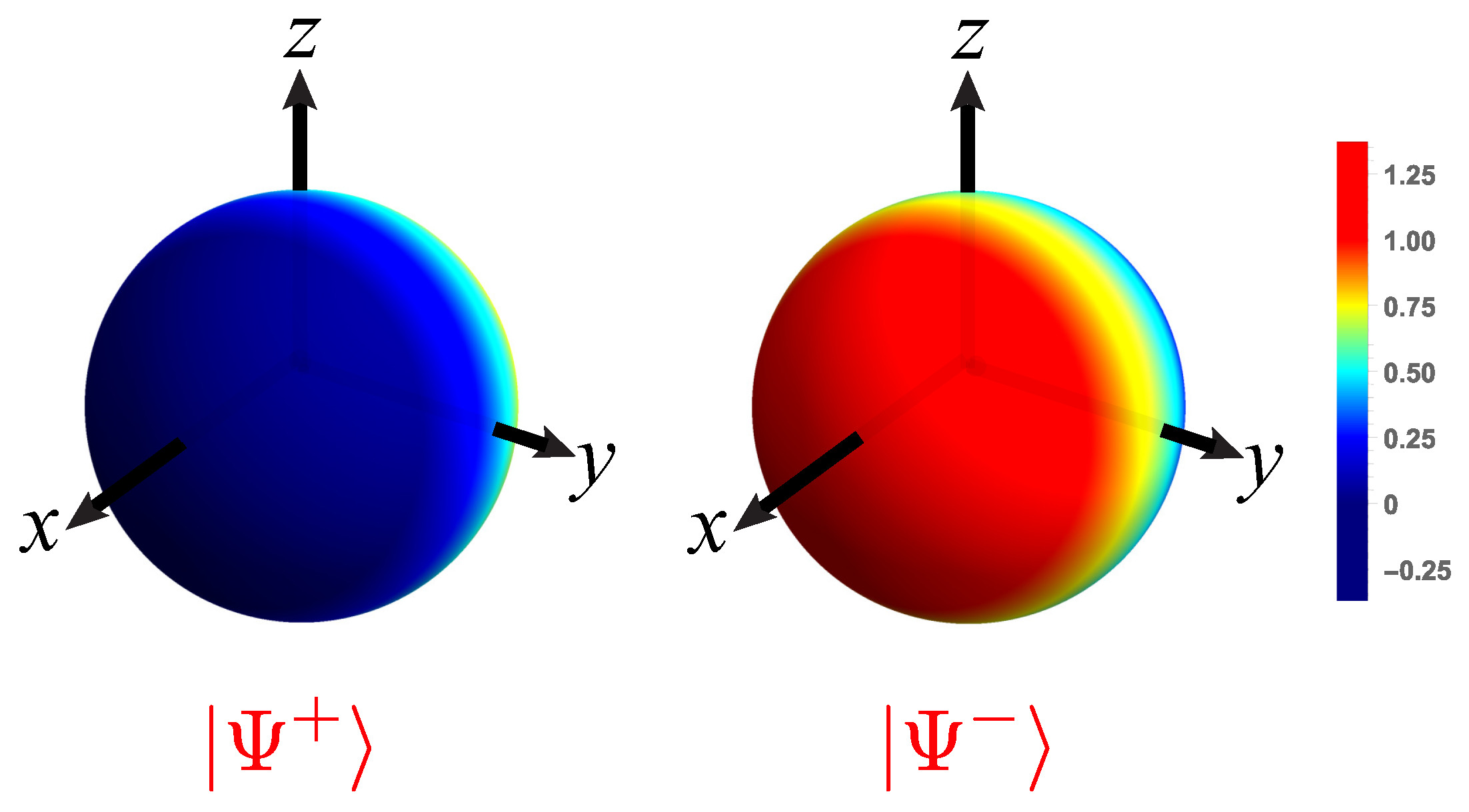
5. Examples
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schroek, F.E. Quantum Mechanics on Phase Space; Kluwer: Dordrecht, The Netherlands, 1996. [Google Scholar]
- Schleich, W.P. Quantum Optics in Phase Space; Wiley-VCH: Berlin, Germany, 2001. [Google Scholar]
- Zachos, C.K.; Fairlie, D.B.; Curtright, T.L. (Eds.) Quantum Mechanics in Phase Space; World Scientific: Singapore, 2005. [Google Scholar]
- Albert, V.V.; Pascazio, S.; Devoret, M.H. General phase spaces: From discrete variables to rotor and continuum limits. J. Phys. A Math. Theor. 2017, 50, 504002. [Google Scholar] [CrossRef]
- Weyl, H. Quantenmechanik und Gruppentheorie. Z. Phys. 1927, 46, 1–46. [Google Scholar] [CrossRef]
- Wigner, E.P. On the quantum correction for thermodynamic equilibrium. Phys. Rev. 1932, 40, 749–759. [Google Scholar] [CrossRef]
- Groenewold, H.J. On the principles of elementary quantum mechanics. Physica 1946, 12, 405–460. [Google Scholar] [CrossRef]
- Moyal, J.E. Quantum mechanics as a statistical theory. Proc. Camb. Philos. Soc. 1949, 45, 99–124. [Google Scholar] [CrossRef]
- Kirillov, A.A. Lectures on the Orbit Method; Graduate Studies in Mathematics; American Mathematical Society: Providence, RI, USA, 2004; Volume 64. [Google Scholar]
- Tatarskii, V.I. The Wigner representation in quantum mechanics. Sov. Phys. Usp. 1983, 26, 311–327. [Google Scholar] [CrossRef]
- Balazs, N.L.; Jennings, B.K. Wigner’s function and other distribution functions in mock phase spaces. Phys. Rep. 1984, 104, 347–391. [Google Scholar] [CrossRef]
- Hillery, M.; Connell, R.F.O.; Scully, M.O.; Wigner, E.P. Distribution functions in physics: Fundamentals. Phys. Rep. 1984, 106, 121–167. [Google Scholar] [CrossRef]
- Lee, H.W. Theory and application of the quantum phase-space distribution functions. Phys. Rep. 1995, 259, 147–211. [Google Scholar] [CrossRef]
- Weinbub, J.; Ferry, D.K. Recent advances in Wigner function approaches. Appl. Phys. Rev. 2018, 5, 041104. [Google Scholar] [CrossRef]
- Ferry, D.K.; Nedjalkov, M. The Wigner Function in Science and Technology; IOP Publishing: Bristol, UK, 2018. [Google Scholar] [CrossRef]
- Glauber, R.J. Coherent and Incoherent States of the Radiation Field. Phys. Rev. 1963, 131, 2766–2788. [Google Scholar] [CrossRef]
- Sudarshan, E.C.G. Equivalence of Semiclassical and Quantum Mechanical Descriptions of Statistical Light Beams. Phys. Rev. Lett. 1963, 10, 277–279. [Google Scholar] [CrossRef]
- Husimi, K. Some Formal Properties of the Density Matrix. Proc. Phys. Math. Soc. Jpn. 1940, 22, 264–314. [Google Scholar] [CrossRef]
- Stratonovich, R.L. On distributions in representation space. JETP 1956, 31, 1012–1020. [Google Scholar]
- Berezin, F.A. General concept of quantization. Commun. Math. Phys. 1975, 40, 153–174. [Google Scholar] [CrossRef]
- Varilly, J.C.; Gracia-Bondía, J.M. The Moyal representation for spin. Ann. Phys. 1989, 190, 107–148. [Google Scholar] [CrossRef]
- Mukunda, N. Wigner distribution for angle coordinates in quantum mechanics. Am. J. Phys. 1979, 47, 182–187. [Google Scholar] [CrossRef]
- Plebański, J.F.; Prazanowski, M.; Tosiek, J.; Turrubiates, F.K. Remarks on deformation quantization on the cylinder. Acta Phys. Pol. B 2000, 31, 561–587. [Google Scholar]
- Chumakov, S.M.; Klimov, A.B.; Wolf, K.B. Connection between two Wigner functions for spin systems. Phys. Rev. A 2000, 61, 034101. [Google Scholar] [CrossRef]
- Rigas, I.; Sánchez-Soto, L.L.; Klimov, A.B.; Řeháček, J.; Hradil, Z. Orbital angular momentum in phase space. Ann. Phys. 2011, 326, 426–439. [Google Scholar] [CrossRef]
- Kastrup, H.A. Wigner functions for the pair angle and orbital angular momentum. Phys. Rev. A 2016, 94, 062113. [Google Scholar] [CrossRef]
- Kastrup, H.A. Wigner functions for angle and orbital angular momentum: Operators and dynamics. Phys. Rev. A 2017, 95, 052111. [Google Scholar] [CrossRef]
- Fabre, N.; Klimov, A.B.; Murenzi, R.; Gazeau, J.P.; Sánchez-Soto, L.L. Majorana stellar representation of twisted photons. Phys. Rev. Res. 2023, 5, L032006. [Google Scholar] [CrossRef]
- Franke-Arnold, S.; Allen, L.; Padgett, M. Advances in optical angular momentum. Laser Photon. Rev. 2008, 2, 299–313. [Google Scholar] [CrossRef]
- Seyfarth, U.; Klimov, A.B.; Guise, H.d.; Leuchs, G.; Sanchez-Soto, L.L. Wigner function for SU(1,1). Quantum 2020, 4, 317. [Google Scholar] [CrossRef]
- Klimov, A.B.; Seyfarth, U.; de Guise, H.; Sánchez-Soto, L.L. SU(1, 1) covariant s-parametrized maps. J. Phys. A Math. Theor. 2021, 54, 065301. [Google Scholar] [CrossRef]
- Wodkiewicz, K.; Eberly, J.H. Coherent states, squeezed fluctuations, and the SU(2) and SU(1,1) groups in quantum-optics applications. J. Opt. Soc. Am. B 1985, 2, 458–466. [Google Scholar] [CrossRef]
- Gerry, C.C. Dynamics of SU(1,1) coherent states. Phys. Rev. A 1985, 31, 2721–2723. [Google Scholar] [CrossRef]
- Gerry, C.C. Correlated two-mode SU(1,1) coherent states: Nonclassical properties. J. Opt. Soc. Am. B 1991, 8, 685–690. [Google Scholar] [CrossRef]
- Gerry, C.C.; Grobe, R. Two-mode intelligent SU(1,1) states. Phys. Rev. A 1995, 51, 4123–4131. [Google Scholar] [CrossRef]
- Yurke, B.; McCall, S.L.; Klauder, J.R. SU(2) and SU(1,1) interferometers. Phys. Rev. A 1986, 33, 4033–4054. [Google Scholar] [CrossRef] [PubMed]
- Jing, J.; Liu, C.; Zhou, Z.; Ou, Z.Y.; Zhang, W. Realization of a nonlinear interferometer with parametric amplifiers. Appl. Phys. Lett. 2011, 99, 011110. [Google Scholar] [CrossRef]
- Hudelist, F.; Kong, J.; Liu, C.; Jing, J.; Ou, Z.Y.; Zhang, W. Quantum metrology with parametric amplifier-based photon correlation interferometers. Nat. Commun. 2014, 5, 3049. [Google Scholar] [CrossRef] [PubMed]
- Chekhova, M.V.; Ou, Z.Y. Nonlinear interferometers in quantum optics. Adv. Opt. Photon. 2016, 8, 104–155. [Google Scholar] [CrossRef]
- Brif, C.; Mann, A. A general theory of phase-space quasiprobability distributions. J. Phys. A Math. Gen. 1998, 31, L9–L17. [Google Scholar] [CrossRef]
- Mukunda, N.; Marmo, G.; Zampini, A.; Chaturvedi, S.; Simon, R. Wigner–Weyl isomorphism for quantum mechanics on Lie groups. J. Math. Phys. 2005, 46, 012106. [Google Scholar] [CrossRef]
- Klimov, A.B.; de Guise, H. General approach to SU(n) quasi-distribution functions. J. Phys. A Math. Theor. 2010, 43, 402001. [Google Scholar] [CrossRef]
- Tilma, T.; Nemoto, K. SU(N)-symmetric quasi-probability distribution functions. J. Phys. A Math. Theor. 2012, 45, 015302. [Google Scholar] [CrossRef]
- Tilma, T.; Everitt, M.J.; Samson, J.H.; Munro, W.J.; Nemoto, K. Wigner Functions for Arbitrary Quantum Systems. Phys. Rev. Lett. 2016, 117, 180401. [Google Scholar] [CrossRef]
- Rundle, R.P.; Tilma, T.; Samson, J.H.; Dwyer, V.M.; Bishop, R.F.; Everitt, M.J. General approach to quantum mechanics as a statistical theory. Phys. Rev. A 2019, 99, 012115. [Google Scholar] [CrossRef]
- Feynman, R.P. Quantum Implications: Essays in Honour of David Bohm; Chapter Negative Probabilities; Routledge: New York, NY, USA, 1987; pp. 235–248. [Google Scholar]
- Wootters, W.K. A Wigner-function formulation of finite-state quantum mechanics. Ann. Phys. 1987, 176, 1–21. [Google Scholar] [CrossRef]
- Galetti, D.; de Toledo-Piza, A.F.R. An extended Weyl-Wigner transformation for special finite spaces. Phys. A 1988, 149, 267–282. [Google Scholar] [CrossRef]
- Galetti, D.; de Toledo-Piza, A.F.R. Discrete quantum phase spaces and the mod N invariance. Phys. A 1992, 186, 513–523. [Google Scholar] [CrossRef]
- Heiss, S.; Weigert, S. Discrete Moyal-type representations for a spin. Phys. Rev. A 2000, 63, 012105. [Google Scholar] [CrossRef]
- Gibbons, K.S.; Hoffman, M.J.; Wootters, W.K. Discrete phase space based on finite fields. Phys. Rev. A 2004, 70, 062101. [Google Scholar] [CrossRef]
- Vourdas, A. Quantum systems with finite Hilbert space. Rep. Prog. Phys. 2004, 67, 267–320. [Google Scholar] [CrossRef]
- Klimov, A.B.; Mu noz, C.; Romero, J.L. Geometrical approach to the discrete Wigner function in prime power dimensions. J. Phys. A 2006, 39, 14471–14497. [Google Scholar] [CrossRef]
- Vourdas, A. Quantum systems with finite Hilbert space: Galois fields in quantum mechanics. J. Phys. A Math. Theor. 2007, 40, R285–R331. [Google Scholar] [CrossRef]
- Björk, G.; Klimov, A.B.; Sánchez-Soto, L.L. The discrete Wigner function. Prog. Opt. 2008, 51, 469–516. [Google Scholar] [CrossRef]
- Klimov, A.B.; Romero, J.L.; Björk, G.; Sánchez-Soto, L.L. Discrete phase-space structure of n-qubit mutually unbiased bases. Ann. Phys. 2009, 324, 53–72. [Google Scholar] [CrossRef]
- Rundle, R.P.; Everitt, M.J. Overview of the Phase Space Formulation of Quantum Mechanics with Application to Quantum Technologies. Adv. Quantum Technol. 2021, 4, 2100016. [Google Scholar] [CrossRef]
- Royer, A. Wigner function as the expectation value of a parity operator. Phys. Rev. A 1977, 15, 449–450. [Google Scholar] [CrossRef]
- Deléglise, S.; Dotsenko, I.; Sayrin, C.; Bernu, J.; Brune, M.; Raimond, J.M.; Haroche, S. Reconstruction of non-classical cavity field states with snapshots of their decoherence. Nature 2008, 455, 510–514. [Google Scholar] [CrossRef] [PubMed]
- Harder, G.; Silberhorn, C.; Rehacek, J.; Hradil, Z.; Motka, L.; Stoklasa, B.; Sánchez-Soto, L.L. Local Sampling of the Wigner Function at Telecom Wavelength with Loss-Tolerant Detection of Photon Statistics. Phys. Rev. Lett. 2016, 116, 133601. [Google Scholar] [CrossRef]
- Agarwal, G.S. Relation between atomic coherent-state representation, state multipoles, and generalized phase-space distributions. Phys. Rev. A 1981, 24, 2889–2896. [Google Scholar] [CrossRef]
- Dowling, J.P.; Agarwal, G.S.; Schleich, W.P. Wigner distribution of a general angular momentum state: Application to a collection of two-level atoms. Phys. Rev. A 1994, 49, 4101–4109. [Google Scholar] [CrossRef]
- Klimov, A.B.; Romero, J.L.; de Guise, H. Generalized SU(2) covariant Wigner functions and some of their applications. J. Phys. A Math. Theor. 2017, 50, 323001. [Google Scholar] [CrossRef]
- Jordan, P. Der Zusammenhang der symmetrischen und linearen Gruppen und das Mehrkörperproblem. Z. Phys. 1935, 94, 531–535. [Google Scholar] [CrossRef]
- Schwinger, J. On angular momentum. In Quantum Theory of Angular Momentum; Biedenharn, L.C., Dam, H., Eds.; Academic: New York, NY, USA, 1965. [Google Scholar]
- Chaturvedi, S.; Marmo, G.; Mukunda, N.; Simon, R.; Zampini, A. The Schwinger representation of a group: Concept and Applications. Rev. Math. Phys. 2006, 18, 887–912. [Google Scholar] [CrossRef]
- Dalton, B.J.; Ghanbari, S. Two mode theory of Bose–Einstein condensates: Interferometry and the Josephson model. J. Mod. Opt. 2012, 59, 287–353. [Google Scholar] [CrossRef]
- Sehat, A.; Söderholm, J.; Björk, G.; Espinoza, P.; Klimov, A.B.; Sánchez-Soto, L.L. Quantum polarization properties of two-mode energy eigenstates. Phys. Rev. A 2005, 71, 033818. [Google Scholar] [CrossRef]
- Marquardt, C.; Heersink, J.; Dong, R.; Chekhova, M.V.; Klimov, A.B.; Sánchez-Soto, L.L.; Andersen, U.L.; Leuchs, G. Quantum Reconstruction of an Intense Polarization Squeezed Optical State. Phys. Rev. Lett. 2007, 99, 220401. [Google Scholar] [CrossRef] [PubMed]
- Klimov, A.B.; Björk, G.; Söderholm, J.; Madsen, L.S.; Lassen, M.; Andersen, U.L.; Heersink, J.; Dong, R.; Marquardt, C.; Leuchs, G.; et al. Assessing the Polarization of a Quantum Field from Stokes Fluctuations. Phys. Rev. Lett. 2010, 105, 153602. [Google Scholar] [CrossRef] [PubMed]
- Müller, C.R.; Stoklasa, B.; Peuntinger, C.; Gabriel, C.; Řeháček, J.; Hradil, Z.; Klimov, A.B.; Leuchs, G.; Marquardt, C.; Sánchez-Soto, L.L. Quantum polarization tomography of bright squeezed light. New J. Phys. 2012, 14, 085002. [Google Scholar] [CrossRef]
- de la Hoz, P.; Klimov, A.B.; Björk, G.; Kim, Y.H.; Müller, C.; Marquardt, C.; Leuchs, G.; Sánchez-Soto, L.L. Multipolar hierarchy of efficient quantum polarization measures. Phys. Rev. A 2013, 88, 063803. [Google Scholar] [CrossRef]
- Sundar, K.; Mukunda, N.; Simon, R. Coherent-mode decomposition of general anisotropic Gaussian Schell-model beams. J. Opt. Soc. Am. A 1995, 12, 560–569. [Google Scholar] [CrossRef]
- Simon, R.; Mukunda, N. Shape-invariant anisotropic Gaussian Schell-model beams: A complete characterization. J. Opt. Soc. Am. A 1998, 15, 1361–1370. [Google Scholar] [CrossRef]
- Simon, R.; Mukunda, N. Optical phase space, Wigner representation, and invariant quality parameters. J. Opt. Soc. Am. A 2000, 17, 2440–2463. [Google Scholar] [CrossRef]
- Aniello, P.; Cagli, R.C. An algebraic approach to linear-optical schemes for deterministic quantum computing. J. Opt. B Quantum Semiclass. Opt. 2005, 7, S711. [Google Scholar] [CrossRef][Green Version]
- Sunko, D.K.; Svrtan, D. Generating function for angular momentum multiplicities. Phys. Rev. C 1985, 31, 1929–1933. [Google Scholar] [CrossRef]
- Berry, M.V.; Robbins, J.M. Indistinguishability for Quantum Particles: Spin, Statistics and the Geometric Phase. Proc. R. Soc. Lond. 1997, A453, 1771–1790. [Google Scholar] [CrossRef]
- Müller, C.R.; Madsen, L.S.; Klimov, A.B.; Sánchez-Soto, L.L.; Leuchs, G.; Marquardt, C.; Andersen, U.L. Parsing polarization squeezing into Fock layers. Phys. Rev. A 2016, 93, 033816. [Google Scholar] [CrossRef]
- Bengtsson, I.; Życzkowski, K. Geometry of Quantum States: An Introduction to Quantum Entanglement; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Goldberg, A.Z.; de la Hoz, P.; Björk, G.; Klimov, A.B.; Grassl, M.; Leuchs, G.; Sánchez-Soto, L.L. Quantum concepts in optical polarization. Adv. Opt. Photon. 2021, 13, 1–73. [Google Scholar] [CrossRef]
- Klimov, A.B. Exact evolution equations for SU(2) quasidistribution functions. J. Math. Phys. 2002, 43, 2202–2213. [Google Scholar] [CrossRef]
- Klimov, A.B.; Chumakov, S.M. A Group-Theoretical Approach to Quantum Optics; Wiley: Meinheim, Germany, 2009. [Google Scholar]
- Fano, U.; Racah, G. Irreducible Tensorial Sets; Academic: New York, NY, USA, 1959. [Google Scholar]
- Blum, K. Density Matrix Theory and Applications; Plenum: New York, NY, USA, 1981. [Google Scholar]
- Varshalovich, D.A.; Moskalev, A.N.; Khersonskii, V.K. Quantum Theory of Angular Momentum; World Scientific: Singapore, 1988. [Google Scholar]
- Goldberg, A.Z.; Klimov, A.B.; de Guise, H.; Leuchs, G.; Agarwal, G.S.; Sánchez-Soto, L.L. From polarization multipoles to higher-order coherences. Opt. Lett. 2022, 47, 477–480. [Google Scholar] [CrossRef]
- Feynman, R.P.; Vernon, F.L., Jr.; Hellwarth, R.W. Geometrical Representation of the Schrödinger Equation for Solving Maser Problems. J. Appl. Phys. 1957, 28, 49–52. [Google Scholar] [CrossRef]
- Perelomov, A. Generalized Coherent States and Their Applications; Springer: Berlin/Heidelberg, Germany, 1986. [Google Scholar]
- Gazeau, J.P. Coherent States in Quantum Physics; Wiley-VCH: Weinheim, Germany, 2009. [Google Scholar]
- Braginsky, V.B. Classical and Quantum Restrictions on the Detection of Weak Disturbances of a Macroscopic Oscillator. JETP 1967, 53, 1434–1441. Available online: http://jetp.ras.ru/cgi-bin/dn/e_026_04_0831.pdf (accessed on 2 May 2025).
- Caves, C.M. Quantum-mechanical noise in an interferometer. Phys. Rev. D 1981, 23, 1693–1708. [Google Scholar] [CrossRef]
- Yuen, H.P. Contractive states and the standard quantum limit for monitoring free-mass position. Phys. Rev. Lett. 1983, 51, 719–722. [Google Scholar] [CrossRef]
- Jaekel, M.T.; Reynaud, S. Quantum limits in interferometric measurements. Europhys. Lett. 1990, 13, 301–305. [Google Scholar] [CrossRef]
- Luis, A.; Sánchez-Soto, L.L. Multimode quantum analysis of an interferometer with moving mirrors. Phys. Rev. A 1992, 13, 8228–8234. [Google Scholar] [CrossRef] [PubMed]
- Holland, M.J.; Burnett, K. Interferometric detection of optical phase shifts at the Heisenberg limit. Phys. Rev. Lett. 1993, 71, 1355–1358. [Google Scholar] [CrossRef] [PubMed]
- Sanders, B.C.; Milburn, G.J. Optimal Quantum Measurements for Phase Estimation. Phys. Rev. Lett. 1995, 75, 2944–2947. [Google Scholar] [CrossRef] [PubMed]
- Giovannetti, V.; Lloyd, S.; Maccone, L. Quantum-Enhanced Measurements: Beating the Standard Quantum Limit. Science 2004, 306, 1330–1336. [Google Scholar] [CrossRef]
- Berry, D.W.; Wiseman, H.M. Optimal States and Almost Optimal Adaptive Measurements for Quantum Interferometry. Phys. Rev. Lett. 2000, 85, 5098–5101. [Google Scholar] [CrossRef]
- Bollinger, J.J.; Itano, W.M.; Wineland, D.J.; Heinzen, D.J. Optimal frequency measurements with maximally correlated states. Phys. Rev. A 1996, 54, R4649–R4652. [Google Scholar] [CrossRef]
- Yurke, B. Input States for Enhancement of Fermion Interferometer Sensitivity. Phys. Rev. Lett. 1986, 56, 1515–1517. [Google Scholar] [CrossRef]
- Combes, J.; Wiseman, H.M. States for phase estimation in quantum interferometry. J. Opt. B Quantum Semiclass. Opt. 2005, 7, 14–21. [Google Scholar] [CrossRef][Green Version]
- Dowling, J.P. Quantum optical metrology—The lowdown on high-N00N states. Contemp. Phys. 2008, 49, 125–143. [Google Scholar] [CrossRef]
- Donati, G.; Bartley, T.J.; Jin, X.M.; Vidrighin, M.D.; Datta, A.; Barbieri, M.; Walmsley, I.A. Observing optical coherence across Fock layers with weak-field homodyne detectors. Nat. Commun. 2014, 5, 5584. [Google Scholar] [CrossRef]
- Klimov, A.B.; Romero, J.L. A generalized Wigner function for quantum systems with the SU(2) dynamical symmetry group. J. Phys. A Math. Theor. 2008, 41, 055303. [Google Scholar] [CrossRef]
- Tan, S.M.; Walls, D.F.; Collett, M.J. Nonlocality of a single photon. Phys. Rev. Lett. 1991, 66, 252–255. [Google Scholar] [CrossRef] [PubMed]
- Björk, G.; Jonsson, P.; Sánchez-Soto, L.L. Single-particle nonlocality and entanglement with the vacuum. Phys. Rev. A 2001, 64, 042106. [Google Scholar] [CrossRef]
- van Enk, S.J. Single-particle entanglement. Phys. Rev. A 2005, 72, 064306. [Google Scholar] [CrossRef]
- Guerreiro, T.; Monteiro, F.; Martin, A.; Brask, J.B.; Vértesi, T.; Korzh, B.; Caloz, M.; Bussières, F.; Verma, V.B.; Lita, A.E.; et al. Demonstration of Einstein–Podolsky-Rosen Steering Using Single-Photon Path Entanglement and Displacement-Based Detection. Phys. Rev. Lett. 2016, 117, 070404. [Google Scholar] [CrossRef]
- Qiao, L.F.; Jiao, Z.Q.; Xu, X.Y.; Gao, J.; Zhang, Z.Y.; Ren, R.J.; Zhou, W.H.; Wang, X.W.; Jin, X.M. Multistage quantum swapping of vacuum-one-photon entanglement. Phys. Rev. A 2021, 104, 022415. [Google Scholar] [CrossRef]
- Hessmo, B.; Usachev, P.; Heydari, H.; Björk, G. Experimental Demonstration of Single Photon Nonlocality. Phys. Rev. Lett. 2004, 92, 180401. [Google Scholar] [CrossRef]


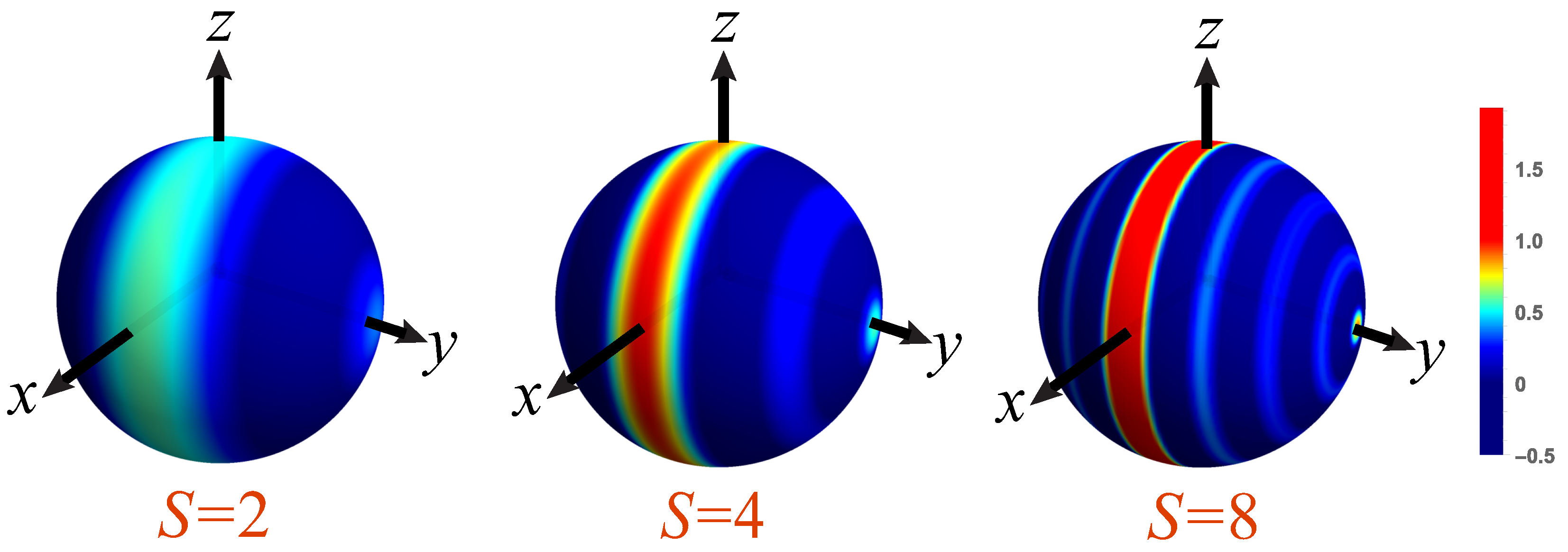

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sánchez-Soto, L.L.; Muñoz, A.; de la Hoz, P.; Klimov, A.B.; Leuchs, G. Phase Space Insights: Wigner Functions for Qubits and Beyond. Appl. Sci. 2025, 15, 5155. https://doi.org/10.3390/app15095155
Sánchez-Soto LL, Muñoz A, de la Hoz P, Klimov AB, Leuchs G. Phase Space Insights: Wigner Functions for Qubits and Beyond. Applied Sciences. 2025; 15(9):5155. https://doi.org/10.3390/app15095155
Chicago/Turabian StyleSánchez-Soto, Luis L., Ariana Muñoz, Pablo de la Hoz, Andrei B. Klimov, and Gerd Leuchs. 2025. "Phase Space Insights: Wigner Functions for Qubits and Beyond" Applied Sciences 15, no. 9: 5155. https://doi.org/10.3390/app15095155
APA StyleSánchez-Soto, L. L., Muñoz, A., de la Hoz, P., Klimov, A. B., & Leuchs, G. (2025). Phase Space Insights: Wigner Functions for Qubits and Beyond. Applied Sciences, 15(9), 5155. https://doi.org/10.3390/app15095155








