A Review of Prediction Methods for Ultra-Low Cycle Fatigue Damage of Steel Piers Under Earthquakes
Abstract
1. Introduction
2. Research Status and Development Trend at Home and Abroad
2.1. Empirical Formula Method
2.2. Cyclic Void Growth Model
2.3. Cyclic Multiaxial Fracture Strain Energy (CMFSE)
2.4. Continuum Damage Mechanics (CDM) Model
2.4.1. The Principle and Development of Traditional CDM Model
2.4.2. The Method of Testing the Critical Value of Damage Variable
2.4.3. Simulation Method for Ultra-Low Cycle Fatigue Crack Propagation
2.4.4. Advantages and Disadvantages of Prediction Methods
- (1)
- The CDM model, designed for ductile fracture prediction, requires parameter calibration from monotonic tensile tests, minimizing the number of tests needed.
- (2)
- This model features multiple prediction functions. It not only forecasts the ultra-low cycle fatigue life of steel and steel structures but also estimates damage cycles.
- (3)
- The CDM model accounts for damage effects on the material constitutive behavior, making it compatible with finite element software and practical for engineering applications.
2.5. Seismic Performance and Seismic Response Calculation Model of Steel Pier
2.5.1. Seismic Performance Evaluation Indicators for Steel Bridge Piers
2.5.2. Seismic Response Calculation Model of Steel Bridge Pier
3. Conclusions
- (1)
- The traditional CDM model is not highly accurate in predicting ultra-low cycle fatigue damage in steel and its welded specimens. Although this model can estimate the fatigue process and life of welded joints under seismic conditions, it fails to incorporate the effects of equivalent plastic strain on damage increments and lack a reliable method for determining the critical value of damage and fracture, leading to discrepancies between predictions and experimental results.
- (2)
- Current prediction methods lack a mixed-element partitioning criterion for steel bridge piers that accounts for the coupling effects of ultra-low cycle fatigue and local buckling. The modeling approach is not universally applicable and poses challenges for practical engineering applications.
- (3)
- Prediction limits for ultra-low cycle fatigue damage should not rely solely on crack initiation. The hysteresis model and skeleton curve utilized for evaluating the seismic performance of steel bridge piers do not incorporate the effects of crack propagation and its interaction with local buckling, which deviates from experimental findings.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, J.L.; Li, W.J.; Feng, M. The Advancement and Retreat of Highway Steel Bridges in China. China Highw. 2016, 23, 25–27. [Google Scholar]
- Sun, C.; Han, L. The Development and Application of Anticorrosion Technology for Steel Bridges at Home and Abroad. Urban Roads Bridges Flood Control 2011, 29, 127–128. [Google Scholar]
- Ministry of Transport of the People’s Republic of China. Guiding Opinions on Promoting the Construction of Highway Steel Structure Bridges; Ministry of Transport of the People’s Republic of China: Beijing, China, 2016.
- He, L.X.; Song, L. The development and application examples of steel bridges and steel-concrete composite bridges. Southwest Highw. 2014, 17, 2–14. [Google Scholar]
- Usami, T. Guidelines for Seismic and Damage Control Design of Steel Bridges; Gihodo Shuppan Co.: Tokyo, Japan, 2007. [Google Scholar]
- Unjoh, S. Seismic retrofit of highway bridges. J. Jpn. Assoc. Earthq. Eng. 2004, 4, 230–248. [Google Scholar] [CrossRef][Green Version]
- Xie, X.; Tang, S. Seismic Design of Steel Bridges; Science Press: Beijing, China, 2019. [Google Scholar]
- Tian, Q.; Hayashikawa, T.; Ren, W.X. Effectiveness of shock absorber device for damage mitigation of curved viaduct with steel bearing supports. Eng. Struct. 2016, 109, 61–74. [Google Scholar] [CrossRef]
- Maruyama. Japanese Society of Civil Engineering Analysis of the Actual State of Earthquake Disasters in Steel Structures During the Hanshin Awaji Earthquake; Maruyama: Tokyo, Japan, 1999. [Google Scholar]
- Miller, D.K. Lessons learned from the Northridge earthquake. Eng. Struct. 1998, 20, 249–260. [Google Scholar] [CrossRef]
- Zhang, Y.; Xiao, J.; Liu, W.; Zhao, A. Effect of Welding Peak Temperature on Microstructure and Impact Toughness of Heat-Affected Zone of Q690 High Strength Bridge Steel. Materials 2021, 14, 2981. [Google Scholar] [CrossRef]
- Kitada, T.; Matsumura, M.; Otoguro, Y. Seismic retrofitting techniques using an energy absorption segment for steel bridge piers. Eng. Struct. 2003, 25, 621–635. [Google Scholar] [CrossRef]
- Goto, Y.; Ebisawa, T.; Lu, X.L. Local buckling restraining behavior of thin-walled circular CFT columns under seismic loads. J. Struct. Eng. 2014, 140, 04013105. [Google Scholar] [CrossRef]
- Wang, Z.F.; Toshitaka, Y. Ultimate strength and ductility of stiffened steel tubular bridge piers. Int. J. Steel Struct. 2011, 11, 81–90. [Google Scholar] [CrossRef]
- Usami, T.; Ge, H.B.; Saizuka, K. Behavior of partially concrete-filled steel bridge piers under cyclic and dynamic loading. J. Constr. Steel Res. 1997, 41, 121–136. [Google Scholar] [CrossRef]
- Wang, Z.; Sui, W.-N.; Li, G.-C.; Wu, Q.; Ge, L. Mechanical behaviour of pertically concrete-filled steel circular bridge piers under cyclic lateral load. China J. Highw. Transp. 2015, 28, 62–70. [Google Scholar]
- Kanvinde, A.M.; Deierlein, G.G. Cyclic void growth model to assess ductile fracture initiation in structural steels due to ultra low cycle fatigue. J. Eng. Mech. 2007, 133, 701–712. [Google Scholar] [CrossRef]
- Bleck, W.; Dahl, W.; Nonn, A.; Amlung, L.; Feldmann, M.; Schäfer, D.; Eichler, B. Numerical and experimental analyses of damage behavior of steel moment connection. Eng. Fract. Mech. 2009, 76, 1531–1547. [Google Scholar] [CrossRef]
- Zhou, H.; Wang, Y.; Shi, Y.; Xiong, J.; Yang, L. Extremely low cycle fatigue prediction of steel beam-to-column connection by using a micro-mechanics based fracture model. Int. J. Fatigue 2013, 48, 90–100. [Google Scholar] [CrossRef]
- Kermajani, M.; Ghaini, F.M.; Miresmaeili, R.; Aghakouchak, A.; Shadmand, M. Effect of weld metal toughness on fracture behavior under ultra-low cycle fatigue loading (earthquake). Mater. Sci. Eng. 2016, 668, 30–37. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhang, J.; Zhao, P.; Huang, Y.; Yu, Z.; Fang, X. Low-cycle fatigue behaviors of a new type of 10% Cr martensitic steel and welded joint with Ni-based weld metal. Int. J. Fatigue 2016, 88, 78–87. [Google Scholar] [CrossRef]
- Anderson, T.L. Fracture Mechanics, Fundamentals and Applications, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Cao, W.Z. Research on Extremely Low Cycle Fatigue Fracture Behavior of Q460 High Strength Steel Frame Cruciform Beam—Column Welded Connections Based on Micromechanism. Master Thesis, Chang’an University, Chang’an, China, 2021. [Google Scholar]
- Nip, K.H.; Gardner, L.; Davies, C.M. Extremely low cycle fatigue tests on structural carbon steel and stainless steel. J. Constr. Steel Res. 2010, 66, 96–110. [Google Scholar] [CrossRef]
- Xue, L. A unified expression for low cycle fatigue and extremely low cycle fatigue and its implication for monotonic loading. Int. J. Fatigue 2008, 30, 1691–1698. [Google Scholar] [CrossRef]
- Kuwamura, H.; Takagi, N. Similitude law of prefracture hysteresis of steel members. J. Struct. Eng. 2004, 130, 752–761. [Google Scholar] [CrossRef]
- Song, F.Y.; Xie, X. Analysis of the Effect of Corrosion on the Low Cycle Fatigue Performance of Steel. J. Zhejiang Univ. (Eng. Ed.) 2018, 52, 1–10. [Google Scholar]
- Coffin, L.F.J. A study of the effects of cyclic thermal stresses on a ductile metal. Trans. Am. Soc. Mech. Eng. 1954, 76, 931–950. [Google Scholar] [CrossRef]
- Manson, S.S. Fatigue, a complex subject-some simple approximations. Exp. Mesh. 1965, 5, 193–226. [Google Scholar] [CrossRef]
- Zhang, S. Numerical Simulation of Q345 Steel Ultra-Low Cycle Fatigue Fracture Process Based on Cyclic Void Growth Model. Master Thesis, Tianjin University, Tianjin, China, 2020. [Google Scholar]
- Bonora, N. A nonlinear CDM model for ductile failure. Eng. Fract. Mech. 1997, 58, 11–28. [Google Scholar] [CrossRef]
- Kuwamura, H.; Akiyama, H. Brittle fracture under repeated high stresses. J. Constr. Steel Res. 1994, 29, 5–19. [Google Scholar] [CrossRef]
- Tateishi, K.; Hanji, T.; Minami, K. A prediction model for extremely low cycle fatigue strength of structural steel. Int. J. Fatigue 2007, 29, 887–896. [Google Scholar] [CrossRef]
- Pereira, J.; de Jesus, A.; Xavier, J.; Fernandes, A. Ultra low-cycle fatigue behaviour of a structural steel. Eng. Struct. 2014, 60, 214–222. [Google Scholar] [CrossRef]
- Liao, F.F.; Wang, W.; Chen, Y.Y. Parameter calibrations and application of micromechanical fracture models of structural steels. Struct. Eng. Mech. 2012, 42, 153–174. [Google Scholar] [CrossRef]
- Liao, F.; Wang, W.; Chen, Y. Prediction of ultra-low cycle fatigue fracture of steel structure nodes under reciprocating loads. J. Tongji Univ. (Nat. Sci. Ed.) 2014, 42, 539–546. [Google Scholar]
- Tian, Q.; Zhuge, H.Q.; Xie, X. Prediction of the ultra-low-cycle fatigue damage of Q345qc steel and its weld joint. Materials 2019, 12, 4014. [Google Scholar] [CrossRef]
- Ge, H.B.; Kang, L. A damage index-based evaluation method for predicting the ductile crack initiation in steel structures. J. Earthq. Eng. 2012, 16, 623–643. [Google Scholar] [CrossRef]
- Ge, H.B.; Kang, L.; Tsumura, Y. Extremely low-cycle fatigue tests of thick-walled steel bridge piers. J. Bridge Eng. 2013, 18, 858–870. [Google Scholar] [CrossRef]
- JTG D60-2015; General Specifications for Design of Highway Bridges and Culverts. China Communications Press: Beijing, China, 2015.
- Japan Road Association. Specifications for Highway Bridges-Part II Steel Bridges; Maruzen: Tokyo, Japan, 2012. [Google Scholar]
- Manson, S.S. Behavior of Materials Under Conditions of Thermal Stress; National Advisory Committee for Aeroautics: Washington, DC, USA, 1954.
- Shi, G.; Gao, Y.; Wang, X. Research on Low Cycle Fatigue Performance of Low Yield Point Steel. J. Civ. Eng. 2019, 52, 20–26. [Google Scholar]
- Han, Y.D.; Zhang, Z.F.; Xu, L.Y. Analysis of High Temperature and Low Cycle Fatigue Behavior of P92 Steel Weld Metal. J. Weld. 2019, 40, 11–14, 31. [Google Scholar]
- Li, S.; Xie, X.; Cheng, C.; Tian, Q. A modified Coffin-Manson model for ultra-low cycle fatigue fracture of structural steels considering the effect of stress triaxiality. Eng. Fract. Mech. 2020, 237, 107223. [Google Scholar] [CrossRef]
- Shimada, K.; Komotori, J.; Shimizu, M. The applicability of the Manson-Coffin law and Miner’s law to extremely low cycle fatigue. Trans. Jpn. Soc. Mech. Eng. Part A 1987, 53, 1178–1185. [Google Scholar] [CrossRef]
- Kim, S.-J.; Choi, P.-H.; Dewa, R.T.; Kim, W.-G.; Kim, M.-H. Low cycle fatigue properties of alloy 617 base metal and weld joint at room temperature. Procedia Mater. Sci. 2014, 3, 2201–2206. [Google Scholar] [CrossRef]
- Kuroda, M. Extremely low cycle fatigue life prediction based on a new cumulative fatigue damage model. Int. J. Fatigue 2002, 24, 699–703. [Google Scholar] [CrossRef]
- Luo, Y.R.; Wang, Q.Y.; Fu, L. Ultra low cycle fatigue performance of Q235 steel structural materials. J. Iron Steel Res. 2016, 28, 47–51. [Google Scholar]
- Kang, L.; Ge, H.B. Predicting ductile crack initiation in steel bridge piers with unstiffened box section under specific cyclic loadings using detailed and simplified evaluation methods. Adv. Struct. Eng. 2015, 18, 1427–1442. [Google Scholar] [CrossRef]
- Xie, X.; Cheng, C.; Li, S.L. A deformation history-based approach for ultra-low cycle fatigue damage evaluation of steel structures. Int. J. Steel Struct. 2020, 20, 1378–1392. [Google Scholar] [CrossRef]
- Huang, X.W.; Zhang, X.; Miao, T.C. Damage prediction model for ultra-low cycle fatigue fracture failure of building structural steel. Eng. Mech. 2017, 34, 101–108. [Google Scholar]
- Liao, F.F.; Wang, W.; Li, W.C. Research Status of Steel Structure Node Fracture. J. Build. Sci. Eng. 2016, 33, 67–75. [Google Scholar]
- Li, S.L.; Xie, X.; Liao, Y.H. Improvement of cyclic void growth model for ultra-low cycle fatigue prediction of steel bridge piers. Materials 2019, 12, 1615. [Google Scholar] [CrossRef]
- Kanvinde, A.M. Micromechanical Simulation of Earthquake-Induced Fracture in Steel Structures. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2004. [Google Scholar]
- Rice, J.R.; Tracey, D.M. On the ductile enlargement of voids in triaxial stress fields. J. Mech. Phys. Solids 1969, 17, 201–217. [Google Scholar] [CrossRef]
- Hancock, J.W.; Mackenzie, A.C. On the mechanics of ductile failure in high-strength steel subjected to multi-axial stress-states. J. Mech. Phys. Solids 1976, 24, 147–169. [Google Scholar] [CrossRef]
- Chi, W.M.; Kanvinde, A.M.; Deierlein, G.G. Prediction of ductile fracture in steel connections using SMCS criterion. J. Struct. Eng. 2006, 132, 171–181. [Google Scholar] [CrossRef]
- Xing, J.H.; Guo, C.L.; Li, Y.Y. Identification of micro fracture model damage coefficient for Q235B steel. J. Harbin Inst. Technol. 2017, 49, 184–188. [Google Scholar]
- Li, S.; Xie, X.; Tian, Q.; Cheng, C.; Zhang, Z. Improved ultra-low cycle fatigue fracture models for structural steels considering the dependence of cyclic damage degradation parameters on stress triaxiality. Int. J. Steel Struct. 2021, 21, 329–348. [Google Scholar] [CrossRef]
- Liao, F.; Wang, M.; Tu, L.; Wang, J.; Lu, L. Micromechanical fracture model parameter influencing factor study of structural steels and welding materials. Constr. Build. Mater. 2019, 215, 898–917. [Google Scholar] [CrossRef]
- Xie, X.; Zhuge, H.; Tang, Z.; Wang, T.; Liao, Y. Damage characteristics of thin-walled steel arch bridges subjected to in-plane earthquake action. J. Constr. Steel Res. 2018, 151, 70–82. [Google Scholar] [CrossRef]
- Liao, Y.H. Research on Ultra-Low Cycle Fatigue Performance and Fracture Mechanism of Steel Bridge Welded Joints. Master’s Thesis, Zhejiang University, Hangzhou, China, 2018. [Google Scholar]
- Luo, J.; Li, S.; Mao, L.; Shi, G. Constitutive model of duplex stainless steel S22053 and its weld under cyclic large plastic strain loading. J. Constr. Steel Res. 2023, 205, 107882. [Google Scholar] [CrossRef]
- Yin, Y.; Zhang, S.; Han, Q.H.; Ma, T. Numerical simulation of ultra-low cycle fatigue fracture of q355 steels based on cyclic void growth model. Eng. Mech. 2021, 38, 246–256. [Google Scholar]
- Qiu, C.; Xing, J.H.; Zhang, L.; Wang, T. Ultra-low cycle fatigue properties of Q460 high strength T-shape butt weld joints. J. Vib. Shock. 2021, 40, 166–174. [Google Scholar]
- Christopher, S.; Andy, Z.; Masao, T.; Amit, K.; Gregory, D. A stress-weighted ductile fracture model for steel subjected to Ultra Low Cycle Fatigue. Eng. Struct. 2021, 245, 112964. [Google Scholar]
- Wang, T.; Wen, J.; Liao, P.; Zhang, X.; Kim, Y.; Tu, S. A study of ultra-low cycle fatigue failure based on a fracture strain energy model. Int. J. Fatigue 2021, 146, 106149. [Google Scholar] [CrossRef]
- Tian, Q.; Liao, Y.; Xie, X.; Zhuge, H. Study on the prediction method of the ultra-low-cycle fatigue damage of steel. Appl. Sci. 2020, 10, 679. [Google Scholar] [CrossRef]
- Rabotnov, Y.N. On the equations of state for creep. In Progress in Applied Mechanics; North-Holland: Amsterdam, The Netherlands, 1963; pp. 307–315. [Google Scholar]
- Lemaitre, J. Evaluation of dissipation and damage in metals submitted to dynamic loading. In Proceedings of the ICM1, Kyoto, Japan, 1 December 1971; pp. 540–549. [Google Scholar]
- Bonora, N. On the effect of triaxial state of stress on ductility using nonlinear CDM model. Int. J. Fract. 1997, 88, 359–371. [Google Scholar] [CrossRef]
- Tong, L.; Huang, X.; Zhou, F.; Chen, Y. Experimental and numerical investigations on extremely-low-cycle fatigue fracture behavior of steel welded joints. J. Constr. Steel Res. 2016, 119, 98–112. [Google Scholar] [CrossRef]
- Cheng, G.X. The investigation of low-cycle fatigue life for pressure vessel by damage mechanics approach. J. Xi’an Jiao Tong Univ. 1994, 28, 59–64. [Google Scholar]
- Lemaitre, J.; Dufailly, J. Damage measurements. Eng. Fract. Mech. 1987, 28, 643–661. [Google Scholar] [CrossRef]
- Policella, M.; Baudin, G.; Cailletaud, G. Mesures de longueur de fissures, déformations et endommagement par une technique de potentiel électrique. In Proceedings of the 60th Meeting of S.M. Panel AGARD, San Antonio, CA, USA, 1–6 May 1985. (In French). [Google Scholar]
- Paris, P.C.; Gomez, M.P.; Anderson, W.P. A rational analytic theory of fatigue. Trend Eng. 1961, 13, 9–14. [Google Scholar]
- Dover, W.D. Fatigue crack growth under COD cycling. Eng. Fract. Mech. 1973, 5, 11–21. [Google Scholar] [CrossRef]
- Shimada, H.; Furuya, Y. Application of crack-tip-strain loop to fatigue-crack propagation. Exp. Mech. 1981, 21, 423–428. [Google Scholar] [CrossRef]
- El Haddad, M.H.; Dowling, N.E.; Topper, T.H.; Smith, K.N. Integral application for short fatigue cracks at notch. Int. J. Fract. 1980, 16, 15–30. [Google Scholar] [CrossRef]
- Zhuge, H.Q.; Xie, X.; Li, S.L.; Wang, J.D. Seismic performance test and practical calculation method of thin-walled square-section steel piers under horizontal bi-directional seismic excitations. China Civ. Eng. J. 2020, 53, 74–85. [Google Scholar]
- Zhu, T.; Gao, S.B. Research on the initiation and propagation of ultra-low cycle fatigue cracks in thick walled box steel bridge piers. Steel Struct. (Chin. Engl.) 2020, 11, 1–11. [Google Scholar]
- Chi, S. Grain Prediction of Ultra-Low Cycle Fatigue Crack Initiation Life for Steel Bridge Piers. Master’s Thesis, Shanghai Jiao Tong University, Shanghai, China, 2016. [Google Scholar]
- Ge, H.B.; Gao, S.B.; Usami, T. Stiffened steel box columns. Part 1, Cyclic behavior. Earthq. Eng. Struct. Dyn. 2000, 29, 1691–1706. [Google Scholar] [CrossRef]
- Usami, T.; Gao, S.B.; Ge, H.B. Stiffened steel box columns. Part 2: Ductility evaluation. Earthq. Eng. Struct. Dyn. 2000, 29, 1707–1722. [Google Scholar] [CrossRef]
- Chen, S.X.; Xie, X.; Zhuge, H.Q. Hysteretic model for steel piers considering the local buckling of steel plates. Eng. Struct. 2019, 183, 303–318. [Google Scholar] [CrossRef]
- Zhuge, H.Q.; Xie, X.; Tang, Z. Station Study on the length of damaged areas of steel bridge piers under horizontal bidirectional seismic action. Chin. J. Highw. 2019, 32, 79–91. [Google Scholar]
- Tian, Q.; Xie, X.; Li, S.L. A model for ultra low cycle fatigue damage prediction of structural steel. J. Constr. Steel Res. 2021, 187, 106956. [Google Scholar] [CrossRef]

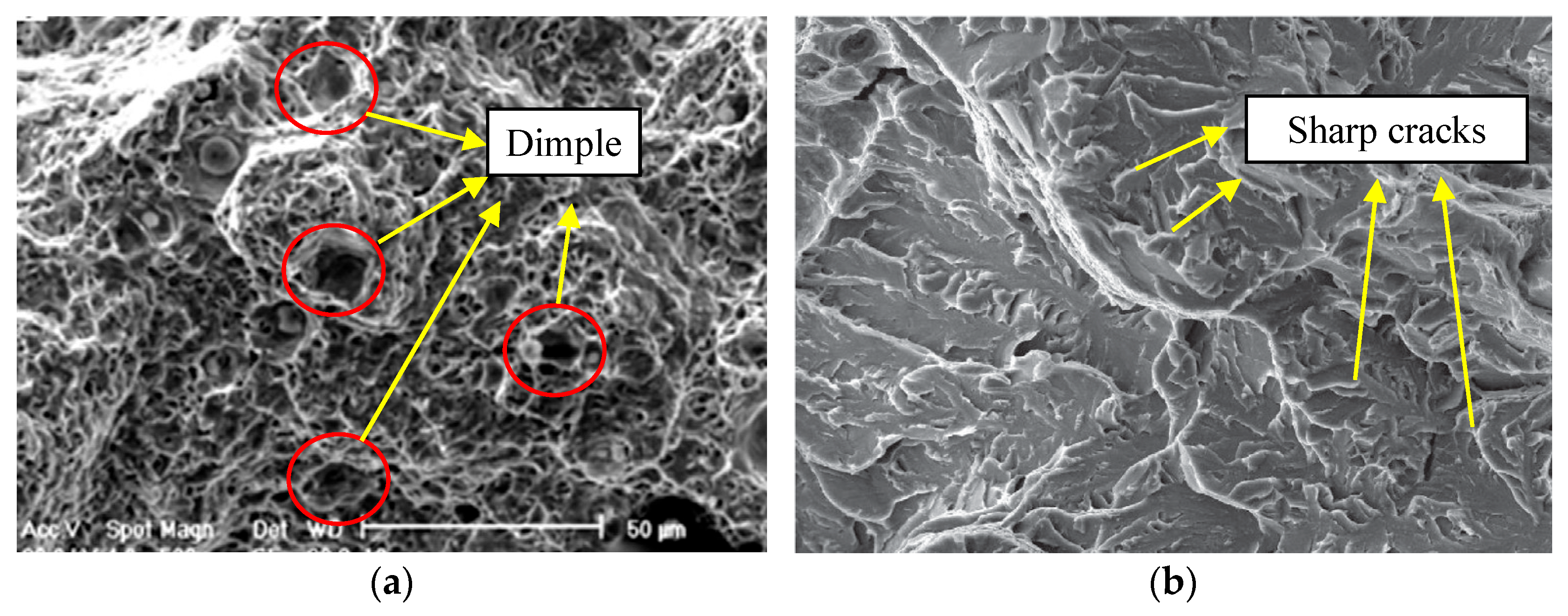


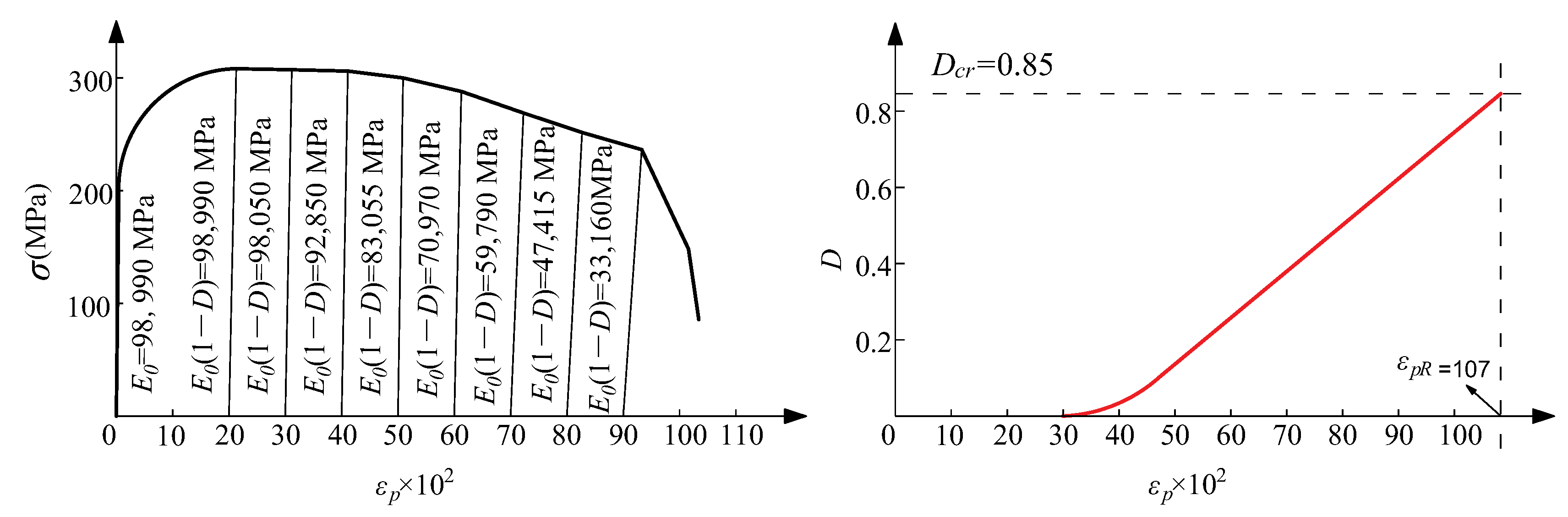
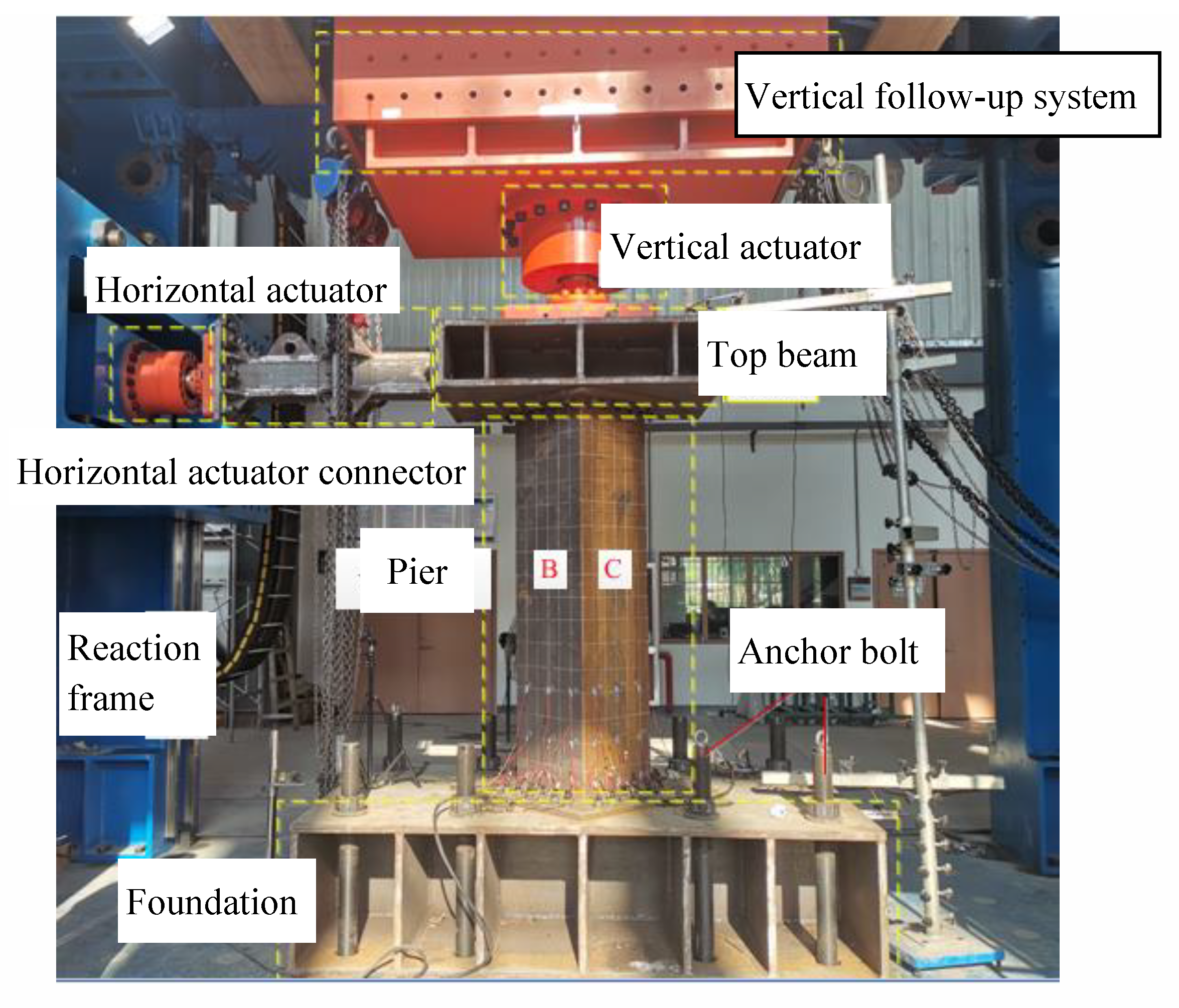


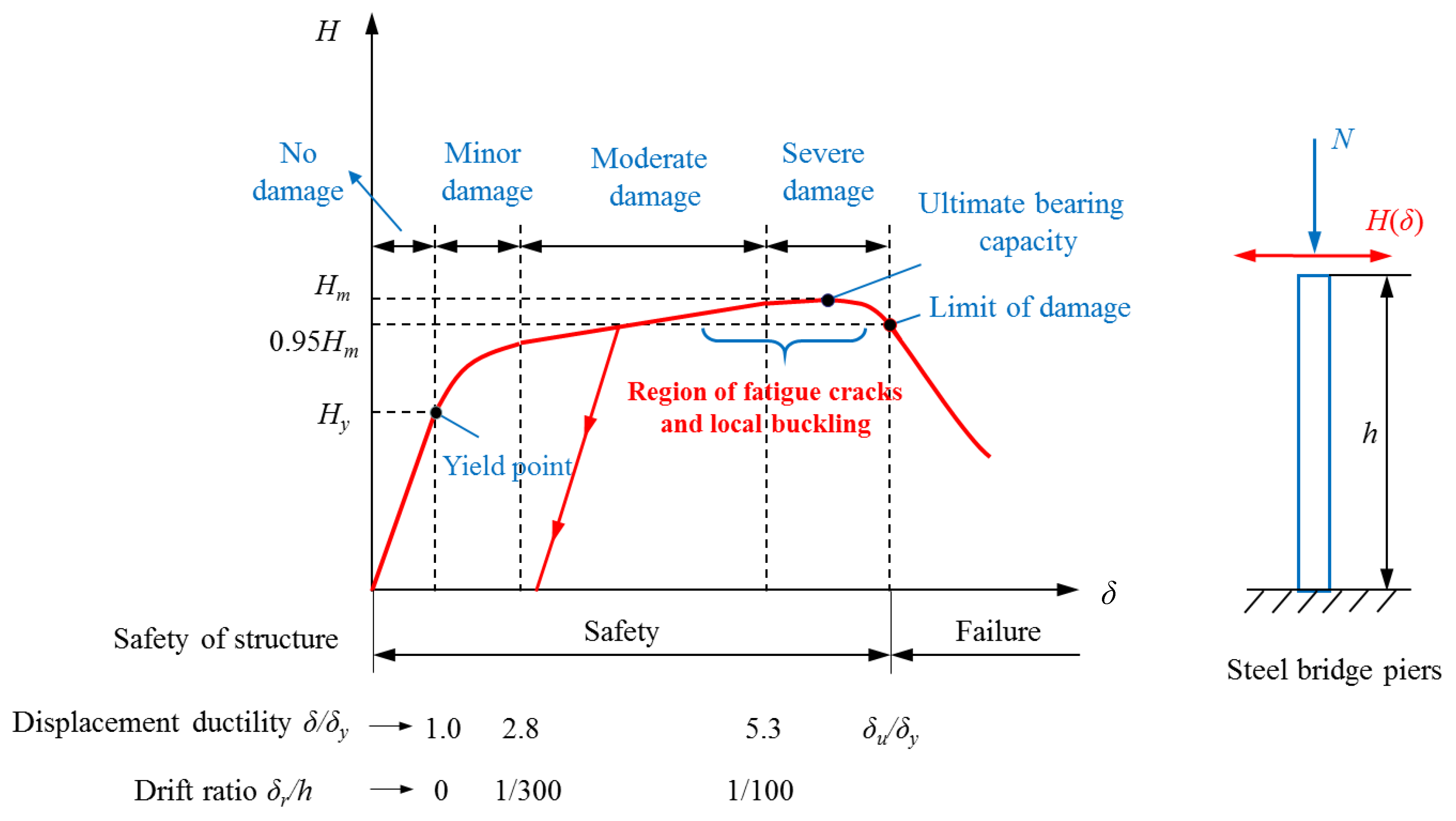
| No | Name | Advantages | Disadvantages |
|---|---|---|---|
| 1 | Empirical formula method | Few parameter calibration experiments; easy to integrate with finite element software; high computational efficiency | Prediction of fatigue life cannot consider the influence of the spatial stress state |
| 2 | CVGM | Prediction of fatigue life can consider the influence of the spatial stress state; medium computational efficiency | Additional parameter calibration experiments; difficult to integrate with finite element software |
| 3 | CDM | Prediction of fatigue life can consider the influence of the spatial stress state; few parameter calibration experiments; easy to integrate with finite element software | Low computational efficiency |
| σy(MPa) | E(MPa) | μ | c | |
|---|---|---|---|---|
| 391.2 | 2.045 × 105 | 0.3 | 0.4441 | −0.6003 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Q.; Cao, S.; Lu, X.; Cheng, H. A Review of Prediction Methods for Ultra-Low Cycle Fatigue Damage of Steel Piers Under Earthquakes. Appl. Sci. 2025, 15, 5114. https://doi.org/10.3390/app15095114
Tian Q, Cao S, Lu X, Cheng H. A Review of Prediction Methods for Ultra-Low Cycle Fatigue Damage of Steel Piers Under Earthquakes. Applied Sciences. 2025; 15(9):5114. https://doi.org/10.3390/app15095114
Chicago/Turabian StyleTian, Qin, Shixin Cao, Xiaoming Lu, and Haigen Cheng. 2025. "A Review of Prediction Methods for Ultra-Low Cycle Fatigue Damage of Steel Piers Under Earthquakes" Applied Sciences 15, no. 9: 5114. https://doi.org/10.3390/app15095114
APA StyleTian, Q., Cao, S., Lu, X., & Cheng, H. (2025). A Review of Prediction Methods for Ultra-Low Cycle Fatigue Damage of Steel Piers Under Earthquakes. Applied Sciences, 15(9), 5114. https://doi.org/10.3390/app15095114





