Modeling and Simulation of Autonomous DC Microgrid with Variable Droop Controller

Abstract

The emergence of highly efficient and cost-effective power converters, coupled with the growing diversity of DC loads, has elevated the importance of DC microgrids to a level comparable with AC microgrids in the modern power industry. DC microgrids are free from synchronization and reactive power dynamics, making them more reliable and cost-effective. In autonomous mode, achieving effective voltage regulation and satisfactory power sharing is critical to ensuring the overall stability of the microgrid. As the common DC bus of the microgrid connects distributed generators (DGs), storage devices, and loads through power electronic converters (PECs), the controllers of these PECs must regulate the bus voltage effectively, track reference signals to meet power demands, and enable satisfactory load sharing. In this work, a real time decentralized droop controller is implemented for an islanded DC microgrid to enhance the voltage regulation at the DC bus and current sharing efficacy between the sources subject to load transients. A novel control strategy is presented in which the conventional droop control is modified considering the load dynamics. The performance of the proposed control strategy is compared with the conventional voltage droop control strategy. The fluctuations in the DC bus voltage, which is the major cause of voltage instability of the DC microgrid is effectively reduced by the proposed strategy. The proposed strategy is validated by comparing it with the conventional fixed droop control method on the MATLAB Simulink platform. The variable droop control strategy outperforms the fixed droop method by addressing sudden voltage fluctuations in the DC bus, which occur due to the inherent load current dependency of the fixed droop approach. This technique achieves enhanced voltage regulation, which is crucial for microgrid stability.

1. Introduction

The integration of renewable energy sources with advanced distributed storage technologies has enabled microgrids to deliver clean, reliable, and cost-effective energy to society [1,2]. Microgrids offer additional benefits, including voltage support, loss minimization, and enhanced power quality. Initially, AC microgrids gained more popularity than DC microgrids, primarily because of the transformers, which are the the core component of AC systems, naturally allowed adaptation to varying voltage and power levels as required. AC microgrids continue to dominate the modern power industry, as most existing power grids are AC-based, and the widespread adoption of purely DC microgrids has been limited. However, the advent of highly efficient, cost-effective power electronic converters (PECs) and the growing variety of DC loads has established a significant role for DC microgrids in the industry. DC microgrids offer distinct advantages, including being free of synchronization issues, reactive power challenges, and harmonic distortions, which makes them increasingly reliable in modern power systems. The benefits and challenges of DC microgrids, particularly in terms of efficiency, cost reduction, and integration with renewable energy, are discussed in [3,4]. In [5], modeling of a DC microgrid with various types of DGs and storage is performed and the scope of the system for sustainable power is investigated. 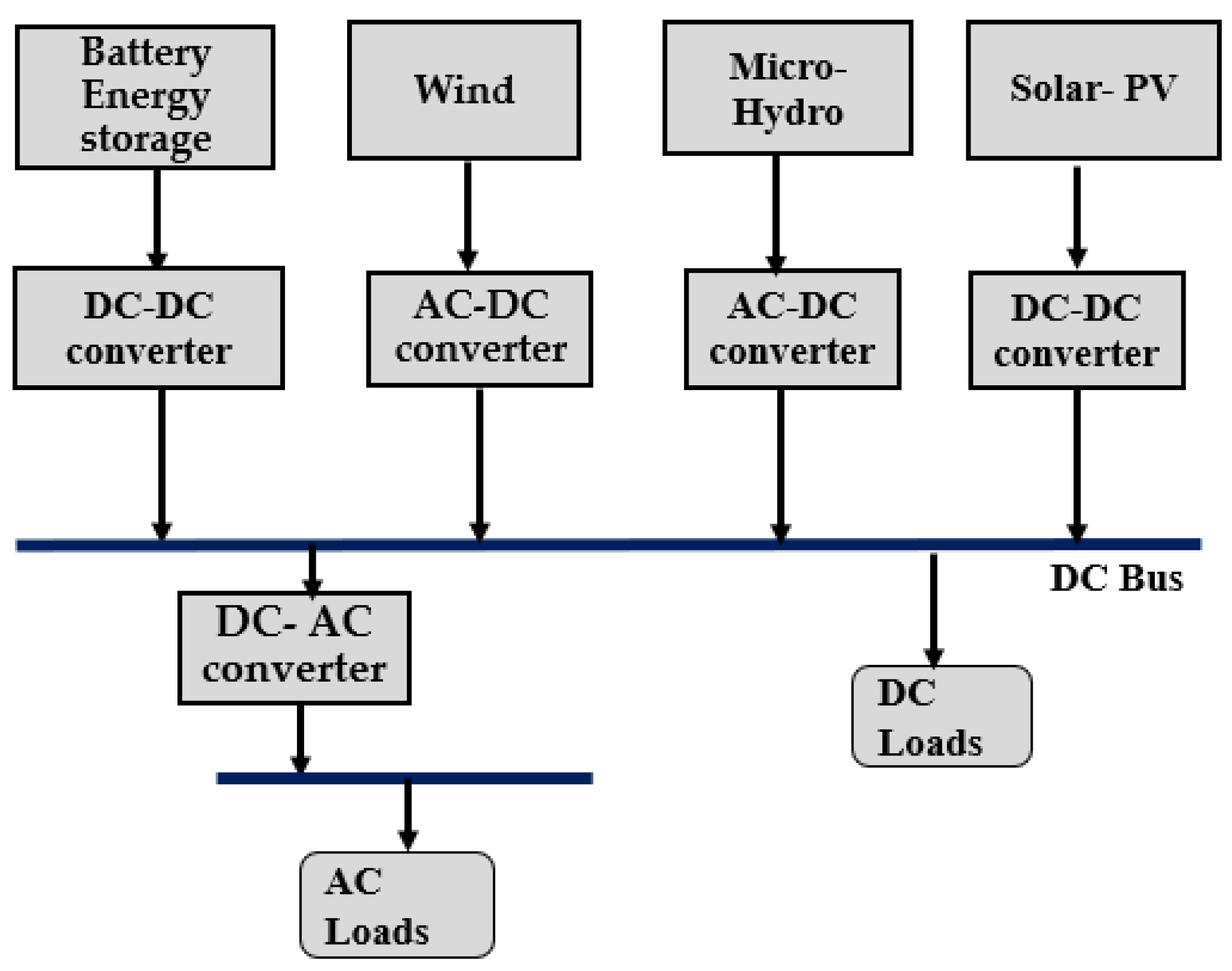Figure 1 shows a general architecture of the DC microgrid.

Various types of DGs are integrated with the DC bus using PECs. DC loads are connected directly, while AC loads are interfaced via AC–DC converters. The bus voltage and power levels vary depending on the specific application. DC microgrid control focuses on maintaining bus voltage stability and ensuring proportional power sharing between the sources. Maintaining stability, especially in autonomous mode, presents a significant challenge in microgrids. To address this, various control strategies have been developed. Voltage control of the DC bus primarily involves regulating the interfacing PECs. In DC microgrid control, decentralized droop control is a well-established technique for islanded operation. The basic principle behind droop control is to enhance the low output resistance of the PEC, which is often the primary cause of inadequate load sharing in a microgrid. This introduces a resistive droop characteristic into the system. However, the literature reveals that fixed droop control cannot fully address the inherent dependency of bus voltage deviation on load current. A decentralized variable droop controller for an autonomous DC microgrid is proposed to improve bus voltage regulation under varying load dynamics. The effectiveness of current sharing with the proposed control technique is also investigated.

This paper is organized as follows: Section 2 offers an in-depth review of the operation and control of DC microgrids. The proposed system is introduced in Section 3. The simulation results and a detailed analysis of the results are given in Section 4. The paper is finally concluded in Section 5, which also provides a summary of the main contributions and possible future research areas.

2. Literature Review

Microgrid operation is typically categorized into two distinct modes: utility-connected and autonomous. A primary challenge in autonomous operation is ensuring system stability, particularly in terms of voltage and frequency regulation. Achieving optimal power sharing between distributed generators (DGs), efficient load management, and seamless transitions between operational modes are critical for maintaining the overall stability and performance of the microgrid system [6]. Developing effective control systems enabling stable operation has been the focus of recent research, especially in islanded or autonomous mode where stability concerns are critical due to the lack of grid support [7,8,9]. The stability of the microgrid is intrinsically linked to the behavior of the DGs and loads, which must be carefully balanced to ensure reliable operation under varying conditions.

To address these challenges, both AC and DC microgrids commonly employ a three-level hierarchical control architecture, which is essential for ensuring effective current sharing and voltage stability. In grid-connected mode, all three levels of control are required: primary, secondary, and tertiary. These controls can be implemented through either centralized or decentralized approaches. In the case of centralized control, a microgrid central controller is responsible for coordinating the operation of all components to meet the desired performance criteria. This central controller typically oversees the management of energy flow, voltage regulation, and power sharing across the system. In contrast, decentralized control involves assigning local controllers to each converter, with the stability of the microgrid maintained through cooperative communication between these local controllers. This decentralized approach can improve system resilience and reduce the dependency on a single point of failure.

In islanded operation, tertiary-level control is often not required, simplifying the control structure. The primary control mechanism in DC microgrids is droop control, a well-established method for regulating power sharing and voltage stability. A virtual droop resistor is connected in series with the converter to achieve droop control, which modulates the output impedance of the power electronic converters (PECs). By adjusting the droop resistor value, the microgrid can achieve dynamic load sharing and voltage regulation without the need for centralized coordination [10]. This approach helps mitigate issues related to load imbalances and voltage deviations, thus enhancing the overall performance and reliability of the system under varying load conditions.

Droop control is the most widely used and effective decentralized method for power sharing in microgrids [11]. In converter-connected microgrids, the droop characteristics of each converter can be adjusted to ensure proper load sharing among distributed generators (DGs), eliminating the need for direct communication between them, particularly in islanded operation. Microgrid control is typically structured into three hierarchical levels. Primary control is the innermost feedback and feed-forward loops that regulate voltage and frequency in real time at the converter level, ensuring local stability and load sharing. Secondary control is the level that compensates for steady-state errors, such as voltage and frequency deviations due to droop control, by adjusting reference values for the primary control through a communication network. Tertiary control manages the power exchange between microgrids or between the microgrid and the utility, optimizing energy distribution and guaranteeing seamless transitions between grid-connected and islanded modes.

A comprehensive review of microgrid control and operation is presented in [12,13], which highlight key advancements and challenges in the field. Traditional droop control strategies have been explored in [14], but these methods often fail to address voltage deviations that occur under changing load conditions. Additionally, inaccurate load sharing due to variations in line impedance remains a significant limitation of fixed droop control. A performance comparison between P and PI controllers for voltage droop control is discussed in [15], revealing that while these methods can regulate voltage, they often suffer from poor current sharing and deviations in the bus voltage under varying load dynamics [16,17].

Ref. [18] proposes a modified droop control method that adaptively adjusts droop coefficients to reduce circulating currents and improve load-sharing accuracy. This approach enhances both voltage regulation and system stability, while mitigating the adverse effects of mismatched line impedances. The effectiveness of this adaptive droop method is validated through simulations and experiments under dynamic operating conditions, as demonstrated in [19].

Communication-based techniques for voltage regulation are explored in [20], where a low-bandwidth secondary control system dynamically adjusts droop coefficients to optimize microgrid performance. Ref. [21] implements a feed-forward compensation-based droop control for improved load sharing and bus voltage stability. In [22], an adaptive approach to tune the droop coefficient based on real-time system feedback is proposed to further enhance system performance.

Moreover, communication-enabled coordination techniques for improving stability in decentralized microgrids are discussed in [23]. In ref. [24] a disturbance observer-based droop control strategy is proposed to address uncertainties arising from renewable energy sources, while in [25] non-linear droop characteristics are used to improve microgrid performance under varying load conditions. This approach improves stability, especially in the presence of large load variations. However, challenges such as design complexity and the need for precise tuning persist.

Coordinated control of multiple sources for enhanced voltage regulation is explored in [26], where a combination of primary droop control with secondary and tertiary control layers leads to improved system performance. However, the need for a communication infrastructure increases the complexity of the system.

The performance comparison of various droop control strategies used in DC microgrids and the operating conditions is also reviewed and summarized as follows. Ref. [27] implemented an adaptive droop control strategy based on the virtual impedance method to improve voltage stability, regulation, and power flow management. This method is more suitable for microgrids with considerable variation in line impedance. In [28] a hybrid droop control strategy is utilized to reduce total generation cost and transmission power loss using suitable weighting factors which makes it more suitable for economical and efficient operating conditions. In [29], a complex droop-based method is used to tune the droop functions mainly to achieve the desired power management in hybrid DC microgrids. This type of droop control is more suitable for microgrids with high source and load dynamics. Ref. [30] proposes a variable droop control strategy for a hybrid energy storage system, with supercapacitors and batteries, to address power fluctuations in ship DC microgrids. This method is suitable for ship-board type applications where load dynamics is very high. Ref. [31] proposes a novel mixed integer-based optimization technique to design the optimal converter size and location in the microgrid network. This method is suitable for microgrids where optimal resource allocation is of high priority.

From the existing literature, the main research gap in the area of conventional fixed droop control strategy is that it cannot change dynamically to the load transients. The sharp voltage bus fluctuations due to the fixed droop resistor deteriorate the power quality. The variation in the droop coefficient with respect to the load in the proposed strategy successfully eliminates the sharp fluctuations in the DC bus voltage, thereby enhancing the transient performance subject to load dynamics. Variable droop control can effectively address the limitations of the fixed droop technique and contribute towards the enhancement of bus voltage stability in a DC microgrids.

3. Proposed Variable Droop Controller for DC Microgrid

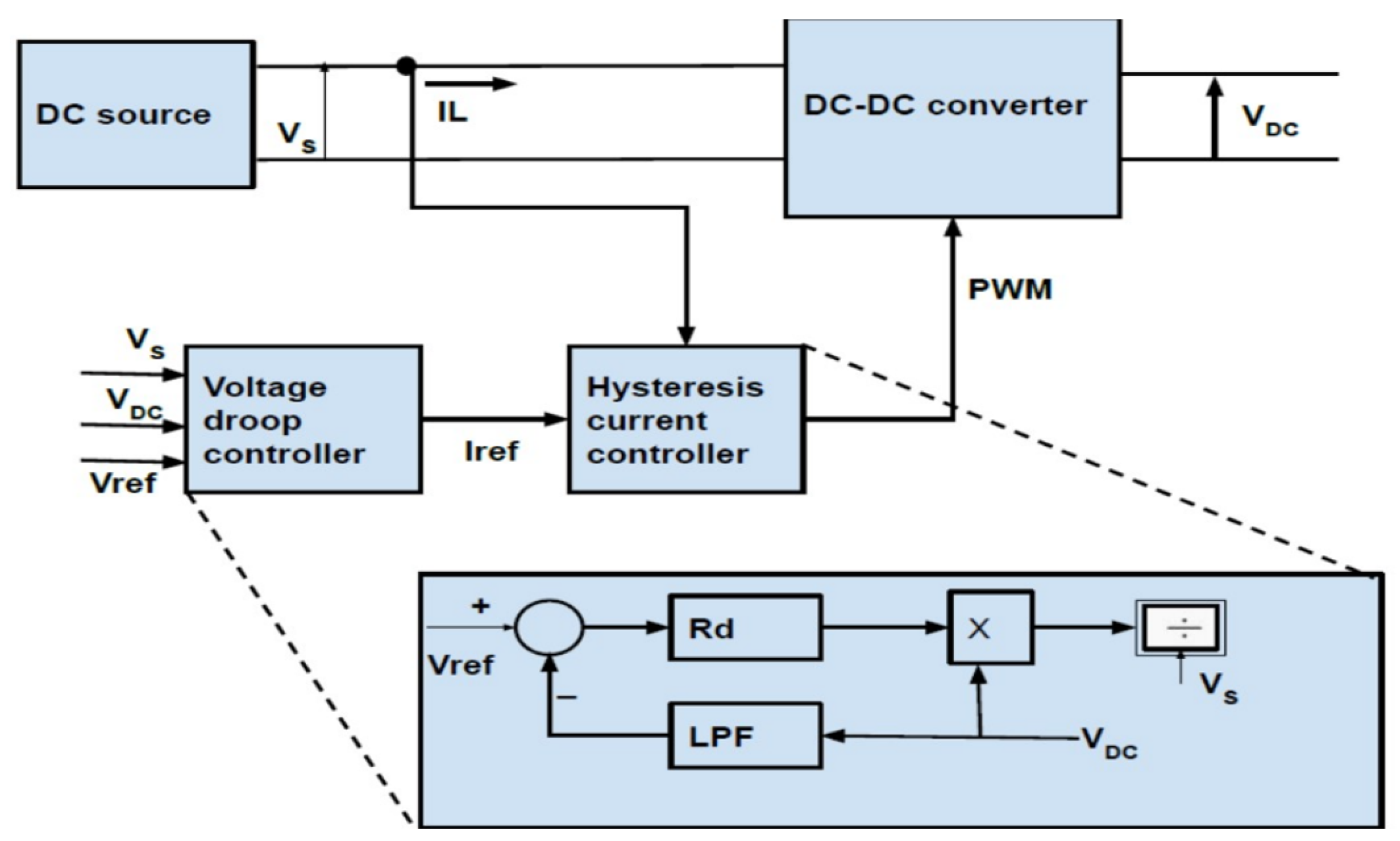
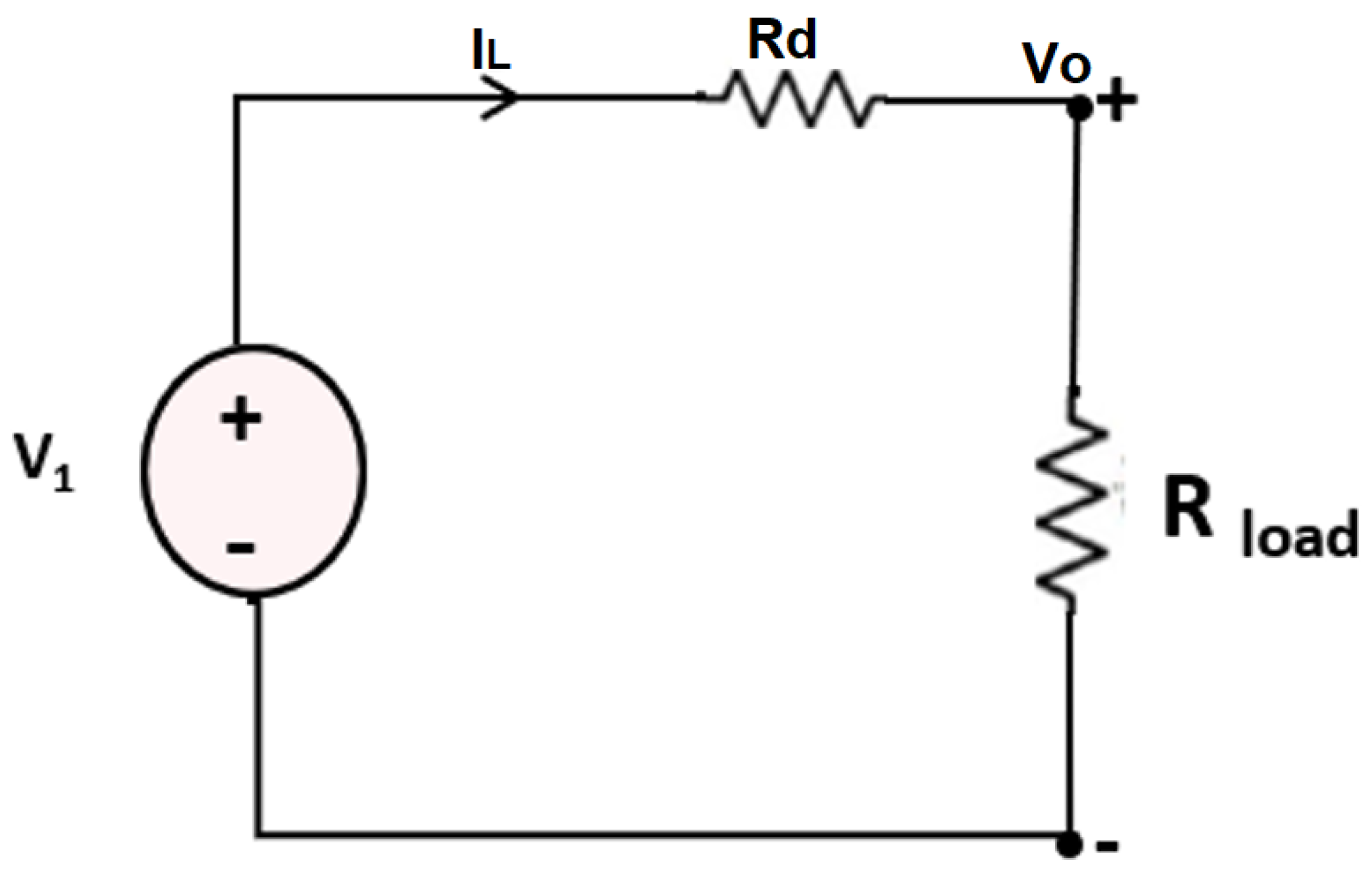
The proposed system shown in Figure 2 uses parallel connected boost converters with its own droop control system and have identical droop characteristics. All the n units have the same structure, but the current values depend on the droop resistor values for each converter. The converter with the highest droop value will contribute to the maximum current share. In the proposed system, identical PWM pattern is assumed for all units to simplify control coordination and accurate current sharing between the units. The DC bus voltage is 300 V. Each unit comprises a source, a PEC, and a droop controller. The DGs are modeled as constant voltage sources of 100 V, with the droop equation applied to define the droop characteristics. The block schematic of the proposed droop control scheme for a single unit is shown in Figure 3. The droop controller models the resistive behavior, resulting in a decrease in the converter output voltage as the source current increases. A low-pass filter is employed to reduce fluctuations at the DC bus. The filtered bus voltage is compared to the reference and the resulting error is processed by a proportional (P) controller. The output of the controller is multiplied by the DC bus voltage ($V_{DC}$) and divided by the source voltage to determine the required source current to meet the demand. The inner current control loop is regulated by a hysteresis controller, implemented using an SR flip-flop, which compares the reference current signal from the voltage control unit with the actual source current.

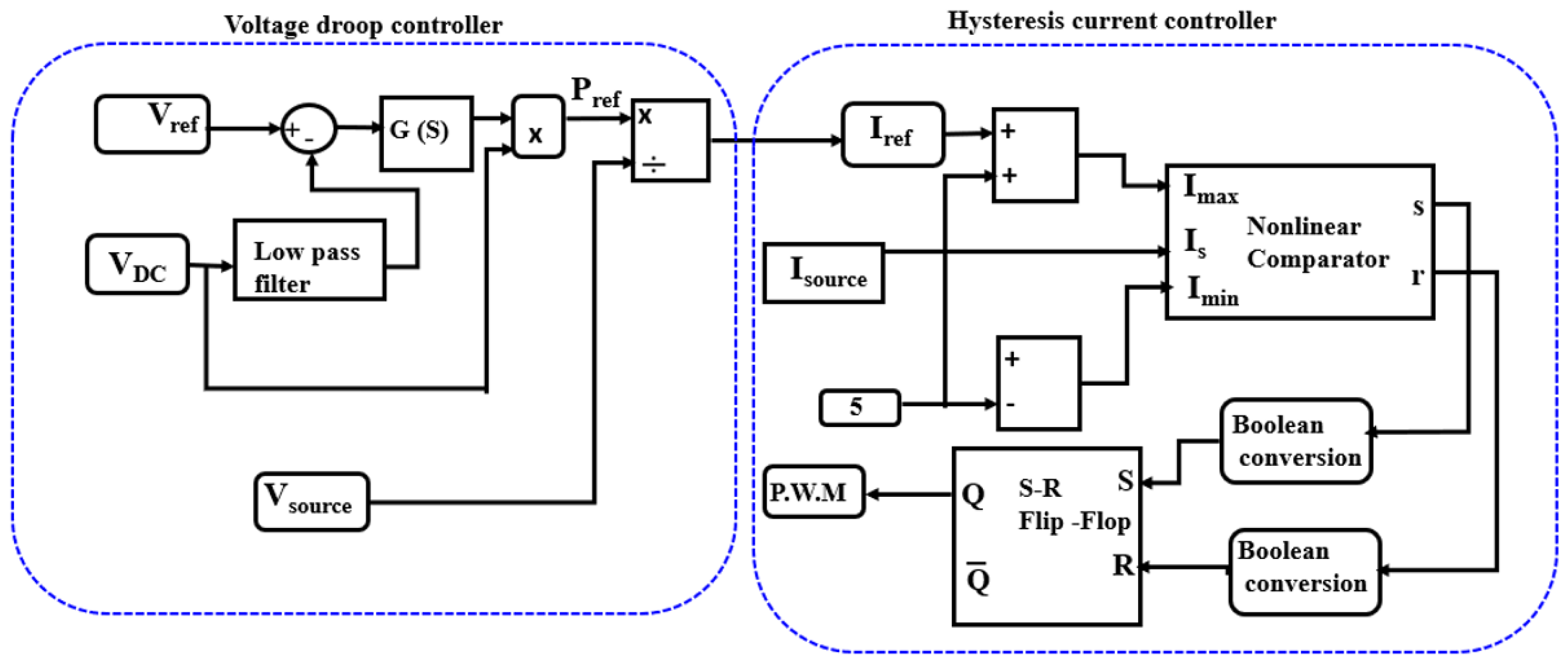
Figure 4 illustrates the proposed droop control scheme combined with hysteresis current control. In this work, the hysteresis current controller is used in the inner current control loop, which offers extremely fast and effective current tracking for microgrid applications. Compared to the classical PI, hysteresis control relies heavily on precise system modeling to a lesser extent and is inherently robust to variations in system parameters. Hysteresis current control is a straightforward technique often employed in power converters to generate an output current that closely follows a reference current waveform. The hysteresis band, defined by upper and lower tolerance limits, is set at ±5 A, based on the current ratings of both the source and the load. The switching signal is generated by comparing the error signal with the hysteresis band, ensuring that the output current remains within the specified tolerance. Equation (1) denotes the reference power generated by the voltage droop controller.

$$P_{ref}=G\left(s\right)(V_{ref}-V_{DC,filtered})·V_{DC}$$

(1)

$$I_{ref}={\displaystyle \frac{P_{ref}}{V_{source}}}$$

(2)

where $P_{ref}$, $V_{ref}$, and $I_{ref}$ are the reference power, voltage, and current, respectively. $G\left(s\right)$ is the proportional controller transfer function as given in Equation (3).

$$G\left(s\right)={\displaystyle \frac{1}{R_D}}$$

(3)

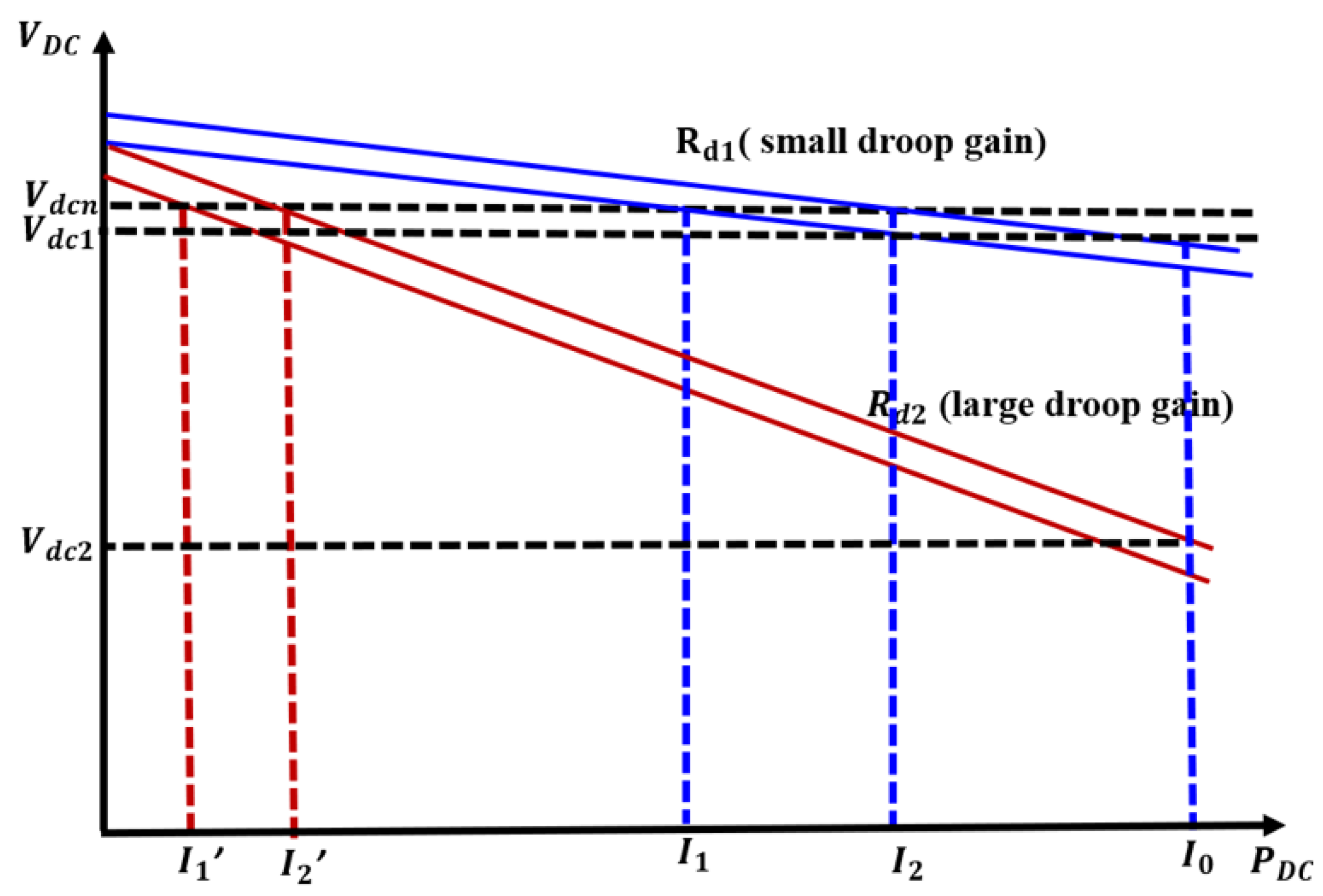
where $R_D$ is the droop coefficient. The relationship between terminal voltages and current sharing as a function of the droop values in a parallel connected converter system is depicted in Figure 5. Two sets of droop resistors are considered, characterized by different droop coefficients, $R_{d1}$ and $R_{d2}$, where $R_{d1}$ is smaller than $R_{d2}$. The nominal bus voltage is denoted as $V_{dcn}$. The resulting current sharing difference is represented by $I_2$-$I_1$ A for the droop coefficient $R_{d1}$ and $I_2^{\prime }$-$I_1^{\prime }$ A for $R_{d2}$.

The current sharing performance improves as the droop gain increases. To investigate the effect of droop gain on terminal voltage, consider a specific load current, $I_0$. For a smaller droop gain $R_{d1}$, the terminal voltage drops to $V_{dc1}$, while for a larger droop gain $R_{d2}$, the terminal voltage drops to $V_{dc2}$. As the droop gain increases, voltage regulation deteriorates. Therefore, the droop control strategy strikes a balance between terminal voltage and current sharing, offering a practical trade-off.

Design of Variable Droop Coefficient

The equivalent circuit of a PEC in the proposed system where each converter is represented as a source with voltage $V_1$ and a series droop resistor $R_d$ is shown in Figure 6. Here, the bus voltage $V_{DC}$ is expressed as in Equation (4).

$$V_o=V_1-I_L·r_d$$

(4)

$\delta$ indicates the voltage deviation at the DC bus when a load is applied and is given in Equation (5). The equation representing the $P-V$ droop characteristics for a DC microgrid is given in Equation (6).

$$\delta =I_L·r_d$$

(5)

$$V_{DC}=V_{NL}-k{P}_{DC},$$

(6)

where $V_{NL}$ is the voltage of the bus without load. $P_{DCmax}$ is the rated power of the source and $V_{min}$ is the minimum voltage corresponding to $P_{DCmax}$ and k is the slope of the droop characteristics accounted as the negative droop coefficient. $P_{DC}$ and $V_{DC}$ correspond to the operating point at any instant. $P_0$ and $V_0$ represent the nominal operating point. From Equation (6),

$$k={\displaystyle \frac{V_{NL}-V_{min}}{P_{DCmax}}}$$

(7)

The droop coefficient is obtained as

$$k={\displaystyle \frac{305-295}{5000}}=0.002$$

(8)

Corresponding to $P_{DCmax}$, $I_{DCmax}$ can be found as

$$I_{DCmax}={\displaystyle \frac{P_{DCmax}}{V_{min}}}=17A$$

(9)

and the droop resistor value is 0.588, obtained by substituting the values in

$$R_D={\displaystyle \frac{305-295}{17}}=0.588$$

(10)

The L and C values are obtained from the filter section design of the converter.

The load dynamics can be accounted by modifying this as Equation (11).

$$\delta \left(t\right)=I_L\left(t\right)·r_d\left(t\right)$$

(11)

The instantaneous value of $\delta$ is denoted as $\delta \left(t\right)$. $r_d\left(t\right)$ and $I_L\left(t\right)$ represent the values of the load current and the droop resistor at any instant. Differentiating both sides with respect to time, Equation (12) is obtained.

$${\displaystyle \frac{d}{dt}}\delta \left(t\right)=I_L\left(t\right)·{\displaystyle \frac{d}{dt}}{r}_d\left(t\right)+r_d\left(t\right)·{\displaystyle \frac{d}{dt}}{I}_L\left(t\right)$$

(12)

$\delta \left(t\right)$ is to be minimized for maximum dynamic stability. Hence, equating Equation (12) to zero, Equations (13) and (14) are obtained.

$$I_L\left(t\right)·{\displaystyle \frac{d}{dt}}{r}_d\left(t\right)=-r_d\left(t\right)·{\displaystyle \frac{d}{dt}}{I}_L\left(t\right)$$

(13)

$${\displaystyle \frac{d}{dt}}{r}_d\left(t\right)=-{\displaystyle \frac{r_d\left(t\right)}{I_L\left(t\right)}}·{\displaystyle \frac{d}{dt}}{I}_L\left(t\right)$$

(14)

Integrating Equation (13) with respect to time, Equation (15) to Equation (17) are obtained.

$${\int }_0^t{\displaystyle \frac{d}{dt}}{r}_d\left(t\right)dt=-{\int }_0^t{\displaystyle \frac{r_d\left(t\right)}{I_L\left(t\right)}}·{\displaystyle \frac{d}{dt}}{I}_L\left(t\right)dt$$

(15)

$$r_d\left(t\right)-r_d\left(0\right)=-{\int }_0^t{\displaystyle \frac{r_d\left(t\right)}{I_L\left(t\right)}}·{\displaystyle \frac{d}{dt}}·I_L\left(t\right)$$

(16)

$$r_d\left(t\right)=r_d\left(0\right)-{\int }_0^t{\displaystyle \frac{r_d\left(t\right)}{I_L\left(t\right)}}·{\displaystyle \frac{d}{dt}}{I}_L\left(t\right)dt$$

(17)

$r_d\left(0\right)$, the initial value of $r_d\left(t\right)$ is set as 0.5. To minimize $\delta \left(t\right)$ a proportional controller is implemented in the voltage control loop. Enhanced DC bus voltage regulation is attained since the value of $r_d$ changes according to the value of the load current. The hysteresis controller is realized in a MATLAB Simulink, R2018 A, with a nonlinear comparator having a dead band of ±5 A, so that a high speed of response for the converter is achieved with accuracy.

4. Results and Discussion

Table 1. System parameters.

System Parameters	Values
$V_{NL}$	305 V
$V_{min}$	295 V
$V_{DC}$ nominal value	300 V
$P_{DCmax}$	5 kW
P-V droop coefficient, k	0.002
Source side inductor	0.763 × 10−4 H
DC bus capacitor	0.01 F
Initial value of $R_d$	0.5

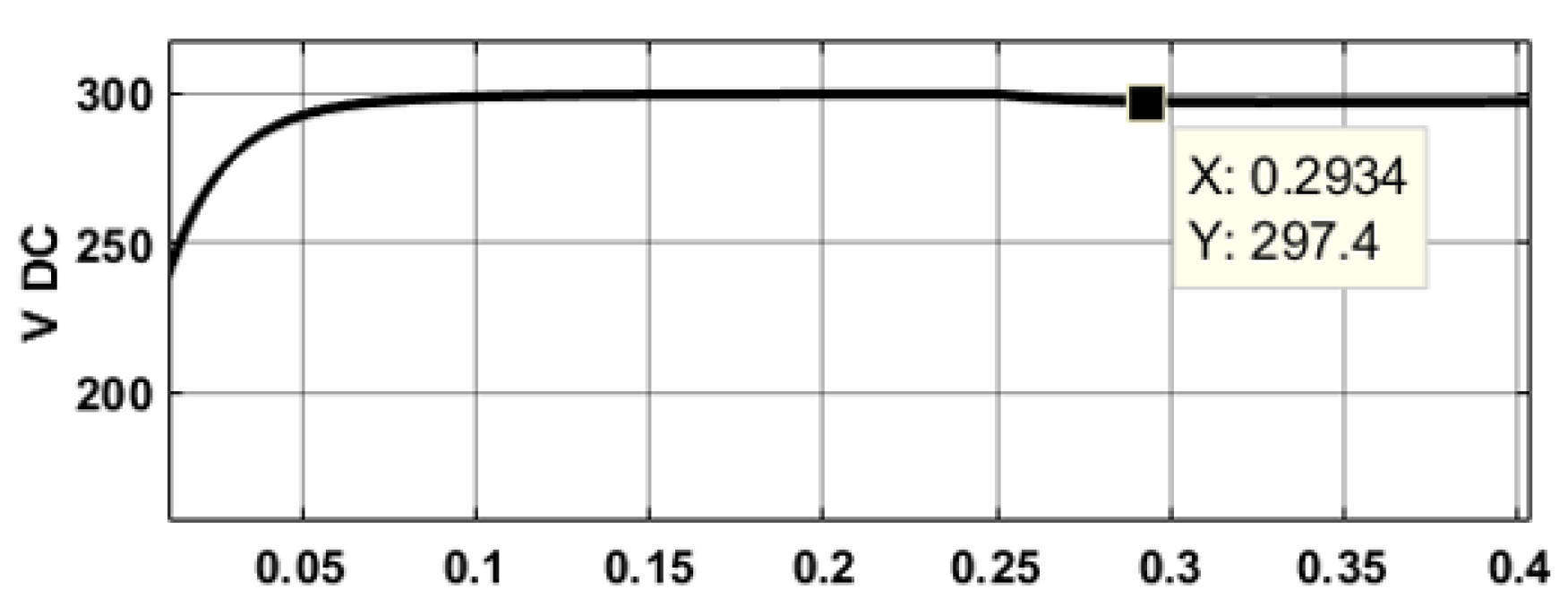
includes the system parameters for MATLAB simulation. With $V_{NL}-V_{min}$ set as 10 V and $P_{DCmax}$ as 5 kW, the P-V droop coefficient was obtained as 0.002. The nominal DC bus voltage was 300 V. The droop resistor was designed for $r_d=0.588$. The voltage regulation subject to varying load was primarily investigated with the variable droop control and compared with the fixed droop control. The current sharing was also compared for different load cases. The load transients of 1.5 KW were introduced at 0.25 s, 0.5 s, and 0.8 s, respectively.

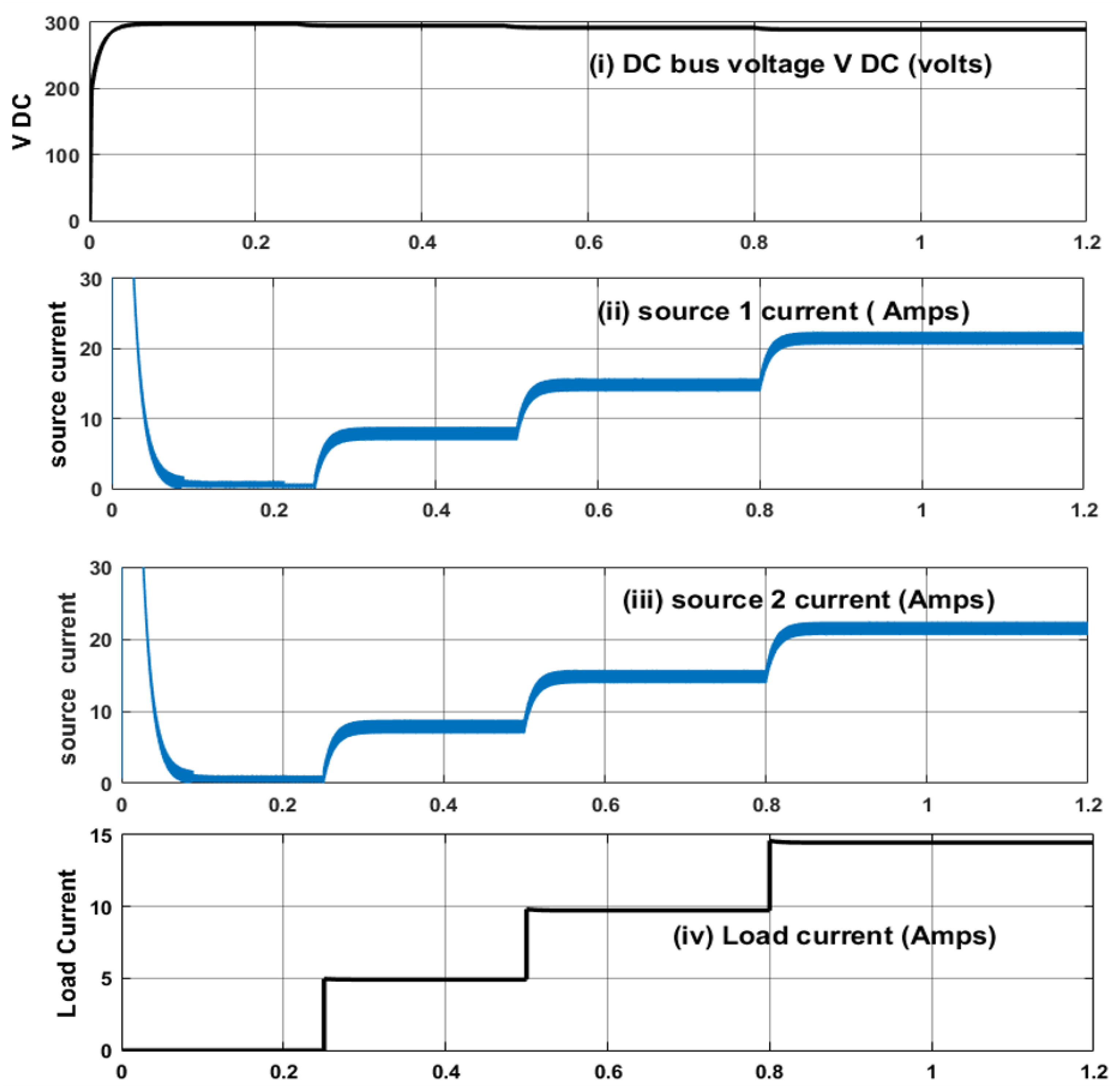
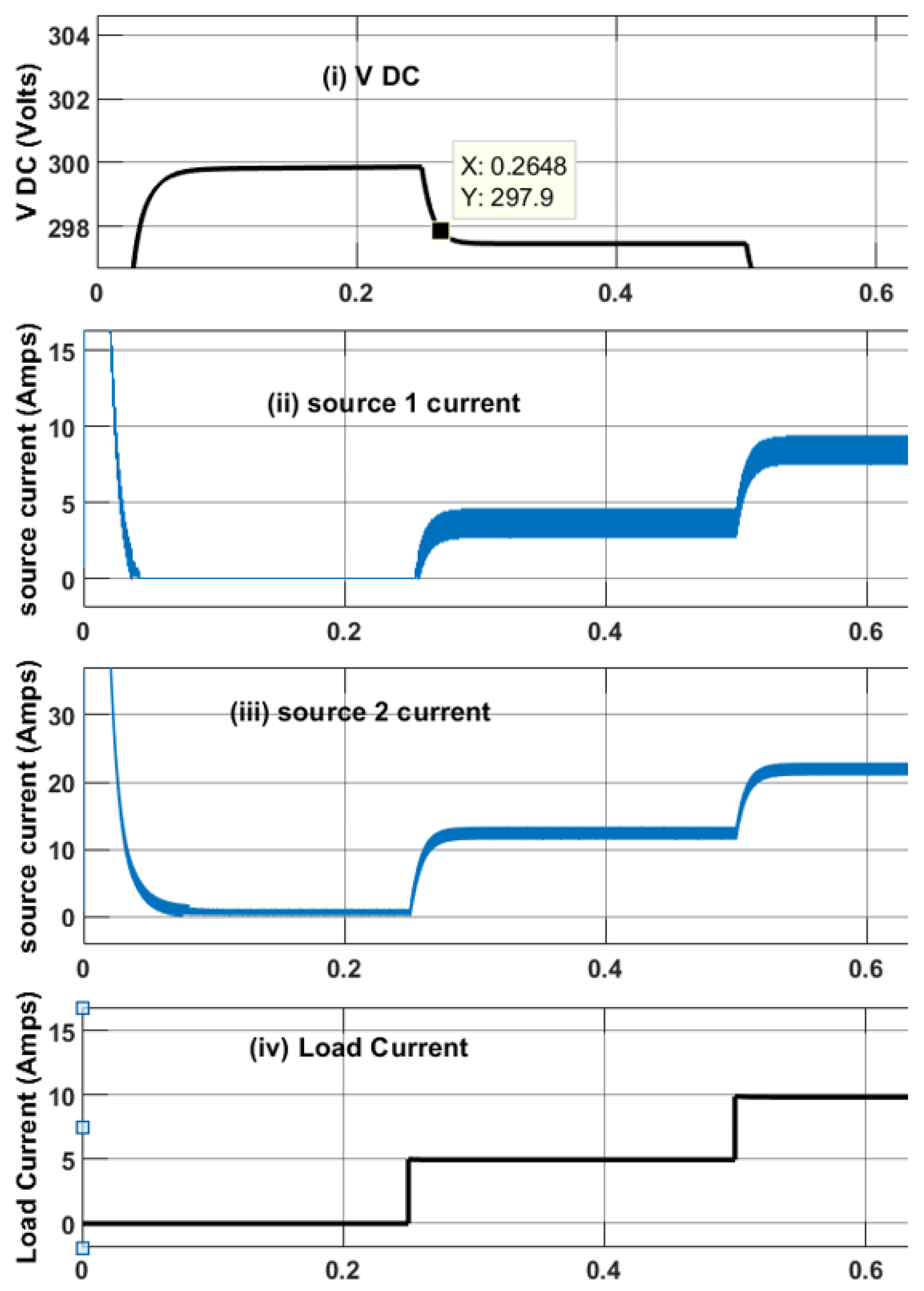
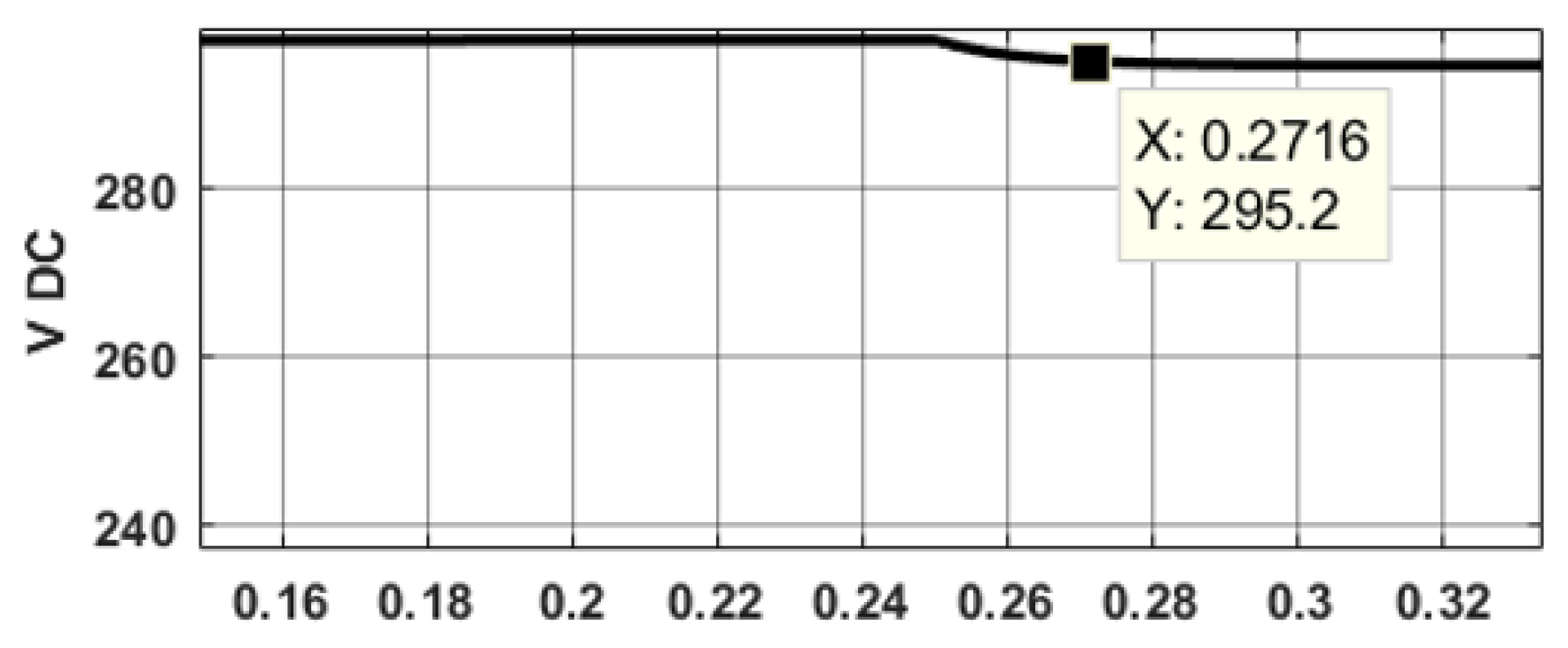
Waveforms in Figure 7 and Figure 8 shows the DC bus voltage, the current shared by the two parallel connected converters, and the load transients with both fixed and variable droop control. With the fixed droop control, the bus voltage drops quickly in response to the transients which will in turn affect the stability of the microgrid. With the variable droop control, the bus voltage obtained for the first load transient is 297.4756 Volts, while for the fixed droop control it is 295.2776 Volts. For a total load current of 15 amperes, the total source current must add up to 45 A. Hence, the current shared by each source is 22.5 A. Since the converter transformation ratio is 3, the total source current is higher than the load current thereby maintaining a power balance in the system.

With variable droop control, the droop resistor adjusts its value according to the change in load and thus eliminates sharp deviations. The value of $r_d$ changes to a lower value at the instant of load switching and then gradually varies to produce a smooth voltage deviation instead of a sudden fluctuation, which is not desirable for the stability of the microgrid.

The droop resistor adjusts its value according to the change in load and thus eliminates sharp deviations. The value of $r_d$ changes to a lower value at the instant of load switching and then gradually varies to produce a smooth voltage deviation instead of a sudden fluctuation that leads to unstable microgrid operation. The voltage regulation achieved with fixed droop control is 1.57 %, while with variable droop it is 0.84 %. The results indicate the supremacy of the variable droop controller in maintaining the voltage regulation in comparison to the fixed droop controller. The waveforms that confirm these results are shown in Figure 9 and Figure 10. 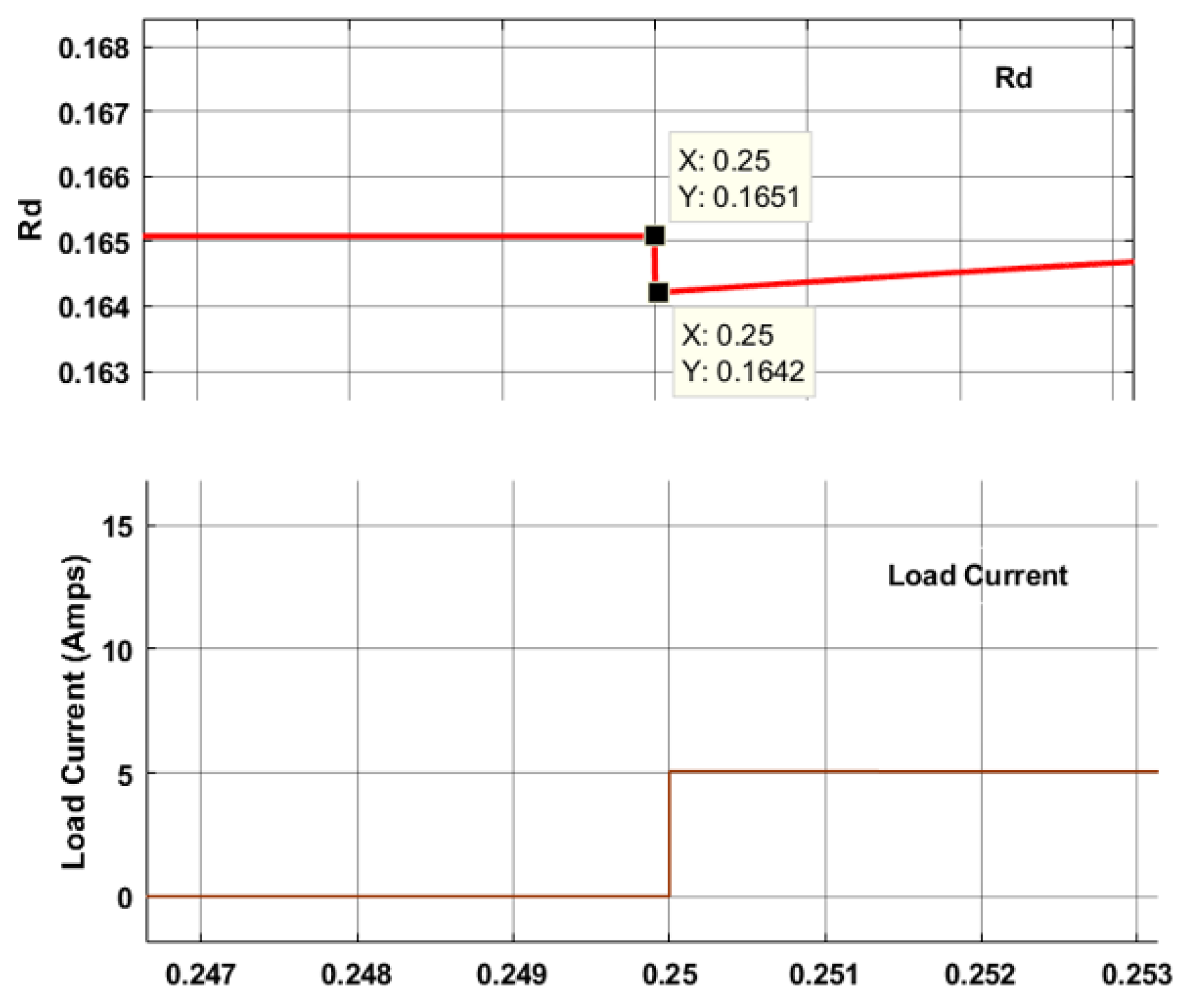Figure 11 shows the variation in the droop resistor subject to load change. At 0.25 seconds, when the load transient of 2.5 kW is introduced, the droop resistor changes its value from 0.1651 to 0.1642. 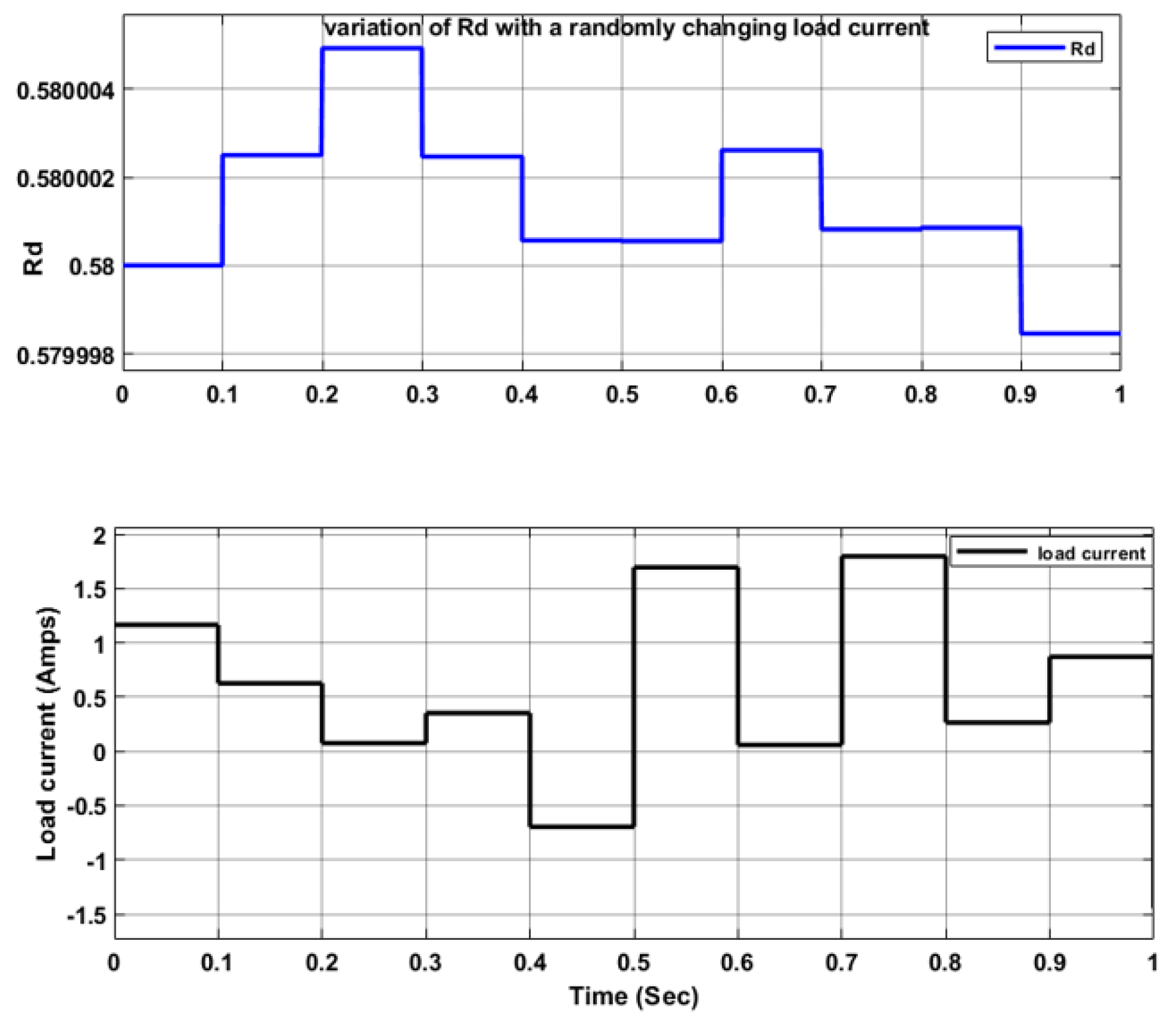Figure 12 illustrates the variation in $r_d$ for a randomly varying load current.

5. Conclusions

In this study, a decentralized voltage droop control strategy for DC microgrids in autonomous operation has been explored. The limitations of the fixed droop control is addressed by introducing a dynamic droop resistor that adapts in real time to load transients. The proposed variable droop control strategy effectively mitigates sharp voltage fluctuations, significantly improving voltage regulation and ensuring higher power quality. Although both fixed and variable droop controllers can achieve satisfactory current sharing, the variable droop approach offers distinct advantages in terms of stabilizing the DC bus voltage under varying load conditions. In situations when demand profiles are variable or unpredictable, this improved voltage stability adds to the overall dependability and effectiveness of DC microgrids. The key assumptions made in the proposed model are as follows:

1.

Approximating the nonlinear model of the DC microgrid system as a linearized model.

2.

Replacing the communication from the secondary level control to the droop controller as manual input.

3.

The generation is assumed stable and the renewable energy generation dynamics is not considered and emphasis is laid on the load power dynamics and the application of droop control strategies for the bus voltage stability problem.

Further studies could also explore the integration of advanced control techniques, such as model predictive control or machine learning-based approaches, to improve real-time performance and resilience under diverse operating conditions. Future extension of this work is possible by realizing a hybrid microgrid in which the DC- or AC-based devices can be connected directly to the network with limited interface elements, decreasing the conversion losses and cost. The need for the synchronization of generation units and storage units is eliminated since they are directly connected to either the DC or AC network. Developing efficient coordinate control strategies for power flow management between the subgrids is a potential area of research. In cases where the AC microgrid is grid connected and the DC loads are managed by the DC subgrid, this control strategy could be adapted with sufficient modifications considering the voltage range and power level of the DC bus. The challenges might include the design of power converters, filter components, droop coefficients, communication for secondary level control, etc.