The Mechanical Properties of Reinforced Concrete Columns with Longitudinal Pre-Embedded Holes
Abstract
1. Introduction
2. Finite Element Model Building
2.1. Model Establishment
2.2. Material Constitutive Model
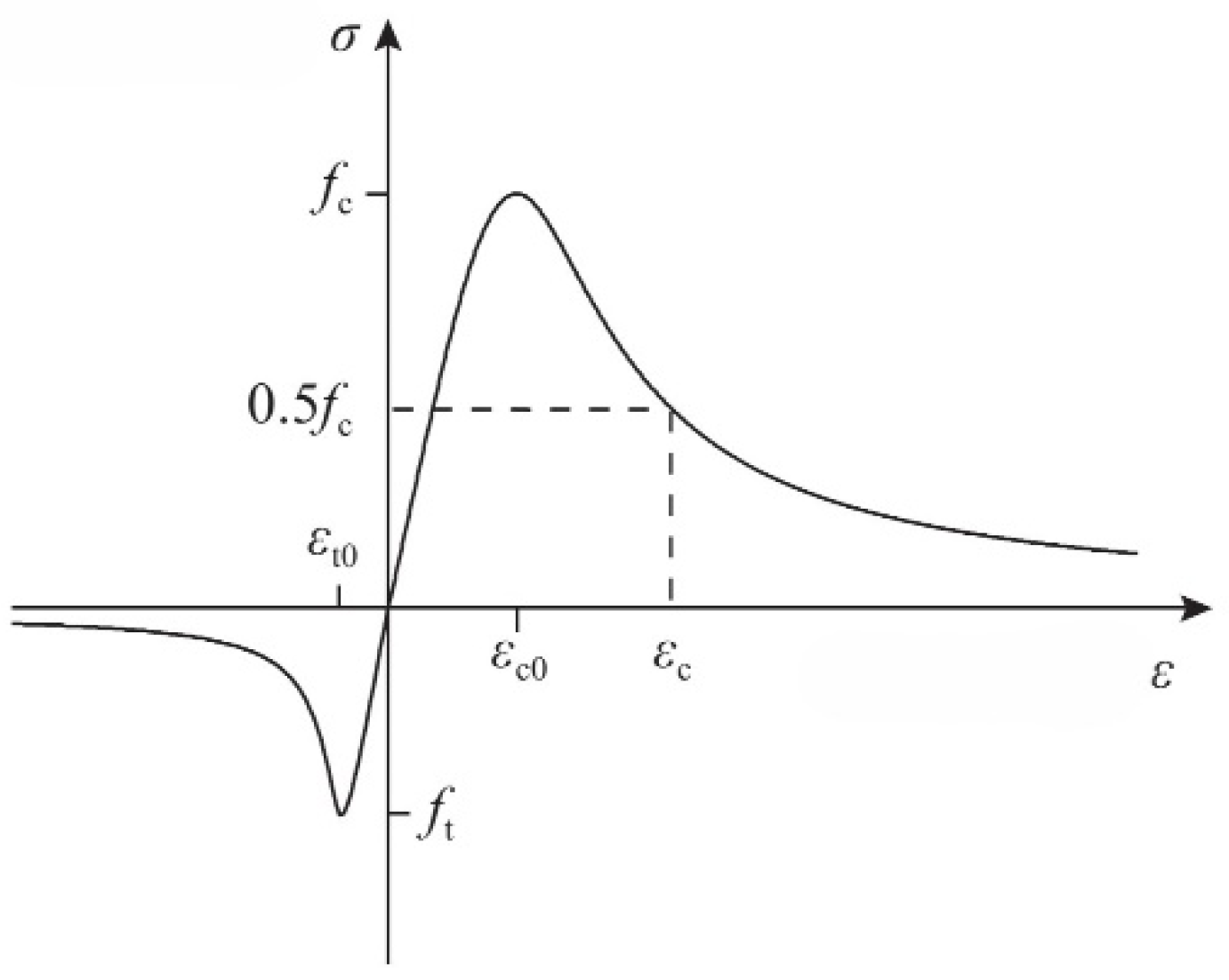
2.2.1. Concrete Constitutive
- where ; ; .
- where —the characteristic value of the uniaxial compressive strength of concrete;
- where ; .
- where —the characteristic value of the uniaxial tensile strength of concrete;
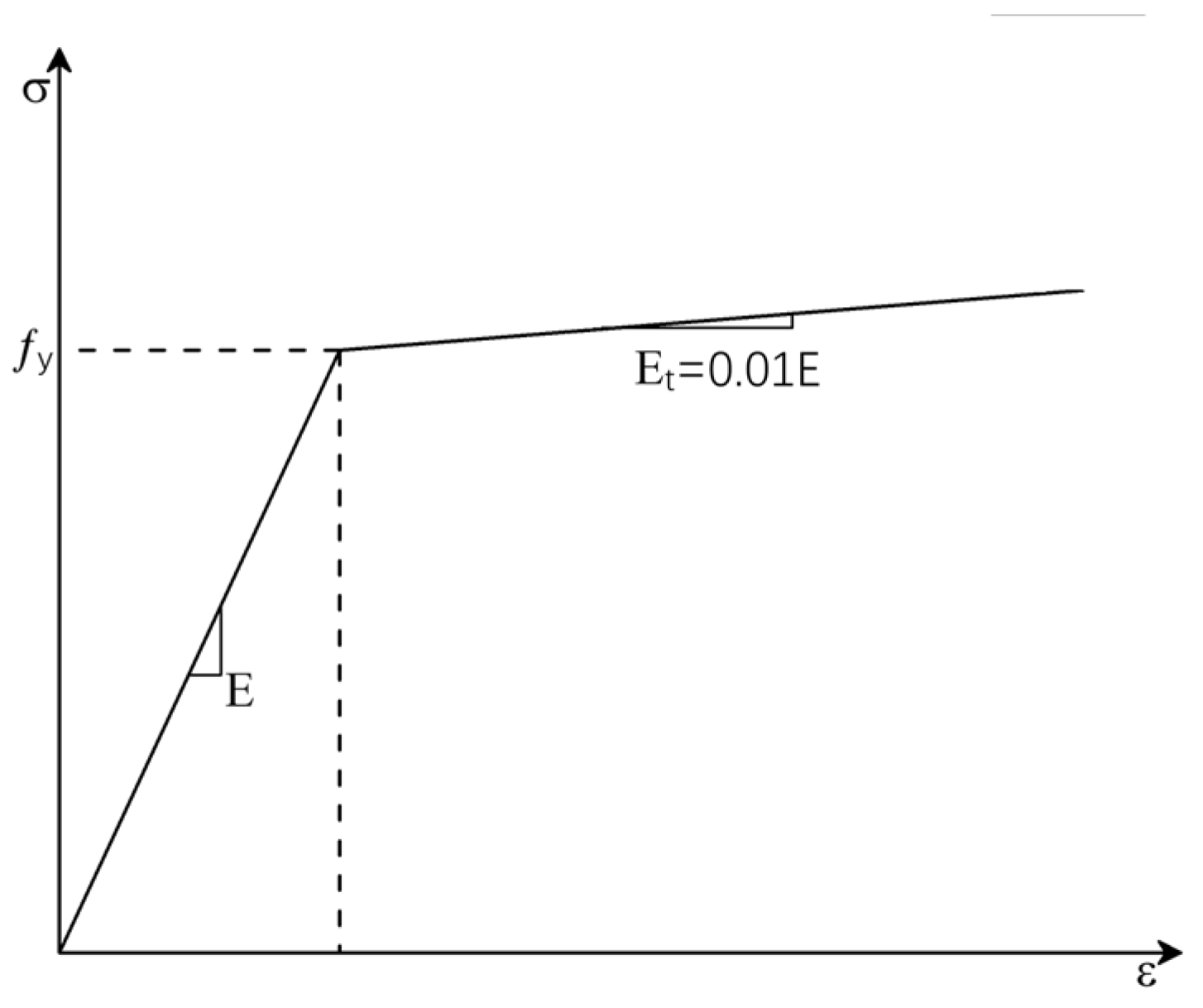
2.2.2. Constitutive Reinforcement
2.3. Element Selection and Mesh Division
2.4. Contact and Boundary Conditions
2.5. Finite Element Model Verification
3. Results and Discussion
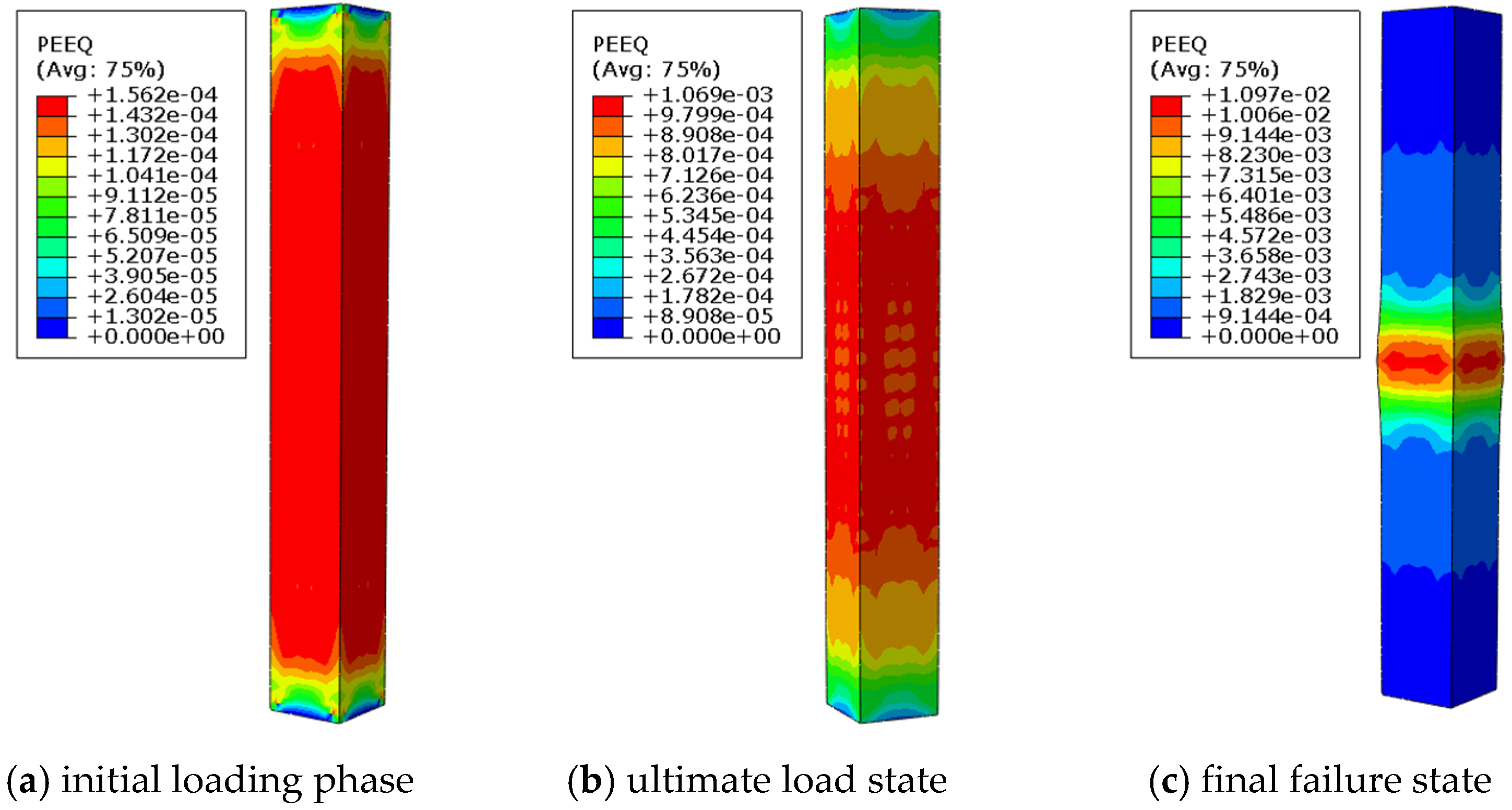
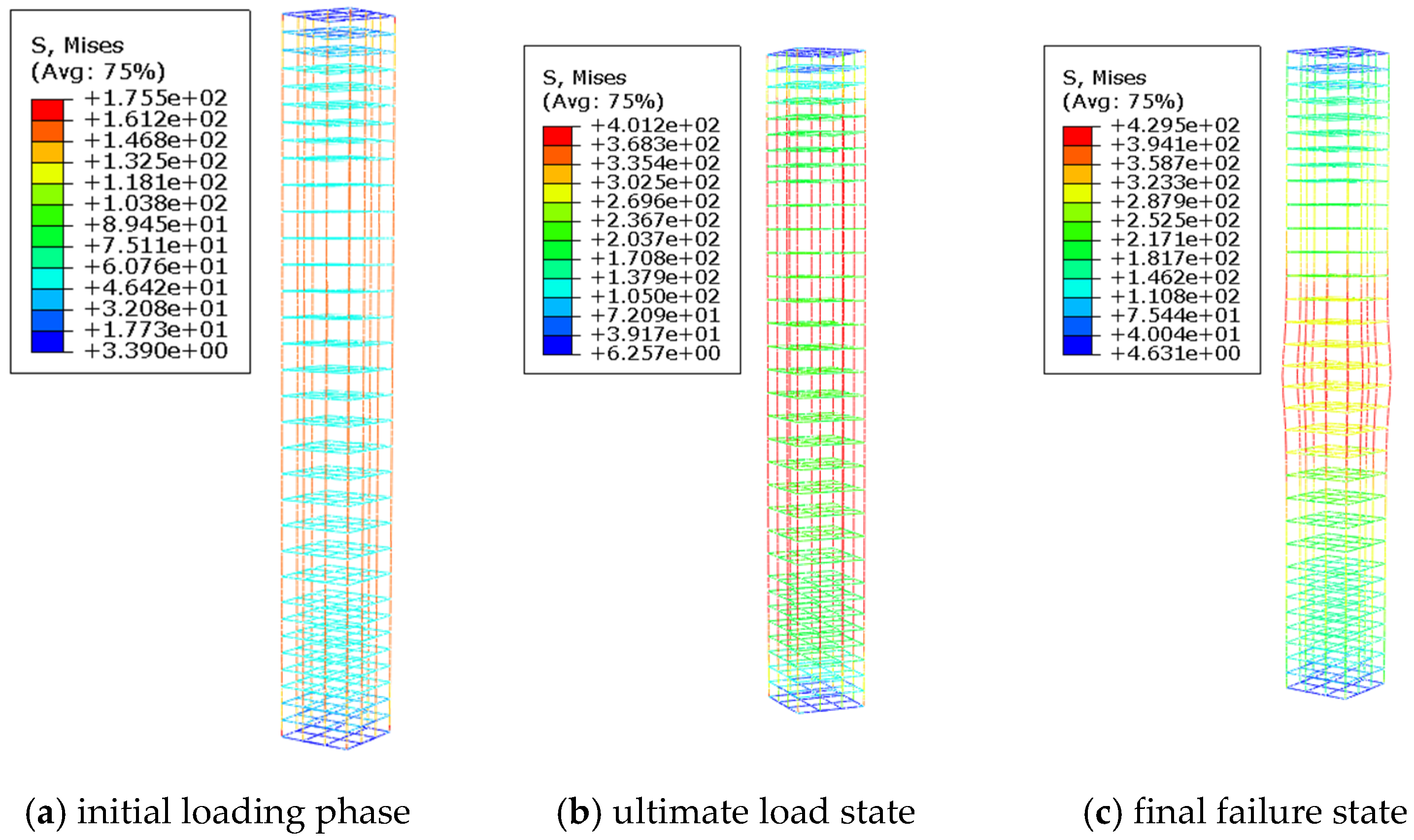
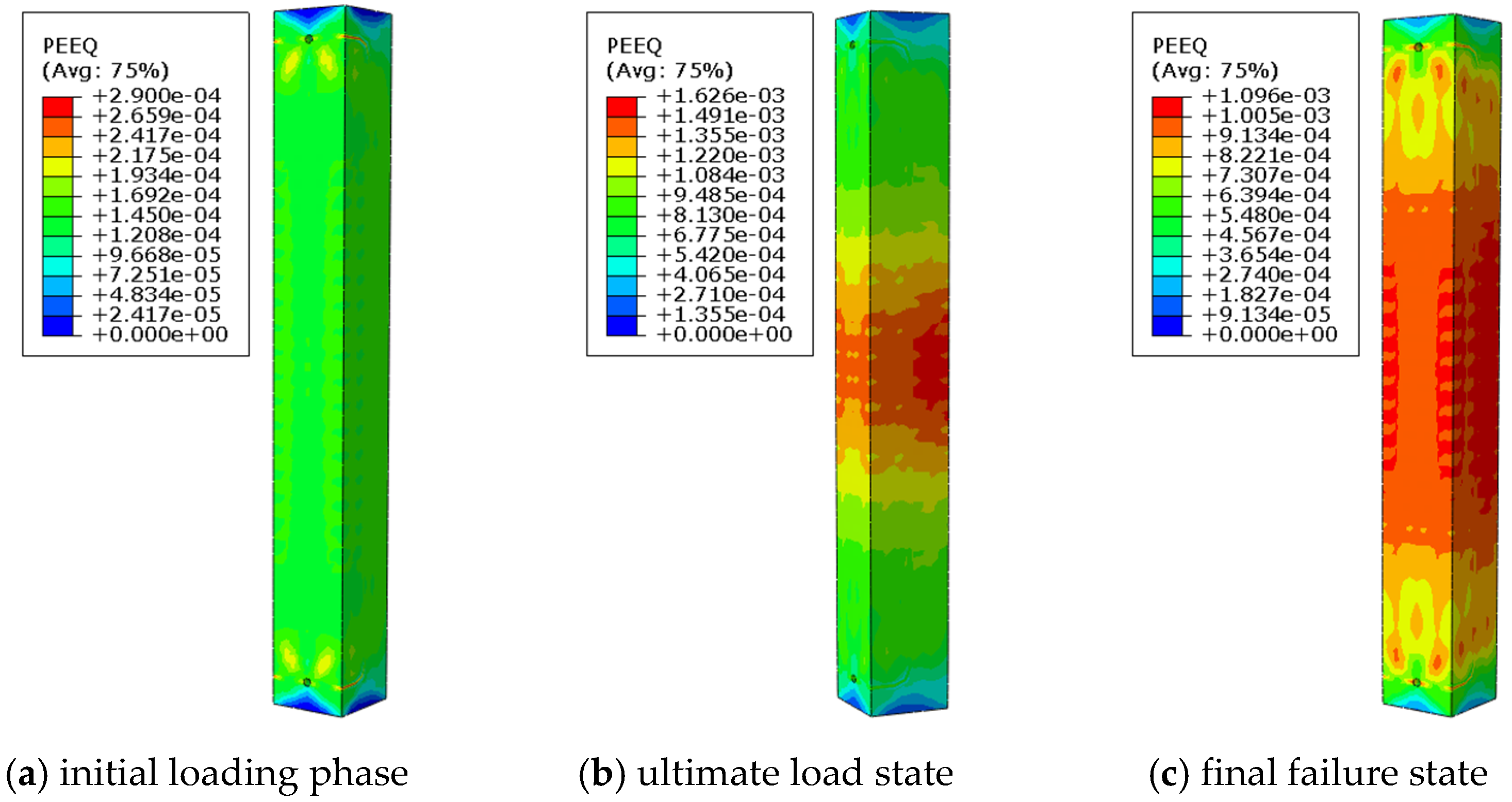
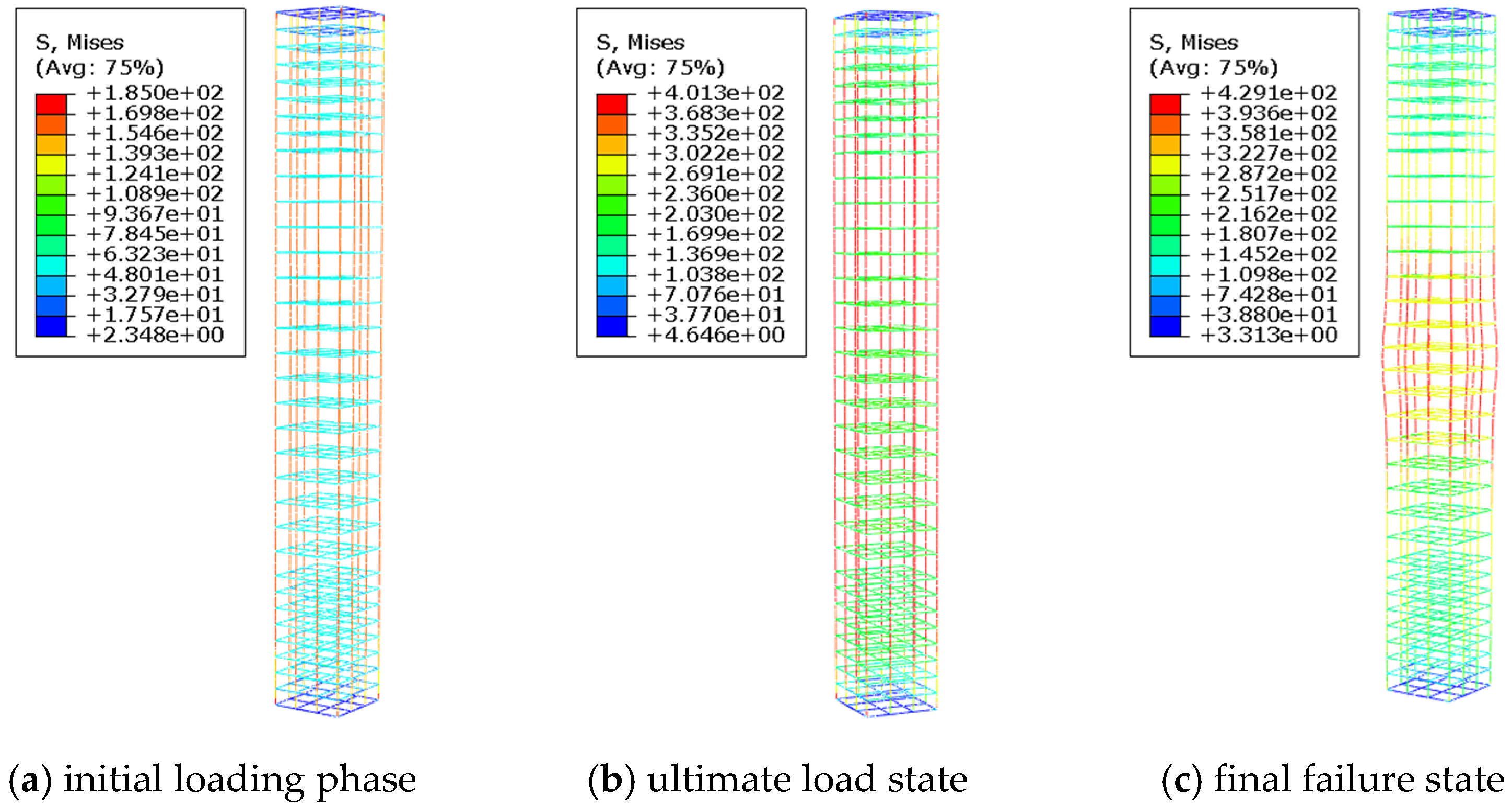
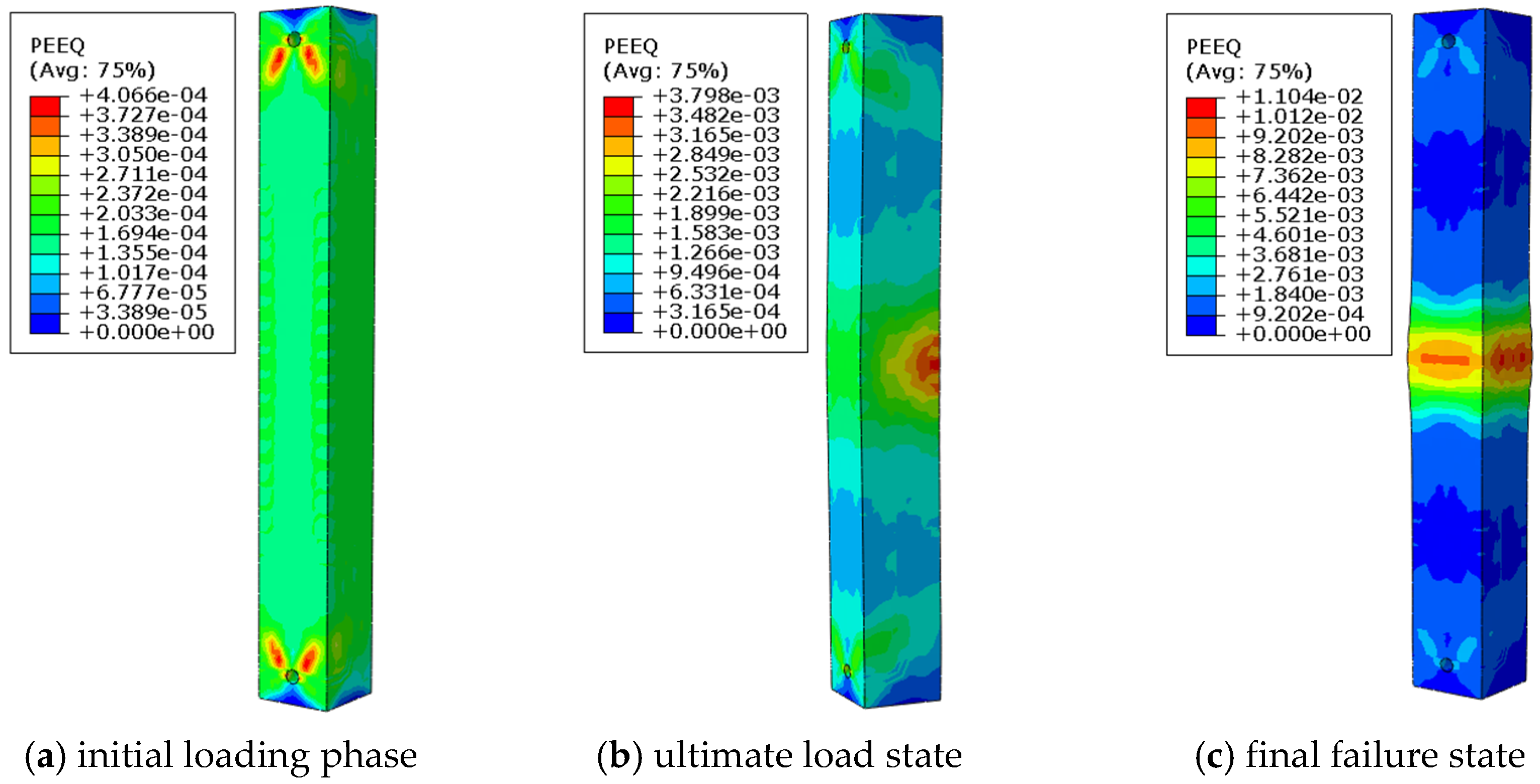
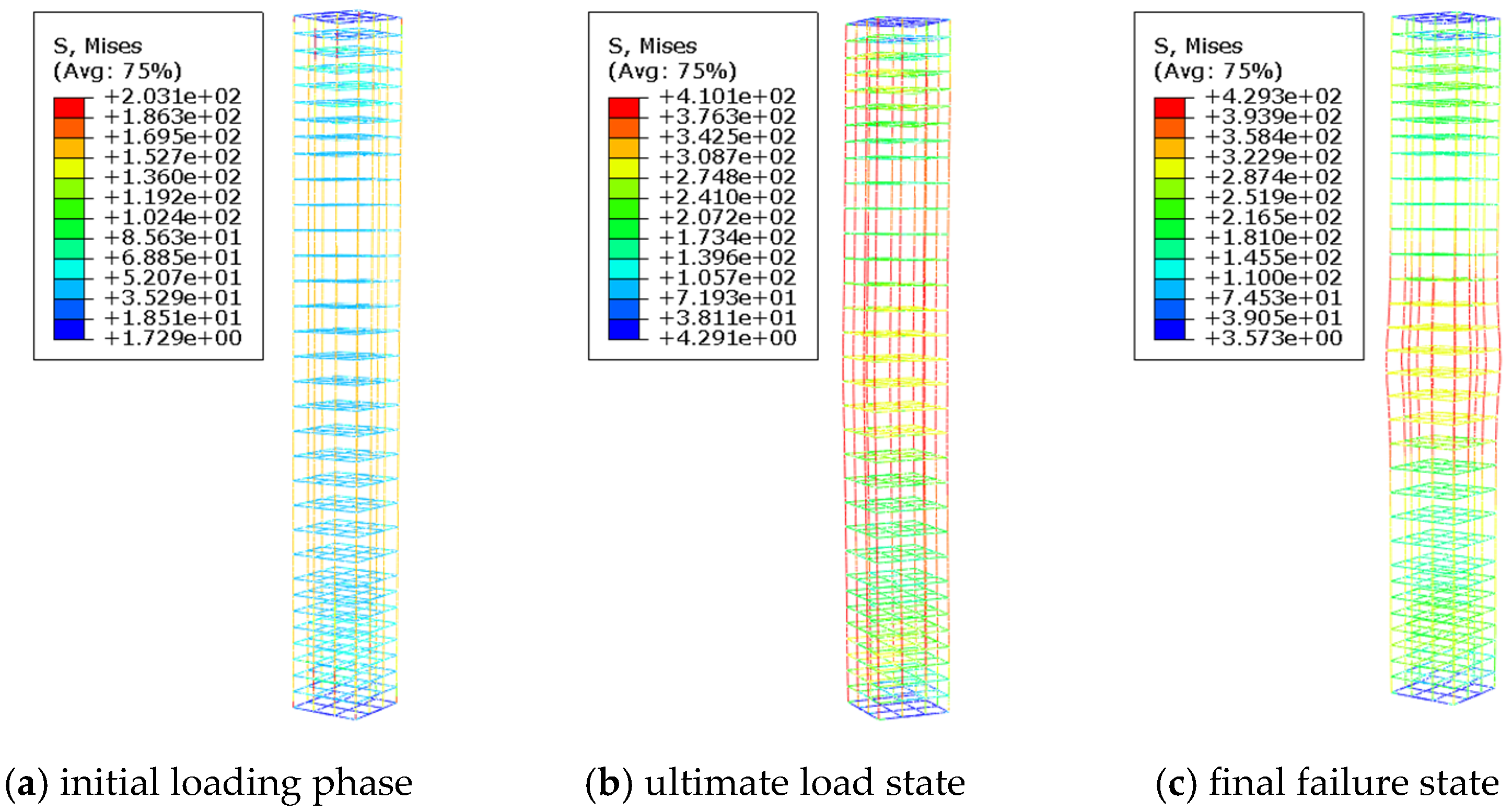
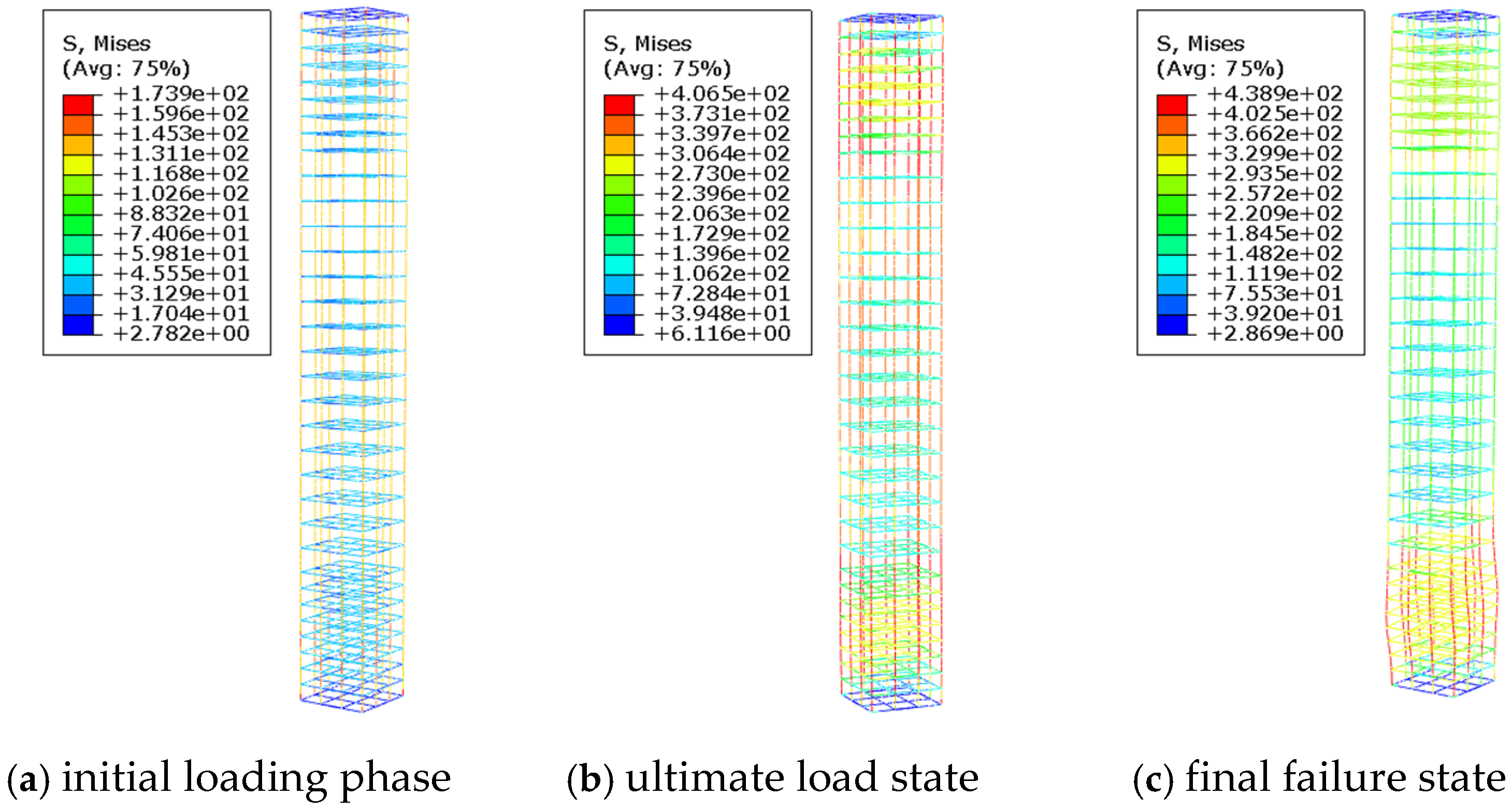
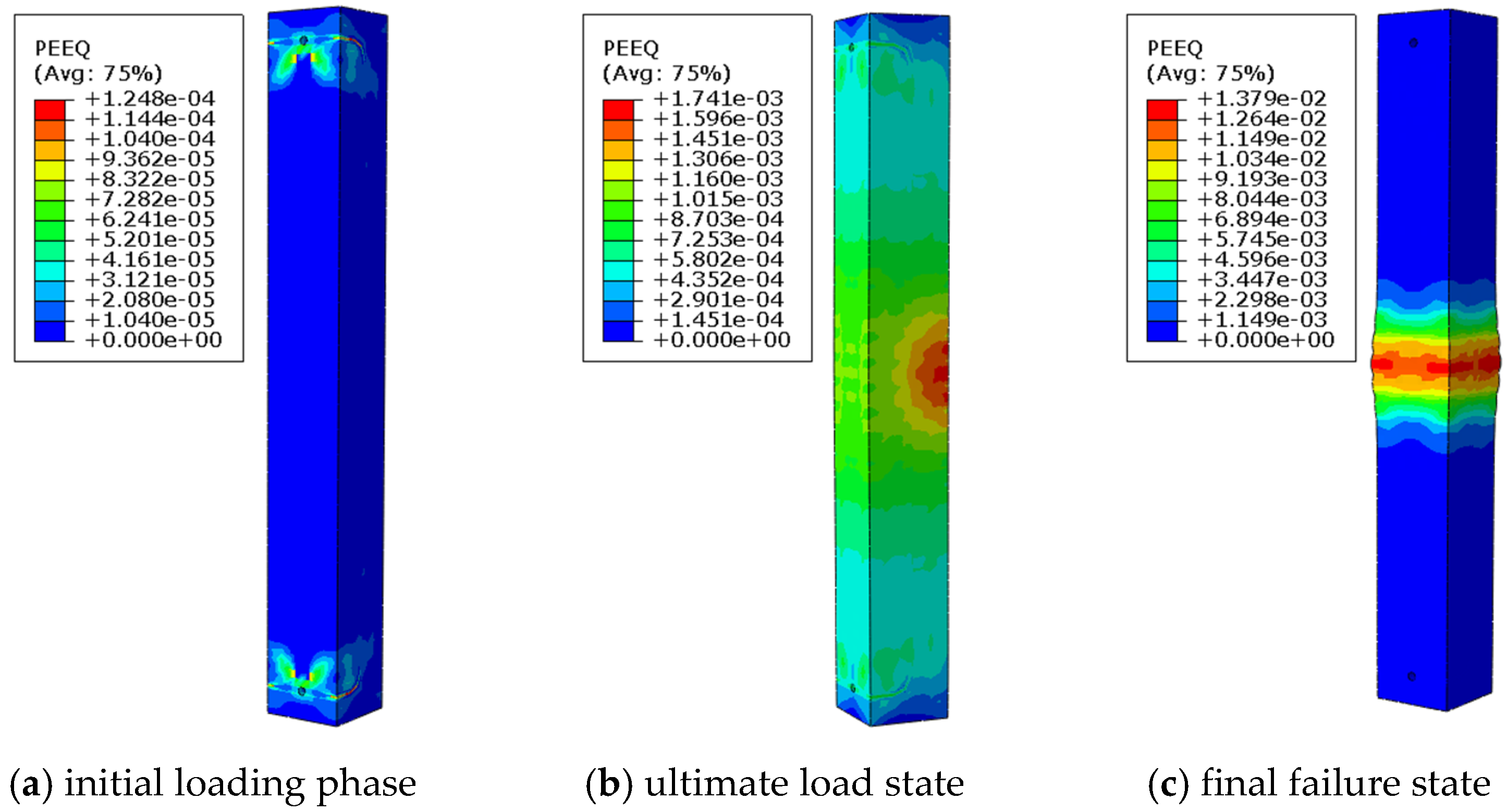
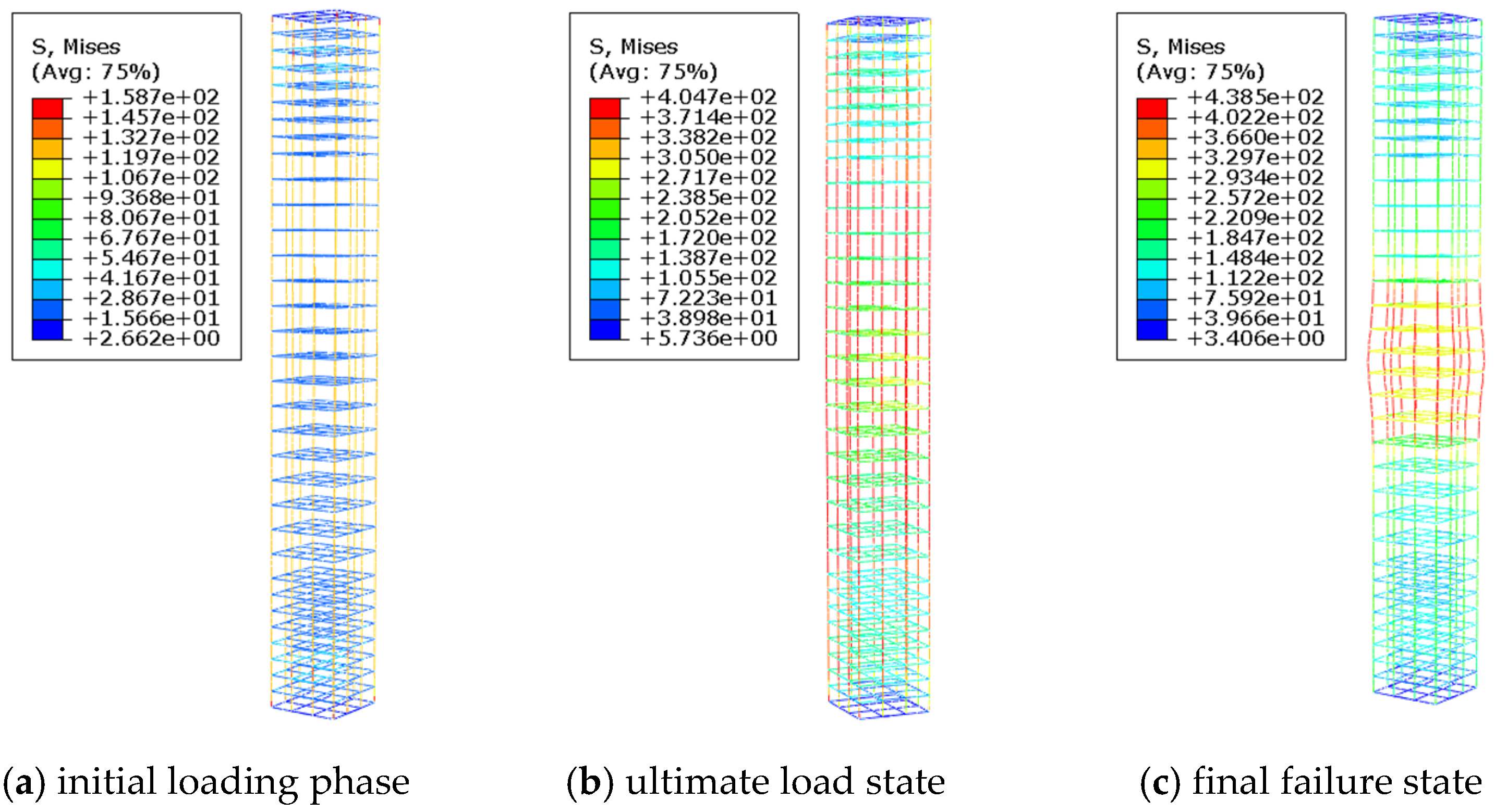
3.1. Failure Mode and Phenomenon
3.2. Bearing Capacity Analysis
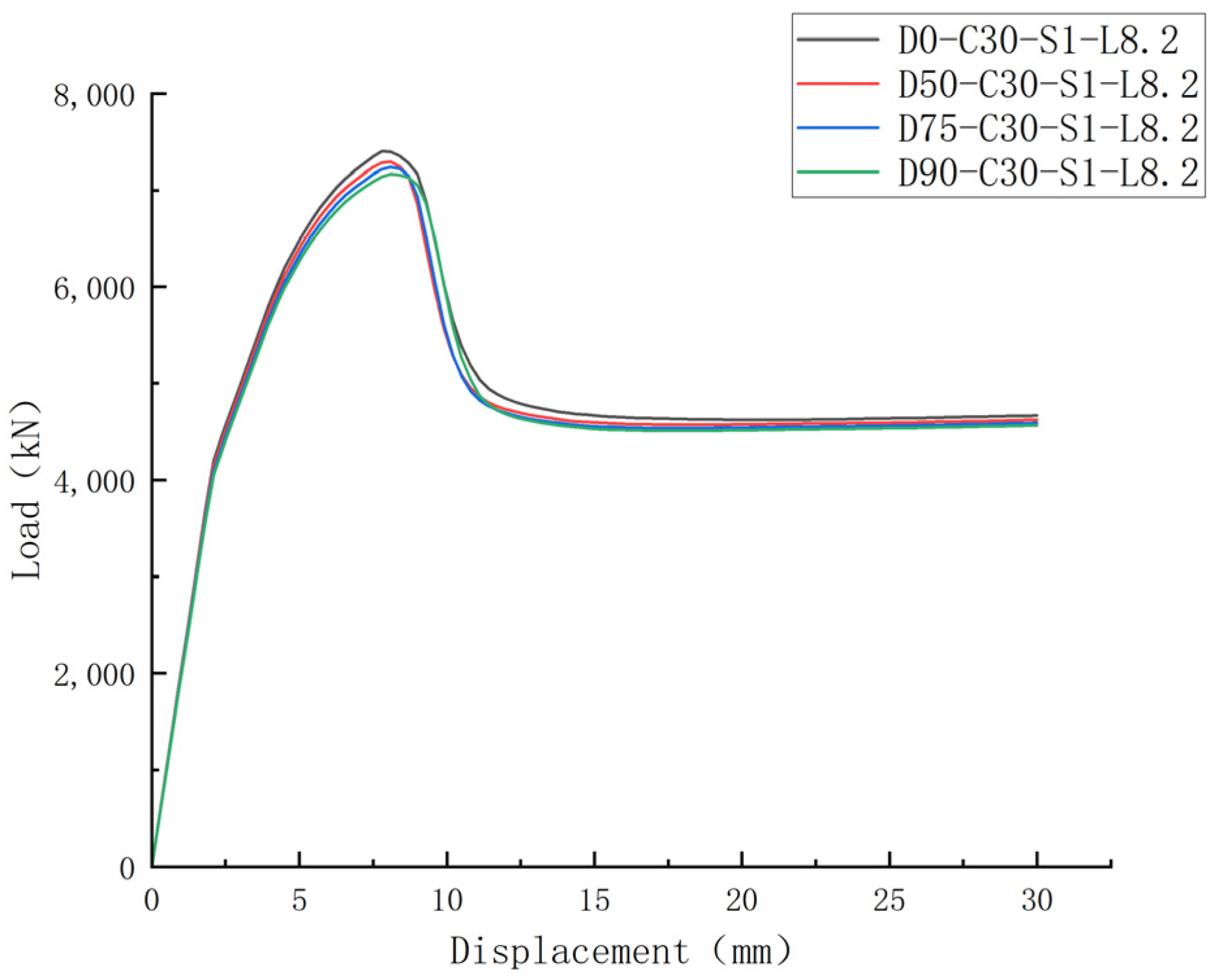
3.2.1. Impact of Pre-Embedded Hole Diameter on the Bearing Capacity of Reinforced Concrete Columns with Embedded Pipes
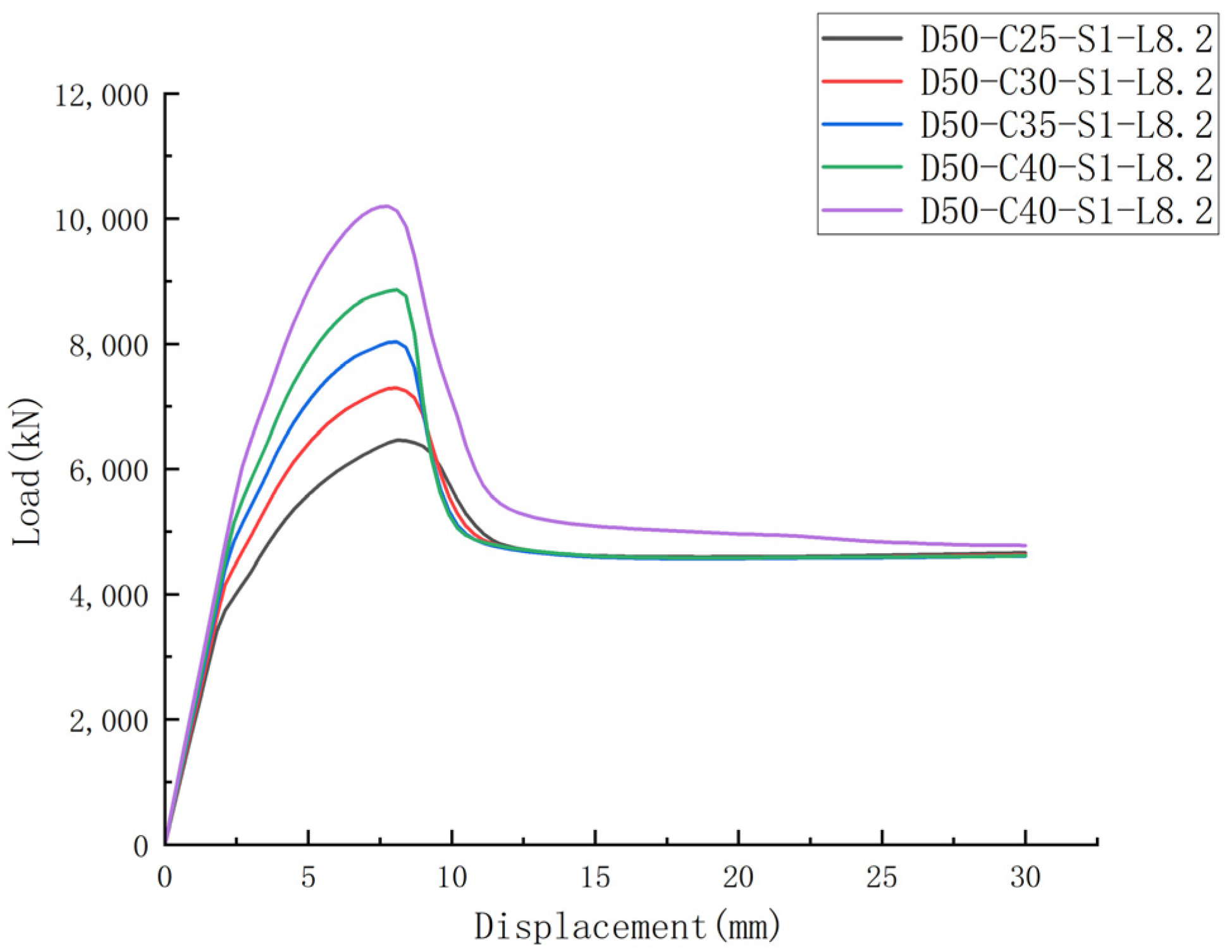
3.2.2. Impact of Concrete Strength on the Bearing Capacity of Reinforced Concrete Columns with Pre-Embedded Holes
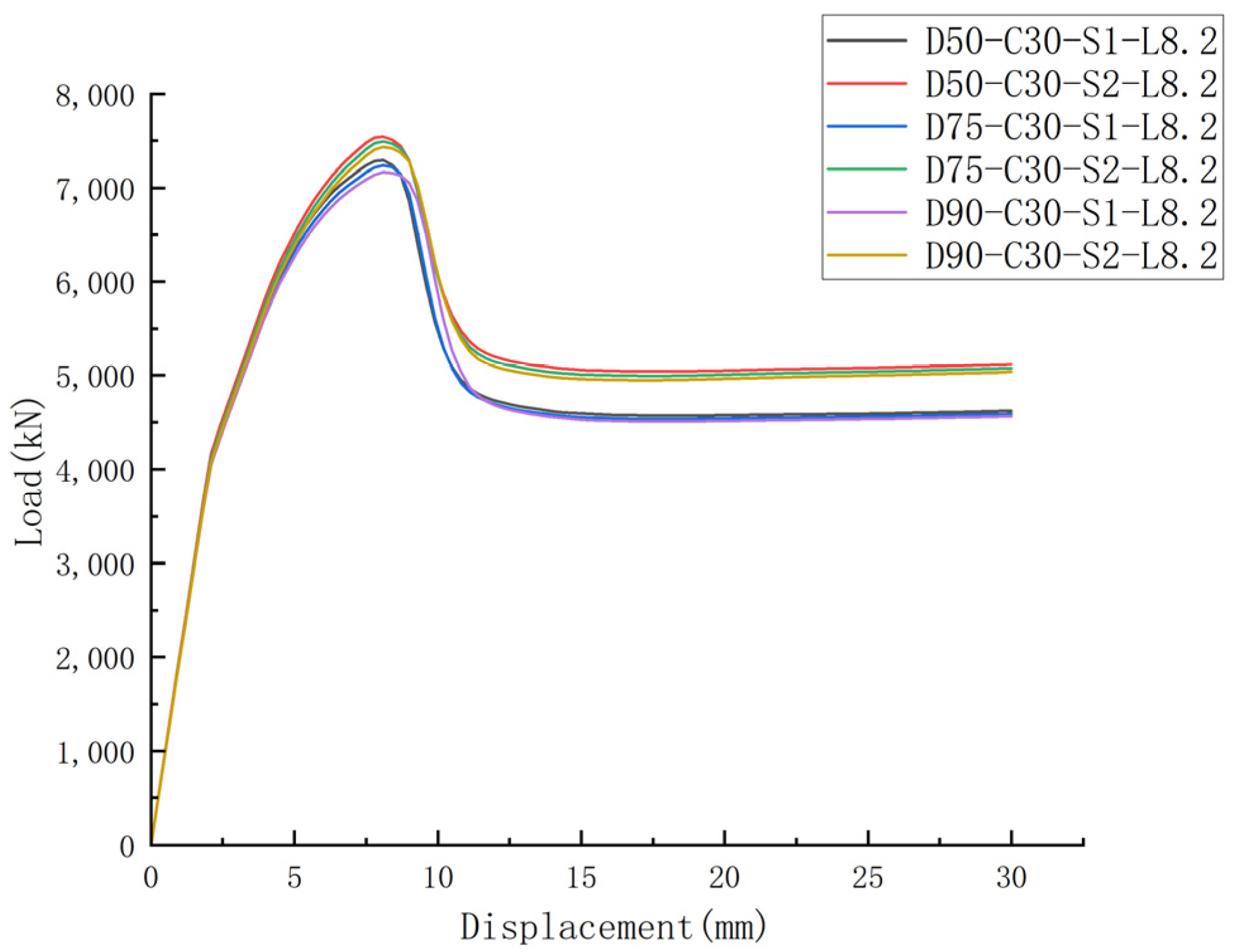
3.2.3. Impact of Stirrup Ratio on the Bearing Capacity of Reinforced Concrete Columns with Pre-Embedded Holes
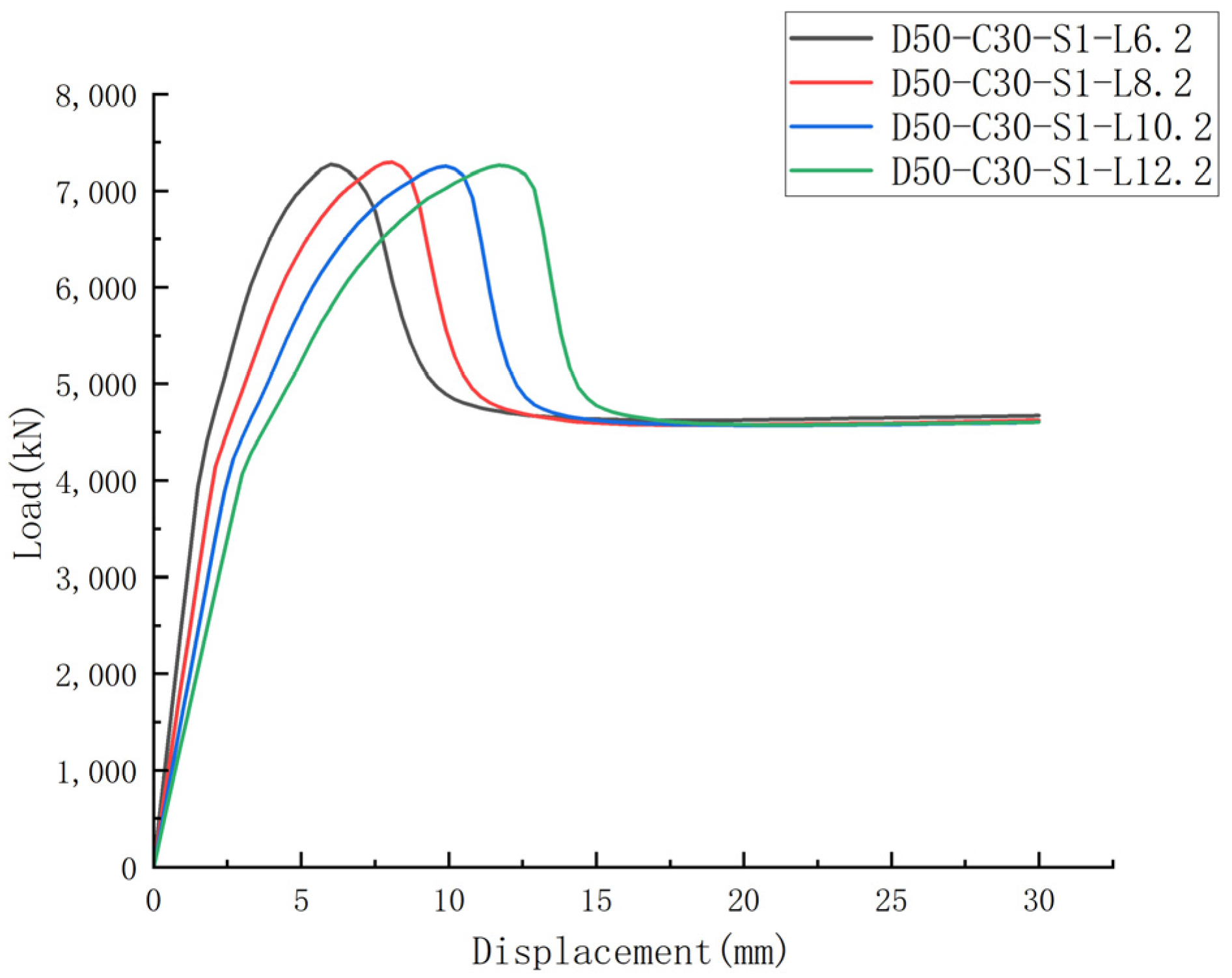
3.2.4. Impact of Slenderness Ratio on the Bearing Capacity of Reinforced Concrete Columns with Pre-Embedded Holes
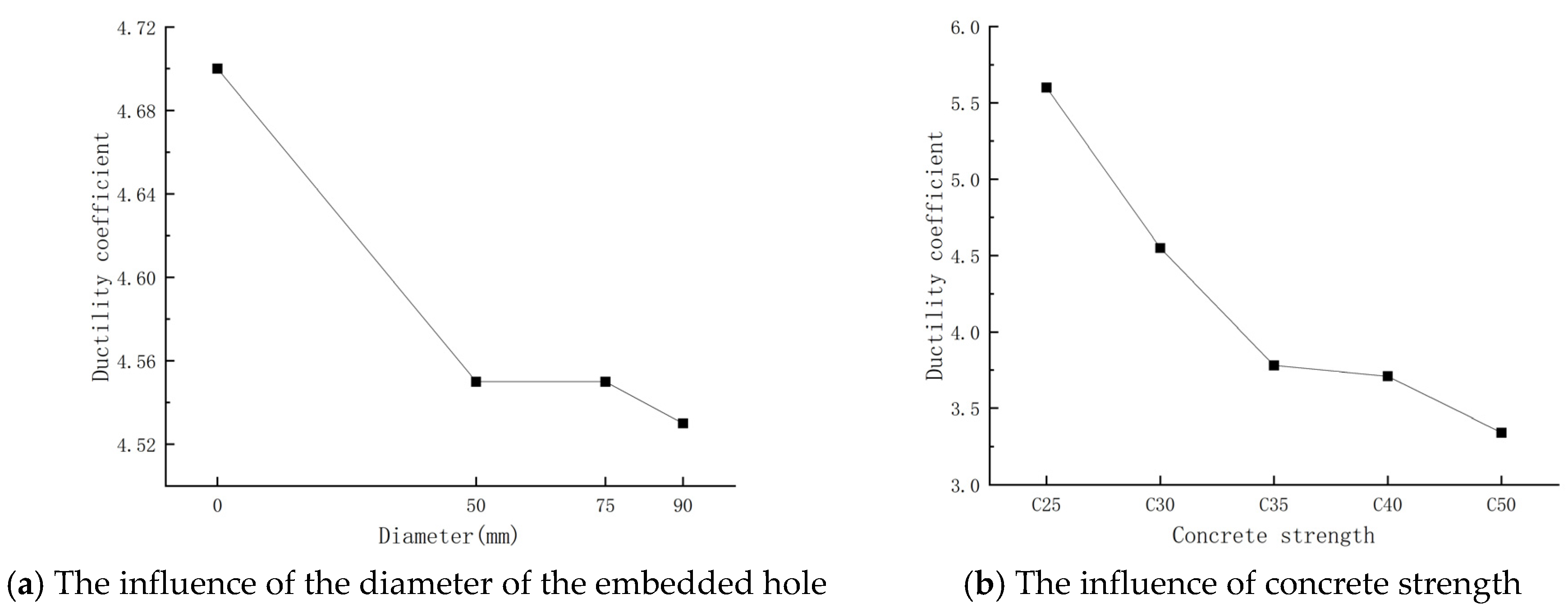
4. Ductility
5. Conclusions
- (1)
- The inclusion of longitudinal pre-embedded holes in reinforced concrete columns leads to a reduction in their ultimate load-bearing capacity. When the diameters of the pre-embedded holes are 50 mm, 75 mm, and 90 mm, the ultimate load-bearing capacity of the specimens decreases by 1.76%, 2.22%, and 3.47%, respectively. This reduction becomes more pronounced as the diameter of the pre-embedded holes increases. The primary reason for this trend is that the presence of pre-embedded holes reduces the effective load-bearing area of the column cross-section, leaving the column more susceptible to entering failure conditions under axial loading.
- (2)
- When the diameter of the embedded hole is ≥75 mm, the presence of the hole alters the stress state of the reinforced concrete column under axial loading. The column experiences an eccentric compression effect due to the hole. However, through stress redistribution and the material’s ductility, the impact of the eccentric compression gradually diminishes. Ultimately, the failure mode does not undergo a fundamental change and remains dominated by axial compression failure. This indicates that, while the embedded hole affects the stress state, the overall failure mechanism of the column does not undergo a fundamental change due to the presence of the hole.
- (3)
- When the concrete strength exceeds C30, the diameter of the embedded hole should not exceed 50 mm. However, if the hole diameter increases to 75 mm or above and the strength of the concrete reaches C35 or above, the existence of the embedded hole will cause the failure mode of the concrete column to change from axial compression failure to shear failure along the plane where the curved part of the embedded hole is located.
- (4)
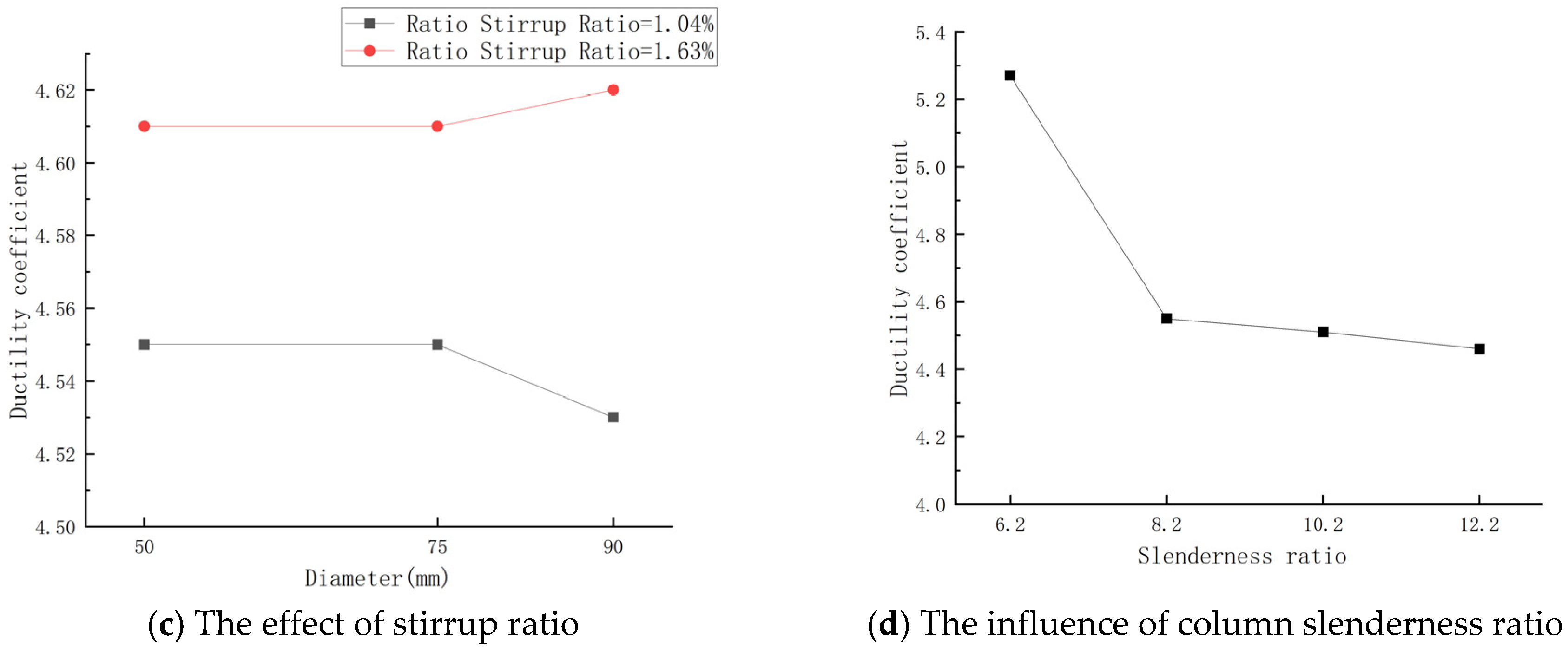
- Increasing the stirrup ratio can effectively improve the performance of reinforced concrete columns with embedded holes. As the stirrup ratio increases, both the load-bearing capacity and ductility of the specimens are enhanced, and the stress concentration is reduced, which helps mitigate the risk of local shear failure.
- (5)
- With the gradual increase in slenderness ratio, both the ultimate bearing capacity and ductility of the column show a decreasing trend, but the change amplitude of the ultimate bearing capacity of the column is not significant and can be ignored. The ductility of the specimen column is more obvious with the increase in the slenderness ratio. This indicates that the slenderness ratio has limited influence on the performance of reinforced concrete columns with embedded holes in practical engineering applications, but its potential influence should still be considered in the design to ensure the safety and reliability of the structure.
- (6)
- In practical engineering, the technology of longitudinally pre-embedded hole reinforced concrete columns demonstrates significant advantages, supporting its use in future frame structures and temporary buildings. Optimizing the cross-sectional design reduces self-weight and saves materials, offering considerable economic benefits. Additionally, the innovative multi-functional hole design improves the utilization efficiency of the holes, significantly enhancing the building’s life cycle benefits and reducing its carbon emissions over the entire lifespan. This provides an innovative solution for the development of green buildings.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xie, X. Development and Prospect of Demolition Blasting Technology. Blasting 2019, 36, 1–12. [Google Scholar] [CrossRef]
- Ye, J.; Cheng, D.; Shu, D.; Li, X.; Ming, J. Green Demolition Blasting Technology for Axially Embedded Holes in Reinforced Concrete Members. Sci. Technol. Eng. 2017, 17, 151–157. [Google Scholar]
- Ye, J.; Cheng, D.; Zhan, X.; Ming, J.; Xiao, H. Blasting Technology of Demolition of Pit-support Beams by Pre-buried Axial Pipes Blast Holes. Blasting 2017, 34, 74–79. [Google Scholar]
- Cheng, D.; Ye, J.; Wang, L.; Ming, J. Blasting Demolition Technology for Reinforced Concrete Beam-Column Members with Long Bags for Axial Embedded Holes Using Water Coupling for Precise Loading. Blasting 2018, 35, 107–113. [Google Scholar] [CrossRef]
- Cai, J.; Huang, T.; Li, J. Experimental Study on Reinforced Concrete Simply Supported Beams with Circular Holes in the Abdomen. J. Civ. Eng. 2009, 42, 27–35. [Google Scholar]
- Han, X. Finite Element Analysis of Bending Capacity of Reinforced Concrete Beams with Holes. Master’s Thesis, Kunming University of Science and Technology, Kunming, China, 2014. [Google Scholar]
- Xu, Z.; Yuan, G.; Nie, M.; Qiu, H. Experimental and Theoretical Study on the Bearing Capacity of RC Beams with Holes. Build. Sci. 2016, 32, 1–8. [Google Scholar] [CrossRef]
- Son, K.; Pilakoutas, K.; Neocleous, K. Behaviour of Concrete Columns with Drilled Holes. Mag. Concr. Res. 2006, 58, 411–419. [Google Scholar] [CrossRef]
- Basravi, A. Finite Element Analysis of Reinforced Concrete Column with Longitudinal Hole; Faculty of Civil Engineering, Universiti Teknologi Malaysia: Johor Bahru, Malaysia, 2010. [Google Scholar]
- Lotfy, F.M. Nonlinear Analysis of Reinforced Concrete Columns with Holes. Int. J. Civ. Struct. Eng. 2013, 3, 655–668. [Google Scholar]
- Campione, G.; Cucchiara, C.; Minafò, G. Effects of circular openings on the compressive behaviour of RC columns. Mater. Struct. 2015, 48, 1995–2008. [Google Scholar] [CrossRef]
- Wilson, P.P.; Nair, E.M.S. Investigation on Reinforced Concrete Columns with Holes. Int. Res. J. Eng. Technol. 2017, 4, 1393–1395. [Google Scholar]
- AL-Shaarbaf, I.A.S.; Allawi, A.A.; AlSalim, N.H. Strength of Reinforced Concrete Columns with Transverse Openings. Eng. J. 2017, 23, 114–133. [Google Scholar] [CrossRef]
- Kassim, M.M.; Ahmad, A.S. Strength Evaluation of Concrete Columns with Cross-Sectional Holes. Pract. Period. Struct. Des. Constr. 2018, 23, 04018027. [Google Scholar] [CrossRef]
- Li, Y. Analysis of the Influence of Core Drilling on the Load-Bearing Performance of Reinforced Concrete Axial Compression Columns Using Finite Element Method. Master’s Thesis, Taiyuan University of Technology, Taiyuan, China, 2019. [Google Scholar]
- Negassa, F. Numerical Investigation of Reinforced Concrete Columns with Transverse Holes. Glob. Sci. J. 2020, 8, 4515–4561. [Google Scholar]
- Tahir, F.M.; Shabbir, F.; Ahmad, A.; Ejaz, N. Experimental and Numerical Investigation of Transverse Circular Holes on Load-Carrying Capacity of RC Columns. Iran. J. Sci. Technol. Trans. Civ. Eng. 2020, 45, 683–696. [Google Scholar] [CrossRef]
- Hailu, B.; Goshu, K. Effect of transverse opening on the strength of reinforced concrete column. Asian J. Civ. Eng. 2022, 24, 639–655. [Google Scholar]
- Zhang, Y.; Zhang, S.; Wang, Z. Effects of Holes and the Confining Pressure on the Mechanical Properties of High-Performance Concrete. Iran. J. Sci. Technol. Trans. Civ. Eng. 2024, 1–14. [Google Scholar] [CrossRef]
- Li, L. Study on the Mechanical Properties of Prefabricated Concrete Columns with Different Hole Rates. Master’s Thesis, Anhui University of Architecture, Hefei, China, 2024. [Google Scholar] [CrossRef]
- Liang, J.; Wang, Y.; Zou, W.; Wang, C.; Li, W. Axial compression performance of partially encased concrete columns with web opening. Sci. Rep. 2024, 14, 11853. [Google Scholar] [CrossRef]
- Bassurucu, M.; Yildiz, O.; Kina, C. Seismic Performance Assessment of an RC Building Due to 2023 Türkiye Earthquakes: A Case Study in Adıyaman, Türkiye. Buildings 2025, 15, 521. [Google Scholar] [CrossRef]
- Hu, C.; Hu, L.; Wang, Y.; Yuan, B.; Wang, B. Influence of reinforcement methods on mechanical properties of reinforced concrete beam-column joints. Civ. Eng. 2023, 12, 1485–1492. [Google Scholar] [CrossRef]
- Vanni, N.; Sandro, C.; Fabrizio, G. Nomograms for the pre-dimensioning of RC beam-column joints according to Eurocode 8. Structures 2022, 39, 39958–39973. [Google Scholar]
- GB 50010-2010; Code for Design of Concrete Structures. China Architecture & Building Press: Beijing, China, 2011.
- Du, X.; Fu, J.; Zhang, J. The experimental study on size effect of the large-size reinforced concrete column under axial loading. Civ. Eng. J. 2010, 43 (Suppl. S2), 1–8. [Google Scholar] [CrossRef]
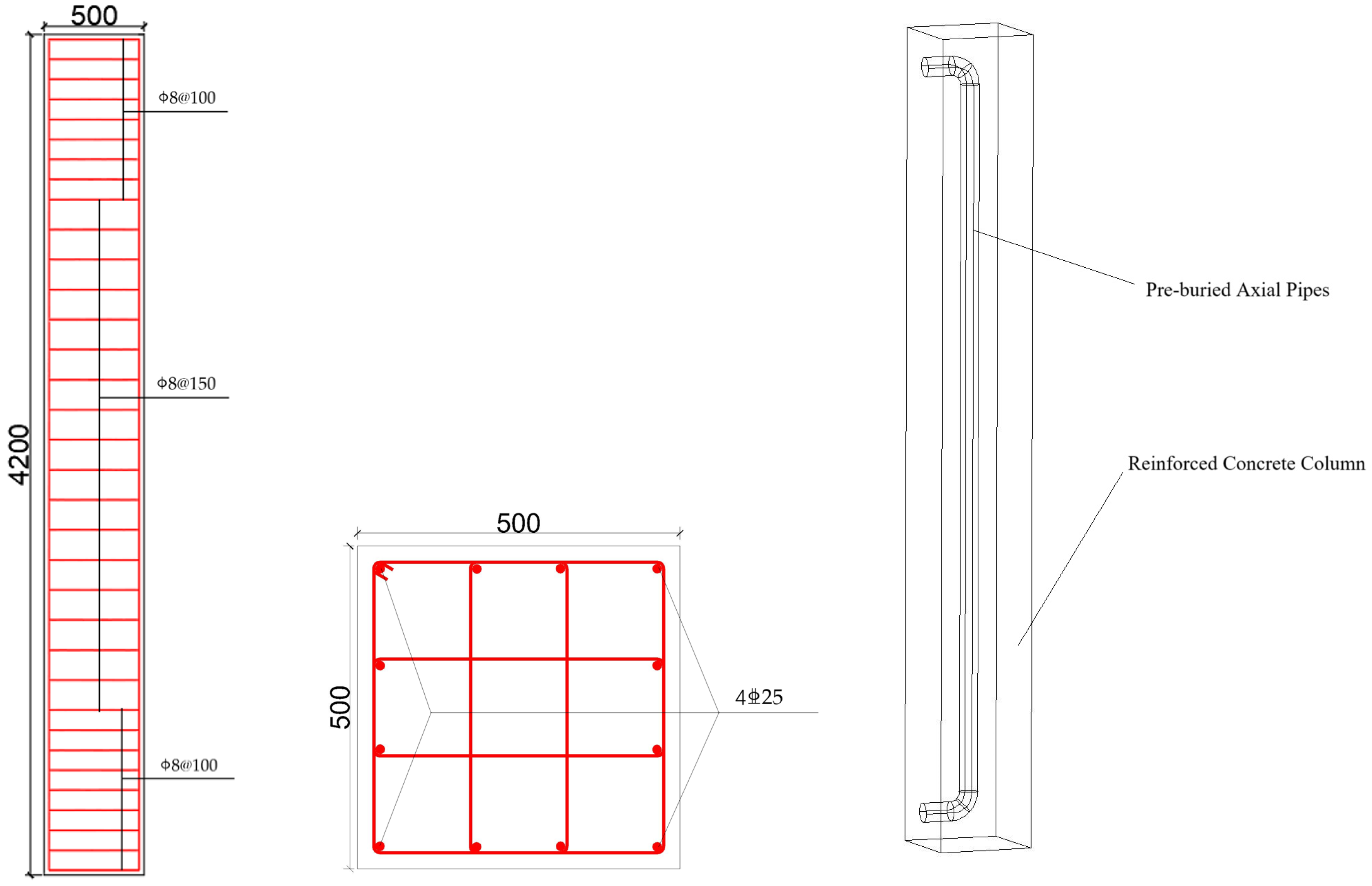




| Concrete Strength Grade | Axial Compressive Strength fc (MPa) | Axial Tensile Strength ft (MPa) | Elastic Modulus (MPa) |
|---|---|---|---|
| C25 | 16.7 | 1.78 | 2.80 × 104 |
| C30 | 20.1 | 2.01 | 3.00 × 104 |
| C35 | 23.4 | 2.20 | 3.15 × 104 |
| C40 | 26.8 | 2.39 | 3.25 × 104 |
| C50 | 32.4 | 2.64 | 3.45 × 104 |
| Type | Diameter (mm) | Yield Strength fy (MPa) | Ultimate Strength fst (MPa) | Elastic Modulus Es (MPa) |
|---|---|---|---|---|
| HRB400 | 25 | 400 | 540 | 2.0 × 105 |
| 22 | ||||
| HPB300 | 8 | 300 | 420 | 2.1 × 105 |
| 10 |
| φ | e | fb0/fco | K | μ |
|---|---|---|---|---|
| 30° | 0.1 | 1.16 | 0.6667 | 0.0005 |
| Number | Pre-Embedded Hole Diameter (mm) | Concrete Strength | Length (mm) | Slenderness Ratio | Stirrup Ratio (%) | Ultimate Bearing Capacity (kN) | Damage Pattern |
|---|---|---|---|---|---|---|---|
| D0-C30-S1-L8.2 | 0 | C30 | 4200 | 8.2 | 1.04% | 7400.07 | Axial compression damage |
| D50-C30-S1-L8.2 | 50 | C30 | 4200 | 8.2 | 1.04% | 7291.99 | Axial compression damage |
| D50-C25-S1-L8.2 | 50 | C25 | 4200 | 8.2 | 1.04% | 6460.96 | Axial compression damage |
| D50-C35-S1-L8.2 | 50 | C35 | 4200 | 8.2 | 1.04% | 8032.88 | Axial compression damage |
| D50-C40-S1-L8.2 | 50 | C40 | 4200 | 8.2 | 1.04% | 8858.84 | Axial compression damage |
| D50-C50-S1-L8.2 | 50 | C50 | 4200 | 8.2 | 1.04% | 10,196.10 | Shear failure |
| D50-C30-S1-L6.2 | 50 | C30 | 3200 | 6.2 | 1.04% | 7269.38 | Axial compression damage |
| D50-C30-S1-L10.2 | 50 | C30 | 5200 | 10.2 | 1.04% | 7251.75 | Axial compression damage |
| D50-C30-S1-L12.2 | 50 | C30 | 6200 | 12.2 | 1.04% | 7262.09 | Axial compression damage |
| D50-C30-S2-L8.2 | 50 | C30 | 4200 | 8.2 | 1.63% | 7541.62 | Axial compression damage |
| D50-C50-S2-L8.2 | 50 | C50 | 4200 | 8.2 | 1.63% | 10,459.50 | Axial compression damage |
| D75-C30-S1-L8.2 | 75 | C30 | 4200 | 8.2 | 1.04% | 7239.18 | Axial compression damage |
| D75-C25-S1-L8.2 | 75 | C25 | 4200 | 8.2 | 1.04% | 6415.63 | Axial compression damage |
| D75-C35-S1-L8.2 | 75 | C35 | 4200 | 8.2 | 1.04% | 7910.02 | Shear failure |
| D75-C40-S1-L8.2 | 75 | C40 | 4200 | 8.2 | 1.04% | 8639.91 | Shear failure |
| D75-C50-S1-L8.2 | 75 | C50 | 4200 | 8.2 | 1.04% | 9883.73 | Shear failure |
| D75-C30-S2-L8.2 | 75 | C30 | 4200 | 8.2 | 1.63% | 7492.84 | Axial compression damage |
| D75-C35-S2-L8.2 | 75 | C35 | 4200 | 8.2 | 1.63% | 8198.16 | Axial compression damage |
| D90-C30-S1-L8.2 | 90 | C30 | 4200 | 8.2 | 1.04% | 7163.81 | Axial compression damage |
| D90-C25-S1-L8.2 | 90 | C25 | 4200 | 8.2 | 1.04% | 6361.33 | Axial compression damage |
| D90-C35-S1-L8.2 | 90 | C35 | 4200 | 8.2 | 1.04% | 7737.69 | Shear failure |
| D90-C40-S1-L8.2 | 90 | C40 | 4200 | 8.2 | 1.04% | 8441.39 | Shear failure |
| D90-C50-S1-L8.2 | 90 | C50 | 4200 | 8.2 | 1.04% | 9593.85 | Shear failure |
| D90-C30-S2-L8.2 | 90 | C30 | 4200 | 8.2 | 1.63% | 7432.91 | Axial compression damage |
| D90-C35-S2-L8.2 | 90 | C35 | 4200 | 8.2 | 1.63% | 8116.65 | Axial compression damage |
| Specimen Number | /mm | /mm | |
|---|---|---|---|
| Z0 | 2.07 | 9.72 | 4.70 |
| Z50-1 | 2.07 | 9.42 | 4.55 |
| Z50-2 | 1.84 | 10.31 | 5.60 |
| Z50-3 | 2.38 | 9.00 | 3.78 |
| Z50-4 | 2.39 | 8.87 | 3.71 |
| Z50-5 | 2.70 | 9.02 | 3.34 |
| Z50-6 | 2.11 | 9.72 | 4.61 |
| Z50-7 | 1.51 | 7.96 | 5.27 |
| Z50-8 | 2.50 | 11.28 | 4.51 |
| Z50-9 | 3.01 | 13.41 | 4.46 |
| Z50-10 | 2.68 | 9.00 | 3.36 |
| Z75-1 | 2.08 | 9.46 | 4.55 |
| Z75-2 | 2.19 | 10.39 | 4.74 |
| Z75-3 | 2.38 | 9.74 | 4.09 |
| Z75-4 | 2.42 | 8.88 | 3.67 |
| Z75-5 | 2.73 | 8.24 | 3.02 |
| Z75-6 | 2.12 | 9.77 | 4.61 |
| Z75-7 | 9.35 | 9.87 | 1.06 |
| Z90-1 | 2.15 | 9.73 | 4.53 |
| Z90-2 | 2.08 | 10.31 | 4.96 |
| Z90-3 | 2.10 | 9.15 | 4.36 |
| Z90-4 | 2.40 | 8.59 | 3.58 |
| Z90-5 | 2.66 | 7.90 | 2.97 |
| Z90-6 | 2.12 | 9.79 | 4.62 |
| Z90-7 | 2.34 | 9.45 | 4.04 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Xu, W.; Ye, J.; Liu, X. The Mechanical Properties of Reinforced Concrete Columns with Longitudinal Pre-Embedded Holes. Appl. Sci. 2025, 15, 5010. https://doi.org/10.3390/app15095010
Zhang J, Xu W, Ye J, Liu X. The Mechanical Properties of Reinforced Concrete Columns with Longitudinal Pre-Embedded Holes. Applied Sciences. 2025; 15(9):5010. https://doi.org/10.3390/app15095010
Chicago/Turabian StyleZhang, Junzheng, Weisheng Xu, Jianjun Ye, and Xuexi Liu. 2025. "The Mechanical Properties of Reinforced Concrete Columns with Longitudinal Pre-Embedded Holes" Applied Sciences 15, no. 9: 5010. https://doi.org/10.3390/app15095010
APA StyleZhang, J., Xu, W., Ye, J., & Liu, X. (2025). The Mechanical Properties of Reinforced Concrete Columns with Longitudinal Pre-Embedded Holes. Applied Sciences, 15(9), 5010. https://doi.org/10.3390/app15095010





