Research on Temporary Support Robot for the Integrated Excavation and Mining System of Section Coal Pillar

Abstract

Facing the support challenges of short-wall working face (15–40m) roadways in the ‘excavation–backfill–retention’ tunneling method for section coal pillars, traditional equipment struggled to achieve stable, reliable, and efficient support. This paper designed a temporary support robot for the excavation and mining system of section coal pillars to ensure the safety of equipment and personnel in short-wall working faces. The support requirements of the section coal pillar excavation and mining system were analyzed, and a general ‘driving under pressure’ temporary support scheme was proposed. The working principle of the temporary support robot was analyzed. A mechanical model for the stable support of the temporary support robot was established. The mechanical properties of the surrounding rock were analyzed, and the allowable range of the temporary support robot’s supporting force was determined while ensuring the stability of the surrounding rock. Based on the Stribeck friction theory, a dynamic model of the temporary support robot in the driving under pressure state was constructed. The boundary conditions of the dynamic model were set, and the corresponding relationship between the temporary support robot’s supporting force and its maximum static friction force was determined. This accurately described the influence of the supporting force and pushing (pulling) force on the movement during the process of driving under pressure. Through finite element simulation, the stress conditions of the temporary support robot and the floor under maximum load were analyzed, indicating that this load condition would not cause damage to the temporary support robot or the surrounding rock. Through multi-body dynamics simulation, the pushing (pulling) forces required for the temporary support robot’s movement under different supporting force conditions were obtained, verifying the feasibility of the driving under pressure action under different supporting force conditions. Moreover, the model-predicted and simulated values of the required pushing (pulling) forces during the process of driving under pressure were consistent, validating the accuracy of the driving under pressure dynamic model. This research provides a new theoretical framework for the design and dynamic analysis of temporary support equipment for short-wall working faces in section coal pillar mining, holding significant academic value and broad application prospects.

1. Introduction

Based on the development trend of China’s excavation and mining technology and the actual needs of coal mining enterprises for solid waste treatment and section coal pillar resources recovery, academician Wang proposed the integrated ‘excavation–backfill–retention’ tunneling method for section coal pillar extraction [1], which can realize the collaborative operation of “roadway excavation, solid waste disposal, and section coal pillar resources recovery”. The new method employs a set of advanced comprehensive mining equipment for short-wall working face excavation, continuously and efficiently filling behind the excavation face. Overall, it replaces the section coal pillar with waste rock, while automatically leaving two side lanes along the filling belt, achieving tunnel excavation, waste rock disposal, and section coal pillar resource recovery.

Temporary support is an indispensable process in roadway excavation, which can ensure the safety and stability of the roof in the area between the drilling head and permanent support [2]. Compared with the permanent support of general roadways, the temporary support strength is far lower than the permanent support strength [3,4]. Therefore, realizing safe and stable temporary support during face section coal pillar excavation is an important engineering problem in integrated ‘excavation–backfill–retention’ mining.

Currently, most of the temporary support equipment used in coal mine roadway excavation do not rely on the tunneling machine and can realize autonomous movement [5,6]. They can be categorized by their movement method: stepping mobile temporary support, crawler-type temporary support, and translational temporary support. The stepping mobile temporary support moves by mimicking a walking motion using independent units that alternately support, release, advance, and re-support. This adapts to complex geology and offers high strength [7]. The crawler-type temporary support uses a crawler mechanism for continuous support and advancement, improving efficiency. It is ideal for long, uniform tunnels, especially in soft soil [8]. The translational temporary support uses large frames pushed forward; during the pushing process, the support force on the roof is usually maintained. It is simple and suited for regular tunnels with good geology [9].

Regarding the stepping mobile temporary support, Yang et al. [10] designed a novel advanced roadway support with a support height of 2~4 m and a support width of 3.2~4.5 m, analyzing the influence of support timing and location on roof load and deformation. This provided a basis for optimizing the support design and improving the support effect. Mao et al. [11] studied the structural stability of the stepping arch advanced supporting equipment and established the multi-point distributed static model of the top support beam. Structural design optimization was carried out through simulation software to ensure the structure had better stability. Wang et al. [12] proposed a novel approach for roadway excavation, support, and anchoring by utilizing the twin-link stepping mobile temporary support method, it can provide support for roadways that are 3.6 m high and 5 m wide. This method laid a theoretical foundation for implementing the parallel operation mode in underground roadway excavation in coal mines and significantly improved safety and efficiency.

Regarding the crawler-type temporary support. Xu [13] introduced a hydraulic-driven crawler-type tunneling support device designed for a tunneling width of 5.0 m and a support height range of 3.0 to 3.8 m, addressing safety and efficiency issues in coal mine roadway support. Utilizing a hydraulic motor and a four-bar linkage mechanism, the device enhances stability, reduces roof damage, and allows rapid forward movement without lowering the frame, thereby improving tunneling efficiency.

Regarding the translational temporary support, Ma et al. [14] proposed a precise control method based on fuzzy PID for the supporting force of a shield-type temporary support robot and verified the stability of the control system through experiments to ensure the safe and stable support of the surrounding rock during the pushing movement.

In summary, the existing temporary support equipment can only address excavation issues in roadways with widths below 5 m, and there is a high risk of damaging the roof during the support process. There are few reports on temporary support equipment for a short-wall working face with a length of 15~40 m required for the face section coal pillar excavation and mining system. Therefore, aiming at the needs of the face section coal pillar excavation and mining system, this paper designs a self-propelled temporary support robot (TSR). The working principle of TSR driving under pressure is analyzed, and mechanical models of TSR for stable support and dynamic models for driving under pressure are constructed. The stable support state, the boundary conditions of driving under pressure, and the process of driving under pressure are simulated and analyzed to solve the support and driving problems of excavation equipment in short-wall working face tunnels.

This paper is organized as follows: Section 1 designs a self-moving TSR and clarifies its working principle. Section 2 constructs a mechanical model of the TSR during stable support. Section 3 constructs a dynamic model of the TSR when driving under pressure. Section 4 establishes a criterion system for the boundary condition of the dynamic model, and clarifies the mechanism of interaction between the TSR and the surrounding rock under the driving under pressure condition. Section 5 conducts a finite element analysis on the TSR and the floor and simulates the critical value of the boundary condition of the dynamic model for driving under pressure, as well as the process of driving under pressure.

2. Analysis of the Principles of the Temporary Support Robot

2.1. Overall Scheme of the Temporary Support System

The ‘excavation–backfill–retention’ system of the section coal pillar is responsible for completing the integrated ‘excavation–backfill–retention’ mining of the section coal pillar, and its scheme is shown in Figure 1. The ‘excavation–backfill–retention’ system of the section coal pillar includes four subsystems: section coal pillar excavation and mining system, waste-filling system, transportation system, and ventilation dust removal system. Among them, the section coal pillar excavation and mining system includes a cutting robot [15], a scraper conveyor [16], a temporary support system, and platforms with drilling and anchoring equipment [17,18], which are responsible for working face excavation and support. The waste-filling system completes the middle waste rock filling and the natural roadway formation on the left and right sides through the waste-filling robot. The transportation system can timely and smoothly load and transport the cut coal rock to the main transport system and transport the materials used for waste-filling to the designated position according to the requirements so as to provide an effective and reliable guarantee for the continuous work of the entire face section coal pillar excavation and mining and waste-filling. The loader and belt conveyor are essential in the mining face and belong to the transportation system. The loader transfers coal from the mining area to the main belt conveyor or storage, providing flexibility and improving efficiency. The belt conveyor enables continuous, stable transport of coal over long distances, reducing manual labor and enhancing safety. Together, they form the backbone of modern coal mining operations. The ventilation dust removal system is mainly responsible for supplying fresh air to the underground, diluting and discharging harmful gases and dust, providing a safe and healthy working environment for miners, preventing gas explosions and dust hazards, and ensuring the safe production of the mine. Each subsystem operates in parallel and collaboratively, which can realize the safety, high efficiency, and intelligence of the excavation and waste-filling process of the section coal pillar extraction system.

The temporary support system is a part of the ‘excavation–backfill–retention’ system of section coal pillar extraction, mainly including the TSR and the end support robot. Considering that the end support robot needs to straddle the scraper conveyor and has a unique structure, this paper only focuses on the TSR. The face of the section coal pillar excavation and mining working face is divided into several sections. The cutting robot cuts a section from left to right for each section. Each section contains a group of two TSRs and a platform with drilling and anchoring equipment (as shown in Figure 1). The working process of each TSR group is as follows: two TSRs wait in the stable support situation for the cutting robot to complete the cutting action of the coal wall in the section. When the cutting work of the section is completed, and the cutting robot is traversed to the next section, the group of TSRs, according to the requirements of the scraper conveyor pushing in the comprehensive working face, and coordinates with the scraper conveyor pushing cylinder of the left TSR by controlling the extension of its scraper conveyor pushing cylinder to form an S-bend in the scraper conveyor pan. When the scraper conveyor pushing cylinder of the group of TSRs reaches the maximum stroke, the group of TSRs drives forward under pressure for a section from left to right in a push–pull sequence. When the group of TSRs completes the driving under pressure action, they jointly pull the platform with drilling and anchoring equipment located in the section forward for a section. All groups of TSRs in the temporary support system complete all the actions from left to right and then enter the next cycle.

2.2. Working Principles of the Temporary Support Robot

The temporary support system needs to provide timely and stable support for the empty top area generated after the cutting robot cuts the coal wall, and also provide a safe working space for personnel and equipment in the excavation and mining working face. To ensure the filling of waste rock and the forming of two roadways, the roof cannot collapse during the tunneling process of the excavation and mining system. Therefore, the temporary support system should avoid damaging the roof as much as possible during the support and driving process [19]. The excavation and mining system requires a temporary support system to provide forward power during tunneling. Therefore, the temporary support system must drive the cutting robot, scraper conveyor, and platform with drilling and anchoring equipment while moving autonomously. The requirements of the temporary support system include support and driving requirements, as shown in Figure 2.

In response to the requirements of the ‘excavation–backfill–retention’ temporary support system for section coal pillar extraction, this paper designs a novel self-propelled TSR. The TSR mainly comprises two parts: support 1 and support 2, as shown in Figure 3. The driving under pressure process of the TSR involves the collaborative operation of support 1 and support 2. Both can adaptively change the supporting force to the roof while maintaining the surrounding rock stability. When support 1 is driving under pressure, its supporting force decreases, while support 2 is in a steady state, and its supporting force remains unchanged. The moving cylinder uses support 2 as a supporting point, pushing support 1 forward. Then, support 2 is dragged forward by support 1 under the same principle. The two TSRs in the same group rely on the friction with the roof and floor to move the platform with drilling and anchoring equipment forward through the pushing cylinder traction. The TSR adopts the method of pushing the scraper conveyor with the hydraulic support of the comprehensive working face. Multiple TSRs cooperate to complete the scraper conveyor pushing action through the pulling cylinder [20].

2.3. Construction of the Principle Model for the Temporary Support Robot

According to the working principle of the TSR and the structure of the chock-type hydraulic support [21], the principle model of the TSR is constructed as shown in Figure 4. The structural components of the simulation include the top beam of support 1, the base of support 1, the top beam of support 2, the base of support 2, the four-bar linkage mechanism of support 1, the four-bar linkage mechanism of support 2, the support hydraulic cylinder of support 1, the support hydraulic cylinder of support 2, roof, floor, and the moving cylinders. The TSR’s top beam is connected to the base through a four-bar linkage mechanism and supporting cylinder [22]. The support hydraulic cylinders of support 1 and support 2 are, respectively, hinged to the corresponding top beams and bases, providing support forces to both support 1 and support 2 [23]. The top beams of support 1 and support 2 make contact with the roof, while the bases of support 1 and support 2 make contact with the floor. The connection method of the four-bar linkage mechanism is hinged. The four-bar linkage can withstand horizontal loads from the rock layer, preventing the bending of the vertical columns. Additionally, it can control the variation in distance between the front end of the top beam and the coal wall within a certain range when the height of the top beam changes, thereby enhancing the control level of the roof.

3. Mathematical Model Construction Based on Theoretical Mechanics Methods

3.1. Construction of Stable Support Mechanical Model

In order to analyze the force distribution of the TSR under stable support conditions, a mechanical model of the TSR in a stable support state is constructed based on the principle model of the temporary support robot. In this model, the force on the TSR is simplified to a plane linkage model on the neutral surface [24], as shown in Figure 5.

The x-axis represents the direction of roadway excavation, and the y-axis represents the roadway height direction; the vertical distance from the roadway roof to the roadway floor is H; and the top beams and bases of support 1 and support 2 are aligned at the tail end. For ease of analysis, the distance from each hinge point on the top beams of support 1 and support 2 to the roof is H₁, and the distance from each hinge point on the bases of support 1 and support 2 to the base is H₂; the distances from points A₁, A₂, A₃, and A₄ to the tail end of support 1 are L₁, L₂, L₃, and L₄, respectively; and the distances from points B₁, B₂, B₃, and B₄ to the tail end of support 2 are L₅, L₆, L₇, and L₈, respectively. The external loading of the roof on the top beam of support 1 is Q₁₁, the supporting force of the floor on the base of support 1 is Q₁₂, and the distances from forces Q₁₁ and Q₁₂ to the tail end of support 1 are L_Q11 and L_Q12, respectively. The external loading of the roof on the top beam of support 2 is Q₂₁, the supporting force of the floor on the base of support 2 is Q₂₂, and the distances from forces Q₂₁ and Q₂₂ to the tail end of support 2 are L_Q21 and L_Q22, respectively. The weight of the top beam of support 1 is G11, and the distance from the center of gravity to the tail end of support 1 is L_G11. The overall weight of support 1 is G₁, and the distance from the center of gravity to the tail end of support 1 is L_G1. The weight of the top beam of support 2 is G₂₁, and the distance from the center of gravity to the tail end of support 2 is LG₂₁. The overall weight of support 2 is G₂, and the distance from the center of gravity to the tail end of support 2 is L_G2. The angles between the front and rear supporting cylinders of support 1 and the top beam are α₁ and α₂, respectively, and the working resistances are F₁ and F₂, respectively. The angles between the front and rear supporting cylinders of support 2 and the top beam are α₃ and α₄, respectively, and the working resistances are F₃ and F₄, respectively. The external loading Q₁₁ and Q₂₁ of the roof on the top beams of support 1 and support 2 are numerically equal to the supporting forces of support 1 and support 2 on the roof. The relative displacement of the top beams and bases of support 1 and support 2 of the TSR in the x-axis direction can be ignored.

When support 1 stably supports the roof, taking the top beam of support 1 as a separate body, the top beam of support 1 is in force equilibrium at this time, then the sum ∑F_y11 of the forces on the top beam of support 1 in the y-axis direction equal zero; the sum ∑M_A1 of all moments about point A₁ on the top beam of support 1 equal zero; and the sum ∑M_A3 of all moments about point A₃ on the top beam of support 1 equal zero. The force equilibrium equations are as follows:

$$\left\{\begin{array}{l}\sum F_{y11}=0\\ \sum M_{A1}=0\\ \sum M_{A3}=0\end{array}\right.,$$

(1)

According to the force equilibrium equations, the following can be obtained:

$$\left\{\begin{array}{l}{F}_1\sin {\alpha }_1+F_2\sin {\alpha }_2-Q_{11}-G_{11}=0\\ F_2\sin {\alpha }_2\left(L_1-L_3\right)-Q_{11}\left(L_1-L_{Q11}\right)-G_{11}\left(L_1-L_{G11}\right)=0\\ G_{11}\left(L_{G11}-L_3\right)+Q_{11}\left(S_1-L_3\right)-F_1\sin {\alpha }_1\left(L_1-L_3\right)=0\end{array}\right.$$

(2)

Taking the overall support 1 as the research object, support 1 as a whole is in force equilibrium, then the sum ∑F_y1 of the forces on support 1 in the y-axis direction equals zero; the sum ∑M_A of all moments about point A on support 1 equal zero; the force equilibrium equations are as follow:

$$\left\{\begin{array}{l}\sum F_y=0\\ \sum M_A=0\end{array}\right.$$

(3)

From the force equilibrium equations, the following can be obtained:

$$\left\{\begin{array}{l}{Q}_{12}-G_1-Q_{11}=0\\ G_1{L}_{G1}+S_1{Q}_{11}-Q_{12}{L}_{Q12}=0\end{array}\right.,$$

(4)

Similarly, when support 2 of the TSR stably supports the roof, it can be seen that

$$\left\{\begin{array}{l}{F}_3\sin {\alpha }_3+F_4\sin {\alpha }_4-Q_{21}-G_{21}=0\\ F_4\sin {\alpha }_4\left(L_5-L_7\right)-Q_{21}\left(L_5-L_{Q21}\right)-G_{21}\left(L_5-L_{G21}\right)=0\\ G_{21}\left(L_{G21}-L_7\right)+Q_{21}\left(L_{Q21}-L_7\right)-F_3\sin {\alpha }_3\left(L_5-L_7\right)=0\\ Q_{22}-Q_{21}-G_2=0\\ G_2{L}_{G2}+Q_{21}{S}_3-Q_{22}{S}_4=0\end{array}\right.,$$

(5)

where

$$\left\{\begin{array}{l}\sin {\alpha }_1={\displaystyle \frac{{\left(H-H_1-H_2\right)}^2}{{\left(L_1-L_2\right)}^2+{\left(H-H_1-H_2\right)}^2}}\\ \sin {\alpha }_2={\displaystyle \frac{{\left(H-H_1-H_2\right)}^2}{{\left(L_4-L_3\right)}^2+{\left(H-H_1-H_2\right)}^2}}\\ \sin {\alpha }_3={\displaystyle \frac{{\left(H-H_1-H_2\right)}^2}{{\left(L_5-L_6\right)}^2+{\left(H-H_1-H_2\right)}^2}}\\ \sin {\alpha }_4={\displaystyle \frac{{\left(H-H_1-H_2\right)}^2}{{\left(L_8-L_7\right)}^2+{\left(H-H_1-H_2\right)}^2}}\end{array}\right..$$

(6)

3.2. Construction of a Dynamic Model for Driving Under Pressure

According to the working principle of driving under pressure for the TSR, analyzing the force on the TSR during the driving under pressure process yields the principle model of driving under pressure for the TSR, as shown in Figure 6.

The x-axis represents the direction of roadway excavation, and the y-axis represents the height direction of the roadway. The pushing forces of the upper and lower sets of moving cylinders connecting support 1 and support 2 are denoted as T₁ and T₂, respectively. Let f₁ and f₂ be the friction coefficients between support 1, support 2, and the surrounding rock. The pushing and friction forces experienced by the two parts, support 1 and support 2, during the driving under pressure process, are parallel to the x-axis. Therefore, the resultant external loading Q₁₁ and Q₂₁ of the roof on the top beam of support 1 and support 2, and the resultant supporting force Q₁₂ and Q₂₂ of the floor on the base of support 1 and support 2, can still be expressed by Equations (2), (4) and (6). Assuming that support 1 is in force equilibrium during the driving under pressure process, the pushing force of the moving cylinder on support 1 and support 2 is equal to its load, i.e., T₁ = T_1z and T₂ = T_2z.

The process of driving under pressure for the TSR includes the driving under pressure of support 1 and the driving under pressure of support 2. When support 1 is driving under pressure, perform force analysis on the entire support 1. Assume that support 1 is in force equilibrium during the driving under pressure process. Then, the sum of forces ∑F_x1 on the entire support 1 in the x-axis direction equals zero, and the sum of forces ∑F_y1 on the entire support 1 in the y-axis direction equals zero. The force equilibrium equations are as follows:

$$\left\{\begin{array}{l}\sum F_{x1}=0\\ \sum F_{y1}=0\end{array}\right..$$

(7)

From the force equilibrium equations, it can be obtained that

$$\left\{\begin{array}{l}{T}_{1z}+T_{2z}-f_1{Q}_{a11}-f_1{Q}_{12}=0\\ Q_{12}-G_1-Q_{11}=0\end{array}\right..$$

(8)

When support 1 is accelerating, according to Newton’s second law, the dynamic model of the moving cylinder pushing support 1 is as follows:

$$T_1+T_2=f_1\left(Q_{11}+Q_{12}\right)+{\displaystyle \frac{m_1{d}^2{x}_1}{d{t}^2}},$$

(9)

where m₁ is the mass of support 1, kg; t is the time, s; x₁ is the displacement of support 1, m; and f₁ is the friction coefficient between support 1 and the surrounding rock.

When support 2 is in the state of driving under pressure, assuming that it is in the state of force equilibrium, similarly, it can be known that the dynamic model of the moving cylinder pulling support 2 is as follows:

$$\left\{\begin{array}{l}{T}_1+T_2=f_2\left(Q_{21}+Q_{22}\right)+{\displaystyle \frac{m_2{d}^2{x}_2}{d{t}^2}}\\ Q_{22}-G_1-Q_{21}=0\end{array}\right.,$$

(10)

where m₂ is the mass of support 2, (kg); x₂ is the displacement of support 2, (m); and f₂ is the friction coefficient between support 2 and the surrounding rock.

When the TSR is driving under pressure, there is a transition from static friction to dynamic friction between the TSR and the roof and floor. To accurately describe this friction process, this paper adopts the Stribeck friction theory [25,26] to analyze the friction coefficient between them. The model is as follows:

$$\left\{\begin{array}{l}{f}_1=f_d+\left(f_s-f_d\right)e^{-{\left(v_1/v_{st}\right)}^2}\\ f_2=f_d+\left(f_s-f_d\right)e^{-{\left(v_2/v_{st}\right)}^2}\end{array}\right.,$$

(11)

where f_d is the dynamic friction coefficient between the TSR and the surrounding rock; f_s is the static friction coefficient between the TSR and the surrounding rock; v_st is the critical Stribeck velocity, (m/s); v₁ is the moving speed of support 1, m/s; and v₂ is the moving speed of support 2, m/s.

3.3. Boundary Condition Analysis of the Dynamic Model for Driving Under Pressure

When studying the driving under pressure process of the TSR, it is necessary to consider the boundary conditions of its dynamic mode and analyze its criterion system. Since support 2 must maintain a stable support state and not move during the driving under the pressure process of support 1, the friction force generated by its contact with the surrounding rock must be able to overcome the pushing force of the moving cylinder. The same applies when support 2 is driving under pressure.

When support 1 is driving under pressure, it can be obtained from Equation (9) that

$$f_1\left(Q_{11}+Q_{12}\right)+{\displaystyle \frac{m_1{d}^2{x}_1}{d{t}^2}}<f_s\left(Q_{21}+Q_{22}\right),$$

(12)

When support 2 is driving under pressure, it can be obtained from Equation (10) that

$$f_2\left(Q_{21}+Q_{22}\right)+{\displaystyle \frac{m_2{d}^2{x}_2}{d{t}^2}}<f_s\left(Q_{11}+Q_{12}\right).$$

(13)

The base area of each support 1 and support 2 is smaller than the top beam area. Due to its weight’s influence, the base’s specific pressure against the floor is greater than the specific pressure of the top beam against the roof. To ensure that the TSR can stably support the roof and not damage the surrounding rock during the driving under pressure process, it is necessary to analyze the relationship between the top beam and the base area of support 1 and support 2, and the specific pressure of the surrounding rock.

Since f₁ ≤ f_s and f₂ ≤ f_s, according to the pressure formula F = PS, it can be obtained that

$$\left\{\begin{array}{l}{P}_{11d}{S}_{11}=P_{12d}{S}_{12}-G_1\\ P_{21d}{S}_{21}=P_{22d}{S}_{22}-G_2\\ P_{22s}{S}_{22}-P_{12d}{S}_{12}>{\displaystyle \frac{m_1{d}^2{x}_1}{2f_{s}d{t}^2}}+{\displaystyle \frac{\left(G_2-G_1\right)}{2}}\\ P_{12s}{S}_{12}-P_{22d}{S}_{22}>{\displaystyle \frac{m_2{d}^2{x}_2}{2f_{s}d{t}^2}}+{\displaystyle \frac{\left(G_1-G_2\right)}{2}}\end{array}\right.,$$

(14)

where P_11d is the specific pressure of the top beam against the roof when support 1 is in the state of driving under pressure, Pa; P_12d is the particular pressure of the base against the floor when support 1 is in the state of driving under pressure, Pa; P_12s is the specific pressure of the base against the floor when support 1 is in stable support, Pa; P_21d is the particular pressure of the top beam against the roof when support 2 is in the state of driving under pressure, Pa; P_22d is the specific pressure of the base against the floor when support 2 is in the state of driving under pressure, Pa; P_22s is the specific pressure of the base against the floor when support 2 is in stable support, Pa; S₁₁ is the area of the top beam of support 1, m²; S₁₂ is the area of the base of support 1, m²; S₂₁ is the area of the top beam of support 2, m²; and S₂₂ is the area of the base of support 2, m².

3.4. Analysis of Mechanical Properties of Roof and Floor

The mechanical properties of the roof and floor are analyzed to obtain the minimum support strength required for the TSR to support the roof stably and the maximum allowable value of the specific pressure between the base and the floor.

To ensure that the roof does not fracture, the support strength of the TSR must ensure that the deformation of the roof is maintained within a certain range, ensuring that the tensile stress of the roof does not exceed the tensile strength limit of the roof. The thin plate theory [27] is used to study the support strength. Assume that the roof is a continuous layered elastic medium, the roof rock mass is an isotropic homogeneous body, and there is no tie force between the roof layers. The roof model of the section coal pillar excavation and mining working face is shown in Figure 7. The TSR is responsible for supporting the empty top area; the two sides of the empty top area are sidewalls with a length of a (m), the area to be excavated is located in front of the direct roof with a width of b (m), the thickness of the roof is δ (m), and the plane of the coordinate axis z = 0 is located at δ/2 (m) of the roof thickness. The anchoring area comprises anchor bolts and bolt nets behind the roof. Therefore, the boundary conditions of the roof can be regarded as three sides with a clamped edge and one side with a simply supported edge.

The boundary conditions of the clamped edge OC, OD, and DE are as follows [28]:

$$\left\{\begin{array}{l}{\left(w\right)}_{y=0}={\left(w\right)}_{x=0}={\left(w\right)}_{y=b}=0\\ {\left({\displaystyle \frac{\partial w}{\partial y}}\right)}_{y=0}={\left({\displaystyle \frac{\partial w}{\partial y}}\right)}_{x=0}={\left({\displaystyle \frac{\partial w}{\partial y}}\right)}_{y=b}=0\end{array}\right.,$$

(15)

where w is the deflection equation of the roof.

The boundary conditions of the simply supported edge CE are as follows:

$$\left\{\begin{array}{l}{\left(w\right)}_{x=a}=0\\ {\left({\displaystyle \frac{{\partial }^{2}w}{{\partial }^{2}y}}\right)}_{x=a}=0\end{array}\right..$$

(16)

Based on the assumption, after the thin plate is deformed, the straight line originally perpendicular to the middle plane remains straight, and the length remains unchanged and is always perpendicular to the deformed middle plane. The strain in the thickness direction can be neglected, and the deflection w is only related to the plane coordinates (x, y) and has nothing to do with the thickness direction coordinate z. The stress state of the thin plate can be approximately regarded as a plane stress state; that is, the normal stress and shear stress perpendicular to the middle plane are much smaller than the normal stress and shear stress in the middle plane and can be ignored. The deformation of the middle plane of the thin plate is very small, and it can be considered that there is no tensile or compressive deformation of the middle plane. The external loading on the roof is q. According to the thin plate theory, the differential equation representing the deflection w of the roof can be obtained as follows:

$$\left\{\begin{array}{l}{\displaystyle \frac{{\partial }^{4}w}{\partial x^4}}+2{\displaystyle \frac{{\partial }^{4}w}{\partial x^2\partial y^2}}+{\displaystyle \frac{{\partial }^{4}w}{\partial y^4}}={\displaystyle \frac{q}{D}}\\ D={\displaystyle \frac{E{\delta }^3}{12(1-{\mu }^2)}}\end{array}\right.,$$

(17)

where D is the bending stiffness of the roof, N/m; E is the elastic modulus of the roof, MPa; and μ is the Poisson’s ratio.

Then, the tensile stresses σ_x and σ_y in the x and y directions of the roof are as follows:

$$\left\{\begin{array}{l}{\sigma }_x=-{\displaystyle \frac{8{\pi }^{2}AEz}{a^2(1-{\mu }^2)}}\left[{\sin }^2{\displaystyle \frac{\pi y}{b}}\cos {\displaystyle \frac{2\pi x}{a}}+\mu {\left({\displaystyle \frac{a}{b}}\right)}^2{\sin }^2{\displaystyle \frac{\pi x}{a}}\cos {\displaystyle \frac{\pi y}{b}}\right]\\ {\sigma }_y=-{\displaystyle \frac{8{\pi }^{2}AEz}{b^2(1-{\mu }^2)}}\left[{\sin }^2{\displaystyle \frac{\pi x}{a}}\cos {\displaystyle \frac{2\pi y}{b}}+\mu {\left({\displaystyle \frac{a}{b}}\right)}^2{\sin }^2{\displaystyle \frac{\pi y}{b}}\cos {\displaystyle \frac{\pi x}{a}}\right]\\ A={\displaystyle \frac{q{a}^2}{4{\pi }^{4}D\left[3+3{\left(\frac{a}{b}\right)}^4+2{\left(\frac{a}{b}\right)}^2\right]}}\end{array}\right.,$$

(18)

Taking the tensile stress on the bottom surface of the roof, that is the z = −δ/2 plane, as the research object, the maximum tensile stress values in the x and y directions of the roof can be obtained as follows:

$$\left\{\begin{array}{c}{\sigma }_{x\max }={\displaystyle \frac{4{\pi }^{2}AE\delta }{a^2(1-{\mu }^2)}}\\ {\sigma }_{y\min }={\displaystyle \frac{4{\pi }^{2}AE\delta }{b^2(1-{\mu }^2)}}\end{array}\right..$$

(19)

For the roof supported by the TSR in actual work, its length a must be less than width b, then the stress on the roof in the y direction must be greater than the stress on the roof in the x direction. From Equation (19), it can be obtained that

$${\displaystyle \frac{12q{a}^2}{{\pi }^2{b}^2{\delta }^2\left[3+3{\left(\frac{a}{b}\right)}^4+2{\left(\frac{a}{b}\right)}^2\right]}}<{\sigma }_{\mu },$$

(20)

where σ_μ is the tensile strength limit of the roof material, Mpa. Considering the support strength p_min of the TSR to the roof and the rock load q_o of the roof, Equation (20) can be rewritten as follows:

$$p>q_o-{\displaystyle \frac{{\pi }^2{b}^2{\delta }^2{\sigma }_{\mu }\left[3+3{\left(\frac{a}{b}\right)}^4+2{\left(\frac{a}{b}\right)}^2\right]}{12a^2}},$$

(21)

Equation (21) provides the minimum allowable value of the TSR’s support strength to the roof.

To prevent the support force of the TSR from being too large and causing the floor to break, it is necessary to calculate the maximum specific pressure p_max that the floor can withstand according to the maximum compressive strength of the floor [29]. Since the actual contact area between the base of the TSR and the floor is smaller than the area of the base, the cutting, pushing, and other behaviors during the excavation process will directly damage the integrity of the floor and generate defects such as cracks and micro-cracks, these defects will reduce the bearing capacity of the floor. Therefore, the coefficient C represents the influence on the maximum specific pressure the floor can withstand.

$$p_{\max }=C{\sigma }_b.$$

(22)

where σ_b is the uniaxial compressive strength limit of the floor material.

4. Simulation Experiment and Mathematical Model Validation

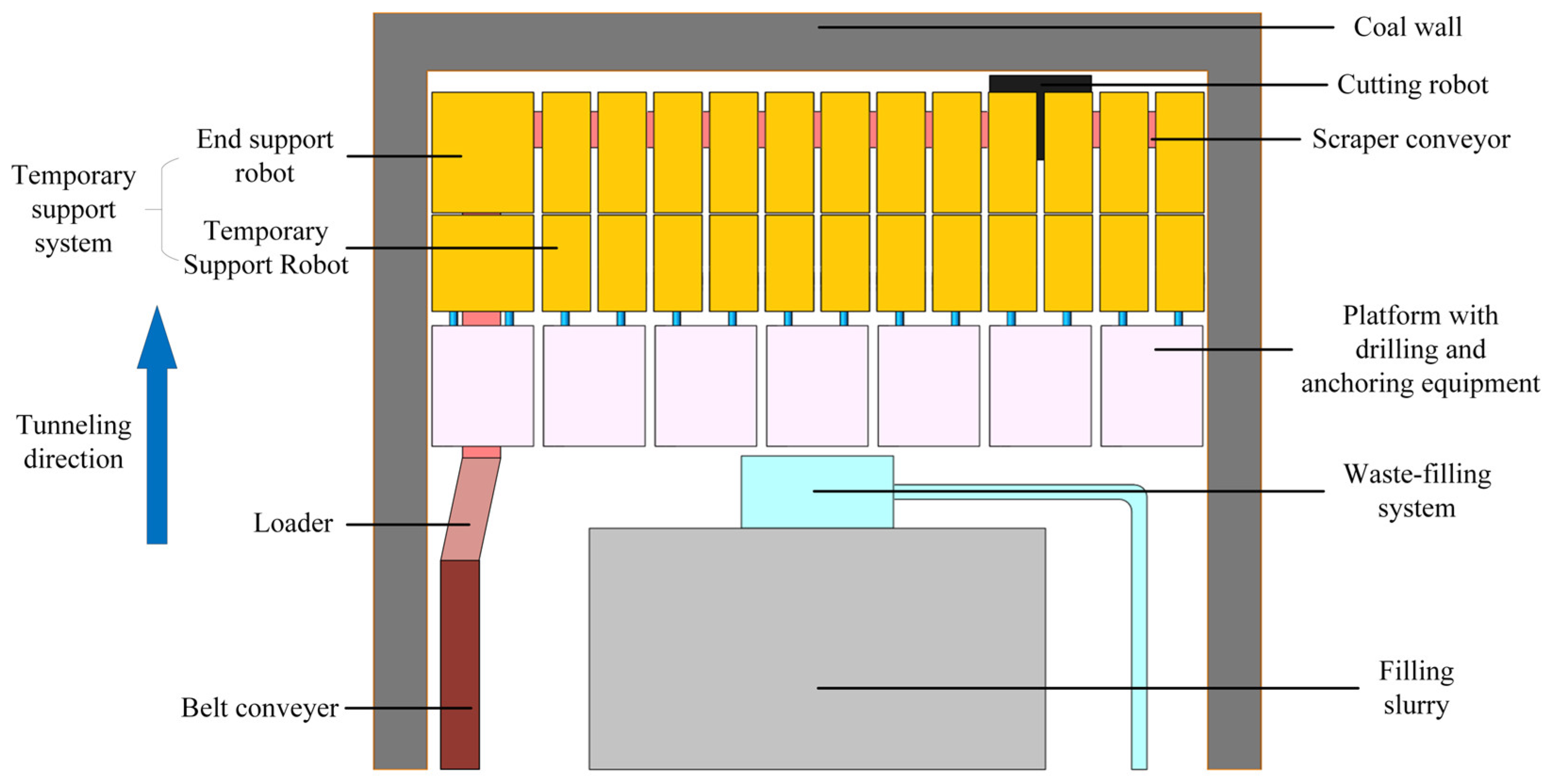
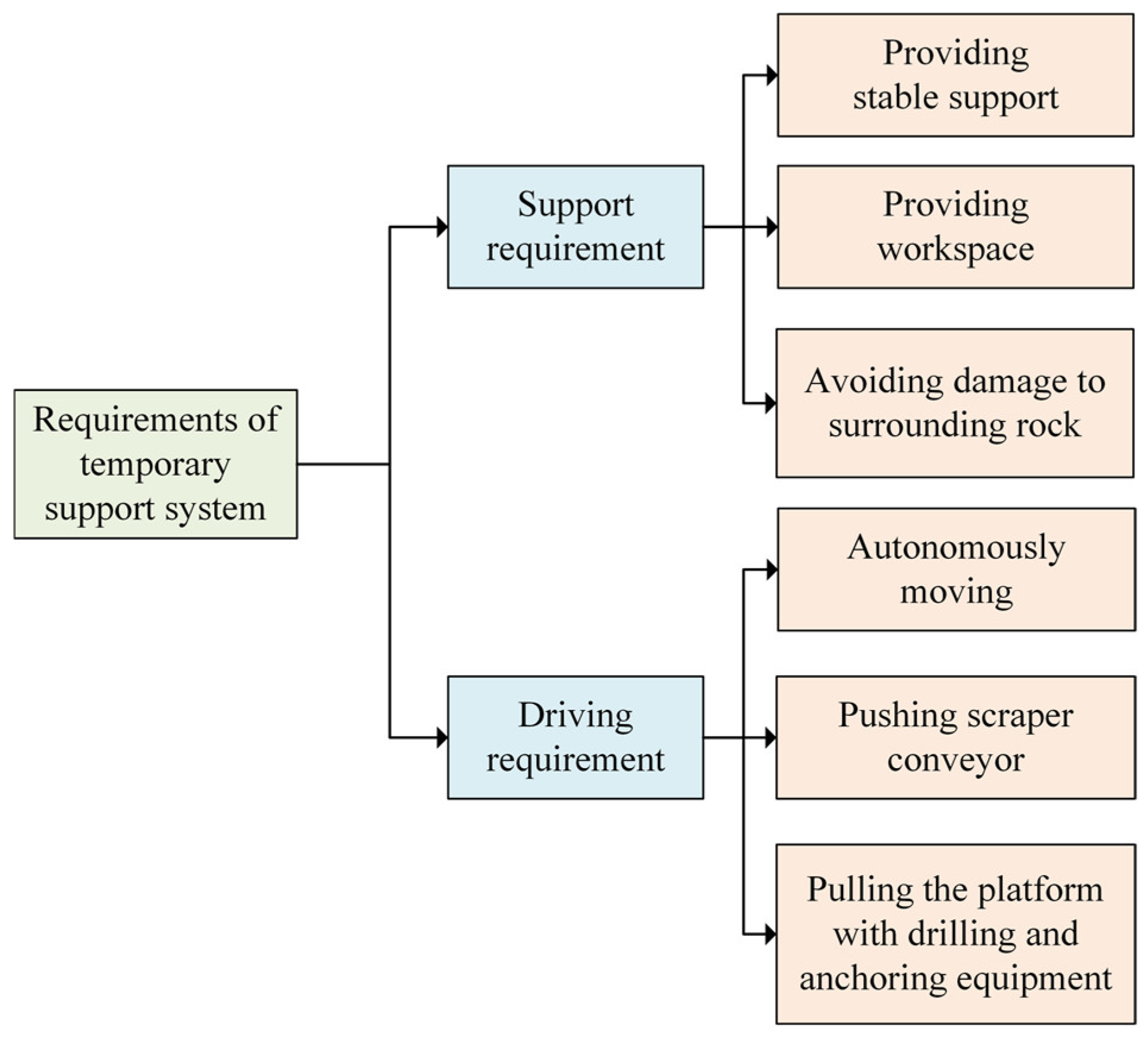
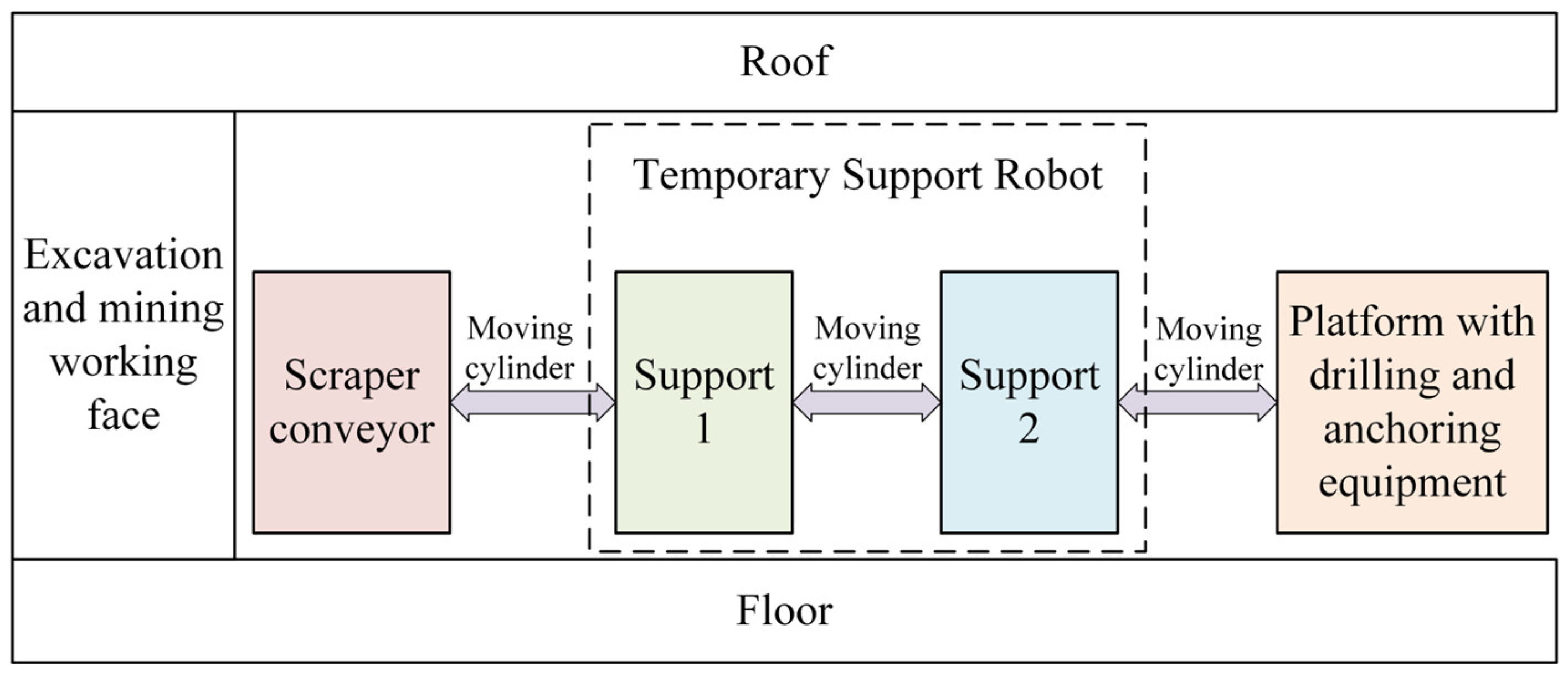
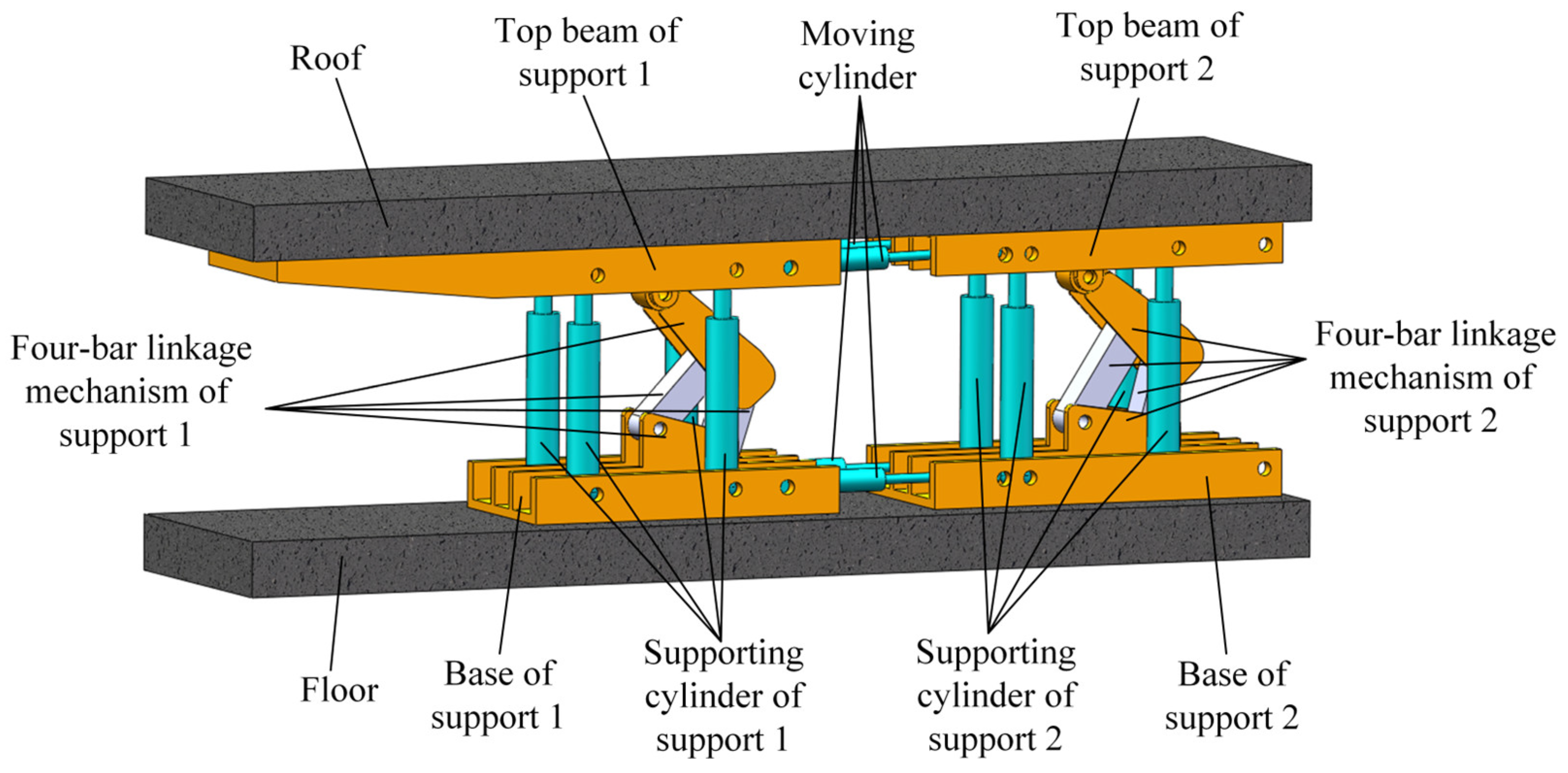
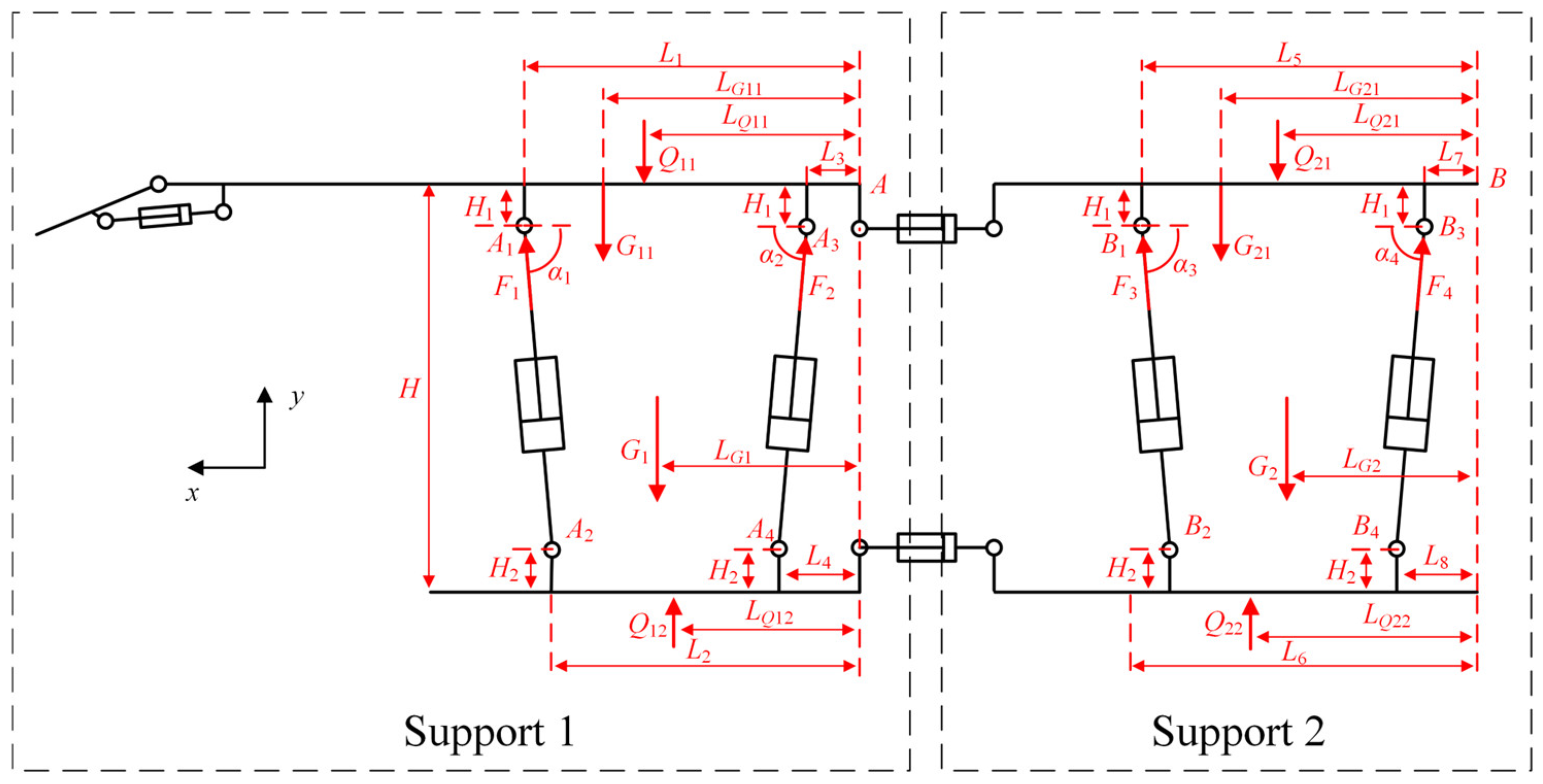
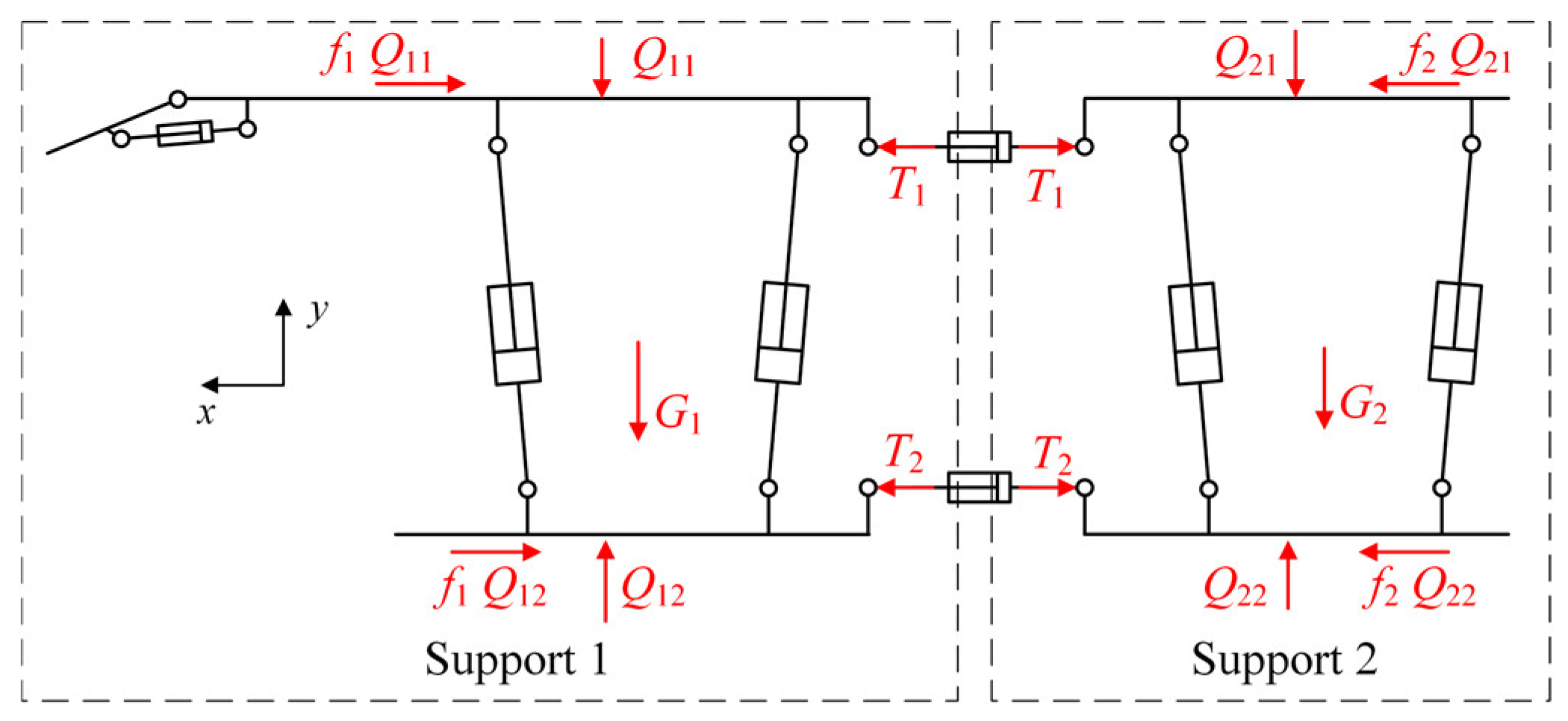
4.1. Determination of Simulation Parameters for the Temporary Support Robot

According to the working environment of the TSR, we set the relevant parameters for the surrounding rock of the working face, as shown in

Table 1. The relevant parameters for the surrounding rock of the working face.

Parameter	Value
the tensile strength limit of the roof σμ/MPa	1.2
the roof rock load is qo/MPa	233
length of the empty top area a/m	15
width of the empty top area b/m	30
the thickness of the roof δ/m	4

. The minimum allowable value of the support strength of the TSR to the roof, p_min = 0.02 MPa, is calculated by Equation (21). The uniaxial compressive strength of the floor material is taken as σ_b = 4.1 MPa; the coefficient is C = 0.05; and the minimum allowable value of the support strength of the TSR to the roof, p_max ≈ 0.2 MPa, is calculated by Equation (22).

To analyze the boundary conditions of the driving under pressure dynamic model of the TSR under given conditions and to obtain the relationship between the specific pressure of the top beam and base of support 1 and support 2 on surrounding rock, the boundary conditions of the driving under pressure dynamic model of the TSR are simulated. Relevant parameters are shown in

Table 2. Simulation parameter table of boundary conditions for driving under pressure dynamic model.

Parameter	Value
overall mass of support 1 m1/kg	18,000
overall mass of support 2 m2/kg	16,000
mass of top beam of support 1 ma/kg	8000
mass of top beam of support 2 mb/kg	5000
dynamic friction coefficient fd	0.28
static friction coefficient fs	0.3

. It is assumed that the support strength of the TSR to the roof cannot be less than 0.02 MPa, and the specific pressure in contact with the surrounding rock cannot be greater than 0.2 MPa. To ensure the stability of the driving under pressure motion process of the TSR, the acceleration of support 1 and support 2 during movement is taken not to exceed 0.2 m/s². According to the cooperation mode between the TSR and the cutting robot, the TSR’s width is 2 m. The space length required for the cutting robot and scraper conveyor located below the top beam of support 1 is at least 3 m, and the length of the top beam and floor of support 2 are the same. Then, the area relationship between the top beam and base of support 1 is S₁₁ − S₁₂ = 6 m², and the area relationship between the top beam and base of support 2 is S₂₁ = S₂₂. The supporting force of support 1 and support 2 is the largest during stable support. Therefore, the specific pressure of the base of support 1 and support 2 against the floor during stable support 1s is taken as P_12s = P_22s = 0.2 MPa. 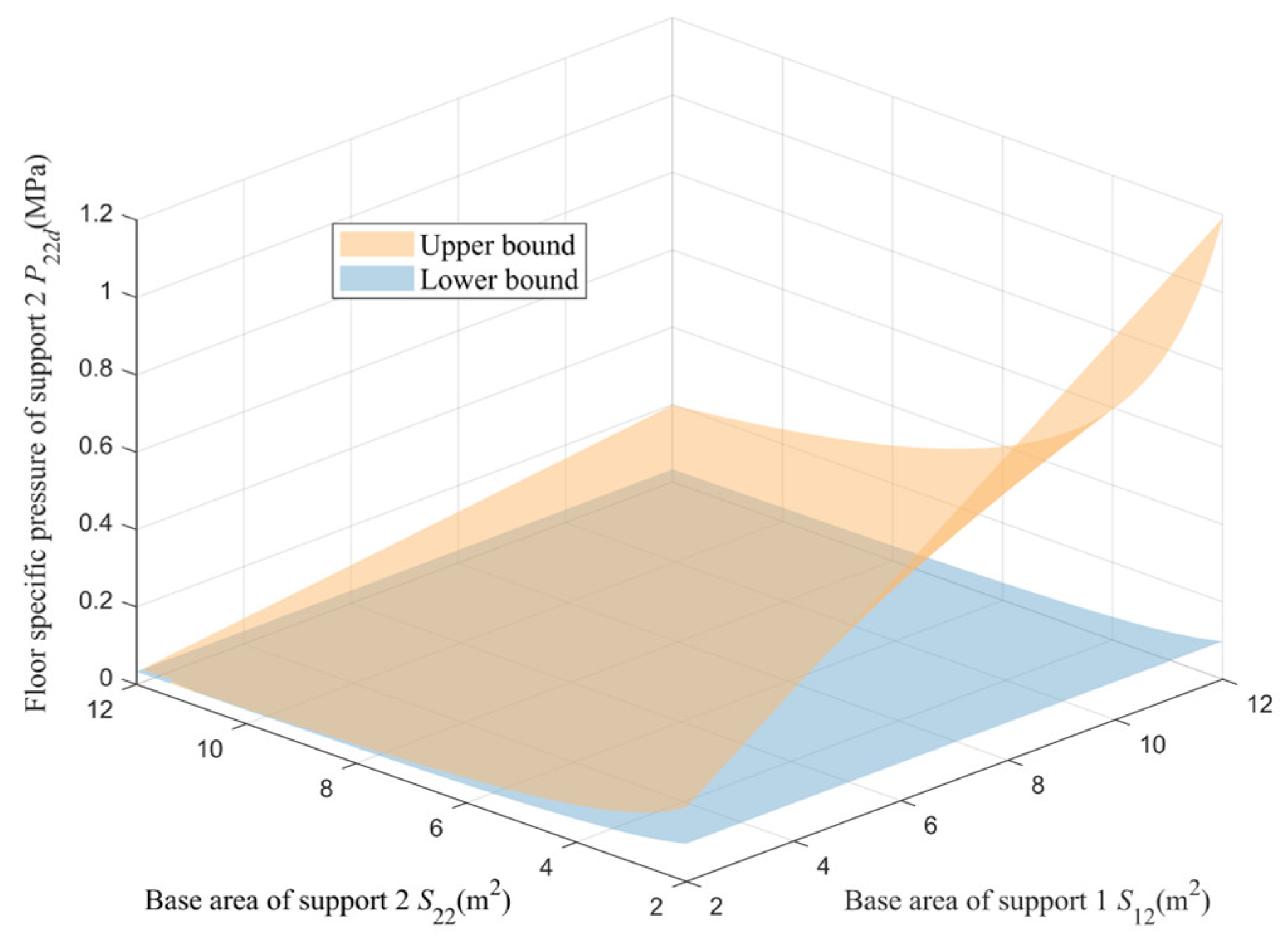From Equation (13), the value range of the specific pressure P_12d of the base of support 1 against the floor during the state of driving under pressure and the value range of the specific pressure P_22d of the base of support 2 against the floor during in the state of driving under pressure can be obtained, as shown in Figure 8 and Figure 9, which vary with the area S₁₂ of the base of support 1 and the support area S₂₂ of support 2.

Analyzing Figure 8 and Figure 9, it can be seen that when the ratio of the base area of support 1 to support 2 is smaller, the value range of P_12d is larger, and the value range of P_22d is smaller. When the ratio of the base area of support 1 to support 2 is larger, the value range of P_12d is smaller, and the value range of P_22d is larger. The upper limit of P_12d and P_22d determines the critical value at which support 1 and support 2 can drive under pressure, and the lower limit determines the critical value for ensuring that the roof does not collapse during the driving under pressure process of support 1 and support 2.

Taking the area of support 1’s top beam as S₁₁ = 8 m²; the area of support 1’s base as S₁₂ = 9 m²; the area of support 2’s top beam as S₂₁ = 8 m²; and the area of support 2’s base as S₂₁ = 8 m², the value range of the specific pressure of the base of support 1 and support 2 against the floor can be calculated by Equation (14) as 0.056 MPa < P_12d < 0.22 MPa and 0.038 MPa < P_22d < 0.17 MPa. For ease of analysis, the angles of the supporting cylinders for both support 1 and support 2 are set to 90 degrees. The other parameters of the TSR are listed in

Table 3. Other parameters for the virtual prototype model.

Parameter	Value
the distance from each hinge point on the top beams of support 1 and support 2 to the roof H1/mm	0.3
the distance from each hinge point on the bases of support 1 and support 2 to the base H2/mm	0.3
the distances from point A1 to the tail end of support 1 L1/mm	2.8
the distances from point A2 to the tail end of support 1 L2/mm	2.8
the distances from point A3 to the tail end of support 1 L3/mm	1.2
the distances from point A4 to the tail end of support 1 L4/mm	1.2
the distances from point B1 to the tail end of support 2 L5/mm	2.9
the distances from point B2 to the tail end of support 2 L6/mm	2.9
the distances from point B3 to the tail end of support 2 L7/mm	1.2
the distances from point B4 to the tail end of support 2 L8/mm	1.2
the vertical distance from the roof to the floor H/m	3

.

4.2. Finite Element Simulation of the Temporary Support Robot and the Floor

To analyze whether the stresses on the TSR itself and the floor under stable support conditions meet the strength requirements, the stress characteristics of the floor and the TSR were simulated and analyzed using finite element analysis. In the finite element analysis process, the model constructed in Section 2.3 of the TSR was imported into the ANSYS Workbench 2023 R1 software, omitting non-load-bearing components such as support columns; small structures like lifting rings and mounting holes were ignored; the effects of rounded corners and welding on the load-bearing characteristics of the support were not considered; and equivalent loads were used to replace the column structure. The relevant parameters of the simulation model are consistent with those described in Section 4.1.

According to the actual working environment of the TSR, appropriate parameters are selected, and the relevant parameters are shown in

Table 4. Relevant material parameters for the finite element simulation.

Parameter	Value
elastic modulus of Q690 Steel/GPa	206
density of Q690 Steel kg/m3	7850
Poisson’s ratio of Q690 Steel	0.26
yield strength of Q690 Steel/MPa	690
bulk modulus of sandstone/GPa	21.3
Young’s modulus of sandstone/GPa	34
Poisson’s Ratio of sandstone	0.233
shear modulus of sandstone/GPa	13.78

. Based on this, the automatic mesh generation function in the software was used to mesh the simulation models of the TSR and its corresponding floor, resulting in a finite element model with 29,282 elements and 13,631 nodes for support 1, and a model with 25,762 elements and 11,204 nodes for support 2. When the TSR achieves a bottom pressure of 0.2 MPa, it can be calculated using the pressure formula and Equation (2) that the bottom pressure of support 1 on the roof is approximately 0.1 MPa. Similarly, when the TSR reaches a bottom pressure of 0.2 MPa, it can be calculated using the pressure formula and Equation (5) that the bottom pressure of support 2 on the roof is approximately 0.18 MPa. Based on the model shown in Figure 4, a simulation analysis of the load on the top beam was conducted, as illustrated in Figure 4 and Figure 10. 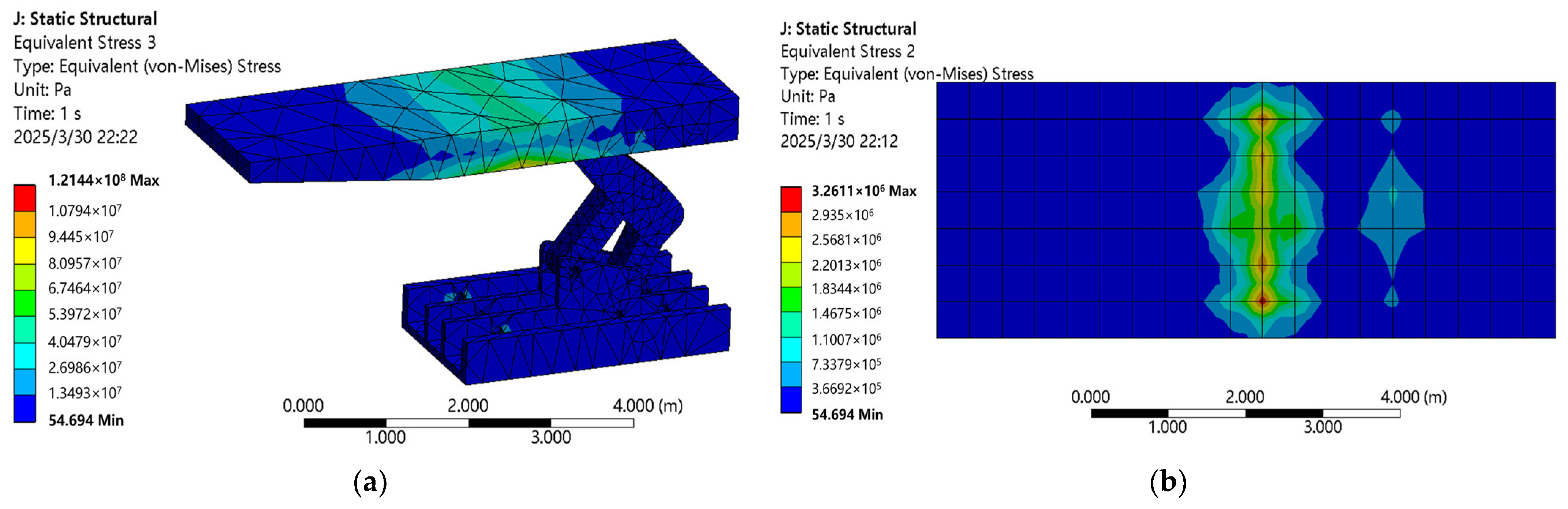Figure 10 shows the simulation results under a uniform load of 0.1 MPa applied to the top beam of support 1, where Figure 10a represents the stress cloud diagram of support 1 and Figure 10b represents the stress cloud diagram of the floor under support 1. 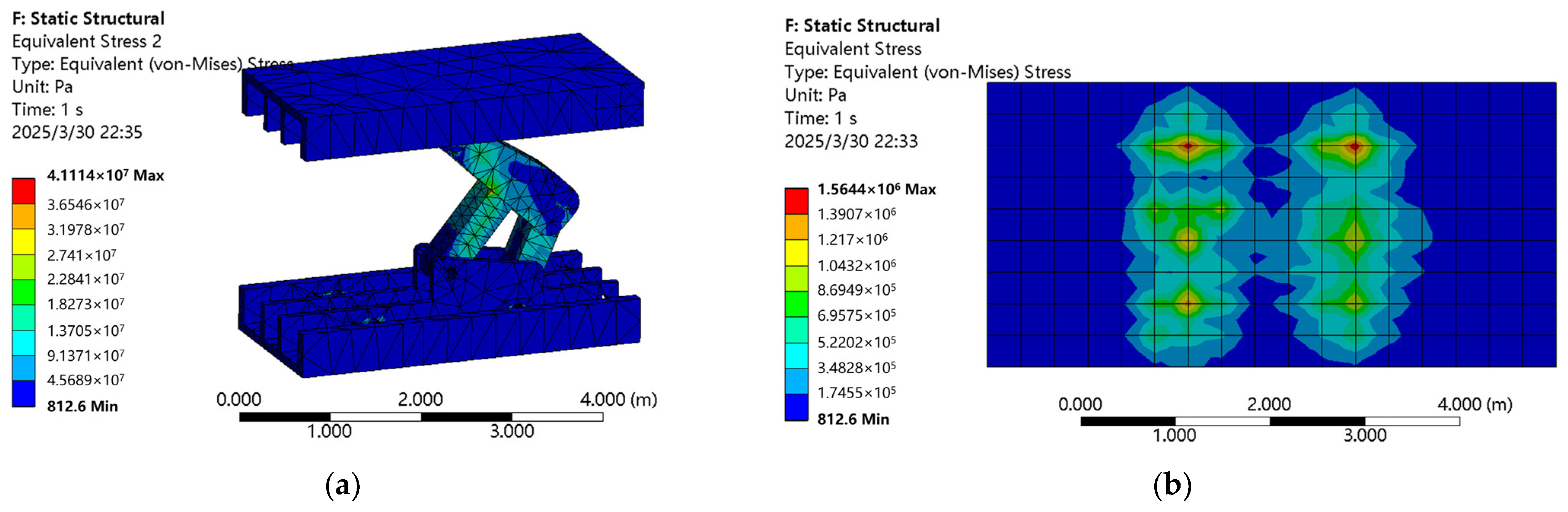Figure 11 shows the simulation results under a uniform load of 0.18 MPa applied to the top beam of support 2, with Figure 11a representing the stress cloud diagram of support 2 and Figure 11b representing the stress cloud diagram of the floor under support 2.

From Figure 10a, it can be seen that under a uniform load of 0.1 MPa applied to the top beam of support 1, the overall stress on support 1 is within the range of 121 MPa, which is below the yield strength of Q690. The peak stress is concentrated on the rib plate at the hinge between the top beam and the support cylinder, indicating that this part of the structure is more prone to damage under these conditions. The displacement of support 1 is within the range of 11 mm. From Figure 10b, it can be seen that regarding the stress conditions of the floor, the stress on the surface of the floor is within the range of 3.26 MPa, which is below the uniaxial compressive strength limit of the floor material. The peak stress is concentrated at the front end of the base of support 1. Therefore, under the 0.1 MPa loading condition on the top beam, the stress on the structure of support 1 is less than the yield strength of its material, meeting the strength requirements of the support, and the stress value on the floor is less than its uniaxial compressive strength, ensuring that the floor will not be damaged.

From Figure 11a, it can be seen that under a uniform load of 0.18 MPa applied to the top beam of support 2, the overall stress on support 2 is within the range of 41 MPa, with a peak value below the yield strength of Q690, concentrated on the four-bar mechanism, indicating that this part of the structure is more prone to damage under these conditions. From Figure 11b, it can be noted that regarding the stress conditions of the floor, the stress on the surface of the floor is within the range of 1.56 MPa, which is below the uniaxial compressive strength limit of the floor material, with peaks mainly concentrated at the installation position of the support cylinder on the base of support 2. Therefore, under a loading condition of 0.18 MPa, the stress on the structure of support 2 is less than the yield strength of its material, satisfying the strength requirements of the support, and the stress value on the floor is less than its uniaxial compressive strength, ensuring that the floor will not be damaged.

4.3. Critical Value Simulation of Boundary Conditions for Driving Under Pressure Dynamic Model

Import the model constructed in Section 2.3 of the TSR into the Adams View 2018 software. The driving under pressure process of support 1 and support 2 is simulated and analyzed using the Adams software to obtain the pushing force of the moving cylinder and the critical values of the movement of support 1 and support 2 to verify the accuracy of the boundary conditions of the driving under pressure dynamic model. The relevant parameters of the simulation model are consistent with those described in Section 4.1. The contact stiffness between the surrounding rock and the TSR is 1 × 108 N/m, the damping coefficient is 1 × 10⁵ Ns/m, and the penetration depth is 0.1 mm.

When support 1 is in the state of driving under pressure, it is known from the value range of the specific pressure of the base of support 1 against the floor, 0.056 MPa < P_12d < 0.22 MPa, that the specific pressure P_12d of the base of support 1 against the floor falls within the range of the maximum allowable specific pressure of 0.2 MPa for the contact between the TSR and the surrounding rock, enabling driving under pressure action. Therefore, two cases, P_12d = 0.1 MPa and P_12d = 0.2 MPa, are used for the simulation analysis. When support 2 is stably supporting, its base is under the specific pressure P_22s = 0.2 MPa. Assuming F₁ = F₂ and F₃ = F₄, it can be calculated from Equations (2) and (5) that the working resistance of each supporting cylinder of support 2 is approximately 423 kN, and the maximum friction force generated by contact with the surrounding rock is approximately 1033 kN. When P_12d = 0.1 MPa, the specific pressure of the top beam of support 1 against the roof, P_11d, is approximately 0.045 MPa, satisfying the support conditions. Currently, each supporting cylinder of support 1 has a working resistance of approximately 175.5 kN, and the maximum friction force generated by contact with the surrounding rock is 427 kN. When P_12d = 0.2 MPa, the specific pressure of the top beam of support 1 against the roof, P_11d, is approximately 0.1 MPa, satisfying the support conditions. Currently, each supporting cylinder of support 1 has a working resistance of approximately 376 kN, and the maximum friction force generated by contact with the surrounding rock is 907 kN. A gradually increasing pushing force is applied synchronously to the four moving cylinders for simulation, and the variation in the displacement of support 1 and support 2, as well as the friction between them and the surrounding rock with the resultant pushing force, are shown in Figure 12 and Figure 13.

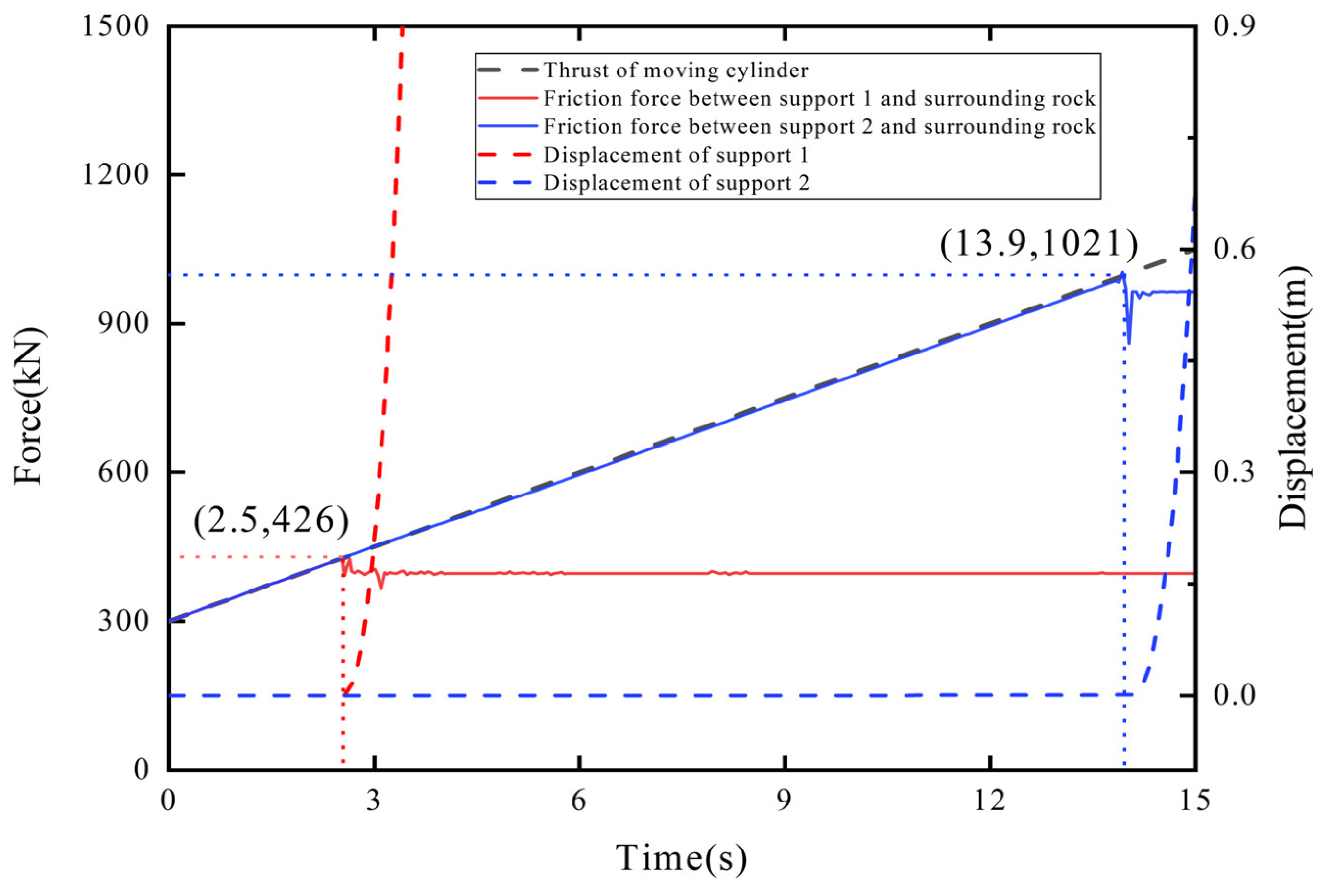
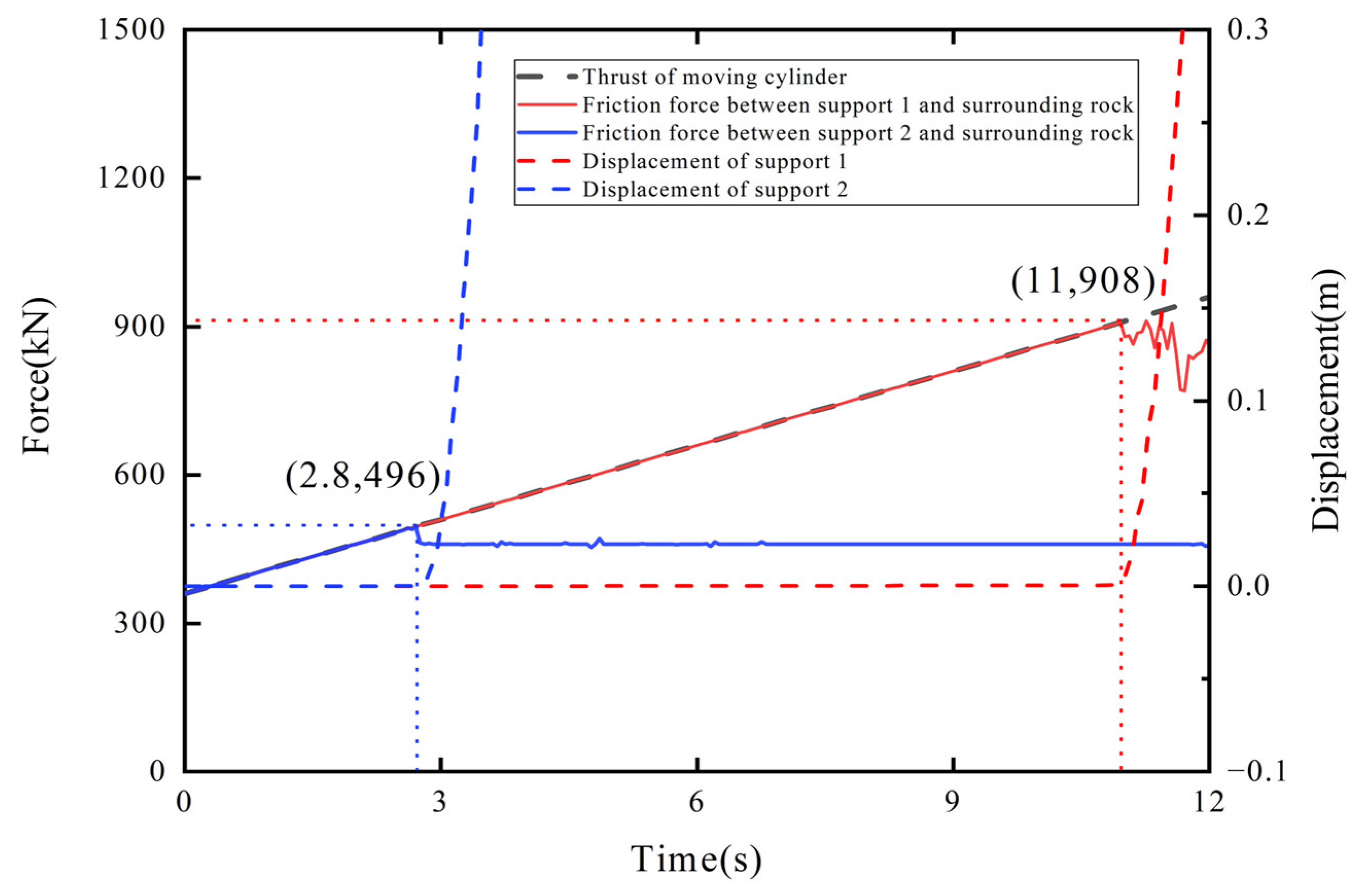
Analyzing Figure 12, it can be seen that under the condition that the specific pressure of the base of support 1 against the floor is P_12d = 0.1 MPa, when the pushing force of the moving cylinder exceeds 426 kN, the friction force between support 1 and the surrounding rock reaches its maximum value, and support 1 begins to move. The friction force between support 2 and the surrounding rock continues to increase with the pushing force of the moving cylinder, and support 2 remains stationary. At this time, support 1 can normally drive under pressure. When the pushing force of the moving cylinder exceeds 1021 kN, the friction force between support 2 and the surrounding rock reaches its maximum value, and support 2 begins to move. At this time, support 1 cannot drive under pressure. Analyzing Figure 13, it can be seen that under the condition that the specific pressure of the base of support 1 against the floor is P_12d = 0.2 MPa when the pushing force of the moving cylinder exceeds 908 kN, the friction force between support 1 and the surrounding rock reaches its maximum value, and support 1 begins to move. The friction force between support 2 and the surrounding rock continues to increase with the pushing force of the moving cylinder, and support 2 remains stationary. At this time, support 1 can normally drive under pressure. When the pushing force of the moving cylinder exceeds 1031 kN, the friction force between support 2 and the surrounding rock reaches its maximum value, and support 2 begins to move. At this time, support 1 cannot drive under pressure. Compared with the theoretical values, the maximum error of the maximum friction force between support 1 and support 2 and the surrounding rock obtained by simulation is 1.2%, less than 5%.

When support 2 is in the state of driving under pressure, it is known from the value range of the specific pressure of the base of support 2 against the floor, 0.038 MPa < P_22d < 0.17 MPa, that support 2 is in the state of driving under pressure when the specific pressure P_22d of the base of support 2 against the floor is taken in the range of 0.038 MPa to 0.17 MPa; support 2 cannot drive under pressure when the specific pressure P_22d of the base of support 2 against the floor is taken in the range of 0.17 MPa to 0.2 MPa. Therefore, two cases, P_22d = 0.1 MPa and P_22d = 0.19 MPa, are used for the simulation analysis. When support 1 is stably supporting, its base is under the specific pressure P_12s = 0.2 MPa. Assuming F₁ = F₂ and F₃ = F₄, it can be calculated from Equations (2) and (5) that each supporting cylinder of support 1 has a working resistance of approximately 376 kN, and the maximum friction force generated by contact with the surrounding rock is approximately 908 kN. When P_22d = 0.1 MPa, the specific pressure of the top beam of support 2 against the roof, P_21d, is approximately 0.082 MPa, satisfying the support conditions. Each supporting cylinder of support 2 has a working resistance of approximately 198 kN, and the maximum friction force generated by contact with the surrounding rock is 492 kN. When P_22d = 0.19 MPa, the specific pressure of the top beam of support 2 against the roof, P_21d, is approximately 0.17 MPa, satisfying the support conditions. Currently, each supporting cylinder of support 1 has a working resistance of approximately 401 kN, and the maximum friction force generated by contact with the surrounding rock is 979 kN. A gradually increasing pushing force is applied synchronously to the four moving cylinders for simulation, and the variation in the displacement of support 1 and support 2, as well as the friction between them and the surrounding rock with the resultant pushing force, are shown in Figure 14 and Figure 15.

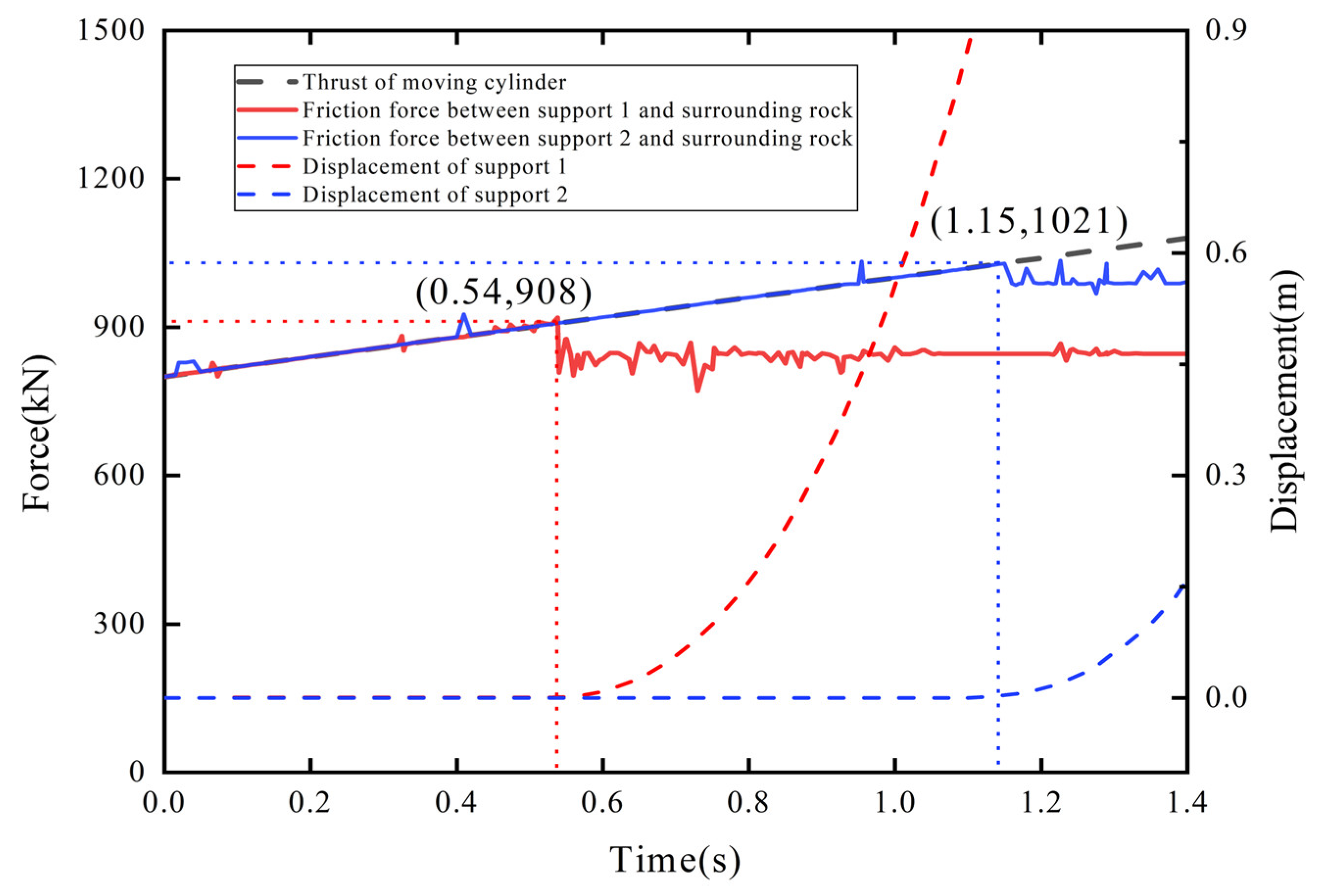
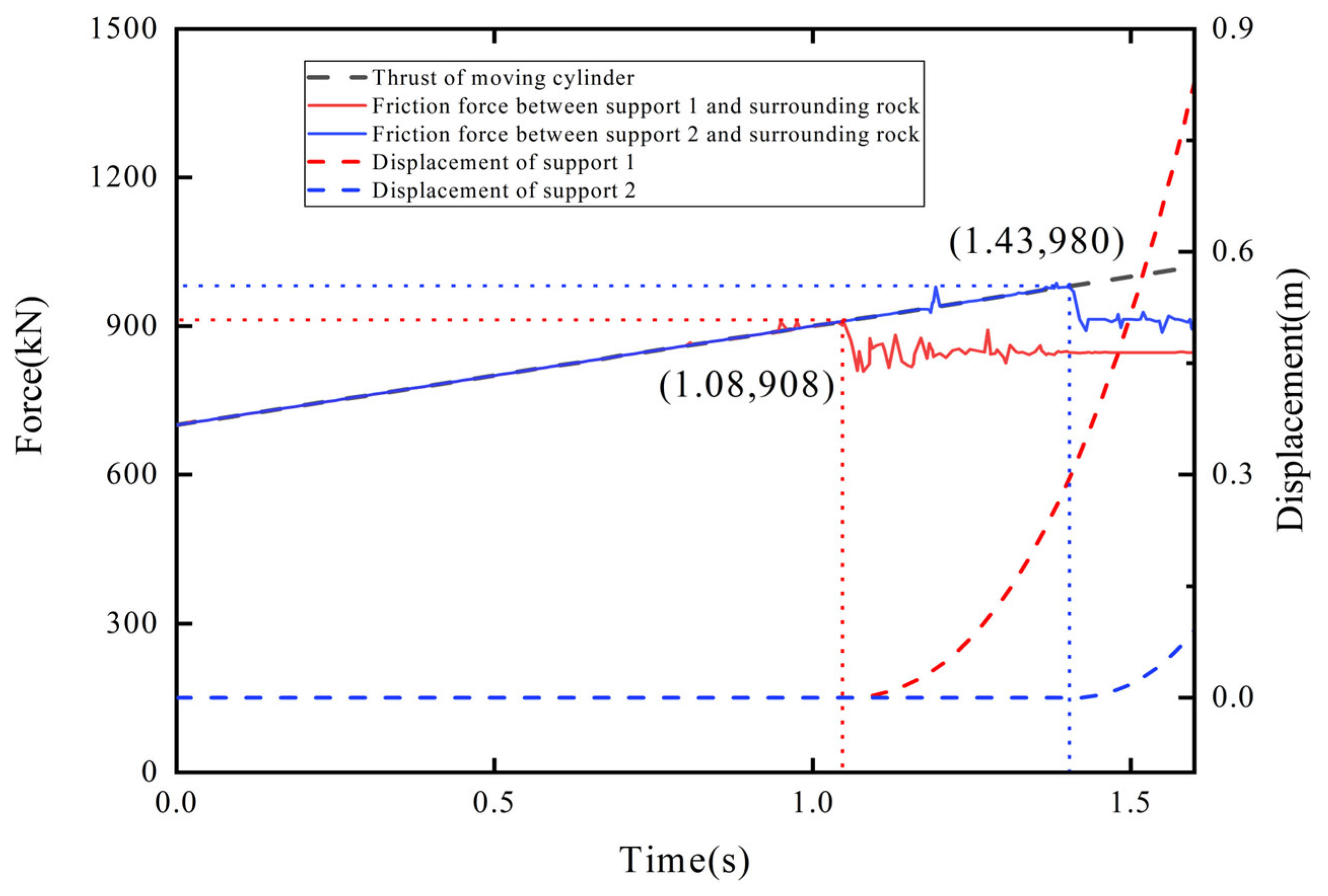
Analyzing Figure 14, it can be seen that under the condition that the specific pressure of the base of support 2 against the floor is P_12d = 0.1 MPa, when the pulling force exceeds 496 kN, the friction force between support 2 and the surrounding rock reaches its maximum value, and support 2 begins to move. The friction force between support 1 and the surrounding rock continues to increase with the pulling force of the moving cylinder, and support 1 remains stationary. At this time, support 2 can normally drive under pressure. When the pulling force exceeds 908 kN, the friction force between support 1 and the surrounding rock reaches its maximum value, and support 1 begins to move. At this time, support 2 cannot drive under pressure.

Analyzing Figure 15, it can be seen that under the condition that the specific pressure of the base of support 2 against the floor is P_12d = 0.19 MPa when the pulling force exceeds 908 kN, the friction force between support 1 and the surrounding rock reaches its maximum value, and support 1 begins to move. The friction force between support 2 and the surrounding rock continues to increase with the pulling force of the moving cylinder, and support 2 remains stationary. When the pulling force exceeds 980 kN, the friction force between support 2 and the surrounding rock reaches its maximum value, and support 2 begins to move. Therefore, support 2 cannot be driven under pressure under this condition. Compared with the theoretical values, the maximum error of the maximum friction force between support 1 and support 2 and the surrounding rock is 1%, which is less than 5%.

4.4. Simulation of Driving Under Pressure Dynamic Model

To analyze the variation in the pushing and pulling forces of the moving cylinder, as well as the supporting force of the top beam of support 1 and support 2 on the roof, and to verify the accuracy of the dynamic model during the driving under pressure motion of support 1 and support 2, the driving under pressure process and its dynamic model of the TSR are simulated through the Adams software based on the simulation model of the boundary condition simulation experiment of the driving under pressure dynamic model taking P_12s = P_22s = 0.2 MPa. The effective area of the piston rod in the piston chamber of the push hydraulic cylinder compared to the effective area of the piston rod in the rod chamber typically ranges from 1.2 to 2. Therefore, the ratio of the thrust to the pull force provided by the push hydraulic cylinder is also between 1.2 and 2. Taking the effective area ratio of the piston chamber piston rod to the rod chamber piston rod as 1.3, during pressurized operation of the temporary support robot, in order to achieve a more rational distribution of thrust and pull force of the push hydraulic cylinder, P_12d is set to 0.1 (MPa). Based on the parameters of the virtual prototype model of the TSR, and assuming that support 1 and support 2 are in uniform motion, the maximum thrust required for support 1 is calculated to be approximately 427 (KN) using the pressure formula, Equations (2) and (9). Consequently, the maximum pull force for support II during pressurized operation is about 427/1.3 ≈ 328 (KN). Further calculations using the pressure formula, Equations (5) and (10), indicate that P_22d is approximately 0.07 (MPa), with both P_12d and P_22d remaining within their respective value ranges. 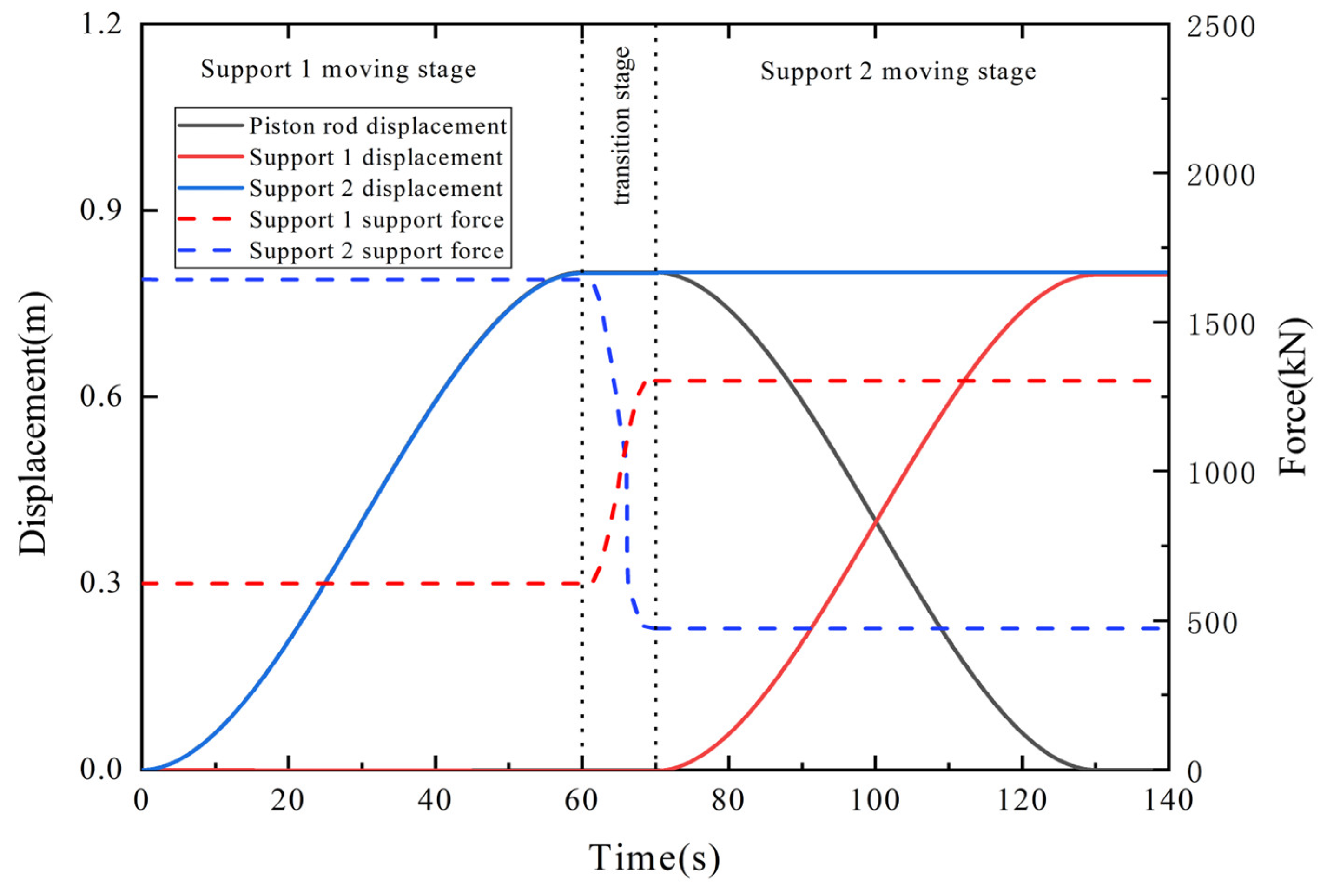And, the critical Stribeck velocity v_st = 0.01 m/s, a step function of the displacement of the moving cylinder piston rod relative to the cylinder, is constructed in the Adams software as a driver for simulation. The displacement increases from 0 m to 0.8 m within 0 to 60 s and decreases from 0.8 m to 0 m within 70 s to 130 s. The simulation time is 140 s. The displacement curve of the moving cylinder piston rod relative to the cylinder and the displacement variation in support 1 and support 2 obtained by simulation are shown in Figure 16. Using the MATLAB R2023a software, the displacement data of support 1 and support 2 are substituted into their respective dynamic models to simulate two cases: considering the Stribeck friction theory and not considering the Stribeck friction theory (when the Stribeck friction theory is not considered, take f₁ = f₂ = 0.28). The theoretical values of the pushing and pulling forces of the moving cylinder are obtained. The force value pushing support 1 is positive, and the force value pulling support 2 is negative. The pushing and pulling forces of the moving cylinder obtained by the Adams simulation are compared with them, and the results are shown in Figure 17.

As shown in Figure 16, the stage from 0 s to 60 s is the driving under pressure stage of support 1. In this stage, driven by the moving cylinder, the speed of support 1 gradually increases from zero within 0 s to 16 s, remains constant within 16 s to 44 s, and gradually decreases to zero within 44 s to 60 s, with a displacement of 0.8 m during the whole process. The stage from 60 s to 70 s is the transition stage. The stage from 70 s to 130 s is the driving under pressure stage of support 2. In this stage, pulled by the moving cylinder, the speed of support 2 gradually increases from zero between 70 s and 86 s, remains constant between 86 s and 114 s, and gradually decreases to zero between 114 s and 130 s, with a displacement of 0.8 m during the whole process. The supporting force of the top beam of support 1 on the roof is 623 kN within 0 s to 60 s, gradually increases to 1303 kN within 60 s to 70 s, and remains stable. The supporting force of the top beam of support 2 on the roof is 1643 kN within 0 s to 60 s, gradually decreases to 473 kN within 60 s to 70 s, and remains stable.

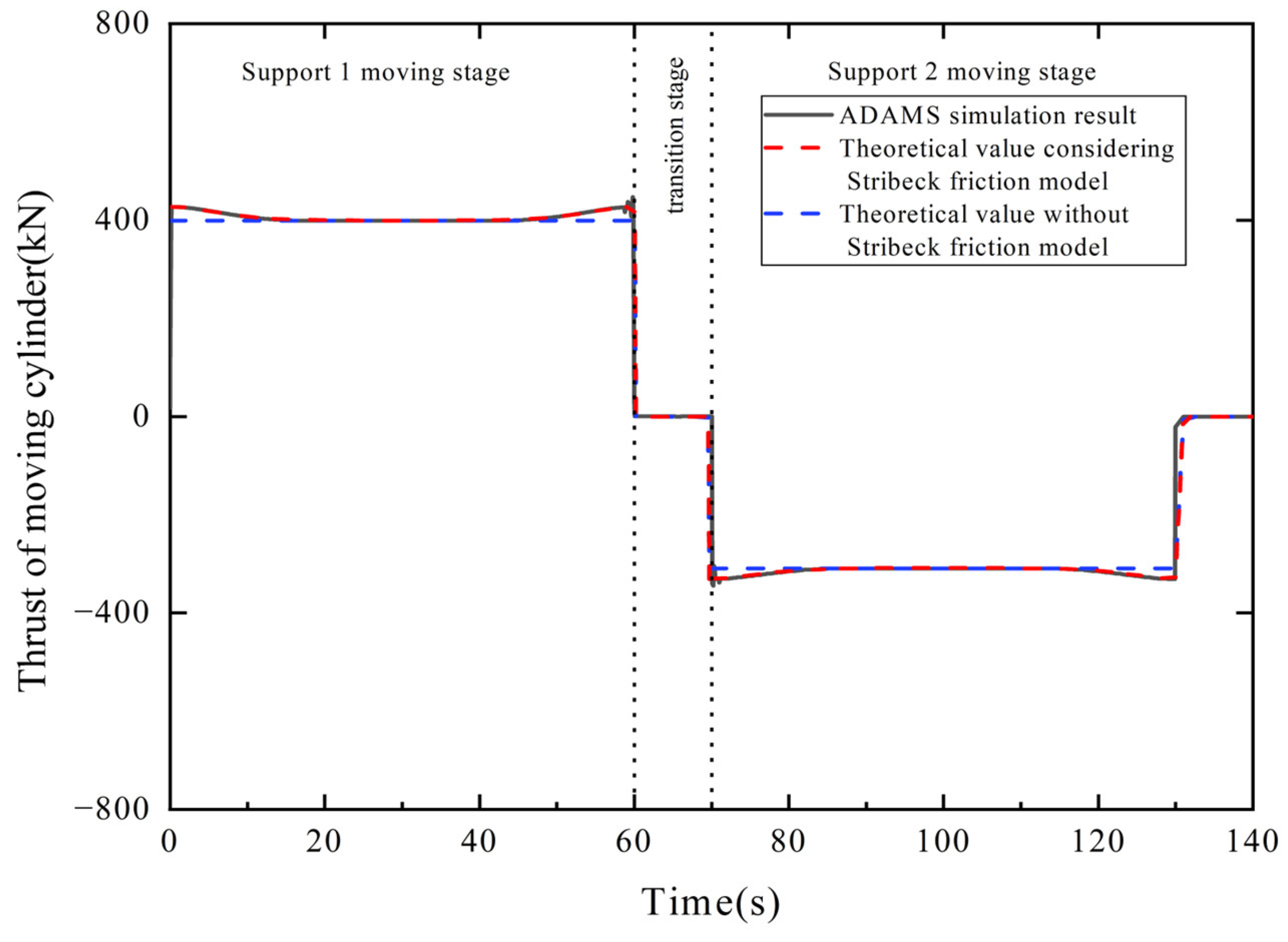
As shown in Figure 17, the pushing force value of the moving cylinder obtained by simulation gradually decreases from 426 kN to 398 kN within 0 s to 16 s, remains stable within 16 s to 44 s, and gradually increases from 399 kN to 426 kN within 44 s to 60 s. The force value decreases to 0 N at 60 s. At 70 s, the pushing force of the moving cylinder changes to pulling force, and the force value is 333 kN, which decreases from 333 kN to 305 kN within 70 s to 86 s, remains stable within 86 s to 114 s, and increases from 305 kN to 330 kN within 114 s to 130 s, and remains constant at 0 N after 130 s. The theoretical values of the pushing and pulling force of the moving cylinder in the dynamic model of driving under pressure considering the Stribeck friction theory gradually decrease from 427 kN to 399 kN within 0 s to 16 s, remain stable within 16 s to 44 s, gradually increase from 399 kN to 427 kN within 44 s to 60 s, and remain constant at 0 N within 60 s to 70 s. At 70 s, the pushing force of the moving cylinder changes to pulling force, and the force value is 331 kN, which decreases from 331 kN to 309 kN within 70 s to 86 s, remains stable within 86 s to 114 s, increases from 309 kN to 331 kN within 114 s to 130 s, and remains constant at 0 N after 130 s. The theoretical value of the pushing and pulling force of the moving cylinder in the driving under pressure dynamic model without considering the Stribeck friction theory is 398 kN within 0 s to 60 s and 0 N within 60 s to 70 s. From 70 s to 130 s, the pushing force of the moving cylinder changes to the pulling force with a force of 309 kN and remains constant at 0 N after 130 s.

The simulation results show that the theoretical value of the dynamic model of driving under pressure, considering the Stribeck friction theory, has the same trend as the pushing force value of the moving cylinder obtained by the simulation, and its maximum error is 1.3%, which does not exceed 5%. The maximum error between the theoretical value of the driving under pressure dynamic model without considering the Stribeck friction theory and the pushing force value of the moving cylinder obtained by the simulation is 7.8%. Therefore, considering the Stribeck friction theory can make the driving under pressure dynamic model reflect the real values of pushing and pulling force required by support 1 and support 2 during the driving under pressure process of TSR more accurately.

5. Conclusions

Facing the support challenges of short-wall working face (15–40 m) roadways in the ‘excavation–backfill–retention’ tunneling method for section coal pillars, traditional equipment struggled to achieve stable, reliable, and efficient support. This paper proposed a temporary support robot for the excavation and mining system of section coal pillars to ensure the safety of equipment and personnel in short-wall working faces. The support requirements of the section coal pillar excavation and mining system were analyzed, and the overall scheme of the temporary support system was proposed. The working principle of the temporary support robot was introduced. The temporary support robot consisted of two parts, support 1 and support 2, which advanced by pushing and pulling each other.

A mechanical model for the stable support of the temporary support robot was established. The mechanical properties of the surrounding rock were analyzed, and the allowable range of the temporary support robot’s supporting force under stable support conditions was determined while ensuring the stability of the surrounding rock. Based on the Stribeck friction theory, a dynamic model of the temporary support robot in the driving under pressure state was constructed. The boundary conditions of the dynamic model were set, and the corresponding relationship between the temporary support robot’s supporting force and its maximum static friction force was determined. Precise dynamic modeling of heavy equipment under complex contact conditions was completed, accurately describing the influence of the supporting force and pushing (pulling) force on the movement during the temporary support robot’s driving under pressure, and limiting the allowable range of the supporting force in the driving under pressure state.

Through finite element simulation, the stress on the temporary support robot and the floor under the given load was analyzed, verifying that this load condition would not cause damage to the temporary support robot or the surrounding rock. Through multi-body dynamics simulation, the pushing (pulling) forces required for the temporary support robot’s movement under different supporting force conditions were obtained. Compared with the theoretical values, the error was less than 1.2%, verifying the feasibility of the driving under pressure action under different supporting force conditions. In addition, through multi-body dynamics simulation, the driving under pressure process of the temporary support robot was analyzed. The changes in supporting force and displacement of support Ⅰ and support Ⅱ in the TSR were obtained. The model-predicted values and simulated values of the required pushing (pulling) forces were compared. The simulation results showed that the model’s prediction accuracy was significantly better than that of the traditional simplified model, with an error of only 1.3%, validating the accuracy of the dynamic model.

This research provided a safe and reliable support scheme for the excavation of short-wall working faces in section coal pillar mining, offering a new theoretical framework for the design and dynamic analysis of temporary support equipment in mining engineering. It holds significant academic value and broad application prospects, driving the development of support equipment towards higher precision, efficiency, and safety, and providing strong technical support for achieving the goals of mine safety production and green mining.